Comprehensive Investigation of Capabilities of the Left-Looking InSAR Observations in Coseismic Surface Deformation Mapping and Faulting Model Estimation Using Multi-Pass Measurements: An Example of the 2016 Kumamoto, Japan Earthquake
Abstract
1. Introduction
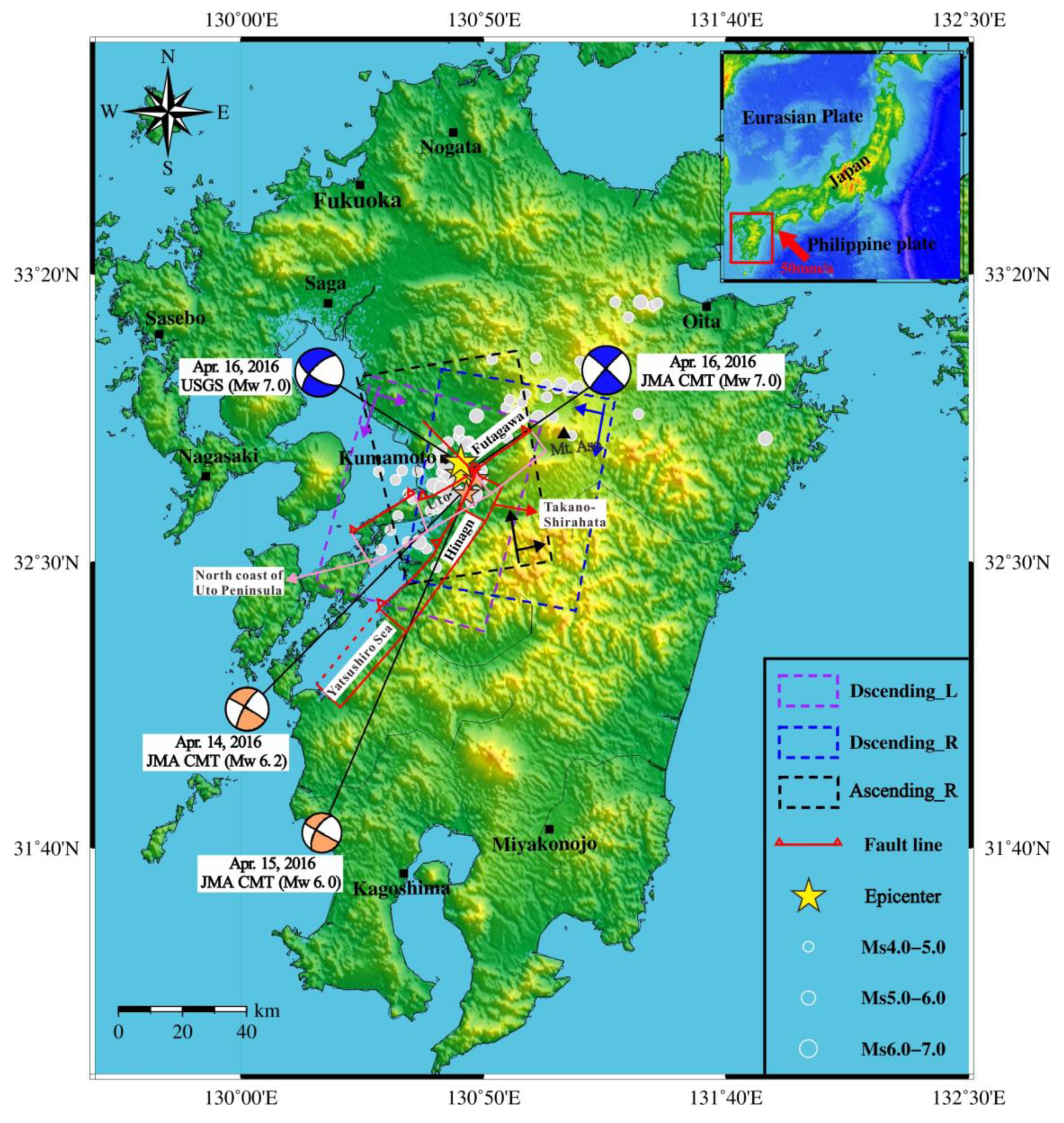
2. Datasets and Processing
2.1. Coseismic InSAR Deformation
2.2. Pixel Offset-Tracking Data
3. Method of Geodetic Modelling
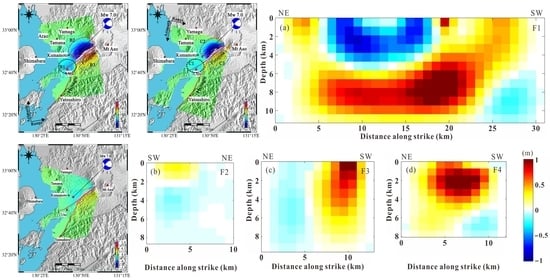
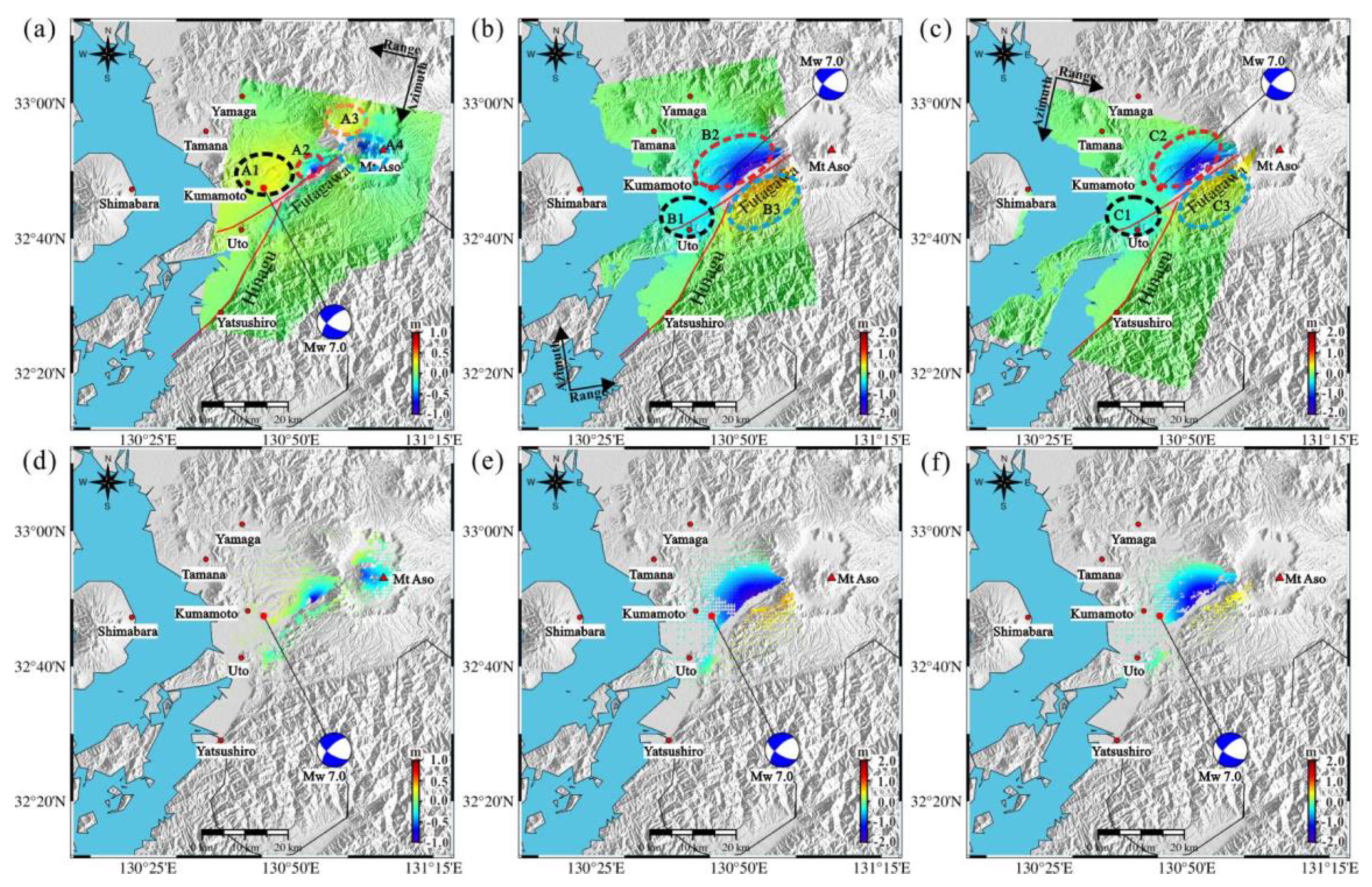
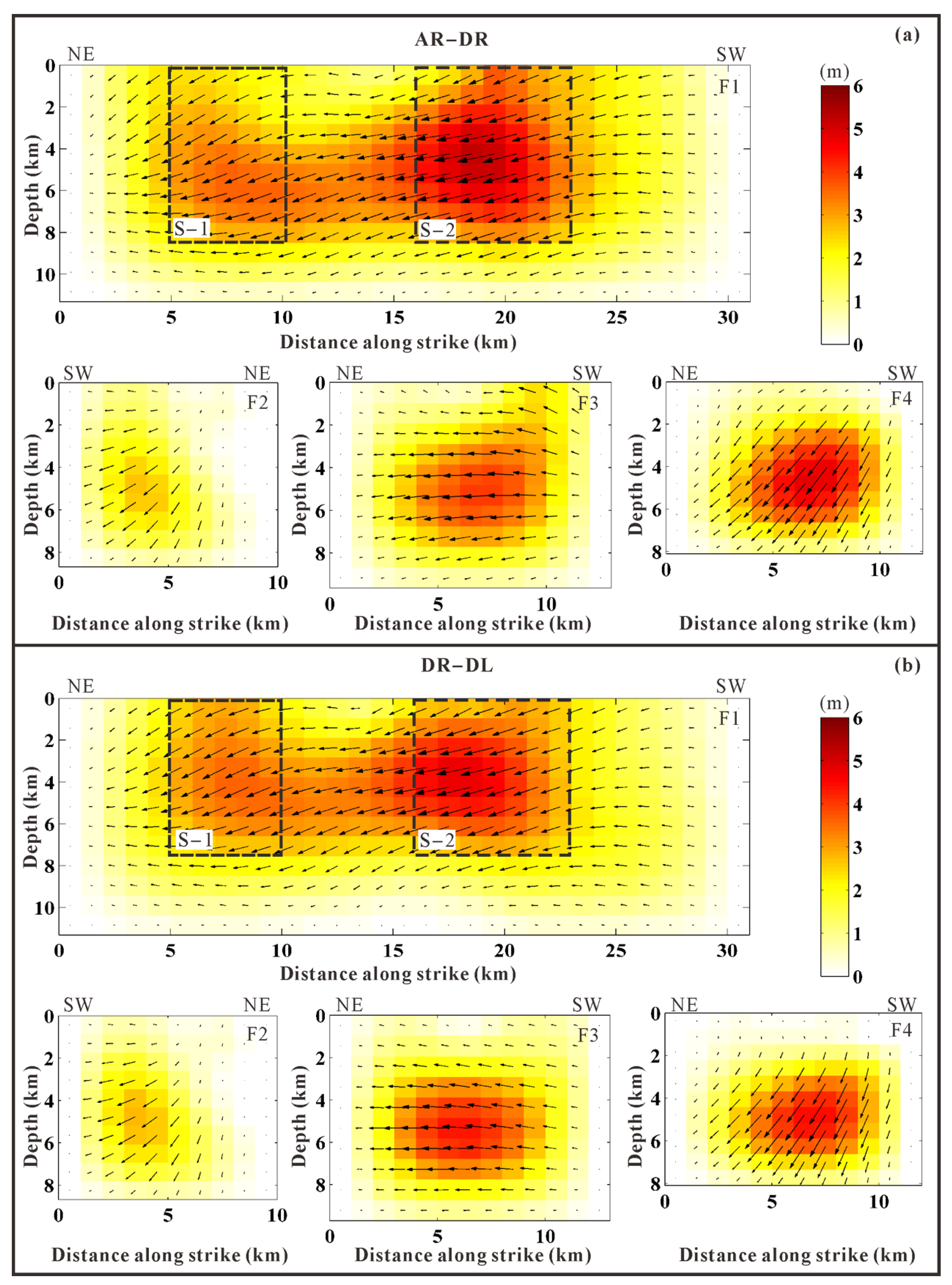
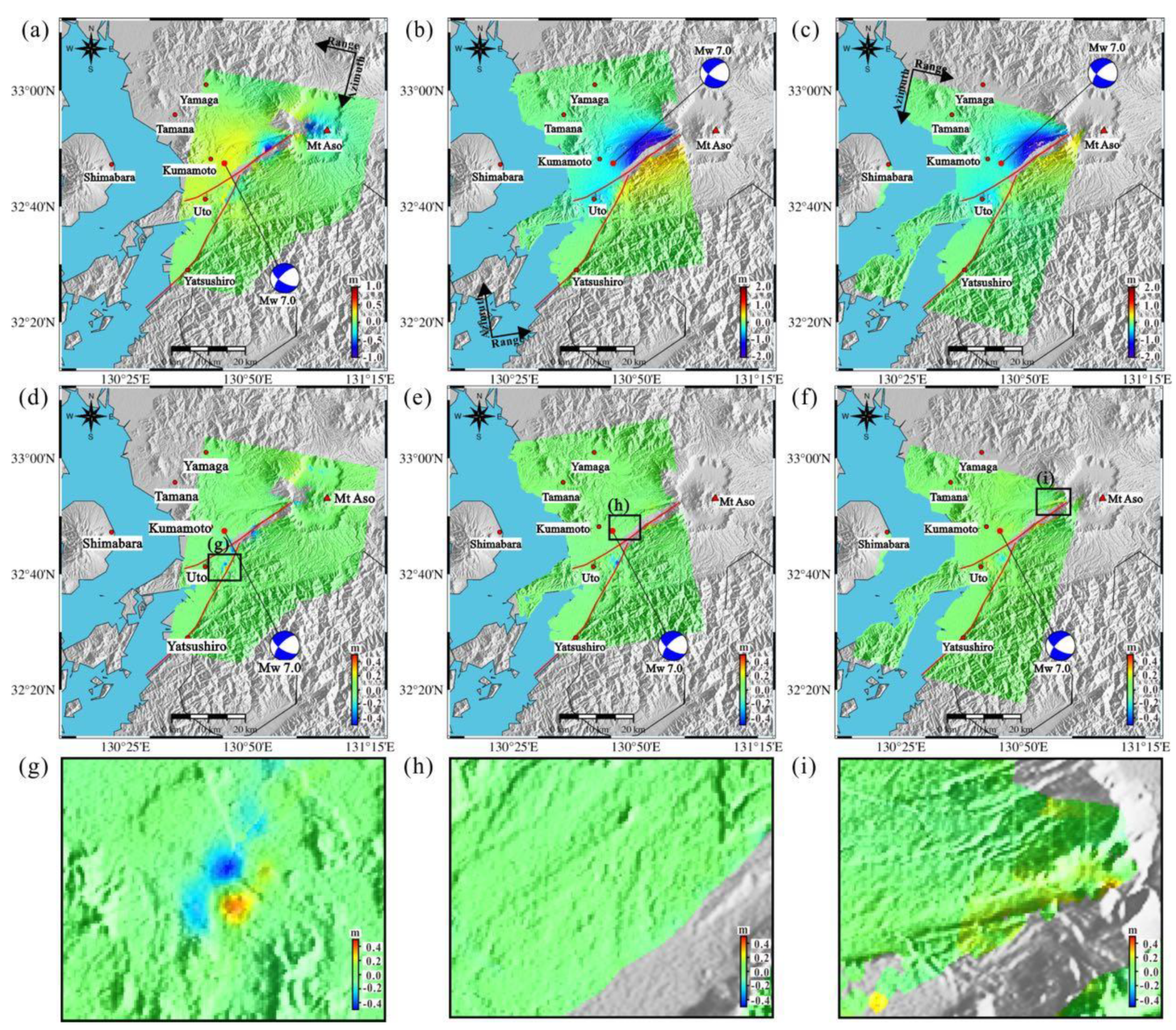
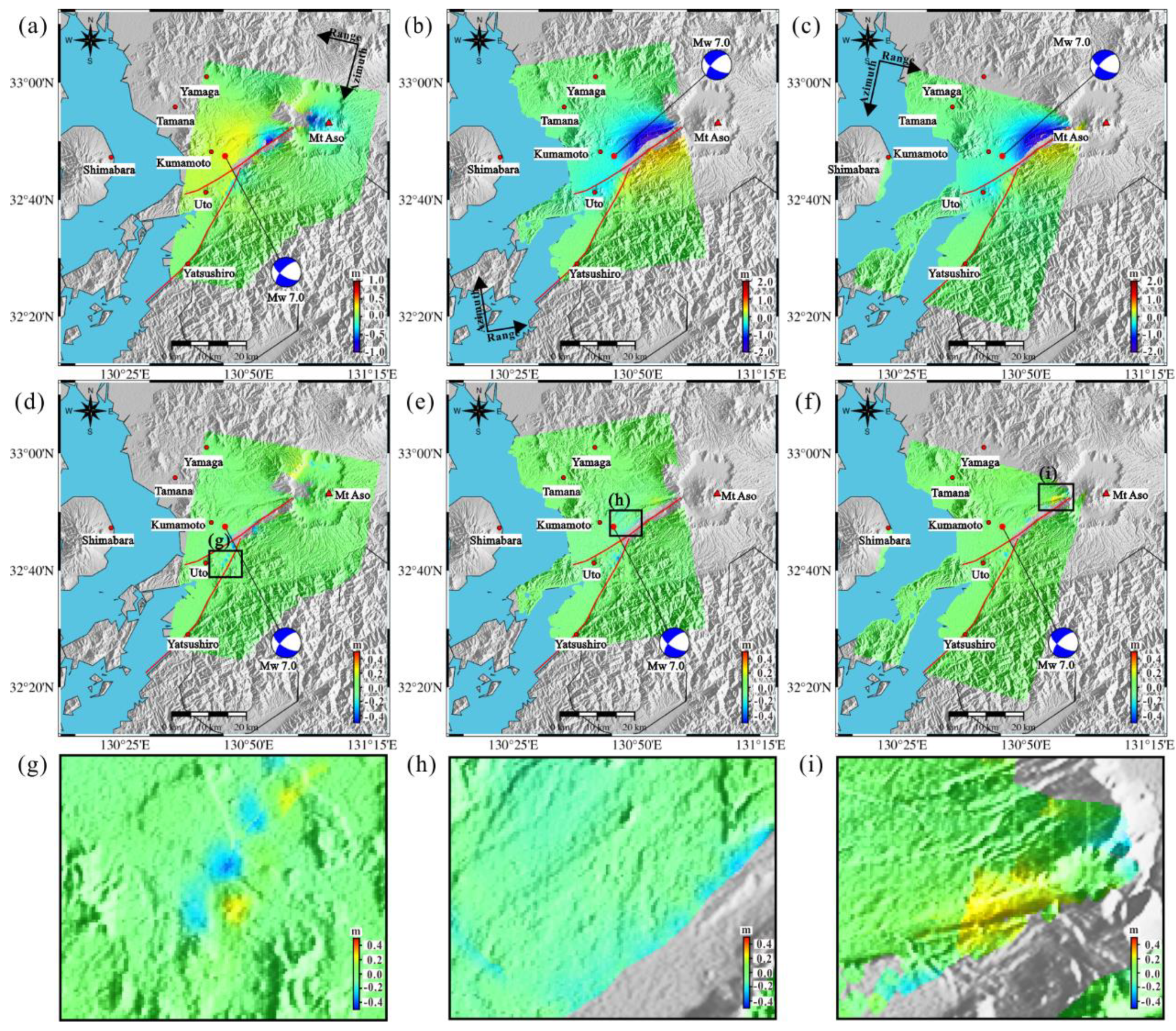
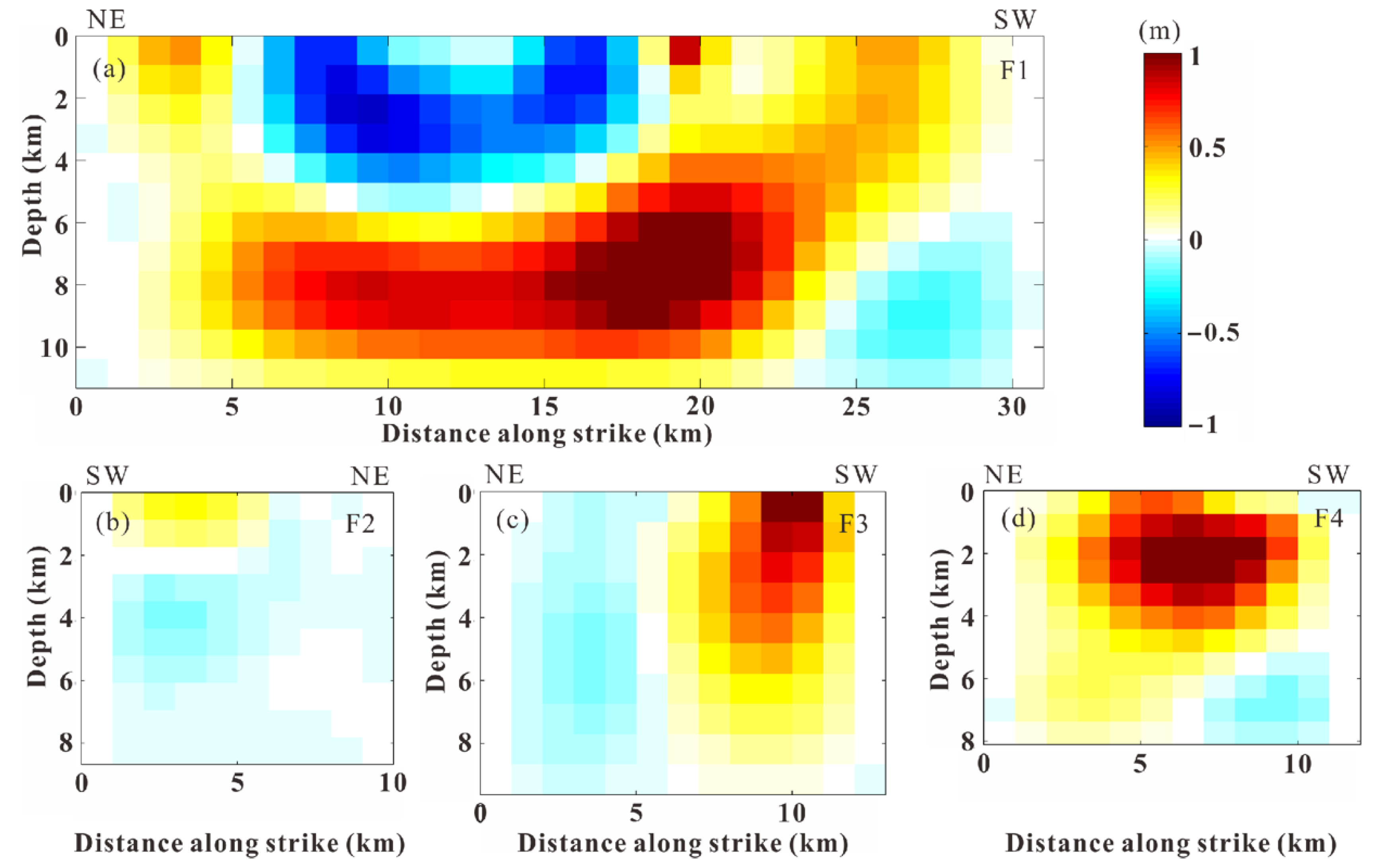
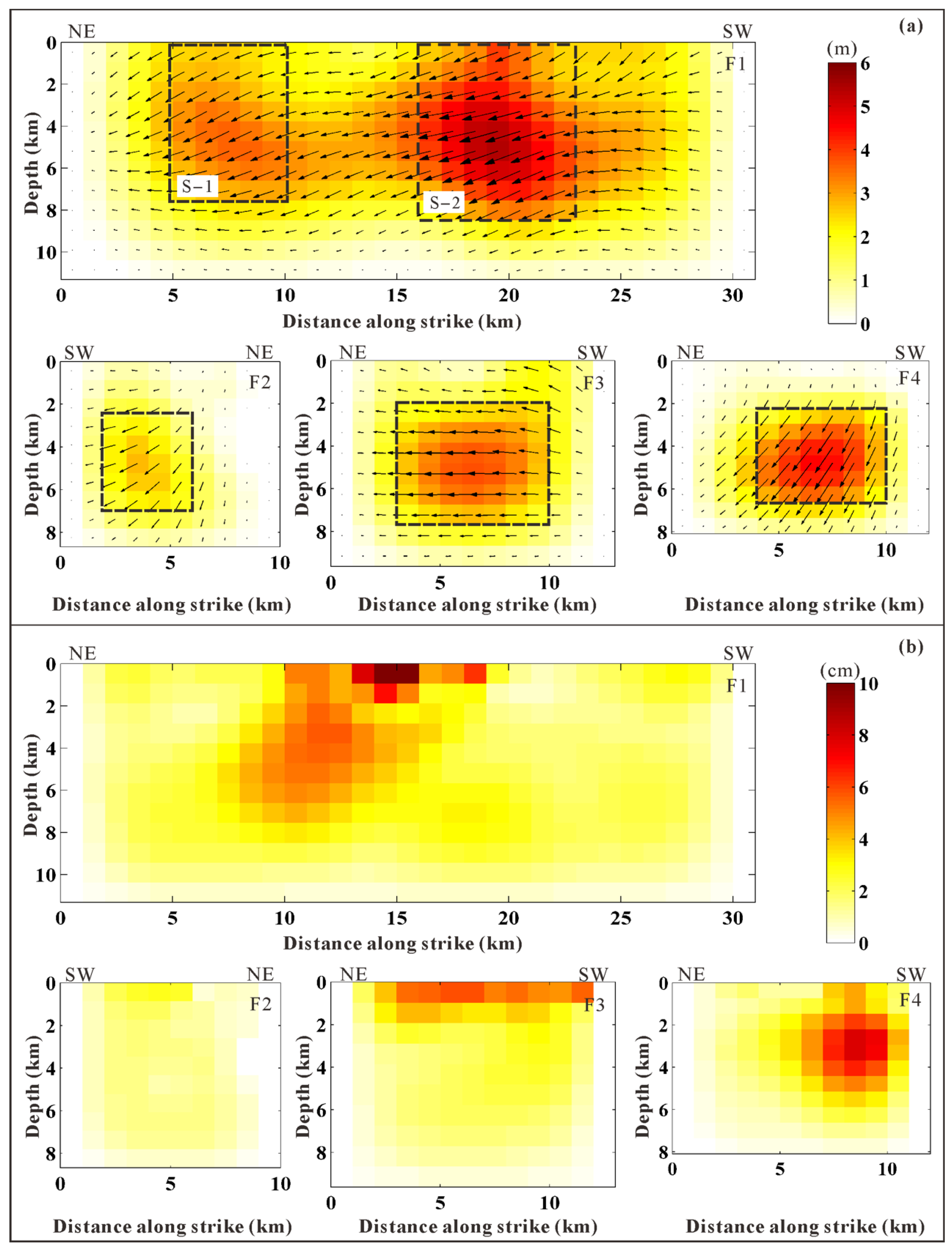
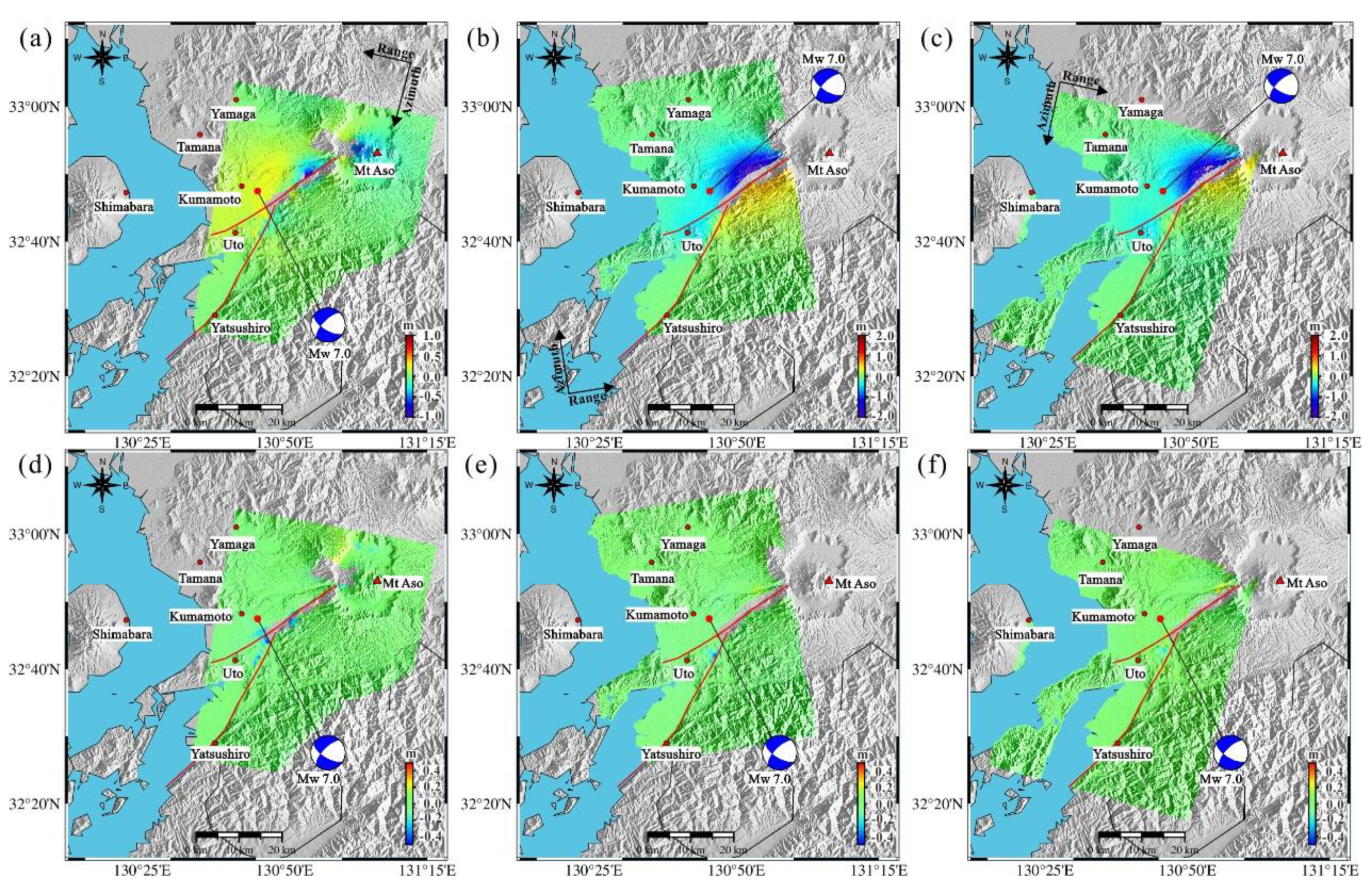
4. Results
5. Discussions
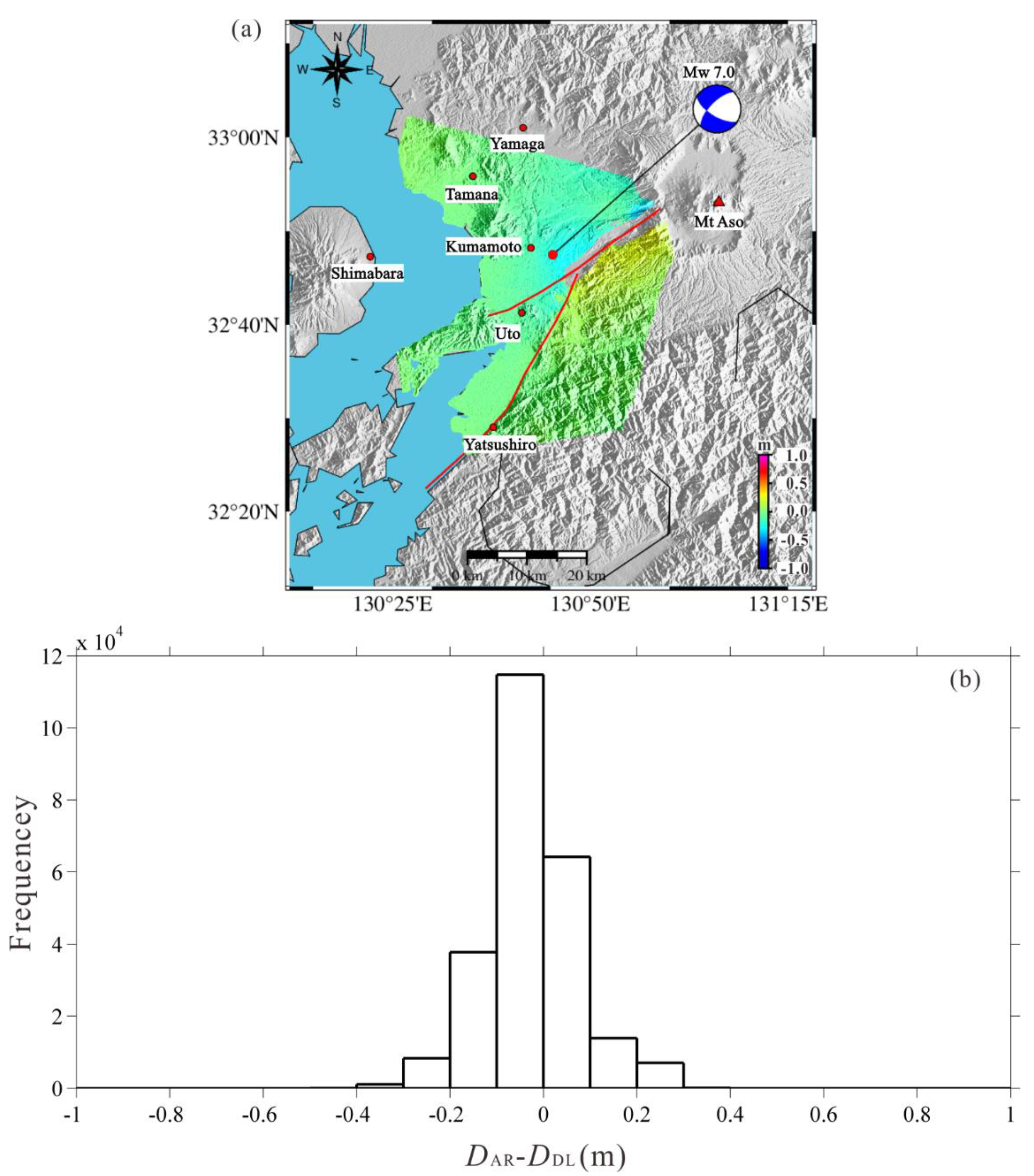
5.1. Difference in Displacements from the AR and DL InSAR
5.2. Optimal Faulting Mechanism Responsible for the 2016 Kumamoto Earthquake
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chen, Q.; Xu, Q.; Zhang, Y.J.; Yang, Y.H.; Yong, Q.; Liu, G.X. Sequential combination of multi-source satellite observations for separation of surface deformation associated with serial seismic events. Int. J. Appl. Earth Obs. Geoinf. 2018, 65, 57–70. [Google Scholar] [CrossRef]
- Massonnet, D.; Rossi, M.; Carmona, C. The displacement filed of the landers earthquake mapped by radar interferometry. Nature 1993, 364, 138–142. [Google Scholar] [CrossRef]
- Tong, X.P.; Sandwell, D.T.; Yuri, F. Coseismic slip model of the 2008 Wenchuan earthquake derived from joint inversion of interferometric synthetic aperture radar, GPS, and feld data. J. Geophys. Res. Solid Earth 2010, 115, B04314. [Google Scholar] [CrossRef]
- Wright, T.J.; Parsons, B.; England, P.C.; Fielding, E.J. InSAR observations of low slip rates on the major faults of western Tibet. Science 2004, 305, 236–239. [Google Scholar] [CrossRef]
- Yang, Y.H.; Tsai, M.C.; Hu, J.C.; Aurelio, M.A.; Hashimoto, M.; Escudero, J.A.P.; Su, Z.; Chen, Q. Coseismic slip deficit of the 2017 Mw 6.5 Ormoc Earthquake that occurred along a creeping segment and geothermal field of the Philippine Fault. Geophys. Res. Lett. 2018, 45, 2659–2668. [Google Scholar] [CrossRef]
- Alatza, S.; Papoutsis, I.; Paradissis, D.; Kontoes, C.; Papadopoulos, G.A. Multi-Temporal InSAR Analysis for Monitoring Ground Deformation in Amorgos Island, Greece. Sensors 2020, 20, 338. [Google Scholar] [CrossRef] [PubMed]
- Hu, J.; Li, Z.W.; Ding, X.L.; Zhu, J.J.; Zhang, L.; Sun, Q. Resolving three-dimensional surface displacements from InSAR measurements: A review. Earth Sci. Rev. 2014, 133, 1–17. [Google Scholar] [CrossRef]
- Wright, T.J.; Parsons, B.E.; Lu, Z. Toward mapping surface deformation in three dimensions using InSAR. Geophys. Res. Lett. 2004, 31, L01607. [Google Scholar] [CrossRef]
- Asano, K.; Iwata, T. Source rupture processes of the foreshock and mainshock in the 2016 Kumamoto earthquake sequence estimated from the kinematic waveform inversion of strong motion data. Earth Planets Space 2016, 68, 147. [Google Scholar] [CrossRef]
- Kubo, H.; Suzuki, W.; Aoi, S.; Sekiguchi, H. Source rupture processes of the 2016 Kumamoto, Japan, earthquakes estimated from strong-motion waveforms. Earth Planets Space 2016, 68, 161. [Google Scholar] [CrossRef]
- Nanjo, K.Z.; Izutsu, J.; Orihara, Y.; Furuse, N.; Togo, S.; Nitta, H.; Okada, T.; Tanaka, R.; Kamogawa, M.; Nagao, T. Seismicity prior to the 2016 Kumamoto earthquakes. Earth Planets Space 2016, 68, 187. [Google Scholar] [CrossRef]
- Fukuda, Y.; Itahara, M.; Kusumoto, S.; Higashi, T.; Takemura, K.; Mawatari, H.; Yusa, Y.; Yamamoto, T.; Kato, T. Crustal movements around the Beppu Bay area, East-Central Kyushu, Japan, observed by GPS 1996–1998. Earth Planets Space 2000, 52, 979–984. [Google Scholar] [CrossRef]
- Miyazaki, S.; Heki, K. Crustal velocity field of southwest Japan: Subduction and arc–arc collision. J. Geophys. Res. 2001, 106, 4305–4326. [Google Scholar] [CrossRef]
- Nishimura, S.; Hashimoto, M. A model with rigid rotations and slip deficits for the GPS-derived velocity field in Southwest Japan. Tectonophysics 2006, 421, 187–207. [Google Scholar] [CrossRef]
- Takayama, H.; Yoshida, A. Crustal deformation in Kyushu derived from GEONET data. J. Geophys. Res. 2007, 112. [Google Scholar] [CrossRef]
- ERC/HERP (Earthquake Research Committee/Headquarters for Earthquake Research Promotion). Elevation of the Futagawa Fault Zone and the Hinagu Fault Zone. 1 February 2013 Version (Partially Revised). (In Japanese). Available online: http://www.jishin.go.jp/main/chousa/13feb_chi_kyushu/k_11.pdf (accessed on 17 June 2016).
- Geological Survey of Japan. Surface Earthquake Faults Associated with the 2016 Kumamoto Earthquake. (In Japanese). Available online: https://www.gsi.jp/hazards/earthquake/kumamoto2016/kumamoto20160513-1.html (accessed on 10 August 2016).
- Okada, S.; Toda, S. Report on the emergency field survey of the 2016 Kumamoto earthquake. In Report Presented at the Second Emergency Meeting, International Research Institute of Disaster Science; Tohoku University: Sendai, Japan, 2016; Available online: http://irides.tohoku.ac.jp/media/files/earthquake/eq/IRIDeS_Kumamoto_earthquake_okada_20160419.pdf (accessed on 16 May 2016).
- Fukahata, Y.; Hashimoto, M. Simultaneous estimation of the dip angles and slip distribution on the faults of the 2016 Kumamoto earthquake through a weak nonlinear inversion of InSAR data. Earth Planets Space 2016, 68, 204. [Google Scholar] [CrossRef]
- Himematsu, Y.; Furuya, M. Fault source model for the 2016 Kumamoto earthquake sequence based on ALOS-2/PALSAR-2 pixel-offset data: Evidence for dynamic slip partitioning (EPSP-D-16-00163). Earth Planets Space 2016, 68, 169. [Google Scholar] [CrossRef]
- Shirahama, Y.; Yoshimi, M.; Awata, Y.; Maruyama, T.; Azuma, T.; Miyashita, Y.; Mori, H.; Imanishi, K.; Takeda, N.; Ochi, T.; et al. Characteristics of the surface ruptures associated with the 2016 Kumamoto earthquake sequence, central Kyushu, Japan. Earth Planets Space 2016, 68, 191. [Google Scholar] [CrossRef]
- National Institute of Advanced Industrial Science and Technology. Active Fault Database of Japan, 28 February 2012 version. Research Information Database DB095, National Institute of Advanced Industrial Science and Technology. 2012. Available online: https://gbank.gsj.jp/activefault/index_e_gmap.html (accessed on 11 July 2016).
- Wegmuller, U.; Werner, C. Gamma SAR processor and ineterferometry software. In Proceedings of the 3rd ERS Symposium, Florence, Italy, 14–21 March 1997; pp. 1687–1692. [Google Scholar]
- Hanssen, R.F. Radar Interferometry: Data Interpretation and Error Analysis; Springer: Dordrecht, The Netherlands, 2001. [Google Scholar] [CrossRef]
- Tralli, D.M.; Blom, R.G.; Zlotnicki, V.; Donnellan, A.; Evans, D.L. Satellite remote sensing of earthquake, volcano, flood, landslide and coastal inundation hazards. ISPRS J. Photogramm. Remote Sens. 2005, 59, 185–198. [Google Scholar] [CrossRef]
- Yang, Y.H.; Chen, Q.; Xu, Q.; Zhang, Y.; Yong, Q.; Liu, G. Coseismic surface deformation of the 2014 Napa earthquake mapped by Sentinel-1A SAR and accuracy assessment with COSMO-SkyMed and GPS data as cross validation. Int. J. Digit. Earth 2017, 10, 1197–1213. [Google Scholar] [CrossRef]
- Tobita, M.; Murakami, M.; Nakagawa, H.; Yarai, H.; Fujiwara, S.; Rosen, P.A. 3-D surface deformation of the 2000 Usu eruption measured by matching of SAR images. Geophys. Res. Lett. 2001, 28, 4291–4294. [Google Scholar] [CrossRef]
- Strozzi, T.; Luckman, A.; Murray, T.; Wegmüller, U.; Werner, U. Glacier motion estimation using SAR offset-tracking procedures. IEEE Trans. Geosci. Remote Sens. 2002, 40, 2384–2391. [Google Scholar] [CrossRef]
- Casu, F.; Manconi, A.; Pepe, A.; Lanari, R. Deformation time-series generation in areas characterized by large displacement dynamics: The SAR amplitude pixel-offset SBAS technique. IEEE Trans. Geosci. Remote Sens. 2011, 49, 2752–2763. [Google Scholar] [CrossRef]
- Chen, Q.; Luo, R.; Yang, Y.H.; Yong, Q. Method and Accuracy of Ex tracting Surface Deformation Field from SAR Image Coregistration. Acta Geod. Cartogr. Sin. 2015, 44, 301–308, In Chinese. [Google Scholar] [CrossRef]
- Jonsson, S.; Zebker, H.; Segall, P.; Amelung, F. Fault slip distribution of the 1999 Mw7.1 Hector Mine, California, Earthquake, estimated from satellite radar and GNSS measurements. Bull. Seismol. Soc. Am. 2002, 92, 1377–1389. [Google Scholar] [CrossRef]
- Ozawa, T.; Fujita, E.; Ueda, H. Crustal deformation associated with the 2016 Kumamoto Earthquake and its effect on the magma system of Aso volcano. Earth Planets Space 2016, 68, 186. [Google Scholar] [CrossRef]
- USGS. Available online: http://earthquake.usgs.gov/earthquakes/eventpage/us20005iis#finite-fault (accessed on 11 June 2016).
- Okada, Y. Surface deformation to shear and tensile fault in a half-space. Bull. Seismol. Soc. Am. 1985, 75, 1135–1154. [Google Scholar]
- Chen, Q.; Liu, X.W.; Zhang, Y.J.; Zhao, J.; Xu, Q.; Yang, Y.; Liu, G.X. A nonlinear inversion of InSAR-observed coseismic surface deformation for estimating variable fault dips in the 2008 Wenchuan earthquake. Int. J. Appl. Earth Obs. Geoinf. 2019, 76, 179–192. [Google Scholar] [CrossRef]
- Xu, Q.; Chen, Q.; Zhao, J.J.; Liu, X.; Yang, Y.; Zhang, Y.; Liu, G. Sequential modelling of the 2016 Central Italy earthquake cluster using multisource satellite observations and quantitative assessment of Coulomb stress change. Geophys. J. Int. 2020, 221, 451–466. [Google Scholar] [CrossRef]
- He, Z.; Chen, T.; Wang, M.; Li, Y. Multi-Segment Rupture Model of the 2016 Kumamoto Earthquake Revealed by InSAR and GPS Data. Remote. Sens. 2020, 12, 3721. [Google Scholar] [CrossRef]
- Fujiwara, S.; Yarai, H.; Kobayashi, T.; Morishita, Y.; Nakano, T.; Miyahara, B.; Nakai, H.; Miura, Y.; Ueshiba, H.; Kakiage, Y.; et al. Small-displacement linear surface ruptures of the 2016 Kumamoto earthquake sequence detected by ALOS-2 SAR interferometry. Earth Planets Space 2016, 68, 160. [Google Scholar] [CrossRef]
- Uchide, T.; Horikawa, H.; Nakai, M.; Matsushita, R.; Shigematsu, N.; Ando, R.; Imanishi, K. The 2016 Kumamoto–Oita earthquake sequence: Aftershock seismicity gap and dynamic triggering in volcanic areas. Earth Planets Space 2016, 68, 180. [Google Scholar] [CrossRef]
- Kobayashi, T. Earthquake rupture properties of the 2016 Kumamoto earthquake foreshocks (Mj 6.5 and Mj 6.4) revealed by conventional and multiple-aperture InSAR. Earth Planets Space 2017, 69, 7. [Google Scholar] [CrossRef]

| Track | Acquisition Data (Y-M-D) | Operation Mode | Flight Direction | Beam Direction | Prep. Baseline (m) | Temp. Baseline (Day) | Heading (°) | Incidence Angle (°) |
|---|---|---|---|---|---|---|---|---|
| T23 | 2016/03/07– 2016/05/02 | SM1 | Descending | Right | −34.6 | 56 | 190.3 | 36.2 |
| T131 | 2016/03/29– 2016/04/26 | SM1 | Ascending | Right | −227.3 | 28 | −9.7 | 42.9 |
| T29 | 2015/01/14– 2016/04/20 | SM1 | Descending | Left | 5.1 | 461 | 195.5 | 43.0 |
| Constraint Data | Seismic Fault | Strike Angle (°) | Dip Angle (°) | Rake Angle (°) |
|---|---|---|---|---|
| AR–DR | F1 | 235.6 ± 1.9 243.4 ± 2.7 223.6 ± 3.2 | 71.8 ± 3.1 | −171.4 ± 5.6 |
| F2 | 40.5 ± 1.8 | 58.9 ± 3.6 | −134.7 ± 6.1 | |
| F3 | 205.0 ± 2.2 | 73.6 ± 3.9 | −171.3 ± 5.9 | |
| F4 | 235.6 | 46.6 ± 2.1 | −117.1 ± 6.5 | |
| DR–DL | F1 | 237.5 ± 2.4 245.7 ± 2.3 220.6 ± 3.6 | 68.8 ± 3.5 | −167.0 ± 4.6 |
| F2 | 39.1 ± 2.1 | 62.2 ± 3.8 | −128.9 ± 6.2 | |
| F3 | 203.5 ± 2.2 | 77.4 ± 4.0 | −176.1 ± 6.4 | |
| F4 | 237.5 | 45.9 ± 1.7 | −123.2 ± 6.6 | |
| AR–DR–DL | F1 | 236.5 ± 2.0 247.1 ± 2.5 222.0 ± 3.3 | 70.6 ± 3.2 | −168.5 ± 4.9 |
| F2 | 41.0 ± 1.9 | 60.2 ± 3.8 | −132.1 ± 6.3 | |
| F3 | 204.5 ± 2.1 | 74.5 ± 4.1 | −173.7 ± 6.2 | |
| F4 | 236.5 | 46.2 ± 1.9 | −120.0 ± 6.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Y.-H.; Chen, Q.; Xu, Q.; Zhao, J.-J.; Hu, J.-C.; Li, H.-L.; Xu, L. Comprehensive Investigation of Capabilities of the Left-Looking InSAR Observations in Coseismic Surface Deformation Mapping and Faulting Model Estimation Using Multi-Pass Measurements: An Example of the 2016 Kumamoto, Japan Earthquake. Remote Sens. 2021, 13, 2034. https://doi.org/10.3390/rs13112034
Yang Y-H, Chen Q, Xu Q, Zhao J-J, Hu J-C, Li H-L, Xu L. Comprehensive Investigation of Capabilities of the Left-Looking InSAR Observations in Coseismic Surface Deformation Mapping and Faulting Model Estimation Using Multi-Pass Measurements: An Example of the 2016 Kumamoto, Japan Earthquake. Remote Sensing. 2021; 13(11):2034. https://doi.org/10.3390/rs13112034
Chicago/Turabian StyleYang, Ying-Hui, Qiang Chen, Qian Xu, Jing-Jing Zhao, Jyr-Ching Hu, Hao-Liang Li, and Lang Xu. 2021. "Comprehensive Investigation of Capabilities of the Left-Looking InSAR Observations in Coseismic Surface Deformation Mapping and Faulting Model Estimation Using Multi-Pass Measurements: An Example of the 2016 Kumamoto, Japan Earthquake" Remote Sensing 13, no. 11: 2034. https://doi.org/10.3390/rs13112034
APA StyleYang, Y.-H., Chen, Q., Xu, Q., Zhao, J.-J., Hu, J.-C., Li, H.-L., & Xu, L. (2021). Comprehensive Investigation of Capabilities of the Left-Looking InSAR Observations in Coseismic Surface Deformation Mapping and Faulting Model Estimation Using Multi-Pass Measurements: An Example of the 2016 Kumamoto, Japan Earthquake. Remote Sensing, 13(11), 2034. https://doi.org/10.3390/rs13112034