Investigation of Combined Heating and Cooling Systems with Short- and Long-Term Storages
Abstract
:1. Introduction
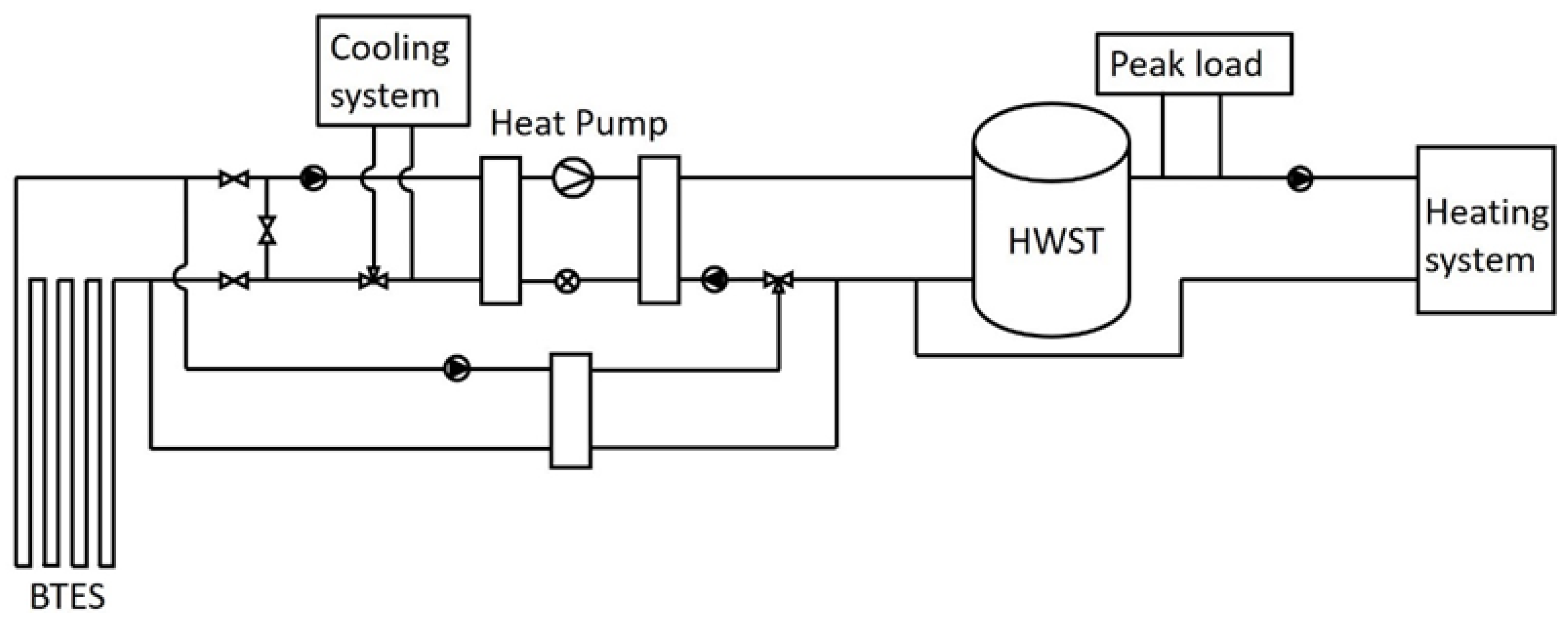
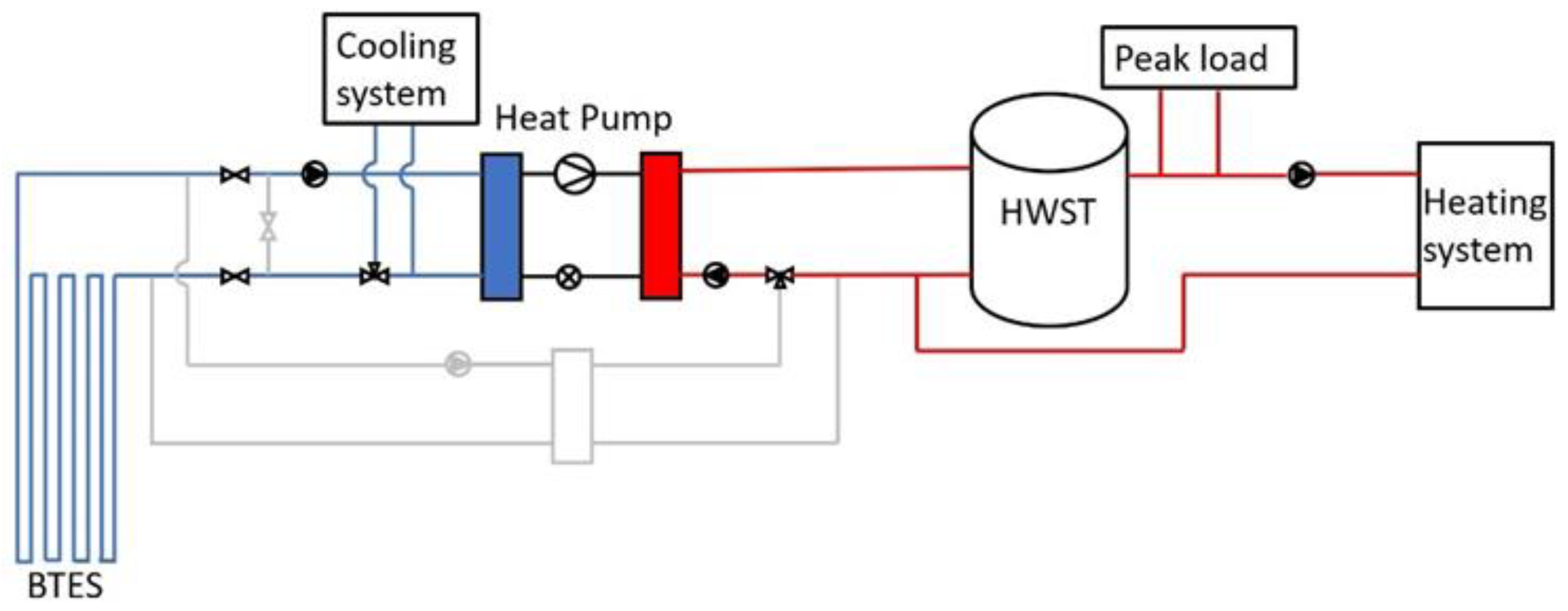
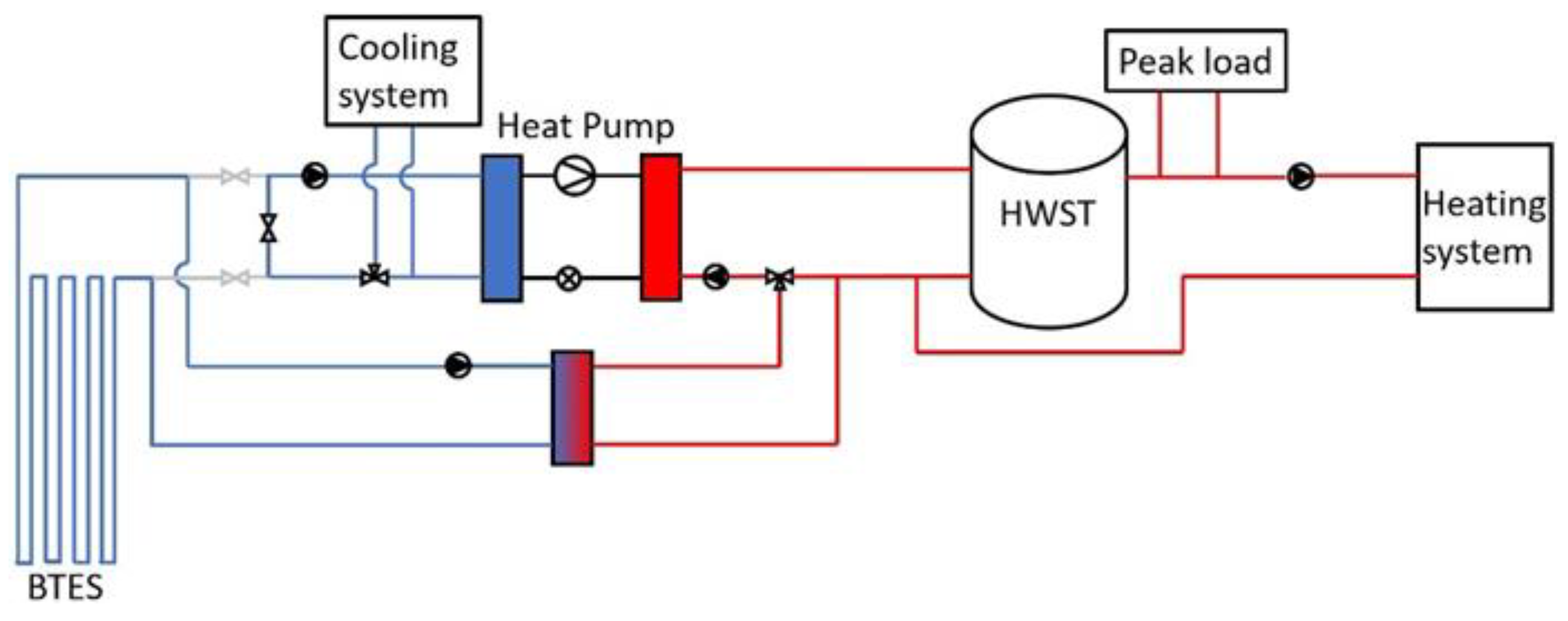
2. System Description
3. Methods
3.1. Heat Pump Model
3.2. Borehole Thermal Energy Storage Model
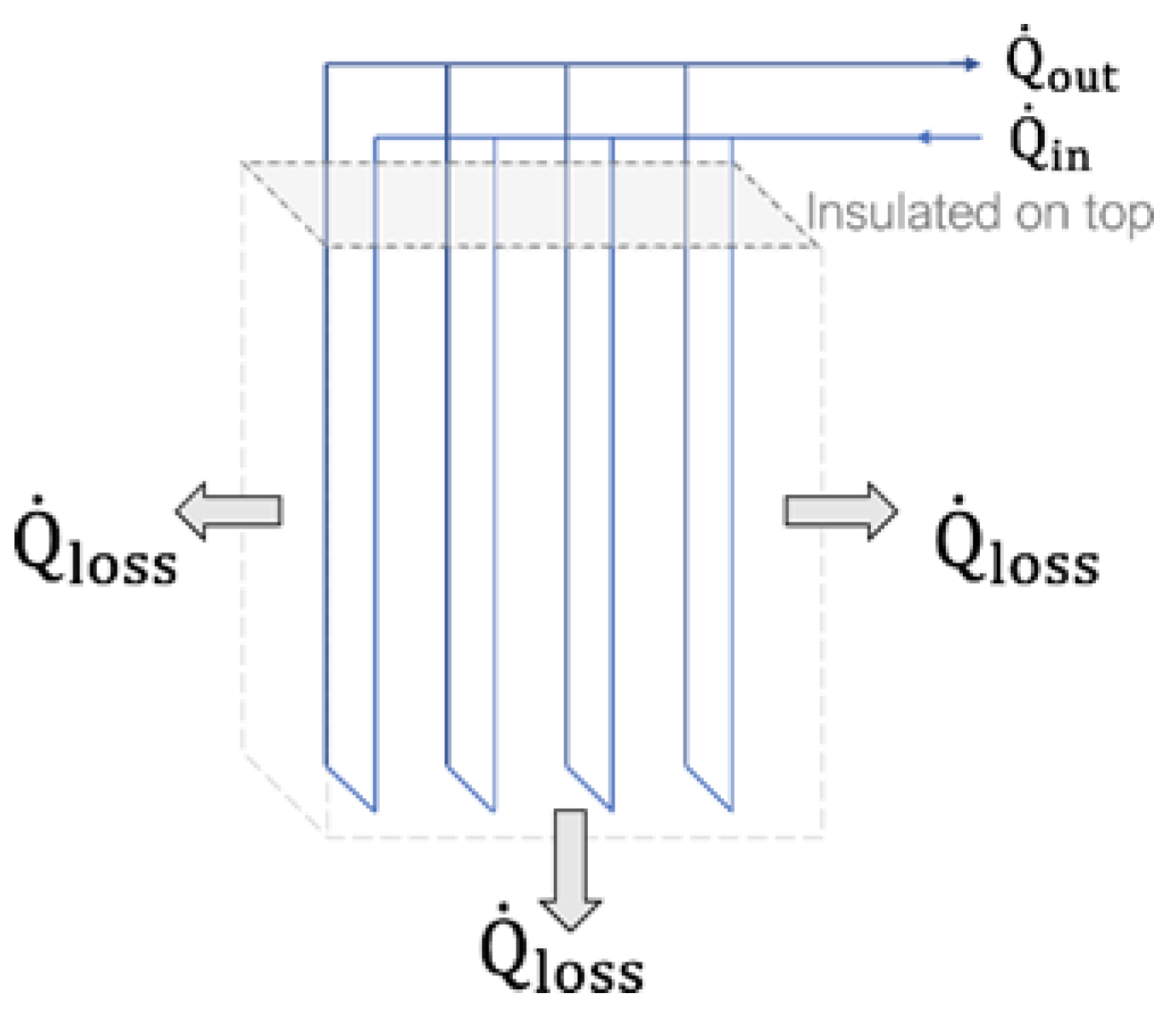
3.3. Thermal Storage Tank Model
3.4. Substation Model
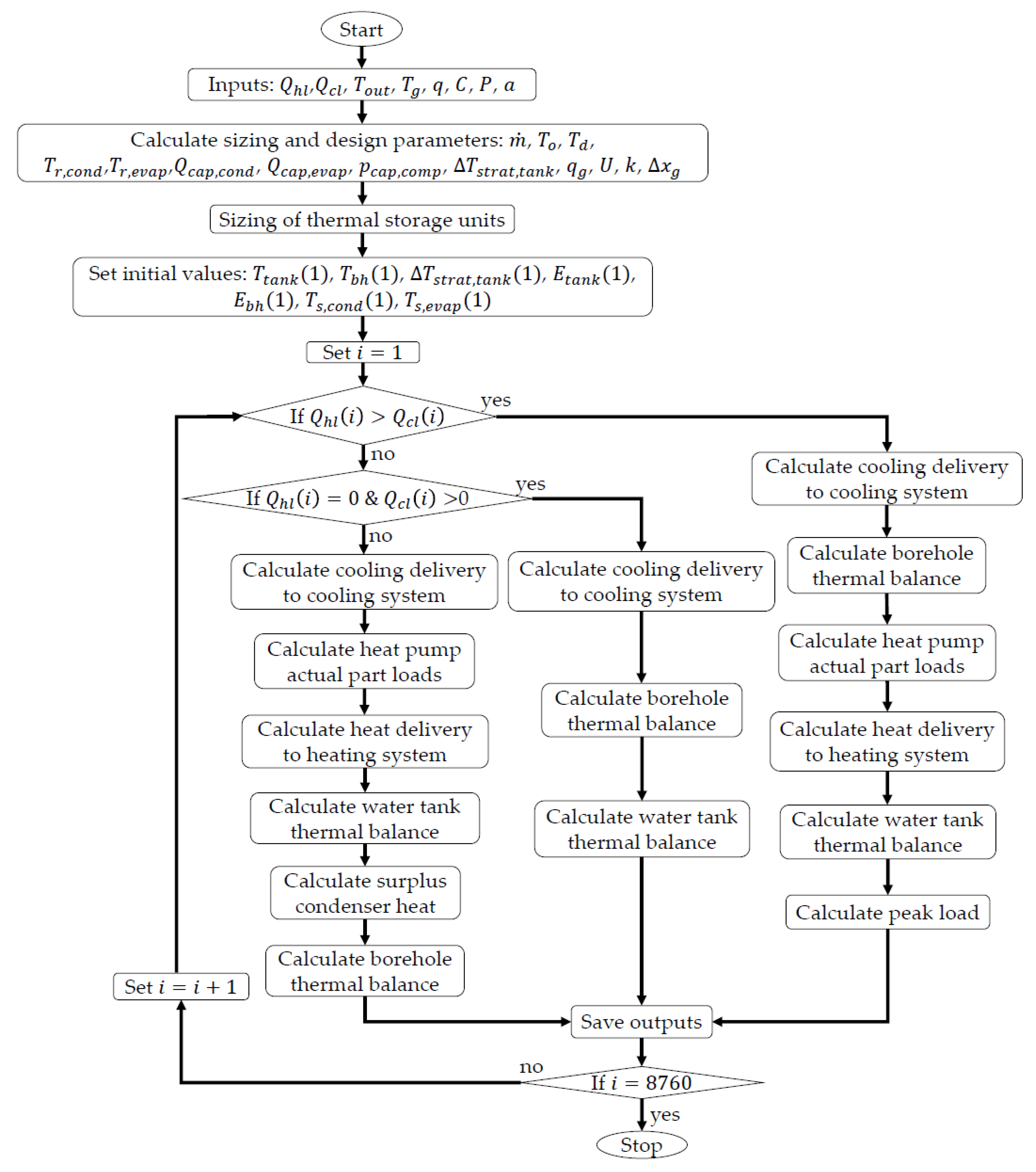
4. Integrated System Model
5. Sizing and Performance Analysis
6. Results
6.1. Annual Performance Analysis
6.2. Sensitivity Analysis
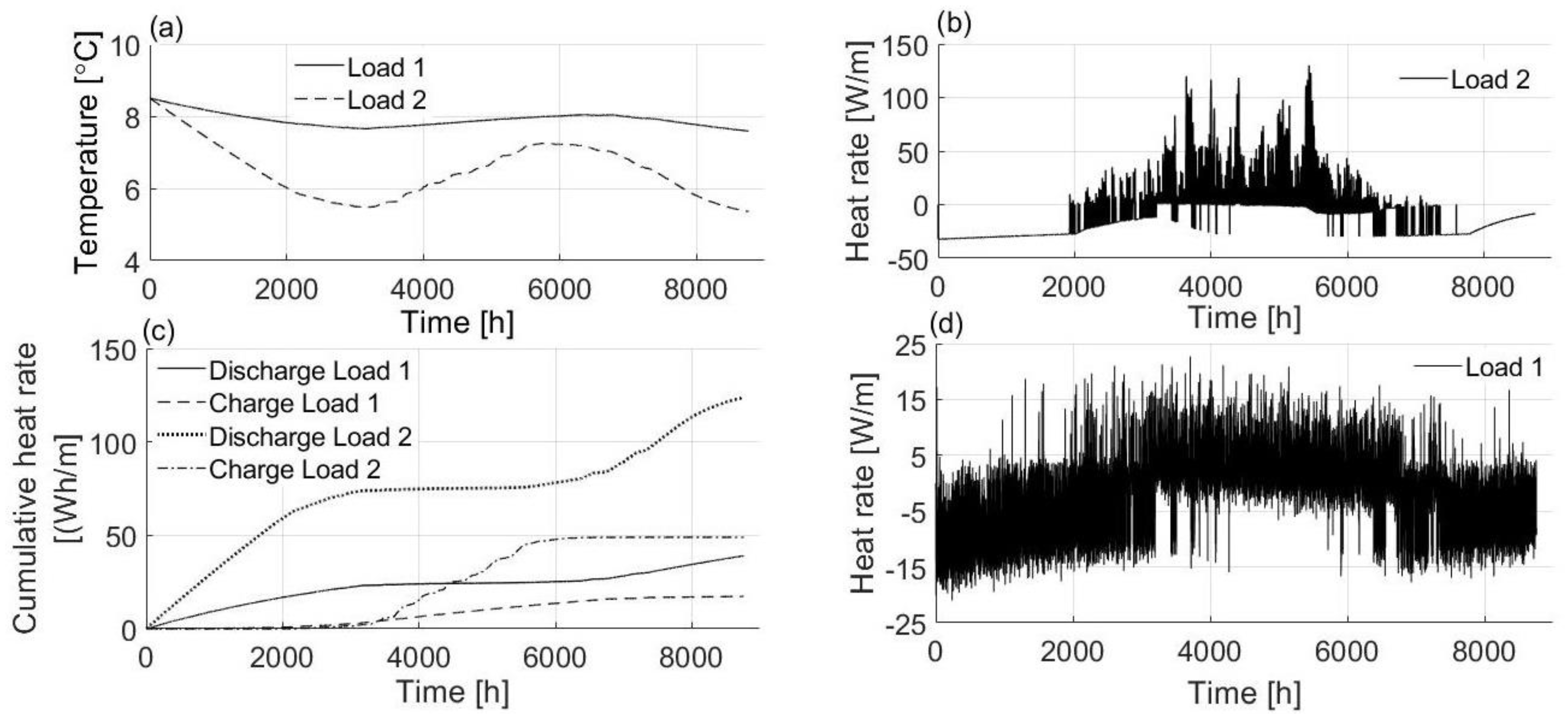
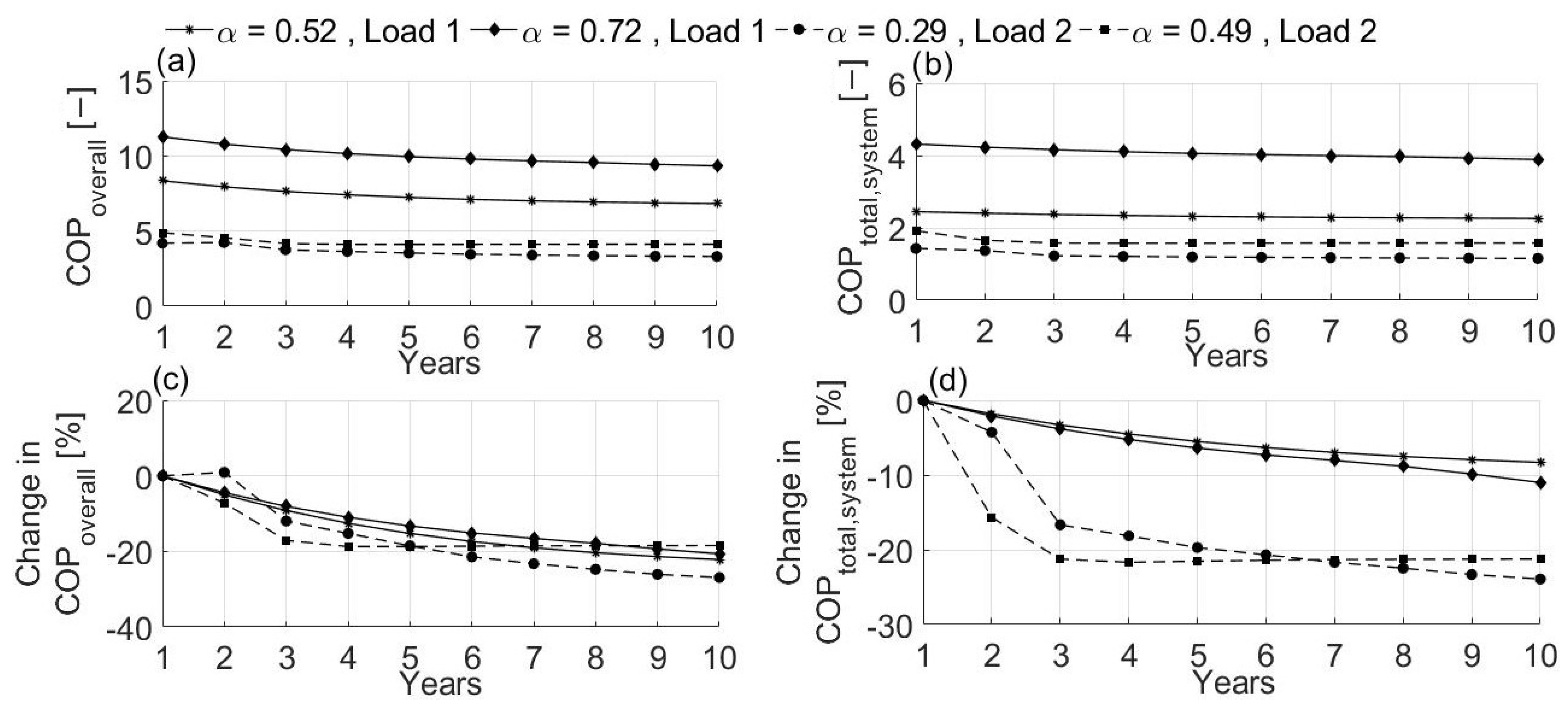
6.3. Long-Term Operation of the System
7. Discussion
8. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Variables and Abbreviations | |
| Cooling to heating ratio (−) | |
| Heat rate (W) | |
| Temperature (°C) | |
| t | Time (s) |
| Compressor power (W) | |
| Mass flow rate (kg/s) | |
| Part load ratio (−) | |
| Specific heat capacity (J/kg.K) | |
| Energy (J) | |
| Length (m) | |
| Volume (m3) | |
| Density (kg/m3) | |
| Thermal conductivity (W/m.K) | |
| Distance (m) | |
| Ground heat extraction rate (W) | |
| Depth (m) | |
| COP | Coefficient of performance |
| GCHP | Ground coupled heat pump |
| GSHP | Ground source heat pump |
| GHE | Ground heat exchanger |
| HWST | Hot water storage tank |
| BTES | Borehole thermal energy storage |
| Subscript | |
| cp | Capacity |
| ev | Evaporator |
| cd | Condenser |
| set | Setpoint |
| hp | Heat pump |
| max | Maximum |
| g | Ground |
| hl | Heating load |
| cl | cooling load |
| r | Return |
| s | Supply |
| strat | Stratification |
References
- Soltani, M.; Kashkooli, F.M.; Dehghani-Sanij, A.; Kazemi, A.; Bordbar, N.; Farshchi, M.; Elmi, M.; Gharali, K.; Dusseault, M.B. A comprehensive study of geothermal heating and cooling systems. Sustain. Cities Soc. 2019, 44, 793–818. [Google Scholar] [CrossRef]
- Eswiasi, A.; Mukhopadhyaya, P. Critical review on efficiency of ground heat exchangers in heat pump systems. Clean Technol. 2020, 2, 204–224. [Google Scholar] [CrossRef]
- Ahmadi, M.H.; Ahmadi, M.A.; Sadaghiani, M.S.; Ghazvini, M.; Shahriar, S.; Alhuyi Nazari, M. Ground source heat pump carbon emissions and ground-source heat pump systems for heating and cooling of buildings: A review. Environ. Prog. Sustain. Energy 2018, 37, 1241–1265. [Google Scholar] [CrossRef]
- Shin, D.U.; Ryu, S.R.; Kim, K.W. Simultaneous heating and cooling system with thermal storage tanks considering energy efficiency and operation method of the system. Energy Build. 2019, 205, 109518. [Google Scholar] [CrossRef]
- Byrne, P.; Ghoubali, R. Exergy analysis of heat pumps for simultaneous heating and cooling. Appl. Therm. Eng. 2019, 149, 414–424. [Google Scholar] [CrossRef]
- Rice, C.K.; Murphy, R.W.; Baxter, V.D. Design approach and performance analysis of a small integrated heat pump (IHP) for net zero energy homes (NEH). In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 14–17 July 2008. [Google Scholar]
- Sarbu, I.; Sebarchievici, C. General review of ground-source heat pump systems for heating and cooling of buildings. Energy Build. 2014, 70, 441–454. [Google Scholar] [CrossRef]
- Lanahan, M.; Tabares-Velasco, P.C. Seasonal thermal-energy storage: A critical review on btes systems, modeling, and system design for higher system efficiency. Energies 2017, 10, 743. [Google Scholar] [CrossRef] [Green Version]
- Moradi, A.; Smits, K.M.; Massey, J.; Cihan, A.; McCartney, J. Impact of coupled heat transfer and water flow on soil borehole thermal energy storage (sbtes) systems: Experimental and modeling investigation. Geothermics 2015, 57, 56–72. [Google Scholar] [CrossRef] [Green Version]
- Beier, R.A. Thermal response tests on deep borehole heat exchangers with geothermal gradient. Appl. Therm. Eng. 2020, 115447. [Google Scholar] [CrossRef]
- Hino, T.; Ooka, R. Integrated utilization of renewable energy by 2-stage heat pump system. In Proceedings of the Conference of the Air Conditioning and Sanitary Engineering Society, Nagoya, Japan, 12–14 September 2018; pp. 1–4. [Google Scholar]
- Rohde, D.; Andresen, T.; Nord, N. Analysis of an integrated heating and cooling system for a building complex with focus on long–term thermal storage. Appl. Therm. Eng. 2018, 145, 791–803. [Google Scholar] [CrossRef]
- Sircar, A.; Shah, M.; Vaidya, D.; Dhale, S.; Sahajpal, S.; Yadav, K.; Garg, S.; Sarkar, P.; Sharma, S.; Mishra, T. Performance simulation of ground source heat pump system based on low enthalpy geothermal systems. Emerg. Trends Chem. Eng. 2017, 4, 1–12. [Google Scholar]
- Rui, Y.; Garber, D.; Yin, M. Modelling ground source heat pump system by an integrated simulation programme. Appl. Therm. Eng. 2018, 134, 450–459. [Google Scholar] [CrossRef]
- Sarbu, I.; Sebarchievici, C. Using ground-source heat pump systems for heating/cooling of buildings. In Advances in Geothermal Energy; Books on Demand: Norderstedt, Germany, 2016. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.; Malhotra, M.; Im, P. Performance analysis of ground source heat pump demonstration projects in the united states, In Proceedings of the 12th IEA Heat Pump Conference, Rotterdam, The Netherlands, 15–18 May 2017.
- Ferrantelli, A.; Fadejev, J.; Kurnitski, J. A tabulated sizing method for the early stage design of geothermal energy piles including thermal storage. Energy Build. 2020, 223, 110178. [Google Scholar] [CrossRef]
- Li, H.; Nord, N. Transition to the 4th generation district heating- possibilities, bottlenecks, and challenges. Energy Procedia 2018, 149, 483–498. [Google Scholar] [CrossRef]
- Li, H.; Hou, J.; Hong, T.; Ding, Y.; Nord, N. Energy, economic, and environmental analysis of integration of thermal energy storage into district heating systems using waste heat from data centres. Energy 2021, 219, 119582. [Google Scholar] [CrossRef]
- Li, H.; Hou, J.; Hong, T.; Nord, N. Distinguish between the economic optimal and lowest distribution temperatures for heat-prosumer-based district heating systems with short-term thermal energy storage. Energy 2022, 248, 123601. [Google Scholar] [CrossRef]
- Li, H.; Hou, J.; Tian, Z.; Hong, T.; Nord, N.; Rohde, D. Optimize heat prosumers’ economic performance under current heating price models by using water tank thermal energy storage. Energy 2022, 239, 122103. [Google Scholar] [CrossRef]
- Cimmino, M.; Wetter, M. Modelling of heat pumps with calibrated parameters based on manufacturer data. In Proceedings of the 12th International Modelica Conference, Prague, Czech Republic, 15–17 May 2017; pp. 219–226. [Google Scholar]
- Kinab, E.; Fau, A.; Marchio, D.; Rivière, P. Model of a reversible heat pump for part load energy based optimization design. In Proceedings of the Conference Clima 2007 WellBeing Indoors, Helsinki, Finland, 10–14 June 2007. [Google Scholar]
- Fuentes, E.; Waddicor, D.; Fannan, M.O.; Salom, J. Improved methodology for testing the part load performance of water-to-water heat pumps, In Proceedings of the 12th IEA Heat Pump Conference, Rotterdam, The Netherlands, 15–18 May 2017.
- Bitzer Software. Available online: https://www.Bitzer.De/websoftware/ (accessed on 3 May 2022).
- Bitzer Software 6.17.0: Selection-Software for the Reliable Selection of Products from the Broad Product Range of Bitzer. Available online: https://www.bitzer.de/gb/en/ (accessed on 3 May 2022).
- Skarphagen, H.; Banks, D.; Frengstad, B.S.; Gether, H. Design considerations for borehole thermal energy storage (btes): A review with emphasis on convective heat transfer. Geofluids 2019, 2019, 4961781. [Google Scholar] [CrossRef]
- Ahmadfard, M.; Bernier, M. A review of vertical ground heat exchanger sizing tools including an inter-model comparison. Renew. Sustain. Energy Rev. 2019, 110, 247–265. [Google Scholar] [CrossRef]
- Javed, S.; Spitler, J. Calculation of borehole thermal resistance. In Advances in Ground-Source Heat Pump Systems; Elsevier: Amsterdam, The Netherlands, 2016; pp. 63–95. [Google Scholar]
- Persson, T.; Stavset, O.; Ramstad, R.K.; Alonso, M.J.; Lorenz, K. Software for modelling and simulation of ground source heating and cooling systems; SINTEF Energi AS: Trondheim, Norway, 2016. [Google Scholar]
- Hellström, G.; Sanner, B.; Earth Energy Designer. User’s Manual, Version 2. 2000. Available online: https://www.buildingphysics.com/manuals/eed.pdf (accessed on 3 May 2022).
- Giordano, N.; Chicco, J.; Mandrone, G.; Verdoya, M.; Wheeler, W.H. Comparing transient and steady-state methods for the thermal conductivity characterization of a borehole heat exchanger field in Bergen, Norway. Environ. Earth Sci. 2019, 78, 460. [Google Scholar] [CrossRef]
- Ramstad, R.K.; Midttømme, K.; Liebel, H.T.; Frengstad, B.S.; Willemoes-Wissing, B. Thermal conductivity map of the oslo region based on thermal diffusivity measurements of rock core samples. Bull. Eng. Geol. Environ. 2015, 74, 1275–1286. [Google Scholar] [CrossRef]
- Ferrantelli, A.; Fadejev, J.; Kurnitski, J. Energy pile field simulation in large buildings: Validation of surface boundary assumptions. Energies 2019, 12, 770. [Google Scholar] [CrossRef] [Green Version]
- Faanes, A.; Skogestad, S. Buffer tank design for acceptable control performance. Ind. Eng. Chem. Res. 2003, 42, 2198–2208. [Google Scholar] [CrossRef] [Green Version]
- Rahman, A.; Fumo, N.; Smith, A.D. Simplified modeling of thermal storage tank for distributed energy heat recovery applications, In Proceedings of the ASME 2015 9th International Conference on Energy Sustainability collocated with the ASME 2015 Power Conference, the ASME 2015 13th International Conference on Fuel Cell Science, Engineering and Technology, and the ASME 2015 Nuclear Forum, San Diego, CA, USA, 28 June–2 July 2015; Volume 2.
- Campos-Celador, Á.; Diarce, G.; Larrinaga, P.; García-Romero, A.M. A simple method for the design of thermal energy storage systems. Energy Storage 2020, 2, e140. [Google Scholar] [CrossRef]
- Cadau, N.; De Lorenzi, A.; Gambarotta, A.; Morini, M.; Rossi, M. Development and analysis of a multi-node dynamic model for the simulation of stratified thermal energy storage. Energies 2019, 12, 4275. [Google Scholar] [CrossRef] [Green Version]
- Abdelhak, O.; Mhiri, H.; Bournot, P. CFD analysis of thermal stratification in domestic hot water storage tank during dynamic mode. In Building Simulation; Springer: Berlin/Heidelberg, Germany, 2015; Volume 8, pp. 421–429. [Google Scholar]
- Chandra, Y.P.; Matuska, T. Stratification analysis of domestic hot water storage tanks: A comprehensive review. Energy Build. 2019, 187, 110–131. [Google Scholar] [CrossRef]
- Fernández, M.; Eguía, P.; Granada, E.; Febrero, L. Sensitivity analysis of a vertical geothermal heat exchanger dynamic simulation: Calibration and error determination. Geothermics 2017, 70, 249–259. [Google Scholar] [CrossRef]
- Han, C.; Yu, X.B. Sensitivity analysis of a vertical geothermal heat pump system. Appl. Energy 2016, 170, 148–160. [Google Scholar] [CrossRef] [Green Version]
- Bonanos, A.; Votyakov, E.V. Sensitivity analysis for thermocline thermal storage tank design. Renew. Energy 2016, 99, 764–771. [Google Scholar] [CrossRef]
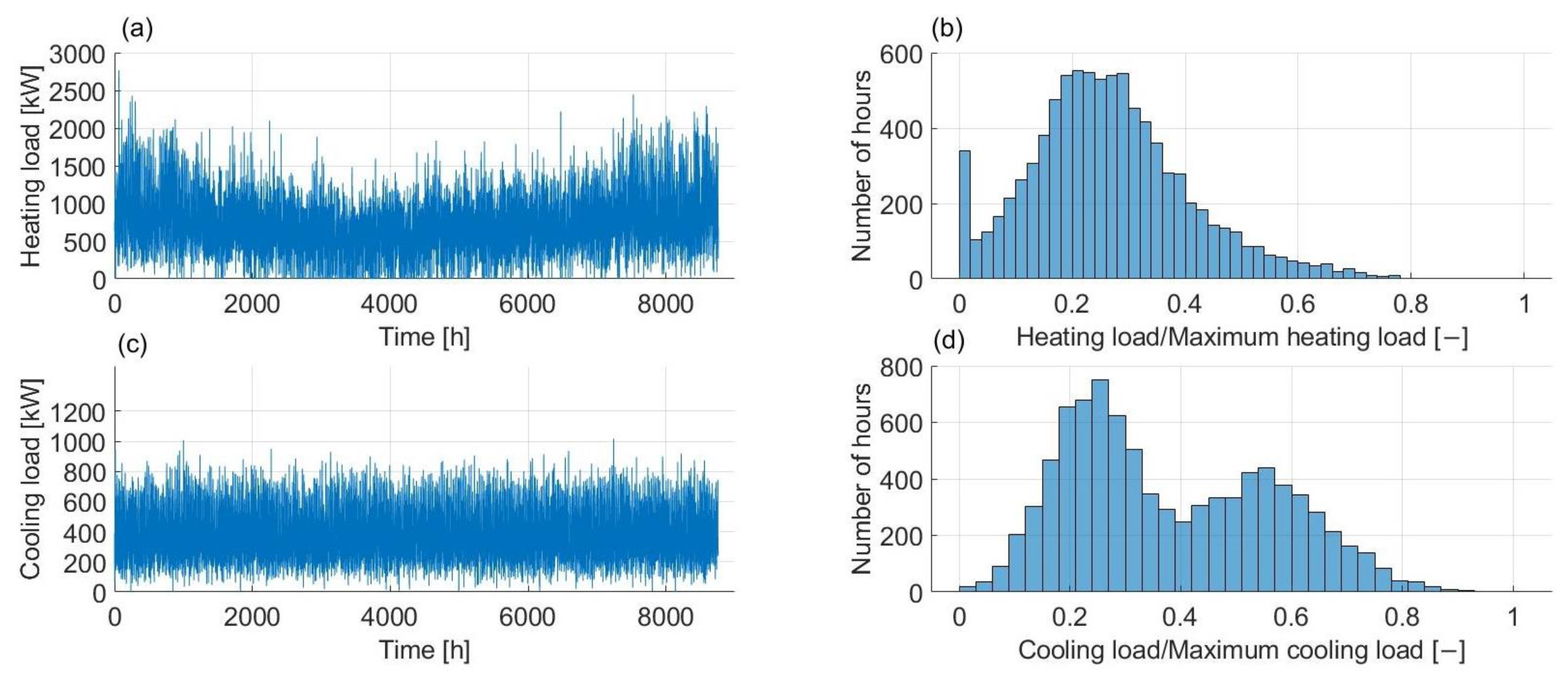
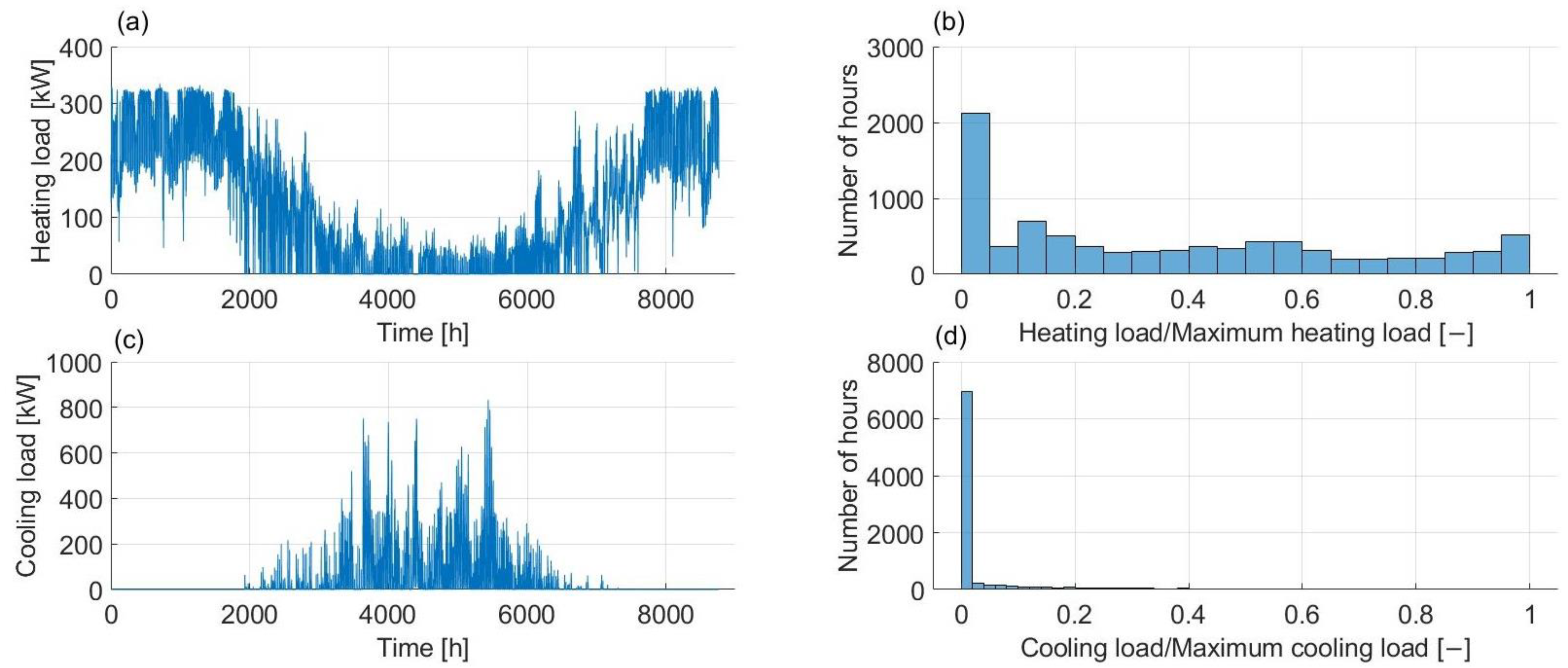

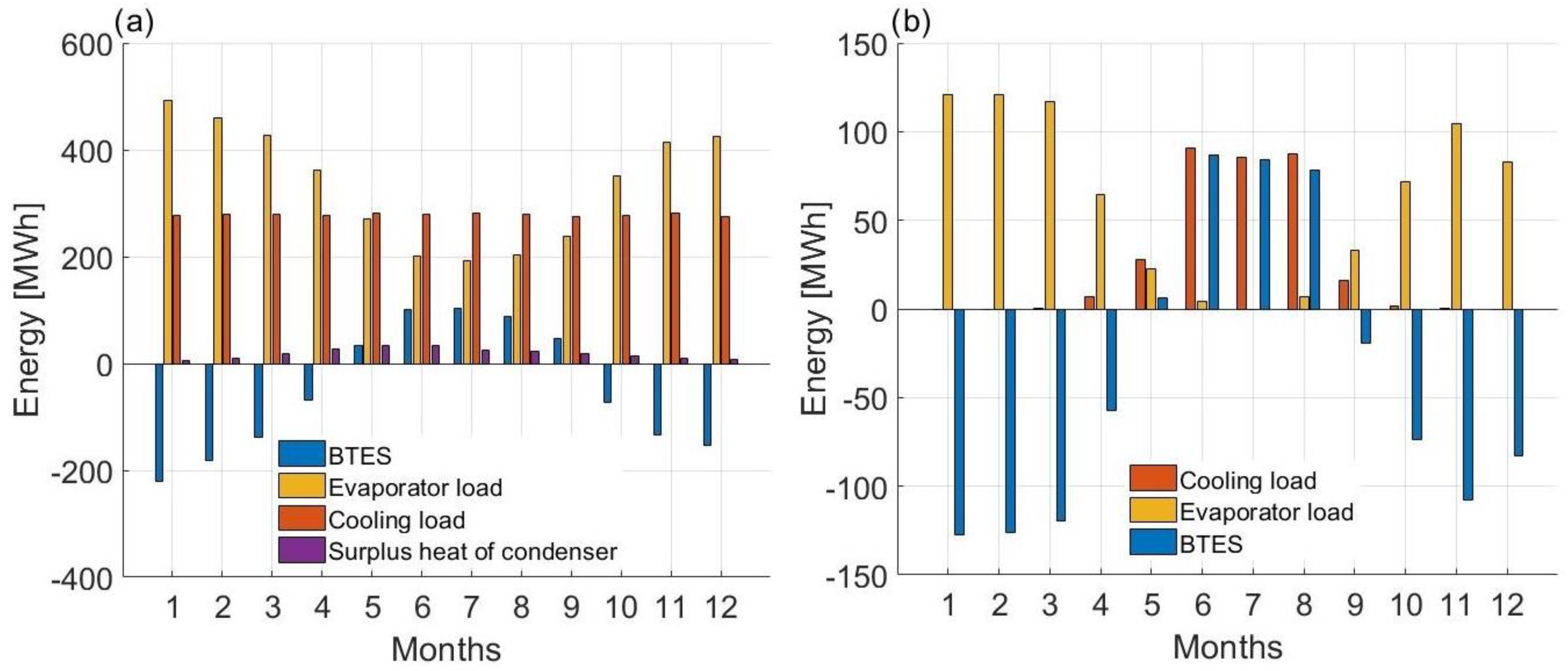

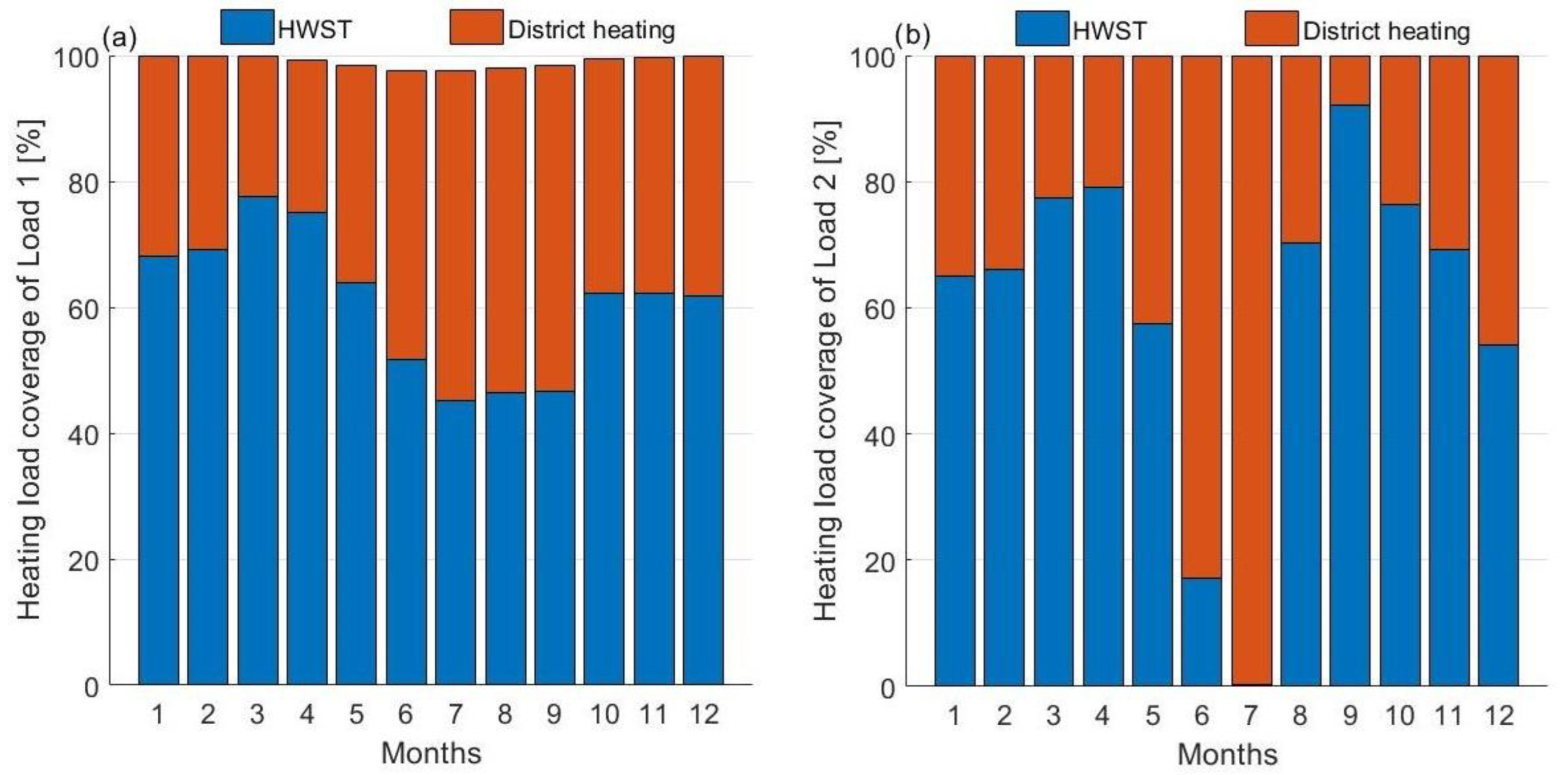

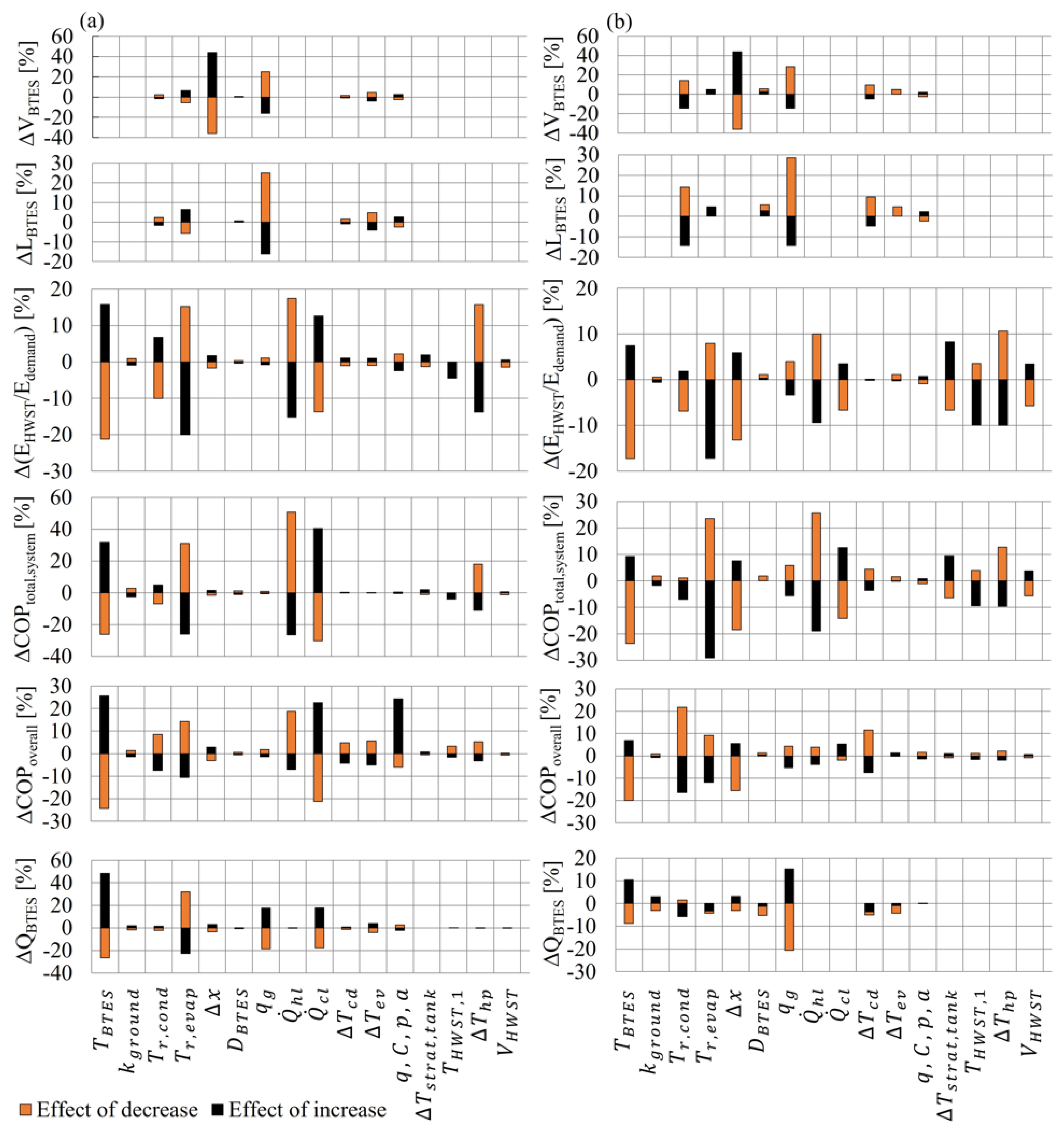
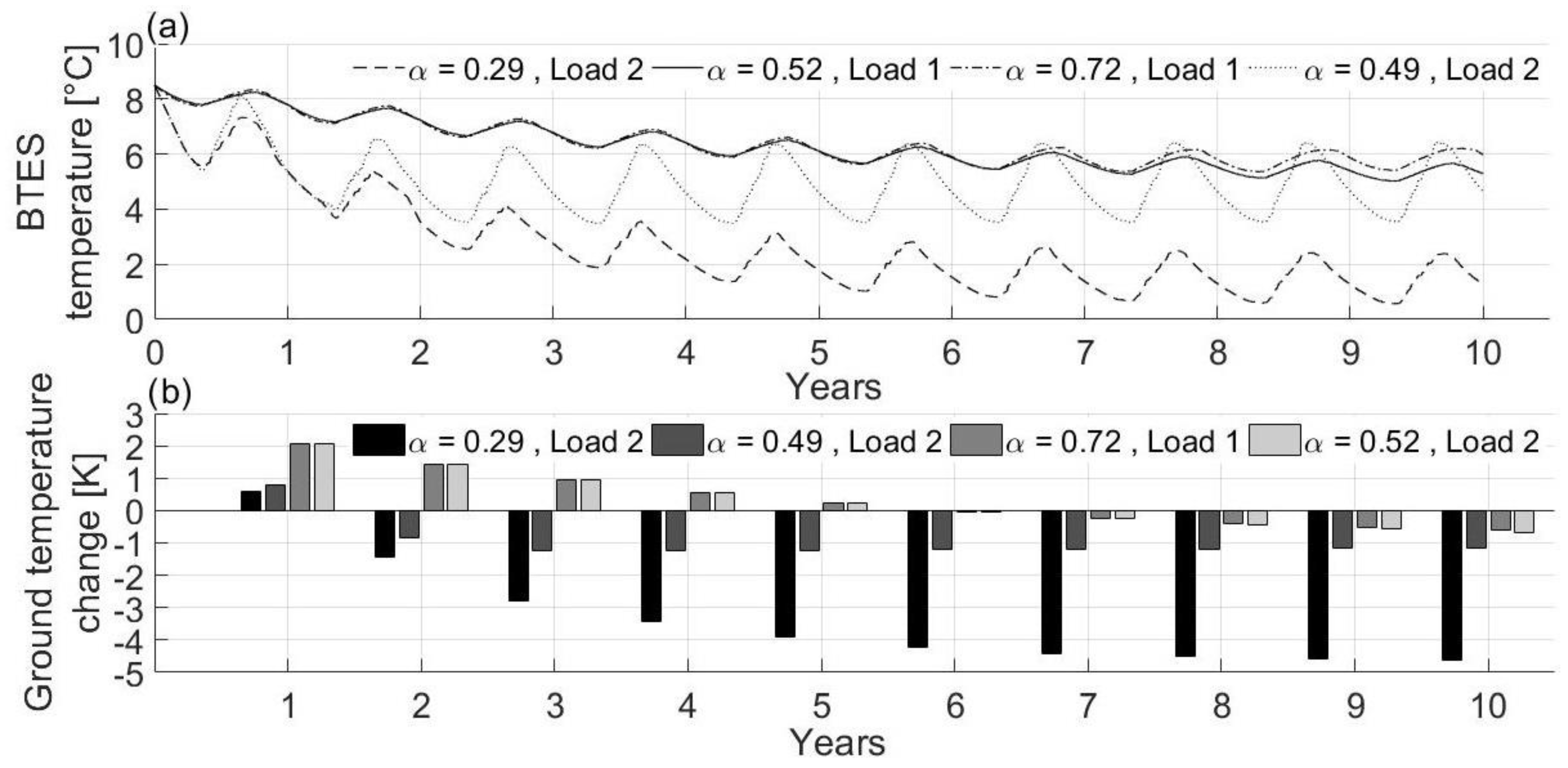
| Profiles | Demand | Maximum (kW) | Total (MWh) | α (−) |
|---|---|---|---|---|
| Load 1 | Heating | 2768 | 6436 | 0.52 |
| Cooling | 1015 | 3386 | ||
| Load 2 | Heating | 334.7 | 1073.4 | 0.29 |
| Cooling | 832.5 | 316.2 |
| Profiles | Load 1 | Load 2 |
|---|---|---|
| Type | OSKA95103-K | 8FE-70Y |
| Working fluid | R717 | R404A |
| Evaporation temperature | 0 °C/2 °C | 0 °C/2 °C |
| Condensation temperature | 25 °C/29 °C | 40 °C/42 °C |
| Evaporator capacity | 1116 kW | 187 kW |
| Condenser capacity | 1248 kW | 248 kW |
| COP (nominal/Carnot) | 9.40/13.02 | 4.06/8.15 |
| Parameter | Value |
|---|---|
| Ground heat extraction rate (W/m) | 30 |
| Ground thermal conductivity (W/m∙K) | 3.5 |
| The average temperature of the unaffected ground (°C) | 6 |
| Length to the unaffected ground (m) | 10 |
| Borehole depth (m) | 300 |
| Ground material density (kg/m3) | 2800 |
| Ground heat capacity (kJ/kg·K) | 0.85 |
| Heat carrier fluid density (kg/m3) | 971 |
| Heat carrier fluid heat capacity (kJ/kg·K) | 4.298 |
| The center-to-center distance between boreholes (m) | 6 |
| Borehole diameter (mm) | 110 |
| U-pipe diameter (mm) | 32 |
| U-pipe thermal conductivity (W/m·K) | 0.42 |
| Filling material thermal conductivity (W/m·K) | 0.6 |
| Heat carrier fluid thermal conductivity (W/m·K) | 0.43 |
| Parameter | Unit | Base Value | Change |
|---|---|---|---|
| Borehole storage initial temperature | °C | 8.5 | ±20% |
| Ground conductivity | W/(m·K) | 2.6 | ±20% |
| Temperature setpoint for heating side | °C | 50/55 | ±20% |
| Temperature setpoint for cooling side | °C | 5/7 | ±20% |
| Borehole pipe distances | m | 6 | ±20% |
| Borehole depth | m | 300 | ±20% |
| Ground heat rate | W/m | 30 | ±20% |
| Heating load | kW | 100% | ±20% |
| Cooling load | kW | 100% | ±20% |
| K | 25 | ±20% | |
| K | 5 | ±20% | |
| Heat pump performance coefficients | - | 100% | ±10% |
| K | 0–40 | ±20% | |
| HWST initial temperature | °C | 40 | ±20% |
| Temperature drop around the condenser | K | 40–45 | ±20% |
| Volume of HWST (Load 1/Load 2) ( | m3 | 35.59/6.45 | ±20% |
| Variable | Unit | Load 1 | Load 2 |
| Average COP (heating/cooling/overall) | - | 4.54/3.57/8.34 | 2.20/1.56/5.99 |
| - | 2.45 | 2.01 | |
| Borehole storage temperature (max/min/mean) | °C | 8.5/7.78/8.05 | 8.5/5.38/6.57 |
| HWST temperature (max/min/mean) | °C | 83.91/42.9/53.48 | 92.56/39.45/58.63 |
| Annual electricity use | MWh | 1117.4 | 303.14 |
| HWST coverage ratio | % | 61 | 65 |
| Annual peak load energy | MWh | 2435.4 | 366.56 |
| Annual surplus heat | MWh | 223.10 | 0 |
| Part load operation hours | h | 5995 | 4505 |
| Maximum BTES extraction/injection | W/m | −19.51/23.82 | −28.31/131.69 |
| Maximum tank extraction/injection | kW | −1450.7/718.45 | −162.55/176.69 |
| Volume of HWST | m3 | 35.59 | 6.45 |
| Total BTES volume | m3 | 1,339,200 | 226,800 |
| Number of boreholes | - | 124 | 21 |
| Total BTES field area | m2 | 4464 | 756 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shakerin, M.; Eikeskog, V.; Li, Y.; Harsem, T.T.; Nord, N.; Li, H. Investigation of Combined Heating and Cooling Systems with Short- and Long-Term Storages. Sustainability 2022, 14, 5709. https://doi.org/10.3390/su14095709
Shakerin M, Eikeskog V, Li Y, Harsem TT, Nord N, Li H. Investigation of Combined Heating and Cooling Systems with Short- and Long-Term Storages. Sustainability. 2022; 14(9):5709. https://doi.org/10.3390/su14095709
Chicago/Turabian StyleShakerin, Mohammad, Vilde Eikeskog, Yantong Li, Trond Thorgeir Harsem, Natasa Nord, and Haoran Li. 2022. "Investigation of Combined Heating and Cooling Systems with Short- and Long-Term Storages" Sustainability 14, no. 9: 5709. https://doi.org/10.3390/su14095709
APA StyleShakerin, M., Eikeskog, V., Li, Y., Harsem, T. T., Nord, N., & Li, H. (2022). Investigation of Combined Heating and Cooling Systems with Short- and Long-Term Storages. Sustainability, 14(9), 5709. https://doi.org/10.3390/su14095709








