Analysis for Ground Deformation Induced by Undercrossed Shield Tunnels at a Small Proximity Based on Equivalent Layer Method
Abstract
:1. Introduction
2. Project Overview
2.1. Geological Conditions
2.2. Driving Paremeters of EPBS
2.3. Arrangement of Measurement
3. Equivalent Layer Method
3.1. Model of Equivalent Layer
3.2. Numerical Model of Equivalent Layer Method
4. Numerical Simulation
4.1. Numerical Model
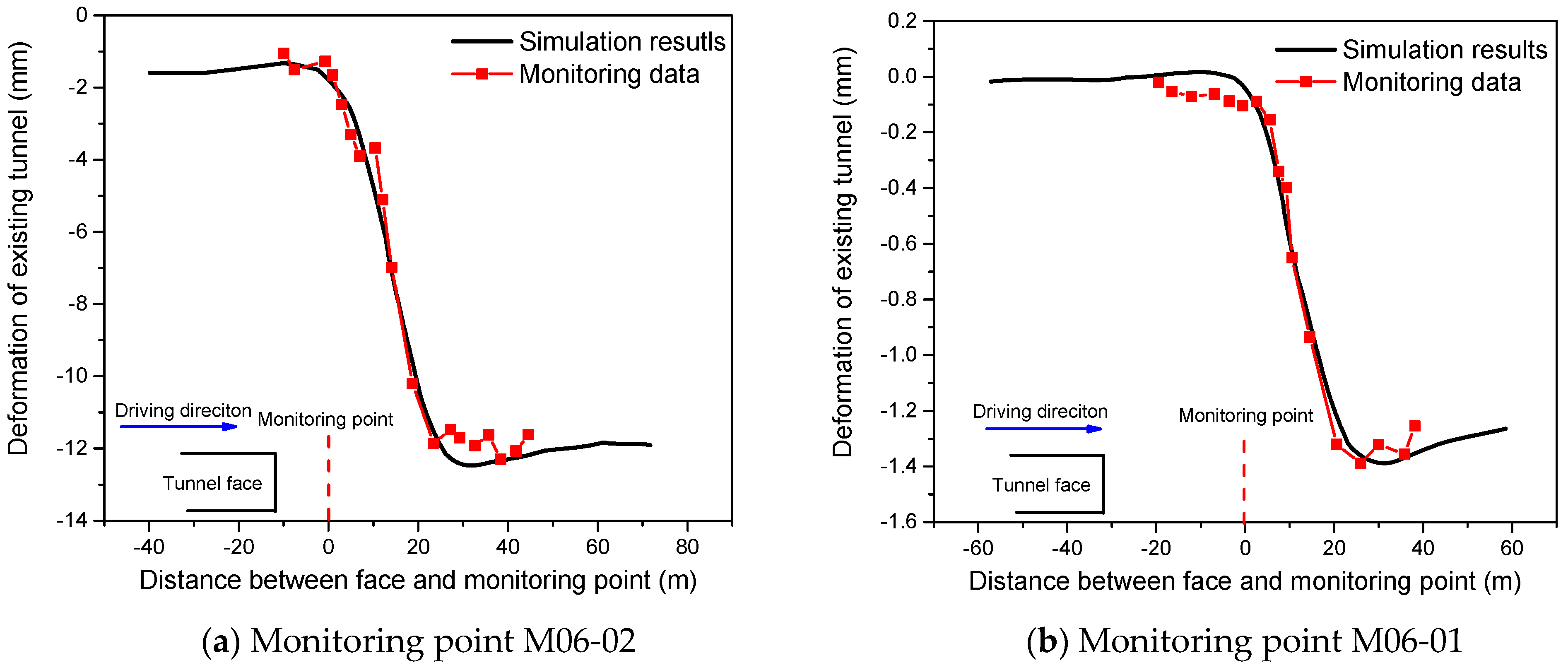
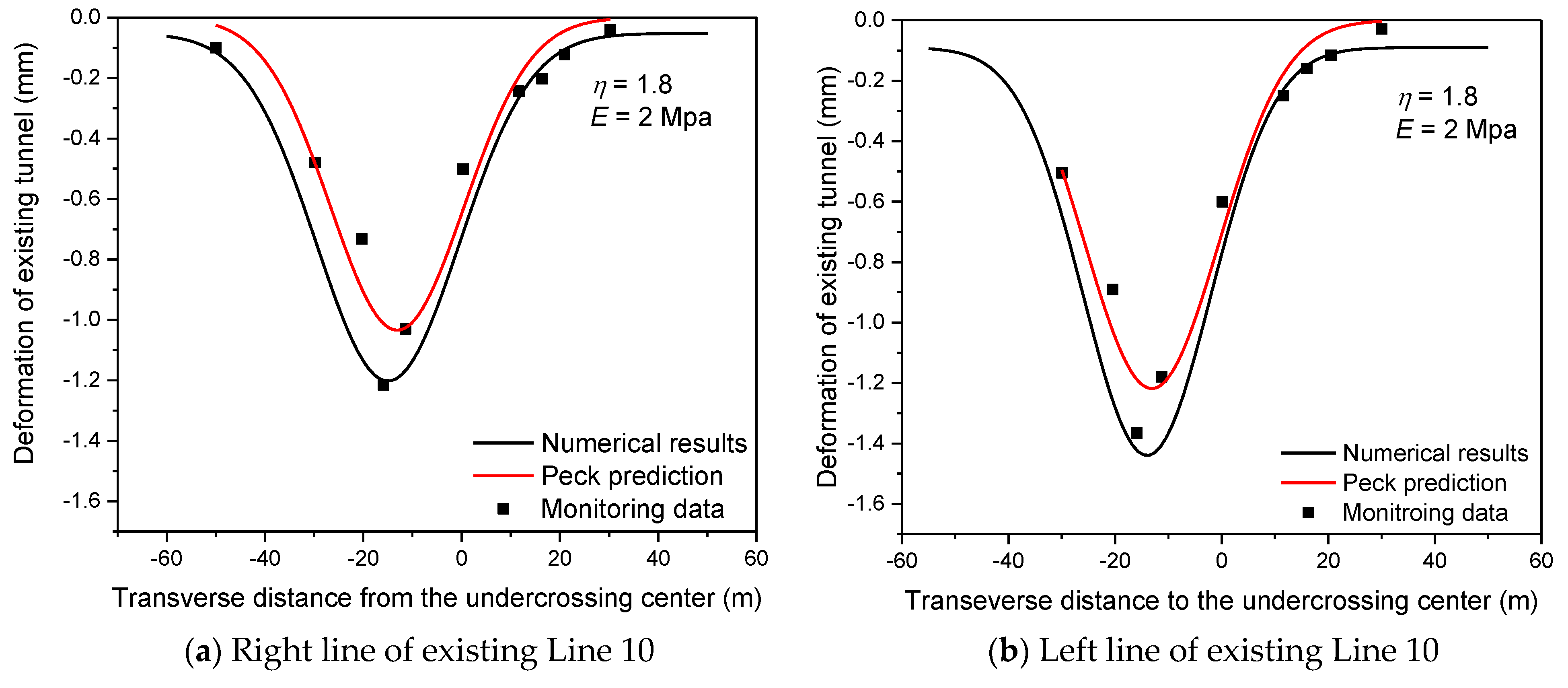
4.2. Numerical Results
5. Conclusions
- (1)
- The equivalent layer method uses a homogeneous, equal thickness, elastic soil layer to simulate the reinforced soil around the tunnel linings. Ground deformation is simulated by replacing the actual soil layer and grouting around the lining with the equivalent layer. The equivalent layer method is simple to use in numerical analysis to analyze the construction and tail grouting of shield tunnel with wide applicability.
- (2)
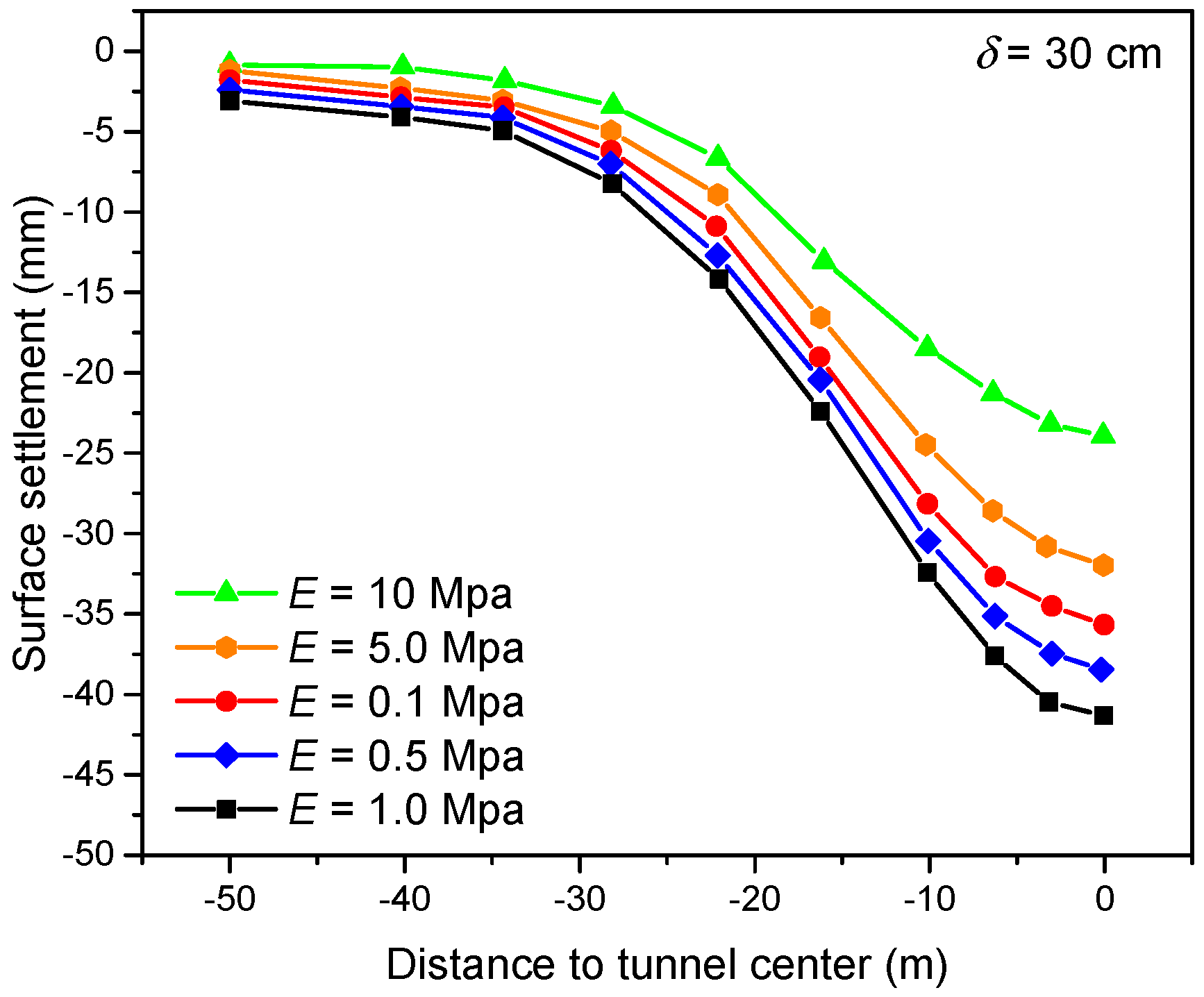
- The parametric analysis was carried out to study the influence of the thickness (δ) and elastic modulus (E) of the equivalent layer on surface settlement. The surface settlement increases almost linearly with the increase in the δ. The surface deformation is insensitive to changes in E. When the E of the equivalent layer is very large (10 MPa) or small (0.1 MPa), the surface settlement is rather small, while when the E of equivalent layer is in the middle value (1 MPa), the surface settlement is large. The δ is the dominating factor affecting the surface settlement.
- (3)
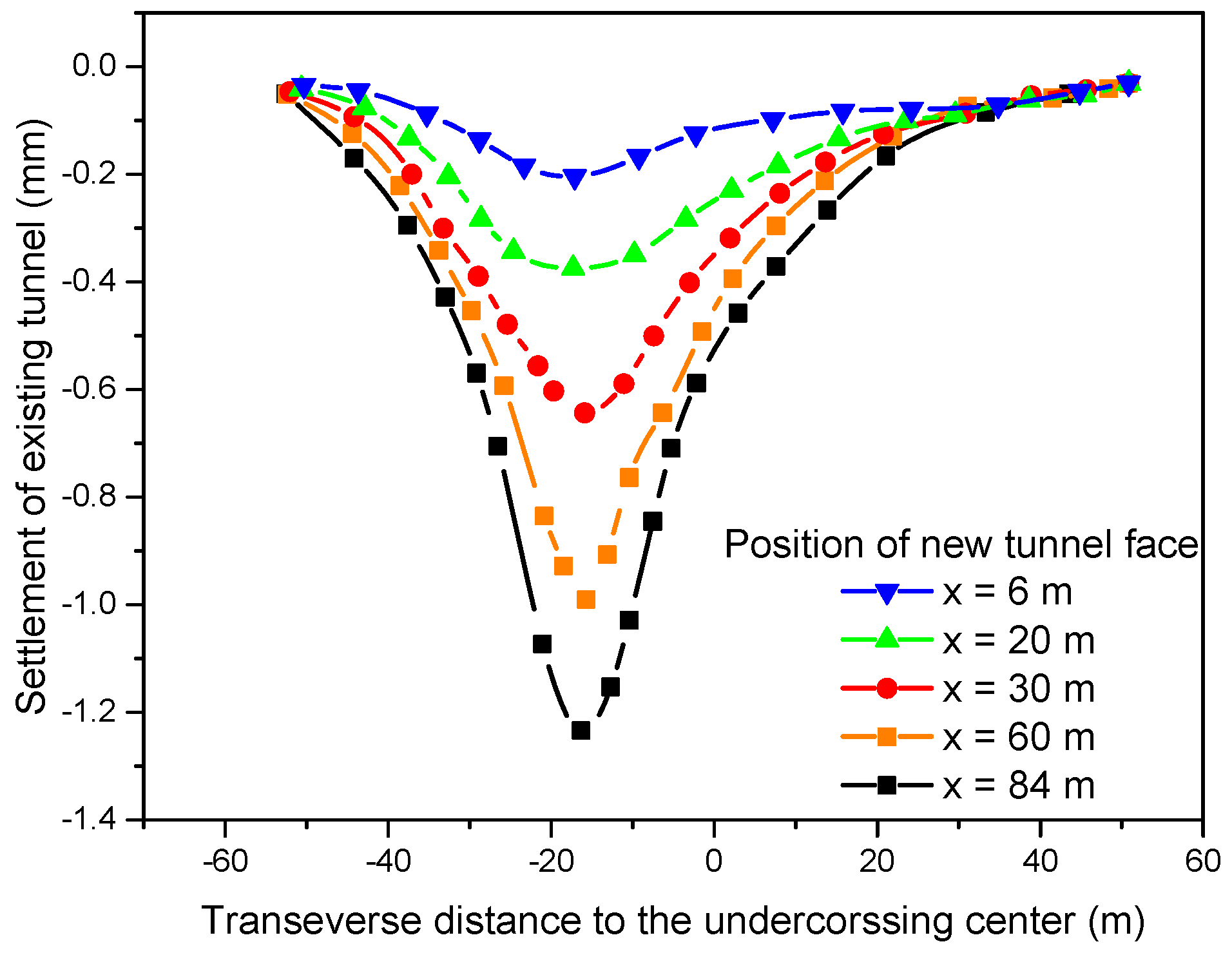
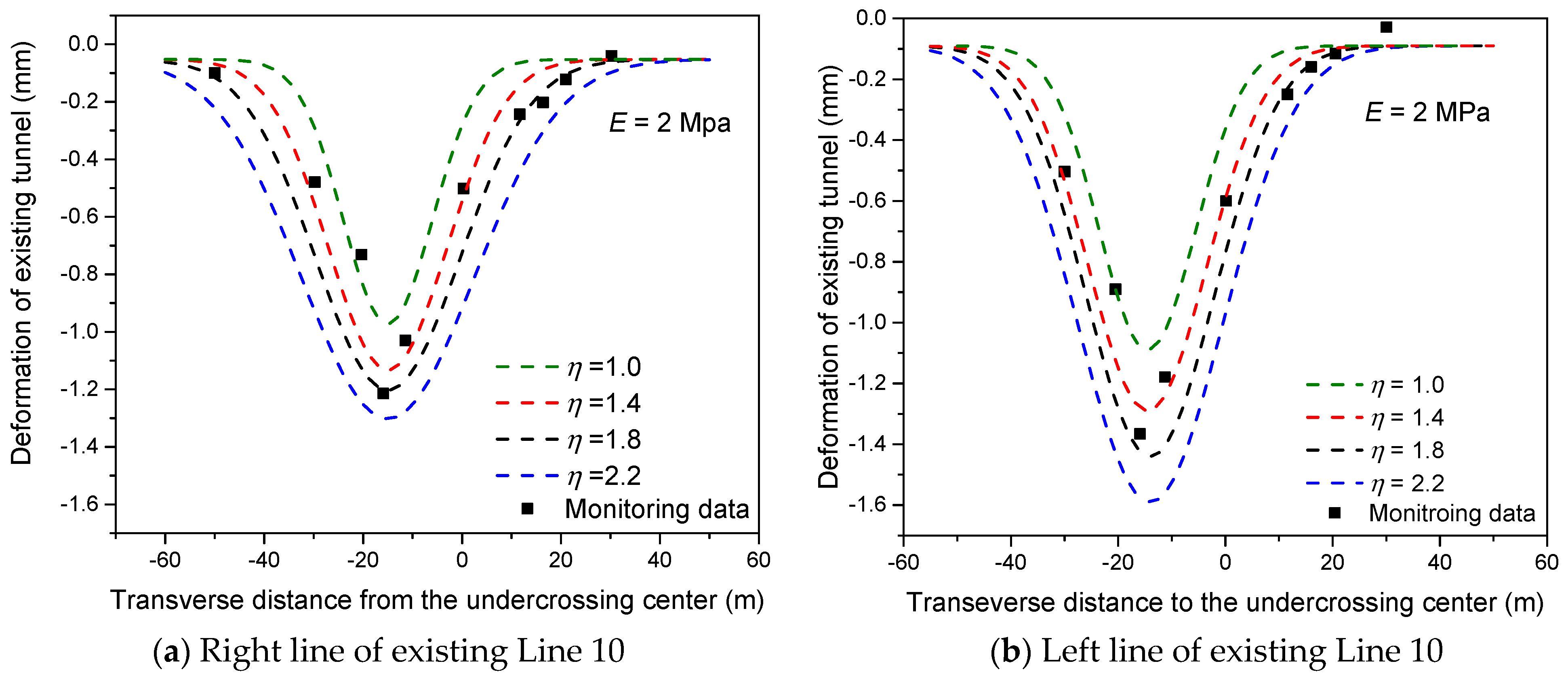
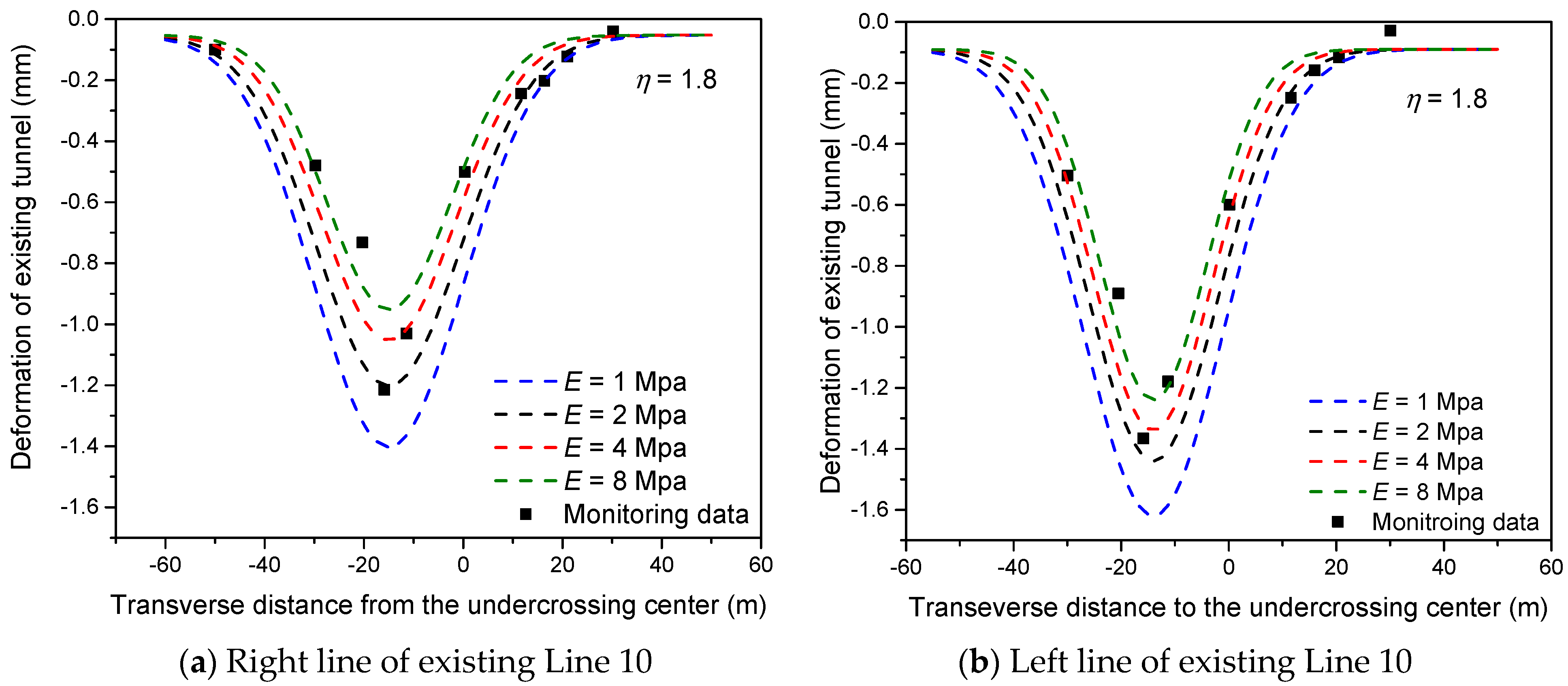
- Based on the case study of Beijing Metro Lines project, a numerical model was established with the equivalent layer method. The predicted surface settlement was analyzed and compared with monitoring data. The applicability of the equivalent layer method was verified. Tunnel face excavation was the main cause of surface settlement. Empirical values for the equivalent layer method were summarized, which were η = 1.8 and E equal to that of cement soil. The empirical values summarized from field data could be a reference for other shield tunnel constructions in soft soil. The accurate prediction using methods in this paper shows the influence of tail grouting on ground deformation; it could control the volume of grouting materials in construction, which is beneficial for reducing budgets and environmental protection.
- (4)
- There are still some limitations in this paper. The research in this paper was carried out based on the case study of Beijing Metro Line 10 and 12, and the empirical values of the equivalent layer method obtained are more suitable for soft soil. The empirical values for sand or other special soils require more back-analysis based on field cases. The E of the equivalent layer is derived from the empirical values and back analysis. If the grouting layer can be sampled on-site, a more accurate prediction can be obtained, and the empirical value can be compared and corrected. In the future, more research can be carried out based on the case of tunnels excavated in different soils.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| Notation | |
| Thickness of equivalent layer; | |
| E | Elastic modulus; |
| Computed shield tail gap; | |
| Coefficient of equivalent layer method; | |
| k | Permeability; |
| Unit weight; | |
| Compression modulus; | |
| c′ | Effective cohesion; |
| Effective internal friction angle; | |
| Poisson’s ratio; | |
| t | Thickness of lining; |
| Secant stiffness in standard drained triaxial test; | |
| Tangent stiffness for primary oedometer loading; | |
| Unloading/reloading stiffness from drained triaxial test; | |
| Reference shear modulus at very small strains; | |
| m | Power exponent; |
| Threshold shear strain; | |
| The settlement at the offset distance x from the tunnel center line; | |
| The maximum settlement above the tunnel center line; | |
| i | The width coefficient of the surface settlement trough. |
Appendix A
References
- Tang, X.W.; Gan, P.L.; Liu, W.; Zhao, Y. Surface Settlements Induced by Tunneling in Permeable Strata: A Case History of Shenzhen Metro. J. Zhejiang Univ.-Sci. A 2017, 18, 757–775. [Google Scholar] [CrossRef]
- Liu, W.; Zhao, Y.; Shi, P.; Li, J.; Gan, P. Face Stability Analysis of Shield-Driven Tunnels Shallowly Buried in Dry Sand Using 1-g Large-Scale Model Tests. Acta Geotech. 2018, 13, 693–705. [Google Scholar] [CrossRef]
- Yang, H.; Shi, H.; Jiang, X.; Liu, C. Influence of Construction Process of Double-Line Shield Tunnel Crossing Frame Structure on Ground Settlement. Geotech. Geol. Eng. 2020, 38, 1531–1545. [Google Scholar] [CrossRef]
- Tang, X.; Liang, J.; Liu, W.; Ye, Y.; Yu, Y. Modification of Peck Formula to Predict Ground Surface Settlement of Twin Tunnels in Low Permeability Soil. Adv. Civ. Eng. 2021, 2021, 6698673. [Google Scholar] [CrossRef]
- Liu, W.; Wu, B.; Shi, P.; Xu, X. Analysis on Face Stability of Rectangular Cross-Sectional Shield Tunneling Based on an Improved Two-Dimensional Rotational Mechanism. Acta Geotech. 2021, 16, 3725–3738. [Google Scholar] [CrossRef]
- Liu, W.; Wu, Y.; Zhao, H.; Xu, X.; Miao, L. Deformations of Subway Tunnels Induced by the Overcrossing Jacked Box Tunnels. Symmetry 2021, 13, 1800. [Google Scholar] [CrossRef]
- Peila, D.; Chieregato, A.; Martinelli, D.; Salazar, C.O.; Shch, R.; Boscaro, A.; Dal Negre, E.; Picchio, A. Long Term Behavior of Two Component Back-Fill Grout Mix Used in Full Face Mechanized Tunneling. 2015. Available online: https://core.ac.uk/download/pdf/76530807.pdf (accessed on 10 July 2022).
- Peck, R.B. Deep Excavations and Tunnelling in Soft Ground. In Proceedings of the 7th International Conference on Soil Mechanics and Foundations, Mexico City, Mexico, 1969; pp. 225–290. Available online: https://www.issmge.org/uploads/publications/1/38/1969_03_0005.pdf (accessed on 10 July 2022).
- O’Reilly, M.P.; New, B.M. Settlement above Tunnels in the United Kingdom—Their Magnitude and Prediction. In Tunnelling 82, Proceedings of the 3rd International Symposium, Brighton, UK, 7–11 June 1982; IMM: London, UK, 1982; pp. 173–181. [Google Scholar]
- Attewell, P.B.; Woodman, J.P. Predicting the Dynamics of Ground Settlement and Its Derivatives Caused by Tunnelling in Soil. Ground Eng. 1982, 15, 13–22. [Google Scholar]
- Suwansawat, S.; Einstein, H.H. Describing Settlement Troughs over Twin Tunnels Using a Superposition Technique. J. Geotech. Geoenviron. Eng. 2007, 133, 445–468. [Google Scholar] [CrossRef]
- Lin, Q.; Tian, Y.; Lu, D.; Gong, Q.; Du, X.; Gao, Z. A Prediction Method of Ground Volume Loss Variation with Depth Induced by Tunnel Excavation. Acta Geotech. 2021, 16, 3689–3707. [Google Scholar] [CrossRef]
- Loganathan, N. Analytical Prediction for Tunneling-Induced Ground Movements in Clays. J. Geotech. Geoenviron. Eng. 1998, 124, 846–856. [Google Scholar] [CrossRef]
- Chou, W.; Bobet, A. Predictions of Ground Deformations in Shallow Tunnels in Clay—ScienceDirect. Tunn. Undergr. Space Technol. Inc. Trenchless Technol. Res. 2002, 17, 3–19. [Google Scholar] [CrossRef]
- Bernat, S.; Cambou, B. Soil-Structure Interaction in Shield Tunnelling in Soft Soil. Comput. Geotech. 1998, 22, 221–242. [Google Scholar] [CrossRef]
- Chakeri, H.; Ozcelik, Y.; Unver, B. Investigation of Ground Surface Settlement in Twin Tunnels Driven with EPBM in Urban Area. Arab. J. Geosci. 2015, 8, 7655–7666. [Google Scholar] [CrossRef]
- Zhang, Z.X.; Liu, C.; Huang, X.; Kwok, C.Y.; Teng, L. Three-Dimensional Finite-Element Analysis on Ground Responses during Twin-Tunnel Construction Using the URUP Method. Tunn. Undergr. Space Technol. 2016, 58, 133–146. [Google Scholar] [CrossRef]
- Liang, J.; Tang, X.; Wang, T.; Ye, Y.; Liu, Y. Numerical Analysis of the Influence of a River on Tunnelling-Induced Ground Deformation in Soft Soil. J. Zhejiang Univ. Sci. A 2022, 23, 564–578. [Google Scholar] [CrossRef]
- Addenbrooke, T.I.; Potts, D.M. Twin Tunnel Interaction: Surface and Subsurface Effects. Int. J. Geomech. 2001, 1, 249–271. [Google Scholar] [CrossRef]
- Mollon, G.; Dias, D.; Soubra, A.-H. Probabilistic Analyses of Tunneling-Induced Ground Movements. Acta Geotech. 2013, 8, 181–199. [Google Scholar] [CrossRef]
- Do, N.-A.; Dias, D.; Oreste, P.; Djeran-Maigre, I. Three-Dimensional Numerical Simulation of a Mechanized Twin Tunnels in Soft Ground. Tunn. Undergr. Space Technol. 2014, 42, 40–51. [Google Scholar] [CrossRef]
- Zhang, Y.; Yin, Z.; Xu, Y. Analysis on Three-Dimensional Ground Surface Deformations due to Shield Tunnel. Chin. J. Rock Mech. Eng. 2002, 21, 388–392. (In Chinese) [Google Scholar]
- Yoo, C. Interaction between Tunneling and Groundwater—Numerical Investigation Using Three Dimensional Stress–Pore Pressure Coupled Analysis. J. Geotech. Geoenviron. Eng. 2005, 131, 240–250. [Google Scholar] [CrossRef]
- Song, J.; Miao, L.; Li, C.; Liu, Z. Construction Effect Analysis of near Tunnel Based on the Ground-Equivalent Circle Zone-Lining Model. In Proceedings of the 2011 International Conference on Electric Technology and Civil Engineering (ICETCE), Jiujiang, China, 22–24 April 2011; pp. 2059–2062. [Google Scholar]
- Liu, C.; Zhang, Z.; Regueiro, R.A. Pile and Pile Group Response to Tunnelling Using a Large Diameter Slurry Shield—Case Study in Shanghai. Comput. Geotech. 2014, 59, 21–43. [Google Scholar] [CrossRef]
- Xie, X.; Yang, Y.; Ji, M. Analysis of Ground Surface Settlement Induced by the Construction of a Large-Diameter Shield-Driven Tunnel in Shanghai, China. Tunn. Undergr. Space Technol. 2016, 51, 120–132. [Google Scholar] [CrossRef]
- Zhou, Z.; Chen, Y.; Liu, Z.; Miao, L. Theoretical Prediction Model for Deformations Caused by Construction of New Tunnels Undercrossing Existing Tunnels Based on the Equivalent Layered Method. Comput. Geotech. 2020, 123, 103565. [Google Scholar] [CrossRef]
- Tong, F.; Yang, J.; Duan, M.Q.; Ma, X.F.; Li, G.C. The Numerical Simulation of Rock Mass Grouting: A Literature Review. Eng. Comput. 2022, 39, 1902–1921. [Google Scholar] [CrossRef]
- Kasper, T.; Meschke, G. A 3D Finite Element Simulation Model for IBM Tunnelling in Soft Ground. Int. J. Numer. Anal. Methods Geomech. 2004, 28, 1441–1460. [Google Scholar] [CrossRef]
- Fu, C.; Gao, Y. Numerical Analysis on the Behavior of Existing Tunnels Subjected to the Undercrossed Shield Tunneling at a Small Proximity. Adv. Civ. Eng. 2020, 2020, 8823331. [Google Scholar] [CrossRef]
- Shiba, Y.; Kawashima, K.; Obinata, N.; Kano, T. An Evaluation Method of Longitudinal Stiffness of Shield Tunnel Linings for Application to Seismic Response Analyses. Doboku Gakkai Ronbunshu 1988, 398, 319–327. [Google Scholar] [CrossRef]
- Uchida, K.; Wasa, Y.; Kanai, M. Design of the Shield Tunnel for the Trans-Tokyo Bay Highway. Tunn. Undergr. Space Technol. 1992, 7, 251–261. [Google Scholar] [CrossRef]
- He, S.; Lai, J.; Wang, L.; Wang, K. A Literature Review on Properties and Applications of Grouts for Shield Tunnel. Constr. Build. Mater. 2020, 239, 117782. [Google Scholar] [CrossRef]
- Mao, J.; Yuan, D.; Jin, D.; Zeng, J. Optimization and Application of Backfill Grouting Material for Submarine Tunnel. Constr. Build. Mater. 2020, 265, 120281. [Google Scholar] [CrossRef]
- Liang, X.; Ying, K.; Ye, F.; Su, E.; Xia, T.; Han, X. Selection of Backfill Grouting Materials and Ratios for Shield Tunnel Considering Stratum Suitability. Constr. Build. Mater. 2022, 314, 125431. [Google Scholar] [CrossRef]
- Janbu, N. Soil Compressibility as Determined by Odometer and Triaxial Tests. In Proceedings of the European Conference on Soil Mechanics and Foundation Engineering, Wiesbaden, Germany, 11 November 1963; Volume 1, pp. 19–25. [Google Scholar]
- Bolton, M.D. The Strength and Dilatancy of Sands. Géotechnique 1986, 36, 65–78. [Google Scholar] [CrossRef]
- Wang, W.; Wang, H.; Xu, Z. Study of parameters of HS-Small model used in numerical analysis of excavations in Shanghai area. Rock Soil Mech. 2013, 34, 1766–1774. [Google Scholar] [CrossRef]

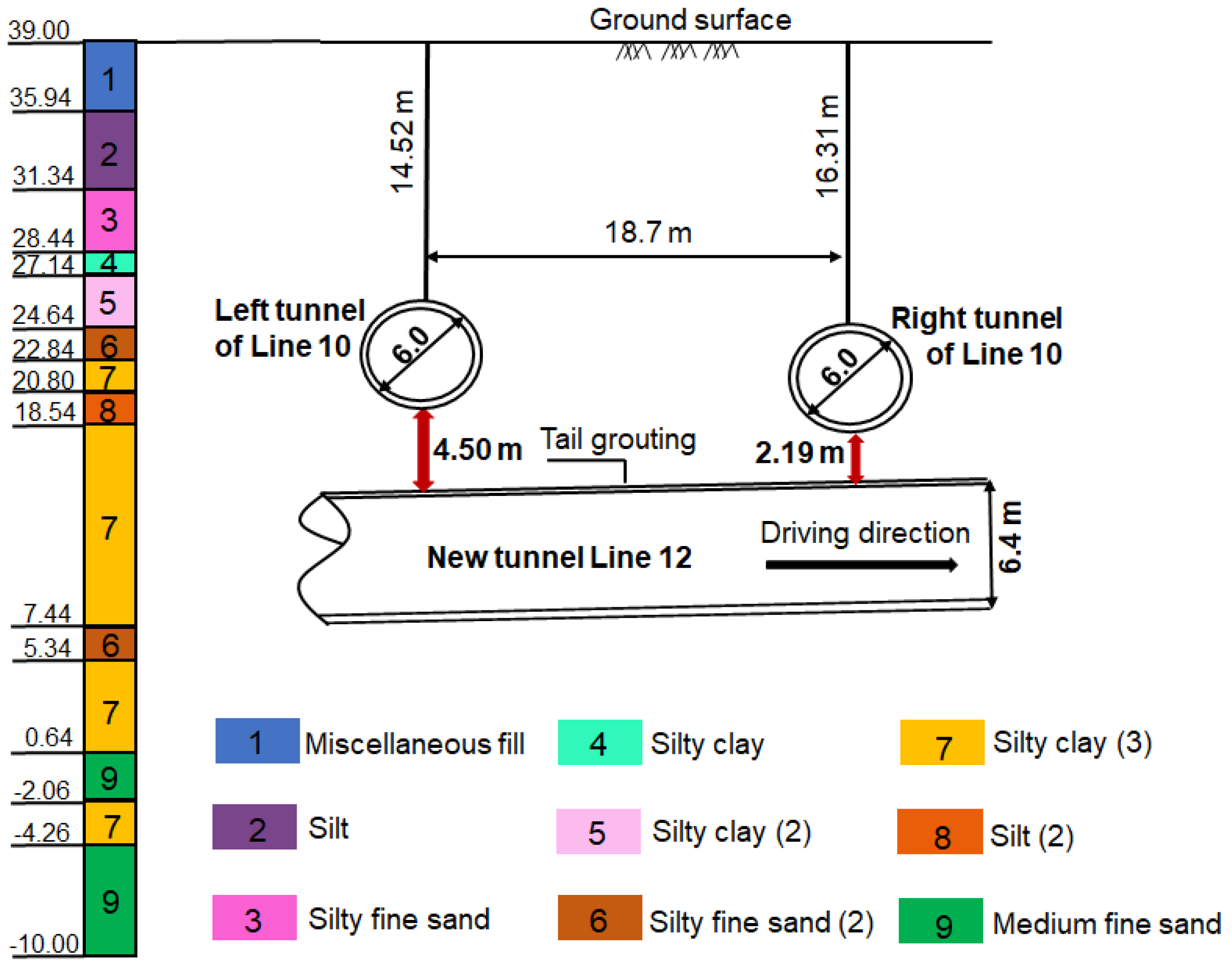

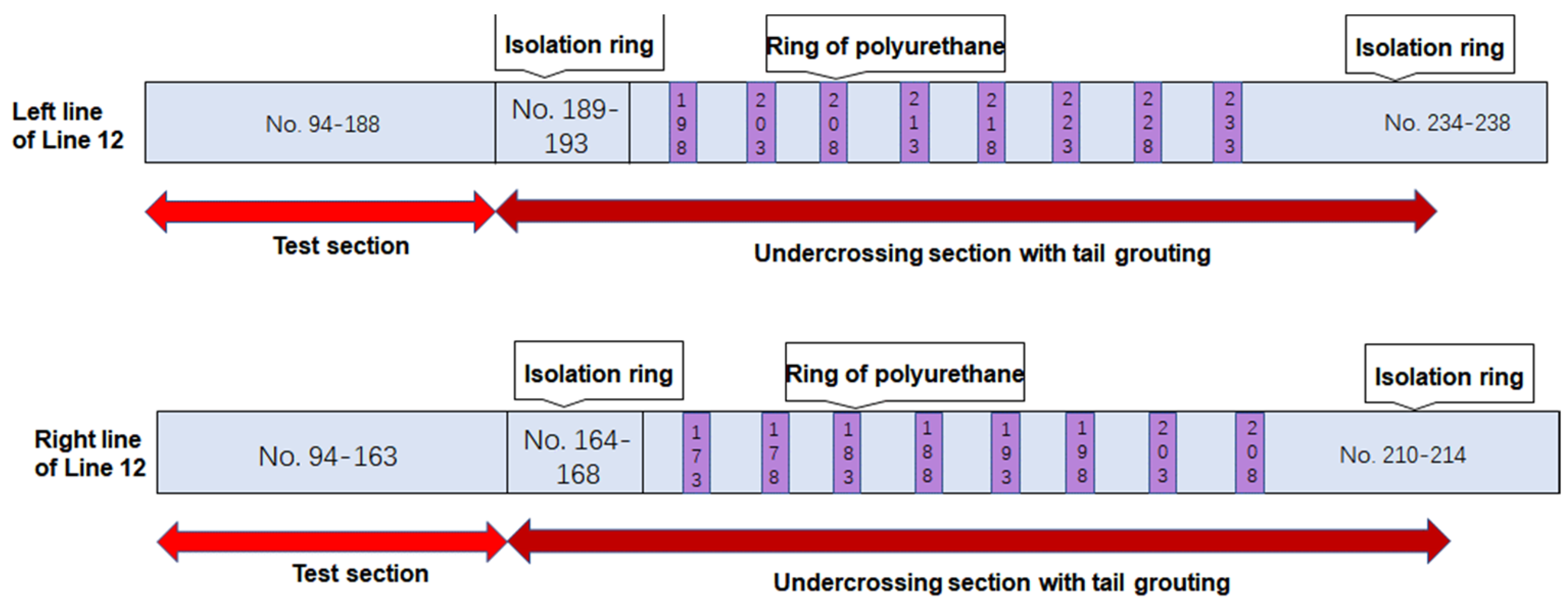

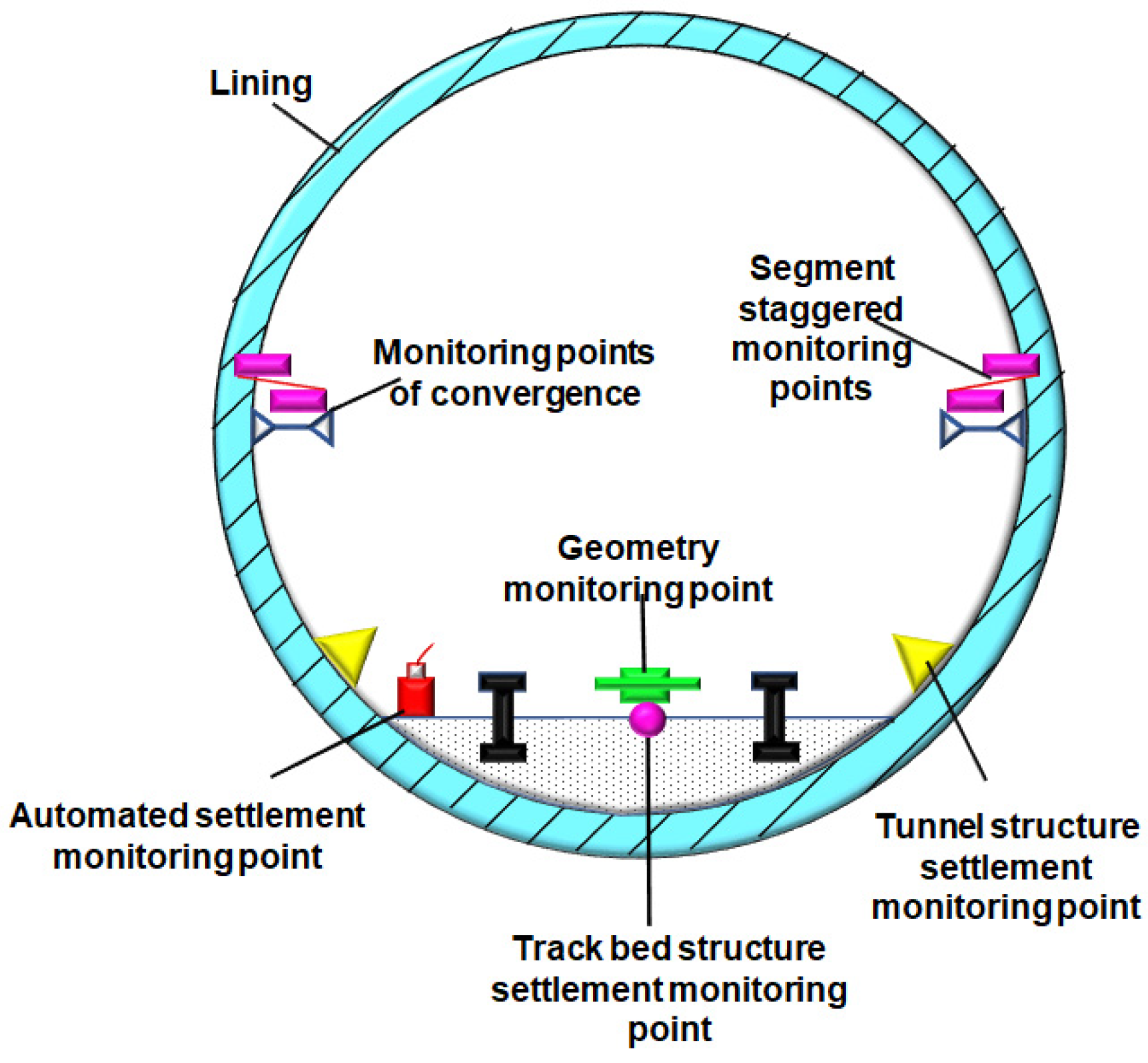


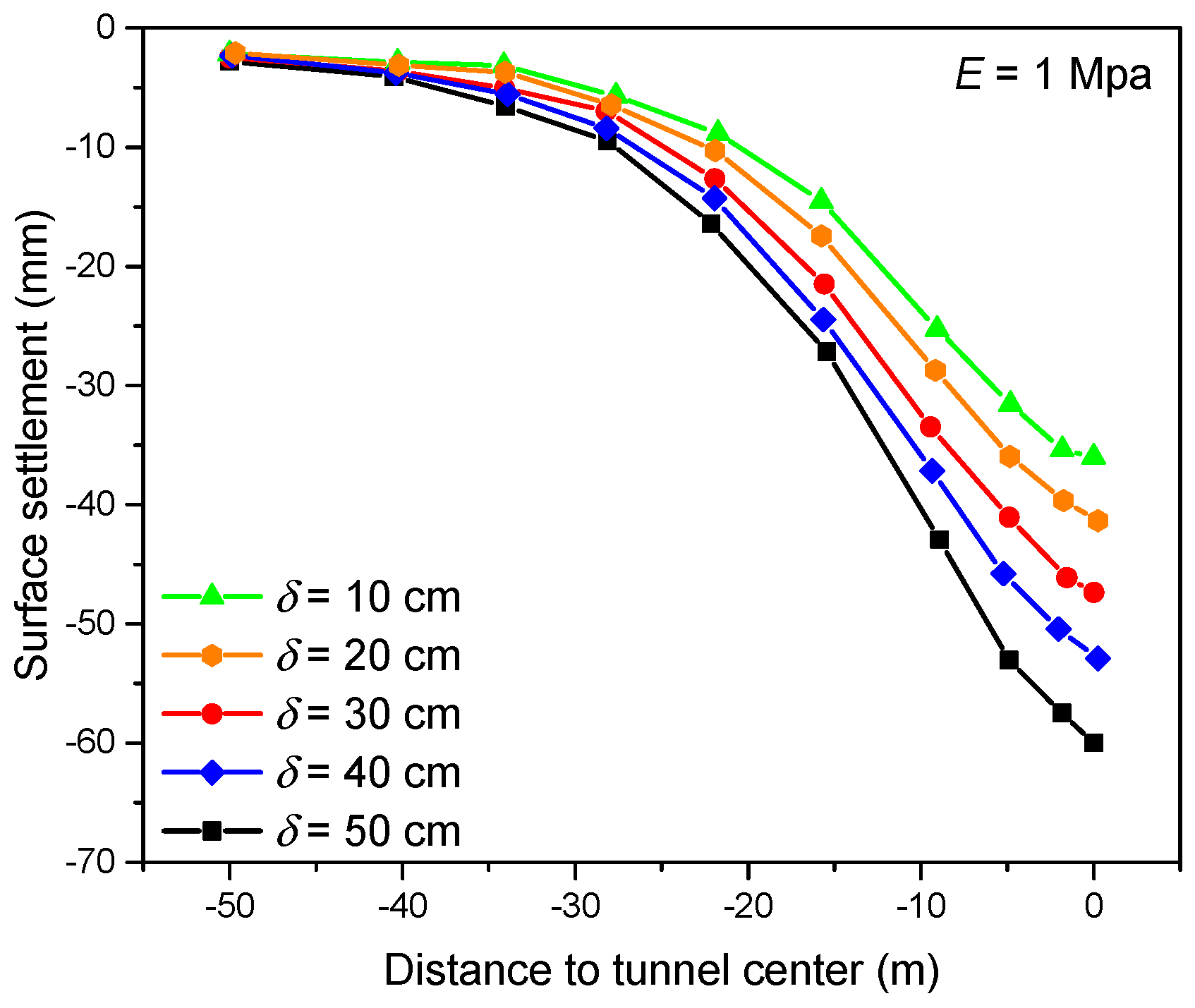

| No. | Scheme | Permeability | Unit Weight | Compression Modulus | Cohesion | Internal Friction Angle | Poisson Ratio |
|---|---|---|---|---|---|---|---|
| k (cm/s) | γ (kN/m3) | Es (MPa) | c′ (kPa) | φ′ (°) | ν | ||
| 1 | Miscellaneous fill | -- | 18.0 | 4.00 | 2 | 10 | 0.28 |
| 2 | Silt | 3.5 × 10−4 | 20.2 | 8.71 | 15.27 | 26.74 | 0.28 |
| 3 | Silty fine sand | 6.9 × 10−3 | 20.1 | 4.52 | 10 | 28 | 0.28 |
| 4 | Silty clay | 5.9 × 10−5 | 20.5 | 8.43 | 26.77 | 13.95 | 0.27 |
| 5 | Silty clay (2) | 5.6 × 10−5 | 19.9 | 14.00 | 32.92 | 13.64 | 0.33 |
| 6 | Silty fine sand (2) | 6.9 × 10−3 | 20.4 | 30.00 | 10 | 32 | 0.26 |
| 7 | Silty clay (3) | 5.6 × 10−5 | 20.2 | 12.21 | 28.2 | 13.28 | 0.29 |
| 8 | Silt (2) | 3.5 × 10−4 | 20.5 | 16.41 | 18.03 | 27.90 | 0.26 |
| 9 | Medium–fine sand | 2.7 × 10−4 | 20.5 | 40.00 | 5 | 36 | 0.23 |
| Items | Parameters | Items | Parameters |
|---|---|---|---|
| Excavation diameter | 6680 mm | Number of drive groups | 9 groups |
| Advance speed | 10~15 mm/min | Rated torque | 7375 kNm |
| Cutter pressure | 0.9~1.2 Bar | Breakout torque | 8600 kNm |
| Cutter opening rate | 60% | Screw machine | 880 × 560 mm |
| Drive form | Hydraulic drive | Maximum design pressure | 5 Bar |
| Minimum horizontal turning radius | 250 m | Maximum slag capacity | 440 m3/h |
| Power of machine | 1750.75 KW | Articulation form | Passive articulation |
| Liquid A | Liquid B | |
|---|---|---|
| Cement (kg) | Water (kg) | Sodium silicate (kg) |
| 608 | 304 | 660.5 |
| Monitoring Items | Monitoring Device | Monitoring Accuracy/mm | Deformation Restriction/mm |
|---|---|---|---|
| Remote real-time monitoring of existing line structures | Static level | 0.1 | 3.0 |
| Tunnel structure settlement and track bed structure settlement | Level | 0.3 | 2.0 |
| Track geometry | Track gauge | 0.1 | / |
| Tunnel convergence | Convergence meter | 0.1 | 1.0 |
| Segment staggering | Vernier caliper | 0.01 | 1.0 |
| Item | Simulation Element | Thickness (t)/m | Unit Weight (γ)/kN/m3 | Elastic Modulus (E)/MPa | Poisson Ratio (ν) |
|---|---|---|---|---|---|
| Lining | Soil element | 0.3 | 27.0 | 31,000 | 0.1 |
| Soil Layers | γ (kN/m3) | / MPa | / MPa | / MPa | / kPa | / MPa | m | ||
|---|---|---|---|---|---|---|---|---|---|
| 1 | 18.0 | 5.40 | 3.60 | 25.20 | 2 | 10 | 100.80 | 0.5 | 0.00020 |
| 2 | 20.2 | 11.76 | 7.84 | 54.88 | 15.27 | 26.74 | 219.52 | 0.5 | 0.00015 |
| 3 | 20.1 | 6.10 | 4.07 | 28.49 | 10 | 28 | 113.96 | 0.6 | 0.00020 |
| 4 | 20.5 | 11.38 | 7.59 | 53.13 | 26.77 | 13.95 | 212.52 | 0.5 | 0.00015 |
| 5 | 19.9 | 18.90 | 12.60 | 88.20 | 32.92 | 13.64 | 352.80 | 0.5 | 0.00015 |
| 6 | 20.4 | 40.50 | 27.00 | 189.00 | 10 | 32 | 756.00 | 0.6 | 0.00020 |
| 7 | 20.2 | 16.48 | 10.99 | 76.93 | 28.2 | 13.28 | 307.72 | 0.5 | 0.00015 |
| 8 | 20.5 | 22.15 | 14.77 | 103.38 | 18.03 | 27.90 | 413.53 | 0.5 | 0.00015 |
| 9 | 20.5 | 54.00 | 36.00 | 252.00 | 5 | 36 | 1008.00 | 0.7 | 0.00020 |
| Items | Simulation Element | Unit Weight (γ)/kN/m3 | Elastic Modulus (E)/MPa | Poisson Ration (ν) |
|---|---|---|---|---|
| Shield shell | Plate element | 120 | 23,000 | 0.15 |
| Equivalent layer | Soil element | 22 | 1~8 | 0.20 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, J.; Tang, X.; Wang, T.; Lin, W.; Yan, J.; Fu, C. Analysis for Ground Deformation Induced by Undercrossed Shield Tunnels at a Small Proximity Based on Equivalent Layer Method. Sustainability 2022, 14, 9972. https://doi.org/10.3390/su14169972
Liang J, Tang X, Wang T, Lin W, Yan J, Fu C. Analysis for Ground Deformation Induced by Undercrossed Shield Tunnels at a Small Proximity Based on Equivalent Layer Method. Sustainability. 2022; 14(16):9972. https://doi.org/10.3390/su14169972
Chicago/Turabian StyleLiang, Jiaxin, Xiaowu Tang, Tianqi Wang, Weikang Lin, Jing Yan, and Chunqing Fu. 2022. "Analysis for Ground Deformation Induced by Undercrossed Shield Tunnels at a Small Proximity Based on Equivalent Layer Method" Sustainability 14, no. 16: 9972. https://doi.org/10.3390/su14169972
APA StyleLiang, J., Tang, X., Wang, T., Lin, W., Yan, J., & Fu, C. (2022). Analysis for Ground Deformation Induced by Undercrossed Shield Tunnels at a Small Proximity Based on Equivalent Layer Method. Sustainability, 14(16), 9972. https://doi.org/10.3390/su14169972







