Effect of Structural Parameters and Operational Characteristic Analysis on Ejector Used in Proton Exchange Membrane Fuel Cell
Abstract
:1. Introduction
2. Ejector Structure Design
2.1. Design Condition
2.2. Thermodynamic Theory Design Procedure
3. Numerical Modeling
3.1. CFD Modeling and Convergence Criteria
3.2. Grid Independence Verification
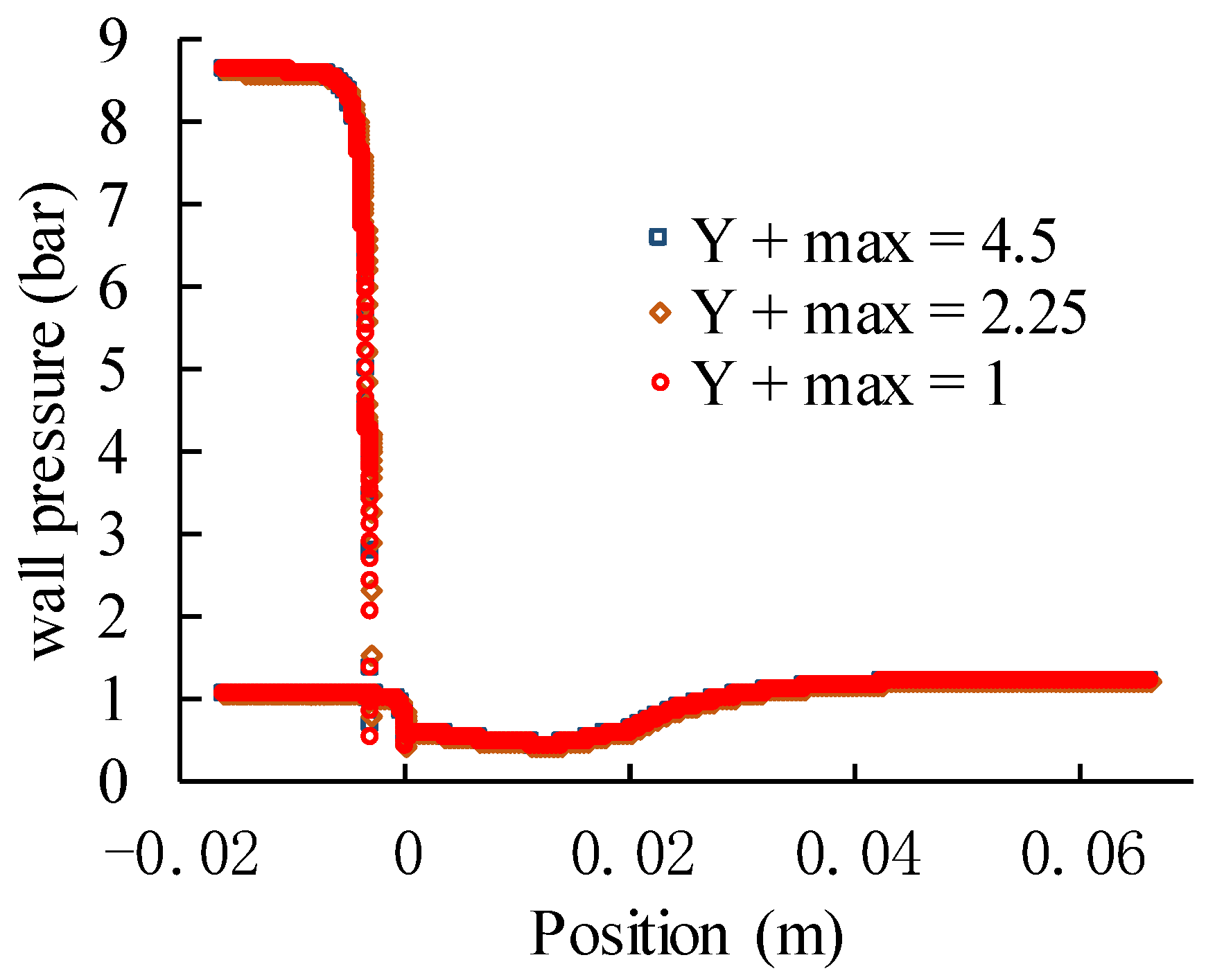
3.3. The y+ Independence
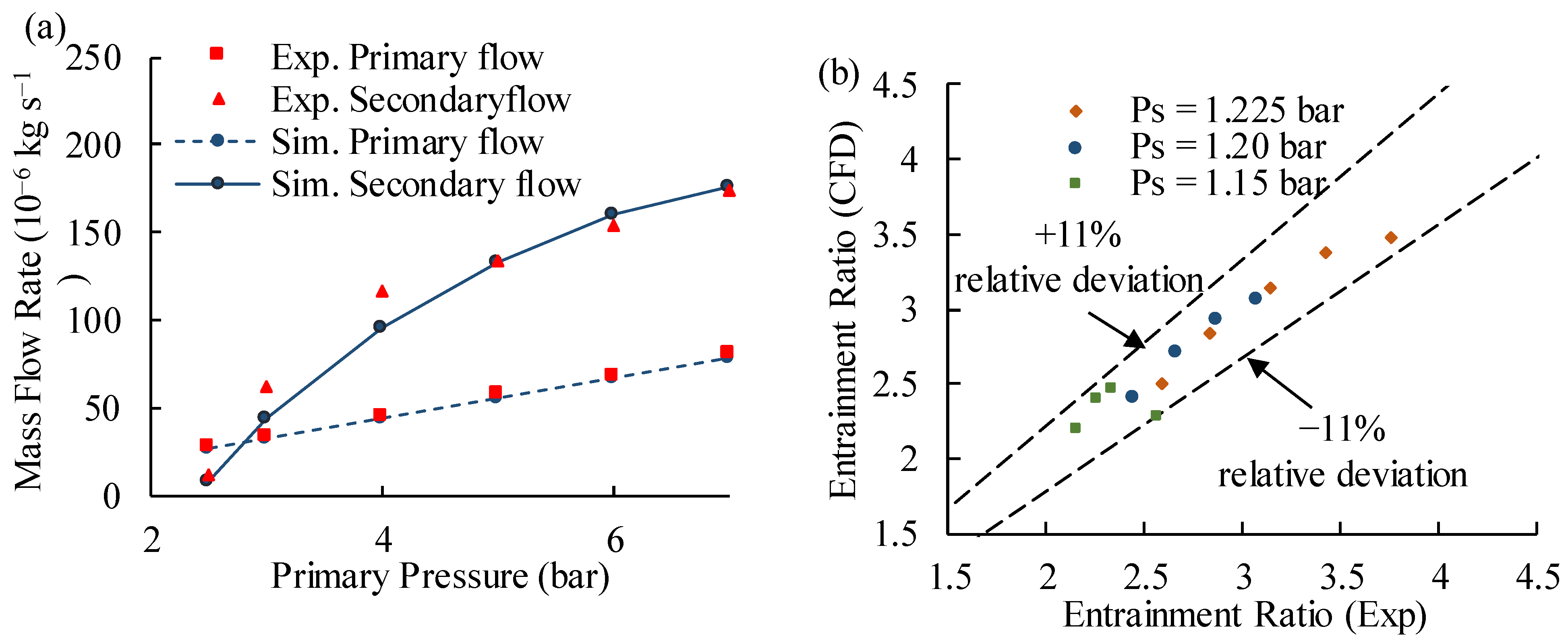
3.4. Model Validation
4. Single-Factor Analysis of the Performance of Ejector in PEMFC
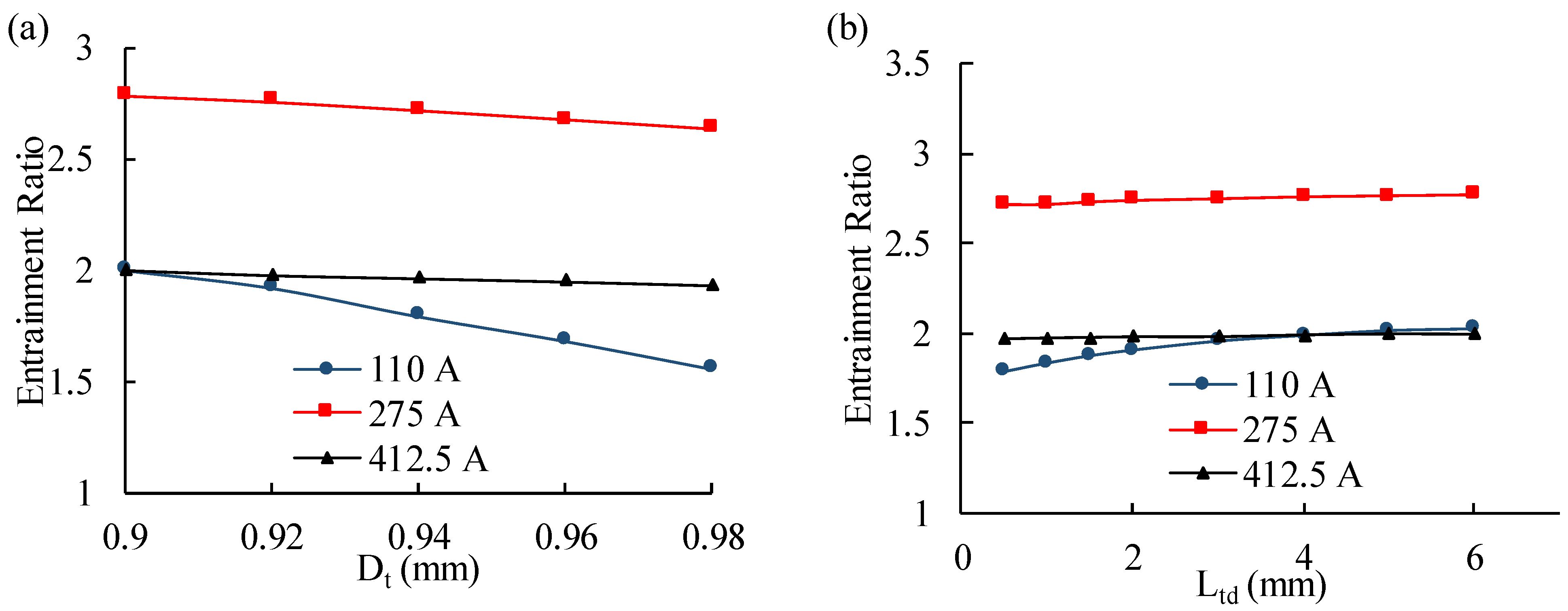
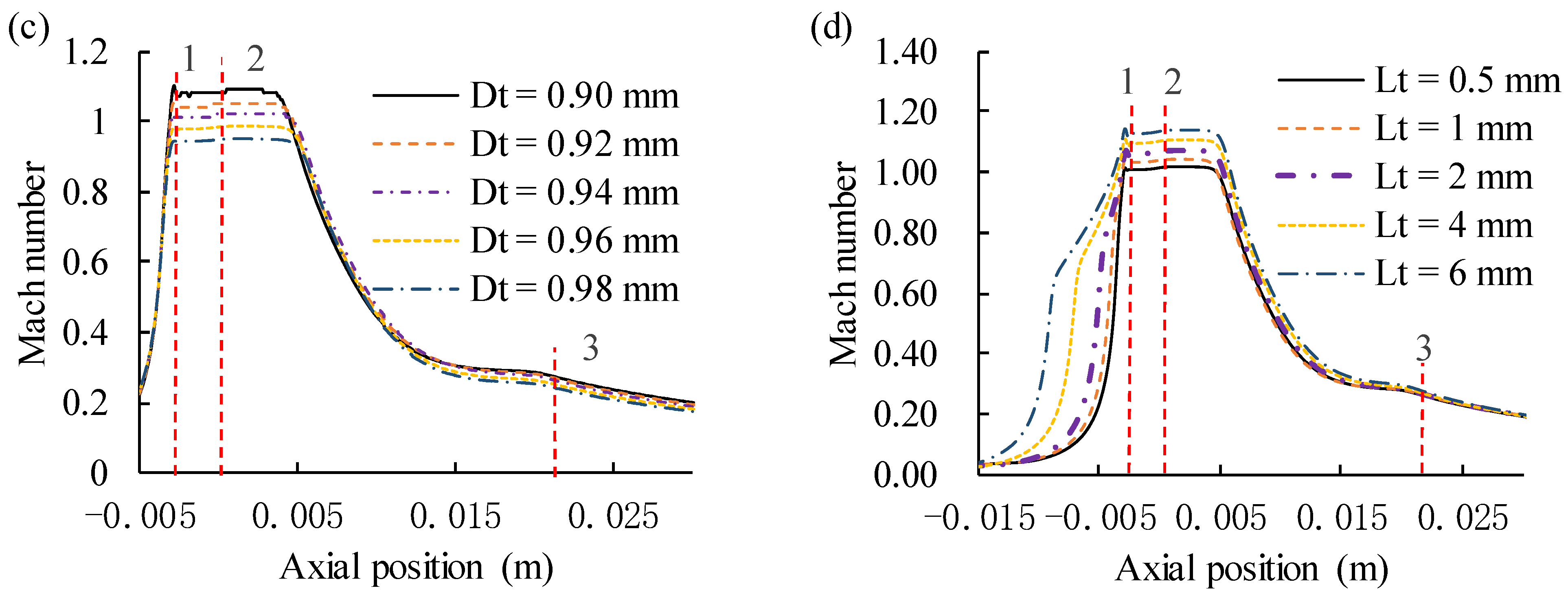
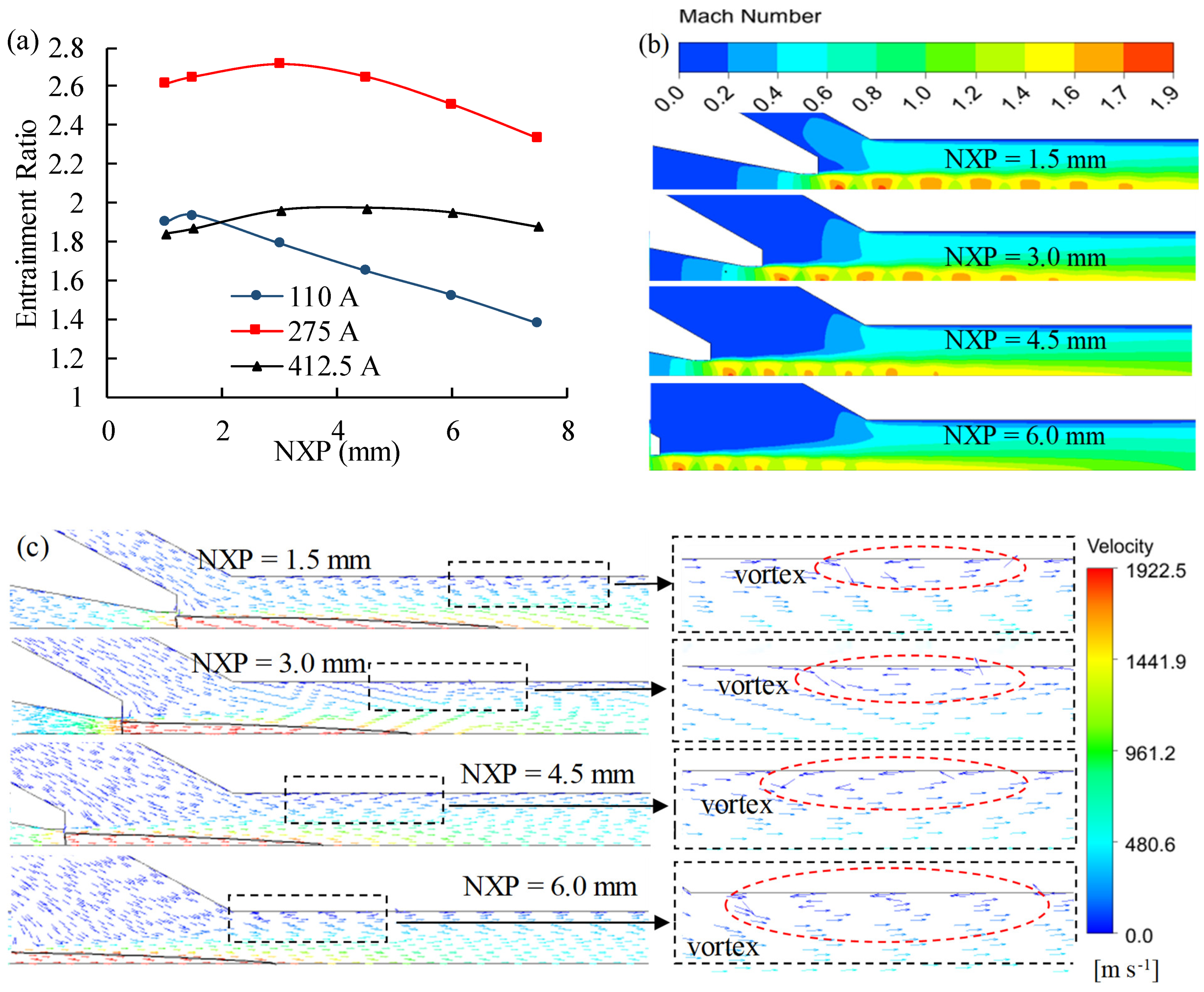
4.1. Effect of Nozzle Dimensions
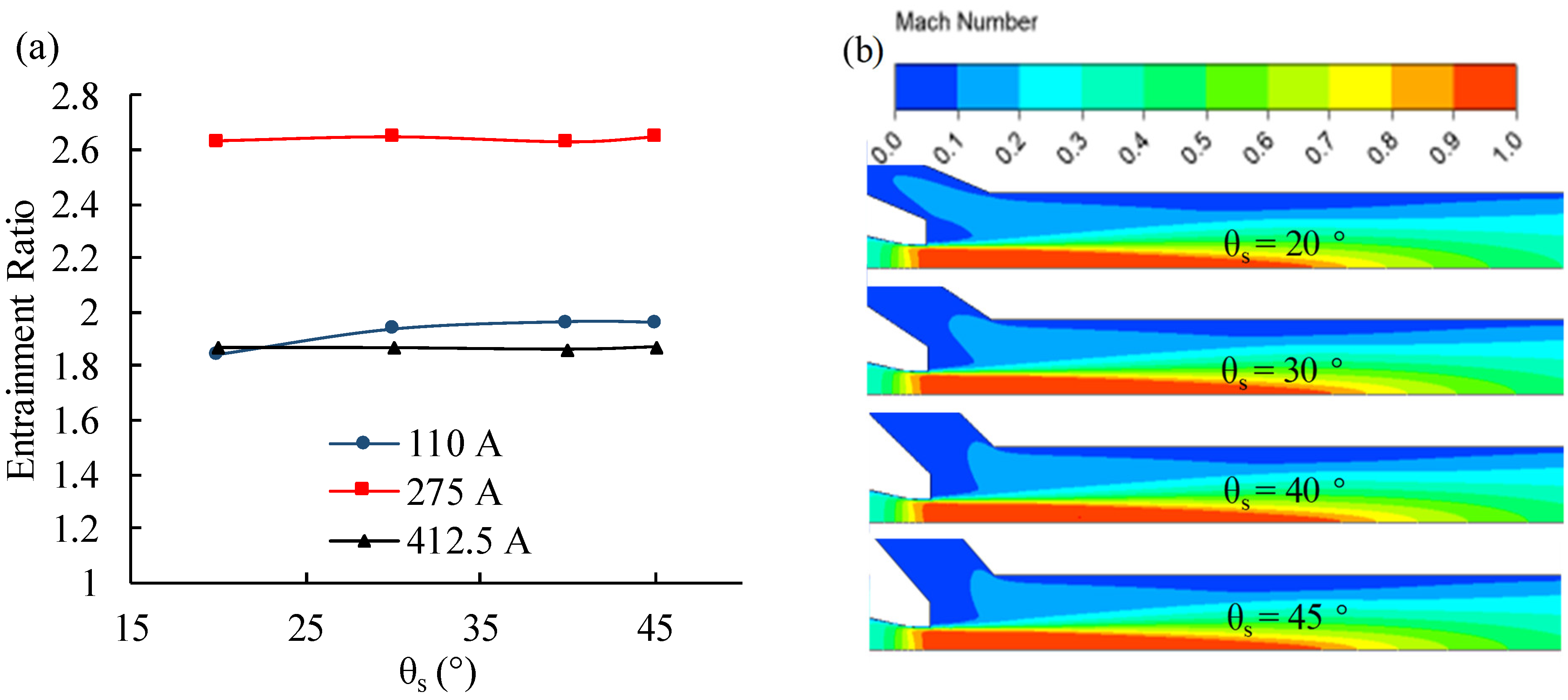
4.2. Effect of Suction Chamber Dimensions
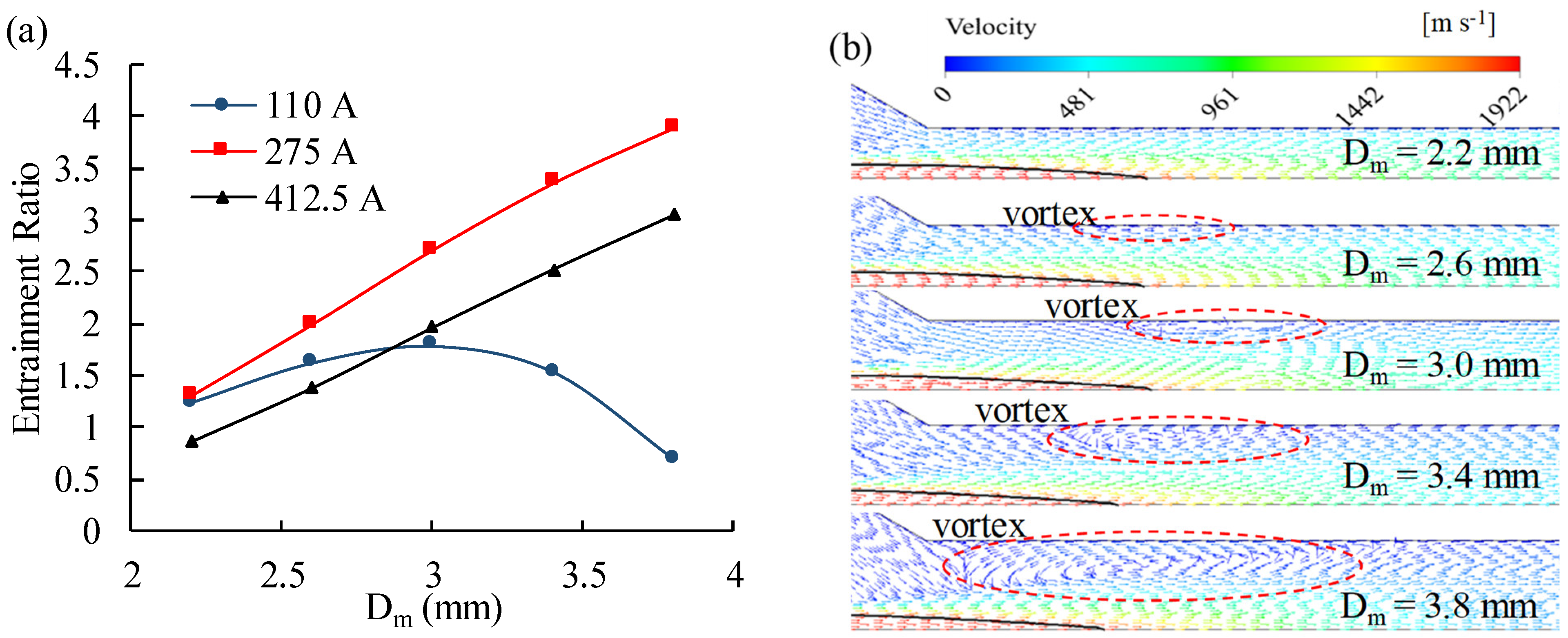
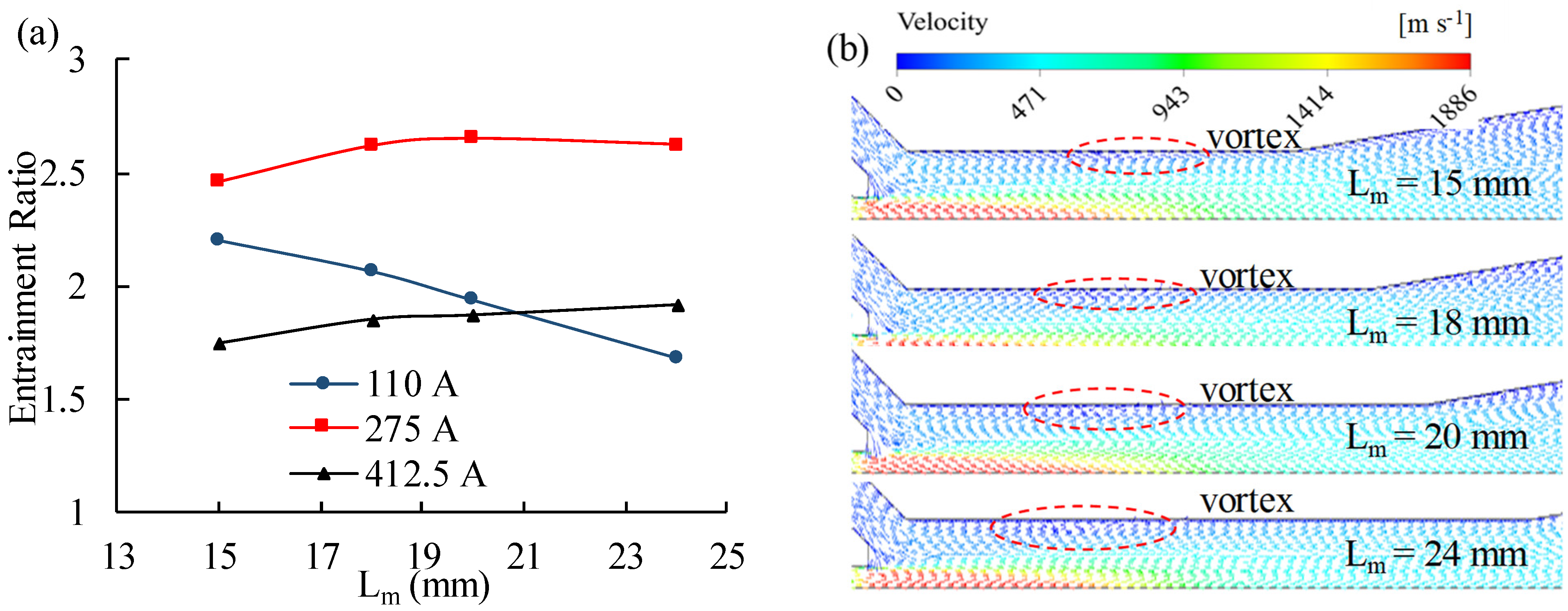
4.3. Effect of Mixing Tube Dimensions
4.4. Effect of Diffuser Dimensions
5. Multi-Factor Analysis of the Performance of Ejector Using Taguchi Method
5.1. Taguchi Experimental Design
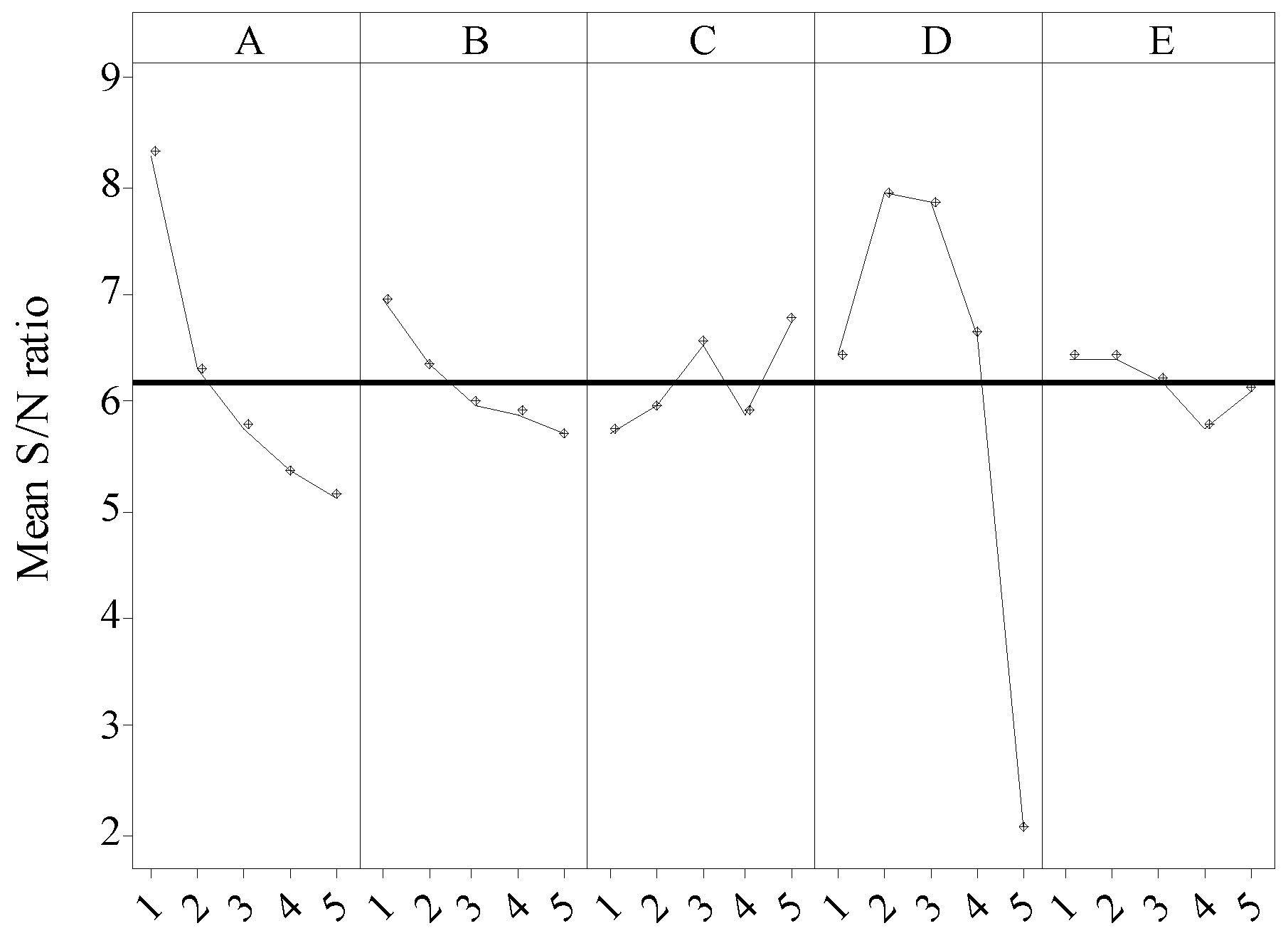
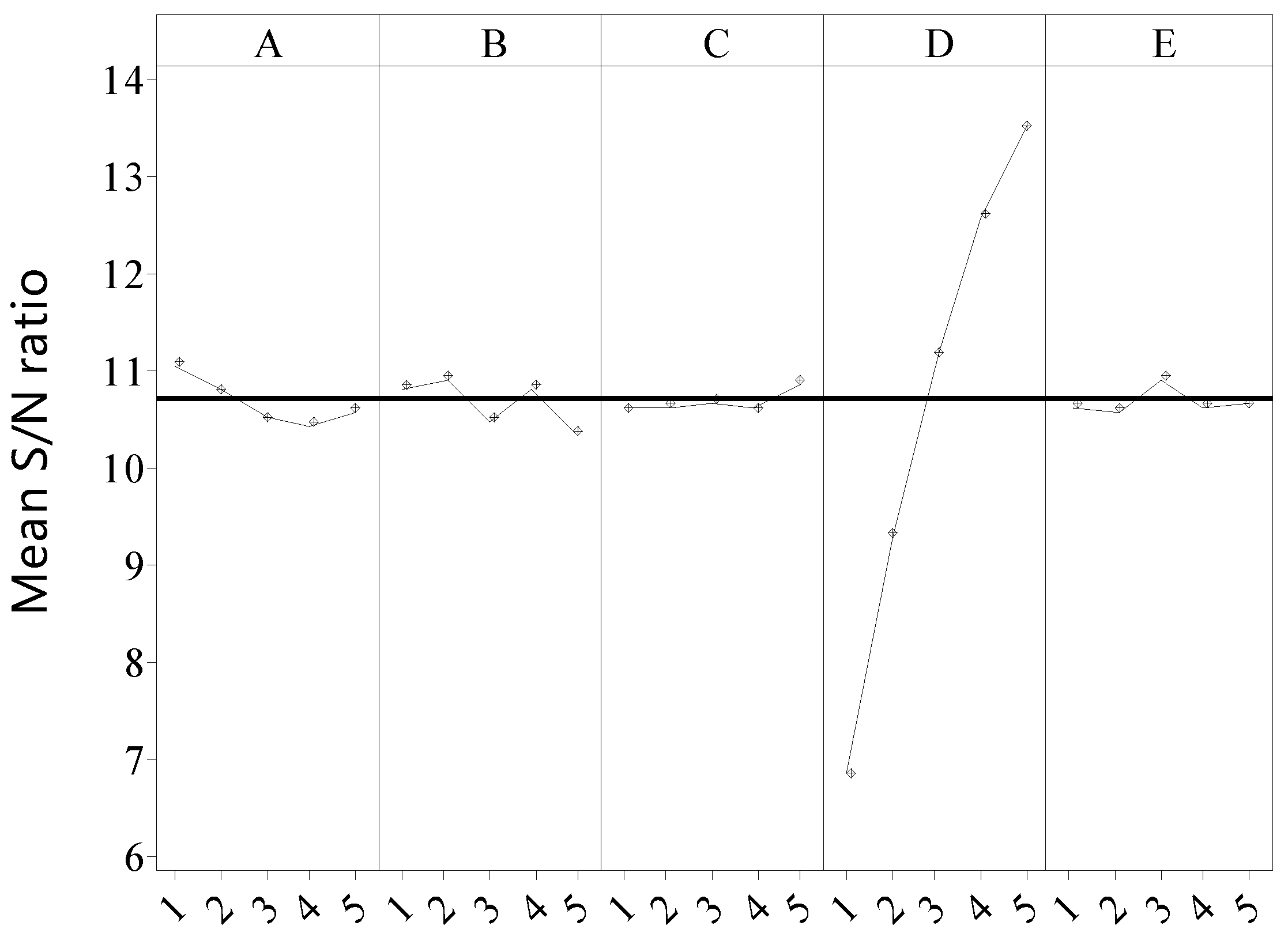
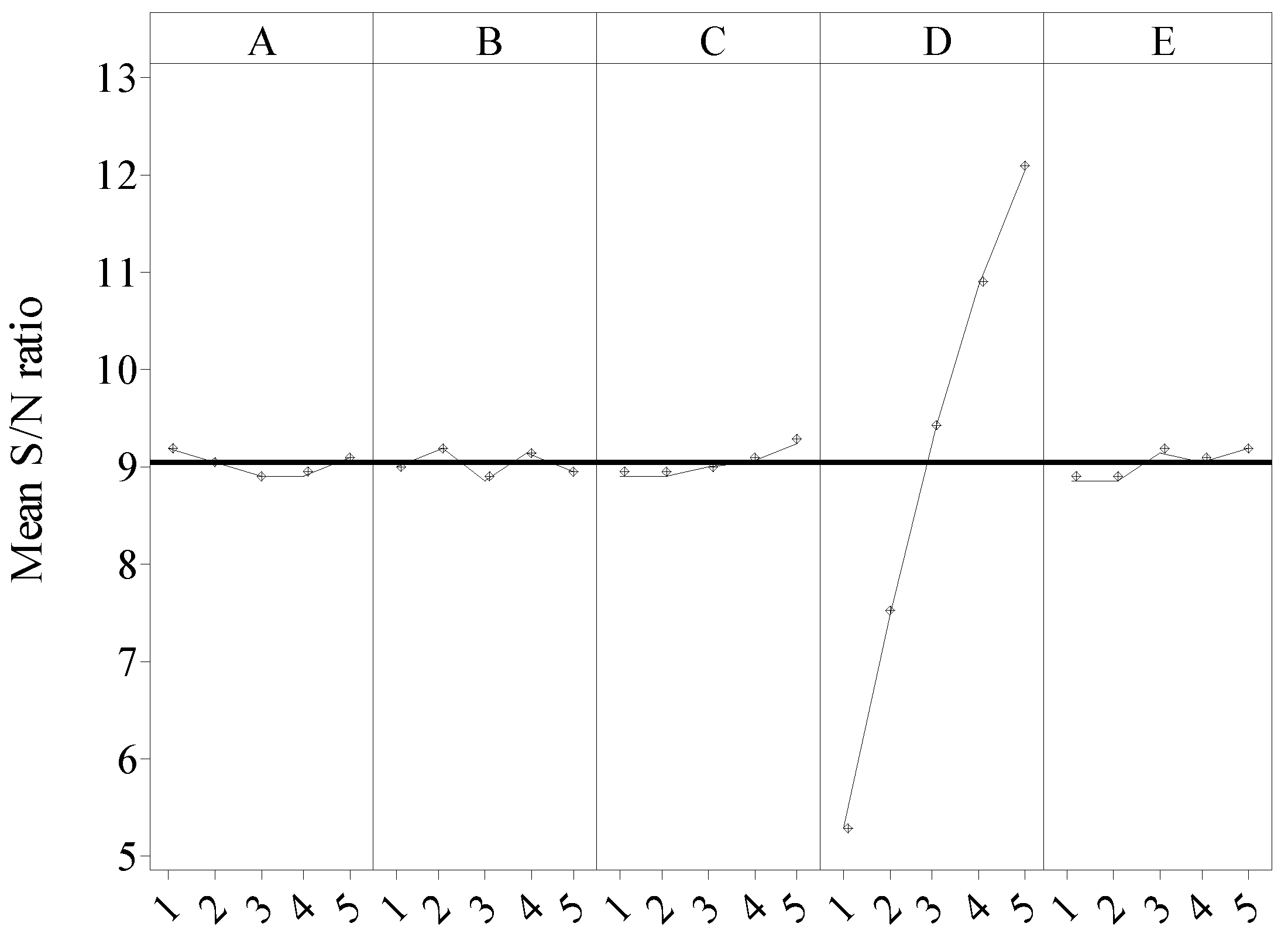
5.2. Correlation Analysis of Factors
5.3. Optimal Combination
5.4. Comparison of the Optimized Result
6. Conclusions
- In the normal range, the nozzle convergence angle, suction chamber angle, and diffuser length are non-essential structural factors that hardly affect the performance of ejector. Nozzle divergent angle and nozzle throat length are important parameters of partial working conditions. Nozzle throat diameter, nozzle exit position, mixing tube diameter and mixing tube length are crucial for wide-range ejector design in PEMFC.
- Convergent nozzle outperforms convergent-divergent nozzle at entrainment ratio of PEMFC ejector. The optimal mixing tube diameter is 3 mm with the current is 110 A, and the optimal diameter as stack current increases. The optimal NXT is 1.5 mm with current is 110 A, while the optimal NXT is 3 mm and 4.5 mm when the current is 275 A and 412.5 A, respectively.
- Multi-factor analysis indicated that mixing tube diameter shall be the most significant parameter affecting performance of PEMFC ejectors except the nozzle diameter. In addition, the nozzle angle and length are more sensitive to the entrainment performance at low and medium currents, while the nozzle exit position is more sensitive at high currents.
- The ejector optimized based on multiple load condition can operate in a wider range, and the entrainment ratio is more uniform. Multi-factor analysis method rather than the single-factor analysis may lead to more effective improvement of operating range. Entrainment ratios of the ejector optimized by multi-factor is about 5% greater than single-factor.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | Area [m−2] |
| D | Diameter [m] |
| ER | Entrainment ratio |
| F | Faraday’s constant [C mol−1] |
| L | Length [m] |
| T | Temperature [K] |
| I | Current [A] |
| m | mass flow rate [kg s−1] |
| M | molar weight [kg mol−1] |
| Ma | Much number |
| N | number of single cell of PEMFC stack |
| P | Pressure [Pa] |
| R | gas constant [J mol−1 K−1] |
| S/N | signal-to-noise ratio |
| V | Velocity [m s−1] |
| Greek letters | |
| θ | Angle [°] |
| λ | excess ratio |
| γ | specific heat ratio |
| ρ | Density [kg m3] |
| η | mean of S/N ratio |
| φ | Efficiency [%] |
| Subscript | |
| p | primary flow |
| s | secondary flow |
| c | exit flow |
| t | nozzle throat |
| y | mixing tube inlet |
| v | velocity distribution |
| h2 | hydrogen |
References
- Barbir, F. PEM Fuel Cells: Theory and Practice; Academic press: London, UK, 2005. [Google Scholar]
- Lee, H.Y.; Su, H.C.; Chen, Y.S. A gas management strategy for anode recirculation in a proton exchange membrane fuel cell. Int. J. Hydrogen Energy 2018, 43, 3803–3808. [Google Scholar] [CrossRef]
- Hou, J.; Yang, M.; Zhang, J. Active and passive fuel recirculation for solid oxide and proton exchange membrane fuel cells. Renew. Energy 2020, 155, 1355–1371. [Google Scholar] [CrossRef]
- Shen, K.Y.; Park, S.; Kim, Y.B. Hydrogen utilization enhancement of proton exchange membrane fuel cell with anode recirculation system through a purge strategy. Int. J. Hydrogen Energy 2020, 45, 16773–16786. [Google Scholar] [CrossRef]
- Tsai, S.W.; Chen, Y.S. A mathematical model to study the energy efficiency of a proton exchange membrane fuel cell with a dead-ended anode. Appl. Energy 2017, 188, 151–159. [Google Scholar] [CrossRef]
- Toghyani, S.; Baniasadi, E.; Afshari, E. Performance analysis and comparative study of an anodic recirculation system based on electrochemical pump in proton exchange membrane fuel cell. Int. J. Hydrogen Energy 2018, 43, 19691–19703. [Google Scholar] [CrossRef]
- Toghyani, S.; Afshari, E.; Baniasadi, E. A parametric comparison of three fuel recirculation system in the closed loop fuel supply system of PEM fuel cell. Int. J. Hydrogen Energy 2019, 44, 7518–7530. [Google Scholar] [CrossRef]
- Hwang, J.J. Passive hydrogen recovery schemes using a vacuum ejector in a proton exchange membrane fuel cell system. J. Power Sources 2014, 247, 256–263. [Google Scholar] [CrossRef]
- Cokojiob, R.; Бpoдянcкий, H.M. Ejector; Science Press: Beijing, China, 1977. [Google Scholar]
- Zhang, L.H.; Li, X.J.; Li, J.; Shao, Z.G.; Yi, B.L. Design and performance test of subsonic ejector for proton exchange membrane fuel cell based on thermodynamic model. Chin. J. Power Sources 2014, 38, 1824–1827+1897. [Google Scholar]
- Jin, K.M.; Jun, S.Y.; Won, C.C.; Yong, L.W.; Soo, K.C. Customized design for the ejector to recirculate a humidified hydrogen fuel in a submarine PEMFC. J. Power Sources 2008, 176, 529–533. [Google Scholar]
- Zhu, Y.H.; Li, Y.Z. New theoretical model for convergent nozzle ejector in the proton exchange membrane fuel cell system. J. Power Sources 2009, 191, 510–519. [Google Scholar] [CrossRef]
- Dadva, M.; Afshar, E. Analysis of design parameters in anodic recirculation system based on ejector technology for PEM fuel cells: A new approach in designing. Int. J. Hydrogen Energy 2014, 39, 12061–12073. [Google Scholar] [CrossRef]
- Brunner, D.A.; Marcks, S.; Bajpai, M.; Prasad, A.K.; Advani, S.G. Design and characterization of an electronically controlled variable flow rate ejector for fuel cell applications. Int. J. Hydrogen Energy 2012, 37, 4457–4466. [Google Scholar] [CrossRef]
- MV, S.R.; Jagadeesh, G. Vector Evaluated Particle Swarm Optimization (VEPSO) of Supersonic Ejector for Hydrogen Fuel Cells. J. Fuel Cell Sci. Technol. 2010, 7, 16–22. [Google Scholar]
- Maghsoodi, A.; Afshari, E.; Ahmadikia, H. Optimization of geometric parameters for design a high-performance ejector in the proton exchange membrane fuel cell system using artificial neural network and genetic algorithm. Appl. Therm. Eng. 2014, 71, 410–418. [Google Scholar] [CrossRef]
- Bai, S.; Lei, W.; Wang, X. Optimization of ejector geometric parameters with hybrid artificial fish swarm algorithm for PEM fuel cell. In Proceedings of the 2017 Chinese Automation Congress (CAC), Jinan, China, 20 October 2017. [Google Scholar]
- Nikiforow, K.; Koski, P.; Karimaki, H.; Ihonen, J.; Alopaeus, V. Designing a hydrogen gas ejector for 5kW stationary PEMFC system—CFD-modeling and experimental validation. Int. J. Hydrogen Energy 2016, 41, 14952–14970. [Google Scholar] [CrossRef]
- Feng, J.; Han, J.; Hou, T.; Peng, X. Performance analysis and parametric studies on the primary nozzle of ejectors in proton exchange membrane fuel cell systems. Energy Sour. Part. A Recovery Util. Environ. Effects 2020, 1–20. [Google Scholar] [CrossRef]
- Lakzian, E.; Ghorbanzadeh, S. A numerical comparison between ejector performance with convergence and convergence-divergence primary nozzle. Modares Mech. Eng. 2016, 16, 324–332. [Google Scholar]
- Yin, Y.; Fan, M.; Jiao, K.; Du, Q.; Qin, Y. Numerical investigation of an ejector for anode recirculation in proton exchange membrane fuel cell system. Energy Convers. Manag. 2016, 126, 1106–1117. [Google Scholar] [CrossRef]
- Wang, X.; Xu, S.; Xing, C. Numerical and experimental investigation on an ejector designed for an 80 kW polymer electrolyte membrane fuel cell stack. J. Power Sources 2019, 415, 25–32. [Google Scholar] [CrossRef]
- Besagni, G.; Mereu, R.; Inzoli, F.; Chiesa, P. Application of an integrated lumped parameter-CFD approach to evaluate the ejector-driven anode recirculation in a PEM fuel cell system. Appl. Therm. Eng. 2017, 121, 628–651. [Google Scholar] [CrossRef]
- Besagni, G.; Mereu, R.; Chiesa, P.; Inzoli, F. An Integrated Lumped Parameter-CFD approach for off-design ejector performance evaluation. Energy Convers. Manag. 2015, 105, 697–715. [Google Scholar] [CrossRef]
- Pei, P.; Ren, P.; Li, Y.; Wu, Z.; Chen, D.; Huang, S.; Jia, X. Numerical studies on wide-operating-range ejector based on anodic pressure drop characteristics in proton exchange membrane fuel cell system. Appl. Energy 2018, 235, 729–738. [Google Scholar] [CrossRef]
- Yang, Y.; Du, W.; Ma, T.; Lin, W.; Cong, M.; Yang, H.; Yu, Z. Numerical studies on ejector structure optimization and performance prediction based on a novel pressure drop model for proton exchange membrane fuel cell anode. Int. J. Hydrogen Energy 2020, 45, 23343–23352. [Google Scholar] [CrossRef]
- Yan, J.; Wen, H. Multi-round optimization of an ejector with different mixing chamber geometries at various liquid volume fractions of inlet fluids. Appl. Therm. Eng. 2022, 200, 117709. [Google Scholar] [CrossRef]
- Wu, Y.; Zhao, H.; Zhang, C.; Wang, L.; Han, J. Optimization analysis of structure parameters of steam ejector based on CFD and orthogonal test. Energy 2018, 151, 79–93. [Google Scholar] [CrossRef]
- Amin, A.; Elbadawy, I.; Elgendy, E.; Fatouh, M. Effect of geometrical factors interactions on design optimization process of a natural gas ejector. Adv. Mech. Eng. 2019, 11, 168781401988036. [Google Scholar] [CrossRef]
- Zhu, Y.H.; Cai, W.J.; Li, Y.Z.; Wen, C.Y. Anode gas recirculation behavior of a fuel ejector in hybrid solid oxide fuel cell systems: Performance evaluation in three operational modes. J. Power Sources 2008, 185, 1122–1130. [Google Scholar] [CrossRef]
- Gu, P.; Xing, L.; Wang, Y.; Feng, J.; Peng, X. A multi-objective parametric study of the claw hydrogen pump for fuel cell vehicles using taguchi method and ANN. Int. J. Hydrogen Energy 2021, 46, 6680–6692. [Google Scholar] [CrossRef]
- Zhang, Q.; Feng, J.; Zhang, Q.; Peng, X. Performance prediction and evaluation of the scroll-type hydrogen pump for FCVs based on CFD–Taguchi method. Int. J. Hydrogen Energy 2019, 44, 15333–15343. [Google Scholar] [CrossRef]
- Amadane, Y.; Mounir, H. Performance improvement of a PEMFC with dead-end anode by using CFD-Taguchi approach. J. Electroanal. Chem. 2022, 904, 115909. [Google Scholar] [CrossRef]






| Current (A) | Power (kW) | Mass Flow Rate of Primary Flow (g/s) | Ejector Exit Pressure (bar) | Ejector Secondary Flow Pressure (bar) | H2 Mass Fraction of Secondary Flow (%) | Entrainment Ratio |
|---|---|---|---|---|---|---|
| 55 | 3.8 | 0.05243 | 1.3 | 1.213 | 0.69 | 4.26 |
| 110 | 7.4 | 0.10336 | 1.52 | 1.42 | 0.65 | 2.68 |
| 165 | 10 | 0.15579 | 1.75 | 1.64 | 0.61 | 2.14 |
| 220 | 14 | 0.20672 | 1.96 | 1.845 | 0.58 | 1.90 |
| 275 | 16.5 | 0.25765 | 2.2 | 2.08 | 0.55 | 1.77 |
| 330 | 20 | 0.31009 | 2.2 | 2.07 | 0.55 | 1.77 |
| 385 | 22 | 0.36102 | 2.2 | 2.055 | 0.55 | 1.77 |
| 412.5 | 23.5 | 0.38798 | 2.2 | 2.039 | 0.55 | 1.77 |
| Parameters | Value | Parameters | Value |
|---|---|---|---|
| Nozzle diameter (Dt) | 0.94 mm | Mixing tube length (Lm) | 20 mm |
| Mixing tube diameter (Dm) | 3.2 mm | Suction chamber angle (θs) | 30° |
| Nozzle convergence angle (θtc) | 11° | Nozzle exit position (NXP) | 3 mm |
| Nozzle throat length (Ltd) | 0.5 mm | Diffuser length (Ld) | 40 mm |
| Nozzle divergent angle (θtd) | 0° |
| Model | Mesh Cells | ER | Relative Deviation |
|---|---|---|---|
| 1 | 53,144 | 1.889706 | 6.78% |
| 2 | 96,729 | 1.982459 | 2.21% |
| 3 | 153,144 | 2.006531 | 1.02% |
| 4 | 199,549 | 2.017966 | 0.46% |
| 5 | 300,339 | 2.027254 | 0.00% |
| y+max | Primary Flow (g·s−1) | Secondary Flow (g·s−1) | Relative Deviation |
|---|---|---|---|
| 4.5 | 0.388 | 0.76183 | 0.021% |
| 2.25 | 0.388 | 0.76218 | 0.068% |
| 1 | 0.388 | 0.76167 | 0 |
| level | θtd (A) | Lt (B) | NXP (C) | Dm (D) | Lm (E) |
|---|---|---|---|---|---|
| 1 | 0 | 0.5 | 1 | 2.2 | 12 |
| 2 | 1.5 | 1.5 | 2 | 2.6 | 15 |
| 3 | 3.5 | 2.5 | 3 | 3.0 | 18 |
| 4 | 4.5 | 3.5 | 4 | 3.4 | 21 |
| 5 | 6 | 4.5 | 5 | 3.8 | 24 |
| L25(55) | A | B | C | D | E |
|---|---|---|---|---|---|
| 1 | 1 | 1 | 1 | 1 | 1 |
| 2 | 1 | 2 | 2 | 2 | 2 |
| 3 | 1 | 3 | 3 | 3 | 3 |
| 4 | 1 | 4 | 4 | 4 | 4 |
| 5 | 1 | 5 | 5 | 5 | 5 |
| 6 | 2 | 1 | 2 | 3 | 4 |
| 7 | 2 | 2 | 3 | 4 | 5 |
| 8 | 2 | 3 | 4 | 5 | 1 |
| 9 | 2 | 4 | 5 | 1 | 2 |
| 10 | 2 | 5 | 1 | 2 | 3 |
| 11 | 3 | 1 | 3 | 5 | 2 |
| 12 | 3 | 2 | 4 | 1 | 3 |
| 13 | 3 | 3 | 5 | 2 | 4 |
| 14 | 3 | 4 | 1 | 3 | 5 |
| 15 | 3 | 5 | 2 | 4 | 1 |
| 16 | 4 | 1 | 4 | 2 | 5 |
| 17 | 4 | 2 | 5 | 3 | 1 |
| 18 | 4 | 3 | 1 | 4 | 2 |
| 19 | 4 | 4 | 2 | 5 | 3 |
| 20 | 4 | 5 | 3 | 1 | 4 |
| 21 | 5 | 1 | 5 | 4 | 3 |
| 22 | 5 | 2 | 1 | 5 | 4 |
| 23 | 5 | 3 | 2 | 1 | 5 |
| 24 | 5 | 4 | 3 | 2 | 1 |
| 25 | 5 | 5 | 4 | 3 | 2 |
| Objective | Combination | 110 A | 220 A | 412.5 A | |||
|---|---|---|---|---|---|---|---|
| S/N | λ | S/N | λ | S/N | λ | ||
| Low current | A1B1C5D2E2 | 11.66 | 3.83 | 9.95 | 3.14 | 7.72 | 2.43 |
| Middle current | A1B2C5D5E3 | 4.94 | 1.76 | 14.57 | 5.35 | 12.76 | 4.34 |
| High current | A1B2C5D5E5 | 4.85 | 1.74 | 14.30 | 5.19 | 12.77 | 4.35 |
| Operating range | A1B1C3D3E2 | 11.35 | 3.69 | 11.64 | 3.82 | 9.40 | 2.95 |
| Structure | Initial Ejector | Optimized by Single-Factor | Optimized by Multi-Factor |
|---|---|---|---|
| Nozzle divergent angle | 0 | 0 | 0 |
| Nozzle throat length | 0.5 | 6 | 0.5 |
| NXP | 3 | 1.5 | 3 |
| Mixing tube length | 20 | 18 | 15 |
| Mixing tube diameter | 3.2 | 3 | 3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, C.; Sun, B.; Luo, Q. Effect of Structural Parameters and Operational Characteristic Analysis on Ejector Used in Proton Exchange Membrane Fuel Cell. Sustainability 2022, 14, 9205. https://doi.org/10.3390/su14159205
Li C, Sun B, Luo Q. Effect of Structural Parameters and Operational Characteristic Analysis on Ejector Used in Proton Exchange Membrane Fuel Cell. Sustainability. 2022; 14(15):9205. https://doi.org/10.3390/su14159205
Chicago/Turabian StyleLi, Chao, Baigang Sun, and Qinghe Luo. 2022. "Effect of Structural Parameters and Operational Characteristic Analysis on Ejector Used in Proton Exchange Membrane Fuel Cell" Sustainability 14, no. 15: 9205. https://doi.org/10.3390/su14159205
APA StyleLi, C., Sun, B., & Luo, Q. (2022). Effect of Structural Parameters and Operational Characteristic Analysis on Ejector Used in Proton Exchange Membrane Fuel Cell. Sustainability, 14(15), 9205. https://doi.org/10.3390/su14159205





