An Investigation of Compression Bearing Capacity of Concrete-Filled Rectangular Stainless Steel Tubular Columns under Axial Load and Eccentric Axial Load
Abstract
:1. Introduction
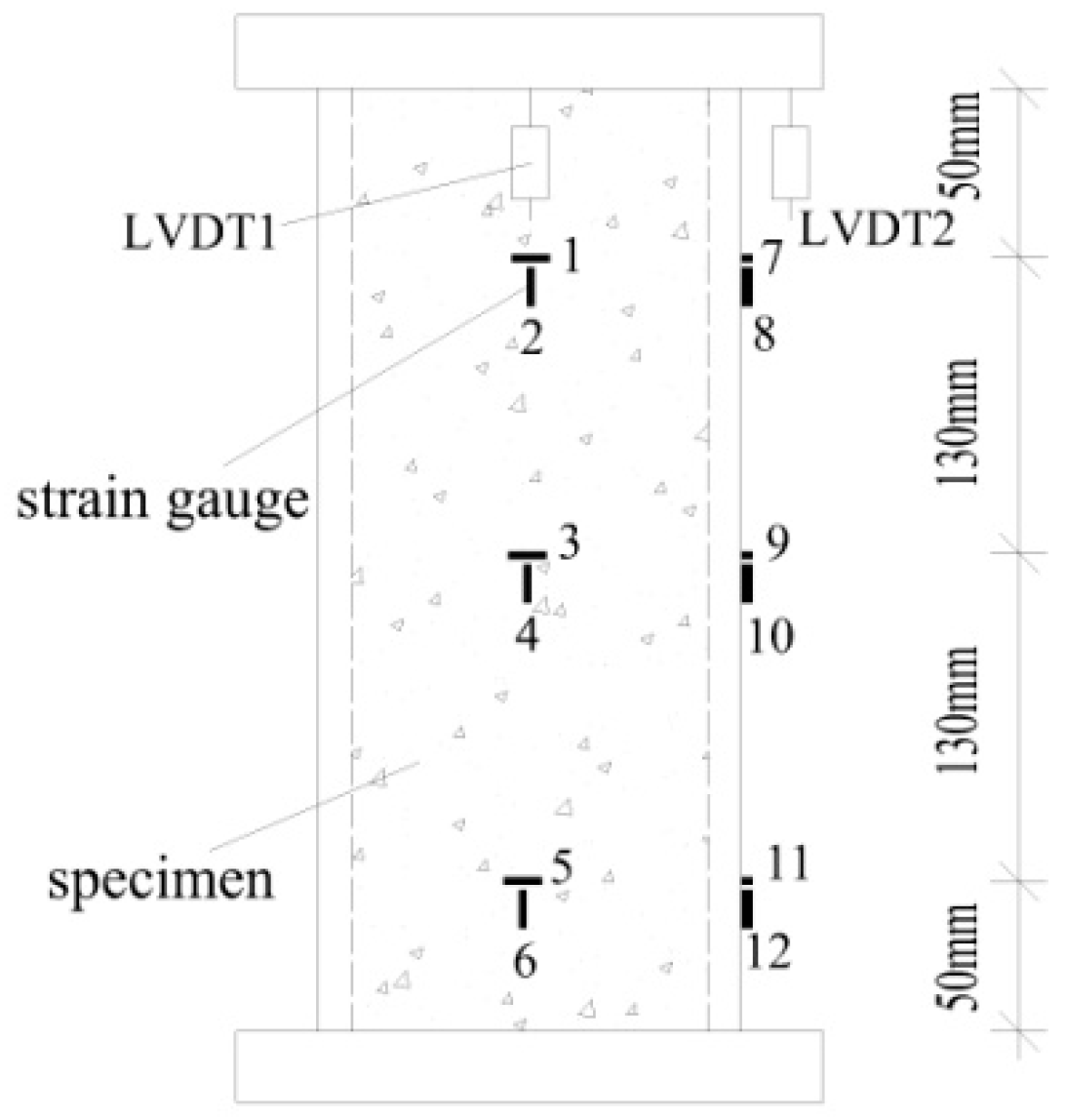
2. Experiment Overview
3. Finite Element Analysis
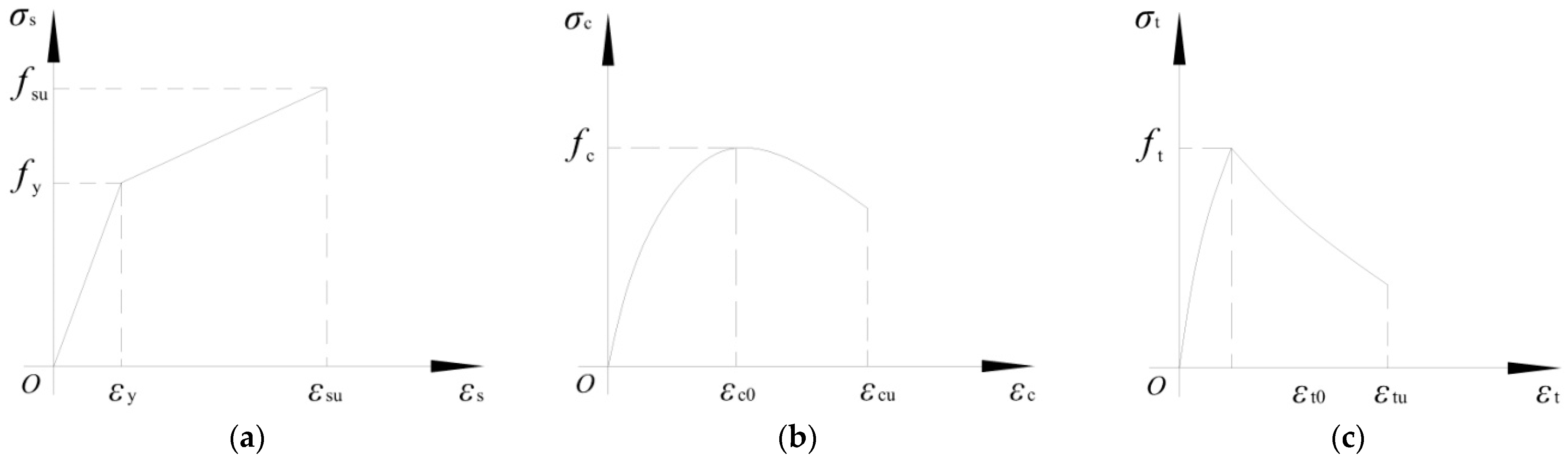
3.1. Finite Element Model
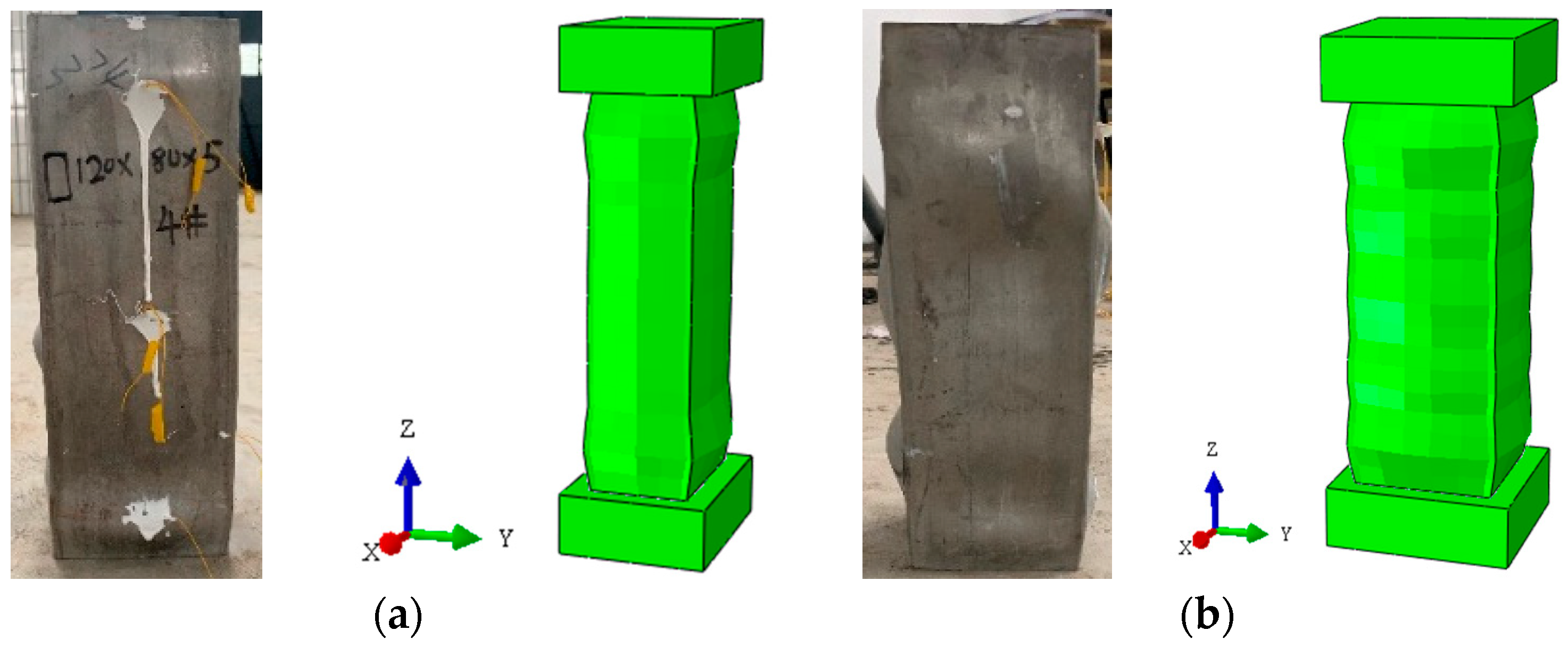
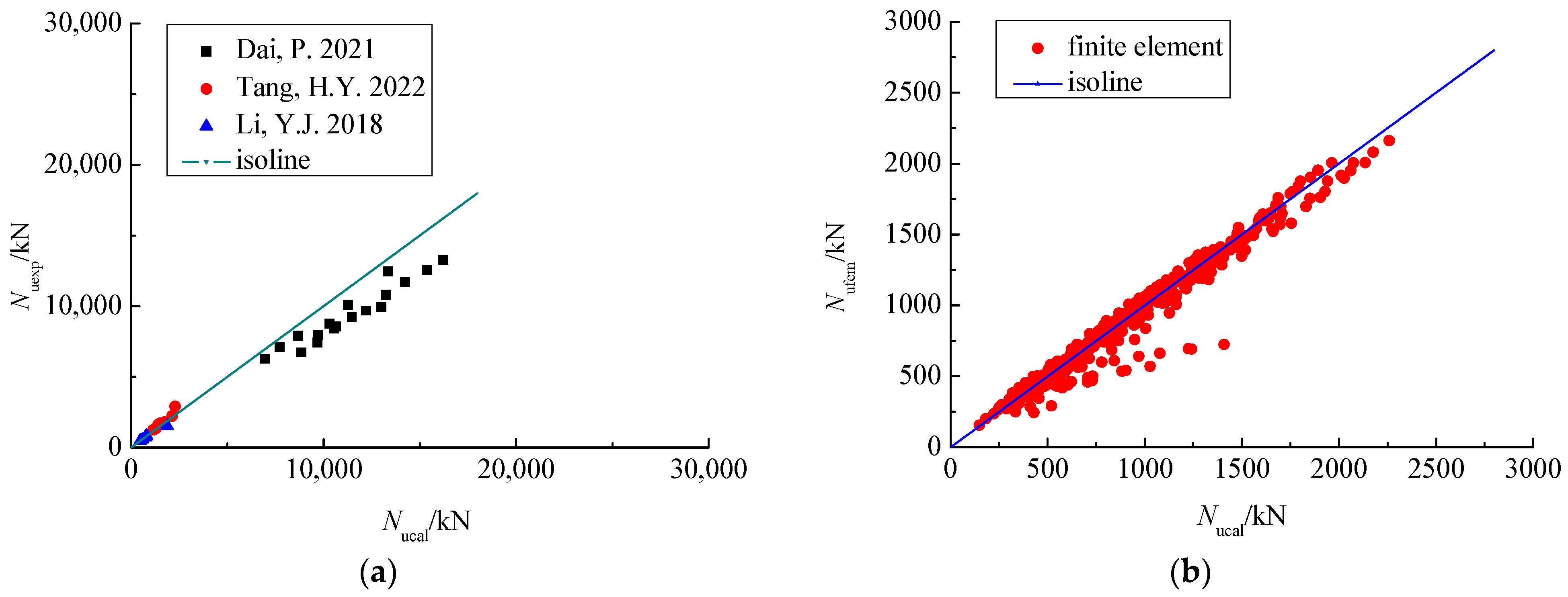
3.2. Finite Element Model Verification
3.3. Analysis of Finite Element Calculation
3.3.1. Analysis of Load–Displacement Curves
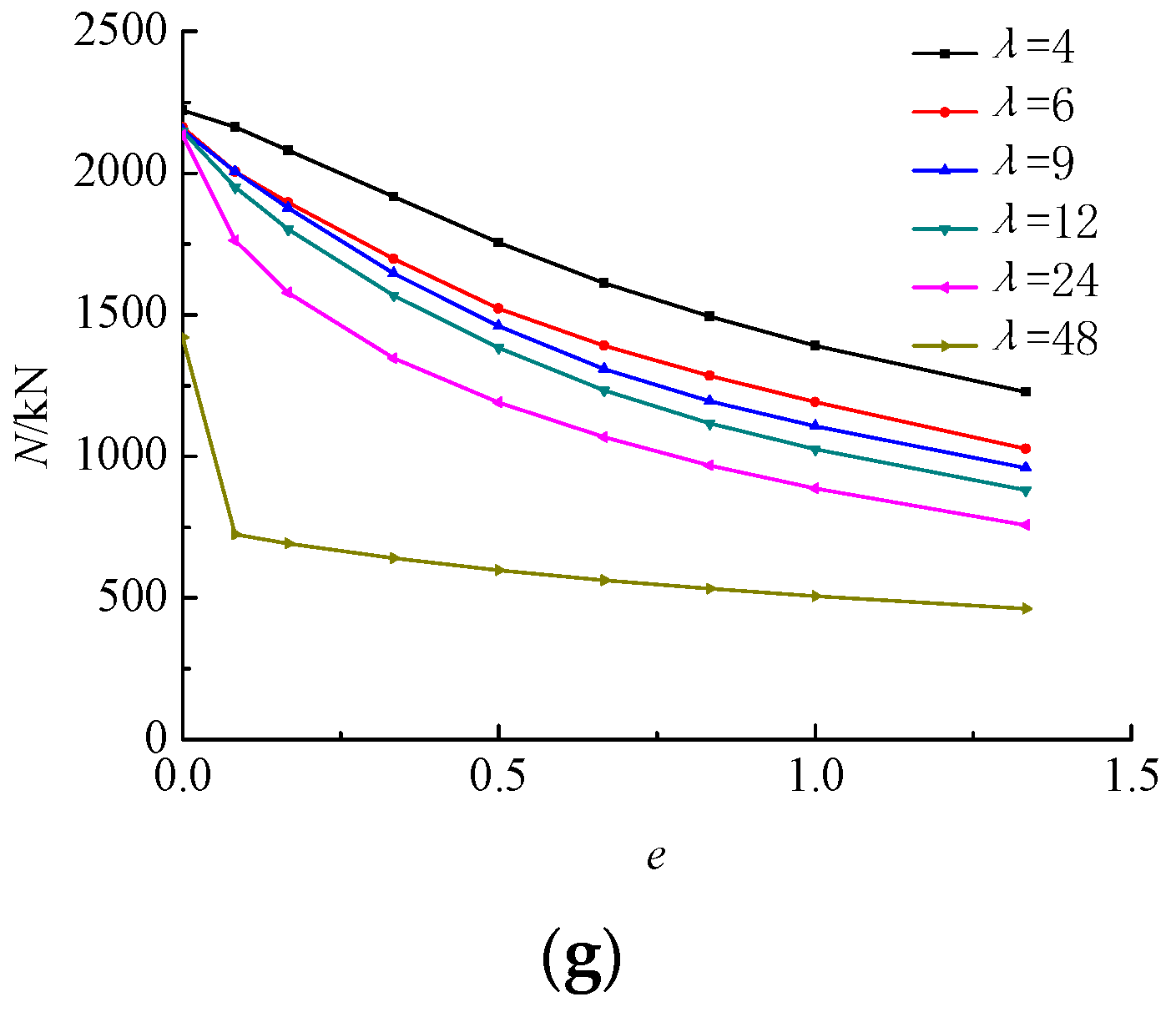
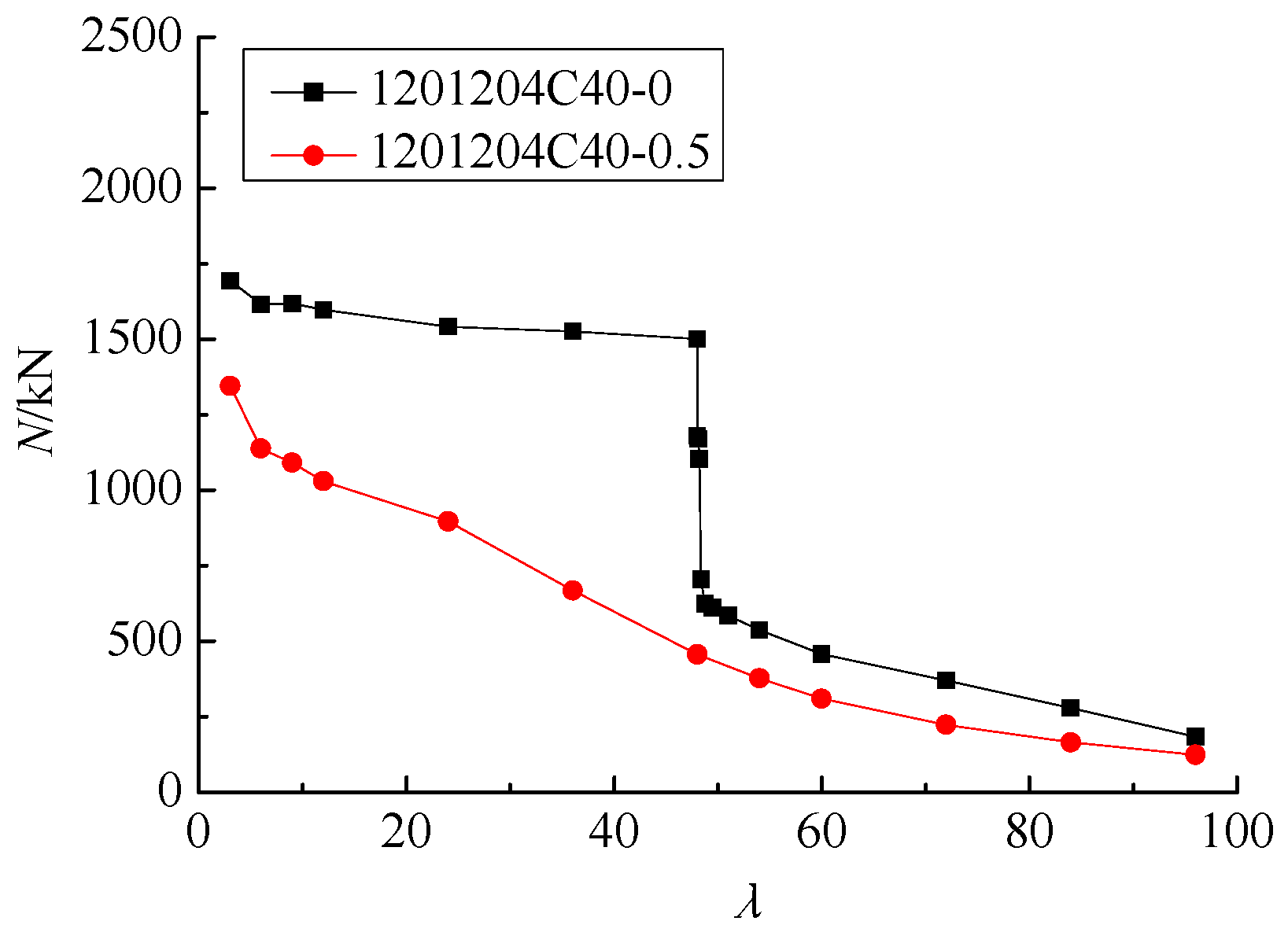
3.3.2. Analysis of Compression Bearing Capacity
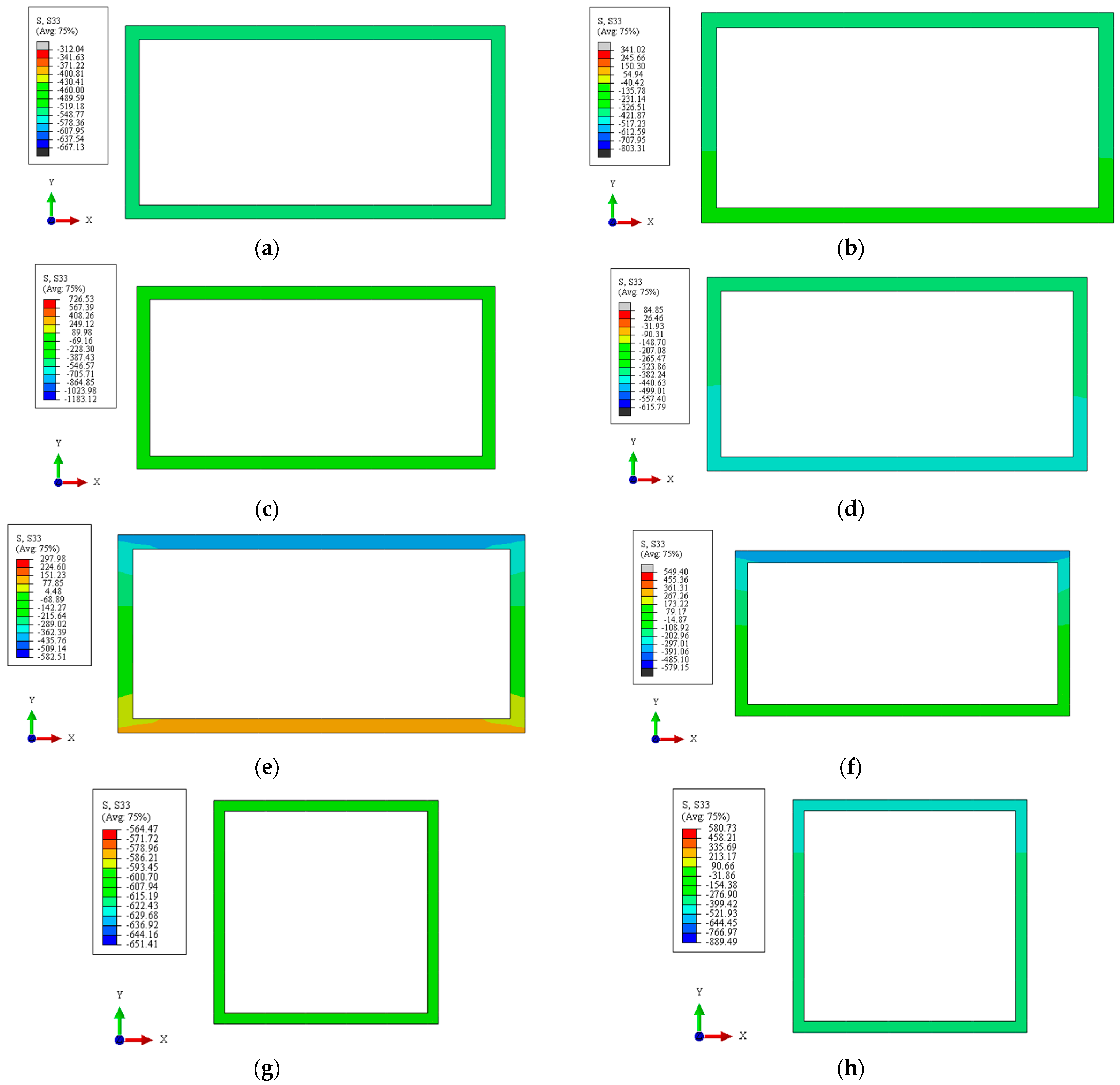
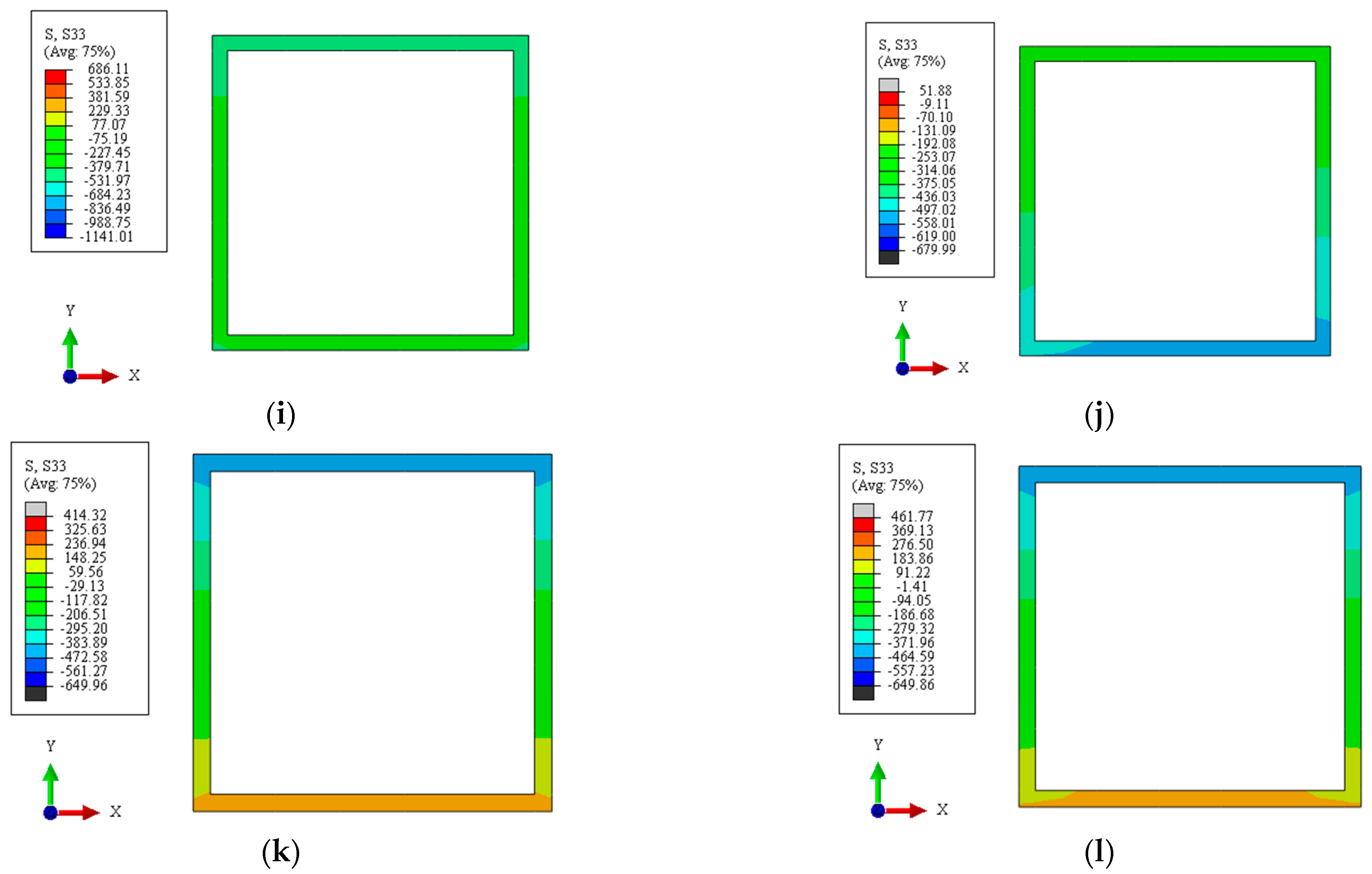
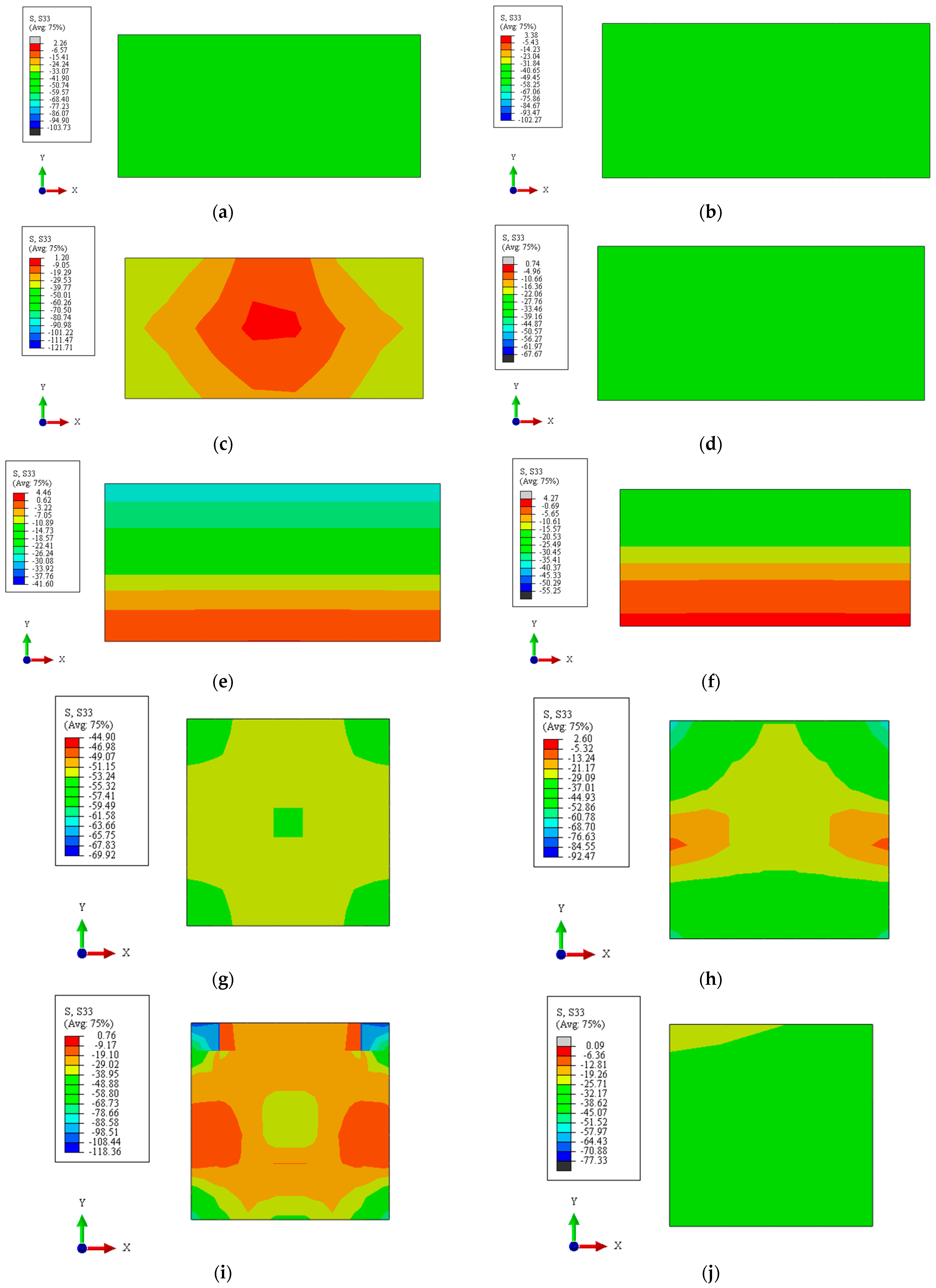
3.3.3. Analysis of Longitudinal Stress Distribution in the Central Cross-Section
- Analysis of longitudinal stress distribution of the stainless steel tubes in the central cross-section
- 2.
- Analysis of longitudinal stress distribution of core concrete in the central cross-section
4. Calculation Formula of Compression Bearing Capacity
4.1. Failure Mode Analysis
4.2. Calculation Method of Compression Bearing Capacity
4.2.1. Calculation Formula of Compression Bearing Capacity of Short Column under Axial Compression Load
4.2.2. Calculation Formula of Compression Bearing Capacity of Long Column under Axial Compression Load
4.2.3. Calculation Formula of Compression Bearing Capacity of Eccentric Column
4.2.4. Verification of the Proposed Formula of Compression Bearing Capacity
5. Conclusions
- (1)
- The finite element model can effectively simulate the compression bearing capacity; the mean of finite element calculations Nufem to the test Nuexp is 0.985, and the variance is 0.000621.
- (2)
- The slenderness ratio and relative eccentricity have a great influence on the load–displacement curves. The thickness of the stainless steel tube has little influence on the load–displacement curves. With the increase in slenderness ratio and relative eccentricity, the compression bearing capacity decreases.
- (3)
- With the increase in the slenderness ratio, the failure model of the specimen gradually changes from plastic failure to elastoplastic failure and then elastic failure.
- (4)
- When the slenderness ratio is the same, if the relative eccentricity is larger, increasing the thickness of the stainless steel tube will be more effective in improving the compression bearing capacity. When the relative eccentricity is the same, if the slenderness ratio is smaller, increasing the thickness of the stainless steel tube will be more effective in improving the compression bearing capacity.
- (5)
- The slenderness ratio and relative eccentricity have a great influence on the longitudinal stress distribution in the cross-section. When the slenderness ratio and relative eccentricity are larger, the longitudinal compressive stress in parts of the cross-section gradually becomes the longitudinal tensile stress.
- (6)
- The proposed formula can effectively predict the compression bearing capacity of concrete-filled rectangular stainless steel tubular columns. The mean of theoretical calculations to the test and the finite element is 1.054, and the variance is 0.0247.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xu, Y.; Tang, H.; Chen, J.; Jia, Y.; Liu, R. Numerical analysis of CFRP-confined concrete-filled stainless steel tubular stub columns under axial compression. J. Build. Eng. 2021, 37, 102130. [Google Scholar] [CrossRef]
- Chen, Z.; Xu, R.; Ning, F.; Liang, Y. Compression behaviour and bearing capacity calculation of concrete filled double skin square steel columns. J. Build. Eng. 2021, 42, 103022. [Google Scholar] [CrossRef]
- Guo, H.W.; Zheng, H. The linear analysis of thin shell problems using the numerical manifold method. Thin Walled Struct. 2018, 124, 366–383. [Google Scholar] [CrossRef]
- Guo, H.W.; Zheng, H.; Zhuang, X.Y. Numerical manifold method for vibration analysis of Kirchhoff’s plates of arbitrary geometry. Appl. Math. Model. 2019, 66, 695–727. [Google Scholar] [CrossRef]
- Lam, D.; Gardner, L. Structural design of stainless-steel concrete filled columns. J. Constr. Steel Res. 2008, 64, 1275–1282. [Google Scholar] [CrossRef]
- Uy, B.; Tao, Z.; Han, L.H. Behaviour of short and slender concrete-filled stainless steel tubular columns. J. Constr. Steel Res. 2010, 67, 360–378. [Google Scholar] [CrossRef]
- Ellobody, E.; Ghazy, M.F. Eperimental investigation of eccentrically loaded fibre reinforced concrete-filled stainless steel tubular columns. J. Constr. Steel Res. 2012, 76, 167–176. [Google Scholar] [CrossRef]
- Ellobody, E.; Ghazy, M.F. Numerical modelling of fibre reinforced concrete-filled stainless steel tubular columns. Thin Walled Struct. 2013, 63, 1–12. [Google Scholar] [CrossRef]
- Ellobody, E. Nonlinear behaviour of eccentrically loaded FR concrete-filled stainless steel tubular columns. J. Constr. Steel Res. 2013, 90, 1–12. [Google Scholar] [CrossRef]
- Tokgoz, S. Tests on plain and steel fiber concrete-filled stainless steel tubular columns. J. Constr. Steel Res. 2015, 114, 129–135. [Google Scholar] [CrossRef]
- Hassanein, M.F.; Shao, Y.B.; Elchalakani, M.; El Hadidy, A.M. Flexural buckling of circular concrete-filled stainless steel tubular columns. Mar. Struct. 2020, 71, 102722. [Google Scholar] [CrossRef]
- Elsisy, A.R.; Shao, Y.B.; Zhou, M.; Hassanein, M.F. A study on the compressive strengths of stiffened and unstiffened concrete-filled austenitic stainless steel tubular short columns. Ocean. Eng. 2022, 248, 110793. [Google Scholar] [CrossRef]
- Al-Mekhlafi, G.M.; Al-Osta, M.A.; Sharif, A.M. Behavior of eccentrically loaded concrete-filled stainless steel tubular stub columns confined by CFRP composites. Eng. Struct. 2020, 205, 110113. [Google Scholar] [CrossRef]
- Patel, V.I.; Liang, Q.Q.; Hadi, M.N.S. Nonlinear analysis of axially loaded circular concrete-filled stainless steel tubular short columns. J. Constr. Steel Res. 2014, 101, 9–18. [Google Scholar] [CrossRef] [Green Version]
- He, A.; Su, A.D.; Liang, Y.T.; Zhao, O. Experimental and numerical investigations of circular recycled aggregate concrete-filled stainless steel tube columns. J. Constr. Steel Res. 2021, 179, 106566. [Google Scholar] [CrossRef]
- Tang, H.Y.; Fang, L.Y.; Zhao, X. Bearing capacity model of circular concrete-filled stainless steel stub columns under uniaxial compression. Adv. Eng. Sci. 2020, 52, 10–20. [Google Scholar]
- Duan, W.F.; Zhao, L.; Leng, J. Finite element analysis of axial compressive bearing capacity of recycled concrete short columns with circular stainless steel tubes. J. Jilin Jianzhu Univ. 2017, 34, 9–12. [Google Scholar]
- Liao, F.Y.; Hou, C.; Zhang, W.J.; Ren, J. Experimental investigation on sea sand concrete-filled stainless steel tubular stub columns. J. Constr. Steel Res. 2019, 155, 46–61. [Google Scholar] [CrossRef]
- Ding, F.X.; Yin, Y.X.; Wang, L.P.; Yu, Y.; Luo, L.; Yu, Z.W. Confinement coefficient of concrete-filled square stainless steel tubular stub columns. Steel Compos. Struct. 2019, 30, 337–350. [Google Scholar]
- Dai, P.; Yang, L.; Wang, J.; Gang, Y.; Yang, S. Experimental study on bearing behavior of concrete-filled square stainless steel tubular stub columns under axial compression. J. Build. Struct. 2021, 42, 17–24. [Google Scholar]
- Tang, H.Y.; Li, Z.Z.; Fang, L.Y.; Hong, Y. Experimental investigation on behavior of rectangular concrete-filled stainless steel stub column under axial loading. J. Southwest Jiaotong Univ. 2022, 57, 855–864. [Google Scholar]
- Li, Y.J.; Liao, F.Y.; Huang, H.Q. Experimental study on the behavior of concrete filled rectangular stainless steel tubular columns under bi-axial eccentric compression. Prog. Steel Build. Struct. 2018, 20, 60–66. [Google Scholar]
- Le, T.T.; Patel, V.I.; Liang, Q.Q.; Huynh, P. Axisymmetric simulation of circular concrete-filled double-skin steel tubular short columns incorporating outer stainless-steel tube. Eng. Struct. 2021, 227, 111416. [Google Scholar] [CrossRef]
- Le, T.T.; Patel, V.I.; Liang, Q.Q.; Huynh, P. Numerical modeling of rectangular concrete-filled double-skin steel tubular columns with outer stainless-steel skin. J. Constr. Steel Res. 2021, 179, 106504. [Google Scholar] [CrossRef]
- EN 1994-1-1; Eurocode 4. Design of Composite Steel and Concrete Structures Part1-1: General Rules and Rules for Building. CEN: Brussels, Belgium, 2004.
- EN 1993-1-1; Eurocode 3. Design of Steel Structures Part1-1: General Rules and Rules for Building. CEN: Brussels, Belgium, 2005.
- EN 1993-1-4; Eurocode 3. Design of Steel Structures Part1-4: General Rules—Supplementary Rules for Stainless Steels. CEN: Brussels, Belgium, 2006.
- GB/T 2975-2018; Steel and Steel Products—Location and Preparation of Samples and Test Pieces for Mechanical Testing. China Architecture and Building Press: Beijing, China, 2018.
- GB/T 228-2010; Metallic Materials—Tensile Testing—Method of Test at Ambient Temperature. China Architecture and Building Press: Beijing, China, 2010.
- GB/T 50081-2002; Standard for Test Method of Mechanical Properties on Ordinary Concrete. China Architecture and Building Press: Beijing, China, 2002.
- GB/T 50152-2012; Standard for Test Method of Concrete Structures. China Architecture and Building Press: Beijing, China, 2012.
- Wang, C.G. Experimental and Analytical Study on Eccentric Compression and Seismic Behavior of Recycled Aggregate Concrete Filled Square Steel Tube Long Columns. Ph.D. Thesis, Heifei University of Technology, Heifei, China, 2018; pp. 67–73. [Google Scholar]
- Cao, B.; Zhang, X.; Liang, N.; Yang, Y.; Shen, D.; Huang, B.; Du, Y.H. Bearing capacity of welded composite T-shaped concrete-filled steel tubular columns under axial compression. Adv. Mech. Eng. 2020, 12, 1687814020923102. [Google Scholar] [CrossRef]
- Han, L.H. Concrete-Filled Steel Tubular Structure-Theory and Practice; Science Press: Beijing, China, 2004. [Google Scholar]
- Luo, X.; Wei, J.G.; Han, J.P. Experimental study on compression performance of ultra-high strength concrete (UHSC) filled high-strength steel tube stub columns subjected to eccentric load. J. Build. Struct. 2021, 42, 271–277. [Google Scholar]



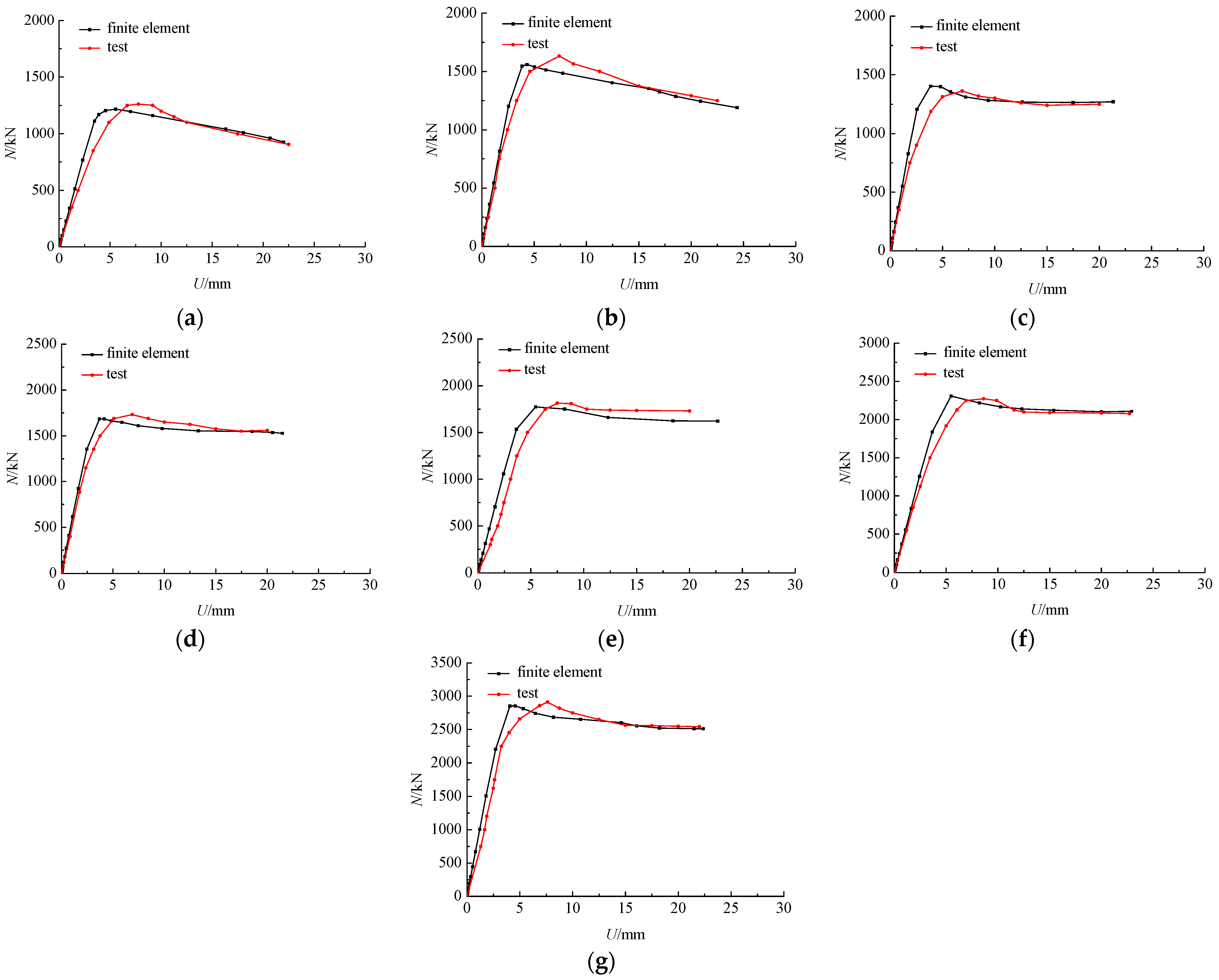



| Specimen Number | Length of Steel Tube a/mm | Width of Steel Tube b/mm | Thickness of Steel Tube t/mm | Length of Specimen L/mm | Compression Bearing Capacity Nuexp/kN |
|---|---|---|---|---|---|
| S1 | 120 | 60 | 4 | 360 | 1261 |
| S2 | 120 | 60 | 5 | 360 | 1632 |
| S3 | 120 | 80 | 4 | 360 | 1362 |
| S4 | 120 | 80 | 5 | 360 | 1732 |
| S5 | 120 | 120 | 4 | 360 | 1814 |
| S6 | 120 | 120 | 5 | 360 | 2224 |
| S7 | 120 | 120 | 6 | 360 | 2913 |
| Finite Element Model Type | Length of Steel Tube a/mm | Width of Steel Tube b/mm | Thickness of Steel Tube t/mm | Yield Strength of Steel Tube fy/MPa | Axial Compressive Strength of Concrete fc/MPa | Slenderness Ratio λ | Relative Eccentricity e |
|---|---|---|---|---|---|---|---|
| FEM 1 | 120 | 60 | 4 | 534.3 | 29.48 | 6~48 | 0~2.667 |
| FEM 2 | 120 | 60 | 5 | 572.3 | 29.48 | 6~48 | 0~2.667 |
| FEM 3 | 120 | 80 | 4 | 534.3 | 29.48 | 6~48 | 0~2 |
| FEM 4 | 120 | 80 | 5 | 572.3 | 29.48 | 6~48 | 0~2 |
| FEM 5 | 120 | 120 | 4 | 534.3 | 29.48 | 3~96 | 0~1.333 |
| FEM 6 | 120 | 120 | 5 | 572.3 | 29.48 | 3~48 | 0~1.333 |
| FEM 7 | 120 | 120 | 6 | 598.0 | 29.48 | 3~48 | 0~1.333 |
| Failure Mode | Neutralization Axis | Tensile Area of the Stainless Steel Tube | Compressive Area of the Stainless Steel Tube | Tensile Area of the Core Concrete | Compressive Area of the Core Concrete |
|---|---|---|---|---|---|
| 1 | Not through the cross-section | No tensile area | All are under compression, which is yielding | No tensile area | All are under compression, which has reached the ultimate compressive strength |
| 2 | Not through the cross-section | No tensile area | All are under compression, which is not yielding | No tensile area | All are under compression, some areas have reached the ultimate compressive strength, while other areas have not reached it |
| 3 | Not through the cross-section | No tensile area | All are under compression, but some areas are yielding and other areas are not yielding | No tensile area | All are under compression, some areas have reached the ultimate compressive strength, while other areas have not reached it |
| 4 | Through the cross-section | There are tensile areas, which are not yielding | There are tensile areas, which are not yielding | No tensile area | All are under compression, some areas have reached the ultimate compressive strength, while other areas have not reached it |
| 5 | Through the cross-section | There are tensile areas, which are not yielding | There are tensile areas, which are not yielding | There are tensile areas, which have reached the ultimate tensile strength | There are compression areas, which have not reached the ultimate compressive strength |
| 6 | Through the cross-section | There are tensile areas, which are not yielding | There are tensile areas, which are not yielding | There are tensile areas, which have reached the ultimate tensile strength | There are compression areas, which have reached the ultimate compressive strength |
| 7 | Through the cross-section | There are tensile areas, which are yielding | There are tensile areas, which are yielding | There are tensile areas, which have reached the ultimate tensile strength | There are compression areas, which have reached the ultimate compressive strength |
| Slenderness Ratio λ | The Formula of η | Scope of Application |
|---|---|---|
| λ ≤ 4 | 0 < e ≤ 2.667 e = max{ex, ey} | |
| 4 < λ ≤ 6 | ||
| 6 < λ ≤ 8 | ||
| 8 < λ ≤ 13.5 | ||
| 13.5 < λ ≤ 18 | ||
| 18 < λ ≤ 24 | ||
| 24 < λ ≤ 36 | ||
| 36 < λ ≤ 48 |
| Specimen Number | Compression Bearing Capacity Nuexp/kN | Data Sources | Specimen Number | Compression Bearing Capacity Nuexp/kN | Data Sources |
|---|---|---|---|---|---|
| 304-t8C50 | 6290 | Reference [20] | 120 × 60 × 4 | 1261 | Reference [21] |
| 304-t10C50 | 7113 | 120 × 60 × 5 | 1632 | ||
| 304-t12C50 | 7924 | 120 × 80 × 4 | 1362 | ||
| 304-t8C70 | 6743 | 120 × 80 × 5 | 1732 | ||
| 304-t10C70 | 7947 | 120 × 120 × 4 | 1814 | ||
| 304-t12C70 | 8575 | 120 × 120 × 5 | 2224 | ||
| 304-t8C80 | 7436 | 120 × 120 × 6 | 2913 | ||
| 304-t10C80 | 8430 | r-0-0-a | 1542 | Reference [22] | |
| 304-t12C80 | 9257 | r-0-0-b | 1498 | ||
| 2205-t8C50 | 8771 | r-0.50-0.50-a | 734 | ||
| 2205-t10C50 | 10,111 | r-0.50-0.50-b | 716 | ||
| 2205-t12C50 | 12,472 | r-0.75-0.75-a | 485 | ||
| 2205-t8C70 | 9686 | r-0.75-0.75-b | 497 | ||
| 2205-t10C70 | 10,820 | rc1-0.5-0.5-a | 533 | ||
| 2205-t12C70 | 12,560 | rc1-0.5-0.5-b | 524 | ||
| 2205-t8C80 | 9962 | rc2-0.5-0.5-a | 824 | ||
| 2205-t10C80 | 11,728 | rc2-0.5-0.5-b | 814 | ||
| 2205-t12C80 | 13,272 | rl1-0.5-0.5-a | 795 | ||
| – | – | rl1-0.5-0.5-b | 778 | ||
| – | – | rl2-0.5-0.5-a | 562 | ||
| – | – | rl2-0.5-0.5-b | 564 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, B.; Zhu, L.; Jiang, X.; Wang, C. An Investigation of Compression Bearing Capacity of Concrete-Filled Rectangular Stainless Steel Tubular Columns under Axial Load and Eccentric Axial Load. Sustainability 2022, 14, 8946. https://doi.org/10.3390/su14148946
Cao B, Zhu L, Jiang X, Wang C. An Investigation of Compression Bearing Capacity of Concrete-Filled Rectangular Stainless Steel Tubular Columns under Axial Load and Eccentric Axial Load. Sustainability. 2022; 14(14):8946. https://doi.org/10.3390/su14148946
Chicago/Turabian StyleCao, Bing, Longfei Zhu, Xintong Jiang, and Changsheng Wang. 2022. "An Investigation of Compression Bearing Capacity of Concrete-Filled Rectangular Stainless Steel Tubular Columns under Axial Load and Eccentric Axial Load" Sustainability 14, no. 14: 8946. https://doi.org/10.3390/su14148946
APA StyleCao, B., Zhu, L., Jiang, X., & Wang, C. (2022). An Investigation of Compression Bearing Capacity of Concrete-Filled Rectangular Stainless Steel Tubular Columns under Axial Load and Eccentric Axial Load. Sustainability, 14(14), 8946. https://doi.org/10.3390/su14148946





