An Energy Storage Assessment: Using Frequency Modulation Approach to Capture Optimal Coordination
Abstract
:1. Introduction
2. Background Study
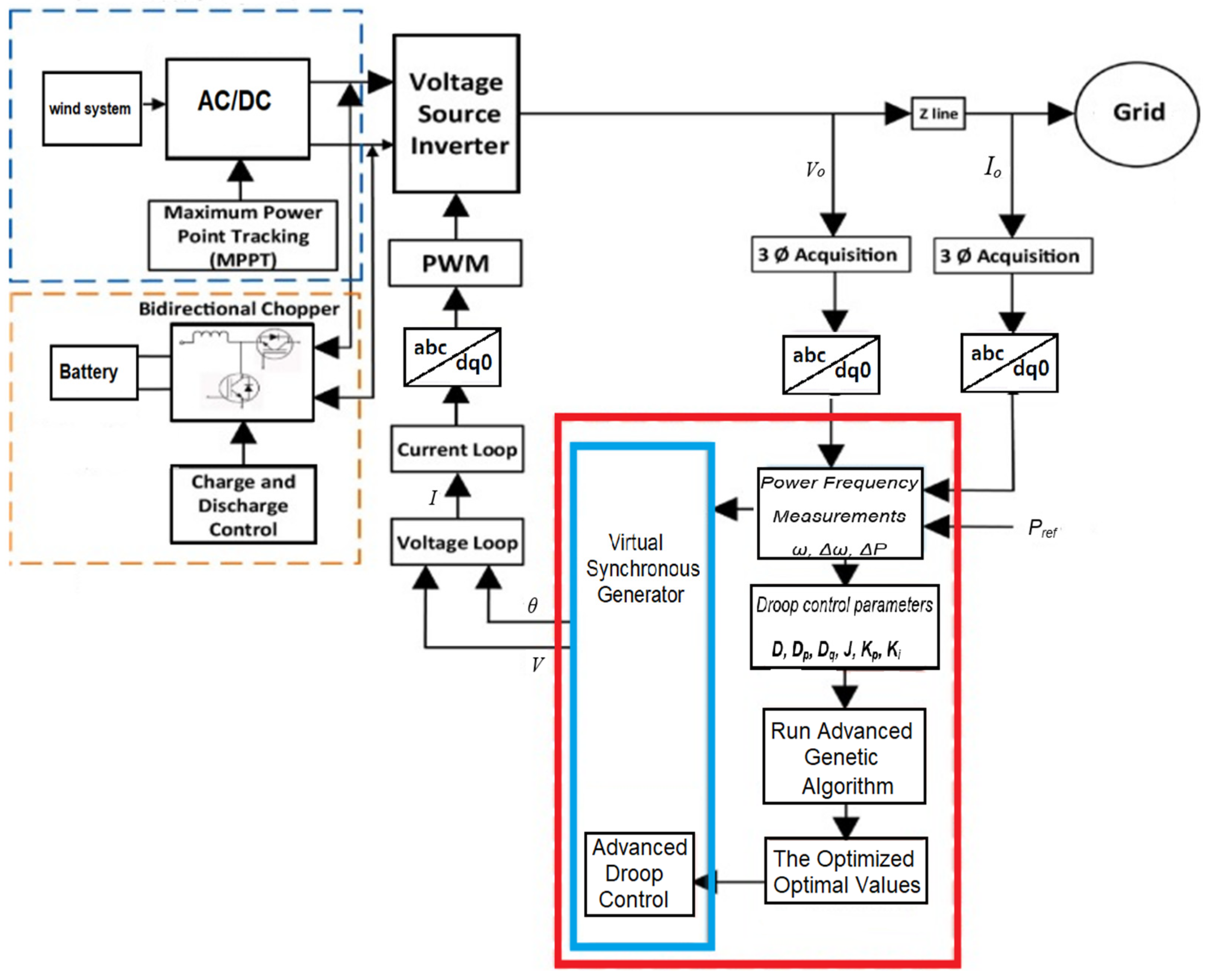
2.1. Virtual Synchronous Generator
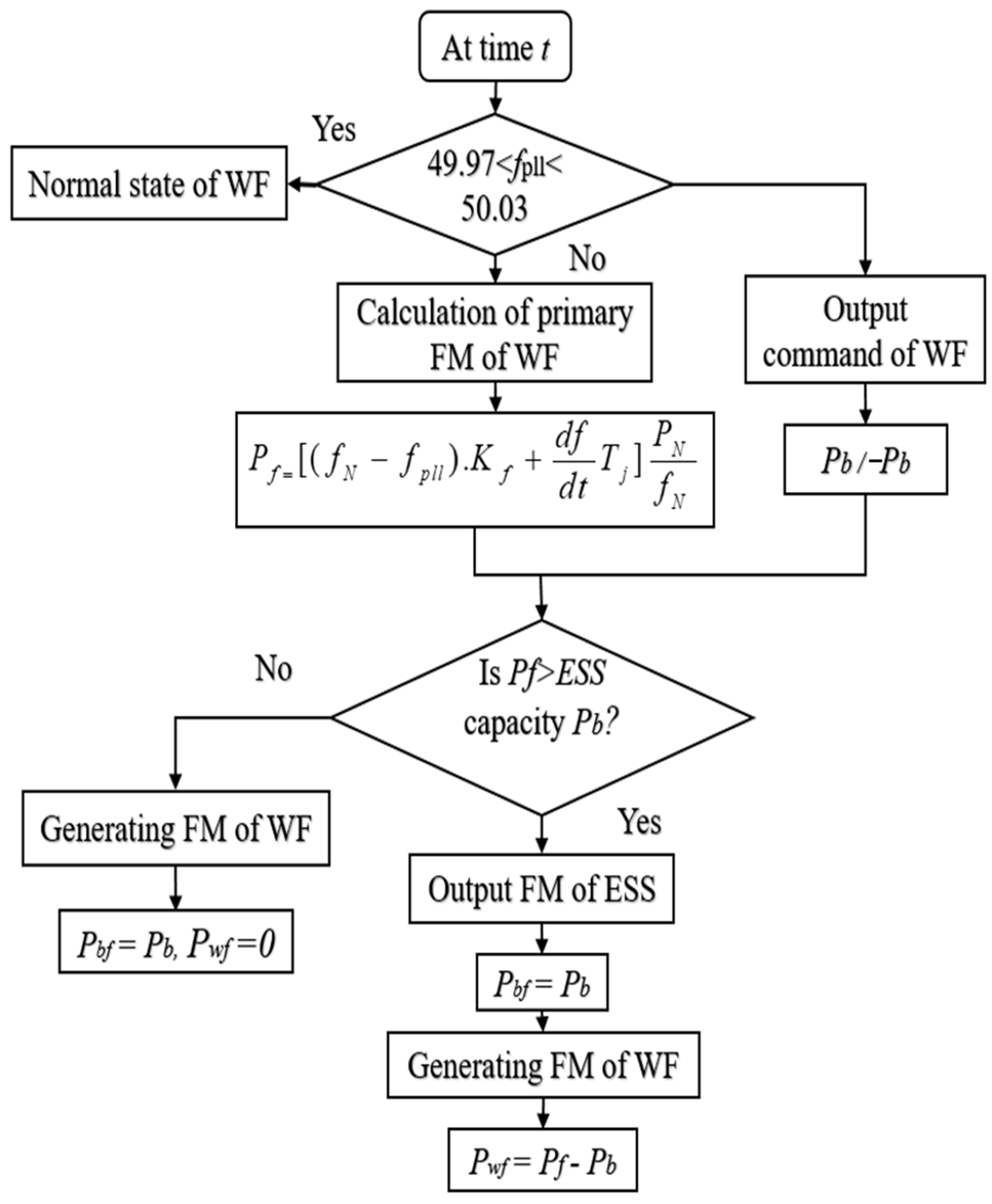
2.2. Frequency Modulation Coordination
2.3. Optimal Energy Storage Capacity
3. Proposed Methods
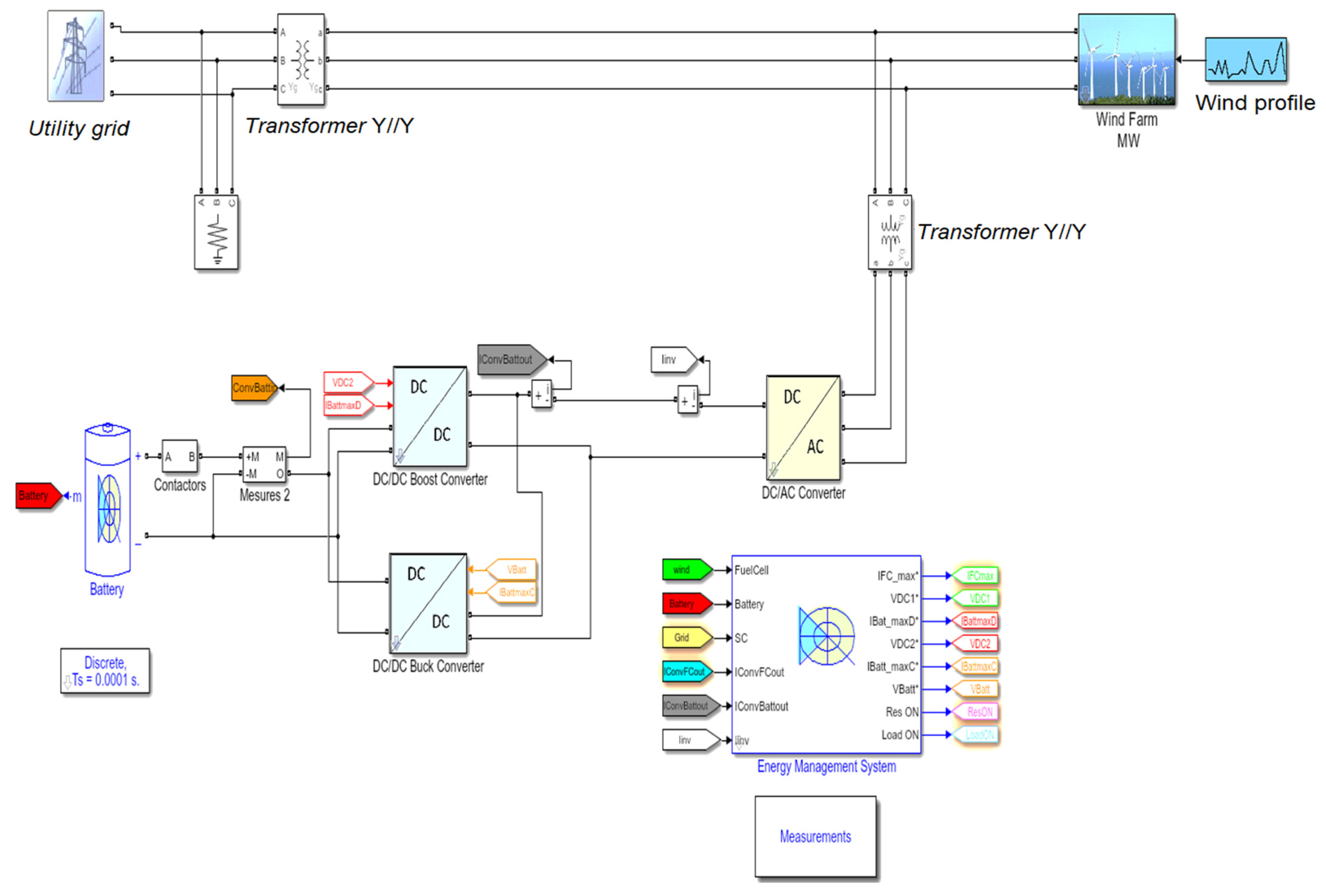
3.1. Virtual Synchronous Generator Model
3.2. Optimized Control Strategy
3.3. Optimal Economic Capacity
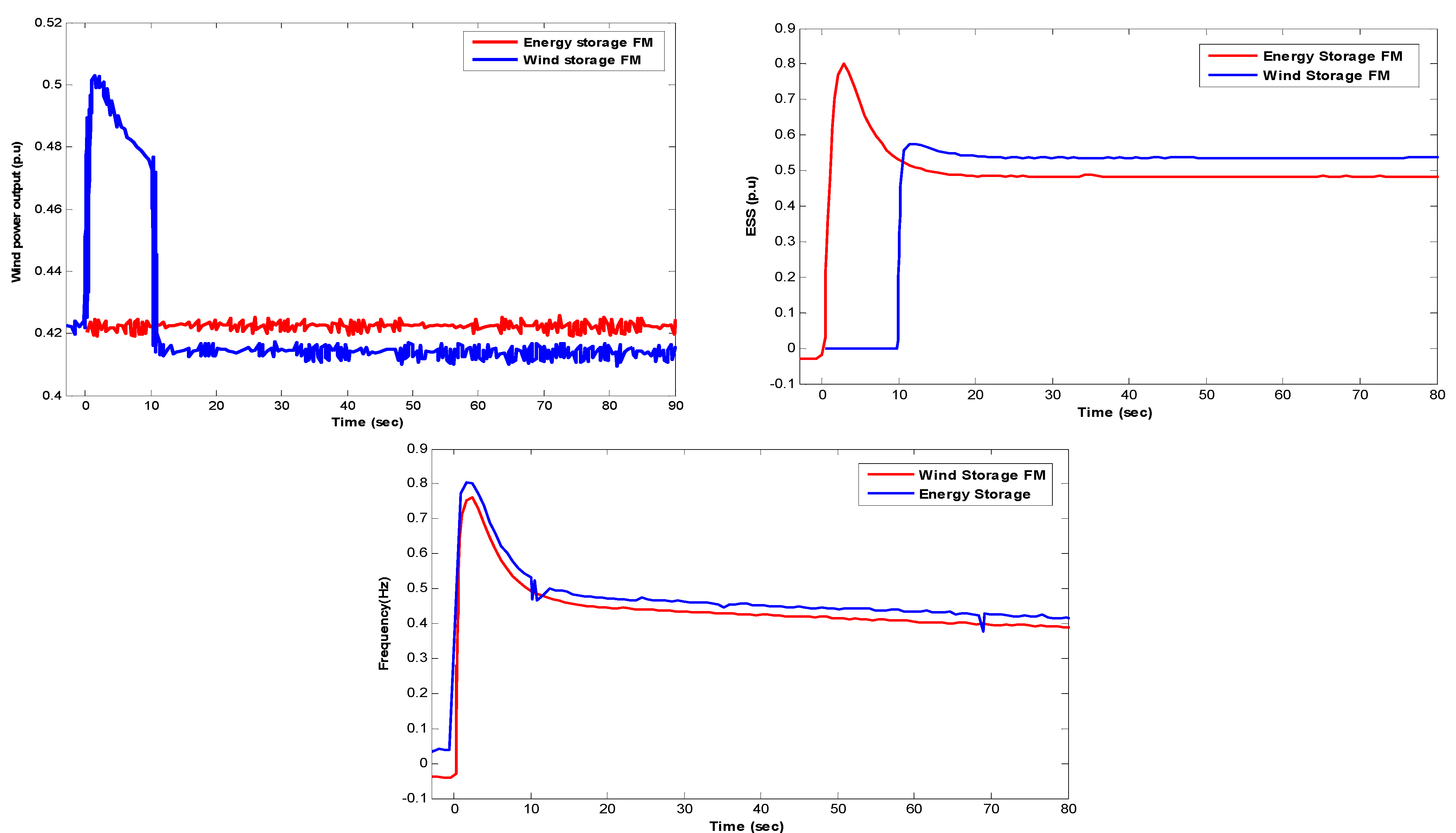
4. Results and Discussion
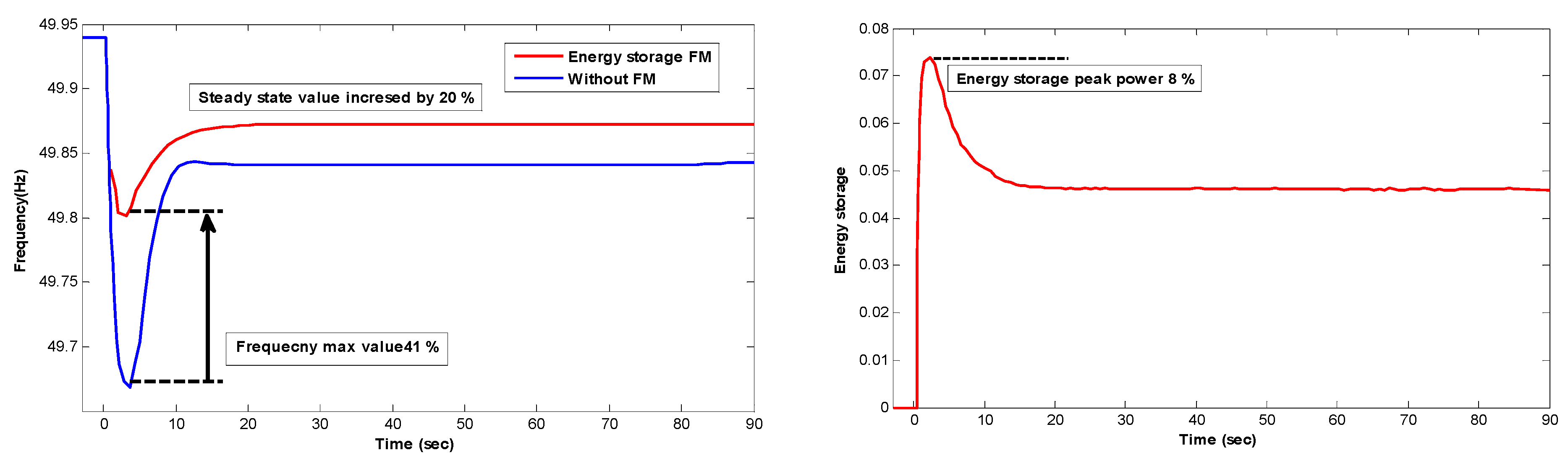
4.1. Results
4.2. Discussion
5. Conclusions
- One can improve the economics of energy storage by determining the design method of its rated power and the capacity according to the FM requirements.
- The energy storage capacity under high wind speeds is configured to be 5.9% of the installed capacity, which is a reduction of 26% compared with the 8% capacity required for independent support of energy storage.
- The comprehensive optimal energy storage capacity configuration of the coordinated FM control strategy is improved.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| FM | Frequency modulation |
| ESS | Energy storage system |
| SOC | State of charge |
| FM power | |
| Rated frequency of the system | |
| Pwf | Power of the wind turbine |
| Inertial time constant | |
| Energy storage capacity | |
| Cbat | Energy storage device cost |
| Cpcs | Power conversion system cost |
| Cbop | Auxiliary equipment cost |
| Erated | Rated power of the ESS |
| CB | Unit electricity price |
| Cf | Operation and maintenance cost |
| J | Optimization objective |
References
- Ramírez, M.; Castellanos, R.; Calderón, G.; Malik, O. Placement and sizing of battery energy storage for primary frequency control in an isolated section of the Mexican power system. Electr. Power Syst. Res. 2018, 160, 142–150. [Google Scholar]
- Bhutta, M.S.; Sarfraz, M.; Ivascu, L.; Li, H.; Rasool, G.; ul Abidin Jaffri, Z.; Farooq, U.; Ali Shaikh, J.; Nazir, M.S. Voltage Stability Index Using New Single-Port Equivalent Based on Component Peculiarity and Sensitivity Persistence. Processes 2021, 9, 1849. [Google Scholar]
- Rahman, F.S.; Kerdphol, T.; Watanabe, M.; Mitani, Y. Optimization of virtual inertia considering system frequency protection scheme. Electr. Power Syst. Res. 2019, 170, 294–302. [Google Scholar]
- El-Bidairi, K.S.; Nguyen, H.D.; Mahmoud, T.S.; Jayasinghe, S.; Guerrero, J.M. Optimal sizing of Battery Energy Storage Systems for dynamic frequency control in an islanded microgrid: A case study of Flinders Island, Australia. Energy 2020, 195, 117059. [Google Scholar]
- Belila, A.; Amirat, Y.; Benbouzid, M.; Berkouk, E.M.; Yao, G. Virtual synchronous generators for voltage synchronisation of a hybrid PV-diesel power system. Int. J. Electr. Power Energy Syst. 2020, 117, 1056776. [Google Scholar]
- Zhu, T.; Nazir, M.S.; Ali Mokhtarzadeh, A.; Abdalla, A.N.; Nazir, H.M.; Chen, W. Improve performance of induction motor drive using weighting factor approach-based gravitational search algorithm. Int. J. Electron. 2022, 109, 900–913. [Google Scholar]
- Nazir, M.S.; Abdalla, A.N.; MMetwally, A.S.; Imran, M.; Bocchetta, P.; Javed, M.S. Cryogenic-Energy-Storage-Based Optimized Green Growth of an Integrated and Sustainable Energy System. Sustainability 2022, 14, 5301. [Google Scholar]
- Abdalla, A.N.; Nazir, M.S.; Tao, H.; Cao, S.; Ji, R.; Jiang, M.; Yao, L. Integration of energy storage system and renewable energy sources based on artificial intelligence: An overview. J. Energy Storage 2021, 40, 102811. [Google Scholar]
- Das, C.K.; Mahmoud, T.S.; Bass, O.; Muyeen, S.; Kothapalli, G.; Baniasadi, A.; Mousavi, N. Optimal sizing of a utility-scale energy storage system in transmission networks to improve frequency response. J. Energy Storage 2020, 29, 101315. [Google Scholar]
- Nazir, M.S.; Abdalla, A.N.; Sohail, H.M.; Tang, Y.; Rashed, G.I.; Chen, W. Optimal planning and investment of Multi-renewable power generation and energy storage system capacity. J. Electr. Syst. 2021, 17, 171–181. [Google Scholar]
- Soni, N.; Doolla, S.; Chandorkar, M.C. Improvement of transient response in microgrids using virtual inertia. IEEE Trans. Power Deliv. 2013, 28, 1830–1838. [Google Scholar]
- D’Arco, S.; Suul, J.A.; Fosso, O.B. A virtual synchronous machine implementation for distributed control of power converters in smartgrids. Electr. Power Syst. Res. 2015, 122, 180–197. [Google Scholar]
- Sakimoto, K.; Miura, Y.; Ise, T. Stabilization of a power system including inverter type distributed generators by the virtual synchronous generator. IEEJ Trans. Power Energy 2012, 132, 341–349. [Google Scholar]
- Alipoor, J.; Miura, Y.; Ise, T. Power system stabilization using virtual synchronous generator with alternating moment of inertia. IEEE J. Emerg. Sel. Top. Power Electron. 2015, 3, 451–458. [Google Scholar]
- Jongudomkarn, J.; Liu, J.; Ise, T. Comparison of current-limiting strategies of virtual synchronous generator control during fault ride-through. IFAC-Pap. Line 2018, 51, 256–261. [Google Scholar]
- Hirase, Y.; Abe, K.; Sugimoto, K.; Shindo, Y. A grid-connected inverter with virtual synchronous generator model of algebraic type. Electr. Eng. Jpn. 2013, 184, 10–21. [Google Scholar]
- Guerrero, J.M.; Vasquez, J.C.; Matas, J.; de Vicuna, L.G.; Castilla, M. Hierarchical control of droop-controlled ac and dc microgrids-a general approach toward standardization. IEEE Trans. Ind. Electron. 2011, 58, 158–172. [Google Scholar]
- Liu, J.; Miura, Y.; Bevrani, H.; Ise, T. Enhanced virtual synchronous generator control for parallel inverters in microgrids. IEEE Trans. Smart Grid. 2015, 8, 2268–2277. [Google Scholar]
- Tamrakar, U.; Galipeau, D.; Tonkoski, R.; Tamrakar, I. Improving transient stability of photovoltaic-hydro microgrids using virtual synchronous machines. In Proceedings of the 2015 IEEE Eindhoven Power Tech, Eindhoven, The Netherlands, 29 June–2 July 2015; pp. 1–6. [Google Scholar]
- Onaolapo, A.K.; Carpanen, R.P.; Dorrell, D.G.; Ojo, E.E. Reliability Evaluation and Financial Viability of an Electricity Power Micro-Grid System with the Incorporation of Renewable Energy Sources and Energy Storage: Case Study of KwaZulu-Natal, South Africa. IEEE Access 2021, 9, 159908–159924. [Google Scholar]
- Kerdphol, T.; Rahman, F.S.; Watanabe, M.; Mitani, Y. Optimization of Virtual Inertia Control Considering System Frequency Protection Scheme. In Virtual Inertia Synthesis and Control; Springer: Berlin/Heidelberg, Germany, 2021; pp. 227–247. [Google Scholar]
- Chen, J.; Jin, T.; Mohamed, M.A.; Annuk, A.; Dampage, U. Investigating the Impact of Wind Power Integration on Damping Characteristics of Low Frequency Oscillations in Power Systems. Sustainability 2022, 14, 3841. [Google Scholar]
- Nazir, M.S.; Abdalla, A.N.; Zhao, H.; Chu, Z.; Nazir, H.M.J.; Bhutta, M.S.; Javed, M.S.; Sanjeevikumar, P. Optimized economic operation of energy storage integration using improved gravitational search algorithm and dual stage optimization. J. Energy Storage 2022, 50, 104591. [Google Scholar]
- Al-Ghussain, L.; Ahmad, A.D.; Abubaker, A.M.; Mohamed, M.A. An integrated photovoltaic/wind/biomass and hybrid energy storage systems towards 100% renewable energy microgrids in university campuses. Sustain. Energy Technol. Assess. 2021, 46, 101273. [Google Scholar]
- Meng, L.; Zafar, J.; Khadem, S.K.; Collinson, A.; Murchie, K.C.; Coffele, F.; Burt, G.M. Fast frequency response from energy storage systems—A review of grid standards, projects and technical issues. IEEE Trans. Smart Grid. 2019, 11, 1566–1581. [Google Scholar]
- Judge, P.D.; Green, T.C. Modular multilevel converter with partially rated integrated energy storage suitable for frequency support and ancillary service provision. IEEE Trans. Power Deliv. 2018, 34, 208–219. [Google Scholar]
- Arifin, Z.; Firmanto, A. Battery Energy Storage System as Frequency Control at Substation based on Defense Scheme Mechanism. In Proceedings of the 2021 International Seminar on Intelligent Technology and Its Applications (ISITIA), Surabaya, Indonesia, 21–22 July 2021. [Google Scholar]
- Alsiraji, H.A.; El-Shatshat, R. Comprehensive assessment of virtual synchronous machine based voltage source converter controllers. IET Gener. Transm. Distrib. 2017, 11, 1762–1769. [Google Scholar]
- Vargas, R.Z.; Lopes, J.C.; Colque, J.C.; Azcue, J.L.; Sousa, T. Energy Storage System Integration with Wind Generation for Primary Frequency Support in the Distribution Grid. Simpósio Bras. Sist. Elétricos-SBSE 2020. [Google Scholar] [CrossRef]





| Type of Battery | Unit Capacity Price CE (USD/kW·h) | Unit Power Price Cp (USD/kW) | O&M Cost Cf (USD)/(kW·h) | Charging Electricity Price Cc (USD/kW.h) | Conversion Efficiency (%) | Life Time Period (Year) |
|---|---|---|---|---|---|---|
| Lithium battery | 21,600 | 7270 | 1040 | 3.5 | 0.85 | 10 |
| Wind Speed | 6.2 m/s | 8.6 m/s | 11.2 m/s |
|---|---|---|---|
| With energy storage supports | 8% | 8% | 8% |
| Original strategy: secondary fall compensation | 6.7% | 5.6% | 13.9% |
| Optimization strategy: energy storage and compensation | 5.5% | 5.2% | 5.9% |
| Model | Parameter | Value |
|---|---|---|
| Robust economic model | Control variable (Tj) | 4 |
| Control variable (Kf) | 5 | |
| Economic evaluation index (J) | 0.131 | |
| Economic evaluation index (CLCC) (103 USD) | 66.30 | |
| Power (%) | 1.4 | |
| Optimal capacity allocation | Control variable (Tj) | 8 |
| Control variable (Kf) | 13 | |
| Economic evaluation index (J) | 0.098 | |
| Economic evaluation index (CLCC) (103) | 150 | |
| Power (%) | 3.7 | |
| Control variable | Control variable (Tj) | 12 |
| Control variable (Kf) | 20 | |
| Economic evaluation index (J) | 0.096 | |
| Economic evaluation index (CLCC) (103) | 23,200 | |
| Power (%) | 5.9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, W.; Liu, B.; Nazir, M.S.; Abdalla, A.N.; Mohamed, M.A.; Ding, Z.; Bhutta, M.S.; Gul, M. An Energy Storage Assessment: Using Frequency Modulation Approach to Capture Optimal Coordination. Sustainability 2022, 14, 8510. https://doi.org/10.3390/su14148510
Chen W, Liu B, Nazir MS, Abdalla AN, Mohamed MA, Ding Z, Bhutta MS, Gul M. An Energy Storage Assessment: Using Frequency Modulation Approach to Capture Optimal Coordination. Sustainability. 2022; 14(14):8510. https://doi.org/10.3390/su14148510
Chicago/Turabian StyleChen, Wan, Baolian Liu, Muhammad Shahzad Nazir, Ahmed N. Abdalla, Mohamed A. Mohamed, Zujun Ding, Muhammad Shoaib Bhutta, and Mehr Gul. 2022. "An Energy Storage Assessment: Using Frequency Modulation Approach to Capture Optimal Coordination" Sustainability 14, no. 14: 8510. https://doi.org/10.3390/su14148510
APA StyleChen, W., Liu, B., Nazir, M. S., Abdalla, A. N., Mohamed, M. A., Ding, Z., Bhutta, M. S., & Gul, M. (2022). An Energy Storage Assessment: Using Frequency Modulation Approach to Capture Optimal Coordination. Sustainability, 14(14), 8510. https://doi.org/10.3390/su14148510










