Investigation into Influence of Wall Roughness on the Hydraulic Characteristics of an Axial Flow Pump as Turbine
Abstract
:1. Introduction
2. Numerical Model and Methods
2.1. Governing Equations
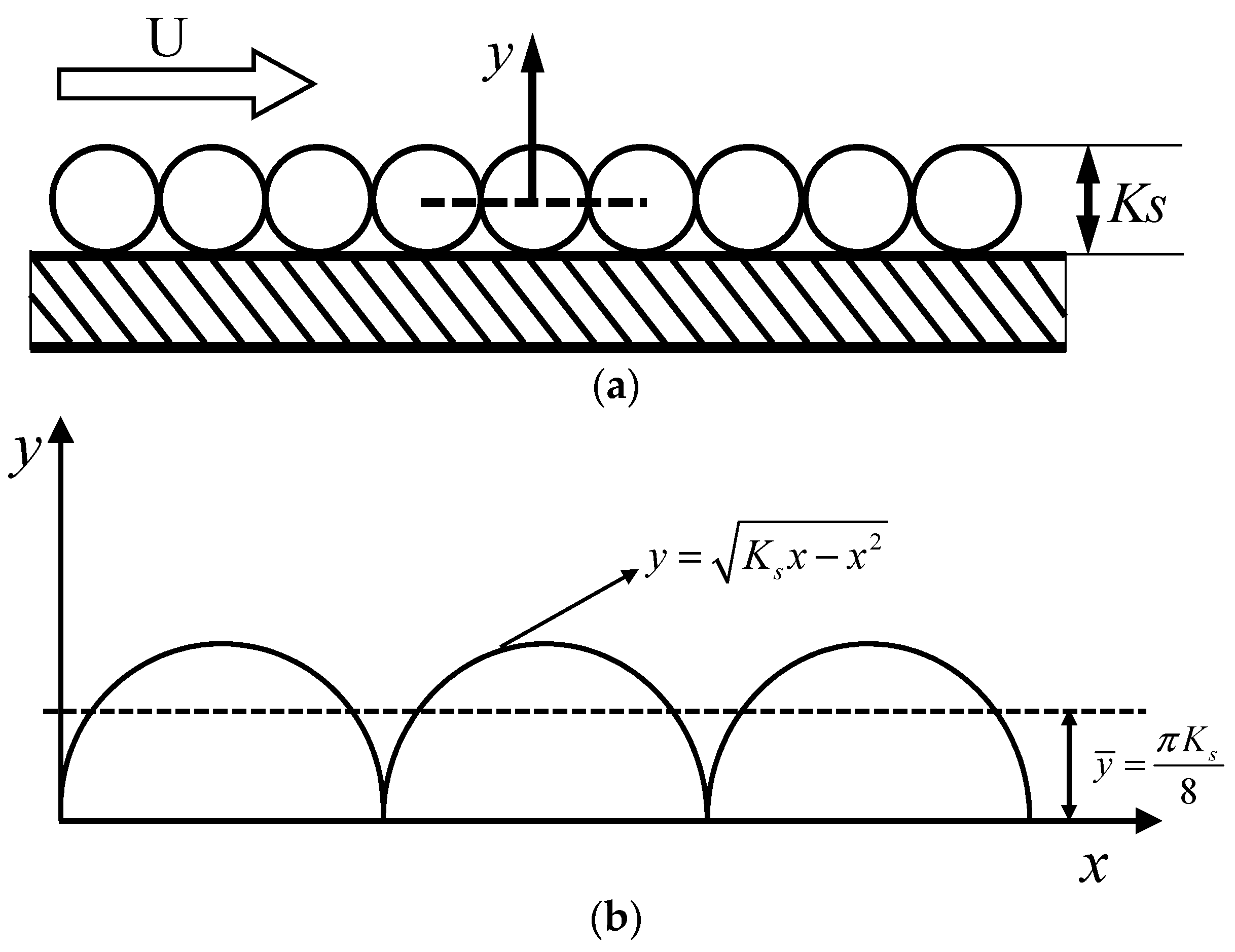
2.2. Equivalent Sand-Grain Roughness
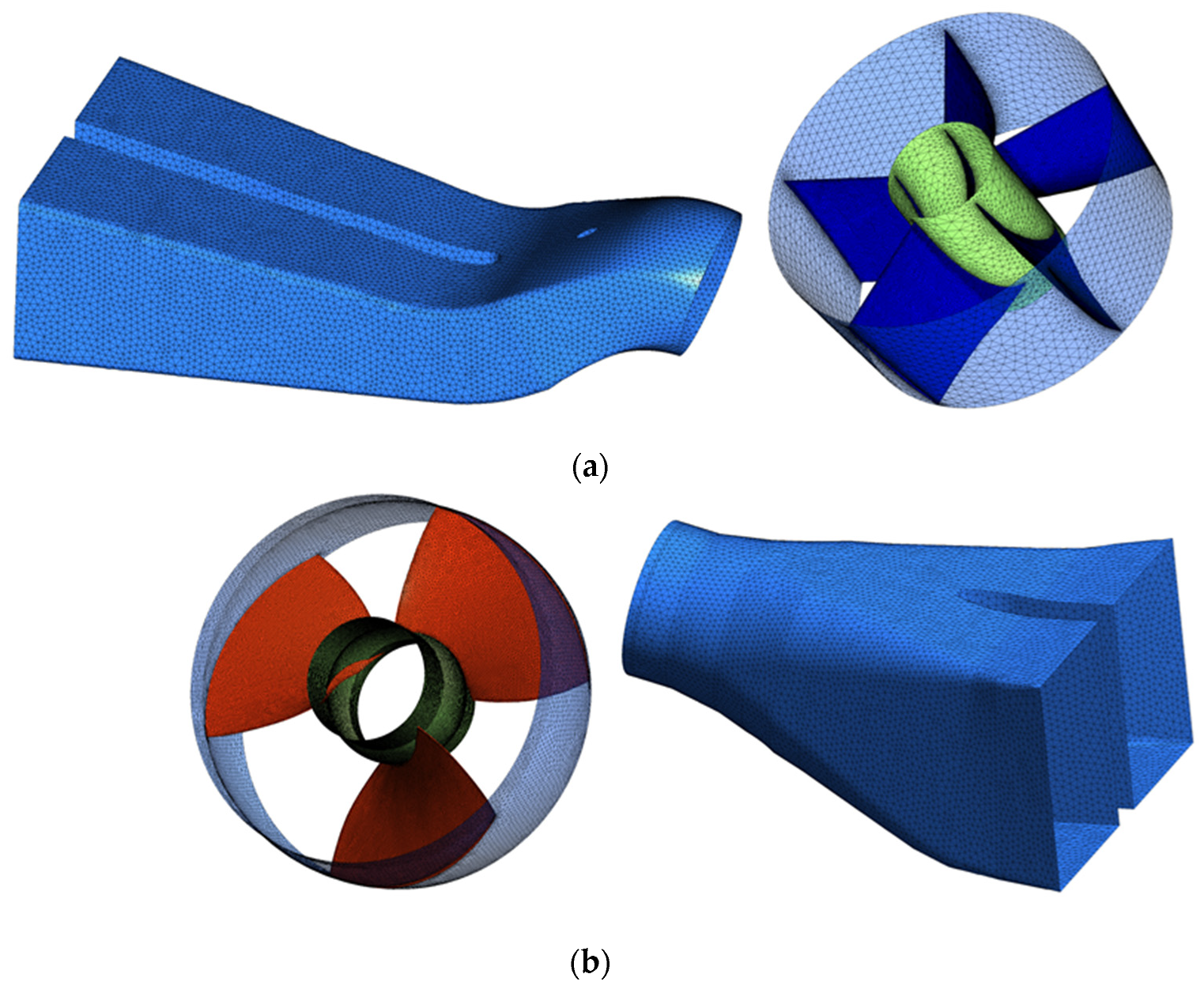
2.3. The Geometric Model Description
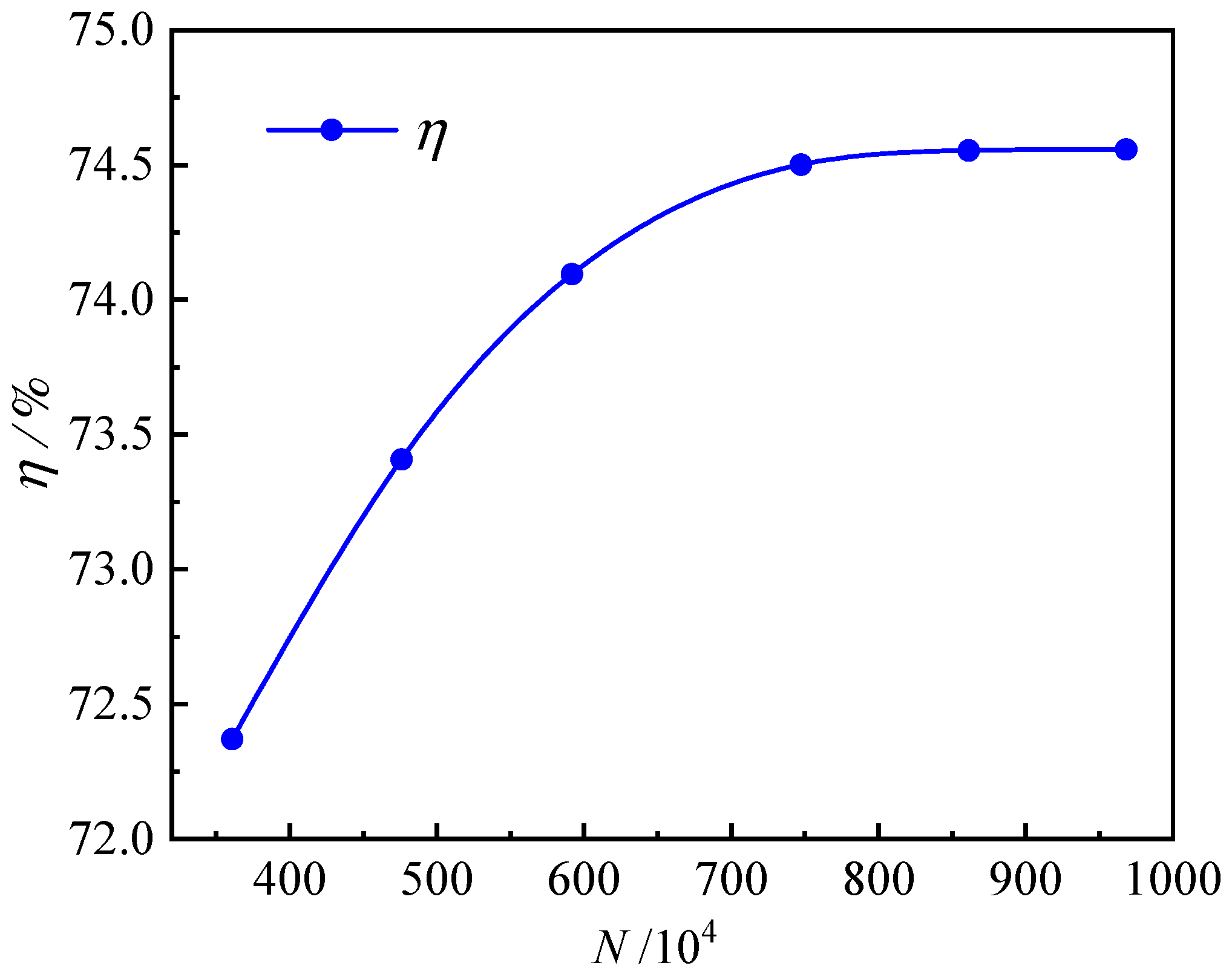
2.4. The Grid Generation Methodology
2.5. Boundary Conditions
3. Results and Discussion
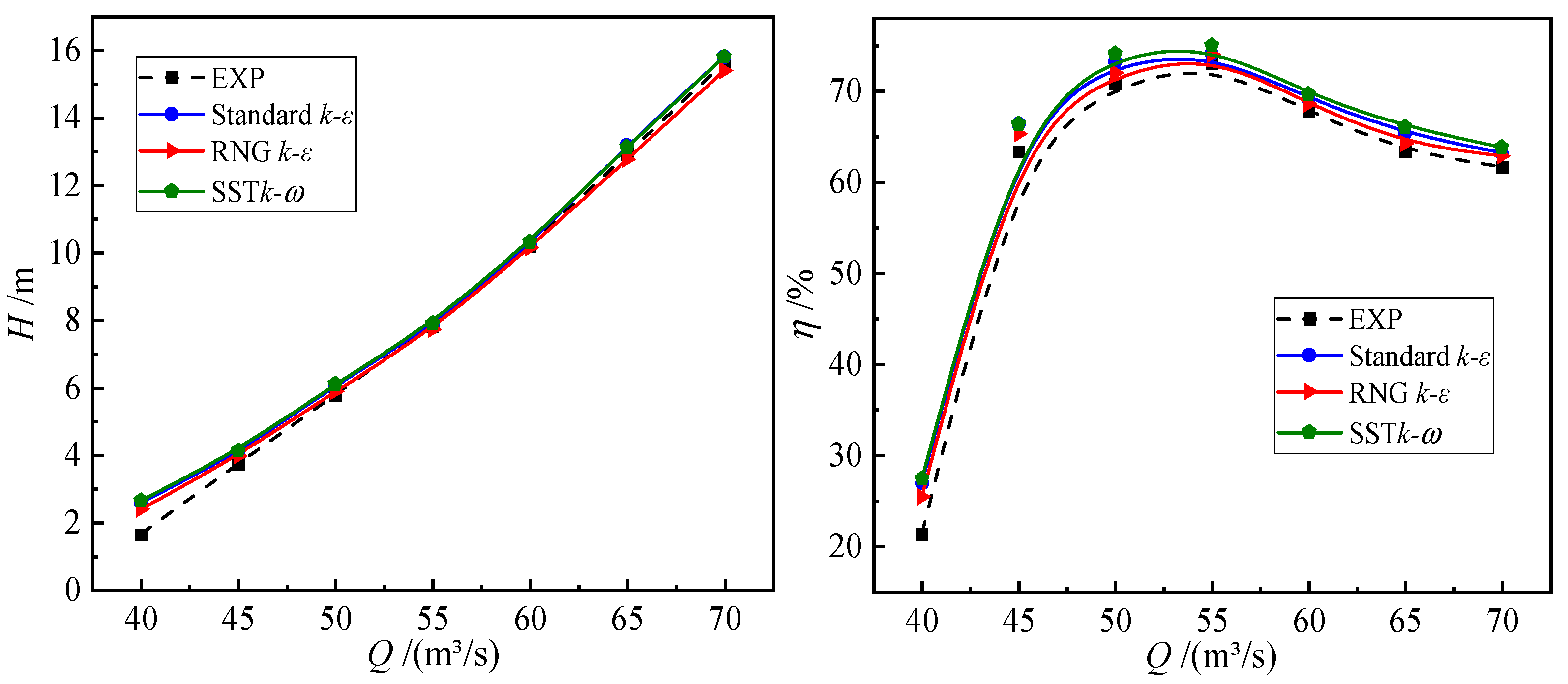
3.1. Experimental Validation of the Numerical Scheme
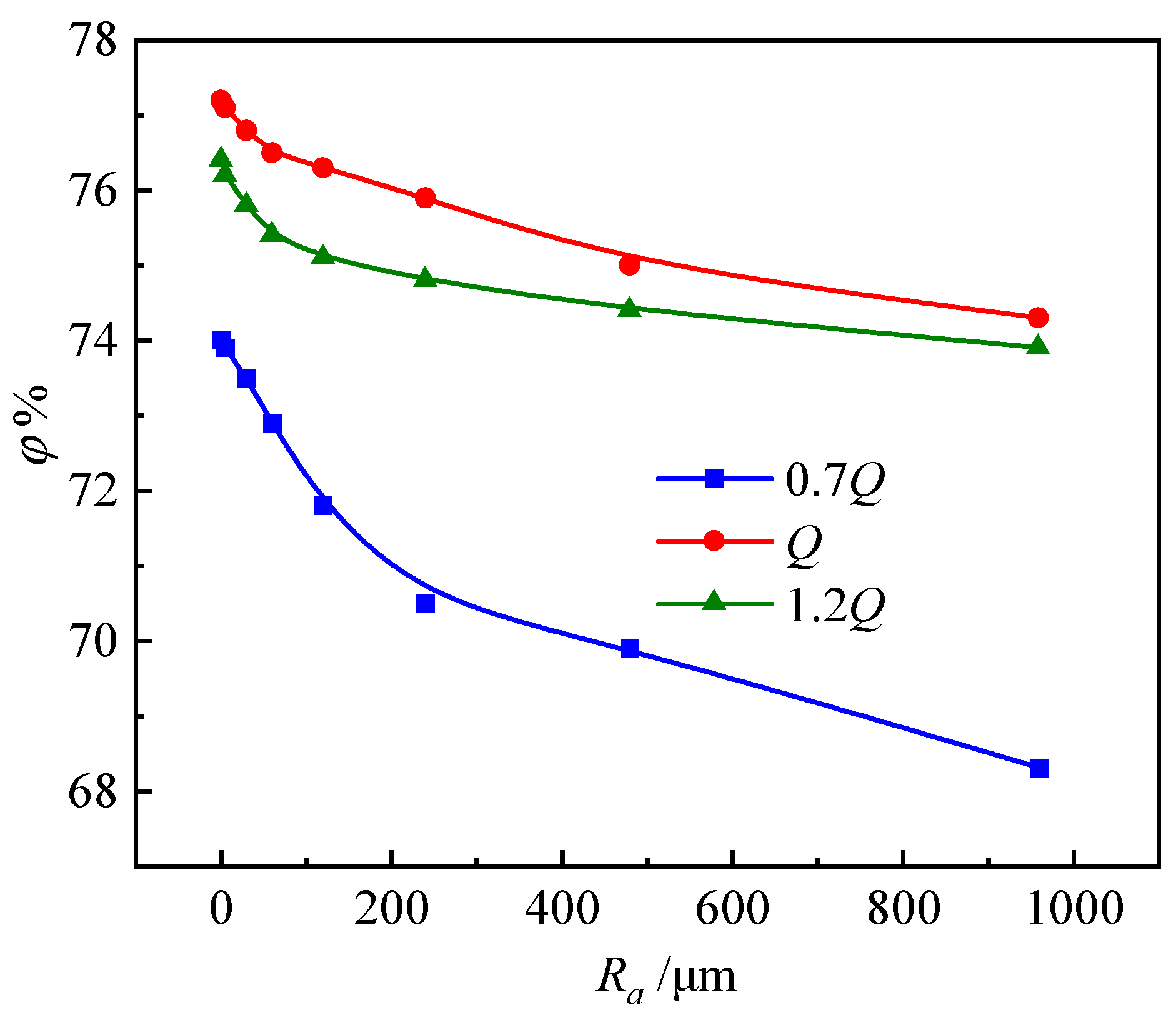
3.2. Effect of Wall Roughness on PAT Performance
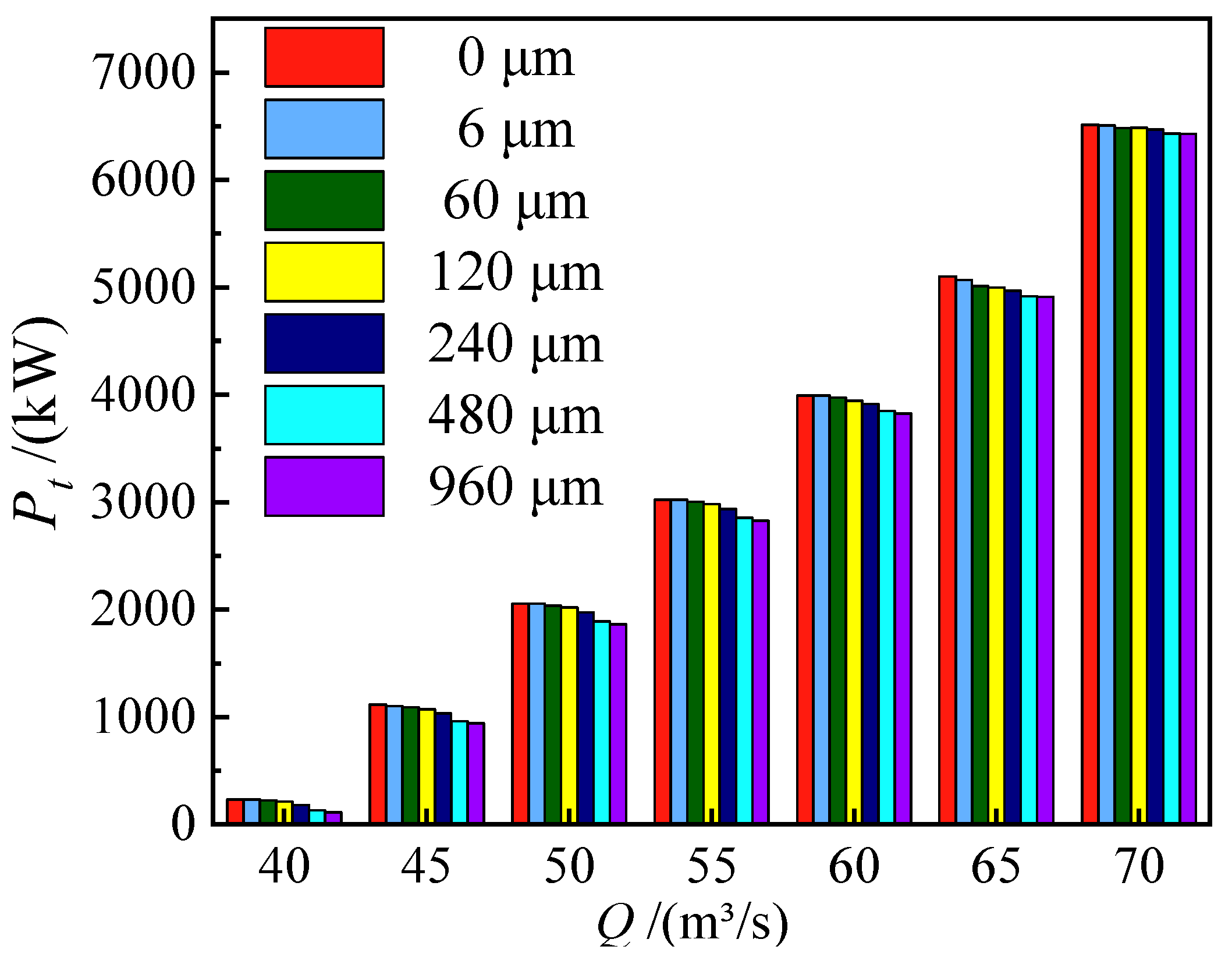
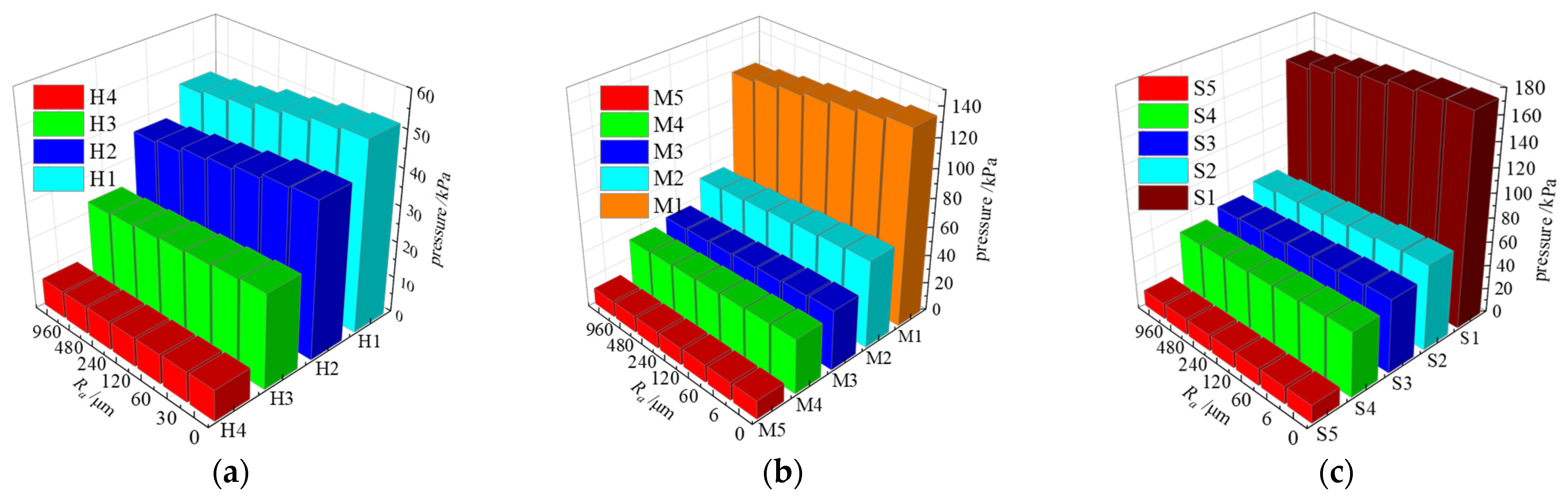
3.3. Effect of Wall Roughness on Shaft Power
3.4. Effect of Wall Roughness on Internal Flow
3.4.1. Wall Roughness Effect on Relative Flow Velocity over the Blade Pressure Surface
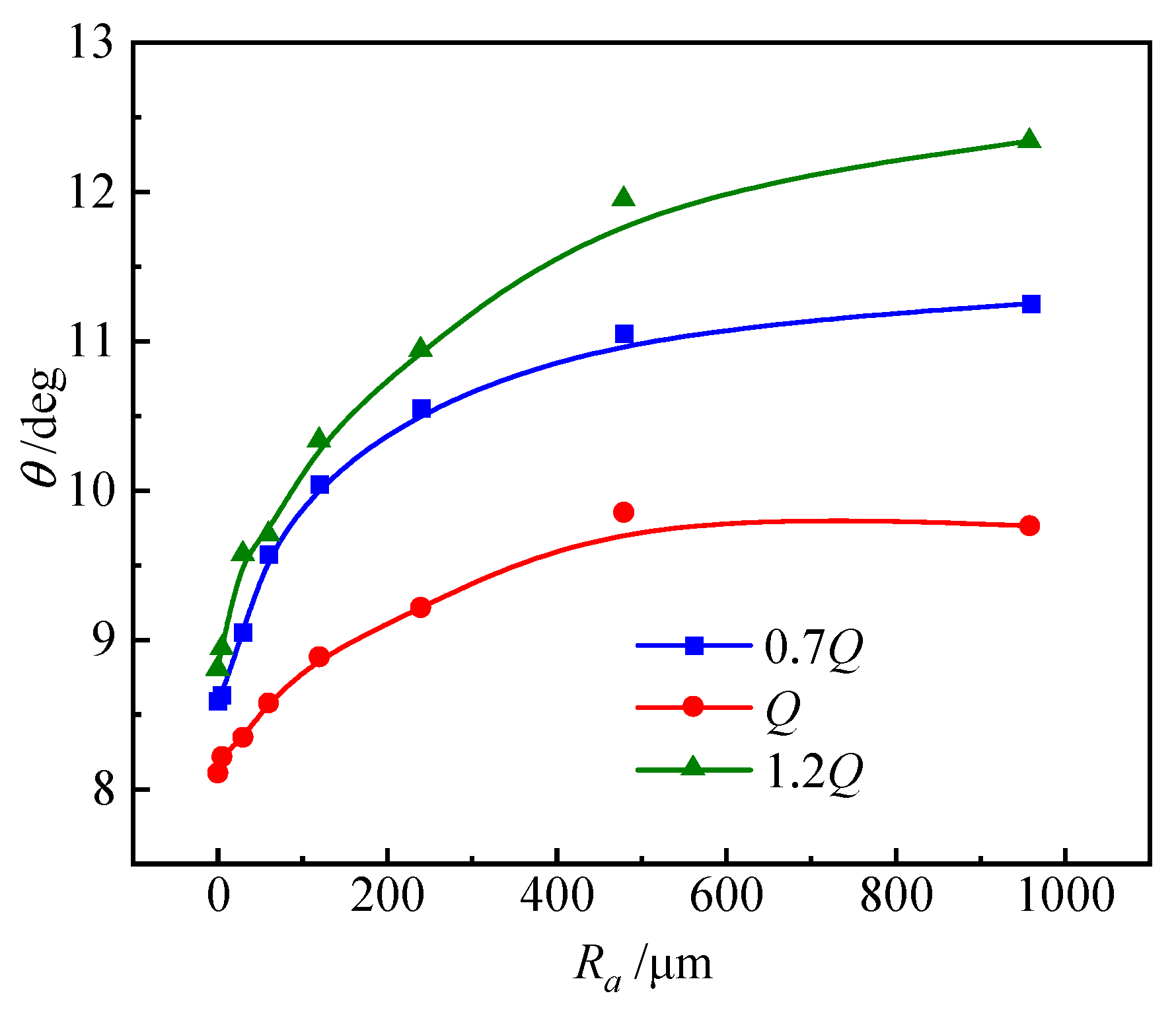
3.4.2. Effect of Wall Roughness on Impeller Outlet Flow
3.4.3. Effect of Wall Roughness on Streamline of Outlet Conduit
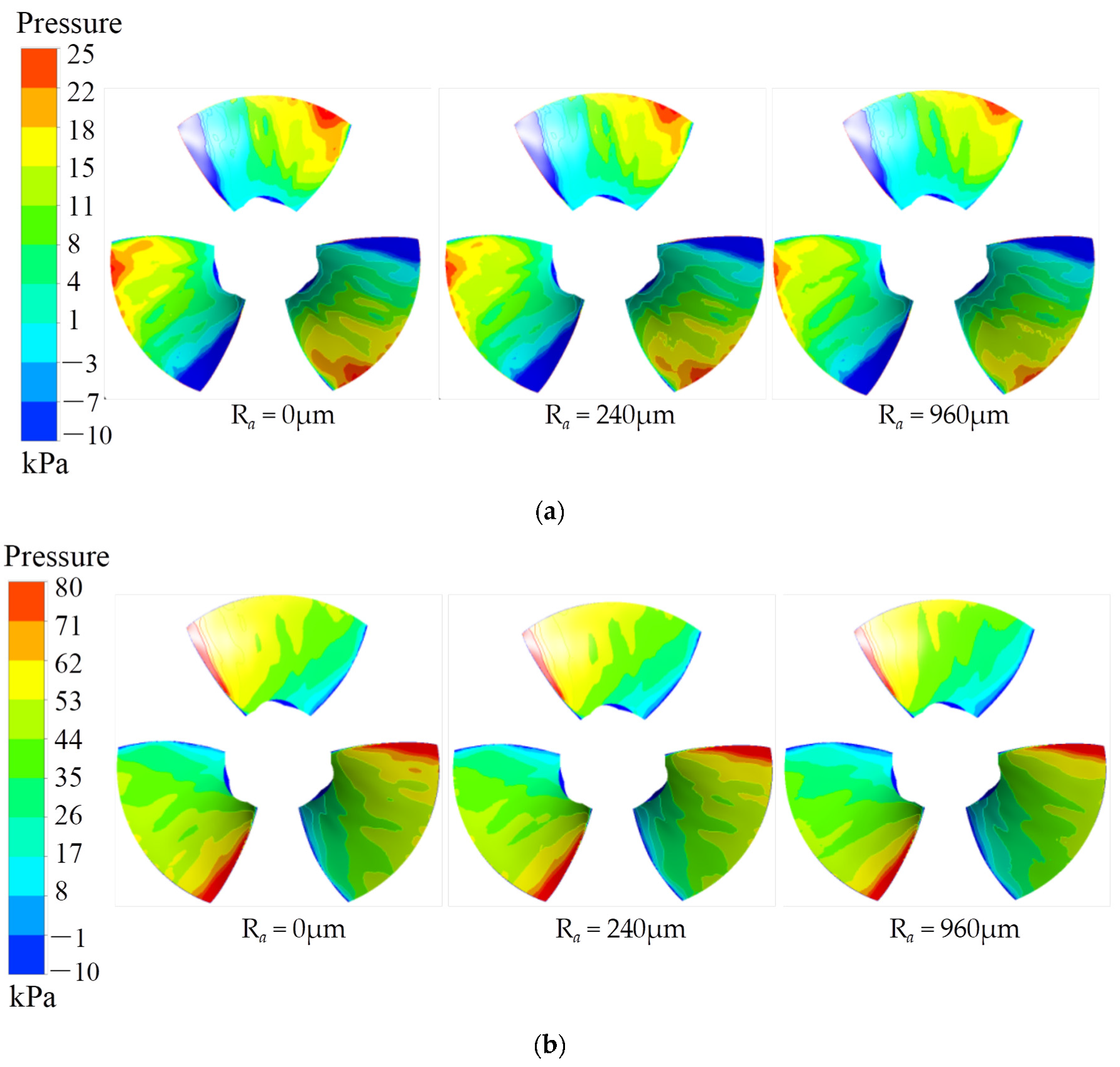
3.4.4. Effect of Wall Roughness on Pressure Distribution over the Blade Pressure Surface
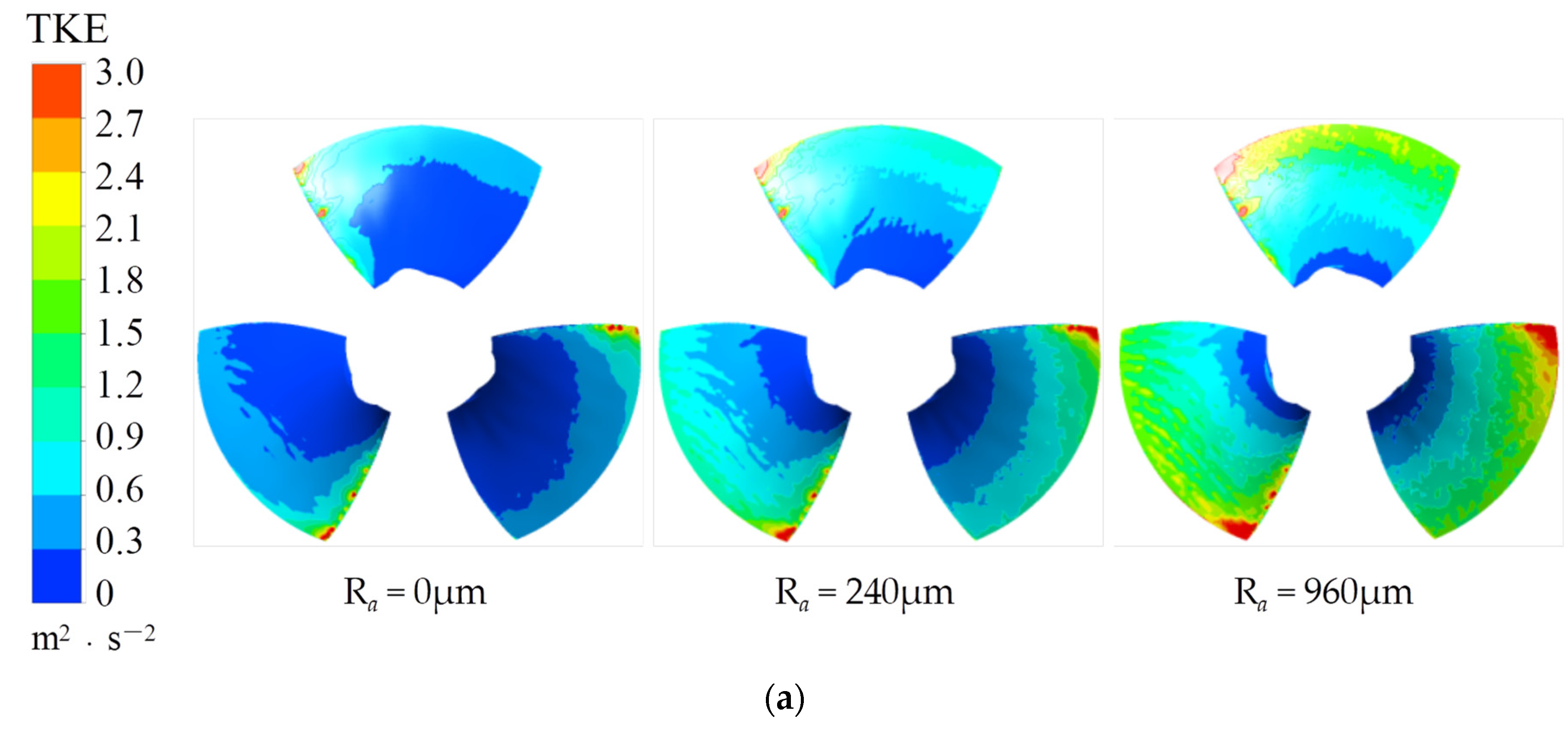
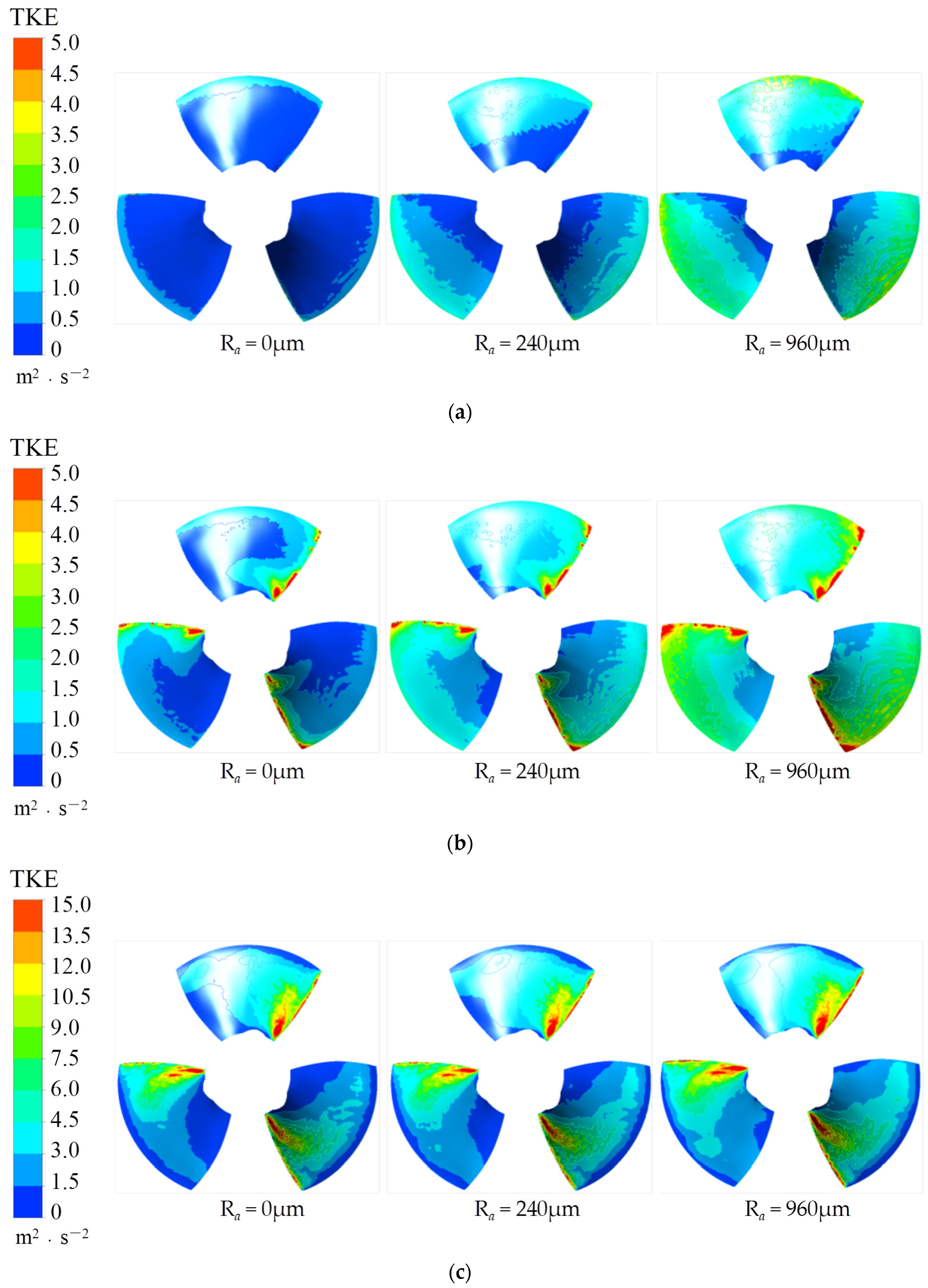
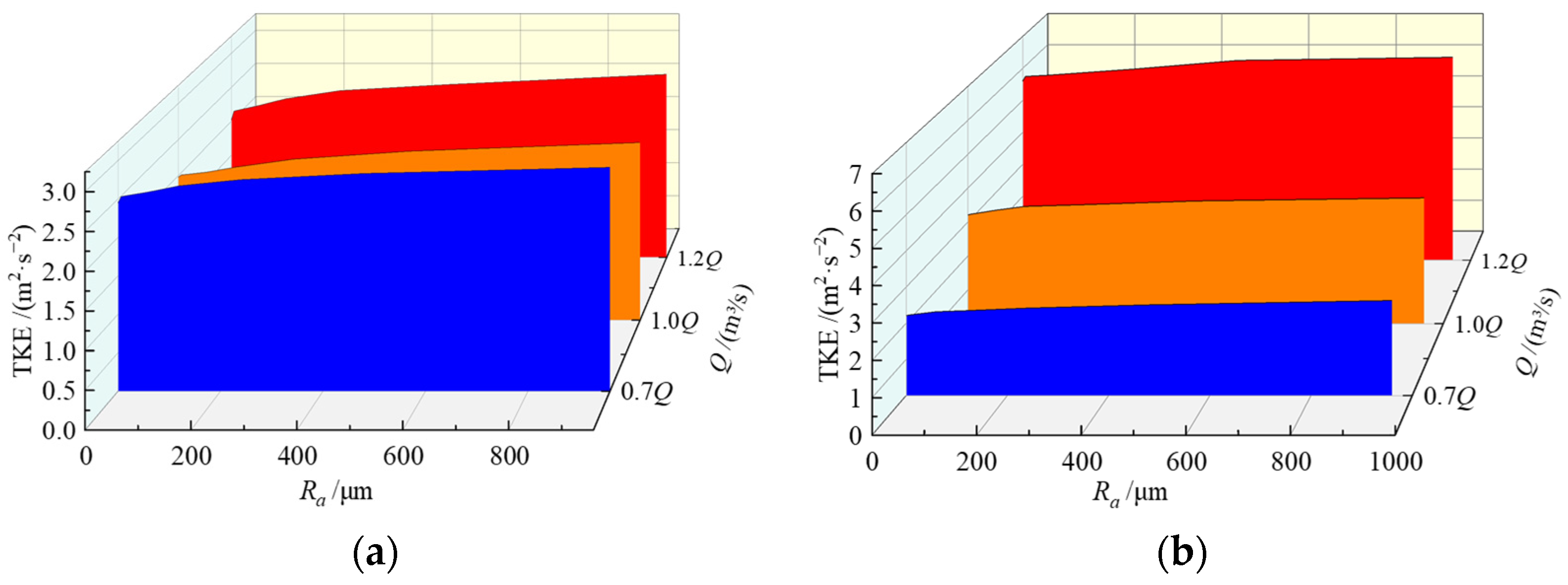
3.4.5. Effect of Wall Roughness on Turbulent Kinetic Energy
4. Conclusions
- (1)
- Under constant individual flow rates, the gradual deterioration of PAT performance (measured through parameters such as the hydraulic efficiency, head, and shaft power) is conspicuously associated with the increase in wall roughness depth. The later displays a minimum impact on PAT performance only under optimal flow conditions, while for off-design flow conditions, the larger the deviation from the best efficient point, the greater the impact on PAT performance characteristics.
- (2)
- Under turbine operating mode, the increase of wall roughness simultaneously brings about a messy non-uniform distribution of axial velocity and an increase of the velocity-weighted average swirl angle. Furthermore, streamlines within the discharge conduit reflect a disorderly flow pattern, eventually giving rise to backflow structures.
- (3)
- Ultimately, the wall roughness accumulation remarkably triggers the increase of energy losses. This is evidenced by the drop of static pressure on the blade pressure surface and the increase of TKE on the blade. The latter is particularly evident near the impeller shroud. Under the same roughness conditions, the TKE on the blade suction surface proves to be greater than that on the blade pressure surface.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yang, F.; Li, Z.; Hu, W.; Liu, C.; Jiang, D.; Liu, D.; Nasr, A. Analysis of flow loss characteristics of slanted axial-flow pump device based on entropy production theory. R. Soc. Open Sci. 2022, 9, 211208. [Google Scholar] [CrossRef] [PubMed]
- Kan, K.; Yang, Z.; Lyu, P.; Zheng, Y.; Shen, L. Numerical study of turbulent flow past a rotating axial-flow pump based on a level-set immersed boundary method. Renew. Energy 2021, 168, 960–971. [Google Scholar] [CrossRef]
- Shi, L.; Zhang, W.; Jiao, H.; Tang, F.; Wang, L.; Sun, D.; Shi, W. Numerical simulation and experimental study on the comparison of the hydraulic characteristics of an axial-flow pump and a full tubular pump. Renew. Energy 2020, 153, 1455–1464. [Google Scholar] [CrossRef]
- Shi, L.; Yuan, Y.; Jiao, H.; Tang, F.; Cheng, L.; Yang, F.; Jin, Y.; Zhu, J. Numerical investigation and experiment on pressure pulsation characteristics in a full tubular pump. Renew. Energy 2021, 163, 987–1000. [Google Scholar] [CrossRef]
- Liu, Y.; Tan, L. Tip clearance on pressure fluctuation intensity and vortex characteristic of a mixed flow pump as turbine at pump mode. Renew. Energy 2018, 129, 606–615. [Google Scholar] [CrossRef]
- Kan, K.; Zhang, Q.; Xu, Z.; Zheng, Y.; Gao, Q.; Shen, L. Energy loss mechanism due to tip leakage flow of axial flow pump as turbine under various operating conditions. Energy 2022, 255, 124532. [Google Scholar] [CrossRef]
- Yang, S.S.; Derakhshan, S.; Kong, F.Y. Theoretical, numerical and experimental prediction of pump as turbine performance. Renewable. Energy 2012, 48, 507–513. [Google Scholar] [CrossRef]
- Jain, S.V.; Patel, R.N. Investigations on pump running in turbine mode: A review of the state-of-the-art. Renew. Sustain. Energy Rev. 2014, 30, 841–868. [Google Scholar] [CrossRef]
- Aldaş, K.; Yapıcı, R. Investigation of effects of scale and surface roughness on efficiency of water jet pumps using CFD. Eng. Appl. Comput. Fluid Mech. 2014, 8, 14–25. [Google Scholar] [CrossRef] [Green Version]
- Han, Y.; Tan, L. Influence of rotating speed on tip leakage vortex in a mixed flow pump as turbine at pump mode. Renew. Energy 2020, 162, 144–150. [Google Scholar] [CrossRef]
- Lin, T.; Zhu, Z.; Li, X.; Li, J.; Lin, Y. Theoretical, experimental, and numerical methods to predict the best efficiency point of centrifugal pump as turbine. Renew. Energy 2021, 168, 31–44. [Google Scholar] [CrossRef]
- Sun-Sheng, Y.; Fan-Yu, K.; Jian-Hui, F.; Ling, X. Numerical research on effects of splitter blades to the influence of pump as turbine. Int. J. Rotating Mach. 2012, 2012, 123093. [Google Scholar] [CrossRef] [Green Version]
- Capurso, T.; Bergamini, L.; Camporeale, S.M.; Fortunato, B.; Torresi, M. CFD analysis of the performance of a novel impeller for a double suction centrifugal pump working as a turbine. In Proceedings of the 13th European Conference on Turbomachinery Fluid dynamics & Thermodynamics. European Turbomachinery Society, Lausanne, Switzerland, 24–28 April 2019. [Google Scholar]
- Derakhshan, S.; Mohammadi, B.; Nourbakhsh, A. Efficiency improvement of centrifugal reverse pumps. J. Fluids Eng. 2009, 131, 021103. [Google Scholar] [CrossRef]
- Morabito, A.; Vagnoni, E.; Di Matteo, M.; Hendrick, P. Numerical investigation on the volute cutwater for pumps running in turbine mode. Renew. Energy 2021, 175, 807–824. [Google Scholar] [CrossRef]
- Gülich, J.F. Effect of Reynolds number and surface roughness on the efficiency of centrifugal pumps. J. Fluids Eng. 2003, 125, 670–679. [Google Scholar] [CrossRef]
- Bai, T.; Liu, J.; Zhang, W.; Zou, Z. Effect of surface roughness on the aerodynamic performance of turbine blade cascade. Propuls. Power Res. 2014, 3, 82–89. [Google Scholar] [CrossRef] [Green Version]
- Bellary, S.A.I.; Samad, A. Exit blade angle and roughness effect on centrifugal pump performance. In Proceedings of the Gas Turbine India Conference, Bangalore, Karnataka, 5–6 December 2013; American Society of Mechanical Engineers: New York, NY, USA; pp. 1–10. [Google Scholar]
- Deshmukh, D.; Samad, A. CFD-based analysis for finding critical wall roughness on centrifugal pump at design and off-design conditions. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 1–18. [Google Scholar] [CrossRef]
- Juckelandt, K.; Bleeck, S.; Wurm, F.H. Analysis of losses in centrifugal pumps with low specific speed with smooth and rough walls. In Proceedings of the 11th European Conference on Turbomachinery Fluid dynamics & Thermodynamics, European Turbomachinery society, Madrid, Spain, 23–27 March 2015. [Google Scholar]
- He, X.; Jiao, W.; Wang, C.; Cao, W. Influence of surface roughness on the pump performance based on Computational Fluid Dynamics. IEEE Access 2019, 7, 105331–105341. [Google Scholar] [CrossRef]
- Limbach, P.; Skoda, R. Numerical and experimental analysis of cavitating flow in a low specific speed centrifugal pump with different surface roughness. J. Fluids Eng. 2017, 139, 101201. [Google Scholar] [CrossRef]
- Lim, S.E.; Sohn, C.H. CFD analysis of performance change in accordance with inner surface roughness of a double-entry centrifugal pump. J. Mech. Sci. Technol. 2018, 32, 697–702. [Google Scholar] [CrossRef]
- Kan, K.; Xu, Z.; Chen, H.; Xu, H.; Zheng, Y.; Zhou, D.; Muhirwa, A.; Maxime, B. Energy loss mechanisms of transition from pump mode to turbine mode of an axial-flow pump under bidirectional conditions. Energy 2022, 257, 124630. [Google Scholar] [CrossRef]
- Kan, K.; Chen, H.; Zheng, Y.; Zhou, D.; Binama, M.; Dai, J. Transient characteristics during power-off process in a shaft extension tubular pump by using a suitable numerical model. Renew. Energy 2021, 164, 109–121. [Google Scholar] [CrossRef]
- He, X.; Zhang, Y.; Wang, C.; Zhang, C.; Cheng, L.; Chen, K.; Hu, B. Influence of critical wall roughness on the performance of double-channel sewage pump. Energies 2020, 13, 464. [Google Scholar] [CrossRef] [Green Version]
- Shockling, M.A.; Allen, J.J.; Smits, A.J. Roughness effects in turbulent pipe flow. J. Fluid Mech. 2006, 564, 267–285. [Google Scholar] [CrossRef]
- Adams, T.; Grant, C.; Watson, H. A simple algorithm to relate measured surface roughness to equivalent sand-grain roughness. Int. J. Mech. Eng. Mechatron. 2012, 1, 66–71. [Google Scholar] [CrossRef] [Green Version]
- Ji, L.; Li, W.; Shi, W.; Tian, F.; Agarwal, R. Diagnosis of internal energy characteristics of mixed-flow pump within stall region based on entropy production analysis model. Int. Commun. Heat Mass Transf. 2020, 117, 104784. [Google Scholar] [CrossRef]
- Sha, L.; Yang, S.; Kong, X.; Sun, H.; Pei, L. Influence of inlet flow field uniformity on the performance of the nuclear main pump. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1081, 012024. [Google Scholar] [CrossRef]
- Skrzypacz, J.; Bieganowski, M. The influence of micro grooves on the parameters of thecentrifugal pμmp impeller. Int. J. Mech. Sci. 2018, 144, 827–835. [Google Scholar] [CrossRef]
- Li, W.; Ji, L.; Li, E.; Shi, W.; Agarwal, R.; Zhou, L. Numerical investigation of energy loss mechanism of mixed-flow pump under stall condition. Renew. Energy 2021, 167, 740–760. [Google Scholar] [CrossRef]






| Parameter | Value |
|---|---|
| Impeller nominal diameter D [m] | 3.25 |
| Design head H [m] | 2.6 |
| Design flow rate Q [m3/s] | 45.5 |
| Design power P [kW] | 1319 |
| Design efficiency η [%] | 88 |
| Rotational speed n [r/min] | 122 |
| Hydraulic Circuit Components | Grid Size [104] |
|---|---|
| Inlet conduit | 79 |
| Guide vane | 241 |
| Impeller | 334 |
| Outlet conduit | 94 |
| An all-embracing grid size | 748 |
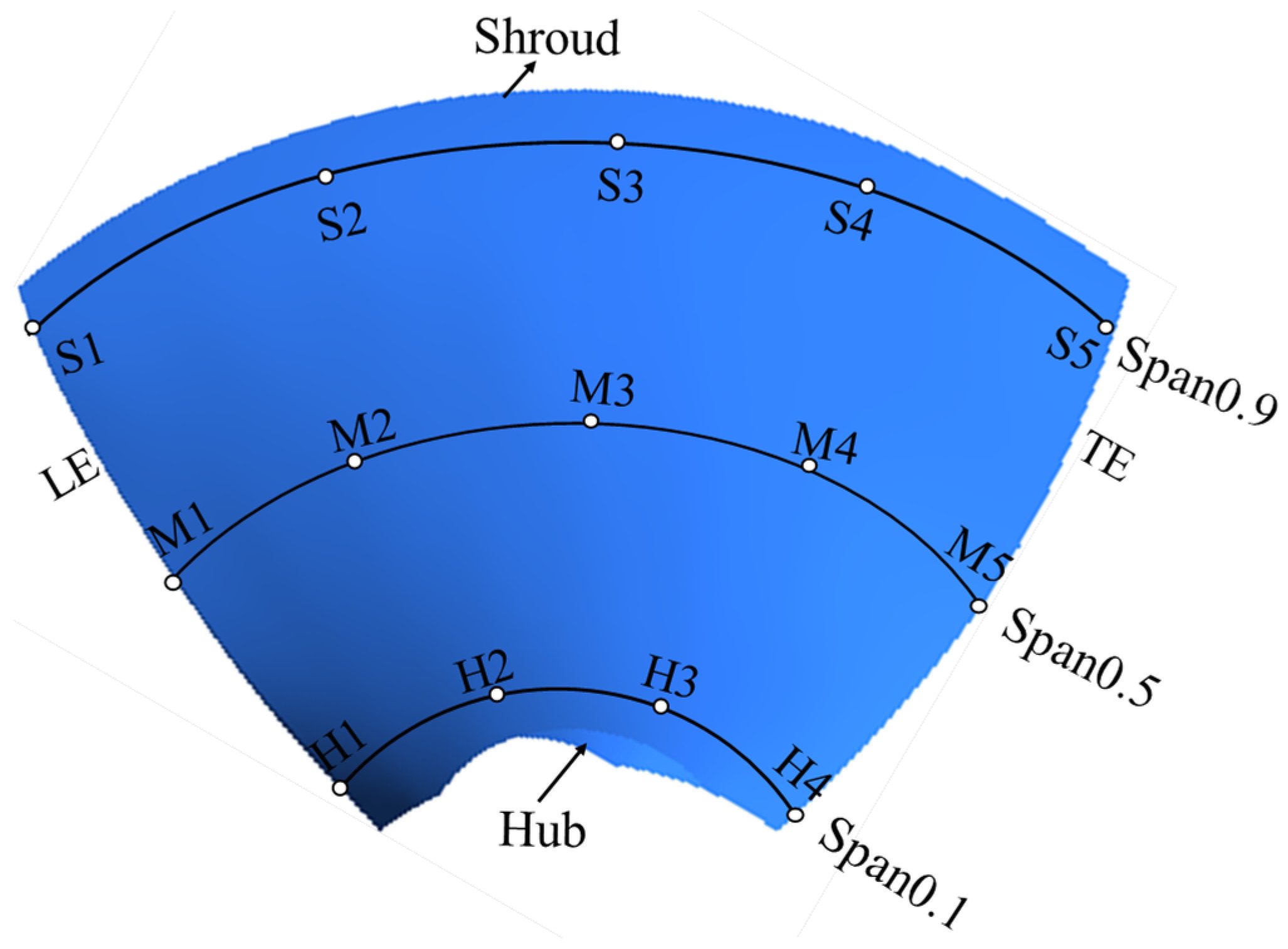
| Ra (μm) | H3 | M4 | S5 | |||
|---|---|---|---|---|---|---|
| Vr (m/s) | Relative Deviation (%) | Vr (m/s) | Relative Deviation (%) | Vr (m/s) | Relative Deviation (%) | |
| 0 | 8.13 | 14.04 | 22.62 | |||
| 6 | 8.06 | 0.87% | 13.73 | 2.23% | 22.42 | 0.89% |
| 60 | 7.91 | 2.75% | 13.34 | 4.98% | 21.81 | 3.58% |
| 120 | 7.55 | 7.15% | 12.90 | 8.13% | 21.16 | 6.45% |
| 240 | 7.31 | 10.14% | 12.47 | 11.21% | 20.36 | 10.01% |
| 480 | 7.22 | 11.23% | 12.16 | 13.41% | 19.83 | 12.34% |
| 960 | 7.13 | 12.30% | 11.89 | 15.31% | 19.42 | 14.12% |
| Span0.1 | Span0.5 | Span0.9 | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Point | H1 | H2 | H3 | H4 | M1 | M2 | M3 | M4 | M5 | S1 | S2 | S3 | S4 | S5 |
| Deviation (%) | 11 | 8 | 14 | 13 | 11 | 5.2 | 3.6 | 3.2 | 1.1 | 11 | 14 | 13 | 11 | 15 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kan, K.; Zhang, Q.; Zheng, Y.; Xu, H.; Xu, Z.; Zhai, J.; Muhirwa, A. Investigation into Influence of Wall Roughness on the Hydraulic Characteristics of an Axial Flow Pump as Turbine. Sustainability 2022, 14, 8459. https://doi.org/10.3390/su14148459
Kan K, Zhang Q, Zheng Y, Xu H, Xu Z, Zhai J, Muhirwa A. Investigation into Influence of Wall Roughness on the Hydraulic Characteristics of an Axial Flow Pump as Turbine. Sustainability. 2022; 14(14):8459. https://doi.org/10.3390/su14148459
Chicago/Turabian StyleKan, Kan, Qingying Zhang, Yuan Zheng, Hui Xu, Zhe Xu, Jianwei Zhai, and Alexis Muhirwa. 2022. "Investigation into Influence of Wall Roughness on the Hydraulic Characteristics of an Axial Flow Pump as Turbine" Sustainability 14, no. 14: 8459. https://doi.org/10.3390/su14148459
APA StyleKan, K., Zhang, Q., Zheng, Y., Xu, H., Xu, Z., Zhai, J., & Muhirwa, A. (2022). Investigation into Influence of Wall Roughness on the Hydraulic Characteristics of an Axial Flow Pump as Turbine. Sustainability, 14(14), 8459. https://doi.org/10.3390/su14148459








