Performance Analysis of a New Electricity and Freshwater Production System Based on an Integrated Gasification Combined Cycle and Multi-Effect Desalination
Abstract
1. Introduction
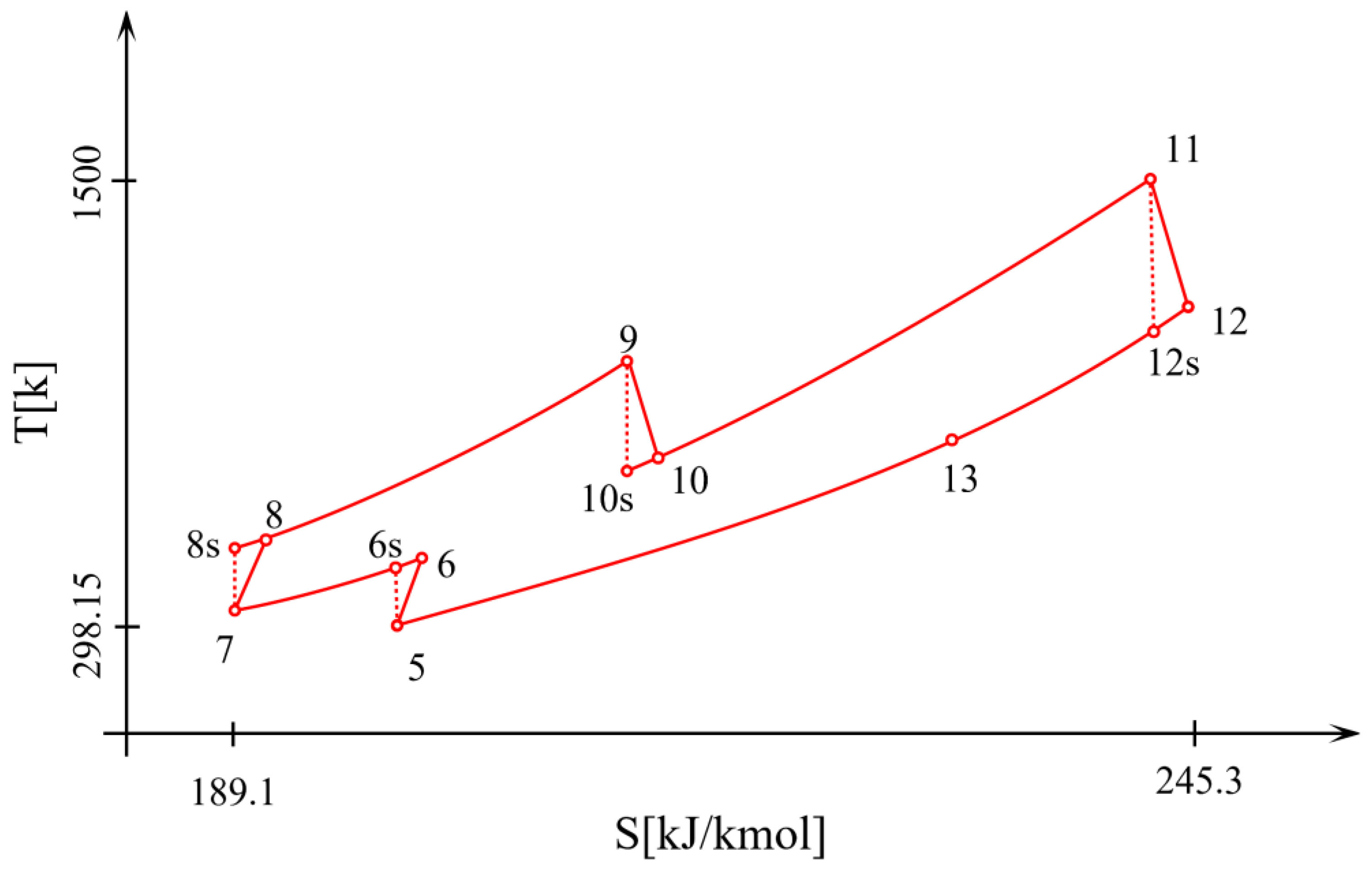
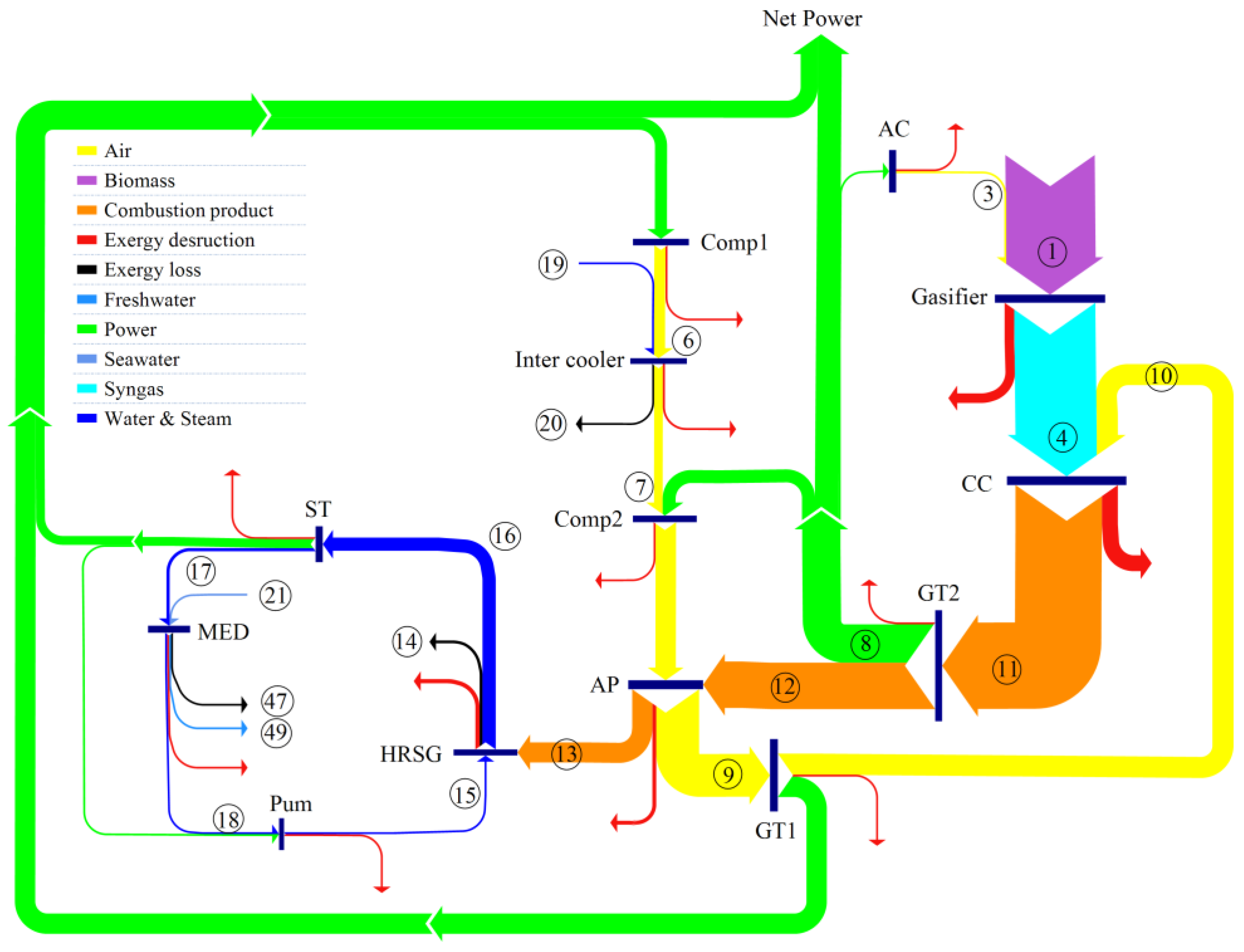
2. System Description
3. Modeling and Assumptions
3.1. Thermodynamic Assumptions
- The operation of each process in the cycle is considered to be at steady state.
- Changes in the potential and kinetic energy rates are ignored; thus, only physical and chemical exergies are considered.
- Pressure drops in the steam Rankine cycle are neglected, and pressure loss of the combustion chamber is taken to be 5%.
- The input air composition is 79% N2 and 21% O2.
- Also, the reference environment properties T0 and P0 are taken to be 298.15 K and 1.013 bra, respectively.
- The temperature differences for the flows in all effects of the MED are equal.
- The spray of seawater in all effects of the MED occurs in an equal flow rate.
3.2. Thermodynamic Modeling
3.2.1. Gasifier
3.2.2. Combustion Chamber
3.2.3. Heat Recovery Steam Generator (HRSG)
3.2.4. MED Desalination Unit
3.3. Exergoeconomic Analysis
3.4. Main Performance Criteria
4. Results and Discussion
4.1. Model Validation
4.2. Main Operating Results
4.3. Parametric Analysis
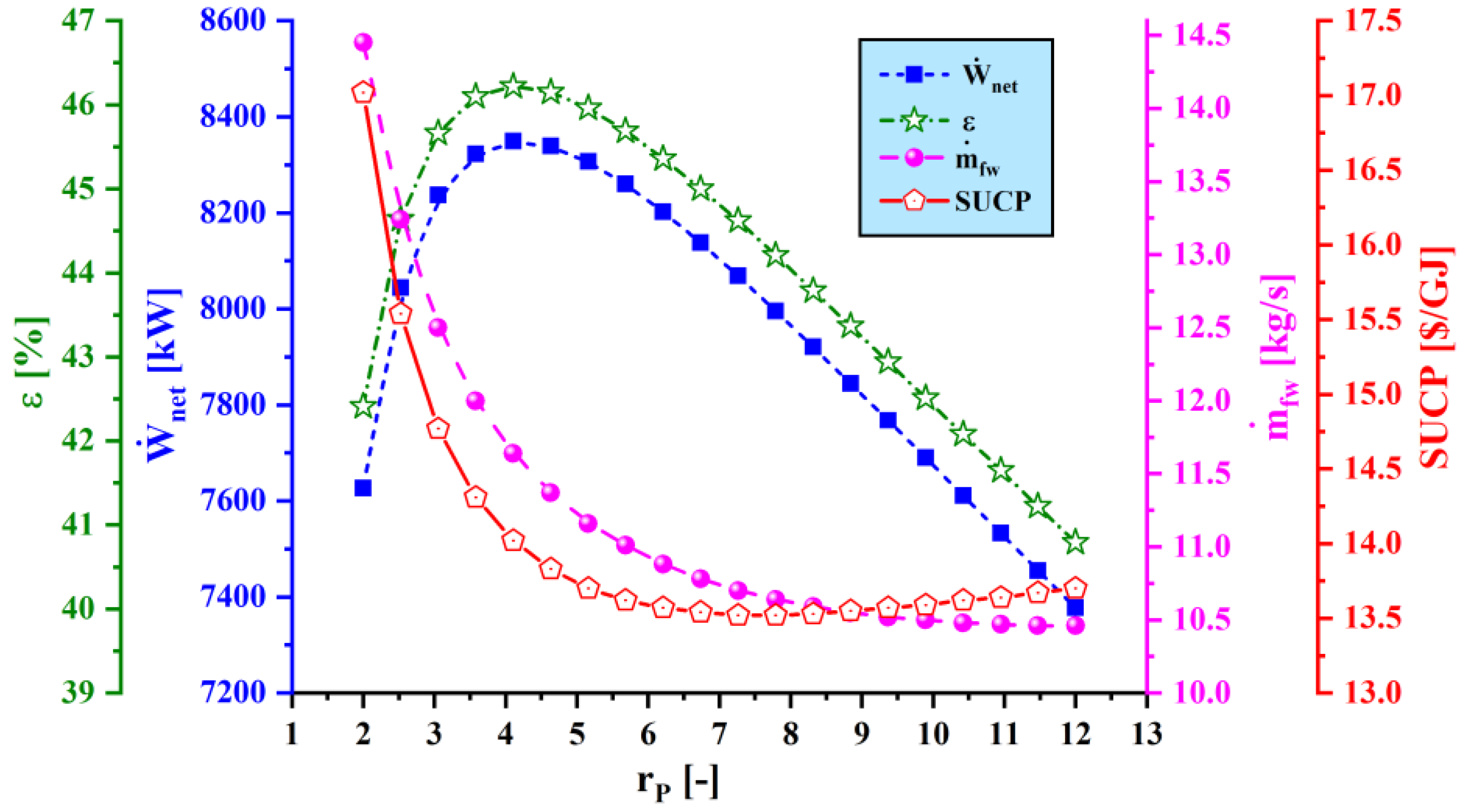
4.3.1. Effect of Compressor Pressure Ratio on System Performance
4.3.2. Effect of Gasification Temperature on System Performance
4.3.3. Effect of Combustion Chamber Temperature on System Performance
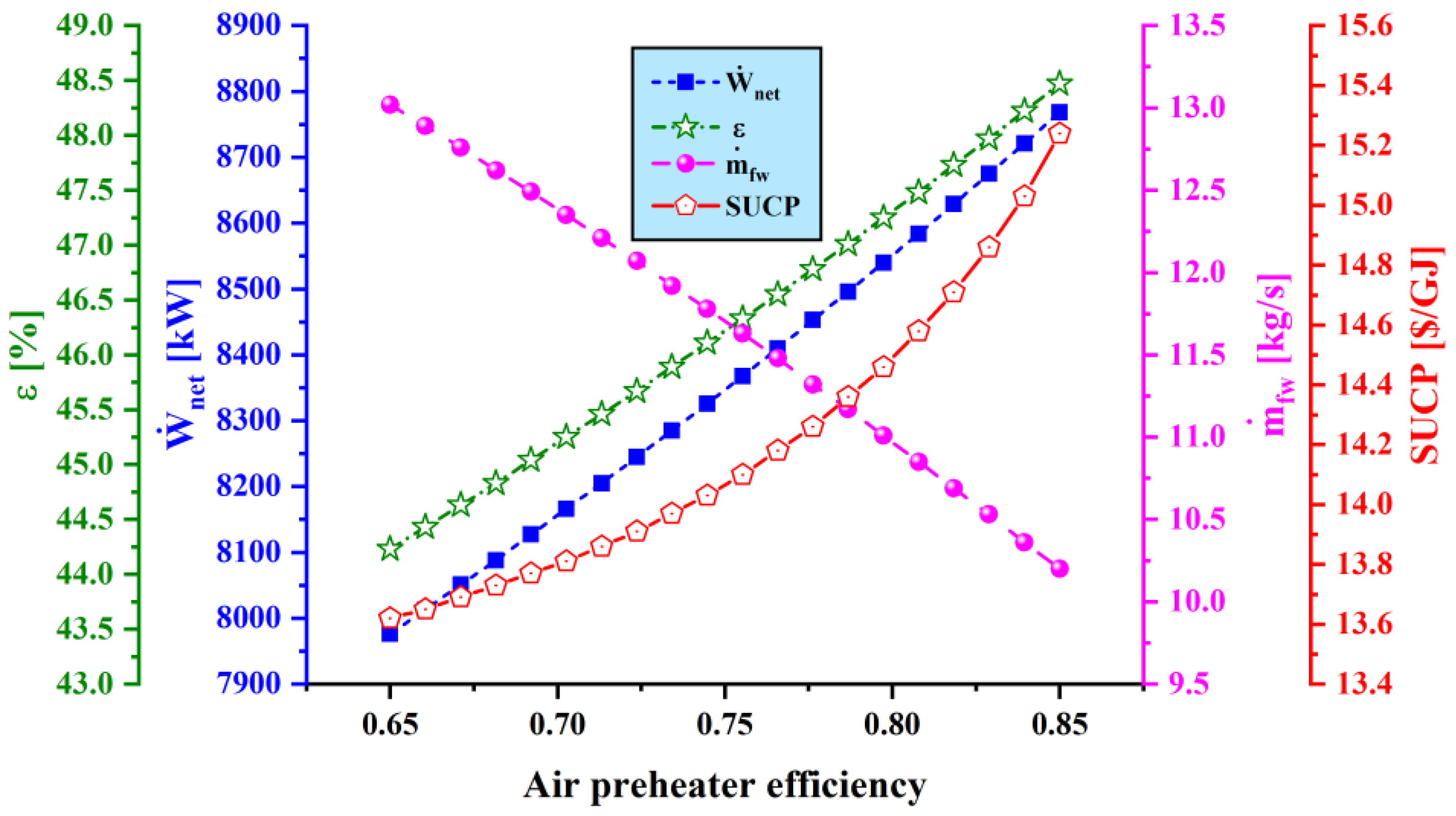
4.3.4. Effect of Air Preheater Effectiveness on System Performance
4.3.5. Effect of Superheating Temperature Difference on System Performance
4.3.6. Effect of Feedwater Temperature () on Water Production Capacity
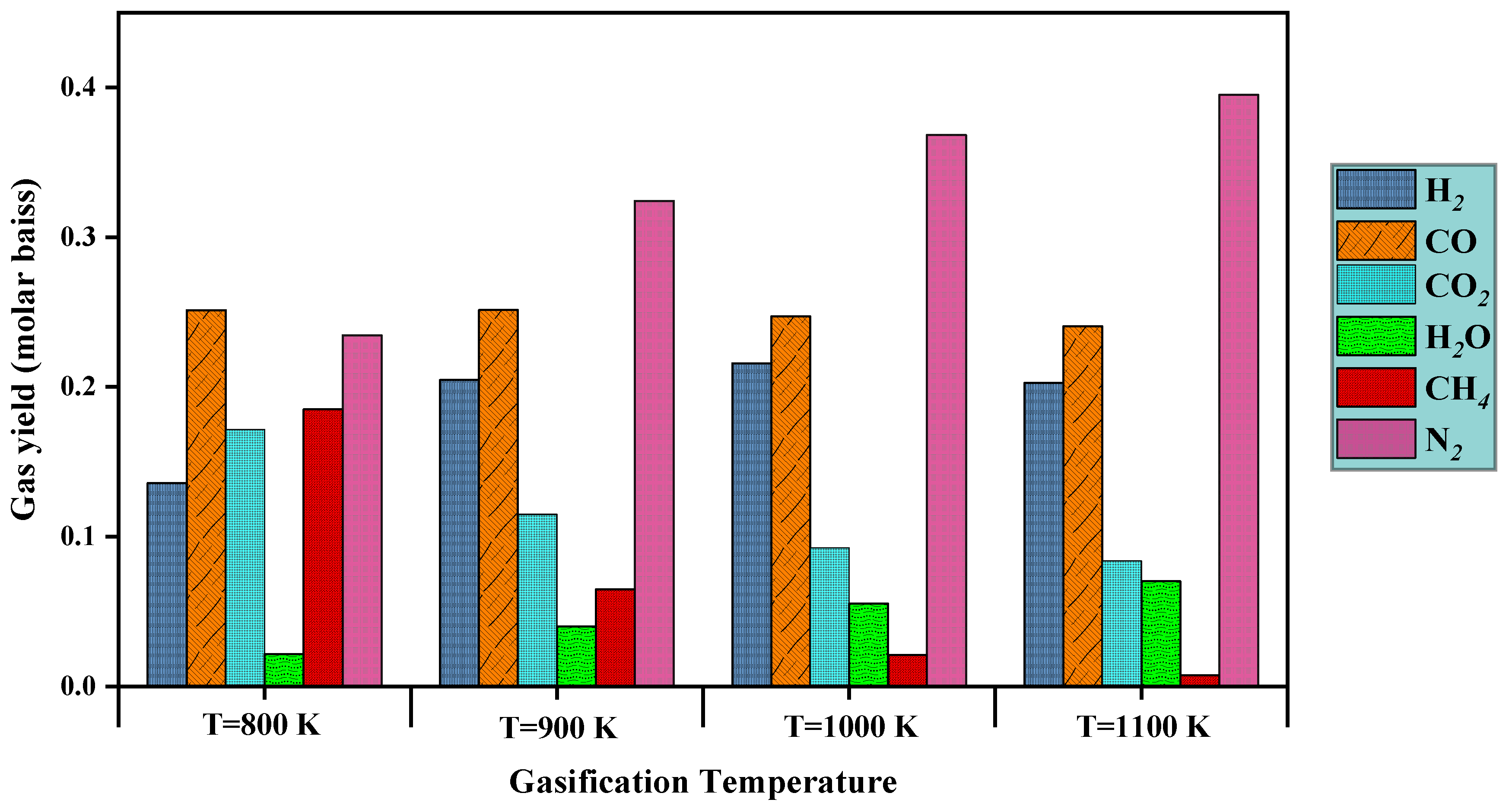
4.3.7. Effect of Gasification Temperature () on Gas Yield
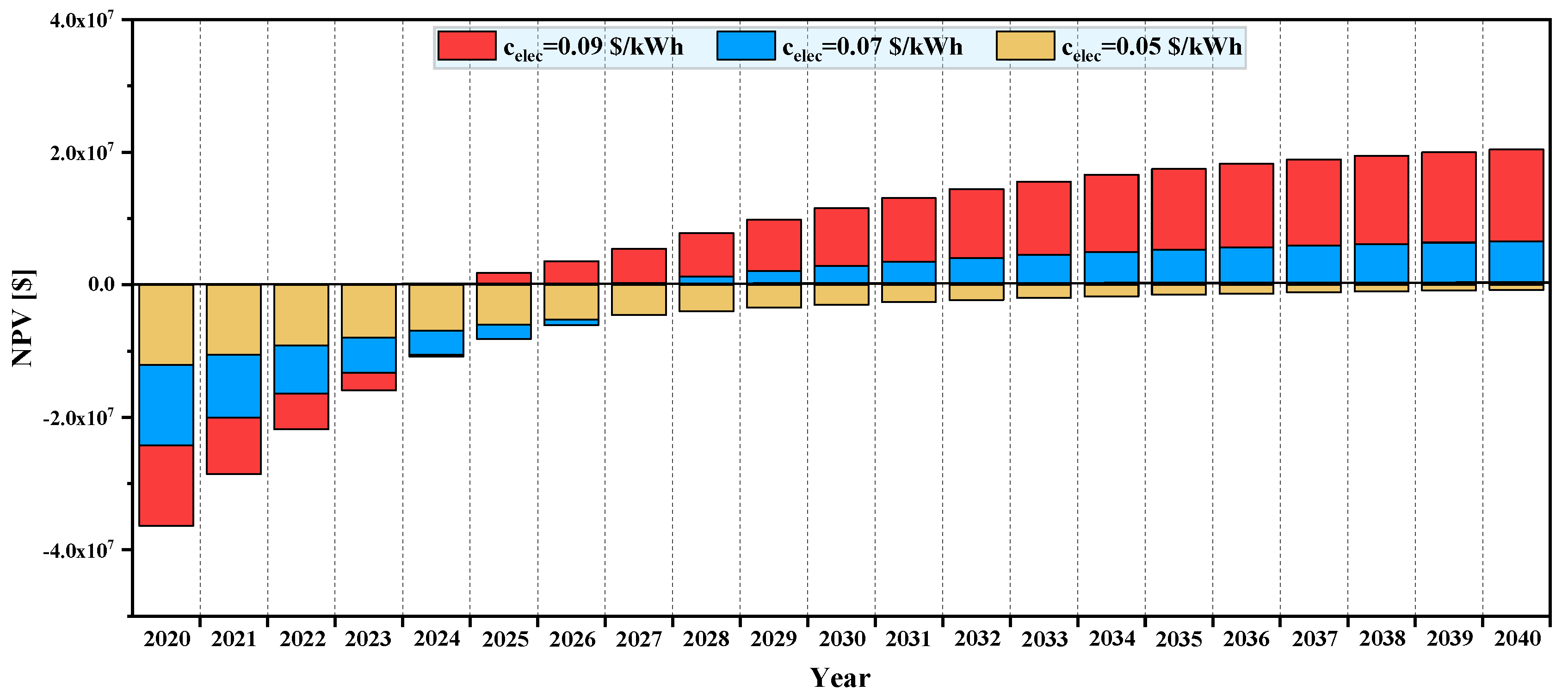
4.4. Net Present Value and Payback Period
5. Conclusions
- For the base case, the system has , , and . Also, the water production rate is calculated as .
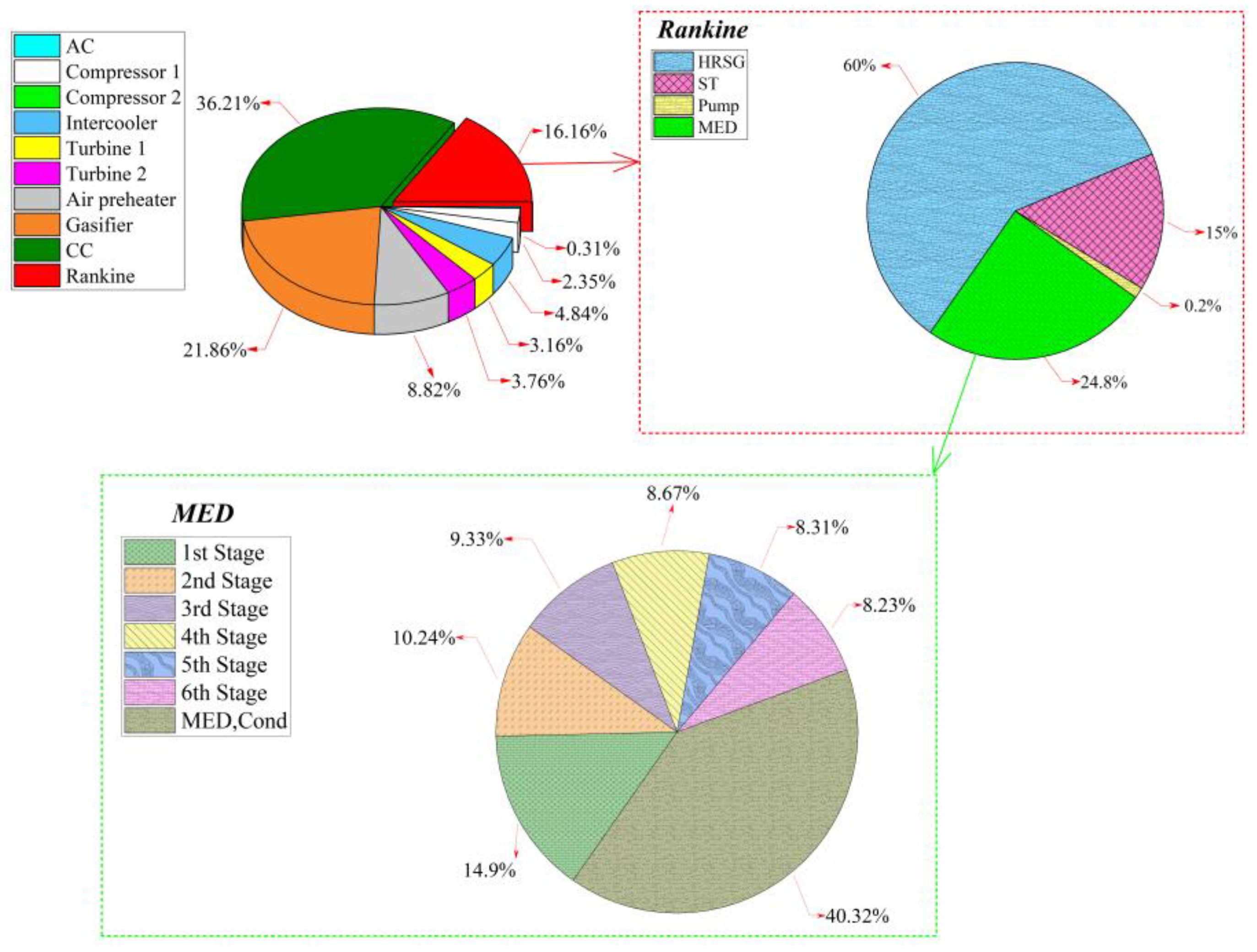
- The exergy analysis shows that, among all system components, the combustion chamber contributes the highest exergy destruction rate (), representing of the overall exergy destruction rate.
- The parametric analysis shows that increasing the gasification temperature results in lower power production.
- For a fuel cost of , a freshwater selling price of , and an electricity price of , the total NPV value at the end of plant lifetime is which means that the plant is feasible from an economic viewpoint. For these values, the payback period is . However, the payback period is sensitive to these values, increasing from to as the fuel cost increases from to .
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Symbols | |
| Area () | |
| Annual income () | |
| Approach point temperature () | |
| Annual savings () | |
| Boiling point elevation (°C) | |
| Rejected mass flow rate () | |
| Cost per unit exergy () | |
| Cost rate () | |
| Capital recovery factor | |
| Mass flow rate of steam in MED effects () | |
| Specific exergy () | |
| Exergy rate () | |
| Mass flow rate of feedwater in MED effects () | |
| Gain output ratio | |
| Gas turbine cycle | |
| Gibbs free energy ( | |
| Specific enthalpy () | |
| Heat recovery steam generator | |
| Integrated gasification combined cycle | |
| Equilibrium constant | |
| Lower heating value () | |
| Mass flow rate () | |
| Molecular weight () | |
| Multi-effect distillation | |
| Moisture content () | |
| Molar rate () | |
| Lifetime of system () | |
| Net present value () | |
| Pressure () | |
| Payback period () | |
| Pinch point temperature difference () | |
| Heat rate () | |
| Pressure ratio | |
| Universal gas constant () | |
| Specific entropy () | |
| Steam Rankine cycle | |
| Sum unit cost of product () | |
| Temperature () | |
| Total gain output ratio | |
| Heat transfer coefficient () | |
| Electricity () | |
| Waste heat recovery | |
| Salinity () | |
| Investment cost of components () | |
| Subscripts | |
| Air compressor | |
| Air preheater | |
| Approach point | |
| Combustion chamber | |
| Chemical | |
| Capital investment | |
| Condenser | |
| Control volume | |
| Destruction | |
| electricity | |
| Environmental | |
| Exergy | |
| Formation | |
| Freshwater | |
| Fuel | |
| Gas turbine | |
| Inlet | |
| Investment | |
| Isentropic | |
| component | |
| Mixer | |
| Net value | |
| Operation and maintenance | |
| Outlet | |
| Physical | |
| Product | |
| Pump | |
| Pinch point | |
| Heat transfer | |
| Regenerator | |
| Saturation | |
| Steam turbine | |
| System | |
| Thermal | |
| Total | |
| Vapor generator | |
| Work | |
| Cycle locations | |
| Dead state | |
| Greek Symbols | |
| Energy efficiency () | |
| Exergy efficiency () | |
| Maintenance factor | |
| Latent heat of vaporization () | |
Appendix A
| Component | Cost Function | Ref. Year | Cost Index |
|---|---|---|---|
| Air compressor | 1994 | 368 | |
| Compressor 1 (Com1) | 1994 | 368 | |
| Compressor 2 (Com2) | 1994 | 368 | |
| Intercooler | 2000 | 394.1 | |
| Air preheater | 1994 | 368 | |
| Gas turbine 1 (GT1) | 1994 | 368 | |
| Gas turbine 2 (GT2) | 1994 | 368 | |
| Combustion chamber | 1994 | 368 | |
| Gasifier | 1994 | 368 | |
| Steam turbine (S) | 2003 | 402 | |
| Pump | 2000 | 394.1 | |
| HRSG | 1994 | 368 | |
| MED | = 6291 [1 −], , , | 2018 | 638.1 |
| First stage | 0.16 | 2018 | 638.1 |
| Second stage | 0.16 | 2018 | 638.1 |
| Third stage | 0.16 | 2018 | 638.1 |
| Fourth stage | 0.16 | 2018 | 638.1 |
| Fifth stage | 0.16 | 2018 | 638.1 |
| Sixth stage | 0.16 | 2018 | 638.1 |
| MED condenser | = 0.04 | 2018 | 638.1 |
| Component | |
|---|---|
| Intercooler | 850 |
| Air preheater | 18 |
| Component | Cost Rate Equations | Auxiliary Equations |
|---|---|---|
| Air compressor | , | |
| Compressor 1 (Com1) | , | |
| Compressor 2 (Com2) | ||
| Intercooler | ||
| Air preheater | ||
| Gas turbine 1 (GT1) | ||
| Gas turbine 2 (GT2) | ||
| Combustion chamber | - | |
| Gasifier | - | |
| Steam turbine (S) | ||
| Pump | ||
| HRSG | ||
| First stage of MED | ||
| Second stage of MED | ||
| Third stage of MED | ||
| Fourth stage of MED | ||
| Fifth stage of MED | ||
| Sixth stage of MED | ||
| MED condenser | ||
| Division point | ||
| Gathering point | + |
| Parameter | Value |
|---|---|
| Maintenance factor, | 1.06 |
| Annual number of hours, (hours) | 7000 |
| Fuel price, () | 2 |
| Plant expected life, (years) | 20 |
| Electricity price, () | 0.07 |
| freshwater price, () | 1.8 |
| CEPCI for 2020 | 668 |
| Interest rate, () | 15 |
References
- Liu, B.; Rajagopal, D. Life-cycle energy and climate benefits of energy recovery from wastes and biomass residues in the United States. Nat. Energy 2019, 4, 700–708. [Google Scholar] [CrossRef]
- Tonini, D.; Hamelin, L.; Alvarado-Morales, M.; Astrup, T.F. GHG emission factors for bioelectricity, biomethane, and bioethanol quantified for 24 biomass substrates with consequential life-cycle assessment. Bioresour. Technol. 2016, 208, 123–133. [Google Scholar] [CrossRef]
- Yao, Z.; You, S.; Ge, T.; Wang, C.-H. Biomass gasification for syngas and biochar co-production: Energy application and economic evaluation. Appl. Energy 2018, 209, 43–55. [Google Scholar] [CrossRef]
- Gambarotta, A.; Morini, M.; Zubani, A. A non-stoichiometric equilibrium model for the simulation of the biomass gasification process. Appl. Energy 2018, 227, 119–127. [Google Scholar] [CrossRef]
- Weil, K.S. Coal gasification and IGCC technology: A brief primer. Proc. Inst. Civ. Eng. Energy 2010, 163, 7–16. [Google Scholar] [CrossRef]
- Parraga, J.; Khalilpour, K.R.; Vassallo, A. Polygeneration with biomass-integrated gasification combined cycle process: Review and prospective. Renew. Sustain. Energy Rev. 2018, 92, 219–234. [Google Scholar] [CrossRef]
- Sahraei, M.H.; McCalden, D.; Hughes, R.W.; Ricardez-Sandoval, L. A survey on current advanced IGCC power plant technologies, sensors and control systems. Fuel 2014, 137, 245–259. [Google Scholar] [CrossRef]
- Soltani, S.; Mahmoudi, S.M.S.; Yari, M.; Rosen, M. Thermodynamic analyses of an externally fired gas turbine combined cycle integrated with a biomass gasification plant. Energy Convers. Manag. 2013, 70, 107–115. [Google Scholar] [CrossRef]
- Gholamian, E.; Mahmoudi, S.M.S.; Zare, V. Proposal, exergy analysis and optimization of a new biomass-based cogeneration system. Appl. Therm. Eng. 2016, 93, 223–235. [Google Scholar] [CrossRef]
- Ahmadi, P.; Rosen, M.A.; Dincer, I. Multi-objective exergy-based optimization of a polygeneration energy system using an evolutionary algorithm. Energy 2012, 46, 21–31. [Google Scholar] [CrossRef]
- Köse, Ö.; Koç, Y.; Yağlı, H. Performance improvement of the bottoming steam Rankine cycle (SRC) and organic Rankine cycle (ORC) systems for a triple combined system using gas turbine (GT) as topping cycle. Energy Convers. Manag. 2020, 211, 112745. [Google Scholar]
- Wang, J.; Dai, Y.; Gao, L. Exergy analyses and parametric optimizations for different cogeneration power plants in cement industry. Appl. Energy 2009, 86, 941–948. [Google Scholar] [CrossRef]
- Singh, O.K.; Kaushik, S.C. Reducing CO2 emission and improving exergy based performance of natural gas fired combined cycle power plants by coupling Kalina cycle. Energy 2013, 55, 1002–1013. [Google Scholar] [CrossRef]
- Cao, L.; Wang, J.; Dai, Y. Thermodynamic analysis of a biomass-fired Kalina cycle with regenerative heater. Energy 2014, 77, 760–770. [Google Scholar] [CrossRef]
- Qi, C.-H.; Feng, H.-J.; Lv, Q.-C.; Xing, Y.-L.; Li, N. Performance study of a pilot-scale low-temperature multi-effect desalination plant. Appl. Energy 2014, 135, 415–422. [Google Scholar] [CrossRef]
- Ghaffour, N.; Bundschuh, J.; Mahmoudi, H.; Goosen, M.F. Renewable energy-driven desalination technologies: A comprehensive review on challenges and potential applications of integrated systems. Desalination 2015, 356, 94–114. [Google Scholar] [CrossRef]
- Al-Karaghouli, A.; Kazmerski, L.L. Energy consumption and water production cost of conventional and renewable-energy-powered desalination processes. Renew. Sustain. Energy Rev. 2013, 24, 343–356. [Google Scholar] [CrossRef]
- Al-Mutaz, I.; Wazeer, I. Comparative performance evaluation of conventional multi-effect evaporation desalination processes. Appl. Therm. Eng. 2014, 73, 1194–1203. [Google Scholar] [CrossRef]
- Saldivia, D.; Rosales, C.; Barraza, R.; Cornejo, L. Computational analysis for a multi-effect distillation (MED) plant driven by solar energy in Chile. Renew. Energy 2019, 132, 206–220. [Google Scholar] [CrossRef]
- Razmi, A.R.; Soltani, M.; Tayefeh, M.; Torabi, M.; Dusseault, M. Thermodynamic analysis of compressed air energy storage (CAES) hybridized with a multi-effect desalination (MED) system. Energy Convers. Manag. 2019, 199, 112047. [Google Scholar] [CrossRef]
- Baccioli, A.; Antonelli, M.; Desideri, U.; Grossi, A. Thermodynamic and economic analysis of the integration of Organic Rankine Cycle and Multi-Effect Distillation in waste-heat recovery applications. Energy 2018, 161, 456–469. [Google Scholar] [CrossRef]
- Mokhtari, H.; Sepahvand, M.; Fasihfar, A. Thermoeconomic and exergy analysis in using hybrid systems (GT+ MED+ RO) for desalination of brackish water in Persian Gulf. Desalination 2016, 399, 1–15. [Google Scholar] [CrossRef]
- Dastgerdi, H.R.; Whittaker, P.; Chua, H.T. New MED based desalination process for low grade waste heat. Desalination 2016, 395, 57–71. [Google Scholar] [CrossRef]
- Wang, Y.; Lior, N. Performance analysis of combined humidified gas turbine power generation and multi-effect thermal vapor compression desalination systems—Part 1: The desalination unit and its combination with a steam-injected gas turbine power system. Desalination 2006, 196, 84–104. [Google Scholar] [CrossRef]
- Al-Sahali, M.; Ettouney, H. Developments in thermal desalination processes: Design, energy, and costing aspects. Desalination 2007, 214, 227–240. [Google Scholar] [CrossRef]
- Rostamzadeh, H.; GhiasiRad, H.; Amidpour, M.; Amidpour, Y. Performance enhancement of a conventional multi-effect desalination (MED) system by heat pump cycles. Desalination 2020, 477, 114261. [Google Scholar] [CrossRef]
- Tsatsaronis, B.A.G.; Moran, M.J. Thermal Design and Optimization; John Wiley & Sons: New York, NY, USA, 1995. [Google Scholar]
- Sayyaadi, H.; Saffari, A. Thermoeconomic optimization of multi effect distillation desalination systems. Appl. Energy 2010, 87, 1122–1133. [Google Scholar] [CrossRef]
- Ahmadi, S.; Ghaebi, H.; Shokri, A. A comprehensive thermodynamic analysis of a novel CHP system based on SOFC and APC cycles. Energy 2019, 186, 115899. [Google Scholar] [CrossRef]
- Singh, O.K. Performance enhancement of combined cycle power plant using inlet air cooling by exhaust heat operated ammonia-water absorption refrigeration system. Appl. Energy 2016, 180, 867–879. [Google Scholar] [CrossRef]
- Behzadi, A.; Houshfar, E.; Gholamian, E.; Ashjaee, M.; Habibollahzade, A. Multi-criteria optimization and comparative performance analysis of a power plant fed by municipal solid waste using a gasifier or digester. Energy Convers. Manag. 2018, 171, 863–878. [Google Scholar] [CrossRef]
- Mehrabadi, Z.K.; Boyaghchi, F.A. Thermodynamic, economic and environmental impact studies on various distillation units integrated with gasification-based multi-generation system: Comparative study and optimization. J. Clean. Prod. 2019, 241, 118333. [Google Scholar] [CrossRef]
- Boyaghchi, F.A.; Chavoshi, M.; Sabeti, V. Multi-generation system incorporated with PEM electrolyzer and dual ORC based on biomass gasification waste heat recovery: Exergetic, economic and environmental impact optimizations. Energy 2018, 145, 38–51. [Google Scholar] [CrossRef]
- Moran, M.J.; Shapiro, H.N.; Boettner, D.D.; Bailey, M.B. Fundamentals of Engineering Thermodynamics; John Wiley & Sons: New York, NY, USA, 2010. [Google Scholar]
- Aklilu, B.; Gilani, S. Mathematical modeling and simulation of a cogeneration plant. Appl. Therm. Eng. 2010, 30, 2545–2554. [Google Scholar] [CrossRef]
- Herold, K.; Radermacher, R.; Klein, S. Engineering Equation Solver; F-Chart Software: Madison, WI, USA, 2002. [Google Scholar]
- Zainal, Z.; Ali, R.; Lean, C.; Seetharamu, K. Prediction of performance of a downdraft gasifier using equilibrium modeling for different biomass materials. Energy Convers. Manag. 2001, 42, 1499–1515. [Google Scholar] [CrossRef]
- Alauddin, Z.A. Performance and Characteristics of a Biomass Gasifier System. Ph.D. Thesis, University of Wales, Cardiff, UK, 1996. [Google Scholar]
- Vidali, H.A.R.; Kousi, P. Modelling and Thermodynamic Analysis of a Multi Effect Distillation (MED) Plant for Seawater Desalination; National Technical University of Athens (NTUA): Athens, Greece, 2003. [Google Scholar]
- CEPCI. The Chemical Engineering Plant Cost Index n.d. 2020. Available online: https://www.chemengonline.com (accessed on 27 September 2020).
- Moghimi, M.; Emadi, M.; Ahmadi, P.; Moghadasi, H. 4E analysis and multi-objective optimization of a CCHP cycle based on gas turbine and ejector refrigeration. Appl. Therm. Eng. 2018, 141, 516–530. [Google Scholar] [CrossRef]








| Parameter | Value | Unit |
|---|---|---|
| Reference pressure, | 1 | bar |
| Reference temperature, | 298.15 | K |
| Isentropic efficiency of gas turbines, | 86 | % |
| Isentropic efficiency of steam turbine, | 88 | % |
| Isentropic efficiency of compressors, | 86 | % |
| Isentropic efficiency of pump, | 86 | % |
| Pressure ratio of compressors, | 4 | |
| Combustion product temperature, | 1500 | K |
| Combustion chamber pressure drop, | 5 | % |
| Gasification temperature, | 1073.15 | K |
| Gasification pressure, | 4 | bar |
| Mass flow rate of biomass, | 1.25 | kg/s |
| Moisture content in biomass | 20 | % |
| Temperature of water entering intercooler, | 303.15 | K |
| Temperature of water exiting intercooler, | 311.15 | k |
| Pinch point temperature difference of Intercooler, | 17 | K |
| Effectiveness of air preheater, | 75 | % |
| Stack temperature, | 423.15 | K |
| PPTD of HRSG, | 10 | K |
| APTD of HRSG, A | 10 | K |
| Steam turbine inlet pressure, | 20 | bar |
| Steam turbine outlet pressure, | 0.25 | bar |
| MED feedwater temperature, | 318.15 | K |
| Seawater temperature, | 303.15 | K |
| Salinity of seawater | 42 | |
| Salinity of brine | 70 | |
| Number of MED stages, | 6 | |
| Temperature difference between effects, | 2.8 | K |
| MED final-stage temperature | 321.15 | K |
| Component | Mass and Energy Rate Balances | |
|---|---|---|
| Air compressor | , | |
| Compressor 1 (Com1) | , | |
| Compressor 2 (Com2) | , | |
| Intercooler | , | |
| Air preheater | , | |
| Gas turbine 1 (GT1) | , | |
| Gas turbine 2 (GT2) | , | |
| Combustion chamber | ||
| Gasifier | ||
| Steam turbine (S) | , | |
| Pump | =, | |
| HRSG | , | |
| First stage | + = + + | |
| Second stage | , + = + + | |
| Third stage | , + = + + | |
| Fourth stage | , = + + | |
| Fifth stage | , + = + + | |
| Sixth stage | , + = + + | |
| MED condenser | , |
| Gasifier | ||||
| Gas Yield | Present Work (%) | Gholamian et al. [9] (%) | Zainal et al. [37] (%) | Experiment [38] (%) |
| MED | ||||
| Parameter | Present Work | Ref. [39] | Error (%) | |
| Water production rate () | 0.8225 | 0.8311 | 1.03 | |
| Salinity of brine () | 55.00 | 55.91 | 1.62 | |
| Operating pressure of first effect () | 0.275 | 0.265 | 3.77 | |
| Performance ratio | 9.452 | 9.5576 | 1.1 | |
| Cycle States | ||||||||
|---|---|---|---|---|---|---|---|---|
| 1 | 298.15 | 1.013 | - | - | 1.25 | 18,237 | 919,133 | 2 |
| 2 | 298.15 | 1.013 | 0 | 6.884 | 1.891 | 0 | 0 | 0 |
| 3 | 464.8 | 4.052 | 169.8 | 6.937 | 1.891 | 293.6 | 65,963 | 8.917 |
| 4 | 1073 | 4.052 | −2727 | 9.674 | 3.141 | 16,568 | 1.134 × 106 | 2.715 |
| 5 | 298.2 | 1.013 | 0 | 6.884 | 14.52 | 0 | 0 | 0 |
| 6 | 464.8 | 4.052 | 169.8 | 6.937 | 14.52 | 2255 | 1.116 × 106 | 19.64 |
| 7 | 320 | 4.052 | 22.06 | 6.556 | 14.52 | 1757 | 869,617 | 19.64 |
| 8 | 498 | 16.21 | 204.1 | 6.608 | 14.52 | 4175 | 1.409 × 106 | 13.39 |
| 9 | 989 | 16.21 | 740.3 | 7.354 | 14.52 | 8735 | 3.395 × 106 | 15.42 |
| 10 | 733.1 | 4.052 | 454.5 | 7.419 | 14.52 | 4300 | 1.671 × 106 | 15.42 |
| 11 | 1500 | 3.849 | −111.3 | 8.443 | 17.67 | 17,618 | 2.821 × 106 | 6.354 |
| 12 | 1153 | 0.9624 | −553.6 | 8.507 | 17.67 | 9466 | 1.516 × 106 | 6.354 |
| 13 | 786 | 0.9624 | −994.5 | 8.048 | 17.67 | 4113 | 658,674 | 6.354 |
| 14 | 423 | 0.9624 | −1398 | 7.362 | 17.67 | 582.4 | 93,253 | 6.354 |
| 15 | 338.3 | 20 | 274.3 | 0.8942 | 2.419 | 35.74 | 13,188 | 14.64 |
| 16 | 660.6 | 20 | 3220 | 7.085 | 2.419 | 2695 | 763,985 | 11.25 |
| 17 | 338.1 | 0.25 | 2468 | 7.388 | 2.419 | 658.9 | 186,754 | 11.25 |
| 18 | 338.1 | 0.25 | 272 | 0.8933 | 2.419 | 30.77 | 8723 | 11.25 |
| 19 | 303.15 | 1.013 | 125.8 | 0.4365 | 64.12 | 171.4 | 0 | 0 |
| 20 | 311.15 | 1.013 | 159.2 | 0.5454 | 64.12 | 234.1 | 247,856 | 42.01 |
| 21 | 303.15 | 1.013 | 118.5 | 0.4102 | 73.11 | 15 | 0 | 0 |
| 22 | 318.15 | 1.013 | 178.1 | 0.602 | 73.11 | 188.1 | 155,024 | 32.7 |
| 23 | 318.15 | 1.013 | 178.1 | 0.602 | 43.86 | 112.9 | 92,997 | 32.7 |
| 24 | 318.15 | 1.013 | 178.1 | 0.602 | 29.25 | 75.28 | 62,027 | 32.7 |
| 25 | 318.15 | 1.013 | 178.1 | 0.602 | 4.876 | 12.55 | 10,338 | 32.7 |
| 26 | 318.15 | 1.013 | 178.1 | 0.602 | 4.876 | 12.55 | 10,338 | 32.7 |
| 27 | 318.15 | 1.013 | 178.1 | 0.602 | 4.876 | 12.55 | 10,338 | 32.7 |
| 28 | 318.15 | 1.013 | 178.1 | 0.602 | 4.876 | 12.55 | 10,338 | 32.7 |
| 29 | 318.15 | 1.013 | 178.1 | 0.602 | 4.876 | 12.55 | 10,338 | 32.7 |
| 30 | 318.15 | 1.013 | 178.1 | 0.602 | 4.876 | 12.55 | 10,338 | 32.7 |
| 31 | 334.4 | 0.2173 | 2611 | 7.888 | 2.109 | 561.2 | 168,907 | 11.94 |
| 32 | 334.4 | 1.013 | 232.8 | 0.7548 | 2.767 | 25.67 | 21,148 | 32.7 |
| 33 | 334.4 | 0.2173 | 256.3 | 0.8467 | 2.109 | 23.08 | 6947 | 11.94 |
| 34 | 331.6 | 0.1907 | 2606 | 7.933 | 1.993 | 493.9 | 157,608 | 12.66 |
| 35 | 331.6 | 1.013 | 222.5 | 0.7246 | 5.649 | 45.54 | 37,524 | 32.7 |
| 36 | 331.6 | 0.1907 | 244.6 | 0.8115 | 1.993 | 19.36 | 6179 | 12.66 |
| 37 | 328.8 | 0.167 | 2601 | 7.979 | 1.916 | 439.2 | 151,719 | 13.71 |
| 38 | 328.8 | 1.013 | 212 | 0.6934 | 8.609 | 59.78 | 49,259 | 32.7 |
| 39 | 328.8 | 0.167 | 232.9 | 0.776 | 1.916 | 16.43 | 5677 | 13.71 |
| 40 | 326 | 0.1459 | 2596 | 8.026 | 1.879 | 395.1 | 150,643 | 15.13 |
| 41 | 326 | 1.013 | 201.3 | 0.6613 | 11.61 | 68.79 | 56,683 | 32.7 |
| 42 | 326 | 0.1459 | 221.1 | 0.7401 | 1.879 | 14.14 | 5392 | 15.13 |
| 43 | 323.15 | 0.127 | 2591 | 8.074 | 1.881 | 359.2 | 153,657 | 16.98 |
| 44 | 323.15 | 1.013 | 190.6 | 0.6284 | 14.6 | 73.19 | 60,300 | 32.7 |
| 45 | 323.15 | 0.127 | 209.4 | 0.7039 | 1.881 | 12.35 | 5285 | 16.98 |
| 46 | 320.15 | 0.1103 | 2586 | 8.124 | 1.923 | 329.2 | 159,928 | 19.28 |
| 47 | 320.15 | 1.013 | 179.7 | 0.5947 | 17.55 | 73.75 | 60,768 | 32.7 |
| 48 | 320.3 | 0.1103 | 197.6 | 0.6673 | 1.923 | 10.96 | 5326 | 19.28 |
| 49 | 327.5 | 0.1529 | 227.6 | 0.76 | 11.7 | 96.34 | 34,805 | 14.34 |
| Performance Index | Value | Unit |
|---|---|---|
| Net output power () | 8.347 | |
| Produced freshwater rate () | 11.7 | |
| Exergy efficiency () | 46.22 | |
| Net present value over considered lifetime () | ||
| Payback period () | 6.75 | years |
| SUCP | 14.07 |
| Component | ||||||
|---|---|---|---|---|---|---|
| Air compressor (AC) | 7.267 | 8.917 | 5033 | 7170 | 27.48 | 91.44 |
| Compressor 1 (Com1) | 17.07 | 19.64 | 90,797 | 55,073 | 211.1 | 91.44 |
| Compressor 2 (Com2) | 7.267 | 8.855 | 41,648 | 55,073 | 227.4 | 91.4 |
| Intercooler | 19.64 | 156.7 | 215,127 | 1678 | 434.8 | 12.61 |
| Air preheater | 6.354 | 17.28 | 126,810 | 1.129 × 106 | 791.9 | 85.2 |
| Gas turbine 1 (GT1) | 15.42 | 17.07 | 110,125 | 61,881 | 283.3 | 93.61 |
| Gas turbine 2 (GT2) | 6.354 | 7.267 | 54,046 | 125,715 | 337.5 | 95.86 |
| Combustion chamber | 5.334 | 6.354 | 436,880 | 16,147 | 3250 | 84.42 |
| Gasifier | 2.11 | 2.715 | 104,310 | 148,578 | 1962 | 89.41 |
| Steam turbine (S) | 11.25 | 29.45 | 61,957 | 771,991 | 218.6 | 89.27 |
| Pump | 29.45 | 35.7 | 518.6 | 262.5 | 0.6988 | 87.66 |
| HRSG | 6.354 | 11.2 | 139,524 | 185,376 | 871.3 | 75.32 |
| First stage of MED | 11.25 | 12.42 | 15,250 | 1686 | 53.8 | 91.43 |
| Second stage of MED | 11.94 | 12.96 | 11,103 | 1686 | 36.89 | 93.14 |
| Third stage of MED | 12.66 | 13.78 | 10,722 | 1686 | 33.6 | 92.92 |
| Fourth stage of MED | 13.71 | 14.97 | 10,794 | 1686 | 31.25 | 92.61 |
| Fifth stage of MED | 15.13 | 16.61 | 11,404 | 1686 | 29.91 | 92.15 |
| Sixth stage of MED | 16.98 | 18.77 | 12,678 | 1686 | 29.64 | 91.46 |
| MED condenser | 19.28 | 35.53 | 70,481 | 421.6 | 145.1 | 54.41 |
| Parameter | Range | Base Case Value |
|---|---|---|
| Compressor pressure ratio, | ||
| Gasification temperature, | ||
| Combustion chamber temperature, | ||
| Air preheater efficiency, | ||
| Superheating temperature difference, | ||
| Feedwater temperature, [°C] |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hamrang, F.; Shokri, A.; Mahmoudi, S.M.S.; Ehghaghi, B.; Rosen, M.A. Performance Analysis of a New Electricity and Freshwater Production System Based on an Integrated Gasification Combined Cycle and Multi-Effect Desalination. Sustainability 2020, 12, 7996. https://doi.org/10.3390/su12197996
Hamrang F, Shokri A, Mahmoudi SMS, Ehghaghi B, Rosen MA. Performance Analysis of a New Electricity and Freshwater Production System Based on an Integrated Gasification Combined Cycle and Multi-Effect Desalination. Sustainability. 2020; 12(19):7996. https://doi.org/10.3390/su12197996
Chicago/Turabian StyleHamrang, Farzad, Afshar Shokri, S. M. Seyed Mahmoudi, Biuk Ehghaghi, and Marc A. Rosen. 2020. "Performance Analysis of a New Electricity and Freshwater Production System Based on an Integrated Gasification Combined Cycle and Multi-Effect Desalination" Sustainability 12, no. 19: 7996. https://doi.org/10.3390/su12197996
APA StyleHamrang, F., Shokri, A., Mahmoudi, S. M. S., Ehghaghi, B., & Rosen, M. A. (2020). Performance Analysis of a New Electricity and Freshwater Production System Based on an Integrated Gasification Combined Cycle and Multi-Effect Desalination. Sustainability, 12(19), 7996. https://doi.org/10.3390/su12197996







