Single-Phase Active Power Harmonics Filter by Op-Amp Circuits and Power Electronics Devices
Abstract
1. Introduction
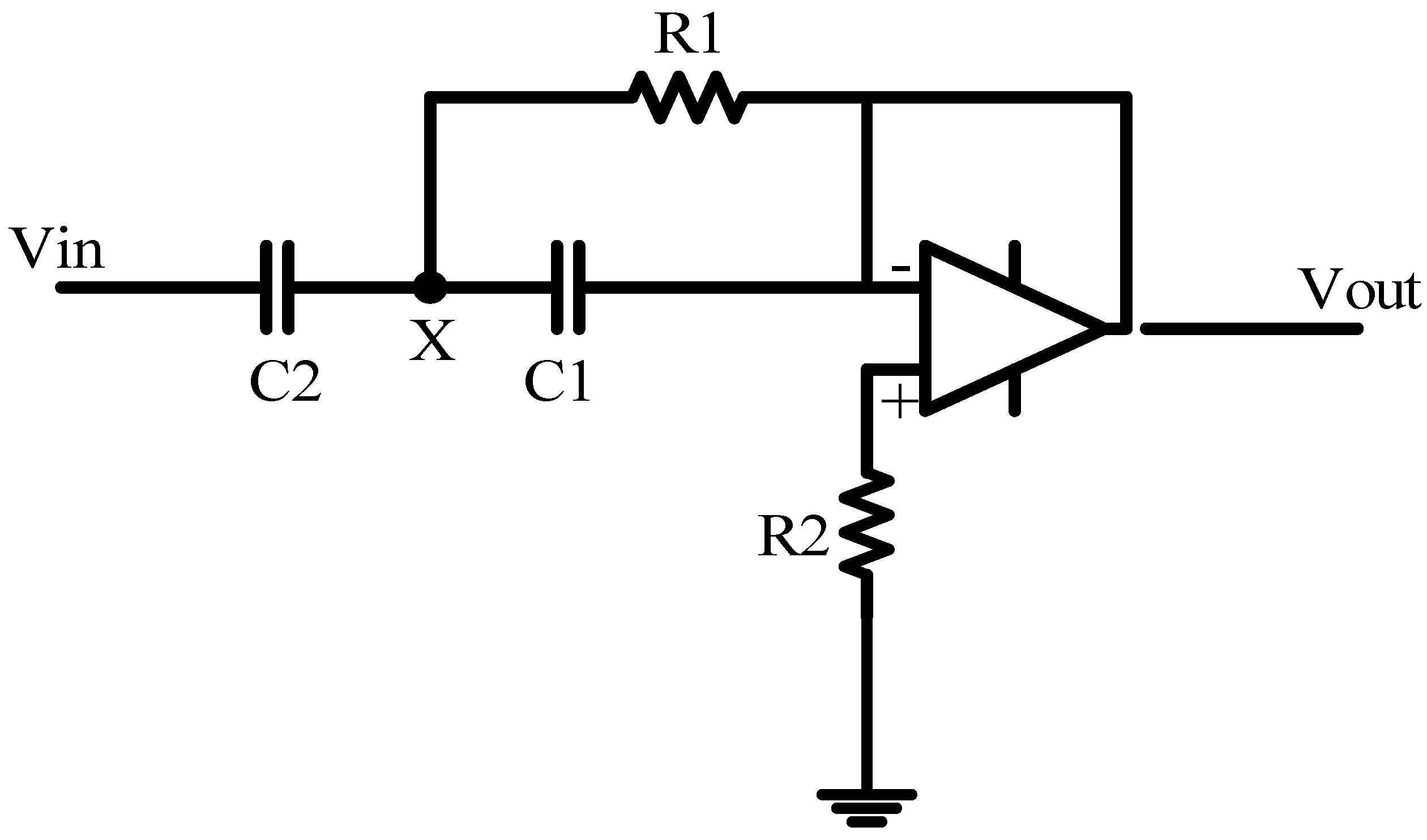
2. The Strategy of Harmonics Detection
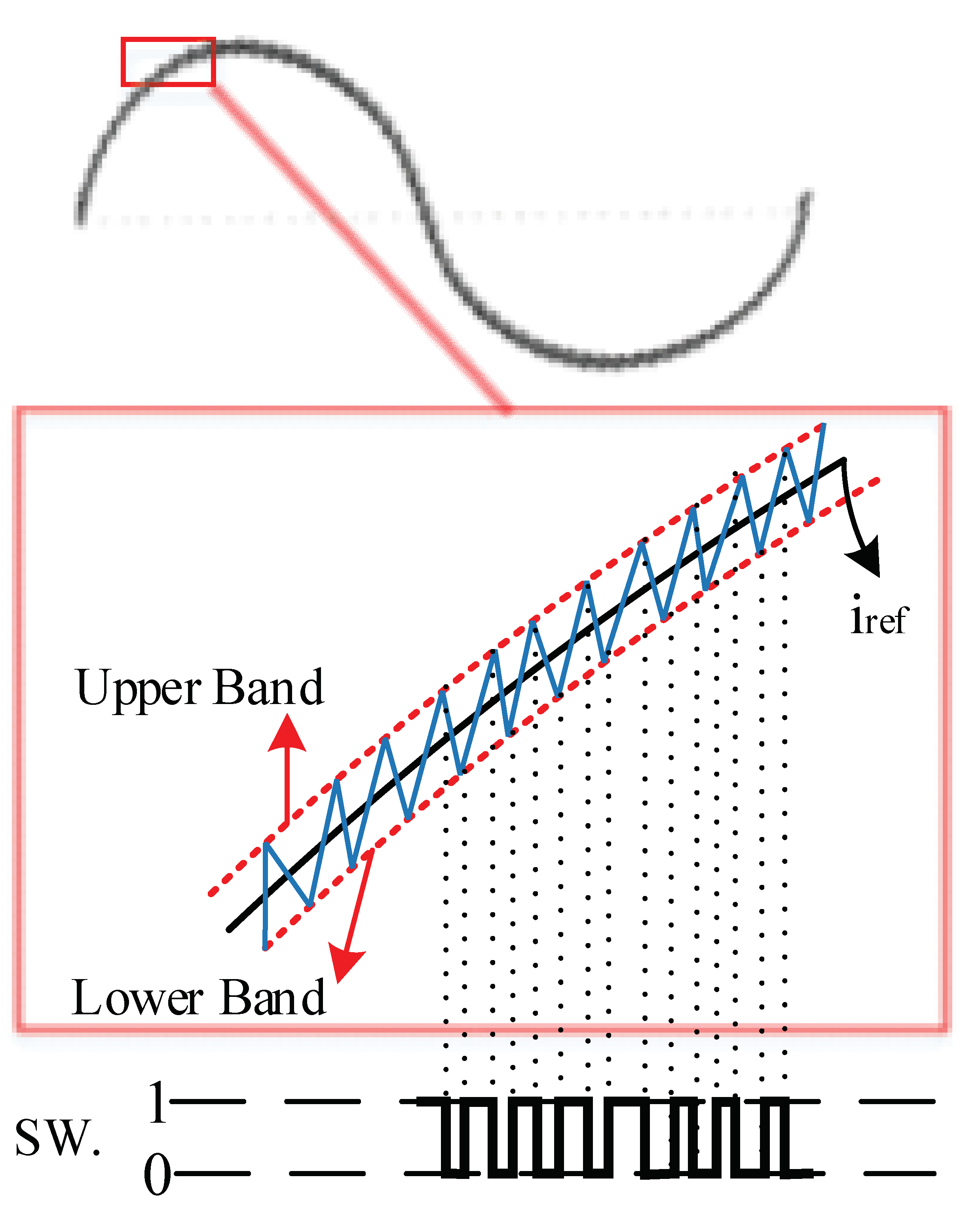
Hysteresis Switching Technique (HYS)
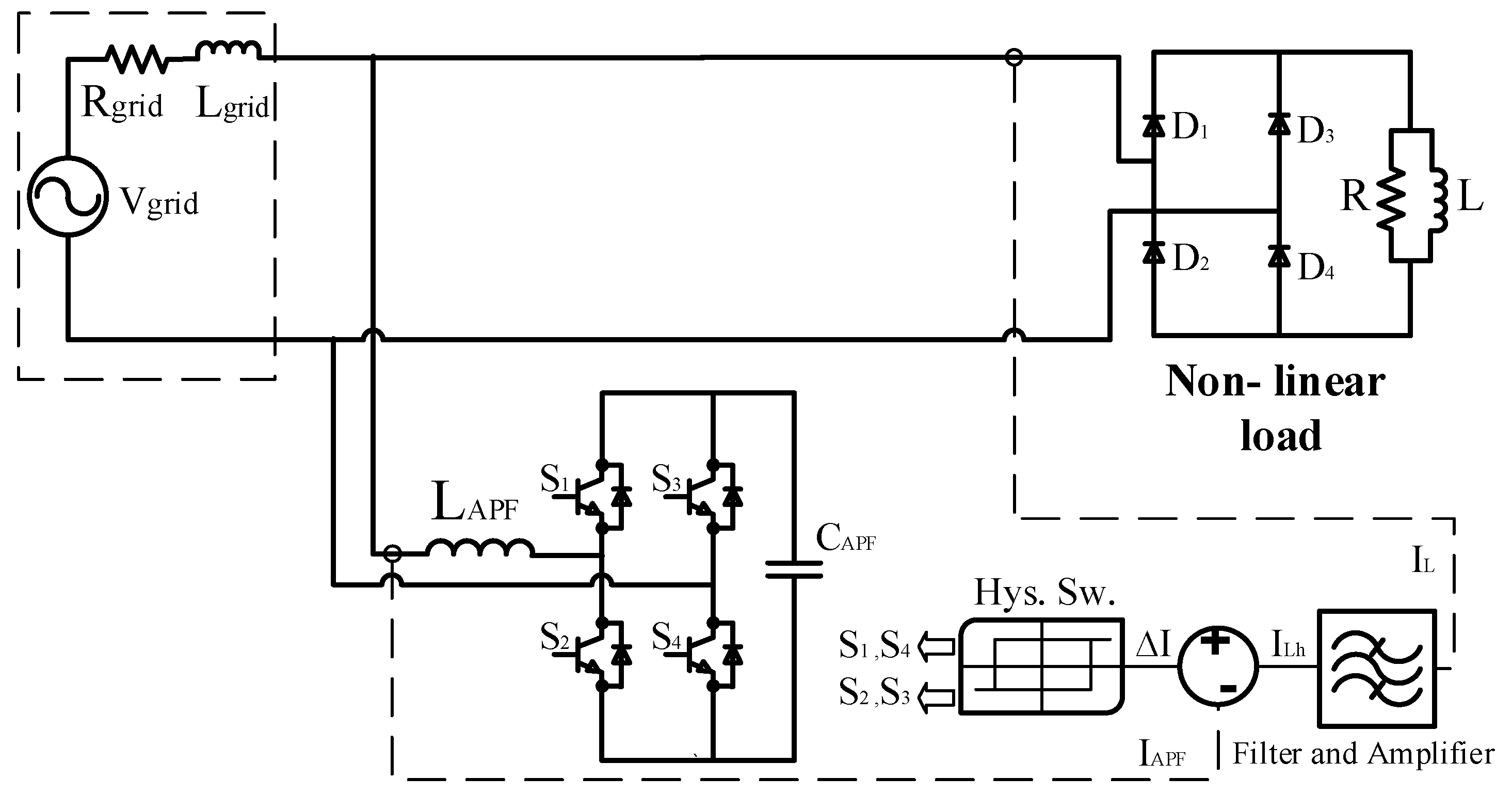
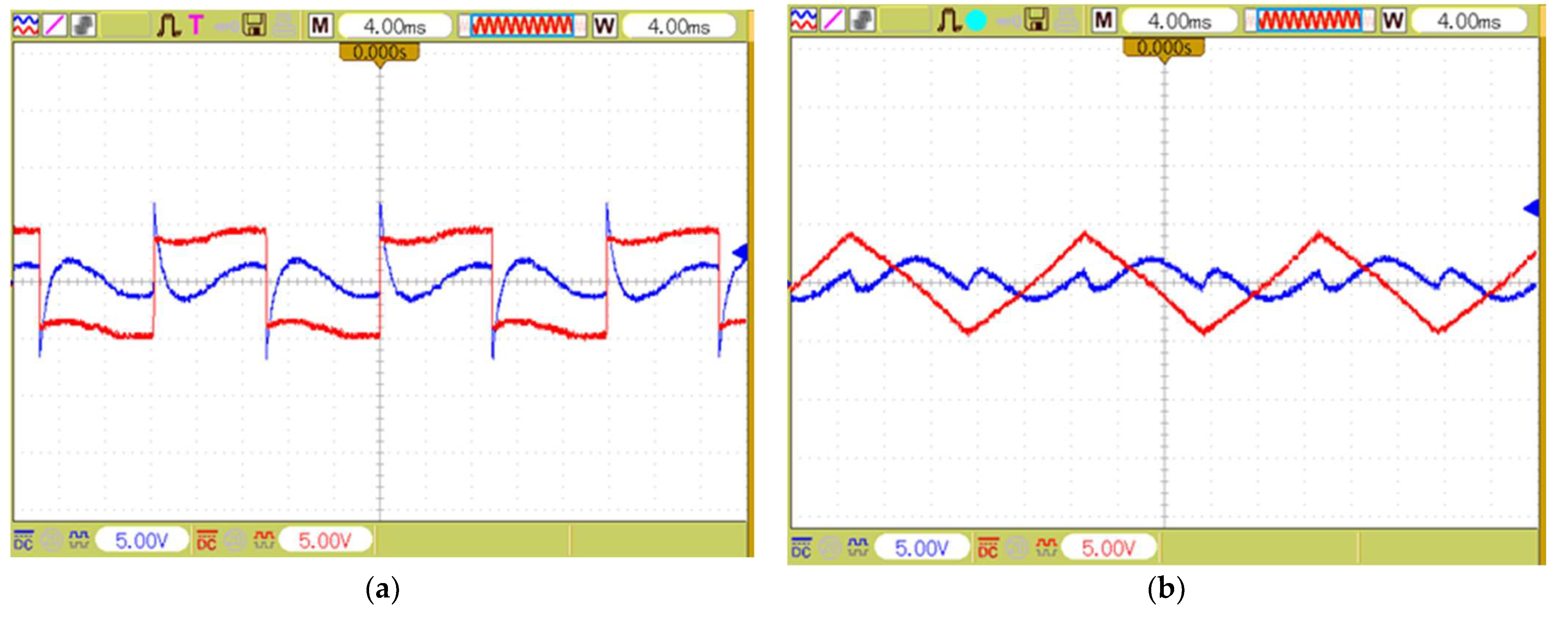
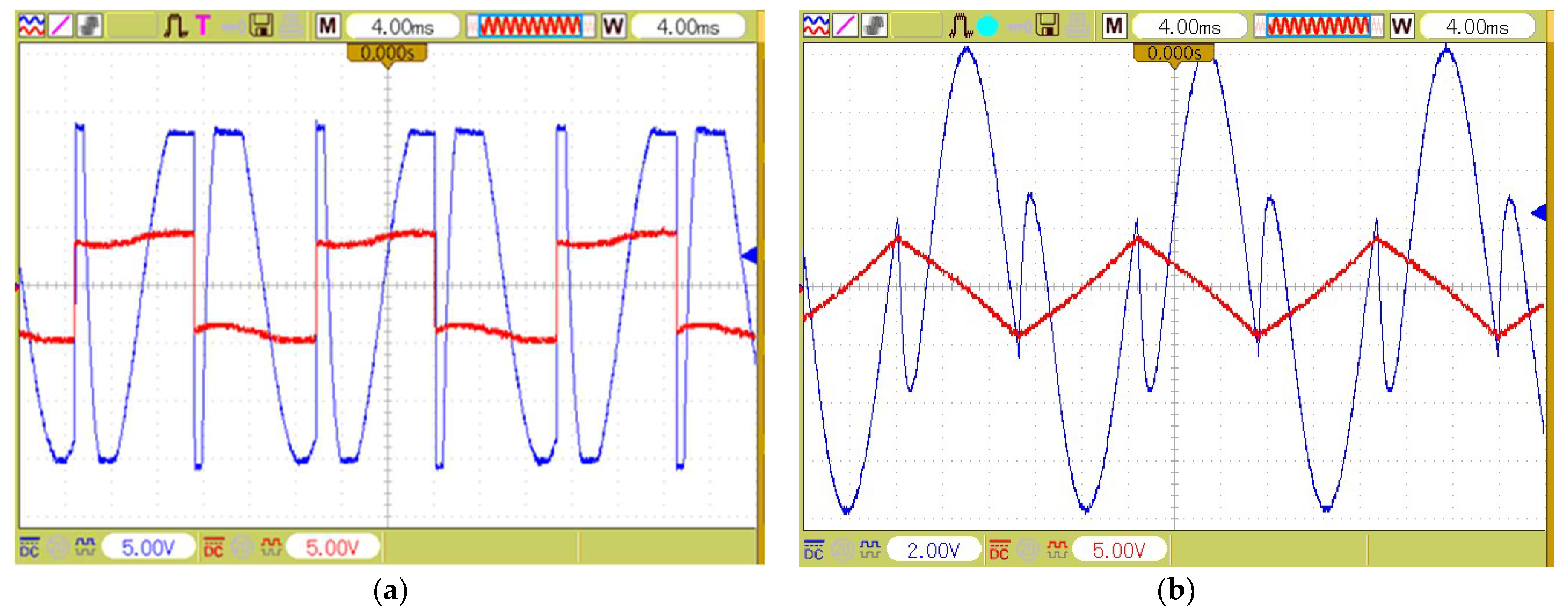
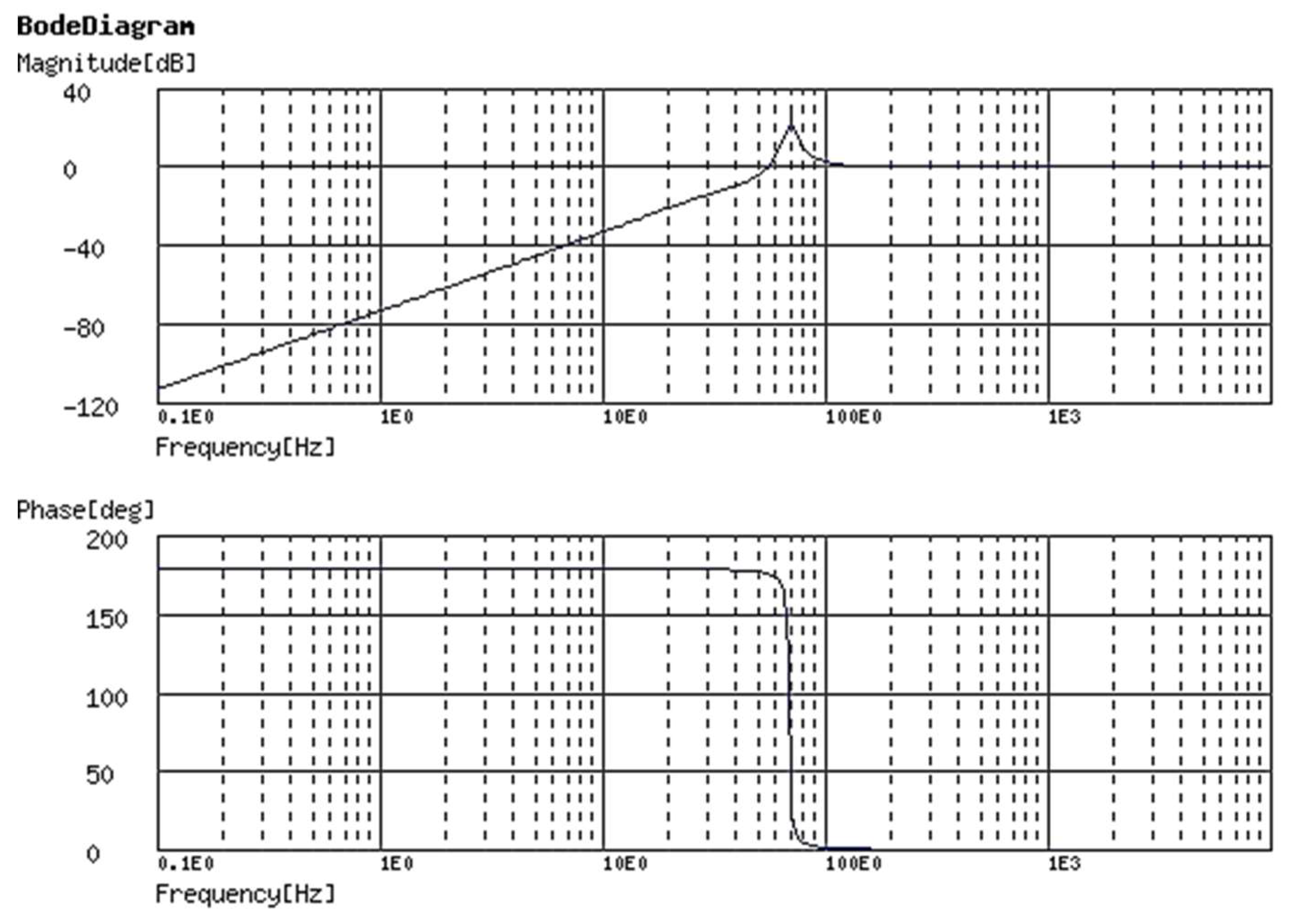
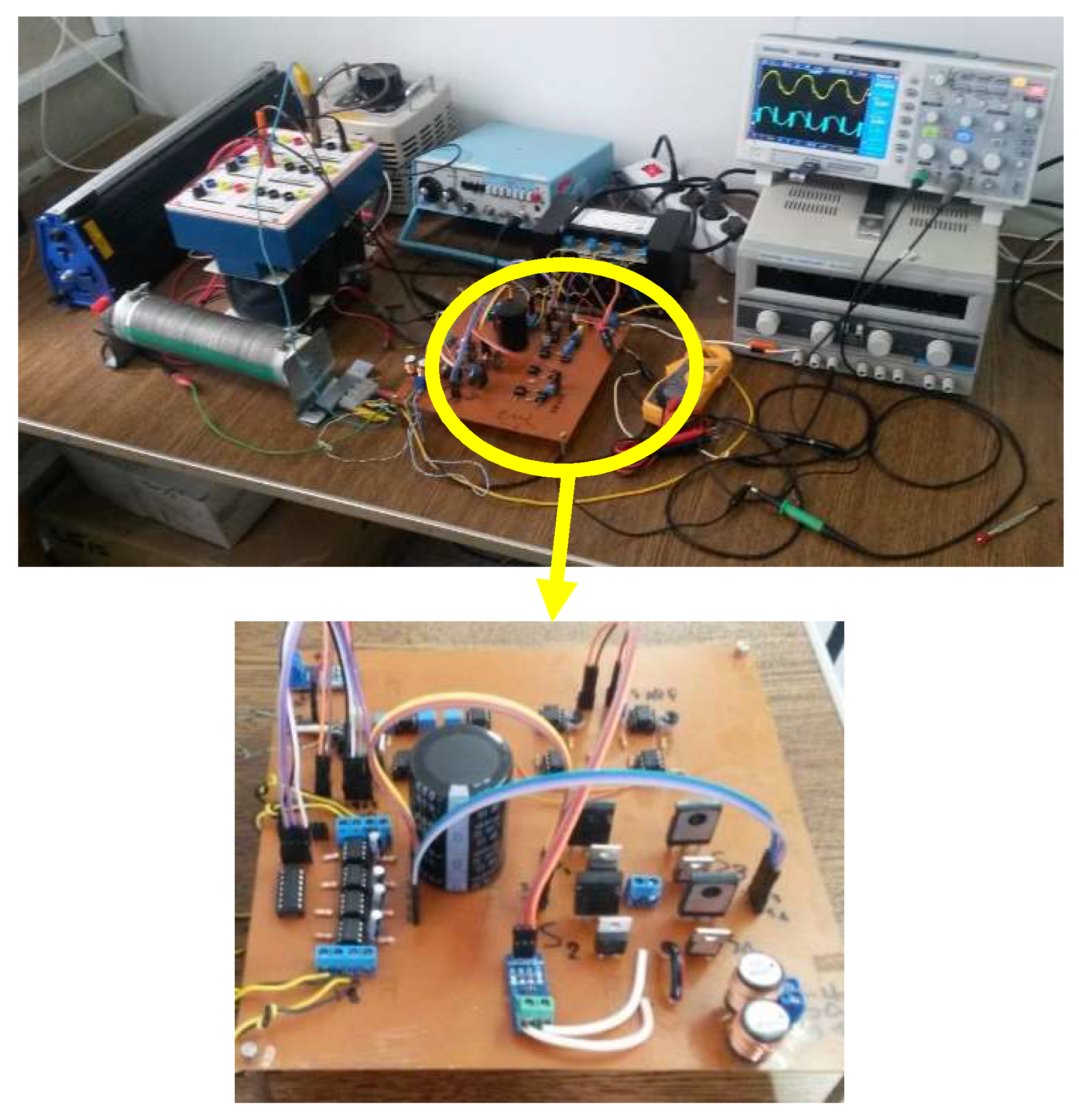
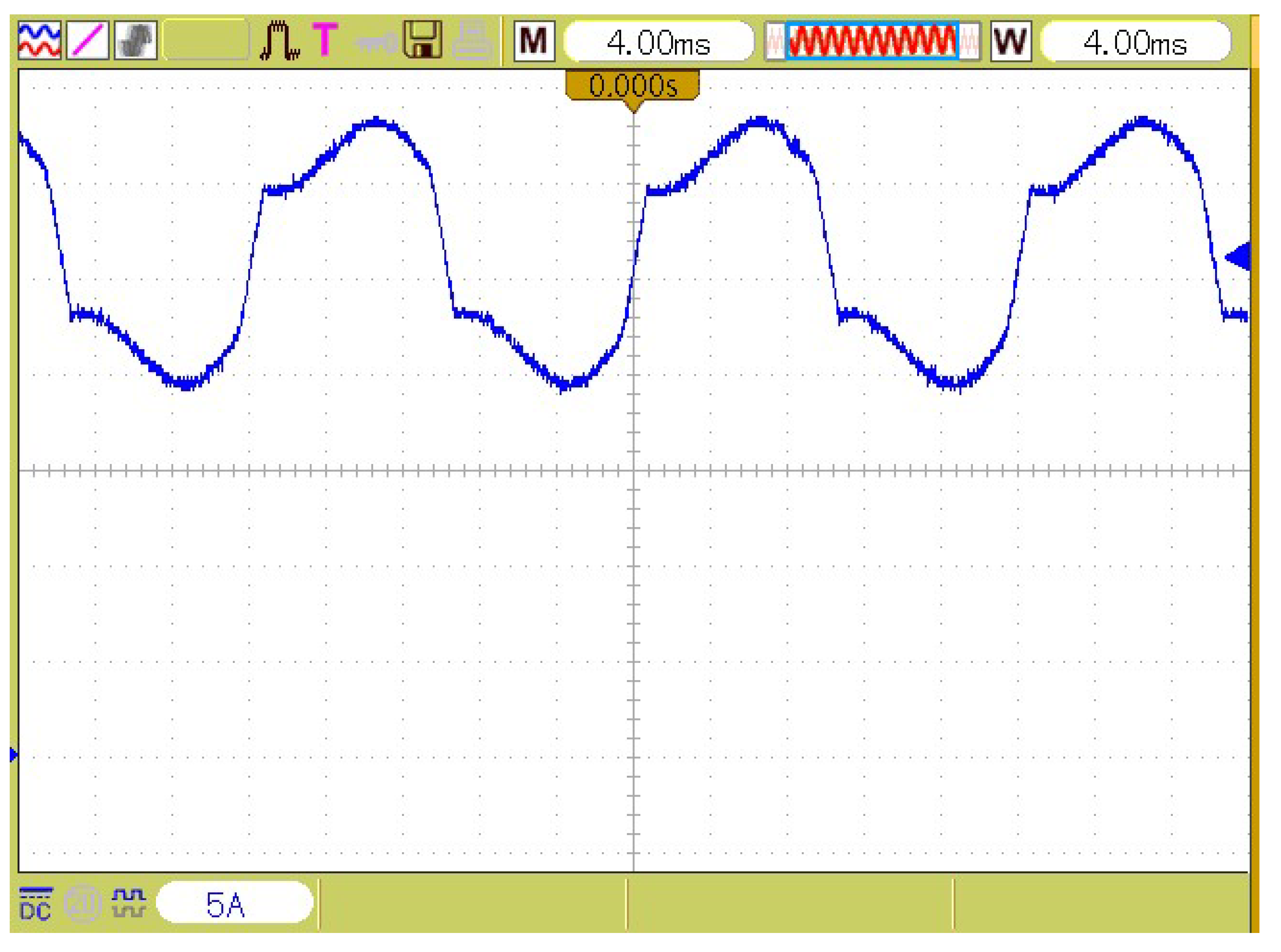
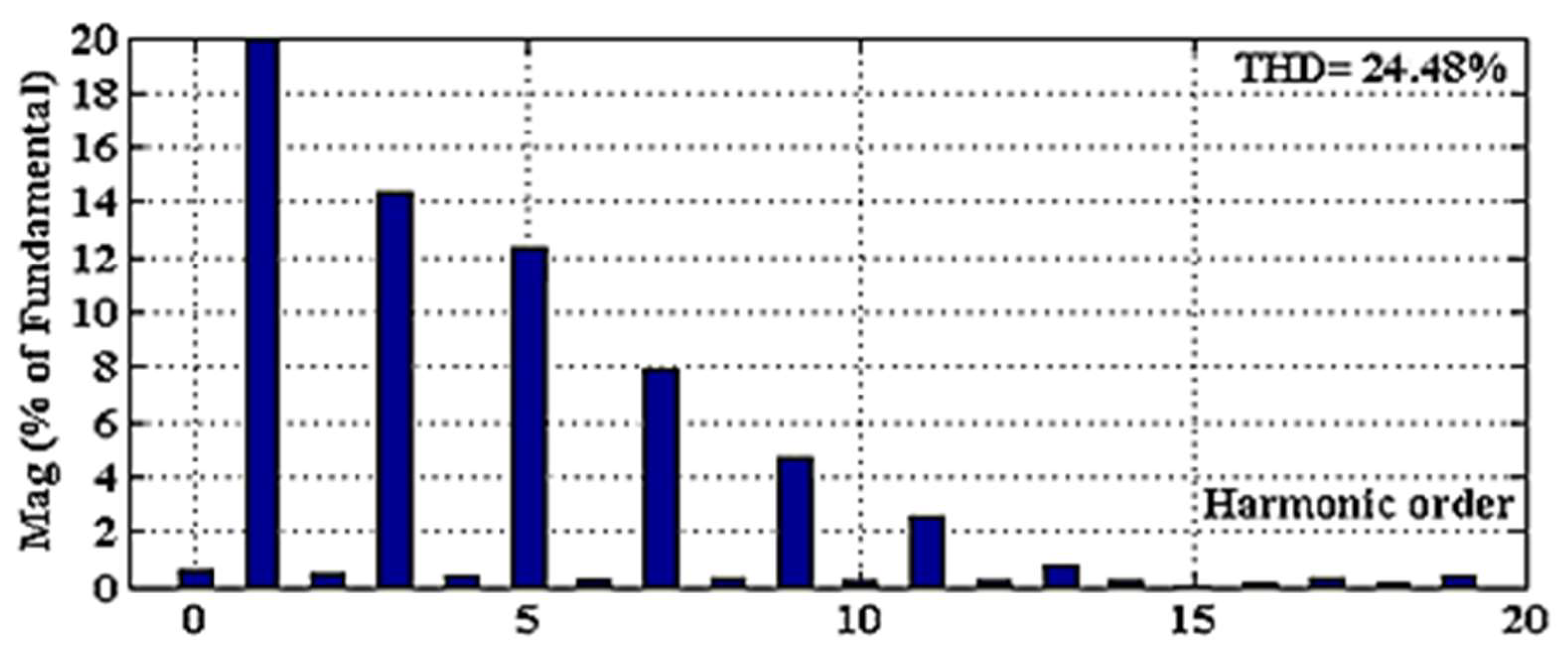
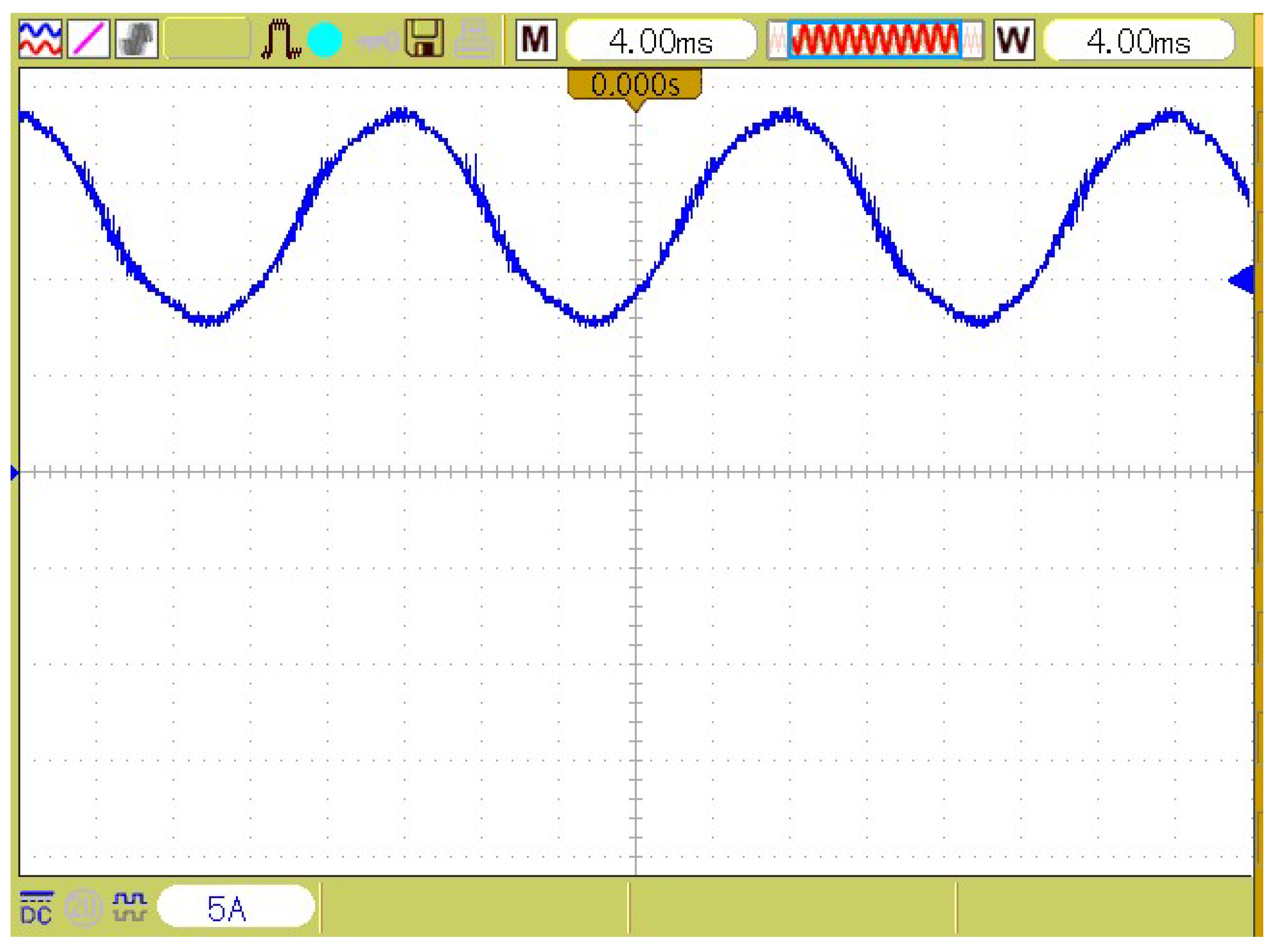
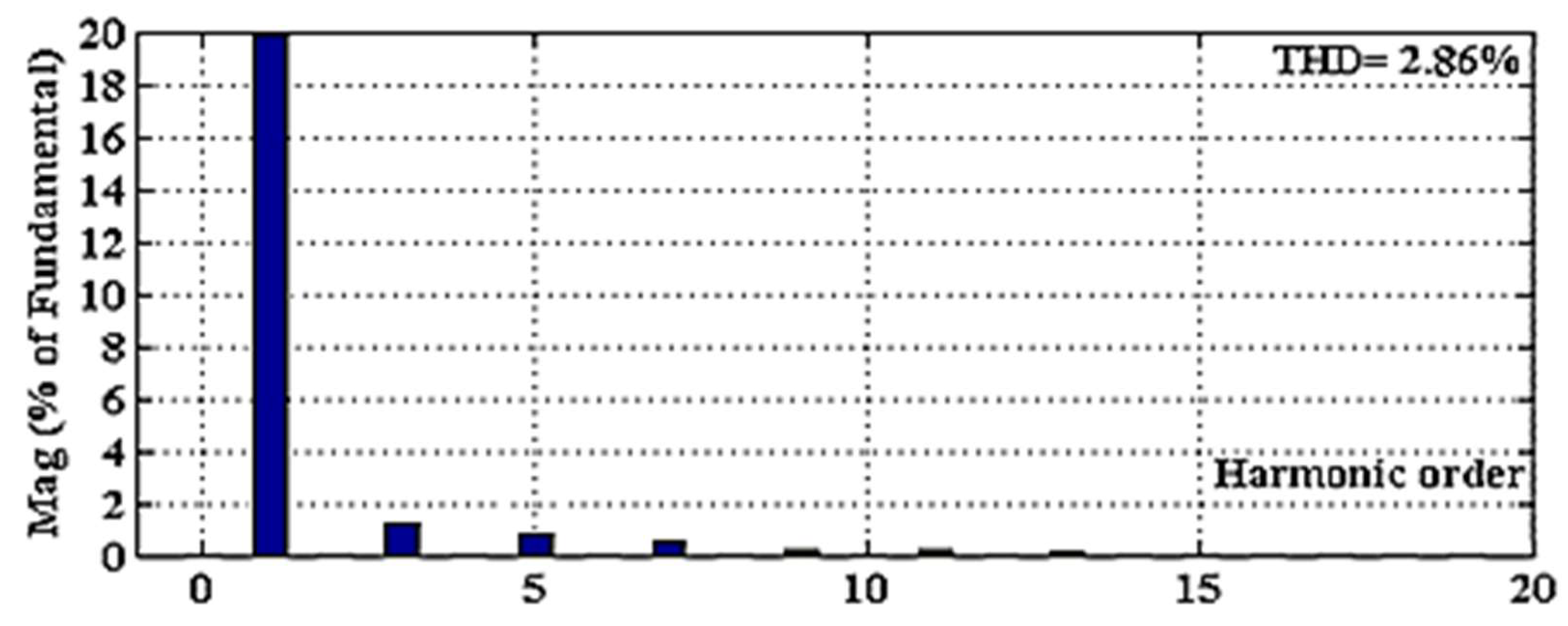
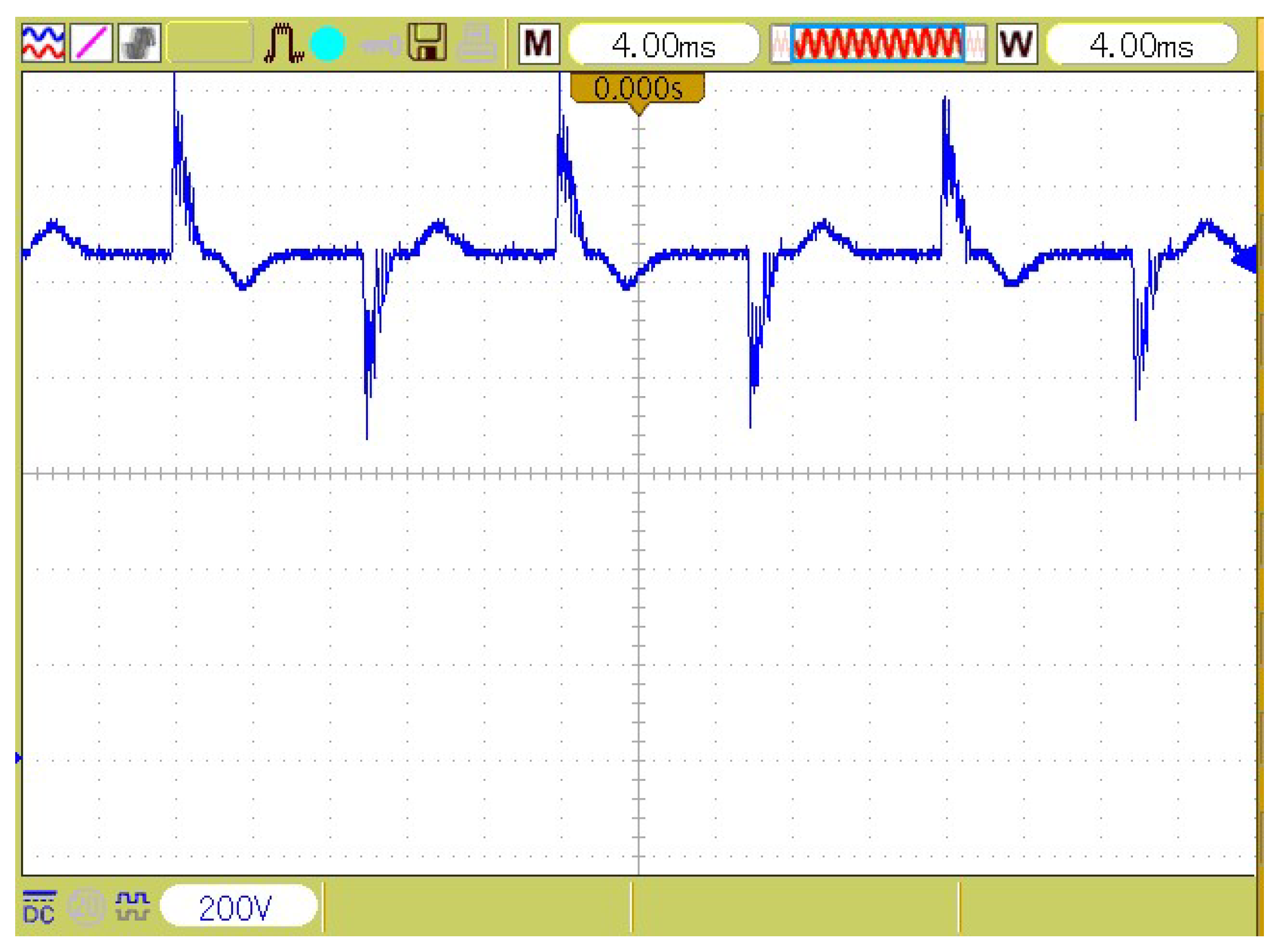
3. Experimental Results
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Singh, R.; Singh, A. Energy loss due to harmonics in residential campus—A case study. In Proceedings of the 45th International Universities Power Engineering Conference UPEC2010, Cardiff, UK, 31 August–3 September 2010; pp. 1–6. [Google Scholar]
- Simpson, R.H. Misapplication of power capacitors in distribution systems with nonlinear loads-three case histories. IEEE Trans. Ind. Appl. 2005, 41, 134–143. [Google Scholar] [CrossRef]
- Yazdani-Asrami, M.; Sadati, S.M.B.; Samadaei, E. Harmonic study for MDF industries: A case study. In Proceedings of the 2011 IEEE Applied Power Electronics Colloquium (IAPEC), Johor Bahru, Malaysia, 18–19 April 2011; pp. 149–154. [Google Scholar] [CrossRef]
- Gao, S.; Li, X.; Ma, X.; Hu, H.; He, Z.; Yang, J. Measurement-based compartmental modeling of harmonic sources in traction power-supply system. IEEE Trans. Power Deliv. 2017, 32, 900–909. [Google Scholar] [CrossRef]
- Grady, W.M.; Santoso, S. Understanding power system harmonics. IEEE Power Eng. Rev. 2001, 21, 8–11. [Google Scholar] [CrossRef]
- Samadaei, E.; Khosravi, A.; Sheikholeslami, A. Optimal Allocation of Active Power Filter On real distribution network for improvement of power quality by use of BBO: A case study. IIUM Eng. J. 2017, 18, 85–99. [Google Scholar] [CrossRef]
- Beres, R.N.; Wang, X.; Liserre, M.; Blaabjerg, F.; Bak, C.L. A review of passive power filters for three-phase grid-connected voltage-source converters. IEEE J. Emerg. Sel. Top. Power Electron. 2016, 4, 54–69. [Google Scholar] [CrossRef]
- Chang, G.W.; Chu, S.Y.; Wang, H.L. A new method of passive harmonic filter planning for controlling voltage distortion in a power system. IEEE Trans. Power Deliv. 2006, 21, 305–312. [Google Scholar] [CrossRef]
- Zobaa, A.F. The optimal passive filters to minimize voltage harmonic distortion at a load bus. IEEE Trans. Power Deliv. 2005, 20, 1592–1597. [Google Scholar] [CrossRef]
- Badrzadeh, B.; Smith, K.S.; Wilson, R.C. Designing passive harmonic filters for an aluminum smelting plant. IEEE Trans. Ind. Appl. 2011, 47, 973–983. [Google Scholar] [CrossRef]
- Chang, G.W.; Wang, H.L.; Chu, S.Y. Strategic placement and sizing of passive filters in a power system for controlling voltage distortion. IEEE Trans. Power Deliv. 2004, 19, 1204–1211. [Google Scholar] [CrossRef]
- Zhao, B.; Abramovitz, A.; Smedley, K. Family of bridgeless buck-boost PFC rectifiers. IEEE Trans. Power Electron. 2015, 30, 6524–6527. [Google Scholar] [CrossRef]
- Zhang, J.; Zhao, C.; Zhao, S.; Wu, X. A family of single-phase hybrid step-down PFC converters. IEEE Trans. Power Electron. 2017, 32, 5271–5281. [Google Scholar] [CrossRef]
- Lange, A.D.B.; Soeiro, T.B.; Ortmann, M.S.; Heldwein, M.L. Three-level single-phase bridgeless PFC rectifiers. IEEE Trans. Power Electron. 2015, 30, 2935–2949. [Google Scholar] [CrossRef]
- Liu, Y.; Sun, Y.; Su, M.; Zhou, M.; Zhu, Q.; Li, X. A Single-Phase PFC Rectifier with Wide Output Voltage and Low-Frequency Ripple Power Decoupling. IEEE Trans. Power Electron. 2018, 33, 5076–5086. [Google Scholar] [CrossRef]
- Akagi, H. Modern active filters and traditional passive filters. Bull. Pol. Acad. Sci. Tech. Sci. 2006, 54, 255–269. [Google Scholar]
- Wu, C.J.; Chiang, J.C.; Yen, S.S.; Liao, C.J.; Yang, J.S.; Guo, T.Y. Investigation and mitigation of harmonic amplification problems caused by single-tuned filters. IEEE Trans. Power Deliv. 1998, 13, 800–806. [Google Scholar] [CrossRef]
- Bhattacharya, S.; Cheng, P.T.; Divan, D.M. Hybrid solutions for improving passive filter performance in high power applications. IEEE Trans. Ind. Appl. 1997, 33, 732–747. [Google Scholar] [CrossRef]
- Peng, F.Z. Harmonic sources and filtering approaches. IEEE Ind. Appl. Mag. 2001, 7, 18–25. [Google Scholar] [CrossRef]
- Salam, Z.; Tan, P.C.; Jusoh, A. Harmonic’s mitigation using active power filter: A technological review. Elektr. J. Electr. Eng. 2006, 8, 17–26. [Google Scholar]
- Bhattacharya, S.; Frank, T.M.; Divan, D.M.; Banerjee, B. Active filter system implementation. IEEE Ind. Appl. Mag. 1998, 4, 47–63. [Google Scholar] [CrossRef]
- Akagi, H. Active harmonic filters. Proc. IEEE 2005, 93, 2128–2141. [Google Scholar] [CrossRef]
- Akagi, H. New trends in active filters for power conditioning. IEEE Trans. Ind. Appl. 1996, 32, 1312–1322. [Google Scholar] [CrossRef]
- Mohan, N.; Peterson, H.A.; Long, W.F.; Dreifuerst, G.R.; Vithayathil, J.J. Active filters for AC harmonic suppression. In Proceedings of the IEEE Power Engineering Society Winter Meeting, New York, NY, USA, 30 January–4 February 1977. [Google Scholar]
- Tuyen, N.D.; Fujita, G. PV-active power filter combination supplies power to nonlinear load and compensates utility current. IEEE Power Energy Technol. Syst. J. 2015, 2, 32–42. [Google Scholar] [CrossRef]
- Javadi, A.; Hamadi, A.; Woodward, L.; Al-Haddad, K. Experimental investigation on a hybrid series active power compensator to improve power quality of typical households. IEEE Trans. Ind. Electron. 2016, 63, 4849–4859. [Google Scholar] [CrossRef]
- Bubshait, A.S.; Mortezaei, A.; Simões, M.G.; Busarello, T.D.C. Power quality enhancement for a grid connected wind turbine energy system. IEEE Trans. Ind. Appl. 2017, 53, 2495–2505. [Google Scholar] [CrossRef]
- Carpinelli, G.; Proto, D.; Russo, A. Optimal Planning of Active Power Filters in a Distribution System Using Trade-off/Risk Method. IEEE Trans. Power Deliv. 2017, 32, 841–851. [Google Scholar] [CrossRef]
- He, J.; Li, Y.W.; Blaabjerg, F.; Wang, X. Active harmonic filtering using current-controlled, grid-connected DG units with closed-loop power control. IEEE Trans. Power Electron. 2014, 29, 642–653. [Google Scholar] [CrossRef]
- Darwish, M.K.; El-Habrouk, M.; Kasikci, I. EMC compliant harmonic and reactive power compensation using passive filter cascaded with shunt active filter. EPE J. 2002, 12, 43–50. [Google Scholar] [CrossRef]
- Javadi, A.; Al-Haddad, K. A single-phase active device for power quality improvement of electrified transportation. IEEE Trans. Ind. Electron. 2015, 62, 3033–3041. [Google Scholar] [CrossRef]
- Antchev, M.H. Classical and Recent Aspects of Active Power Filters for Power Quality Improvement. In Classical and Recent Aspects of Power System Optimization; Academic Press: Cambridge, MA, USA, 2018; pp. 219–254. [Google Scholar]
- Ko, W.H.; Gu, J.C. Impact of shunt active harmonic filter on harmonic current distortion of voltage source inverter-fed drives. IEEE Trans. Ind. Appl. 2016, 52, 2816–2825. [Google Scholar] [CrossRef]
- Samadaei, E.; Lesan, S.; Cherati, S.M. A new schematic for hybrid active power filter controller. In Proceedings of the 2011 IEEE Applied Power Electronics Colloquium (IAPEC), Johor Bahru, Malaysia, 18–19 April 2011; pp. 143–148. [Google Scholar] [CrossRef]
- Samedaei, E.; Vahedi, H.; Sheikholeslami, A.; Lesan, S. Using “STF-PQ” algorithm and hysteresis current control in hybrid active power filter to eliminate source current harmonic. In Proceedings of the 2010 First Power Quality Conferance (PQC), Tehran, Iran, 14–15 September 2010; pp. 1–6. [Google Scholar]
- Singh, B.; Solanki, J. An implementation of an adaptive control algorithm for a three-phase shunt active filter. IEEE Trans. Ind. Electron. 2009, 56, 2811–2820. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, Q.; Yao, W.; Liu, J. A series active power filter adopting hybrid control approach. IEEE Trans. Power Electron. 2001, 16, 301–310. [Google Scholar] [CrossRef]
- Kanjiya, P.; Khadkikar, V.; Zeineldin, H.H. Optimal control of shunt active power filter to meet IEEE Std. 519 current harmonic constraints under nonideal supply condition. IEEE Trans. Ind. Electron. 2015, 62, 724–734. [Google Scholar] [CrossRef]
- Saribulut, L.; Teke, A.; Tümay, M. Artificial neural network-based discrete-fuzzy logic controlled active power filter. IET Power Electron. 2014, 7, 1536–1546. [Google Scholar] [CrossRef]
- Suresh, Y.; Panda, A.K.; Suresh, M. Real-time implementation of adaptive fuzzy hysteresis-band current control technique for shunt active power filter. IET Power Electron. 2012, 5, 1188–1195. [Google Scholar] [CrossRef]
- Antoniewicz, K.; Jasinski, M. Experimental comparison of hysteresis based control and finite control state set Model Predictive Control of Shunt Active Power Filter. In Proceedings of the 2015 Selected Problems of Electrical Engineering and Electronics (WZEE), Kielce, Poland, 17–19 September 2015; pp. 1–6. [Google Scholar] [CrossRef]
- Zhivich, M.; Cunningham, R.K. The Real Cost of Software Errors. IEEE Secur. Priv. 2009, 7, 87–90. [Google Scholar] [CrossRef]
- Williamson, G.F. Software safety and reliability. IEEE Potentials 1997, 16, 32–36. [Google Scholar] [CrossRef]
- De Almeida, J.R.; Camargo, J.B.; Cugnasca, P.S. Software Safety in Subway and Air Traffic Control Applications. IEEE Latin Am. Trans. 2008, 6, 106–113. [Google Scholar] [CrossRef]
- Mosalikanti, P.; Kurd, N.; Mozak, C.; Oshita, T. Low power analog circuit techniques in the 5th generation intel core TM microprocessor (broadwell). In Proceedings of the 2015 IEEE Custom Integrated Circuits Conference (CICC), San Jose, CA, USA, 28–30 September 2015; pp. 1–4. [Google Scholar] [CrossRef]
- Caloz, C.; Gupta, S.; Zhang, Q.; Nikfal, B. Analog signal processing: A possible alternative or complement to dominantly digital radio schemes. IEEE Microw. Mag. 2013, 14, 87–103. [Google Scholar] [CrossRef]
- Gonzalez-Diaz, V.R.; Peña-Perez, A.; Maloberti, F. Opamp gain compensation technique for continuous-time ΣΔ modulators. Electron. Lett. 2014, 50, 355–356. [Google Scholar] [CrossRef]
- Millman, J. Microelectronics: Digital and Analog Circuits and Systems; Entire Document; McGraw-Hill: New York, NY, USA, 1979; pp. 523–527. ISBN 0-07-042327-X. [Google Scholar]
- Mao, H.; Yang, X.; Chen, Z.; Wang, Z. A hysteresis current controller for single-phase three-level voltage source inverters. IEEE Trans. Power Electron. 2012, 27, 3330–3339. [Google Scholar] [CrossRef]
- Antchev, M.; Petkova, M.; Petkov, M. Single-phase shunt active power filter using frequency limitation and hysteresis current control. In Proceedings of the Power Conversion Conference (PCC’07), Nagoya, Japan, 2–5 April 2007; pp. 97–102. [Google Scholar] [CrossRef]
- Lipiansky, E. Ch5. In The Operational Amplifier as a Circuit Element; Electrical, Electronics and Digital Hardware Essentials for Scientists and Engineers; Wiley-IEEE Press: Hoboken, NJ, USA, 2013; Volume 1, pp. 287–353. ISBN 9781118414552. [Google Scholar]
- Kazmierkowski, M.P.; Malesani, L. Current control techniques for three-phase voltage source PWM converters: A survey. IEEE Trans. Ind. Electron. 1998, 45, 691–703. [Google Scholar] [CrossRef]


| Parameters | Magnitude |
|---|---|
| Power | 3 kW |
| Vgrid | 220 v |
| Rgrid | 1 Ω |
| Lgrid | 600 μH |
| Rload | 1 Ω |
| Lload | 10 mH |
| CAPF | 680 μF |
| LAPF | 300 mH |
| VDC Link | 310 v |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Samadaei, E.; Iranian, M.; Rezanejad, M.; Godina, R.; Pouresmaeil, E. Single-Phase Active Power Harmonics Filter by Op-Amp Circuits and Power Electronics Devices. Sustainability 2018, 10, 4406. https://doi.org/10.3390/su10124406
Samadaei E, Iranian M, Rezanejad M, Godina R, Pouresmaeil E. Single-Phase Active Power Harmonics Filter by Op-Amp Circuits and Power Electronics Devices. Sustainability. 2018; 10(12):4406. https://doi.org/10.3390/su10124406
Chicago/Turabian StyleSamadaei, Emad, Mina Iranian, Mohammad Rezanejad, Radu Godina, and Edris Pouresmaeil. 2018. "Single-Phase Active Power Harmonics Filter by Op-Amp Circuits and Power Electronics Devices" Sustainability 10, no. 12: 4406. https://doi.org/10.3390/su10124406
APA StyleSamadaei, E., Iranian, M., Rezanejad, M., Godina, R., & Pouresmaeil, E. (2018). Single-Phase Active Power Harmonics Filter by Op-Amp Circuits and Power Electronics Devices. Sustainability, 10(12), 4406. https://doi.org/10.3390/su10124406







