Proposing a Hybrid Thermal Management System Based on Phase Change Material/Metal Foam for Lithium-Ion Batteries
Abstract
:1. Introduction
- To Analyze the performance of the BTMS using active and passive cooling techniques under forced air and paraffin/aluminum composite.
- To assess the effect of PCM on the BTMS heat dissipation performance, the thickness of PCM is proposed. A more reasonable optimal PCM for the BTMS is also suggested.
- To prevent thermal runaway, the distance between the cells and the airflow has been studied as factors that contribute to maintaining a uniform temperature distribution in the cells.
- PCM thermal conductivity and phase transition temperature are investigated in detail for paraffin and paraffin/Al foams to determine the battery temperature and PCM utilization.
| Research | Ref. | Year | Elements of Cooling System | Battery Type | C-Rate | ||
|---|---|---|---|---|---|---|---|
| Yoongi et al. | [32] | 2017 | PCM + Forced air | Prismatic | 4C | 322.3 | 3.2 |
| Qin et al. | [33] | 2019 | PCM + Forced air | Cylindrical | 3C | 289.1 | 1.2 |
| Kermani et al. | [31] | 2019 | PCM + Copper foam | Pouchy | 5C | 308.9 | 4.6 |
| Zhang et al. | [25] | 2021 | PCM | Prismatic | 5C | 314.5 | 2.5 |
| Lamrani et al. | [28] | 2021 | PCM | Cylindrical | 3C | 305.1 | - |
| Yang et al. | [34] | 2022 | Forced air | Prismatic | 4C | 324.8 | 2.8 |
| Current study | - | - | PCM + Aluminum foam + Forced air | Cylindrical | 5C | 308.1 | 2 |
2. Materials and Methods
2.1. Modelling and Governing Equations
- The fluid flow is incompressible, and the flow regime is laminar and turbulent for different fluid velocities.
- Radiative heat transfer is neglected.
- The Boussinesq approximation estimates natural convection due to buoyancy.
- The metal foam is assumed to be isotropic and homogeneous.
- Volume expansion is neglected.
2.1.1. Battery
2.1.2. Coolant
2.1.3. Phase Change Material
2.2. Boundary and Initial Conditions
- The battery cells are fully charged.
- Initially, all module components have a temperature equal to the ambient temperature (24.5 °C).
- The cell heat generated is considered at 5C.
- The convective heat transfer coefficient between the battery and the environment equals 5 (W/m·K).
- The bottom wall of the battery module is thermally insulated.
- This study investigates five different cooling air temperatures (10, 15, 20, 24.5, and 30 °C) to dissipate heat and create suitable working conditions.
3. Numerical Method and Mesh Independency
4. Validation of Numerical Model
5. Results and Discussion
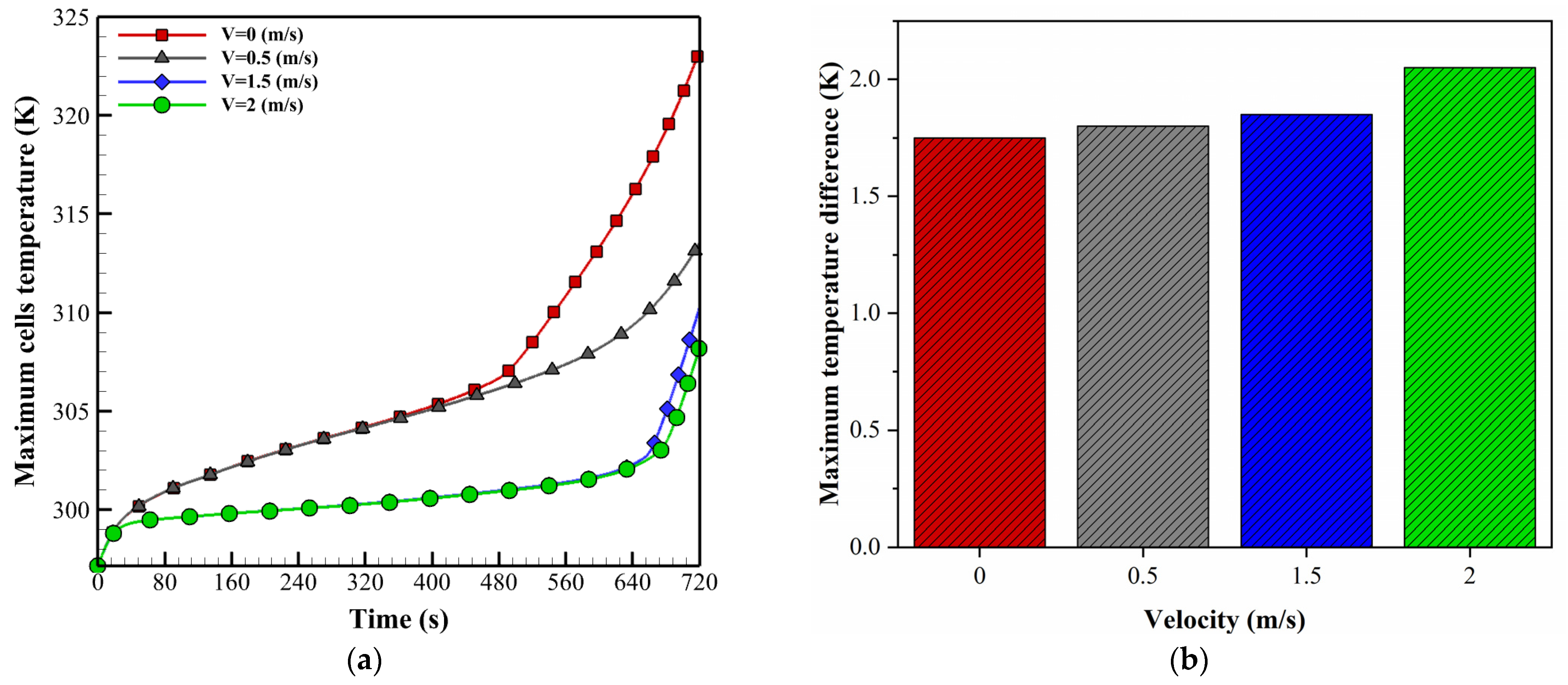
5.1. Effect of Air Inlet Velocity
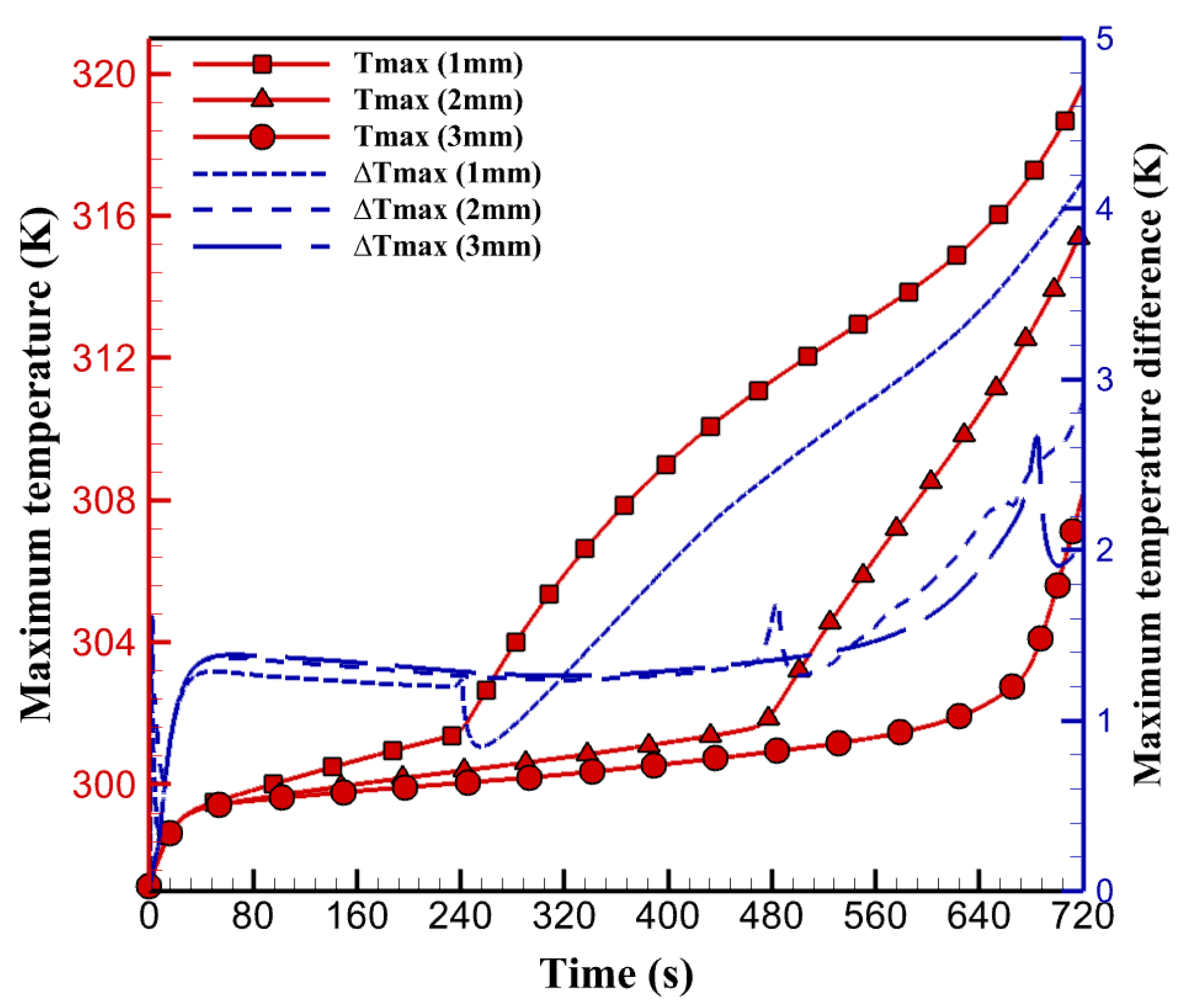
5.2. The Effect of PCM Thickness
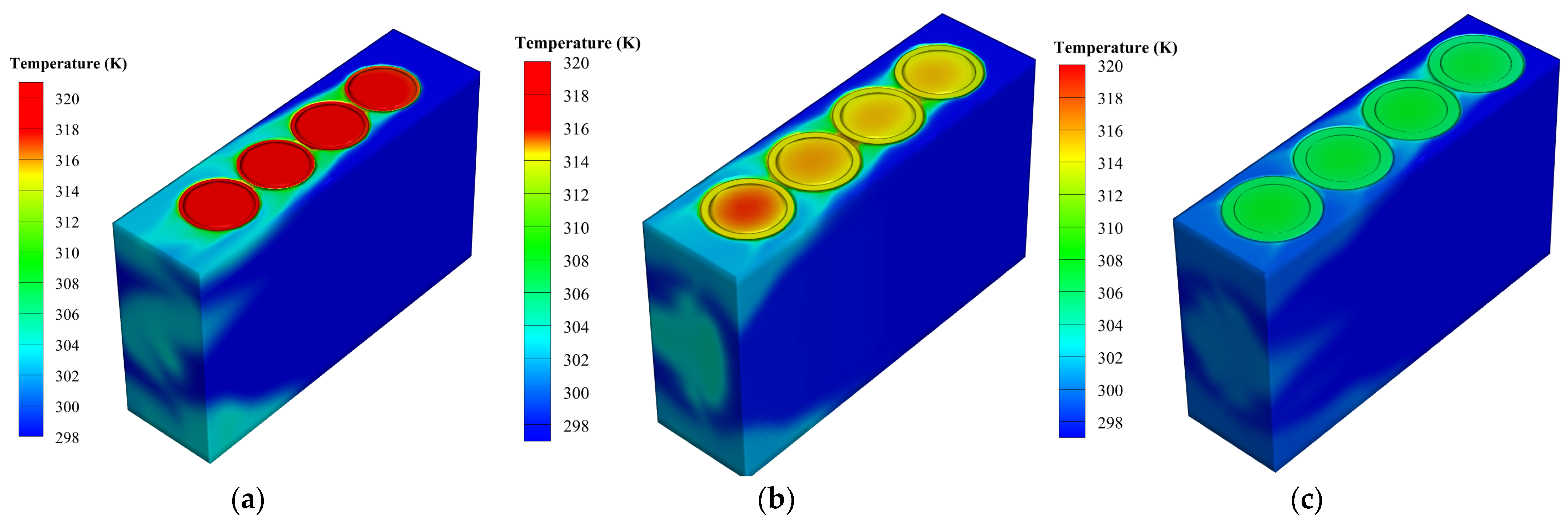
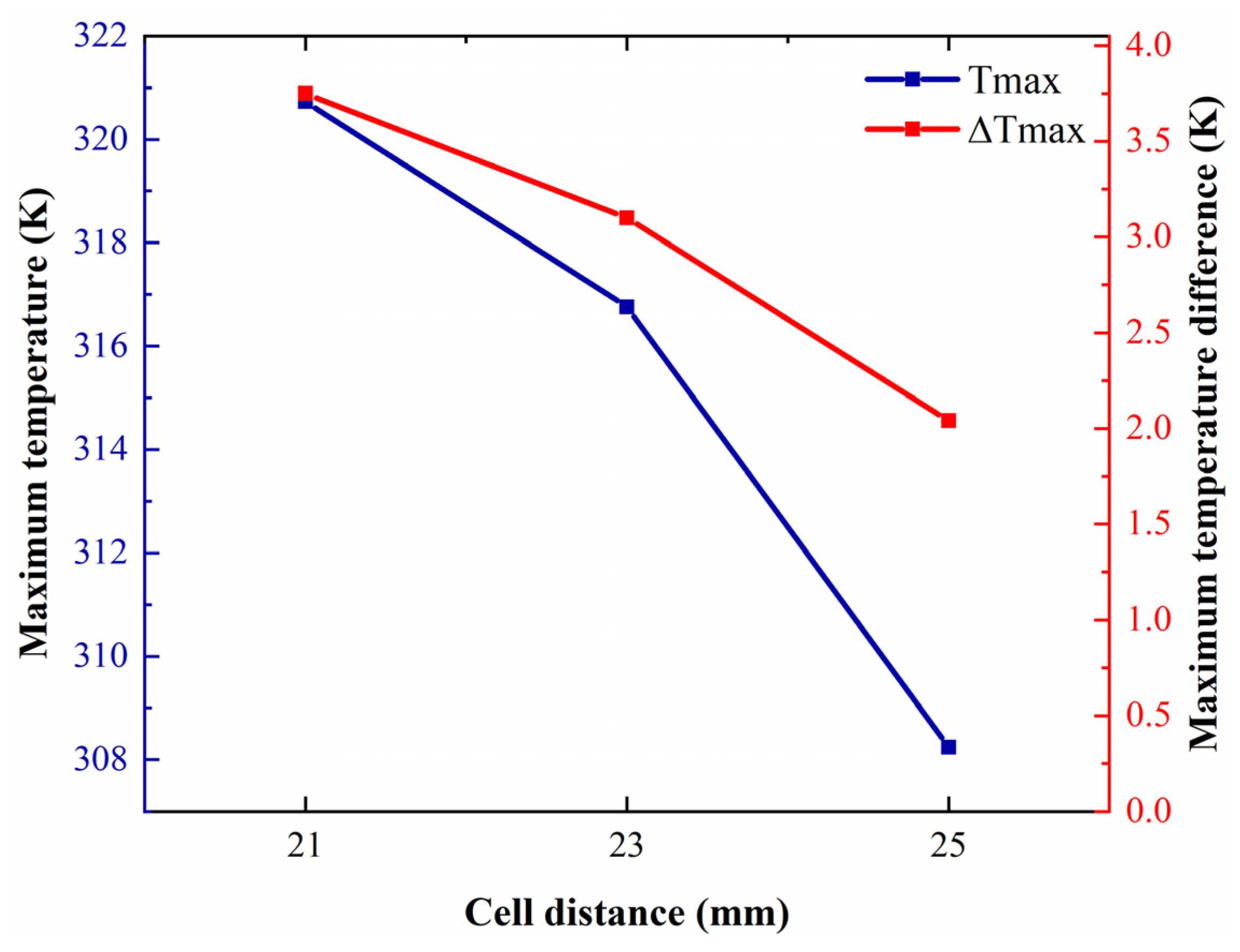
5.3. Effect of Cell Distance
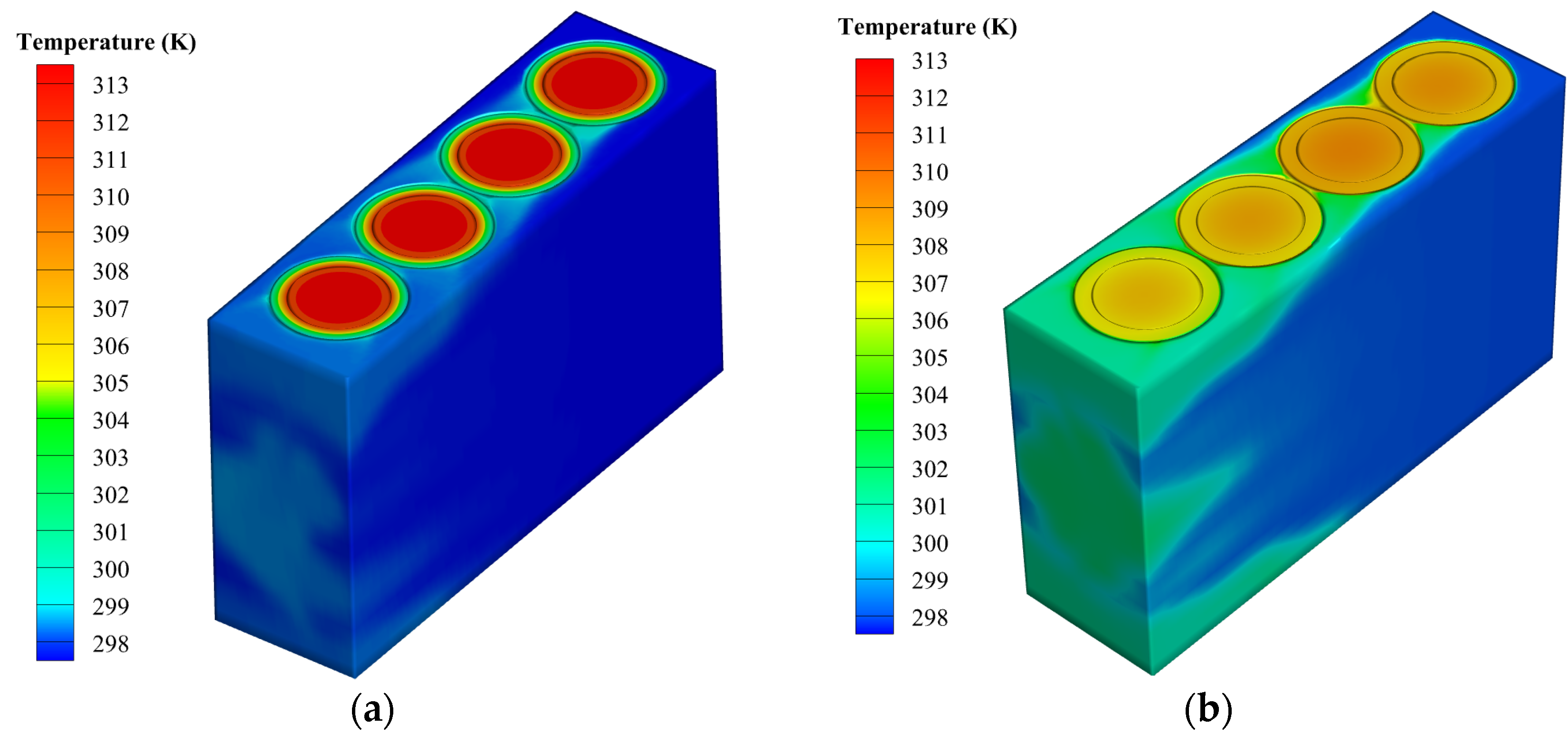
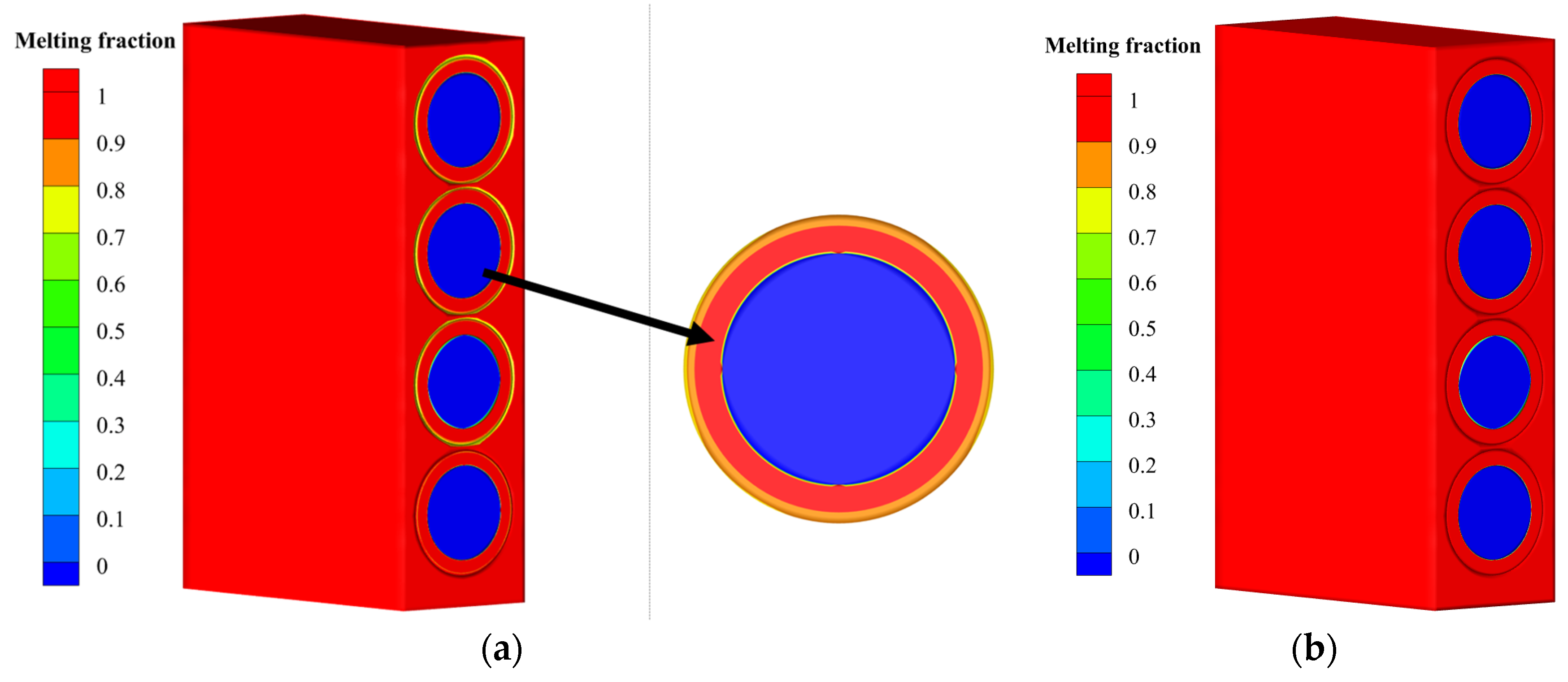
5.4. Impact of Metal Foam
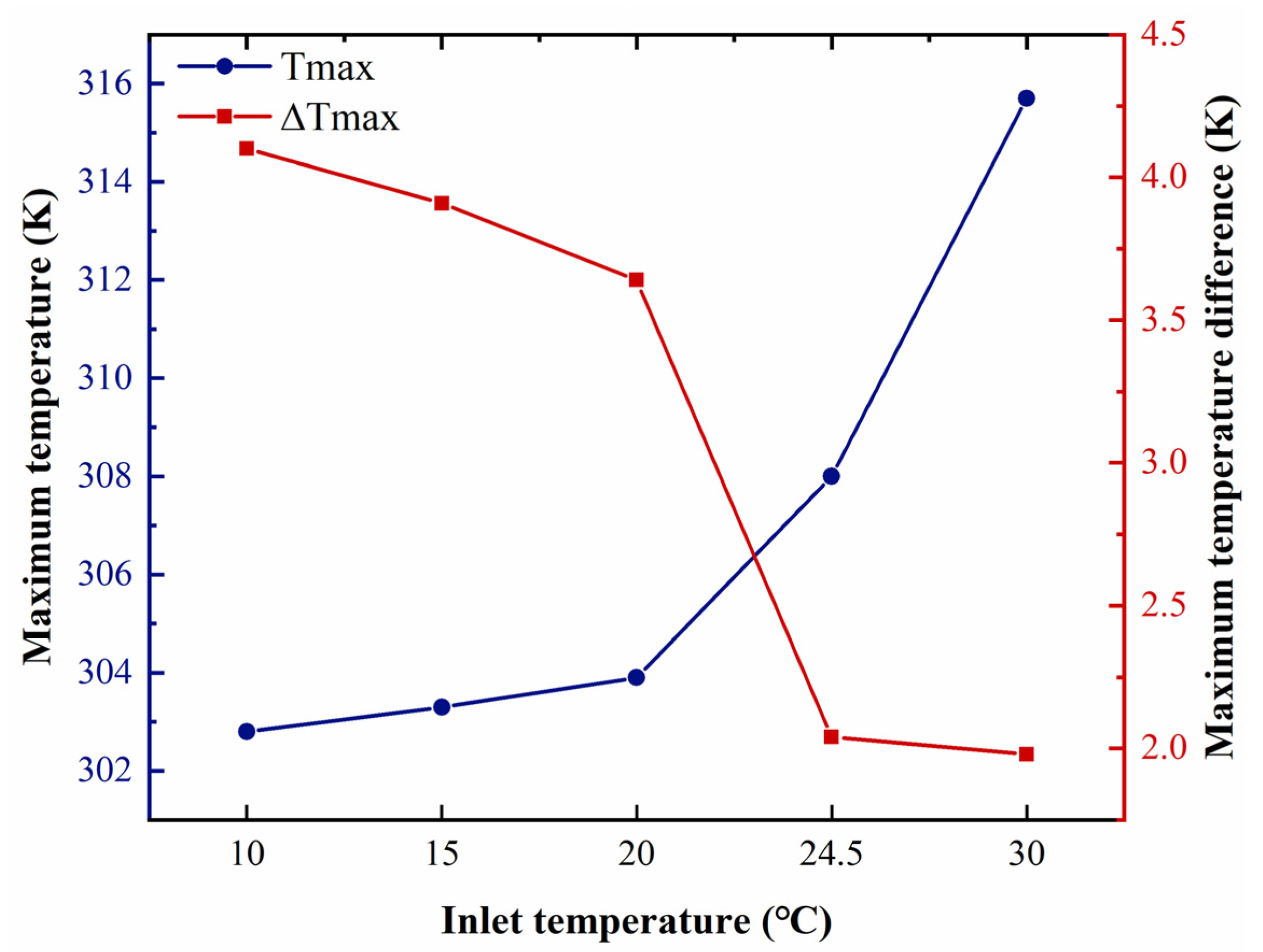
5.5. Effect of Air Inlet Temperature
6. Conclusions
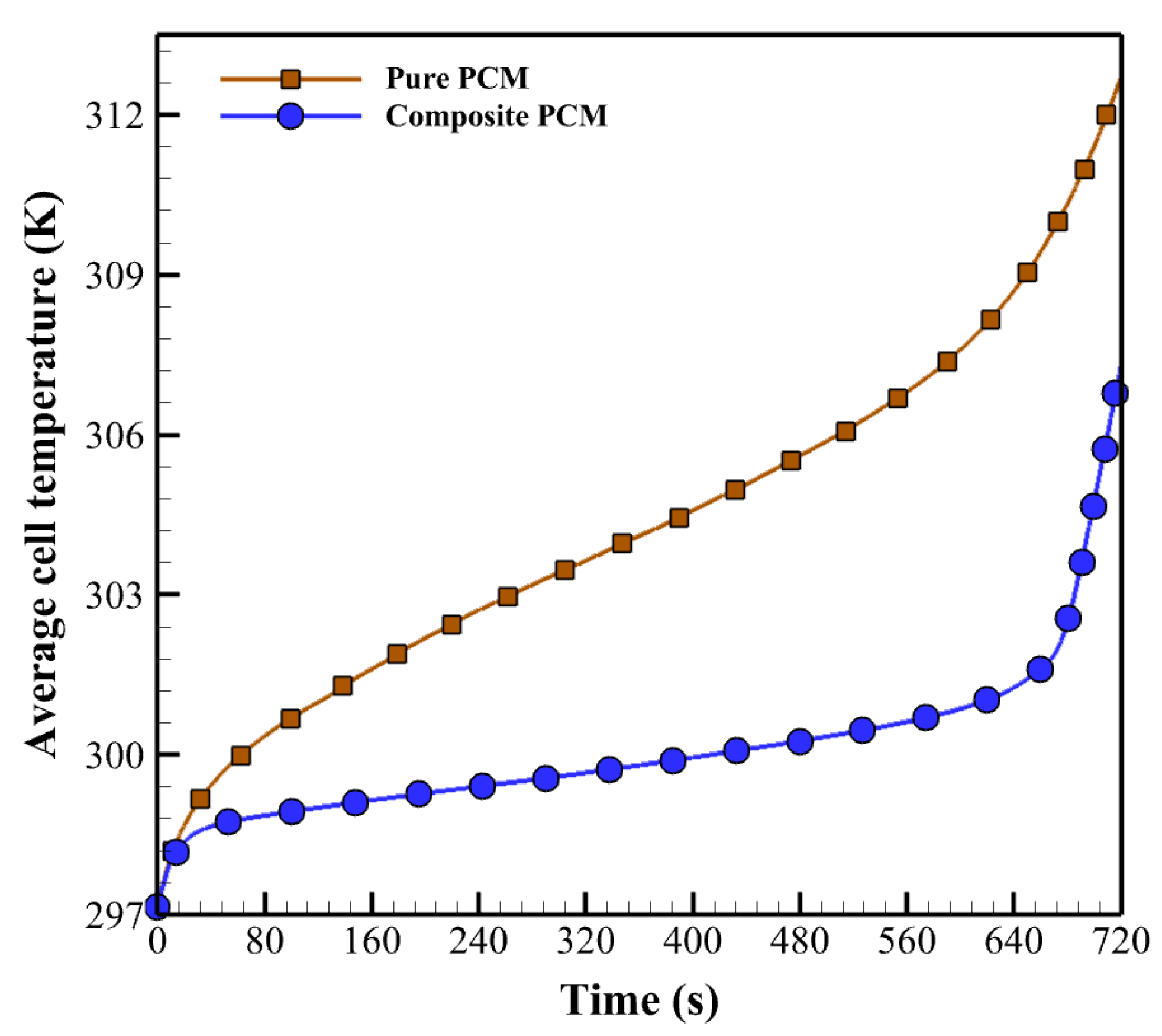
- The paraffin/aluminum composite provides better thermal performance than paraffin alone for battery packs, since the high thermal conductivity of paraffin can transfer the heat produced by the batteries to the PCM and surrounding environment. As a result, the temperature distribution becomes more uniform, and the temperature decreases.
- With an increased air temperature, the temperature increases, followed by a decrease in the temperature difference. The proposed system can maintain the uniformity of temperature and the temperature in the optimal range for the battery under a cooling temperature of 10 to 24.5 °C.
- Increasing the thickness of paraffin can affect the efficiency of the BTMS. The proposed system is a paraffin/aluminum composite with a thickness of 3 mm. Increasing the thickness and combining aluminum foam with paraffin improves the capacity for temperature control in the thermal management system and maintains the cell temperature at 35 °C.
- Increasing the inlet air velocity improves temperature control, but the uniformity of the battery pack temperature worsens. Under a discharge rate of 5C and an environment temperature of 24.5 °C, with an inlet speed of 2 m/s, the proposed system can maintain the temperature difference and maximum temperature at 2.04 K and 308 K, respectively.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| C: | Discharge rate (C) |
| H: | |
| : | |
| : | |
| : | |
| : | |
| : | |
| S: | Heat source |
| k: | Thermal conductivity (W/m·K) |
| : | Air thermal conductivity (W/m·K) |
| K: | Turbulent kinetic energy (m2/s2) |
| : | Turbulence production |
| : | Empirical parameters |
| : | Parameters of the turbulence model |
| R: | |
| : | Temperature coefficient of open-circuit voltage (V/K) |
| : | Open-circuit voltage (V) |
| : | Battery voltage (V) |
| I: | Cell charge/discharge current (A) |
| m: | Mass (kg) |
| : | Battery mass (kg) |
| L: | Characteristic length |
| : | Volume (m3) |
| : | Battery volume (m3) |
| : | Specific heat capacity (J/kg·K) |
| : | Battery specific heat capacity (J/kg·K) |
| : | Air specific heat capacity (J/kg·K) |
| T: | Temperature (K) |
| : | Air temperature (K) |
| : | Melting temperature (K) |
| : | Solidus temperature (K) |
| : | Maximum temperature (K) |
| : | Maximum temperature difference (K) |
| t: | Time (s) |
| V: | Velocity (m/s) |
| Re: | Reynolds number |
| Greek symbols | |
| : | Melting fraction of phase change material |
| : | |
| : | ) |
| : | ) |
| : | ) |
| : | Viscosity (kg·m/S) |
| : | Air viscosity (kg·m/S) |
| : | Turbulent viscosity coefficient |
| ε: | Turbulent dissipation rate |
| : | |
| Abbreviations | |
| BTMS: | Battery thermal management system |
| PCM: | Phase change materials |
| SOC: | State of charge |
| PPI: | Pores per inch |
| LIB: | Lithium-ion battery |
| TMS: | Thermal management system |
| CFD: | Computational fluid dynamics |
| UDF: | User-defined function |
References
- Han, X.; Ouyang, M.; Lu, L.; Li, J.; Zheng, Y.; Li, Z. A Comparative Study of Commercial Lithium-Ion Battery Cycle Life in Electrical Vehicle: Aging Mechanism Identification. J. Power Sources 2014, 251, 38–54. [Google Scholar] [CrossRef]
- Liu, X.; Li, K.; Wu, J.; He, Y.; Liu, X. An Extended Kalman Filter Based Data-Driven Method for State of Charge Estimation of Li-Ion Batteries. J. Energy Storage 2021, 40, 102655. [Google Scholar] [CrossRef]
- Lin, M.; Zeng, X.; Wu, J. State of Health Estimation of Lithium-Ion Battery Based on an Adaptive Tunable Hybrid Radial Basis Function Network. J. Power Source 2021, 504, 230063. [Google Scholar] [CrossRef]
- Ravdel, B.; Abraham, K.M.; Gitzendanner, R.; DiCarlo, J.; Lucht, B.; Campion, C. Thermal Stability of Lithium-Ion Battery Electrolytes. J. Power Source 2003, 119–121, 805–810. [Google Scholar] [CrossRef]
- Zhang, S.S.; Xu, K.; Jow, T.R. The Low Temperature Performance of Li-Ion Batteries. J. Power Source 2003, 115, 137–140. [Google Scholar] [CrossRef]
- Mehrenjani, J.R.; Gharehghani, A.; Nasrabadi, A.M.; Moghimi, M. Design, Modeling and Optimization of a Renewable-Based System for Power Generation and Hydrogen Production. Int. J. Hydrogen Energy 2022, 47, 14225–14242. [Google Scholar] [CrossRef]
- Ali, H.M. Thermal Management Systems for Batteries in Electric Vehicles: A Recent Review. Energy Rep. 2023, 9, 5545–5565. [Google Scholar] [CrossRef]
- Moradi, J.; Gharehghani, A.; Mirsalim, M. Numerical Comparison of Combustion Characteristics and Cost between Hydrogen, Oxygen and Their Combinations Addition on Natural Gas Fueled HCCI Engine. Energy Convers. Manag. 2020, 222, 113254. [Google Scholar] [CrossRef]
- Moradi, J.; Gharehghani, A.; Mirsalim, M. Numerical Investigation on the Effect of Oxygen in Combustion Characteristics and to Extend Low Load Operating Range of a Natural-Gas HCCI Engine. Appl. Energy 2020, 276, 115516. [Google Scholar] [CrossRef]
- Jiang, Z.Y.; Li, H.B.; Qu, Z.G.; Zhang, J.F. Recent Progress in Lithium-Ion Battery Thermal Management for a Wide Range of Temperature and Abuse Conditions. Int. J. Hydrogen Energy 2022, 47, 9428–9459. [Google Scholar] [CrossRef]
- Wu, M.S.; Liu, K.H.; Wang, Y.Y.; Wan, C.C. Heat Dissipation Design for Lithium-Ion Batteries. J. Power Source 2002, 109, 160–166. [Google Scholar] [CrossRef]
- Wang, T.; Tseng, K.J.; Zhao, J. Development of Efficient Air-Cooling Strategies for Lithium-Ion Battery Module Based on Empirical Heat Source Model. Appl. Therm. Eng. 2015, 90, 521–529. [Google Scholar] [CrossRef]
- Chen, K.; Chen, Y.; Li, Z.; Yuan, F.; Wang, S. Design of the Cell Spacings of Battery Pack in Parallel Air-Cooled Battery Thermal Management System. Int. J. Heat Mass Transf. 2018, 127, 393–401. [Google Scholar] [CrossRef]
- Fan, Y.; Bao, Y.; Ling, C.; Chu, Y.; Tan, X.; Yang, S. Experimental Study on the Thermal Management Performance of Air Cooling for High Energy Density Cylindrical Lithium-Ion Batteries. Appl. Therm. Eng. 2019, 155, 96–109. [Google Scholar] [CrossRef]
- Kirad, K.; Chaudhari, M. Design of Cell Spacing in Lithium-Ion Battery Module for Improvement in Cooling Performance of the Battery Thermal Management System. J. Power Source 2021, 481, 229016. [Google Scholar] [CrossRef]
- Abdulrasool Hasan, H.; Togun, H.; Abed, A.M.; Mohammed, H.I.; Biswas, N. A Novel Air-Cooled Li-Ion Battery (LIB) Array Thermal Management System–a Numerical Analysis. Int. J. Therm. Sci. 2023, 190, 108327. [Google Scholar] [CrossRef]
- Rabiei, M.; Gharehghani, A.; Andwari, A.M. Enhancement of Battery Thermal Management System Using a Novel Structure of Hybrid Liquid Cold Plate. Appl. Therm. Eng. 2023, 232, 121051. [Google Scholar] [CrossRef]
- Liu, Z.; Gao, Y.; Chen, H.; Wang, C.; Sun, Y.; Yan, P. Thermal Performance of Lithium Titanate Oxide Anode Based Battery Module under High Discharge Rates. World Electr. Veh. J. 2021, 12, 158. [Google Scholar] [CrossRef]
- Sha, Y.; Zhang, Z.; Chen, Y.; Lin, Q.; Zhong, Y.; Xu, X.; Shao, Z. One-Pot Combustion Synthesis of Li3VO4-Li4Ti5O12 Nanocomposite as Anode Material of Lithium-Ion Batteries with Improved Performance. Electrochim. Acta 2016, 222, 587–595. [Google Scholar] [CrossRef]
- Kshetrimayum, K.S.; Yoon, Y.G.; Gye, H.R.; Lee, C.J. Preventing Heat Propagation and Thermal Runaway in Electric Vehicle Battery Modules Using Integrated PCM and Micro-Channel Plate Cooling System. Appl. Therm. Eng. 2019, 159, 113797. [Google Scholar] [CrossRef]
- Kizilel, R.; Lateef, A.; Sabbah, R.; Farid, M.M.; Selman, J.R.; Al-Hallaj, S. Passive Control of Temperature Excursion and Uniformity in High-Energy Li-Ion Battery Packs at High Current and Ambient Temperature. J. Power Source 2008, 183, 370–375. [Google Scholar] [CrossRef]
- Kizilel, R.; Sabbah, R.; Selman, J.R.; Al-Hallaj, S. An Alternative Cooling System to Enhance the Safety of Li-Ion Battery Packs. J. Power Source 2009, 194, 1105–1112. [Google Scholar] [CrossRef]
- Ling, Z.; Wang, F.; Fang, X.; Gao, X.; Zhang, Z. A Hybrid Thermal Management System for Lithium-Ion Batteries Combining Phase Change Materials with Forced-Air Cooling. Appl. Energy 2015, 148, 403–409. [Google Scholar] [CrossRef]
- Verma, A.; Shashidhara, S.; Rakshit, D. A Comparative Study on Battery Thermal Management Using Phase Change Material (PCM). Therm. Sci. Eng. Prog. 2019, 11, 74–83. [Google Scholar] [CrossRef]
- Zhang, W.; Liang, Z.; Ling, G.; Huang, L. Influence of Phase Change Material Dosage on the Heat Dissipation Performance of the Battery Thermal Management System. J. Energy Storage 2021, 41, 102849. [Google Scholar] [CrossRef]
- Iasiello, M.; Mameli, M.; Filippeschi, S.; Bianco, N. Metal Foam/PCM Melting Evolution Analysis: Orientation and Morphology Effects. Appl. Therm. Eng. 2021, 187, 116572. [Google Scholar] [CrossRef]
- Javani, N.; Dincer, I.; Naterer, G.F.; Yilbas, B.S. Heat Transfer and Thermal Management with PCMs in a Li-Ion Battery Cell for Electric Vehicles. Int. J. Heat Mass Transf. 2014, 72, 690–703. [Google Scholar] [CrossRef]
- Lamrani, B.; Lebrouhi, B.E.; Khattari, Y.; Kousksou, T. A Simplified Thermal Model for a Lithium-Ion Battery Pack with Phase Change Material Thermal Management System. J. Energy Storage 2021, 44, 103377. [Google Scholar] [CrossRef]
- Bais, A.R.; Subhedhar, D.G.; Joshi, N.C.; Panchal, S. Numerical Investigation on Thermal Management System for Lithium Ion Battery Using Phase Change Material. Mater. Today Proc. 2022, 66, 1726–1733. [Google Scholar] [CrossRef]
- Karimi, D.; Behi, H.; Van Mierlo, J.; Berecibar, M. Novel Hybrid Thermal Management System for High-Power Lithium-Ion Module for Electric Vehicles: Fast Charging Applications. World Electr. Veh. J. 2022, 13, 86. [Google Scholar] [CrossRef]
- Mehrabi-Kermani, M.; Houshfar, E.; Ashjaee, M. A Novel Hybrid Thermal Management for Li-Ion Batteries Using Phase Change Materials Embedded in Copper Foams Combined with Forced-Air Convection. Int. J. Therm. Sci. 2019, 141, 47–61. [Google Scholar] [CrossRef]
- Xie, Y.; Tang, J.; Shi, S.; Xing, Y.; Wu, H.; Hu, Z.; Wen, D. Experimental and Numerical Investigation on Integrated Thermal Management for Lithium-Ion Battery Pack with Composite Phase Change Materials. Energy Convers. Manag. 2017, 154, 562–575. [Google Scholar] [CrossRef]
- Qin, P.; Liao, M.; Zhang, D.; Liu, Y.; Sun, J.; Wang, Q. Experimental and Numerical Study on a Novel Hybrid Battery Thermal Management System Integrated Forced-Air Convection and Phase Change Material. Energy Convers. Manag. 2019, 195, 1371–1381. [Google Scholar] [CrossRef]
- Yang, R.; Wang, M.; Xi, H. Thermal Investigation and Forced Air-Cooling Strategy of Battery Thermal Management System Considering Temperature Non-Uniformity of Battery Pack. Appl. Therm. Eng. 2023, 219, 119566. [Google Scholar] [CrossRef]
- Cicconi, P.; Kumar, P.; Varshney, P. A Support Approach for the Modular Design of Li-Ion Batteries: A Test Case with PCM. J. Energy Storage 2020, 31, 101684. [Google Scholar] [CrossRef]
- EIIdi, M.M.M.; Karkri, M.; Andwari, M.; Vincent, S. Hybrid Cooling Based Battery Thermal Management Using Composite Phase Change Materials and Forced Convection. J. Energy Storage 2021, 41, 102946. [Google Scholar] [CrossRef]
- Gharehghani, A.; Kakoee, A.; Andwari, A.M.; Megaritis, T.; Pesyridis, A. Numerical investigation of an RCCI engine fueled with natural gas/dimethyl-ether in various injection strategies. Energies 2021, 14, 1638. [Google Scholar] [CrossRef]
- Shmueli, H.; Ziskind, G.; Letan, R. Melting in a Vertical Cylindrical Tube: Numerical Investigation and Comparison with Experiments. Int. J. Heat Mass Transf. 2010, 53, 4082–4091. [Google Scholar] [CrossRef]
- Severino, B.; Gana, F.; Palma-Behnke, R.; Estévez, P.A.; Calderón-Muñoz, W.R.; Orchard, M.E.; Reyes, J.; Cortés, M. Multi-Objective Optimal Design of Lithium-Ion Battery Packs Based on Evolutionary Algorithms. J. Power Source 2014, 267, 288–299. [Google Scholar] [CrossRef]
- Lai, Y.; Wu, W.; Chen, K.; Wang, S.; Xin, C. A Compact and Lightweight Liquid-Cooled Thermal Management Solution for Cylindrical Lithium-Ion Power Battery Pack. Int. J. Heat Mass Transf. 2019, 144, 118581. [Google Scholar] [CrossRef]
- Zhang, B.; Ren, G. Li-Ion Battery State of Charge Prediction for Electric Vehicles Based on Improved Regularized Extreme Learning Machine. World Electr. Veh. J. 2023, 14, 202. [Google Scholar] [CrossRef]
- Li, W.; Xiao, M.; Peng, X.; Garg, A.; Gao, L. A Surrogate Thermal Modeling and Parametric Optimization of Battery Pack with Air Cooling for EVs. Appl. Therm. Eng. 2019, 147, 90–100. [Google Scholar] [CrossRef]
- Sahin, R.C.; Gocmen, S.; Cetkin, E. Thermal Management System for Air-Cooled Battery Packs with Flow-Disturbing Structures. J. Power Source 2022, 551, 232214. [Google Scholar] [CrossRef]
- Jefford, C.W.; Kabengele, T.; Kovacs, J.; Burger, U. Additions of fluorocarbenes to norbornadiene by linear cheletropic reaction. Tetrahedron Lett. 1974, 3, 257–260. [Google Scholar] [CrossRef]
- Jones, B.J.; Sun, D.; Krishnan, S.; Garimella, S.V. Experimental and Numerical Study of Melting in a Cylinder. Int. J. Heat Mass Transf. 2006, 49, 2724–2738. [Google Scholar] [CrossRef]
- Fadl, M.; Eames, P. A Numerical Investigation into the Heat Transfer and Melting Process of Lauric Acid in a Rectangular Enclosure with Three Values of Wall Heat Flu. Energy Procedia 2019, 158, 4502–4509. [Google Scholar] [CrossRef]
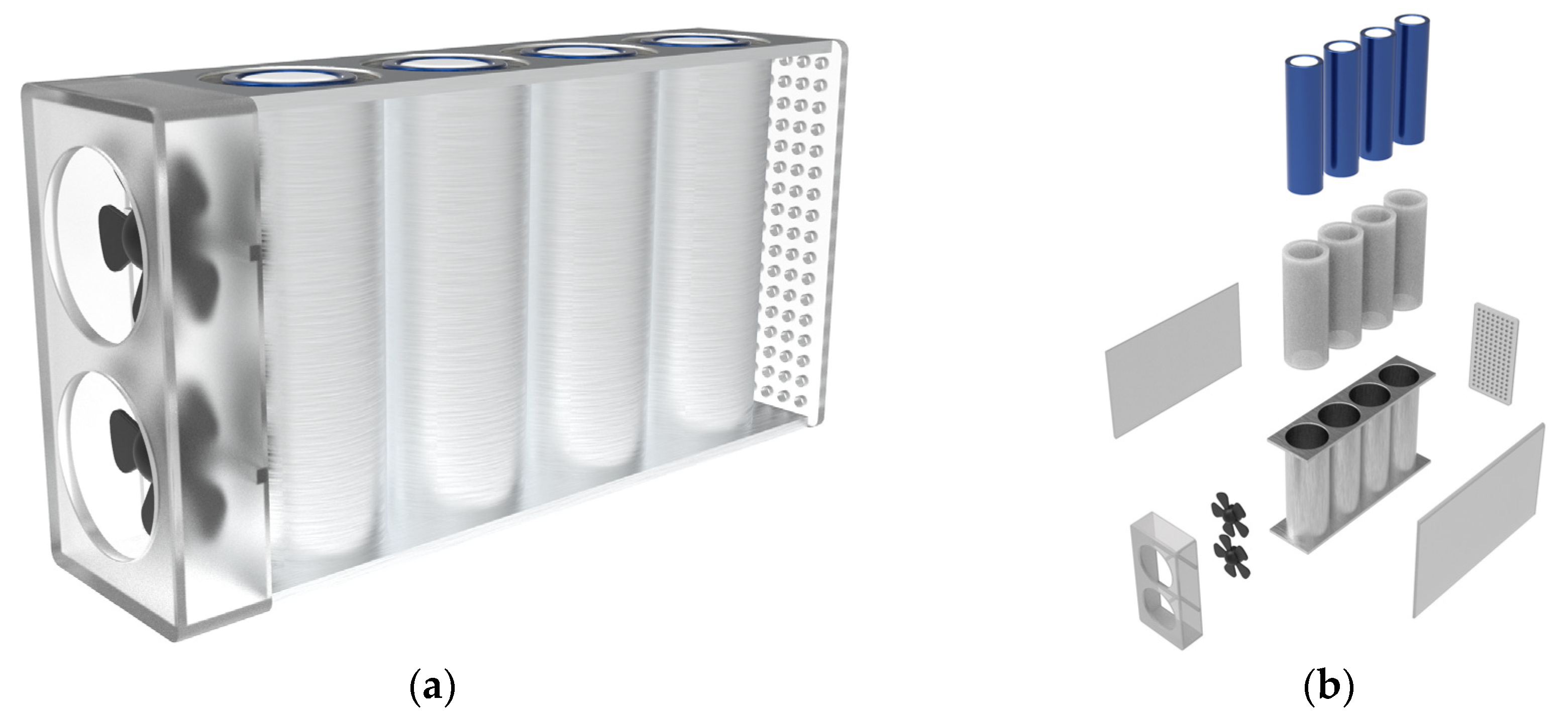
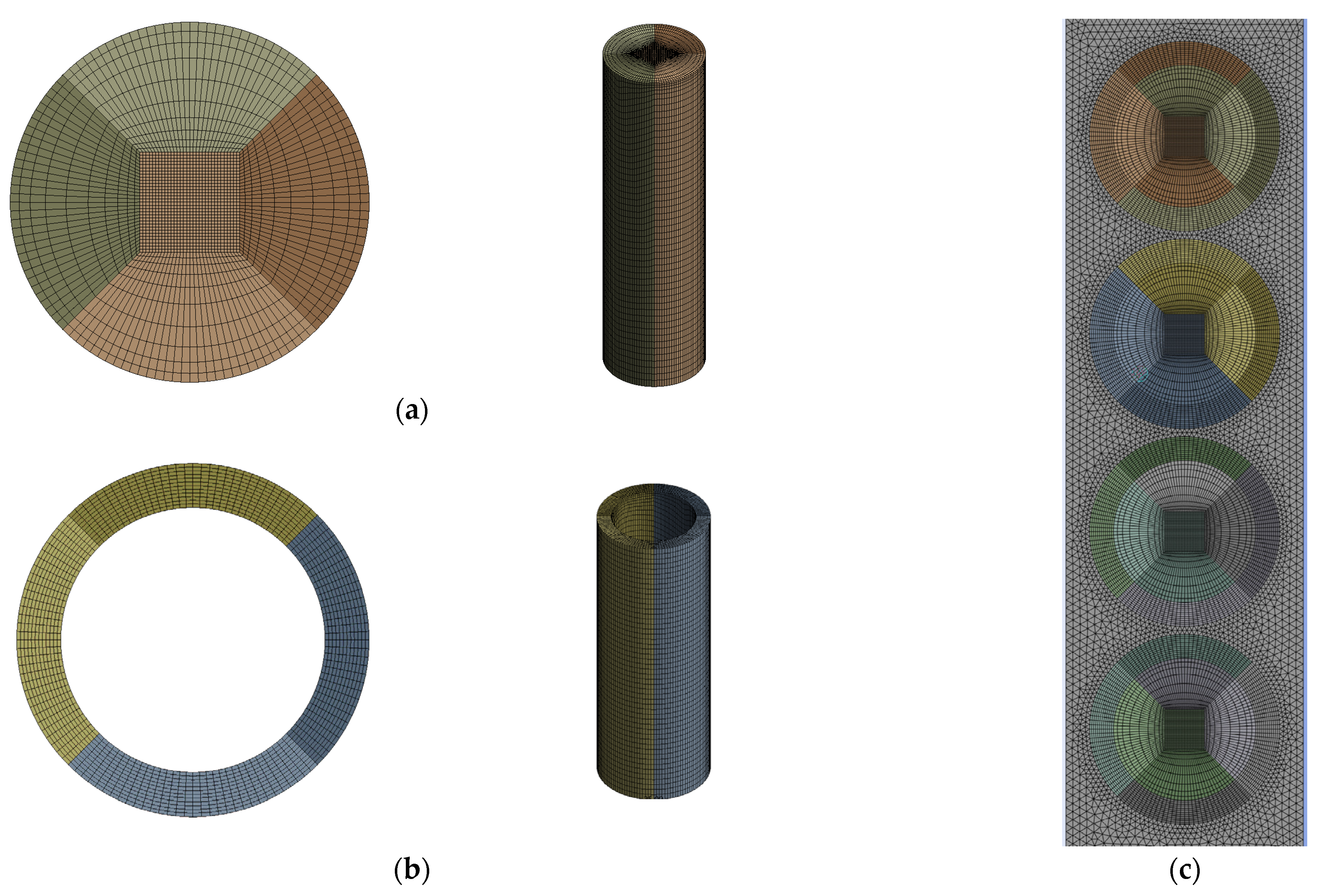
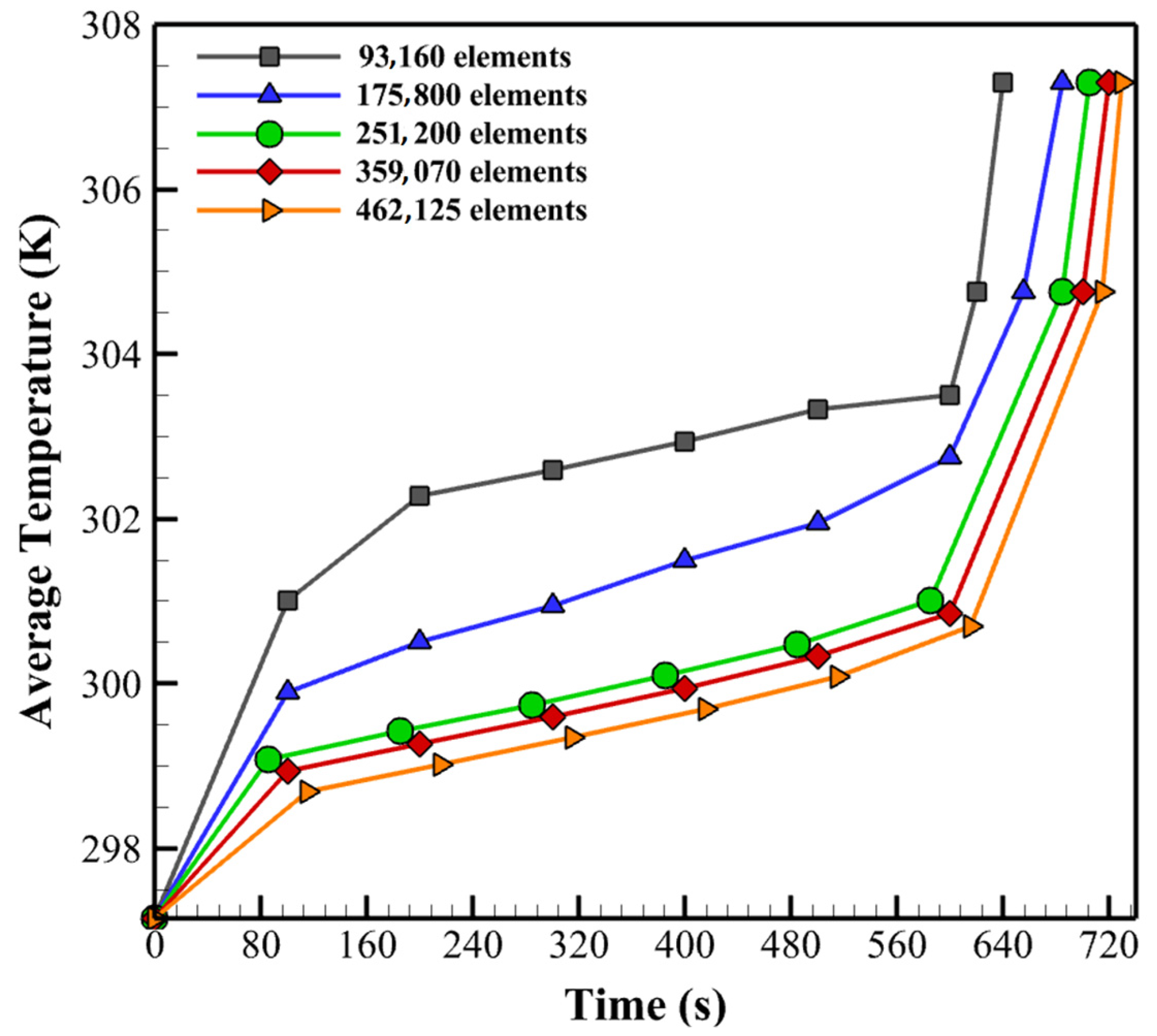
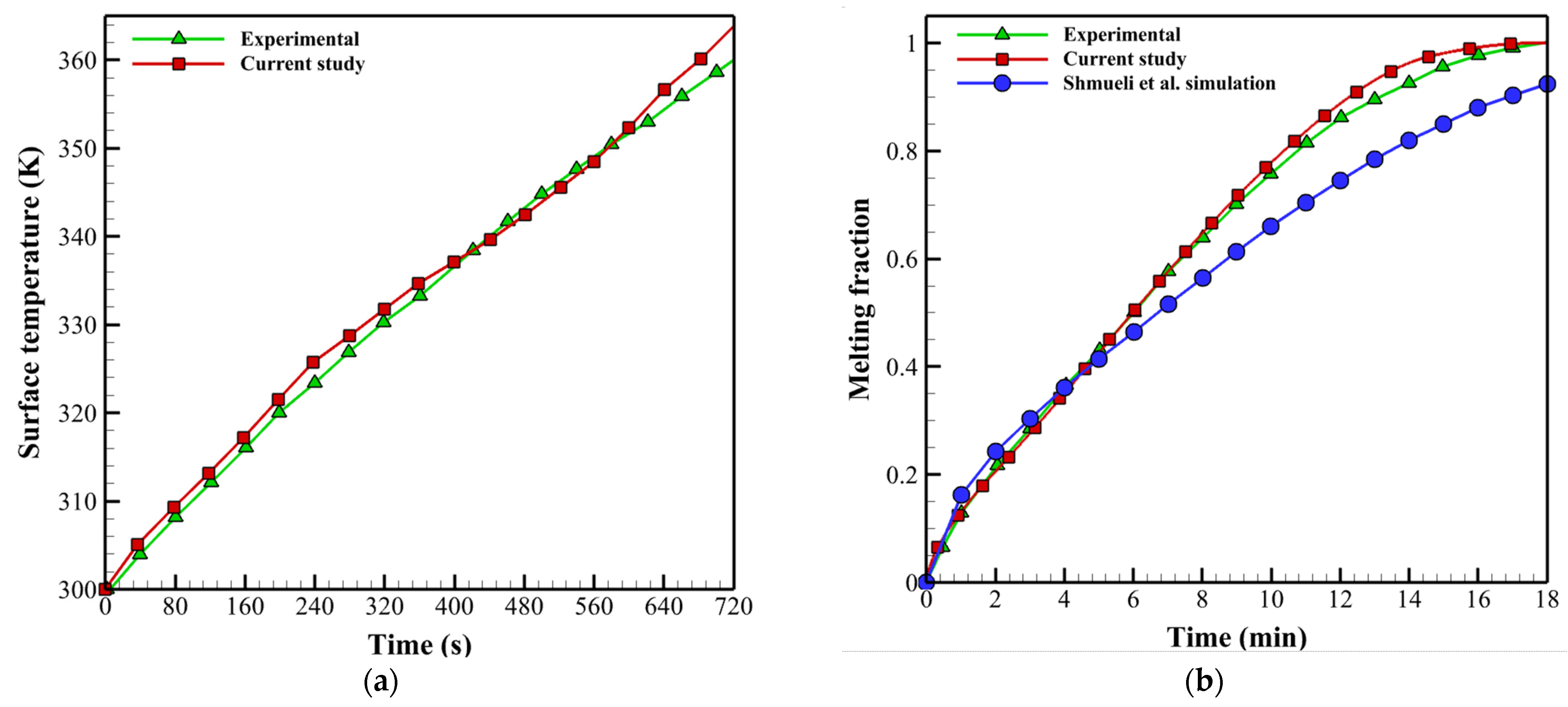
| Properties | Value |
|---|---|
| Density (kg/m3) | 2700 |
| Thermal conductivity (radial) (W/m·K) | 0.2 |
| Thermal conductivity (axial) (W/m·K) | 31.15 |
| Specific heat capacity (J/kg·K) | 1726 |
| Height (mm) | 65 |
| Diameter (mm) | 18 |
| Nominal capacity (mAh) | 3000 |
| Charging voltage (V) | 4.20 |
| Nominal voltage (V) | 3.6 |
| Lifecycles (cycles) | 300 @0.5C to 80% |
| Discharge end voltage (V) | 2.5 |
| Weight (g) | 45.50 |
| Max. continuous discharge current (A) | 15 |
| Properties | Aluminum [36] | Air [37] | Paraffin RT27 [38] | RT27—Metal Foam Composite [36] |
|---|---|---|---|---|
| Specific heat capacity (J/kg·K) | 910 | 1005 | 2500 | 1195.68 |
| Thermal conductivity (W/m·K) | 237 | 0.0267 | 0.2 | 4.49 |
| Latent heat (kJ/kg) | - | - | 179 | - |
| Density (kg/m3) | 2800 | 1.165 | 870 at 299 K 781.5 at 301 K 750 at 343 K | 1005 (Solid) 902 (Liquid) |
| Viscosity (kg·m/S) | - | 1.86 × 10−5 | −1.137439E − 8T3 + 1.178188E − 5T2–0.004111388T + 0.4857203 | - |
| Solidus temperature (K) | - | - | 297.65 | - |
| Melting temperature (K) | - | - | 300.15 | 300.15 |
| Polynomial Fitting of Equivalent Internal Resistance (R) | Temperature (K) |
|---|---|
| 58 − 355 × SOC + 1898 × SOC2 − 5121 × SOC3 + 7376 × SOC4 − 5374 × SOC5 + 1559 × SOC6 | 333 |
| 58 − 355 × SOC + 1898 × SOC2 − 5121 × SOC3 + 7376 × SOC4 − 5374 × SOC5 + 1559 × SOC6 | 323 |
| 66 − 382 × SOC + 1962 × SOC2 − 5181 × SOC3 + 7378 × SOC4 − 5365 × SOC5 + 1559 × SOC6 | 313 |
| 107 − 793 × SOC + 4036 × SOC2 − 10,514 × SOC3 + 14,700 × SOC4 − 10,480 × SOC5 + 2989 × SOC6 | 303 |
| 166 − 1334 × SOC + 6559 × SOC2 − 16,531 × SOC3 + 22,391 × SOC4 − 15,496 × SOC5 + 4301 × SOC6 | 293 |
| Velocity (m/s) | Reynolds |
|---|---|
| 0 | 0 |
| 0.5 | 1403.4 |
| 1.5 | 4210.2 |
| 2 | 5613.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saeedipour, S.; Gharehghani, A.; Ahbabi Saray, J.; Andwari, A.M.; Mikulski, M. Proposing a Hybrid Thermal Management System Based on Phase Change Material/Metal Foam for Lithium-Ion Batteries. World Electr. Veh. J. 2023, 14, 240. https://doi.org/10.3390/wevj14090240
Saeedipour S, Gharehghani A, Ahbabi Saray J, Andwari AM, Mikulski M. Proposing a Hybrid Thermal Management System Based on Phase Change Material/Metal Foam for Lithium-Ion Batteries. World Electric Vehicle Journal. 2023; 14(9):240. https://doi.org/10.3390/wevj14090240
Chicago/Turabian StyleSaeedipour, Soheil, Ayat Gharehghani, Jabraeil Ahbabi Saray, Amin Mahmoudzadeh Andwari, and Maciej Mikulski. 2023. "Proposing a Hybrid Thermal Management System Based on Phase Change Material/Metal Foam for Lithium-Ion Batteries" World Electric Vehicle Journal 14, no. 9: 240. https://doi.org/10.3390/wevj14090240
APA StyleSaeedipour, S., Gharehghani, A., Ahbabi Saray, J., Andwari, A. M., & Mikulski, M. (2023). Proposing a Hybrid Thermal Management System Based on Phase Change Material/Metal Foam for Lithium-Ion Batteries. World Electric Vehicle Journal, 14(9), 240. https://doi.org/10.3390/wevj14090240









