Improvement of the Vehicle Seat Suspension System Incorporating the Mechatronic Inerter Element
Abstract
1. Introduction
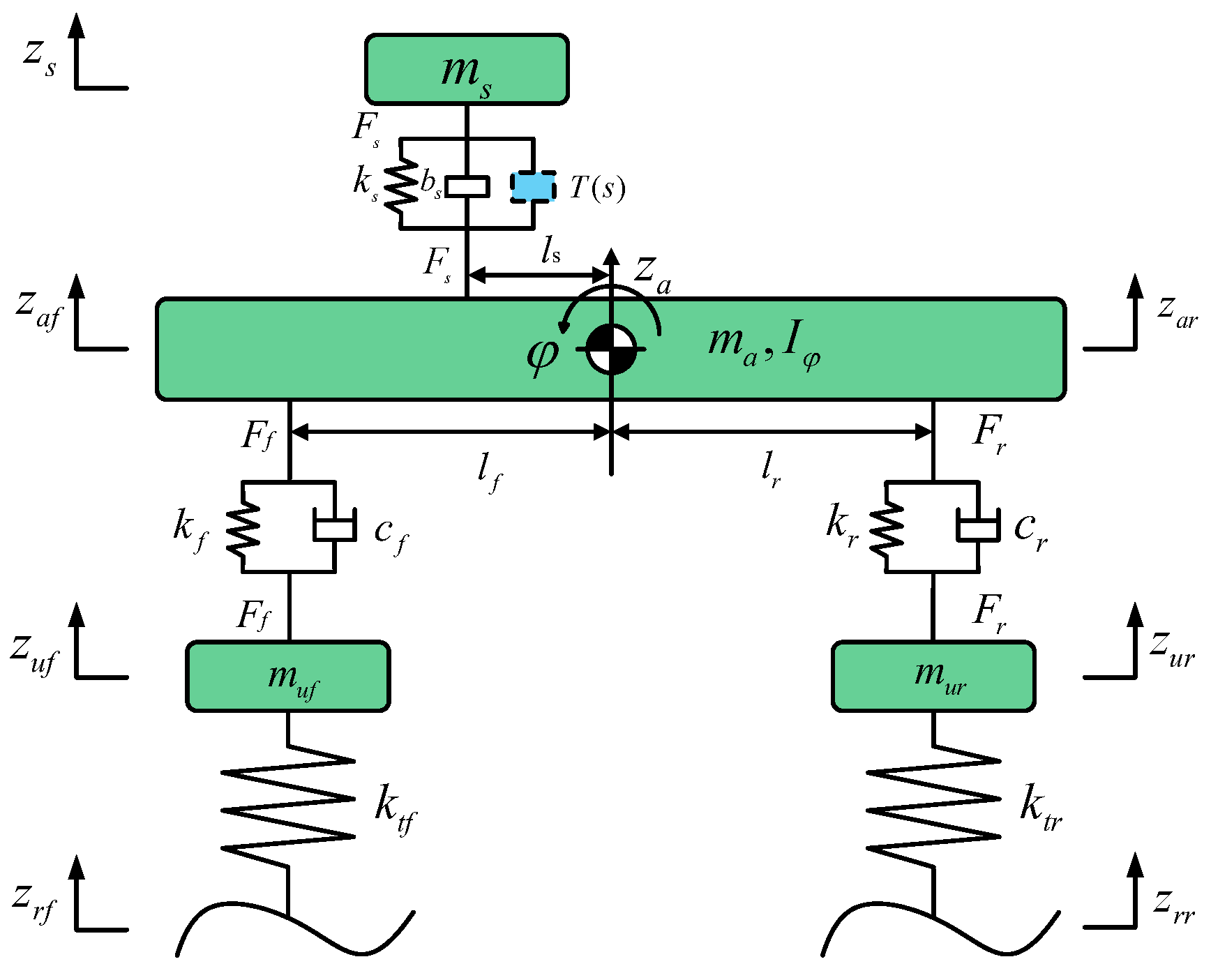
2. Half Vehicle Model
3. Seat Suspension Layout
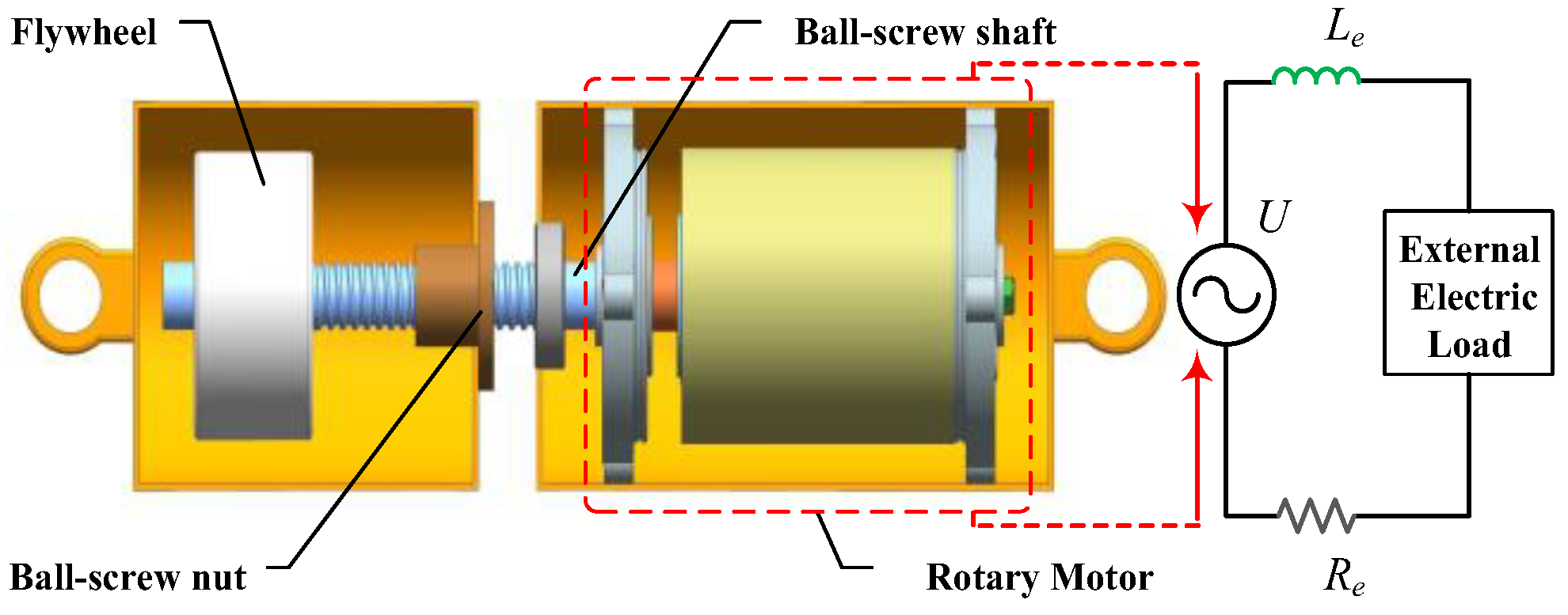
3.1. The Ball-Screw Mechatronic Inerter
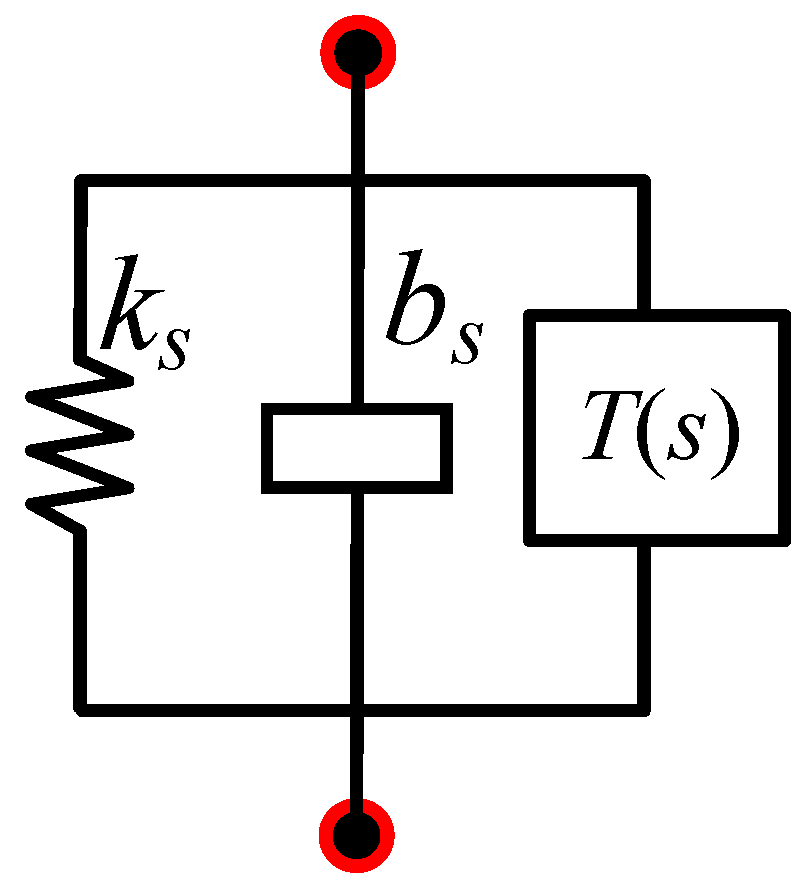
3.2. The Seat Suspension Layout
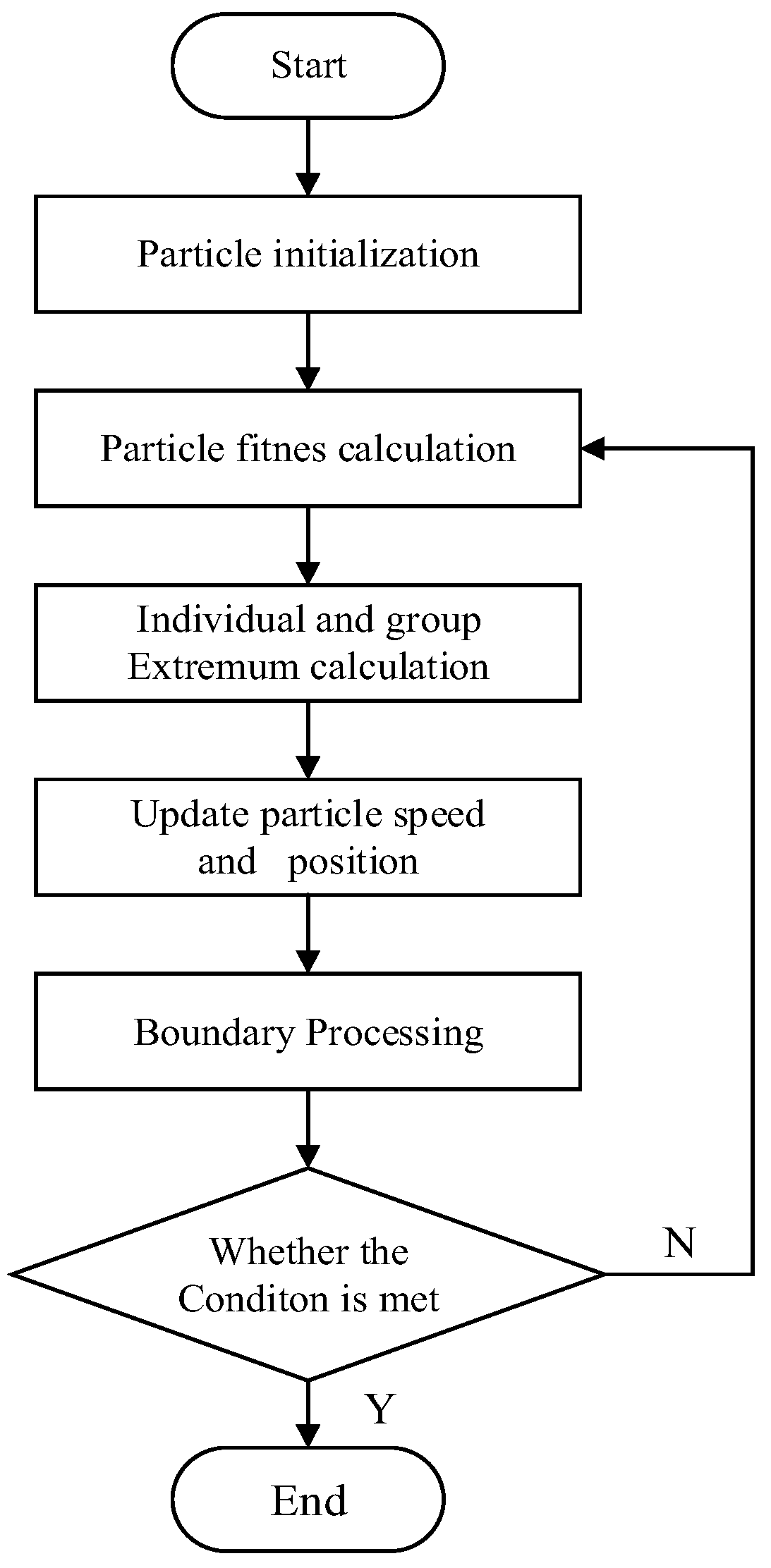
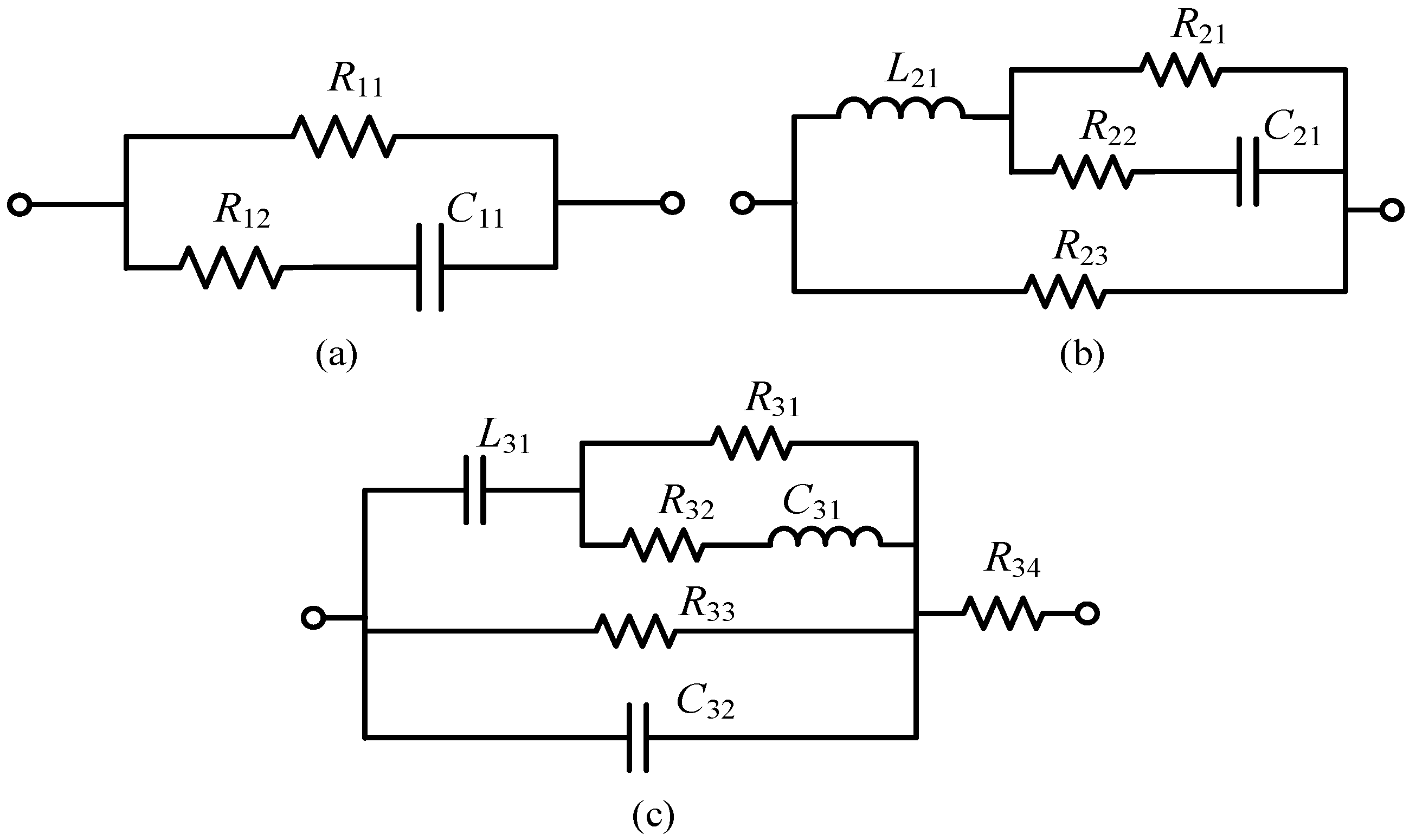
4. Optimal Design of the Mechatronic Seat Suspension
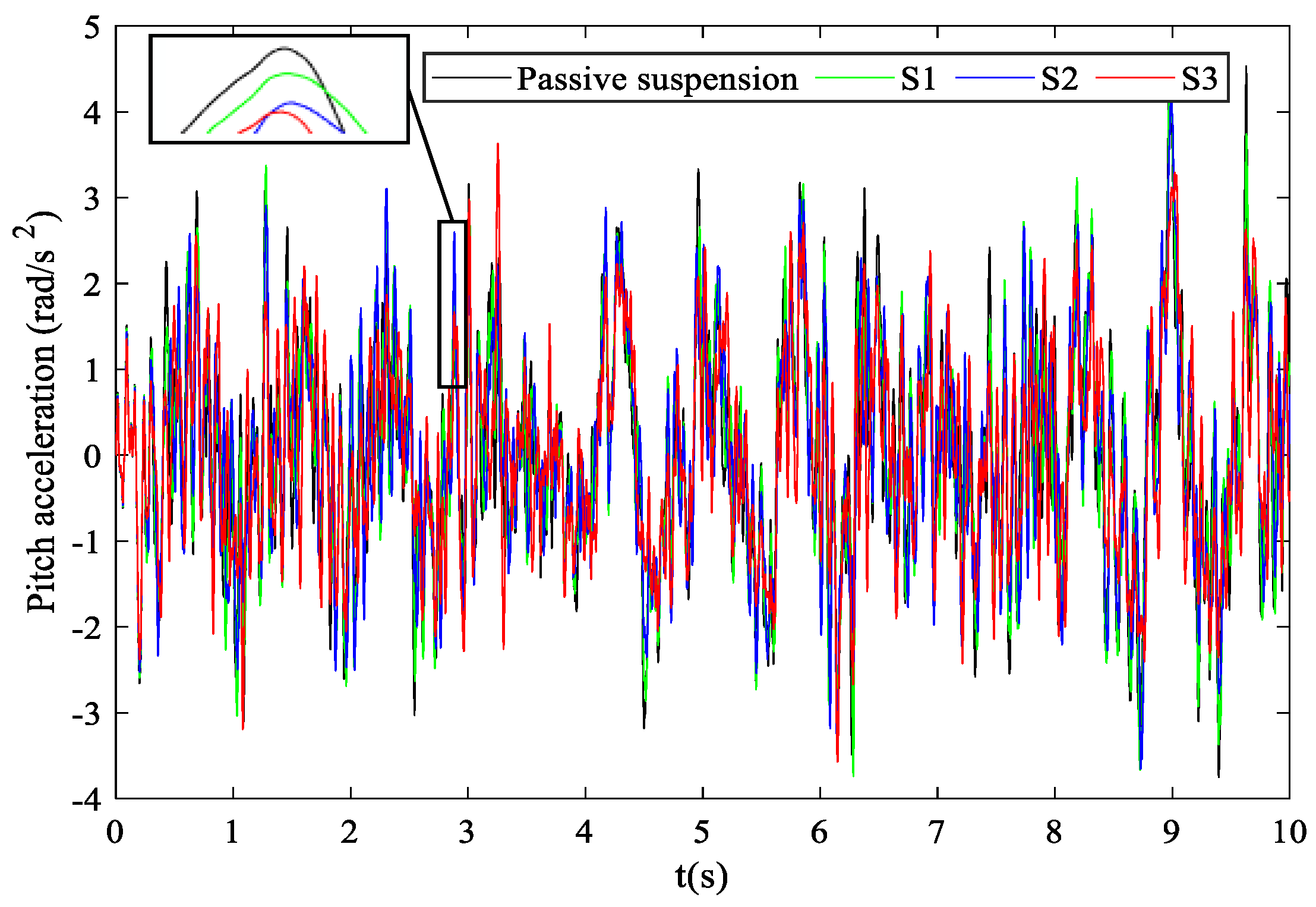
5. Performance Evaluation
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Choi, S.; Choi, Y.T.; Chang, E.G.; Han, S.J.; Kim, C.S. Control characteristics of a continuously variable ER damper. Mechatronics 1998, 8, 143–161. [Google Scholar] [CrossRef]
- Rakheja, S.; Afework, Y.; Sankar, S. An analytical and experimental investigation of the driver-seat-suspension system. Veh. Syst. Dyn. 1994, 23, 501–524. [Google Scholar] [CrossRef]
- Tong, R.T.; Amirouche, F. Ride control-a two state suspension design for cabs and seats. Veh. Syst. Dyn. 1999, 33, 578–589. [Google Scholar] [CrossRef]
- Wereley, N.M.; Pang, L. Nondimensional analysis of semi-active electrorheological and magnetorheological dampers using approximate parallel plate models. Smart Mater. Struct. 1997, 7, 732–743. [Google Scholar] [CrossRef]
- Amirouche, F.; Palkovics, L.; Woodrooffe, J. Optimal driver seat suspension design for heavy trucks. Transp. Syst. ASME 1994, 2, 277–291. [Google Scholar]
- Andersson, R. The low back pain of bus drivers in an urban area of California. Spine 1982, 17, 1481–1488. [Google Scholar] [CrossRef]
- Deng, H.; Deng, J.; Yue, R.; Han, G.; Zhang, J.; Ma, M.; Zhong, X. Design and verification of a seat suspension with variable stiffness and damping. Smart Mater. Struct. 2019, 28, 65015. [Google Scholar] [CrossRef]
- Ning, D.; Du, H.; Sun, S.; Li, W.; Zhang, N.; Dong, M. A novel electrical variable stiffness device for vehicle seat suspension control with mismatched disturbance compensation. IEEE ASME Trans. Mechatron. 2019, 24, 2019–2030. [Google Scholar] [CrossRef]
- Liao, X.; Du, X.; Li, S. Design of cab seat suspension system for construction machinery based on negative stiffness structure. Adv. Mech. Eng. 2021, 13, 1–15. [Google Scholar] [CrossRef]
- Liu, P.; Ning, D.; Luo, L.; Zhang, N.; Du, H. An electromagnetic variable inertance and damping seat suspension with controllable circuits. IEEE Trans. Ind. Electron. 2021, 69, 2811–2821. [Google Scholar] [CrossRef]
- Smith, M.C.; Wang, F. Performance benefits in passive vehicle suspensions employing inerters. Veh. Syst. Dyn. 2004, 42, 235–257. [Google Scholar] [CrossRef]
- Zhang, C.; Zhang, Z.; Zhao, H. Analysis of dynamic characteristics and ride performance of automobile active suspension. Chin. Agric. Mech. 2015, 36, 176–179. [Google Scholar] [CrossRef]
- Huang, C.; Chen, L.; Yuan, Z.; Jiang, H.; Niu, L. Hybrid fuzzy control of semi-active suspension system. Automot. Eng. 2014, 36, 999–1018. [Google Scholar]
- Smith Malcolm, C. Synthesis of mechanical networks: The inerter. IEEE Trans. Autom. Control 2002, 47, 1648–1662. [Google Scholar] [CrossRef]
- Zhao, Z.-P.; Chen, Q.-J.; Zhang, R.-F.; Pan, P.; Jian, Y.-Y. Energy dissipation mechanism of inerter systems. Int. J. Mech. Sci. 2020, 184, 105845. [Google Scholar] [CrossRef]
- Chen, M.Z.Q.; Hu, Y.L.; Li, C.Y.; Chen, G. Application of semi-active inerter in semi-active suspensions via force tracking. J. Vib. Acoust. 2016, 138, 041014. [Google Scholar] [CrossRef]
- Hu, Y.L.; Wang, K.; Chen, Y.H.; Chen, M.Z.Q. Inerter-based semi-active suspensions with low-order mechanical admittance via network synthesis. Trans. Inst. Meas. Control 2018, 40, 4233–4245. [Google Scholar] [CrossRef]
- Liu, Y.L.; Zhao, W.T.; Yang, X.F.; Shen, Y.J. Predictive control of vehicle ISD suspension based on a hydraulic electric inerter. Shock Vib. 2019, 2019, 9230736. [Google Scholar] [CrossRef]
- Zhang, S.Y.; Jiang, J.Z.; Neild, S.A. Optimal configurations for a linear vibration suppression device in a multi-storey building. Struct. Control Health Monit. 2016, 24, e1887. [Google Scholar] [CrossRef]
- Giaralis, A.; Petrini, F. Wind-induced vibration mitigation in tall buildings using the tuned mass-damper-inerter. J. Struct. Eng. 2017, 142, 04017127. [Google Scholar] [CrossRef]
- Yang, L.; Wang, R.; Ding, R.; Liu, W.; Zhu, Z. Investigation on the dynamic performance of a new semi-active hydro-pneumatic inerter-based suspension system with MPC control strategy. Mech. Syst. Signal Process. 2021, 154, 107569. [Google Scholar] [CrossRef]
- Shen, Y.; Hua, J.; Fan, W.; Liu, Y.; Yang, X.; Chen, L. Optimal design and dynamic performance analysis of a fractional-order electrical network-based vehicle mechatronic ISD suspension. Mech. Syst. Signal Process. 2023, 184, 109718. [Google Scholar] [CrossRef]
- Li, Y.; Yang, X.; Shen, Y.; Liu, Y.; Wang, W. Optimal design and dynamic control of the HMDV inertial suspension based on the ground-hook positive real network. Adv. Eng. Softw. 2022, 171, 103171. [Google Scholar] [CrossRef]
- Shen, Y.; Chen, L.; Liu, Y.; Zhang, X. Influence of fluid inerter nonlinearities on vehicle suspension performance. Adv. Mech. Eng. 2017, 9, 1687814017737257. [Google Scholar] [CrossRef]
- Sun, X.Q.; Chen, L.; Wang, S.H.; Zhang, X.L.; Yang, X.F. Performance investigation of vehicle suspension system with nonlinear ball-screw inerter. Int. J. Automot. Technol. 2016, 17, 399–408. [Google Scholar] [CrossRef]
- Liu, C.; Chen, L.; Zhang, X.; Yang, Y.; Nie, J. Design and tests of a controllable inerter with fluid-air mixture condition. IEEE Access 2020, 8, 125620–125629. [Google Scholar] [CrossRef]
- Yang, X.; He, T.; Shen, Y.; Liu, Y.; Yan, L. Research on predictive coordinated control of ride comfort and road friendliness for heavy vehicle ISD suspension based on the hybrid-hook damping strategy. Proc. Inst. Mech. Eng. D J. Automob. Eng. 2022. [Google Scholar] [CrossRef]
- Shen, Y.; Hua, J.; Wu, B.; Chen, Z.; Xiong, X.; Chen, L. Optimal design of the vehicle mechatronic ISD suspension system using the structure-immittance approach. Proc. Inst. Mech. Eng. D J. Automob. Eng. 2022, 236, 512–521. [Google Scholar] [CrossRef]
- ISO 8608:2016; Mechanical Vibration—Road Surface Profiles—Reporting of Measured Data. International Organization for Standardization: Geneva, Switzerland, 2016. Available online: https://www.iso.org/standard/71202.html. (accessed on 22 November 2022).


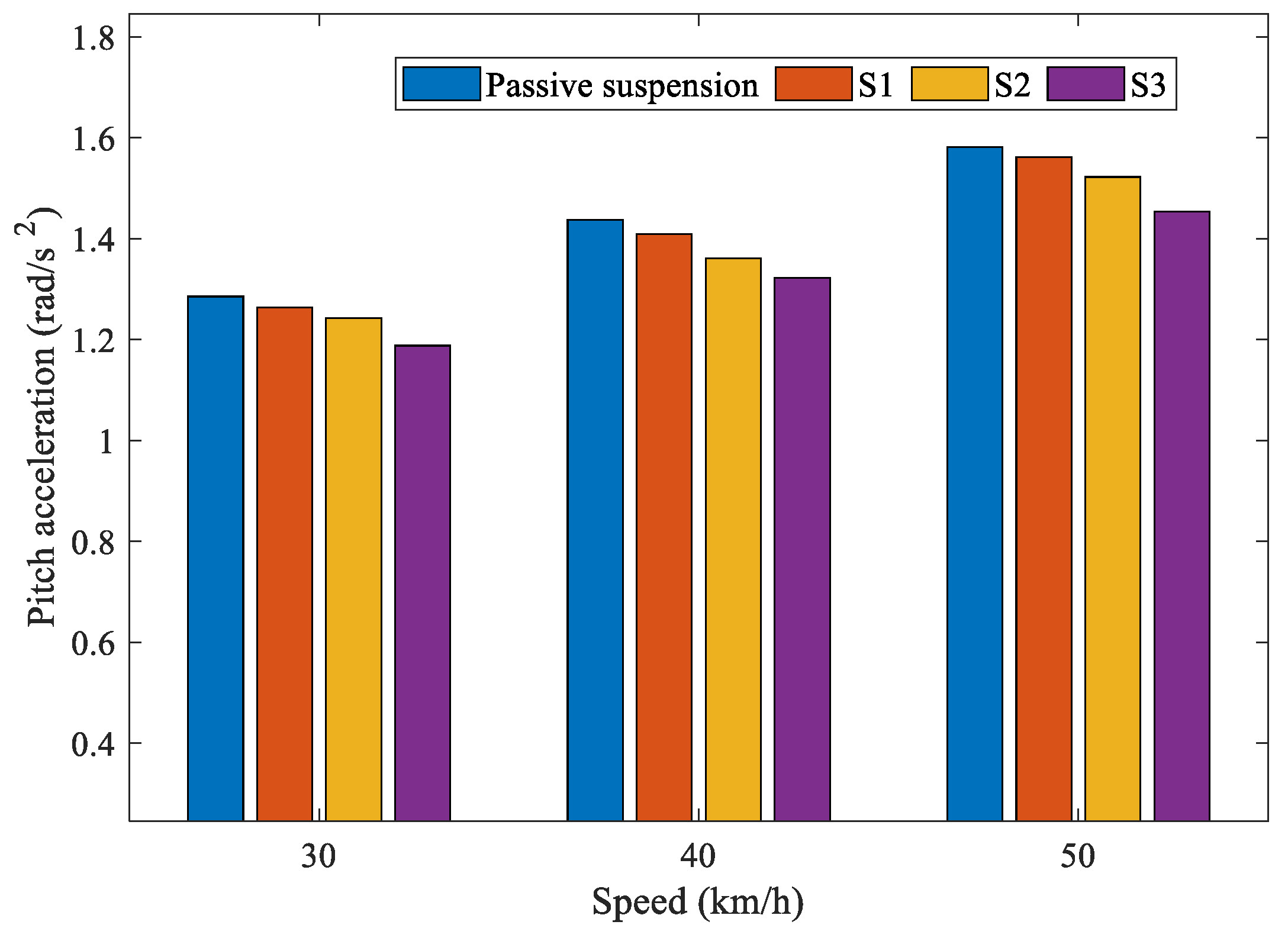
| Name | Value |
|---|---|
| Seat mass ms (kg) | 48 |
| Body centroid mass ma (kg) | 928.2 |
| Unsprung mass of front wheels muf (kg) | 26.5 |
| Unsprung mass of rear wheels mur (kg) | 24.4 |
| Distance from seat to centroid ls (m) | 0.324 |
| Distance from front axle to centroid lf (m) | 0.968 |
| Distance from rear axle to centroid lr (m) | 1.392 |
| Moment of inertia aound the Y axis Iφ (kg·m2) | 1058 |
| Front suspension stiffness kf (kN·m−1) | 25 |
| Rear suspension stiffness kr (kN·m−1) | 22 |
| Front suspension damping cf (Ns/m) | 1500 |
| Rear suspension damping cr (Ns/m) | 1300 |
| Tire stiffness kt (kN·m−1) | 192 |
| Name | Value |
|---|---|
| Resistor R11 (Ω) | 4877 |
| Resistor R12 (Ω) | 654 |
| Capacitor C11 (F) | 0.0047 |
| Resistor R21 (Ω) | 98,754 |
| Resistor R22 (Ω) | 35,789 |
| Resistor R23 (Ω) | 5711 |
| Capacitor C21 (F) | 0.0079 |
| Inductor L21 (H) | 0.34 |
| Resistor R31 (Ω) | 81 |
| Resistor R32 (Ω) | 6998 |
| Resistor R33 (Ω) | 74,223 |
| Resistor R34 (Ω) | 158 |
| Capacitor C31 (F) | 0.0024 |
| Capacitor C32 (F) | 0.0017 |
| Inductor L31 (H) | 0.76 |
| RMS of Seat Acceleration | Improvement | RMS of Pitch Acceleration | Improvement | |
|---|---|---|---|---|
| Passive suspension | 0.9913 | / | 1.2852 | / |
| Layout S1 | 0.9664 | 2.51% | 1.2638 | 1.67% |
| Layout S2 | 0.9459 | 4.58% | 1.2414 | 3.41% |
| Layout S3 | 0.8866 | 10.56% | 1.1879 | 7.57% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiu, C.; Liu, X.; Shen, Y. Improvement of the Vehicle Seat Suspension System Incorporating the Mechatronic Inerter Element. World Electr. Veh. J. 2023, 14, 29. https://doi.org/10.3390/wevj14020029
Qiu C, Liu X, Shen Y. Improvement of the Vehicle Seat Suspension System Incorporating the Mechatronic Inerter Element. World Electric Vehicle Journal. 2023; 14(2):29. https://doi.org/10.3390/wevj14020029
Chicago/Turabian StyleQiu, Chengqun, Xiaofu Liu, and Yujie Shen. 2023. "Improvement of the Vehicle Seat Suspension System Incorporating the Mechatronic Inerter Element" World Electric Vehicle Journal 14, no. 2: 29. https://doi.org/10.3390/wevj14020029
APA StyleQiu, C., Liu, X., & Shen, Y. (2023). Improvement of the Vehicle Seat Suspension System Incorporating the Mechatronic Inerter Element. World Electric Vehicle Journal, 14(2), 29. https://doi.org/10.3390/wevj14020029








