An Inductor-Based and Capacitor-Free Bipolar Pulse Converter with Overvoltage Protection
Abstract
:1. Introduction
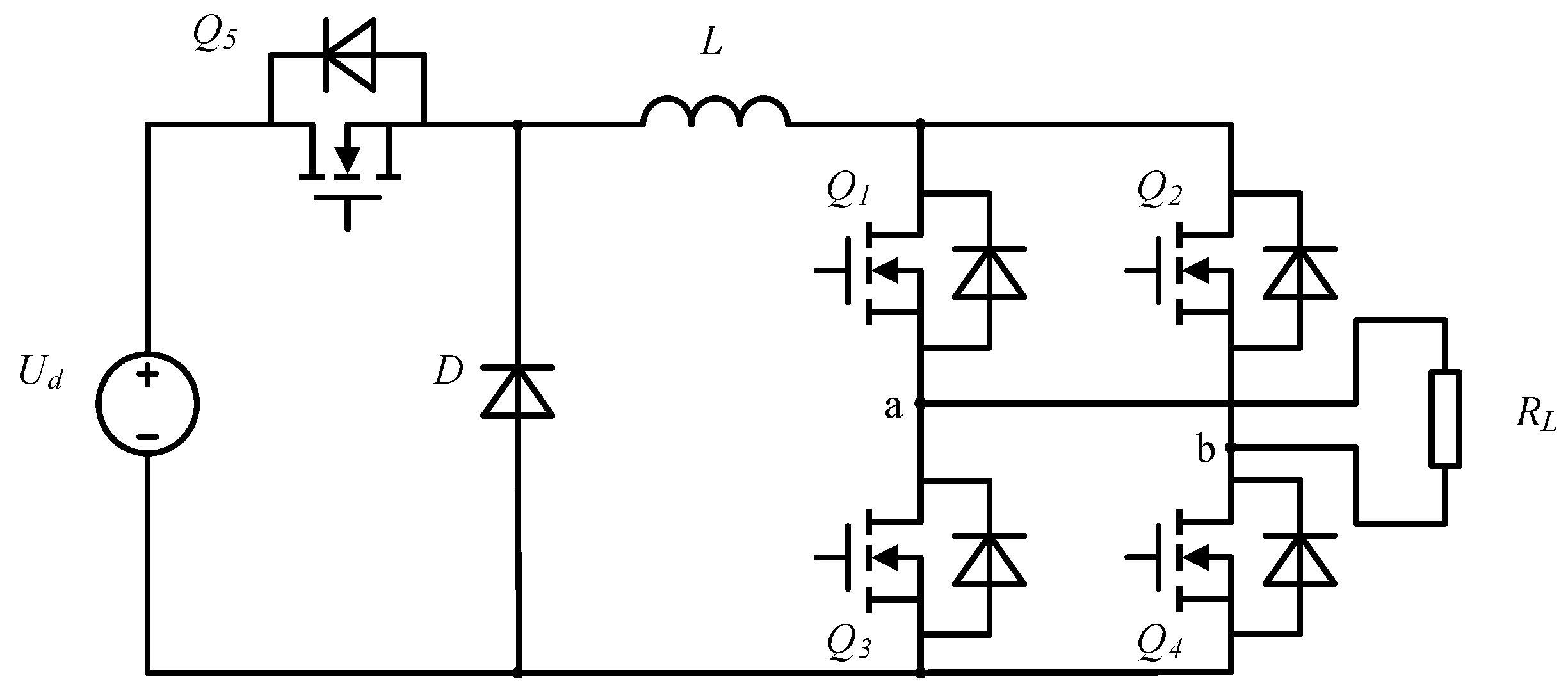
2. Converter Circuit Topology and Overvoltage Protection
2.1. The Circuit Topology and Working Process
- (1)
- The switches are ideal MOSFETs except for the constant on-resistance.
- (2)
- The freewheeling diode is ideal except for the constant threshold voltage.
- (3)
- The power inductor is ideal except for the constant ESR.
- (1)
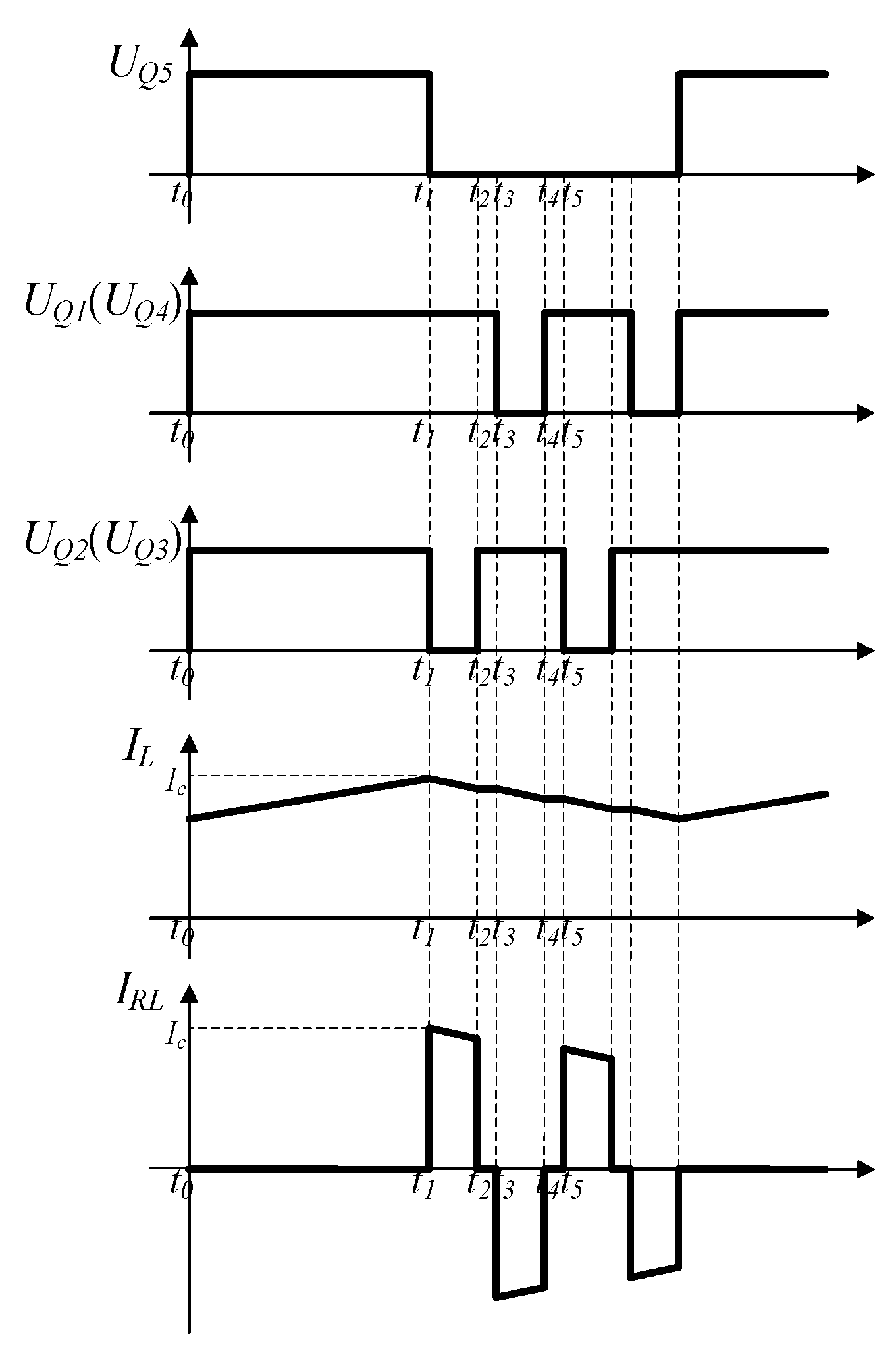
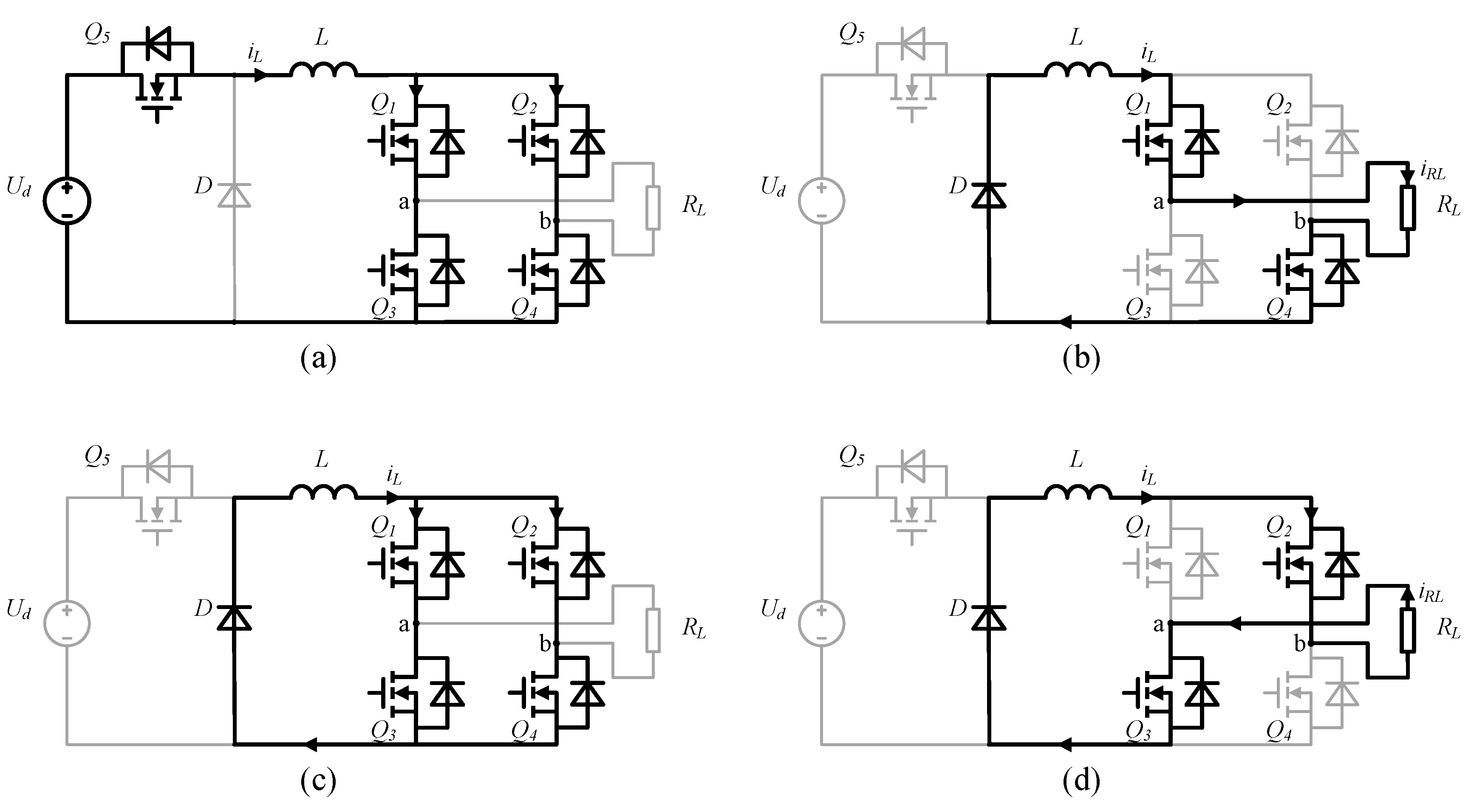
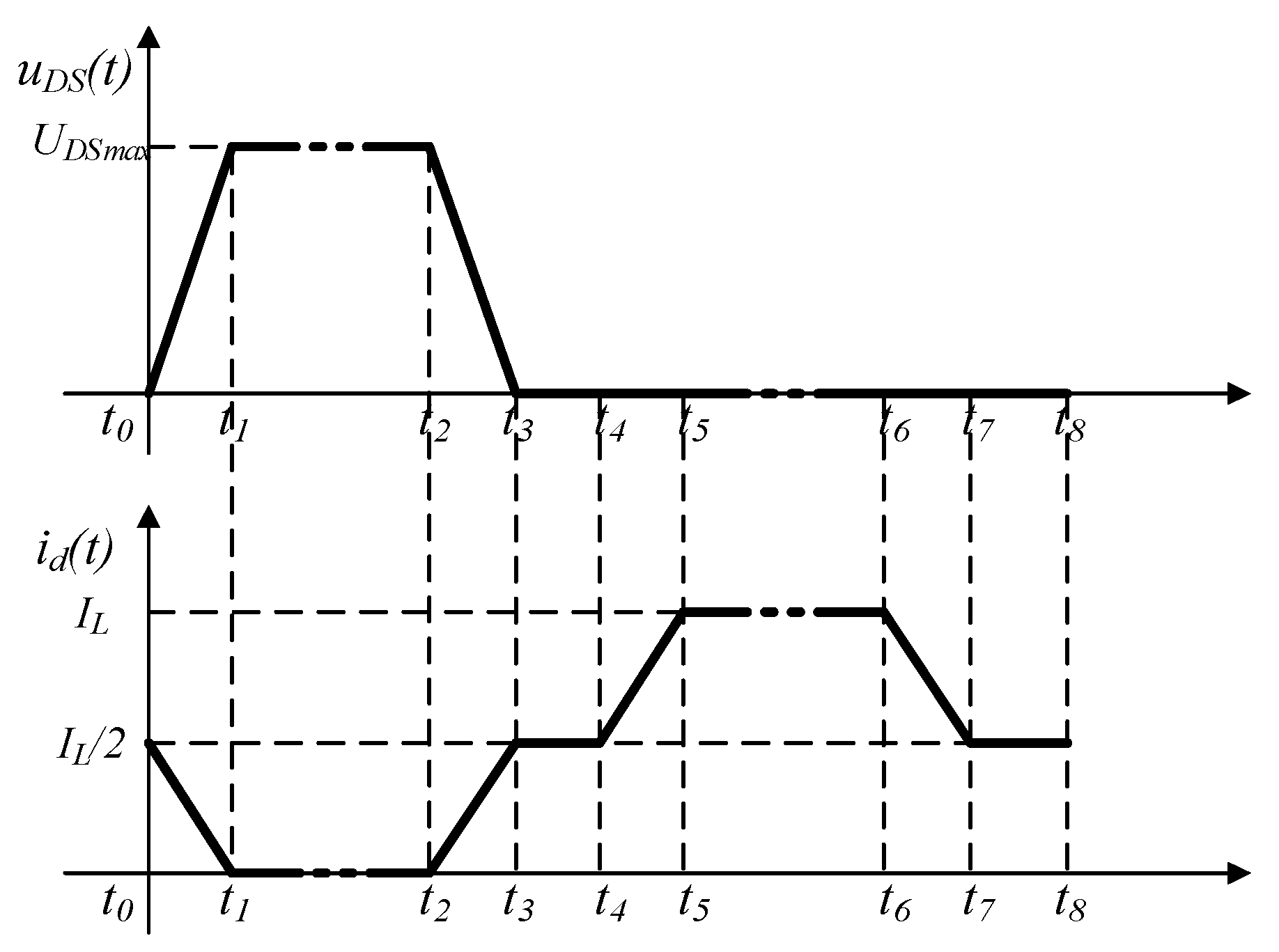
- Charging stage: (t0 − t1)
- (2)
- Discharging stage: (t1 − t5)
- (a)
- Positive pulse: (t1 − t2)
- (b)
- Deadtime: (t2 − t3) and (t4 − t5)
- (c)
- Negative pulse: (t3 − t4)
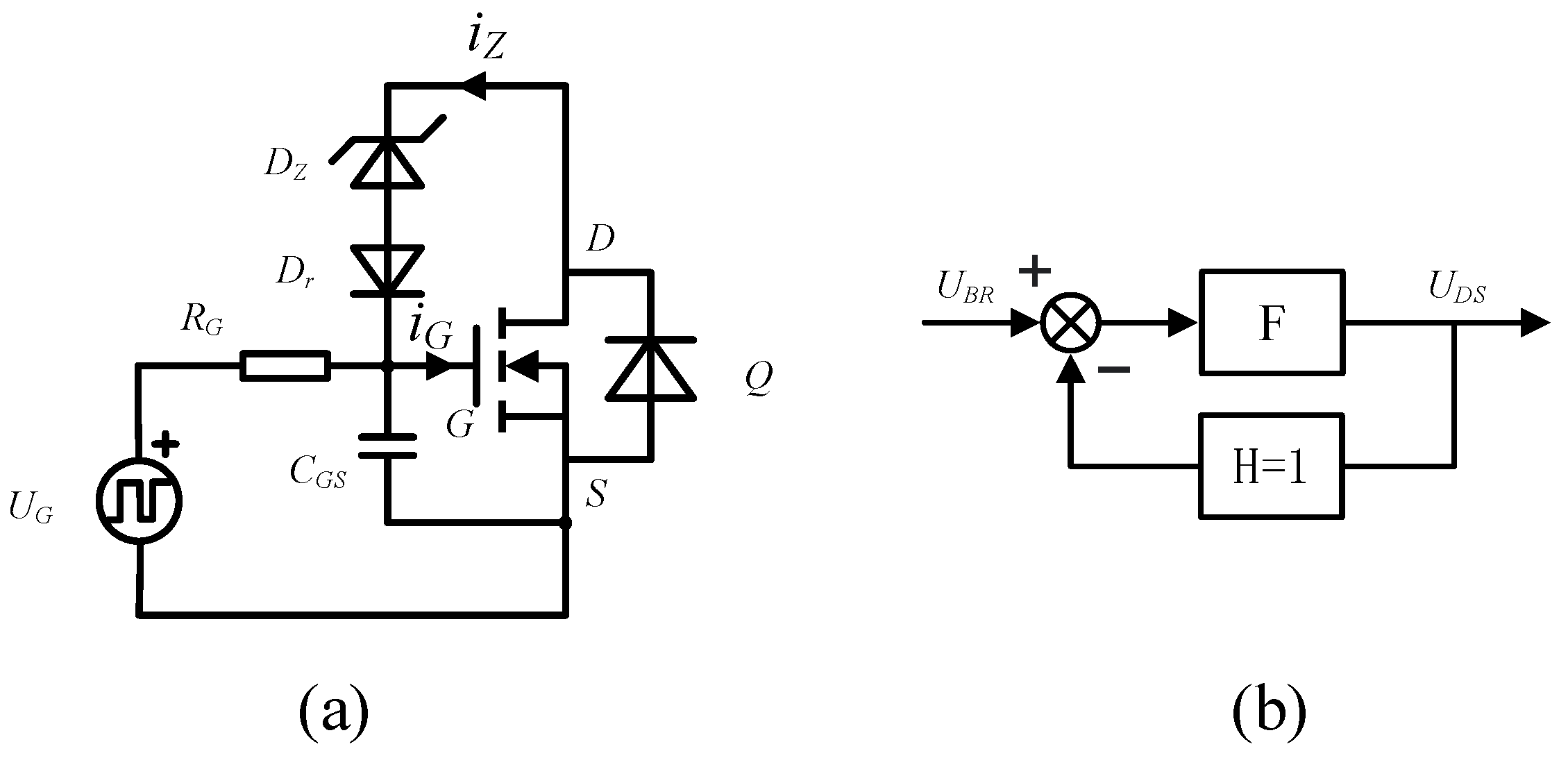
2.2. Overvoltage Protection Scheme
3. Results Simulation of the Converter and Overvoltage Protection
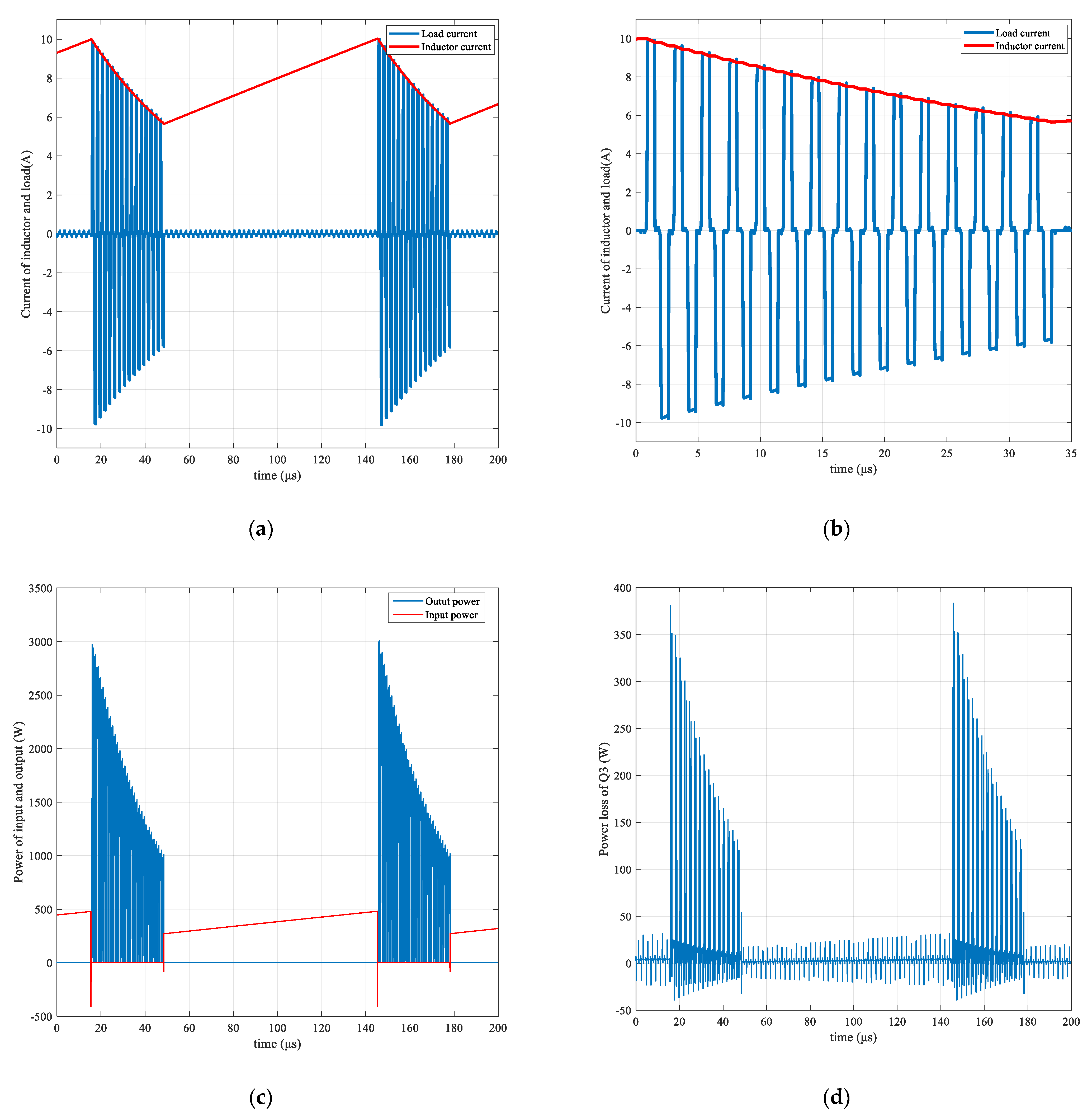
3.1. Simulation of the Converter
3.2. Simulation of the Overvoltage Protection
4. Experiments and Results
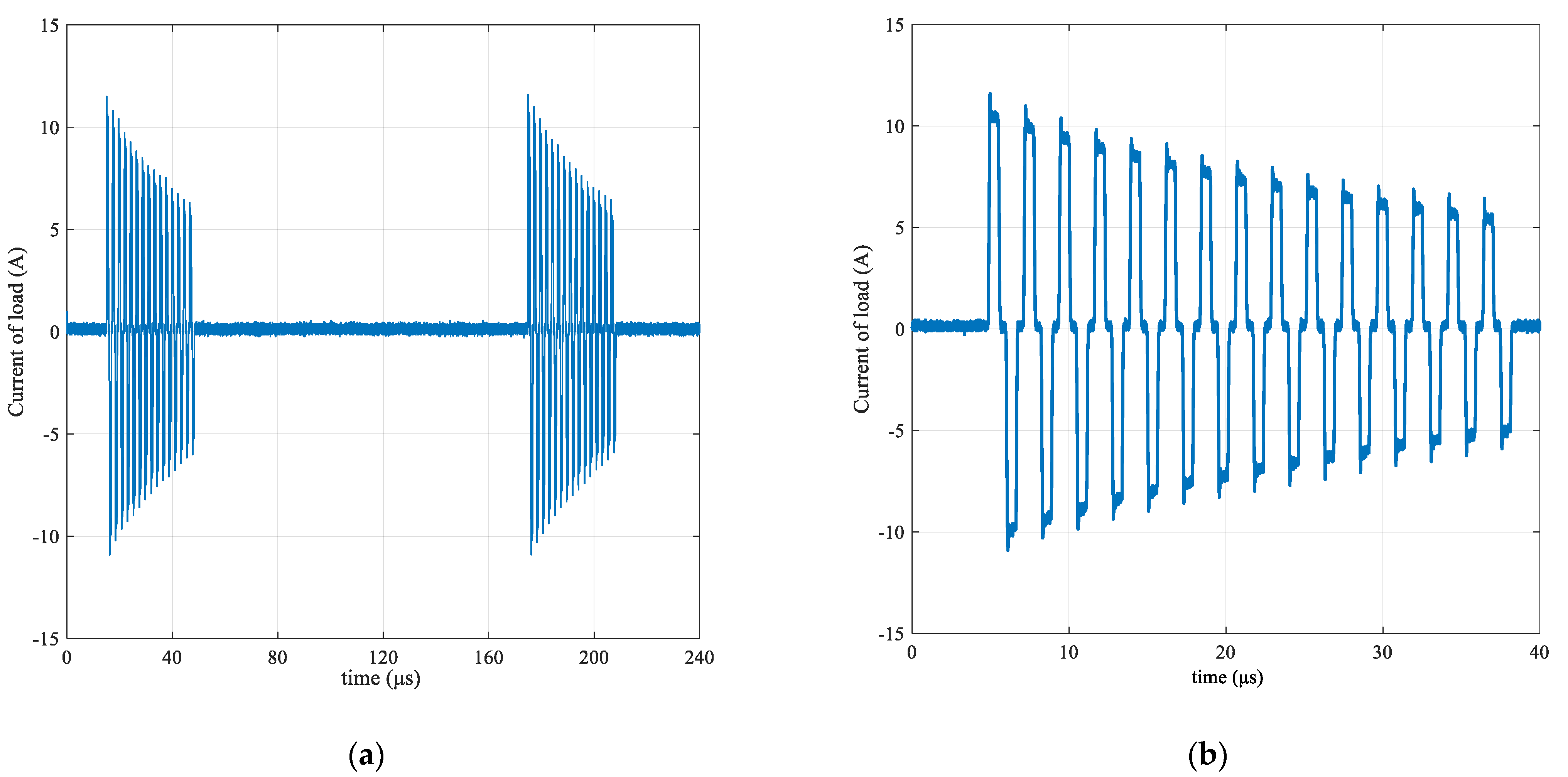
4.1. Output under Normal Conditions
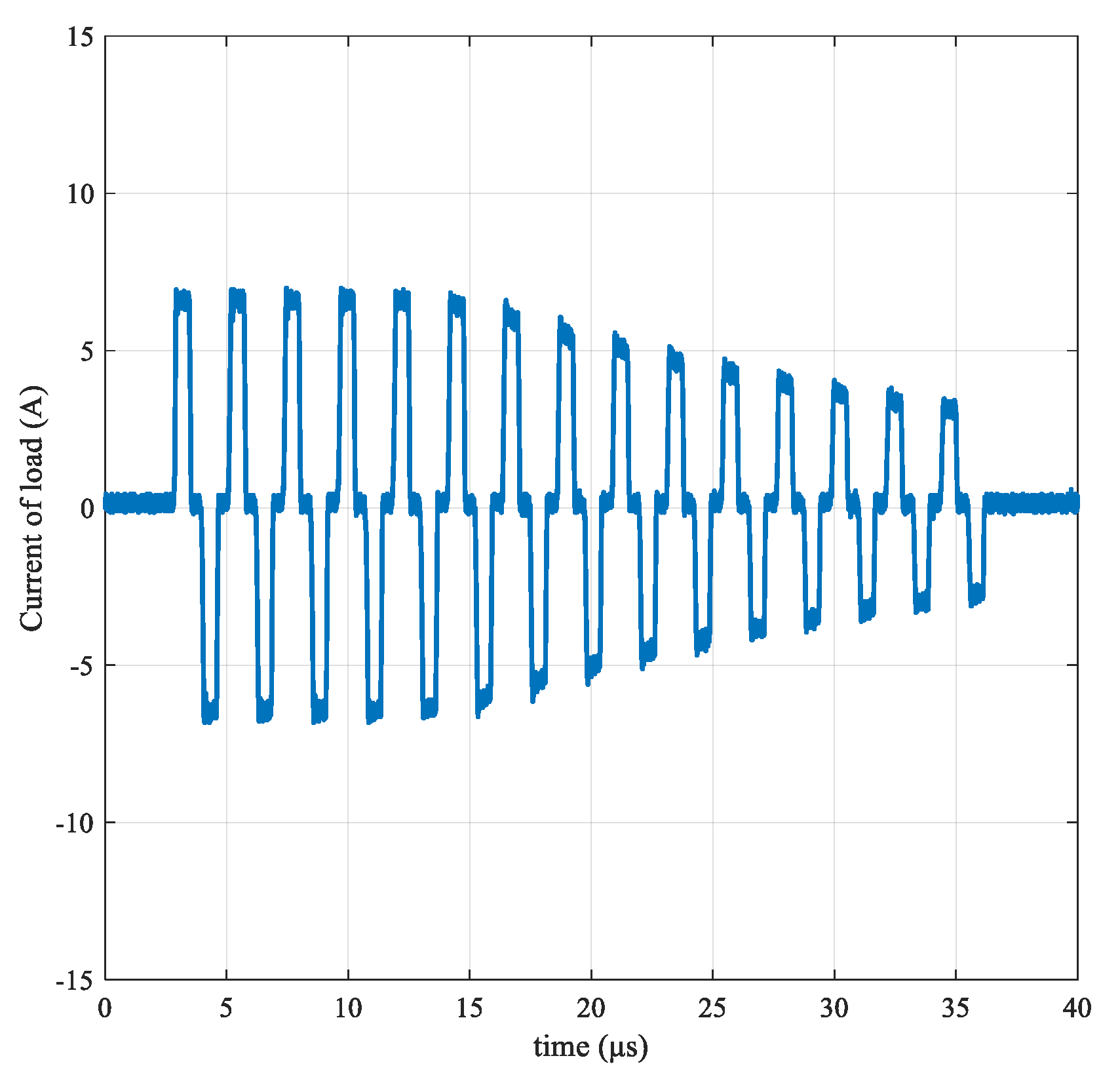
4.2. Output When Overvoltage Protection Takes Effect
4.3. Model and Calculation of the Power Loss on MOSFETs
4.3.1. Switching Loss
4.3.2. Conduction Loss
- (1)
- Charging stage
- (2)
- Discharging stage
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
| Parameter | Value |
|---|---|
| Power inductor | L = 1 mH, ESR = 50 mΩ |
| Input dc voltage | Ud = 48 V |
| Load impedance | RL = 30 Ω |
| Reference current | Ic = 10 A |
| Positive pulse width | tp_pulse = 5000 ns |
| Negative pulse width | tn_pulse = 400 ns |
| deadtime | tdead = 300 ns |
| Number of bipolar pulses in discharge stage | n = 5 |
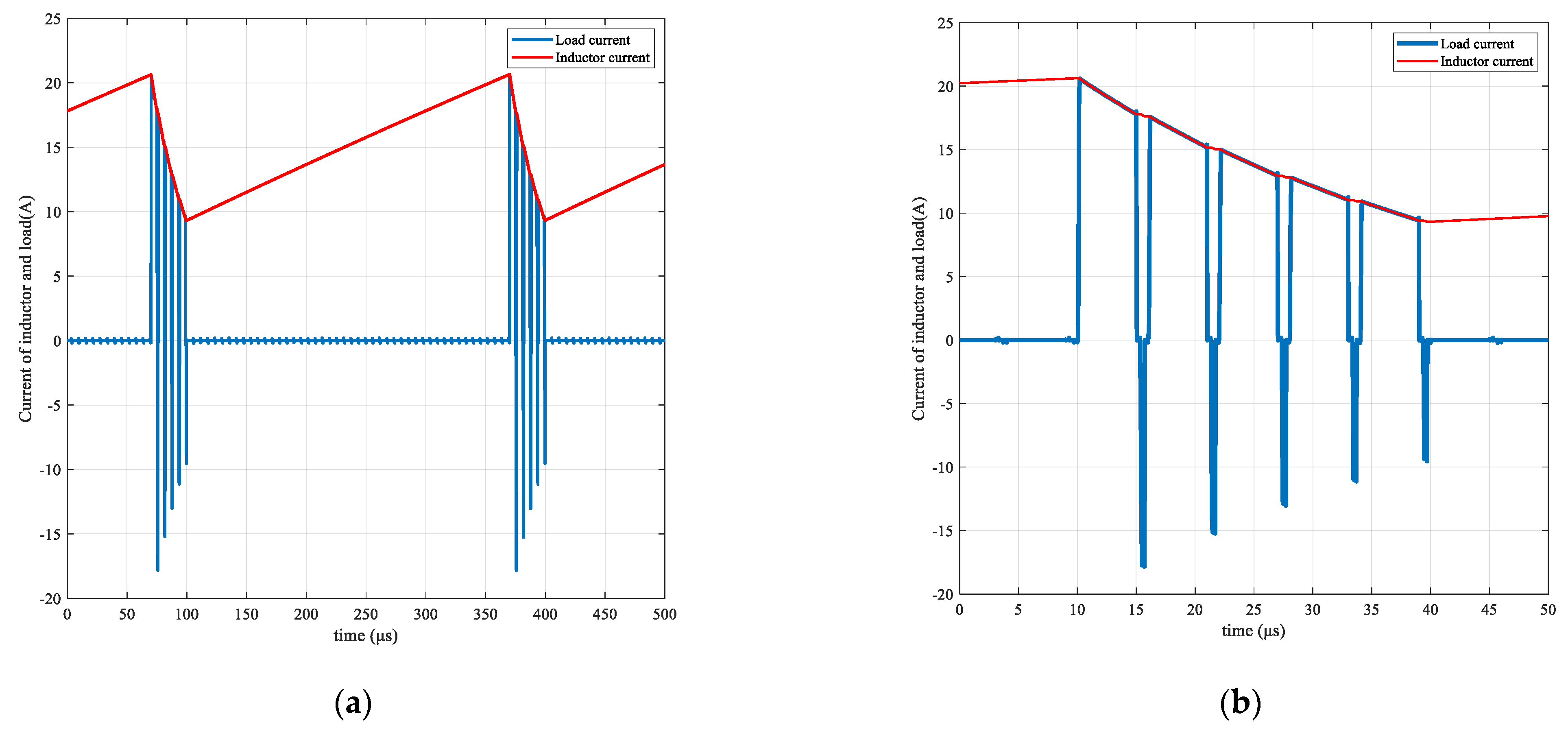
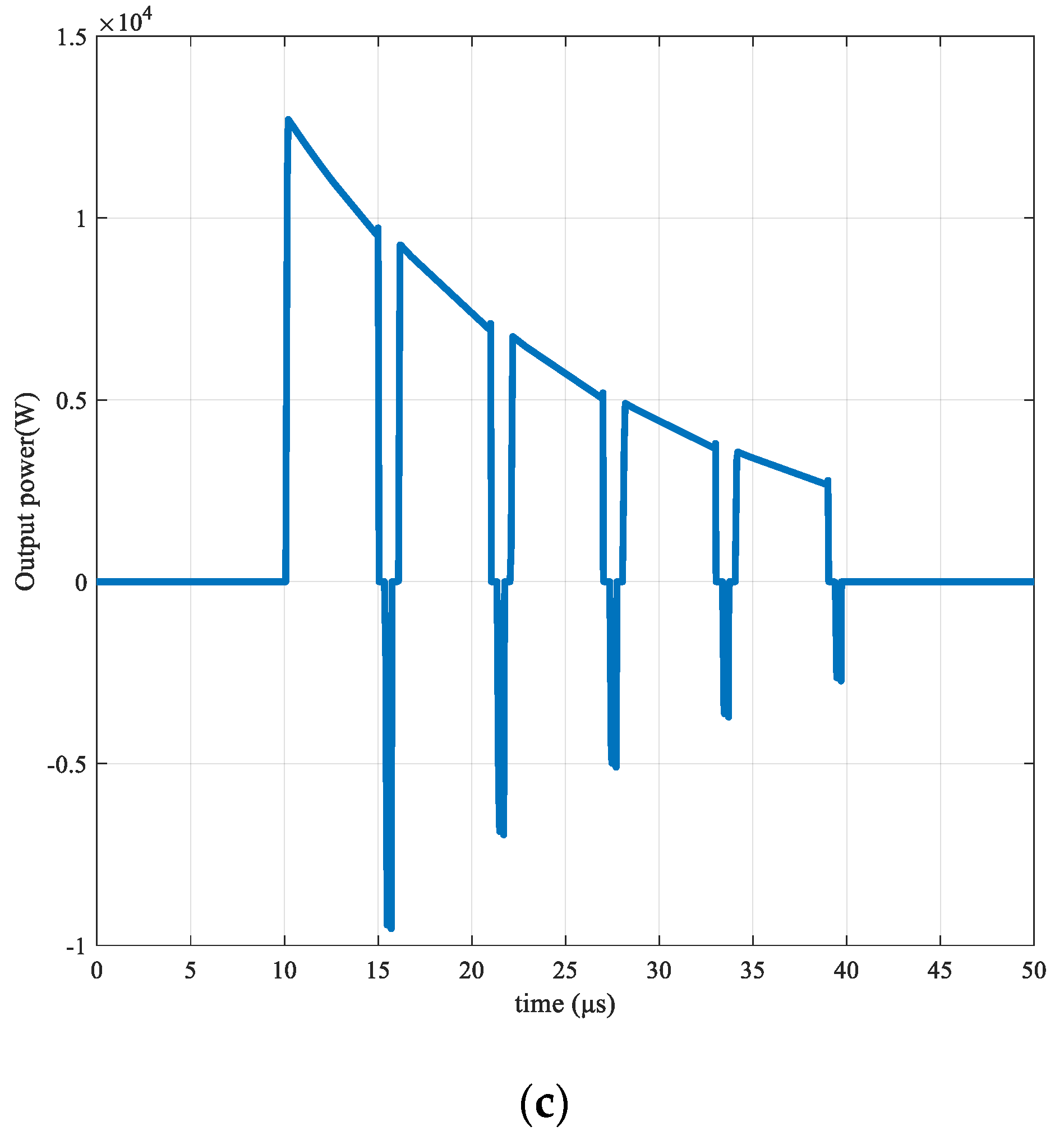
Appendix B
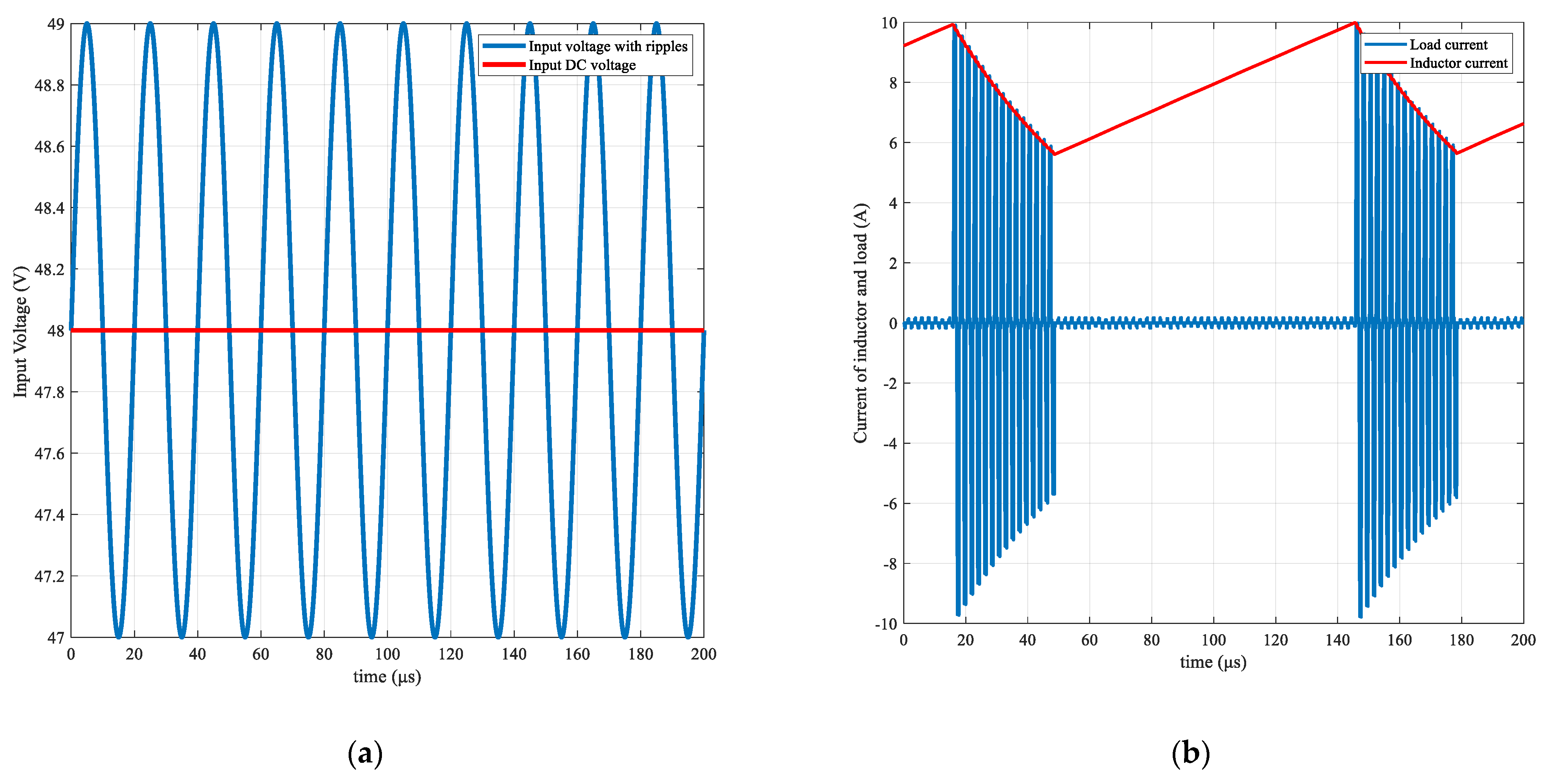
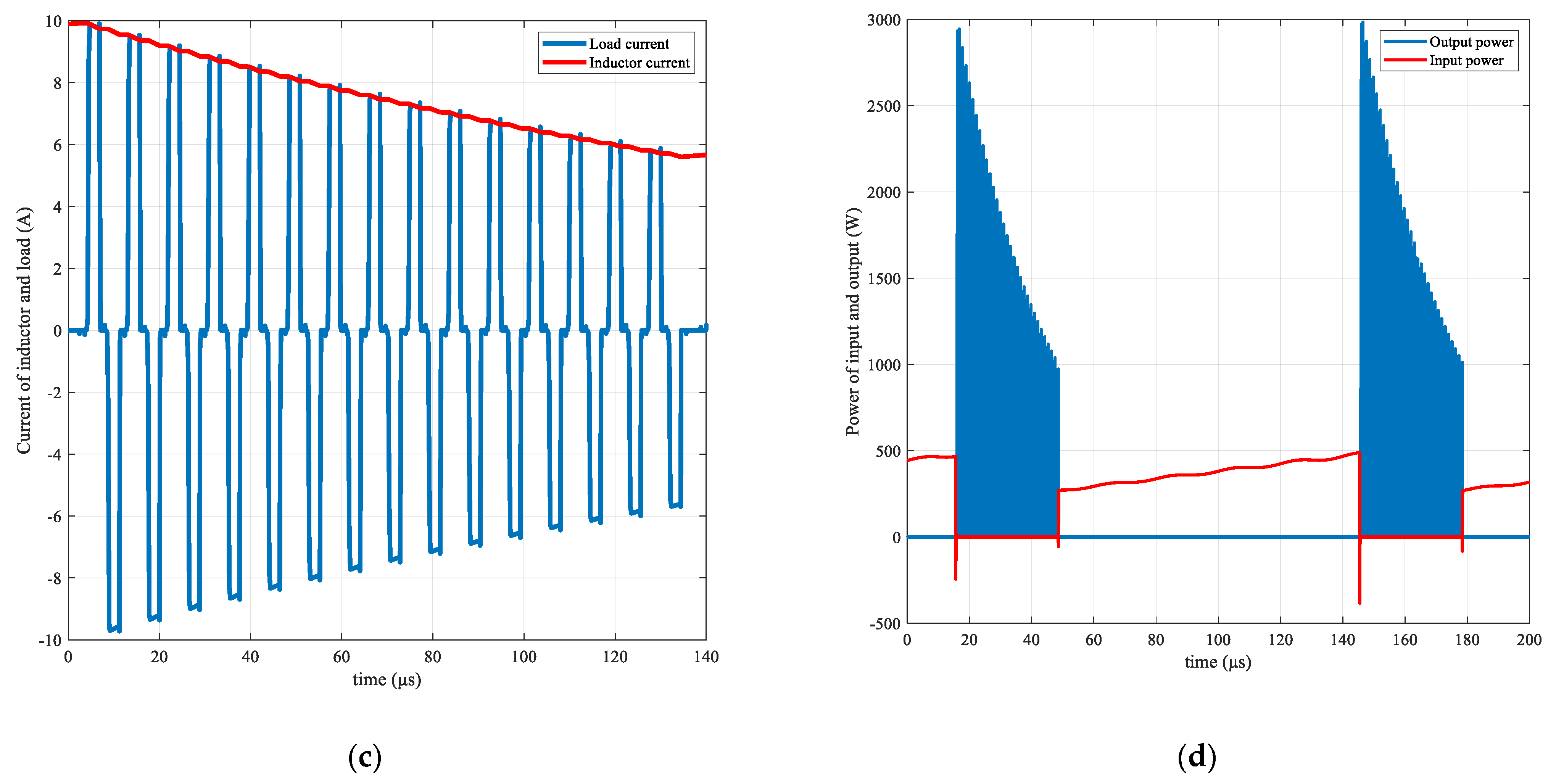
References
- Akiyama, H.; Sakugawa, T.; Namihira, T.; Takaki, K.; Minamitani, Y.; Shimomura, N. Industrial Applications of Pulsed Power Technology. IEEE Trans. Dielectr. Electr. Insul. 2007, 14, 1051–1064. [Google Scholar] [CrossRef] [Green Version]
- Mankowski, J.; Kristiansen, M. A review of short pulse generator technology. IEEE Trans. Plasma Ence 2002, 28, 102–108. [Google Scholar] [CrossRef]
- Curtiss, L.E. High frequency currents in endoscopy: A review of principles and precautions. Gastrointest. Endosc. 1973, 20, 9–12. [Google Scholar] [CrossRef]
- Morris, M.L.; Tucker, R.D.; Baron, T.H.; Song, L.M.W.K. Electrosurgery in gastrointestinal endoscopy: Principles to practice. Am. J. Gastroenterol. 2009, 104, 1563–1574. [Google Scholar] [CrossRef] [PubMed]
- Sujitha, N.; Krithiga, S. RES based EV battery charging system: A review. Renew. Sustain. Energy Rev. 2017, 75, 978–988. [Google Scholar] [CrossRef]
- Huet, F. A review of impedance measurements for determination of the state-of-charge or state-of-health of secondary batteries. J. Power Sources 2012, 70, 59–69. [Google Scholar] [CrossRef]
- Guo, Y.; Zhang, C. Study on the fast charging method of lead-acid battery with negative pulse discharge. In Proceedings of the 4th International Conference on Power Electronics Systems and Applications, Hongkong, China, 8–10 June 2011. [Google Scholar]
- Marcos, J.; Dios, J.; Cao, A.M.; Doval, J.; Poza, F. Fast lead-acid battery charge strategy. In Proceedings of the Twenty-First Annual IEEE Applied Power Electronics Conference and Exposition, 2006, Beijing, China, 15–19 May 2006. [Google Scholar]
- Hua, C.C.; Lin, M.Y. A study of charging control of lead-acid battery for electric vehicles. In Proceedings of the 2000 IEEE International Symposium on Industrial Electronics, Cholula, Mexico, 4–8 December 2000. [Google Scholar]
- Pagar, O.; Darekar, M.; Gawde, S.; Bhartiy, J.; Deshmukh, B. Comparative Evaluation of Fast Charging Systems for the Advanced Electric Vehicles with Pulse Charging & Reflex Charging. In Proceedings of the International Conference on IoT Based Control Networks & Intelligent Systems—ICICNIS 2021, Kottayam, India, 28–29 June 2021. [Google Scholar]
- Lai, C.-M.; Teh, J.; Cheng, Y.-H.; Li, Y.-H. A reflex-charging based bidirectional DC charger for light electric vehicle and DC-microgrids. In Proceedings of the TENCON 2017–2017 IEEE Region 10 Conference, Penang, Malaysia, 5–8 November 2017; pp. 280–284. [Google Scholar]
- Vignan, M.; Hemarani, P. Implementation of Variable Duty Ratio Reflex Charging of Li-ion Batteries. In Proceedings of the 2021 IEEE International Power and Renewable Energy Conference (IPRECON), Kollam, India, 24–26 September 2021; pp. 1–5. [Google Scholar]
- Ahmadi, F.; Adib, E.; Azari, M. Soft Switching Bidirectional Converter for Reflex Charger With Minimum Switches. IEEE Trans. Ind. Electron. 2020, 67, 8355–8362. [Google Scholar] [CrossRef]
- Kumar, G.K.; Elangovan, D. Review on fault-diagnosis and fault-tolerance for DC–DC converters. IET Power Electron. 2020, 13, 1–13. [Google Scholar] [CrossRef]
- Wang, H.; Liserre, M.; Blaabjerg, F.; Peter, D.; Jacobsen, J.B.; Kvisgaard, T.; Landkildehus, J. Transitioning to Physics-of-Failure as a Reliability Driver in Power Electronics. IEEE J. Emerg. Sel. Top. Power Electron. 2014, 2, 97–114. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, H.; Sangwongwanich, A.; Blaabjerg, F. Design for Reliability of Power Electronic Systems. In Power Electronic Handbook, 4th ed.; Butterworth-Heinemann: Oxford, UK, 2018; pp. 1423–1440. [Google Scholar]
- Pang, H.M.; Bryan, P. A life prediction scheme for electrolytic capacitors in power converters without current sensor. In Proceedings of the Applied Power Electronics Conference & Exposition, Palm Springs, CA, USA, 21–25 February 2010. [Google Scholar]
- Hao, M.; Wang, L. Fault diagnosis and failure prediction of aluminum electrolytic capacitors in power electronic converters. In Proceedings of the Conference of IEEE Industrial Electronics Society, Raleigh, NC, USA, 6–10 November 2005. [Google Scholar]
- Kulkarni, C.; Biswas, G.; Koutsoukos, X.; Goebel, K.; Ce Laya, J. Physics of Failure Models for Capacitor Degradation in DC-DC Converters. In Proceedings of the The Maintenance and Reliability Conference, San Antonio, TX, USA, 25–28 May 2010. [Google Scholar]
- Adler, P.; Mallwitz, R. Time-based Reliability Analysis of Electrolytic Capacitors for Automotive Applications Using Multi-Domain Simulation. In Proceedings of the 2021 22nd International Conference on Thermal, Mechanical and Multi-Physics Simulation and Experiments in Microelectronics and Microsystems (EuroSimE), St. Julian, Malta, 19–21 April 2021; pp. 1–6. [Google Scholar]
- Gasperi, M.L. Life prediction model for aluminum electrolytic capacitors. In Proceedings of the IAS Meeting, San Diego, CA, USA, 6–10 October 1996. [Google Scholar]
- Friedrichs, D.A.; Erickson, R.W.; Gilbert, J. A new system architecture improves output power regulation in electrosurgical generators. Annu. Int. Conf. IEEE Eng. Med. Biol. Soc. 2011, 2011, 6870–6873. [Google Scholar] [CrossRef] [PubMed]
- Friedrichs, D.A.; Erickson, R.W.; Gilbert, J. A new dual current-mode controller improves power regulation in electrosurgical generators. IEEE Trans. Biomed. Circuits Syst. 2012, 6, 39–44. [Google Scholar] [CrossRef] [PubMed]
- Sarnago, H.; Lucia, O.; Burdío, J. High performance boost inverter featuring GaN-based devices for electro surgical units. In Proceedings of the Applied Power Electronics Conference & Exposition, Tampa, FL, USA, 26–30 March 2017. [Google Scholar]
- Jiang, C.; Chau, K.T.; Liu, C.; Lee, C.H.T. An Overview of Resonant Circuits for Wireless Power Transfer. Energies 2017, 10, 894. [Google Scholar] [CrossRef]
- Liu, X.; Colli-Menchi, A.; Gilbert, J.; Friedrichs, D.A.; Malang, K.; Sanchez-Sinencio, E. An Automatic Resonance Tracking Scheme With Maximum Power Transfer for Piezoelectric Transducers. IEEE Trans. Ind. Electron. 2015, 62, 7136–7145. [Google Scholar] [CrossRef]
- Wang, X.; Wei, X.; Zhu, J.; Dai, H.; Zheng, Y.; Xu, X.; Chen, Q. A review of modeling, acquisition, and application of lithium-ion battery impedance for onboard battery management. ETransportation 2021, 7, 100093. [Google Scholar] [CrossRef]
- Middlebrook, R.D. Modeling Current-Programmed Buck and Boost Regulators. IEEE Trans. Power Electron. 1989, 4, 36–52. [Google Scholar] [CrossRef] [Green Version]
- Tan, F.D.; Middlebrook, R.D. A Unified Model for Current-Programmed Converters. IEEE Trans. Power Electron. 1995, 10, 397–408. [Google Scholar] [CrossRef] [Green Version]
- Zhang, C.; Liang-Deng, H.U. Research of Active Clamping Circuit for Medium-high Voltage High Power IGBT Driver. Power Electron. 2017, 51, 5. [Google Scholar]
- Xiong, Y.; Sun, S.; Jia, H.; Shea, P.; Shen, Z.J. New Physical Insights on Power MOSFET Switching Losses. IEEE Trans. Power Electron. 2009, 24, 525–531. [Google Scholar] [CrossRef]
- Acquaviva, A.; Rodionov, A.; Kersten, A.; Thiringer, T.; Liu, Y. Analytical conduction loss calculation of a mosfet three-phase inverter accounting for the reverse conduction and the blanking time. IEEE Trans. Ind. Electron. 2020, 68, 6682–6691. [Google Scholar] [CrossRef]
- Yao, C.; Dong, S.; Zhao, Y.; Zhou, Y.; Mi, Y.; Li, C. High-frequency composite pulse generator based on full-bridge inverter and soft switching for biological applications. IEEE Trans. Dielectr. Electr. Insul. 2016, 23, 2730–2737. [Google Scholar] [CrossRef]
- Seeman, M.D.; Ng, V.W.; Le, H.-P.; John, M.; Alon, E.; Sanders, S.R. A comparative analysis of Switched-Capacitor and inductor-based DC-DC conversion technologies. In Proceedings of the 2010 IEEE 12th Workshop on Control and Modeling for Power Electronics (COMPEL), Boulder, CO, USA, 28–30 June 2010; pp. 1–7. [Google Scholar]


| Parameter | Value |
|---|---|
| Power inductor | L = 1 mH, ESR = 50 mΩ |
| Input dc voltage | Ud = 48 V |
| Load impedance | RL = 30 Ω |
| Reference current | Ic = 10 A |
| Pulse width | tpulse = 800 ns |
| deadtime | tdead = 300 ns |
| Number of bipolar pulses in discharge stage | n = 15 |
| Parameter | Value |
|---|---|
| Power inductor | L = 1 mH, ESR = 50 mΩ |
| Input dc voltage | Ud = 48 V |
| Load impedance | RL = 60 Ω |
| Reference current | Ic = 10 A |
| Pulse width | tpulse = 800 ns |
| deadtime | tdead = 300 ns |
| Breakdown voltage of TVS | UBR = 350 V |
| Number of bipolar pulses in discharge stage | n = 15 |
| Parameter | Value |
|---|---|
| Power inductor | L = 1 mH, ESR = 50 mΩ |
| Input dc voltage | Ud = 48 V |
| Reference current | Ic = 10 A |
| Pulse width | tpulse = 800 ns |
| deadtime | tdead = 300 ns |
| Number of bipolar pulses in discharge stage | n = 15 |
| MOSFET Q1~Q5 | SPP21N50C3 |
| Drivers for Q1~Q4 | IR2110STRPBF |
| Driver for Q5 | TLP250H(F) |
| TVS DZ | 1.5KE350A |
| Breakdown voltage of TVS DZ | UBR = 350 V |
| Controller (FPGA) | EP1C3T100C8N |
| Parameter | Value |
|---|---|
| Pulse width | tpulse = 800 ns |
| Time of turn-on process | tturnon = 136 ns |
| Time of turn-off process | tturnoff = 112 ns |
| Time of charging stage | tcharge = 123 μs |
| Inductor current when the charging stage starts | IL0 = 5.10 A |
| Energy of switching loss | Esw(total) = 545.27 μJ |
| Energy of conduction loss | Econ(total) = 503.47 μJ |
| Average power loss of Q1~Q4 | Ploss = 6.72 W |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, J.; Yang, X.; Zhao, H.; Qiu, J.; Liu, K. An Inductor-Based and Capacitor-Free Bipolar Pulse Converter with Overvoltage Protection. World Electr. Veh. J. 2022, 13, 91. https://doi.org/10.3390/wevj13050091
Xu J, Yang X, Zhao H, Qiu J, Liu K. An Inductor-Based and Capacitor-Free Bipolar Pulse Converter with Overvoltage Protection. World Electric Vehicle Journal. 2022; 13(5):91. https://doi.org/10.3390/wevj13050091
Chicago/Turabian StyleXu, Jianzhi, Xingjian Yang, Hui Zhao, Jian Qiu, and Kefu Liu. 2022. "An Inductor-Based and Capacitor-Free Bipolar Pulse Converter with Overvoltage Protection" World Electric Vehicle Journal 13, no. 5: 91. https://doi.org/10.3390/wevj13050091
APA StyleXu, J., Yang, X., Zhao, H., Qiu, J., & Liu, K. (2022). An Inductor-Based and Capacitor-Free Bipolar Pulse Converter with Overvoltage Protection. World Electric Vehicle Journal, 13(5), 91. https://doi.org/10.3390/wevj13050091









