Impacts of Early Thinning of a Eucalyptus globulus Labill. Pulplog Plantation in Western Australia on Economic Profitability and Harvester Productivity
Abstract
1. Introduction
2. Materials and Methods
2.1. Stocking and Harvesting Trial
2.2. Yield and Harvest Productivity Modelling
2.3. Economic Analysis
- LEV = Land Expectation Value per hectare,
- R = rotation length (years),
- E = stand establishment cost per hectare,
- A = annual land leasing cost per hectare,
- It = thinning cost per hectare occurring after plantation establishment and before the final harvest,
- Y = expected pulplog yield (m3) per hectare at the end of the rotation,
- P = mill gate price of pulplogs per m3,
- H = harvesting and transportation cost per m3, and
- r = real interest rate.
3. Results
3.1. Tree and Stand Factors at Time of Harvest
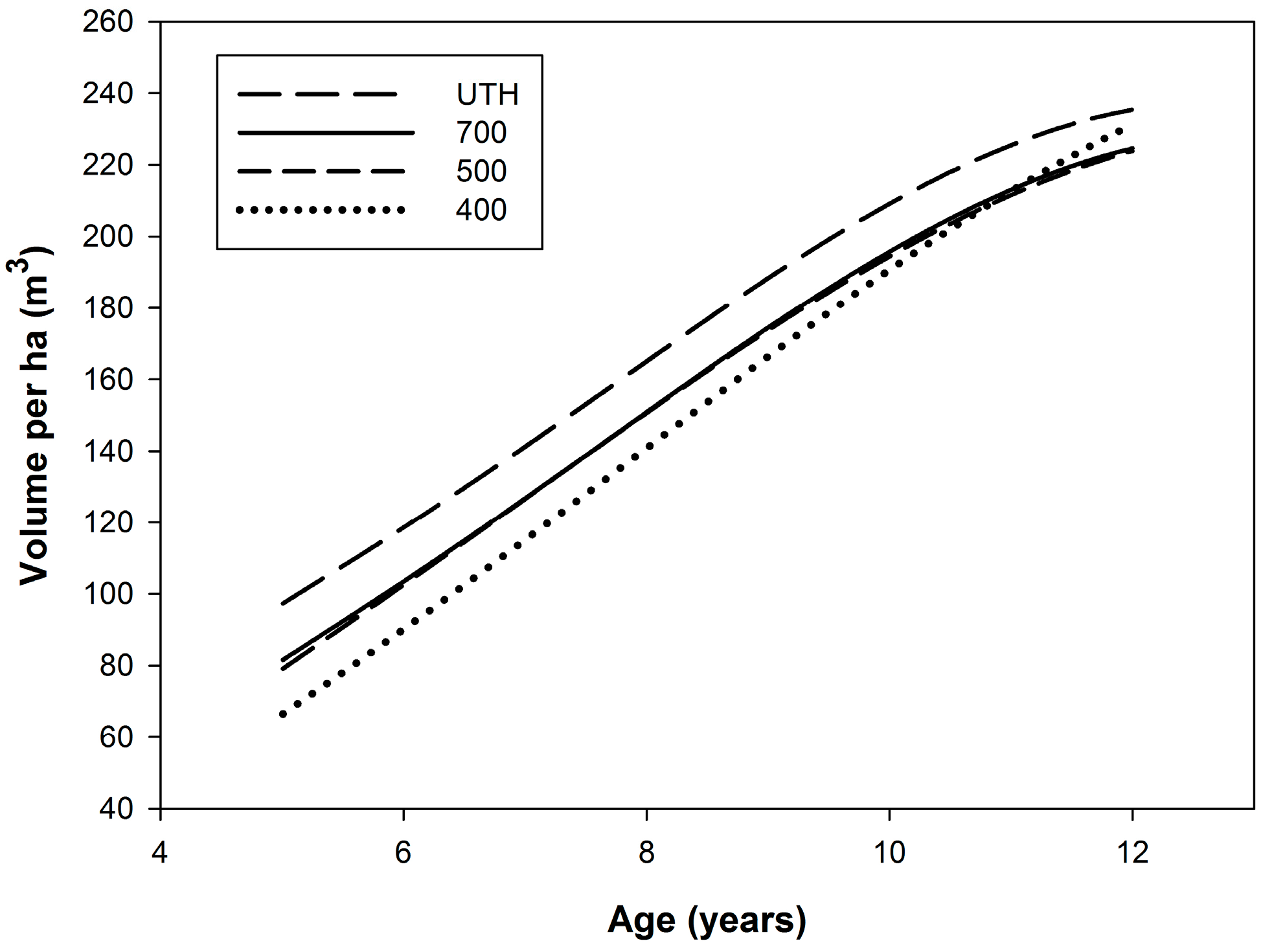
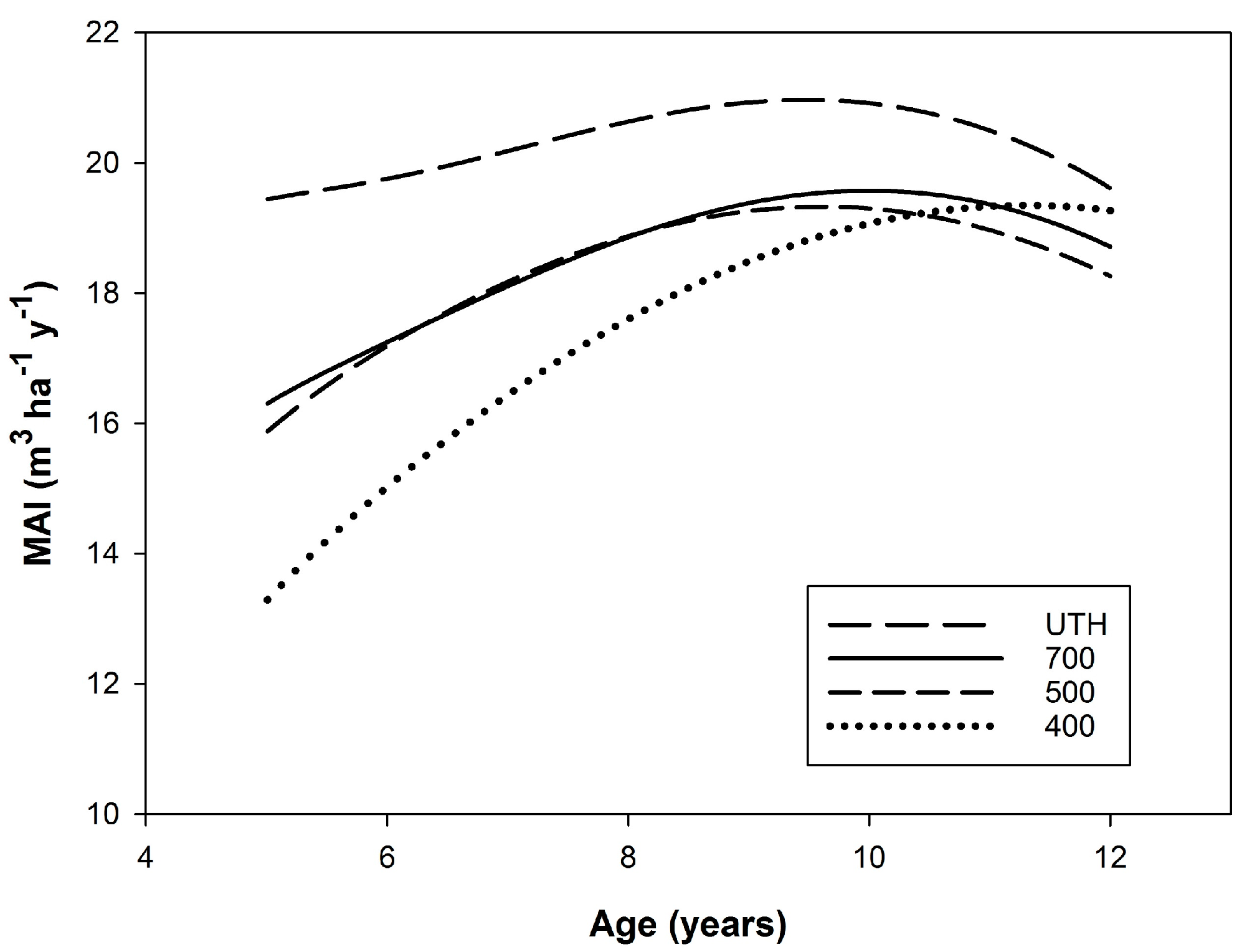
3.2. Yield and MAI Curves
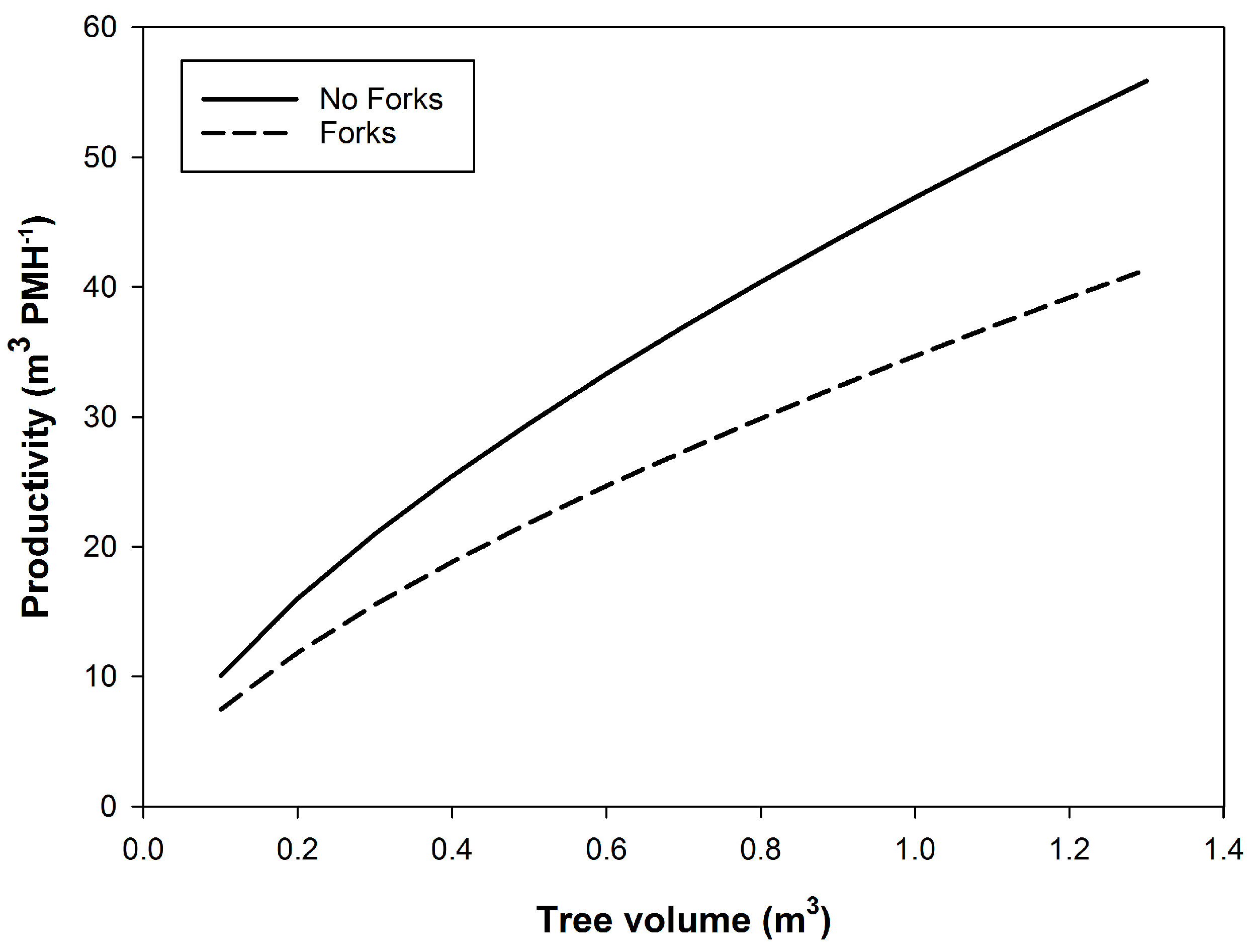
3.3. Harvest Producivity Study and Modelling
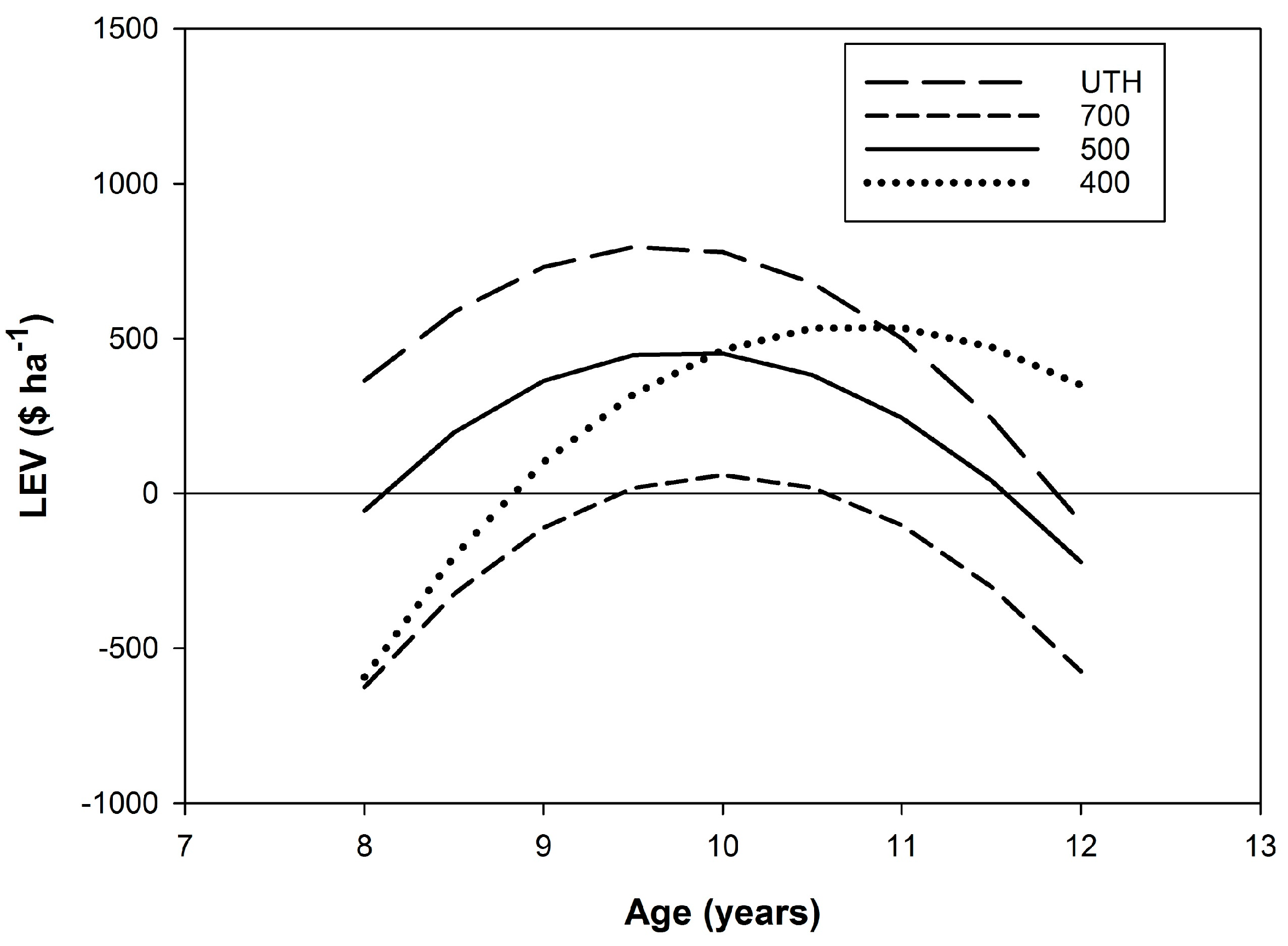
3.4. LEV by Stocking Treatment
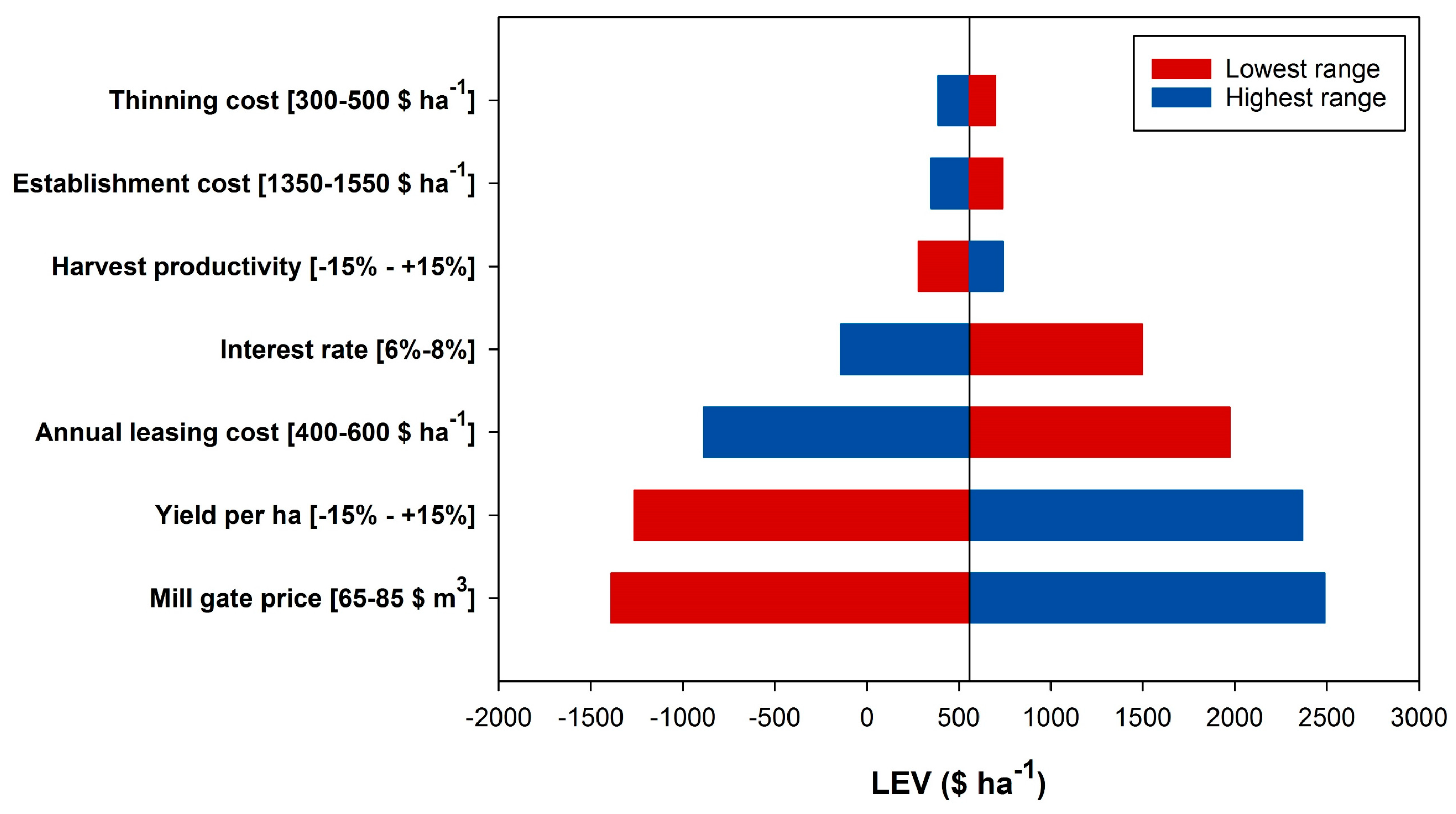
3.5. Sensitivity Analysis on LEV
4. Discussion
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Australian Bureau of Agricultural and Resource Economics and Sciences (ABARES). Australian Plantation Statistics; Australian Bureau of Agricultural and Resource Economics and Sciences: Canberra, Australia, 2016.
- Sutton, W.R.J. Initial spacing and financial return of Pinus radiata on coastal sands. N. Z. J. For. 1968, 13, 203–219. [Google Scholar]
- Peltola, H.; Gort, J.; Pulkkinen, P.; Zubizarreta Gerendiain, A.; Karppinen, J.; Ikonen, V.-P. Differences in growth and wood density traits in Scots pine (Pinus sylvestris L.) genetic entries grown at different spacing and sites. Silva Fenn 2009, 43, 339–354. [Google Scholar] [CrossRef]
- McClain, K.M.; Morris, D.M.; Hills, S.C.; Buse, L.J. The effects of initial spacing on growth and crown development for planted northern conifers: 37-year results. For. Chron. 1994, 70, 174–182. [Google Scholar] [CrossRef]
- Neilsen, W.A.; Gerrand, A.M. Growth and branching habit of Eucalyptus nitens at different spacing and the effect of final crop selection. For. Ecol. Manag. 1999, 123, 217–229. [Google Scholar] [CrossRef]
- Schonau, A.P.G. The effect of planting espacement and pruning on growth, yield and timber density of Eucalyptus grandis. S. Afr. For. J. 1974, 88, 16–23. [Google Scholar]
- Gascon, R.J.; Krinard, R.M. Biological Response of Plantation Cottonwood to Spacing, Pruning and Thinning; Symposium on Eastern Cottonwood and Related Species; Thielges, B.A., Land, S.B., Eds.; Louisiana State University: Baton Rouge, LA, USA, 1976; pp. 385–391. [Google Scholar]
- Meade, D.J. Opportunities for improving plantation productivity. How much? How quickly? How realistic? Biomass Bioenergy 2005, 28, 249–266. [Google Scholar] [CrossRef]
- Pollack, J.C.; Johnstone, W.C.; Coates, K.D.; LePage, P. The Influence of Initial Spacement on the Growth of a 32-Year-Old White Spruce Plantation; Research Note 111; BC Ministry of Forests: Fort Fraser, BC, Canada, 1992; p. 16.
- Poynton, R.J. The Silvicultural Treatment of Eucalypt Plantations in Southern Africa. S. Afr. For. J. 1981, 116, 11–16. [Google Scholar] [CrossRef]
- Gerrand, A.M.; Neilsen, W.A.; Medhurst, J.L. Thinning and pruning eucalypt plantations in Tasmania. Tasforests 1997, 9, 15–34. [Google Scholar]
- Forrester, D.I.; Medhurst, J.L.; Wood, M.; Beadle, C.L.; Valencia, J.C. Growth and physiological responses to silviculture for producing solid-wood products from Eucalyptus plantations: An Australian perspective. For. Ecol. Manag. 2010, 259, 1819–1835. [Google Scholar] [CrossRef]
- Opie, J.E.; Curtin, R.A.; Incoll, W.D. Stand Management. In Eucalypts for Wood Production; Hillis, W.E., Brown, A.G., Eds.; Academic: London, UK, 1984; pp. 179–197. [Google Scholar]
- West, P.W. Growing Plantation Forests, 2nd ed.; Springer: Cham, Switzerland, 2014. [Google Scholar]
- Bulman, L.S. Cyclaneusma needle-cast and Dothistroma needle blight in NZ pine plantations. N. Z. For. 1993, 38, 21–24. [Google Scholar]
- Waring, K.M.; O‘Hara, K.L. Silvicultural strategies in forest ecosystems affected by introduced pests. For. Ecol. Manag. 2005, 209, 27–41. [Google Scholar] [CrossRef]
- Butcher, T.B.; Havel, J.J. Influence of moisture relationships on thinning practice. N. Z. J. For. Sci. 1976, 6, 158–170. [Google Scholar]
- White, D.A.; Crombie, D.S.; Kinal, J.; Battaglia, M.; McGrath, J.F.; Mendham, D.S.; Walker, S.N. Managing productivity and drought risk in Eucalyptus globulus plantations in south-western Australia. For. Ecol. Manag. 2009, 259, 33–44. [Google Scholar] [CrossRef]
- Medhurst, J.L.; Beadle, C.L. Crown structure and leaf area index development in thinned and unthinned Eucalyptus nitens plantations. Tree Physiol. 2001, 21, 989–999. [Google Scholar] [CrossRef] [PubMed]
- Shepherd, K. Plantation Silviculture; Nijhoff Publishers: Doordrecht, The Netherlands, 1986. [Google Scholar]
- Reed, W.; Apaloo, J. Evaluating the Effects of Risk on the Economics of Juvenile Spacing and Commercial Thinning. Can. J. For. Res. 1991, 21, 1390–1400. [Google Scholar] [CrossRef]
- Leask, J.; Smith, R. Guidelines for Plantation Fire Protection; Fires and Emergency Services Authority of Western Australia, FESA: Perth, Australia, 2011; p. 36. [Google Scholar]
- Jactel, H.; Nicoll, B.C.; Branco, M.; Gonzalez-Olabarria, J.R.; Grodzki, W.; Långström, B.; Moreira, F.; Netherer, S.; Orazio, C.; Piou, D.; et al. The influences of forest stand management on biotic and abiotic risks of damage. Ann. For. Sci. 2009, 66, 701. [Google Scholar] [CrossRef]
- Forrester, D.I.; Elms, S.R.; Baker, T.G. Relative, but not absolute, thinning responses decline with increasing thinning age in a Eucalyptus nitens plantation. Aust. For. 2013, 76, 121–127. [Google Scholar] [CrossRef]
- Stone, C.; Penman, T.; Turner, R. Managing drought-induced mortality in Pinus radiata plantations under climate change conditions: A local approach using digital camera data. For. Ecol. Manag. 2012, 265, 94–101. [Google Scholar] [CrossRef]
- Medhurst, J.L.; Beadle, C.L.; Neilsen, W.A. Early-age and later-age thinning affects growth, dominance, and intraspecific competition in Eucalyptus nitens plantations. Can. J. For. Res. 2001, 31, 187–197. [Google Scholar] [CrossRef]
- Acuna, M.A.; Kellogg, L. Evaluation of Alternative Cut-to-Length Harvesting Technology for Native Forest Thinning in Australia. Int. J. For. Eng. 2009, 20, 17–25. [Google Scholar]
- Jiroušek, R.; Klvač, R.; Skoupy, A. Productivity and costs of the mechanised cut-to-length wood harvesting system in clear-felling operations. J. For. Sci. 2007, 53, 476–482. [Google Scholar]
- Spinelli, R.; Owende, P.M.O.; Ward, S. Productivity and cost of CTL harvesting of Eucalyptus globulus stands using excavator-based harvesters. For. Prod. J. 2002, 52, 67–77. [Google Scholar]
- Strandgard, M.; Walsh, D.; Acuna, M.A. Estimating harvester productivity in Pinus radiata plantations using StanForD stem files. Scand. J. For. Res. 2013, 28, 73–80. [Google Scholar] [CrossRef]
- Plamondon, J.; Pitt, D.G. Effects of precommercial thinning on the forest value chain in northwestern New Brunswick: Part 2—Efficiency gains in cut-to-length harvesting. For. Chron. 2013, 89, 458–463. [Google Scholar] [CrossRef]
- Strandgard, M.; Walsh, D.; Mitchell, R. Productivity and cost of whole tree harvesting without debarking in a Eucalyptus nitens plantation in Tasmania, Australia. South For. 2015, 77, 173–178. [Google Scholar]
- Hartsough, B.; Spinelli, R.; Pottle, S.; Klepac, J. Fiber Recovery with Chain Flail Delimbing/Debarking and Chipping of Hybrid Poplar. J. For. Eng. 2000, 11, 59–68. [Google Scholar]
- Nakagawa, M.; Hamatsu, J.; Saitou, T.; Ishida, H. Effect of tree size on productivity and time required for work elements in selective thinning by a harvester. Int. J. For. Eng. 2007, 18, 24–28. [Google Scholar]
- Tong, Q.J.; Zhang, S.Y.; Thompson, M. Evaluation of growth response, stand value and financial return for pre-commercially thinned jack pine stands in Northwestern Ontario. For. Ecol. Manag. 2005, 209, 225–235. [Google Scholar] [CrossRef]
- Samuelson, P. The economics of forestry in an evolving society. Econ. Inq. 1976, 14, 466–492. [Google Scholar] [CrossRef]
- Faustmann, M. Calculation of the value which forestland and immature stands possess for forestry. J. For. Econ. 1995, 1, 7–44. [Google Scholar]
- Brazee, R.; Mendelsohn, R. Timber Harvesting with Fluctuating Timber Prices. For. Sci. 1988, 34, 359–372. [Google Scholar]
- Hartman, R. The Harvesting Decision when a forest stand has value. Econ. Inq. 1976, 14, 52–55. [Google Scholar] [CrossRef]
- Shedley, E.; Adams, M. Parrot Damage in Tasmanian Bluegum Plantations in the South-West of Western Australia. In Proceedings of the Eleventh Australian Vertebrate Pest Control Conference, Bunbury, WA, Australia, 3–8 May 1998; pp. 247–253. [Google Scholar]
- Abdi, H. Bonferroni and Šidák corrections for multiple comparisons. In Encyclopedia of Measurement and Statistics; Salkind, N.J., Ed.; Sage: Thousand Oaks, CA, USA, 2007. [Google Scholar]
- Holm, S. A simple sequentially rejective multiple test procedure. Scand. J. Stat. 1979, 6, 65–70. [Google Scholar]
- Chang, S.J. The determination of the optimal rotation age: A theoretical analysis. For. Ecol. Manag. 1984, 8, 137–147. [Google Scholar] [CrossRef]
- Coordes, R. Optimal Thinning within the Faustmann Approach; Springer Vieweg: Berlin, Germany, 2014; p. 246. [Google Scholar]
- Strandgard, M.; Mitchell, R. Impact of number of stems per stool on mechanical harvesting of a Eucalyptus globulus coppiced plantation in south-west Western Australia. South For. 2017, 1–6. [Google Scholar] [CrossRef]
- Hamilton, M.G.; Acuna, M.; Wiedemann, J.C.; Mitchell, R.; Pilbeam, D.J.; Brown, M.W.; Potts, B.M. Genetic control of Eucalyptus globulus harvest traits. Can. J. For. Res. 2015, 45, 615–624. [Google Scholar] [CrossRef]
- Labelle, E.R.; Soucy, M.; Cyr, A.; Pelletier, G. Effect of Tree Form on the Productivity of a Cut-to-Length Harvester in a Hardwood Dominated Stand. Croat. J. For. Eng. 2016, 37, 175–183. [Google Scholar]
- Strandgard, M.; Mitchell, R.; Acuna, M. General productivity model for single grip harvesters in Australian eucalypt plantations. Aust. For. 2016, 79, 108–113. [Google Scholar] [CrossRef]
- Van den Berg, G.J.; Little, K.M. Effect of rainfall and under-canopy vegetation on the ability to debark Eucalyptus grandis x E. camaldulensis when felled in Zululand, South Africa. S. Afr. For. J. 2004, 200, 71–75. [Google Scholar]
- Ovaskainen, H.; Uusitalo, J.; Väätäinen, K. Characteristics and Significance of a Harvester Operators’ Working Technique in Thinnings. Int. J. For. Eng. 2004, 15, 67–76. [Google Scholar]
- Sutton, W.R.J. Comparison of Alternative Silvicultural Regimes for Radiata Pine. N. Z. J. For. Sci. 1976, 6, 350–356. [Google Scholar]
- Yang, J.-L.; Fife, D.; Waugh, G.; Downes, G.; Blackwell, P. The effect of growth strain and other defects on the sawn timber quality of 10-year-old Eucalyptus globulus Labill. Aust. For. 2002, 65, 31–37. [Google Scholar] [CrossRef]
- McGavin, R.L.; Bailleres, H.; Hamilton, M.; Blackburn, D.; Vega, M.; Ozarska, B. Variation in Rotary Veneer Recovery from Australian Plantation Eucalyptus globulus and Eucalyptus nitens. Bioresources 2015, 10, 313–329. [Google Scholar] [CrossRef][Green Version]
- Nolan, G.B.; Greaves, B.L.; Washusen, R.; Parsons, M.; Jennings, S. Eucalypt Plantations for Solid Wood Products in Australia—A Review ‘If You Don’t Prune It, We Can’t Use It’; PN04.3002; Forest Wood Products Research and Develoment Corporation: Melbourne, VIC, Australia, 2005; p. 130. [Google Scholar]

| Work Element | Description |
|---|---|
| Felling | Begins when crane starts to engage the tree and ends when processing commences |
| Processing | Debarking, delimbing, and bucking (i.e., cross-cutting) of logs. Commences when tree is horizontal or feed rollers commence turning |
| Brushing or clearing | Removal or movement of slash, undergrowth, or unmerchantable trees |
| Moving | Not associated with felling and processing, harvester moving within a pass (3 harvested rows per pass) |
| Travelling | Movement between passes or bays |
| Delay | Any interruption that causes the harvester to cease working during a shift |
| Tree and Stand Factors | Target Stocking (Trees ha−1) | |||
|---|---|---|---|---|
| UTH | 700 | 500 | 400 | |
| Number of treatment plots | 3 | 3 | 6 | 6 |
| Actual merchantable stocking, trees ha−1 | 978 | 637 | 489 | 393 |
| Mean tree diameter (DBHOB), mm | 167 a | 205 b | 226 b | 253 c |
| Mean tree height, m | 19.8 a | 21.4 ab | 22.2 ab | 23.7 b |
| Mean standing tree volume, m3 tree−1 | 0.233 a | 0.286 ab | 0.366 b | 0.464 c |
| Stem form (Forking), % of trees | ||||
| Class 1 | 62 | 62 | 77 | 77 |
| Class 2 | 38 | 38 | 23 | 23 |
| Merchantable yield, tonnes ha−1 * | 194.6 | 179.9 | 178.1 | 180.2 |
| Differential | −8% | −8% | −7% | |
| UTH | 700 | 500 | 400 | |||||
|---|---|---|---|---|---|---|---|---|
| Work Element | Mean time per cycle, sec. | % of cycle time | Mean time per cycle, sec. | % of cycle time | Mean time per cycle, sec. | % of cycle time | Mean time per cycle, sec. | % of cycle time |
| Felling | 16.1 | 17.6 | 14.4 | 16.9 | 14.3 | 14.8 | 15.7 | 15.8 |
| Processing | 71.3 a | 78.1 | 66.5 a | 78.2 | 77.3 b | 79.9 | 77.8 b | 78.2 |
| Brushing or cleaning | 0.8 | 0.8 | 0.12 | 0.1 | 0.26 | 0.3 | 0.20 | 0.2 |
| Moving | 3.0 a | 3.3 | 3.8 ab | 4.5 | 4.7 bc | 4.9 | 5.6 c | 5.6 |
| Travelling | 0.2 | 0.2 | 0.2 | 0.2 | 0.2 | 0.2 | 0.2 | 0.2 |
| Total | 91.4 a | 100.0 | 85.0 a | 100.0 | 96.8 b | 100.0 | 99.5 b | 100.0 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Acuna, M.; Strandgard, M.; Wiedemann, J.; Mitchell, R. Impacts of Early Thinning of a Eucalyptus globulus Labill. Pulplog Plantation in Western Australia on Economic Profitability and Harvester Productivity. Forests 2017, 8, 415. https://doi.org/10.3390/f8110415
Acuna M, Strandgard M, Wiedemann J, Mitchell R. Impacts of Early Thinning of a Eucalyptus globulus Labill. Pulplog Plantation in Western Australia on Economic Profitability and Harvester Productivity. Forests. 2017; 8(11):415. https://doi.org/10.3390/f8110415
Chicago/Turabian StyleAcuna, Mauricio, Martin Strandgard, John Wiedemann, and Rick Mitchell. 2017. "Impacts of Early Thinning of a Eucalyptus globulus Labill. Pulplog Plantation in Western Australia on Economic Profitability and Harvester Productivity" Forests 8, no. 11: 415. https://doi.org/10.3390/f8110415
APA StyleAcuna, M., Strandgard, M., Wiedemann, J., & Mitchell, R. (2017). Impacts of Early Thinning of a Eucalyptus globulus Labill. Pulplog Plantation in Western Australia on Economic Profitability and Harvester Productivity. Forests, 8(11), 415. https://doi.org/10.3390/f8110415





