The Influence of Different Moisture Contents on the Acoustic Vibration Characteristics of Wood
Abstract
1. Introduction
2. Materials and Methods
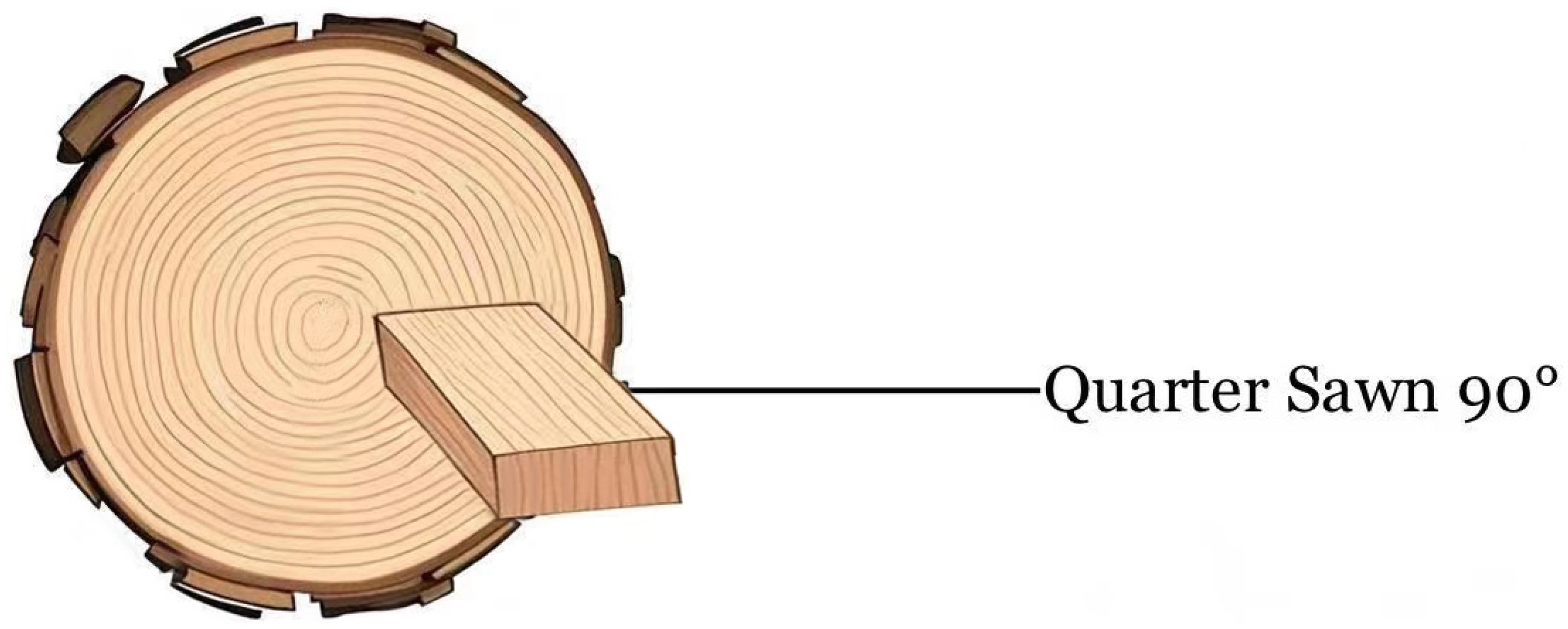
2.1. Experiment Materials
2.2. Adjustment of Specimen Moisture Content
2.3. Experimental Device
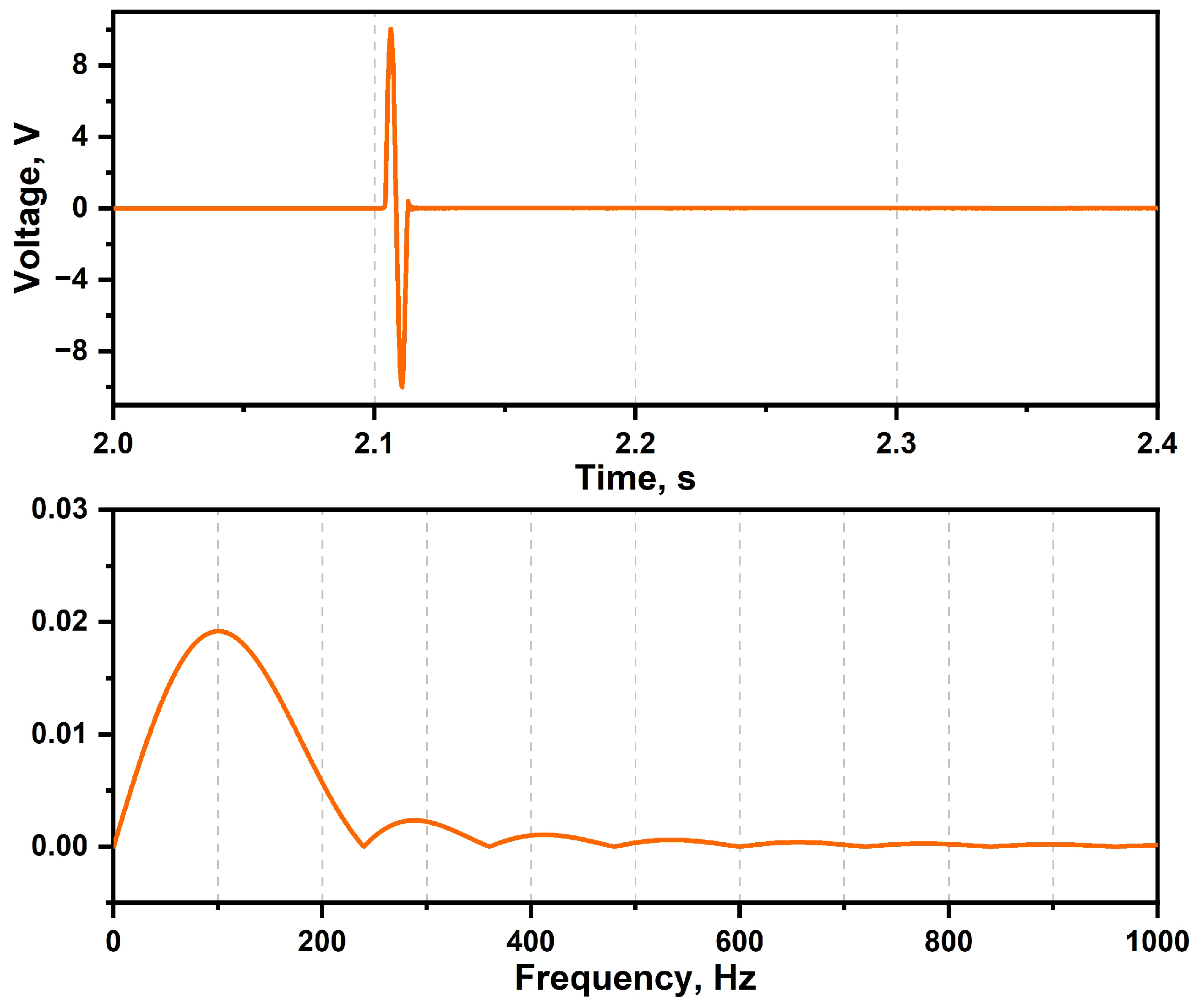
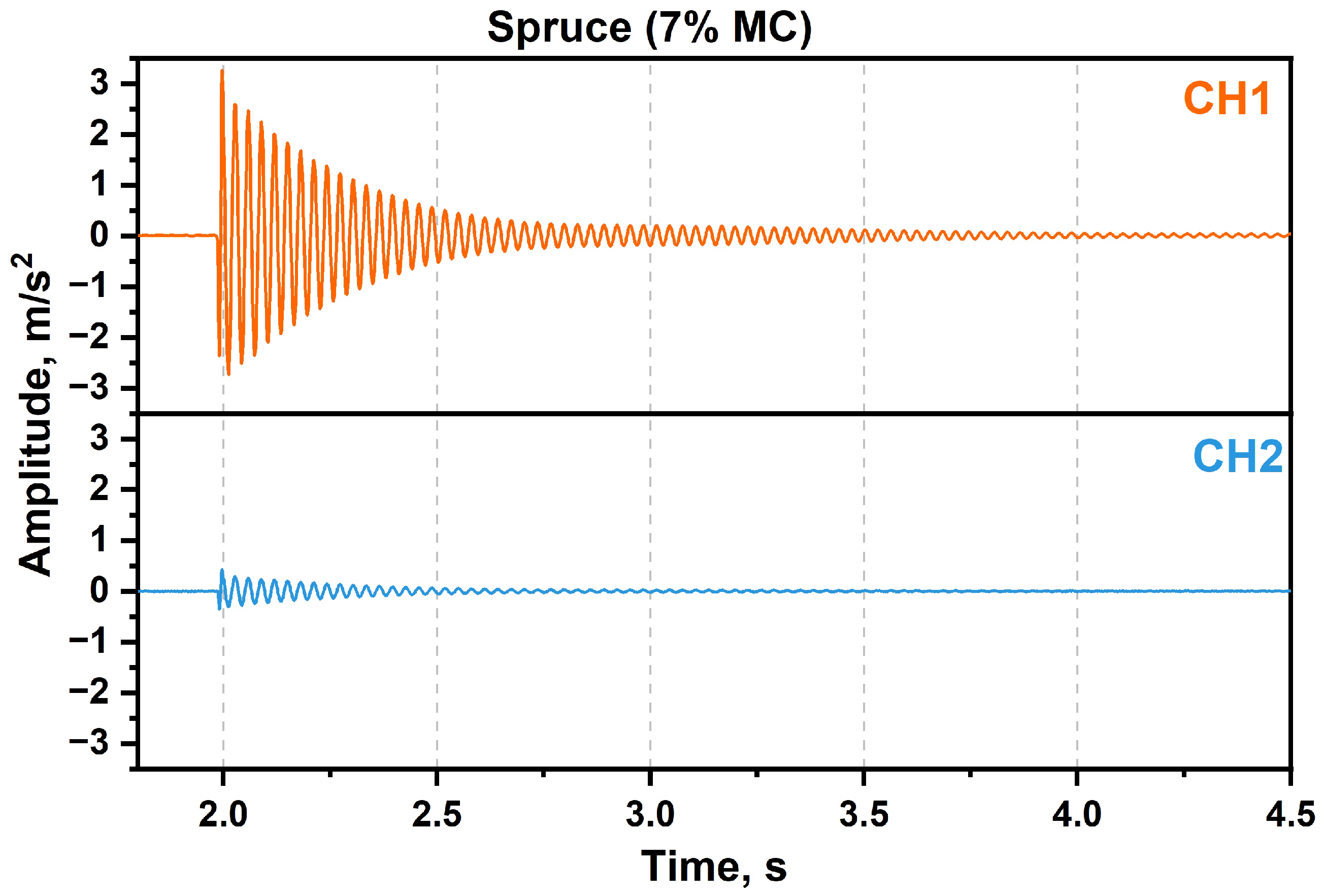
2.4. Experimental Method
3. Results and Discussion
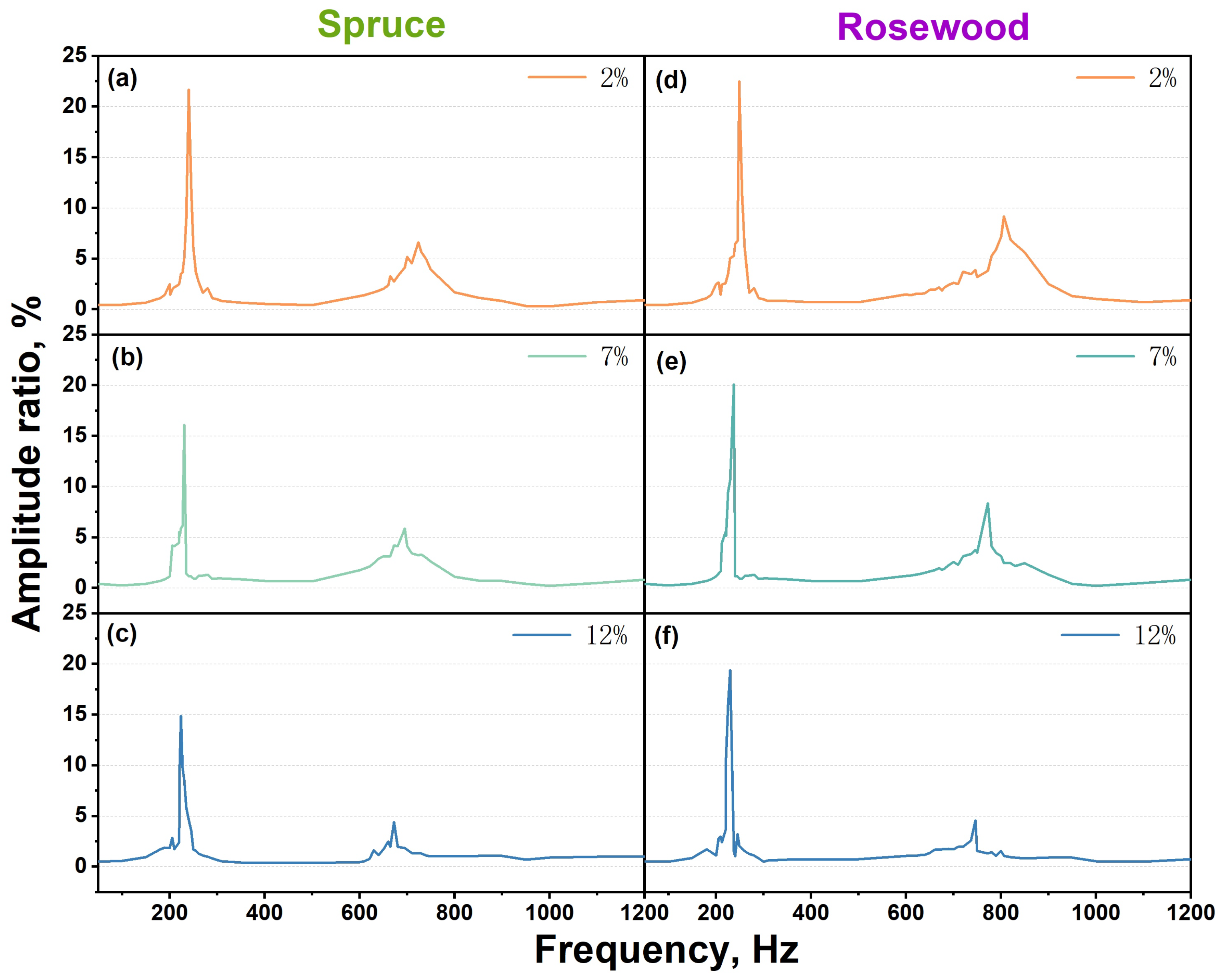
3.1. The Influence of Moisture Content on the Resonance Frequency of Specimens
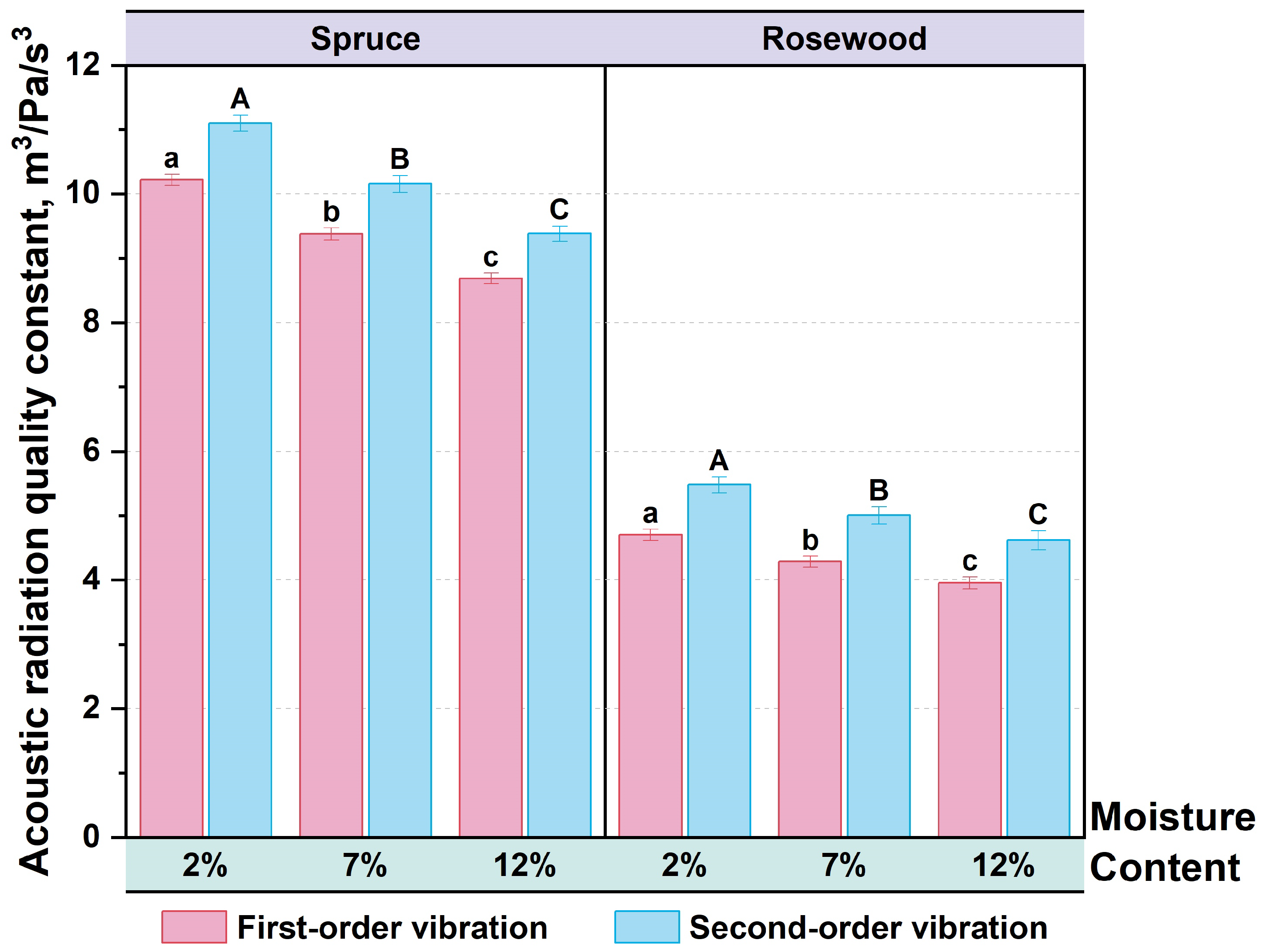
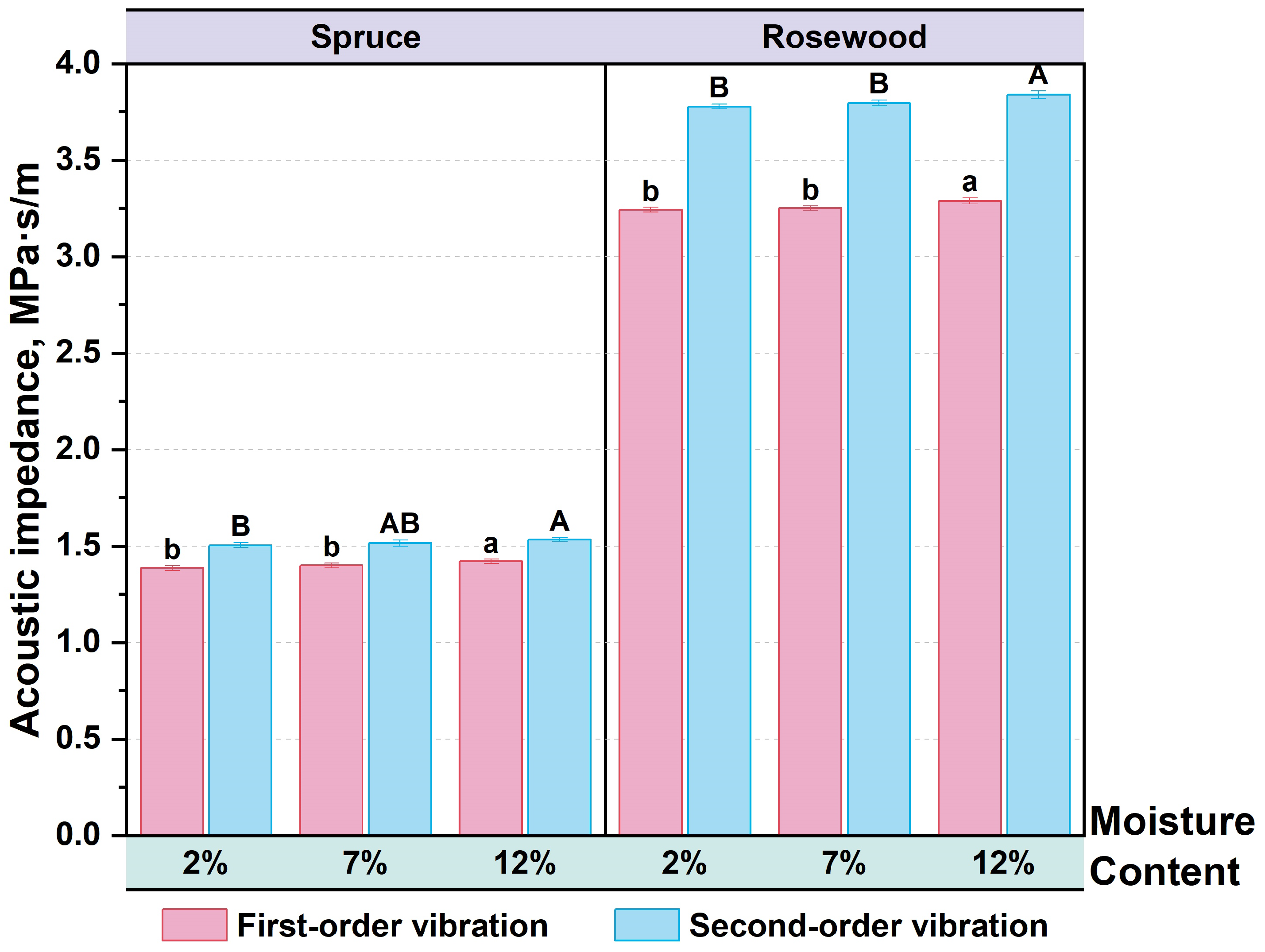
3.2. The Influence of Moisture Content on the Acoustic Vibration Parameters of Specimens
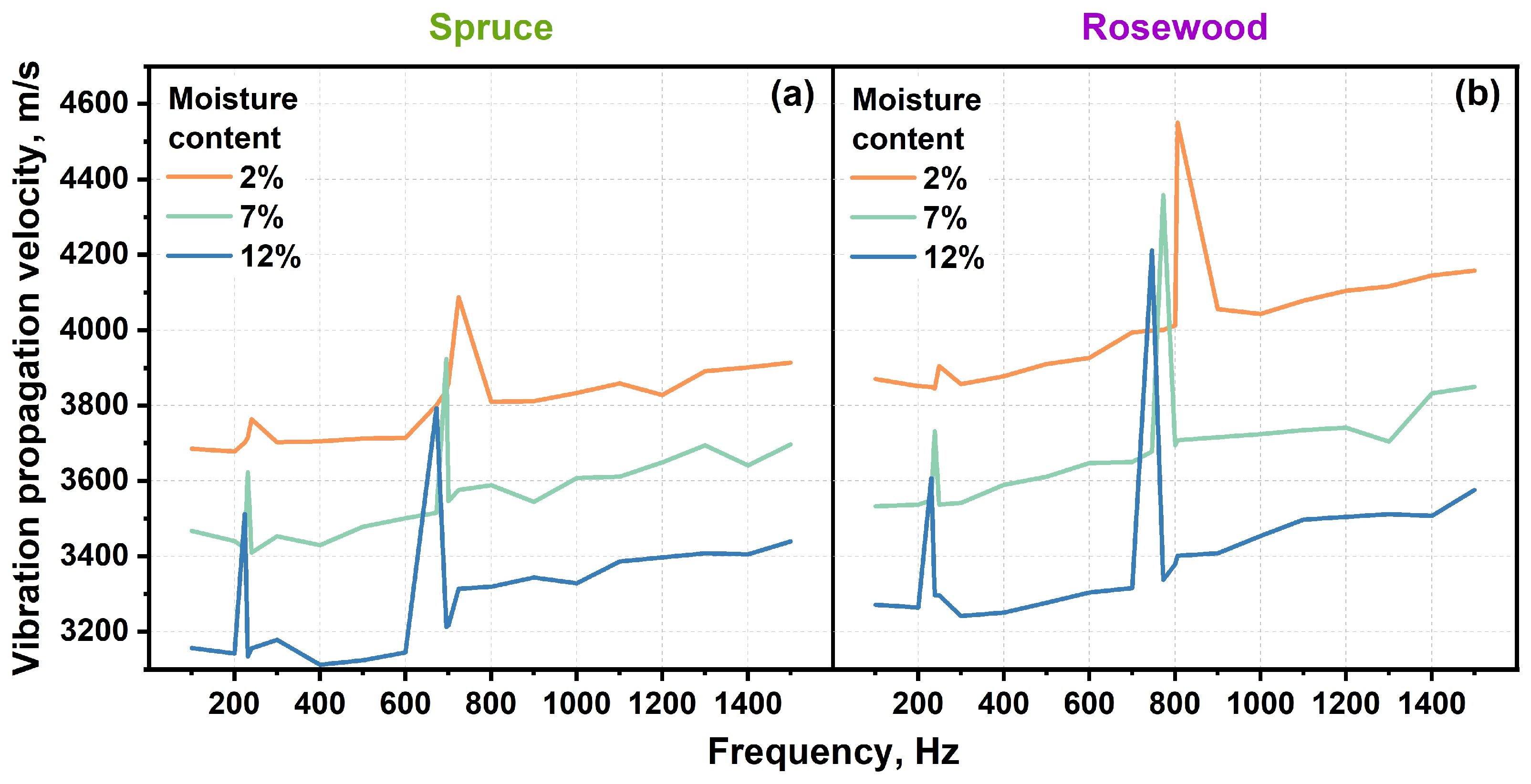
3.3. The Influence of Moisture Content on the Vibration Propagation Velocity of Specimens
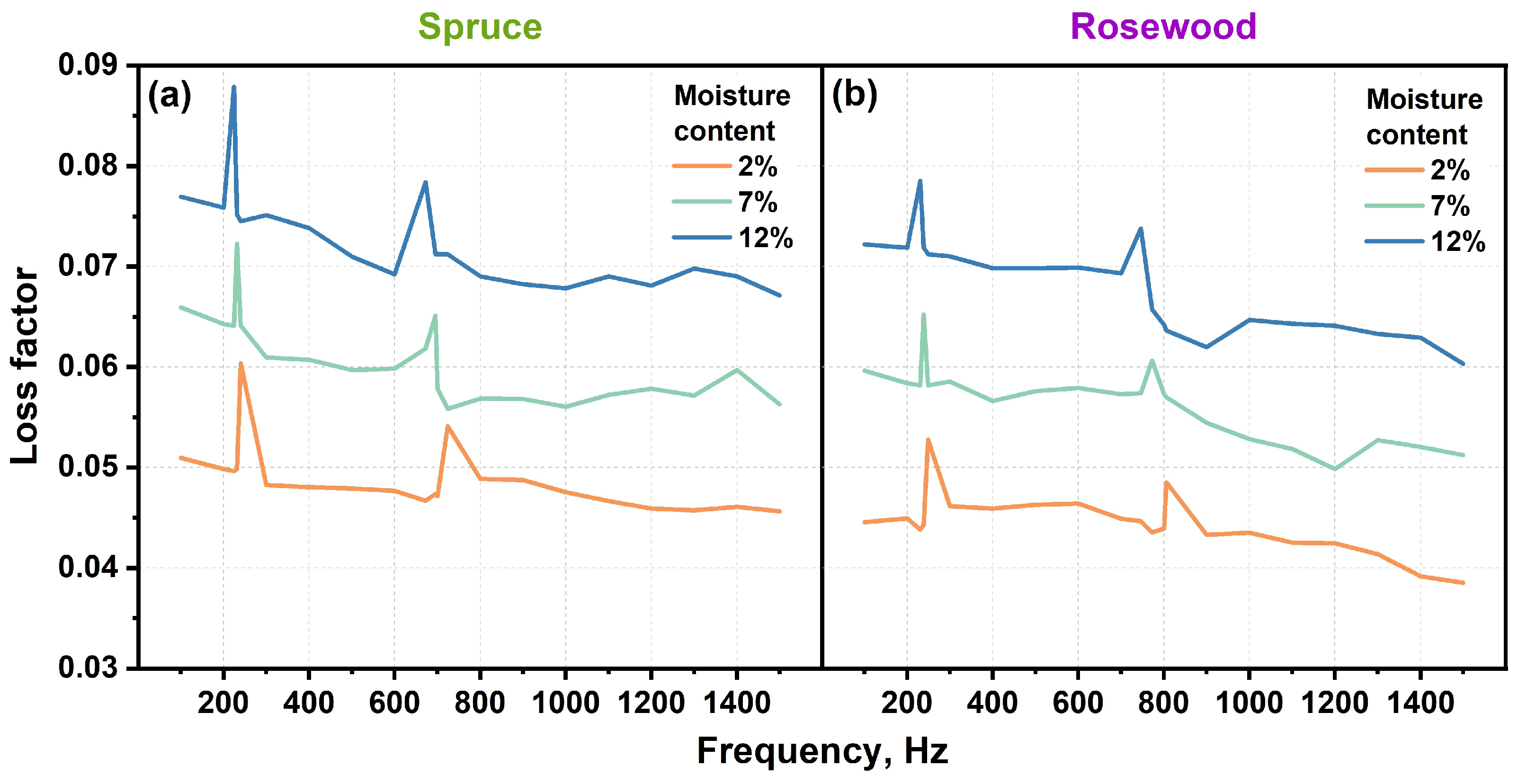
3.4. The Influence of Moisture Content on the Loss Factor of Specimens
4. Conclusions
- The first-order and second-order resonance frequencies and amplitude ratios of spruce and rosewood specimens decreased with the increase in moisture content. At the same moisture content, the resonance frequencies and amplitude ratios at the first-order and second-order resonance points of the rosewood specimen were higher than those of the spruce specimen.
- The first-order and second-order dynamic elastic modulus, specific dynamic elastic modulus, acoustic radiation quality constant, and acoustic conversion efficiency of spruce and rosewood specimens decreased with increasing moisture content, while acoustic impedance increased significantly with increasing moisture content. The decreases in these parameters of the rosewood specimen were higher than those of the spruce specimen.
- As the moisture content increased, the vibration transmission velocity of the spruce and rosewood specimens decreased. Except at the resonance frequency point, the vibration transmission velocity increased with the increase in signal frequency. At the first-order and second-order resonance frequencies, the vibration transmission velocity of the spruce and rosewood specimens significantly increased. The increase in the vibration propagation velocity of the specimens at the first-order resonance frequency was lower than that at the second-order resonance frequency.
- With the increase in moisture content, the loss factor of the spruce and rosewood specimens significantly increased. Additionally, as frequency increased, the loss factor of the spruce and rosewood specimens showed a decreasing trend. At the first-order and second-order resonance frequencies, the loss factor of spruce and rosewood significantly increased. The increase in the loss factor of specimens at the first-order resonance frequency was higher than that at the second-order resonance frequency.
- The acoustic vibration characteristics of the spruce and rosewood specimens decreased with increasing moisture content. In actual production, excessively dry wood may crack and warp, and even affect the sound quality of musical instruments. Lower moisture content results in higher acoustic vibration characteristics. Therefore, between 7% and 12%, it is more recommended to use wood with a moisture content of 7%.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Essien, C. Fundamental Considerations and Application of Acoustics as a Nondestructive Evaluation Technique of Wood Quality Properties. Ph.D. Thesis, Auburn University, Auburn, AL, USA, 2017. [Google Scholar]
- Lewandowski, M.; Foltynowicz, Z. Selected aspects of resonance properties of different woods and the construction of string instruments. Drewno 2022, 65, 1–16. [Google Scholar] [CrossRef]
- Xu, H.; Xu, G.; Wang, L.; Yu, L. Propagation behavior of acoustic wave in wood. J. For. Res. 2014, 25, 671–676. [Google Scholar] [CrossRef]
- Kamperidou, V.; Aidinidis, E.; Barboutis, I. Impact of structural defects on the surface quality of hardwood species sliced veneers. Appl. Sci. 2020, 10, 6265. [Google Scholar] [CrossRef]
- Dinulica, F.; Stanciu, M.D.; Savin, A. Correlation between Anatomical Grading and Acoustic-Elastic Properties of Resonant Spruce Wood Used for Musical Instruments. Forests 2021, 12, 1122. [Google Scholar] [CrossRef]
- Yang, Y. Vibration properties of Paulownia wood for Ruan sound quality using machine learning methods. J. For. Res. 2024, 35, 9. [Google Scholar] [CrossRef]
- Spycher, M.; Schwarze, F.W.M.R.; Steiger, R. Assessment of resonance wood quality by comparing its physical and histological properties. Wood Sci. Technol. 2008, 42, 325–342. [Google Scholar] [CrossRef]
- Bucur, V. A Review on Acoustics of Wood as a Tool for Quality Assessment. Forests 2023, 14, 1545. [Google Scholar] [CrossRef]
- Obataya, E. Effects of natural and artificial ageing on the physical and acoustic properties of wood in musical instruments. J. Cult. Herit. 2017, 27, S63–S69. [Google Scholar] [CrossRef]
- Rolleri, A.; Wentzel, M.; Aguilera, A.; Barros, J.L. Vibroacoustic properties as a function of crystallinity changes in heat-treated Pinus radiata D. Don wood. Wood Mater. Sci. Eng. 2024, 19, 247–252. [Google Scholar] [CrossRef]
- Zhu, L.j.; Jiang, T.; Wu, G. Improvement of Acoustic-Vibration Performance of E. urophylla by High-Temperature Heat Treatment. In Proceedings of the 6th International Conference on Measurement, Instrumentation and Automation (ICMIA), Zhuhai, China, 29–30 June 2017; Volume 154, pp. 148–153. [Google Scholar]
- Mania, P.; Molinski, W.; Roszyk, E.; Gorska, M. Optimization of Spruce (Picea abies L.) Wood Thermal Treatment Temperature to Improve Its Acoustic Properties. Bioresources 2020, 15, 505–516. [Google Scholar] [CrossRef]
- Buchelt, B.; Krueger, R.; Wagenfuehr, A. The vibrational properties of native and thermally modified wood in dependence on its moisture content. Eur. J. Wood Wood Prod. 2023, 81, 947–956. [Google Scholar] [CrossRef]
- Karami, E.; Bardet, S.; Matsuo, M.; Bremaud, I.; Gaff, M.; Gril, J. Effects of mild hygrothermal treatment on the physical and vibrational properties of spruce wood. Compos. Struct. 2020, 253, 112736. [Google Scholar] [CrossRef]
- Li, J.Z.; Furuno, T.; Zhou, W.R.; Ren, Q.; Han, X.Z.; Zhao, J.P. Properties of Acetylated Wood Prepared at Low Temperature in the Presence of Catalysts. J. Wood Chem. Technol. 2009, 29, 241–250. [Google Scholar] [CrossRef]
- Obataya, E.; Minato, K.; Tomita, B. Influence of moisture content on the vibrational properties of hematoxylin-impregnated wood. J. Wood Sci. 2001, 47, 317–321. [Google Scholar] [CrossRef]
- Hassen, M.F.; Hamdan, S.; Rahman, M.R. Investigation of the Acoustic Properties of Chemically Impregnated Kayu Malam Wood Used for Musical Instrument. Adv. Mater. Sci. Eng. 2018, 2018, 7829613. [Google Scholar] [CrossRef]
- Zeniya, N.; Endo-Ujiie, K.; Obataya, E.; Nakagawa-Izumi, A.; Matsuo-Ueda, M. Effects of water-soluble extractives on the vibrational properties and color of hygrothermally treated spruce wood. Wood Sci. Technol. 2019, 53, 151–164. [Google Scholar] [CrossRef]
- Arnold, M. Effect of moisture on the bending properties of thermally modified beech and spruce. J. Mater. Sci. 2010, 45, 669–680. [Google Scholar] [CrossRef]
- Bremaud, I.; Gril, J. Moisture content dependence of anisotropic vibrational properties of wood at quasi equilibrium: Analytical review and multi-trajectories experiments. Holzforschung 2021, 75, 313–327. [Google Scholar] [CrossRef]
- Viala, R.; Cabaret, J.; Sedighi-Gilani, M.; Placet, V.; Cogan, S. Effect of indented growth rings on spruce wood mechanical properties and subsequent violin dynamics. Holzforschung 2024, 78, 189–201. [Google Scholar] [CrossRef]
- Ono, T.; Norimoto, M. Anisotropy of dynamic Young’s modulus and internal friction in wood. Jpn. J. Appl. Phys. 1985, 24, 960. [Google Scholar] [CrossRef]
- Matsunaga, M.; Sugiyama, M.; Minato, K.; Norimoto, M. Physical and Mechanical Properties Required for Violin Bow Materials. Holzforschung 1996, 50, 511–517. [Google Scholar] [CrossRef]
- Kubojima, Y.; Okano, T.; Ohta, M. Vibrational properties of Sitka spruce heat-treated in nitrogen gas. J. Wood Sci. 1998, 4, 73–77. [Google Scholar] [CrossRef]
- Danihelova, A.; Spisiak, D.; Reinprecht, L.; Gergel, T.; Vidholdova, Z.; Ondrejka, V. Acoustic Properties of Norway Spruce Wood Modified with Staining Fungus (Sydowia polyspora). Bioresources 2019, 14, 3432–3444. [Google Scholar] [CrossRef]
- Mirbolouk, P.; Roohnia, M. Evaluation of Dynamic Modulus of Elasticity of Medium Density Fiberboard Panel from Longitudinal Vibration Tests on Specimens. Bioresources 2015, 10, 613–621. [Google Scholar] [CrossRef]
- Zhang, L.; He, L.; Liang, Y.; Zhang, J.; Zhang, H.; Zhou, J.; Cui, H.; Li, M.; Miao, Y.; Liu, Z. Modulating the Acoustic Vibration Performance of Wood by Introducing a Periodic Annular Groove Structure. Forests 2023, 14, 2360. [Google Scholar] [CrossRef]
- Deng, L.; Chen, X.; Chen, F.; Liu, X.e.; Jiang, Z. Effect of Environmental Humidity on the Acoustic Vibration Characteristics of Bamboo. Forests 2022, 13, 329. [Google Scholar] [CrossRef]
- Kohantorabi, M.; Hossein, M.A.; Shahverdi, M.; Roohnia, M. Vibration Based NDT Methods to Verify Wood Drying Efficiency. Drv. Ind. 2015, 66, 221–228. [Google Scholar] [CrossRef]
- Ahmed, S.A.; Adamopoulos, S. Acoustic properties of modified wood under different humid conditions and their relevance for musical instruments. Appl. Acoust. 2018, 140, 92–99. [Google Scholar] [CrossRef]
- Guan, C.; Zhang, H.; Zhou, L.; Wang, X. Dynamic determination of modulus of elasticity of full-size wood composite panels using a vibration method. Constr. Build. Mater. 2015, 100, 201–206. [Google Scholar] [CrossRef]
- Guan, C.; Zhang, H.; Hunt, J.F.; Yan, H. Determining shear modulus of thin wood composite materials using a cantilever beam vibration method. Constr. Build. Mater. 2016, 121, 285–289. [Google Scholar] [CrossRef]
- Wang, Z.; Xu, B.; Sayed, U.; Gu, X.; Qi, L.; Wang, X.; Wang, J. Testing of wood shear modulus based on cantilevered square plate torsional vibration method. Wood Mater. Sci. Eng. 2024, 1–13. [Google Scholar] [CrossRef]
- ASTM D6874-12; Standard Test Methods for Nondestructive Nvaluation of Wood-Based Flexural Members Using Transverse Vibration. ASTM International: West Conshohocken, PA, USA, 2012.
- Yang, H.; Yu, L.; Wang, L. Effect of moisture content on the ultrasonic acoustic properties of wood. J. For. Res. 2015, 26, 753–757. [Google Scholar] [CrossRef]
- Dumond, P.; Baddour, N. Experimental investigation of the mechanical properties and resonance frequencies of simply supported Sitka spruce plates. Wood Sci. Technol. 2015, 49, 1137–1155. [Google Scholar] [CrossRef]
- Yoshihara, H.; Maruta, M. Determining the Young’s modulus of solid wood by considering the fundamental frequency under the free-free flexural vibration mode. Wood Sci. Technol. 2021, 55, 919–936. [Google Scholar] [CrossRef]
- Bucur, V. Mechanical Characterisation of Materials for String Instrument. In Handbook of Materials for String Musical Instruments; Springer International Publishing: Cham, Switzerland, 2016; pp. 93–131. [Google Scholar]
- Brancheriau, L.; Baillères, H. Natural vibration analysis of clear wooden beams: A theoretical review. Appl. Sci. 2002, 36, 347–365. [Google Scholar] [CrossRef]
- Wegst, U.G.K. Wood for sound. Am. J. Bot. 2006, 93, 1439–1448. [Google Scholar] [CrossRef]
- Miao, Y.; Zhong, M.; Liu, Z.; Sun, F. Analysis of Wood Vibration Energy Attenuation Based on FFT Vibration Signal. Bioresources 2015, 10, 272–281. [Google Scholar] [CrossRef][Green Version]
- Fredriksson, M.; Rueggeberg, M.; Nord-Larsen, T.; Beck, G.; Thybring, E.E. Water sorption in wood cell walls-data exploration of the influential physicochemical characteristics. Cellulose 2023, 30, 1857–1871. [Google Scholar] [CrossRef]
- Goncalves, R.; Trinca, A.J.; Pellis, B.P. Elastic constants of wood determined by ultrasound using three geometries of specimens. Wood Sci. Technol. 2014, 48, 269–287. [Google Scholar] [CrossRef]
- Yang, R.; Dong, A.; Meng, X.; Sheng, Y.; Wang, F.; Xia, C.; Aladejana, J.T.; Fang, Z.; Zhao, R.; Zhan, X.; et al. Ultra-Thin Wood-Based Acoustic Diaphragms Fabricated via an Environmentally Friendly Strategy. ACS Appl. Mater. Interfaces 2022, 14, 47089–47099. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, Y.; Yu, H.; Yuan, J. Measurement of the dynamic modulus of elasticity of wood panels. Front. For. China 2006, 1, 425–430. [Google Scholar] [CrossRef]
- Simic, K.; Gendvilas, V.; O’Reilly, C.; Harte, A.M. Predicting structural timber grade-determining properties using acoustic and density measurements on young Sitka spruce trees and logs. Holzforschung 2019, 73, 139–149. [Google Scholar] [CrossRef]
- Silva, L.S.Z.R.S.; Panzera, T.H.; Garcia, C.T.; Silva, D.A.L.; Lahr, F.A.R.; Christoforo, A.L. Physical-mechanical properties of Cajueiro wood estimated by non-destructive methods based on apparent density and natural vibration frequency. Cienc. Florest. 2023, 33, 1–22. [Google Scholar]
- Pantelic, F.; Mijic, M.; Pavlovic, D.S.; Ridley-Ellis, D.; Dudes, D. Analysis of a wooden specimen’s mechanical properties through acoustic measurements in the very near field. J. Acoust. Soc. Am. 2020, 147, EL320–EL325. [Google Scholar] [CrossRef] [PubMed]
- De Moura Aquino, V.B.; Zanini Ribeiro Soares, L.S.; Ruthes, H.C.; Arroyo, F.N.; Fraga, I.F.; Christoforo, A.L.; Dos Santos, H.F.; Chahud, E.; Melgaco Nunes Branco, L.A.; Rocco Lahr, F.A. Analysis of Moisture Content Variation on Strength and Stiffness Properties of Cedrela Odorata Wood Species. Wood Res. 2022, 67, 231–240. [Google Scholar] [CrossRef]
- Miao, Y.; Li, R.; Qian, X.; Yin, Y.; Yang, Y.; Jin, X.; Lin, B.; Liu, Y.; Liu, Z. Effect of extraction on the acoustic vibrational properties of Picea jezoensis var. microsperma (Lindl.) WCCheng & LKFu. Ann. For. Sci. 2021, 78, 24. [Google Scholar]
- Hu, W.; Li, S.; Liu, Y. Vibrational Characteristics of Four Wood Species Commonly Used in Wood Products. Bioresources 2021, 16, 7100–7110. [Google Scholar] [CrossRef]
- Viala, R.; Placet, V.; Cogan, S. Simultaneous non-destructive identification of multiple elastic and damping properties of spruce tonewood to improve grading. J. Cult. Herit. 2020, 42, 108–116. [Google Scholar] [CrossRef]
- Yang, Y.; Liu, Z.; Liu, Y. Prediction of lute acoustic quality based on soundboard vibration performance using multiple choice model. J. For. Res. 2017, 28, 855–861. [Google Scholar] [CrossRef]
- Hilde, C.; Woodward, R.; Avramidis, S.; Hartley, I.D. The Acoustic Properties of Water Submerged Lodgepole Pine (Pinus contorta) and Spruce (Picea spp.) Wood and Their Suitability for Use as Musical Instruments. Materials 2014, 7, 5688–5699. [Google Scholar] [CrossRef]
- Sotomayor Castellanos, J.R.; Villaseñor Aguilar, J.M. Material properties and acoustic performance indexes of twenty-two Mexican woods. Measurement by ultrasound. Madera Bosques 2018, 24, e2431132. [Google Scholar]
- Munk, C.; Pfriem, A. Influence of thermal modification and subsequent linseed oil impregnation on the sound propagation velocity and related acoustic properties of various wood species. In Proceedings of the Tenth European Conference on Wood Modification, Nancy, France, 25–26 April 2022. [Google Scholar]
- Montero, M.J.; de la Mata, J.; Esteban, M.; Hermoso, E. Influence of moisture content on the wave velocity to estimate the mechanical properties of large cross-section pieces for structural use of scots pine from spain. Maderas-Cienc. Tecnol. 2015, 17, 407–420. [Google Scholar] [CrossRef]
- Llana, D.F.; Iniguez-Gonzalez, G.; Martinez, R.D.; Arriaga, F. Influence of timber moisture content on wave time-of-flight and longitudinal resonance frequency in coniferous species for different instruments. Holzforschung 2018, 72, 405–411. [Google Scholar] [CrossRef]
- Llana, D.F.; Iniguez-Gonzalez, G.; Esteban, M.; Hermoso, E.; Arriaga, F. Timber moisture content adjustment factors for nondestructive testing (NDT): Acoustic, vibration and probing techniques. Holzforschung 2020, 74, 817–827. [Google Scholar] [CrossRef]
- Nkibeu, J.B.; Makomra, V.; Sali, M.; Jeremie, M. Damping properties of some tropical wood species determined by free transverse vibration method. Wood Mater. Sci. Eng. 2024, 1–7. [Google Scholar] [CrossRef]
- Sedik, Y.; Hamdan, S.; Jusoh, I.; Hasan, M. Acoustic Properties of Selected Tropical Wood Species. J. Nondestruct. Eval. 2010, 29, 38–42. [Google Scholar] [CrossRef]
- Goeken, J.; Fayed, S.; Schaefer, H.; Enzenauer, J. A Study on the Correlation between Wood Moisture and the Damping Behaviour of the Tonewood Spruce. Acta Phys. Pol. A 2018, 133, 1241–1260. [Google Scholar] [CrossRef]
- Zhang, L.; Tiemann, A.; Zhang, T.; Gauthier, T.; Hsu, K.; Mahamid, M.; Moniruzzaman, P.K.; Ozevin, D. Nondestructive assessment of cross-laminated timber using non-contact transverse vibration and ultrasonic testing. Eur. J. Wood Wood Prod. 2021, 79, 335–347. [Google Scholar] [CrossRef]
- Kohantorabi, M.; Hemmasi, A.; Talaeipour, M.; Roohnia, M.; Bazyar, B. Effect of Artificial Inhomogeneity of Density and Drilling on Dynamic Properties Developed by Poplar Block Species (Populus nigra) Jointed with Oak Wood (Quercus castaneifolia) Beams. Bioresources 2020, 15, 4711–4726. [Google Scholar] [CrossRef]
- Brancheriau, L.; Kouchade, C.; Bremaud, I. Internal friction measurement of tropical species by various acoustic methods. J. Wood Sci. 2010, 56, 371–379. [Google Scholar] [CrossRef]





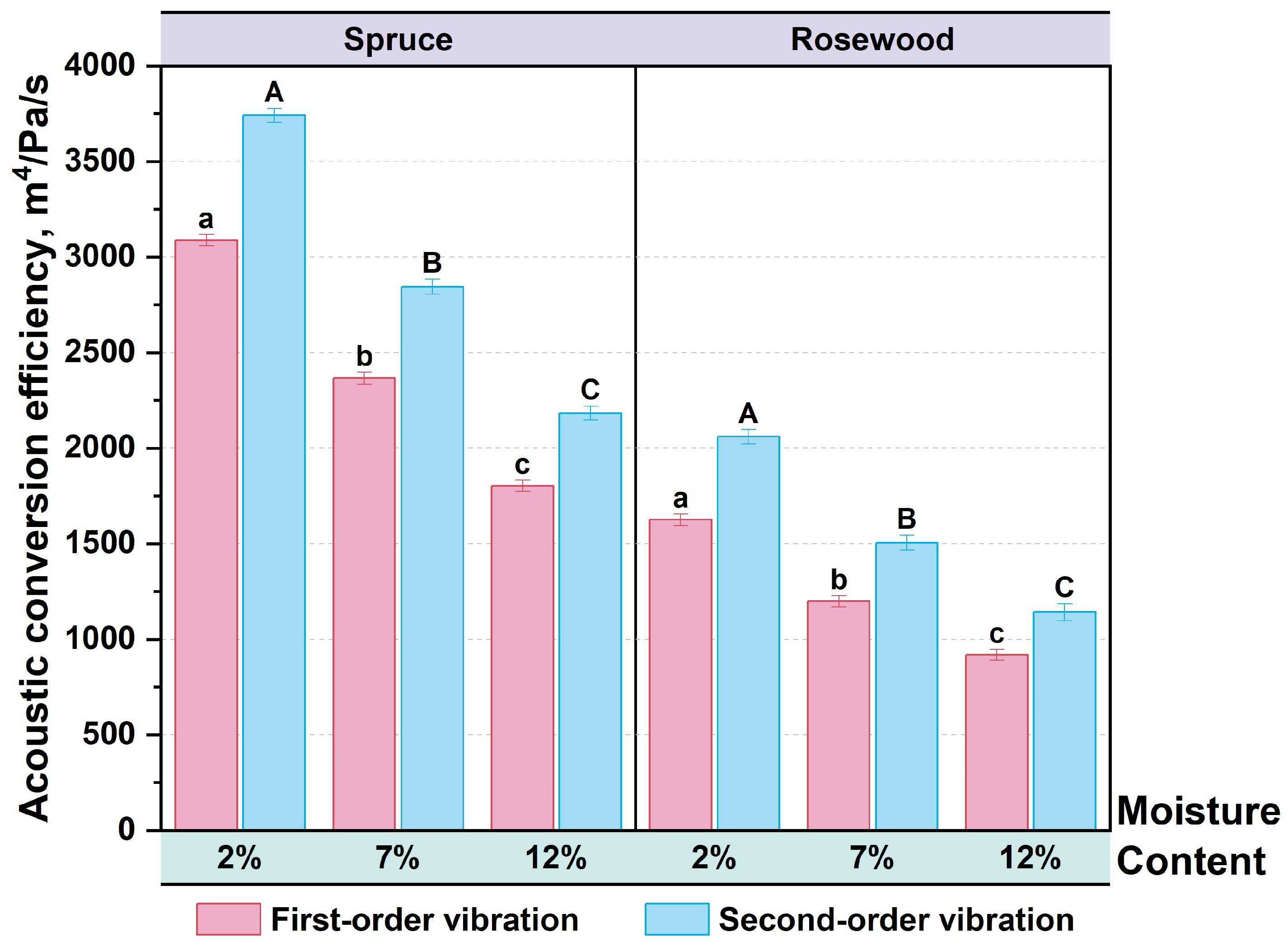
| E | E/ρ | R | Z | ACE | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Mean | SD | Mean | SD | Mean | SD | Mean | SD | Mean | SD | ||
| 2% Spruce | first-order | 5.23 | 0.10 | 14.19 | 0.27 | 10.23 | 0.09 | 1.39 | 0.01 | 3090.52 | 30.06 |
| second-order | 6.16 | 0.13 | 16.74 | 0.35 | 11.11 | 0.12 | 1.51 | 0.01 | 3744.52 | 37.42 | |
| 7% Spruce | first-order | 5.08 | 0.09 | 13.15 | 0.28 | 9.39 | 0.09 | 1.40 | 0.01 | 2638.77 | 31.56 |
| second-order | 6.16 | 0.13 | 16.74 | 0.35 | 11.11 | 0.12 | 1.51 | 0.02 | 3744.52 | 37.42 | |
| 12% Spruce | first-order | 5.00 | 0.09 | 12.36 | 0.26 | 8.70 | 0.09 | 1.42 | 0.01 | 1804.48 | 29.06 |
| second-order | 5.83 | 0.12 | 14.42 | 0.34 | 9.39 | 0.12 | 1.54 | 0.01 | 2184.99 | 36.42 | |
| 2% Rosewood | first-order | 12.69 | 0.10 | 15.28 | 0.27 | 4.71 | 0.09 | 3.25 | 0.02 | 1626.77 | 29.55 |
| second-order | 17.23 | 0.13 | 20.75 | 0.35 | 5.49 | 0.12 | 3.78 | 0.01 | 2062.12 | 37.42 | |
| 7% Rosewood | first-order | 12.16 | 0.09 | 13.96 | 0.26 | 4.29 | 0.09 | 3.25 | 0.02 | 1199.52 | 29.22 |
| second-order | 16.58 | 0.13 | 19.03 | 0.36 | 5.01 | 0.13 | 3.80 | 0.01 | 1506.26 | 39.06 | |
| 12% Rosewood | first-order | 11.88 | 0.10 | 13.04 | 0.27 | 3.96 | 0.09 | 3.29 | 0.02 | 919.75 | 28.34 |
| second-order | 16.20 | 0.15 | 17.77 | 0.41 | 4.62 | 0.15 | 3.84 | 0.02 | 1142.47 | 441.90 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiu, H.; Cui, Y.; Zhang, L.; Ding, T.; Zhu, N. The Influence of Different Moisture Contents on the Acoustic Vibration Characteristics of Wood. Forests 2025, 16, 680. https://doi.org/10.3390/f16040680
Qiu H, Cui Y, Zhang L, Ding T, Zhu N. The Influence of Different Moisture Contents on the Acoustic Vibration Characteristics of Wood. Forests. 2025; 16(4):680. https://doi.org/10.3390/f16040680
Chicago/Turabian StyleQiu, Hongru, Yunqi Cui, Liangping Zhang, Tao Ding, and Nanfeng Zhu. 2025. "The Influence of Different Moisture Contents on the Acoustic Vibration Characteristics of Wood" Forests 16, no. 4: 680. https://doi.org/10.3390/f16040680
APA StyleQiu, H., Cui, Y., Zhang, L., Ding, T., & Zhu, N. (2025). The Influence of Different Moisture Contents on the Acoustic Vibration Characteristics of Wood. Forests, 16(4), 680. https://doi.org/10.3390/f16040680





