Research on the Equivalent Span of Hybrid Girder Bridges
Abstract
1. Introduction
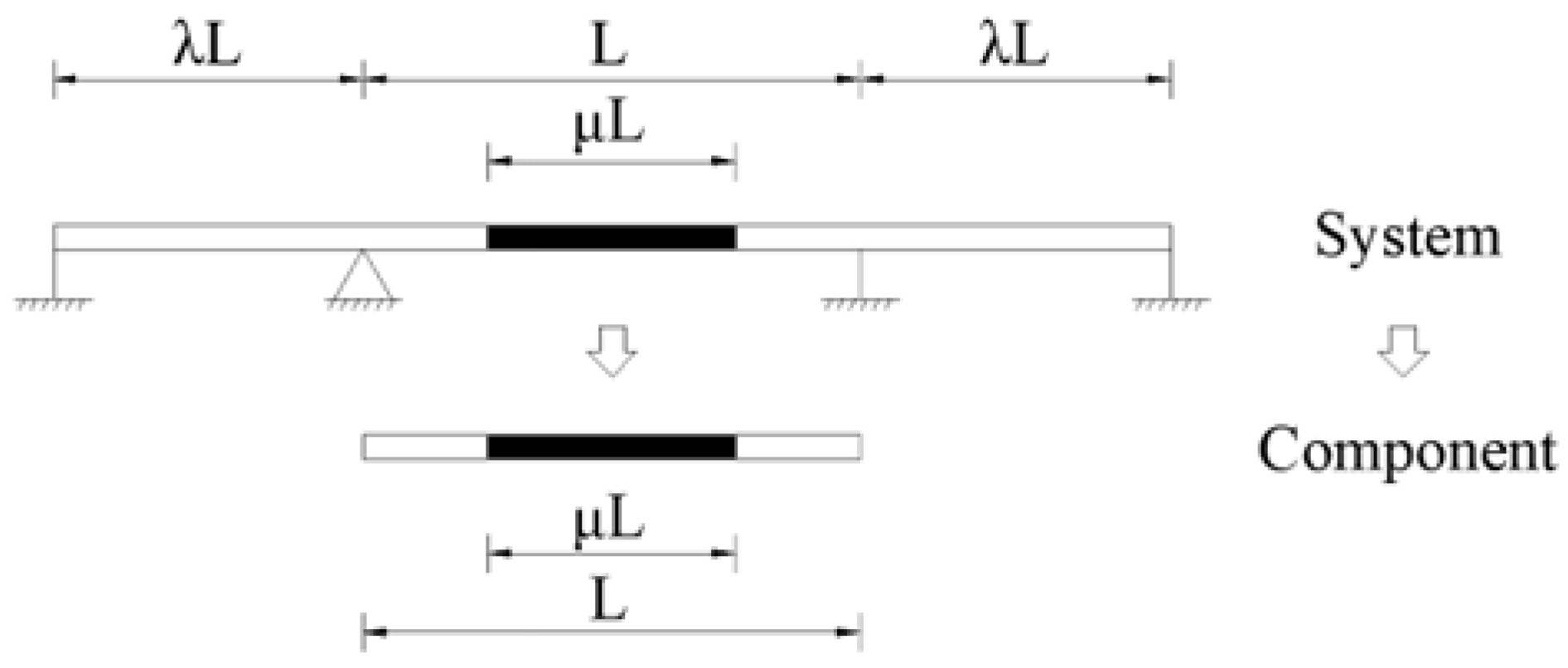
2. Research Assumptions
- (1)
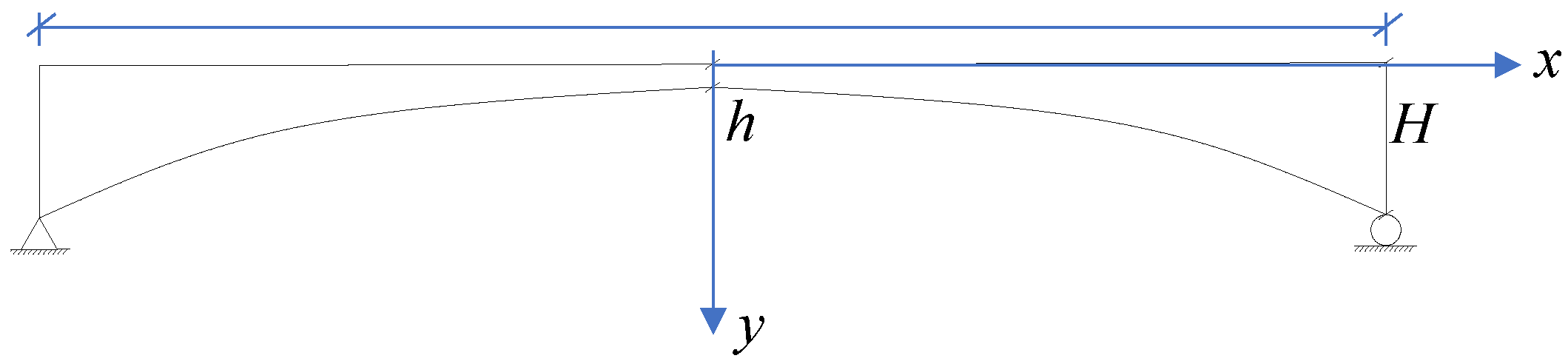
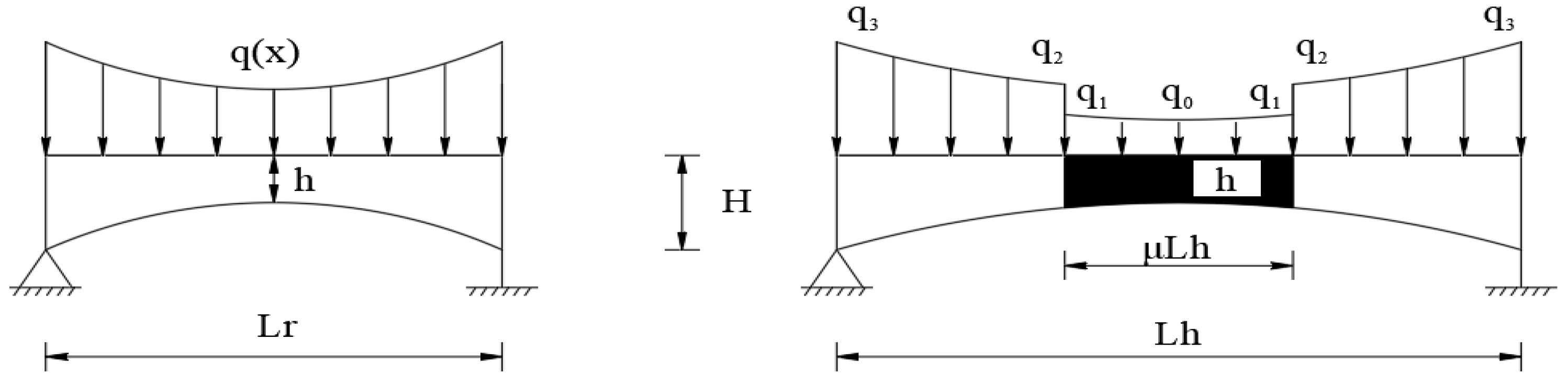
- Load. The load in the bending model of hybrid girder components is represented as a line load perpendicular to the hybrid girder. The intensity of the line load is related to factors such as the material’s unit weight and quantity.
- (2)
- Cross-sectional properties. The bending model of hybrid girder components is classified into two types based on cross-sectional properties: uniform cross-section beams and variable cross-section beams. For variable cross-section beams, the beam height varies according to a power function with an exponent α.
- (3)
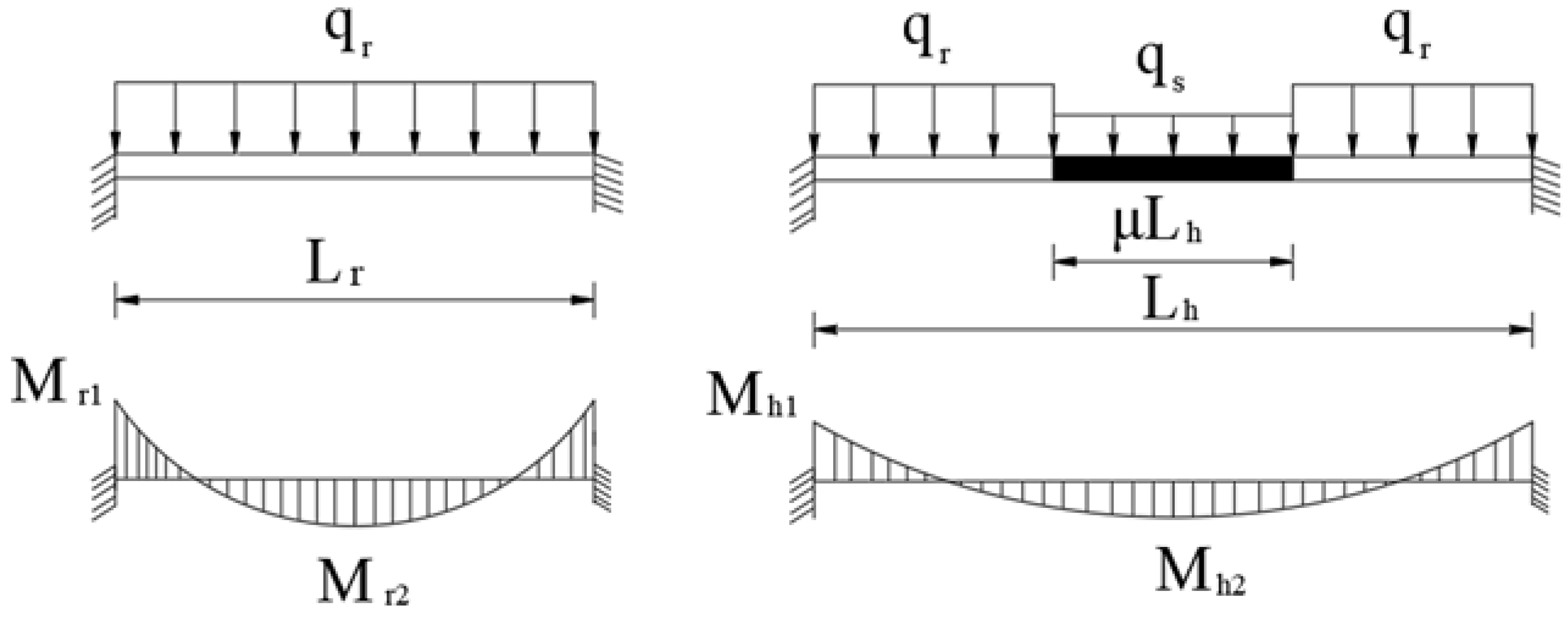
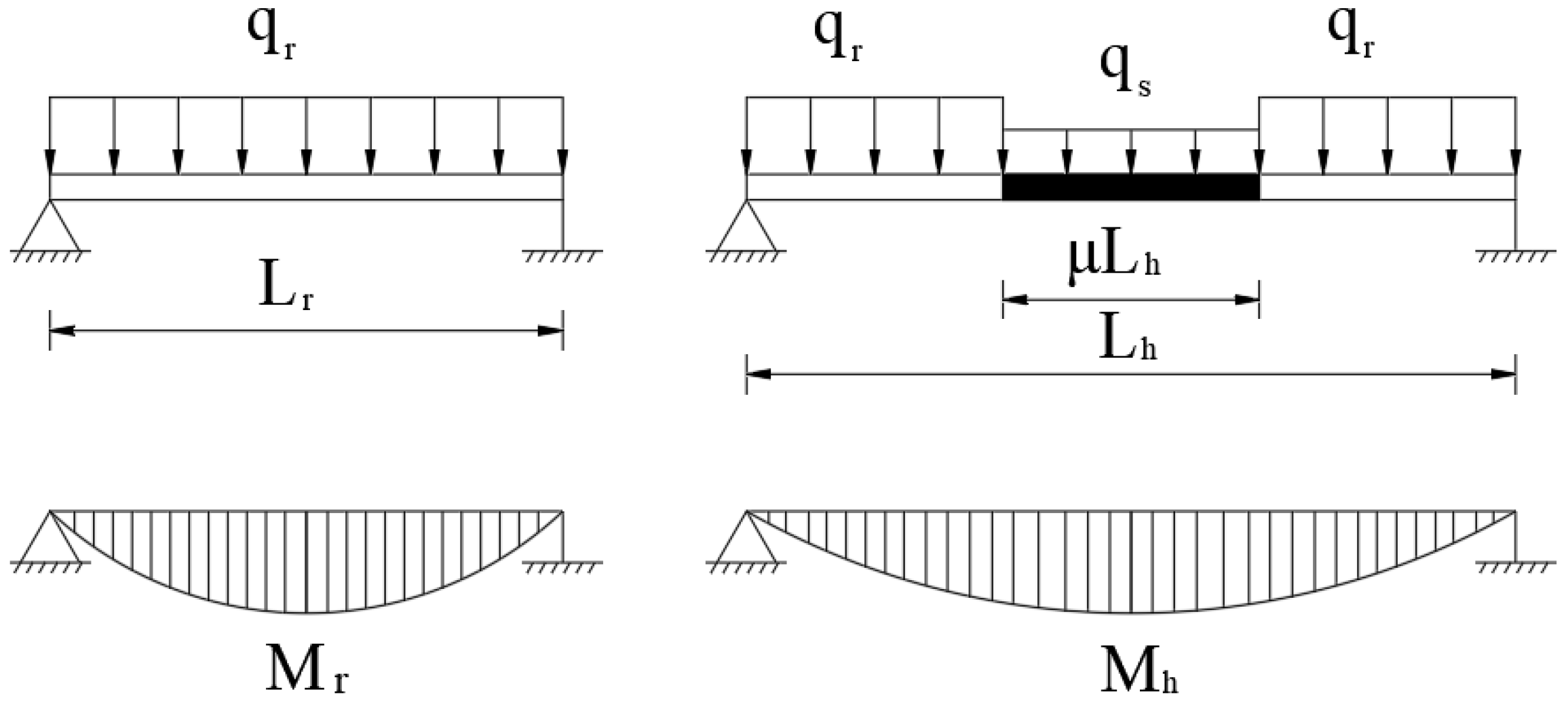
- Boundary Conditions. The boundary conditions of the bending model for hybrid girder components are classified into two types: fixed at both ends and hinged at both ends. Fixed at both ends (referred to as the fixed-end beam model): This model has complete boundary constraints, capable of providing vertical reactions, horizontal reactions, and fixed-end moments. Additionally, if initial moments are applied at the fixed ends, the model can fully simulate the bending behavior of hybrid girder components. However, the fixed-end beam model involves numerous parameters, making it challenging to derive explicit analytical solutions during theoretical derivations. Hinged at both ends (referred to as the simply supported beam model): This model provides boundary constraints that offer only vertical and horizontal reactions but no fixed-end moments. Despite this limitation, the maximum bending moment, Mmax, in a simply supported beam model is equivalent to the difference, ΔM, between the maximum and minimum bending moments in a fixed-end beam model. Since ΔM and Mmax have analogous significance in evaluating the spanning capacity of girder bridges, the simply supported beam model holds value as an alternative. Its primary advantage lies in having fewer parameters, which simplifies theoretical analysis and allows for easier derivation of explicit analytical solutions.
- (4)
- Equivalent internal force terms. When the maximum bending moment of the rootstock beam component is set equal to the maximum bending moment of the hybrid girder component as the equivalence principle, the resulting equivalent span increase coefficient is referred to as the equivalent bending moment span increase coefficient . When the maximum shear force of the rootstock beam component is set equal to the maximum shear force of the hybrid girder component as the equivalence principle, the resulting equivalent span increase coefficient is referred to as the equivalent shear force span increase coefficient .
3. Study on the Equivalent Span of Uniform Cross-Section Hybrid Girders
3.1. Based on the Fixed-End Beam Model
3.2. Based on Simply Supported Beam Model
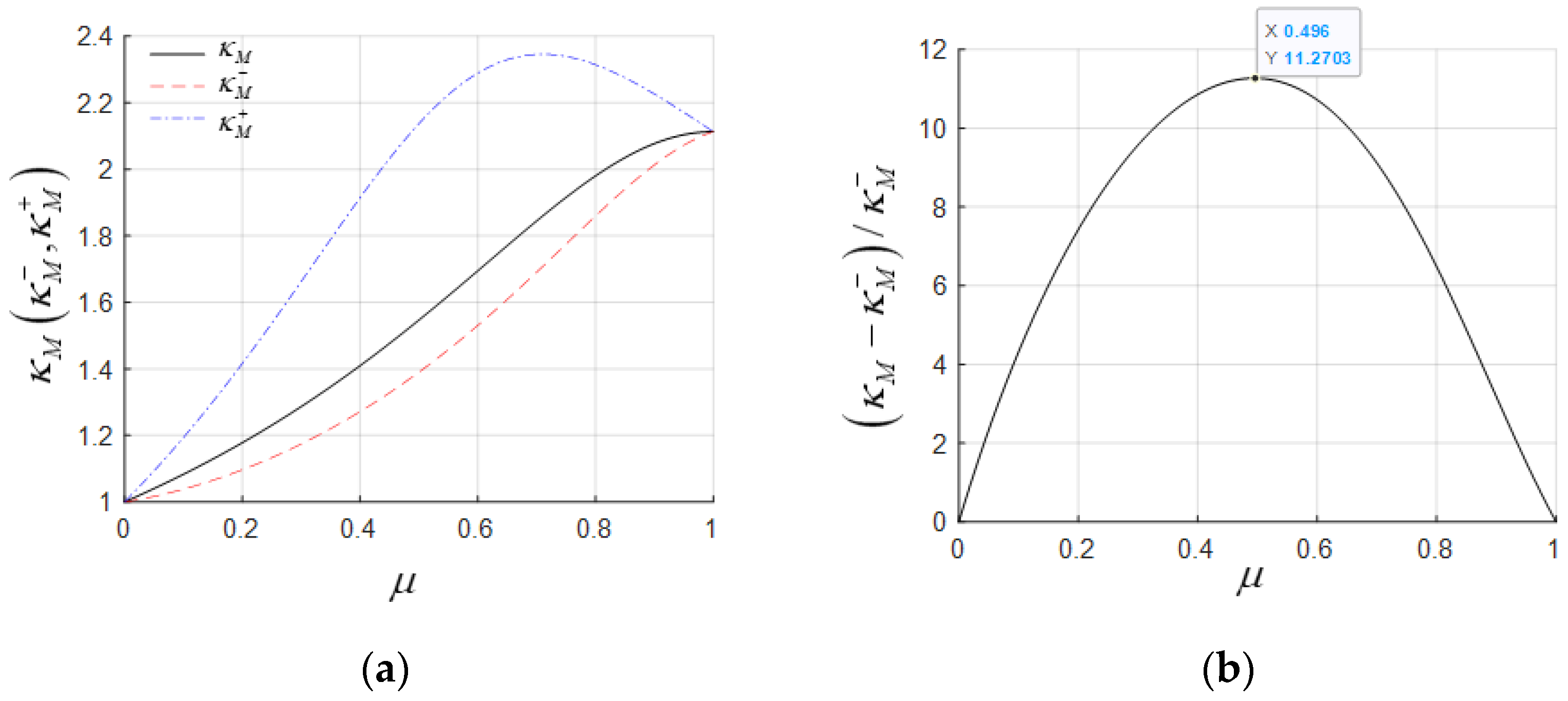
3.3. Comparison of
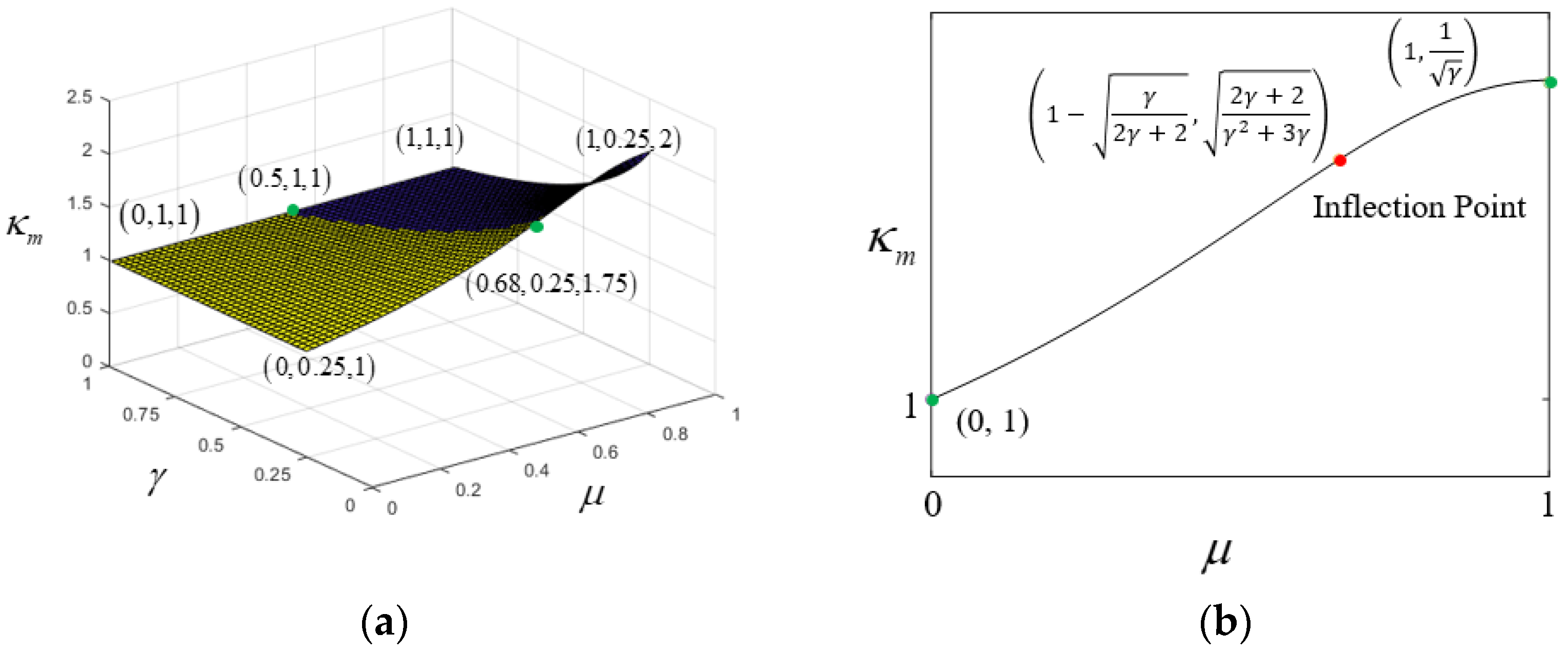
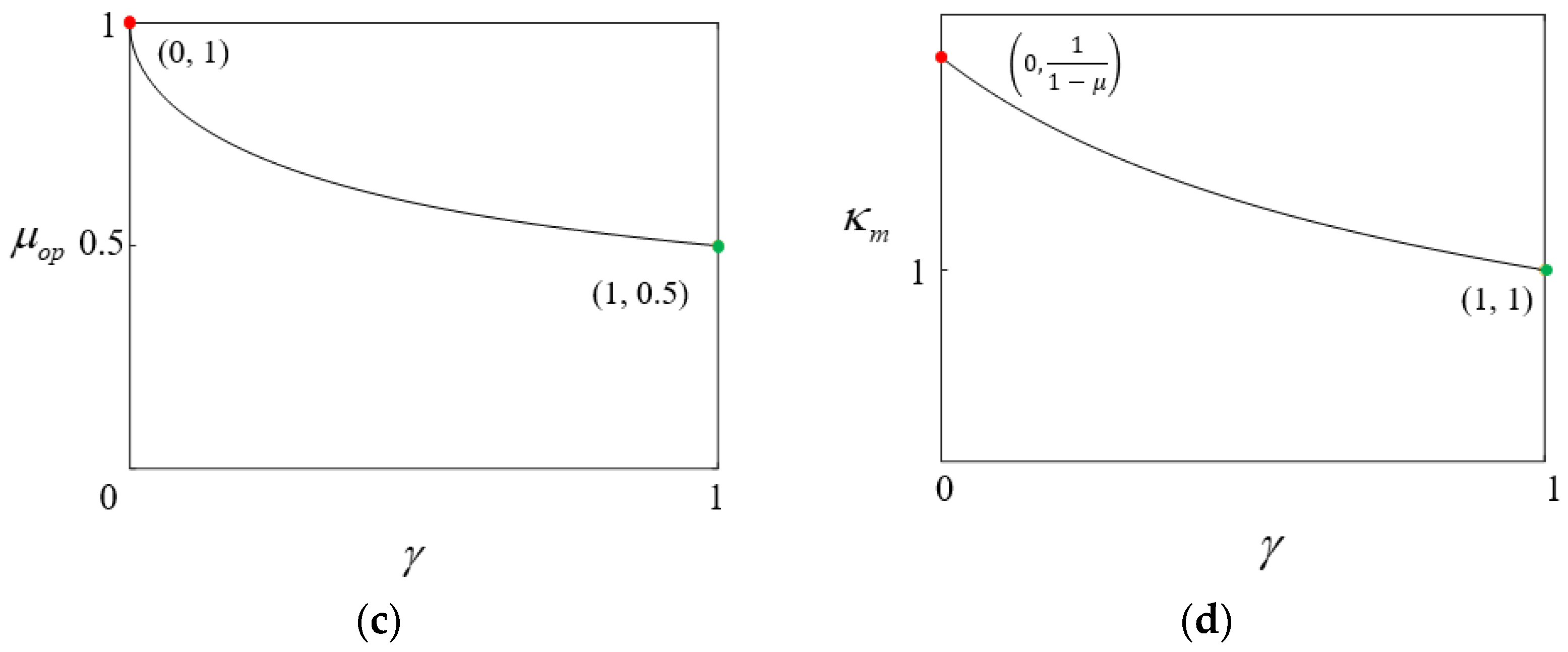
3.4. Characteristics of
4. Study on Equivalent Span Increase Coefficient of Variable Cross-Section Hybrid Girders
5. Engineering Case Comparison of Between Uniform and Variable Cross-Sections
6. Applications
6.1. Span Arrangement Design
6.2. Limit Span Prediction
7. Conclusions
- 1.
- Research on the equivalent span of hybrid girder bridges in this paper is based on hybrid girder components and does not involve factors such as the span-to-span ratio and boundary conditions in system analysis, making it simpler than system analysis. , as the core indicator in the equivalent span research, not only represents the mechanical advantage of the hybrid girder components but also serves as the key to the design of the hybrid structure beam bridge system.
- 2.
- Through the study of the bending characteristics of uniform hybrid girder components, it was found that the simply supported beam serves as a reliable substitute in the calculation of . The Equation (8) based on the simply supported beam model does not rely on the stiffness ratio but is only related to and , providing good calculation accuracy. The analysis of the three-dimensional surface and mathematical characteristics of derived from Equation (8) enhances engineers’ understanding of hybrid girders.
- 3.
- Research on the flexural characteristics of variable cross-section hybrid girders using the simply supported beam model, results in Equation (16). A comparison of five hybrid structure beam bridges with Equation (8) demonstrates that Equation (8) provides sufficient accuracy for conceptual design when empirical values are used. This approach greatly simplifies calculations, highlighting both the simplicity and precision of the equivalent span method.
- 4.
- The equivalent span method, verified by fourteen hybrid structure beam bridges, effectively resolves span layout design during the conceptual phase of hybrid structure beam bridges. It determines side-span lengths under fixed hybrid ratios or identifies hybrid ratios for fixed span layouts.
- 5.
- By applying Equation (8) in conceptual design, Equation (18) was derived to predict the limit span of hybrid structure beam bridges. This approach is conceptually clear and yields reliable results.
- 6.
- The Leq method proposed in this paper also has certain limitations. For example, the hybrid girder component model presented here is based on the mid-span hybrid girder, making it inapplicable to other hybrid girder configurations. Additionally, due to the exclusion of factors such as live load, the calculation accuracy is limited, rendering it suitable only for the conceptual and preliminary design stages of long-span hybrid girder bridges. Addressing these limitations will require further research in the future.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Notation
| a | Power function order of the height curve for variable cross-section beams; |
| b | Bending stiffness ratio of the scion girder and the rootstock girder, i.e., (EsIs)/(ErIr); |
| b1 | Linear load coefficient related to beam height; |
| b1r | Linear load coefficient of the rootstock girder related to beam height; |
| b1s | Linear load coefficient of the scion girder related to beam height; |
| b2 | Linear load coefficient independent of beam height; |
| b2r | Linear load coefficient of the rootstock girder independent of beam height; |
| bsr | Linear load coefficient of the scion girder independent of beam height; |
| g | Linear load concentration ratio of the scion girder and the rootstock girder, i.e., qs/qr; |
| Span increase coefficient; | |
| Equivalent negative bending moment span increase coefficient based on the uniform cross-section fixed-ended beam model; | |
| Equivalent positive bending moment span increase coefficient based on the uniform cross-section fixed-ended beam model; | |
| Equivalent bending moment span increase coefficient; | |
| Equivalent shear force span increase coefficient; | |
| Hybrid ratio, i.e., scion girder component ratio; | |
| Leq | Equivalent internal force span; |
| Lh | Hybrid girder span; |
| Lr | Rootstock girder span; |
| Lrmax | Maximum span record of rootstock girder; |
| Lsmax | Maximum span record of scion girder; |
Appendix A
Appendix B
References
- Ingebrigtsen, T. Stolma Bridge, Norway. Struct. Eng. Int. 1999, 9, 100–102. [Google Scholar] [CrossRef]
- Deng, W.Z.; Dai, T. Overall Design of the Auxiliary Bridge of Chongqing Shibanpo Yangtze River Bridge. Bridge Constr. 2006, 6, 28–32. (In Chinese) [Google Scholar]
- Fuentes, I.; Stegemann, S.; Golczyk, H.; Karcher, D.; Bock, R. Horizontal genome transfer as an asexual path to the formation of new species. Nature 2014, 511, 232–235. [Google Scholar] [CrossRef] [PubMed]
- Melnyk, C.W.; Meyerowitz, E.M. Plant grafting. Curr. Biol. 2015, 25, 183–188. [Google Scholar] [CrossRef] [PubMed]
- Kim, G.; LeBlanc, M.L.; Wafula, E.K.; Depamphilis, C.W.; Westwood, J.H. Genomic-scale exchange of mRNA between a parasitic plant and its hosts. Science 2014, 345, 808–811. [Google Scholar] [CrossRef] [PubMed]
- Mudge, K.; Janick, J.; Scofield, S.; Goldschmidt, E.E. A History of Grafting. Hortic. Rev. 2009, 35, 437–493. [Google Scholar]
- Xu, C.; Zhang, L.P.; Su, Q.T.; Abbas, S. Mechanical behavior of a novel steel-concrete joint in concrete-composited hybrid continuous bridges. Structures 2022, 36, 291–302. [Google Scholar] [CrossRef]
- Shangguan, B.; Su, Q.T.; Casas Joan, R.; Su, H.; Wang, S.Y.; Zhao, R.X. Modeling and testing of a composite steel–concrete joint for hybrid girder bridges. Materials 2023, 16, 3265. [Google Scholar] [CrossRef] [PubMed]
- Pei, H.T.; Jia, L.J.; Li, J.W.; Li, K.W.; Xia, R.J. A Study of the Mechanical Behavior of a Steel-Concrete Hybrid Beam Bridge during Construction. Buildings 2023, 13, 1781. [Google Scholar] [CrossRef]
- Chen, D.B.; Lin, Z.P.; Su, Q.T.; Ouyang, M.G.; Shangguan, B. Structural Optimization and Experiment of Steel-concrete Composite Segment in Hybrid Girder Rigid Frame Bridges. Eng. Mech. 2023, 40, 149–160. [Google Scholar]
- Wang, H.B.; Zeng, H.Z.; Wu, X.Y. Fangcong. Simplified Calculation Model and Experimental Validation of the Force Transfer Ratio of Steel–Concrete Joint of Hybrid Box Girder. Materials 2023, 16, 5091. [Google Scholar] [CrossRef] [PubMed]
- Su, Q.; Qin, F. A new type hybrid-beam bridge structural system and its design parameters. J. Tongji Univ. 2013, 41, 799–805. (In Chinese) [Google Scholar]
- Zuo, Z. Research on Key Parameters and Beam Height Optimization of Steel-Concrete Hybrid Continuous Rigid Frame Bridges. Master’s Thesis, Southwest Jiaotong University, Chengdu, China, 2014. (In Chinese). [Google Scholar]
- Guan, C. Research on Structural Performance and Design of Steel-Concrete Longitudinal Composite Continuous Beam Bridges; Southeast University: Nanjing, China, 2020. (In Chinese) [Google Scholar]
- Zeng, M.; Xu, G.; Lin, Z.; Chen, D.; Su, Q. Mechanical calculation and rational structural system of hybrid beam rigid frame bridge. J. Tongji Univ. 2020, 48, 1687–1695. (In Chinese) [Google Scholar]
- Chen, K.; Yang, Y.; Wu, Q.; Luo, J. Research on the Reasonable Length of Steel Box Girder Sections in Steel-Concrete Hybrid Variable Cross-Section Continuous Beam Bridges. J. Fuzhou Univ. 2022, 50, 237–243. (In Chinese) [Google Scholar]
- He, Z.; Chen, J.; Liu, Z.; Ma, Z.J. An Explicit Approach for Determining the Rational Length of Steel Portion in Steel-Concrete Hybrid Girder Bridges. J. Bridge Eng. 2023, 28, 05022011. [Google Scholar] [CrossRef]
- Mao, M.W.; He, Z.Q.; Liu, G. An analytical method for determining the rational position of joint section in steel-concrete hybrid girder bridges. Structrues 2024, 69, 107431. [Google Scholar] [CrossRef]
- Xiao, R. Bridge Structural Systems; Thomson Press: New Delhi, India, 2016. [Google Scholar]
- Tang, M.-C. The New Shibanpo Bridge, Chongqing, China. Struct. Eng. Int. 2010, 20, 157–160. [Google Scholar] [CrossRef]
- Carrera, E.; Giunta, G.; Petrolo, M. Beam Structures: Classical and Advanced Theories; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Öchsner, A. Euler–Bernoulli Beam Theory; Springer International Publishing: Cham, Switzerland, 2021; pp. 7–66. [Google Scholar]
- Tang, F.; Luo, S.; Yang, H. Overall Design of the Oujiang Bridge. Highway 2012, 5, 127–131. (In Chinese) [Google Scholar]
- Xu, G.; Zeng, M.; Su, Q. Layout and Optimization of the External Prestressing Tendons of Hybrid Beam Rigid Frame Bridges. IOP Conf. Ser. Earth Environ. Sci. 2021, 719, 032061. [Google Scholar] [CrossRef]
- Xu, J. Design Modification of Construction Drawings for the Anhai Bay Grand Bridge on the Haixi Expressway Quanzhou Section; Fujian Transportation Planning and Design Institute: Fuzhou, China, 2018. (In Chinese) [Google Scholar]
- Xu, J. Construction Drawing Design for the Taoer River Grand Bridge on the G228 Dandong Line Binzhou Section; CCCC Highway Planning and Design Institute Co., Ltd.: Beijing, China, 2020. (In Chinese) [Google Scholar]


| Indicators | Stolma Bridge | Shibanpo Bridge | Oujiang Bridge | Anhaiwan Bridge | Taoerhe Bridge |
|---|---|---|---|---|---|
| Mid-span length (m) | 301 | 330 | 200 | 300 | 338 |
| End-span length (m) | 94 | 138 | 84 | 135 | 128 |
| Support beam height (m) | 15 | 16 | 9 | 15 | 17 |
| Mid-span beam height (m) | 3.5 | 4.5 | 3.5 | 4.5 | 5.2 |
| Beam height variation curve a | 2 | 1.6 | 1.6 | 2 | 2 |
| Contact face beam height (m) | 7.64 | 6.28 | 4.77 | 5.74 | 8.29 |
| Actual bridge weight ratio g | 0.630 | 0.340 | 0.365 | 0.326 | 0.278 |
| Estimated weight ratio g | 0.6 | 0.3 | 0.3 | 0.3 | 0.3 |
| Hybrid ratio m | 0.600 | 0.312 | 0.400 | 0.343 | 0.512 |
| 1.166 | 1.185 | 1.256 | 1.221 | 1.388 | |
| 1.204 | 1.238 | 1.298 | 1.273 | 1.491 | |
| 1.227 | 1.259 | 1.346 | 1.289 | 1.464 | |
| 3.2% | 4.5% | 3.3% | 4.3% | 7.4% | |
| 5.2% | 6.2% | 7.1% | 5.6% | 5.4% |
| Bridges | L (m) | g | m | Leq (m) | Reasonable Span for End Spans (m) | Actual End Span (m) | Conformance | |
|---|---|---|---|---|---|---|---|---|
| Stolma Bridge | 301 | 0.6 | 0.600 | 1.227 | 245 | 123~167 | 94 | × |
| Shibanpo Bridge | 330 | 0.3 | 0.312 | 1.259 | 262 | 131~178 | 138 | √ |
| Oujiang Bridge | 200 | 0.3 | 0.400 | 1.346 | 149 | 74~101 | 84 | √ |
| Anhaiwan Bridge | 300 | 0.3 | 0.343 | 1.289 | 233 | 116~158 | 135 | √ |
| Ta’erhe Bridge | 338 | 0.3 | 0.512 | 1.464 | 231 | 115~157 | 128 | √ |
| Cheviré Bridge | 242 | 0.3 | 0.670 | 1.630 | 148 | 74~101 | 69.3 | × |
| Zhoushan Bridge | 260 | 0.3 | 0.327 | 1.273 | 204 | 102~139 | 125 | √ |
| Maogang Bridge | 135 | 0.3 | 0.407 | 1.354 | 100 | 50~68 | 65 | √ |
| Zhongshan Xiaolan Bridge | 220 | 0.3 | 0.395 | 1.341 | 164 | 82~112 | 98 | √ |
| Longxiang Bridge | 202 | 0.3 | 0.396 | 1.342 | 151 | 75~102 | 93 | √ |
| Hangzhou-Wenzhou Railway Bridge | 216 | 0.3 | 0.380 | 1.326 | 163 | 81~111 | 100 | √ |
| Guangzhan Railway Bridge | 200 | 0.5 | 0.375 | 1.199 | 167 | 83~113 | 99 | √ |
| Raftsundet Bridge | 298 | 0.6 | 0.752 | 1.265 | 236 | 118~160 | 125 | √ |
| Niitsu River Bridge, Japan | 144 | 0.7 | 1.000 | 1.195 | 120 | 60~82 | 80 | √ |
| Hybrid Girder Bridge (Rootstock Beam Scion Beam) | Lrmax | Lsmax | Lhmax | Remarks | |
|---|---|---|---|---|---|
| Concrete Beam—Corrugated Steel Web Beam | 0.70 | 270 | 180 | 311 | |
| Concrete Beam—Steel Box Beam | 0.30 | 270 | 300 | 442 | |
| Concrete Beam—Steel Truss Beam | 0.25 | 270 | 549 | 540 | , Results are meaningless. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shangguan, B.; Wang, F.; Su, Q.; Matanmi, F.O.; Xu, J. Research on the Equivalent Span of Hybrid Girder Bridges. Materials 2025, 18, 1278. https://doi.org/10.3390/ma18061278
Shangguan B, Wang F, Su Q, Matanmi FO, Xu J. Research on the Equivalent Span of Hybrid Girder Bridges. Materials. 2025; 18(6):1278. https://doi.org/10.3390/ma18061278
Chicago/Turabian StyleShangguan, Bing, Feng Wang, Qingtian Su, Fawas O. Matanmi, and Jun Xu. 2025. "Research on the Equivalent Span of Hybrid Girder Bridges" Materials 18, no. 6: 1278. https://doi.org/10.3390/ma18061278
APA StyleShangguan, B., Wang, F., Su, Q., Matanmi, F. O., & Xu, J. (2025). Research on the Equivalent Span of Hybrid Girder Bridges. Materials, 18(6), 1278. https://doi.org/10.3390/ma18061278







