Investigations on the Deflection of Carbon-Reinforced Concrete Hollow-Core Slabs
Abstract
1. Introduction

2. Materials and Methods
2.1. Materials
2.1.1. Carbon Reinforcement
2.1.2. Concrete
2.2. Material Properties of Carbon Reinforcement
2.2.1. Small Scale Specimens and Test Setup
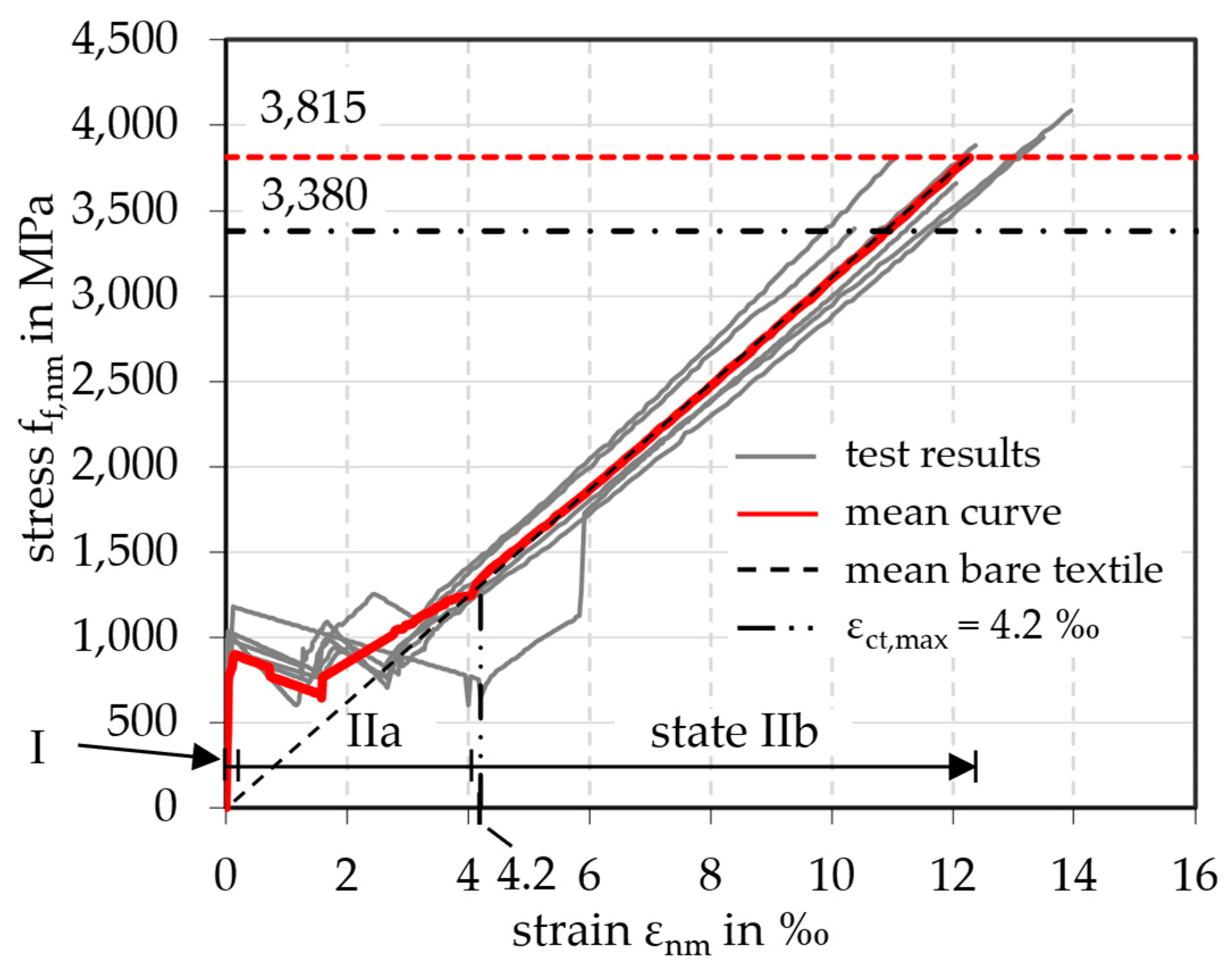
2.2.2. Tensile Test Results
3. Slab Elements and Test Concept
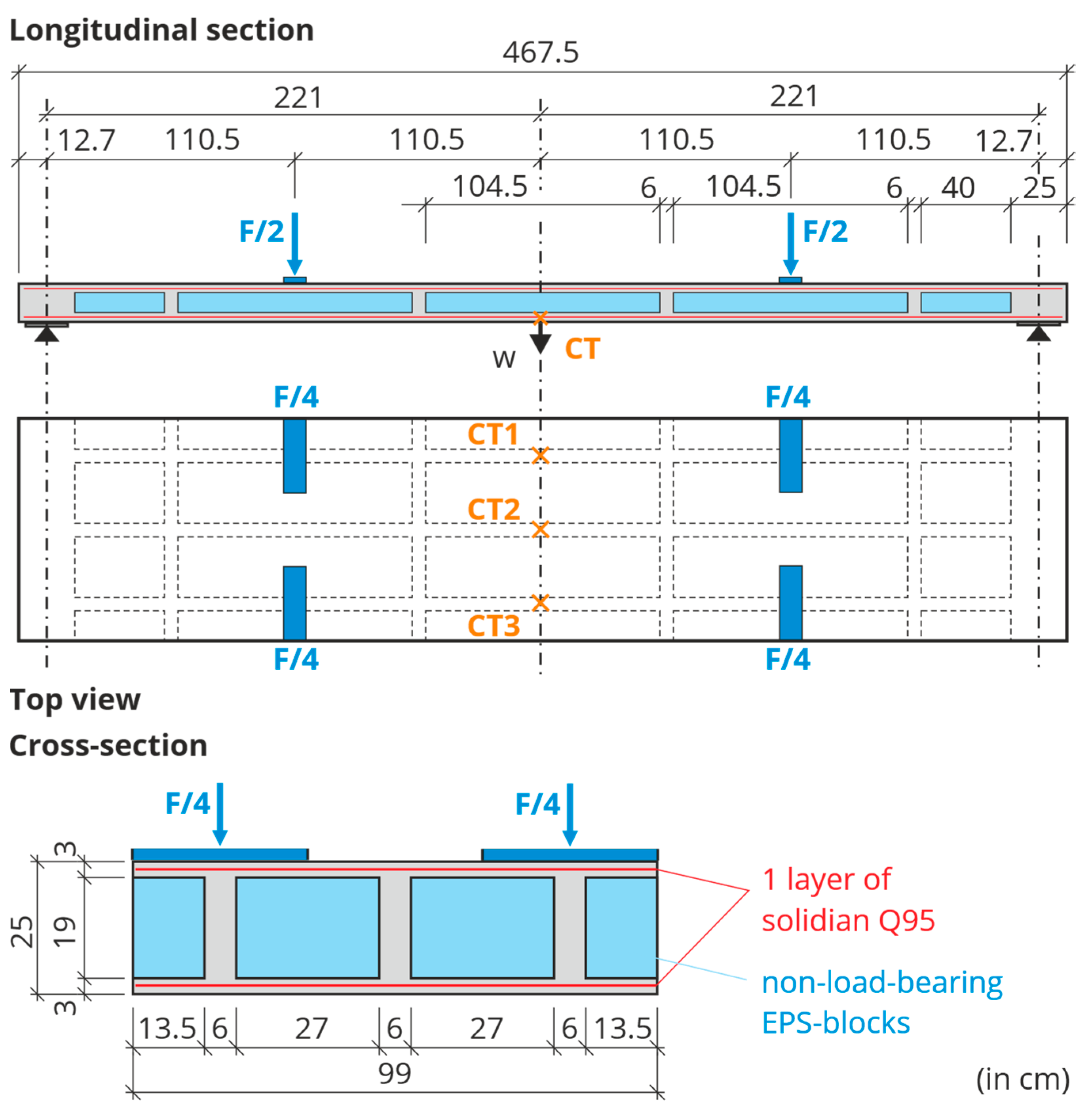
3.1. Slab System in the BOX
3.2. Production of the Large-Scale Test Specimens
3.3. Test Concept
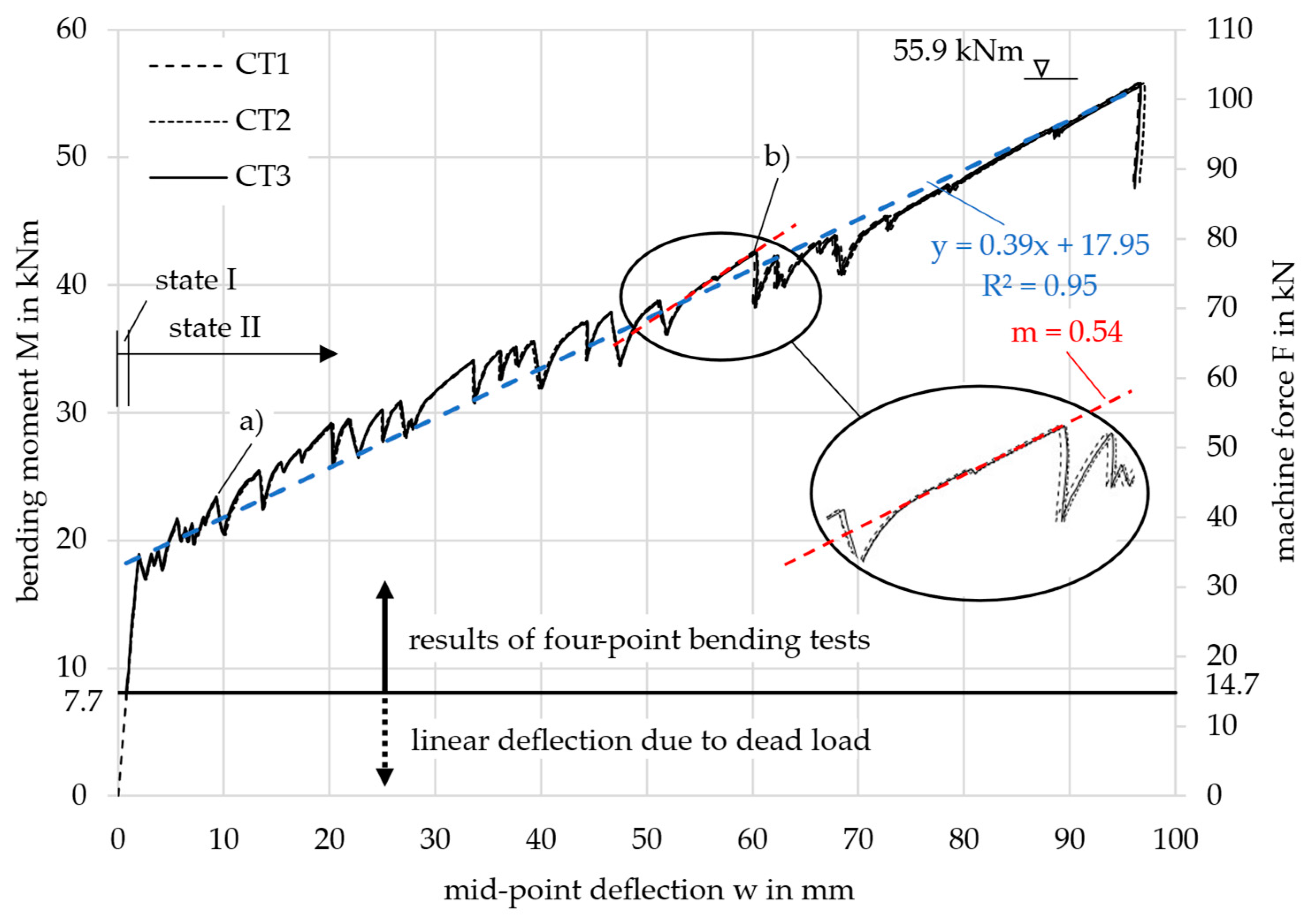
3.4. Slab Test Results
| Dead load | = 3.1 kN/m | |
| Service load | qk | = 2.5 kN/m |
| Load combination | pSLS = gk + qk = 3.1 + 2.5 | = 5.6 kN/m |
| Bending moment | = 13.7 kNm |
4. Investigations on the Deflection
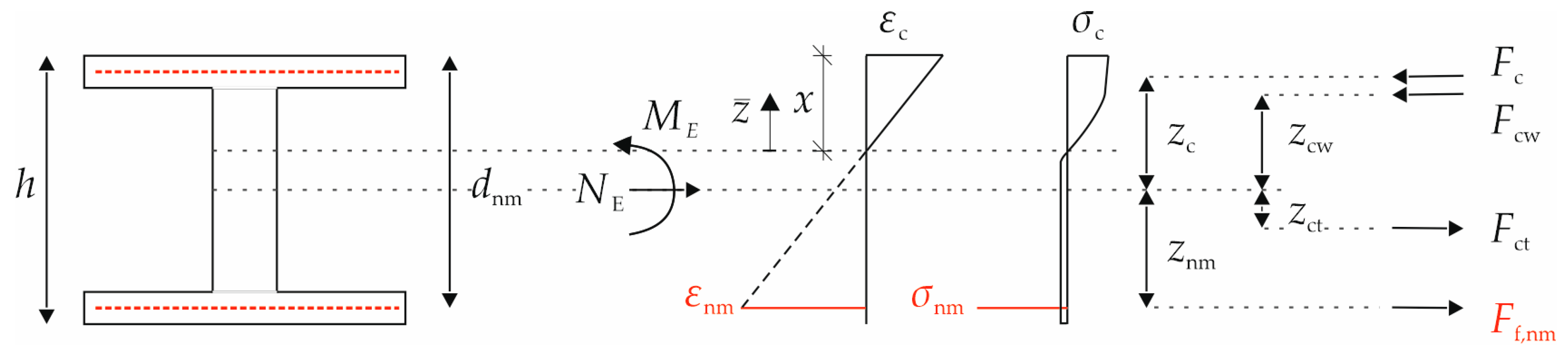
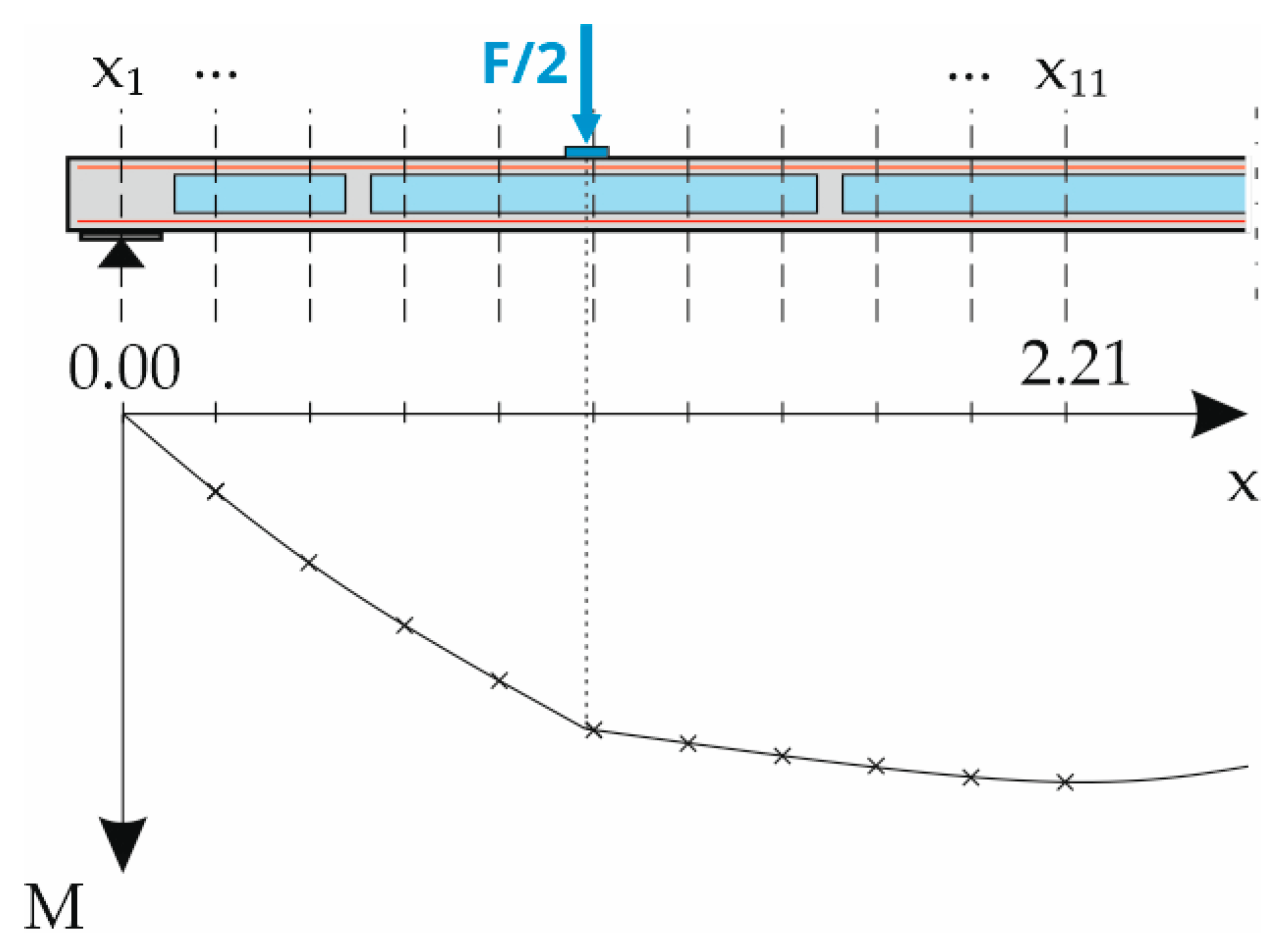
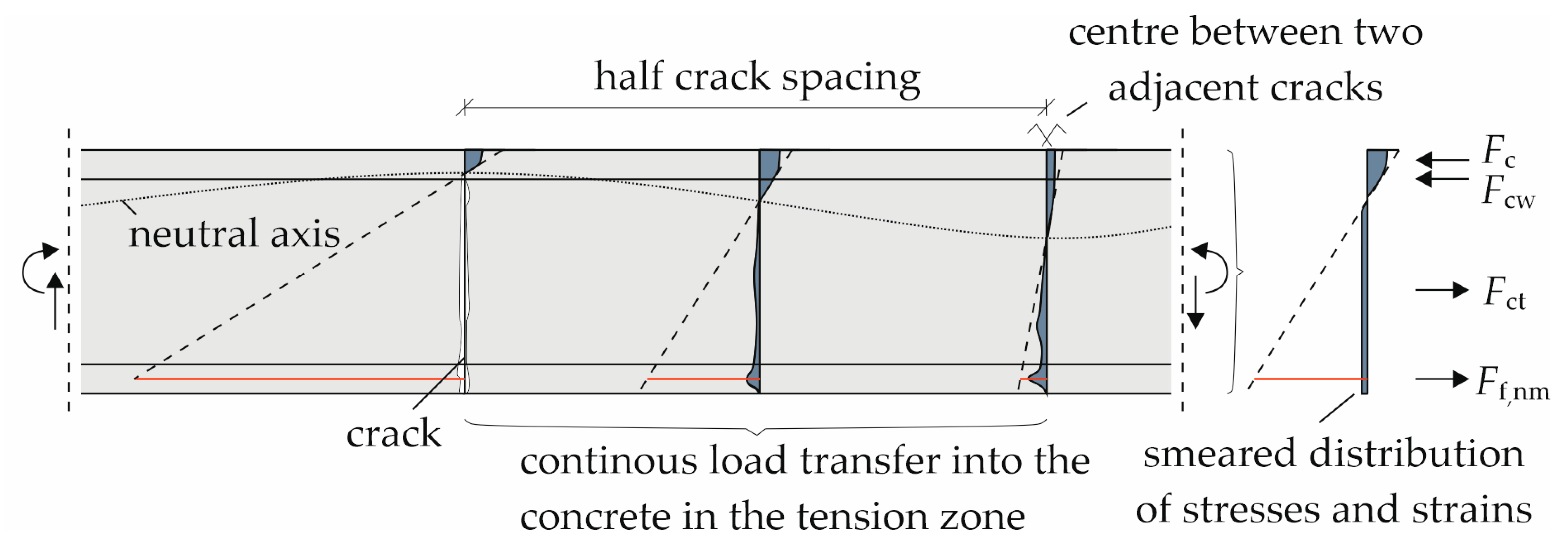
4.1. Calculation Methodology
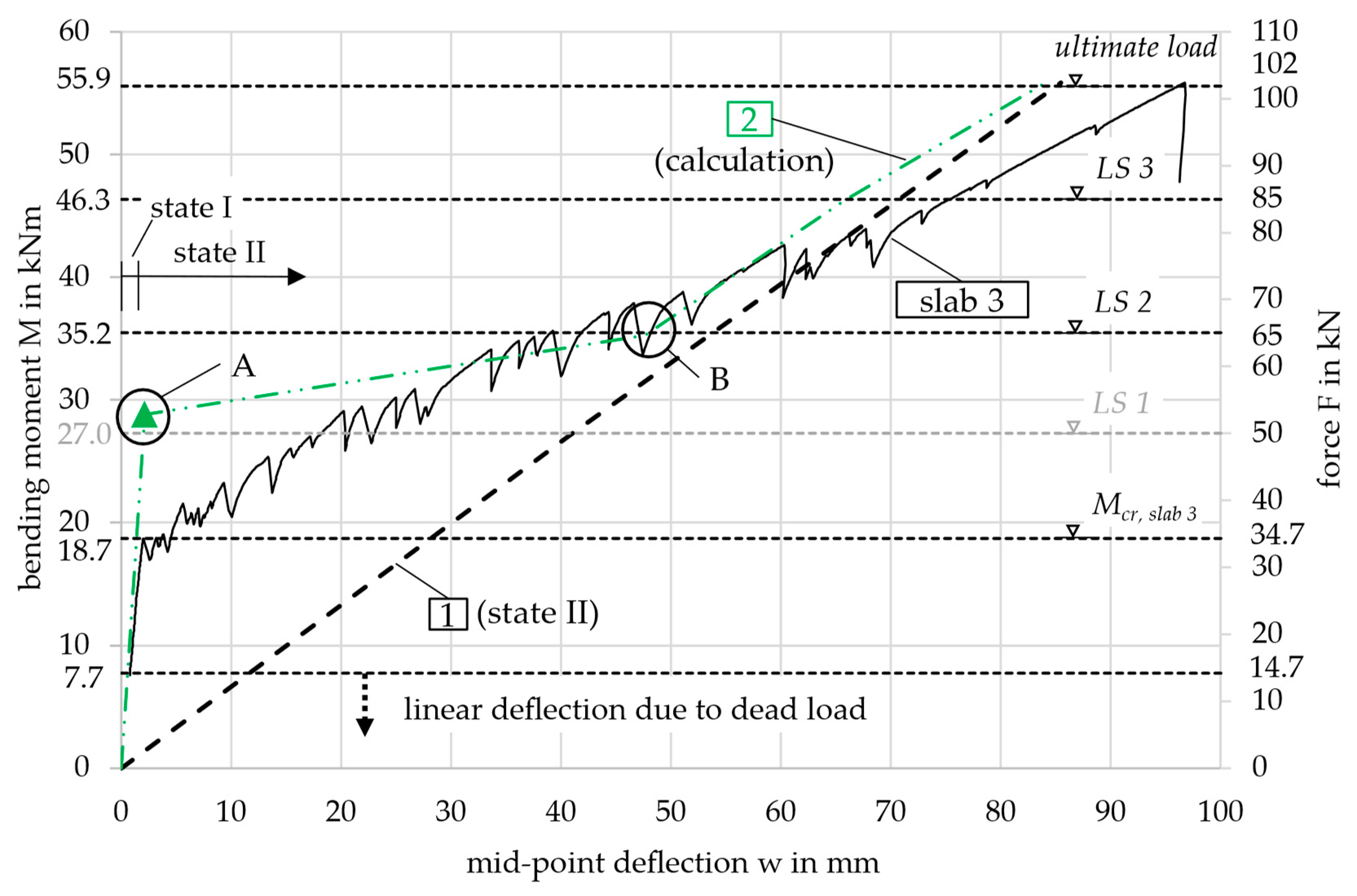
4.2. Calculation Results
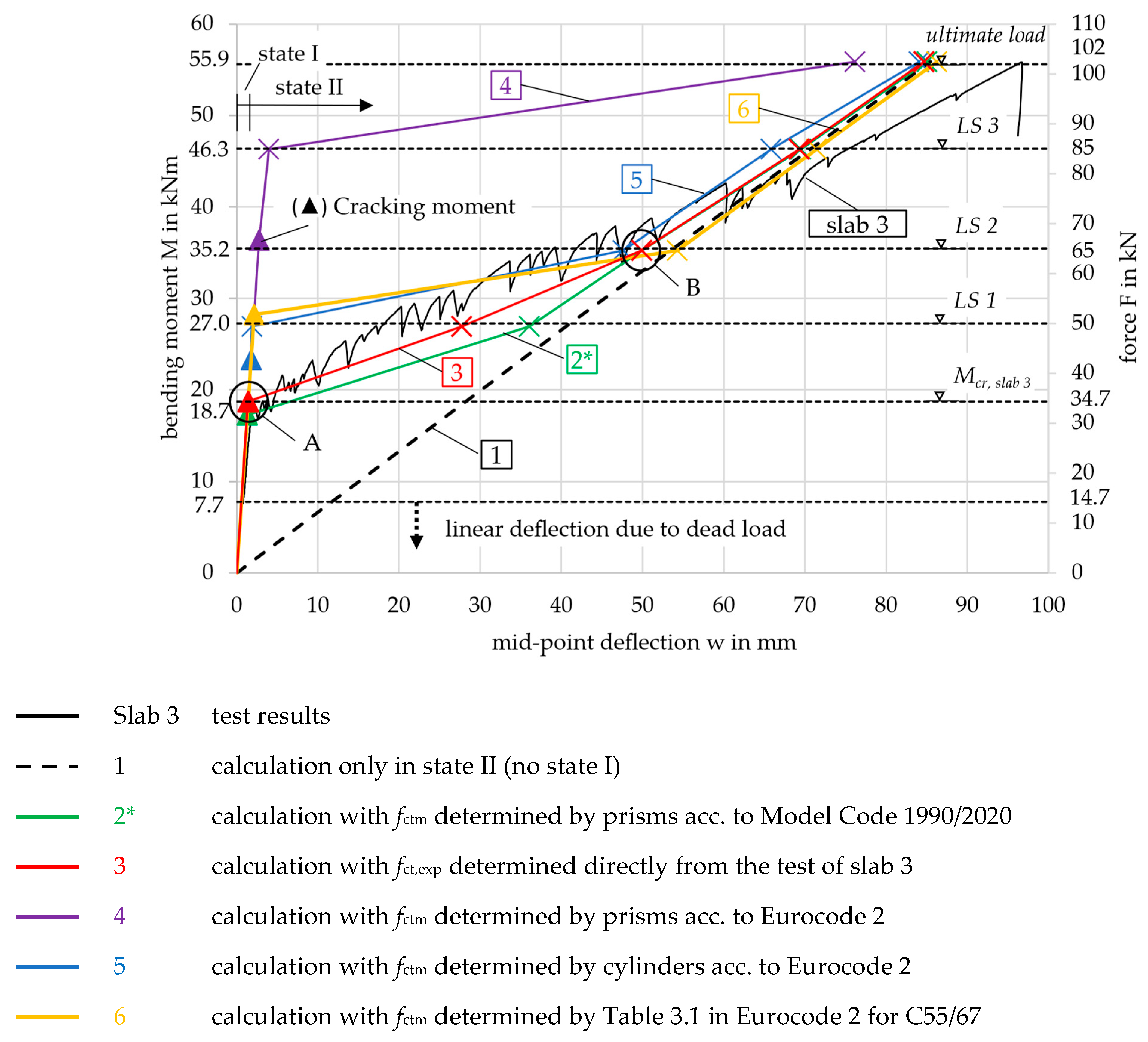
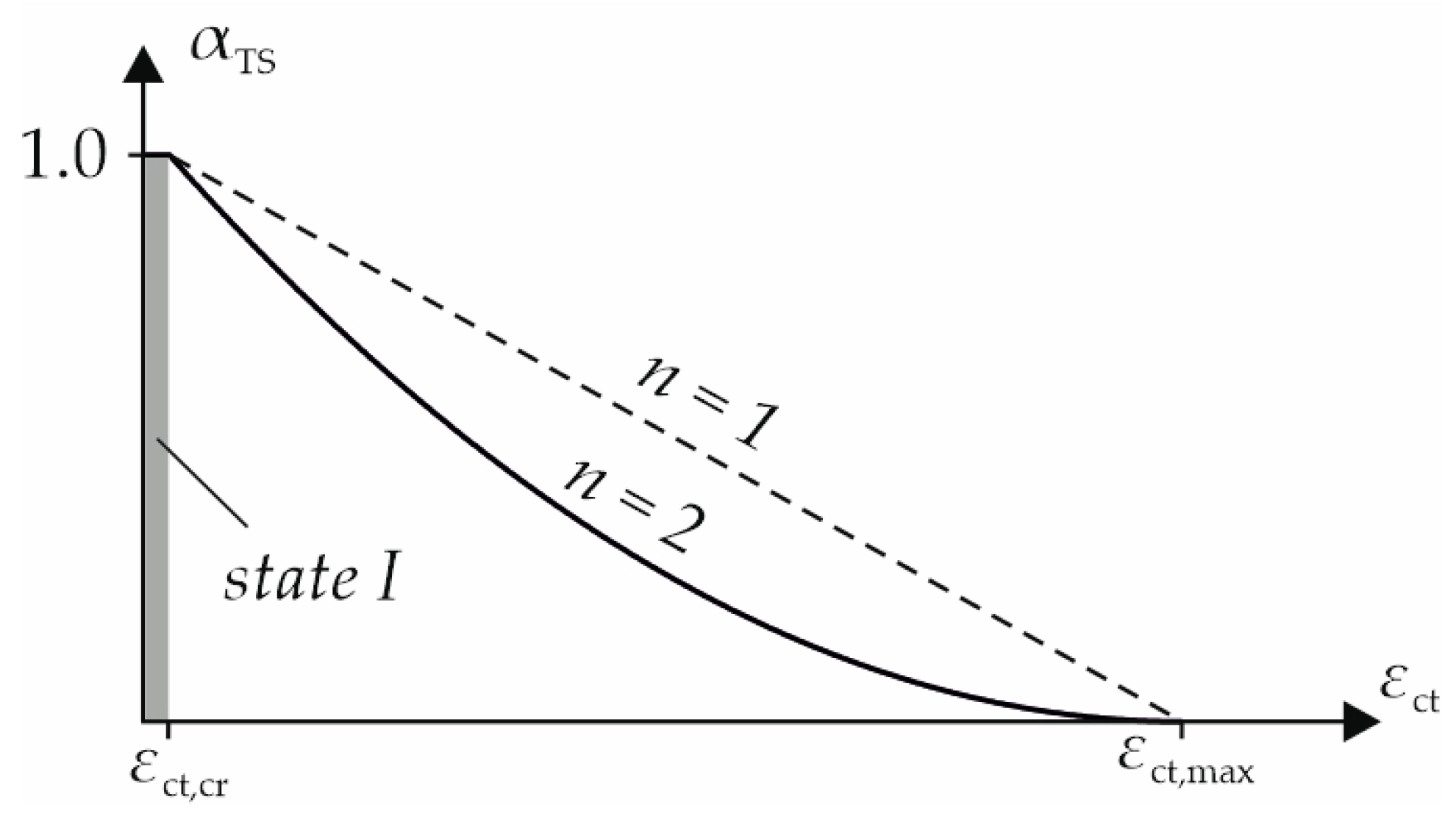
4.3. Discussion on the Concrete Tensile Strength
4.3.1. Simplified Approach
- Cracking moment (state I, EII = 29.0 MNm2)
- →
- Machine force Fcr = 37.4 kN (Mcr,6 = 28.3 kNm), Fcr/2 = 18.7 kN
- →
- →
- →
- Failure load level (state II, EIII = 1.44 MNm2):
- →
- Machine force Fu = 87.3 kN (Mu = 55.9 kNm), Fu/2 = 43.7 kN
- →
- →
- →
4.3.2. Modification of the Concrete Tensile Strength Considering Scaling Effects
4.3.3. Smeared Crack Model and Updated Calculation Results
5. Conclusions
- The production of CRC slab elements for the CUBE and three slab specimens with very slender cross-sections and hollow-core bodies was successfully completed in a prefabrication factory. The many individual steps required were carried out with great care and attention to quality assurance. The correct positioning of the reinforcement in the chords of the cross-section was of particular importance.
- The Ultimate Limit State (ULS) design in bending was adapted from conventional steel-reinforced concrete and successfully applied using the new material parameters of the carbon reinforcement. Small-scale tests provided valuable input values for accurately predicting the load-bearing behavior.
- In addition to the Ultimate Limit State (ULS), the design of the slabs in the Serviceability Limit State (SLS) was also of significant importance. Specifically, the deformation of the slabs needed to be investigated to demonstrate their minimal deflections and to provide an early indication of failure. In the tests, only small deflections were observed at moderate load levels. Up to the SLS, the specimens remained uncracked. A considerable distance to the ultimate load remained, with large deflections of over 80.0 mm and a high number of cracks indicating impending failure.
- The detailed calculation approach for accurately determining the deflections of CRC elements was also adapted from steel-reinforced concrete. The overall results were in good agreement with the experimental tests and also on the safe side for moderate loads. As presented, simplified approaches could also be adopted and modified from reinforced concrete.
- The effects of shrinkage and concrete tensile forces must be carefully considered to ensure accurate deflection calculations. In particular, the determination of tensile strength is crucial, as it significantly impacts the results at moderate load levels. The selected approach must ensure that the deflection calculations remain on the safe side. For the presented slab elements, the estimation of tensile strength from prisms using approaches from Model Code 1990 and 2020 (curve 2*), as well as from cylinders using methods from Eurocode 2 (curve 5), yielded good results. The computational method using tabulated parameters from Eurocode 2 (curve 6) also provided a good approximation of the tests, considering that the experimental value fct,exp is lower than the slab’s mean tensile strength. The approach with prisms in Eurocode 2 (curve 4), however, does not seem suitable for estimating the tensile strength of thin carbon-reinforced concrete components or for calculating their deflection. It significantly overestimates the cracking moment, leading to an underestimation of deformations.
- Shrinkage led to noticeable load drops in displacement-controlled tests and resulted in weaker bending stiffness than initially estimated in the calculations, particularly at higher load levels. These effects could be even more pronounced in very slender structures and cross-sections, necessitating further investigation.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Algorithm for Iteration: Formulas According to [19]
| |
| |
| |
| : Starting from the neutral axis, upward direction (see Figure A1). Only very small concrete compressive strains of were reached. Therefore, also a simplified approach for the compressive force on the linear-elastic branch of the concrete material law (Figure 3) can be used: |
| Simplified approach: |
| |
| |
| |
| |
| If the moment equilibrium is reached, the iteration ends and the correct strain distribution has been determined. If the calculated bending moment from the internal forces does not match the external bending moment, the iteration must be repeated starting from step 1 with the strains adjusted. | |
Appendix B. Integration of Moment–Curvature Relationship
|
|
|
|
|
|
|
|
|
Appendix C. Calculation of the Shrinkage Strain of Slab 3 According to [19]
| (A1) | |||
, with t = 28 d (concrete age) and ts = 1 d (concrete age at the beginning of drying) | ||||
| (A2) | |||
| (A3) | |||
| ||||
| (A4) | |||
| (A5) | |||
| (A6) | |||
| ||||
| (A7) | |||
| ||||
| (A8) | |||
| (A9) | |||
| (A10) | |||
| (A11) | |||
References
- May, S.; Steinbock, O.; Michler, H.; Curbach, M. Precast Slab Structures Made of Carbon Reinforced Concrete. Structures 2019, 18, 20–27. [Google Scholar] [CrossRef]
- Lorenz, E.; Peter, A.; May, S.; Bösche, T. Planung und Neubau einer Straßenbrücke aus Carbonbeton—Carbonbeton, The Next Step. Beton-und Stahlbetonbau 2023, 118, 705–712. [Google Scholar] [CrossRef]
- Kranich, S.; Schladitz, F. C3—Carbon Concrete Composite—Vom größten Bauforschungsprojekt zum bedeutendsten Verband für Carbonbetonbau. Beton-und Stahlbetonbau 2023, 118, 4–6. [Google Scholar] [CrossRef]
- CUBE Das Carbonbetongebäude S2; Ernst & Sohn: Berlin, Germany, 2023; ISBN 0005-9900 (print), 1437-1006 (online).
- CUBE—Neues Bauen mit Carbonbeton: New Building with Carbon Concrete; Curbach, M., Ed.; Wasmuth & Zohlen: Berlin, Germany, 2022; ISBN 978 3 8030 2372 8. [Google Scholar]
- Frenzel, M.; Schmidt, A.; Scheerer, S. ZiE für Carbonbetonbauteile in Halbfertigteil-und Fertigteilbauweise im Gebäudeteil BOX des CUBE. Beton-und Stahlbetonbau 2023, 118, 49–56. [Google Scholar] [CrossRef]
- Frenzel, M.; Sandmann, D.; Curbach, M. Der Forschungsbau CUBE demonstriert eindrucksvoll die Praxistauglichkeit des Innovativen Baustoffs Carbonbeton. Der Prüfingenieur 2023, 62, 21–27. Available online: https://www.bvpi.de/bvpi/downloads/der-pruefingenieur/Pruefingenieur_62.pdf (accessed on 10 June 2024).
- Frenzel, M.; Tietze, M.; Kahnt, A. The Carbon-Reinforced Concrete Building—Cube|Box Manufacturing and Assembling. In Developing Materials and Structures for Sustainable Engineering, Proceedings of the International Structural Engineering and Construction. The Fifth European and Mediterranean Structural Engineering and Construction Conference, Vilnius Gediminas Technical University, Vilnius, Lithuania, 13–18 May 2024; Gribniak, V., Rimkus, A., Holschemacher, K., Quapp, U., Singh, A., Yazdani, S., Eds.; ISEC Press: Fargo, ND, USA, 2024; pp. 1–6. [Google Scholar]
- Solidian GmbH. Solidian Q95/95-CCE-38-E2 [Technical Data Sheet]; Solidian GmbH: Albstadt, Germany, 2017. [Google Scholar]
- Frenzel, M.; Scheerer, S.; Schmidt, A. Übersicht über die im CUBE verwendeten Materialien. Beton-und Stahlbetonbau 2023, 118, 25–36. [Google Scholar] [CrossRef]
- Morales Cruz, C.; Raupach, M. Influence of the surface modification by sanding of carbon textile reinforcements on the bond and load-bearing behavior of textile reinforced concrete. MATEC Web Conf. 2019, 289, 4006. [Google Scholar] [CrossRef]
- Preinstorfer, P.; Yanik, S.; Kirnbauer, J.; Lees, J.M.; Robisson, A. Cracking behaviour of textile-reinforced concrete with varying concrete cover and textile surface finish. Compos. Struct. 2023, 312, 116859. [Google Scholar] [CrossRef]
- DIN EN 206:2021-06; Beton—Festlegung, Eigenschaften, Herstellung und Konformität; Deutsche Fassung EN 206:2013+A2:2021. Beuth Verlag GmbH: Berlin, Germany, 2021; 91.100.30.
- DIN EN 12390-1:2021-09; Prüfung von Festbeton—Teil 1: Form, Maße und Andere Anforderungen für Probekörper und Formen; Deutsche Fassung EN 12390-1:2021. Beuth Verlag GmbH: Berlin, Germany, 2021.
- DIN EN 12390-2:2019-10; Prüfung von Festbeton—Teil 2: Herstellung und Lagerung von Probekörpern für Festigkeitsprüfungen; Deutsche Fassung EN 12390-2:2019. Beuth Verlag GmbH: Berlin, Germany, 2019.
- DIN EN 12390-3:2019-10; Prüfung von Festbeton—Teil 3: Druckfestigkeit von Probekörpern; Deutsche Fassung EN 12390-3:2019. Beuth Verlag GmbH: Berlin, Germany, 2019.
- DIN EN 12390-6:2010-09; Prüfung von Festbeton—Teil 6: Spaltzugfestigkeit von Probekörpern; Deutsche Fassung EN 12390-6:2009. Beuth Verlag GmbH: Berlin, Germany, 2010.
- DIN EN 12390-13:2021-09; Prüfung von Festbeton—Teil 13: Bestimmung des Elastizitätsmoduls Unter Druckbelastung (Sekantenmodul); Deutsche Fassung EN 12390-13:2021. Beuth Verlag GmbH: Berlin, Germany, 2021.
- DIN EN 1992-1-1; Eurocode 2: Bemessung und Konstruktion von Stahlbeton- und Spannbetontragwerken—Teil 1-1: Allgemeine Bemessungsregeln und Regeln für den Hochbau; Deutsche Fassung EN 1992-1-1:2004 + AC:2010. Beuth Verlag GmbH: Berlin, Germany, 2011; 91.010.30.
- Zilch, K.; Zehetmaier, G. Bemessung im Konstruktiven Betonbau; Springer: Berlin/Heidelberg, Germany, 2010; ISBN 978-3-540-70637-3. [Google Scholar]
- CEB-FIP Model Code 1990; Fédération Internationale de la Précontrainte: Design Code. Telford: London, UK, 1993; 213/214.
- International Federation for Structural Concrete. Fib Model Code for Concrete Structures 2020; International Federation of Structural Concrete: Lausanne, Switzerland, 2023; Available online: https://fib-international.org/publications/fib-bulletins/fib-model-code-2020-detail.html (accessed on 10 June 2024).
- Deutscher Ausschuss für Stahlbeton. DAfStb-Richtlinie Betonbauteile mit Nichtmetallischer Bewehrung: 2024-01; Beuth Verlag GmbH: Berlin, Germany, 2024. [Google Scholar]
- Schütze, E.; Bielak, J.; Scheerer, S.; Hegger, J.; Curbach, M. Einaxialer Zugversuch für Carbonbeton mit textiler Bewehrung. Beton-und Stahlbetonbau 2018, 113, 33–47. [Google Scholar] [CrossRef]
- DIN EN 1990:2021-10; Eurocode: Grundlagen der Tragwerksplanung; Deutsche Fassung EN 1990:2002 + A1:2005 + A1:2005/AC:2010. Beuth Verlag GmbH: Berlin, Germany, 2021; 91.010.30 (DIN EN 1990).
- Dhahir, M.K.; Marx, S. Shrinkage behaviour of high-strength concrete plates reinforced with carbon textile reinforcement. Structures 2024, 69, 107504. [Google Scholar] [CrossRef]
- Bauministerkonferenz. Musterbauordnung: MBO; Deutsches Institut für Bautechnik (DIBt): Berlin, Germany, 2023. [Google Scholar]
- Quast, U. Zur Mitwirkung des Betons in der Zugzone. Beton-und Stahlbetonbau 1981, 76, 247–250. [Google Scholar] [CrossRef]
- Quast, U. Zur Auswahl eines geeigneten Verfahrens für die Berücksichtigung der Mitwirkung des Betons auf Zug. Bautechnik 2010, 87, 397–403. [Google Scholar] [CrossRef]
- Espion, B. Contribution à L’analyse non Linéaire des Ossatures Planes. Application Aux Structures en Béton Armé. Ph.D. Thesis, Université Libre de Bruxelles, Bruxelles, Belgium, 1985. [Google Scholar]
- Pfeiffer, U. Die Nichtlineare Berechnung Ebener Rahmen aus Stahl-Oder Spannbeton mit Berücksichtigung der Durch das Aufreißen Bedingten Achsendehnung. Ph.D. Thesis, Technische Universität Hamburg-Harburg, Hamburg-Harburg, Germany, 2004. [Google Scholar]
- Hiesch, D. Bemessung von Betonfertigteilen mit Vorgespannter Bewehrung aus Faserverbundkunststoff: Entwicklung eines Bemessungskonzeptes unter Berücksichtigung Wirklichkeitsnaher Bauteilverformungen. Ph.D. Thesis, Technische Universität Darmstadt, Darmstadt, Germany, 2023. [Google Scholar]
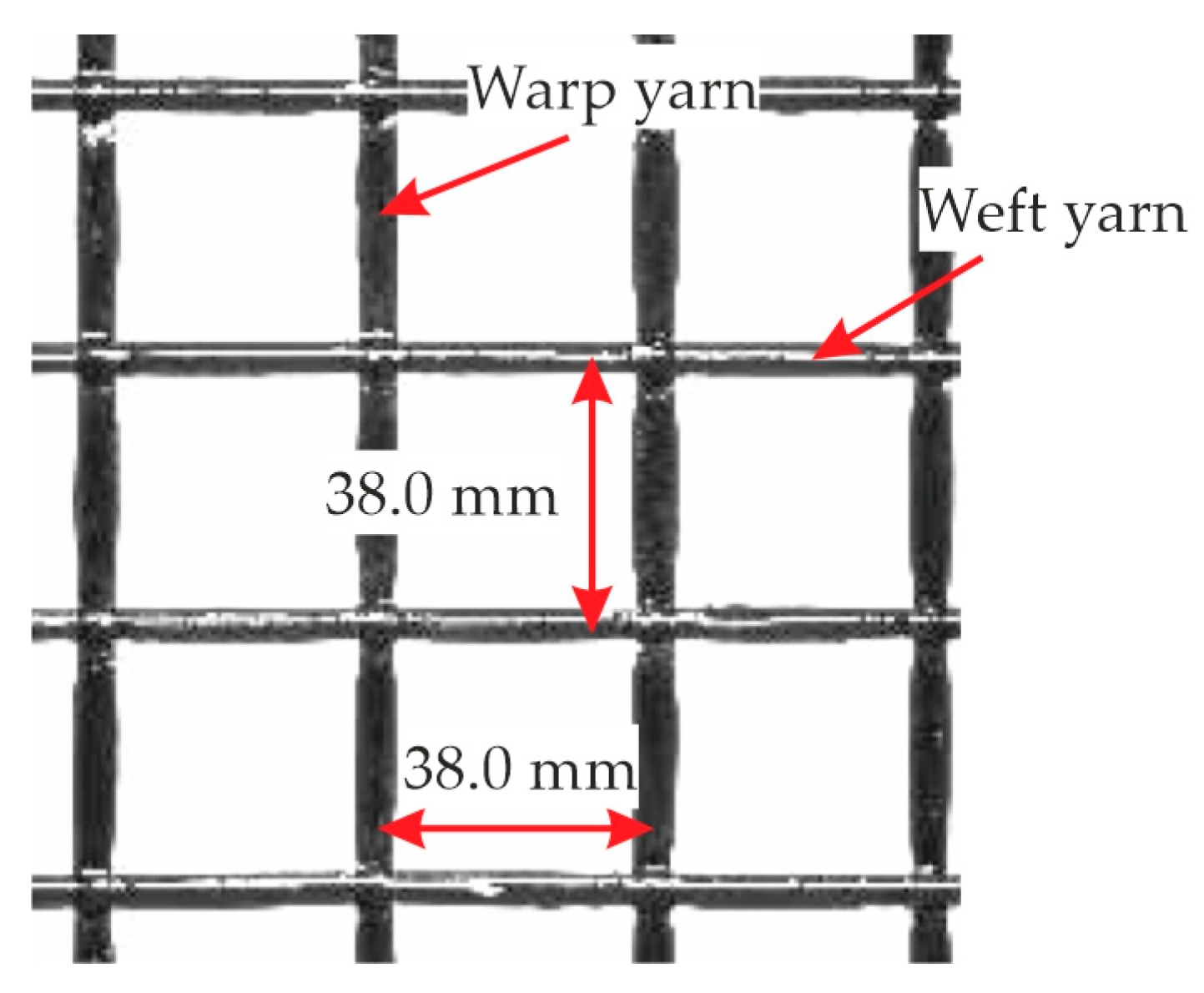





| Test Series | Unit | Value |
|---|---|---|
| Fiber cross-sectional area of fiber strand | mm2 | 3.62 |
| Fiber cross-sectional area | mm2/m | 95.3 |
| Grid width in warp and weft direction | mm | 38 |
| Fiber strands per Meter | - | 26.3 |
| Test Series 1 | Unit | Value | σ | CoV | Age |
|---|---|---|---|---|---|
| Mean concrete compressive strength fcm 2,a | MPa | 68.3 | 0.93 | 0.01 | 31 |
| Mean splitting tensile strength fctm,sp 2,a | MPa | 3.9 | 0.24 | 0.06 | 34 |
| Mean flexural tensile strength fct,fl,m 3,b | MPa | 9.7 | 0.11 | 0.01 | 28 |
| Mean Young’s modulus Ecm 2,a | MPa | 34.733 | 901.9 | 0.03 | 31 |
| Conversion | fctm in MPa | εct,cr in ‰ |
|---|---|---|
| From fctm,sp by Equation (1) acc. to Eurocode 2 [19] | 3.5 | 0.10 |
| From fct,fl,m by Equation (2) acc. to Eurocode 2 [19] | 6.2 | 0.18 |
| From fct,fl,m by Equation (3) acc. to Model Code 1990/2020 [21,22] | 4.3 | 0.12 |
| From Table 3.1 in Eurocode 2 [19] | 4.2 | 0.12 |
| Mean tensile strength regarding Af,nm | ff,nm,m | 3815 MPa |
| Ultimate strain | εnm | 12.3‰ |
| Coefficient of variation | 0.064 | |
| Characteristic value | 3383 MPa |
| Load Steps | Force in kN | Moment in kNm |
|---|---|---|
| LS 1 | 50.0 | 27.0 |
| LS 2 | 65.0 | 35.2 |
| LS 3 | 85.0 | 46.3 |
| Ultimate failure load | 101.9 | 55.9 |
 | slab 3 | test results |
 | 1 | calculation only in state II (no state I) |
 | 2/2* | calculation with fctm determined by prisms acc. to [21,22] |
 | 3 | calculation with fct,exp determined directly from the test of slab 3 |
 | 4 | calculation with fctm determined by prisms acc. to [19] |
 | 5 | calculation with fctm determined by cylinders acc. to [19] |
 | 6 | calculation with fctm determined by Table 3.1 in [19] for C55/67 |
| fctm in MPa | Mcr in kNm | wcr in mm | ||
|---|---|---|---|---|
 | 1 | - | - | - |
 | 2 | 4.3 | 28.8 | 2.2 |
 | 2* | 2.6 | 17.3 | 1.3 |
 | 3 | 2.8 | 18.7 | 1.4 |
 | 4 | (6.2) 1 5.4 | (41.8) 1 36.4 | 2.8 |
 | 5 | 3.5 | 23.3 | 1.7 |
 | 6 | 4.2 | 28.3 | 2.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sandmann, D.; Frenzel, M.; Marx, S.; Curbach, M. Investigations on the Deflection of Carbon-Reinforced Concrete Hollow-Core Slabs. Materials 2025, 18, 1212. https://doi.org/10.3390/ma18061212
Sandmann D, Frenzel M, Marx S, Curbach M. Investigations on the Deflection of Carbon-Reinforced Concrete Hollow-Core Slabs. Materials. 2025; 18(6):1212. https://doi.org/10.3390/ma18061212
Chicago/Turabian StyleSandmann, David, Michael Frenzel, Steffen Marx, and Manfred Curbach. 2025. "Investigations on the Deflection of Carbon-Reinforced Concrete Hollow-Core Slabs" Materials 18, no. 6: 1212. https://doi.org/10.3390/ma18061212
APA StyleSandmann, D., Frenzel, M., Marx, S., & Curbach, M. (2025). Investigations on the Deflection of Carbon-Reinforced Concrete Hollow-Core Slabs. Materials, 18(6), 1212. https://doi.org/10.3390/ma18061212







