Vibration Analysis of Shape Memory Alloy Enhanced Multi-Layered Composite Beams with Asymmetric Material Behavior
Abstract
:1. Introduction
2. A Brief Review on PM
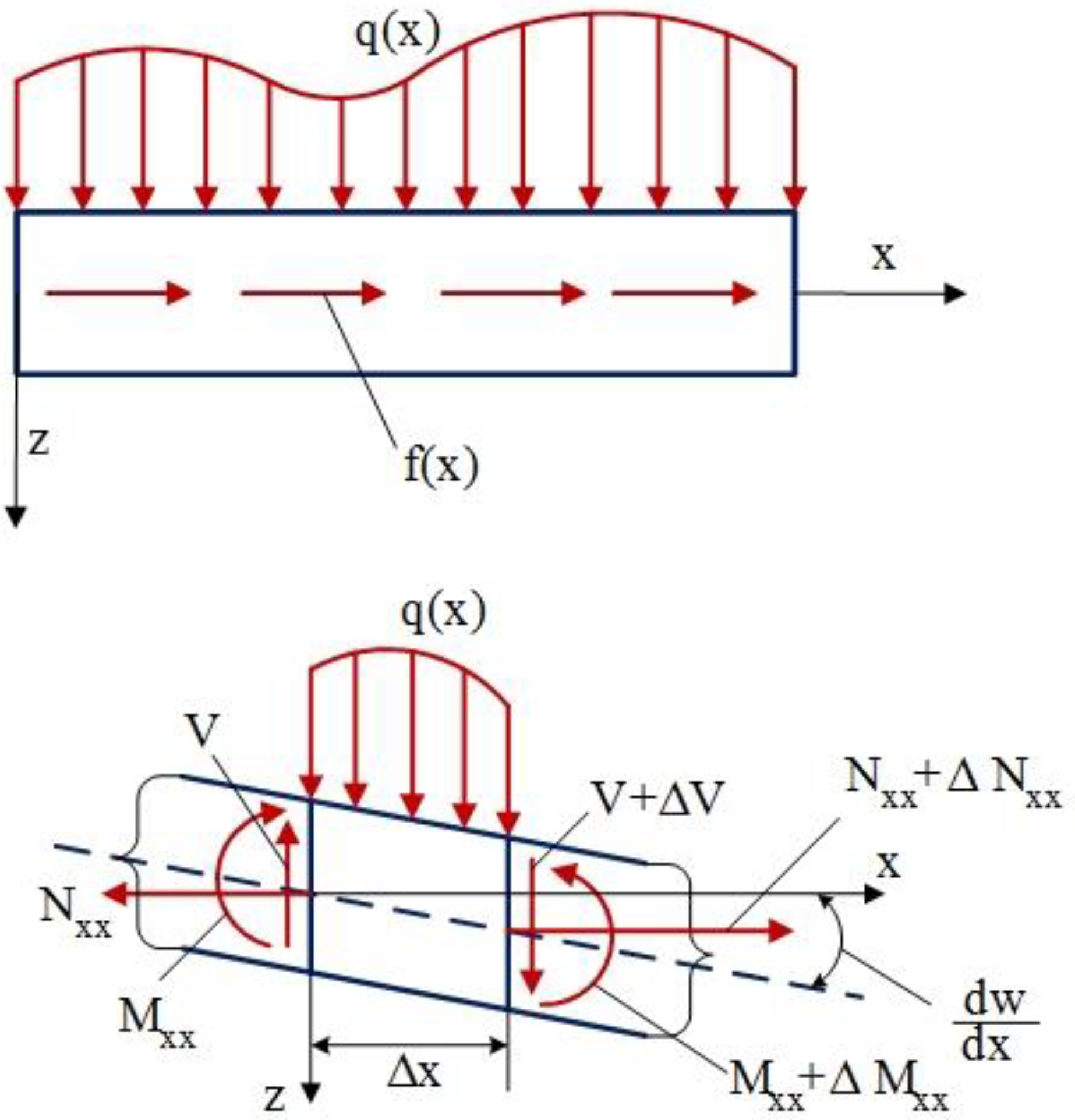
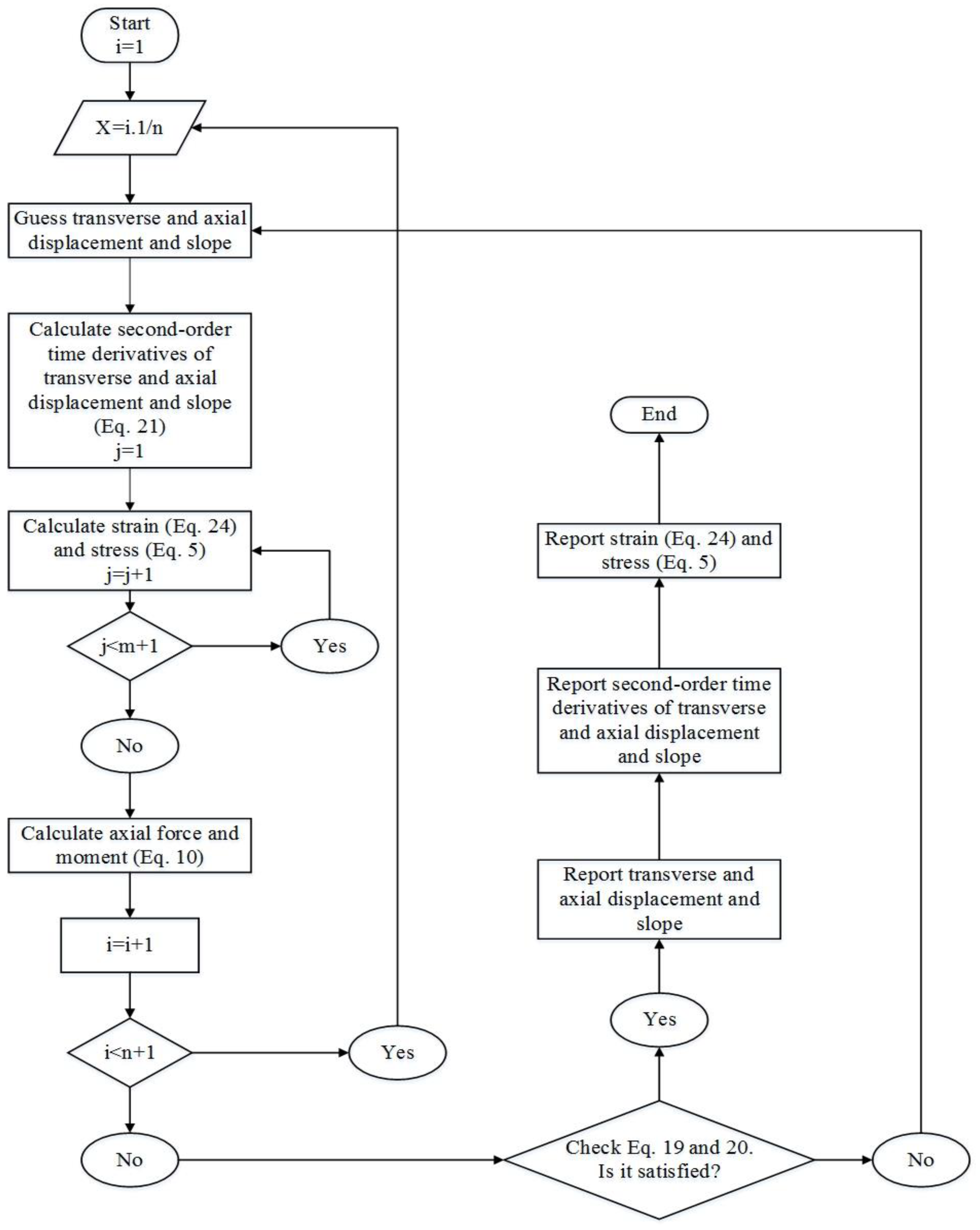
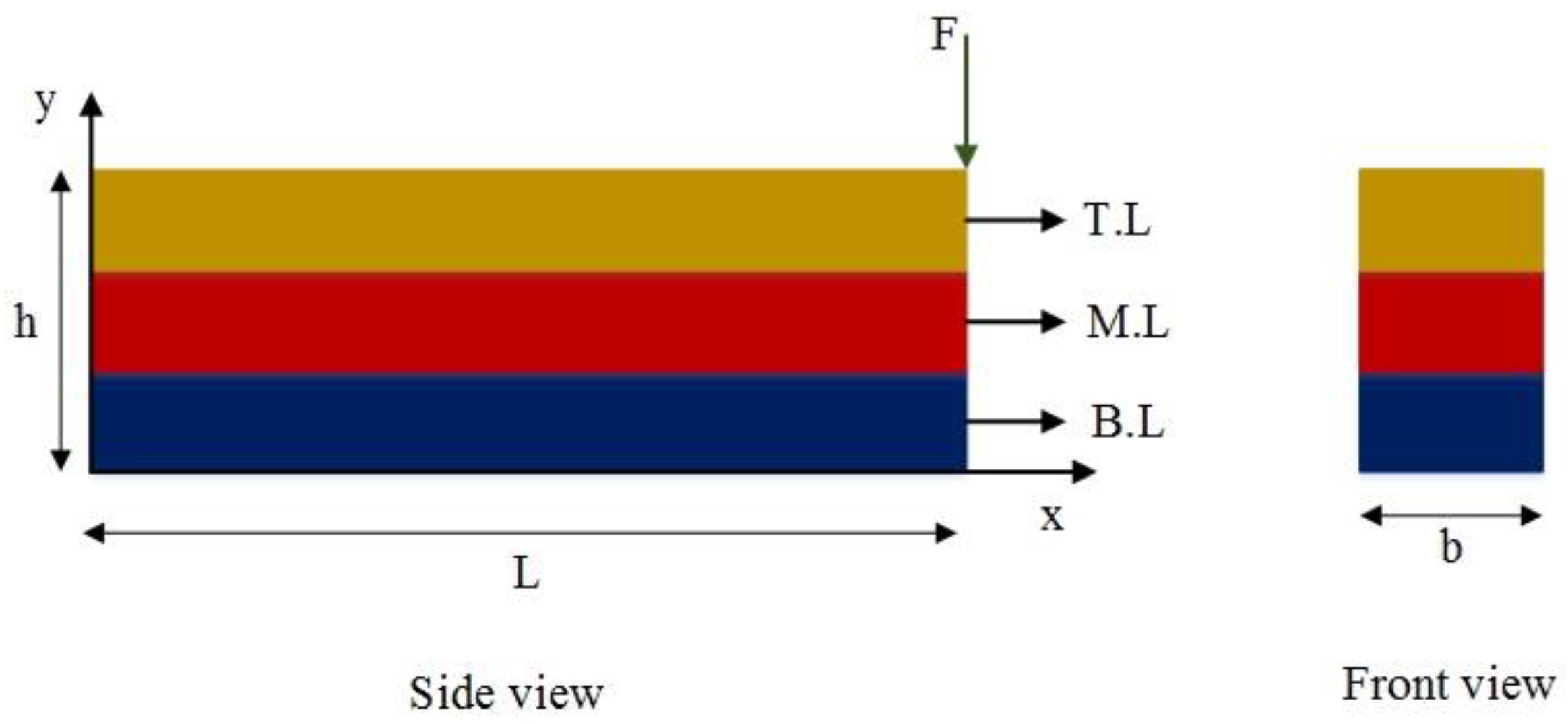
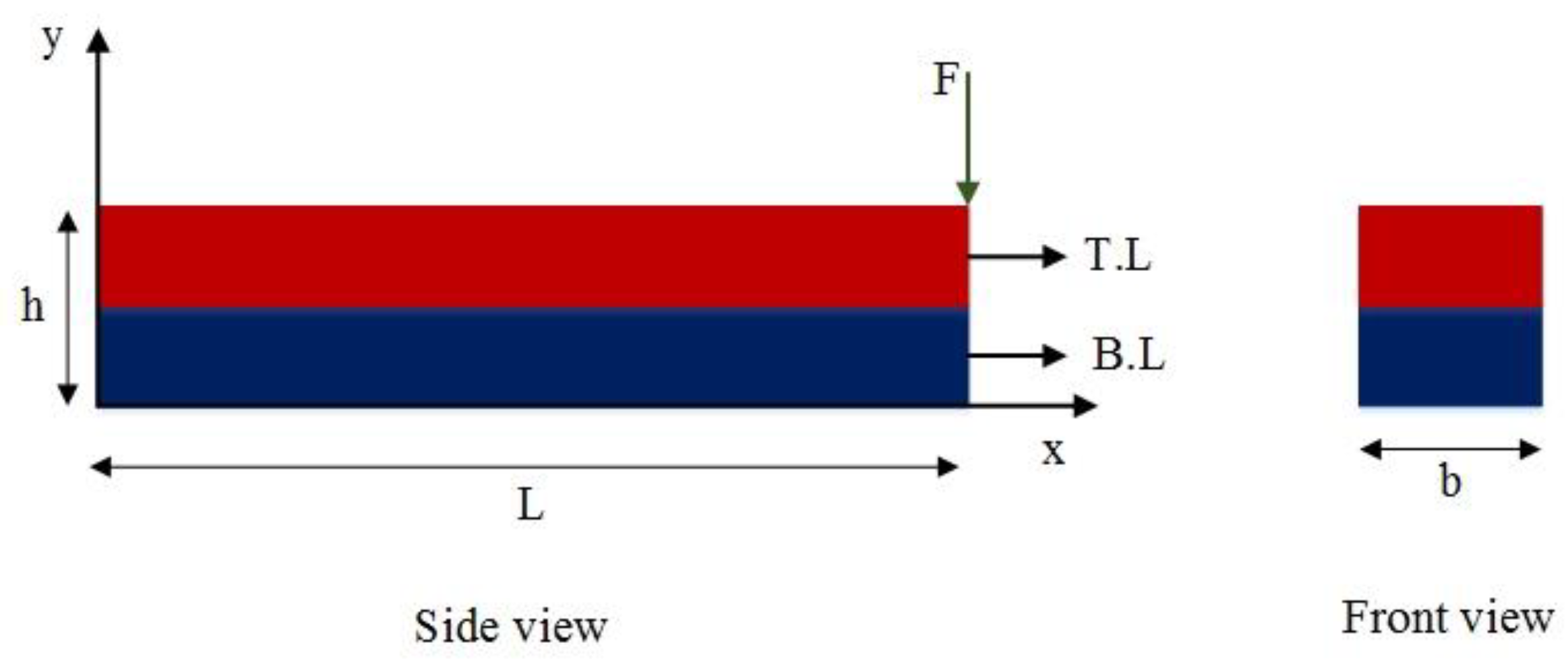
3. Beam Model Development
4. Numerical Results
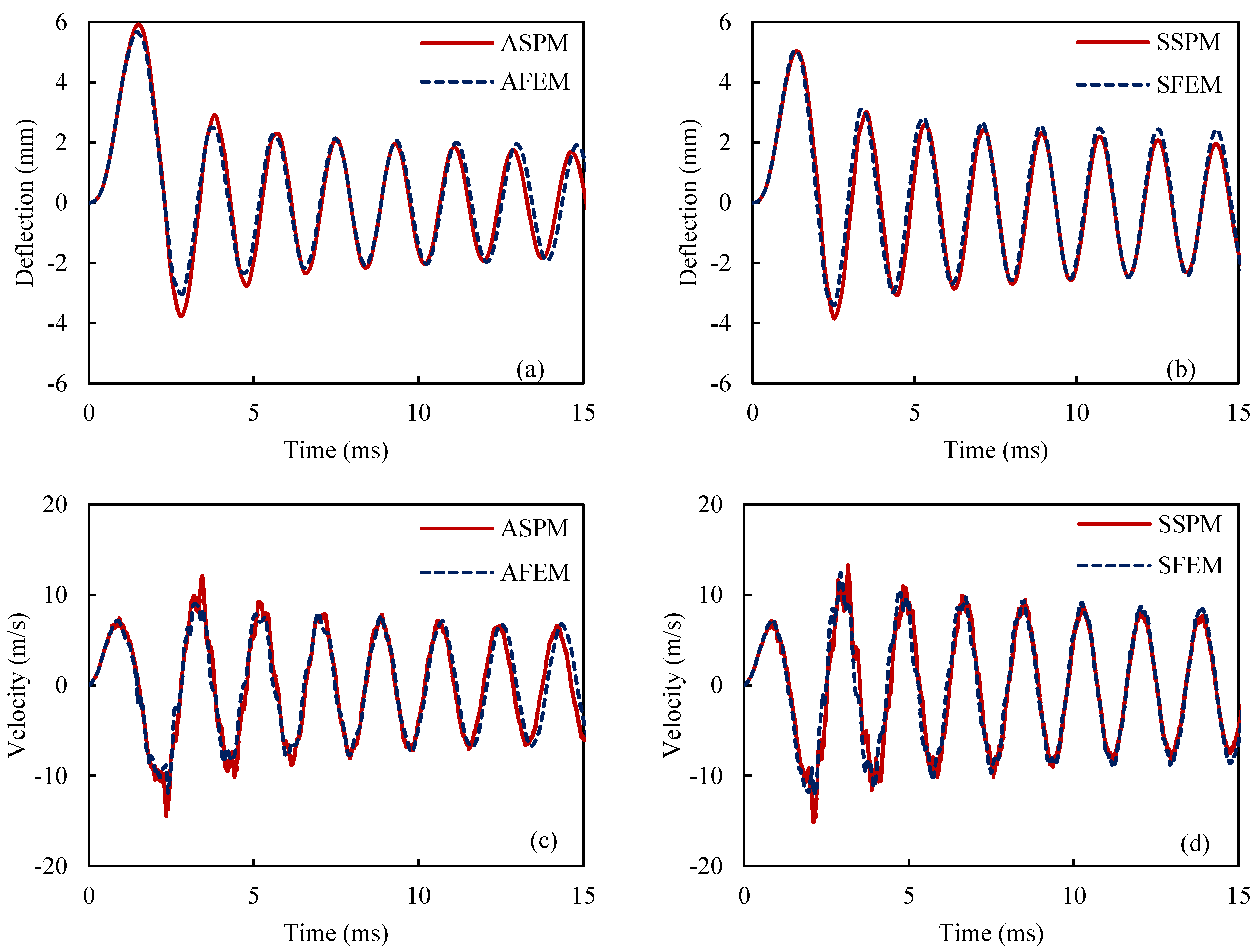
4.1. Validation
4.2. Free Vibration Behavior of a Tri-Layer SMA Beam
4.2.1. Free Vibration of the Tri-Layer SMA Composite Beam
4.2.2. Parametric Study
4.3. Free Vibration Behavior of a Bi-Layer SMA Beam
4.3.1. Free Vibration of the Bi-Layer SMA Composite Beam
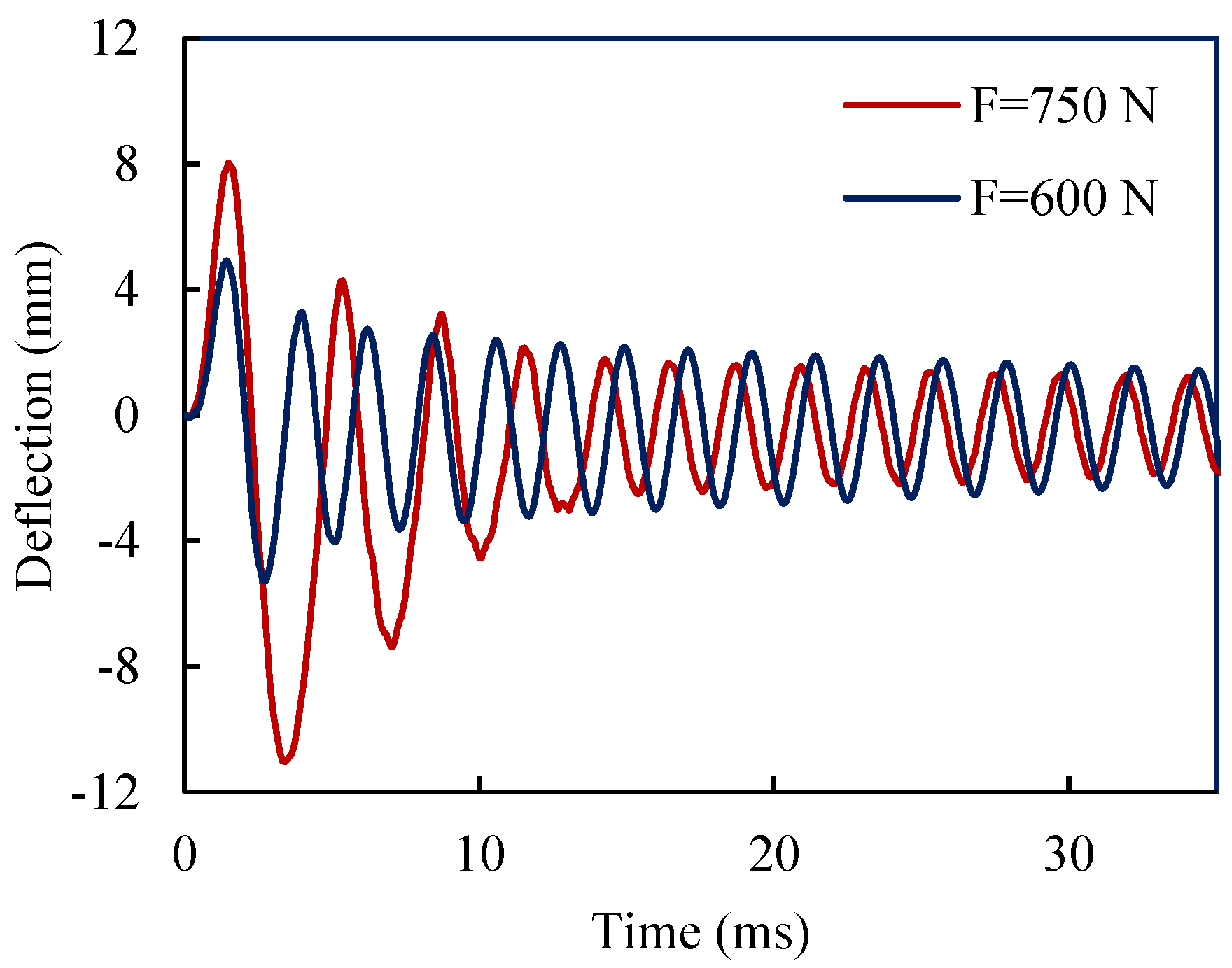
4.3.2. Effect of Loading Amplitude on Free Vibration Response
5. Concluding Remarks
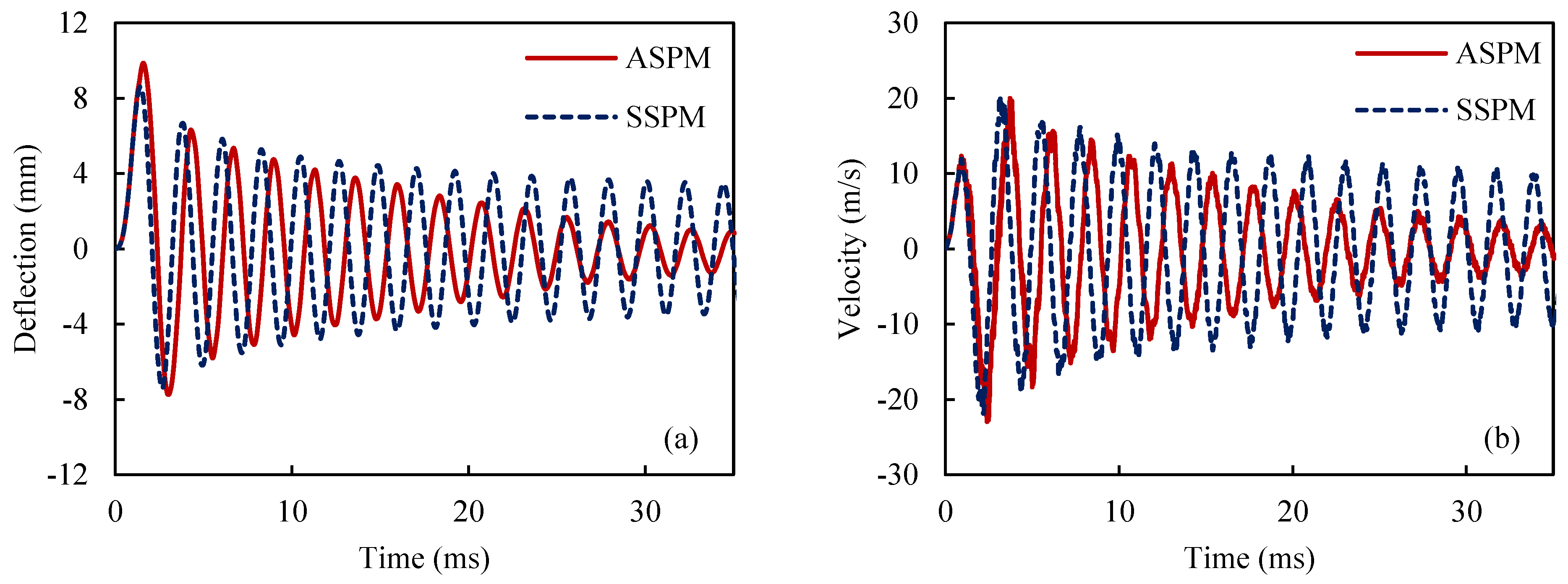
- Due to the hysteresis property of SMAs, the vibration amplitude of the beam is reduced effectively a short time after the beginning of the oscillation.
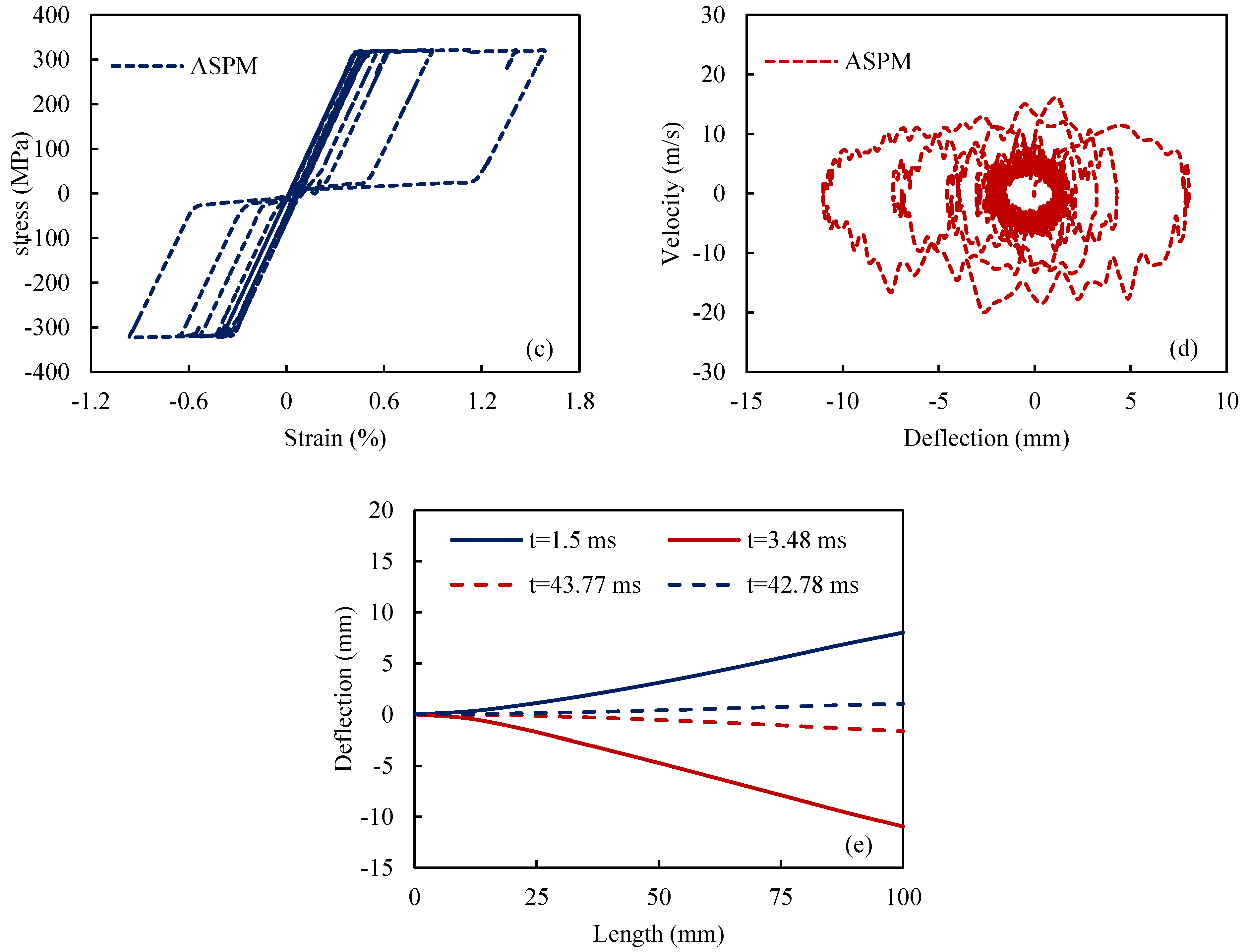
- In the steady-state part of the solution, the SMA composite beam vibrates as an elastic material.
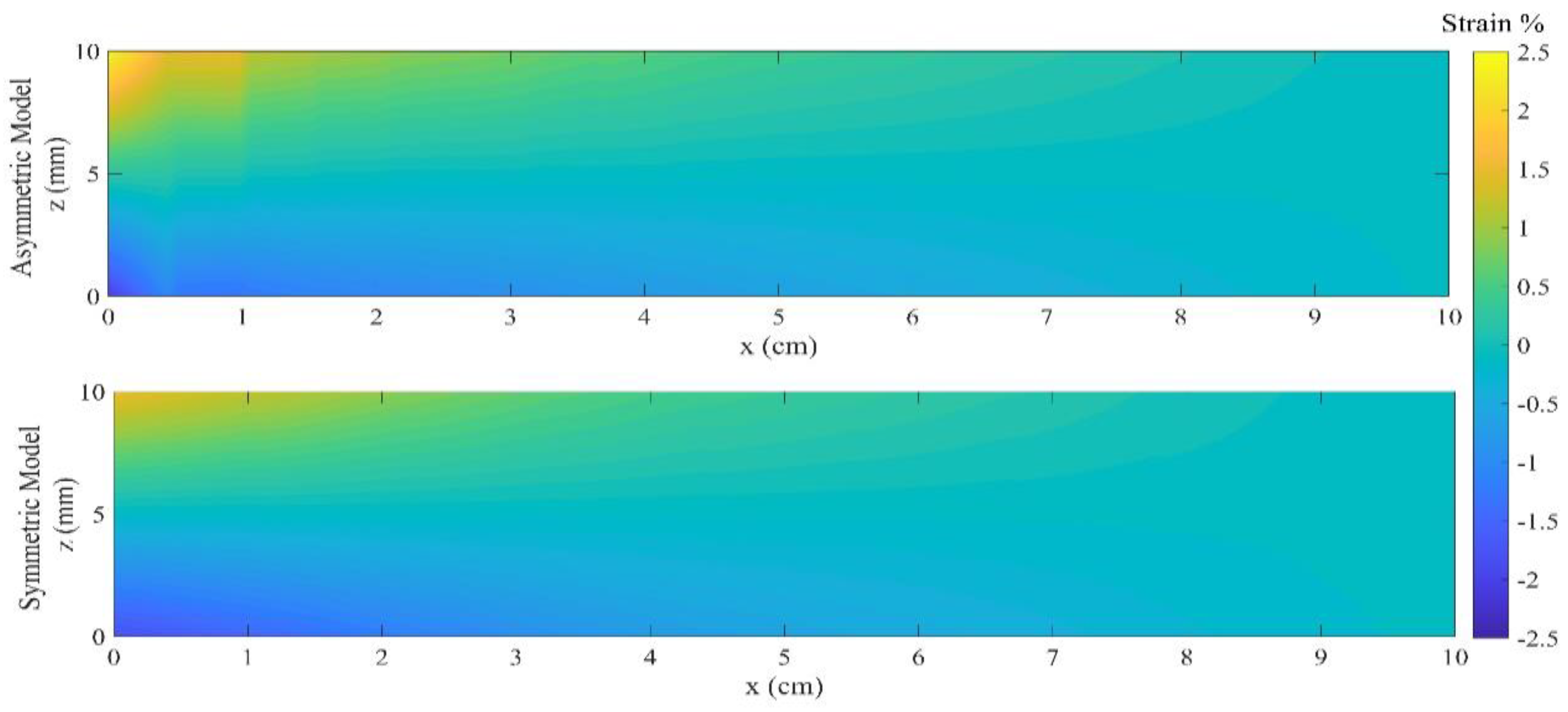
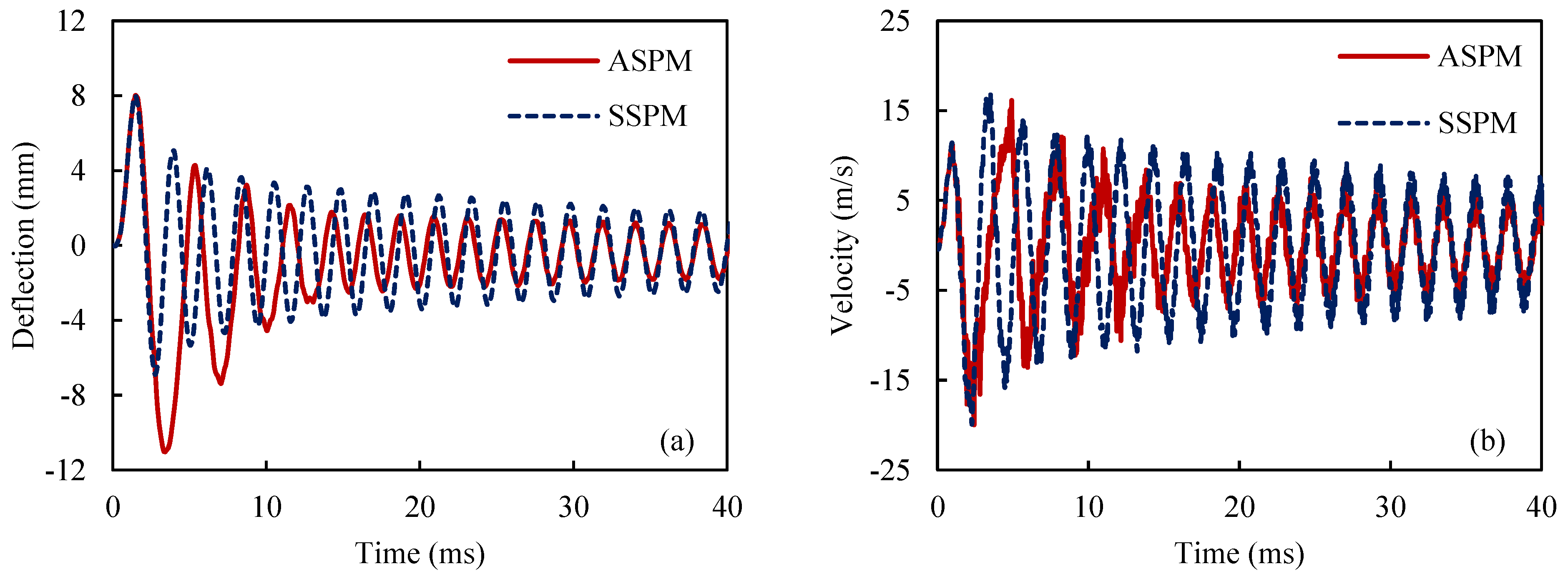
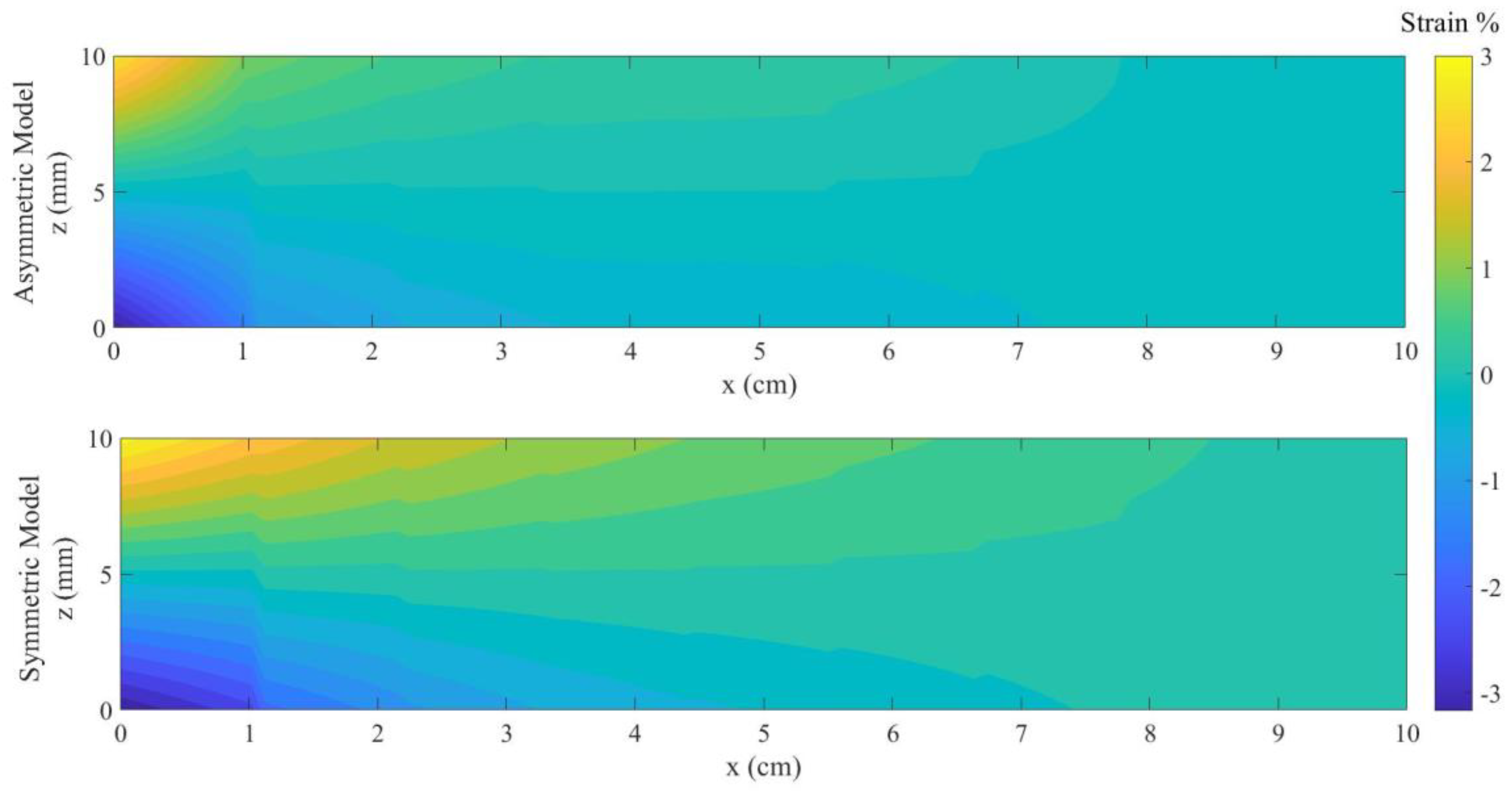
- Regarding the tip deflection and the velocity–time diagrams, there is a noticeable deviation between the asymmetric and symmetric models.
- Due to the energy-dissipating behavior of SMAs, the hysteresis loop in the stress–strain diagram shrinks steadily until it takes a linear form.
- Regarding the phase diagram, the transient state and steady state are observed.
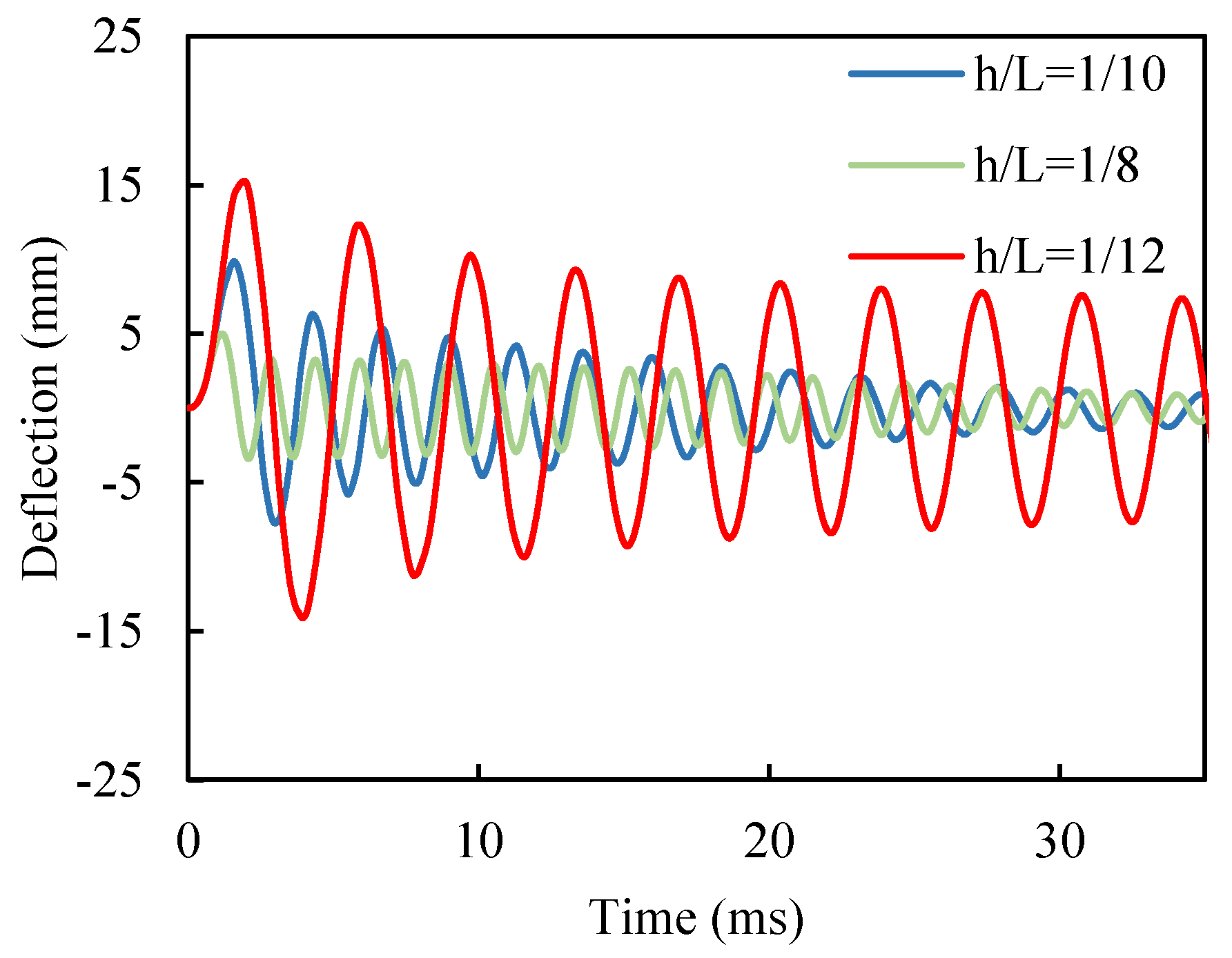
- Performing a parametric study on the multi-layer SMA beam, the results revealed that the steady-state amplitude rises in a smaller height to length ratio.
- SMA layers show higher capability in damping the vibration and dissipating energy of the multi-layer beam under a larger intensity of loading.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ghabussi, A.; Ashrafi, N.; Shavalipour, A.; Hosseinpour, A.; Habibi, M.; Moayedi, H.; Babaei, B.; Safarpour, H. Free vibration analysis of an electro-elastic GPLRC cylindrical shell surrounded by viscoelastic foundation using modified length-couple stress parameter. Mech. Based Des. Struct. Mach. 2021, 49, 738–762. [Google Scholar] [CrossRef]
- Ghabussi, A.; Habibi, M.; NoormohammadiArani, O.; Shavalipour, A.; Moayedi, H.; Safarpour, H. Frequency characteristics of a viscoelastic graphene nanoplatelet–reinforced composite circular microplate. J. Vib. Control 2021, 27, 101–118. [Google Scholar] [CrossRef]
- Huo, J.; Zhang, G.; Ghabussi, A.; Habibi, M. Bending analysis of FG-GPLRC axisymmetric circular/annular sector plates by considering elastic foundation and horizontal friction force using 3D-poroelasticity theory. Compos. Struct. 2021, 276, 114438. [Google Scholar]
- Ma, R.; Karimzadeh, M.; Ghabussi, A.; Zandi, Y.; Baharom, S.; Selmi, A.; Maureira-Carsalade, N. Assessment of composite beam performance using GWO–ELM metaheuristic algorithm. Eng. Comput. 2021, 38, 2083–2099. [Google Scholar] [CrossRef]
- Morasaei, A.; Ghabussi, A.; Aghlmand, S.; Yazdani, M.; Baharom, S.; Assilzadeh, H. Simulation of steel–concrete composite floor system behavior at elevated temperatures via multi-hybrid metaheuristic framework. Eng. Comput. 2022, 38, 2567–2582. [Google Scholar]
- Fahimi, P.; Eskandari, A.H.; Baghani, M.; Taheri, A. A semi-analytical solution for bending response of SMA composite beams considering SMA asymmetric behavior. Compos. Part B Eng. 2019, 163, 622–633. [Google Scholar]
- Samadi-Aghdam, K.; Fahimi, P.; Baniassadi, M.; Baghani, M. Development and implementation of a geometrically nonlinear beam theory model for SMA composite beams with asymmetric behavior. Compos. Struct. 2021, 259, 113417. [Google Scholar]
- Fahimi, P.; Hajarian, A.; Eskandari, A.H.; Taheri, A.; Baghani, M. Asymmetric bending response of shape memory alloy beam with functionally graded porosity. J. Intell. Mater. Syst. Struct. 2020, 31, 1935–1949. [Google Scholar]
- Auricchio, F.; Andrea, P. Numerical modeling of shape-memory alloys in orthodontics. J. Orthod. 2003, 4, 365–380. [Google Scholar]
- Miura, F.; Mogi, M.; Ohura, Y.; Karibe, M. The super-elastic Japanese NiTi alloy wire for use in orthodontics part III. Studies on the Japanese NiTi alloy coil springs. Am. J. Orthod. Dentofac. Orthop. 1988, 94, 89–96. [Google Scholar]
- Saadat, S.; Salichs, J.; Noori, M.; Hou, Z.; Davoodi, H.; Bar-On, I.; Suzuki, Y.; Masuda, A. An overview of vibration and seismic applications of NiTi shape memory alloy. Smart Mater. Struct. 2002, 11, 218. [Google Scholar] [CrossRef]
- Bil, C.; Massey, K.; Abdullah, E.J. Wing morphing control with shape memory alloy actuators. J. Intell. Mater. Syst. Struct. 2013, 24, 879–898. [Google Scholar] [CrossRef]
- Furuya, Y.; Shimada, H. Shape memory actuators for robotic applications. Mater. Des. 1991, 12, 21–28. [Google Scholar]
- Williams, K.; Chiu, G.-C.; Bernhard, R. Dynamic modelling of a shape memory alloy adaptive tuned vibration absorber. J. Sound Vib. 2005, 280, 211–234. [Google Scholar] [CrossRef]
- Savi, M.A.; De Paula, A.S.; Lagoudas, D.C. Numerical investigation of an adaptive vibration absorber using shape memory alloys. J. Intell. Mater. Syst. Struct. 2011, 22, 67–80. [Google Scholar] [CrossRef]
- Brinson, L.C. One-dimensional constitutive behavior of shape memory alloys: Thermomechanical derivation with non-constant material functions and redefined martensite internal variable. J. Intell. Mater. Syst. Struct. 1993, 4, 229–242. [Google Scholar] [CrossRef]
- Poorasadion, S.; Arghavani, J.; Naghdabadi, R.; Sohrabpour, S. An improvement on the Brinson model for shape memory alloys with application to two-dimensional beam element. J. Intell. Mater. Syst. Struct. 2014, 25, 1905–1920. [Google Scholar] [CrossRef]
- Aguiar, R.A.; Savi, M.A.; Pacheco, P.M. Experimental investigation of vibration reduction using shape memory alloys. J. Intell. Mater. Syst. Struct. 2013, 24, 247–261. [Google Scholar]
- dos Santos, F.A.; Cismaşiu, C. Adaptive underslung beam using shape-memory alloys for frequency-tuning. J. Intell. Mater. Syst. Struct. 2017, 28, 1260–1271. [Google Scholar] [CrossRef]
- Jeong, H.-K.; Han, J.-H.; Youn, S.-H.; Lee, J. Frequency tunable vibration and shock isolator using shape memory alloy wire actuator. J. Intell. Mater. Syst. Struct. 2014, 25, 908–919. [Google Scholar]
- Feng, Z.; Li, D. Dynamics of a mechanical system with a shape memory alloy bar. J. Intell. Mater. Syst. Struct. 1996, 7, 399–410. [Google Scholar] [CrossRef]
- Hashemi, S.; Khadem, S. Modeling and analysis of the vibration behavior of a shape memory alloy beam. Int. J. Mech. Sci. 2006, 48, 44–52. [Google Scholar] [CrossRef]
- Jafari, A.; Ghiasvand, H. Dynamic response of a pseudoelastic shape memory alloy beam to a moving load. J. Sound Vib. 2008, 316, 69–86. [Google Scholar] [CrossRef]
- Zbiciak, A. Dynamic analysis of pseudoelastic SMA beam. Int. J. Mech. Sci. 2010, 52, 56–64. [Google Scholar] [CrossRef]
- Jose, S.; Chakraborty, G.; Bhattacharyya, R. Coupled thermo-mechanical analysis of a vibration isolator made of shape memory alloy. Int. J. Solids Struct. 2017, 115, 87–103. [Google Scholar] [CrossRef]
- Ozbulut, O.E.; Mir, C.; Moroni, M.O.; Sarrazin, M.; Roschke, P.N. A fuzzy model of superelastic shape memory alloys for vibration control in civil engineering applications. Smart Mater. Struct. 2007, 16, 818. [Google Scholar] [CrossRef]
- Araki, Y.; Kimura, K.; Asai, T.; Masui, T.; Omori, T.; Kainuma, R. Integrated mechanical and material design of quasi-zero-stiffness vibration isolator with superelastic Cu–Al–Mn shape memory alloy bars. J. Sound Vib. 2015, 358, 74–83. [Google Scholar] [CrossRef]
- Razavilar, R.; Fathi, A.; Dardel, M.; Hadi, J.A. Dynamic analysis of a shape memory alloy beam with pseudoelastic behavior. J. Intell. Mater. Syst. Struct. 2018, 29, 1835–1849. [Google Scholar] [CrossRef]
- Birman, V.; Rusnak, I. Vibrations of plates with superelastic shape memory alloy wires. J. Eng. Math. 2013, 78, 223–237. [Google Scholar] [CrossRef]
- Gilat, R.; Aboudi, J. Dynamic response of active composite plates: Shape memory alloy fibers in polymeric/metallic matrices. Int. J. Solids Struct. 2004, 41, 5717–5731. [Google Scholar] [CrossRef]
- Khalili, S.; Dehkordi, M.B.; Shariyat, M. Modeling and transient dynamic analysis of pseudoelastic SMA hybrid composite beam. Appl. Math. Comput. 2013, 219, 9762–9782. [Google Scholar] [CrossRef]
- Nekouei, M.; Raghebi, M.; Mohammadi, M. Free vibration analysis of hybrid laminated composite cylindrical shells reinforced with shape memory alloy fibers. J. Vib. Control 2020, 26, 610–626. [Google Scholar] [CrossRef]
- Tsai, X.-Y.; Chen, L.-W. Dynamic stability of a shape memory alloy wire reinforced composite beam. Compos. Struct. 2002, 56, 235–241. [Google Scholar] [CrossRef]
- Katsiropoulos, C.V.; Pappas, P.; Koutroumanis, N.; Kokkinos, A.; Galiotis, C. Improving the damping behavior of fiber-reinforced polymer composites with embedded superelastic shape memory alloys (SMA). Smart Mater. Struct. 2020, 29, 025006. [Google Scholar] [CrossRef]
- Alebrahim, R.; Haris, S.M.; Mohamed, N.A.N.; Abdullah, S. Vibration analysis of self-healing hybrid composite beam under moving mass. Compos. Struct. 2015, 119, 463–476. [Google Scholar] [CrossRef]
- Zhang, R.-X.; Ni, Q.-Q.; Masuda, A.; Yamamura, T.; Iwamoto, M. Vibration characteristics of laminated composite plates with embedded shape memory alloys. Compos. Struct. 2006, 74, 389–398. [Google Scholar] [CrossRef]
- Balapgol, B.S.; Bajoria, K.M.; Kulkarni, S.A. Natural frequencies of a multilayer SMA laminated composite cantilever plate. Smart Mater. Struct. 2006, 15, 1021. [Google Scholar] [CrossRef]
- Damanpack, A.; Bodaghi, M.; Aghdam, M.; Shakeri, M. On the vibration control capability of shape memory alloy composite beams. Compos. Struct. 2014, 110, 325–334. [Google Scholar] [CrossRef]
- Yongsheng, R.; Shuangshuang, S. Large amplitude flexural vibration of the orthotropic composite plate embedded with shape memory alloy fibers. Chin. J. Aeronaut. 2007, 20, 415–424. [Google Scholar] [CrossRef]
- Lau, K.-T.; Zhou, L.-M.; Tao, X.-M. Control of natural frequencies of a clamped–clamped composite beam with embedded shape memory alloy wires. Compos. Struct. 2002, 58, 39–47. [Google Scholar] [CrossRef]
- Srivastava, R.; Bhattacharya, B. Thermoelastic and vibration response analysis of shape memory alloy reinforced active bimorph composites. Smart Mater. Struct. 2020, 30, 015033. [Google Scholar] [CrossRef]
- Ni, Q.-Q.; Zhang, R.-X.; Natsuki, T.; Iwamoto, M. Stiffness and vibration characteristics of SMA/ER3 composites with shape memory alloy short fibers. Compos. Struct. 2007, 79, 501–507. [Google Scholar] [CrossRef]
- Zardian, M.G.; Moslemi, N.; Mozafari, F.; Gohery, S.; Yahya, M.Y.; Burvill, C.; Ayob, A.; Gohari, S. Flexural and free vibration control of smart epoxy composite beams using shape memory alloy wires actuator. J. Intell. Mater. Syst. Struct. 2020, 31, 1557–1566. [Google Scholar] [CrossRef]
- Chen, Q.; Levy, C. Active vibration control of elastic beam by means of shape memory alloy layers. Smart Mater. Struct. 1996, 5, 400. [Google Scholar] [CrossRef]
- Matsumori, H.; Deng, M.-C.; Noge, Y. An Operator-based nonlinear vibration control system using a flexible arm with shape memory alloy. Int. J. Autom. Comput. 2020, 17, 139–150. [Google Scholar] [CrossRef]
- Mohammadsalehi, M.; Zakerzadeh, M.R.; Baghani, M. Analysis of nonlinear free vibration of a beam with magnetic shape memory alloy elements. J. Intell. Mater. Syst. Struct. 2016, 27, 2216–2228. [Google Scholar] [CrossRef]
- Vishal, P.; Kaliperumal, D.; Padhi, R. Active vibration suppression of nonlinear cantilever beam using shape memory alloy actuators. IFAC-PapersOnLine 2018, 51, 130–135. [Google Scholar] [CrossRef]
- Ashrafi, M.J.; Ghaffari, I.; Elahinia, M.; Nematollahi, M.R. Nonlinear free vibration and damping analysis of a microbeam with pseudoelastic shape memory alloy layer based on the modified couple stress theory. J. Vib. Control 2020, 27, 957–968. [Google Scholar] [CrossRef]
- Kim, W.; Argento, A.; Mohanty, P.S. Damping characteristics of a spray-deposited shape memory alloy beam. J. Sound Vib. 2014, 333, 3356–3366. [Google Scholar] [CrossRef]
- De la Flor, S.; Urbina, C.; Ferrando, F. Asymmetrical bending model for NiTi shape memory wires: Numerical simulations and experimental analysis. Strain 2011, 47, 255–267. [Google Scholar] [CrossRef]
- Gall, K.; Sehitoglu, H.; Chumlyakov, Y.; Kireeva, I. Tension–compression asymmetry of the stress–strain response in aged single crystal and polycrystalline NiTi. Acta Mater. 1999, 47, 1203–1217. [Google Scholar] [CrossRef]


| ASYM Model | SYM Model | |
|---|---|---|
| Modulus [GPa] | ||
| Stresses [MPa] | ||
| Slopes [MPa/°C] | ||
| Strains [-] | ||
| Temperatures [°C] | ||
| ASYM Model | SYM Model | |
|---|---|---|
| Modulus [GPa] | ||
| Stresses [MPa] | ||
| Slopes [MPa/°C] | ||
| Strains [-] | ||
| Temperatures [°C] | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Samadi-Aghdam, K.; Fahimi, P.; Shahsavari, H.; Rahmatabadi, D.; Baghani, M. Vibration Analysis of Shape Memory Alloy Enhanced Multi-Layered Composite Beams with Asymmetric Material Behavior. Materials 2025, 18, 1181. https://doi.org/10.3390/ma18051181
Samadi-Aghdam K, Fahimi P, Shahsavari H, Rahmatabadi D, Baghani M. Vibration Analysis of Shape Memory Alloy Enhanced Multi-Layered Composite Beams with Asymmetric Material Behavior. Materials. 2025; 18(5):1181. https://doi.org/10.3390/ma18051181
Chicago/Turabian StyleSamadi-Aghdam, Kosar, Pouya Fahimi, Hamid Shahsavari, Davood Rahmatabadi, and Mostafa Baghani. 2025. "Vibration Analysis of Shape Memory Alloy Enhanced Multi-Layered Composite Beams with Asymmetric Material Behavior" Materials 18, no. 5: 1181. https://doi.org/10.3390/ma18051181
APA StyleSamadi-Aghdam, K., Fahimi, P., Shahsavari, H., Rahmatabadi, D., & Baghani, M. (2025). Vibration Analysis of Shape Memory Alloy Enhanced Multi-Layered Composite Beams with Asymmetric Material Behavior. Materials, 18(5), 1181. https://doi.org/10.3390/ma18051181







