Deformation Control of Adjustable-Ring-Mode (ARM) Laser Welding for Aluminum Alloys
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
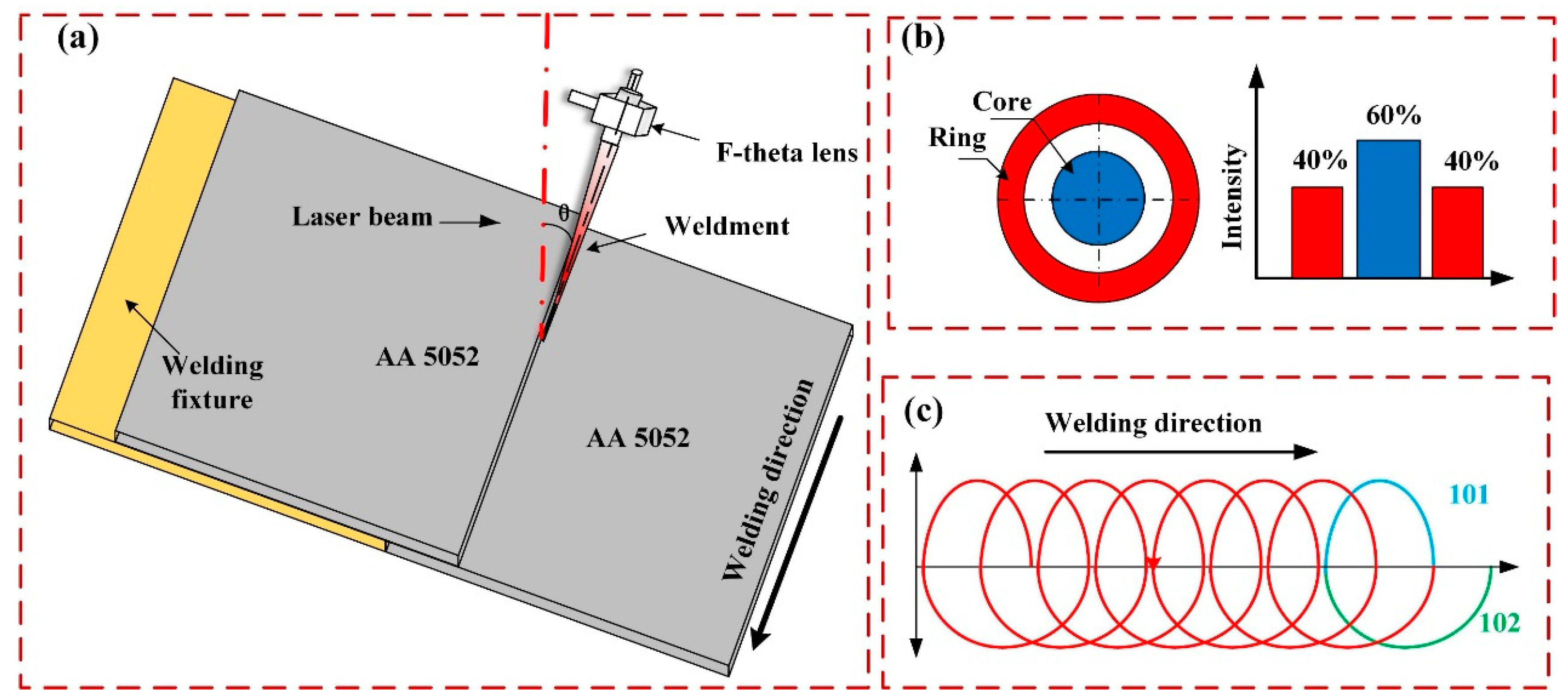
2.2. Experimental Procedure
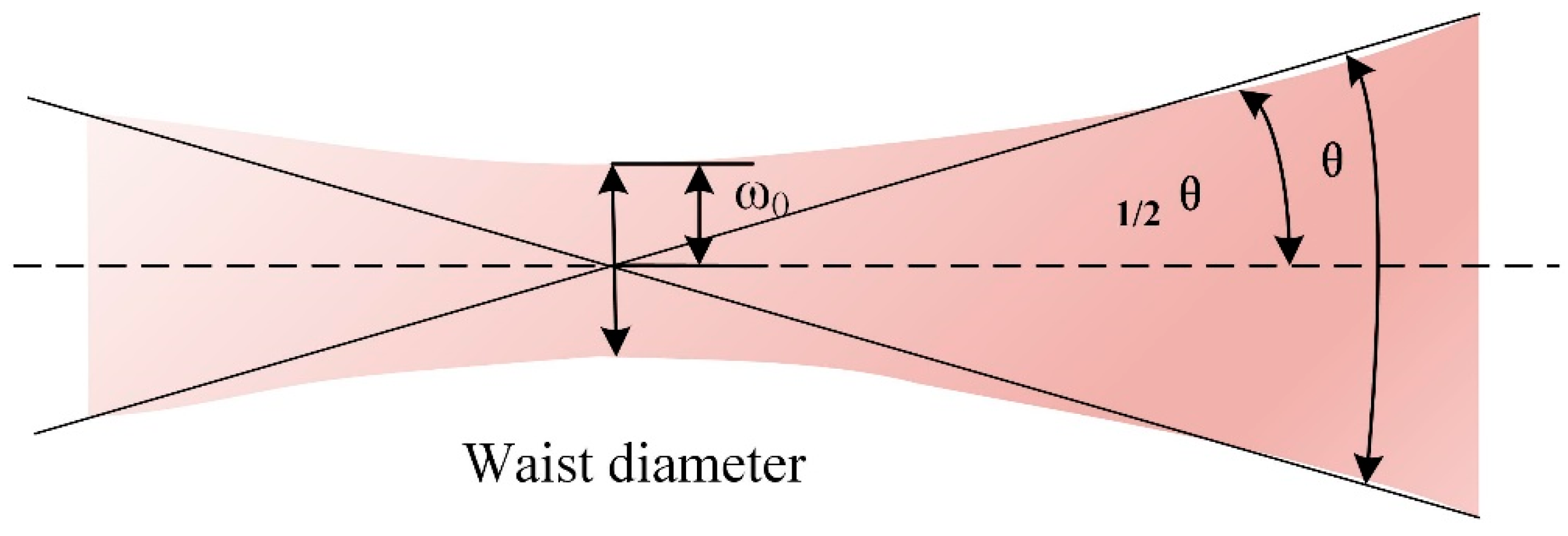
2.3. Analysis Methods
3. Results and Discussion
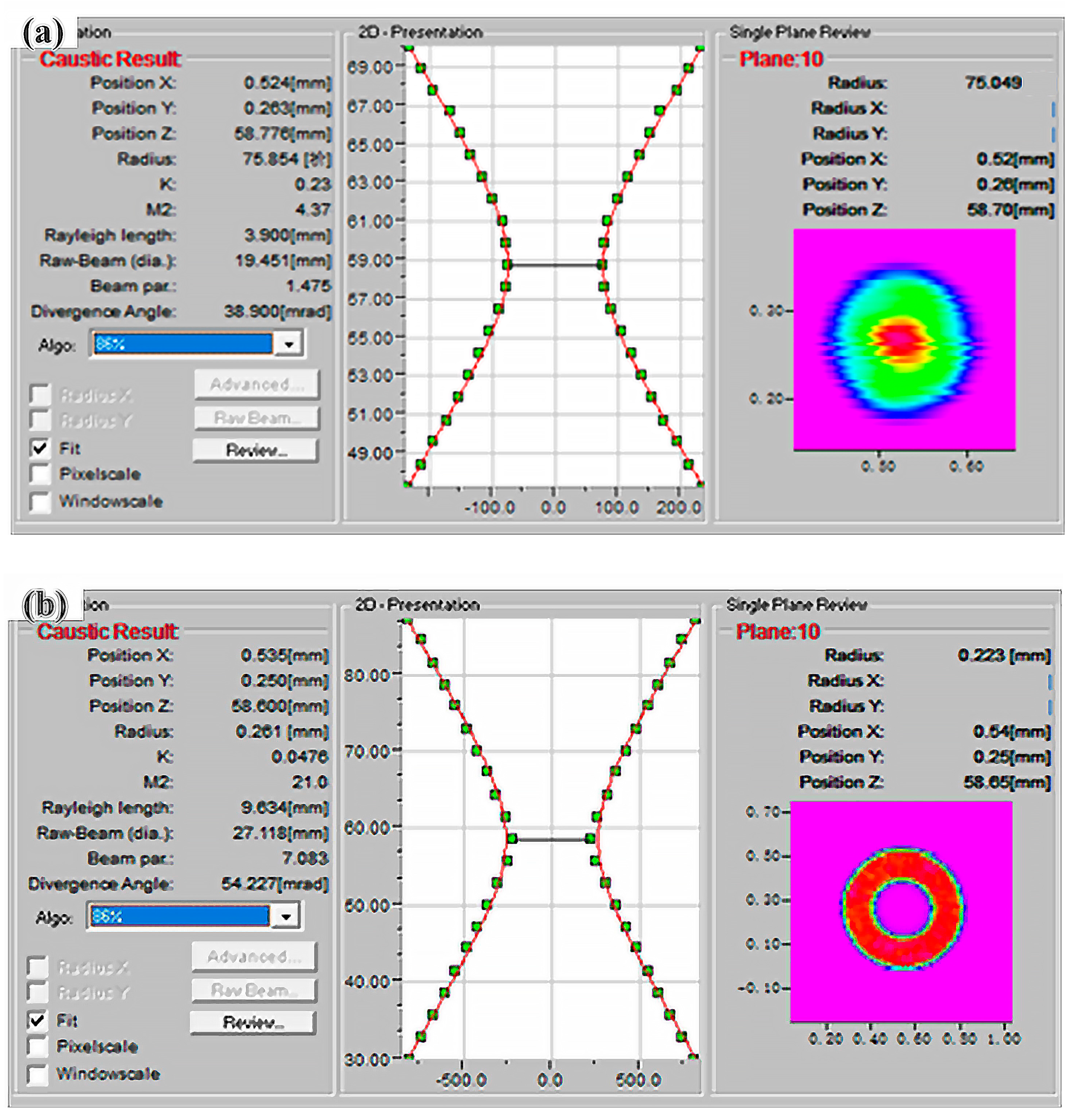
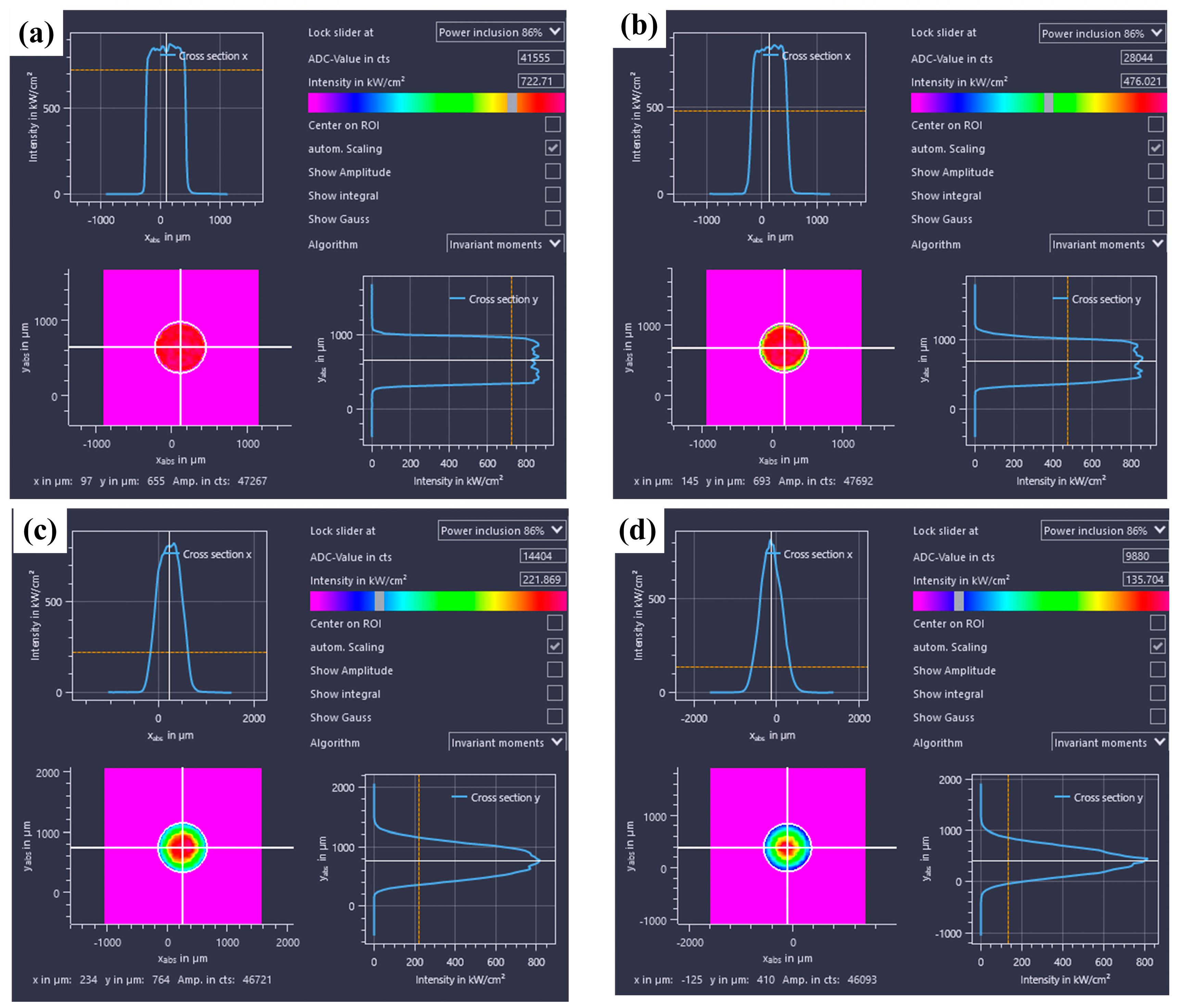
3.1. Effect of Optical Fiber Core Diameter
3.2. Effect of Light-Field Distribution
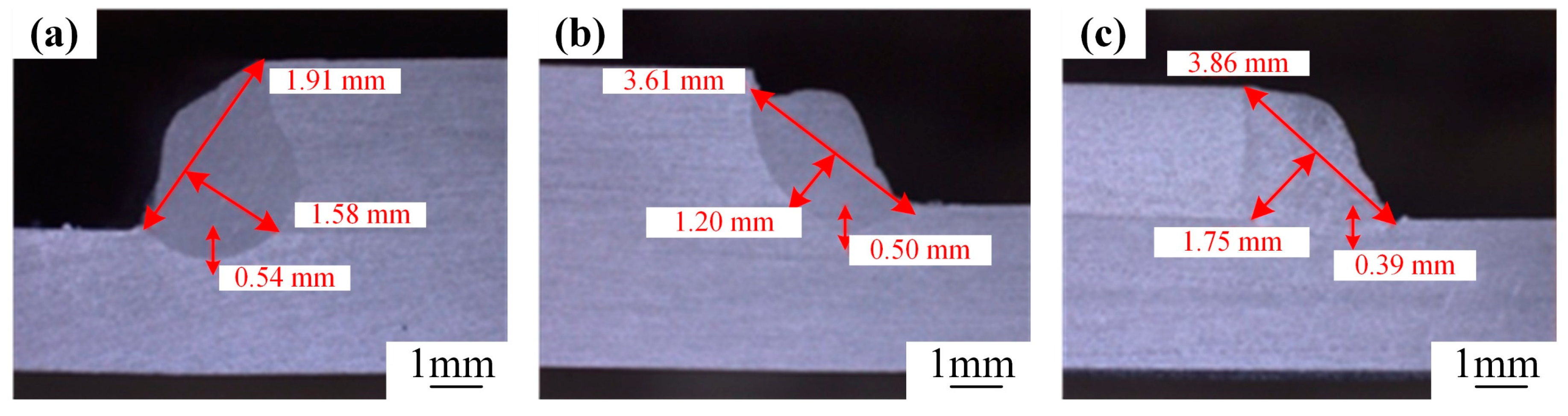
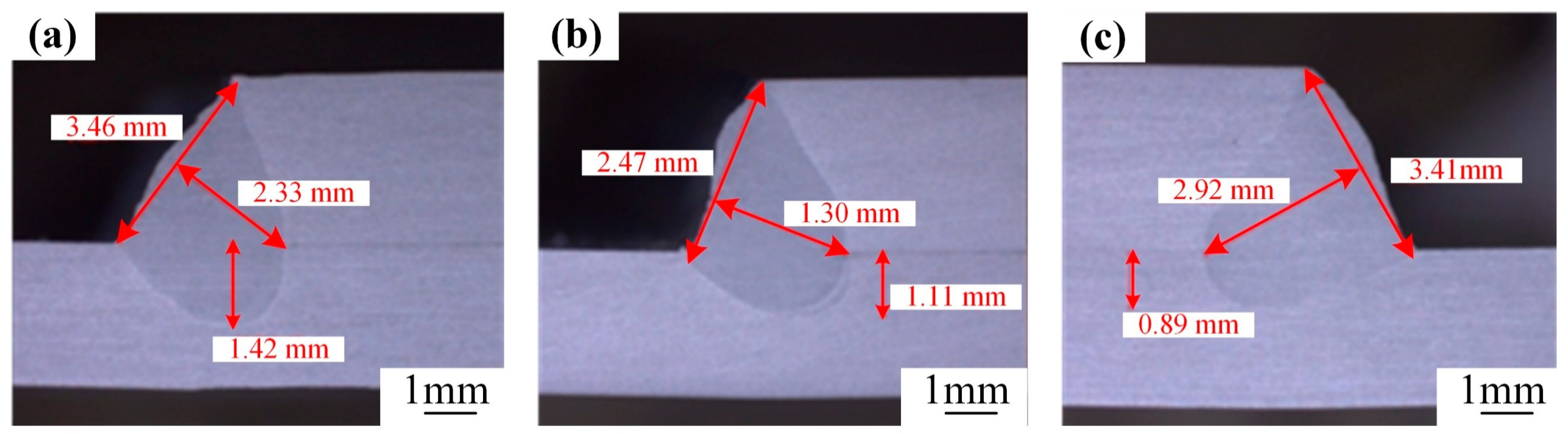
3.3. Effect of Welding Technology Parameters
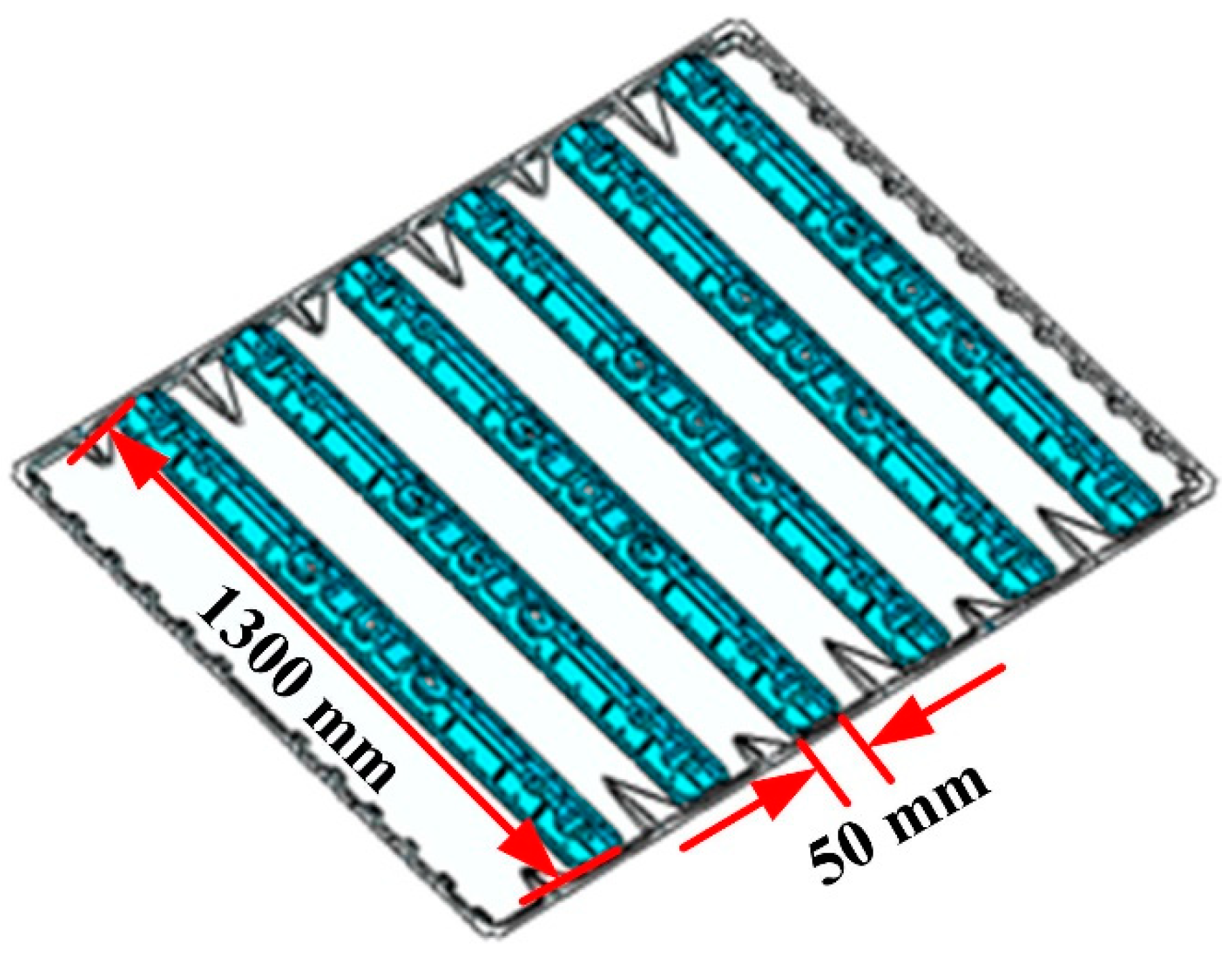
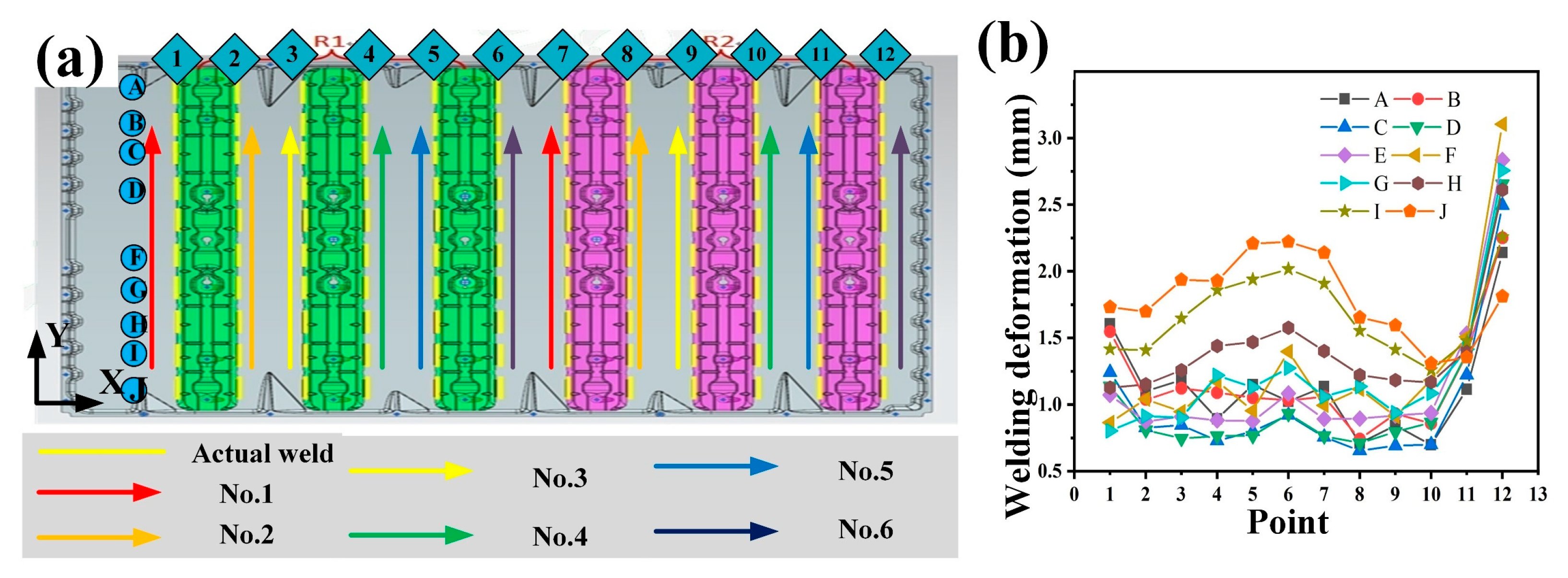
3.4. Effect of Welding Path
4. Conclusions
- (1)
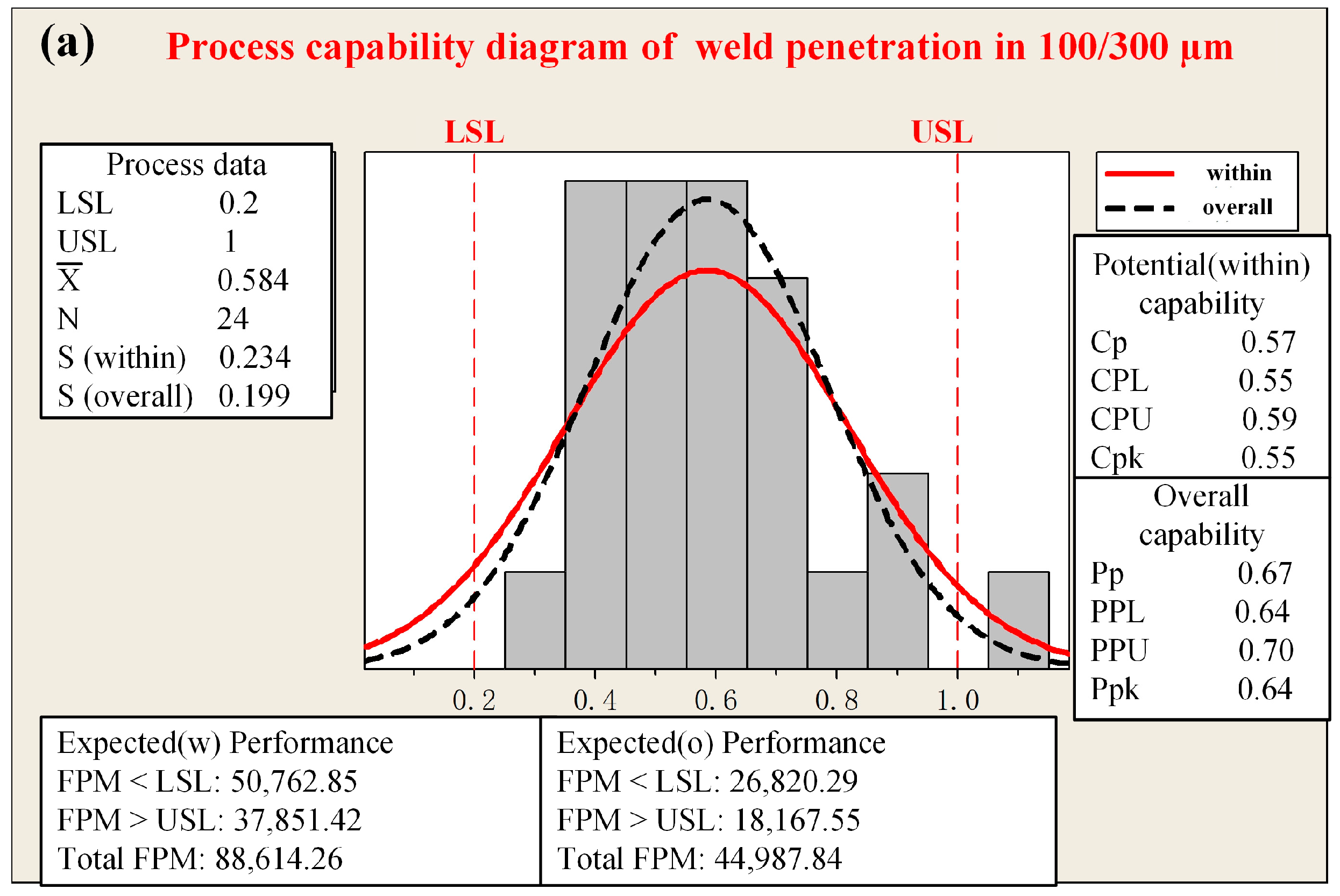
- Using the process capability diagram to evaluate the optical fiber core diameter’s impact, it was found that a 50/150 µm core diameter offers more stable welding. The Cpk and Ppk values for weld penetration and height are higher. The BPP values are 1.475 (core laser) and 21.0 (ring laser). When the light field luminance distribution is H, weld dimensional stability is maximized, ensuring optimal welding.
- (2)
- The effects of oscillation frequency, amplitude, tilt angles, and spot position on weld dimensions were studied. It was determined that at p = 5300 W, v = 5.4 m/min, A = 1.6 mm, f = 120 Hz, and θ = 40°, with the spot at the bottom of the upper substrate’s side, a lap joint with few defects and good weld formation can be obtained.
- (3)
- The influence of different welding paths on weld deformation was examined. After optimization, the maximum Z-axis weld deformation decreased to 1.403 mm, a reduction of 1.702 mm. During cross-symmetric welding, the second weld’s deformation offsets part of the first weld. The closer the symmetric welds are to the outer part, the smaller the post-welding deformation peak, enabling effective control of overall post-welding deformation.
- (4)
- In future research, this work can be combined with real-time monitoring and feedback control of the welding process. Further research on the compatibility of ARM laser welding with newly developed thin aluminum alloys should be carried out to ensure that the technology remains applicable and effective in the face of the ever-changing material demands in the industry. In this way, its strong industrial potential, demonstrated in various industries such as automotive, aerospace, electronics, shipbuilding, and railway, can be fully realized.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xu, J.; Rong, Y.; Huang, Y.; Wang, P.; Wang, C. Keyhole-induced porosity formation during laser welding. J. Mater. Process. Technol. 2018, 252, 720–727. [Google Scholar] [CrossRef]
- Jarwitz, M.; Fetzer, F.; Weber, R.; Graf, T. Weld Seam Geometry and Electrical Resistance of Laser-Welded, Aluminum-Copper Dissimilar Joints Produced with Spatial Beam Oscillation. Metals 2018, 8, 510. [Google Scholar] [CrossRef]
- Yan, S.; Li, Z.; Song, L.; Zhang, Y.; Wei, S. Research and development status of laser micro-welding of aluminum-copper dissimilar metals: A review. Opt. Lasers Eng. 2023, 161, 107312. [Google Scholar] [CrossRef]
- Kumar, N.; Masters, I.; Das, A. In-depth evaluation of laser-welded similar and dissimilar material tab-to-busbar electrical interconnects for electric vehicle battery pack. J. Manuf. Process. 2021, 70, 78–96. [Google Scholar] [CrossRef]
- Sadeghian, A.; Iqbal, N. A review on dissimilar laser welding of steel-copper, steel-aluminum, aluminum-copper, and steel-nickel for electric vehicle battery manufacturing. Opt. Laser Technol. 2022, 146, 107595. [Google Scholar] [CrossRef]
- Mayyas, A.; Omar, M.; Hayajneh, M.; Mayyas, A. Vehicle’s lightweight design vs. electrification from life cycle assessment perspective. J. Clean. Prod. 2017, 167, 687–701. [Google Scholar] [CrossRef]
- Sun, T.; Mohan, A.; Liu, C.; Franciosa, P.; Ceglarek, D. The impact of Adjustable-Ring-Mode (ARM) laser beam on the microstructure and mechanical performance in remote laser welding of high strength aluminium alloys. J. Mater. Res. Technol. 2022, 21, 2247–2261. [Google Scholar] [CrossRef]
- Ceglarek, D.; Colledani, M.; Váncza, J.; Kim, D.-Y.; Marine, C.; Kogel-Hollacher, M.; Mistry, A.; Bolognese, L. Rapid deployment of remote laser welding processes in automotive assembly systems. CIRP Ann. Manuf. Technol. 2015, 64, 389–394. [Google Scholar] [CrossRef]
- Bai, Y.; Yan, F.; Feng, T.; Han, W.; Zhang, L.; Cheng, D.; Bai, Z.; Wen, X. Temperature fiber sensor based on single longitudinal mode fiber laser in 2 μm band with Sagnac interferometer. Opt. Fiber Technol. 2019, 51, 71–76. [Google Scholar] [CrossRef]
- Park, H.M.; Oh, Y.J.; Park, E.J.; Park, J.S.; Jeong, H.; Kim, J.W. High-power ytterbium-doped fibre master-oscillator power-amplifier at 1018 nm. Laser Phys. 2022, 32, 025103. [Google Scholar] [CrossRef]
- Lim, L.T.; Abu, B.M.H.; Mahdi, M.A. Wavelength-tunable single longitudinal mode fiber optical parametric oscillator. Opt. Express 2017, 25, 5501–5508. [Google Scholar] [CrossRef] [PubMed]
- Raza, M.M.; Lo, Y.L.; Lee, H.B.; Chang, Y.-T. Computational modeling of laser welding for aluminumecopper joints using a circular strategy. J. Mater. Res. Technol. 2023, 25, 3350–3364. [Google Scholar] [CrossRef]
- Ma, B.; Gao, X.; Huang, Y.; Zhang, Y.; Huang, Y. Effect of different pulse shapes on the laser welding of aluminum and copper. Opt. Laser Technol. 2024, 171, 110312. [Google Scholar] [CrossRef]
- Duocastella, M.; Arnold, C.B. Bessel and annular beams for materials processing. Laser Photonics Rev. 2012, 6, 607–621. [Google Scholar] [CrossRef]
- Wang, L.; Mohammadpour, M.; Gao, X.; Lavoie, J.; Kleine, K.; Kong, F.; Kovacevic, R. Adjustable Ring Mode (ARM) laser welding of stainless steels. Opt. Lasers Eng. 2021, 137, 106360. [Google Scholar] [CrossRef]
- Xia, H.; Xu, Z.; Mi, G.; Wang, C. Microstructure and Properties of Multilayer 5052 Aluminum Alloy Sheet by Laser Welding. J. Mater. Eng. Perform. 2024, 1–12. [Google Scholar] [CrossRef]
- Pamarthi, V.V.; Sun, T.; Das, A.; Franciosa, P. Tailoring the weld microstructure to prevent solidification cracking in remote laser welding of AA6005 aluminium alloys using adjustable ringmode beam. J. Mater. Res. Technol. 2023, 25, 7154–7168. [Google Scholar] [CrossRef]
- Pamarthi, V.V.; Sun, T.; Das, A.; Franciosa, P. A novel methodology for investigating the through-thickness molten pool shape during remote laser beam welding. Opt. Laser Technol. 2024, 175, 110794. [Google Scholar] [CrossRef]
- Yang, L.; Geng, S.; Jiang, P.; Wang, Y.; Xiong, J. Investigation on the keyhole/molten pool dynamic behavior during adjustable ring-mode laser welding of medium-thick aluminum alloy. Int. J. Therm. Sci. 2024, 196, 108723. [Google Scholar] [CrossRef]
- Li, J.; Geng, S.; Wang, Y.; Wang, C.; Jiang, P. Mitigation of porosity in adjustable-ring-mode laser welding of medium-thick aluminum alloy. Int. J. Heat Mass Transf. 2024, 227, 125514. [Google Scholar] [CrossRef]
- Li, J.; Jiang, P.; Geng, S.; Xiong, J. Numerical and experimental study on keyhole dynamics and pore formation mechanisms during adjustable-ring-mode laser welding of medium-thick aluminum alloy. Int. J. Heat Mass Transf. 2023, 214, 124443. [Google Scholar] [CrossRef]
- Huang, S.; Lu, R.; Lou, M.; Lv, T.; Yao, J.; Li, Y. Effect of oscillation parameters on adjustable-ring mode (ARM) laser beam welding of aluminum alloys. J. Manuf. Process. 2024, 113, 307–318. [Google Scholar] [CrossRef]
- Li, S.; Shu, L.; Jiang, P.; Jiang, S.; Wu, W.; Gao, Y.; Wang, Y. A novel real-time aluminum-copper dissimilar metal ARM laser welding seam width at the interface monitoring method based on multi-diagnostic signals. Opt. Laser Technol. 2025, 181, 111972. [Google Scholar] [CrossRef]
- Guo, K.; Jin, H.; Wei, Y.; Wang, Q.; Chen, J. Experimental study on morphology, microstructure and mechanical properties of adjustable-ring-mode (ARM) laser welded Al-Mg alloy. CIRP J. Manuf. Sci. Technol. 2024, 53, 81–94. [Google Scholar] [CrossRef]
- Li, X.; Hu, L.; Deng, D. Influence of contact behavior on welding distortion and residual stress in a thin-plate butt-welded joint performed by partial-length welding. Thin-Walled Struct. 2022, 176, 109302. [Google Scholar] [CrossRef]
- Fahlström, K.; Andersson, O.; Melander, A.; Karlsson, L.; Svensson, L.E. Correlation between laser welding sequence and distortions for thin sheet structures. Sci. Technol. Weld. Join. 2017, 22, 150–156. [Google Scholar] [CrossRef]
- Geng, S.; Li, Y.; Jiang, P.; Wang, Y.; Jin, J.; Han, C. Influence of welding sequences on residual stress and deformation of U-rib components fabricated by laser-arc hybrid welding. J. Mater. Res. Technol. 2025, 35, 726–742. [Google Scholar] [CrossRef]
- Guo, Z.; Bai, R.; Lei, Z.; Jiang, H.; Zou, J.; Yan, C. Experimental and numerical investigation on ultimate strength of laser-welded stiffened plates considering welding deformation and residual stresses. Ocean Eng. 2021, 234, 109239. [Google Scholar] [CrossRef]
- Huang, Z.; Luo, Z.; Ao, S.; Cai, Y. Effect of Laser Welding Parameters on Weld Bowing Distortion of Thin Plates. High Temp. Mater. Process. 2018, 37, 299–311. [Google Scholar] [CrossRef]
- He, Y.; Zeng, Y.; Li, Z.; Guo, Z.; Fang, P.; Liu, Z.; Liu, Z.; Yang, Z.; Manladan, S.M. The effect of laser segmented skip welding on welding distortion and residual stress in butt weld of 6061 aluminum alloy thin plate. Int. J. Adv. Manuf. Technol. 2023, 124, 3293–3309. [Google Scholar] [CrossRef]
- Jang, H.; Ryu, H.; Kang, S. A Study on the Effects of Welding Deformation According to Weld Sequence in Overlay-Welded Structures. Metals 2024, 14, 684. [Google Scholar] [CrossRef]
- Jin, Q.; Jiang, W.; Wang, C.; Yang, Z.; Luo, Y.; Yan, G.; Tu, S.; He, Y. A rigid-flexible coordinated method to control weld residual stress and deformation during local PWHT for ultra-large pressure vessels. Int. J. Press. Vessel. Pip. 2021, 191, 104323. [Google Scholar] [CrossRef]
- Ganji, Z.A. Multivariate process incapability vector. Qual. Reliab. Eng. Int. 2019, 35, 902–919. [Google Scholar] [CrossRef]
- Lin, G.; Zhao, P.; Dong, Z.; Lin, X. Beam-shaping technique for fiber-coupled diode laser system by homogenizing the beam quality of two laser diode stacks. Opt. Laser Technol. 2020, 123, 105919. [Google Scholar] [CrossRef]
- Rana, H.; Badheka, V.; Patel, P.; Patel, V.; Li, W.; Andersson, J. Augmentation of weld penetration by flux assisted TIG welding and its distinct variants for oxygen free copper. J. Mater. Res. Technol. 2021, 10, 138–151. [Google Scholar] [CrossRef]









| Si | Mg | Fe | Mn | Cu | Al |
|---|---|---|---|---|---|
| 0.2~0.5 | 4.0~5.0 | ≤0.35 | 0.2~0.5 | ≤0.15 | Bal. |
| Material | Tensile Strength (MPa) | Yield Strength (MPa) | Elongation (%) | Elastic Modulus (GPa) | Brinell Hardness (HB) |
|---|---|---|---|---|---|
| AA5182 | 290~305 | 180~200 | 12~18 | 71 | 60~75 |
| Welding Parameters | Value |
|---|---|
| Laser power (p), kW | 3.0, 5.3 |
| Welding speed (v), m/min | 2.7, 5.4 |
| Oscillation amplitude (A), mm | 1.2, 1.6, 2.0 |
| Oscillation frequency (f), Hz | 120, 200, 250, 300 |
| Tilt angle (θ), degree | 20, 30, 40 |
| Spot position | Upper, middle, lower parts of the upper plate’s side |
| Shape | Type | Light-Field Distribution Standard |
|---|---|---|
 | H | The X-axis red energy distribution area and the green energy distribution area are 74%, and the Y-axis is 70%. |
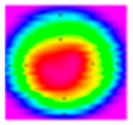 | HH | The X-axis red energy distribution area and the green energy distribution area are 61%, and the Y-axis is 61%. |
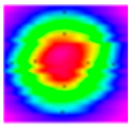 | HHH | The X-axis red energy distribution area and the green energy distribution area are 50%, and the Y-axis is 58%. |
 | HHHH | The X-axis red energy distribution area and the green energy distribution area are 21.3%, and the Y-axis is 34.5%. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, J.; Hu, M.; Su, J.; Guo, Q.; Wang, X.; Luo, Z. Deformation Control of Adjustable-Ring-Mode (ARM) Laser Welding for Aluminum Alloys. Materials 2025, 18, 860. https://doi.org/10.3390/ma18040860
Tang J, Hu M, Su J, Guo Q, Wang X, Luo Z. Deformation Control of Adjustable-Ring-Mode (ARM) Laser Welding for Aluminum Alloys. Materials. 2025; 18(4):860. https://doi.org/10.3390/ma18040860
Chicago/Turabian StyleTang, Jinglong, Minglie Hu, Jie Su, Qijun Guo, Xiaohua Wang, and Zhen Luo. 2025. "Deformation Control of Adjustable-Ring-Mode (ARM) Laser Welding for Aluminum Alloys" Materials 18, no. 4: 860. https://doi.org/10.3390/ma18040860
APA StyleTang, J., Hu, M., Su, J., Guo, Q., Wang, X., & Luo, Z. (2025). Deformation Control of Adjustable-Ring-Mode (ARM) Laser Welding for Aluminum Alloys. Materials, 18(4), 860. https://doi.org/10.3390/ma18040860






