Performance and Life Prediction of Recycled Concrete Against Sulfate Dry–Wet Cycle Corrosion
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Materials
2.2. Experimental Program
2.3. Testing Methods and Data Processing
2.3.1. Cube Compressive Strength
2.3.2. Dynamic Elastic Modulus
2.3.3. Mass Loss Rate
2.3.4. Data Processing
3. Results and Discussion
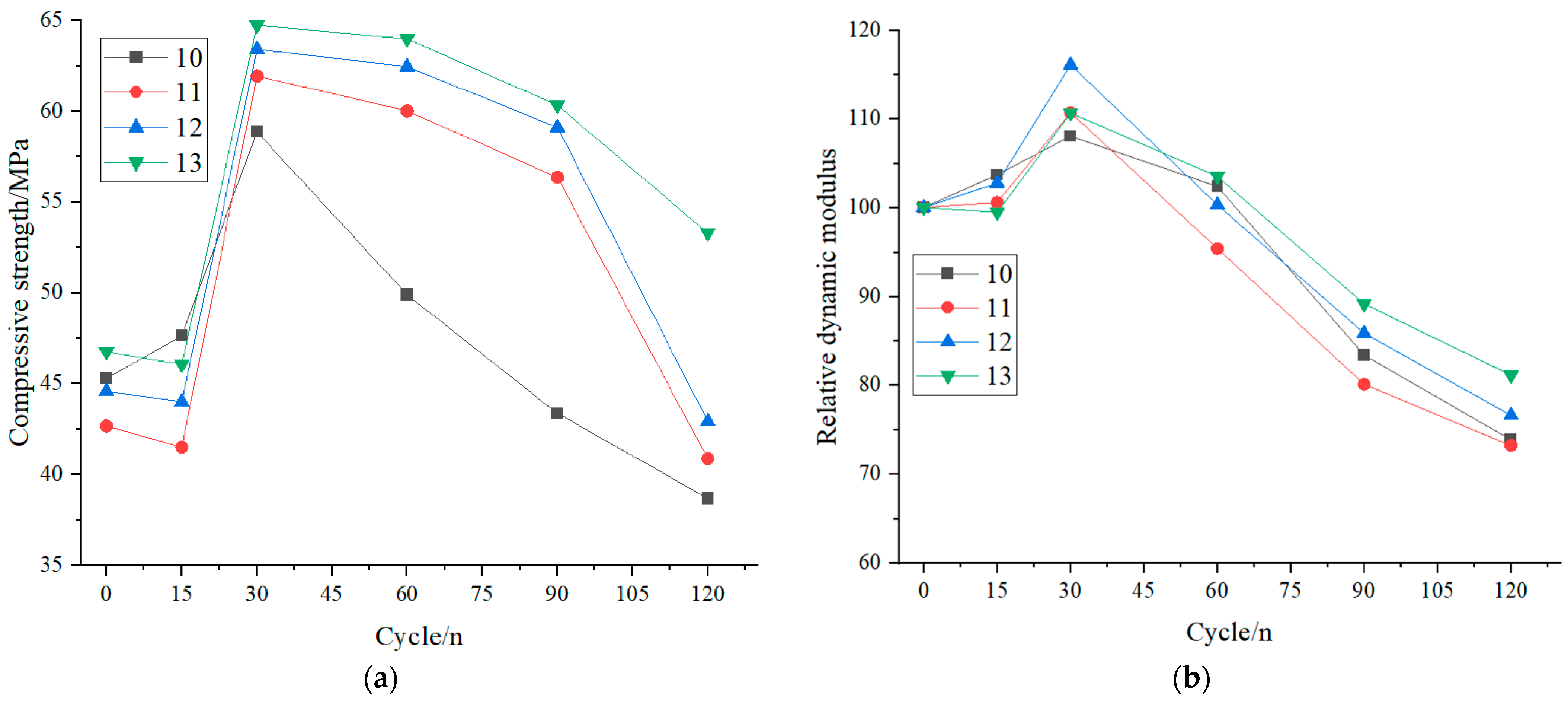
3.1. The Influence Law of Single and Compound Doping
3.2. Results Analysis of the Orthogonal Test
3.2.1. Extreme Range Analysis
3.2.2. Variance Analysis
3.2.3. Regression Analysis
3.2.4. Regression Model Verification
3.3. Determination of Influence Function
3.3.1. Determination of Influence Function of the Replacement Rate of Recycled Coarse Aggregate
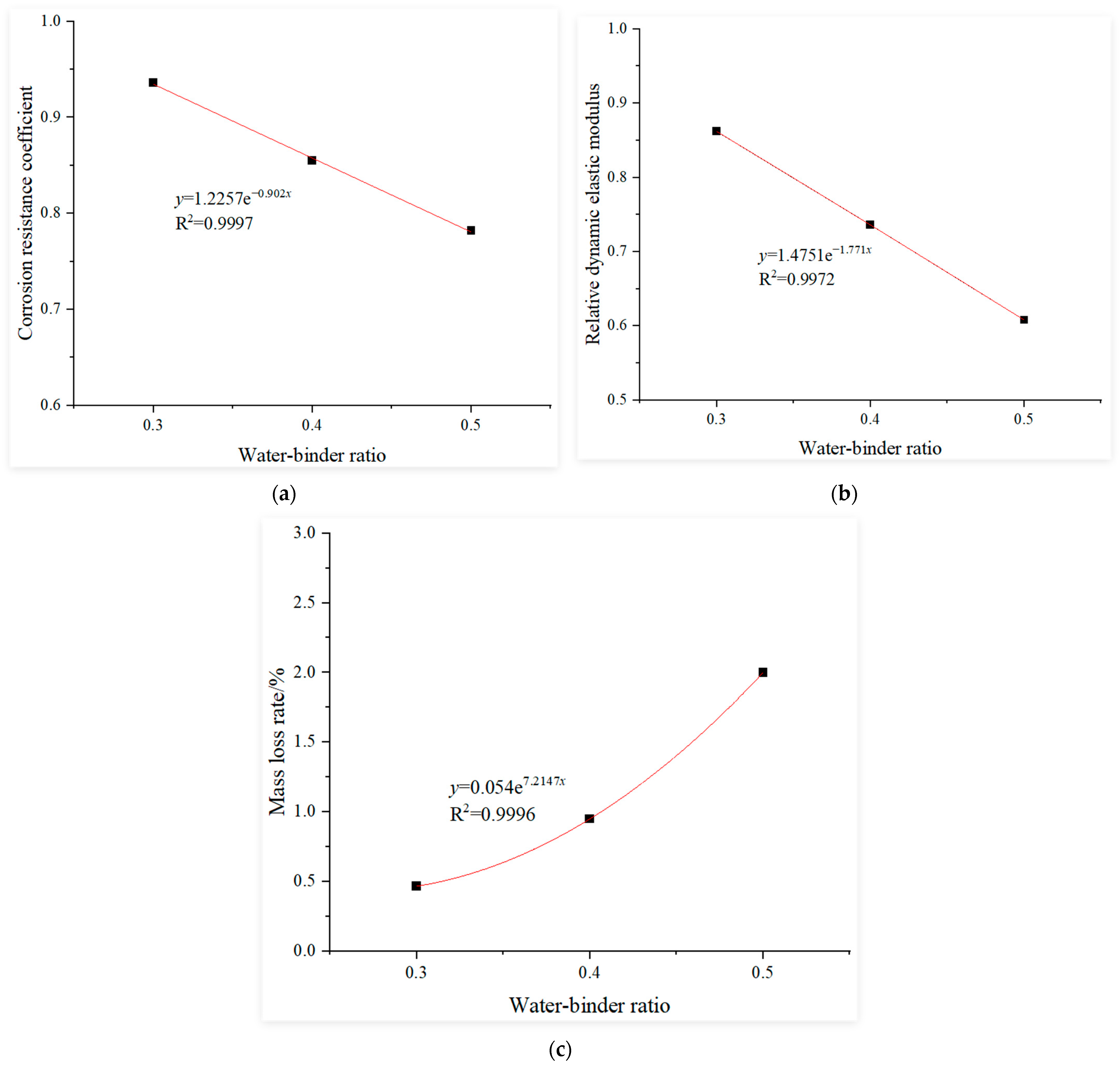
3.3.2. Determination of the Water–Binder Ratio Influence Function
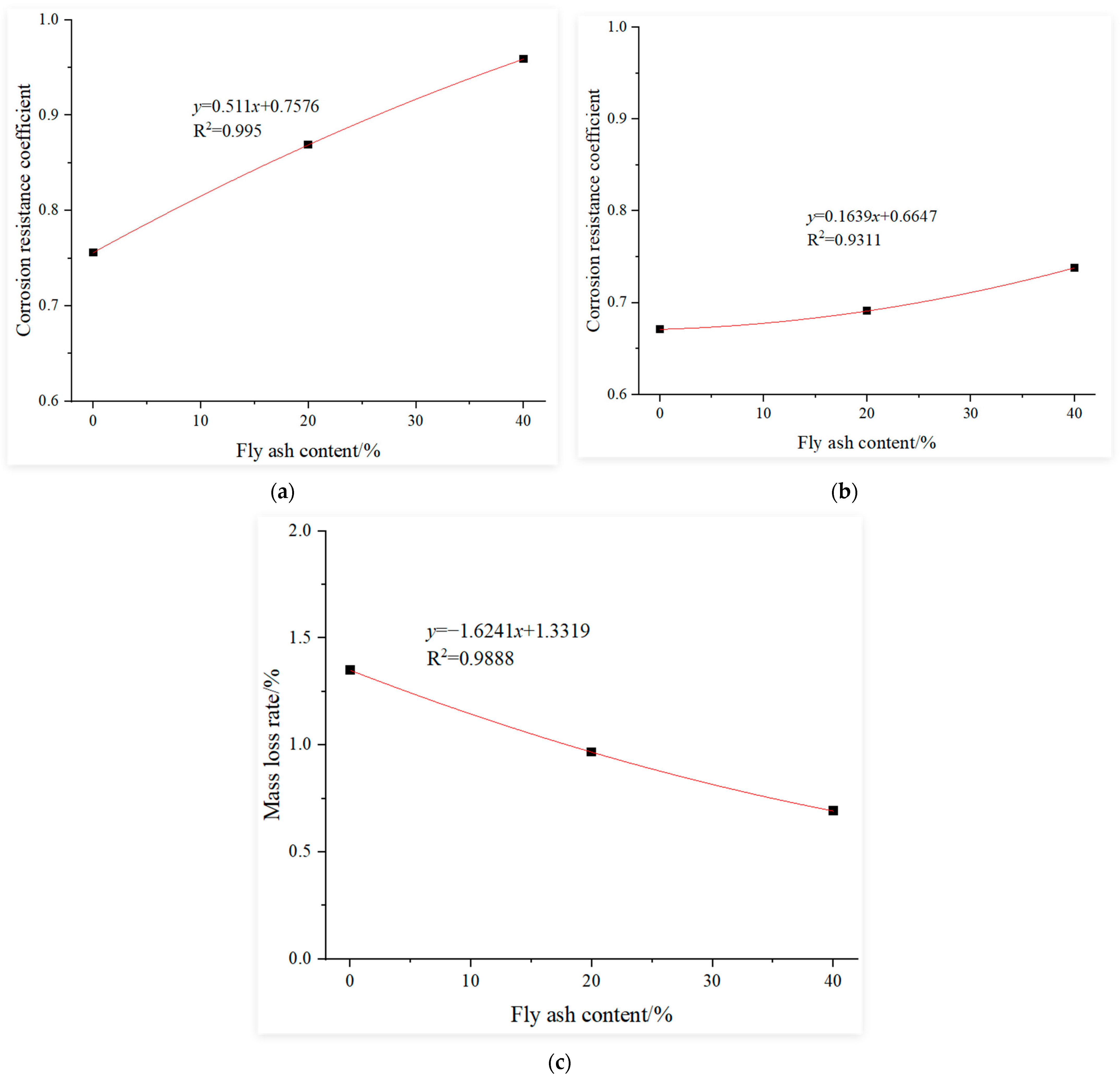
3.3.3. Determination of the Influence Function of Fly Ash Content
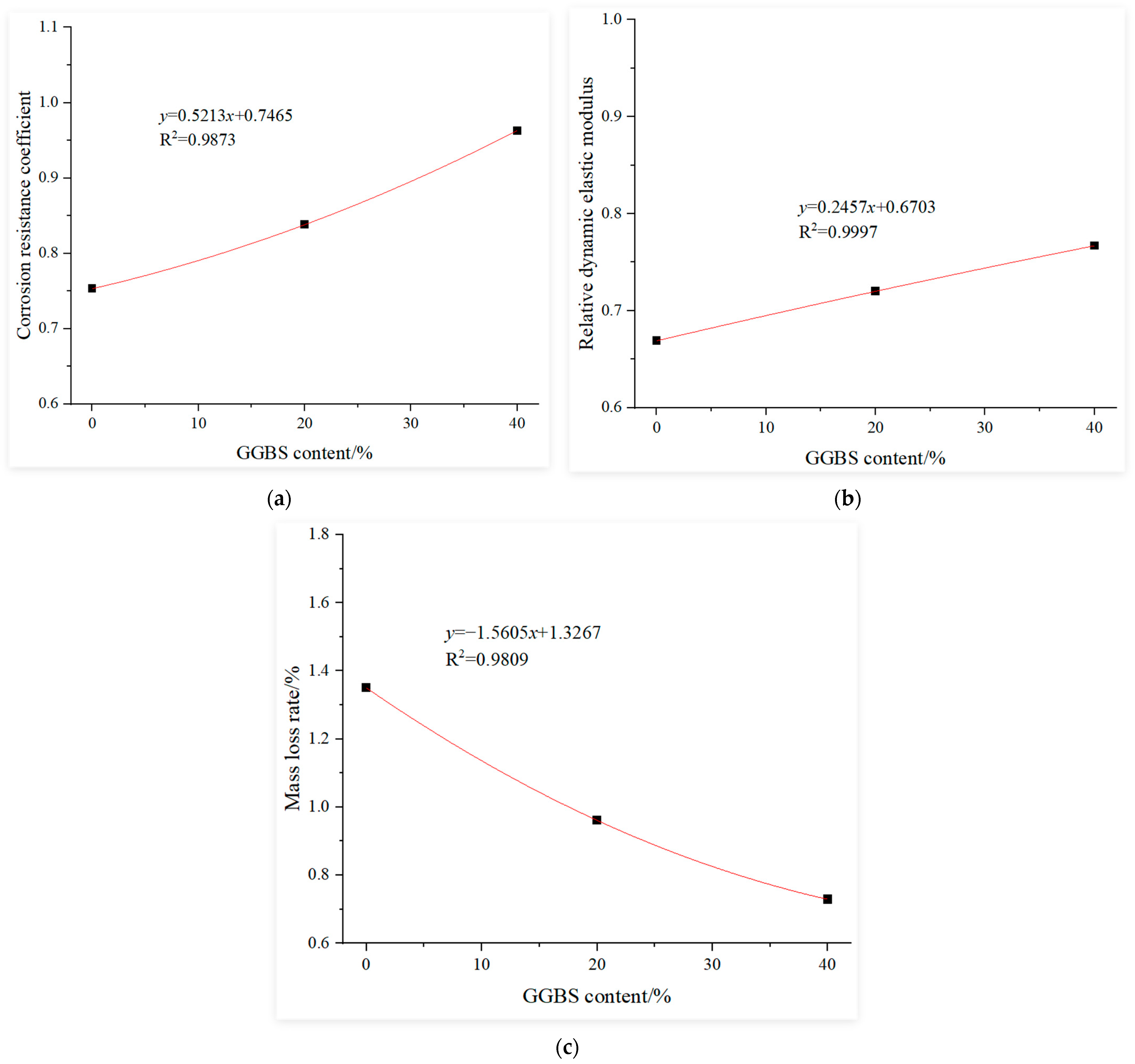
3.3.4. Determination of Influence Function of the GGBS Content
3.4. Establish Life Prediction Model Based on the Single-Factor Design
3.4.1. Proposal of the Service Life Prediction Model
3.4.2. Determination of Natural Decay Coefficient
3.5. Establishment of Life Prediction Equation Based on Three Indices
3.5.1. Life Prediction Model Establishment
3.5.2. Case Study
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- UNEP. Sand and Sustainability: Finding New Solutions for Environmental Governance of Global Sand Resources; United Nations Environment Programme (UNEP): Geneva, Switzerland, 2019. [Google Scholar]
- Huang, B.; Wang, X.; Kua, H.; Geng, Y.; Bleischwitz, R.; Ren, J.; Kim, J.; Lee, S. Deep neural network-based durability prediction for marine concrete structures. Cem. Concr. Res. 2021, 143, 106398. [Google Scholar]
- Wang, B.; Yan, L.; Fu, Q.; Kasal, B. A comprehensive review on recycled aggregate and recycled aggregate concrete. Resour. Conserv. Recycl. 2021, 171, 105565. [Google Scholar]
- Barbhuiya, S.; Das, B.B.; Adak, D. Roadmap to a net-zero carbon cement sector: Strategies, innovations and policy imperatives. J. Environ. Manag. 2024, 359, 121052. [Google Scholar] [CrossRef] [PubMed]
- Panghal, H.; Kumar, A. Enhancing concrete performance: Surface modification of recycled coarse aggregates for sustainable construction. Constr. Build. Mater. 2024, 411, 134432. [Google Scholar] [CrossRef]
- Théréné, F.; Keita, E.; Naël-Redolfi, J.; Boustingorry, P.; Bonafous, L.; Roussel, N. Water absorption of recycled aggregates: Measurements, influence of temperature and practical consequences. Cem. Concr. Res. 2020, 137, 106196. [Google Scholar] [CrossRef]
- Li, Y.; Fu, T.; Wang, R.; Li, Y. An assessment of microcracks in the interfacial transition zone of recycled concrete aggregates cured by CO2. Constr. Build. Mater. 2020, 236, 117543. [Google Scholar] [CrossRef]
- Jiang, T.; Cao, X.; Duan, H.; Shu, K. Recycling efficiency mechanism of recycled concrete aggregate to improve the CO2 uptake and anti-leakage properties of CO2 functional backfills. Constr. Build. Mater. 2025, 458, 139663. [Google Scholar] [CrossRef]
- Xiao, J.; Li, W.; Sun, Z.; Lange, D.A.; Shah, S.P. Properties of interfacial transition zones in recycled aggregate concrete tested by nanoindentation. Cem. Concr. Compos. 2013, 37, 276–292. [Google Scholar] [CrossRef]
- Lu, C.; Yu, Q.; Wei, J.; Niu, Y.; Zhang, Y.; Lin, C.; Chen, P.; Shi, C.; Yang, P. Influence of interface transition zones (ITZ) and pore structure on the compressive strength of recycled aggregate concrete. Constr. Build. Mater. 2024, 456, 139299. [Google Scholar] [CrossRef]
- ACI Committee 201. Guide to Durable Concrete; American Concrete Institute: Farmington Hills, MI, USA, 2016. [Google Scholar]
- Yu, X.; Hu, T.; Khodadadi, N.; Liu, Q.; Nanni, A. Modeling chloride ion diffusion in recycled aggregate concrete: A fuzzy neural network approach integrating material and environmental factors. Structures 2025, 73, 108372. [Google Scholar] [CrossRef]
- Du, T.; Xiao, J.; Li, C.; Gan, Y.; Jiang, X. Experimental and numerical study on the chloride ions penetration in recycled aggregate concrete. Constr. Build. Mater. 2024, 451, 138702. [Google Scholar] [CrossRef]
- Li, W.; Xiao, J.; Sun, Z.; Kawashima, S.; Shah, S.P. Interfacial transition zones in recycled aggregate concrete with different mixing approaches. Constr. Build. Mater. 2012, 35, 1045–1055. [Google Scholar] [CrossRef]
- Jin, M.; Ma, Y.; Li, W.; Huang, J.; Zeng, H.; Lu, C.; Zhang, J.; Liu, J. Degradation of C–S–H(I) at different decalcification degrees. J. Mater. Sci. 2022, 57, 19260–19279. [Google Scholar] [CrossRef]
- Wei, H.; Wan, H.; Yuan, S.; Liu, G.; Teng, J.; Shi, N.; Liu, Z. A new gelling material: Properties of recycled aggregate concrete under conditions of complete cement replacement using steel slag, ore slag, and fly ash. Constr. Build. Mater. 2025, 464, 140180. [Google Scholar] [CrossRef]
- Li, Y.; Long, J.; Chen, X. Property enhancement of recycled coarse aggregate and its concrete under CO2-accelerated curing treatment. Materials 2024, 17, 4194. [Google Scholar] [CrossRef]
- Poupelloz, E.; Gauffinet, S.; Nonat, A. Study of nucleation and growth processes of ettringite in diluted conditions. Cem. Concr. Res. 2020, 127, 105915. [Google Scholar] [CrossRef]
- Souza, M.T.; Onghero, L.; Sakata, R.D.; Neto, F.C.; de Campos, C.E.M.; de Castro Pessôa, J.R.; Repette, W.L.; de Oliveira, A.P.N. Insights into the acting mechanism of ettringite in expansive Portland cement. Mater. Lett. 2023, 345, 134496. [Google Scholar] [CrossRef]
- Huang, L.; An, M.; Wang, Y.; Xie, Y.; Han, S.; Yu, Z. Effect mechanisms of prolonged dry-hot exposure on C-S-H gel properties in cementitious materials. Constr. Build. Mater. 2025, 462, 140013. [Google Scholar] [CrossRef]
- Liao, W.; Lu, C.; Xu, Z.; Xu, Z. The deterioration of C-S-H gel in a severe sulfate environment. Symmetry 2024, 16, 703. [Google Scholar] [CrossRef]
- Yang, J.; Du, Q.; Bao, Y. Concrete with recycled concrete aggregate and crushed clay bricks. Constr. Build. Mater. 2011, 25, 1935–1945. [Google Scholar] [CrossRef]
- Lotfi, S.; Deja, J.; Rem, P.; Mróz, R.; van Roekel, E.; van der Stelt, H. Mechanical recycling of EOL concrete into high-grade aggregates. Resour. Conserv. Recycl. 2014, 87, 117–125. [Google Scholar] [CrossRef]
- Grabiec, A.M.; Klama, J.; Zawal, D.; Krupa, D. Modification of recycled concrete aggregate by calcium carbonate biodeposition. Constr. Build. Mater. 2012, 34, 145–150. [Google Scholar] [CrossRef]
- Wang, Y. Numerical prediction for life of damaged concrete under the action of fatigue loads. Eng. Fail. Anal. 2024, 162, 108368. [Google Scholar] [CrossRef]
- Cao, H.; Xu, Z.; Peng, X. An improved fick model for predicting carbonation depth of concrete. Coatings 2024, 14, 1345. [Google Scholar] [CrossRef]
- Li, J.; Ren, X. Stochastic damage model for concrete based on energy equivalent strain. Int. J. Solids Struct. 2009, 46, 2407–2419. [Google Scholar] [CrossRef]
- Nguyen, H.; Vu, T.; Vo, T.P.; Thai, H.-T. Efficient machine learning models for prediction of concrete strengths. Constr. Build. Mater. 2021, 266, 120950. [Google Scholar] [CrossRef]
- GB/T50082-2009; Standard for Test Methods of Long-term Performance and Durability of Ordinary Concrete. National Standard of the People’s Republic of China: Beijing, China, 2009.
- Zhang, H.; Ji, T.; Liu, H. Performance evolution of recycled aggregate concrete (RAC) exposed to external sulfate attacks under full-soaking and dry-wet cycling conditions. Constr. Build. Mater. 2020, 248, 118675. [Google Scholar] [CrossRef]
- Du, T.; Chen, J.; Qu, F.; Li, C.; Zhao, H.; Xie, B.; Yuan, M.; Li, W. Degradation prediction of recycled aggregate concrete under sulphate wetting–drying cycles using BP neural network. Structures 2022, 46, 1837–1850. [Google Scholar] [CrossRef]
- Santhanam, M.; Cohen, M.D.; Olek, J. Sulfate attack research whither now. Cem. Concr. Res. 2001, 2, 1072–1075. [Google Scholar] [CrossRef]
- Liu, D.; Chen, H.; Tang, Y.; Gong, C.; Jian, Y.; Cao, K. Analysis and prediction of sulfate erosion damage of concrete in service tunnel based on ARIMA model. Materials 2021, 14, 5904. [Google Scholar] [CrossRef]
- Feng, T.; Miao, Y.; Tan, Y.; Yang, Z.; Cao, T.; Wang, F.; Jiang, J. Prediction methodology for the service life of concrete structures in marine environment: From materials to performance. Engineering 2025, in press. [Google Scholar] [CrossRef]
- Alexander, M.; Beushausen, H. Durability, service life prediction, and modelling for reinforced concrete structures—Review and critique. Cem. Concr. Res. 2019, 122, 17–29. [Google Scholar] [CrossRef]


| Name | SiO2 | Al2O3 | Fe2O3 | CaO | MgO | SO3 |
|---|---|---|---|---|---|---|
| Fly ash/wt.% | 56.17 | 20.96 | 6.05 | 5.86 | 2.49 | 1.13 |
| GGBS/wt.% | 35.43 | 16.77 | 0.84 | 35.59 | 1.95 | 0.20 |
| Category | No. | Replacement Rate of Recycled Aggregate (A)/% | Water–Binder Ratio (B) | Content of GGBS + Fly Ash (C)/% | The Amount of Component Materials in Concrete/(kg m−3) | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Cement | Fly Ash | GGBS | Water | Sand | RCA | Natural Stone | Water Reducing Agent | |||||
| Orthogonal test group | 1 | 1 (30) | 1 (0.3) | 1 (0 + 0) | 400 | 0 | 0 | 120 | 837 | 307 | 716 | 4.0 |
| 2 | 1 (30) | 2 (0.4) | 2 (10 + 10) | 240 | 80 | 80 | 160 | 764 | 307 | 716 | 2.8 | |
| 3 | 1 (30) | 3 (0.5) | 3 (20 + 20) | 80 | 160 | 160 | 200 | 712 | 307 | 716 | 2.0 | |
| 4 | 2 (50) | 1 (0.3) | 2 (10 + 10) | 160 | 80 | 160 | 120 | 837 | 512 | 512 | 4.0 | |
| 5 | 2 (50) | 2 (0.4) | 3 (20 + 20) | 240 | 160 | 0 | 160 | 764 | 512 | 512 | 2.8 | |
| 6 | 2 (50) | 3 (0.5) | 1 (0 + 0) | 320 | 0 | 80 | 200 | 712 | 512 | 512 | 2.0 | |
| 7 | 3 (70) | 1 (0.3) | 3 (20 + 20) | 160 | 160 | 80 | 120 | 837 | 716 | 307 | 4.0 | |
| 8 | 3 (70) | 2 (0.4) | 1 (0 + 0) | 240 | 0 | 160 | 160 | 764 | 716 | 307 | 2.8 | |
| 9 | 3 (70) | 3 (0.5) | 2 (10 + 10) | 320 | 80 | 0 | 200 | 712 | 716 | 307 | 2.0 | |
| Single and compound doping test group | 10 | 30 | 0.4 | (0 + 0) | 400 | 0 | 0 | 400 | 160 | 307 | 716 | 2.8 |
| 11 | 30 | 0.4 | (0 + 40) | 240 | 160 | 0 | 240 | 160 | 307 | 716 | 2.8 | |
| 12 | 30 | 0.4 | (40 + 0) | 240 | 0 | 160 | 240 | 160 | 307 | 716 | 2.8 | |
| 13 | 30 | 0.4 | (20 + 20) | 240 | 80 | 80 | 240 | 160 | 307 | 716 | 2.8 | |
| No. | Orthogonal Factor | 0 Cycles of Corrosion/MPa | 60 Cycles of Corrosion/MPa | 120 Cycles of Corrosion/MPa | |||
|---|---|---|---|---|---|---|---|
| A | B | C | D (Empty) | ||||
| 1 | 30% | 0.3 | 0% | 1 | 66.73 | 57.95 | 44.04 |
| 2 | 30% | 0.4 | 20% | 2 | 48.55 | 51.07 | 36.92 |
| 3 | 30% | 0.5 | 40% | 3 | 39.68 | 48.65 | 39.82 |
| 4 | 50% | 0.3 | 20% | 3 | 55.53 | 60.29 | 45.82 |
| 5 | 50% | 0.4 | 40% | 1 | 49.88 | 53.22 | 43.30 |
| 6 | 50% | 0.5 | 0% | 2 | 51.09 | 38.40 | 29.18 |
| 7 | 70% | 0.3 | 40% | 2 | 41.86 | 58.02 | 46.00 |
| 8 | 70% | 0.4 | 0% | 3 | 44.31 | 34.02 | 25.86 |
| 9 | 70% | 0.5 | 20% | 1 | 31.28 | 39.32 | 29.89 |
| k1 | 51.65 | 54.71 | 54.04 | 49.30 | |||
| k2 | 52.17 | 47.58 | 45.12 | 47.17 | |||
| k3 | 39.15 | 40.68 | 43.81 | 46.51 | |||
| R | 13.02 | 14.02 | 10.24 | 2.79 | |||
| k1 | 52.56 | 58.75 | 43.46 | 50.16 | |||
| k2 | 50.64 | 46.11 | 50.23 | 49.16 | |||
| k3 | 43.79 | 42.12 | 53.30 | 47.65 | |||
| R | 8.77 | 16.63 | 9.84 | 2.51 | |||
| k1 | 40.26 | 45.29 | 33.03 | 39.08 | |||
| k2 | 39.43 | 35.36 | 37.54 | 37.37 | |||
| k3 | 33.92 | 32.97 | 43.04 | 37.17 | |||
| R | 6.35 | 12.32 | 10.01 | 1.91 | |||
| No. | Orthogonal Factor | 0 Cycles of Corrosion/GPa | 60 Cycles of Corrosion/GPa | 120 Cycles of Corrosion/GPa | |||
|---|---|---|---|---|---|---|---|
| A | B | C | D (Empty) | ||||
| 1 | 30% | 0.3 | 0% | 1 | 48.73 | 40.72 | 33.34 |
| 2 | 30% | 0.4 | 20% | 2 | 46.43 | 38.75 | 31.76 |
| 3 | 30% | 0.5 | 40% | 3 | 41.67 | 39.62 | 32.45 |
| 4 | 50% | 0.3 | 20% | 3 | 47.58 | 39.73 | 32.55 |
| 5 | 50% | 0.4 | 40% | 1 | 45.63 | 39.02 | 34.07 |
| 6 | 50% | 0.5 | 0% | 2 | 42.02 | 34.03 | 25.90 |
| 7 | 70% | 0.3 | 40% | 2 | 46.70 | 40.88 | 34.03 |
| 8 | 70% | 0.4 | 0% | 3 | 45.33 | 34.01 | 27.21 |
| 9 | 70% | 0.5 | 20% | 1 | 39.01 | 34.11 | 25.39 |
| k1 | 45.61 | 47.67 | 45.36 | 44.46 | |||
| k2 | 45.08 | 45.80 | 44.34 | 45.05 | |||
| k3 | 43.68 | 40.90 | 44.67 | 44.86 | |||
| R | 1.93 | 6.77 | 1.02 | 0.59 | |||
| k1 | 39.69 | 40.44 | 36.25 | 37.95 | |||
| k2 | 37.59 | 37.26 | 37.53 | 37.89 | |||
| k3 | 36.33 | 35.92 | 39.84 | 37.78 | |||
| R | 3.36 | 4.52 | 3.58 | 0.16 | |||
| k1 | 32.52 | 33.30 | 28.81 | 30.93 | |||
| k2 | 30.84 | 31.01 | 29.90 | 30.56 | |||
| k3 | 28.88 | 27.91 | 33.52 | 30.74 | |||
| R | 3.64 | 5.39 | 4.70 | 0.37 | |||
| Indicator | Corrosion Age | Source of Variance | Sum of Squared Deviations | Degrees of Freedom | Mean Square | F-Value | Critical Value Fa | Significance |
|---|---|---|---|---|---|---|---|---|
| Cubic compressive strength | 0 cycle | Recycling aggregate replacement rate | 325.50 | 2 | 162.75 | 25.82 | F0.1(2.2) = 9 | *** |
| Water–binder ratio | 296.83 | 2 | 148.41 | 23.55 | F0.05(2.2) = 19 | *** | ||
| GGBS + fly ash content | 186.99 | 2 | 93.49 | 14.83 | F0.01(2.2) = 99 | ** | ||
| Error | 12.61 | 2 | 6.30 | |||||
| Total | 21,213.76 | 9 | ||||||
| 60 cycle | Recycling aggregate replacement rate | 127.46 | 2 | 63.73 | 13.32 | F0.1(2.2) = 9 | ** | |
| Water–binder ratio | 452.24 | 2 | 226.12 | 47.25 | F0.05(2.2) = 19 | *** | ||
| GGBS + fly ash content | 152.18 | 2 | 76.00 | 15.90 | F0.01(2.2) = 99 | ** | ||
| Error | 9.57 | 2 | 4.79 | |||||
| Total | 22,345.27 | 9 | ||||||
| 120 cycle | Recycling aggregate replacement rate | 71.42 | 2 | 35.71 | 10.81 | F0.1(2.2) = 9 | ** | |
| Water–binder ratio | 256.11 | 2 | 128.05 | 38.75 | F0.05(2.2) = 19 | *** | ||
| GGBS + fly ash content | 150.88 | 2 | 75.44 | 22.83 | F0.01(2.2) = 99 | *** | ||
| Error | 6.61 | 2 | 3.31 | |||||
| Total | 13,392.36 | 9 | ||||||
| Dynamic modulus of elasticity | 0 cycle | Recycling aggregate replacement rate | 5.96 | 2 | 2.98 | 10.82 | F0.1(2.2) = 9 | ** |
| Water–binder ratio | 73.32 | 2 | 36.66 | 133.11 | F0.05(2.2) = 19 | *** | ||
| GGBS + fly ash content | 1.63 | 2 | 0.81 | 2.96 | F0.01(2.2) = 99 | * | ||
| Error | 0.55 | 2 | 0.28 | |||||
| Total | 18,135.86 | 9 | ||||||
| 60 cycle | Recycling aggregate replacement rate | 17.29 | 2 | 8.65 | 41.69 | F0.1(2.2) = 9 | *** | |
| Water–binder ratio | 32.37 | 2 | 16.19 | 782.01 | F0.05(2.2) = 19 | **** | ||
| GGBS + fly ash content | 19.80 | 2 | 9.90 | 47.85 | F0.01(2.2) = 99 | *** | ||
| Error | 0.04 | 2 | 0.02 | |||||
| Total | 12,979.01 | 9 | ||||||
| 120 cycle | Recycling aggregate replacement rate | 19.90 | 2 | 9.95 | 96.52 | F0.1(2.2) = 9 | *** | |
| Water–binder ratio | 43.90 | 2 | 21.95 | 212.93 | F0.05(2.2) = 19 | **** | ||
| GGBS + fly ash content | 36.37 | 2 | 18.19 | 176.43 | F0.01(2.2) = 99 | **** | ||
| Error | 0.21 | 2 | 0.10 | |||||
| Total | 8606.59 | 9 |
| Indicator | Corrosion Cycles/n | Regression Model | Correlation Coefficient (R2) |
|---|---|---|---|
| Cubic compressive strength | 0 cycle | y = 96.49 − 31.25x1 − 70.33x2 − 25.67x3 | 0.84 |
| 60 cycles | y = 88.29 − 21.92x1 − 83.14x2 + 24.61x3 | 0.91 | |
| 120 cycles | y = 65.44 − 15.87x1 − 61.61x2 + 25.03x3 | 0.9 | |
| Dynamic modulus of elasticity | 0 cycle | y = 61.09 − 4.83x1 − 33.85x2 − 1.73x3 | 0.92 |
| 60 cycles | y = 49.32 − 8.40x1 − 22.61x2 + 8.961x3 | 0.96 | |
| 120 cycles | y = 43.72 − 9.1x1 − 26.94x2 + 11.75x3 | 0.96 |
| Corrosion Cycles | Replacement Ratio/% | Water-to-Binder Ratio | Fly Ash Content/% | Measured Compressive Strength/MPa | Measured Dynamic Elastic Modulus/GPa | Calculated Compressive Strength/MPa | Calculated Dynamic Elastic Modulus/GPa | Compressive Strength Error/% | Dynamic Elastic Modulus Error/% |
|---|---|---|---|---|---|---|---|---|---|
| 0 cycle | 30 | 0.3 | 0 | 66.73 | 48.73 | 66.02 | 49.49 | −1.07 | 1.55 |
| 30 | 0.4 | 20 | 48.55 | 46.43 | 53.85 | 45.76 | 10.91 | −1.45 | |
| 30 | 0.5 | 40 | 39.68 | 41.67 | 48.72 | 42.02 | 22.77 | 0.85 | |
| 50 | 0.3 | 20 | 55.53 | 47.58 | 54.63 | 48.17 | −1.62 | 1.25 | |
| 50 | 0.4 | 40 | 49.88 | 45.63 | 42.47 | 44.44 | −14.87 | −2.61 | |
| 50 | 0.5 | 0 | 51.09 | 42.02 | 45.7 | 41.75 | −10.55 | −0.64 | |
| 70 | 0.3 | 40 | 41.86 | 46.7 | 43.25 | 46.86 | 3.32 | 0.35 | |
| 70 | 0.4 | 0 | 44.31 | 45.33 | 46.48 | 44.17 | 4.90 | −2.56 | |
| 70 | 0.5 | 2 | 31.28 | 39.01 | 34.32 | 40.44 | 9.71 | 3.66 | |
| 60 cycles | 30 | 0.3 | 0 | 57.95 | 40.72 | 56.77 | 40.02 | −2.03 | −1.72 |
| 30 | 0.4 | 20 | 51.07 | 38.75 | 53.38 | 39.55 | 4.52 | 2.06 | |
| 30 | 0.5 | 40 | 48.65 | 39.62 | 49.99 | 39.08 | 2.74 | −1.35 | |
| 50 | 0.3 | 20 | 60.29 | 39.73 | 57.31 | 40.13 | −4.94 | 1.01 | |
| 50 | 0.4 | 40 | 53.22 | 39.02 | 53.92 | 39.66 | 1.31 | 1.65 | |
| 50 | 0.5 | 0 | 38.40 | 34.03 | 35.76 | 33.82 | −6.88 | −0.63 | |
| 70 | 0.3 | 40 | 58.02 | 40.88 | 57.85 | 40.24 | −0.30 | −1.56 | |
| 70 | 0.4 | 0 | 34.02 | 34.01 | 39.69 | 34.4 | 16.66 | 1.13 | |
| 70 | 0.5 | 20 | 39.32 | 34.11 | 36.3 | 33.92 | −7.69 | −0.57 | |
| 120 cycles | 30 | 0.3 | 0 | 44.04 | 33.34 | 42.2 | 32.91 | −4.19 | −1.28 |
| 30 | 0.4 | 20 | 36.92 | 31.76 | 41.04 | 32.56 | 11.17 | 2.54 | |
| 30 | 0.5 | 40 | 39.82 | 32.45 | 39.89 | 32.22 | 0.16 | −0.71 | |
| 50 | 0.3 | 20 | 45.82 | 32.55 | 44.03 | 33.44 | −3.91 | 2.74 | |
| 50 | 0.4 | 40 | 43.30 | 34.07 | 42.87 | 33.09 | −0.99 | −2.86 | |
| 50 | 0.5 | 0 | 29.18 | 25.90 | 26.70 | 25.7 | −8.51 | −0.76 | |
| 70 | 0.3 | 40 | 46.00 | 34.03 | 45.86 | 33.97 | −0.30 | −0.18 | |
| 70 | 0.4 | 0 | 25.86 | 27.21 | 29.69 | 26.57 | 14.80 | −2.33 | |
| 70 | 0.5 | 20 | 29.89 | 25.39 | 28.53 | 26.63 | −4.53 | 4.87 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, L.; Wu, F.; Liu, D.; Huang, C.; Pu, S.; Wang, J.; Luo, P. Performance and Life Prediction of Recycled Concrete Against Sulfate Dry–Wet Cycle Corrosion. Materials 2025, 18, 2201. https://doi.org/10.3390/ma18102201
Chen L, Wu F, Liu D, Huang C, Pu S, Wang J, Luo P. Performance and Life Prediction of Recycled Concrete Against Sulfate Dry–Wet Cycle Corrosion. Materials. 2025; 18(10):2201. https://doi.org/10.3390/ma18102201
Chicago/Turabian StyleChen, Liangliang, Fufei Wu, Daqing Liu, Chuanteng Huang, Shuang Pu, Jing Wang, and Pengfei Luo. 2025. "Performance and Life Prediction of Recycled Concrete Against Sulfate Dry–Wet Cycle Corrosion" Materials 18, no. 10: 2201. https://doi.org/10.3390/ma18102201
APA StyleChen, L., Wu, F., Liu, D., Huang, C., Pu, S., Wang, J., & Luo, P. (2025). Performance and Life Prediction of Recycled Concrete Against Sulfate Dry–Wet Cycle Corrosion. Materials, 18(10), 2201. https://doi.org/10.3390/ma18102201







