3D DEM Simulations and Experiments on Spherical Impactor Penetrating into the Elongated Particles
Abstract
1. Introduction
2. Numerical Model and Methodology
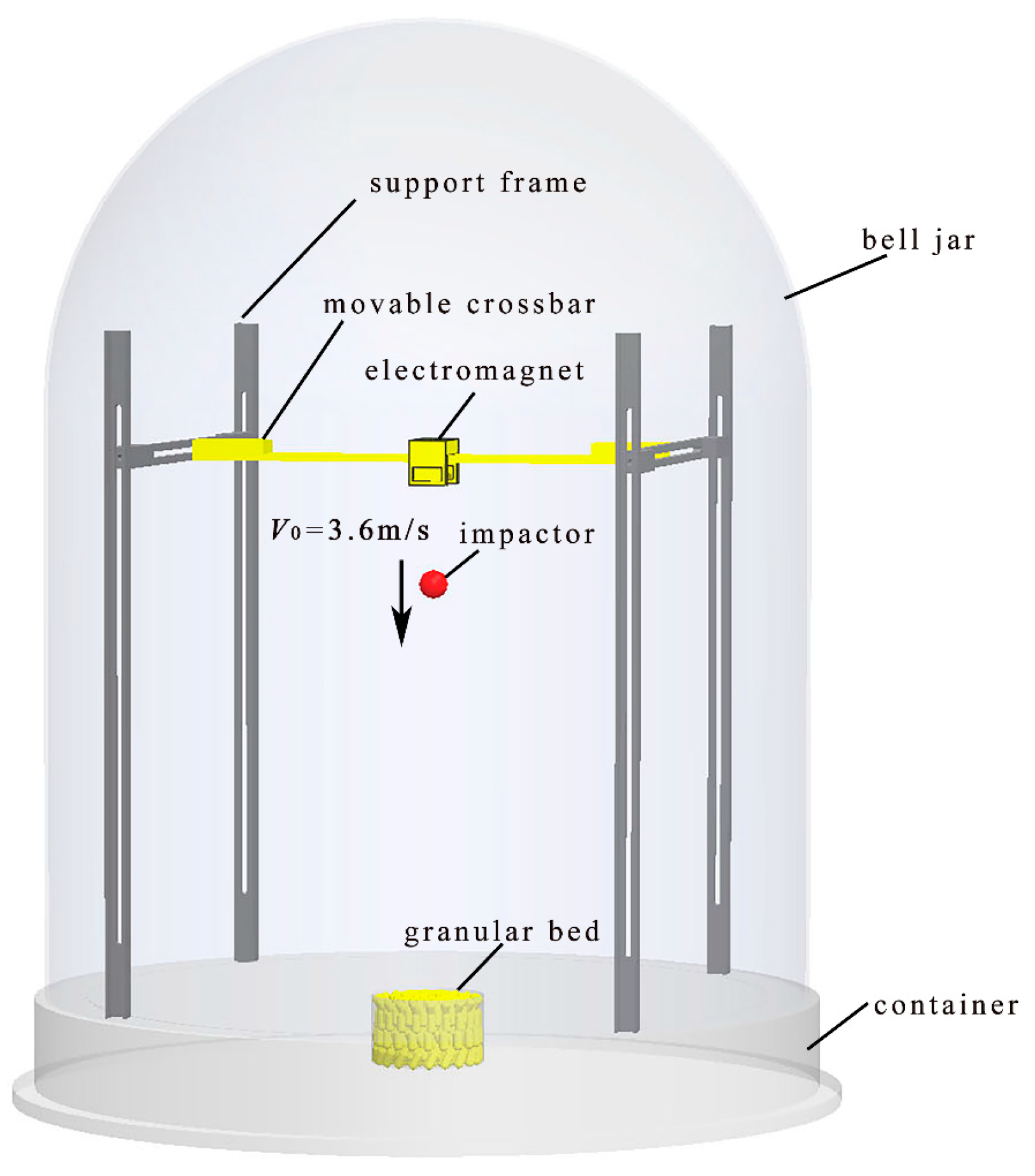
3. Experimental and Numerical Setup
4. Results and Discussion
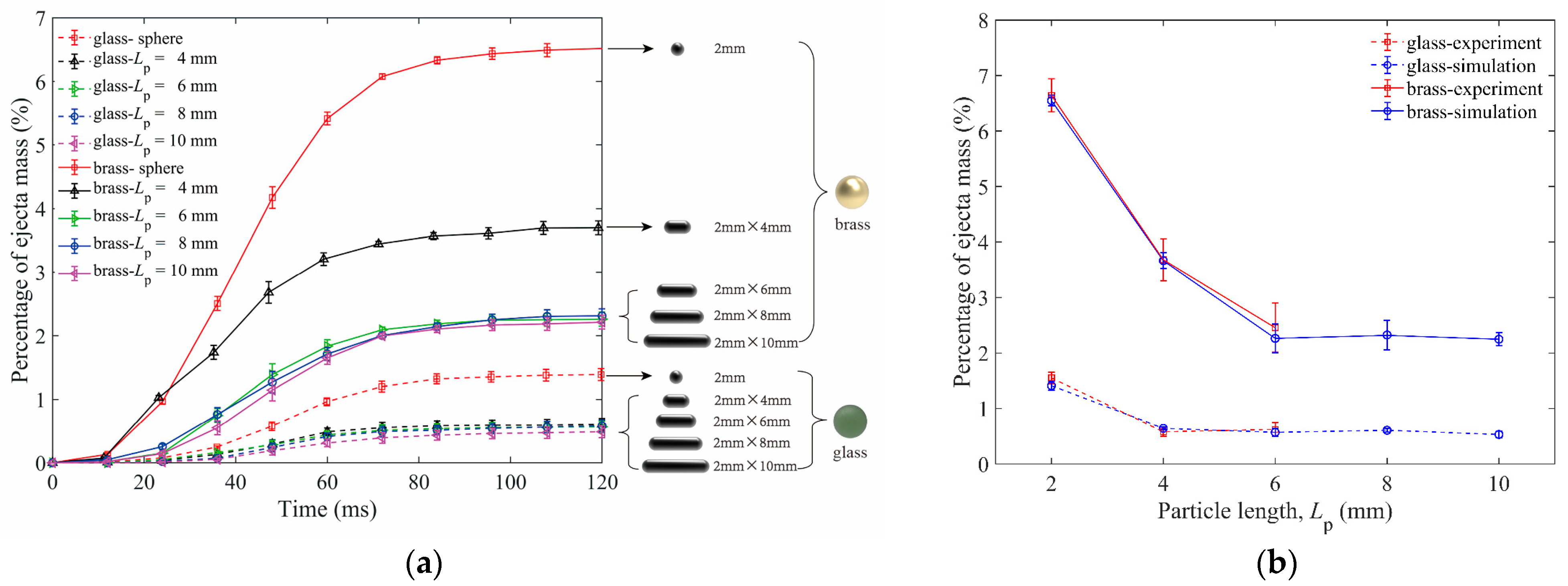
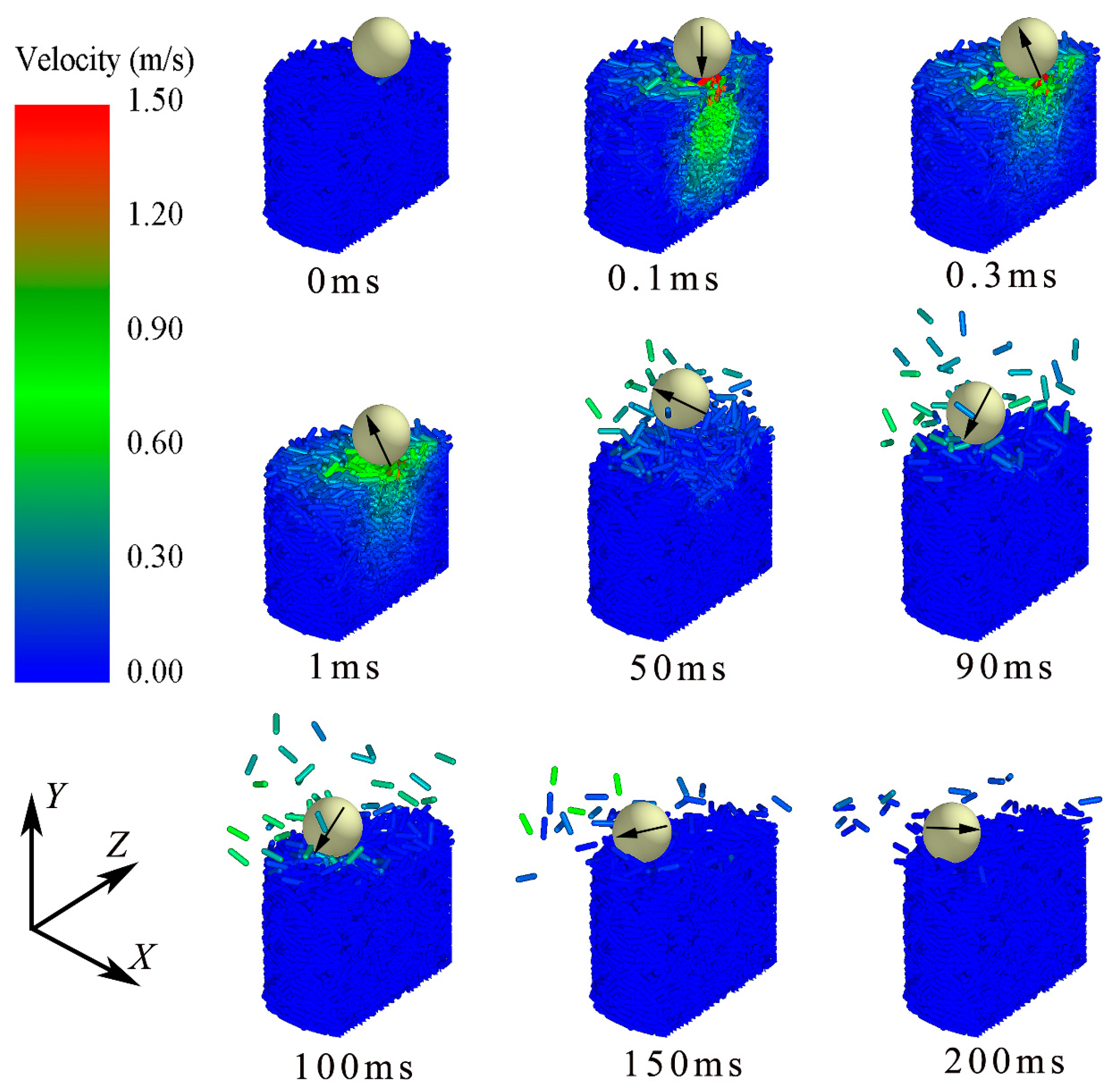
4.1. Effect of Particle Length
4.1.1. The Particles out of the Cup
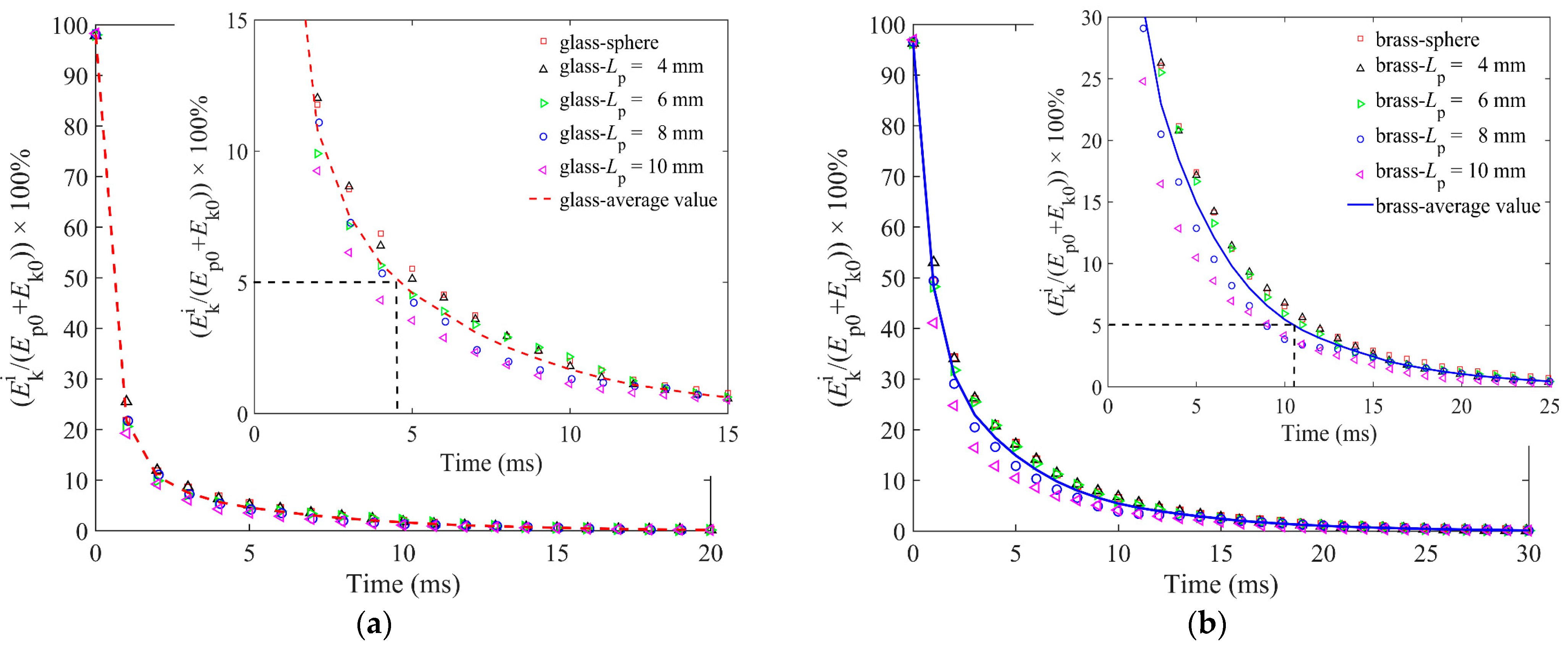
4.1.2. The Impactor
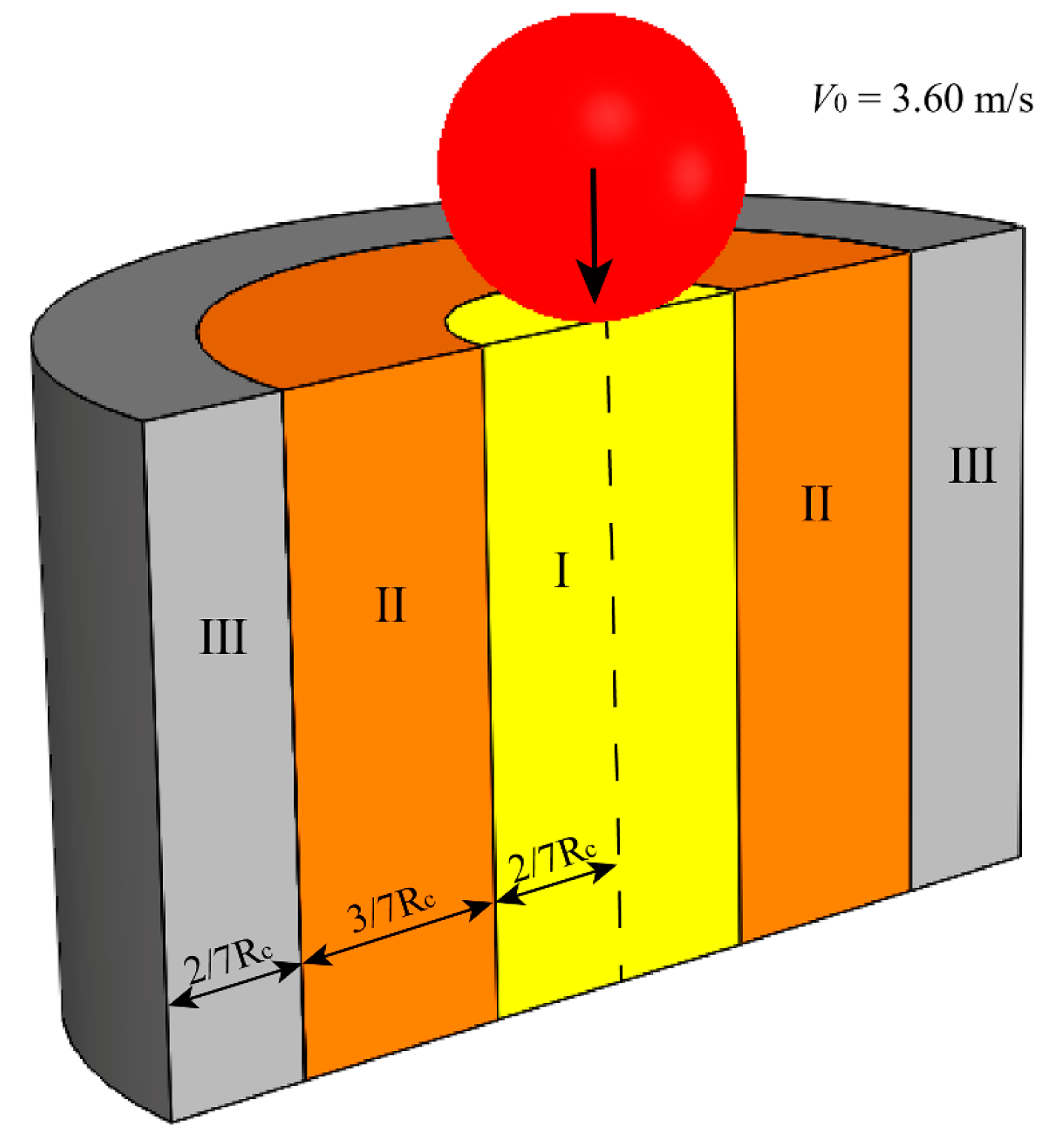
4.1.3. The Particles in the Cup
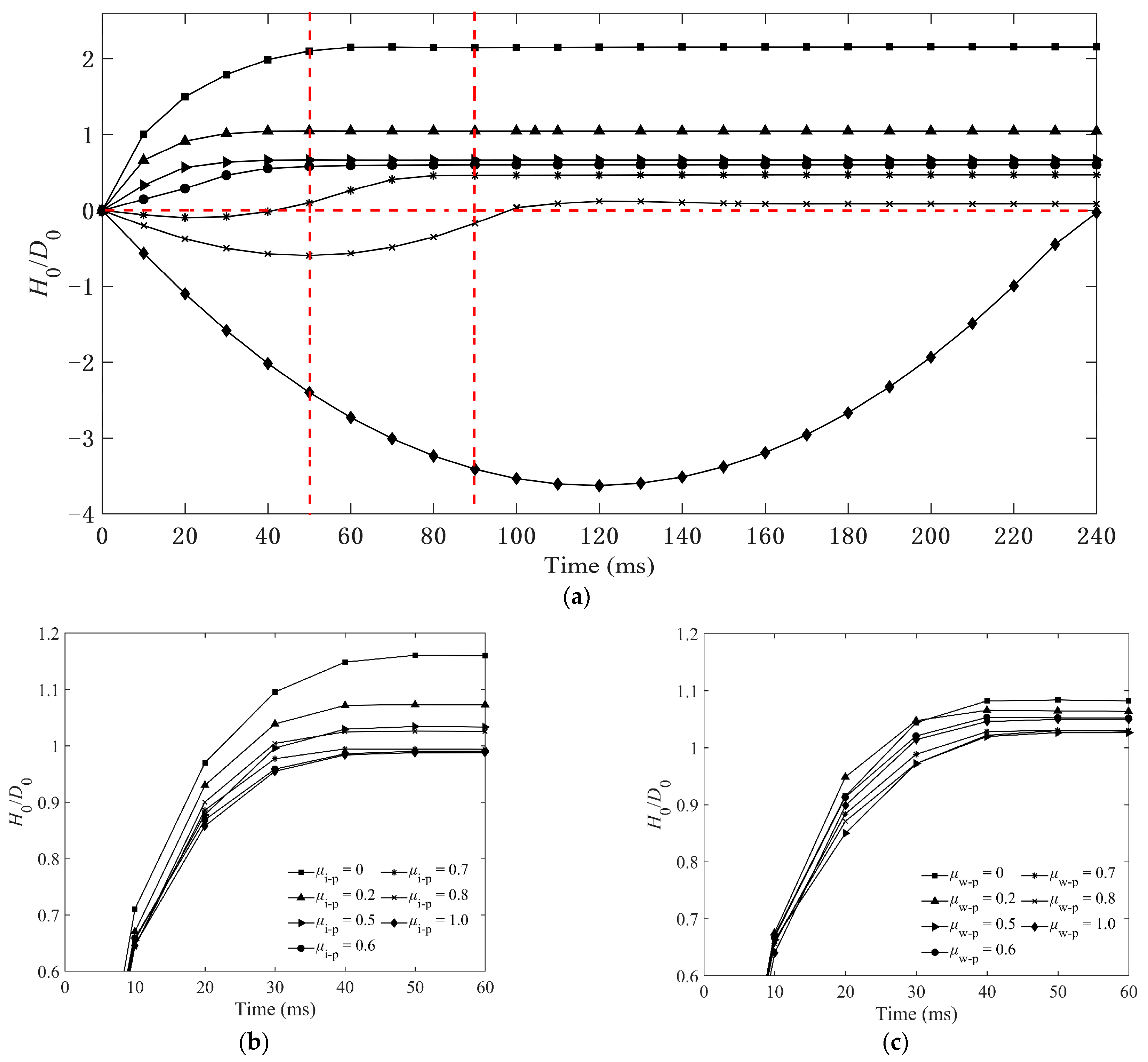
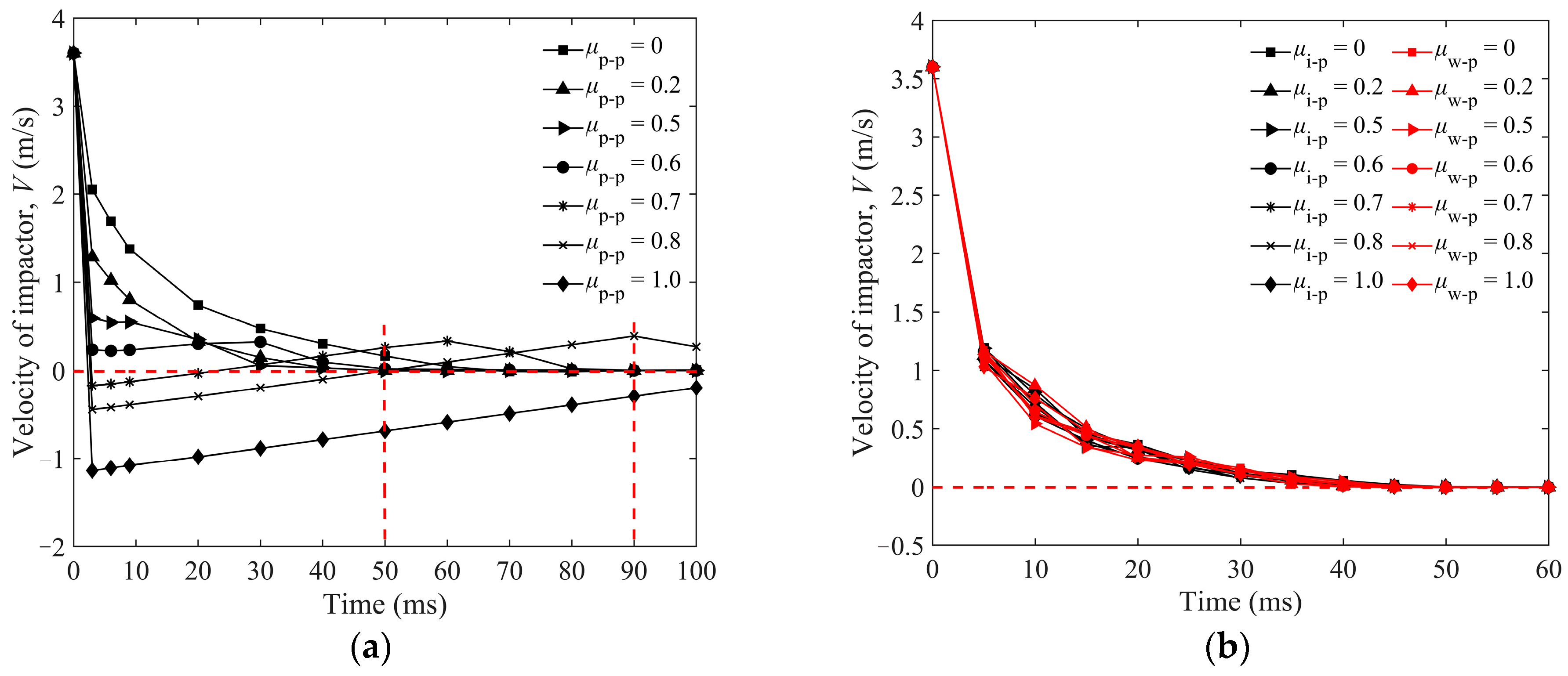
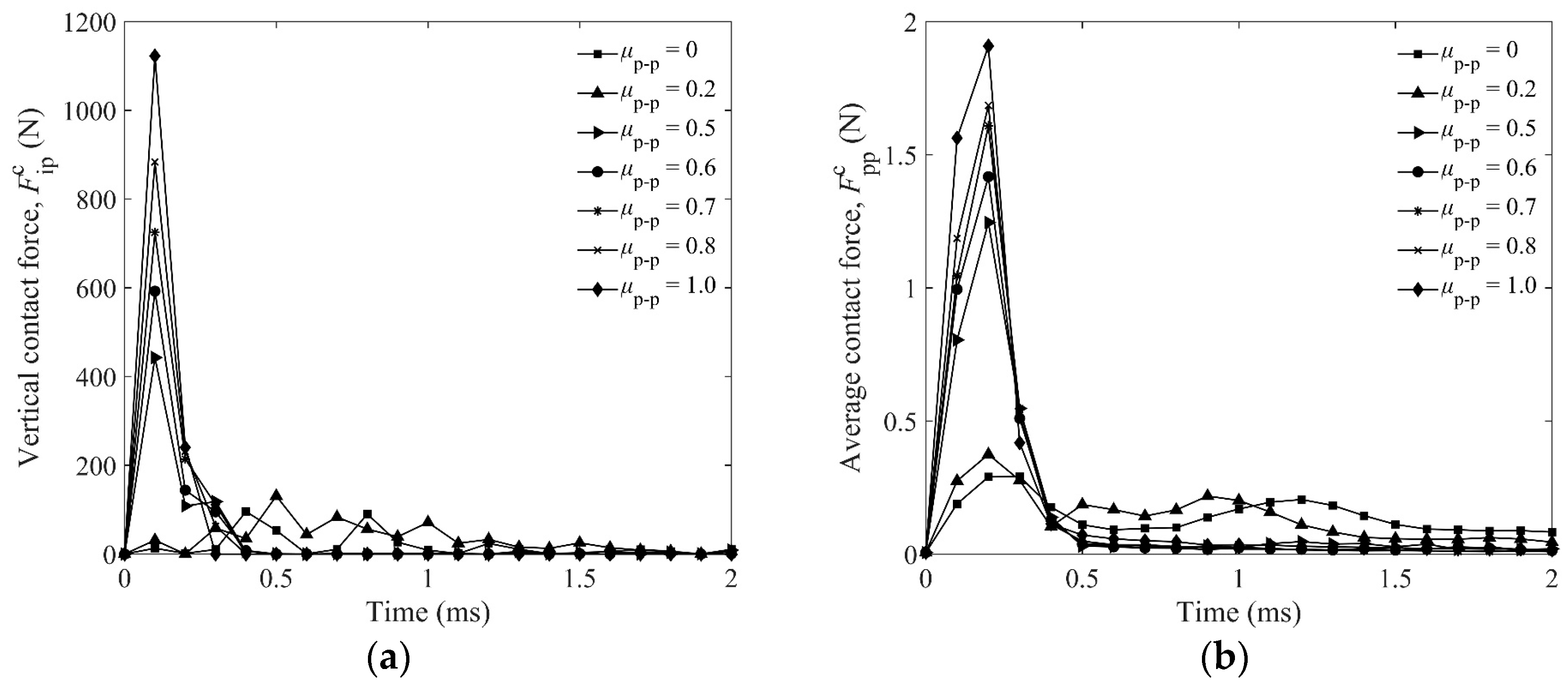
4.2. Effect of Friction Coefficient
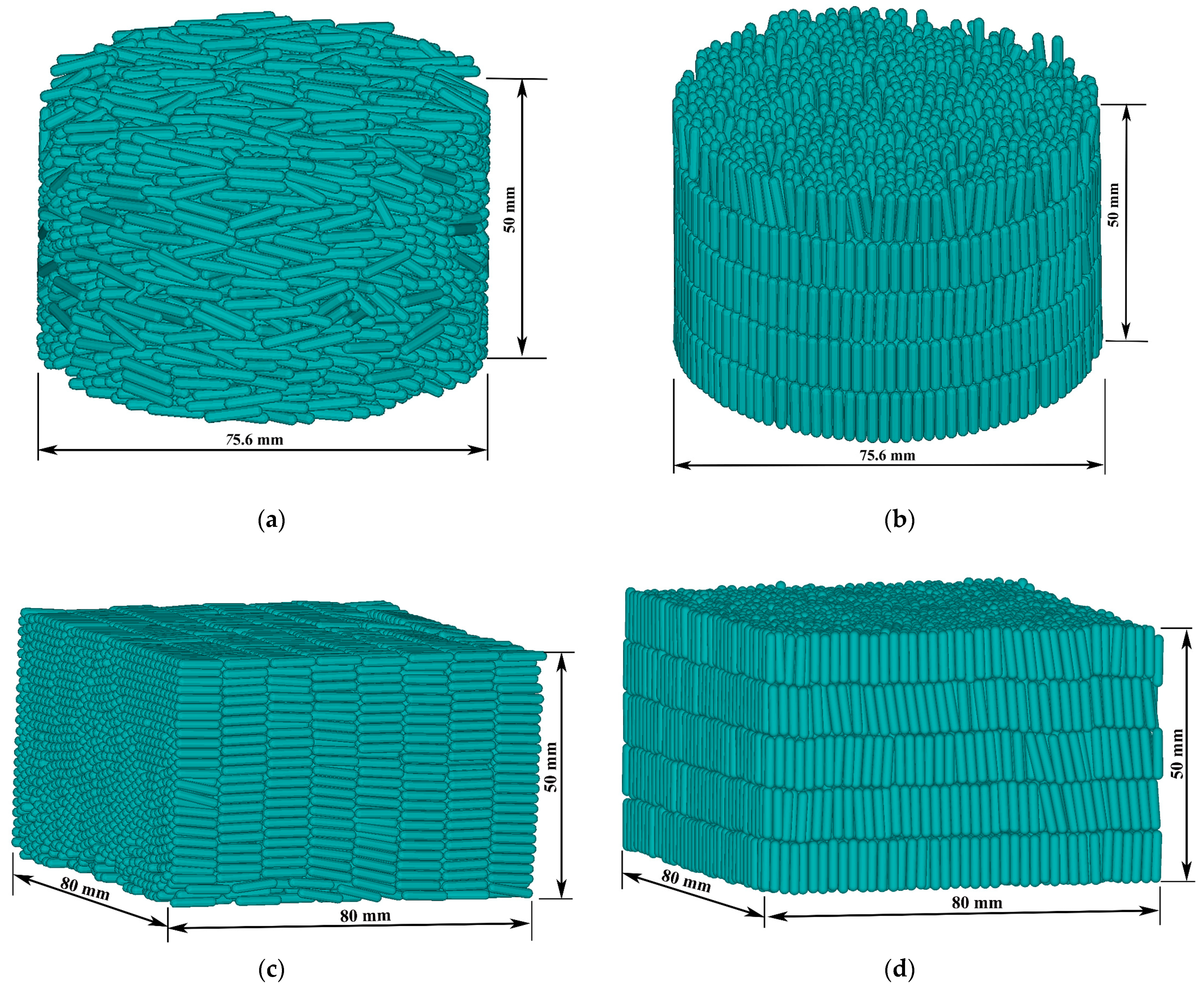
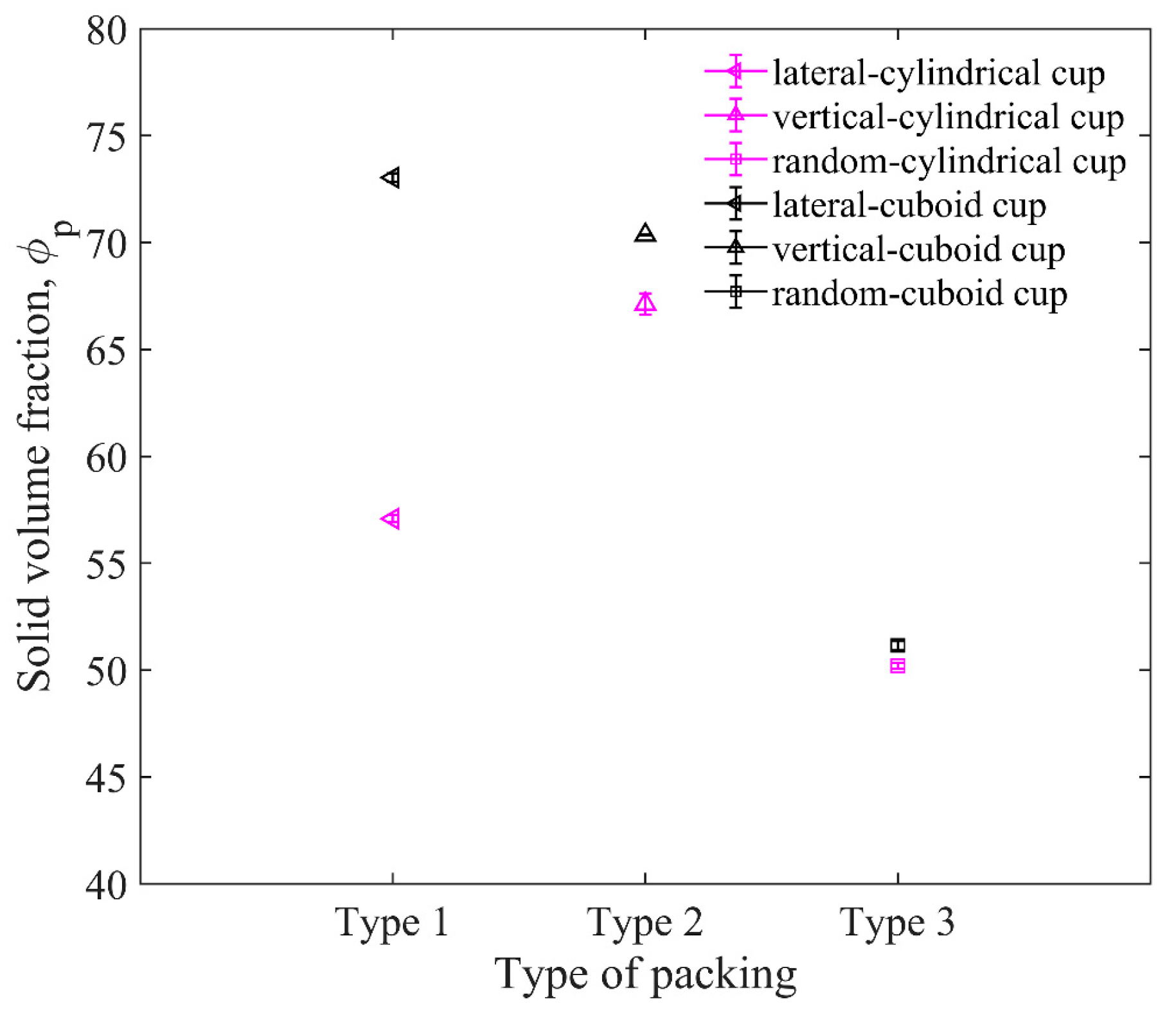
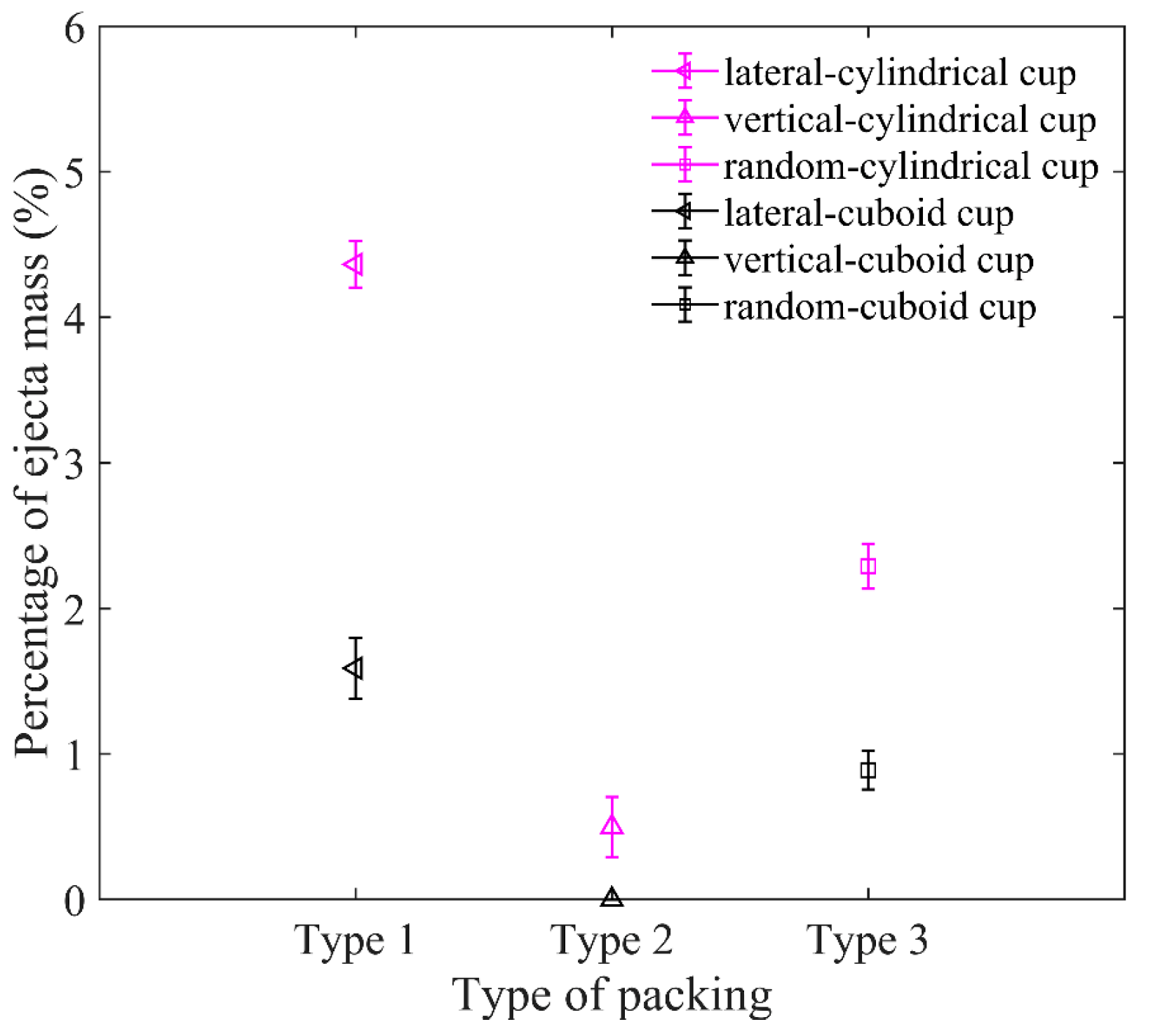
4.3. Effect of Particle Configuration
5. Conclusions
- The effect of particle length. The ejecta mass of the spherical particle bed is obviously larger than that of the elongated particle bed. The granular bed of longer particles has a smaller penetration depth due to the spatial structure of elongated particles, although the solid volume fraction is smaller. In addition, the average contact force between particles is positively correlated to particle length. The average kinetic energy of the impactor obeys an exponential-like dissipation, and the particle length of the elongated particles has little effect on the energy allocation from the impactor to the ejected particles and particles in the cup.
- The effect of friction. The μp-p has a significant effect on the ejecta mass and penetration depth of the impactor, while μi-p and μw-p have a limited effect. The ejecta mass and penetration depth are negatively correlated to μp-p. The contact force between particles and particles or impactors are positively correlated to μp-p.
- The effect of particle configuration. The cuboid cup can obtain a more dense and regular granular bed. The ejecta mass and penetration depth of vertical arrangement are the smallest. For the same arrangement of elongated particles, the penetration depth is negatively correlated to order parameters and solid volume fraction.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Fi | external forces of particle i (N) |
| Ti | external torques of particle i (N·m) |
| mi | mass of particle i (g) |
| vi | translational velocity of particle i (m/s) |
| Ii | moment of inertia of particle i (kg·m2) |
| ωi | rotational velocity of the particle i (rad/s) |
| δn | overlap in normal direction (mm) |
| Fn | normal contact force (N) |
| E* | equivalent Young’s modulus |
| R* | equivalent radius of two objects |
| Fnmin | normal contact force when θ = 0° (N) |
| Fnmax | normal contact force when θ = 90° (N) |
| l | length of contact area along the major axis (mm) |
| b | width of contact area (mm) |
| Ft | tangential contact force in the current time step (N) |
| Ft0 | tangential force vectors in the previous time step (N) |
| G* | equivalent shear modulus |
| a | effective radius of contact (mm) |
| dt | incremental tangential displacement (mm) |
| normal damping force (N) | |
| tangential damping force (N) | |
| m* | equivalent mass |
| vn | normal component of relative velocity (m/s) |
| vt | tangential component of relative velocity (m/s) |
| β | contact damping coefficient |
| Sn | normal contact stiffness |
| St | tangential contact stiffness |
| V | vertical velocity of impactor (m/s) |
| g | acceleration of gravity (N/kg) |
| m0 | total mass of particles in cup before impact (g) |
| m1 | total mass of particles in cup after impact (g) |
| Δm | mass of ejected particles (g) |
| H0 | penetration depth of impactor (mm) |
| D0 | diameter of impactor(mm) |
| Lp | particle length (mm) |
| Ek0 | the initial kinetic energy of the impactor (J) |
| Ep0 | the initial potential energy of the impactor (J) |
| kinetic energy of the impactor (J) | |
| kinetic energy of the particles in the cup (J) | |
| kinetic energy of the ejected particles (J) | |
| Tp | granular temperature (m2/s2) |
| ϕp | solid volume fraction |
| average contact force between particles (N) | |
| μp-p | coefficient of friction between target particles |
| μw-p | coefficient of friction between wall and particles |
| μi-p | coefficient of friction between impactor and particles |
| Tp,q | granular temperature of particles along q (q = x, y, z) direction (m2/s2) |
| vertical contact force between impactor and particles (N) | |
| O | orientational parameter |
| Sr | the order parameter |
References
- Bötschi, S.; Rajagopalan, A.K.; Rombaut, I.; Morari, M.; Mazzotti, M. From needle-like toward equant particles: A controlled crystal shape engineering pathway. Comput. Chem. Eng. 2019, 131, 106581. [Google Scholar] [CrossRef]
- Aust, A.E.; Cook, P.M.; Dodson, R.F. Morphological and chemical mechanisms of elongated mineral particle toxicities. J. Toxicol. Environ. Health Part B 2011, 14, 40–75. [Google Scholar] [CrossRef] [PubMed]
- Doshi, N.; Mitragotri, S. Needle-shaped polymeric particles induce transient disruption of cell membranes. J. R. Soc. Interface 2010, 7, S403–S410. [Google Scholar] [CrossRef] [PubMed]
- Guidelli, E.J.; Ramos, A.P.; Zaniquelli, M.E.D.; Nicolucci, P.; Baffa, O. Synthesis and characterization of silver/alanine nanocomposites for radiation detection in medical applications: The influence of particle size on the detection properties. Nanoscale 2012, 4, 2884. [Google Scholar] [CrossRef] [PubMed]
- Khodadadian, A.; Noii, N.; Parvizi, M.; Abbaszadeh, M.; Wick, T.; Heitzinger, C. A Bayesian estimation method for variational phase-field fracture problems. Comput. Mech. 2020, 66, 827–849. [Google Scholar] [CrossRef]
- Noii, N.; Khodadadian, A.; Aldakheel, F. Probabilistic failure mechanisms via Monte Carlo simulations of complex microstructures. Comput. Methods Appl. Mech. Eng. 2022, 399, 115358. [Google Scholar] [CrossRef]
- Pop, N. A finite element solution for a three-dimensional quasistatic frictional contact problem. Rev Roum. Sci. Tech Ser. Mec Appliq Ed. L’Academie Roum. 1997, 42, 1–2. [Google Scholar]
- Scutaru, M.L.; Vlase, S.; Marin, M.; Modrea, A. New analytical method based on dynamic response of planar mechanical elastic systems. Bound. Value Probl. 2020, 2020, 104. [Google Scholar] [CrossRef]
- Cundall, P.A.; Strack, O.D.L. A discrete numerical model for granular assemblies. Géotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Bester, C.S.; Cox, N.; Zheng, H.; Behringer, R.P. Dynamics of oblique impact in a quasi two-dimensional granular medium. Granul. Matter 2020, 22, 51. [Google Scholar] [CrossRef]
- Prieur, N.C.; Rolf, T.; Luther, R.; Wünnemann, K.; Xiao, Z.; Werner, S.C. The effect of target properties on transient crater scaling for simple craters: Target properties and simple craters. J. Geophys. Res. Planets 2017, 122, 1704–1726. [Google Scholar] [CrossRef]
- Seguin, A.; Bertho, Y.; Gondret, P.; Crassous, J. Sphere penetration by impact in a granular medium: A collisional process. EPL Europhys. Lett. 2009, 88, 44002. [Google Scholar] [CrossRef]
- Ye, X.; Wang, D.; Zheng, X. Effect of packing fraction on dynamic characteristics of granular materials under oblique impact. Powder Technol. 2018, 339, 211–222. [Google Scholar] [CrossRef]
- Escobar-Ortega, Y.Y.; Hidalgo-Caballero, S.; Marston, J.O.; Pacheco-Vázquez, F. The viscoelastic-like response of a repulsive granular medium during projectile impact and penetration. J. Non-Newton. Fluid Mech. 2020, 280, 104295. [Google Scholar] [CrossRef]
- Takada, S.; Hayakawa, H. Drag acting on an intruder in a three-dimensional granular environment. Granul. Matter 2020, 22, 6. [Google Scholar] [CrossRef]
- Miyai, S.; Kobayakawa, M.; Tsuji, T.; Tanaka, T. Influence of particle size on vertical plate penetration into dense cohesionless granular materials (large-scale DEM simulation using real particle size). Granul. Matter 2019, 21, 105. [Google Scholar] [CrossRef]
- Li, Y.; Dove, A.; Curtis, J.S.; Colwell, J.E. 3D DEM simulations and experiments exploring low-velocity projectile impacts into a granular bed. Powder Technol. 2016, 288, 303–314. [Google Scholar] [CrossRef]
- Huang, K.; Delfin, D.H.; Rech, F.; Dichtl, V.; Hidalgo, R.C. The role of initial speed in projectile impacts into light granular media. Sci. Rep. 2020, 10, 3207. [Google Scholar] [CrossRef]
- Holmen, J.K.; Olovsson, L.; Børvik, T. Discrete modeling of low-velocity penetration in sand. Comput. Geotech. 2017, 86, 21–32. [Google Scholar] [CrossRef]
- Güttler, C.; Hirata, N.; Nakamura, A.M. Cratering experiments on the self-armoring of coarse-grained granular targets. Icarus 2012, 220, 1040–1049. [Google Scholar] [CrossRef]
- Davison, T.M.; Collins, G.S.; Elbeshausen, D.; Wünnemann, K.; Kearsley, A. Numerical modeling of oblique hypervelocity impacts on strong ductile targets: Oblique hypervelocity impacts on ductile targets. Meteorit. Planet. Sci. 2011, 46, 1510–1524. [Google Scholar] [CrossRef]
- Horabik, J.; Sochan, A.; Beczek, M.; Mazur, R.; Ryżak, M.; Parafiniuk, P.; Kobyłka, R.; Bieganowski, A. Discrete Element Method simulations and experimental study of interactions in 3D granular bedding during low-velocity impact. Powder Technol. 2018, 340, 52–67. [Google Scholar] [CrossRef]
- Kodam, M.; Bharadwaj, R.; Curtis, J.; Hancock, B.; Wassgren, C. Cylindrical object contact detection for use in Discrete Element Method simulations. Part I—Contact detection algorithms. Chem. Eng. Sci. 2010, 65, 5852–5862. [Google Scholar] [CrossRef]
- Guo, Y.; Wassgren, C.; Curtis, J.S.; Xu, D. A bonded sphero-cylinder model for the discrete element simulation of elasto-plastic fibers. Chem. Eng. Sci. 2018, 175, 118–129. [Google Scholar] [CrossRef]
- Kidokoro, T.; Arai, R.; Saeki, M. Investigation of dynamics simulation of granular particles using sphero-cylinder model. Granul. Matter 2015, 17, 743–751. [Google Scholar] [CrossRef]
- Xie, C.; Song, T.; Zhao, Y. Discrete element modeling and simulation of non-spherical particles using polyhedrons and super-ellipsoids. Powder Technol. 2020, 368, 253–267. [Google Scholar] [CrossRef]
- Azéma, E.; Radjai, F.; Dubois, F. Packings of irregular polyhedral particles: Strength, structure, and effects of angularity. Phys. Rev. E 2013, 87, 062203. [Google Scholar] [CrossRef]
- Zhao, Y.; Xu, L.; Umbanhowar, P.B.; Lueptow, R.M. Discrete element simulation of cylindrical particles using super-ellipsoids. Particuology 2019, 46, 55–66. [Google Scholar] [CrossRef]
- You, Y.; Zhao, Y. Discrete element modelling of ellipsoidal particles using super-ellipsoids and multi-spheres: A comparative study. Powder Technol. 2018, 331, 179–191. [Google Scholar] [CrossRef]
- Liu, Z.; Ma, H.; Zhao, Y. Comparative study of discrete element modeling of tablets using multi-spheres, Multi-Super-Ellipsoids, and Polyhedrons. Powder Technol. 2021, 390, 34–49. [Google Scholar] [CrossRef]
- Liu, Z.; Zhao, Y. Multi-super-ellipsoid model for non-spherical particles in DEM simulation. Powder Technol. 2020, 361, 190–202. [Google Scholar] [CrossRef]
- Feng, Y.T.; Han, K.; Owen, D.R.J. Energy-conserving contact interaction models for arbitrarily shaped Discrete Elements. Comput. Methods Appl. Mech. Eng. 2012, 205–208, 169–177. [Google Scholar] [CrossRef]
- Tangri, H.; Guo, Y.; Curtis, J.S. Packing of cylindrical particles: DEM simulations and experimental measurements. Powder Technol. 2017, 317, 72–82. [Google Scholar] [CrossRef]
- Tangri, H.; Guo, Y.; Curtis, J.S. Hopper discharge of elongated particles of varying aspect ratio: Experiments and DEM simulations. Chem. Eng. Sci. X 2019, 4, 100040. [Google Scholar] [CrossRef]
- Wang, S.; Ji, S. Discrete element analysis of buffering capacity of non-spherical granular materials based on super-quadric method. Acta Phys. Sin. 2018, 67, 182–193. (In Chinese) [Google Scholar]
- Guo, Y.; Liu, Q.; Li, Y.; Li, Z.; Jin, H.; Wassgren, C.; Curtis, J.S. Discrete element method models of elastic and elastoplastic fiber assemblies. AIChE J. 2021, 67, e17296. [Google Scholar] [CrossRef]
- Shackelford, J.F.; Alexander, W. CRC materials science and engineering handbook (Third Edition). Chem. Eng. Sci. 1999, 11, 41. [Google Scholar] [CrossRef]
- Hao, J.; Li, Y.; Liu, Y.; Curtis, J.S.; Guo, Y. Jamming in granular shear flows of frictional, polydisperse cylindrical particles. Adv. Powder Technol. 2021, 32, 3746–3759. [Google Scholar] [CrossRef]
- Qian, Q.; An, X.; Zhao, H.; Dong, K.; Wu, Y.; Fu, H.; Zhang, H.; Yang, X. Particle scale study on the crystallization of mono-sized cylindrical particles subject to vibration. Powder Technol. 2019, 352, 470–477. [Google Scholar] [CrossRef]











| Scenarios | Models | ||
|---|---|---|---|
| Group I | 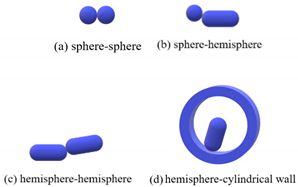 | (3) | |
| in which δn is the overlap in normal direction, E* is equivalent Young’s modulus, and R* is equivalent radius of two objects in contact, which are defined as and , respectively, where E1 and E2, R1 and R2, ν1 and ν2 are the Young’s moduli, the radii, and the Poisson’s ratios of two contacting particles, respectively. | |||
| Group II |  | (4) | |
| in which α is determined by the shape of contact area and determined to be 0.974 here. | |||
| Group III |  | (5) | |
| in which θ is the angle between the two major axes of particles. , when θ is equal to zero. is the same as Equation (6). | |||
| Group IV |  | (6) | |
| in which k is a constant determined as 2.5, l is the length of contact area along the major axis, b is the width of contact area, . | |||
| Tangential force model | (7) | ||
| where Ft0 and Ft are the tangential force vectors in the previous time step and the current time step, respectively. G* is governed by , in which G1 and G2 are the shear moduli of the two objects in contact, ν1 and ν2 are the corresponding Poisson’s ratios. is the effective radius of contact, , and dt represents the incremental tangential displacement in the present time step. | |||
| Parameters | Steel Objective Particles | Impactor | Granular Cup |
|---|---|---|---|
| diameter (mm) | Dp = 2.00 | Dgls = 19.71/Dbrs = 19.01 | Dc = 75.60 |
| height (mm) | / | / | Hc = 46.10 |
| length (mm) | Lp = 4, 6, 8 *, 10 * | / | / |
| Young’s modulus (GPa) Poisson’s ratio | Ep = 182.00 | Egls = 71.70/Ebrs = 117.00 | Ec = 70.00 |
| νp = 0.30 | νgls = 0.24/νbrs = 0.34 | νc = 0.35 | |
| density (kg/m3) | ρp = 8000.45 | ρgls = 2478.36/ρbrs = 8700.20 | ρc = 2700.30 |
| coefficient of friction | μp-p = 0.20/0 # | μi-p = 0.20/0 # | μw-p = 0.34/0 # |
| coefficient of restitution | ep-p = 0.95 | ei-p = 0.88 (gls)/0.65(brs) | ew-p = 0. 69 |
| time step (s) | ∆t = 2.30 × 10−7 | ||
| gravity (N/kg) | g = 9.81 | ||
| impact velocity (m/s) | V0 = 3.60 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, P.; Li, Y.; Hua, X.; Guo, Y.; Curtis, J.S. 3D DEM Simulations and Experiments on Spherical Impactor Penetrating into the Elongated Particles. Materials 2023, 16, 1664. https://doi.org/10.3390/ma16041664
Li P, Li Y, Hua X, Guo Y, Curtis JS. 3D DEM Simulations and Experiments on Spherical Impactor Penetrating into the Elongated Particles. Materials. 2023; 16(4):1664. https://doi.org/10.3390/ma16041664
Chicago/Turabian StyleLi, Ping, Yanjie Li, Xia Hua, Yu Guo, and Jennifer Sinclair Curtis. 2023. "3D DEM Simulations and Experiments on Spherical Impactor Penetrating into the Elongated Particles" Materials 16, no. 4: 1664. https://doi.org/10.3390/ma16041664
APA StyleLi, P., Li, Y., Hua, X., Guo, Y., & Curtis, J. S. (2023). 3D DEM Simulations and Experiments on Spherical Impactor Penetrating into the Elongated Particles. Materials, 16(4), 1664. https://doi.org/10.3390/ma16041664







