Effects of Noncontact Shoulder Tool Velocities on Friction Stir Joining of Polyamide 6 (PA6)
Abstract
:1. Introduction
2. Process Modelling
2.1. Model Description
2.2. Weld Metal Model
2.3. Boundary Conditions
2.4. Heat Transfer Model
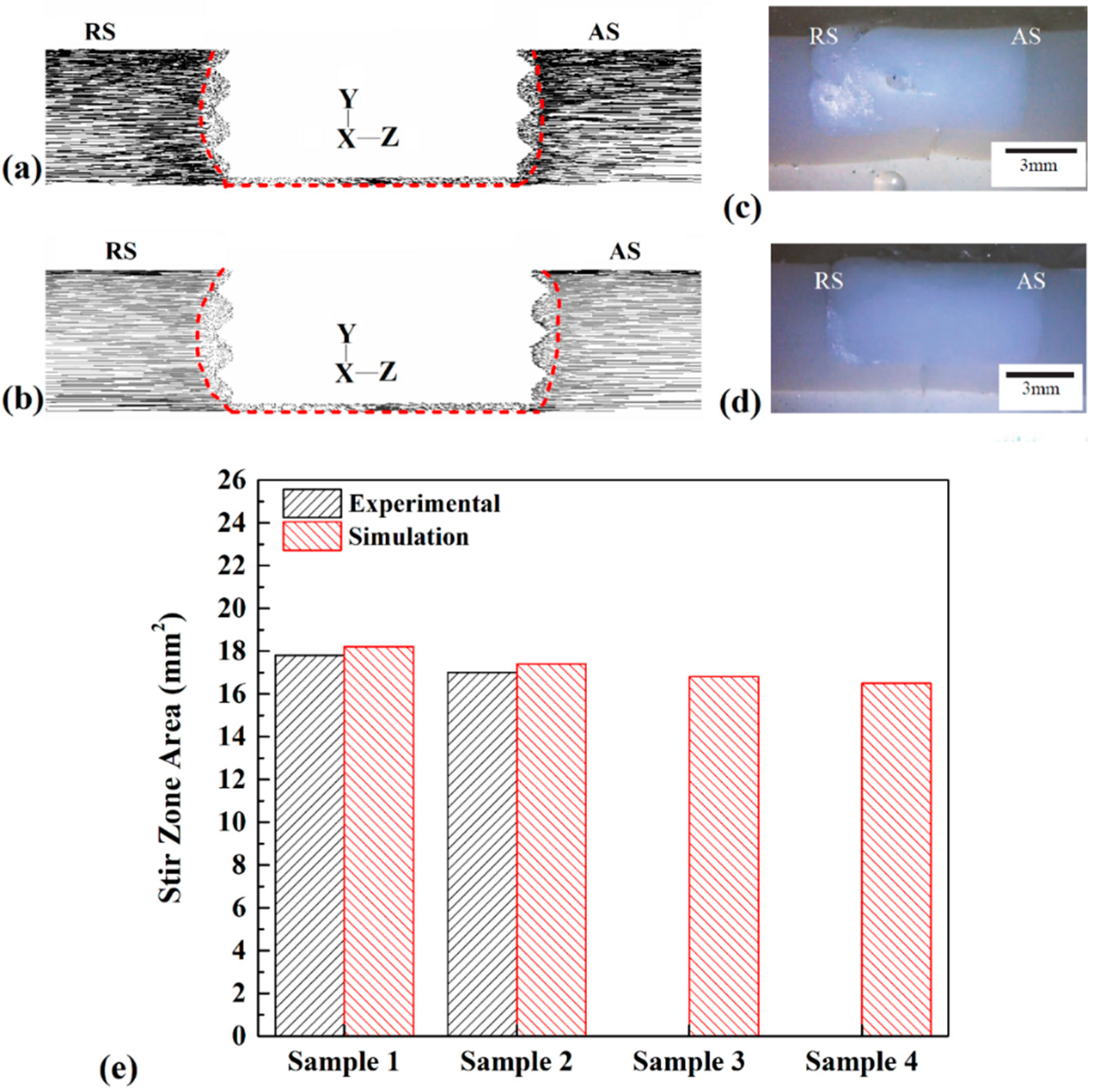
3. Model Validation
4. Results and Discussion
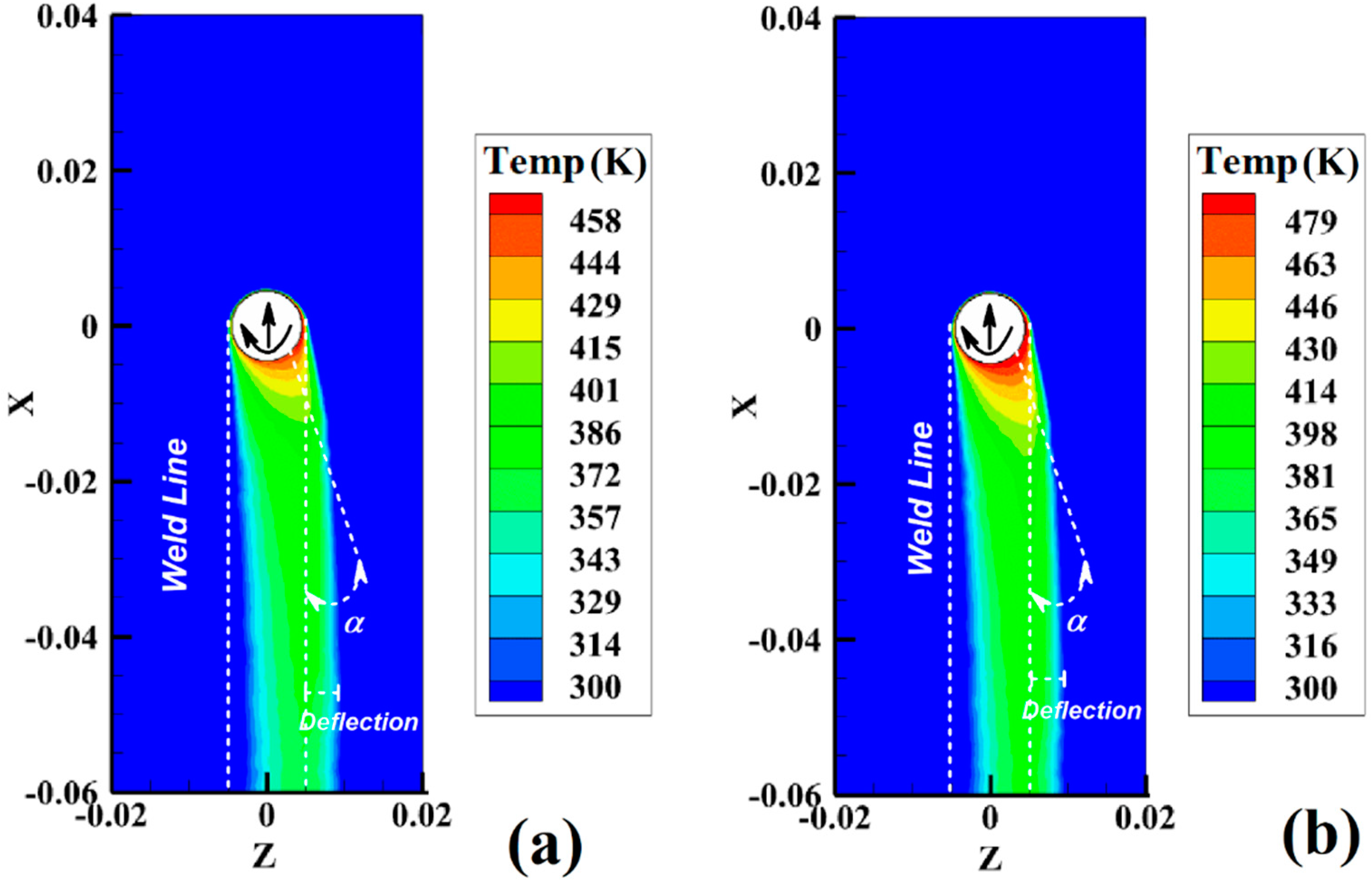
4.1. Heat Generation
4.2. Strain Rate
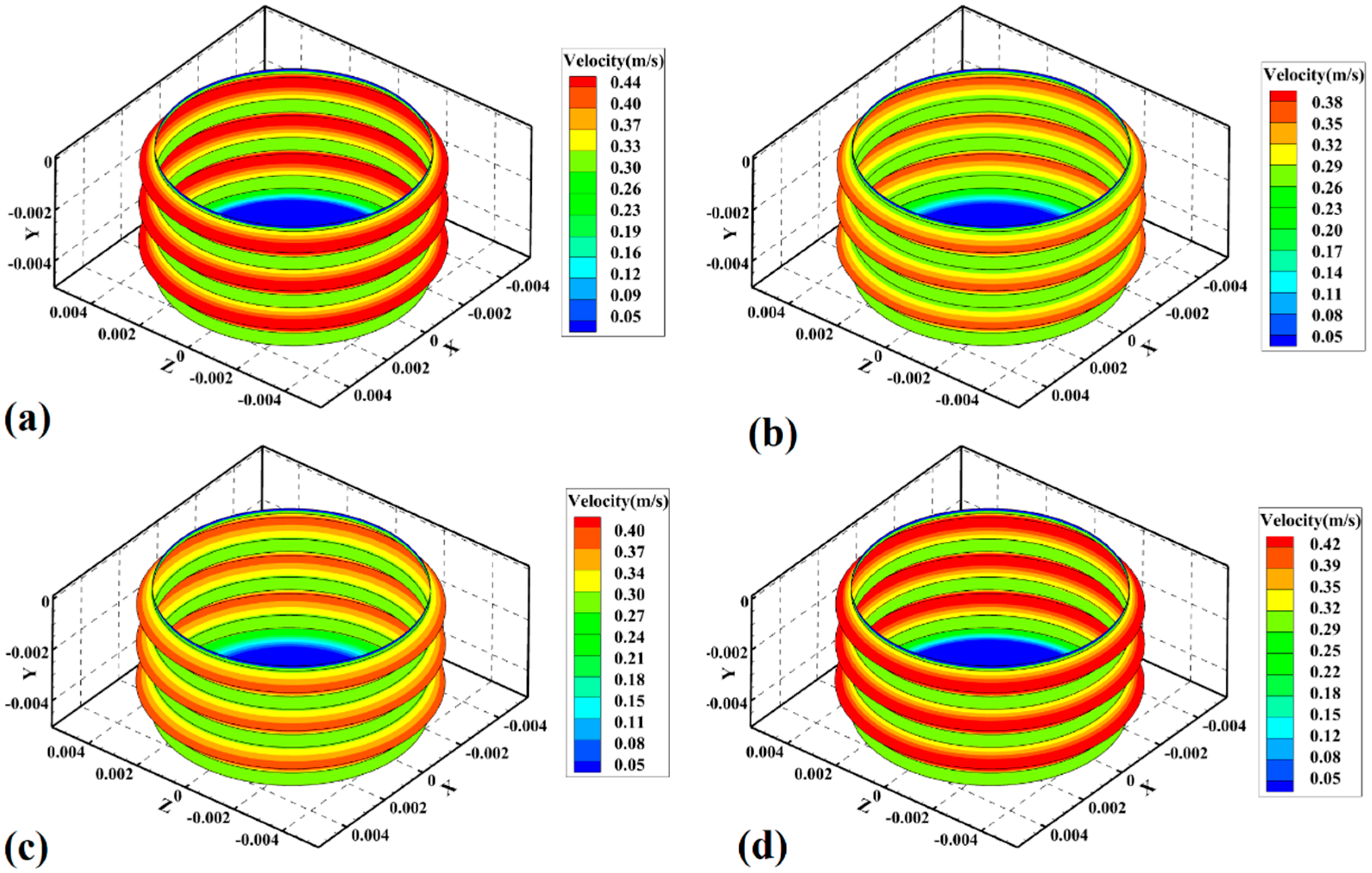
4.3. Materials Velocity
4.4. Internal Material Flow
5. Conclusions
- Due to the lack of a contact surface between the tool shoulder and workpiece, the SZ’s joint morphology and heat generation were concentrated around the pin area. The results showed that the heat generation increased with increasing tool rotational velocity. Heat generation increased by 8% when the tool velocity increased from 380 rpm to 500 rpm.
- This joining type’s internal and surface heat flow differed from the normal FSJ process. The internal heat distribution was rectangular, and the surface temperature gradient was tilted from the joint line.
- The velocity and strain rate of the materials in SZ were not uniform. The screw pin shape led to a higher strain rate and material velocity at the exterior area of the pin. This behavior caused the formation of a rectangular stir zone. This SZ type minimized the size of the joint line compared to the regular SZ of polymeric material welded with the conventional FSJ process.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Khalaf, H.I.; Al-Sabur, R.; Abdullah, M.E.; Kubit, A.; Derazkola, H.A. Effects of Underwater Friction Stir Welding Heat Generation on Residual Stress of AA6068-T6 Aluminum Alloy. Materials 2022, 15, 2223. [Google Scholar] [CrossRef] [PubMed]
- Aghajani Derazkola, H.; Simchi, A.; Lambiase, F. Friction stir welding of polycarbonate lap joints: Relationship between processing parameters and mechanical properties. Polym. Test. 2019, 79, 105999. [Google Scholar] [CrossRef]
- Aghajani Derazkola, H.; Simchi, A. Processing and characterizations of polycarbonate/alumina nanocomposites by additive powder fed friction stir processing. Thin Walled Struct. 2020, 157, 107086. [Google Scholar] [CrossRef]
- Iftikhar, S.H.; Mourad, A.-H.I.; Sheikh-Ahmad, J.; Almaskari, F.; Vincent, S. A Comprehensive Review on Optimal Welding Conditions for Friction Stir Welding of Thermoplastic Polymers and Their Composites. Polymers 2021, 13, 1208. [Google Scholar] [CrossRef] [PubMed]
- Ahmed, M.M.Z.; Habba, M.I.A.; Jouini, N.; Alzahrani, B.; Seleman, M.M.E.-S.; El-Nikhaily, A. Bobbin Tool Friction Stir Welding of Aluminum Using Different Tool Pin Geometries: Mathematical Models for the Heat Generation. Metals 2021, 11, 438. [Google Scholar] [CrossRef]
- Al-Sabur, R. Tensile strength prediction of aluminium alloys welded by FSW using response surface methodology—Comparative review. Mater. Today Proc. 2021, 45, 4504–4510. [Google Scholar] [CrossRef]
- Asmare, A.; Al-Sabur, R.; Messele, E. Experimental Investigation of Friction Stir Welding on 6061-T6 Aluminum Alloy using Taguchi-Based GRA. Metals 2020, 10, 1480. [Google Scholar] [CrossRef]
- Al-Sabur, R.; Jassim, A.K.; Messele, E. Real-time monitoring applied to optimize friction stir spot welding joint for AA1230 Al-alloys. Mater. Today Proc. 2021, 42, 2018–2024. [Google Scholar] [CrossRef]
- Derazkola, H.A.; Khodabakhshi, F.; Gerlich, A.P. Fabrication of a nanostructured high strength steel tube by friction-forging tubular additive manufacturing (FFTAM) technology. J. Manuf. Process. 2020, 58, 724–735. [Google Scholar] [CrossRef]
- Aghajani Derazkola, H.; Khodabakhshi, F.; Gerlich, A.P. Friction-forging tubular additive manufacturing (FFTAM): A new route of solid-state layer-upon-layer metal deposition. J. Mater. Res. Technol. 2020, 9, 15273–15285. [Google Scholar] [CrossRef]
- Li, T.; Shi, Q.Y.; Li, H.K.; Wang, W.; Cai, Z.P. Residual Stresses of Friction Stir Welded 2024-T4 Joints. Mater. Sci. Forum 2008, 580–582, 263–266. [Google Scholar] [CrossRef]
- Vahdati, M.; Moradi, M. Statistical analysis and optimization of tensile strength of Al7075 butt joint produced by friction stir welding and submerged friction stir welding via response surface methodology and desirability approach. Amirkabir J. Mech. Eng. 2021, 53, 18. [Google Scholar] [CrossRef]
- Pereira, A.B.; Fernandes, F.A.O.; de Morais, A.B.; Quintão, J. Mechanical Strength of Thermoplastic Polyamide Welded by Nd:YAG Laser. Polymers 2019, 11, 1381. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Stokes, V.K. Experiments on the hot-tool welding of three dissimilar thermoplastics. Polymer 1998, 39, 2469–2477. [Google Scholar] [CrossRef]
- Pereira, M.A.R.; Amaro, A.M.; Reis, P.N.B.; Loureiro, A. Effect of Friction Stir Welding Techniques and Parameters on Polymers Joint Efficiency—A Critical Review. Polymers 2021, 13, 2056. [Google Scholar] [CrossRef] [PubMed]
- Maggiore, S.; Banea, M.D.; Stagnaro, P.; Luciano, G. A Review of Structural Adhesive Joints in Hybrid Joining Processes. Polymers 2021, 13, 3961. [Google Scholar] [CrossRef]
- Mendes, N.; Loureiro, A.; Martins, C.; Neto, P.; Pires, J.N. Effect of friction stir welding parameters on morphology and strength of acrylonitrile butadiene styrene plate welds. Mater. Des. 2014, 58, 457–464. [Google Scholar] [CrossRef]
- Simões, F.; Rodrigues, D.M. Material flow and thermo-mechanical conditions during Friction Stir Welding of polymers: Literature review, experimental results and empirical analysis. Mater. Des. 2014, 59, 344–351. [Google Scholar] [CrossRef]
- Azarsa, E.; Mostafapour, A. Experimental investigation on flexural behavior of friction stir welded high density polyethylene sheets. J. Manuf. Process. 2014, 16, 149–155. [Google Scholar] [CrossRef]
- Saeedy, S.; Givi, M.K.B. Investigation of the effects of critical process parameters of friction stir welding of polyethylene. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2011, 225, 1305–1310. [Google Scholar] [CrossRef]
- Panneerselvam, K.; Lenin, K. Investigation on Effect of Tool Forces and Joint Defects During FSW of Polypropylene Plate. Procedia Eng. 2012, 38, 3927–3940. [Google Scholar] [CrossRef] [Green Version]
- Panneerselvam, K.; Lenin, K. Joining of Nylon 6 plate by friction stir welding process using threaded pin profile. Mater. Des. 2014, 53, 302–307. [Google Scholar] [CrossRef]
- Lambiase, F.; Derazkola, H.A.; Simchi, A. Friction Stir Welding and Friction Spot Stir Welding Processes of Polymers—State of the Art. Materials 2020, 13, 2291. [Google Scholar] [CrossRef] [PubMed]
- Myalski, J.; Godzierz, M.; Olesik, P. Effect of Carbon Fillers on the Wear Resistance of PA6 Thermoplastic Composites. Polymers 2020, 12, 2264. [Google Scholar] [CrossRef] [PubMed]
- Khan, I.; Hussain, G.; Al-Ghamdi, K.A.; Umer, R. Investigation of Impact Strength and Hardness of UHMW Polyethylene Composites Reinforced with Nano-Hydroxyapatite Particles Fabricated by Friction Stir Processing. Polymers 2019, 11, 1041. [Google Scholar] [CrossRef] [Green Version]
- Derazkola, H.A.; Eyvazian, A.; Simchi, A. Modeling and experimental validation of material flow during FSW of polycarbonate. Mater. Today Commun. 2020, 22, 100796. [Google Scholar] [CrossRef]
- Aghajani Derazkola, H.; Simchi, A. Experimental and thermomechanical analysis of friction stir welding of poly(methyl methacrylate) sheets. Sci. Technol. Weld. Join. 2018, 23, 209–218. [Google Scholar] [CrossRef]
- Sheikh-Ahmad, J.Y.; Deveci, S.; Almaskari, F.; Rehman, R.U.R. Effect of process temperatures on material flow and weld quality in the friction stir welding of high density polyethylene. J. Mater. Res. Technol. 2022, 18, 1692–1703. [Google Scholar] [CrossRef]
- Rehman, R.U.; Sheikh-Ahmad, J.; Deveci, S. Effect of preheating on joint quality in the friction stir welding of bimodal high density polyethylene. Int. J. Adv. Manuf. Technol. 2021, 117, 455–468. [Google Scholar] [CrossRef]
- Shete, M.T.; Yarasu, R.B. Experimental investigation and finite element simulation of friction stir spot welding (FSSW) of high-density polyethylene joints. Mater. Today Proc. 2021, 47, 4274–4280. [Google Scholar] [CrossRef]
- Khodabakhshi, F.; Derazkola, H.A.; Gerlich, A.P. Monte Carlo simulation of grain refinement during friction stir processing. J. Mater. Sci. 2020, 55, 13438–13456. [Google Scholar] [CrossRef]
- Aghajani Derazkola, H.; Garcia, E.; Elyasi, M. Underwater friction stir welding of PC: Experimental study and thermo-mechanical modelling. J. Manuf. Process. 2021, 65, 161–173. [Google Scholar] [CrossRef]
- Aghajani Derazkola, H.; Simchi, A.; Hamed Aghajani Derazkola, A.S. Experimental and thermomechanical analysis of the effect of tool pin profile on the friction stir welding of poly(methyl methacrylate) sheets. J. Manuf. Process. 2018, 34, 412–423. [Google Scholar] [CrossRef]
- Mark, J.E. Polymer Data Handbook; Oxford University Press: London, UK, 1999. [Google Scholar]
- Wypych, G. (Ed.) Handbook of Polymers; ChemTec Publishing: Ontario, CA, USA, 2012. [Google Scholar]
- Utracki, L.A. (Ed.) Polymer Blends Handbook; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2002; Volume 1. [Google Scholar]
- Rosato, V.D. (Ed.) Plastics Processing Data Handbook; Springer: New York, NY, USA, 1997. [Google Scholar]
- Talebizadehsardari, P.; Musharavati, F.; Khan, A.; Sebaey, T.A.; Eyvaziana, A.; Derazkola, H.A. Underwater friction stir welding of Al-Mg alloy: Thermo-mechanical modeling and validation. Mater. Today Commun. 2021, 26, 101965. [Google Scholar] [CrossRef]
- Aghajani Derazkola, H.; Kordani, N.; Aghajani Derazkola, H. Effects of friction stir welding tool tilt angle on properties of Al-Mg-Si alloy T-joint. CIRP J. Manuf. Sci. Technol. 2021, 33, 264–276. [Google Scholar] [CrossRef]
- Elyasi, M.; Derazkola, H.A. Experimental and thermomechanical study on FSW of PMMA polymer T-joint. Int. J. Adv. Manuf. Technol. 2018, 97, 1445–1456. [Google Scholar] [CrossRef]
- Aghajani Derazkola, H.; Khodabakhshi, F. Intermetallic compounds (IMCs) formation during dissimilar friction-stir welding of AA5005 aluminum alloy to St-52 steel: Numerical modeling and experimental study. Int. J. Adv. Manuf. Technol. 2019, 100, 2401–2422. [Google Scholar] [CrossRef]
- Memon, S.; Fydrych, D.; Fernandez, A.C.; Derazkola, H.A.; Derazkola, H.A. Effects of FSW Tool Plunge Depth on Properties of an Al-Mg-Si Alloy T-Joint: Thermomechanical Modeling and Experimental Evaluation. Materials 2021, 14, 4754. [Google Scholar] [CrossRef]
- Bokov, D.O.; Jawad, M.A.; Suksatan, W.; Abdullah, M.E.; Świerczyńska, A.; Fydrych, D.; Derazkola, H.A. Effect of Pin Shape on Thermal History of Aluminum-Steel Friction Stir Welded Joint: Computational Fluid Dynamic Modeling and Validation. Materials 2021, 14, 7883. [Google Scholar] [CrossRef]
- Inaniwa, S.; Kurabe, Y.; Miyashita, Y.; Hori, H. Application of Friction Stir Welding for Several Plastic Materials. In Proceedings of the 1st International Joint Symposium on Joining and Welding, Osaka, Japan, 6–8 November 2013; Fujii, H.B.T.-P., Ed.; Woodhead Publishing: Sawston, UK, 2013; pp. 137–142, ISBN 978-1-78242-163-4. [Google Scholar]
- Iwaszko, J.; Kudła, K. Evolution of Microstructure and Properties of Air-Cooled Friction-Stir-Processed 7075 Aluminum Alloy. Materials 2022, 15, 2633. [Google Scholar] [CrossRef]
- Śnieżek, L.; Kosturek, R.; Wachowski, M.; Kania, B. Microstructure and Residual Stresses of AA2519 Friction Stir Welded Joints under Different Heat Treatment Conditions. Materials 2020, 13, 834. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Omer, M.A.E.; Rashad, M.; Elsheikh, A.H.; Showaib, E.A. A review on friction stir welding of thermoplastic materials: Recent advances and progress. Weld. World 2022, 66, 1–25. [Google Scholar] [CrossRef]
- Griffiths, R.J.; Garcia, D.; Song, J.; Vasudevan, V.K.; Steiner, M.A.; Cai, W.; Yu, H.Z. Solid-state additive manufacturing of aluminum and copper using additive friction stir deposition: Process-microstructure linkages. Materialia 2021, 15, 100967. [Google Scholar] [CrossRef]
- Bagheri, B.; Abbasi, M.; Hamzeloo, R. The investigation into vibration effect on microstructure and mechanical characteristics of friction stir spot vibration welded aluminum: Simulation and experiment. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2020, 234, 1809–1822. [Google Scholar] [CrossRef]
- Memon, S.; Tomków, J.; Derazkola, H.A. Thermo-Mechanical Simulation of Underwater Friction Stir Welding of Low Carbon Steel. Materials 2021, 14, 4953. [Google Scholar] [CrossRef] [PubMed]
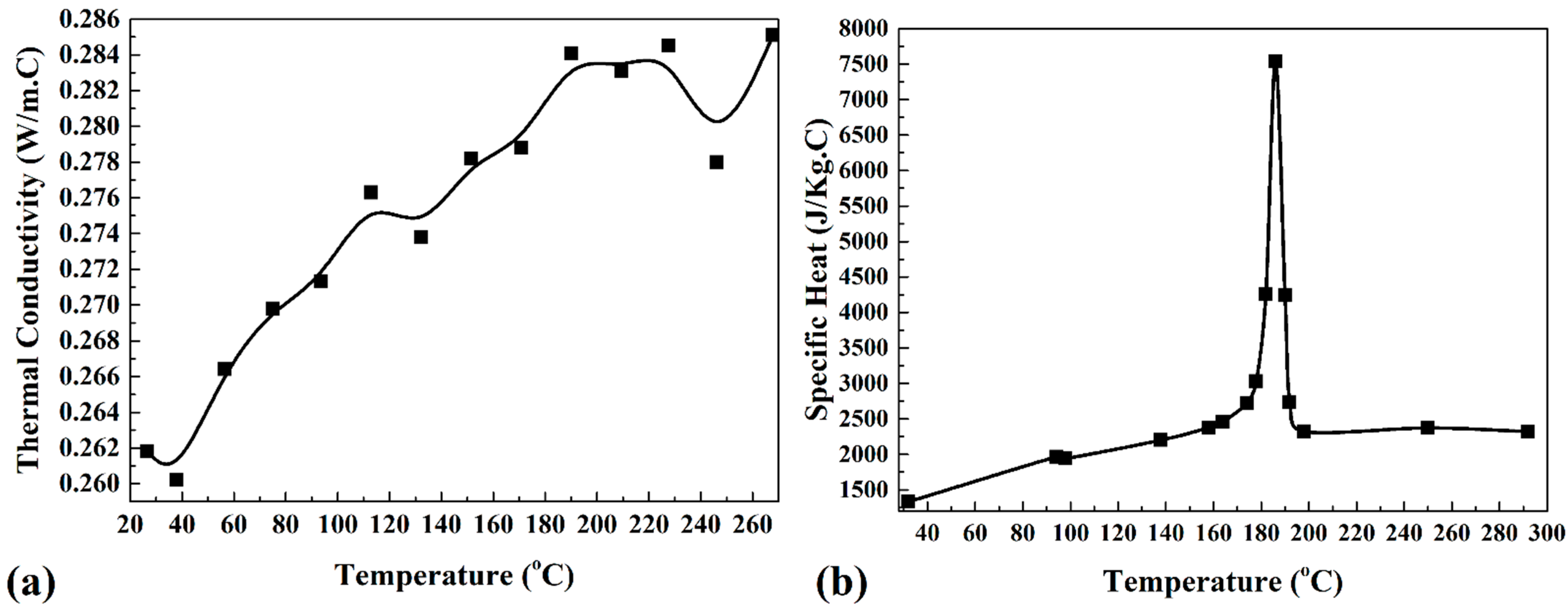
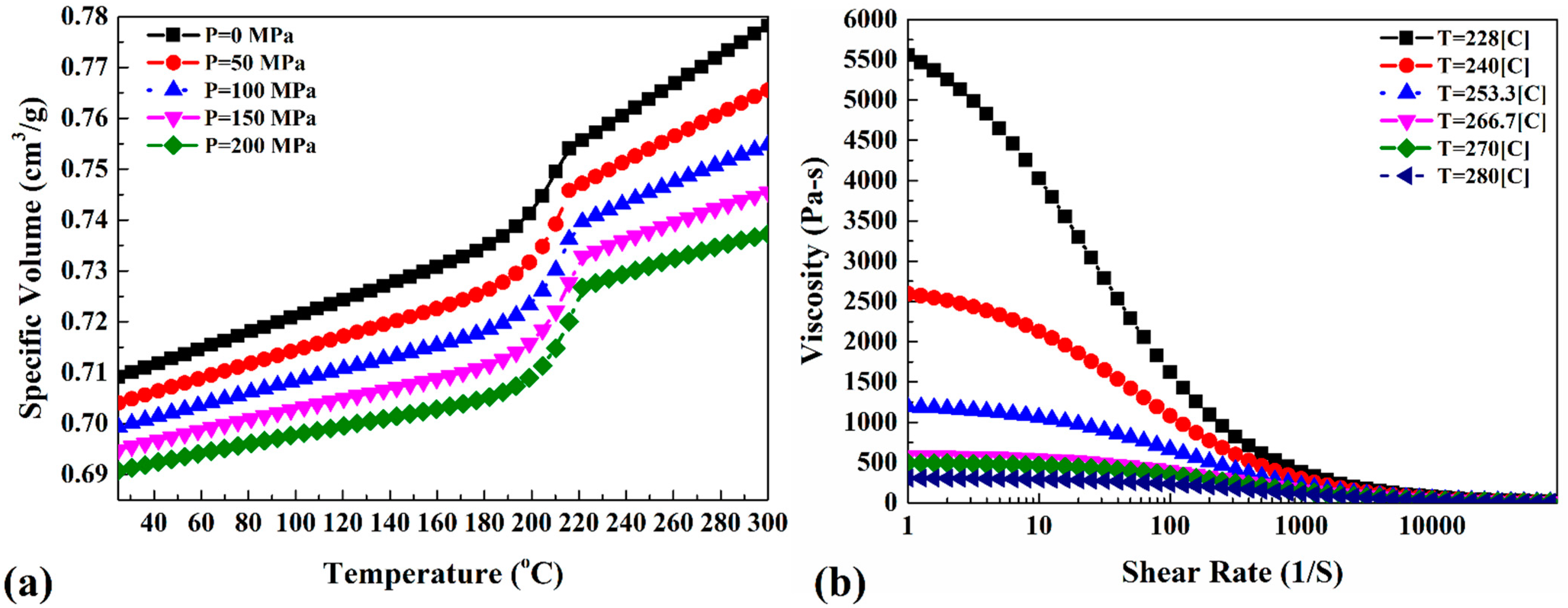
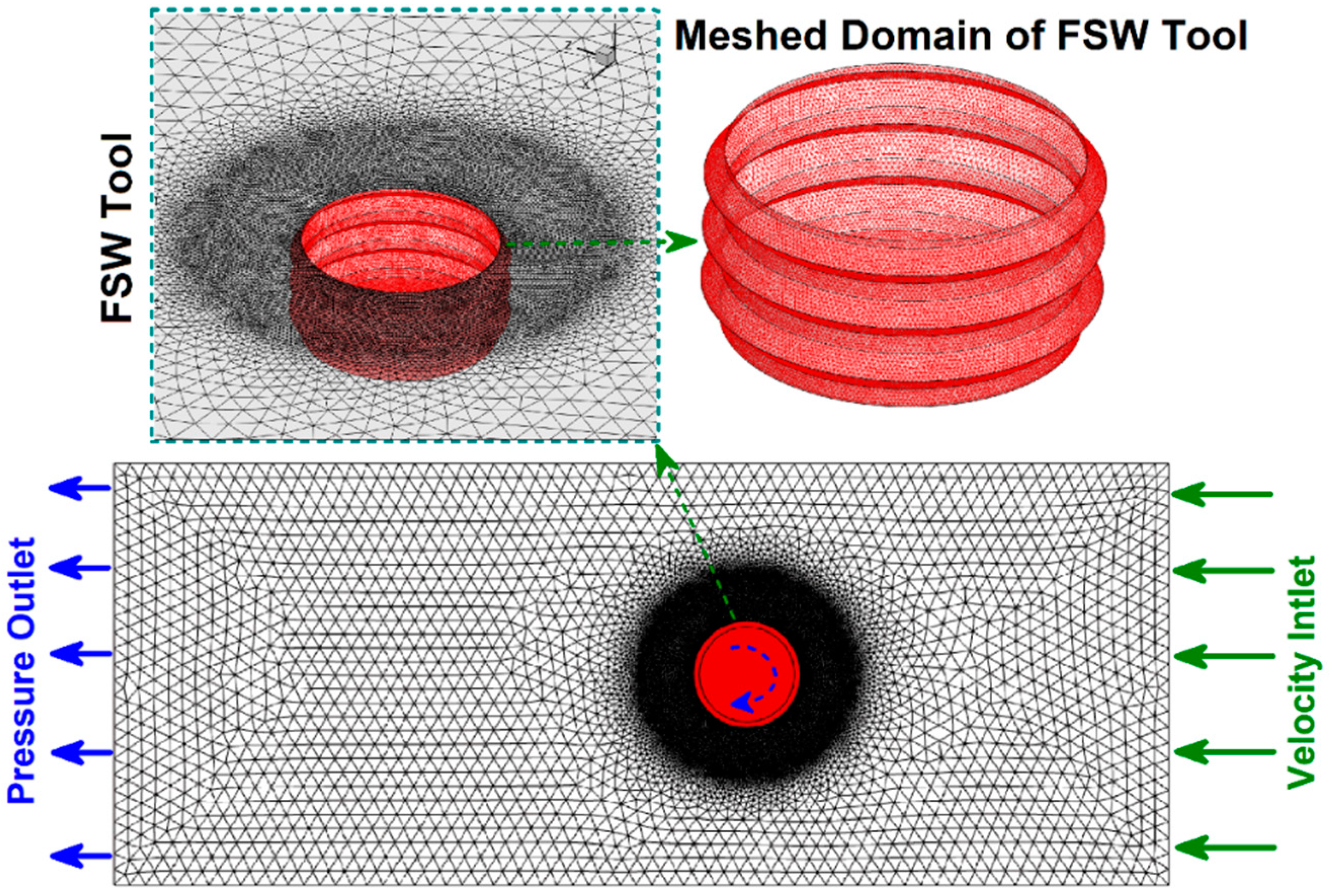


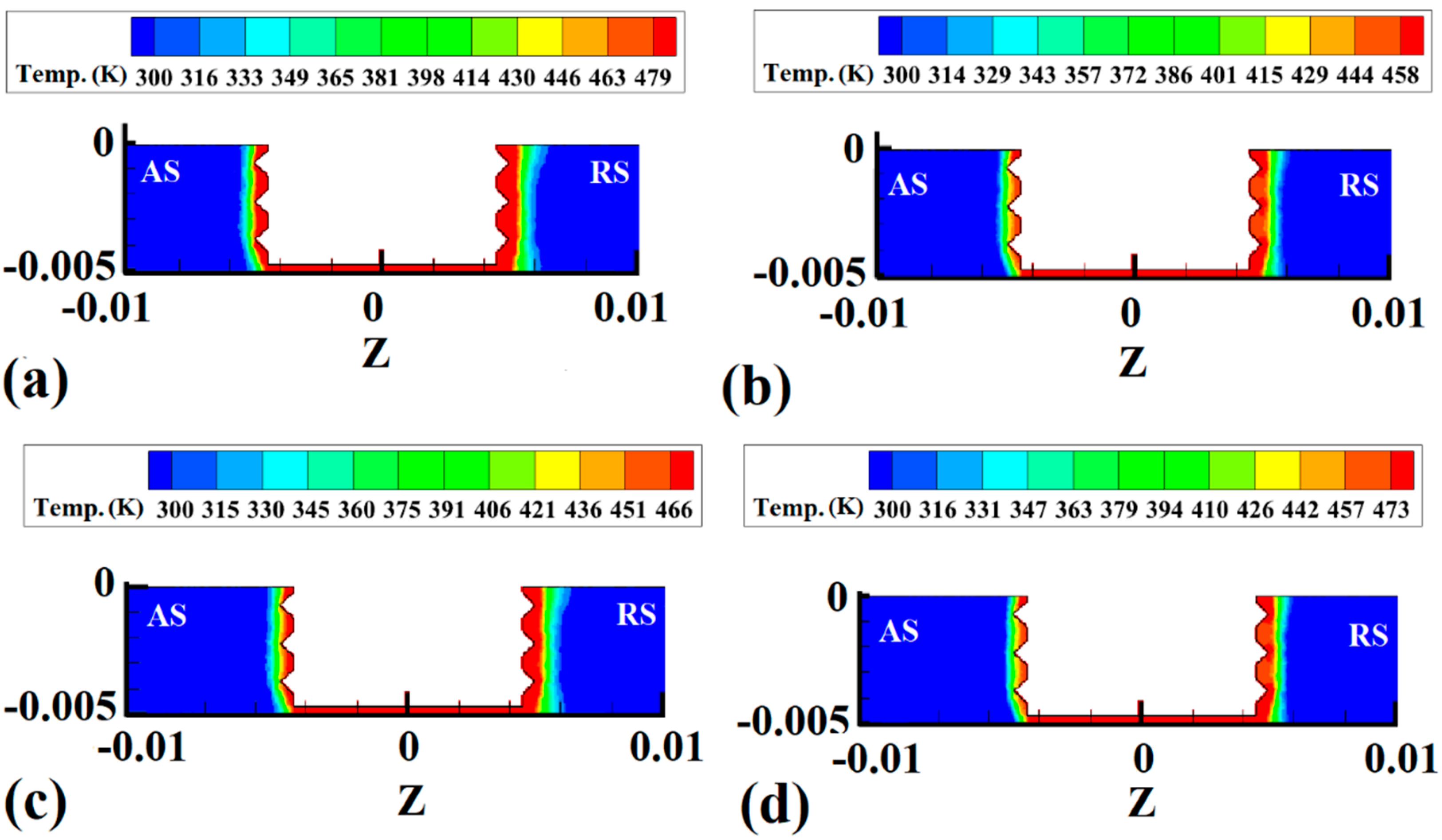

| Properties | Unit | Value |
|---|---|---|
| Young’s modulus | GPa | 2.63 |
| Tensile strength | MPa | 67.1 |
| Thermal conductivity | W/m·k | 0.21 |
| Glass transition temperature | °C | 50 |
| Melting temperature | °C | 225 |
| Crystallinity | % | 20–25 |
| Melt viscosity | Pa·s | 2 × 10 (230 °C) |
| Sample | Traverse Speed (mm/min) | Rotational Speed (rpm) |
|---|---|---|
| 1 | 40 | 440 |
| 2 | 50 | 380 |
| 3 | 50 | 440 |
| 4 | 50 | 500 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Sabur, R.; Khalaf, H.I.; Świerczyńska, A.; Rogalski, G.; Derazkola, H.A. Effects of Noncontact Shoulder Tool Velocities on Friction Stir Joining of Polyamide 6 (PA6). Materials 2022, 15, 4214. https://doi.org/10.3390/ma15124214
Al-Sabur R, Khalaf HI, Świerczyńska A, Rogalski G, Derazkola HA. Effects of Noncontact Shoulder Tool Velocities on Friction Stir Joining of Polyamide 6 (PA6). Materials. 2022; 15(12):4214. https://doi.org/10.3390/ma15124214
Chicago/Turabian StyleAl-Sabur, Raheem, Hassanein I. Khalaf, Aleksandra Świerczyńska, Grzegorz Rogalski, and Hesamoddin Aghajani Derazkola. 2022. "Effects of Noncontact Shoulder Tool Velocities on Friction Stir Joining of Polyamide 6 (PA6)" Materials 15, no. 12: 4214. https://doi.org/10.3390/ma15124214
APA StyleAl-Sabur, R., Khalaf, H. I., Świerczyńska, A., Rogalski, G., & Derazkola, H. A. (2022). Effects of Noncontact Shoulder Tool Velocities on Friction Stir Joining of Polyamide 6 (PA6). Materials, 15(12), 4214. https://doi.org/10.3390/ma15124214







