Model of the Temperature Influence on Additively Manufactured Carbon Fibre Reinforced Polymer Samples with Embedded Fibre Bragg Grating Sensors
Abstract
:1. Introduction
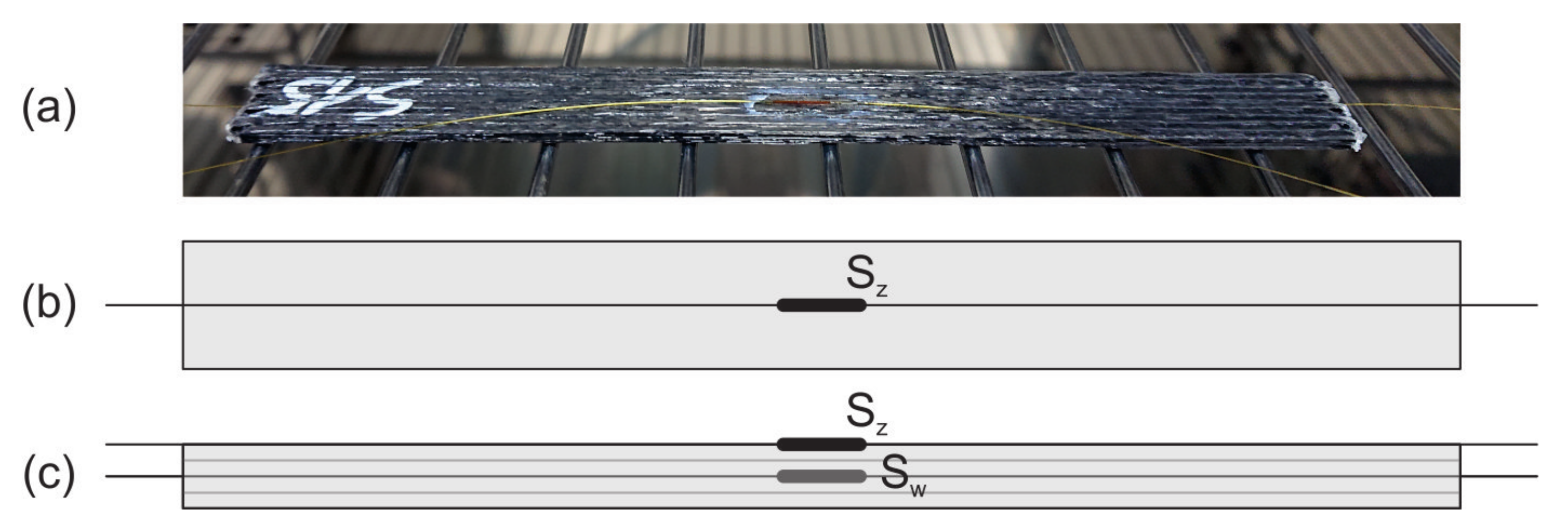
2. Samples
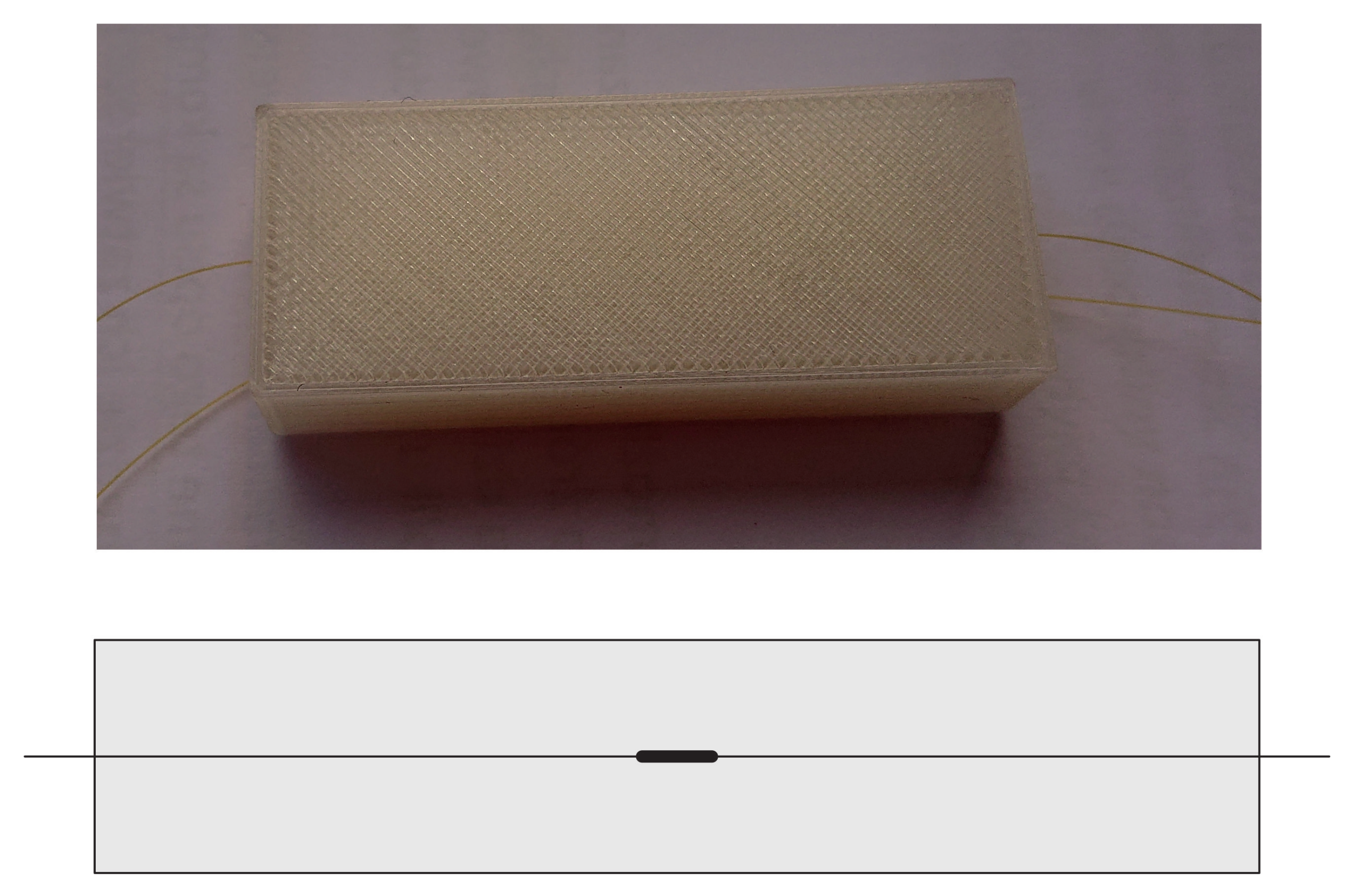
2.1. Manufacturing Method
2.2. Sample
3. Experimental and Numerical Investigation
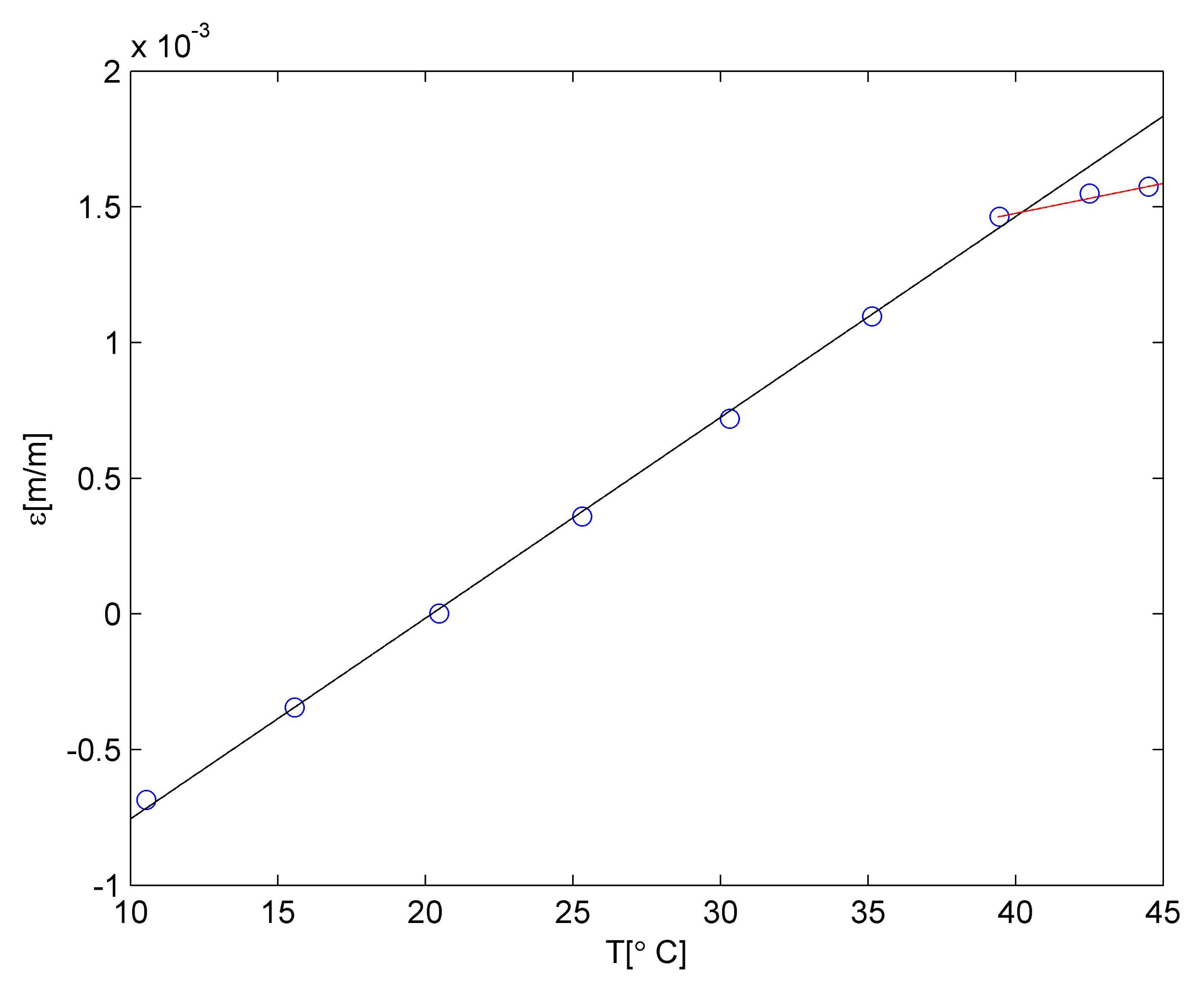
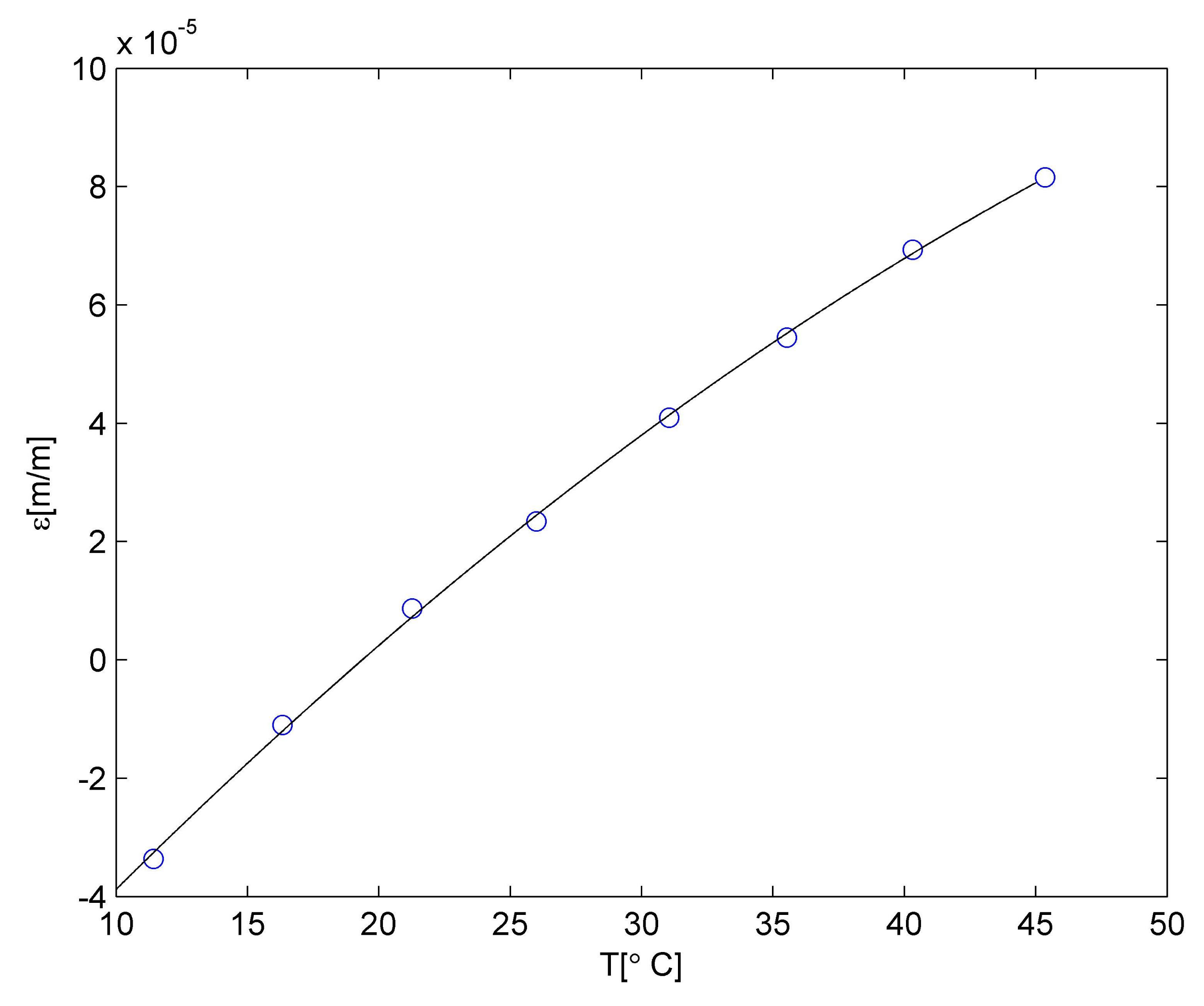
3.1. Coefficient of Thermal Expansion of PLA
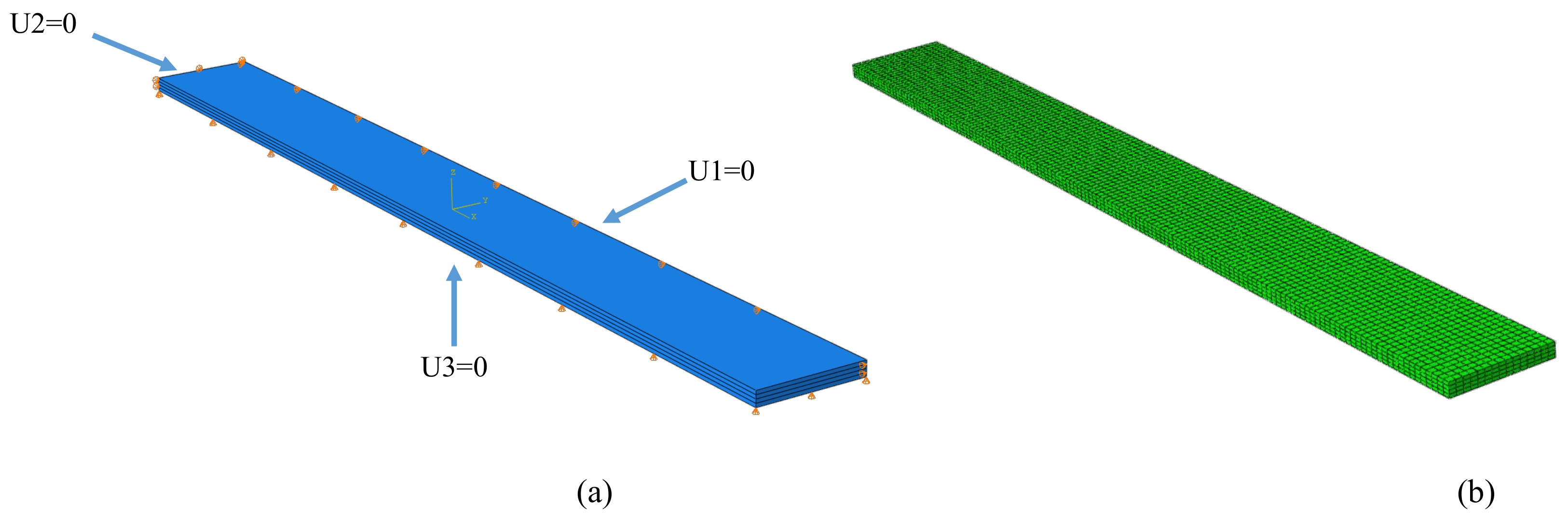
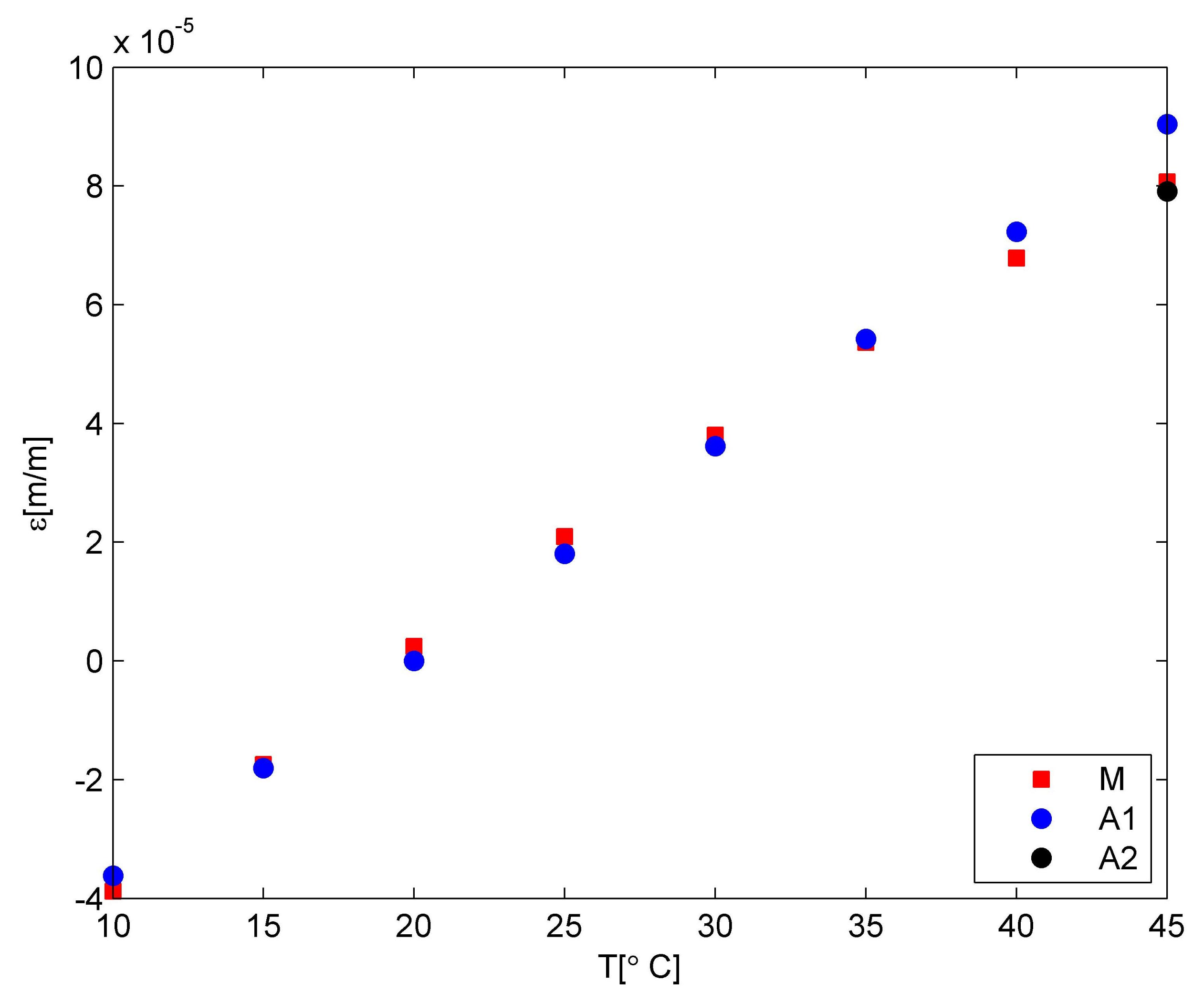
3.2. Numerical Calculation
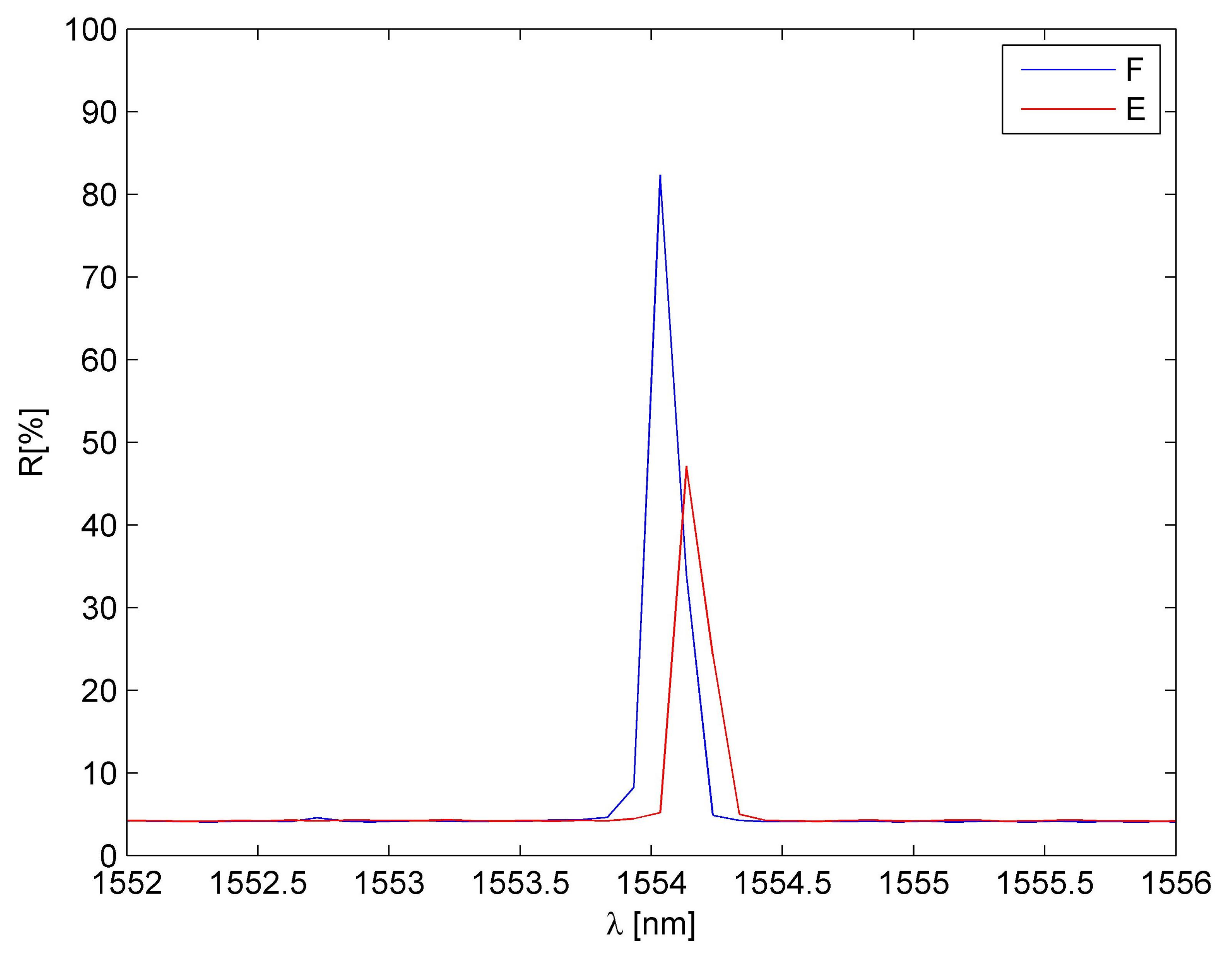
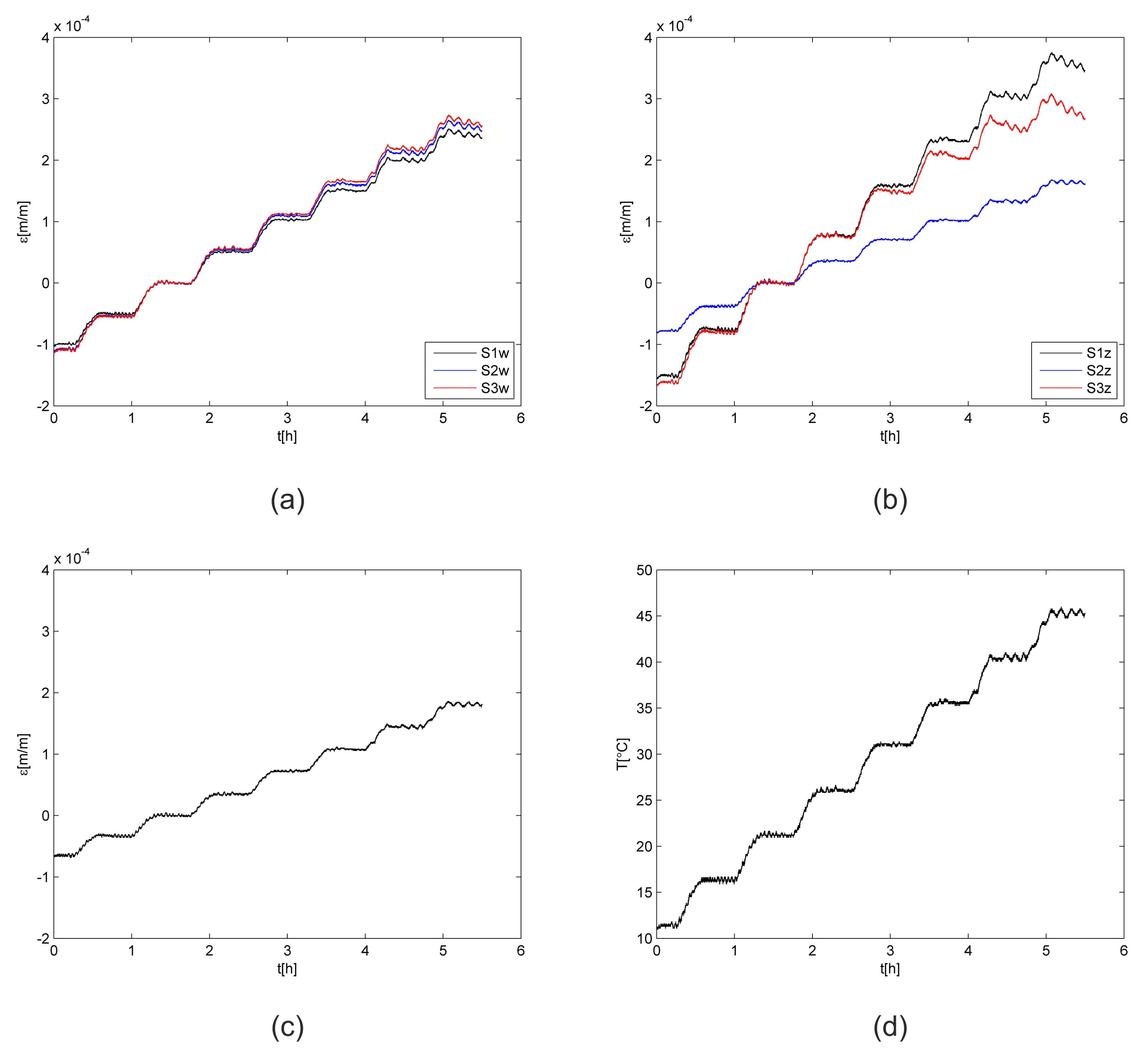
3.3. Experimental Investigation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mieloszyk, M.; Andrearczyk, A.; Majewska, K.; Jurek, M.; Ostachowicz, W. Polymeric structure with embedded fiber Bragg grating sensor manufactured using multi-jet printing method. Measurement 2020, 166, 108229. [Google Scholar] [CrossRef]
- Liu, Z.; Jiang, Q.; Ning, F.; Kim, H.; Cong, W.; Xu, C.; Zhang, H. Investigation of Energy Requirements and Environmental Performance for Additive Manufacturing Processes. Sustainability 2018, 10, 2018. [Google Scholar] [CrossRef] [Green Version]
- Shafighfard, T.; Cender, T.A.; Demir, E. Additive manufacturing of compliance optimized variable stiffness composites through short fiber alignment along curvilinear paths. Addit. Manuf. 2020, 37, 101728. [Google Scholar] [CrossRef]
- Cardeal, G.; Sequeira, D.; Mendonça, J.; Leite, M.; Ribeiro, I. Additive manufacturing in the process industry: A process-based cost model to study life cycle cost and the viability of additive manufacturing spare parts. Procedia CIRP 2021, 98, 211–216. [Google Scholar] [CrossRef]
- Shafighfard, T.; Demir, E.; Yildiz, M. Design of fiber-reinforced variable-stiffness composites for different open-hole geometries with fiber continuity and curvature constraints. Compos. Struct. 2019, 226, 111280. [Google Scholar] [CrossRef]
- Munghen, D.; Iacobellis, V.; Behdinan, K. Incorporation of fiber Bragg grating sensors in additive manufactured Acrylonitrile butadiene styrene for strain monitoring during fatigue loading. Int. J. Fatigue 2022, 154, 106485. [Google Scholar] [CrossRef]
- Minakuchi, S.; Takeda, N.; Takeda, S.I.; Nagao, Y.; Franceschetti, A.; Liu, X. Life cycle monitoring of large-scale CFRP VARTM structure by fiber-optic-based distributed sensing. Compos. Part A Appl. Sci. Manuf. 2011, 42, 669–676. [Google Scholar] [CrossRef]
- Kinet, D.; Mégret, P.; Goossen, K.W.; Qiu, L.; Heider, D.; Caucheteur, C. Fiber Bragg grating sensors toward structural health monitoring in composite materials: Challenges and solutions. Sensors 2014, 14, 7394–7419. [Google Scholar] [CrossRef]
- Matveenko, V.; Kosheleva, N.; Serovaev, G.; Fedorov, A. Analysis of Reliability of Strain Measurements Made with the Fiber Bragg Grating Sensor Rosettes Embedded in a Polymer Composite Material. Sensors 2021, 21, 5050. [Google Scholar] [CrossRef]
- Mieloszyk, M.; Majewska, K.; Ostachowicz, W. Application of embedded fibre Bragg grating sensors for structural health monitoring of complex composite structures for marine applications. Mar. Struct. 2021, 76, 102903. [Google Scholar] [CrossRef]
- Basu, M.; Ghorai, S. Sequential interrogation of multiple FBG sensors using LPG modulation and an artificial neural network. Meas. Sci. Technol. 2015, 26, 045104. [Google Scholar] [CrossRef]
- Leng, J.; Asundi, A. Structural health monitoring of smart composite materials by using EFPI and FBG sensors. Sens. Actuators A Phys. 2003, 103, 330–340. [Google Scholar] [CrossRef]
- Quan, Z.; Wu, A.; Keefe, M.; Qin, X.; Yu, J.; Suhr, J.; Byun, J.H.; Kim, B.S.; Chou, T.W. Additive manufacturing of multi-directional preforms for composites: Opportunities and challenges. Mater. Today 2015, 18, 503–512. [Google Scholar] [CrossRef]
- Mieloszyk, M. Fatigue Crack Propagation Monitoring Using Fibre Bragg Grating Sensors. Vibration 2021, 4, 700–721. [Google Scholar] [CrossRef]
- Chen, J.; Wang, J.; Li, X.; Sun, L.; Li, S.; Ding, A. Monitoring of temperature and cure-induced strain gradient in laminated composite plate with FBG sensors. Compos. Struct. 2020, 242, 112168. [Google Scholar] [CrossRef]
- Arena, M.; Viscardi, M. Strain state detection in composite structures: Review and new challenges. J. Compos. Sci. 2020, 4, 60. [Google Scholar] [CrossRef]
- Lau, K. Structural health monitoring for smart composites using embedded FBG sensor technology. Mater. Sci. Technol. 2014, 30, 1642–1654. [Google Scholar] [CrossRef]
- Oromiehie, E.; Prusty, B.G.; Compston, P.; Rajan, G. Characterization of process-induced defects in automated fiber placement manufacturing of composites using fiber Bragg grating sensors. Struct. Health Monit. 2018, 17, 108–117. [Google Scholar] [CrossRef]
- Homa, D.; Hill, C.; Floyd, A.; Pickrell, G.; Hall, H. Fiber Bragg gratings embedded in 3D printed prototypes. Sci. Adv. Today 2016, 2, 25242. [Google Scholar]
- Mieloszyk, M.; Majewska, K.; Andrearczyk, A. Influence of Temperature on Additive Manufacturing Polymer Structure with Embedded Fibre Bragg Grating Sensors. In European Workshop on Structural Health Monitoring; Springer: Berlin/Heidelberg, Germany, 2020; pp. 679–686. [Google Scholar]
- Kousiatza, C.; Karalekas, D. In-situ monitoring of strain and temperature distributions during fused deposition modeling process. Mater. Des. 2016, 97, 400–406. [Google Scholar] [CrossRef]
- Economidou, S.N.; Karalekas, D. Optical sensor-based measurements of thermal expansion coefficient in additive manufacturing. Polym. Test. 2016, 51, 117–121. [Google Scholar] [CrossRef]
- Zhong, W.; Li, F.; Zhang, Z.; Song, L.; Li, Z. Short fiber reinforced composites for fused deposition modeling. Mater. Sci. Eng. A 2001, 301, 125–130. [Google Scholar] [CrossRef]
- Ning, F.; Cong, W.; Qiu, J.; Wei, J.; Wang, S. Additive manufacturing of carbon fiber reinforced thermoplastic composites using fused deposition modeling. Compos. Part B Eng. 2015, 80, 369–378. [Google Scholar] [CrossRef]
- Ibrahim, Y.; Melenka, G.W.; Kempers, R. Fabrication and tensile testing of 3D printed continuous wire polymer composites. Rapid Prototyp. J. 2018, 24, 1131–1141. [Google Scholar] [CrossRef]
- Dugbenoo, E.; Arif, M.F.; Wardle, B.L.; Kumar, S. Enhanced Bonding via Additive Manufacturing-Enabled Surface Tailoring of 3D Printed Continuous-Fiber Composites. Adv. Eng. Mater. 2018, 20, 1800691. [Google Scholar] [CrossRef]
- Chacón, J.; Caminero, M.; Núñez, P.; García-Plaza, E.; García-Moreno, I.; Reverte, J. Additive manufacturing of continuous fibre reinforced thermoplastic composites using fused deposition modelling: Effect of process parameters on mechanical properties. Compos. Sci. Technol. 2019, 181, 107688. [Google Scholar] [CrossRef]
- Kabir, S.F.; Mathur, K.; Seyam, A.F.M. A critical review on 3D printed continuous fiber-reinforced composites: History, mechanism, materials and properties. Compos. Struct. 2020, 232, 111476. [Google Scholar] [CrossRef]
- Syrlybayev, D.; Zharylkassyn, B.; Seisekulova, A.; Akhmetov, M.; Perveen, A.; Talamona, D. Optimisation of Strength Properties of FDM Printed Parts—A Critical Review. Polymers 2021, 13, 1587. [Google Scholar] [CrossRef]
- Wickramasinghe, S.; Do, T.; Tran, P. FDM-based 3D printing of polymer and associated composite: A review on mechanical properties, defects and treatments. Polymers 2020, 12, 1529. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Feng, Z.; Hao, L.; Huang, L.; Xin, C.; Wang, Y.; Bilotti, E.; Essa, K.; Zhang, H.; Li, Z.; et al. A review on functionally graded materials and structures via additive manufacturing: From multi-scale design to versatile functional properties. Adv. Mater. Technol. 2020, 5, 1900981. [Google Scholar] [CrossRef]
- Guessasma, S.; Belhabib, S.; Nouri, H. Effect of printing temperature on microstructure, thermal behavior and tensile properties of 3D printed nylon using fused deposition modeling. J. Appl. Polym. Sci. 2021, 138, 50162. [Google Scholar] [CrossRef]
- Paul, S. Finite Element Analysis in Fused Deposition Modeling Research: A Literature Review. Measurement 2021, 178, 109320. [Google Scholar] [CrossRef]
- Economidou, S.N.; Karalekas, D. Characterization of fused deposition modeling polymeric structures using embedded fiber Bragg grating sensors. In Additive Manufacturing; Elsevier: Amsterdam, The Netherlands, 2018; pp. 163–180. [Google Scholar]
- Kantaros, A.; Karalekas, D. FBG based in situ characterization of residual strains in FDM process. In Residual Stress, Thermomechanics & Infrared Imaging, Hybrid Techniques and Inverse Problems; Springer: Berlin/Heidelberg, Germany, 2014; Volume 8, pp. 333–337. [Google Scholar]
- Kousiatza, C.; Tzetzis, D.; Karalekas, D. In-situ characterization of 3D printed continuous fiber reinforced composites: A methodological study using fiber Bragg grating sensors. Compos. Sci. Technol. 2019, 174, 134–141. [Google Scholar] [CrossRef]
- Mekid, S.; Daraghma, H. Manufacturing Processes of Sensorial Materials: Sensors Placement and Experimental Validation. Iop Conf. Ser. Mater. Sci. Eng. 2019, 538, 012014. [Google Scholar] [CrossRef] [Green Version]
- Hong, C.; Bao, C.; Fei, J.; Zhang, Y.; Wang, X. Application of FBG Technology in Additive Manufacturing: Monitoring Real-time Internal Temperature of Products. IEEE Sens. J. 2020, 21, 6003–6011. [Google Scholar] [CrossRef]
- Kousiatza, C.; Karalekas, D. Experimental study of fabrication-induced residual strains and distortions in polymeric square plates built using fused deposition modeling process. Mater. Des. Process. Commun. 2021, 3, e149. [Google Scholar] [CrossRef] [Green Version]
- Muna, I.I.; Mieloszyk, M. Temperature Influence on Additive Manufactured Carbon Fiber Reinforced Polymer Composites. Materials 2021, 14, 6413. [Google Scholar] [CrossRef] [PubMed]
- Rimašauskas, M.; Kuncius, T.; Rimašauskienė, R. Processing of carbon fiber for 3D printed continuous composite structures. Mater. Manuf. Process. 2019, 34, 1528–1536. [Google Scholar] [CrossRef]
- Cristea, M.; Ionita, D.; Iftime, M.M. Dynamic mechanical analysis investigations of pla-based renewable materials: How are they useful? Materials 2020, 13, 5302. [Google Scholar] [CrossRef]
- ABAQUS/Standard User’s Manual; Version 6.14; Dassault Systèmes Simulia Corp: Providence, RI, USA, 2017.
| Speed | Temperature | Extrusion | ||||
|---|---|---|---|---|---|---|
| First Layer [mm/s] | Printing [mm/s] | Fan [%] | Extruder [°] | Bed [°] | Multiplier [mm] | Width [mm] |
| 1.20 | 4 | 50/80/100 | 200 | 70 | 0.6 | 1.6 |
| Sample | Layer | Stacking | |||
|---|---|---|---|---|---|
| Lenght [mm] | Width [mm] | Tickness [mm] | Tickness [mm] | Number | Sequence |
| 150 | 15 | 2 | 0.5 | 4 | [0, 0] s |
| E [GPa] | ρ [g/cm3] | ν | Xt [MPa] | v [%] | κ [W/mK] | C [J/kgK] | |
|---|---|---|---|---|---|---|---|
| Fibre | 230 | 176 | 0.33 | 3530 | 18 | 10.46 | 794 |
| Matrix | 2.315 | 1.24 | 0.29 | 51 | 82 | 0.13 | 1800 |
| First Approximation (× 10−5) | Second Approximation (× 10−5) | |
|---|---|---|
| P1 | 7.40 | −149.55 |
| P2 | 2.20 | 59.58 |
| line in Figure 4 | black | red |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shafighfard, T.; Mieloszyk, M. Model of the Temperature Influence on Additively Manufactured Carbon Fibre Reinforced Polymer Samples with Embedded Fibre Bragg Grating Sensors. Materials 2022, 15, 222. https://doi.org/10.3390/ma15010222
Shafighfard T, Mieloszyk M. Model of the Temperature Influence on Additively Manufactured Carbon Fibre Reinforced Polymer Samples with Embedded Fibre Bragg Grating Sensors. Materials. 2022; 15(1):222. https://doi.org/10.3390/ma15010222
Chicago/Turabian StyleShafighfard, Torkan, and Magdalena Mieloszyk. 2022. "Model of the Temperature Influence on Additively Manufactured Carbon Fibre Reinforced Polymer Samples with Embedded Fibre Bragg Grating Sensors" Materials 15, no. 1: 222. https://doi.org/10.3390/ma15010222
APA StyleShafighfard, T., & Mieloszyk, M. (2022). Model of the Temperature Influence on Additively Manufactured Carbon Fibre Reinforced Polymer Samples with Embedded Fibre Bragg Grating Sensors. Materials, 15(1), 222. https://doi.org/10.3390/ma15010222






