Extrusion-Based 3D Printing of Ceramic Pastes: Mathematical Modeling and In Situ Shaping Retention Approach
Abstract
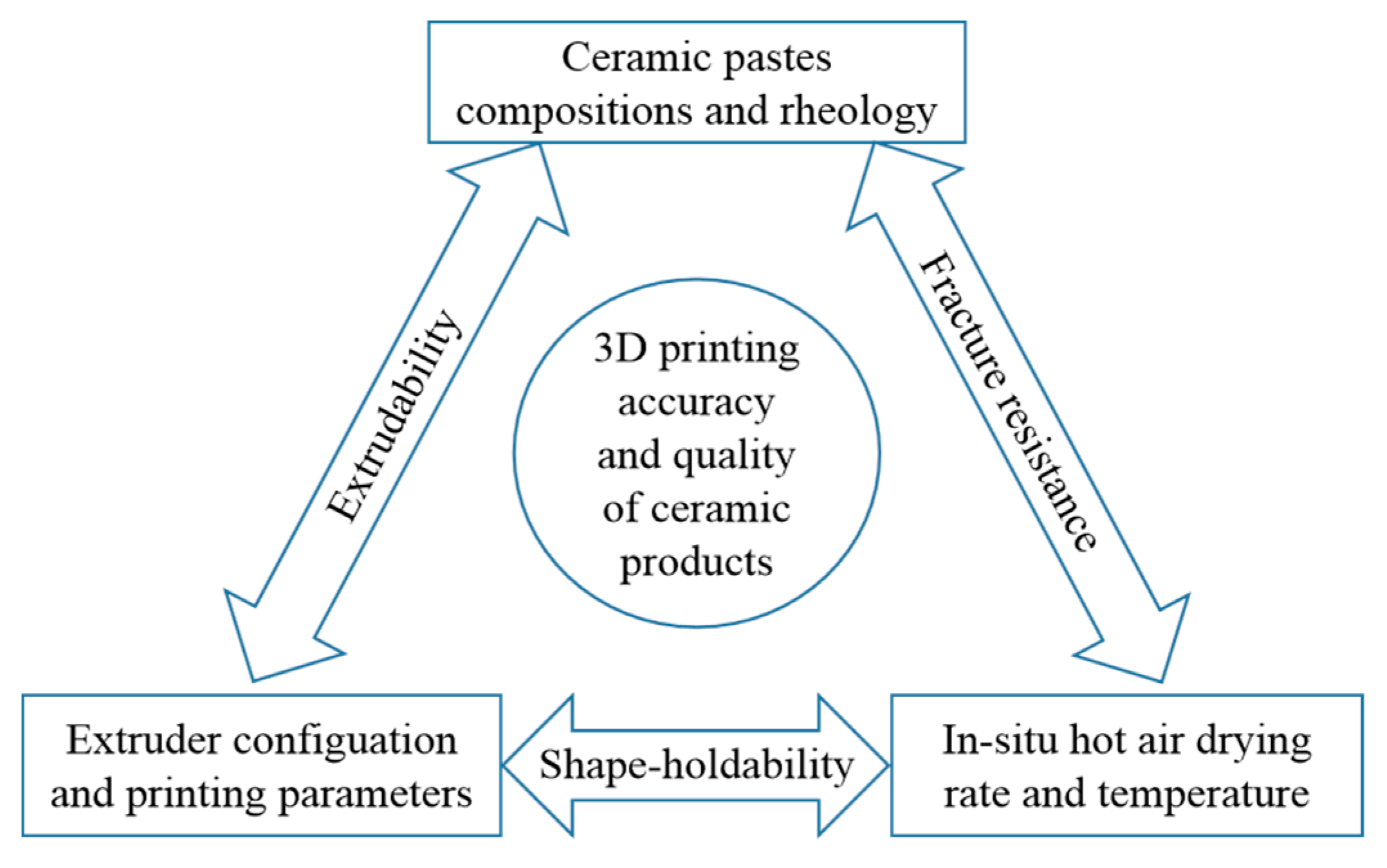
1. Introduction
2. Theoretical Complexity and Enabling Schemes of Extrusion-Based 3D-Printing
2.1. Complexity of Theoretical Modeling for Extrusion-Based 3D-Printing Process
2.2. Technical Solutions for Extrusion-Based 3D-Printing Process
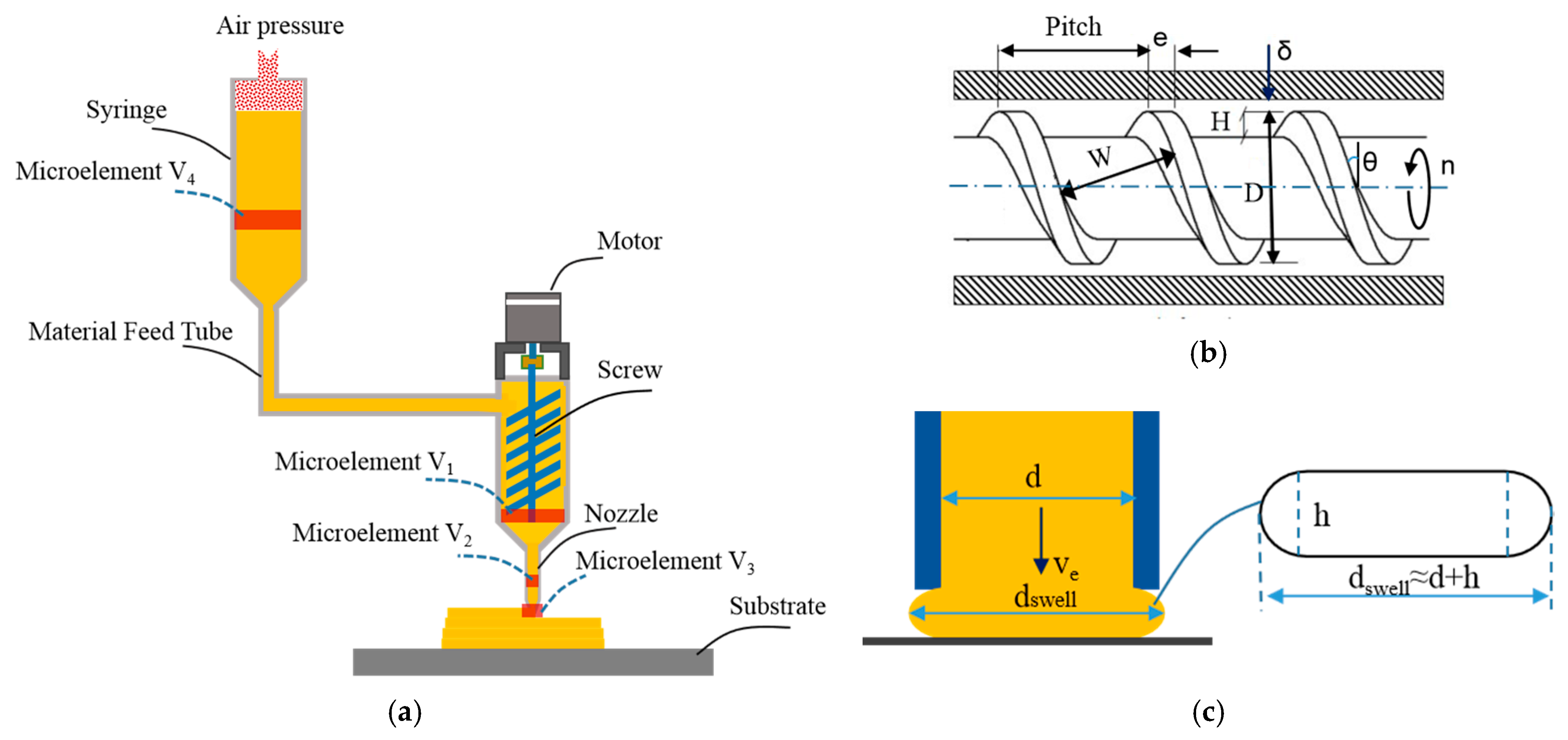
3. Mathematical Representations of Extrusion-Based 3D-Printing Process
4. Limitations of Extrusion-Based 3D-Printing without Shape-Holding Strategies
4.1. Experimental Conditions and Data Preparation
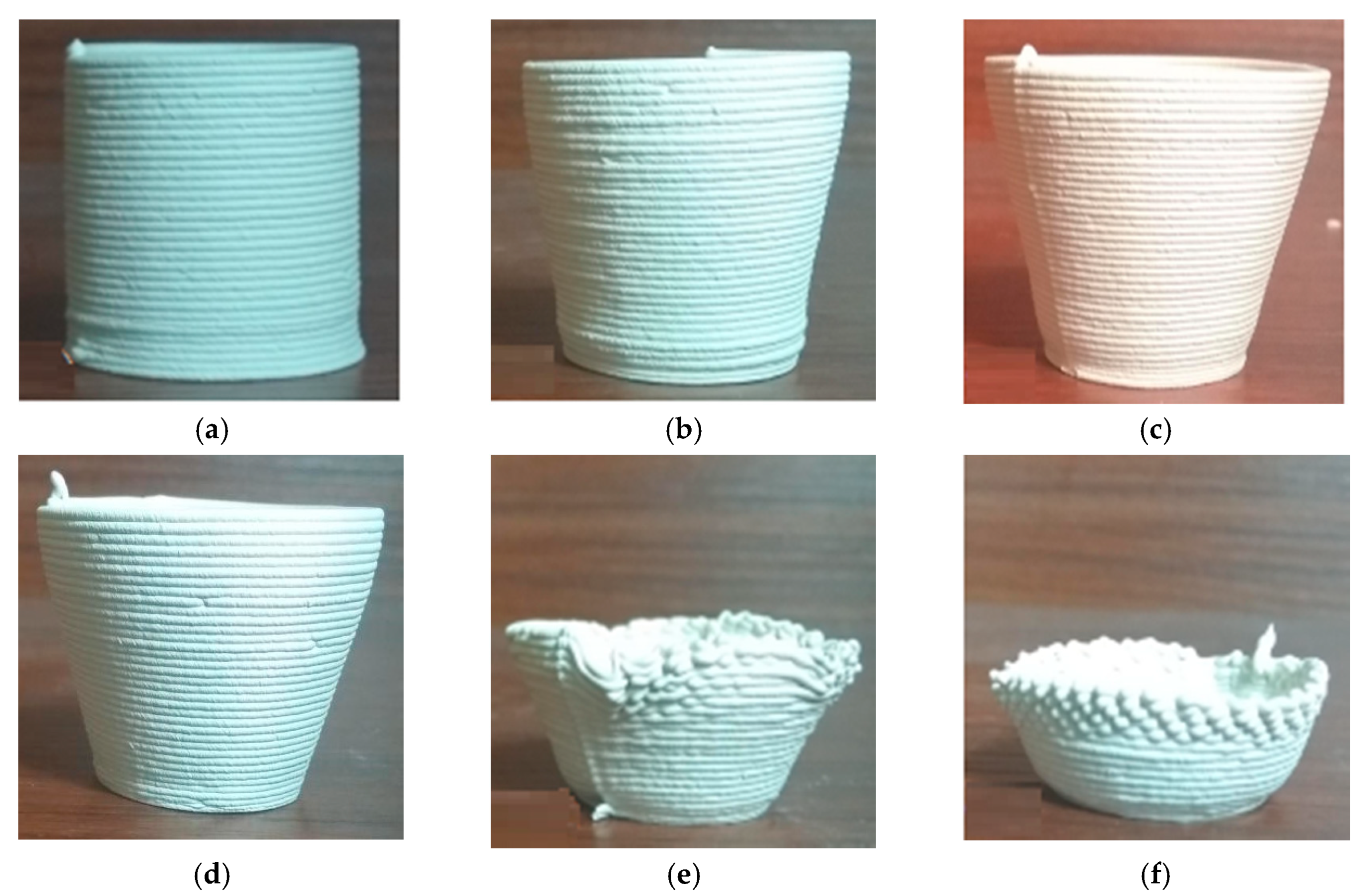
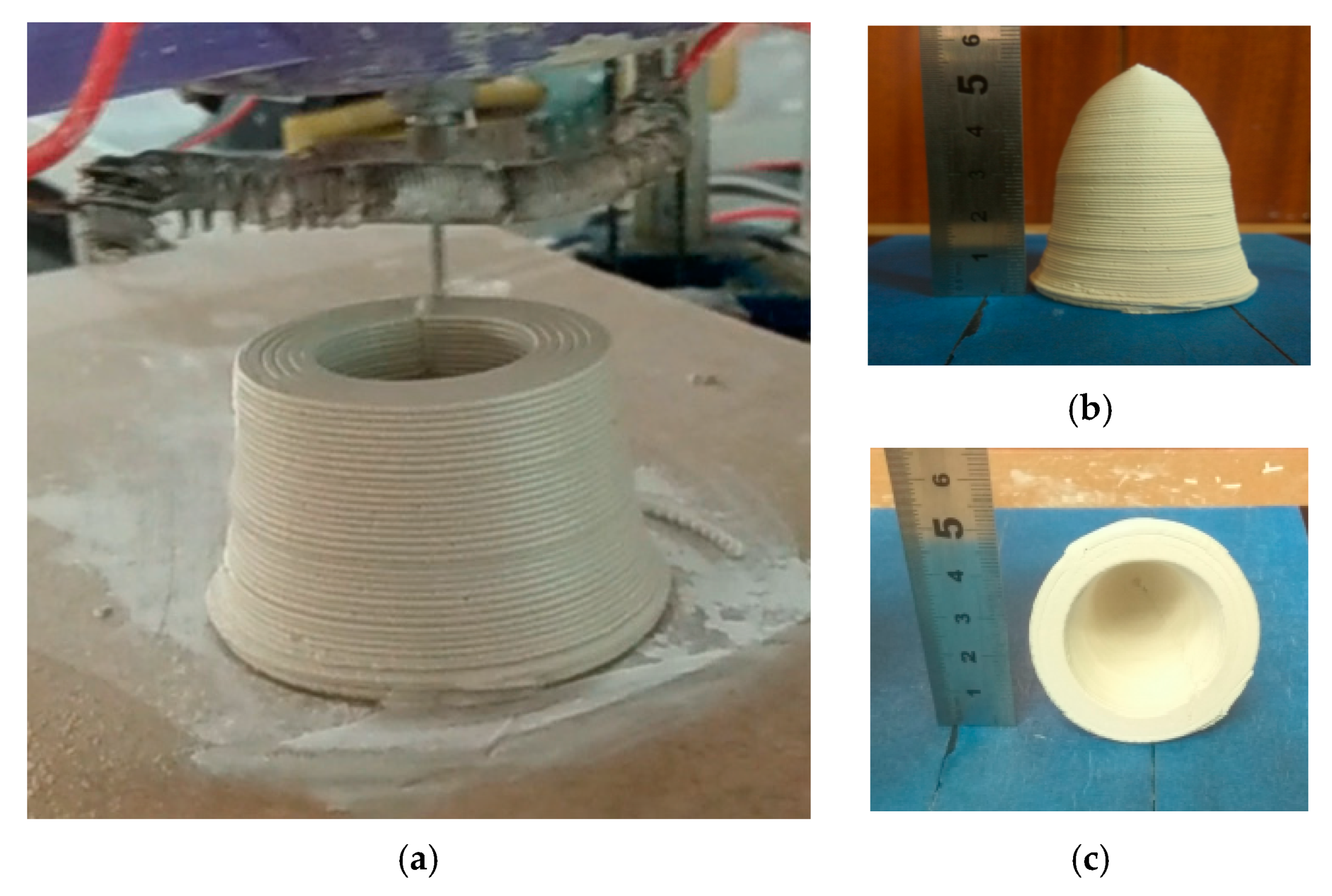
4.2. Weakness of Shape-Retention Ability
4.3. Crack Formation Due to Nonuniform Drying
5. Extrusion-Based 3D-Printing Method with In Situ Hot Air Flow Drying
5.1. Drying Kinetics of Porous Materials
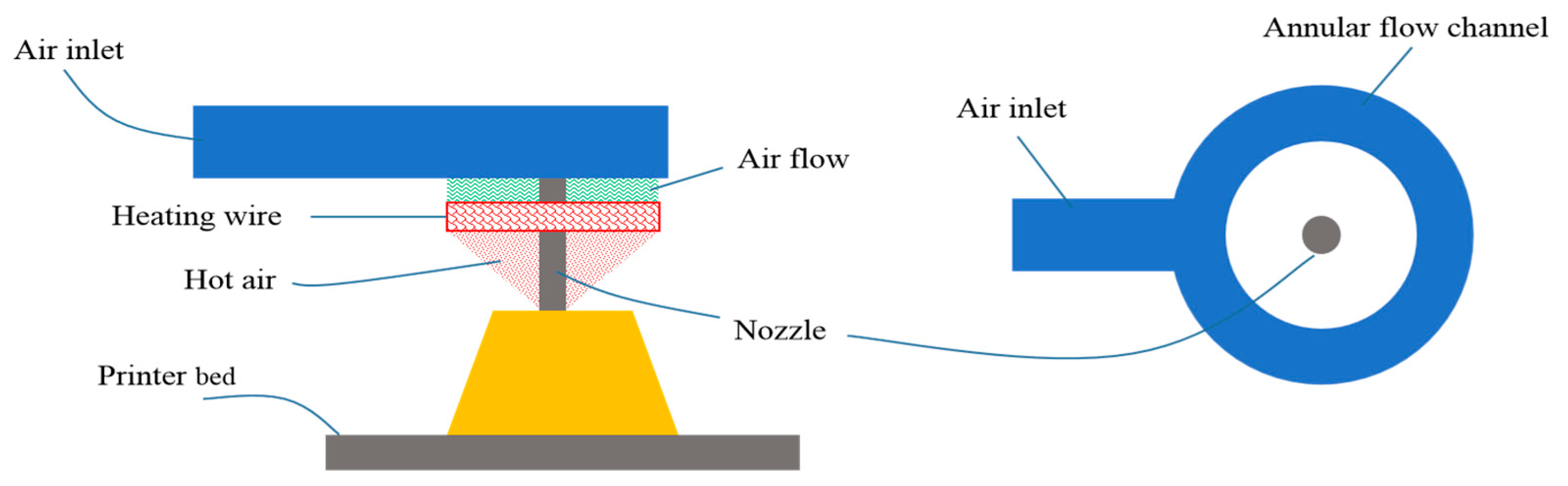
5.2. In Situ Hot Air Flow Drying Device
- The in situ hot air flow drying device is easy to install on the existing 3D printer without any big revamp.
- The heating capacity of this in situ hot air flow drying device can be numerically controlled by programming the speed of fan and the temperature of heating wire. Therefore, this device can partly adapt to different solids loading pastes or different shaping processes [40].
- The main components of this device, i.e., the annular flow channel, can be easily fabricated by an FDM-type printer.
5.3. Synthesis Framework of Full 3D Printability
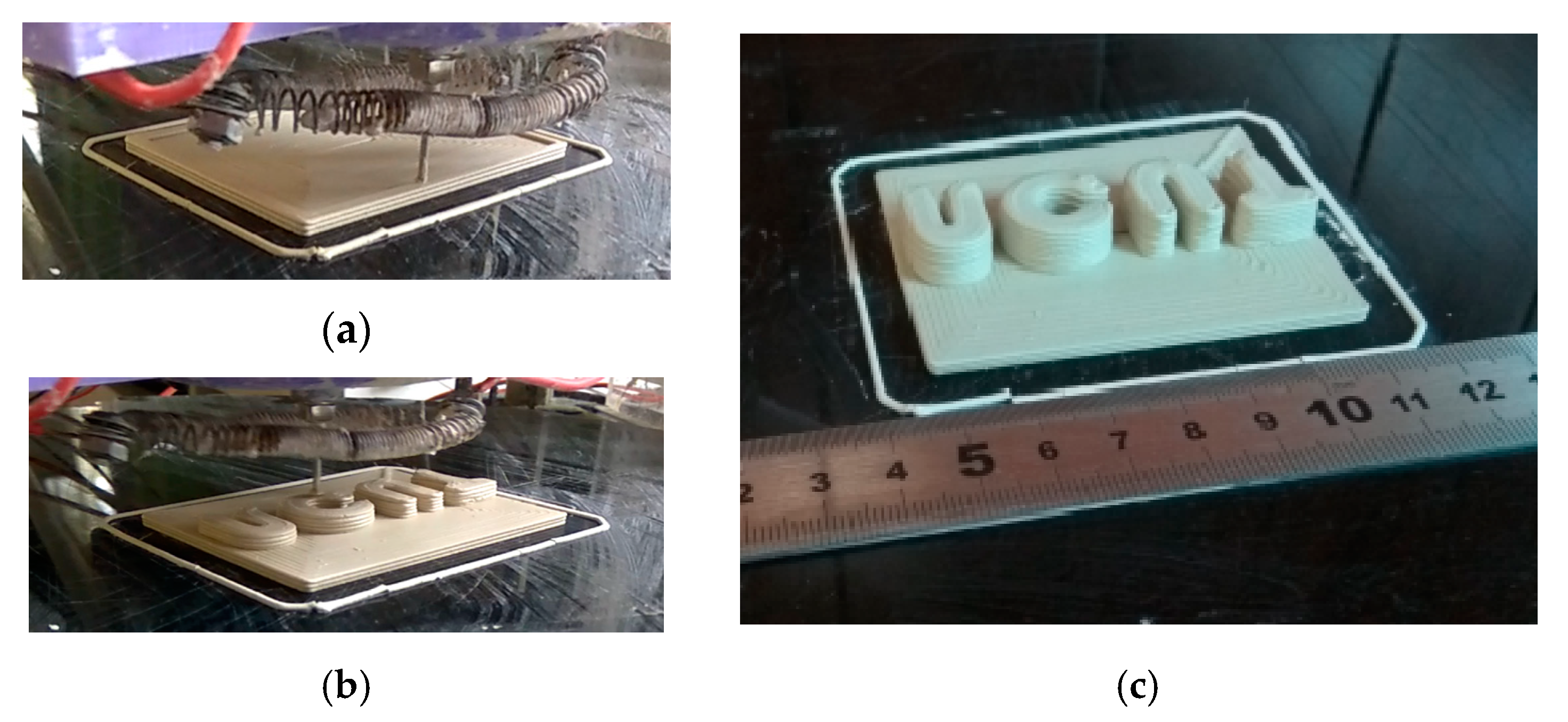
5.4. Verification of In Situ Hot Air Flow Drying Method
5.5. Shaping Capacity and Accuracy
5.6. Comparison with Other Shape-Holding Methods
6. Sintering Experiment of 3D-Printed Ceramic Samples
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| ABEF | Aqueous-Based Extrusion Fabrication |
| AM | Additive Manufacturing |
| CODE | Ceramic On-Demand Extrusion |
| DED | Direct Energy Deposition |
| DIW | Direct Ink Writing |
| FDC | Fused Deposition of Ceramics |
| FDM | Fused Deposition Modeling |
| FEF | Freeze-Form Extrusion Fabrication |
| ME | Material Extrusion |
| RC | Robocasting |
| SLM | Selective Laser Melting |
| LPM | Liquid Phase Migration |
References
- Paoletti, I. Mass Customization with Additive Manufacturing: New Perspectives for Multi Performative Building Components in Architecture. Procedia Eng. 2017, 180, 1150–1159. [Google Scholar] [CrossRef]
- Hu, F.; Cheng, J.; He, Y. Interactive design for additive manufacturing: A creative case of synchronous belt drive. Int. J. Interact. Des. Manuf. (IJIDeM) 2018, 12, 889–901. [Google Scholar] [CrossRef]
- International Organization for Standardization. Additive Manufacturing-General Principles Part 2: Overview of Process Categories and Feedstock. Available online: https://www.iso.org/standard/61626.html.2015 (accessed on 24 February 2021).
- Deckers, J.; Vleugels, J.; Kruth, J.P. Additive manufacturing of ceramics: A review. J. Ceram. Sci. Technol. 2014, 5, 245–260. [Google Scholar]
- Zocca, A.; Colombo, P.; Gomes, C.M.; Günster, J. Additive Manufacturing of Ceramics: Issues, Potentialities, and Opportunities. J. Am. Ceram. Soc. 2015, 98, 1983–2001. [Google Scholar] [CrossRef]
- Travitzky, N.; Bonet, A.; Dermeik, B.; Fey, T.; Filbert-Demut, I.; Schlier, L.; Schlordt, T.; Greil, P. Additive Manufacturing of Ceramic-Based Materials. Adv. Eng. Mater. 2014, 16, 729–754. [Google Scholar] [CrossRef]
- Lv, X.; Ye, F.; Cheng, L.; Fan, S.; Liu, Y. Binder jetting of ceramics: Powders, binders, printing parameters, equipment, and post-treatment. Ceram. Int. 2019, 45, 12609–12624. [Google Scholar] [CrossRef]
- Wang, J.-C.; Dommati, H.; Hsieh, S.-J. Review of additive manufacturing methods for high-performance ceramic materials. Int. J. Adv. Manuf. Technol. 2019, 103, 2627–2647. [Google Scholar] [CrossRef]
- Yves-Christian, H.; Jan, W.; Wilhelm, M.; Konrad, W.; Reinhart, P. Net shaped high performance oxide ceramic parts by selective laser melting. Phys. Procedia 2010, 5, 587–594. [Google Scholar] [CrossRef]
- Sing, S.L.; Yeong, W.Y.; Wiria, F.E.; Tay, B.Y.; Zhao, Z.; Zhao, L.; Tian, Z.; Yang, S. Direct selective laser sintering and melting of ceramics: A review. Rapid Prototyp. J. 2017, 23, 611–623. [Google Scholar] [CrossRef]
- Mason, M.S.; Huang, T.; Landers, R.G.; Leu, M.C.; Hilmas, G.E. Aqueous-based extrusion of high solids loading ceramic pastes: Process modeling and control. J. Mater. Process. Technol. 2009, 209, 2946–2957. [Google Scholar] [CrossRef]
- Onagoruwa, S.; Bose, S.; Bandyopadhyay, A. Fused deposition of ceramics (FDC) and composites. In Proceedings of the 2001 International Solid Freeform Fabrication Symposium SFF, Austin, TX, USA, 6–8 August 2001; pp. 224–231. [Google Scholar]
- Stuecker, J.N.; Cesarano, J.A.; Hirschfeld, D. Control of the viscous behavior of highly concentrated mullite suspensions for robocasting. J. Mater. Process. Technol. 2003, 142, 318–325. [Google Scholar] [CrossRef]
- Huang, T.; Mason, M.S.; Hilmas, G.E.; Leu, M.C. Freeze-form extrusion fabrication of ceramic parts. Virtual Phys. Prototyp. 2006, 1, 93–100. [Google Scholar] [CrossRef]
- Lewis, J.A.; Smay, J.E.; Stuecker, J.; Cesarano, J. Direct Ink Writing of Three-Dimensional Ceramic Structures. J. Am. Ceram. Soc. 2006, 89, 3599–3609. [Google Scholar] [CrossRef]
- Li, W.; Ghazanfari, A.; Leu, M.C.; Landers, R.G. Methods of extrusion on demand for high solids loading ceramic paste in freeform extrusion fabrication. In Proceedings of the Solid Freeform Fabrication Symposium, Austin, TX, USA, 10–12 August 2015; pp. 332–345. [Google Scholar]
- Vitorino, N.; Freitas, C.; Ribeiro, M.J.; Abrantes, J.C.C.; Frade, J.R. Extrusion of ceramic emulsions: Plastic behavior. Appl. Clay Sci. 2014, 101, 315–319. [Google Scholar] [CrossRef]
- Kim, N.P.; Cho, D.; Zielewski, M. Optimization of 3D printing parameters of Screw Type Extrusion (STE) for ceramics using the Taguchi method. Ceram. Int. 2019, 45, 2351–2360. [Google Scholar] [CrossRef]
- Tang, S.; Yang, L.; Li, G.; Liu, X.; Fan, Z. 3D printing of highly-loaded slurries via layered extrusion forming: Parameters optimization and control. Addit. Manuf. 2019, 28, 546–553. [Google Scholar] [CrossRef]
- Li, H.; Liu, S.; Lin, L. Rheological study on 3D printability of alginate hydrogel and effect of graphene oxide. Int. J. Bioprinting 2016, 2, 163–175. [Google Scholar] [CrossRef]
- Godoi, F.C.; Prakash, S.; Bhandari, B.R. 3d printing technologies applied for food design: Status and prospects. J. Food Eng. 2016, 179, 44–54. [Google Scholar] [CrossRef]
- Liu, H.; Li, Y.; Li, D. Research on rheological properties and extrusion behavior of aqueous alumina paste in paste-extrusion-based SFF processes. Int. J. Adv. Manuf. Technol. 2016, 83, 2039–2047. [Google Scholar] [CrossRef]
- Guilherme, P.; Ribeiro, M.; Labrincha, J.A. Behaviour of different industrial ceramic pastes in extrusion process. Adv. Appl. Ceram. 2009, 108, 347–351. [Google Scholar] [CrossRef]
- Patel, M.; Blackburn, S.; Wilson, D. Modelling of paste ram extrusion subject to liquid phase migration and wall friction. Chem. Eng. Sci. 2017, 172, 487–502. [Google Scholar] [CrossRef]
- Bardesley, M.A.; Bridgwater, J. Evaluation of Liquid Phase Migration in Pastes and Gels. Ind. Eng. Chem. Res. 2011, 51, 1774–1781. [Google Scholar] [CrossRef]
- Liu, H.; Leu, M.C. Liquid Phase Migration In Extrusion Of Aqueous Alumina Paste For Freeze-Form Extrusion Fabrication. Int. J. Mod. Phys. B 2009, 23, 1861–1866. [Google Scholar] [CrossRef]
- Guo, C.F.; Zhang, M.; Bhandari, B. A comparative study between syringe-based and screw-based 3D food printers by com-putational simulation. Comput. Electron. Agr. 2019, 162, 397–404. [Google Scholar] [CrossRef]
- Masuda, H.; Ohta, Y.; Kitayama, M. Additive Manufacturing of SiC Ceramics with Complicated Shapes Using the FDM Type 3D-Printer. J. Mater. Sci. Chem. Eng. 2019, 7, 1–12. [Google Scholar] [CrossRef]
- Ghazanfari, A.; Li, W.; Leu, M.C.; Hilmas, G.E. A novel freeform extrusion fabrication process for producing solid ceramic components with uniform layered radiation drying. Addit. Manuf. 2017, 15, 102–112. [Google Scholar] [CrossRef]
- Biswas, P.; Mamatha, S.; Naskar, S.; Rao, Y.S.; Johnson, R.; Padmanabham, G. 3D extrusion printing of magnesium aluminate spinel ceramic parts using thermally induced gelation of methyl cellulose. J. Alloy. Compd. 2019, 770, 419–423. [Google Scholar] [CrossRef]
- Ren, X.; Shao, H.; Lin, T.; Zheng, H. 3D gel-printing—An additive manufacturing method for producing complex shape parts. Mater. Des. 2016, 101, 80–87. [Google Scholar] [CrossRef]
- Scheithauer, U.; Schwarzer, E.; Richter, H.-J.; Moritz, T. Thermoplastic 3D Printing-An Additive Manufacturing Method for Producing Dense Ceramics. Int. J. Appl. Ceram. Technol. 2014, 12, 26–31. [Google Scholar] [CrossRef]
- Li, W.; Ghazanfari, A.; McMillen, D.; Leu, M.C.; Hilmas, G.E.; Watts, J. Fabricating ceramic components with water dissolvable support structures by the Ceramic On-Demand Extrusion process. CIRP Ann. 2017, 66, 225–228. [Google Scholar] [CrossRef]
- Yang, L.; Tang, S.; Li, G.; Qian, L.; Mei, J.; Jiang, W.; Fan, Z.; Mei, L.Q.J. Layered extrusion forming of complex ceramic structures using starch as removable support. Ceram. Int. 2019, 45, 21843–21850. [Google Scholar] [CrossRef]
- Montiel, R.; Patiño-Herrera, R.; Gonzalez-Calderón, J.A.; Pérez, E. Novel twin screw co-extrusion-electrospinning apparatus. Am. J. Biomed. Eng. 2016, 6, 19–24. [Google Scholar]
- Hong, S.; Sanchez, C.; Du, H.; Kim, N. Fabrication of 3D Printed Metal Structures by Use of High-Viscosity Cu Paste and a Screw Extruder. J. Electron. Mater. 2015, 44, 836–841. [Google Scholar] [CrossRef]
- Lu, X.; Lee, Y.; Yang, S.; Hao, Y.; Evans, J.R.; Parini, C.G. Fine lattice structures fabricated by extrusion freeforming: Process variables. J. Mater. Process. Technol. 2009, 209, 4654–4661. [Google Scholar] [CrossRef]
- Khan, A.U.; Briscoe, B.J.; Luckham, P.F. Evaluation of slip in capillary extrusion of ceramic pastes. J. Eur. Ceram. Soc. 2001, 21, 483–491. [Google Scholar] [CrossRef]
- Hu, F.; Cheng, J.; Jiang, X. Investigation on dimensional accuracy of extrusion-based 3D printing and sintering of ceramic paste. Ind. Technol. Innov. 2018, 5, 12–17. [Google Scholar] [CrossRef]
- Jiang, X.; Hu, F. Study on mechanical properties of 3D printed carbon fiber reinforced ceramic matrix composites. Ind. Technol. Innov. 2018, 5, 26–29. [Google Scholar] [CrossRef]
- Oummadi, S.; Nait-Ali, B.; Alzina, A.; Victor, J.L.; Launay, Y.; Mirdrikvand, M.; Dreher, W.; Rezwan, K.; Smith, D.S. Distri-bution of water in ceramic green bodies during drying. J. Eur. Ceram. Soc. 2019, 39, 3164–3172. [Google Scholar] [CrossRef]
- Lan, W.; Xiao, P. Drying stress of yttria-stabilized-zirconia slurry on a metal substrate. J. Eur. Ceram. Soc. 2007, 27, 3117–3125. [Google Scholar] [CrossRef]
- Chiu, R.C.; Garino, T.J.; Cima, M.J. Drying of Granular Ceramic Films: I, Effect of Processing Variables on Cracking Behavior. J. Am. Ceram. Soc. 1993, 76, 2257–2264. [Google Scholar] [CrossRef]
- Chiu, R.C.; Cima, M.J. Drying of Granular Ceramic Films: II, Drying Stress and Saturation Uniformity. J. Am. Ceram. Soc. 1993, 76, 2769–2777. [Google Scholar] [CrossRef]
- Inoue, T.; Iyota, H.; Nishimura, N. Prediction Method for Drying Time of Wet Porous Material in Humid Hot Air and Superheated Steam. Dry. Technol. 2010, 28, 608–614. [Google Scholar] [CrossRef]
- Ol’Shanskii, A.I. Regular Heat Regime of Heating of Moist Capillary-Porous Materials in the Process of Their Drying. J. Eng. Phys. Thermophys. 2014, 87, 1362–1373. [Google Scholar] [CrossRef]
- Faes, M.; Valkenaers, H.; Vogeler, F.; Vleugels, J.; Ferraris, E. Extrusion-based 3D Printing of Ceramic Components. Procedia CIRP 2015, 28, 76–81. [Google Scholar] [CrossRef]
- Finke, B.; Hesselbach, J.; Schütt, A.; Tidau, M.; Hampel, B.; Schilling, M.; Kwade, A.; Schilde, C. Influence of formulation parameters on the freeform extrusion process of ceramic pastes and resulting product properties. Addit. Manuf. 2020, 32, 101005. [Google Scholar] [CrossRef]
- Azzolini, A.; Sglavo, V.M.; Downs, J.A. Novel method for the identification of the maximum solid loading suitable for optimal extrusion of ceramic pastes. J. Adv. Ceram. 2014, 3, 7–16. [Google Scholar] [CrossRef]
- Papazetis, G.; Vosniakos, G.-C. Improving deposition quality at higher rates in material extrusion additive manufacturing. Int. J. Adv. Manuf. Technol. 2020, 111, 1221–1235. [Google Scholar] [CrossRef]
- Joh, C.; Lee, J.; Bui, T.Q.; Park, J.; Yang, I.-H. Buildability and Mechanical Properties of 3D Printed Concrete. Materials 2020, 13, 4919. [Google Scholar] [CrossRef]
- Bociaga, D.; Bartniak, M.; Sobczak, K.; Rosinska, K. An Integration of a Peristaltic Pump-Based Extruder into a 3D Bioprinter Dedicated to Hydrogels. Materials 2020, 13, 4237. [Google Scholar] [CrossRef]
- Mechtcherine, V.; Michael, A.; Liebscher, M.; Schmeier, T. Extrusion-Based Additive Manufacturing with Carbon Reinforced Concrete: Concept and Feasibility Study. Materials 2020, 13, 2568. [Google Scholar] [CrossRef]







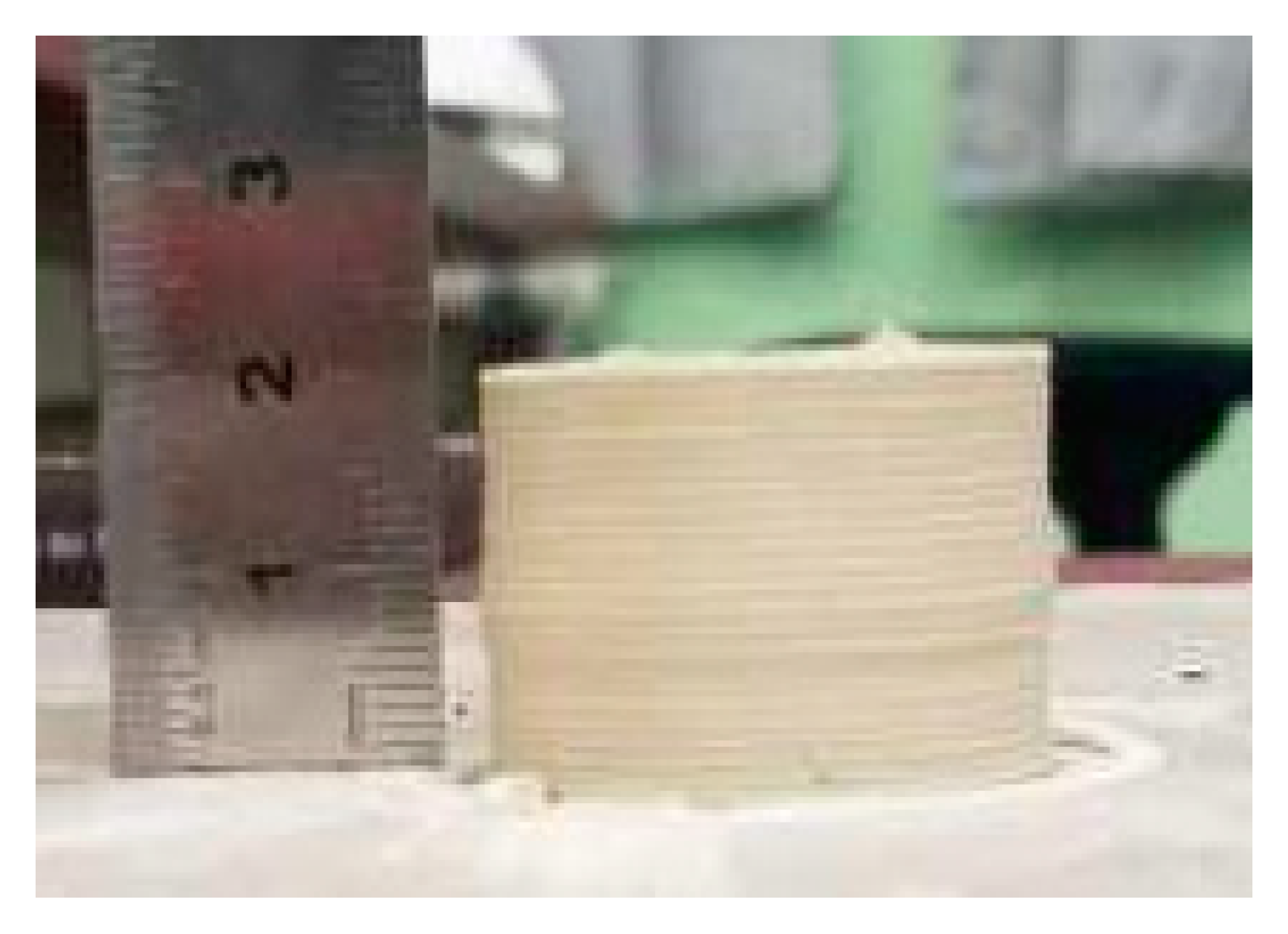

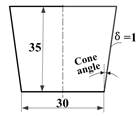
| Thin-Walled Benchmark Part | Layer Height | Extrusion Speed | d by Equation (1) | by Equation (11) | by Equation (12) | by Equation (13) | by Equation (14) | by Equation (15) | by Equation (16) | |
|---|---|---|---|---|---|---|---|---|---|---|
| Estimates | Actual | |||||||||
 | 0.8 dmm | 2.49 mm/s | 2.6 mm/s | 2.04 mm3/s | 144 Pa·s | 0.3 MPa | 21 1/s | 0.0355 MPa | 1.16 mm | 3.0 × 10−3 MPa |
| 0.7 dmm | 2.07 mm/s | 2.2 mm/s | 1.73 mm3/s | 142 Pa·s | 0.25 MPa | 18 1/s | 0.0354 MPa | 1.12 mm | 2.5 × 10−3 MPa | |
| Shape-Holding Methods | Principle Effects | Main Advantages | Implicit Disadvantages |
|---|---|---|---|
| Without auxiliary shape holding method [11,22] | – | Extrusion-based layered forming process with directly atmospheric drying is an eco-friendly process to create 3D objects. | This process has large uncontrollable variability owing to paste property variability. The green body needs a long-time for bulk drying before sintering. |
| Deposition in freezing environment [14,26] | Physical | The freezing environment using a liquid nitrogen injection system enabled the aqueous pastes to solidify at temperatures below the freezing point of water, thus avoiding part deformation and enabling fabrication of larger parts. | Besides the freezing system, a heating jacket was also needed to keep paste temperatures above the freezing point of water until it was deposited. Before sintering, the moisture still needs a long drying time. |
| Using removable support structures [34] | Physical and Chemical | To make complex ceramic components, double-head extrusion was used to print two materials: one to form the main part and another to form the support structure. | The support material must meet the typical requirements of 3D printability. The support material should be compatible with the main material and easily removed in postprocessing. |
| Using chemical binders [13,15] | Chemical | Concentrated colloidal gels with tailored viscoelastic properties were designed to form self-supporting features and were deposited in a layer-by-layer sequence to directly write the desired 3D objects. | The gelling of ceramic colloids or polymers must exhibit a controlled viscoelastic response and contain high colloid volume fractions to minimize shrinkage. During sintering, the gels may release harmful chemicals. |
| Using thermally induced gelation [30] | Chemical | Shaping of ceramics by thermally induced gelation of methyl cellulose is eco-friendly as it is a naturally occurring polymer and is effective in very low concentrations. | The part while printing also needs to be exposed to the gelation temperatures using a honeycomb-based energy efficient air heater. Before sintering, the moisture still needs bulk drying. |
| Uniform layered radiation drying [29] | Physical | Optimal partial drying with the infrared lamp enables strong bonding between layers. The liquid oil surrounding the part could preclude crack formation, warpage, and moisture gradient in the part. | The oil filling and infrared heating systems make the whole system too complicated. In postprocessing, the oil bath needs draining and the remaining water further needs a long drying time. |
| In situ hot air flow drying | Physical | The in situ hot air flow drying device provides online local drying on the deposited spot in sync with the digital material deposition. The auxiliary heating system is very simple and eco-friendly. The green body needs a very short bulk drying time before sintering. | According to current experiments, no obvious disadvantages were found. In the future, we will explore more precise regulations of heating manners and drying efficiency. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, F.; Mikolajczyk, T.; Pimenov, D.Y.; Gupta, M.K. Extrusion-Based 3D Printing of Ceramic Pastes: Mathematical Modeling and In Situ Shaping Retention Approach. Materials 2021, 14, 1137. https://doi.org/10.3390/ma14051137
Hu F, Mikolajczyk T, Pimenov DY, Gupta MK. Extrusion-Based 3D Printing of Ceramic Pastes: Mathematical Modeling and In Situ Shaping Retention Approach. Materials. 2021; 14(5):1137. https://doi.org/10.3390/ma14051137
Chicago/Turabian StyleHu, Fuwen, Tadeusz Mikolajczyk, Danil Yurievich Pimenov, and Munish Kumar Gupta. 2021. "Extrusion-Based 3D Printing of Ceramic Pastes: Mathematical Modeling and In Situ Shaping Retention Approach" Materials 14, no. 5: 1137. https://doi.org/10.3390/ma14051137
APA StyleHu, F., Mikolajczyk, T., Pimenov, D. Y., & Gupta, M. K. (2021). Extrusion-Based 3D Printing of Ceramic Pastes: Mathematical Modeling and In Situ Shaping Retention Approach. Materials, 14(5), 1137. https://doi.org/10.3390/ma14051137









