Diffraction Methods for Qualitative and Quantitative Texture Analysis of Ferroelectric Ceramics †
Abstract
:1. Introduction
2. Experimental Methods
2.1. Sample Preparation
2.2. Bragg-Brentano X-ray Diffraction
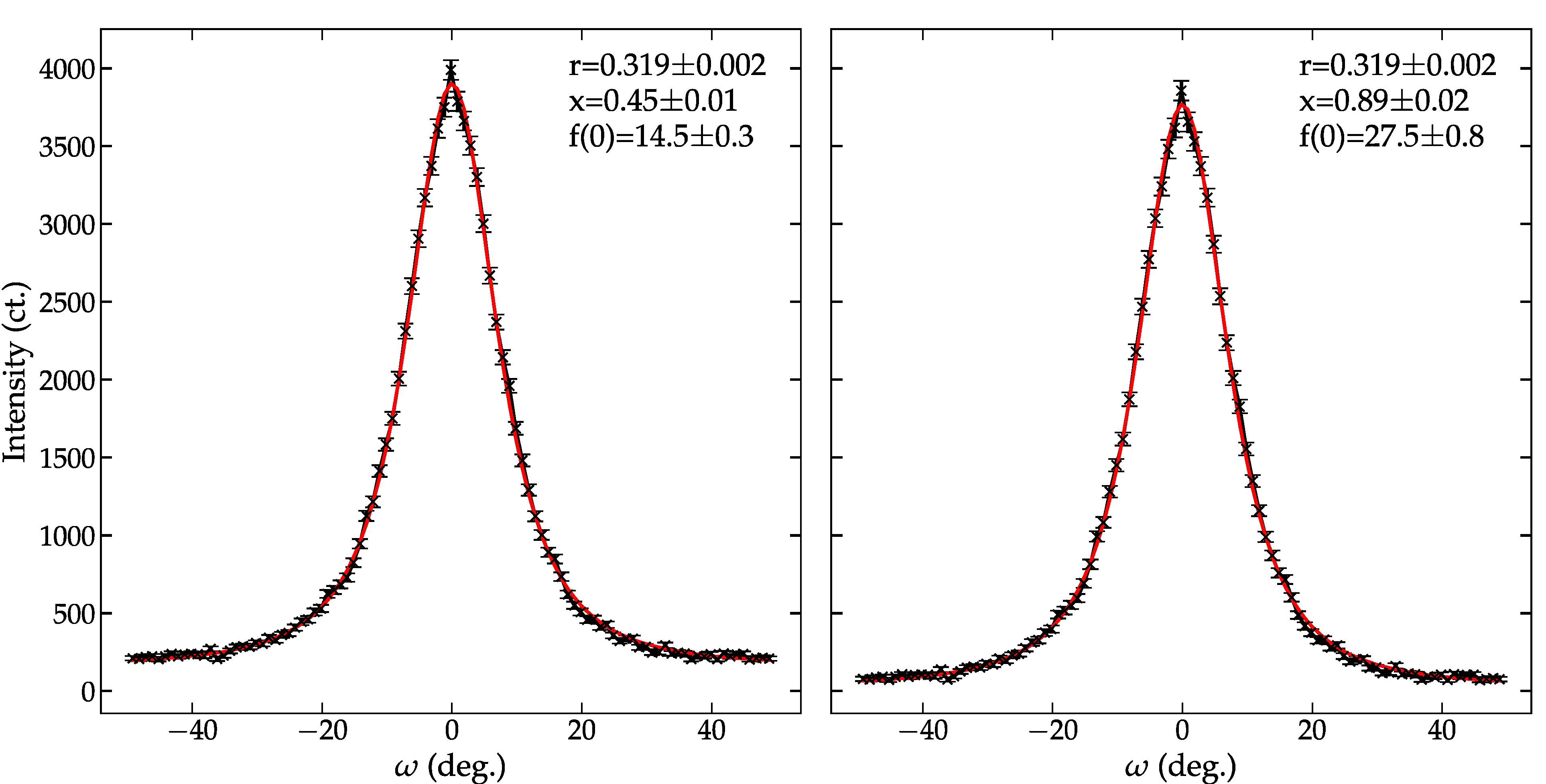
2.3. Neutron Rocking Curves
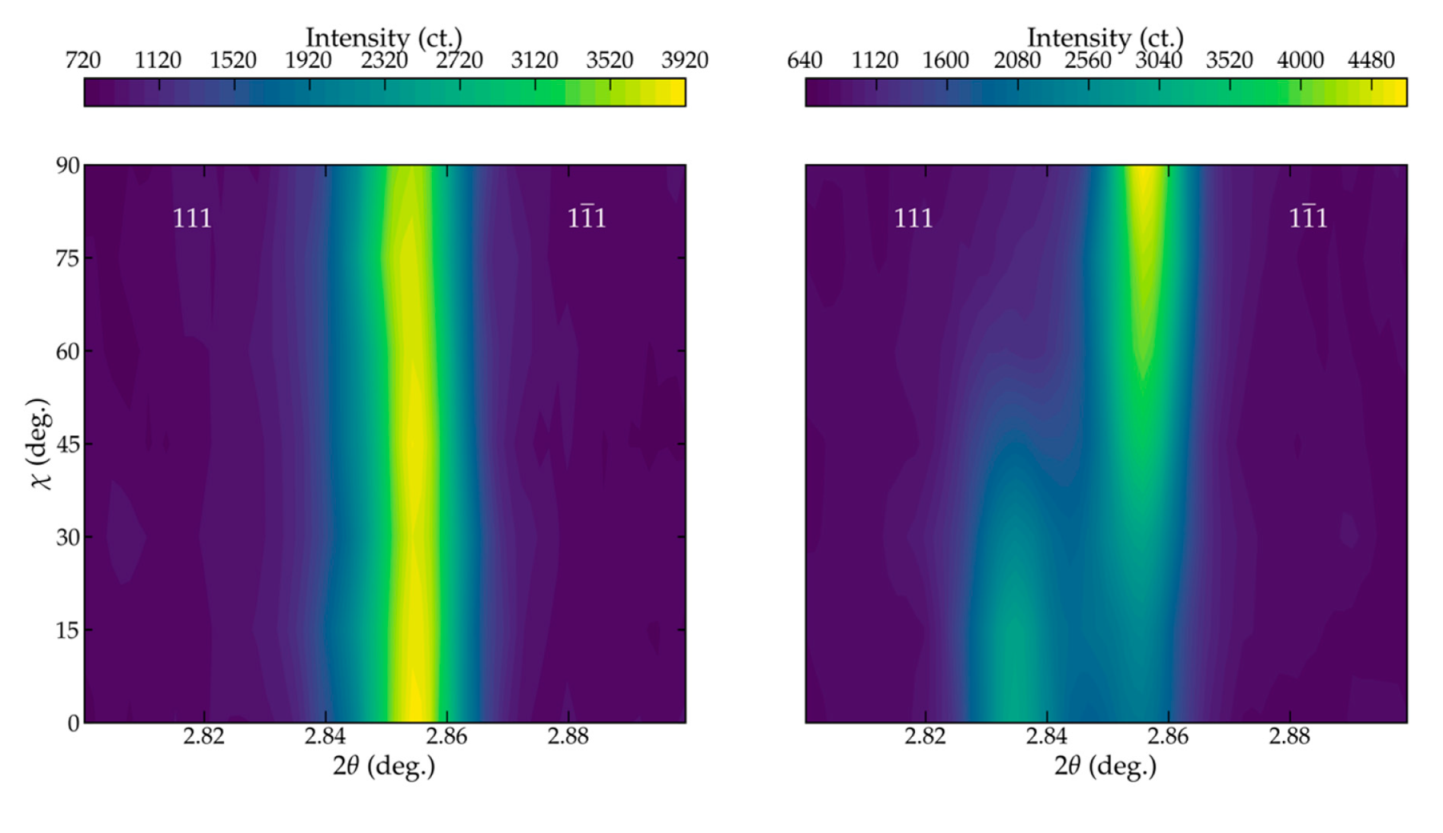
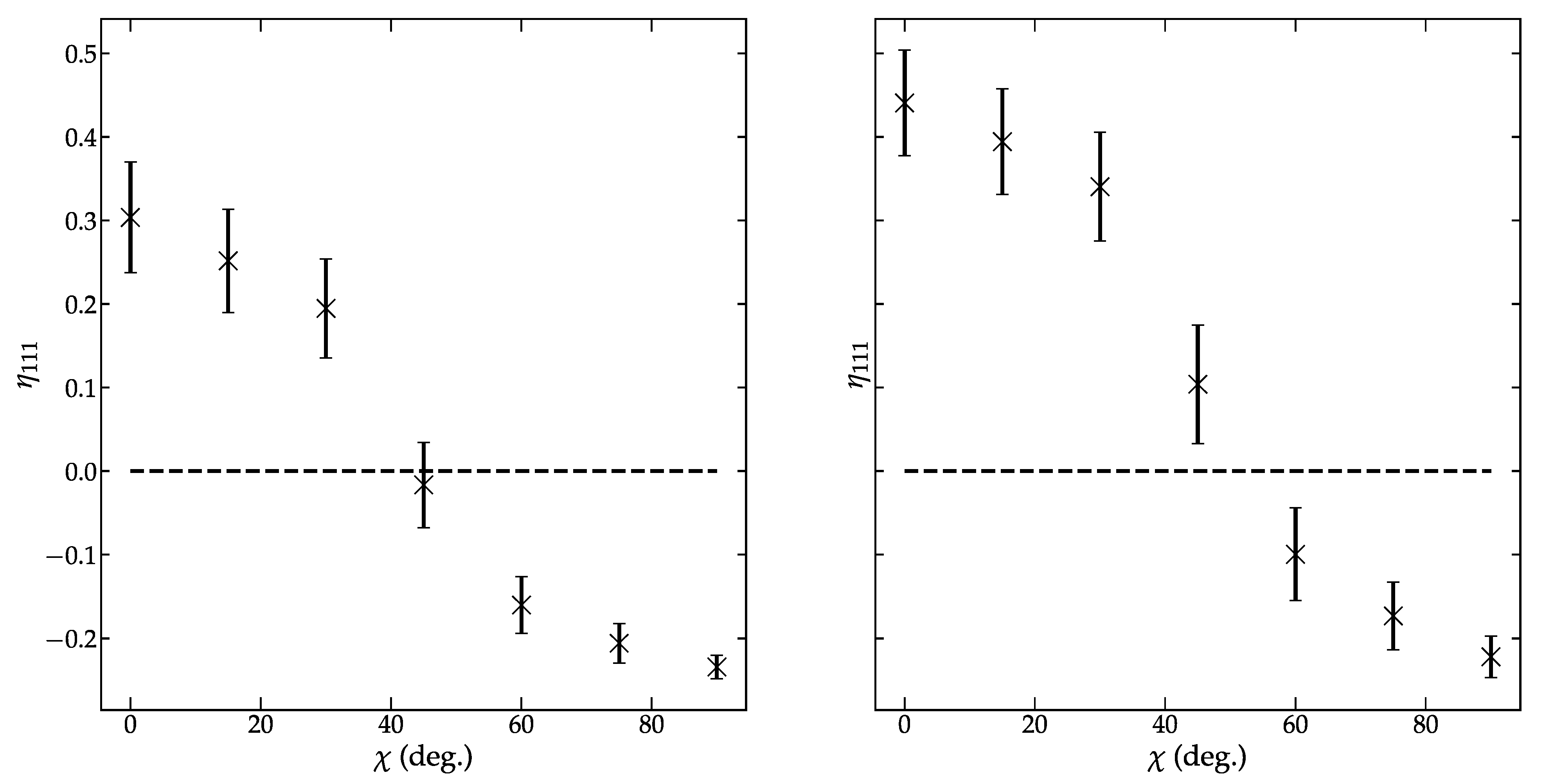
2.4. Angular Dependent Diffraction Data
2.5. Field Dependent Diffraction
3. Mathematical Methods
3.1. Lotgering Factor
3.2. March-Dollase
3.3. Orientation Distribution Functions
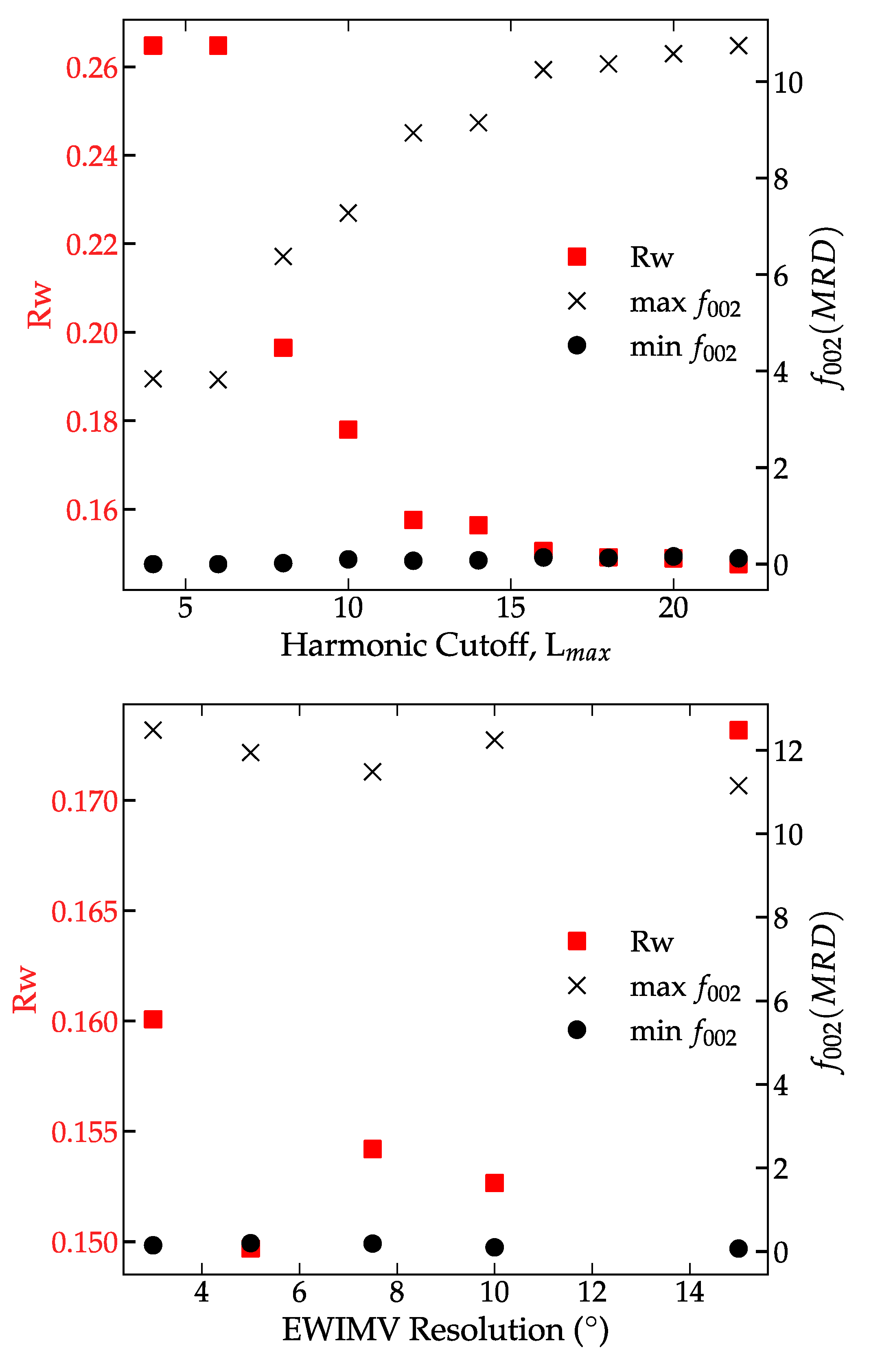
3.3.1. Harmonic ODF
3.3.2. Discrete ODF
3.4. Dipole Distribution
4. Results and Discussion
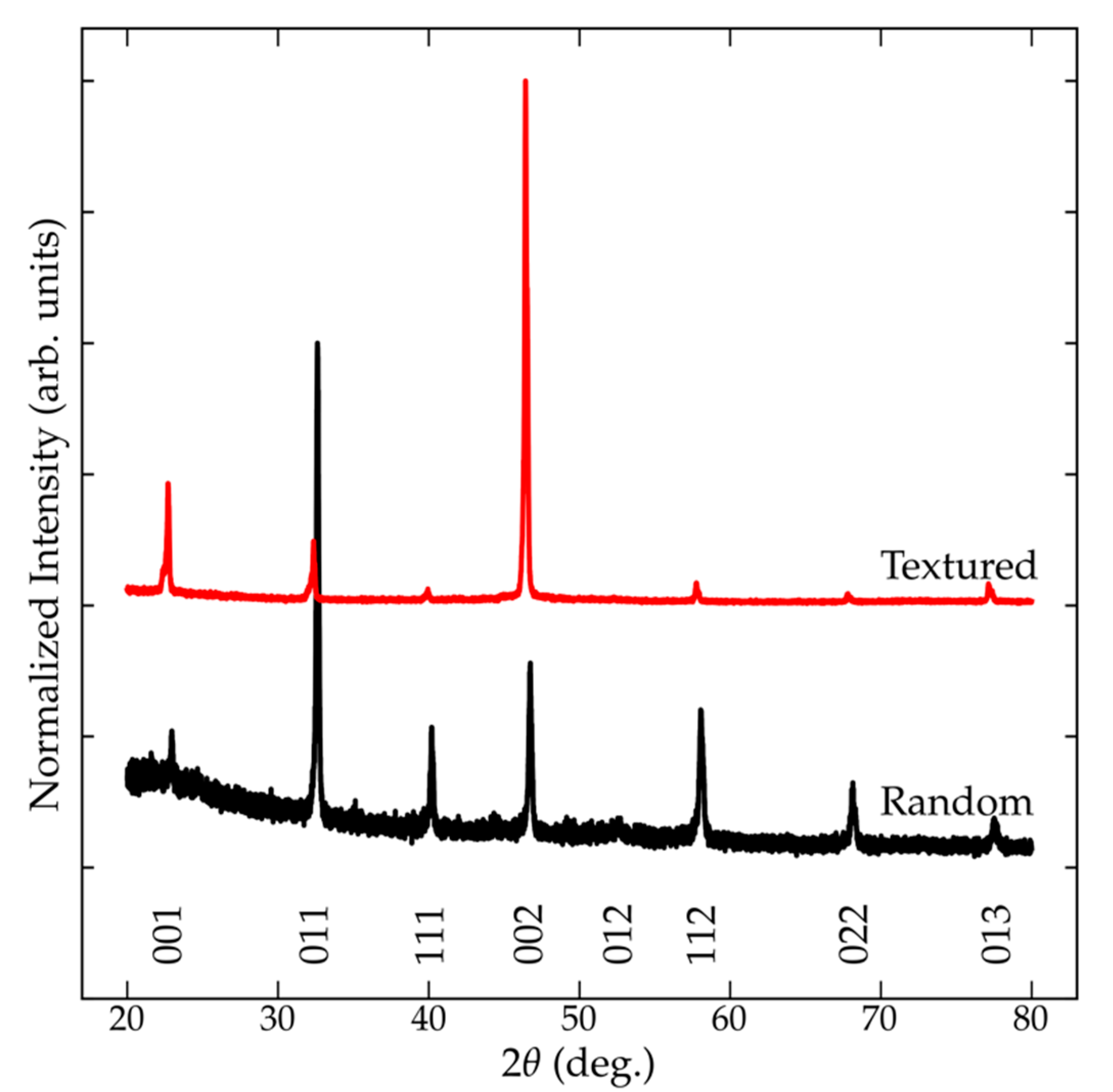
4.1. Lotgering Factor
4.2. March–Dollase
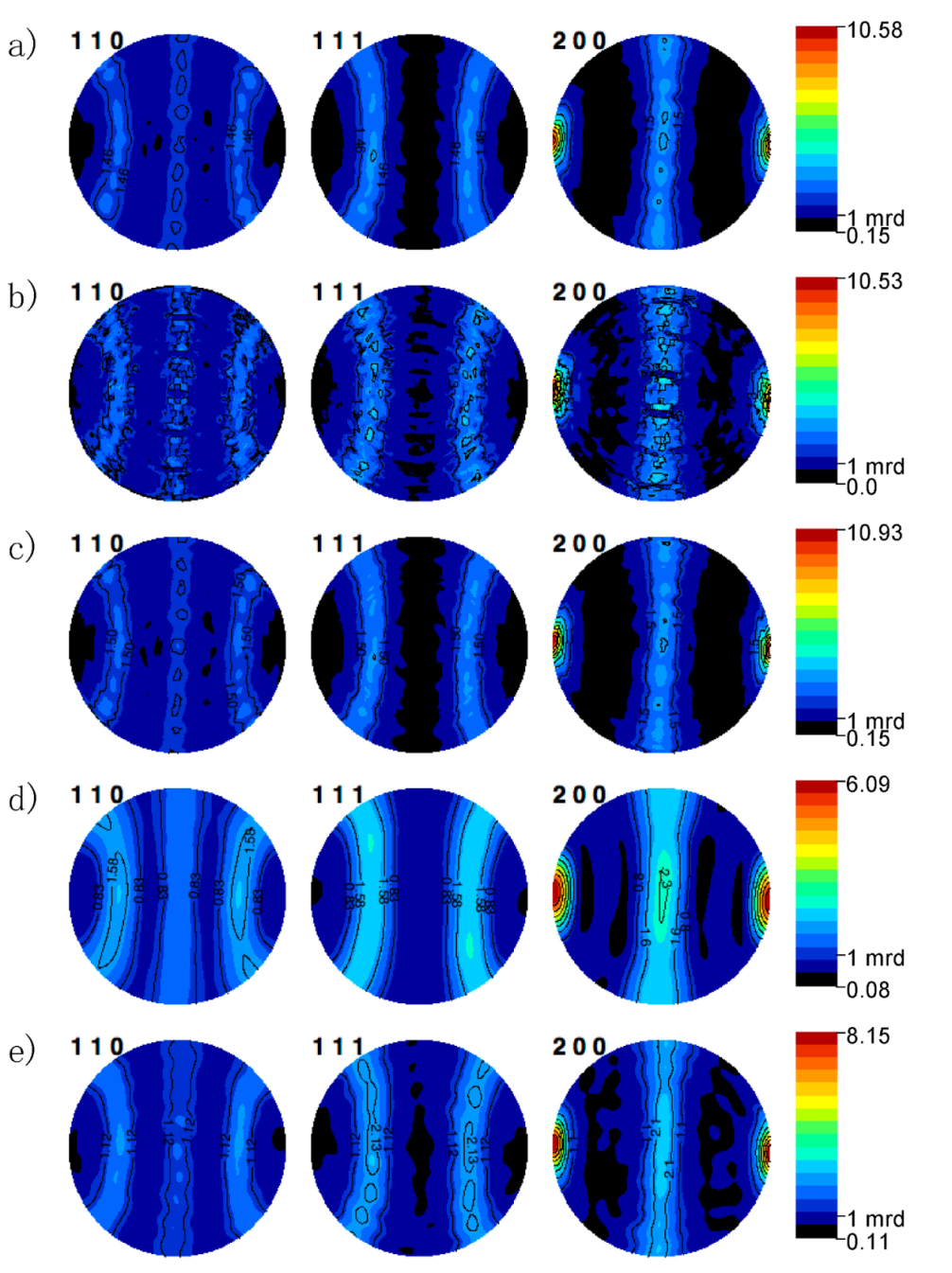
4.3. Rietveld Texture Analysis
4.4. Ferroelastic Domain Texture
5. Comparison of Methods
Supplementary Materials
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xie, Y.X.; Lutterotti, L.; Wenk, H.-R.; Kovacs, F. Texture analysis of ancient coins with TOF neutron diffraction. J. Mater. Sci. 2004, 39, 3329–3337. [Google Scholar] [CrossRef] [Green Version]
- Kocks, U.F.; Tome, C.N.; Wenk, H.-R. Texture and Anisotropy; Cambridge University Press: Cambridge, UK, 2000; ISBN 978-0-521-79420-6. [Google Scholar]
- Dehoff, R.R.; Kirka, M.M.; Sames, W.J.; Bilheux, H.; Tremsin, A.S.; Lowe, L.E.; Babu, S.S. Site specific control of crystallographic grain orientation through electron beam additive manufacturing. Mater. Sci. Technol. 2015, 31, 931–938. [Google Scholar] [CrossRef]
- Messing, G.L.; Trolier-McKinstry, S.; Sabolsky, E.M.; Duran, C.; Kwon, S.; Brahmaroutu, B.; Park, P.; Yilmaz, H.; Rehrig, P.W.; Eitel, K.B.; et al. Templated grain growth of textured piezoelectric ceramics. Crit. Rev. Solid State Mater. Sci. 2004, 29, 45–96. [Google Scholar] [CrossRef]
- Daniel, L.; Hall, D.A.; Koruza, J.; Webber, K.G.; King, A.; Withers, P.J. Revisiting the blocking force test on ferroelectric ceramics using high energy x-ray diffraction. J. Appl. Phys. 2015, 117, 174104. [Google Scholar] [CrossRef]
- Chen, J.-H.; Hwang, B.-H.; Hsu, T.-C.; Lu, H.-Y. Domain switching of barium titanate ceramics induced by surface grinding. Mater. Chem. Phys. 2005, 91, 67–72. [Google Scholar] [CrossRef]
- Kockelmann, W.; Siano, S.; Bartoli, L.; Visser, D.; Hallebeek, P.; Traum, R.; Linke, R.; Schreiner, M.; Kirfel, A. Applications of TOF neutron diffraction in archaeometry. Appl. Phys. A Mater. Sci. Process. 2006, 83, 175–182. [Google Scholar] [CrossRef]
- Ghosh, D.; Sakata, A.; Carter, J.; Thomas, P.A.; Han, H.; Nino, J.C.; Jones, J.L. Domain Wall Displacement is the Origin of Superior Permittivity and Piezoelectricity in BaTiO3 at Intermediate Grain Sizes. Adv. Funct. Mater. 2014, 24, 885–896. [Google Scholar] [CrossRef] [Green Version]
- Fancher, C.M.; Brewer, S.; Chung, C.C.; Röhrig, S.; Rojac, T.; Esteves, G.; Deluca, M.; Bassiri-Gharb, N.; Jones, J.L. The contribution of 180° domain wall motion to dielectric properties quantified from in situ X-ray diffraction. Acta Mater. 2017, 126, 36–43. [Google Scholar] [CrossRef]
- Schenk, T.; Fancher, C.M.; Hyuk Park, M.; Richter, C.; Kunneth, C.; Kersch, A.; Jones, J.L.; Mikolajick, T.; Schroeder, U. On the Origin of the Large Remanent Polarization in La:HfO2. Adv. Electron. Mater. 2019, 5, 1900303. [Google Scholar] [CrossRef]
- Bowman, K.J.; Chen, I.-W.W. Transformation Textures in Zirconia. J. Am. Ceram. Soc. 1993, 76, 113–122. [Google Scholar] [CrossRef]
- Esteves, G.; Fancher, C.M.; Röhrig, S.; Maier, G.A.G.A.; Jones, J.L.; Deluca, M. Electric-field-induced structural changes in multilayer piezoelectric actuators during electrical and mechanical loading. Acta Mater. 2017, 132, 96–105. [Google Scholar] [CrossRef]
- Daniels, J.E.; Jo, W.; Rődel, J.; Honkimaki, V.; Jones, J.L. Electric-field-induced phase-change behavior in (Bi0.5Na0.5)TiO3-BaTiO3-(K0.5Na0.5)NbO3: A combinatorial investigation. Acta Mater. 2010, 58, 2103–2111. [Google Scholar] [CrossRef]
- Jones, J.L.; Aksel, E.; Tutuncu, G.; Usher, T.-M.; Chen, J.; Xing, X.; Studer, A.J. Domain wall and interphase boundary motion in a two-phase morphotropic phase boundary ferroelectric: Frequency dispersion and contribution to piezoelectric and dielectric properties. Phys. Rev. B 2012, 86, 024104. [Google Scholar] [CrossRef] [Green Version]
- Jones, J.L.; Hoffman, M.; Bowman, K.J. Saturated domain switching textures and strains in ferroelastic ceramics. J. Appl. Phys. 2005, 98, 024115. [Google Scholar] [CrossRef]
- Jones, J.L.; Hoffman, M.; Daniels, J.E.; Studer, A.J. Direct measurement of the domain switching contribution to the dynamic piezoelectric response in ferroelectric ceramics. Appl. Phys. Lett. 2006, 89, 092901. [Google Scholar] [CrossRef]
- Saito, Y.; Takao, H.; Tani, T.; Nonoyama, T.; Takatori, K.; Homma, T.; Nagaya, T.; Nakamura, M. Lead-free piezoceramics. Nature 2004, 432, 84–87. [Google Scholar] [CrossRef] [PubMed]
- Jones, J.L.; Slamovich, E.B.; Bowman, K.J. Product and component grain and domain textures in ferroelectric ceramics. Mater. Sci. Forum 2005, 495–497, 1401–1406. [Google Scholar] [CrossRef]
- Fancher, C.M.; Blendell, J.E.; Bowman, K.J. Decoupling of superposed textures in an electrically biased piezoceramic with a 100 preferred orientation. Appl. Phys. Lett. 2017, 110, 062901. [Google Scholar] [CrossRef]
- Wenk, H.-R.; Grigull, S. Synchrotron texture analysis with area detectors. J. Appl. Crystallogr. 2003, 36, 1040–1049. [Google Scholar] [CrossRef]
- Ischia, G.; Wenk, H.-R.; Lutterotti, L.; Berberich, F. Quantitative Rietveld texture analysis of zirconium from single synchrotron diffraction images. J. Appl. Crystallogr. 2005, 38, 377–380. [Google Scholar] [CrossRef]
- Matthies, S.; Pehl, J.; Wenk, H.-R.; Lutterotti, L.; Vogel, S.C. Quantitative texture analysis with the HIPPO neutron TOF diffractometer. J. Appl. Crystallogr. 2005, 38, 462–475. [Google Scholar] [CrossRef]
- Vogel, S.C.; Hartig, C.; Lutterotti, L.; Von Dreele, R.B.; Wenk, H.-R.; Williams, D.J. Texture measurements using the new neutron diffractometer HIPPO and their analysis using the Rietveld method. Powder Diffr. 2004, 19, 65–68. [Google Scholar] [CrossRef] [Green Version]
- Fancher, C.M.; Hoffmann, C.M.M.; Frontzek, M.D.D.; Bunn, J.R.R.; Payzant, E.A.A. Probing orientation information using 3-dimensional reciprocal space volume analysis. Rev. Sci. Instrum. 2019, 90, 013902. [Google Scholar] [CrossRef]
- Xu, P.; Harjo, S.; Ojima, M.; Suzuki, H.; Ito, T.; Gong, W.; Vogel, S.C.; Inoue, J.; Tomota, Y.; Aizawa, K.; et al. High stereographic resolution texture and residual stress evaluation using time-of-flight neutron diffraction. J. Appl. Crystallogr. 2018, 51, 746–760. [Google Scholar] [CrossRef]
- Peterson, N.E.; Einhorn, J.R.; Fancher, C.M.; Bunn, J.R.; Payzant, E.A.; Agnew, S.R. Quantitative texture analysis using the NOMAD time-of-flight neutron diffractometer. J. Appl. Crystallogr. 2021, 54, 867–877. [Google Scholar] [CrossRef]
- Gorfman, S. Sub-microsecond X-ray crystallography: Techniques, challenges, and applications for materials science. Crystallogr. Rev. 2014, 20, 210–232. [Google Scholar] [CrossRef]
- Daniels, J.E.; Finlayson, T.R.; Studer, A.J.; Hoffman, M.; Jones, J.L. Time-resolved diffraction measurements of electric-field-induced strain in tetragonal lead zirconate titanate. J. Appl. Phys. 2007, 101, 094104. [Google Scholar] [CrossRef]
- Zeng, J.T.; Kwok, K.W.; Tam, W.K.; Tian, H.Y.; Jiang, X.P.; Chan, H.L.W. Plate-like Na0.5Bi0.5TiO3 template synthesized by a topochemical method. J. Am. Ceram. Soc. 2006, 89, 3850–3853. [Google Scholar] [CrossRef]
- Fancher, C.M.; Blendell, J.E.; Bowman, K.J. Poling effect on d33 in textured Bi0.5Na0.5TiO3-based materials. Scr. Mater. 2013, 68, 443–446. [Google Scholar] [CrossRef]
- Fancher, C.M.; Jo, W.; Rödel, J.; Blendell, J.E.; Bowman, K.J. Effect of Texture on Temperature-Dependent Properties of K0.5 Na0.5 NbO3 Modified Bi1/2 Na1/2 TiO3-x BaTiO3. J. Am. Ceram. Soc. 2014, 8, 2557–2563. [Google Scholar] [CrossRef]
- Newville, M.; Otten, R.; Nelson, A.; Ingargiola, A.; Stensitzki, T.; Allan, D.; Fox, A.; Carter, F.; Michał; Pustakhod, D.; et al. lmfit/lmfit-py 1.0.2 (Version 1.0.2). 2021. Available online: https://zenodo.org/record/4516651 (accessed on 5 June 2021).
- Chakoumakos, B.C.; Cao, H.; Ye, F.; Stoica, A.D.; Popovici, M.; Sundaram, M.; Zhou, W.; Hicks, J.S.; Lynn, G.W.; Riedel, R.A. Four-circle single-crystal neutron diffractometer at the High Flux Isotope Reactor. J. Appl. Crystallogr. 2011, 44, 655–658. [Google Scholar] [CrossRef]
- Lutterotti, L. Total pattern fitting for the combined size-strain-stress-texture determination in thin film diffraction. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. At. 2010, 268, 334–340. [Google Scholar] [CrossRef]
- Lutterotti, L.; Matthies, S.; Wenk, H.-R.; Schultz, A.S.; Richardson, J.W. Combined texture and structure analysis of deformed limestone from time-of-flight neutron diffraction spectra. J. Appl. Phys. 1997, 81, 594–600. [Google Scholar] [CrossRef]
- Matthies, S.; Lutterotti, L.; Wenk, H.R. Advances in Texture Analysis from Diffraction Spectra. J. Appl. Crystallogr. 1997, 30, 31–42. [Google Scholar] [CrossRef]
- Matthies, S. 20 Years WIMV, History, Experience and Contemporary Developments. Mater. Sci. Forum 2002, 408–412, 95–100. [Google Scholar] [CrossRef]
- Bernier, J.V.; Miller, M.P.; Boyce, D.E. A novel optimization-based pole-figure inversion method: Comparison with WIMV and maximum entropy methods. J. Appl. Crystallogr. 2006, 39, 697–713. [Google Scholar] [CrossRef]
- Kieffer, J.; Karkoulis, D. PyFAI, a versatile library for azimuthal regrouping. J. Phys. Conf. Ser. 2013, 425, 202012. [Google Scholar] [CrossRef]
- Lotgering, F. Topotactical reactions with ferrimagnetic oxides having hexagonal crystal structures—I. J. Inorg. Nucl. Chem. 1959, 9, 249–254. [Google Scholar] [CrossRef]
- Lonardelli, I.; Gey, N.; Wenk, H.-R.; Humbert, M.; Vogel, S.C.; Lutterotti, L. In situ observation of texture evolution during α→β and β→α phase transformations in titanium alloys investigated by neutron diffraction. Acta Mater. 2007, 55, 5718–5727. [Google Scholar] [CrossRef]
- Wenk, H.-R.; Lonardelli, I.; Pehl, J.; Devine, J.; Prakapenka, V.; Shen, G.; Mao, H.; Lonardeli, I. In situ observation of texture development in olivine, ringwoodite, magnesiowustite and silicate perovskite at high pressure. Earth Planet. Sci. Lett. 2004, 226, 507–519. [Google Scholar] [CrossRef]
- Wenk, H.-R.; Cont, L.; Xie, Y.; Lutterotti, L.; Ratschbacher, L.; Richardson, J. Rietveld texture analysis of Dabie Shan eclogite from TOF neutron diffraction spectra. J. Appl. Crystallogr. 2001, 34, 442–453. [Google Scholar] [CrossRef] [Green Version]
- Lonardelli, I.; Wenk, H.-R.; Lutterotti, L.; Goodwin, M. Texture analysis from synchrotron diffraction images with the Rietveld method: Dinosaur tendon and salmon scale. J. Synchrotron Radiat. 2005, 12, 354–360. [Google Scholar] [CrossRef] [PubMed]
- March, A. Mathematical theory of regularity according to grain-form for affine deformation. Zeitschrift Krist. Krist. Krist. Krist. 1932, 81, 285–297. [Google Scholar]
- Dollase, W.A. Correction of intensities for preferred orientation in powder diffractometry: Application of the March model. J. Appl. Crystallogr. 1986, 19, 267–272. [Google Scholar] [CrossRef]
- Yilmaz, H.; Trolier-McKinstry, S.; Messing, G.L. Reactive templated grain growth of textured sodium bismuth titanate (Na1/2Bi1/2TiO3-BaTiO3) ceramics—II dielectric and piezoelectric properties. J. Electroceramics 2003, 11, 217–226. [Google Scholar] [CrossRef]
- Bunge, H.J. Texture Analysis in Materials Science: Mathematical Methods; Butterworths: Boston, UK, 1982. [Google Scholar]
- Roe, R.-J.J.; Krigbaum, W.R. Description of crystallite orientation in polycrystalline materials having fiber texture. J. Chem. Phys. 1964, 40, 2608–2615. [Google Scholar] [CrossRef]
- Roe, R.J. Description of crystallite orientation in polycrystalline materials. 3. general solution to pole figure inversion. J. Appl. Phys. 1965, 36, 2024–2031. [Google Scholar] [CrossRef]
- Matthies, S.; Vinel, G.W. On the reproduction of the orientation distribution function of texturized samples from reduced pole figures using the conception of a conditional ghost correction. Phys. Status Solidi B Basic Solid State Phys. 1982, 112, K111–K114. [Google Scholar] [CrossRef]
- Vanhoutte, P. A method for the generation of various ghost correction algorithms—The example of the positivity method and the exponential method. Textures Microstruct. 1991, 13, 199–212. [Google Scholar] [CrossRef] [Green Version]
- Williams, R.O. Analytical methods for representing complex textures by biaxial pole figures. J. Appl. Phys. 1968, 39, 4329–4335. [Google Scholar] [CrossRef]
- Imhof, J. Determination of a approximation of orientation distribution function using one pole figure. Zeitschrift Met. 1977, 68, 38–43. [Google Scholar]
- Pawlik, K.; Pospieeh, J.; Lficke, K.; Pospiech, J.; Lucke, K. The odf approximation from pole figures with the aid of the adc method. Textures Microstruct. 1991, 14, 25–30. [Google Scholar] [CrossRef]
- Schaeben, H. Entropy optimization in quantitative texture analysis. 2. application to pole-to-orientation density inversion. J. Appl. Phys. 1991, 69, 1320–1329. [Google Scholar] [CrossRef]
- Li, S.; Huang, C.-Y.; Bhalla, A.S.; Cross, L.E. 90°-Domain reversal in Pb(Zrx, Ti1−x)O3 ceramics. Ferroelectr. Lett. Sect. 1993, 16, 7–19. [Google Scholar] [CrossRef]
- Wan, S.; Bowman, K.J. Modeling of electric field induced texture in lead zirconate titanate ceramics. J. Mater. Res. 2001, 16, 2306–2313. [Google Scholar] [CrossRef]
- Jones, J.L.; Vogel, S.C.; Slamovich, E.B.; Bowman, K.J. Quantifying texture in ferroelectric bismuth titanate ceramics. Scr. Mater. 2004, 51, 1123–1127. [Google Scholar] [CrossRef]
- Jones, J.L.; Slamovich, E.B.; Bowman, K.J. Domain texture distributions in tetragonal lead zirconate titanate by x-ray and neutron diffraction. J. Appl. Phys. 2005, 97, 034113. [Google Scholar] [CrossRef]
- Vaudin, M.D.; Rupich, M.W.; Jowett, M.; Riley, G.N.; Bingert, J.F. A method for crystallographic texture investigations using standard X-ray equipment. J. Mater. Res. 1998, 13, 2910–2919. [Google Scholar] [CrossRef] [Green Version]
- Seabaugh, M.M.; Vaudin, M.D.; Cline, J.P.; Messing, G.L. Comparison of texture analysis techniques for highly oriented alpha-Al2O3. J. Am. Ceram. Soc. 2000, 83, 2049–2054. [Google Scholar] [CrossRef]
- Jones, J.L.; Slamovich, E.B.; Bowman, K.J. Critical evaluation of the Lotgering degree of orientation texture indicator. J. Mater. Res. 2011, 19, 3414–3422. [Google Scholar] [CrossRef]
- Poterala, S.F.; Trolier-McKinstry, S.; Meyer, R.J.; Messing, G.L. Processing, texture quality, and piezoelectric properties of <001>C textured (1−x)Pb(Mg1/3Nb2/3)TiO3-xPbTiO3 ceramics. J. Appl. Phys. 2011, 110, 8. [Google Scholar]
- Matthies, S.; Priesmeyer, H.G.; Daymond, M.R. On the diffractive determination of single-crystal elastic constants using polycrystalline samples. J. Appl. Crystallogr. 2001, 34, 585–601. [Google Scholar] [CrossRef] [Green Version]
- Wenk, H.-R.; Pawlik, K.; Pospiech, J.; Kallend, J.S. Deconvolution of Superposed Pole Figures by Discrete ODF Methods: Comparison of ADC and WIMV for Quartz and Calcite with Trigonal Crystal and Triclinic Specimen Symmetry. Textures Microstruct. 1994, 22, 233–260. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.; Daniels, J.E. Quantitative analysis of domain textures in ferroelectric ceramics from single high-energy synchrotron X-ray diffraction images. J. Appl. Phys. 2017, 121, 164102. [Google Scholar] [CrossRef] [Green Version]
- Jones, J.L.; Iverson, B.J.; Bowman, K.J. Texture and anisotropy of polycrystalline piezoelectrics. J. Am. Ceram. Soc. 2007, 90, 2297–2314. [Google Scholar] [CrossRef]
| Lotgering Factor | Experimental Reference | Predicted Reference |
|---|---|---|
| f | 0.945 (7) | 0.946 (7) |
| Methods | Benefits | Limitations |
|---|---|---|
| Lotgering Factor | Qualitative metric to assess the volume fraction of textured material. Helpful in determining the effect of processing on the crystallographic texture. | Only utilizes a single diffraction pattern. Analysis requires data measured using a Bragg-Brentano geometry and does not provide information about angular dependences of the texture. |
| March–Dollase | Determines an estimate for the crystallographic texture of a given hkl pole of interest. | The commonly used formulation is limited to the analysis of materials with a fiber texture. Researchers must account for background and absorption contributions. Analysis requires angular-dependent diffraction data. |
| ODFs | Rigorous method for determining the strength and symmetry of a materials crystallographic texture. | Determining an ODF through Rietveld texture analysis requires information about the crystallographic structure (space group and atomic positions). Analysis requires angular-dependent diffraction data. |
| DOD | Metric to quantify the evolution in the domain alignment in response to a thermomechanical poling process. | Requires precise information about the initial state with a random domain configuration. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fancher, C.M. Diffraction Methods for Qualitative and Quantitative Texture Analysis of Ferroelectric Ceramics. Materials 2021, 14, 5633. https://doi.org/10.3390/ma14195633
Fancher CM. Diffraction Methods for Qualitative and Quantitative Texture Analysis of Ferroelectric Ceramics. Materials. 2021; 14(19):5633. https://doi.org/10.3390/ma14195633
Chicago/Turabian StyleFancher, Chris M. 2021. "Diffraction Methods for Qualitative and Quantitative Texture Analysis of Ferroelectric Ceramics" Materials 14, no. 19: 5633. https://doi.org/10.3390/ma14195633
APA StyleFancher, C. M. (2021). Diffraction Methods for Qualitative and Quantitative Texture Analysis of Ferroelectric Ceramics. Materials, 14(19), 5633. https://doi.org/10.3390/ma14195633





