Figure 1.
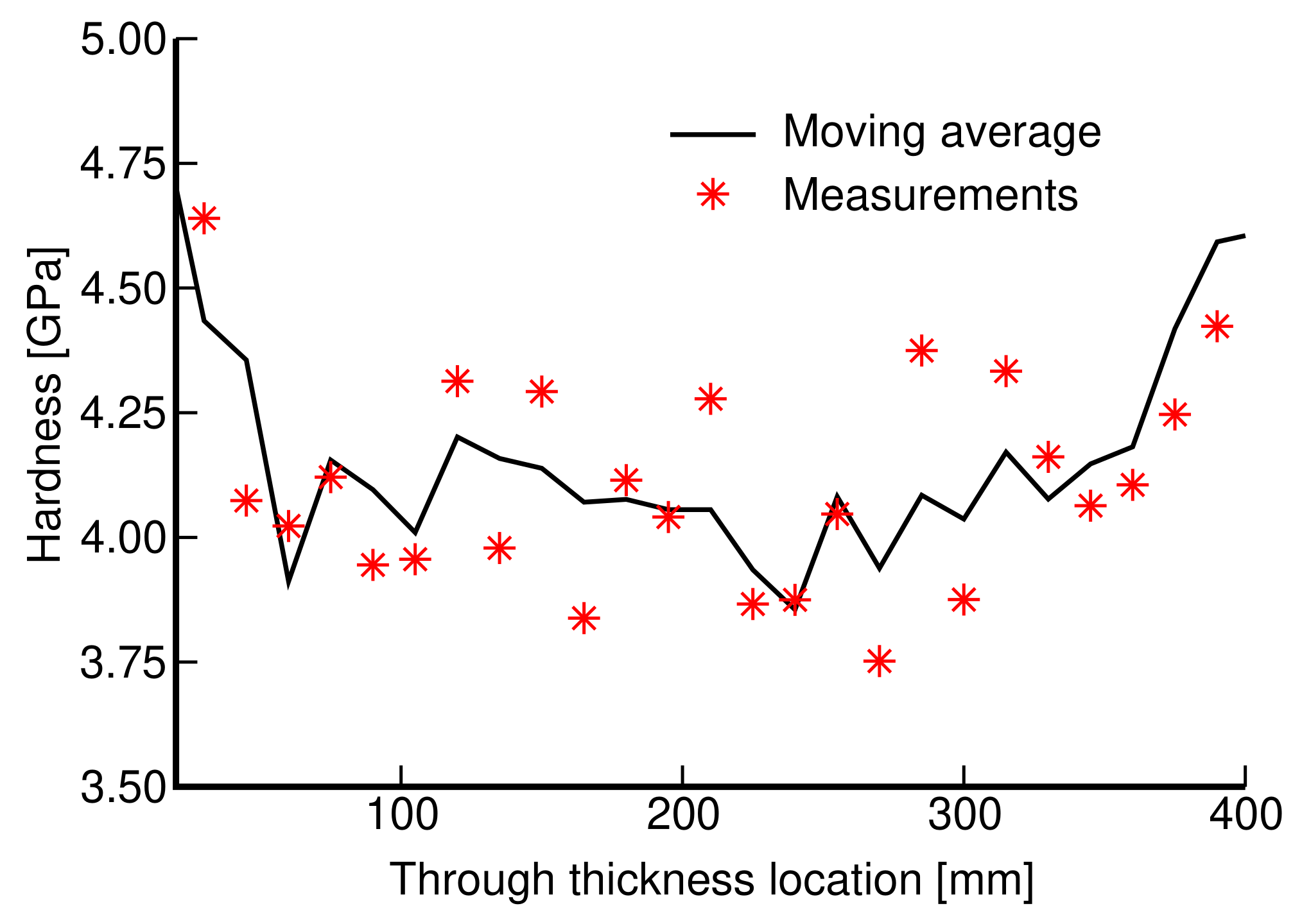
Variation in microhardness throughout the wall thickness.
Figure 1.
Variation in microhardness throughout the wall thickness.
Figure 2.
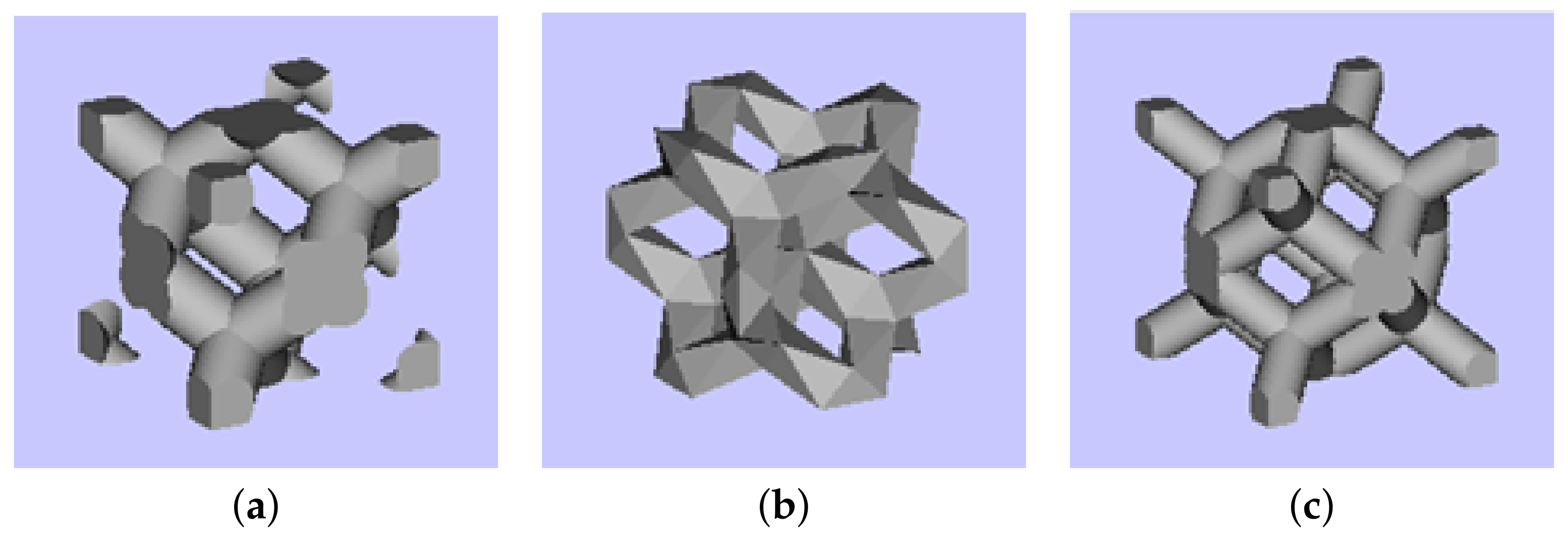
Basic cells of trabecular structures: (a) diamond (D30), relative density 30%, (b) Dode Thick (DT), (c) rhombic dodecahedron (RD30, relative density 30%).
Figure 2.
Basic cells of trabecular structures: (a) diamond (D30), relative density 30%, (b) Dode Thick (DT), (c) rhombic dodecahedron (RD30, relative density 30%).
Figure 3.
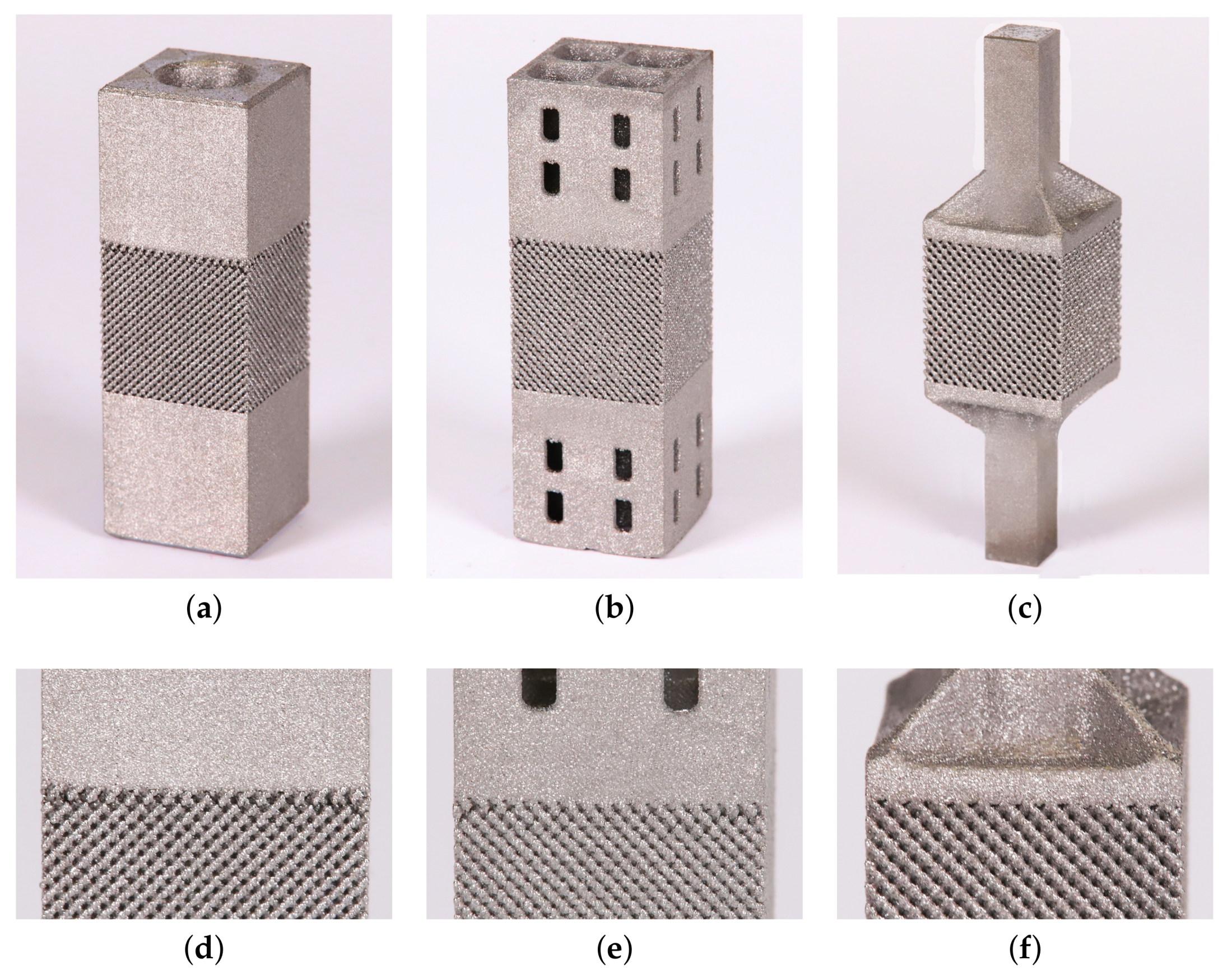
Examples of trabecular specimens made for compression tests: (
a) D30-2, (
b) DT-2, (
c) RD30-2 (
Table 1).
Figure 3.
Examples of trabecular specimens made for compression tests: (
a) D30-2, (
b) DT-2, (
c) RD30-2 (
Table 1).
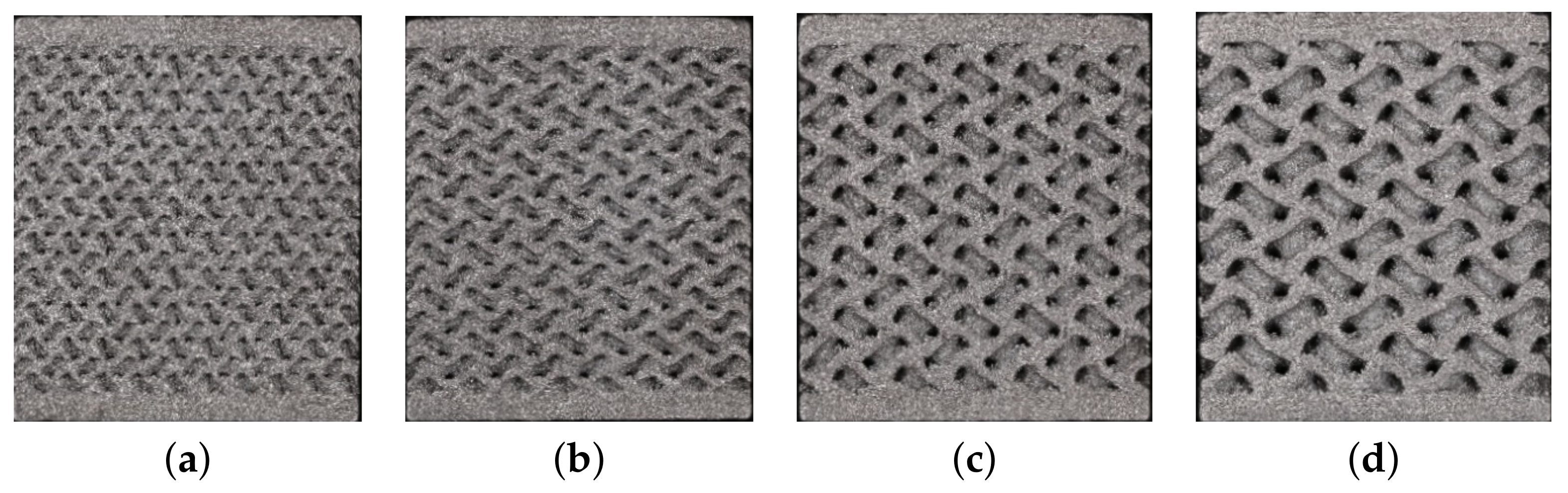
Figure 4.
Defects of trabecular specimens: (a) clusters of slag inside trabecular structure, (b) discontinuities within trabecular structure and variability in strut thickness, (c) discontinuities (debonding) at interface between trabecular and homogeneous part (photographs provided by high-resolution camera Canon 6D Mark II).
Figure 4.
Defects of trabecular specimens: (a) clusters of slag inside trabecular structure, (b) discontinuities within trabecular structure and variability in strut thickness, (c) discontinuities (debonding) at interface between trabecular and homogeneous part (photographs provided by high-resolution camera Canon 6D Mark II).
Figure 5.
Trabecular specimens made for tension tests: (a) variant 1 (TT-V1), (b) variant 2 (TT-V2), (c) variant 3 (TT-V3), (d) visible interface defects TT-V1, (e) visible interface defects TT-V2, (f) no visible interface defects TT-V3.
Figure 5.
Trabecular specimens made for tension tests: (a) variant 1 (TT-V1), (b) variant 2 (TT-V2), (c) variant 3 (TT-V3), (d) visible interface defects TT-V1, (e) visible interface defects TT-V2, (f) no visible interface defects TT-V3.
Figure 6.
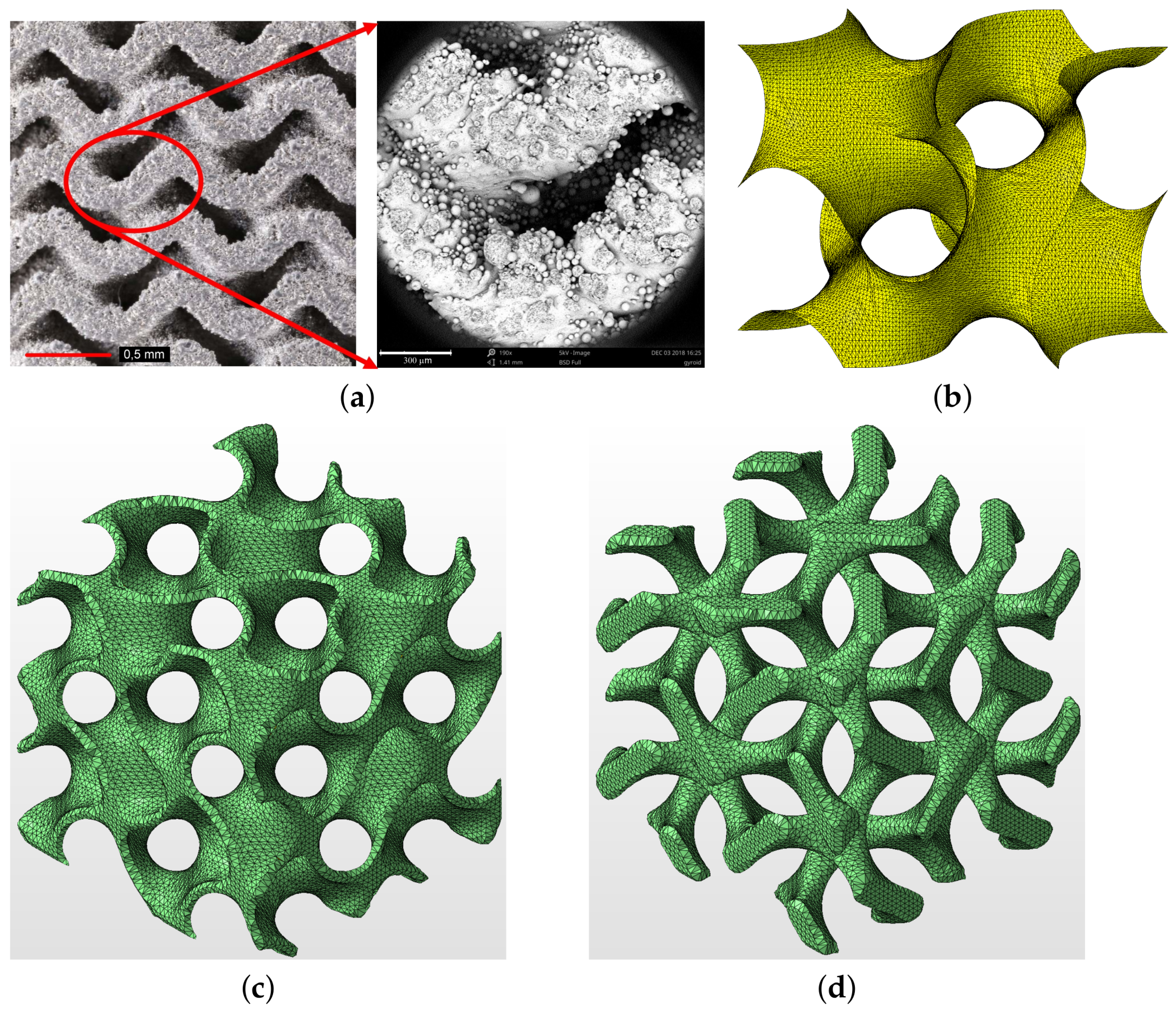
(a) Printed gyroid structure with no visible internal defects (left—photograph provided by high-resolution camera Canon 6D Mark II, right—microscopic image provided by electron microscope SEM Phenom XL), (b) example of one-cell gyroid surface with t = 0, (c) matrix phase gyroid with finite wall thickness μm, (d) inverted matrix phase gyroid assuming μm ((b–d) were generated using Autodesk Netfabb software).
Figure 6.
(a) Printed gyroid structure with no visible internal defects (left—photograph provided by high-resolution camera Canon 6D Mark II, right—microscopic image provided by electron microscope SEM Phenom XL), (b) example of one-cell gyroid surface with t = 0, (c) matrix phase gyroid with finite wall thickness μm, (d) inverted matrix phase gyroid assuming μm ((b–d) were generated using Autodesk Netfabb software).
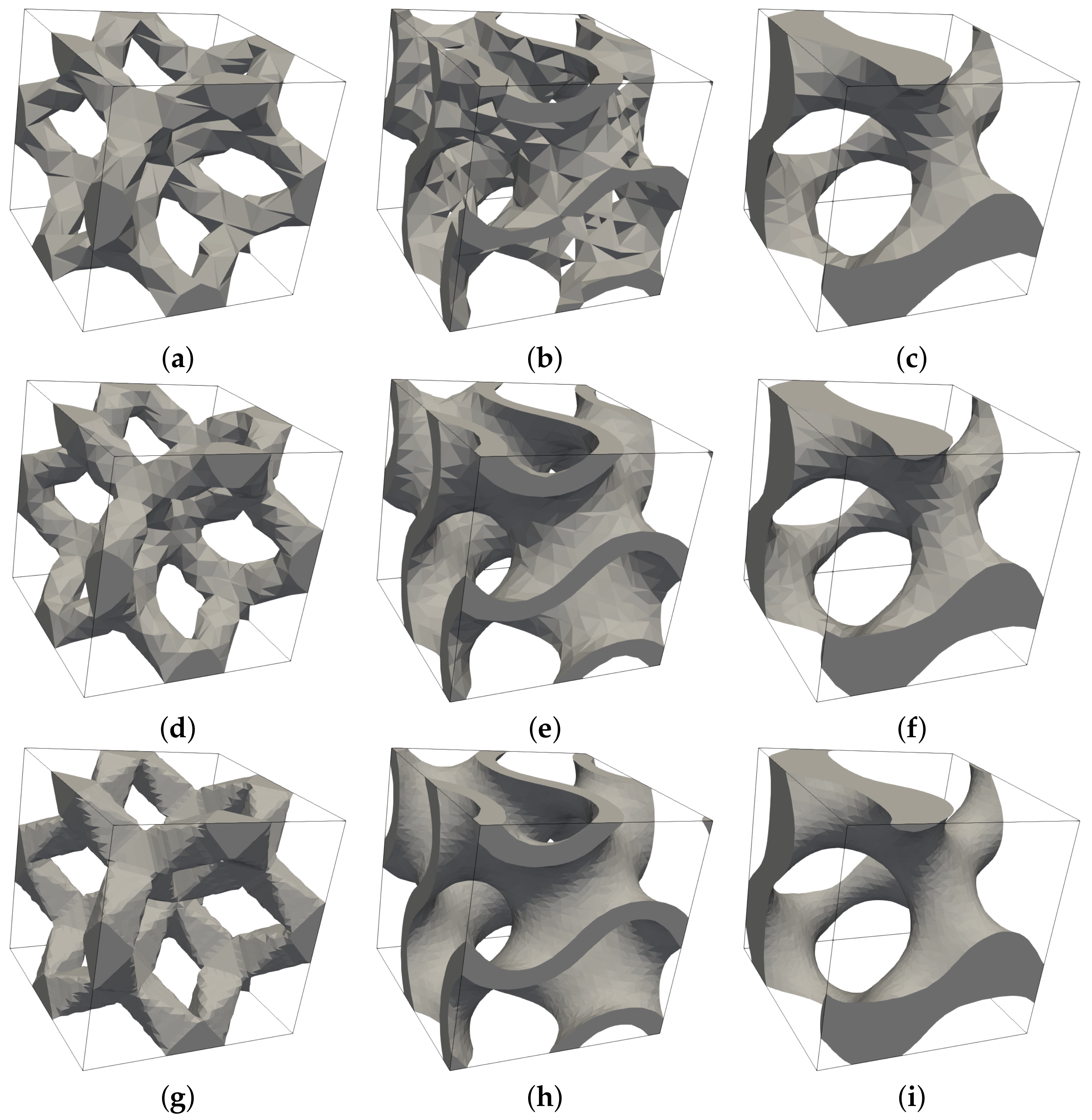
Figure 7.
Basic cells of gyroid structures—Phase I: (a) G1-I, (b) G2-I, (c) G3-I, (d) G4-I.
Figure 7.
Basic cells of gyroid structures—Phase I: (a) G1-I, (b) G2-I, (c) G3-I, (d) G4-I.
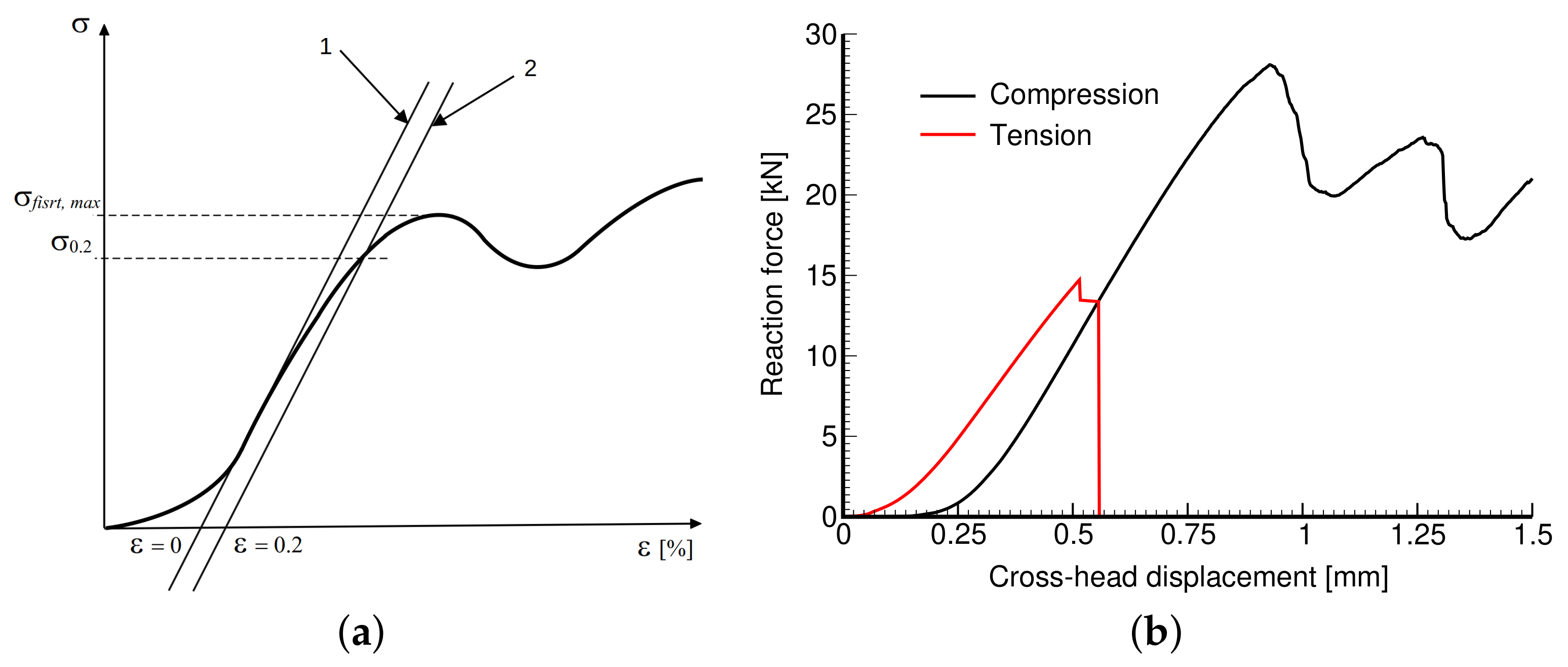
Figure 8.
Engineering stress vs. engineering strain curve: (a) definition of basic mechanical parameters, (b) illustration of compressive and tensile stress–strain diagrams obtained experimentally.
Figure 8.
Engineering stress vs. engineering strain curve: (a) definition of basic mechanical parameters, (b) illustration of compressive and tensile stress–strain diagrams obtained experimentally.
Figure 9.
Fractured specimens undergoing tension: (a) failure at gripping section (samples 2, 3—porous structure corresponds to GII-2), (b) location of fracture surfaces near top base (porous structure corresponds to GII-3, specimens are positioned upside-down from the printing direction point of view).
Figure 9.
Fractured specimens undergoing tension: (a) failure at gripping section (samples 2, 3—porous structure corresponds to GII-2), (b) location of fracture surfaces near top base (porous structure corresponds to GII-3, specimens are positioned upside-down from the printing direction point of view).
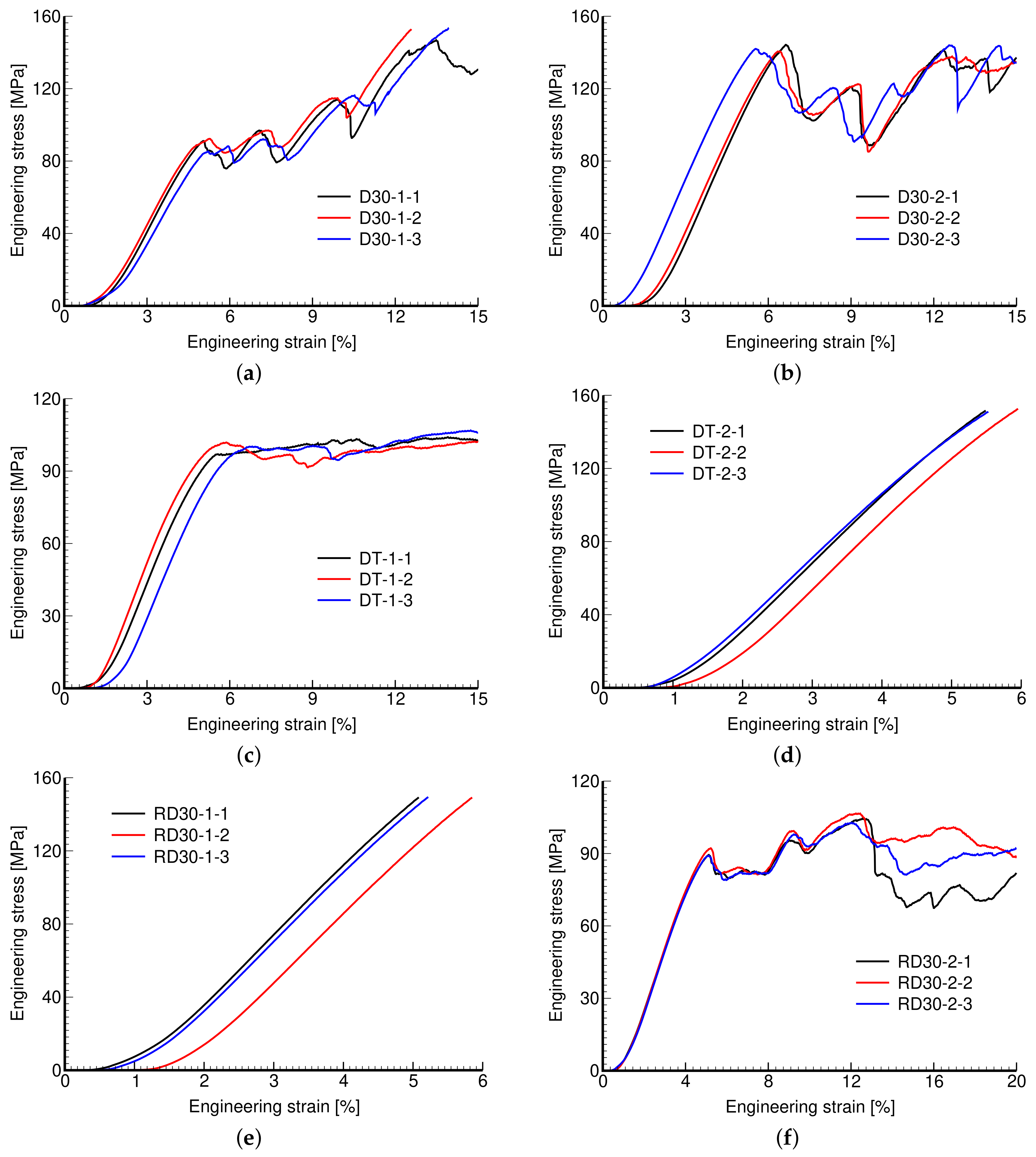
Figure 10.
Engineering stress vs. engineering strain curves: (a) D30-1, (b) D30-2, (c) DT-1, (d) DT-2, (e) RD30-1, (f) RD30-2.
Figure 10.
Engineering stress vs. engineering strain curves: (a) D30-1, (b) D30-2, (c) DT-1, (d) DT-2, (e) RD30-1, (f) RD30-2.
Figure 11.
Engineering stress vs. engineering strain curves: (a) GI-1, (b) GI-2, (c) GI-3, (d) GI-4.
Figure 11.
Engineering stress vs. engineering strain curves: (a) GI-1, (b) GI-2, (c) GI-3, (d) GI-4.
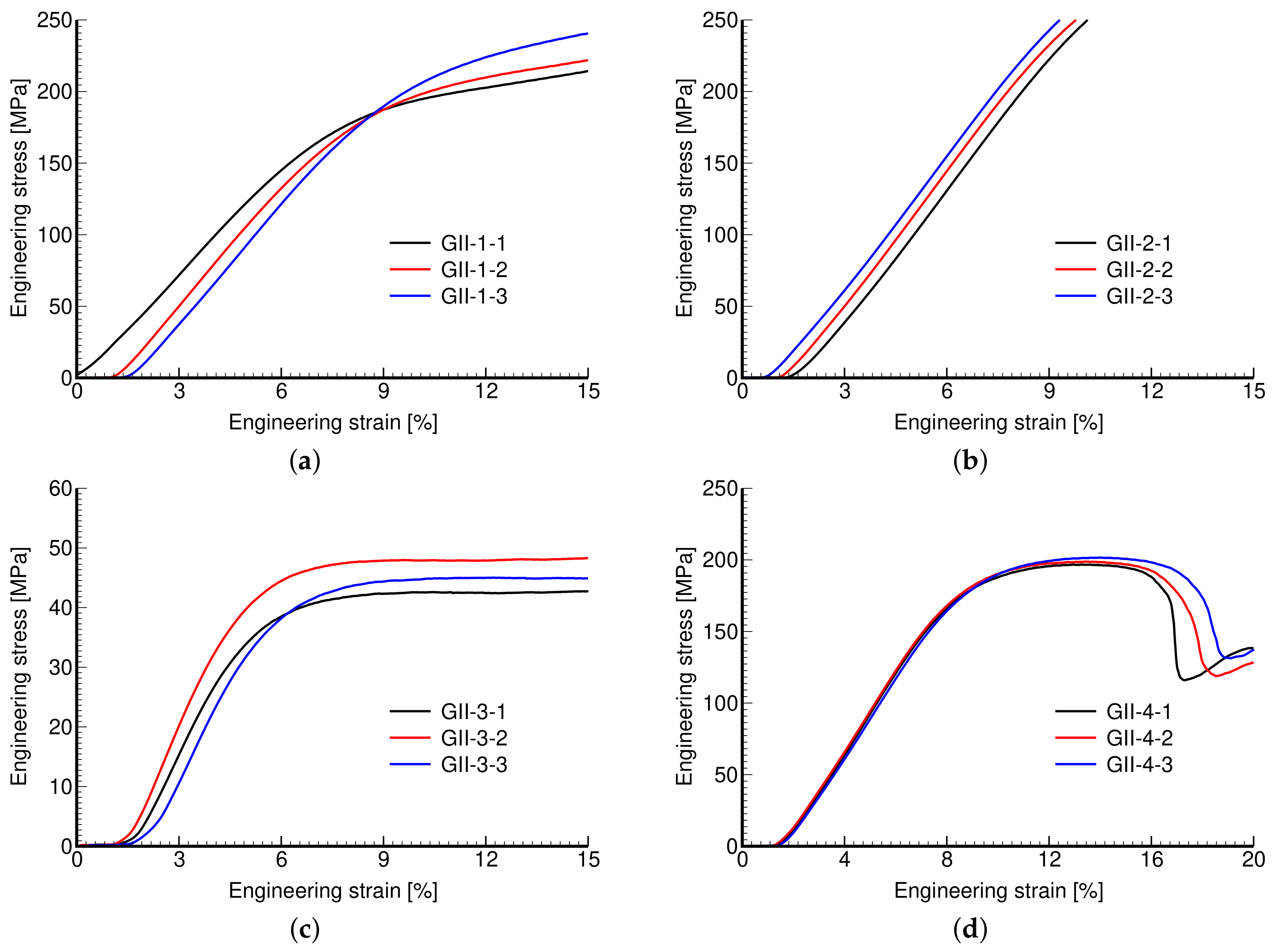
Figure 12.
Engineering stress vs. engineering strain curves: (a) GII-1, (b) GII-2, (c) GII-3, (d) GII-4.
Figure 12.
Engineering stress vs. engineering strain curves: (a) GII-1, (b) GII-2, (c) GII-3, (d) GII-4.
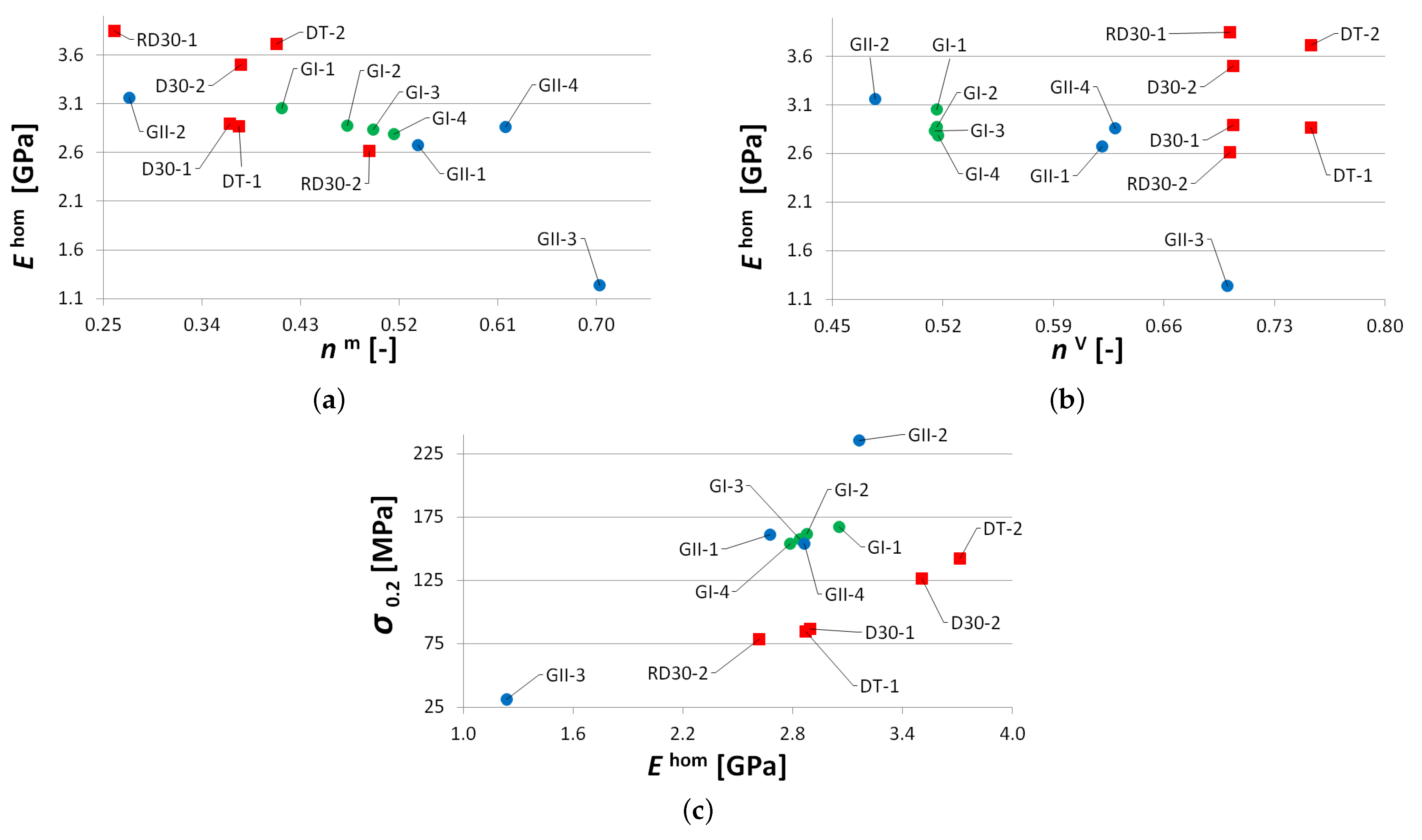
Figure 13.
Comparing mechanical properties of trabecular and gyroid structures: (a) Young’s modulus vs. actual porosity , (b) Young’s modulus vs. theoretical porosity , (c) yield strength in compression vs. Young’s modulus.
Figure 13.
Comparing mechanical properties of trabecular and gyroid structures: (a) Young’s modulus vs. actual porosity , (b) Young’s modulus vs. theoretical porosity , (c) yield strength in compression vs. Young’s modulus.
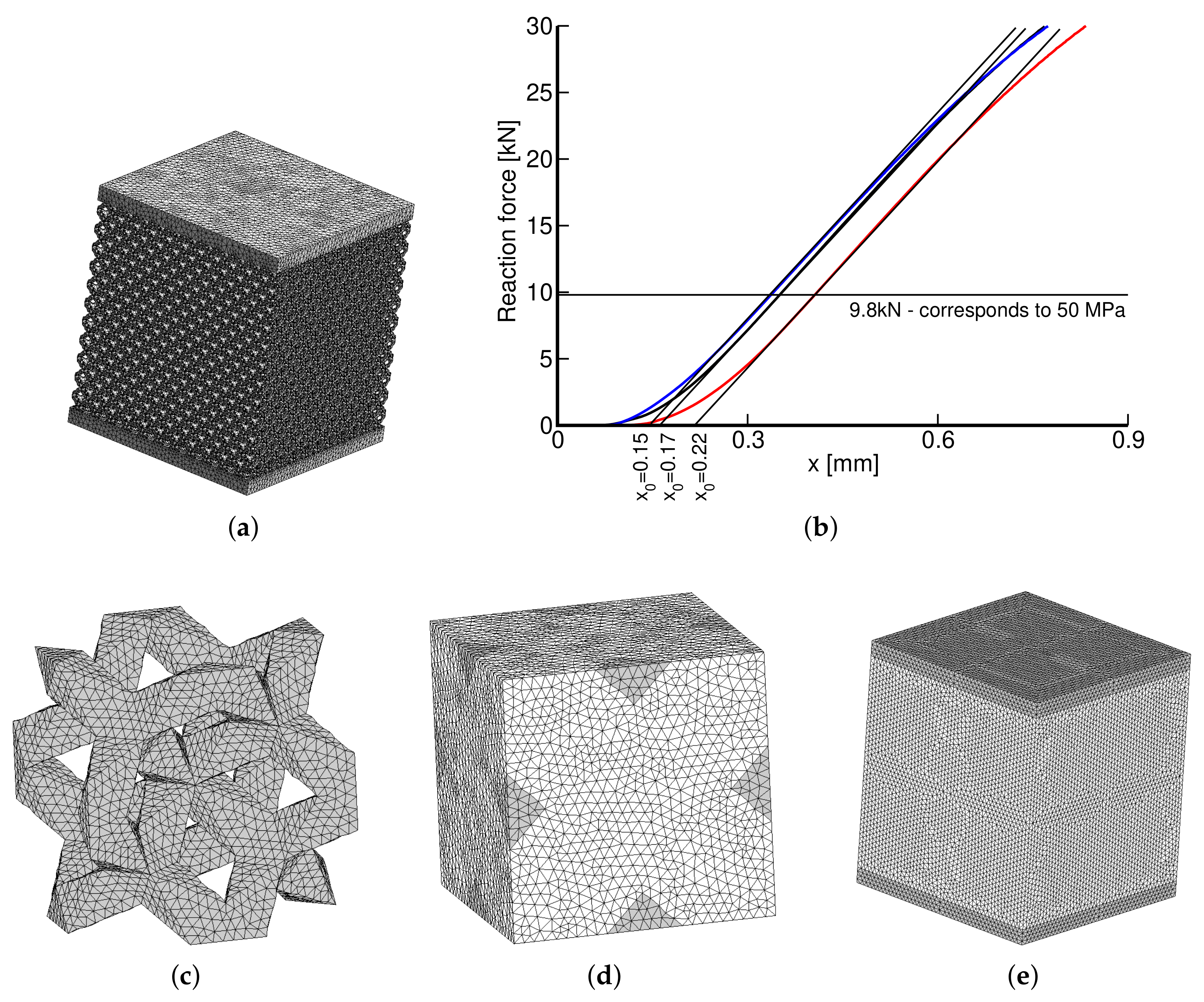
Figure 14.
(
a) Detailed FE model of 1/8 of DT-2 specimen, (
b) measured loading curves (
x—cross-head displacement), (
c) solid part of DT-2 PUC (recall
Figure 2b), (
d) PUC finite element mesh, (
e) coarse FE model of homogenized specimen.
Figure 14.
(
a) Detailed FE model of 1/8 of DT-2 specimen, (
b) measured loading curves (
x—cross-head displacement), (
c) solid part of DT-2 PUC (recall
Figure 2b), (
d) PUC finite element mesh, (
e) coarse FE model of homogenized specimen.
Figure 15.
Finite element meshes of basic cells of trabecular structure: (a) D30-1, (b) DT-1, (c) RD30-1.
Figure 15.
Finite element meshes of basic cells of trabecular structure: (a) D30-1, (b) DT-1, (c) RD30-1.
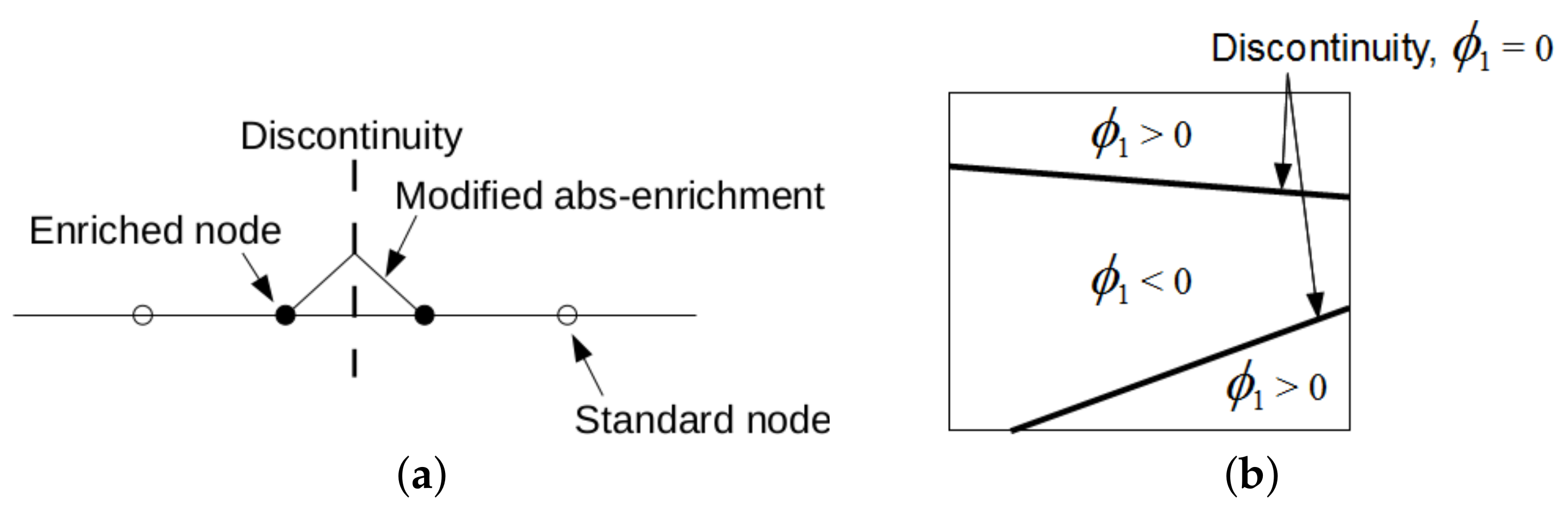
Figure 16.
(a) Modified abs-enrichment for 1D problem, (b) element crossed by two interfaces of the same material phase.
Figure 16.
(a) Modified abs-enrichment for 1D problem, (b) element crossed by two interfaces of the same material phase.
Figure 17.
(a–c) Geometry of specimens tested experimentally in uniaxial compression: (a) DT-2, (b) GS, (c) GT; (d–f) basic unit cells: (d) DT-2, (e) GS, (f) GT.
Figure 17.
(a–c) Geometry of specimens tested experimentally in uniaxial compression: (a) DT-2, (b) GS, (c) GT; (d–f) basic unit cells: (d) DT-2, (e) GS, (f) GT.
Figure 18.
Experimentally derived force×displacement curves for specimens in
Figure 17a–c.
Figure 18.
Experimentally derived force×displacement curves for specimens in
Figure 17a–c.
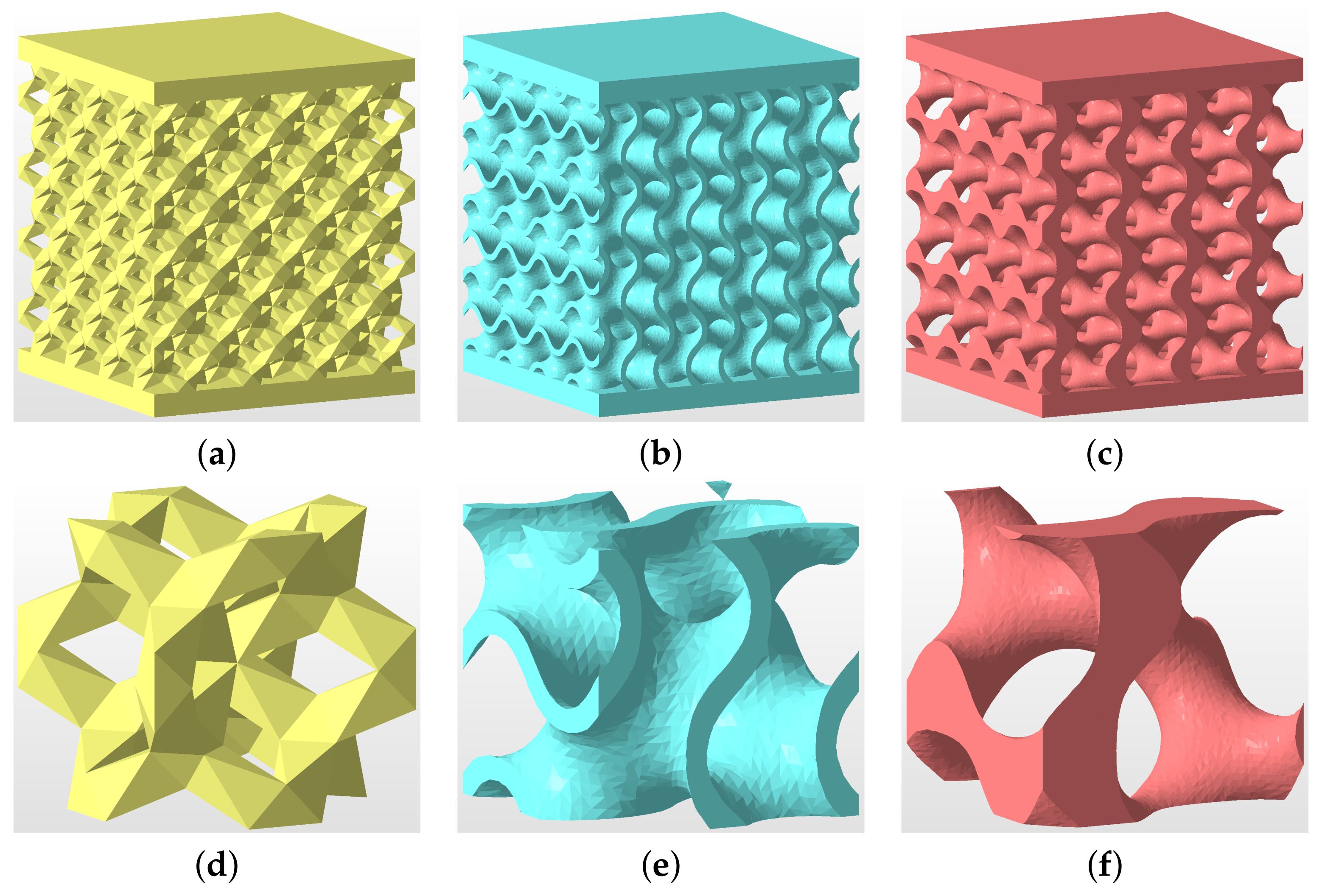
Figure 19.
Influence of mesh refinement on approximation of solid phase by X-FEM: (a–c) subdivision into 10 × 10 ×10 brick elements, (d–f) subdivision into 15 × 15 × 15 brick elements, (g–i) subdivision into 35 × 35 × 35 brick elements.
Figure 19.
Influence of mesh refinement on approximation of solid phase by X-FEM: (a–c) subdivision into 10 × 10 ×10 brick elements, (d–f) subdivision into 15 × 15 × 15 brick elements, (g–i) subdivision into 35 × 35 × 35 brick elements.
Table 1.
Types and geometry of basic trabecular cell units (D30—diamond, relative density 30%, DT—Dode Thick, RD30—rhombic dodecahedron, relative density 30%).
Table 1.
Types and geometry of basic trabecular cell units (D30—diamond, relative density 30%, DT—Dode Thick, RD30—rhombic dodecahedron, relative density 30%).
| Unit Type | L [μm] | δs [μm] | d [μm] | [-] | [-] | NofCellsEdge |
|---|
| D30-1 | 750 | 200 | 350 | 0.37 | 0.70 | 18 |
| D30-2 | 1000 | 260 | 450 | 0.38 | 0.70 | 14 |
| DT-1 | 1000 | 200 | 500 | 0.37 | 0.75 | 14 |
| DT-2 | 1250 | 250 | 630 | 0.41 | 0.75 | 11.5 |
| RD30-1 | 1250 | 230 | 640 | 0.26 | 0.70 | 11.5 |
| RD30-2 | 1500 | 290 | 800 | 0.49 | 0.70 | 9.5 |
Table 2.
Types and geometry of basic gyroid cell units—Phase I.
Table 2.
Types and geometry of basic gyroid cell units—Phase I.
| Unit Type | L [μm] | d [μm] | [-] | [-] | NofCellsEdge |
|---|
| GI-1 | 1400 | 400 | 0.41 | 0.52 | 10 |
| GI-2 | 1800 | 450 | 0.47 | 0.52 | 7.78 |
| GI-3 | 2400 | 700 | 0.50 | 0.52 | 5.83 |
| GI-4 | 3000 | 800 | 0.52 | 0.52 | 4.67 |
Table 3.
Types and geometry of basic gyroid cell units—Phase II.
Table 3.
Types and geometry of basic gyroid cell units—Phase II.
| Unit Type | L [μm] | δs [μm] | d [μm] | [-] | [-] | NofCellsEdge |
|---|
| GII-1 | 1800 | 150 | 450 | 0.54 | 0.62 | 7.78 |
| GII-2 | 1800 | 250 | 450 | 0.27 | 0.48 | 7.78 |
| GII-3 | 2700 | 150 | 750 | 0.70 | 0.70 | 5.18 |
| GII-4 | 2700 | 250 | 750 | 0.62 | 0.63 | 5.18 |
Table 4.
Mechanical properties of trabecular structures from experiments.
Table 4.
Mechanical properties of trabecular structures from experiments.
| Unit Type | E [GPa] | σ0.2 [MPa] | σfirst,max [MPa] | [-] | [-] |
|---|
| D30-1 | 2.88 | 86.7 | 88.7 | 0.37 | 0.70 |
| D30-2 | 3.51 | 126.5 | 141.9 | 0.38 | 0.70 |
| DT-1 | 2.84 | 84.7 | 98.2 | 0.37 | 0.75 |
| DT-2 | 3.71 | 142.2 | - | 0.41 | 0.75 |
| RD30-1 | 3.82 | - | - | 0.26 | 0.70 |
| RD30-2 | 2.63 | 78.4 | 90.2 | 0.49 | 0.70 |
Table 5.
Mechanical properties of gyroid structures from experiments.
Table 5.
Mechanical properties of gyroid structures from experiments.
| Unit Type | E [GPa] | σ0.2 [MPa] | σfirst,max [MPa] | [-] | [-] |
|---|
| GI-1 | 3.05 | 166.9 | 228.5 | 0.41 | 0.52 |
| GI-2 | 2.87 | 161.6 | 214.5 | 0.47 | 0.52 |
| GI-3 | 2.84 | 157.8 | 190.7 | 0.50 | 0.52 |
| GI-4 | 2.77 | 154.1 | 191.4 | 0.52 | 0.52 |
| GII-1 | 2.67 | 161.3 | - | 0.54 | 0.62 |
| GII-2 | 3.16 | 235.8 | - | 0.27 | 0.48 |
| GII-3 | 1.24 | 31.0 | - | 0.70 | 0.70 |
| GII-4 | 2.86 | 154.1 | 199.0 | 0.62 | 0.63 |
Table 6.
Details of finite element models.
Table 6.
Details of finite element models.
| Mesh Details | Detailed Model | Homogeneous Model | PUC |
|---|
| Number of nodes | 304,289 | 3461 | 25,061 |
| Number of elements | 1,016,821 | 17,285 | 110,427 |
| Computational time | 2 h and 13 min | 6 s | 34 s |
Table 7.
Results from initial comparative study on DT-2 geometry.
Table 7.
Results from initial comparative study on DT-2 geometry.
| Model | E [GPa] | ν [-] | Δh [mm] |
|---|
| Detailed model | 4.11 | - | 0.17 |
| Homogeneous model | - | - | 0.18 |
| PUC | 3.56 | 0.244 | - |
| Experiment | 3.71 | - | 0.19 |
Table 8.
Effective elastic properties of trabecular structures from homogenization.
Table 8.
Effective elastic properties of trabecular structures from homogenization.
|
Unit Type | Experiment | Homogenization |
|---|
| E [GPa] | E [GPa] | ν [-] | [-] | Num. Nodes | Num. Elems |
|---|
| D30-1 | 2.88 | 4.42 | 0.296 | 0.704 | 50,045 | 263,404 |
| D30-2 | 3.51 | 4.41 | 0.295 | 0.705 | 49,551 | 260,533 |
| DT-1 | 2.84 | 3.39 | 0.246 | 0.754 | 47,573 | 232,019 |
| DT-2 | 3.71 | 3.56 | 0.244 | 0.756 | 25,061 | 110,427 |
| RD30-1 | 3.82 | 5.94 | 0.296 | 0.703 | 61,172 | 329,417 |
| RD30-2 | 2.63 | 5.97 | 0.277 | 0.703 | 60,916 | 328,003 |
Table 9.
Effective elastic properties of structures in
Figure 17 from measurements and homogenization.
Table 9.
Effective elastic properties of structures in
Figure 17 from measurements and homogenization.
| Unit Type | Experiment | Homogenization |
|---|
| E [MPa] | E [MPa] (X-FEM) | E [MPa] (FEM) |
|---|
| DT-2 | 27.3 ± 3.8 | 26.4 | 25.9 |
| GS | 28.7 ± 1.8 | 33.2 | - |
| GT | 72.4 ± 6.1 | 82.9 | - |
Table 10.
Effective elastic properties of gyroid structures from homogenization.
Table 10.
Effective elastic properties of gyroid structures from homogenization.
| Unit Type | Experiment | Homogenization |
|---|
| E [GPa] | E [MPa] | ν [-] | () [-] | Num. Dofs. |
|---|
| GI | 2.88 (mean) | 28.6 | 0.29 | 0.51 (0.52) | 191,043 |
| GII-1 | 2.67 | 19.6 | 0.29 | 0.62 (0.62) | 194,436 |
| GII-2 | 3.16 | 32.3 | 0.28 | 0.47 (0.48) | 189,432 |
| GII-3 | 1.24 | 14.0 | 0.31 | 0.70 (0.70) | 195,636 |
| GII-4 | 2.86 | 18.2 | 0.30 | 0.63 (0.63) | 194,724 |