Structural Properties of Thin ZnO Films Deposited by ALD under O-Rich and Zn-Rich Growth Conditions and Their Relationship with Electrical Parameters
Abstract
:1. Introduction
2. Growth Details and Used Experimental Techniques
3. Experimental Results and Discussion
3.1. XRD: Film Texture/Preferred Orientation and Dislocation Density
3.1.1. AFM: Surface Morphology
3.1.2. Crystallite Size
3.1.3. Structural Defects and Dislocation Density
3.1.4. Strain Analysis
3.2. Connection between XRD and Optical Data
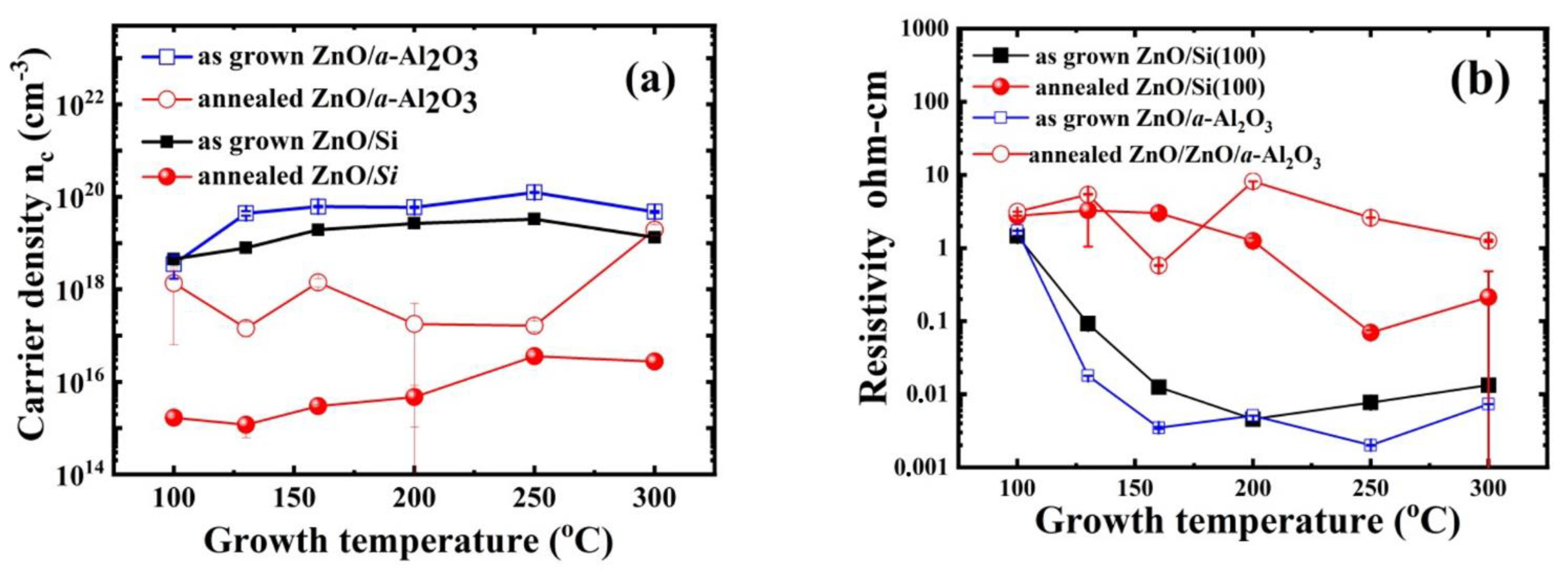
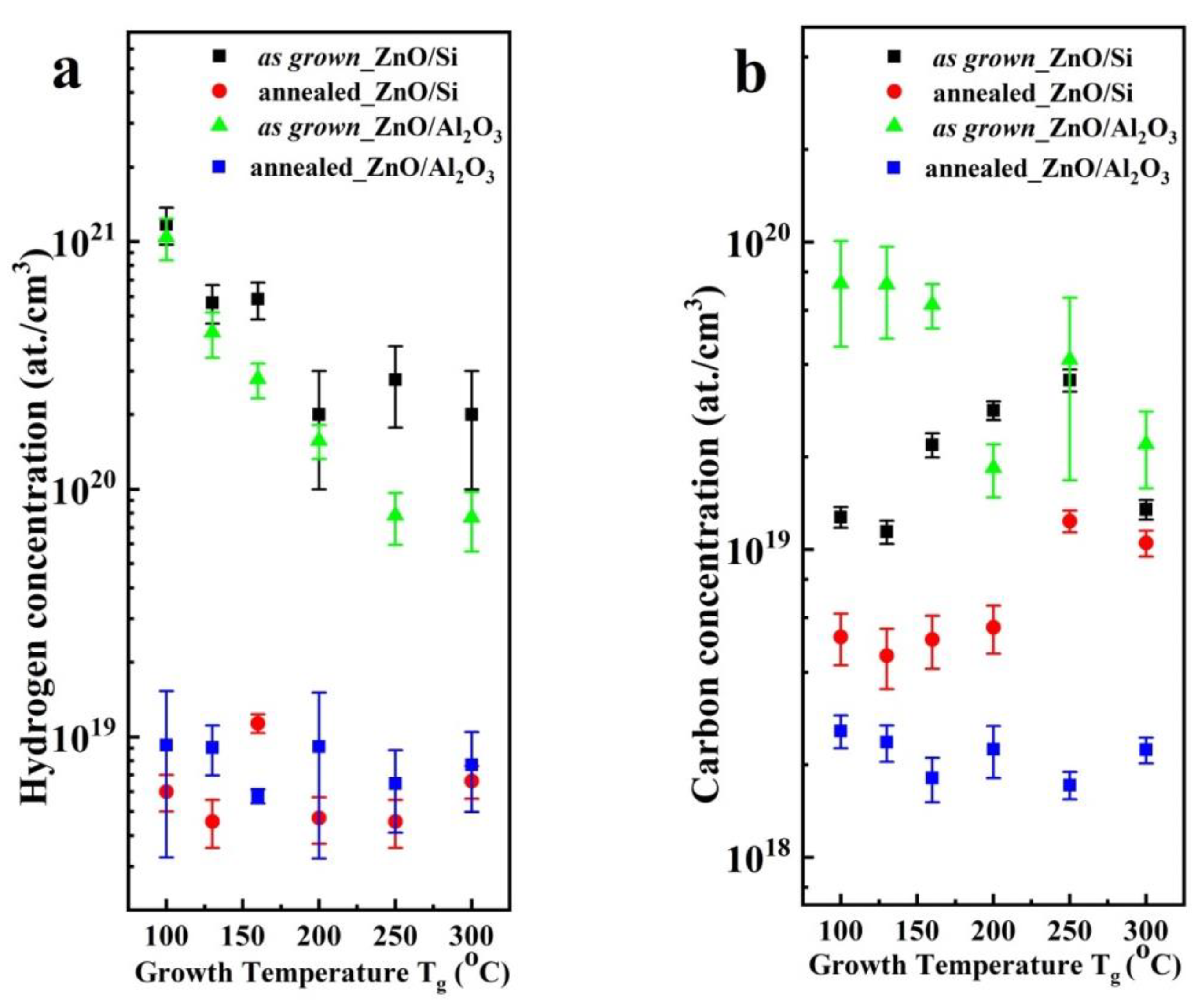
3.3. Electrical Properties and Impurity Concentration
3.4. Grain Boundary Effect on Electrical Conductivity
4. Summary and Outlook
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Özgür, Ü.; Alivov, Y.I.; Liu, C.; Teke, A.; Reshchikov, M.A.; Doğan, S.; Avrutin, V.; Cho, S.-J.; Morkoç, H. A comprehensive review of ZnO materials and devices. J. Appl. Phys. 2005, 98, 041301. [Google Scholar] [CrossRef] [Green Version]
- Janotti, A.; de Walle, C.G. Van Hydrogen multicentre bonds. Nat. Mater. 2006, 6, 44–47. [Google Scholar] [CrossRef] [PubMed]
- Janotti, A.; Van de Walle, C.G. Native point defects in ZnO. Phys. Rev. B 2007, 76, 165202. [Google Scholar] [CrossRef]
- Oba, F.; Choi, M.; Togo, A.; Tanaka, I. Point defects in ZnO: An approach from first principles. Sci. Technol. Adv. Mater. 2011, 12, 034302. [Google Scholar] [CrossRef] [PubMed]
- Vidya, R.; Ravindran, P.; Fjellvåg, H.; Svensson, B.G.; Monakhov, E.; Ganchenkova, M.; Nieminen, R.M. Energetics of intrinsic defects and their complexes in ZnO investigated by density functional calculations. Phys. Rev. B 2011, 83, 045206. [Google Scholar] [CrossRef] [Green Version]
- Janotti, A.; de Walle, C.G. Van Fundamentals of zinc oxide as a semiconductor. Rep. Prog. Phys. 2009, 72, 126501. [Google Scholar] [CrossRef] [Green Version]
- Van de Walle, C.G. Hydrogen as a Cause of Doping in Zinc Oxide. Phys. Rev. Lett. 2000, 85, 1012–1015. [Google Scholar] [CrossRef] [Green Version]
- Park, Y.R.; Kim, J.; Kim, Y.S. Effect of hydrogen doping in ZnO thin films by pulsed DC magnetron sputtering. Appl. Surf. Sci. 2009, 255, 9010–9014. [Google Scholar] [CrossRef]
- Shi, G.A.; Saboktakin, M.; Stavola, M.; Pearton, S.J. “Hidden hydrogen” in as-grown ZnO. Appl. Phys. Lett. 2004, 85, 5601–5603. [Google Scholar] [CrossRef]
- Du, M.-H.; Biswas, K. Anionic and Hidden Hydrogen in ZnO. Phys. Rev. Lett. 2011, 106, 115502. [Google Scholar] [CrossRef] [Green Version]
- Villafuerte, J.; Chaix-Pluchery, O.; Kioseoglou, J.; Donatini, F.; Sarigiannidou, E.; Pernot, J.; Consonni, V. Engineering nitrogen- and hydrogen-related defects in ZnO nanowires using thermal annealing. Phys. Rev. Mater. 2021, 5, 056001. [Google Scholar] [CrossRef]
- Li, W.; Zhang, H.; Zhang, X.; Qin, G.; Li, H.; Xiong, Y.; Ye, L.; Ruan, H.; Tong, C.; Kong, C.; et al. Non-axial NO-VZn shallow acceptor complexes in nitrogen implanted p-type ZnO thin films. Appl. Surf. Sci. 2020, 529, 147168. [Google Scholar] [CrossRef]
- Wang, Z.; Luo, C.; Anwand, W.; Wagner, A.; Butterling, M.; Rahman, M.A.; Phillips, M.R.; Ton-That, C.; Younas, M.; Su, S.; et al. Vacancy cluster in ZnO films grown by pulsed laser deposition. Sci. Rep. 2019, 9, 3534. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Abliz, A.; Xue, X.; Liu, X.; Li, G.; Tang, L. Rational design of hydrogen and nitrogen co-doped ZnO for high performance thin-film transistors. Appl. Phys. Lett. 2021, 118, 123504. [Google Scholar] [CrossRef]
- Frodason, Y.K.; Johansen, K.M.; Bjørheim, T.S.; Svensson, B.G.; Alkauskas, A. Zn vacancy-donor impurity complexes in ZnO. Phys. Rev. B 2018, 97, 104109. [Google Scholar] [CrossRef] [Green Version]
- Schifano, R.; Jakiela, R.; Galeckas, A.; Kopalko, K.; Herklotz, F.; Johansen, K.M.H.; Vines, L. Role of intrinsic and extrinsic defects in H implanted hydrothermally grown ZnO. J. Appl. Phys. 2019, 126, 125707. [Google Scholar] [CrossRef]
- Heinhold, R.; Neiman, A.; Kennedy, J.V.; Markwitz, A.; Reeves, R.J.; Allen, M.W. Hydrogen-related excitons and their excited-state transitions in ZnO. Phys. Rev. B 2017, 95, 054120. [Google Scholar] [CrossRef]
- Guziewicz, E.; Krajewski, T.A.; Przezdziecka, E.; Korona, K.P.; Czechowski, N.; Klopotowski, L.; Terziyska, P. Zinc Oxide Grown by Atomic Layer Deposition: From Heavily n-Type to p-Type Material. Phys. Status Solidi 2019, 257, 1900472. [Google Scholar] [CrossRef]
- Lyons, J.L.; Varley, J.B.; Steiauf, D.; Janotti, A.; Van de Walle, C.G. First-principles characterization of native-defect-related optical transitions in ZnO. J. Appl. Phys. 2017, 122, 035704. [Google Scholar] [CrossRef]
- Zhang, S.B.; Wei, S.-H.; Zunger, A. Intrinsic n-type versus p-type doping asymmetry and the defect physics of ZnO. Phys. Rev. B 2001, 63, 075205. [Google Scholar] [CrossRef] [Green Version]
- Przezdziecka, E.; Guziewicz, E.; Jarosz, D.; Snigurenko, D.; Sulich, A.; Sybilski, P.; Jakiela, R.; Paszkowicz, W. Influence of oxygen-rich and zinc-rich conditions on donor and acceptor states and conductivity mechanism of ZnO films grown by ALD—Experimental studies. J. Appl. Phys. 2020, 127, 075104. [Google Scholar] [CrossRef]
- Przeździecka, E.; Wachnicki, Ł.; Paszkowicz, W.; Łusakowska, E.; Krajewski, T.; Łuka, G.; Guziewicz, E.; Godlewski, M. Photoluminescence, electrical and structural properties of ZnO films, grown by ALD at low temperature. Semicond. Sci. Technol. 2009, 24, 105014. [Google Scholar] [CrossRef]
- Krajewski, T.A.; Dybko, K.; Luka, G.; Guziewicz, E.; Nowakowski, P.; Witkowski, B.S.; Jakiela, R.; Wachnicki, L.; Kaminska, A.; Suchocki, A.; et al. Dominant shallow donors in zinc oxide layers obtained by low-temperature atomic layer deposition: Electrical and optical investigations. Acta Mater. 2014, 65, 69–75. [Google Scholar] [CrossRef]
- Lin, Y.-Y.; Hsu, C.-C.; Tseng, M.-H.; Shyue, J.-J.; Tsai, F.-Y. Stable and High-Performance Flexible ZnO Thin-Film Transistors by Atomic Layer Deposition. ACS Appl. Mater. Interfaces 2015, 7, 22610–22617. [Google Scholar] [CrossRef]
- Fang, L.; Li, H.; Ma, X.; Song, Q.; Chen, R. Optical properties of ultrathin ZnO films fabricated by atomic layer deposition. Appl. Surf. Sci. 2020, 527, 146818. [Google Scholar] [CrossRef]
- Guziewicz, E.; Godlewski, M.; Wachnicki, L.; Krajewski, T.A.; Luka, G.; Gieraltowska, S.; Jakiela, R.; Stonert, A.; Lisowski, W.; Krawczyk, M.; et al. ALD grown zinc oxide with controllable electrical properties. Semicond. Sci. Technol. 2012, 27, 074011. [Google Scholar] [CrossRef] [Green Version]
- Krajewski, T.A.; Luka, G.; Wachnicki, L.; Zakrzewski, A.J.; Witkowski, B.S.; Lukasiewicz, M.I.; Kruszewski, P.; Lusakowska, E.; Jakiela, R.; Godlewski, M.; et al. Electrical parameters of ZnO films and ZnO-based junctions obtained by atomic layer deposition. Semicond. Sci. Technol. 2011, 26, 085013. [Google Scholar] [CrossRef]
- Elam, J.W.; Sechrist, Z.A.; George, S.M. ZnO/Al2O3 nanolaminates fabricated by atomic layer deposition: Growth and surface roughness measurements. Thin Solid Film. 2002, 414, 43–55. [Google Scholar] [CrossRef]
- Kowalik, I.A.; Guziewicz, E.; Kopalko, K.; Yatsunenko, S.; Godlewski, M.; Wójcik, A.; Osinniy, V.; Krajewski, T.; Story, T.; Łusakowska, E.; et al. Extra-Low Temperature Growth of ZnO by Atomic Layer Deposition with Diethylzinc Precursor. Acta Phys. Pol. A 2007, 112, 401–406. [Google Scholar] [CrossRef]
- Adhikari, A.; Przezdziecka, E.; Mishra, S.; Sybilski, P.; Sajkowski, J.; Guziewicz, E. Optical Properties of ZnO Deposited by Atomic Layer Deposition on Sapphire: A Comparison of Thin and Thick Films. Phys. Status Solidi 2020, 2000669. [Google Scholar] [CrossRef]
- Lautenschlaeger, S.; Eisermann, S.; Hofmann, M.N.; Roemer, U.; Pinnisch, M.; Laufer, A.; Meyer, B.K.; von Wenckstern, H.; Lajn, A.; Schmidt, F.; et al. Morphological, structural and electrical investigations on non-polar a-plane ZnO epilayers. J. Cryst. Growth 2010, 312, 2078–2082. [Google Scholar] [CrossRef]
- Liu, Y.; Gorla, C.R.; Liang, S.; Emanetoglu, N.; Lu, Y.; Shen, H.; Wraback, M. Ultraviolet detectors based on epitaxial ZnO films grown by MOCVD. J. Electron. Mater. 2000, 29, 69–74. [Google Scholar] [CrossRef]
- Przezdziecka, E.; Stachowicz, M.; Lisowski, W.; Guziewicz, E.; Sobczak, J.W.; Jakieła, R.; Jablonski, A.; Jarosz, D.; Kozanecki, A. The chemical states of As 3d in highly doped ZnO grown by Molecular Beam Epitaxy and annealed in different atmospheres. Thin Solid Films 2016, 605, 283–288. [Google Scholar] [CrossRef]
- Guziewicz, E.; Kowalik, I.A.; Godlewski, M.; Kopalko, K.; Osinniy, V.; Wójcik, A.; Yatsunenko, S.; Łusakowska, E.; Paszkowicz, W.; Guziewicz, M. Extremely low temperature growth of ZnO by atomic layer deposition. J. Appl. Phys. 2008, 103, 033515. [Google Scholar] [CrossRef]
- Aggarwal, R.; Zhou, H.; Jin, C.; Narayan, J.; Narayan, R.J. Semipolar r-plane ZnO films on Si(100) substrates: Thin film epitaxy and optical properties. J. Appl. Phys. 2010, 107, 113530. [Google Scholar] [CrossRef] [Green Version]
- Samanta, P.K.; Chaudhuri, P.R. Substrate effect on morphology and photoluminescence from ZnO monopods and bipods. Front. Optoelectron. China 2011, 4, 130–136. [Google Scholar] [CrossRef]
- Kang, J.S.; Kang, H.S.; Pang, S.S.; Shim, E.S.; Lee, S.Y. Investigation on the origin of green luminescence from laser-ablated ZnO thin film. Thin Solid Films 2003, 443, 5–8. [Google Scholar] [CrossRef]
- Pung, S.-Y.; Choy, K.-L.; Hou, X.; Shan, C. Preferential growth of ZnO thin films by the atomic layer deposition technique. Nanotechnology 2008, 19, 435609. [Google Scholar] [CrossRef] [PubMed]
- Gieraltowska, S.; Witkowski, B.S.; Godlewski, M.; Guziewicz, E. Properties of thin films of high-k oxides grown by atomic layer deposition at low temperature for electronic applications. Opt. Appl. 2013, 43, 17–25. [Google Scholar]
- Debye, P.; Scherrer, P. Interferenzen a regello orientierten Teilchen im Röntgenlicht. I. Nachr. Ges. Wiss. Göttingen Math. Phys. Kl. 1916, 1916, 1–15. [Google Scholar]
- Scherrer, P. Bestimmung der Größe und der inneren Struktur von Kolloidteilchen mittels Röntgenstrahlen. Nachr. Ges. Wiss. Göttingen Math. Phys. Kl. 1918, 1918, 98–100. [Google Scholar]
- Ungár, T. The meaning of size obtained from broadened x-ray diffraction peaks. Adv. Eng. Mater. 2003, 5, 323–329. [Google Scholar] [CrossRef]
- Saha, D.; Das, A.K.; Ajimsha, R.S.; Misra, P.; Kukreja, L.M. Effect of disorder on carrier transport in ZnO thin films grown by atomic layer deposition at different temperatures. J. Appl. Phys. 2013, 114, 043703. [Google Scholar] [CrossRef]
- Gay, P.; Hirsch, P.B.; Kelly, A. X-ray studies of polycrystalline metals deformed by rolling. III. The physical interpretation of the experimental results. Acta Crystallogr. 1954, 7, 41–49. [Google Scholar] [CrossRef]
- Ben Ameur, S.; Bel hadjltaief, H.; Barhoumi, A.; Duponchel, B.; Leroy, G.; Amlouk, M.; Guermazi, H. Physical investigations and photocatalytic activities on ZnO and SnO2 thin films deposited on flexible polymer substrate. Vacuum 2018, 155, 546–552. [Google Scholar] [CrossRef]
- Williamson, G.K.; Smallman, R.E., III. Dislocation densities in some annealed and cold-worked metals from measurements on the X-ray Debye-Scherrer spectrum. Philos. Mag. 1956, 1, 34–46. [Google Scholar] [CrossRef]
- McCluskey, M.D.; Jokela, S.J. Defects in ZnO. J. Appl. Phys. 2009, 106, 071101. [Google Scholar] [CrossRef] [Green Version]
- Ohno, Y.; Koizumi, H.; Taishi, T.; Yonenaga, I.; Fujii, K.; Goto, H.; Yao, T. Optical properties of dislocations in wurtzite ZnO single crystals introduced at elevated temperatures. J. Appl. Phys. 2008, 104, 073515. [Google Scholar] [CrossRef]
- Müller, E.; Gerthsen, D.; Brückner, P.; Scholz, F.; Gruber, T.; Waag, A. Probing the electrostatic potential of charged dislocations in n−GaN and n−ZnO epilayers by transmission electron holography. Phys. Rev. B 2006, 73, 245316. [Google Scholar] [CrossRef]
- Enigochitra, A.S.; Perumal, P.; Sanjeeviraja, C.; Deivamani, D.; Boomashri, M. Influence of substrate temperature on structural and optical properties of ZnO thin films prepared by cost-effective chemical spray pyrolysis technique. Superlattices Microstruct. 2016, 90, 313–320. [Google Scholar] [CrossRef]
- Singh, D.; Kumar, R.; Ganguli, T.; Srinivasa, R.S.; Major, S.S. Effect of Substrate Temperature on Microstructure of Epitaxial ZnO Films Grown on Sapphire by Sputtering; American Institute of Physics: College Park, MD, USA, 2012; pp. 769–770. [Google Scholar] [CrossRef]
- Klug, H.P.; Alexander, L.E. X-Ray Diffraction Procedures: For Polycrystalline and Amorphous Materials, 2nd ed.; Wiley: Hoboken, NJ, USA, 1974. [Google Scholar]
- Hong, R.; Huang, J.; He, H.; Fan, Z.; Shao, J. Influence of different post-treatments on the structure and optical properties of zinc oxide thin films. Appl. Surf. Sci. 2005, 242, 346–352. [Google Scholar] [CrossRef]
- Warren, B.E. X-ray Diffraction; Dover Books on Physics: New York, NY, USA, 1990. [Google Scholar]
- Jeon, S.; Bang, S.; Lee, S.; Kwon, S.; Jeong, W.; Jeon, H.; Chang, H.J.; Park, H.-H. Structural and Electrical Properties of ZnO Thin Films Deposited by Atomic Layer Deposition at Low Temperatures. J. Electrochem. Soc. 2008, 155, H738. [Google Scholar] [CrossRef]
- Nanostructured Materials for Advanced Technological Applications; Reithmaier, J.P.; Petkov, P.; Kulisch, W.; Popov, C. (Eds.) NATO Science for Peace and Security Series B: Physics and Biophysics; Springer: Dordrecht, The Netherlands, 2009; ISBN 978-1-4020-9915-1. [Google Scholar]
- Dimova-Malinovska, D.; Nichev, H.; Angelov, O. Correlation between the stress in ZnO thin films and the Urbach band tail width. Phys. Status Solidi 2008, 5, 3353–3357. [Google Scholar] [CrossRef]
- Mittemeijer, E.J. Fundamentals of Materials Science: The Microstructure-Property Relationship Using Metals as Model Systems; Springer: Berlin/Heidelberg, Germany, 2011; ISBN 9783642104992. [Google Scholar]
- Morkoç, H.; Özgür, Ü. Zinc Oxide: Fundamentals, Materials and Device Technology; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Wang, A.; Chen, T.; Lu, S.; Wu, Z.; Li, Y.; Chen, H.; Wang, Y. Effects of doping and annealing on properties of ZnO films grown by atomic layer deposition. Nanoscale Res. Lett. 2015, 10, 75. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kennedy, J.; Murmu, P.P.; Leveneur, J.; Markwitz, A.; Futter, J. Controlling preferred orientation and electrical conductivity of zinc oxide thin films by post growth annealing treatment. Appl. Surf. Sci. 2016, 367, 52–58. [Google Scholar] [CrossRef]
- Lin, W.; Chen, D.; Zhang, J.; Lin, Z.; Huang, J.; Li, W.; Wang, Y.; Huang, F. Hydrothermal Growth of ZnO Single Crystals with High Carrier Mobility. Cryst. Growth Des. 2009, 9, 4378–4383. [Google Scholar] [CrossRef]
- Ellmer, K.; Mientus, R. Carrier transport in polycrystalline transparent conductive oxides: A comparative study of zinc oxide and indium oxide. Thin Solid Film. 2008, 516, 4620–4627. [Google Scholar] [CrossRef] [Green Version]
- Guziewicz, E.; Wozniak, W.; Mishra, S.; Jakiela, R.; Guziewicz, M.; Ivanov, V.Y.; Lusakowska, E.; Schifano, R. Hydrogen in As-Grown and Annealed ZnO Films Grown by Atomic Layer Deposition. Phys. Status Solidi 2021, 218, 2000318. [Google Scholar] [CrossRef]
- Seto, J.Y.W. The electrical properties of polycrystalline silicon films. J. Appl. Phys. 1975, 46, 5247–5254. [Google Scholar] [CrossRef]
- Orton, J.W.; Powell, M.J. The Hall effect in polycrystalline and powdered semiconductors. Rep. Prog. Phys. 1980, 43, 1263–1307. [Google Scholar] [CrossRef]
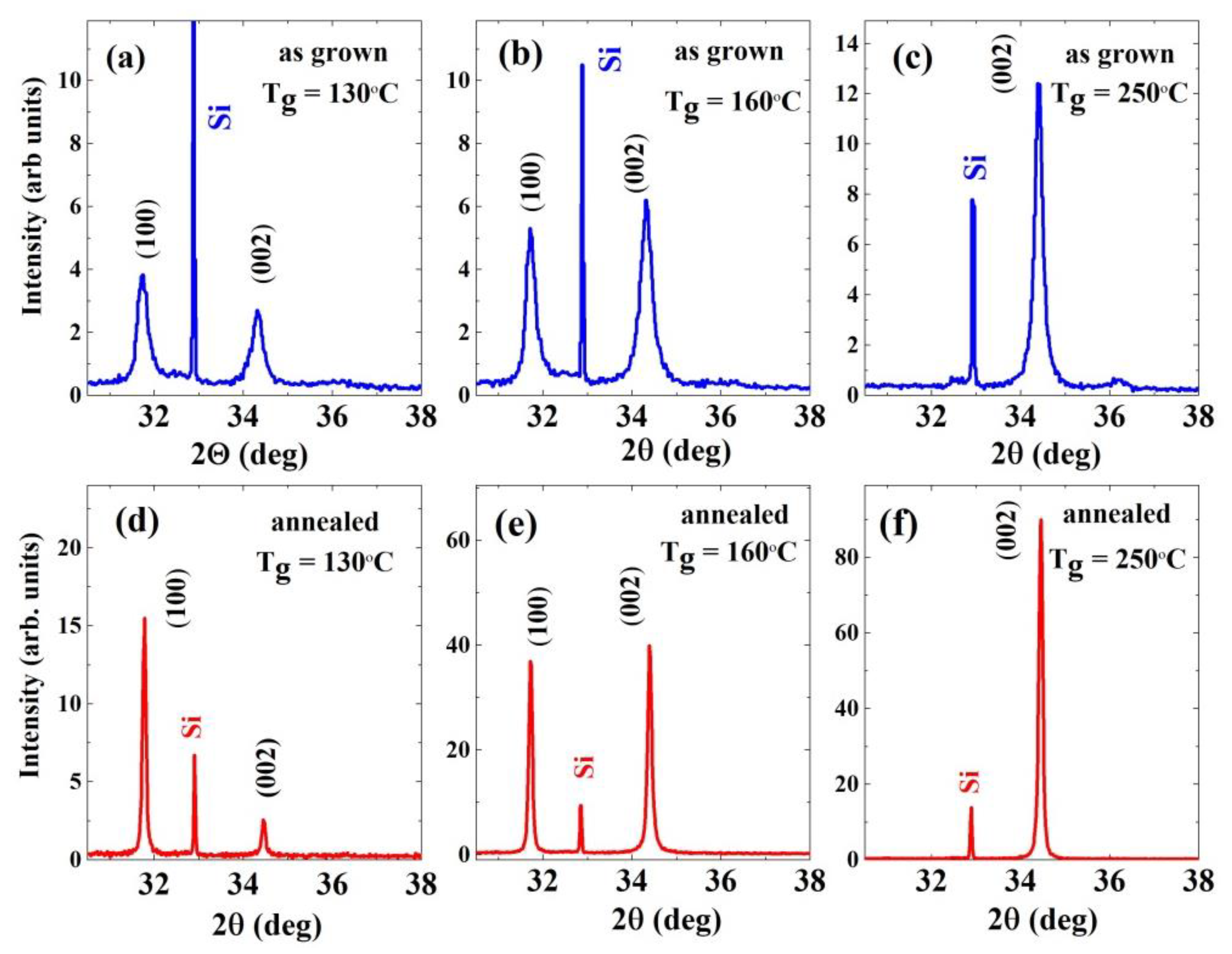
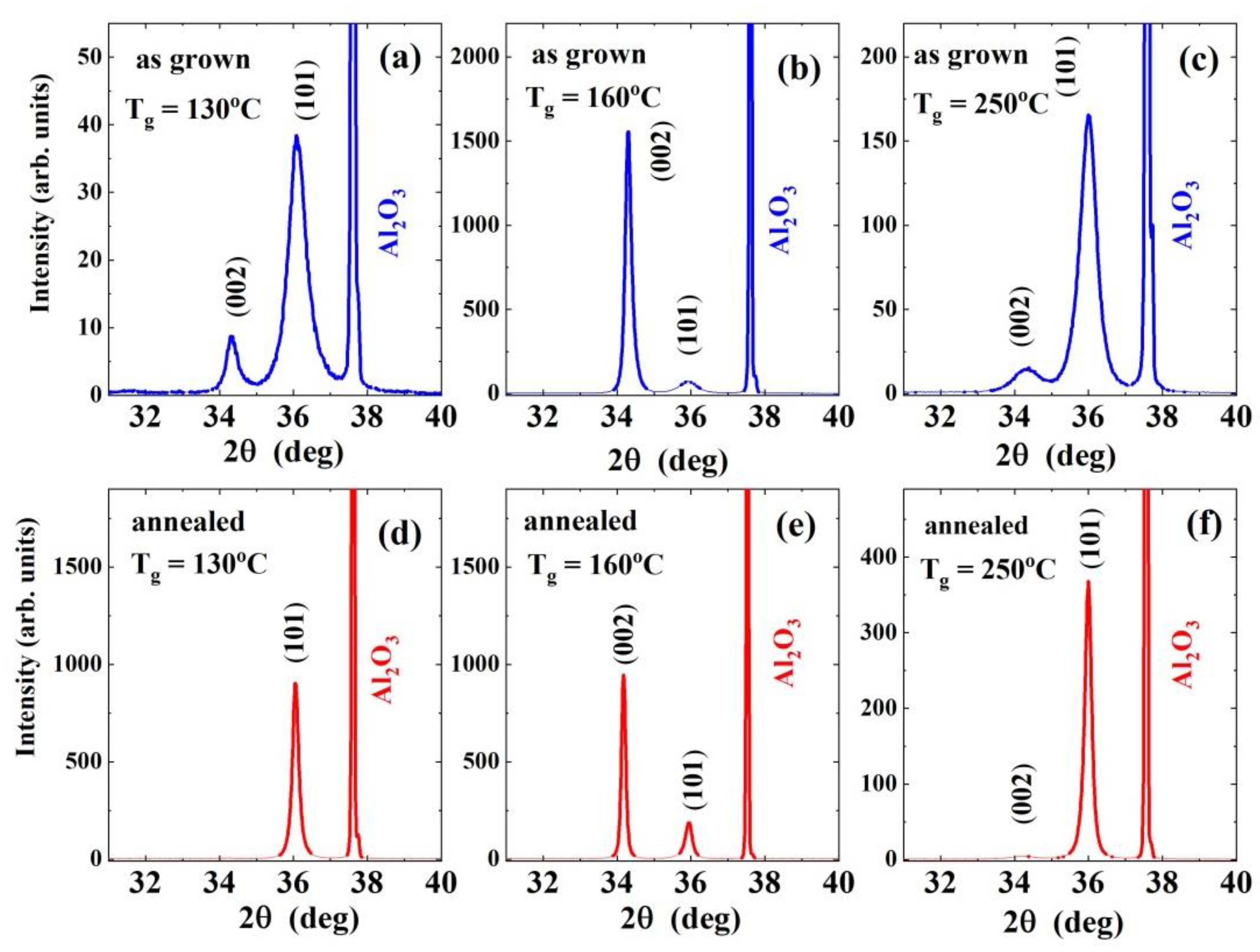
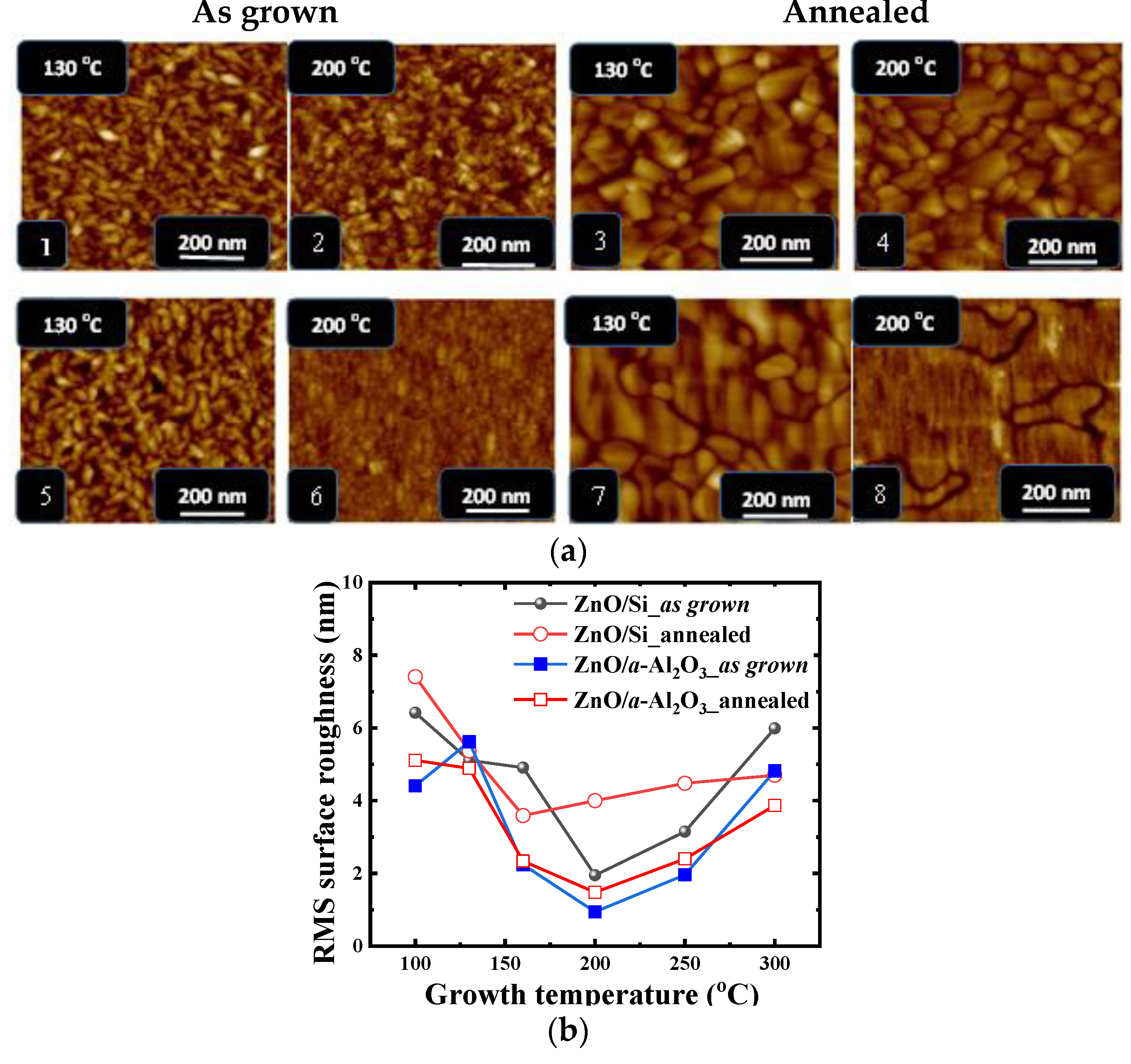

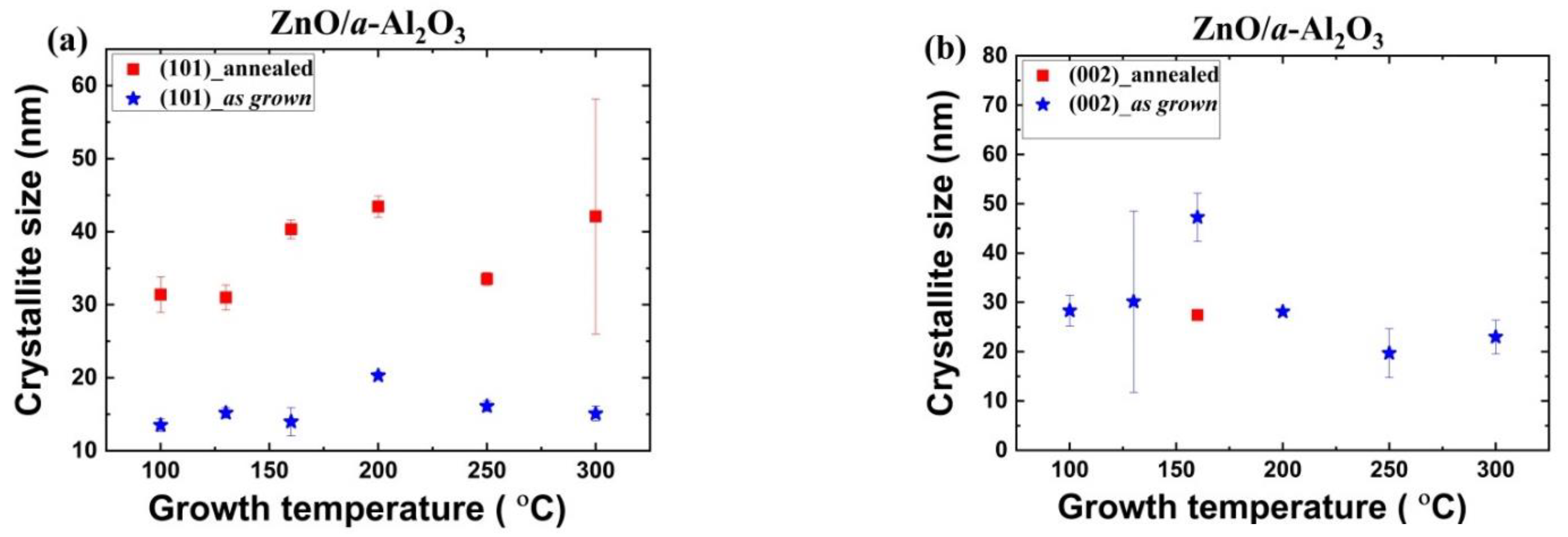
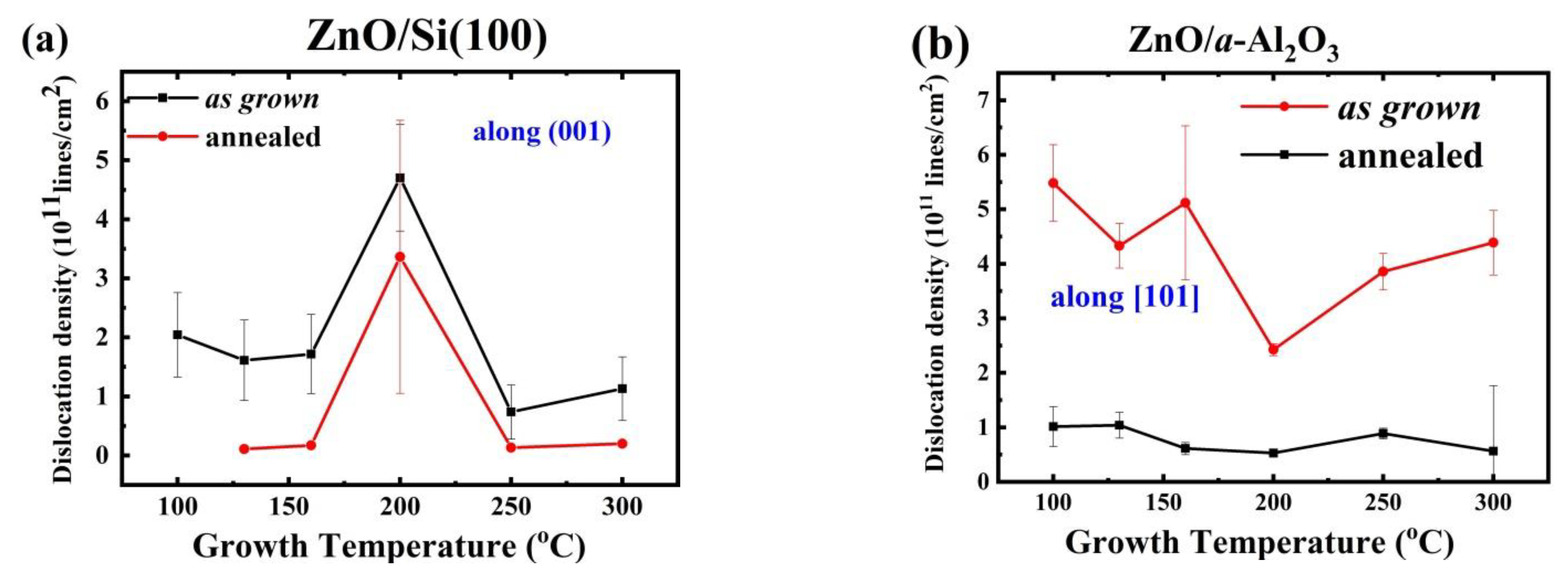

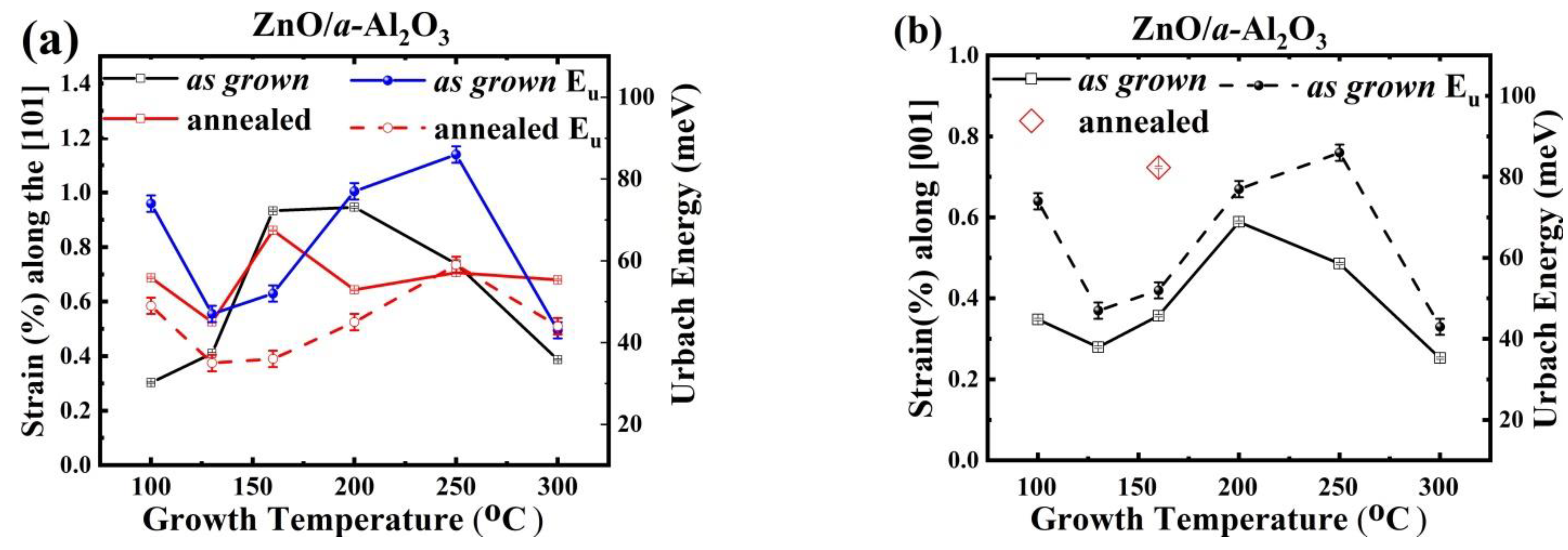
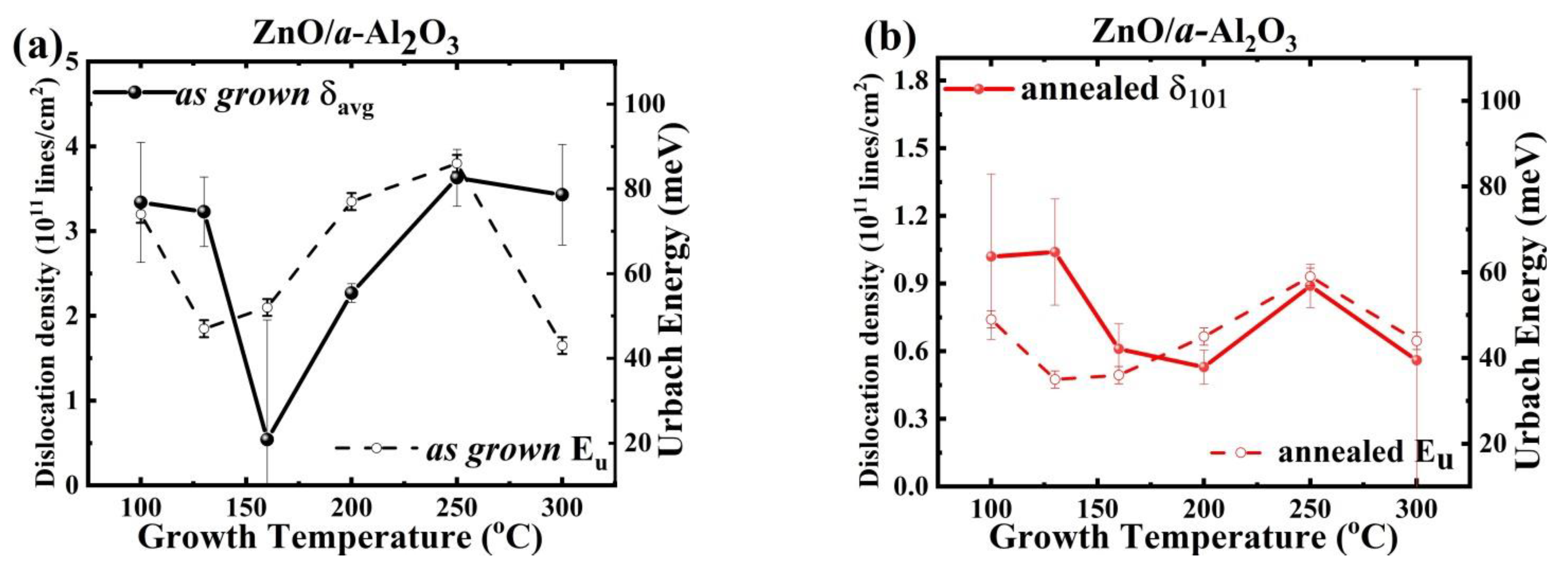
| Tg (°C) | δ(002) (1011 lines/cm2) | δ(100) (1011 lines/cm2) | δavg (1011 lines/cm2) | δ(002) (1011 lines/cm2) | δ(100) (1011 lines/cm2) | δavg (1011 lines/cm2) |
|---|---|---|---|---|---|---|
| ZnO/Si As Grown | ZnO/Si Annealed | |||||
| 100 | 2.05 | 1.22 | 1.62 | - | 0.11 | 0.11 |
| 130 | 1.61 | 1.16 | 1.38 | 0.11 | 0.10 | 0.10 |
| 160 | 1.72 | 0.98 | 1.43 | 0.17 | 0.10 | 0.14 |
| 200 | 4.70 | 1.49 | 3.51 | 3.37 | 0.10 | 0.46 |
| 250 | 0.74 | - | 0.74 | 0.14 | - | 0.14 |
| 300 | 1.13 | - | 1.13 | 0.20 | - | 0.20 |
| Tg (°C) | δ(101) (1011 lines/cm2) | δ(002) (1011 lines/cm2) | δavg (1011 lines/cm2) | δ(101) (1011 lines/cm2) | δ(002) (1011 lines/cm2) | δavg (1011 lines/cm2) |
|---|---|---|---|---|---|---|
| ZnO/a-Al2O3 As Grown | ZnO/a-Al2O3 Annealed | |||||
| 100 | 5.49 | 1.25 | 3.34 | 1.02 | - | 1.02 |
| 130 | 4.33 | 1.10 | 3.23 | 1.04 | - | 1.04 |
| 160 | 5.12 | 0.45 | 0.54 | 0.61 | 1.33 | 1.22 |
| 200 | 2.43 | 1.27 | 2.27 | 0.53 | - | 0.53 |
| 250 | 3.85 | 2.58 | 3.63 | 0.89 | - | 0.89 |
| 300 | 4.39 | 1.90 | 3.43 | 0.56 | - | 0.56 |
| Tg (°C) | As Grown Samples Carrier Density nc (cm−3)/Mobility(cm2/Vs) ZnO/a-Al2O3 nc(cm−3)/µ (cm2/Vs) ρ(Ωcm) | Annealed Samples Carrier Density nc (cm−3)/Mobility(cm2/Vs) ZnO/a-Al2O3 nc(cm−3)/µ (cm2/Vs) ρ(Ωcm) | ||
|---|---|---|---|---|
| 100 | 3.5 × 1018/1.2 | 1.7 | 1.4 × 1018/3.7 | 3.15 |
| 130 | 4.4 × 1019/8.3 | 1.8 × 10−2 | 1.4 × 1017/10.0 | 5.38 |
| 160 | 6.2 × 1019/29.2 | 3.5 × 10−3 | 1.4 × 1018/8.8 | 0.58 |
| 200 | 5.9 × 1019/20.6 | 5.1 × 10−3 | 1.8 × 1017/10.0 | 8.14 |
| 250 | 1.2 × 1020/25.1 | 2.0 × 10−3 | 1.6 × 1017/19.5 | 2.60 |
| 300 | 4.7 × 1019/25.1 | 7.3 × 10−3 | 2.0 × 1019/8.0 | 1.25 |
| Tg (°C) | As Grown Samples Carrier Density nc (cm−3)/Mobility(cm2/Vs) ZnO/Si(100) nc(cm−3)/µ (cm2/Vs) ρ(Ωcm) | Annealed Samples Carrier Density nc (cm−3) & Resistivity ρ(Ωcm), ZnO/Si(100) nc(cm−3) ρ(Ωcm) | ||
|---|---|---|---|---|
| 100 | 4.5 × 1018/17.3 | 8.4 × 10−2 | 1.7 × 1015 | 3.12 |
| 130 | 7.9 × 1018/15.2 | 5.2 × 10−2 | 1.2 × 1015 | 3.52 |
| 160 | 1.9 × 1019/22.5 | 1.4 × 10−2 | 3.0 × 1015 | 2.96 |
| 200 | 2.7 × 1019/22.6 | 1.0 × 10−2 | 4.7 × 1015 | 8.68 |
| 250 | 3.3 × 1019/22.4 | 8.5 × 10−3 | 3.6 × 1016 | 2.9 × 10−1 |
| 300 | 1.3 × 1019/31.9 | 1.5 × 10−2 | 2.7 × 1016 | 7.6 × 10−1 |
| ZnO/a-Al2O3 | ||||||||
| As Grown ZnO/a-Al2O3 | Annealed ZnO/a-Al2O3 | |||||||
| Tg (°C) | Hall Conc. (cm−3) | Half of Crystallite Size, D/2 (nm) | Hall Conc. (cm−3) | LD (nm) | Half of Crystallite Size, D/2 (nm) | |||
| [101] | [002] | [101] | [002] | |||||
| 100 | 3.50 × 1018 | 1.86 | 6.75 | 14.15 | 1.36 × 1018 | 2.98 | 15.7 | NA |
| 130 | 4.40 × 1019 | 0.52 | 7.6 | 15.08 | 1.44 × 1017 | 9.18 | 15.49 | NA |
| 160 | 6.15 × 1019 | 0.44 | 6.99 | 23.64 | 1.41 × 1018 | 2.93 | 20.18 | 13.72 |
| 200 | 5.93 × 1019 | 0.45 | 10.15 | 14.06 | 1.78 × 1017 | 8.26 | 21.73 | NA |
| 250 | 1.24 × 1020 | 0.05 | 8.05 | 9.85 | 1.64 × 1017 | 8.61 | 16.78 | NA |
| 300 | 4.73 × 1019 | 0.51 | 7.55 | 11.49 | 1.95 × 1019 | 0.79 | 21.06 | NA |
| ZnO/Si(100) | ||||||||
| As Grown ZnO/Si(100) | Annealed ZnO/Si(100) | |||||||
| Tg (°C) | Hall Conc.(cm−3) | LD(nm) | Half of Crystallite Size D/2 (nm) | Hall Conc.(cm−3) | LD(nm) | Half of Crystallite Size D/2 (nm) | ||
| [100] | [002] | [100] | [002] | |||||
| 100 | 4.53 × 1018 | 1.64 | 14.3 | 11.0 | 1.67 × 1015 | 85.16 | 46.8 | NA |
| 130 | 7.87 × 1018 | 1.24 | 14.7 | 12.5 | 1.18 × 1015 | 101.31 | 49.4 | 47.9 |
| 160 | 1.93 × 1019 | 0.79 | 16 | 12.1 | 2.99 × 1015 | 63.64 | 49.1 | 38.1 |
| 200 | 2.66 × 1019 | 0.68 | 12.9 | 7.3 | 4.71 × 1015 | 50.71 | 51.1 | NA |
| 250 | 3.29 × 1019 | 0.61 | NA | 18.4 | 3.57 × 1016 | 18.42 | NA | 43.0 |
| 300 | 1.33 × 1019 | 0.95 | NA | 14.9 | 2.74 × 1016 | 21.02 | NA | 35.4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mishra, S.; Przezdziecka, E.; Wozniak, W.; Adhikari, A.; Jakiela, R.; Paszkowicz, W.; Sulich, A.; Ozga, M.; Kopalko, K.; Guziewicz, E. Structural Properties of Thin ZnO Films Deposited by ALD under O-Rich and Zn-Rich Growth Conditions and Their Relationship with Electrical Parameters. Materials 2021, 14, 4048. https://doi.org/10.3390/ma14144048
Mishra S, Przezdziecka E, Wozniak W, Adhikari A, Jakiela R, Paszkowicz W, Sulich A, Ozga M, Kopalko K, Guziewicz E. Structural Properties of Thin ZnO Films Deposited by ALD under O-Rich and Zn-Rich Growth Conditions and Their Relationship with Electrical Parameters. Materials. 2021; 14(14):4048. https://doi.org/10.3390/ma14144048
Chicago/Turabian StyleMishra, Sushma, Ewa Przezdziecka, Wojciech Wozniak, Abinash Adhikari, Rafal Jakiela, Wojciech Paszkowicz, Adrian Sulich, Monika Ozga, Krzysztof Kopalko, and Elzbieta Guziewicz. 2021. "Structural Properties of Thin ZnO Films Deposited by ALD under O-Rich and Zn-Rich Growth Conditions and Their Relationship with Electrical Parameters" Materials 14, no. 14: 4048. https://doi.org/10.3390/ma14144048
APA StyleMishra, S., Przezdziecka, E., Wozniak, W., Adhikari, A., Jakiela, R., Paszkowicz, W., Sulich, A., Ozga, M., Kopalko, K., & Guziewicz, E. (2021). Structural Properties of Thin ZnO Films Deposited by ALD under O-Rich and Zn-Rich Growth Conditions and Their Relationship with Electrical Parameters. Materials, 14(14), 4048. https://doi.org/10.3390/ma14144048







