Fracturing and Damage of 3D-Printed Materials with Two Intermittent Fissures under Compression
Abstract
:1. Introduction
2. Sample Preparation and Testing
2.1. 3D-Printed Sample Preparation
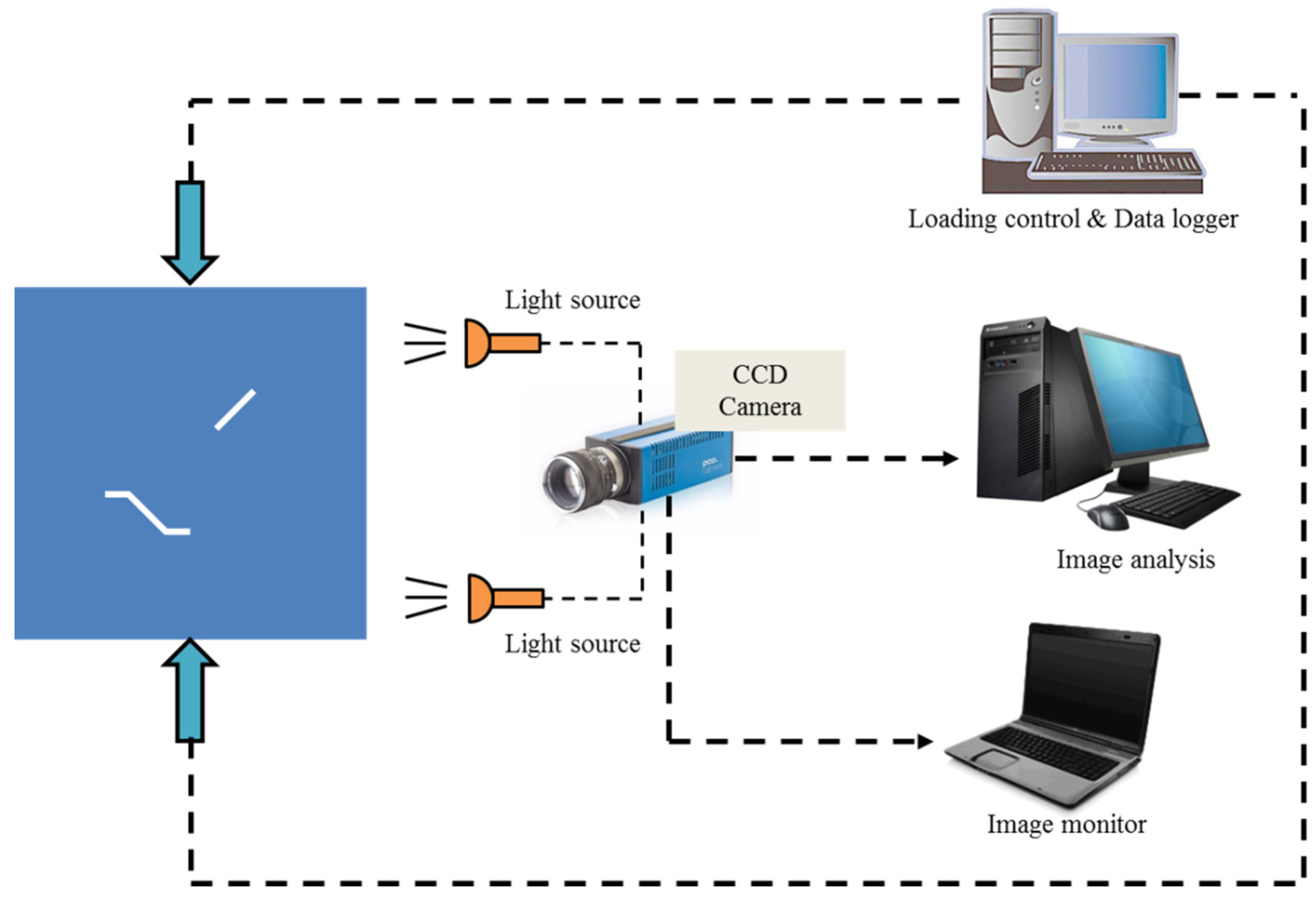
2.2. DIC Equipment Preparation
2.3. Testing Procedure
3. Experimental Results and Discussion
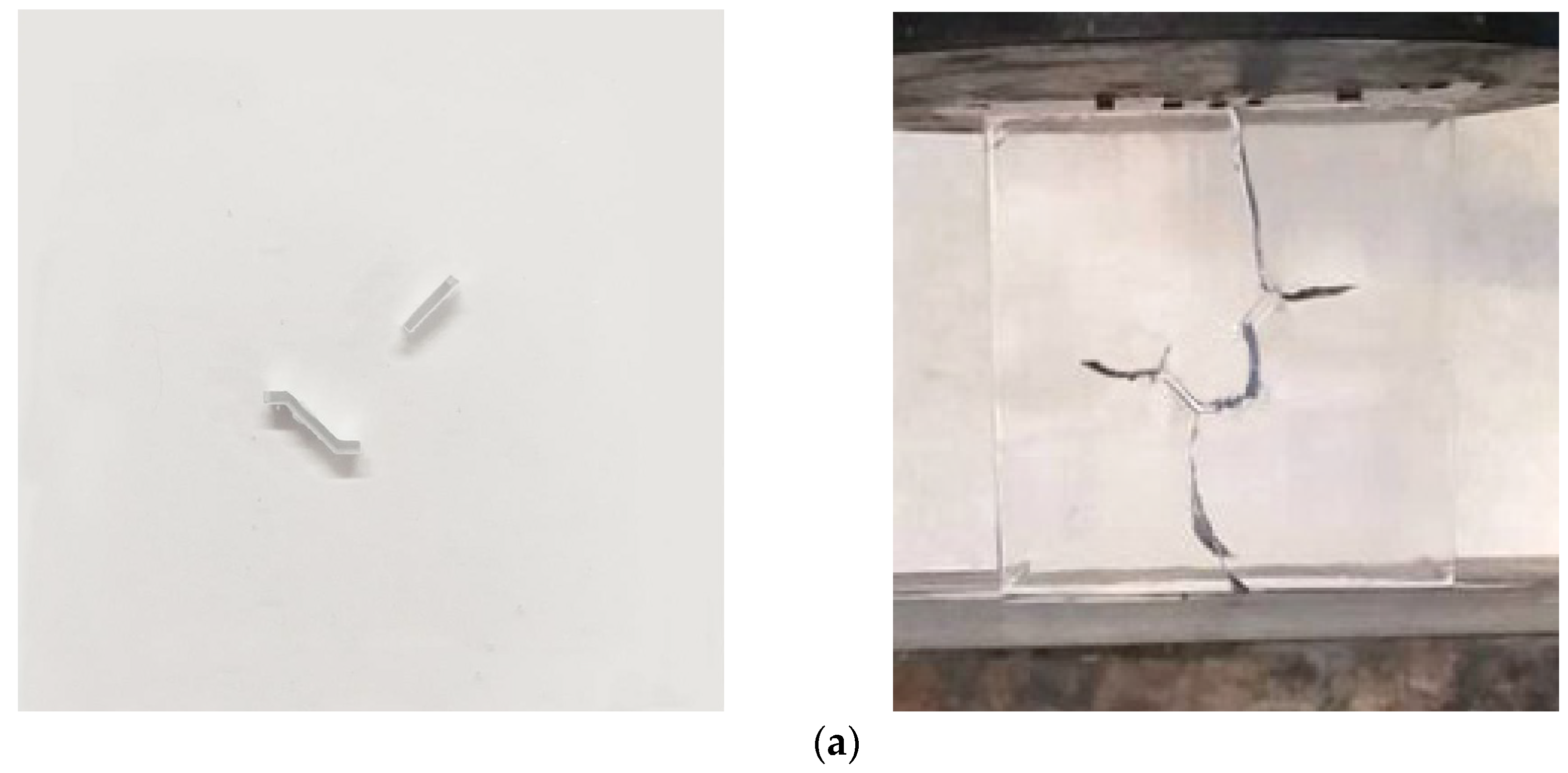
3.1. Certification of the Failure Mode
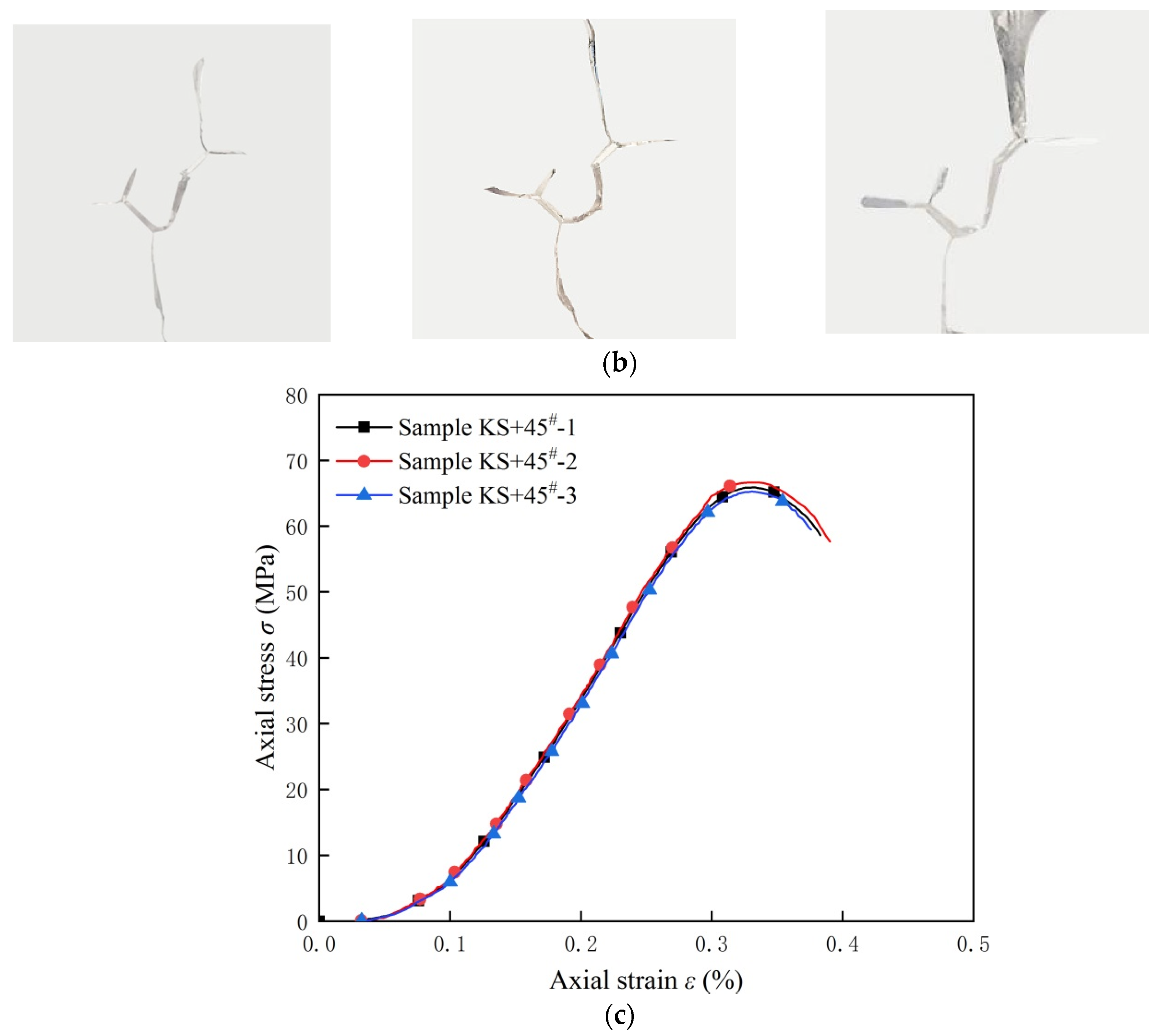
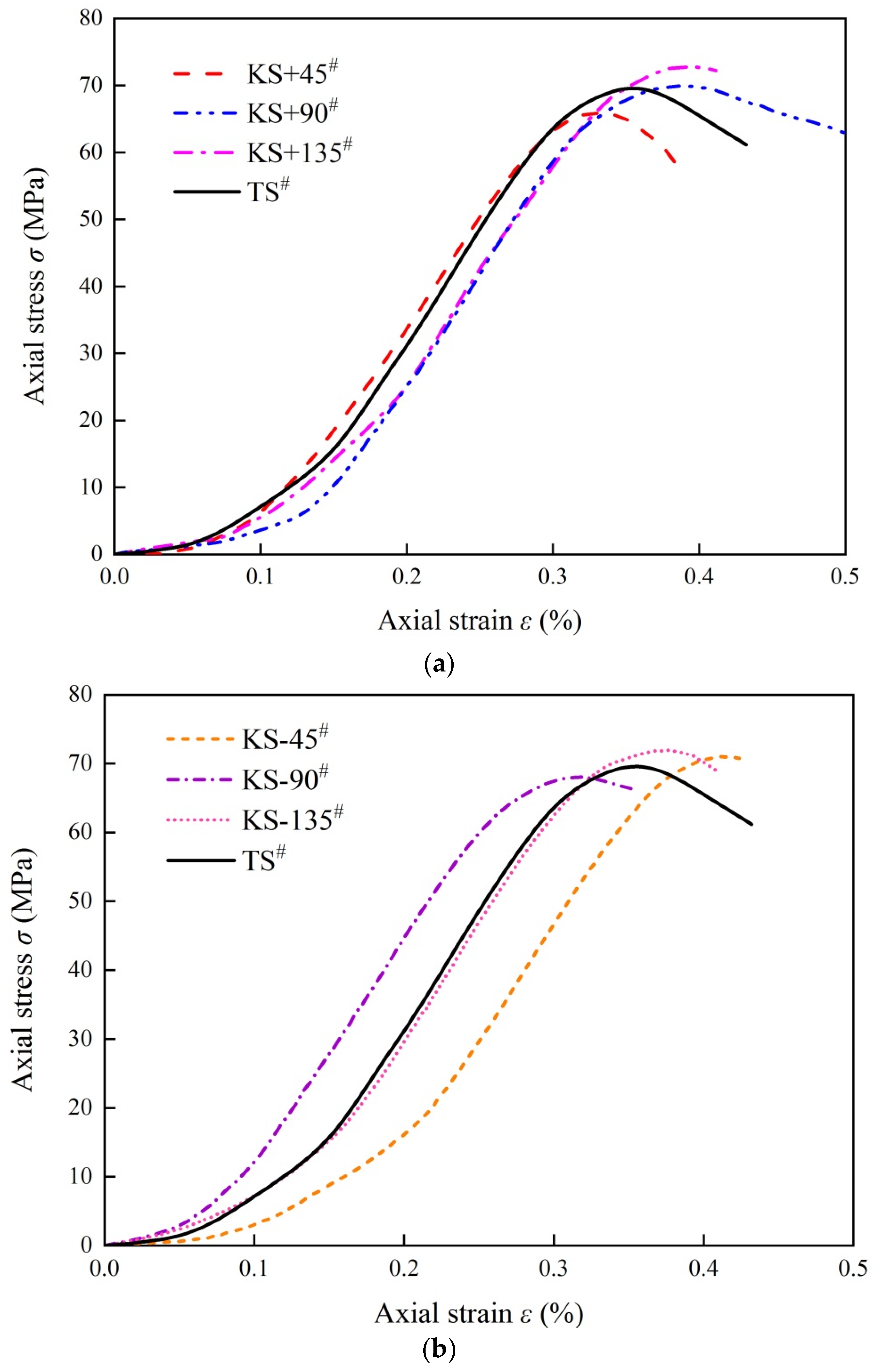
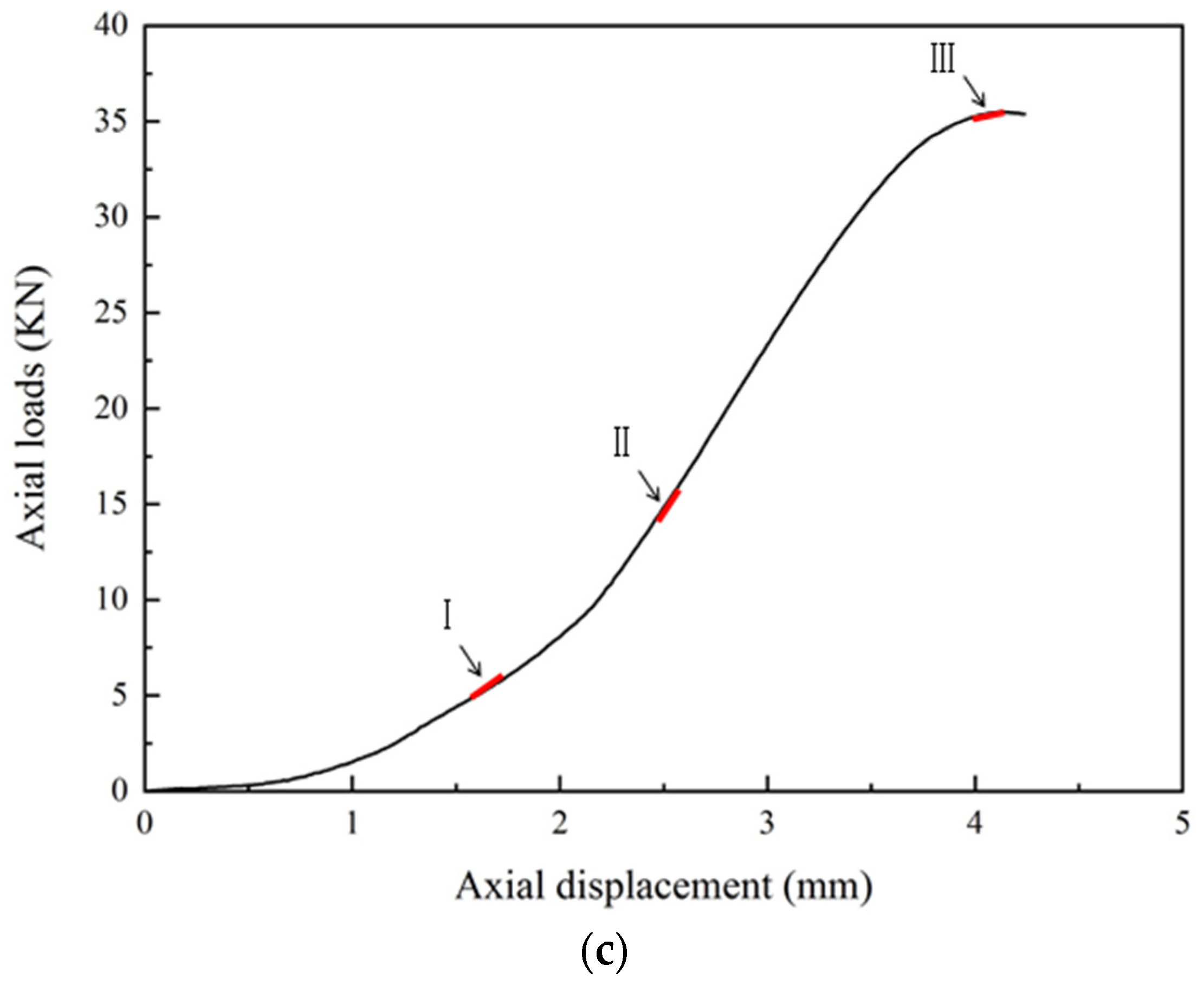
3.2. Analysis of the Axial Stress–Strain Curves of Specimens
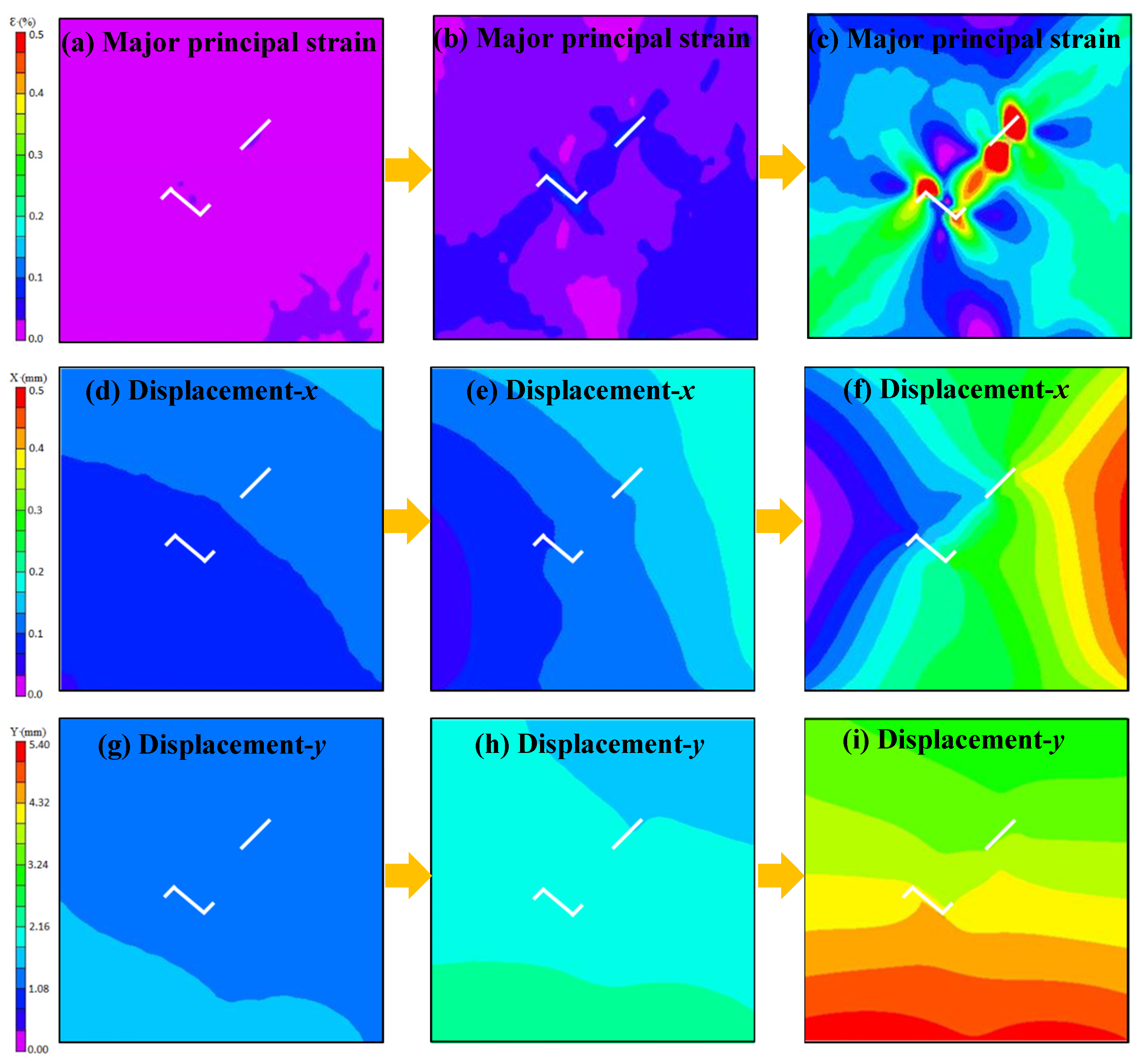
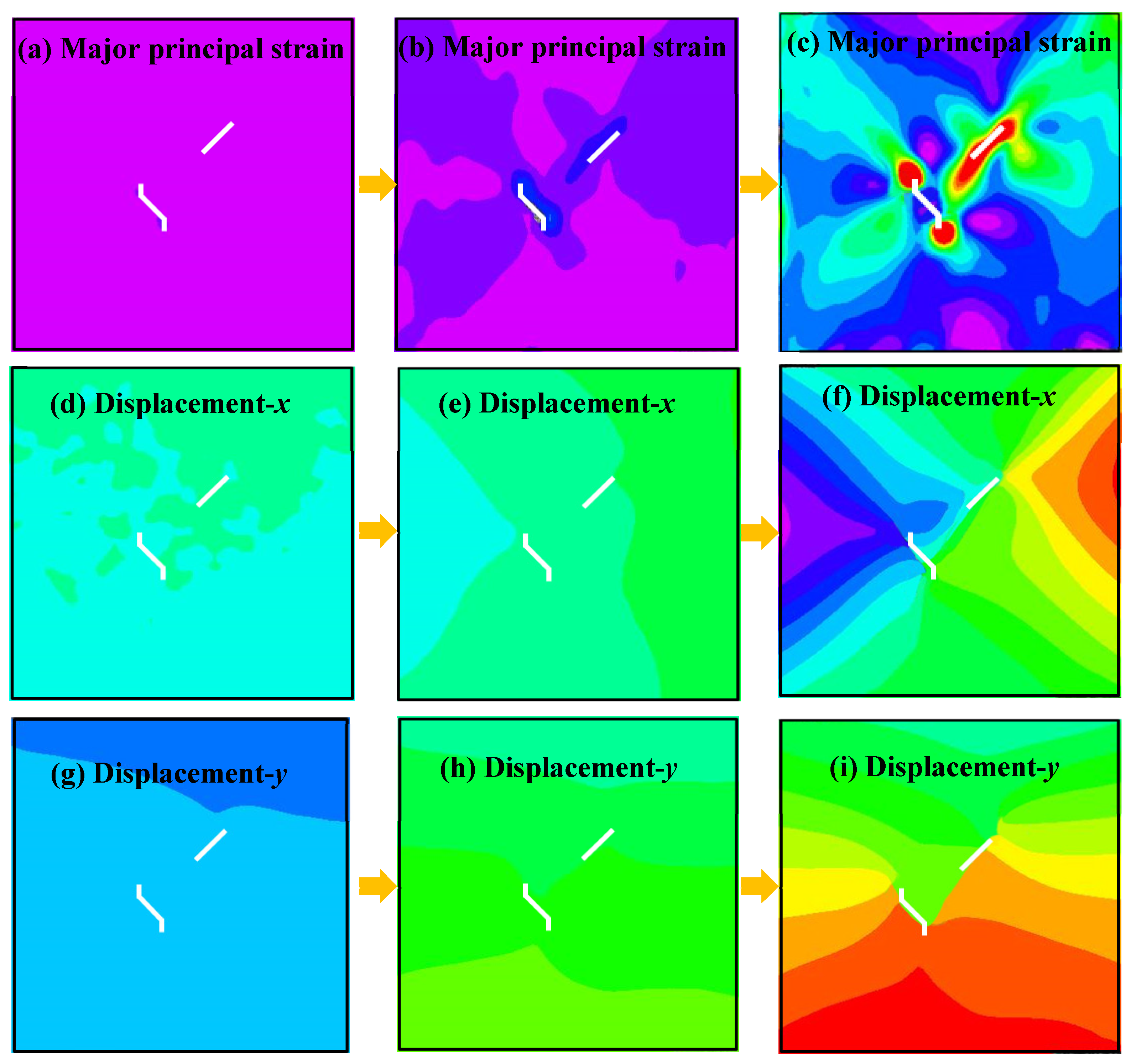
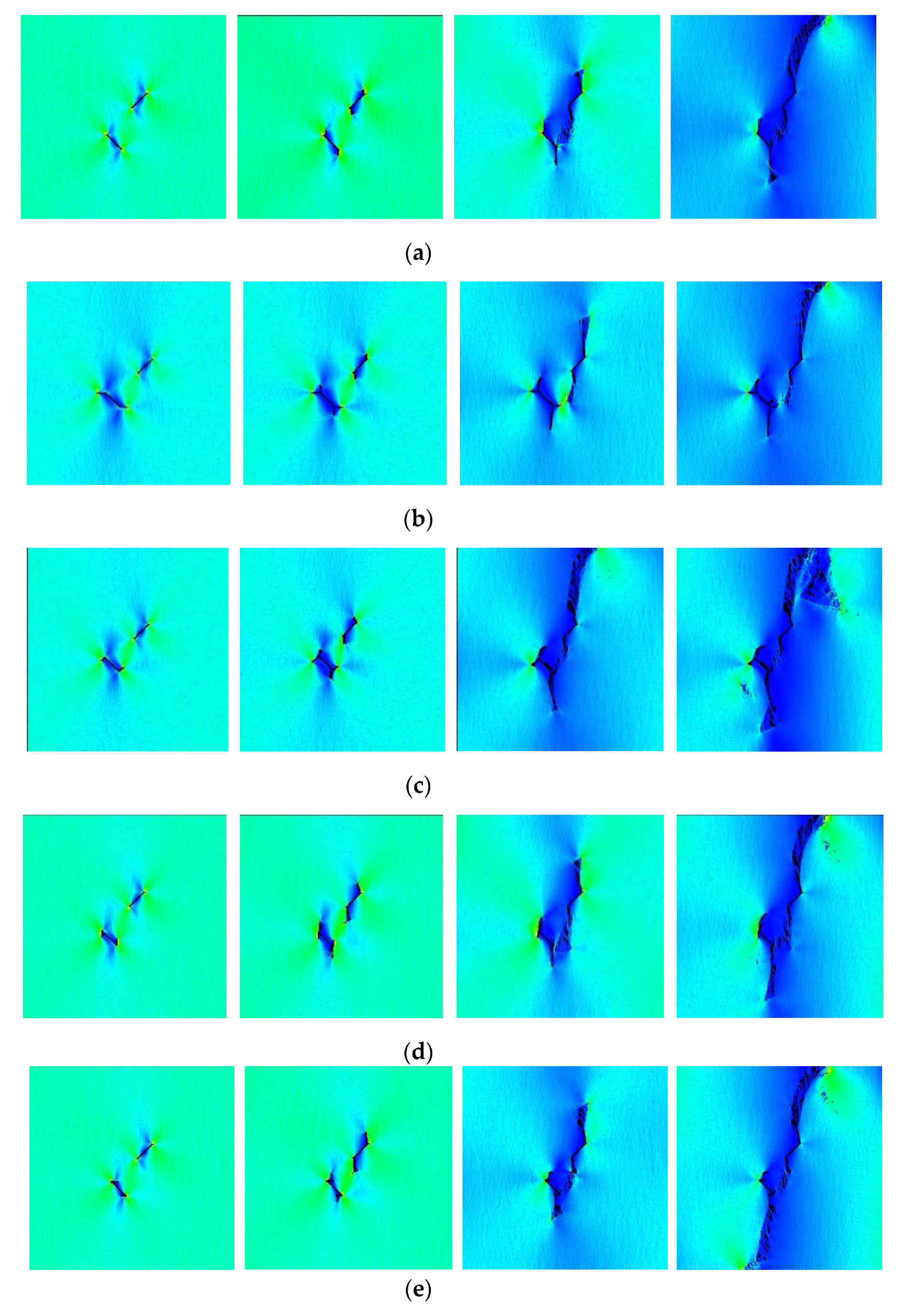
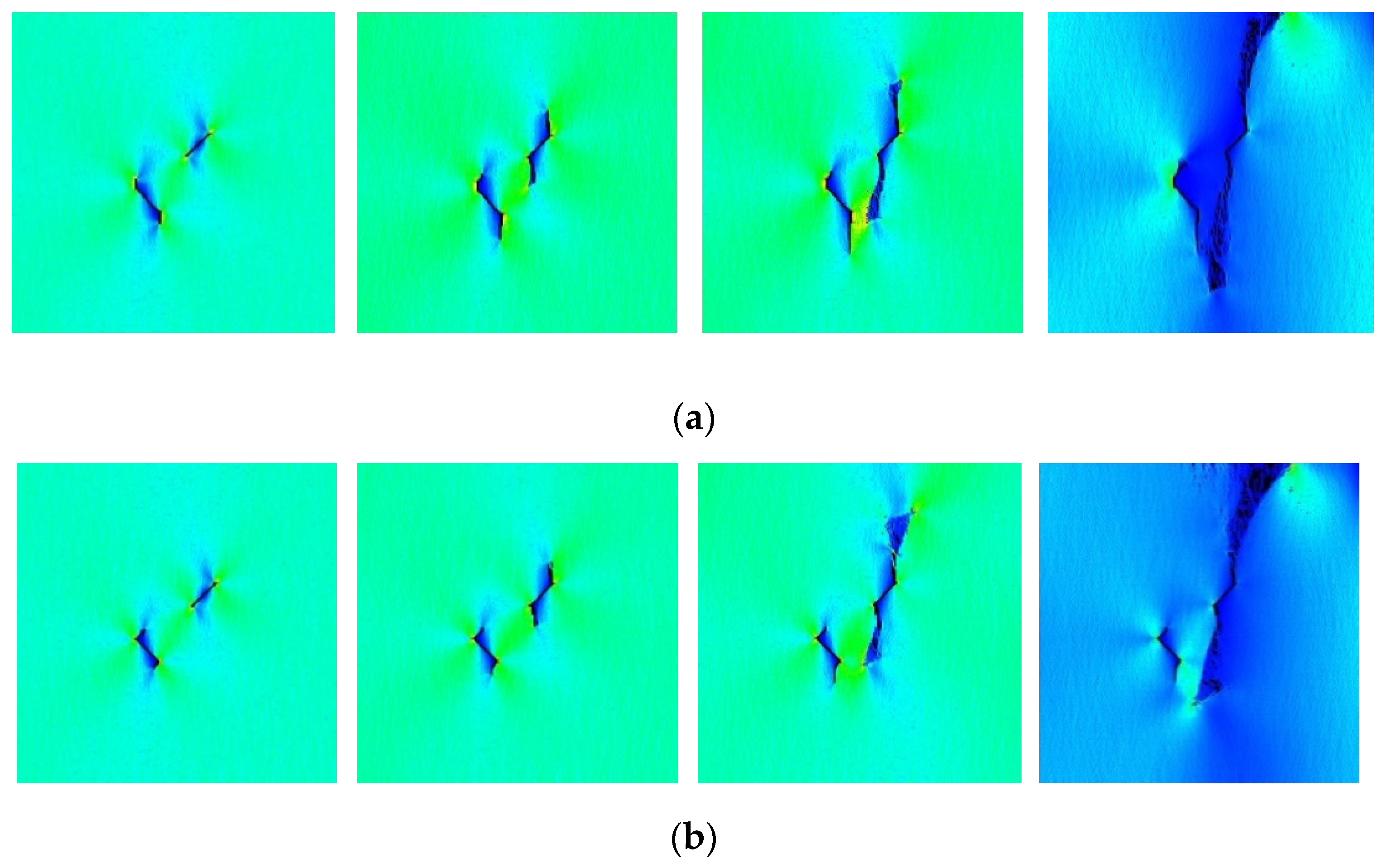
3.3. Cracking Process Based on the DIC Analysis
3.4. The Crack Propagation and Failure Behavior
4. Numerical Simulations
5. Conclusions
- The kinked branches do not change the stiffness and strength of the samples too much. When the branch inclination angle of the kinked fissure rotates clockwise (β = +45°, +90°, or +135°), the peak strength increases. When the branch inclination angle of the kinked fissure rotates counterclockwise (β = −45°, −90°, or −135°), the peak strength does not change significantly, and the difference between the maximum and minimum strength does not exceed 10%.
- The primary crack may initiate from the inflection point or the end tip depending on the inclination angle β of the kink branch. When β = +45°, +90°, or +135°, the kinked fissures form crack from the inflection point. When β = −45°, −90°, or −135°), the kinked fissures incur crack from the end tip.
- The small kink branch may change the failure mode of the samples completely, and the inclination angle β of the kinked fissure has an important effect on the failure mode. When β = +45°, +90°, +135°, or –135°, a tension–shear composite failure mode (Mode I) occur in the rock bridge. When β = −45° and −90°, tensile failure is incurred throughout the whole samples, while the rock bridge does not damage.
- The numerical simulation failure modes of the models are well consistent with the cracking and failure modes of the physical experimental samples.
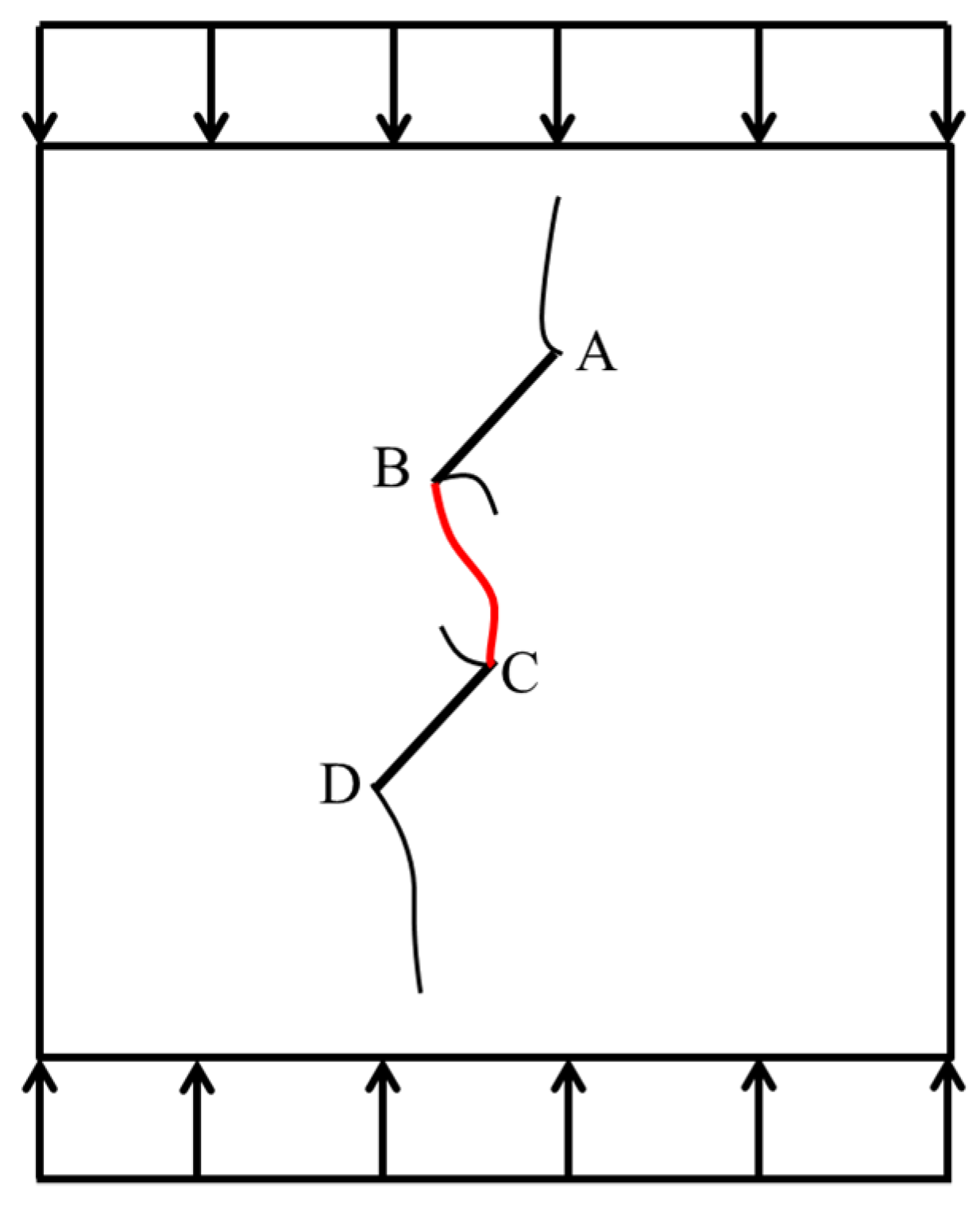
- I = Kinked fissure
- II = Straight fissure
- θ1= Angle between fissure I and the horizontal axis
- θ2= Angle between fissure II and the horizontal axis
- β = Angle between fissure II and the y-axis
- 2a1 = Main fracture length of fissure I
- 2a2 = Length of fissure II
- 2c = Distance between kinked fissure I and straight fissure II
Author Contributions
Funding
Conflicts of Interest
References
- Bobet, A.; Einstein, H.H. Fracture coalescence in rock-type materials under uniaxial and biaxial compression. Int. J. Rock Mech. Min. 1998, 35, 863–888. [Google Scholar] [CrossRef]
- Lee, H.; Jeon, S. An experimental and numerical study of fracture coalescence in pre-cracked specimens under uniaxial compression. Int. J. Solids Struct. 2011, 48, 979–999. [Google Scholar] [CrossRef] [Green Version]
- Yang, S.; Jing, H. Strength failure and crack coalescence behavior of brittle sandstone samples containing a single fissure under uniaxial compression. Int. J. Fract. 2011, 168, 227–250. [Google Scholar] [CrossRef]
- Haeri, H.; Shahriar, K.; FatehiMarji, M.; Moarefvand, P. On the crack propagation analysis of rock like brazilian disc specimens containing cracks under compressive line loading. Lat. Am. J. Solids Struct. 2014, 11, 1400–1416. [Google Scholar] [CrossRef] [Green Version]
- Shlyannikov, V.N.; Tumanov, A.V. An inclined surface crack subject to biaxial loading. Int. J. Solids Struct. 2011, 48, 1778–1790. [Google Scholar] [CrossRef] [Green Version]
- Sagong, M.; Bobet, A. Coalescence of multiple flaws in a rock model material in uniaxial compression. Int. J. Rock Mech. Min. 2002, 39, 229–241. [Google Scholar] [CrossRef]
- Fan, X.; Lin, H.; Lai, H.; Cao, R.; Liu, J. Numerical analysis of the compressive and shear failure behavior of rock containing multi-intermittent joints. Comptes Rendus Mécanique. 2019, 347, 33–48. [Google Scholar] [CrossRef]
- Huang, D.; Gu, D.M.; Yang, C.; Huang, R.Q.; Fu, G.Y. Investigation on Mechanical Behaviors of Sandstone with Two Preexisting Flaws under Triaxial Compression. Rock Mech. Rock Eng. 2015, 49, 375–399. [Google Scholar] [CrossRef]
- Yang, S.Q.; Huanga, Y.H.; Ranjitha, P.G. Failure mechanical and acoustic behavior of brine saturated sandstone containing two pre-existing flaws under different confining pressures. Eng. Fract. Mech. 2018, 193, 108–121. [Google Scholar] [CrossRef]
- Zhang, Y.M.; Lacknera, R.; Zeimla, M.; Mang, H.A. Strong discontinuity embedded approach with standard SOS formulation: Element formulation, energy-based crack-tracking strategy, and validations. Comput Method Appl. 2015, 287, 335–366. [Google Scholar] [CrossRef]
- Rabczuk, T.; Ren, H.L. A peridynamics formulation for quasi-static fracture and contact in rock. Eng. Geol. 2017, 225, 42–48. [Google Scholar] [CrossRef]
- Zhang, Y.M.; Zhuang, X.Y. Cracking elements: A self-propagating Strong Discontinuity embedded Approach for quasi-brittle fracture. Finite Elem. Anal. Des. 2018, 144, 84–100. [Google Scholar] [CrossRef]
- Isida, M.; Noguchi, H. Stress intensity factors at tips of branched cracks under various loadings. Int. J. Fract. 1992, 54, 293–316. [Google Scholar] [CrossRef]
- Chaker, C.; Barquins, M. Sliding effect on branch crack. Phys. Chem. Earth. 1996, 21, 319–323. [Google Scholar] [CrossRef]
- Meggiolaro, M.A.; Miranda, A.C.O.; Castro, J.T.P.; Martha, L.F. Stress intensity factor equations for branched crack growth. Eng. Fract. Mech. 2005, 72, 2647–2671. [Google Scholar] [CrossRef]
- Carpinter, A.; Spagnoli, A.; Vantadori, S.; Viappiani, D. Influence of the crack morphology on the fatigue crack growth rate: A continuously-kinked crack model based on fractals. Eng. Fract. Mech. 2008, 75, 579–589. [Google Scholar] [CrossRef]
- Li, Y.P.; Chen, L.Z.; Wang, Y.H. Experimental research on pre-cracked marble under compression. Int. J. Solids Struct. 2005, 42, 2505–2616. [Google Scholar] [CrossRef]
- Ma, G.W.; Dong, Q.Q.; Fan, L.F.; Gao, J.W. An investigation of non-straight fissures cracking under uniaxial compression. Eng. Fract. Mech. 2017, 191, 300–310. [Google Scholar] [CrossRef]
- Ma, G.W.; Dong, Q.Q.; Wang, L. Experimental Investigation on the Cracking Behavior of 3D Printed Kinked Fissure. Sci. China Technol. Sci. 2018, 61, 1872–1881. [Google Scholar] [CrossRef]
- Dong, Q.Q.; Xiong, C.W.; Ma, C.L.; Wei, H.J. Experimental Study on Cracking Behaviour of Intermittent Double S-shaped Fissures under Uniaxial Compression. KSCE J. Civ. Eng. 2019, 23, 2483–2494. [Google Scholar] [CrossRef]
- Wong, L.N.Y.; Einstein, H.H. Crack coalescence in molded gypsum and carrara marble: Part 1: Macroscopic observations and interpretation. Rock Mech. Rock Eng. 2008, 42, 475–511. [Google Scholar] [CrossRef]
- Park, C.H.; Bobet, A. Crack initiation, propagation and coalescence from frictional flaws in uniaxial compression. Eng. Fract. Mech. 2010, 77, 2727–2748. [Google Scholar] [CrossRef]
- Chen, X.; Liao, Z.H.; Xi, P. Cracking process of rock mass models under uniaxial compression. J. Cent. South Univ. 2013, 20, 1661–1678. [Google Scholar] [CrossRef]
- Yang, S.Q.; Yang, D.S.; Jing, H.W.; Li, Y.H.; Wang, S.Y. An experimental study of the fracture coalescence behaviour of brittle sandstone specimens containing three fissures. Rock Mech. Rock Eng. 2012, 45, 563–582. [Google Scholar] [CrossRef]
- Morgan, S.P.; Stephen, P.; Johnson, C.A.; Einstein, H.H. Cracking processes in Barre granite: Fracture process zones and crack coalescence. Int. J. Fract. 2013, 180, 177–204. [Google Scholar] [CrossRef]
- Yin, P.; Wong, R.H.C.; Chau, K.T. Coalescence of two parallel pre-existing surface cracks in granite. Int. J. Rock Mech. Min. 2014, 68, 66–84. [Google Scholar] [CrossRef]
- Wong, R.H.C.; Chau, K.T.; Tang, C.A.; Lin, P. Analysis of crack coalescence in rock-like materials containing three flaws-part I: Experimental approach. Int. J. Rock Mech. Min. 2001, 38, 909–924. [Google Scholar] [CrossRef]
- Yang, S.Q.; Dai, Y.H.; Han, L.J.; Jin, Z.Q. Experimental study on mechanical behavior of brittle marble samples containing different flaws under uniaxial compression. Eng. Fract. Mech. 2009, 76, 1833–1845. [Google Scholar] [CrossRef]
- Ju, Y.; Xie, H.; Zheng, Z.; Lu, J.; Mao, L.; Gao, F.; Peng, R. Visualization of the complex structure and stress field inside rock by means of 3D printing technology. Chin. Sci. Bull. 2014, 59, 5354–5365. [Google Scholar] [CrossRef]
- Zhou, T.; Zhu, J.B.; Ju, Y.; Xie, H.P. Volumetric Fracturing Behavior of 3D Printed Artificial Rocks Containing Single and Double 3D Internal Flaws under Static Uniaxial Compression. Eng. Fract. Mech. 2018, 205, 190–204. [Google Scholar] [CrossRef]
- Tang, C.A.; Kou, S.Q. Crack propagation and coalescence in brittle materials under compression. Eng. Fract. Mech. 1998, 61, 311–324. [Google Scholar] [CrossRef]
- Tang, C.A.; Lin, P.; Wong, R.H.C. Analysis of crack coalescence in rock-like materials containing three flaws Part II: Numerical approach. Int. J. Rock Mech. Min. 2001, 38, 925–939. [Google Scholar] [CrossRef]
- Wu, J.Y.; Li, F.B. An improved stable XFEM (Is-XFEM) with a novel enrichment function for the computational modeling of cohesive cracks. Comput. Methods Appl. Mech. Eng. 2015, 295, 77–107. [Google Scholar] [CrossRef]
- Vásárhelyi, B.; Bobet, A. Modeling of Crack Initiation, Propagation and Coalescence in Uniaxial Compression. Rock Mech. Rock Eng. 2000, 33, 119–139. [Google Scholar] [CrossRef]
- Chong, W.L.; Haque, A.; Gamage, R.P.; Shahinuzzamanet, A. Modelling of intact and jointed mudstone samples under uniaxial and triaxial compression. Arab. J. Geosci. 2013, 6, 1639–1646. [Google Scholar] [CrossRef]
- Fan, L.F.; Yi, X.W.; Ma, G.W. Numerical manifold method (NMM) simulation of stress wave propagation through fractured rock mass. Int. J. Appl. Mech. 2013, 5, 238–249. [Google Scholar] [CrossRef]
- Fan, L.F.; Wong, L.N.Y. Stress wave transmission across a filled joint with different loading/unloading behavior. Int. J. Rock Mech. Min. 2013, 60, 227–234. [Google Scholar] [CrossRef]
- Verma, A.K.; Singh, T.N. Modeling of a jointed rock mass under triaxial conditions. Arab. J. Geosci. 2010, 3, 91–103. [Google Scholar] [CrossRef]
- Tang, C.; Tang, S.; Gong, B.; Bai, H. Discontinuous deformation and displacement analysis: From continuous to discontinuous. Sci. China Technol. Sci. 2015, 58, 1567–1574. [Google Scholar] [CrossRef]
- Lin, Q.; Labuz, J.F. Fracture of sandstone characterized by digital image correlation. Int. J. Rock Mech. Min. 2013, 60, 235–245. [Google Scholar] [CrossRef]
- Wang, H.; Kang, Y.L. Improved digital speckle correlation method and its application in fracture analysis of metallic foil. Opt. Eng. 2002, 41, 2793–2798. [Google Scholar] [CrossRef]
- Réthoré, J.; Gravouil, A.; Morestin, F.; Combescure, A. Estimation of mixed-mode stress intensity factors using digital image correlation and an interaction integral. Int. J. Fract. 2005, 132, 65–79. [Google Scholar] [CrossRef]
- Roux, S.; Hild, F. Stress intensity factor measurements from digital image correlation: Post-processing and integrated approaches. Int. J. Fract. 2006, 140, 141–157. [Google Scholar] [CrossRef]
- Yoneyama, S.; Ogawa, T.; Kobayashi, Y. Evaluating mixed-mode stress intensity factors from full-field displacement fields obtained by optical methods. Eng. Fract. Mech. 2007, 74, 1399–1412. [Google Scholar] [CrossRef]
- Zhang, H.; Huang, G.Y.; Song, H.P.; Kang, Y.L. Experimental investigation of deformation and failure mechanisms in rock under indentation by digital image correlation. Eng. Fract. Mech. 2012, 96, 667–675. [Google Scholar] [CrossRef]
- Cao, R.H.; Cao, P.; Lin, H.; Ma, G.W.; Chen, Y. Failure characteristics of intermittent fissures under a compressive-shear test: Experimental and numerical analyses. Theor. Appl. Fract. Mech. 2017, 96, 740–757. [Google Scholar] [CrossRef]
- Li, S.Y.; He, T.M.; Yin, X.C. Introduction to Rock Fracture Mechanics; Hefei University of Science and Technology of China Press: Hefei, China, 2010. [Google Scholar]
- Zhu, W.S.; Chen, W.Z.; Shen, J. Model test of fracture propagation in the shape of a goose and study of fracture mechanics. Chin. J. Solid Mech. 1998, 19, 355–360. [Google Scholar]






| Elastic Modulus (GPa) | Yield Strain (%) | Compressive Strength (Mpa) | Ultimate Strain (%) | Density (g/cm3) |
|---|---|---|---|---|
| 2.7 | 3.3 | 60 | 11.0 | 1.12 |
| Poisson’s Ratio (γ) | Elastic Modulus (Gpa) | Tensile Strength (Mpa) | Compressive Strength (Mpa) |
|---|---|---|---|
| 0.24 | 7.1 | 6.7 | 75 |
| Number | Sample | β/° | Note |
|---|---|---|---|
| 1 | TS# | 1. θ1 of all the samples is −45°; 2. θ2 of all the samples is +45°; 3. The length of b for the K–S fissures samples is 2 mm | |
| 2 | KS + 45# | +45 | |
| 3 | KS + 90# | +90 | |
| 4 | KS + 135# | +135 | |
| 5 | KS - 45# | −45 | |
| 6 | KS - 90# | −90 | |
| 7 | KS - 135# | −135 |
| Sample | Experimental Figures | Sketch of the Specimen | Cracking Pattern |
|---|---|---|---|
| TS# |  | 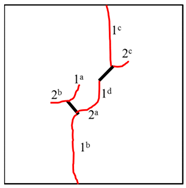 | T: 1a, 1b, 1c, 1d; S: 2a; L: 2b,2c |
| KS + 45# |  | 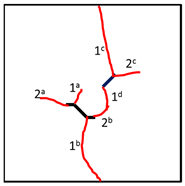 | T: 1a, 1b, 1c, 1d; S: 2b; L: 2a,2c |
| KS + 90# |  | 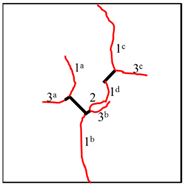 | T: 1a, 1b, 1c, 1d; S: 2, 3b; L: 3a,3c |
| KS + 135# |  |  | T: 1a, 1b, 1c, 1d; S: 2a; L: 2b, 2c |
| KS - 45# |  | 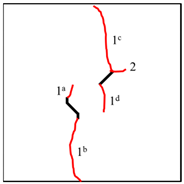 | T: 1a, 1b, 1c, 1d; L: 2 |
| KS - 90# |  | 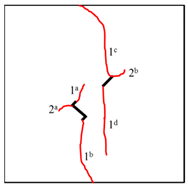 | T: 1a, 1b, 1c, 1d; L: 2a, 2b |
| KS - 135# |  | 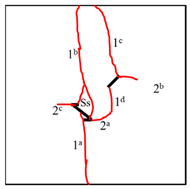 | T: 1a, 1b, 1c, 1d; S: 2a; L: 2b, 2c |
| Internal Friction Angle (°) | Elastic Modulus (GPa) | Coefficient of Deformation | Press Pull Ratio | Poisson’s Ratio (γ) | Pull Coefficient | Density (g/cm3) |
|---|---|---|---|---|---|---|
| 1.12 | 2.7 | 100 | 10 | 0.24 | 1.5 | 1.12 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, D.; Dong, Q. Fracturing and Damage of 3D-Printed Materials with Two Intermittent Fissures under Compression. Materials 2020, 13, 1607. https://doi.org/10.3390/ma13071607
Zhang D, Dong Q. Fracturing and Damage of 3D-Printed Materials with Two Intermittent Fissures under Compression. Materials. 2020; 13(7):1607. https://doi.org/10.3390/ma13071607
Chicago/Turabian StyleZhang, Duan, and Qianqian Dong. 2020. "Fracturing and Damage of 3D-Printed Materials with Two Intermittent Fissures under Compression" Materials 13, no. 7: 1607. https://doi.org/10.3390/ma13071607
APA StyleZhang, D., & Dong, Q. (2020). Fracturing and Damage of 3D-Printed Materials with Two Intermittent Fissures under Compression. Materials, 13(7), 1607. https://doi.org/10.3390/ma13071607




