Evaluation of the Thermal Stability and Surface Characteristics of Thermoplastic Polyurethane V-Belt
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
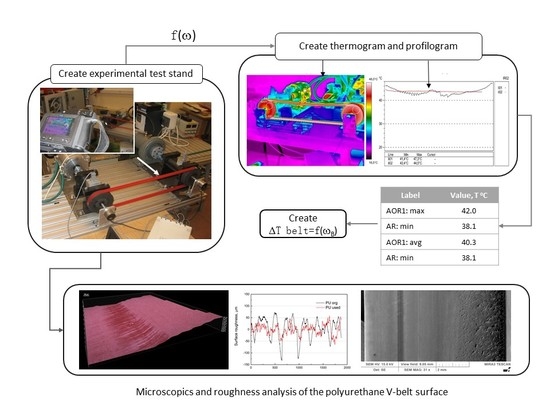
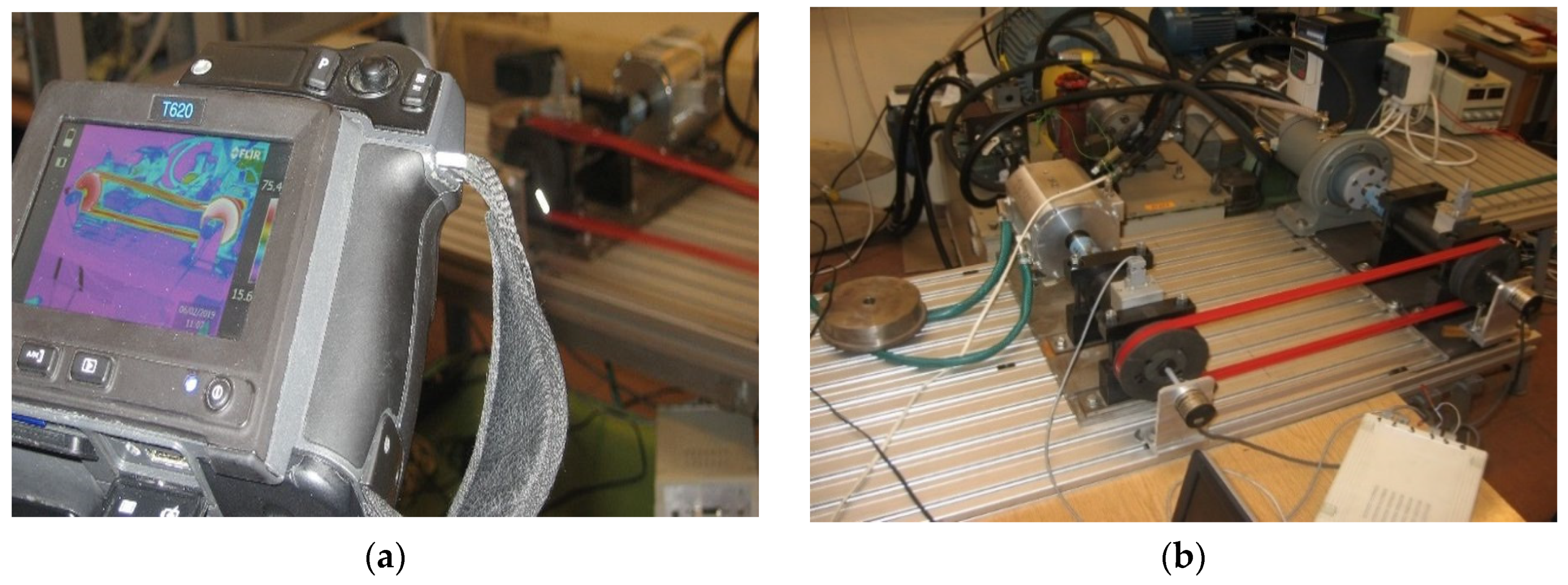
2.2. Experimental Test Stand
2.3. Thermal Tests
2.4. Hardness Test
2.5. Optical Microscopy
2.6. Surface Roughness
2.7. Scanning Electron Microscopy (SEM)
3. Results and Discussions
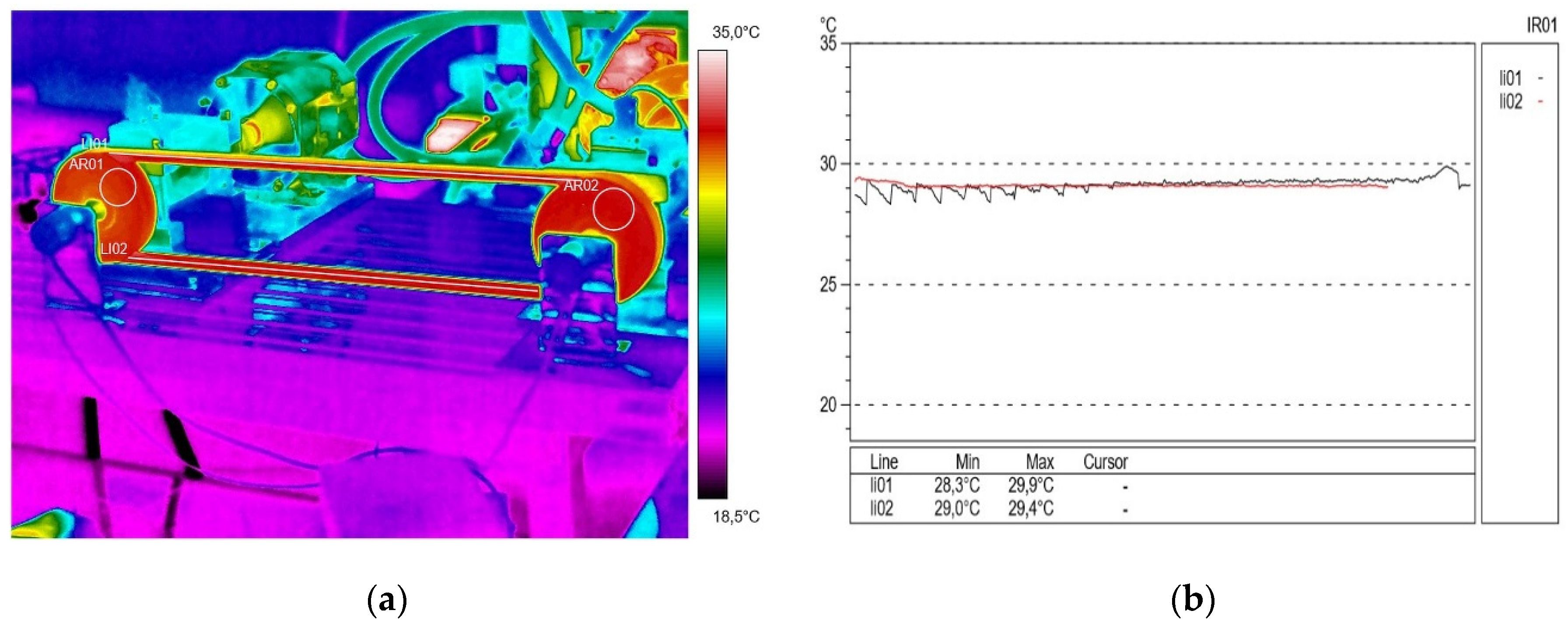
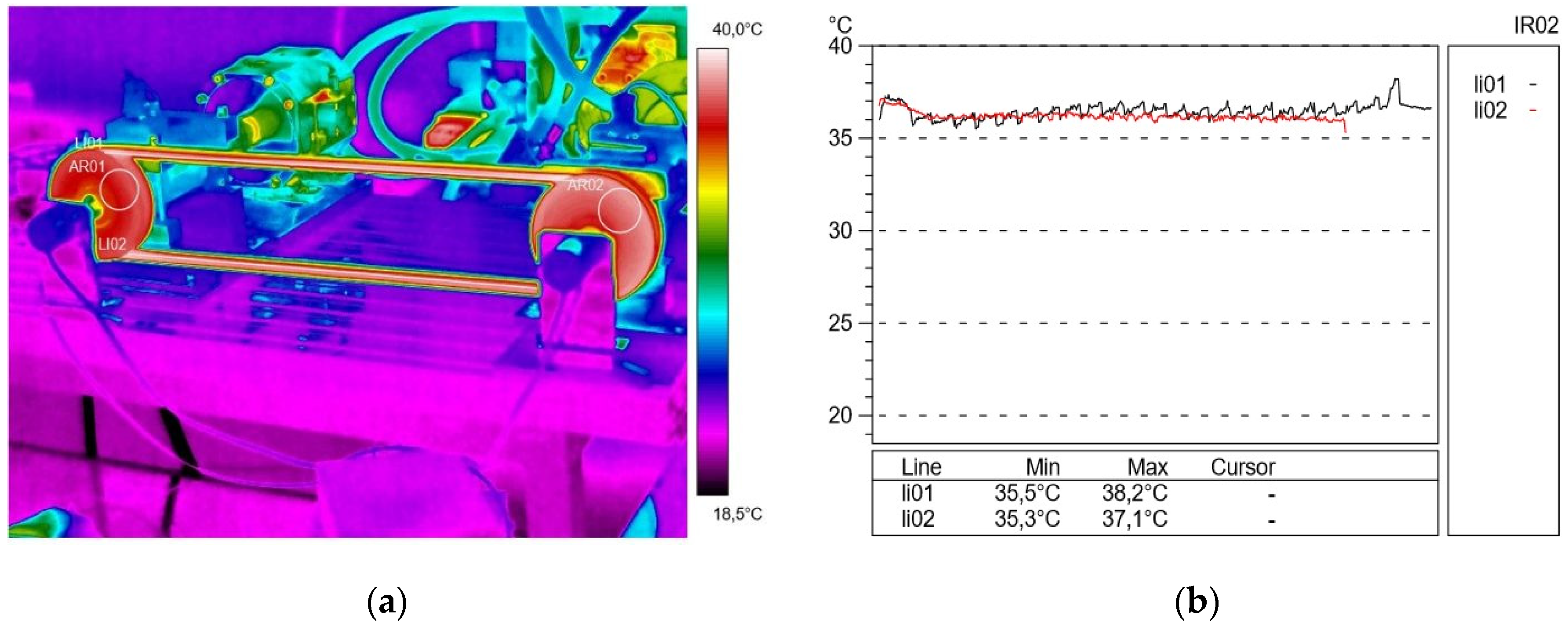
3.1. Thermal Stability Analysis
3.2. Hardness and Surface Roughness Analysis
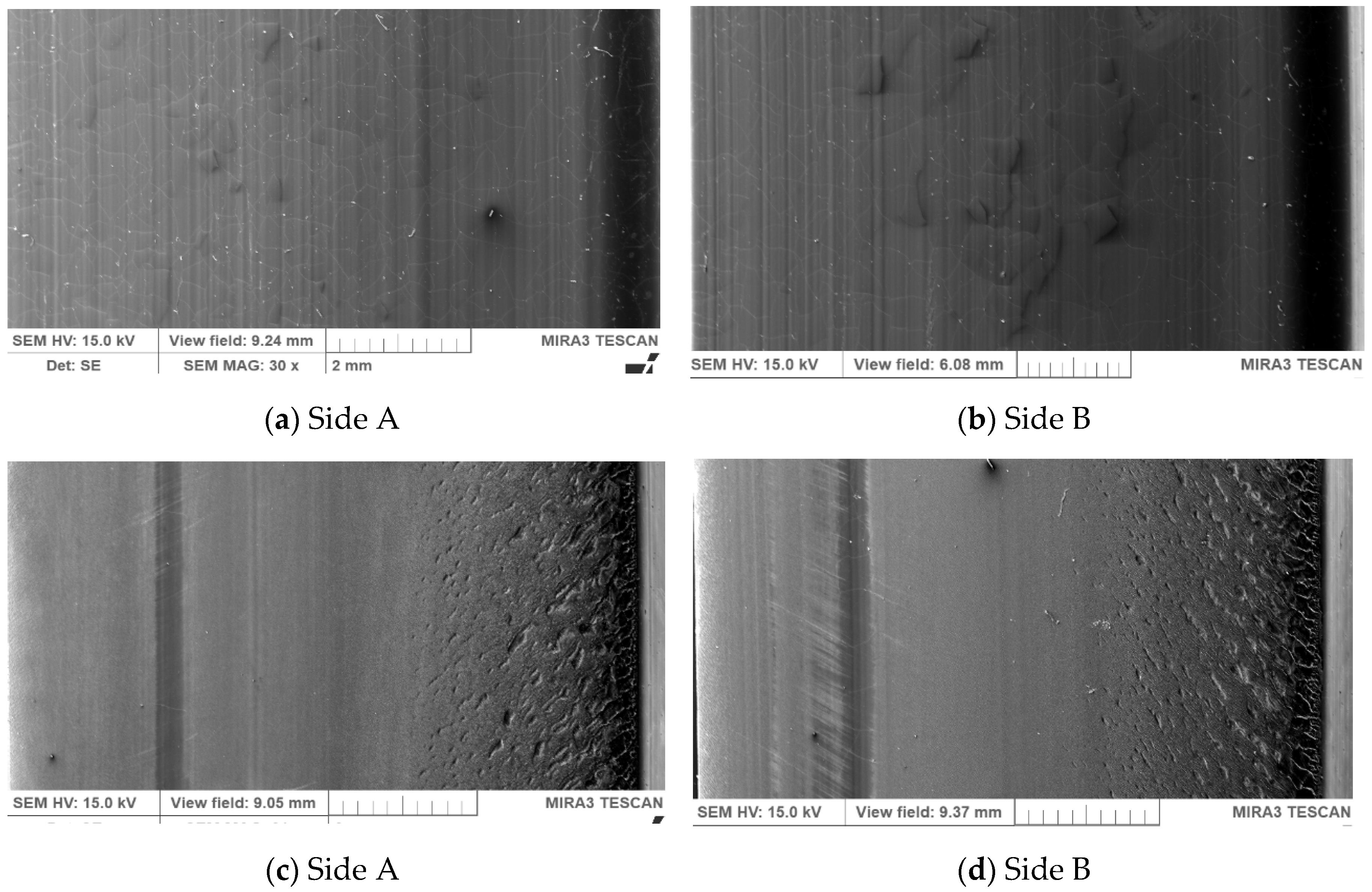
3.3. Optical and SEM Surface Morphology Analysis
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Amanov, A.; Sembiring, J.P.B.A.; Amanov, T. Experimental Investigation on Friction and Wear Behavior of the Vertical Spindle and V-belt of a Cotton Picker. Materials 2019, 12, 773. [Google Scholar] [CrossRef] [PubMed]
- Yu, D.; Childs, T.H.C.; Dalgarno, K.W. V-ribbed belt design, wear and traction capacity. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 1998, 212, 333–344. [Google Scholar] [CrossRef]
- Kong, L.; Parker, R.G. Coupled Belt-Pulley Vibration in Serpentine Drives With Belt Bending Stiffness, ASME. J. Appl. Mech. 2004, 71, 109–119. [Google Scholar] [CrossRef]
- Gao, P.; Xie, L.; Pan, J. Reliability and Availability Models of Belt Drive Systems Considering Failure Dependence. Chin. J. Mech. Eng. 2019, 32, 30. [Google Scholar] [CrossRef]
- Czarnecka-Komorowska, D.; Struski, P. Kompozyty Polimerowe Wytworzone z Odpadów Polioksymetylenu (rPOM) i Poliuretanu Termoplastycznego—Nowe Materiały. Nowoczesne Materiały Polimerowe i ich Przetwórstwo; Klepka, T., Ed.; Wyd. Politechnika Lubelska: Lublin, Poland, 2017. [Google Scholar]
- Thomas, S.; Yang, W. Advances in Polymer Processing: From Macro- To Nano- Scales; Woodhead Publishing: Cambridge, UK, 2009. [Google Scholar]
- Whm_catalogue.pdf. Available online: https://www.whm.pl/oferta/technika-napedow/termozgrzewalne-pasy-okragle-i-klinowe-z-pu.html (accessed on 22 October 2019).
- Behabelt_cataloque.pdf. Available online: http://www.behabelt.com/terms/agb.pdf (accessed on 22 October 2019).
- Kong, L.; Parker, R.G. Steady Mechanics of Belt-Pulley Systems. J. Appl. Mech. 2005, 72, 25. [Google Scholar] [CrossRef]
- Merghache, S.M.; Ghernaout, M.E.A. Experimental and numerical study of heat transfer through asynchronous belt transmission type AT10. Appl. Therm. Eng. 2017, 127, 705–717. [Google Scholar] [CrossRef]
- Silva, C.A.F.; Manin, L.; Rinaldi, R.G.; Remond, D.; Besnier, E.; Andrianoely, M.A. Modeling of power losses in poly-V belt transmissions: Hysteresis phenomena (enhanced analysis). Mech. Mach. Theory. 2018, 121, 373–397. [Google Scholar] [CrossRef]
- Czarnecka-Komorowska, D.; Mencel, K. Modification of polyamide 6 and polyoxymethylene with [3-(2-aminoethyl)amino]propyl-heptaisobutyl-polysilsesquioxane nanoparticles. Przem. Chem. 2014, 93, 392–396. [Google Scholar]
- De Almeida, A.; Greenberg, S. Technology assessment: Energy-efficient belt transmissions. Energy Build. 1995, 22, 245–253. [Google Scholar] [CrossRef]
- Shim, H.J.; Kim, J.K. Cause of failure and optimization of a V-belt pulley considering fatigue life uncertainty in automotive applications. Eng. Fail. Anal. 2009, 16, 1955–1963. [Google Scholar] [CrossRef]
- Krawiec, P.; Grzelka, M.; Kroczak, J.; Domek, G.; Kołodziej, A. A proposal of measurement methodology and assessment of manufacturing methods of nontypical cog belt pulleys. Measurement 2019, 132, 182–190. [Google Scholar] [CrossRef]
- Krawiec, P. Numerical Analysis of Geometrical Characteristics of Machine Elements Obtained Through CMM Scanning. In Progress in Industrial Mathematics; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Kujawski, M.; Krawiec, P. Analysis of Generation Capabilities of Noncircular Cog belt Pulleys on the Example of a Gear with an Elliptical Pitch Line. J. Manuf. Sci. Eng. Trans. ASME 2011, 133, 051006. [Google Scholar] [CrossRef]
- Krawiec, P. Analysis of selected dynamic features of a two-wheeled transmission system. J. Theor. Appl. Mech. 2017, 55, 461–467. [Google Scholar] [CrossRef]
- Krawiec, P.; Marlewski, A. Profile design of noncircular belt pulleys. J. Theor. Appl. Mech. 2016, 54, 561–570. [Google Scholar] [CrossRef]
- Krawiec, P.; Waluś, K.; Warguła, Ł.; Adamiec, J. Wear evaluation of elements of V-belt transmission with the application of optical microscope. In Proceedings of the MATEC Web of Conferences, Proceedings of the Machine Modelling and Simulations, Sklené Teplice, Slovak Republic, 5–8 September 2017; Volume 157. [Google Scholar]
- Krawiec, P.; Warguła, Ł.; Waluś, K.; Adamiec, J. Wear evaluation study of the multiple grooved pulleys with optical method. In Proceedings of the MATEC Web of Conferences, Proceedings of the XXIII Polish-Slovak Scientific Conference on Machine Modelling and Simulations, Rydzyna, Poland, 4–7 September 2018; Volume 254. [Google Scholar]
- Krawiec, P.; Warguła, Ł.; Dziechciarz, A.; Małozięć, D.; Ondrušová, D. Evaluation of chemical compound emissions during thermal decomposition and combustion of V-belts. Przem. Chem. 2020, 99, 92–98. [Google Scholar]
- Krawiec, P.; Różański, L. Usage of IR Thermography in Researching of Uneven Strand Transmissions with Cogbelts. Pomiary Autom. Kontrola 2009, 11, 966–969. [Google Scholar]
- Walentynowicz, J.; Trawiński, G.; Wieczorek, M.; Polak, F.; Boruta, G. Methods of verification of main transmission gear boxes on test bench using vibrat;on measurement. J. KONES Powertrain Transp. 2013, 20, 471–478. [Google Scholar] [CrossRef]
- Jakubek, B.; Barczewski, R.; Rukat, W.; Różański, L.; Wrobel, M. Stabilization of vibro-thermal processes during post-production testing of rolling bearings. Diagnostyka 2019, 20, 53–62. [Google Scholar] [CrossRef]
- Lepiarczyk, D.; Tarnowski, J.; Uhrynski, A.; Gawedzki, W. Usage of thermo-vision in research concerning kinematic pair of friction in machines and mechanical devices. Технології дизайн Машини обладнання легкої Татекстильної промисловості 2012, 4, 1–7. [Google Scholar]
- Kuchynkova, H.; Hajek, V. Measurement of temperature of electrical Machines using thermovision camera. Zesz. Probl. Masz. Elektr. 2010, 87, 139–144. [Google Scholar]
- Barański, M.; Polak, A. Thermal diagnostic in electrical machines. Przegląd Elektrotech. Electr. Rev. 2011, 87, 305–308. [Google Scholar]
- Rosca, D.; Rosca, A. Thermovision predictive maintenance for large capacity milling process in food industry. In Annals of DAAAM for 2011 & Proceedings of the 22nd International DAAAM Symposium; Katalinic, B., Ed.; DAAAM International: Vienna, Austria, 2011; pp. 1407–1408. [Google Scholar]
- Wargula, Ł.; Waluś, K.J.; Krawiec, P. The problems of measuring the temperature of the small engines (SI) on the example of a drive for non-road mobile machines. In MATEC Web of Conferences, Proceedings of the XXIII Polish-Slovak Scientific Conference on Machine Modelling and Simulations, Rydzyna, Poland, 4–7 September 2019; EDP Sciences: Lez Ili, France, 2019; p. 254. [Google Scholar]
- Worsztynowicz, B.; Uhryński, A. The analysis of heating process of catalytic converter using thermo-vision. Combust. Engines 2015, 162, 41–51. [Google Scholar]
- Urzędowska, W.; Stępień, Z. Selected issues regarding the change of engine lubricating oil properties in operation (orginal title in Polish: Wybrane zagadnienia dotyczące zmiany właściwości silnikowego oleju smarowego w eksploatacji). NAFTA-GAZ 2012, 12, 1102–1110. [Google Scholar]
- Warguła, Ł.; Kaczmarzyk, P.; Dziechciarz, A. The assessment of fire risk of non-road mobile wood chopping machines. J. Res. Appl. Agric. Eng. 2019, 64, 58–64. [Google Scholar]
- Kaczmarzyk, P.; Małozięć, D.; Warguła, Ł. Research on electrical wiring used in the construction of working machines and vehicles in the aspect of fire protection. J. Mech. Transp. Eng. 2018, 70, 13–24. [Google Scholar]
- Hakami, F.; Pramanik, A.; Ridgway, N.; Basak, A.K. Developments of rubber material wear in conveyer belt system. Tribol. Int. 2017, 111, 148–158. [Google Scholar] [CrossRef]
- Osornio-Rios, R.; Antonino-Daviu, J.; de Jesus Romero-Troncoso, R. Recent industrial applications of infrared thermography: A review. IEEE Trans. Ind. Inform. 2018, 15, 615–625. [Google Scholar] [CrossRef]
- Królczyk, G.; Li, Z.; Antonino Daviu, J.A. Fault Diagnosis of Rotating Machine. Appl. Sci. 2020, 10, 1961. [Google Scholar] [CrossRef]
- Bagavathiappan, S.; Lahiri, B.B.; Saravanan, T.; Philip, J.; Jayakumar, T. Infrared thermography for condition monitoring—A review. Infrared Phys. Technol. 2013, 60, 35–55. [Google Scholar] [CrossRef]
- Dudek, K.; Banasiak, J.; Bieniek, J. Diagnosis of kinematic pairs in the rotary mowers. Maint. Reliab. 2003, 20, 17–21. [Google Scholar]
- Tor-Świątek, A.; Samujło, B. Wykorzystanie badań termowizyjnych do analizy stabilności procesu wytłaczania mikroporującego poli(chlorku winylu). Maint. Reliab. 2013, 15, 58–61. [Google Scholar]
- Szymiczek, M. Ultrasonic and thermal testing as a diagnostic tool for the evaluation of cumulative discontinuities of the polyester-glass pipes structure. Maint. Reliab. 2017, 19, 1–7. [Google Scholar] [CrossRef]
- Jia, S.S.; Song, Y.M. Elastic dynamic analysis of synchronous belt drive system using absolute nodal coordinate formulation. Nonlinear Dyn. 2015, 81, 1393–1410. [Google Scholar] [CrossRef]
- Li, W.B.; Xin, Z.X. Flexural fatigue life prediction of a tooth V-belt made of fiber reinforced rubber. Int. J. Fatigue 2018, 111, 269–277. [Google Scholar] [CrossRef]
- Różański, L.; Ziopaja, K. Applicability analysis of IR thermography and discrete wavelet transform for technical conditions assessment of bridge elements. Quant. InfraRed Thermogr. J. 2019, 16, 87–110. [Google Scholar] [CrossRef]
- Sundararaman, S.; Hu, J.; Chen, J.; Chandrashekhara, K. Temperature dependent fatigue-failure analysis of V-ribbed serpentine belts. Int. J. Fatigue 2009, 31, 1262–1270. [Google Scholar] [CrossRef]
- Chelladurai, V.; Jayas, D.; White, N. Thermal imaging for detecting fungal infection in stored wheat. J. Stored Prod. Res. 2010, 46, 174–179. [Google Scholar] [CrossRef]
- Chrzanowski, K. Błędy Metod Zdalnego Pomiaru Temperatury za Pomocą Urządzeń Podczerwieni; Wyd. WAT: Warszawa, Poland, 1996. [Google Scholar]
- Chrzanowski, K. Non-Contact Thermometry—Measurement Errors; Polski Oddział SPIE: Warszawa, Poland, 2000. [Google Scholar]
- Fidali, M.; Urbanek, G. Application of evolutionary algorithm to limitation of a set of statistical features of thermovision images. Comput. Assist. Mech. Eng. Sci. 2007, 14, 601–609. [Google Scholar]
- Gade, R.; Moeslund, T. Thermal cameras and applications: A survey. Mach. Vis. Appl. 2014, 25, 245–262. [Google Scholar] [CrossRef]
- Gawdzińska, K.; L. Chybowski, L.; Przetakiewicz, W. Study of Thermal Properties of Cast Metal-Ceramic Composite Foams. Arch. Foundry Eng. 2017, 17, 47–50. [Google Scholar]
- Jóźwik, J. Thermographic evaluation of temperature distribution on surface of workpiece during non-orthogonal turning. Maint. Reliab. 2001, 5, 58–61. [Google Scholar]
- Minkina, W. Pomiary Termowizyjne—Przyrządy i Metody; Wyd. Politechniki Częstochowskiej: Częstochowa, Poland, 2014. [Google Scholar]
- Stawicki, T.; Sędłak, P. Thermal Imaging Studies on Grain Harvester Belt Transmissions. Diagnostyka 2015, 16, 37–42. [Google Scholar]
- Muniz, P.R.; de Araújo Kalid, R.; Cani, K.R.S.; da Silva Magalhães, R. Handy method to estimate uncertainty of temperature measurement by infrared thermography. Opt. Eng. 2014, 53, 074101. [Google Scholar] [CrossRef]
- Minkina, W.; Dudzik, S. Infrared thermography. In Errors and Uncertainty; John Wiley & Sons, Ltd.: Chichester, UK, 2009. [Google Scholar]
- Minkina, W.; Dudzik, S. Simulation analysis of uncertainty of infrared camera measurement and processing path. Measurement 2006, 39, 758–763. [Google Scholar] [CrossRef]
- Gao, P.; Xie, L. Reliability and Lifetime Distribution Analysis of Belt Drive Systems considering Time-Dependent Stiffness of Belts. Hindawi Shock Vib. 2018, 2018, 1–9. [Google Scholar] [CrossRef]
- Eurobelt_catalogue.pdf. Available online: http://www.eurobelt.pl (accessed on 22 October 2019).
- Budinski, K.G. Resistance to particle abrasion of selected plastics. Wear 1997, 203–204, 302–309. [Google Scholar] [CrossRef]
- Hooke, C.J.; Kukureka, S.N.; Liao, P.; Rao, M.; Chen, Y.K. The friction and wear of polymers in non-conformal contacts. Wear 1996, 200, 83–94. [Google Scholar] [CrossRef]
- Nachman, M.; Kwiatkowski, K. The effect of thermal annealing on the abrasion resistance of a segmented block copolymer urethane elastomers. Wear 2013, 306, 113–118. [Google Scholar] [CrossRef]
- Czarnecka-Komorowska, D.; Sterzynski, T.; Dutkiewicz, M. Polyoxymethylene/polyhedral oligomeric silsesquioxane composites: Processing, crystallization, morphology and thermo-mechanical behavior. Int. Polym. Process. 2016, 31, 598–606. [Google Scholar] [CrossRef]
- Czarnecka-Komorowska, D.; Sterzynski, T. Effect of Polyhedral Oligomeric Silsesquioxane on the Melting, Structure, and Mechanical Behavior of Polyoxymethylene. Polymers 2018, 10, 203. [Google Scholar] [CrossRef]
- Wieleba, W.; Dobrowolska, A. Frictional resistance of polymer materials during sliding on ice. Tribologia 2011, 5, 231–238. [Google Scholar]
- Wróblewska-Krepsztul, J.; Rydzkowski, T.; Michalska-Pożoga, I.; Thakur, V.K. Biopolymers for Biomedical and Pharmaceutical Applications: Recent Advances and Overview of Alginate Electrospinning. Nanomaterials 2019, 9, 404. [Google Scholar] [CrossRef] [PubMed]
- Pawlus, P.; Reizer, R.; Wieczorowski, M. Reverse Problem in Surface Texture Analysis—One-Process Profile Modeling on the Basis of Measured Two-Process Profile after Machining or Wear. Materials 2019, 12, 4169. [Google Scholar] [CrossRef] [PubMed]
- Firas, A.; Zhang, S.; Tripathi, M.; Nikiforov, A.; Pugno, N. Cracks, microcracks and fracture in polymer structures: Formation, detection, autonomic repair. Prog. Mater. Sci. 2016, 83, 536–573. [Google Scholar]
- Capanidis, D. The influence of hardness of polyurethane on its abrasive wear resistance. Tribologia 2016, 4, 29–39. [Google Scholar] [CrossRef]
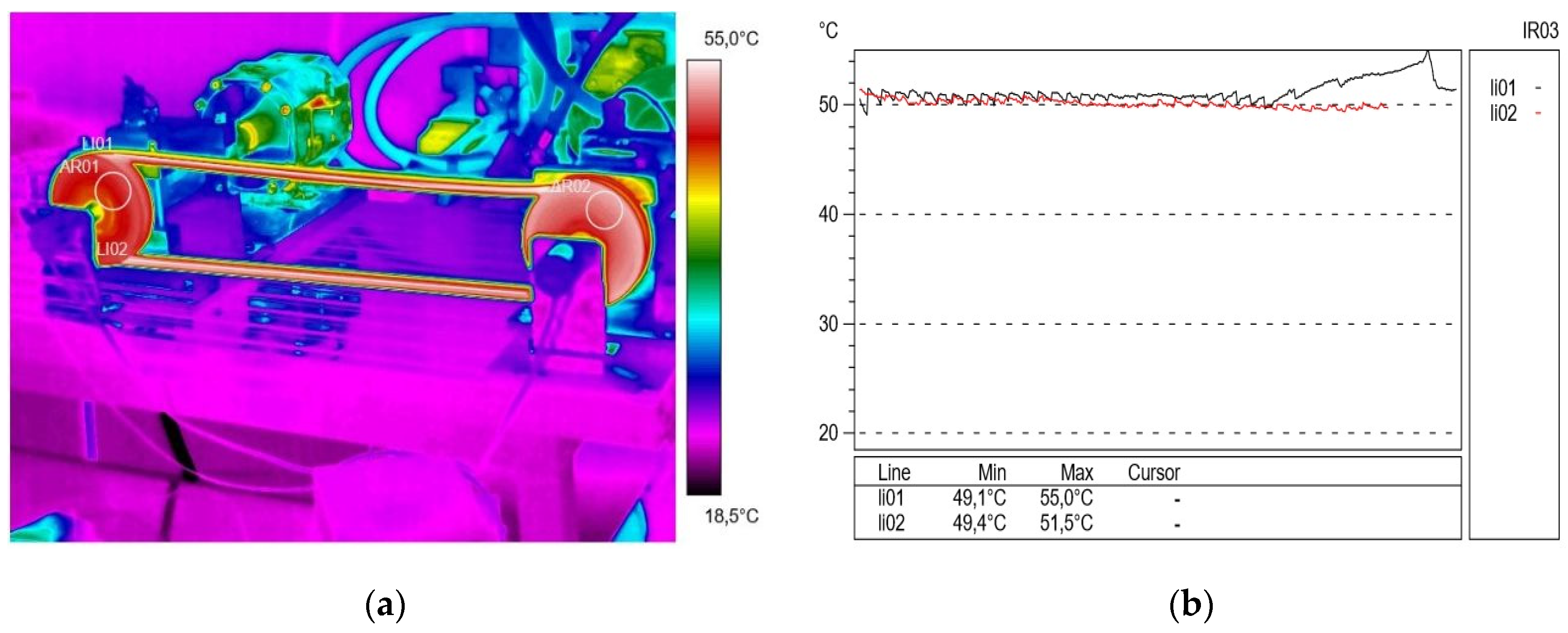
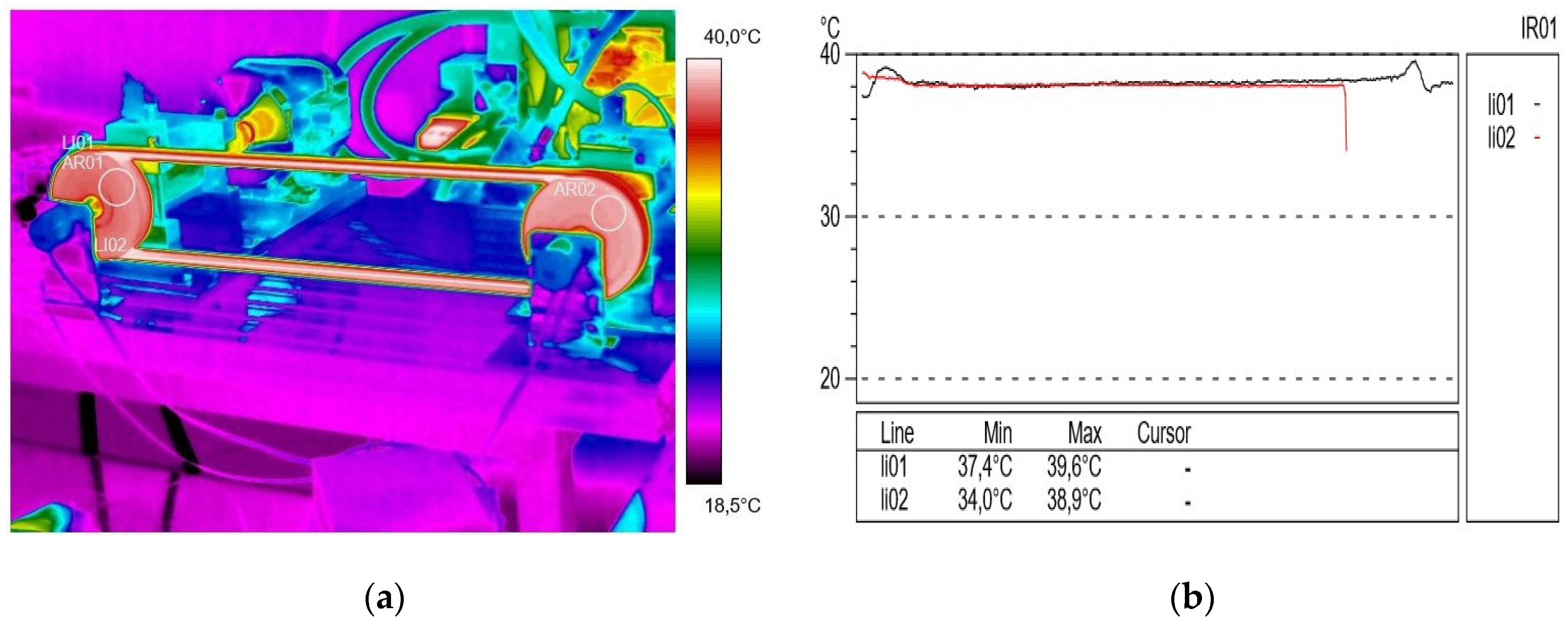
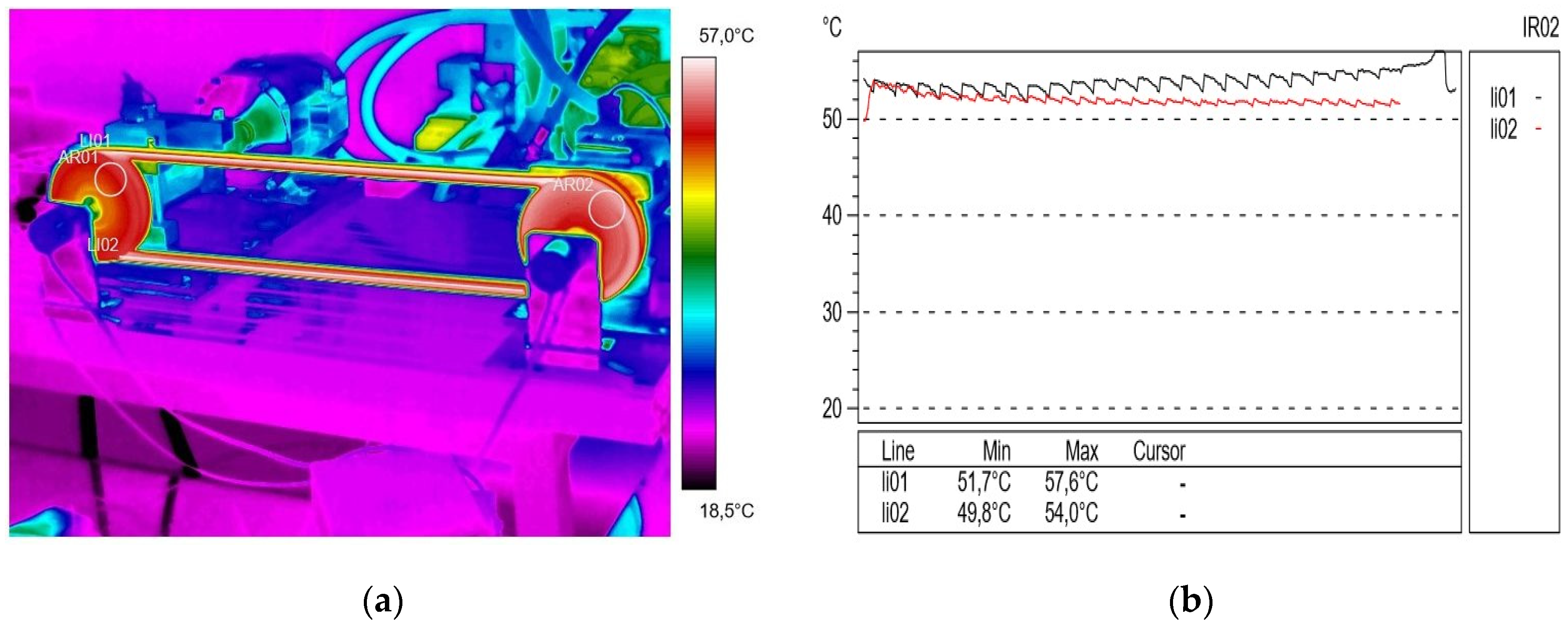
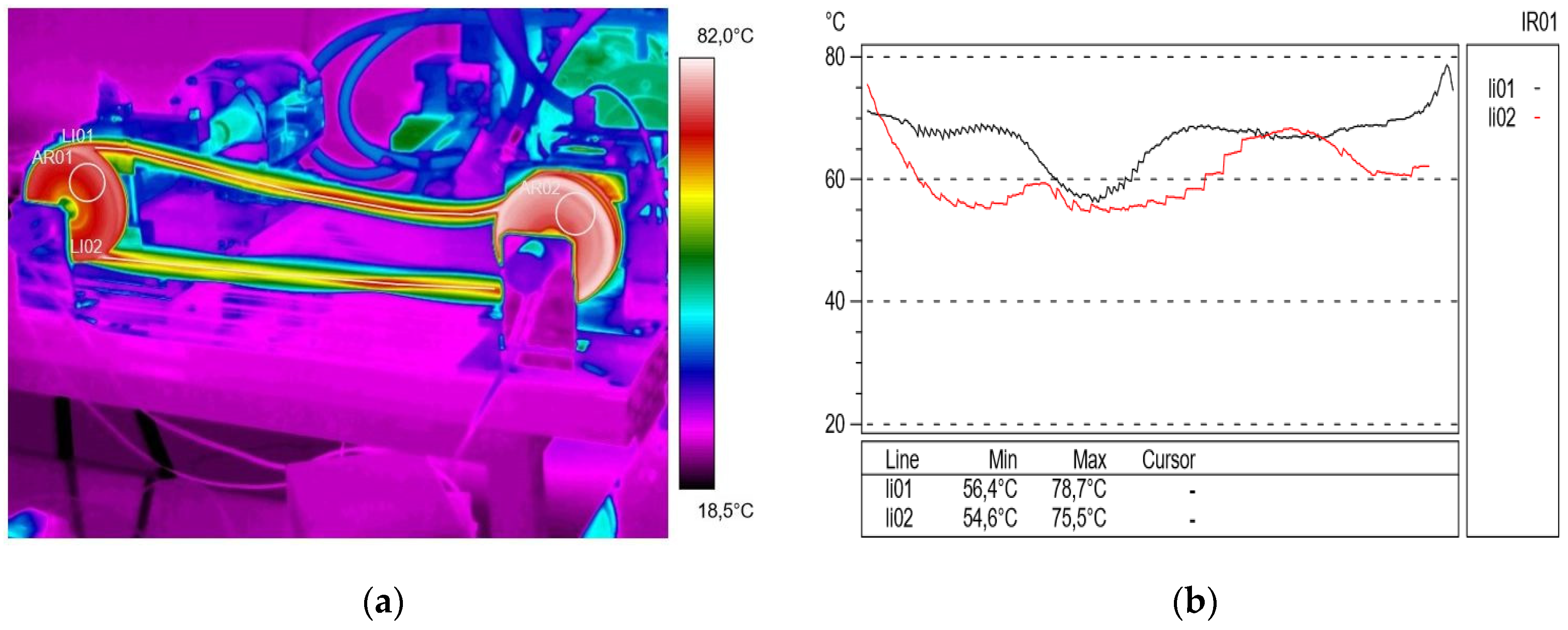
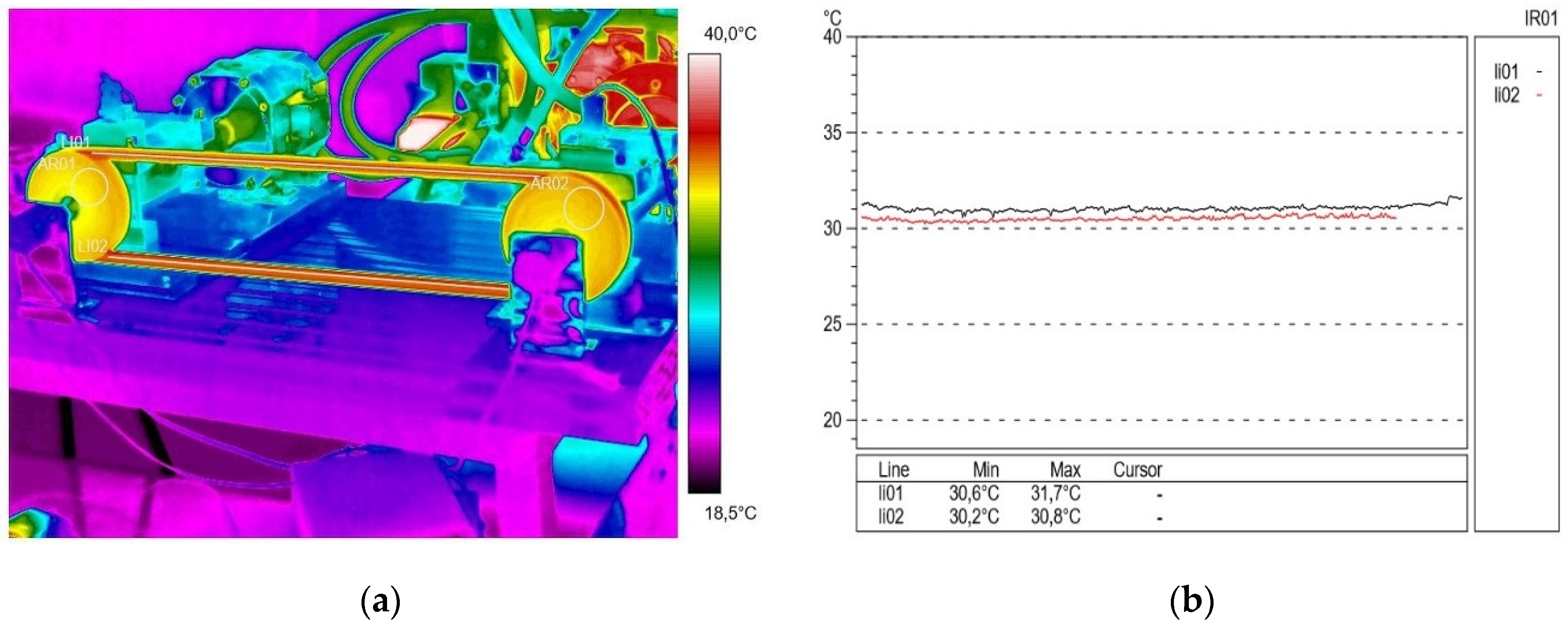
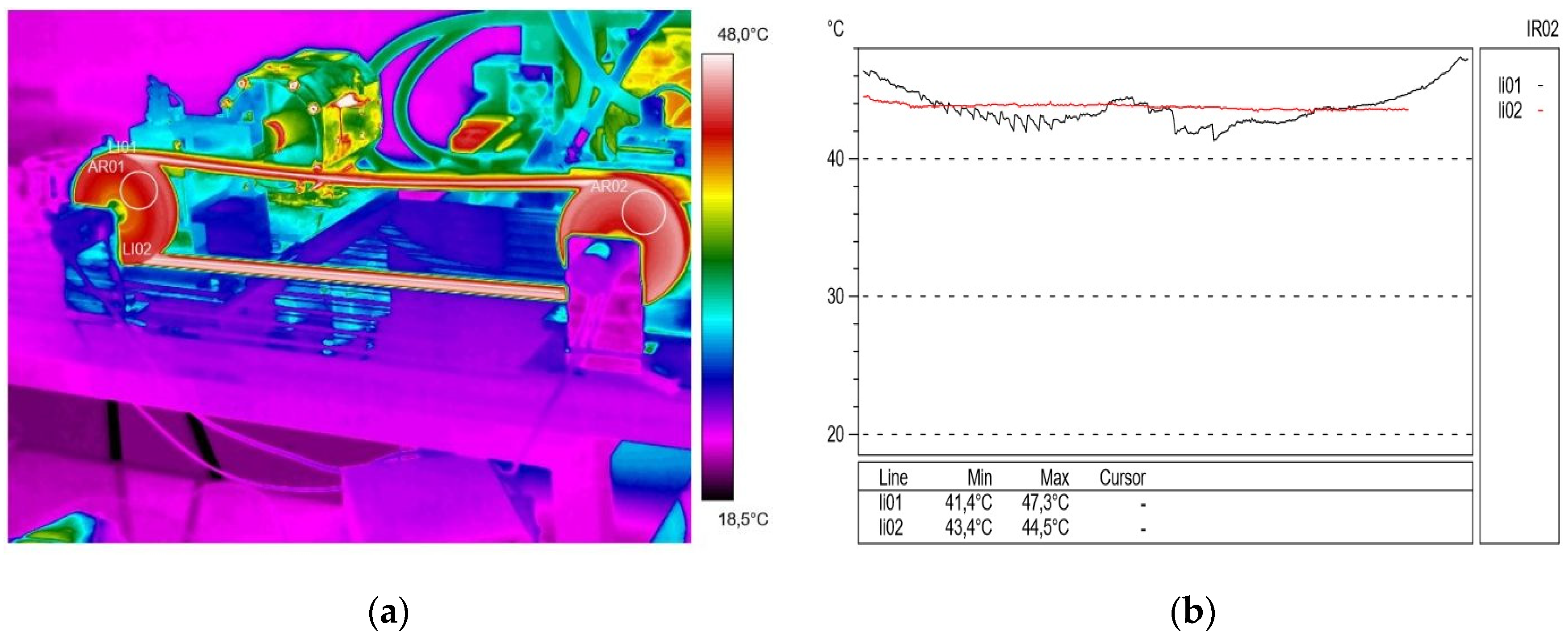
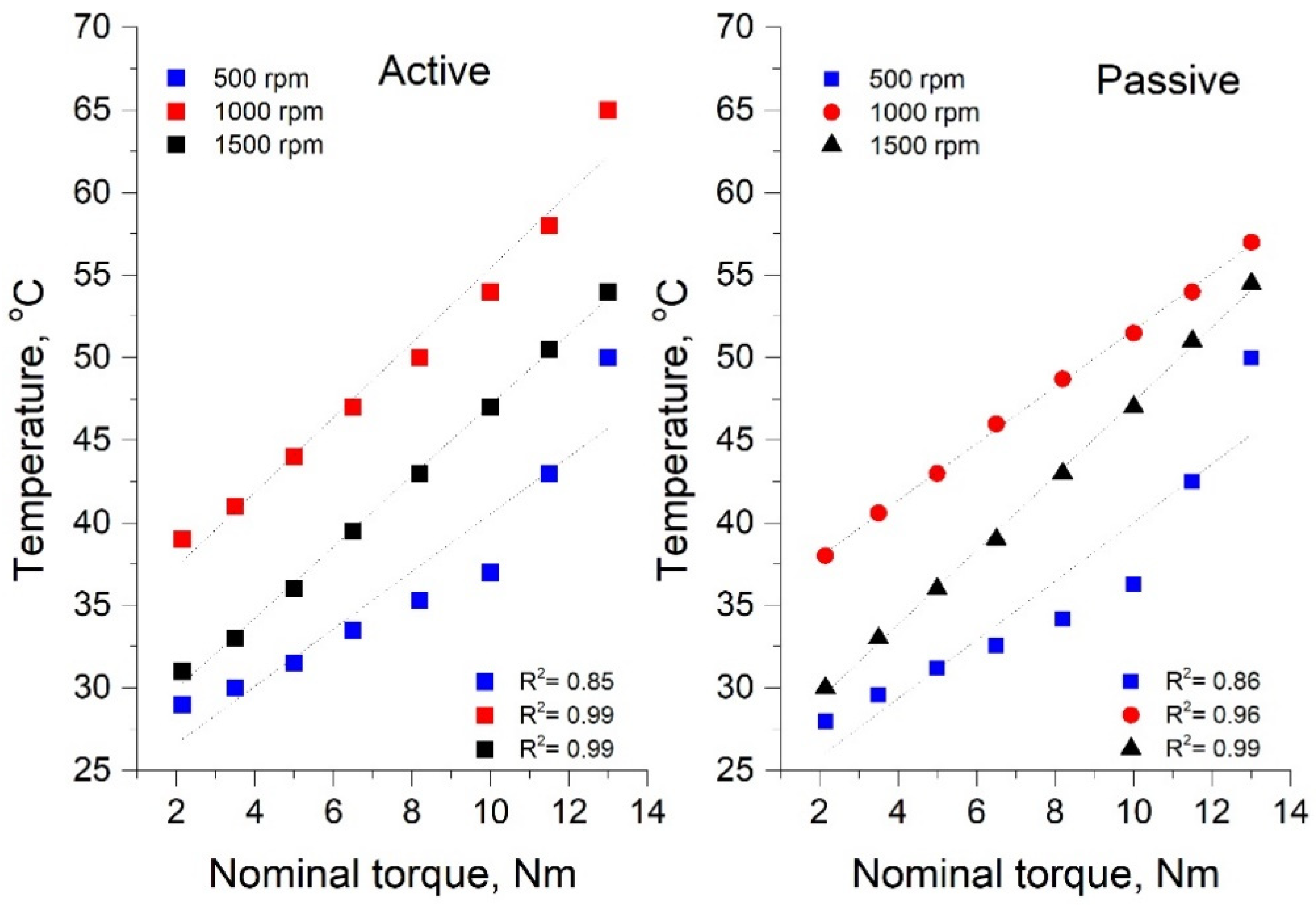

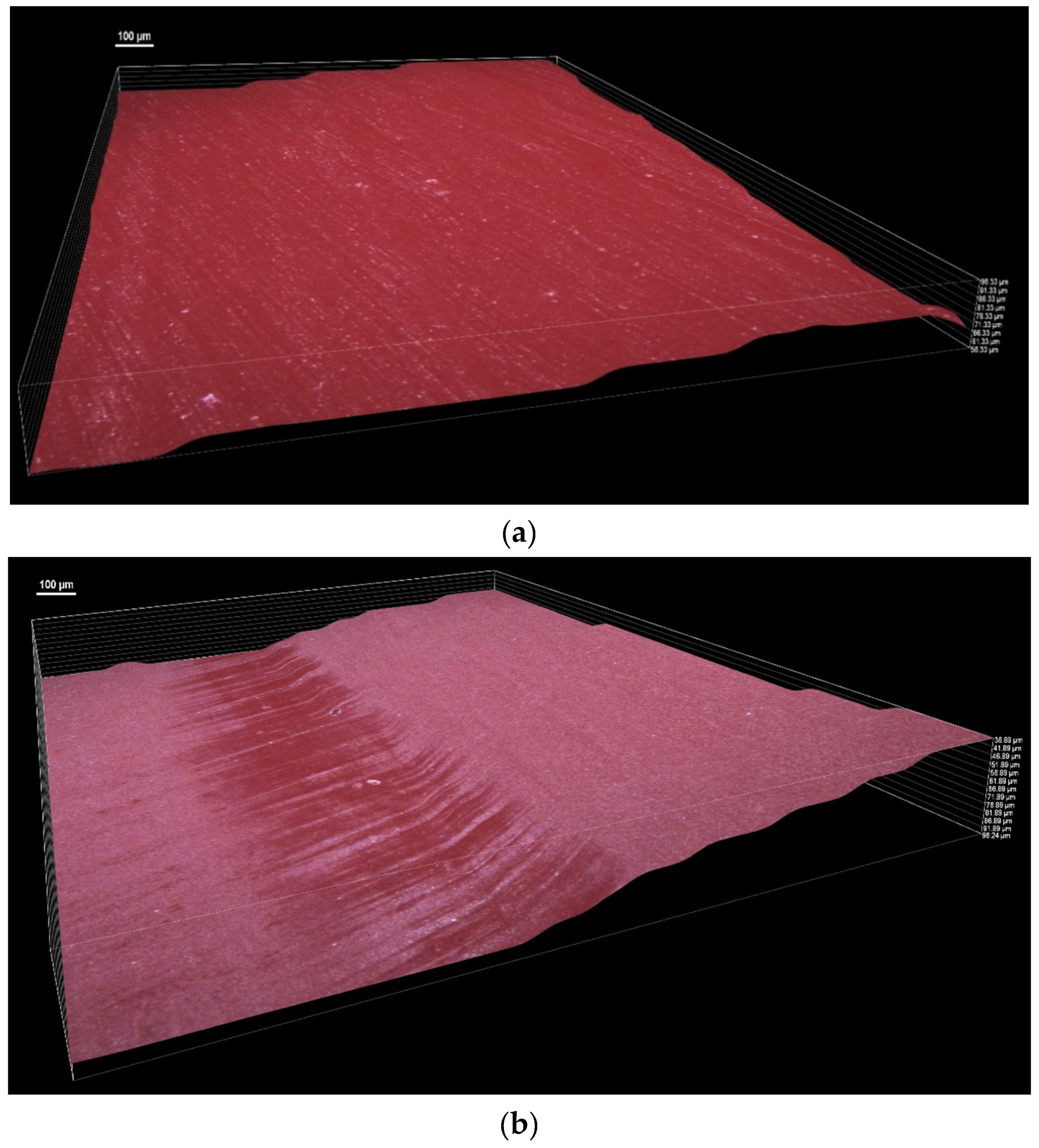

| Properties | Profile Dimension | Hardness | Coefficient Friction Steel Topside | Recommended Min. Pulley | Working Tension |
|---|---|---|---|---|---|
| PU 75A | 17 × 11 mm | 80 °ShA | 0.7 µ | 100 mm | 19 dN/belt |
| Profilometry Parameter | Original PU Belt | Used PU Belt |
|---|---|---|
| Ra (μm) | 0.349 ± 0.15 | 0.179 ± 0.13 |
| Rq (μm) Rz (μm) | 0.423 ± 0.12 1.576 ± 0.11 | 0.894 ± 0.09 0.216 ± 0.10 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Krawiec, P.; Różański, L.; Czarnecka-Komorowska, D.; Warguła, Ł. Evaluation of the Thermal Stability and Surface Characteristics of Thermoplastic Polyurethane V-Belt. Materials 2020, 13, 1502. https://doi.org/10.3390/ma13071502
Krawiec P, Różański L, Czarnecka-Komorowska D, Warguła Ł. Evaluation of the Thermal Stability and Surface Characteristics of Thermoplastic Polyurethane V-Belt. Materials. 2020; 13(7):1502. https://doi.org/10.3390/ma13071502
Chicago/Turabian StyleKrawiec, Piotr, Leszek Różański, Dorota Czarnecka-Komorowska, and Łukasz Warguła. 2020. "Evaluation of the Thermal Stability and Surface Characteristics of Thermoplastic Polyurethane V-Belt" Materials 13, no. 7: 1502. https://doi.org/10.3390/ma13071502
APA StyleKrawiec, P., Różański, L., Czarnecka-Komorowska, D., & Warguła, Ł. (2020). Evaluation of the Thermal Stability and Surface Characteristics of Thermoplastic Polyurethane V-Belt. Materials, 13(7), 1502. https://doi.org/10.3390/ma13071502