Coupled Free Vibration of Spinning Functionally Graded Porous Double-Bladed Disk Systems Reinforced with Graphene Nanoplatelets
Abstract
1. Introduction
2. Theoretical Formulations
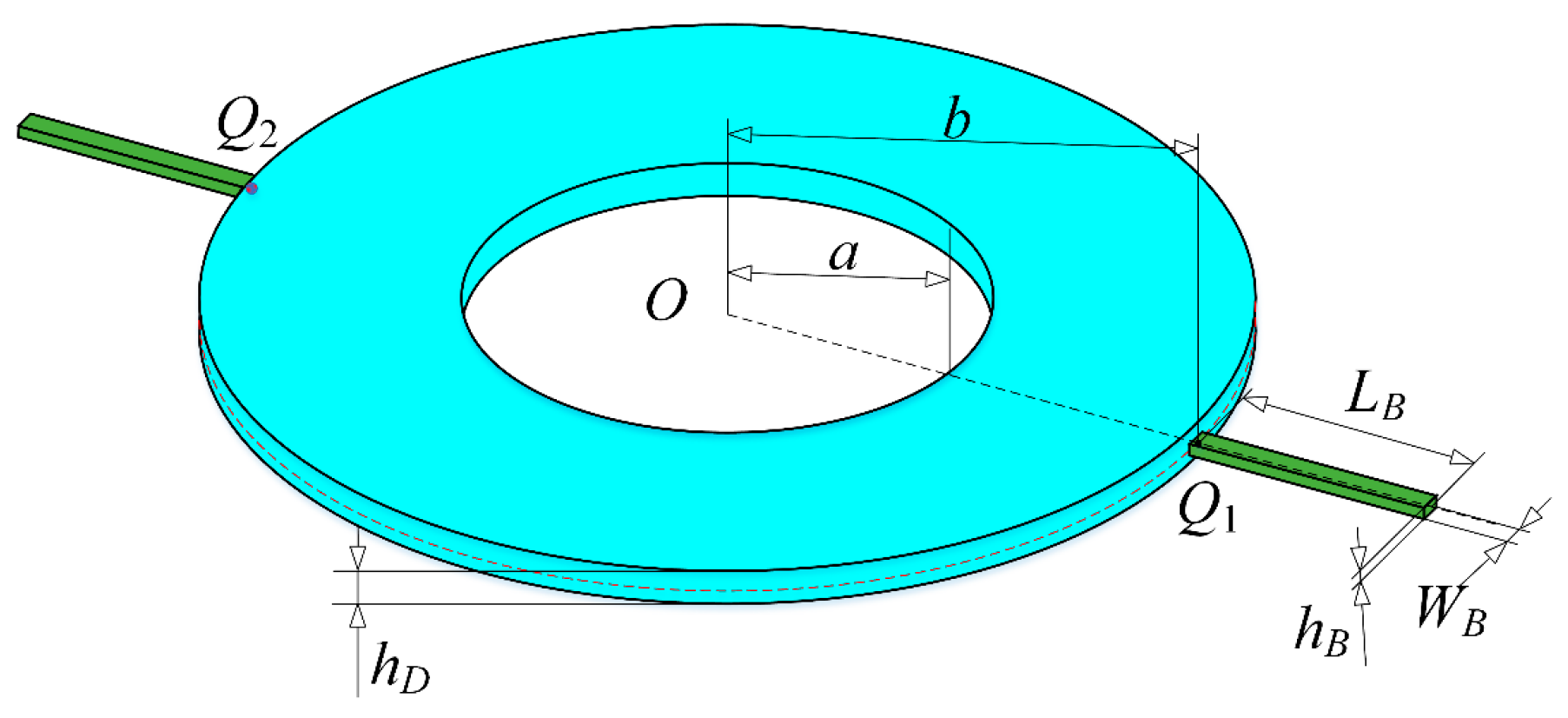
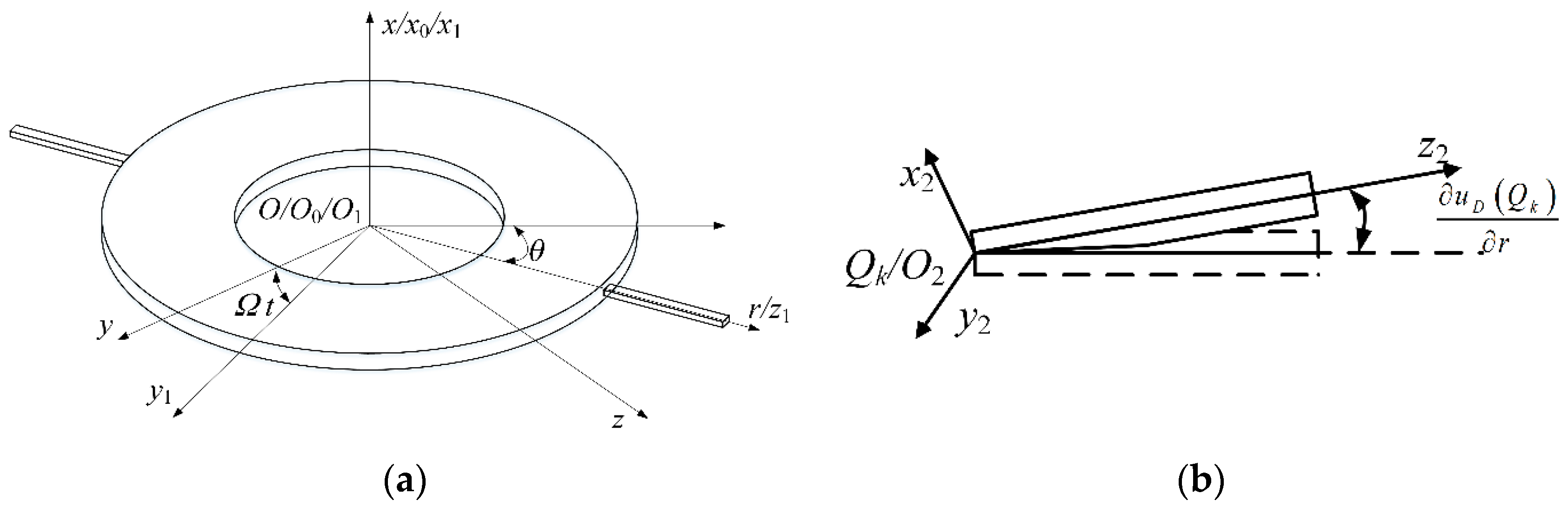
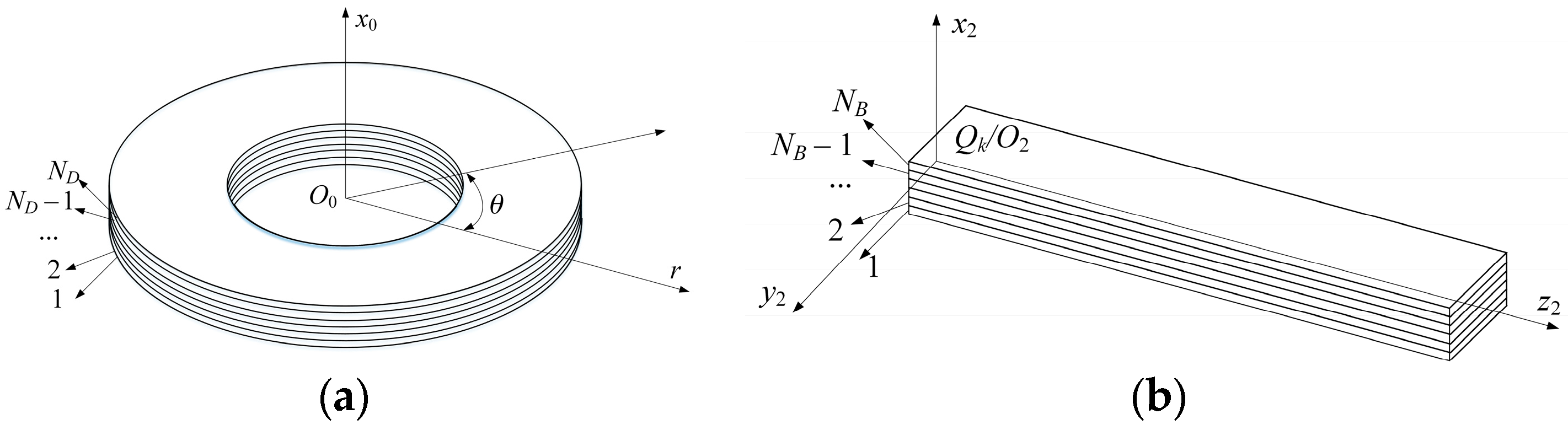
2.1. Modeling
2.2. Material Properties
2.3. Energy Functions
2.4. Equations of Motion
3. Results and Discussion
3.1. Convergence and Comparison Study
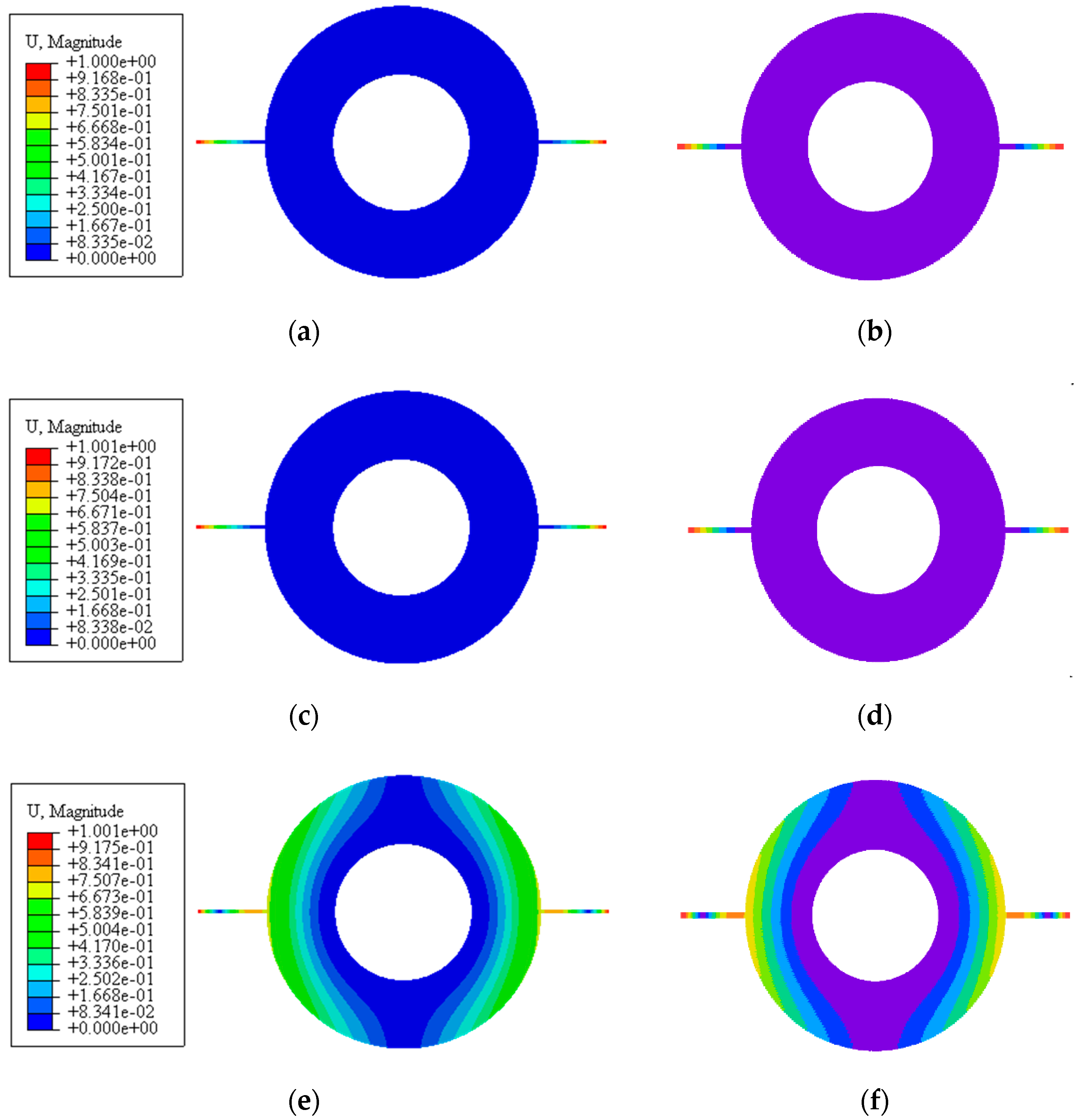
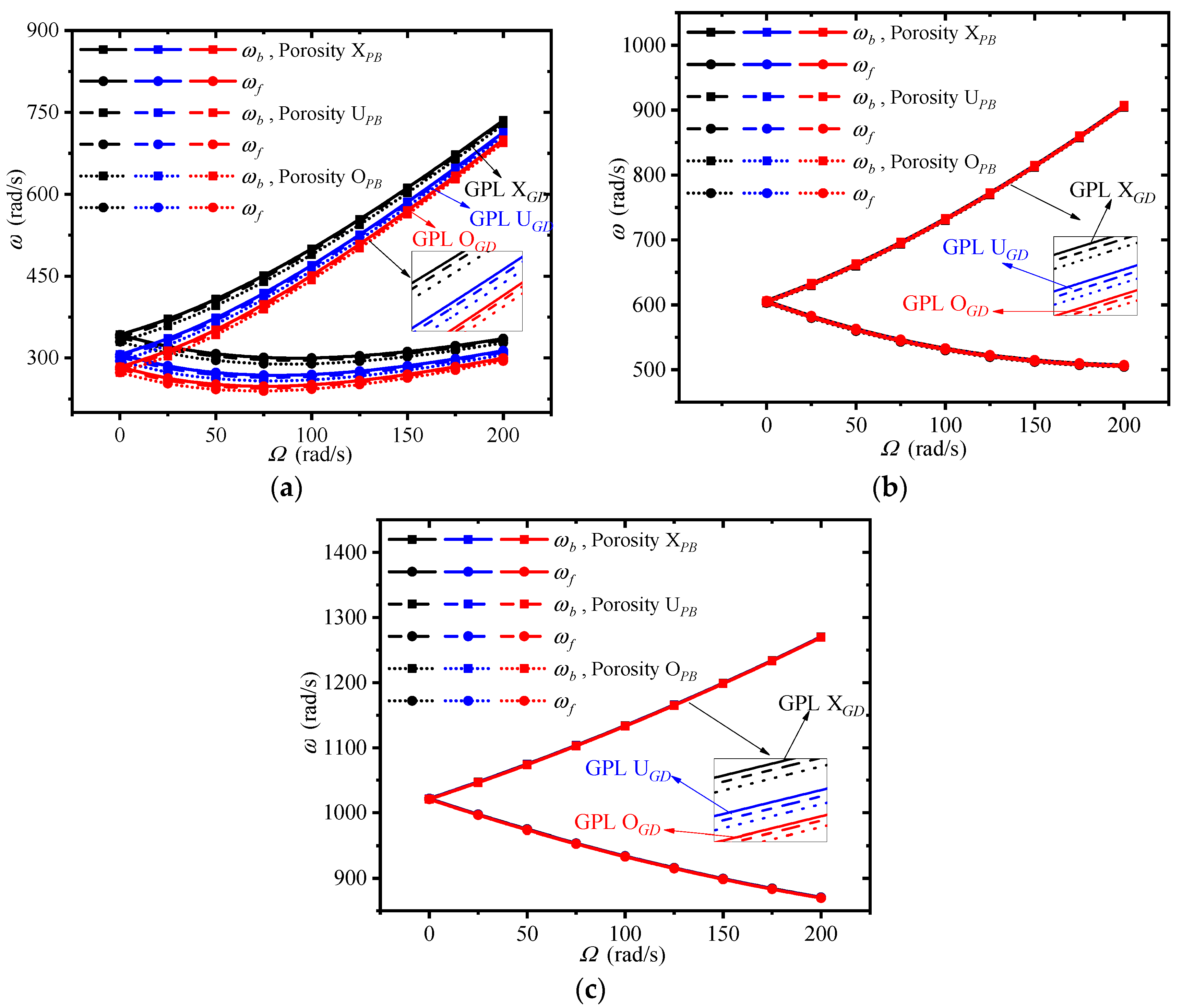
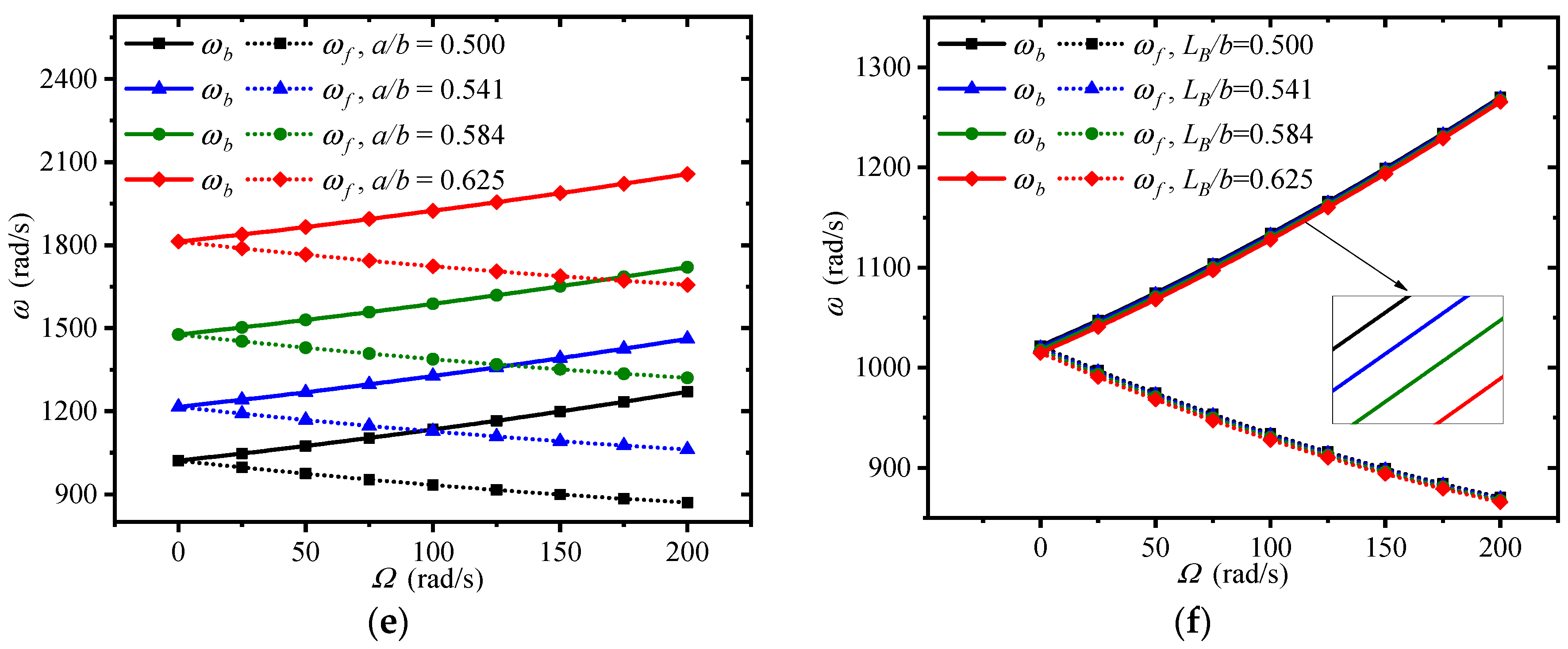
3.2. Free Vibration Analysis
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
References
- Chen, Y.G.; Zhai, J.Y.; Han, Q.K. Vibration and damping analysis of the bladed disk with damping hard coating on blades. Aerosp. Sci. Technol. 2016, 58, 248–257. [Google Scholar] [CrossRef]
- Zhao, T.Y.; Yuan, H.Q.; Li, B.B.; Li, Z.J.; Liu, L.M. Analytical solution for rotational rub-impact plate under thermal shock. J. Mech. 2016, 32, 297–311. [Google Scholar] [CrossRef]
- AI-Khudairi, O.; Hadavinia, H.; Little, C.; Gillmore, G.; Greaves, P.; Dyer, K. Full-scale fatigue testing of a wind turbine blade in flapwise direction and examining the effect of crack propagation on the blade performance. Materials 2017, 10, 1152. [Google Scholar] [CrossRef] [PubMed]
- Wieland, B.; Ropte, S. Process modeling of composite materials for wind-turbine rotor blades: Experiments and numerical modeling. Materials 2017, 10, 1157. [Google Scholar] [CrossRef]
- Zhao, T.Y.; Yuan, H.Q.; Yang, W.J.; Sun, H.G. Genetic particle swarm parallel algorithm analysis of optimization arrangement on mistuned blades. Eng. Optim. 2017, 49, 2095–2116. [Google Scholar] [CrossRef]
- Zhao, T.Y.; Li, H.X.; Sun, H.G. Parallel intelligent algorithm analysis of optimization arrangement on mistuned blades based on compute unified device architecture. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2019, 233, 2207–2218. [Google Scholar] [CrossRef]
- Ma, H.; Wang, D.; Tai, X.Y.; Wen, B.C. Vibration response analysis of blade-disk dovetail structure under blade tip rubbing condition. J. Vib. Control 2017, 23, 252–271. [Google Scholar] [CrossRef]
- Battiato, G.; Firrone, C.M.; Berruti, T.M. Forced response of rotating bladed disks: Blade tip-timing measurements. Mech. Syst. Signal Process. 2017, 85, 912–926. [Google Scholar] [CrossRef]
- Bai, B.; Li, H.; Zhang, W.; Cui, Y.C. Application of extremum response surface method-based improved substructure component modal synthesis in mistuned turbine bladed disk. J. Sound Vib. 2020, 472, 115210. [Google Scholar] [CrossRef]
- Rafiee, M.A.; Rafiee, J.; Wang, Z.; Song, H.H.; Yu, Z.Z.; Koratkar, N. Enhanced mechanical properties of nanocomposites at low graphene content. ACS Nano 2009, 3, 3884–3890. [Google Scholar] [CrossRef]
- Liu, D.Y.; Kitipornchai, S.; Chen, W.Q.; Yang, J. Three-dimensional buckling and free vibration analyses of initially stressed functionally graded graphene reinforced composite cylindrical shell. Compos. Struct. 2018, 189, 560–569. [Google Scholar] [CrossRef]
- Reddy, R.M.R.; Karunasena, W.; Lokuge, W. Free vibration of functionally graded-GPL reinforced composite plates with different boundary conditions. Aerosp. Sci. Technol. 2018, 78, 147–156. [Google Scholar] [CrossRef]
- Zhao, T.Y.; Wang, Y.X.; Pan, H.G.; Gao, X.S.; Cai, Y. Analytical solution for vibration characteristics of rotating graphene nanoplatelet-reinforced plates under rub-impact and thermal shock. Adv. Compos. Lett. 2020, 29. [Google Scholar] [CrossRef]
- Amirabadi, H.; Farhatnia, F.; Eftekhari, S.A.; Hosseini-Ara, R. Free vibration analysis of rotating functionally graded GPL-reinforced truncated thick conical shells under different boundary conditions. Mech. Based Des. Struct. Mach. 2020. [Google Scholar] [CrossRef]
- Feng, C.; Kitipornchai, S.; Yang, J. Nonlinear free vibration of functionally graded polymer composite beams reinforced with graphene nanoplatelets (GPLs). Eng. Struct. 2017, 140, 110–119. [Google Scholar] [CrossRef]
- Tam, M.F.; Yang, T.Z.; Zhao, S.Y.; Yang, J. Vibration and buckling characteristics of functionally graded graphene nanoplatelets reinforced composite beams with open edge cracks. Materials 2019, 12, 1412. [Google Scholar] [CrossRef]
- Zhao, Z.; Feng, C.; Wang, Y.; Yang, J. Bending and vibration analysis of functionally graded trapezoidal nanocomposite plates reinforced with graphene nanoplatelets (GPLs). Compos. Struct. 2017, 180, 799–808. [Google Scholar] [CrossRef]
- Guo, H.L.; Cao, S.Q.; Yang, T.Z.; Chen, Y.S. Vibration of laminated composite quadrilateral plates reinforced with graphene nanoplatelets using the element-free IMLS-Ritz method. Int. J. Mech. Sci. 2018, 142, 610–621. [Google Scholar] [CrossRef]
- Wu, H.L.; Zhu, J.; Kitipornchai, S.; Wang, Q.; Ke, L.L.; Yang, J. Large amplitude vibration of functionally graded graphene nanocomposite annular plates in thermal environments. Compos. Struct. 2020, 239, 112047. [Google Scholar] [CrossRef]
- Song, M.T.; Gong, Y.H.; Yang, J.; Zhu, W.D.; Kitipornchai, S. Nonlinear free vibration of cracked functionally graded graphene platelet-reinforced nanocomposite beams in thermal environments. J. Sound Vib. 2020, 468, 115115. [Google Scholar] [CrossRef]
- Yang, Z.C.; Zhao, S.Y.; Yang, J.; Lv, J.G.; Liu, A.R.; Fu, J.Y. In-plane and out-of-plane free vibrations of functionally graded composite arches with graphene reinforcements. Mech. Adv. Mater. Struct. 2020. [Google Scholar] [CrossRef]
- Wattanasakulpong, N.; Ungbhakorn, V. Linear and nonlinear vibration analysis of elastically restrained ends FGM beams with porosities. Aerosp. Sci. Technol. 2014, 32, 111–120. [Google Scholar] [CrossRef]
- Wang, Y.Q.; Zu, J.W. Large-amplitude vibration of sigmoid functionally graded thin plates with porosities. Thin Walled Struct. 2017, 119, 911–924. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Ghasemi, F.; Salari, E. Investigating thermal effects on vibration behavior of temperature-dependent compositionally graded Euler beams with porosities. Meccanica 2016, 51, 223–249. [Google Scholar] [CrossRef]
- Wang, Y.W.; Wu, D.F. Free vibration of functionally graded porous cylindrical shell using a sinusoidal shear deformation theory. Aerosp. Sci. Technol. 2017, 66, 83–91. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Zia, M. Large amplitude nonlinear vibration analysis of functionally graded Timoshenko beams with porosities. Acta Astronaut. 2015, 116, 117–125. [Google Scholar] [CrossRef]
- Wang, Y.Q.; Zu, J.W. Vibration behaviors of functionally graded rectangular plates with porosities and moving in thermal environment. Aerosp. Sci. Technol. 2017, 69, 550–562. [Google Scholar] [CrossRef]
- Kim, J.; Zur, K.K.; Reddy, J.N. Bending, free vibration, and buckling of modified couples stress-based functionally graded porous micro-plates. Compos. Struct. 2019, 209, 879–888. [Google Scholar] [CrossRef]
- Chen, D.; Yang, J.; Kitipornchai, S. Nonlinear vibration and postbuckling of functionally graded graphene reinforced porous nanocomposite beams. Compos. Sci. Technol. 2017, 142, 235–245. [Google Scholar] [CrossRef]
- Dong, Y.H.; Li, Y.H.; Chen, D.; Yang, J. Vibration characteristics of functionally graded graphene reinforced porous nanocomposite cylindrical shells with spinning motion. Compos. Part B Eng. 2018, 145, 1–13. [Google Scholar] [CrossRef]
- Kitipornchai, S.; Chen, D.; Yang, J. Free vibration and elastic buckling of functionally graded porous beams reinforced by graphene platelets. Mater. Des. 2017, 116, 656–665. [Google Scholar] [CrossRef]
- Zhao, S.Y.; Zhao, Z.; Yang, Z.C.; Ke, L.L.; Kitipornchai, S.; Yang, J. Functionally graded graphene reinforced composite structures: A review. Eng. Struct. 2020, 210, 110339. [Google Scholar] [CrossRef]




| e0 | e1 | e2 |
|---|---|---|
| 0.1 | 0.1738 | 0.9361 |
| 0.2 | 0.3442 | 0.8716 |
| 0.3 | 0.5103 | 0.8064 |
| 0.4 | 0.6708 | 0.7404 |
| Frequency | M = 4 N1 = N2 = 14 P1 = P2 = 11 | M = 5 N1 = N2 = 16 P1 = P2 = 13 | M = 5 N1 = N2 = 16 P1 = P2 = 15 | M = 5 N1 = N2 = 18 P1 = P2 = 13 | M = 6 N1 = N2 = 16 P1 = P2 = 13 |
|---|---|---|---|---|---|
| First | 342.50824 | 342.41852 | 342.41852 | 342.41842 | 342.41559 |
| Second | 606.54032 | 606.39077 | 606.39076 | 606.39077 | 606.39077 |
| Third | 1021.84498 | 1021.61920 | 1021.61920 | 1021.61920 | 1021.61826 |
| Fourth | 2149.98662 | 2149.78629 | 2149.78629 | 2149.78492 | 2149.78629 |
| Fifth | 3801.04613 | 3800.18608 | 3800.18590 | 3800.18608 | 3800.18608 |
| Frequency | ND = NB = 4 | ND = NB = 8 | ND = NB = 16 | ND = NB = 100 |
|---|---|---|---|---|
| First | 333.419 | 340.665 | 342.418 | 342.982 |
| Second | 605.834 | 606.284 | 606.390 | 606.424 |
| Third | 995.799 | 1016.59 | 1021.61 | 1023.23 |
| Fourth | 2093.27 | 2138.77 | 2149.78 | 2153.33 |
| Fifth | 3796.69 | 3799.51 | 3800.18 | 3800.40 |
| Frequency | Ω (rad/s) | Present | Finite Element | Error |
|---|---|---|---|---|
| First | 0 | 301.7845 | 296.1836 | 1.84% |
| 100 | 604.6508 | 594.0126 | 1.74% | |
| 200 | 904.2086 | 900.3008 | 0.38% | |
| Second | 0 | 365.8797 | 167.3024 | 1.51% |
| 100 | 432.1137 | 425.2251 | 1.62% | |
| 200 | 918.1062 | 914.1168 | 0.39% | |
| Third | 0 | 509.8145 | 503.8130 | 1.14% |
| 100 | 705.8883 | 695.0076 | 1.51% | |
| 200 | 959.1757 | 958.6897 | 0.44% |
| Frequency | Ω | WGPLD = p WGPLB = q | f = 0% | f = 0.33% | f = 0.67% | f = 1% |
|---|---|---|---|---|---|---|
| First | 0 | p = 0, q = f | 240.683 | 278.0561 | 311.9853 | 341.7586 |
| p = f, q = 0 | 240.683 | 240.7882 | 240.8503 | 240.8894 | ||
| p = q = f | 240.683 | 278.2255 | 312.3795 | 342.4185 | ||
| 100 | p = 0, q = f | 417.1995 | 446.2748 | 473.9031 | 498.9130 | |
| p = f, q = 0 | 417.1995 | 417.4847 | 417.6507 | 417.7542 | ||
| p = q = f | 417.1995 | 446.6563 | 474.6823 | 500.1056 | ||
| Second | 0 | p = 0, q = f | 475.4040 | 522.0961 | 566.3625 | 606.3908 |
| p = f, q = 0 | 475.4040 | 475.4040 | 475.4040 | 475.4040 | ||
| p = q = f | 475.4040 | 522.0961 | 566.3625 | 606.3908 | ||
| 100 | p = 0, q = f | 609.1708 | 653.0075 | 694.9589 | 733.1512 | |
| p = f, q = 0 | 609.1708 | 609.1910 | 609.2009 | 609.2066 | ||
| p = q = f | 609.1708 | 653.0347 | 695.0128 | 733.2381 | ||
| Third | 0 | p = 0, q = f | 724.8524 | 725.4668 | 726.0604 | 726.6336 |
| p = f, q = 0 | 724.8524 | 834.5594 | 933.3375 | 1019.349 | ||
| p = q = f | 724.8524 | 835.2214 | 934.7485 | 1021.619 | ||
| 100 | p = 0, q = f | 842.1417 | 842.7876 | 843.4427 | 844.0990 | |
| p = f, q = 0 | 842.1417 | 949.6112 | 1046.8747 | 1131.864 | ||
| p = q = f | 842.1417 | 950.2492 | 1048.1906 | 1133.928 |
| Frequency | Ω | lD/tD = p lB/tB = q | f = 10 | f = 40 | f = 70 | f = 100 |
|---|---|---|---|---|---|---|
| First | 0 | p = 10, q = f | 320.7607 | 337.5028 | 340.8724 | 342.3215 |
| p = f, q = 10 | 320.7607 | 320.8224 | 320.8336 | 320.8383 | ||
| p = q = f | 320.7607 | 337.5763 | 340.9623 | 342.4185 | ||
| 100 | p = 10, q = f | 481.7063 | 495.8319 | 498.6986 | 499.9337 | |
| p = f, q = 10 | 481.7063 | 481.8224 | 481.8435 | 481.8523 | ||
| p = q = f | 481.7063 | 495.9637 | 498.8583 | 500.1056 | ||
| Second | 0 | p = 10, q = f | 578.3558 | 600.044 | 604.4773 | 606.3908 |
| p = f, q = 10 | 578.3558 | 578.3558 | 578.3558 | 578.3558 | ||
| p = q = f | 578.3558 | 600.044 | 604.4773 | 606.3908 | ||
| 100 | p = 10, q = f | 706.4411 | 727.1565 | 731.3988 | 733.2307 | |
| p = f, q = 10 | 706.4411 | 706.4463 | 706.4472 | 706.4476 | ||
| p = q = f | 706.4411 | 727.1622 | 731.4057 | 733.2381 | ||
| Third | 0 | p = 10, q = f | 957.0838 | 957.3238 | 957.3725 | 957.3935 |
| p = f, q = 10 | 957.0838 | 1006.949 | 1016.991 | 1021.310 | ||
| p = q = f | 957.0838 | 1007.189 | 1017.28 | 1021.619 | ||
| 100 | p = 10, q = f | 1070.217 | 1070.468 | 1070.519 | 1070.541 | |
| p = f, q = 10 | 1070.217 | 1119.428 | 1129.346 | 1133.613 | ||
| p = q = f | 1070.217 | 1119.673 | 1129.640 | 1133.928 |
| Frequency | Ω | lD/wD = p lB/wB = q | f = 2 | f = 4 | f = 6 | f = 8 |
|---|---|---|---|---|---|---|
| First | 0 | p = 1, q = f | 342.4185 | 339.9449 | 337.7323 | 335.7413 |
| p = f, q = 1 | 342.4185 | 342.4085 | 342.3992 | 342.3908 | ||
| p = q = f | 342.4185 | 339.9351 | 337.7139 | 335.7154 | ||
| 100 | p = 1, q = f | 500.1056 | 497.9969 | 496.1141 | 494.4228 | |
| p = f, q = 1 | 500.1056 | 500.0878 | 500.0715 | 500.0565 | ||
| p = q = f | 500.1056 | 497.9794 | 496.0812 | 494.3762 | ||
| Second | 0 | p = 1, q = f | 606.3908 | 603.1327 | 600.2314 | 597.6311 |
| p = f, q = 1 | 606.3908 | 606.3908 | 606.3908 | 606.3908 | ||
| p = q = f | 606.3908 | 603.1327 | 600.2314 | 597.6311 | ||
| 100 | p = 1, q = f | 733.2381 | 730.1191 | 727.3429 | 724.8556 | |
| p = f, q = 1 | 733.2381 | 733.2374 | 733.2367 | 733.2361 | ||
| p = q = f | 733.2381 | 730.1184 | 727.3415 | 724.8537 | ||
| Third | 0 | p = 1, q = f | 1021.619 | 1021.584 | 1021.552 | 1021.524 |
| p = f, q = 1 | 1021.619 | 1014.254 | 1007.666 | 1001.74 | ||
| p = q = f | 1021.619 | 1014.219 | 1007.6 | 1001.644 | ||
| 100 | p = 1, q = f | 1133.928 | 1133.891 | 1133.859 | 1133.830 | |
| p = f, q = 1 | 1133.928 | 1126.653 | 1120.147 | 1114.295 | ||
| p = q = f | 1133.928 | 1126.616 | 1120.078 | 1114.197 |
| Frequency | Ω | lD/wD = p lB/wB = q | f = 0 | f = 0.1 | f = 0.2 | f = 0.3 |
|---|---|---|---|---|---|---|
| First | 0 | p = 0, q = f | 343.4377 | 342.4425 | 341.5784 | 340.8869 |
| p = f, q = 0 | 343.4377 | 343.4126 | 343.3854 | 343.3555 | ||
| p = q = f | 343.4377 | 342.4185 | 341.5304 | 340.8149 | ||
| 100 | p = 0, q = f | 500.9792 | 500.1474 | 499.4285 | 498.8582 | |
| p = f, q = 0 | 500.9792 | 500.9357 | 500.8884 | 500.8366 | ||
| p = q = f | 500.9792 | 500.1056 | 499.3448 | 498.7324 | ||
| Second | 0 | p = 0, q = f | 615.0141 | 606.3908 | 597.4517 | 588.1804 |
| p = f, q = 0 | 615.0141 | 615.0141 | 615.0141 | 615.0141 | ||
| p = q = f | 615.0141 | 606.3908 | 597.4517 | 588.1804 | ||
| 100 | p = 0, q = f | 741.5002 | 733.2395 | 724.6866 | 715.8272 | |
| p = f, q = 0 | 741.5002 | 741.4987 | 741.4970 | 741.4952 | ||
| p = q = f | 741.5002 | 733.2381 | 724.6838 | 715.8232 | ||
| Third | 0 | p = 0, q = f | 1024.607 | 1024.751 | 1024.905 | 1025.070 |
| p = f, q = 0 | 1024.607 | 1021.471 | 1018.718 | 1016.468 | ||
| p = q = f | 1024.607 | 1021.619 | 1019.033 | 1016.975 | ||
| 100 | p = 0, q = f | 1136.880 | 1136.997 | 1137.122 | 1137.257 | |
| p = f, q = 0 | 1136.880 | 1133.809 | 1131.118 | 1128.928 | ||
| p = q = f | 1136.880 | 1133.928 | 1131.372 | 1129.338 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, T.; Ma, Y.; Zhang, H.; Yang, J. Coupled Free Vibration of Spinning Functionally Graded Porous Double-Bladed Disk Systems Reinforced with Graphene Nanoplatelets. Materials 2020, 13, 5610. https://doi.org/10.3390/ma13245610
Zhao T, Ma Y, Zhang H, Yang J. Coupled Free Vibration of Spinning Functionally Graded Porous Double-Bladed Disk Systems Reinforced with Graphene Nanoplatelets. Materials. 2020; 13(24):5610. https://doi.org/10.3390/ma13245610
Chicago/Turabian StyleZhao, Tianyu, Yu Ma, Hongyuan Zhang, and Jie Yang. 2020. "Coupled Free Vibration of Spinning Functionally Graded Porous Double-Bladed Disk Systems Reinforced with Graphene Nanoplatelets" Materials 13, no. 24: 5610. https://doi.org/10.3390/ma13245610
APA StyleZhao, T., Ma, Y., Zhang, H., & Yang, J. (2020). Coupled Free Vibration of Spinning Functionally Graded Porous Double-Bladed Disk Systems Reinforced with Graphene Nanoplatelets. Materials, 13(24), 5610. https://doi.org/10.3390/ma13245610






