Changes in the Fracture Toughness under Mode II Loading of Low Calcium Fly Ash (LCFA) Concrete Depending on Ages
Abstract
1. Introduction
- energy intensive,
- very expensive,
- a process that produces harmful greenhouse gases (GHG), such as: CO2, NO, NO2,
- an activity that degrades natural mineral deposits, such as limestone.
- binder production costs,
- energy consumption,
- CO2 emissions.
- corrosion resistance, e.g., [44],
- drying shrinkage, e.g., [51],
- porosity and pore volume distribution, e.g., [46],
- heat development, e.g., [46],
- water absorption and water permeability, e.g., [48],
- hydration process, e.g., [52],
- crack propagation, e.g., [45],
- fracture toughness evaluated in mortar tests with LCFA, e.g., [54].
2. The Importance of Research
- support zones of RC beams,
- short cantilevers,
- undercut beams,
- beams loaded with point loads,
- corners of the rigid frame bridges,
- deep beams, and
- connections in precast segments.
- analysis of influence of the curing time on the values of critical stress intensity factor KIIc,
- evaluation of brittleness of concretes depending on the adopted material modification, and
- diagnosis of the basic pattern of crack development in specimens subjected to shear.
3. Experimental Section
3.1. Materials
- without the LCFA addition (LCFA-00),
- with 20% LCFA addition (LCFA-20),
- with 30% LCFA addition (LCFA-30).
3.2. Methods
- compressive strength (fcm) and
- fracture toughness at shear (KIIc).
- its dimensions,
- loading conditions,
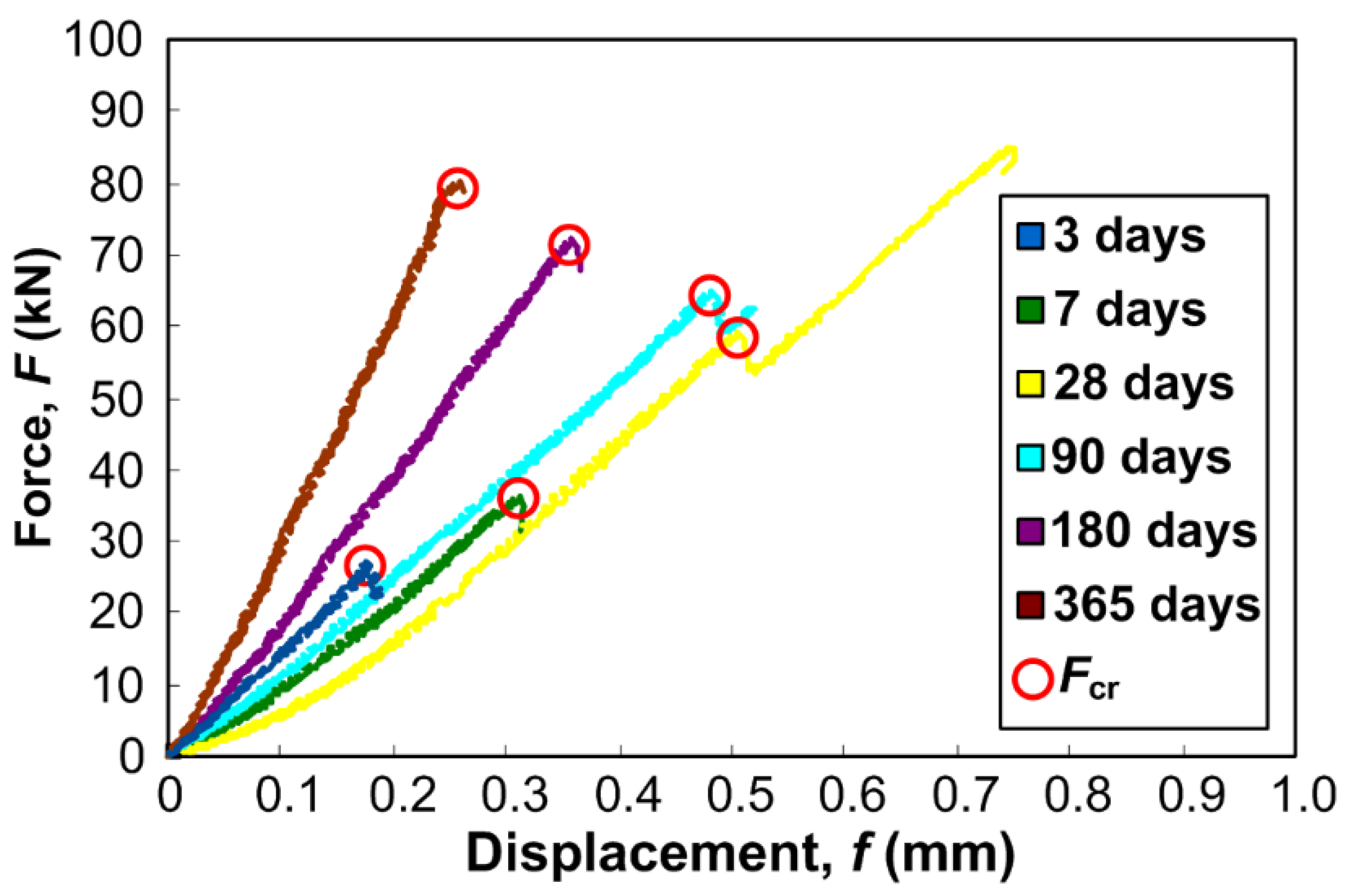
- designation of force Fcr causing the development of initial cracks, and
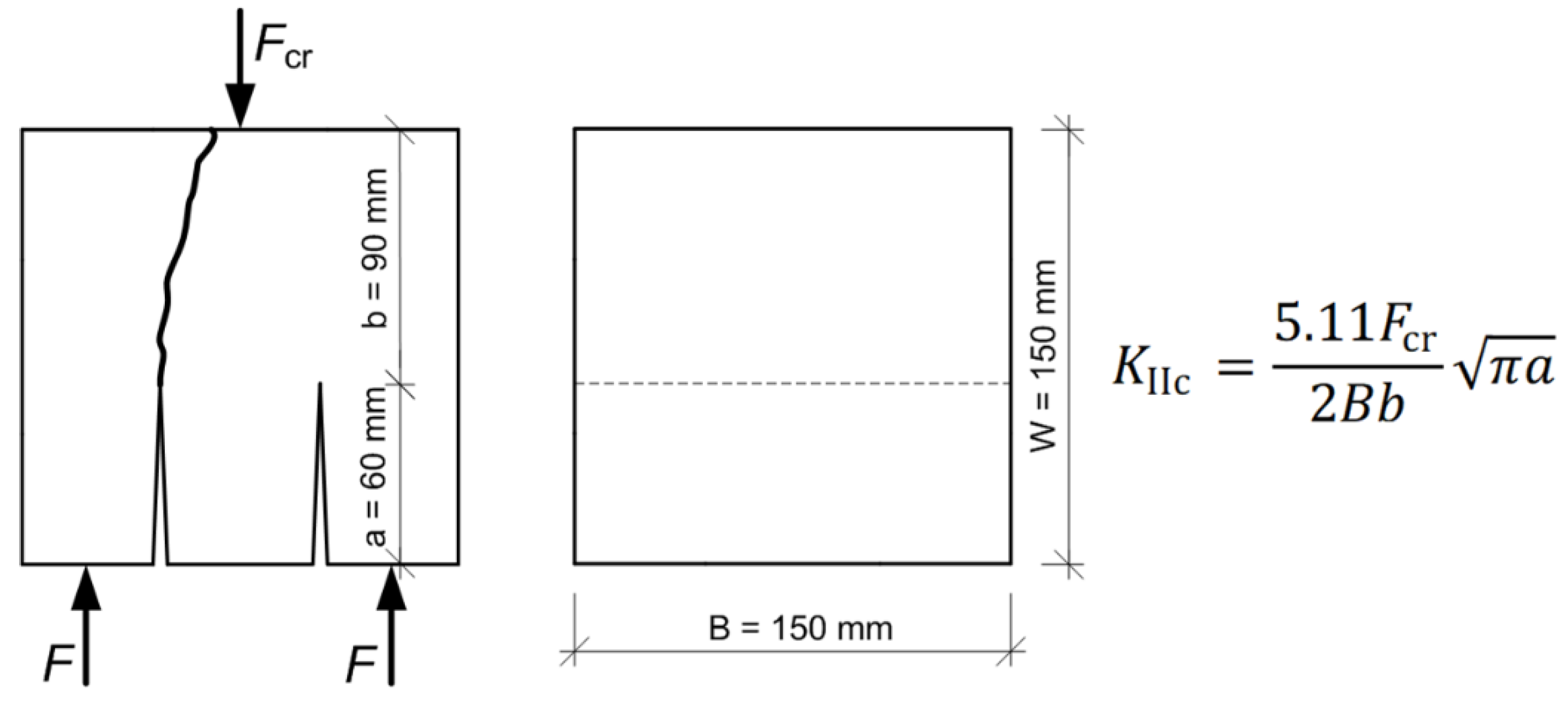
- equation for determining KIIc parameter, according to Watkins [112].
- ABAQUS program, created by Dassault Systèmes Simulia Corp. from Providence, Rhode Island, USA supported by Extended Finite Element Method (XFEM) during numerical simulations,
- peak principal stress criterion for description of the crack grow.
- a displacement controlled type of tests with the MTS head velocity equal to 0.25 mm/min,
- quasi-static increase of the loading force (F) up to the final failure of the specimens.
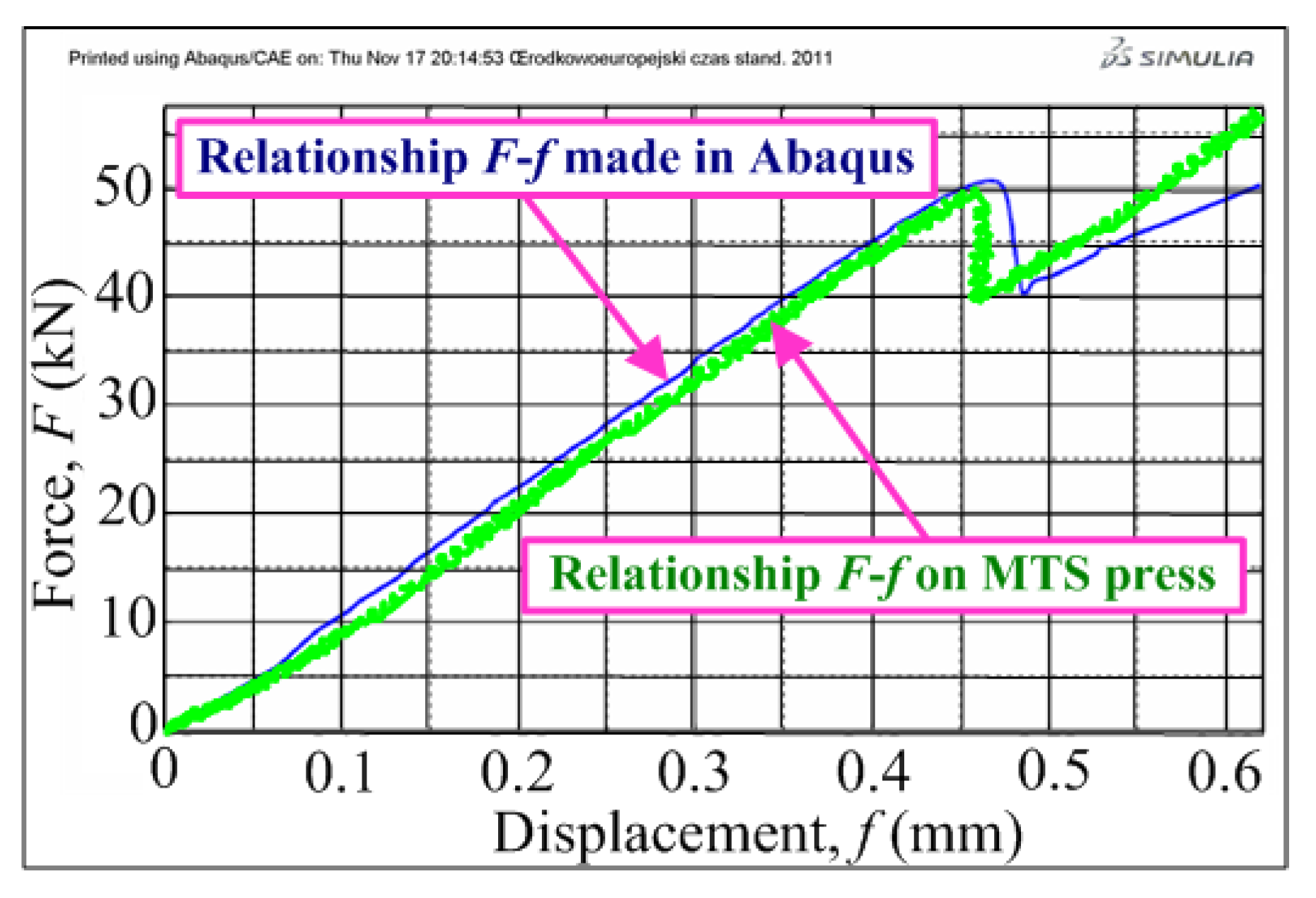
- load (F)–displacement (f) relationship and
- fracture toughness KIIc parameter.
4. Results and Discussions
4.1. Analysis of Changes in Concretes Mechanical Parameters Depending on Ages
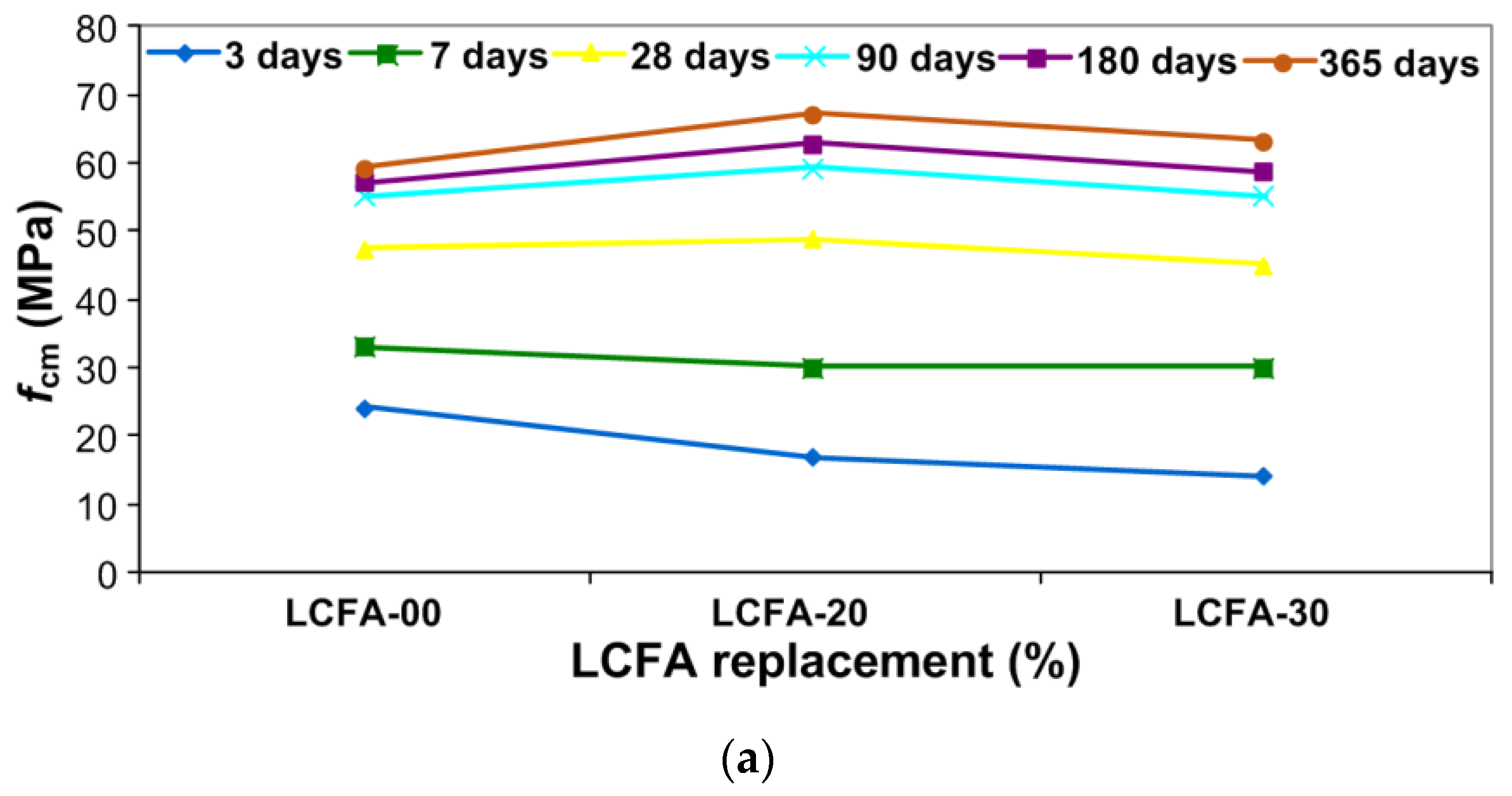
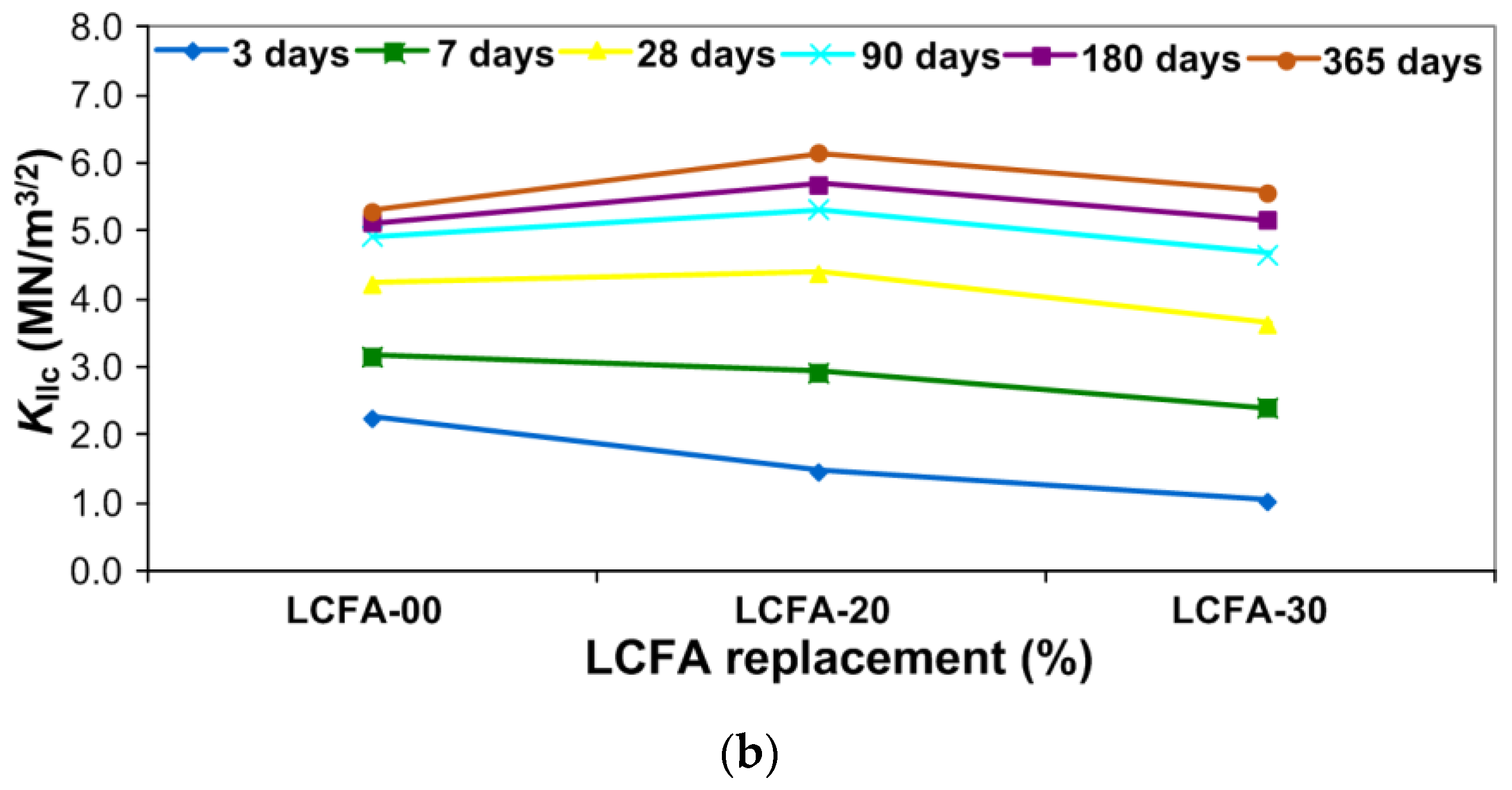
- the applied LCFA additive reduces the early fracture toughness of cement concrete, as well as compressive strength of composite, i.e., in the first week of curing;
- 1 month old concretes with addition of 20% LCFA are characterized by higher KIIc and fcm parameters than the reference concrete sample;
- during the standard period, i.e., after 28 days and longer periods of curing LCFA-20 had the highest strength and fracture toughness, which was influenced by the intensification of the pozzolanic reaction after a longer time of curing (Figure 1);
- the LCFA-30 composite only after 3 months achieved parameters similar to those obtained for LCFA-00 and LCFA-20 concretes samples;
- concrete with more LCFA will achieve higher KIIc and fcm values after half a year compared to the reference concrete, while they were still lower than the results obtained for LCFA-20 (even after one year); and
- the greatest increases in KIIc and fcm were observed: in the case of reference concrete during the first 28 days, in the case of concretes containing LCFA in the time period above 4 weeks.
- the greatest dispersion of results, represented by the highest values of ν, was observed in the concrete with the shortest curing time (Table 6).
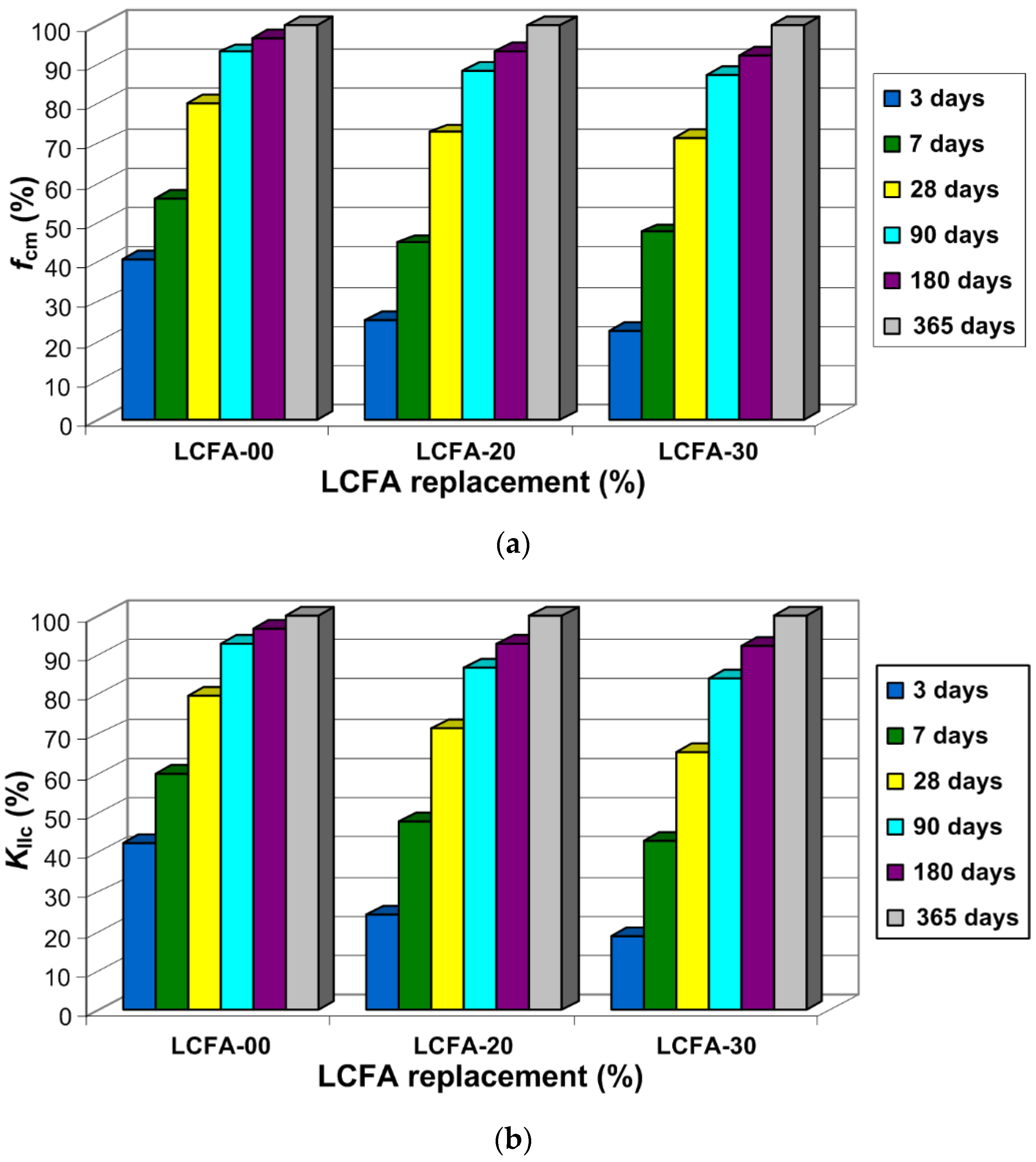
- significantly higher values of both analyzed mechanical parameters in the reference concrete samples compared to concrete samples modified by LCFA in the first of the analyzed curing periods., i.e., after 3 days; during this period, reference concrete had more than 40% of the annual compressive strength, whereas, in concretes with LCFA additives, this strength did not even reach 30% of the final strength (Figure 9a), whereas fracture toughness was lower by 35% and 54% for LCFA-20 and LCFA-30, respectively, in comparison to the value obtained for LCFA-00 (Figure 9b);
- a clearer dynamics of strength and fracture toughness increase in composites with LCFA after a week; nonetheless, during this period, concrete without the LCFA additive was still characterized by a higher relative strength and fracture toughness (Figure 9);
- sharp increase of fcm and KIIc for concretes with the LCFA additive between the 7th and 90th day of curing (Figure 9); during this time, the values of analyzed mechanical parameters for these materials increased more than 80% for LCFA-20 and above 90% for LCFA-30; and
- stable and small increase in fcm and KIIc in all series of concretes after 180 and 365 days of curing; during these periods, the most significant changes were observed in concrete with a greater amount of pozzolanic additive, i.e., LCFA-30 (Figure 9).
4.2. Assessment of Material Fracture Processes at Mode II Fracture
- quasi-straight crack, occurring mainly in the most brittle concrete, i.e., LCFA-00-00 (Figure 11a),
- slightly curved crack, which is related with the most common LCFA-20 concrete (Figure 11b), and
- clearly and strongly curved crack with small branches (visible in the final stage of crack propagation and marked with a red line) which was observed mainly in the LCFA-30 concrete (Figure 11c).
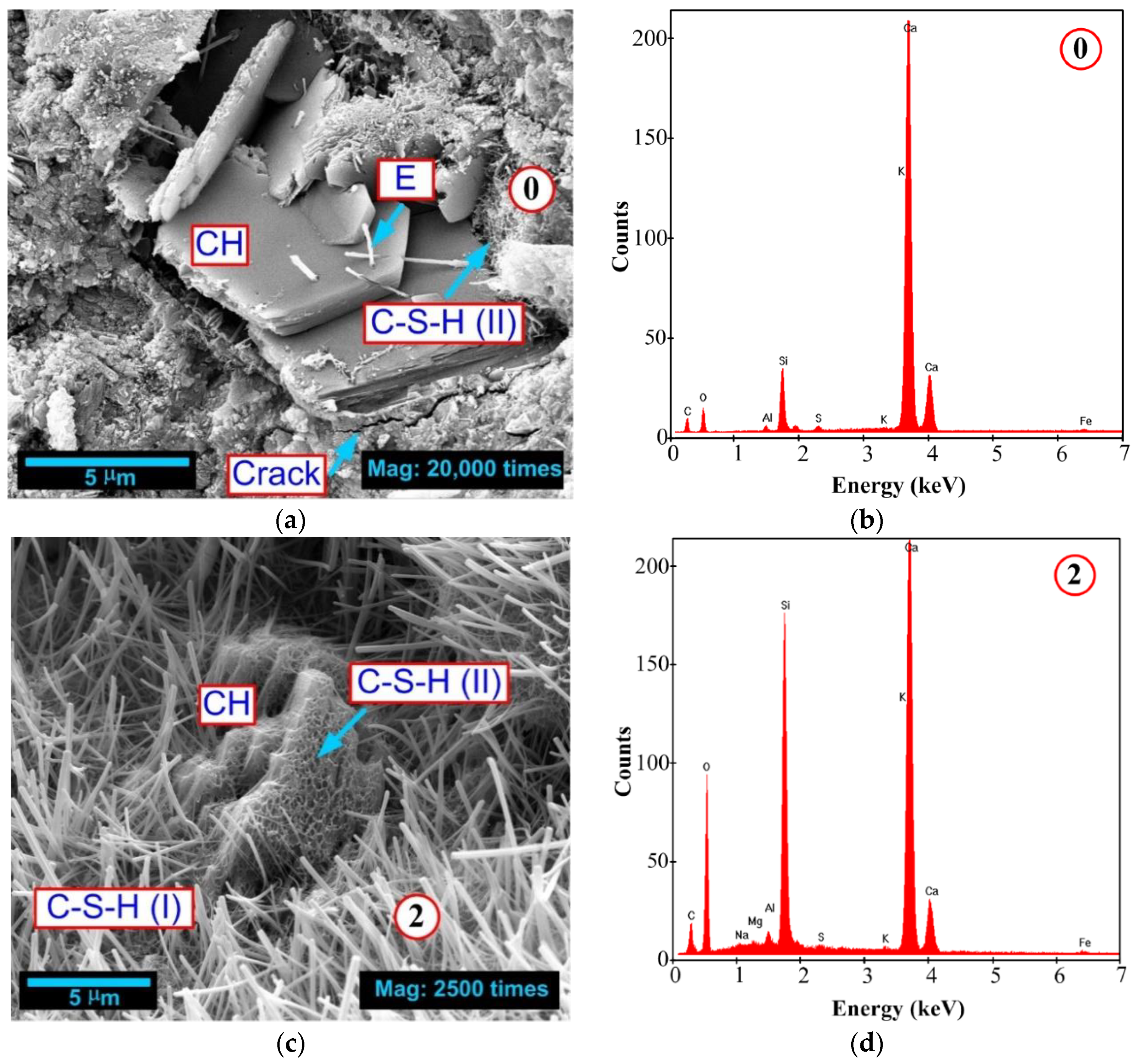
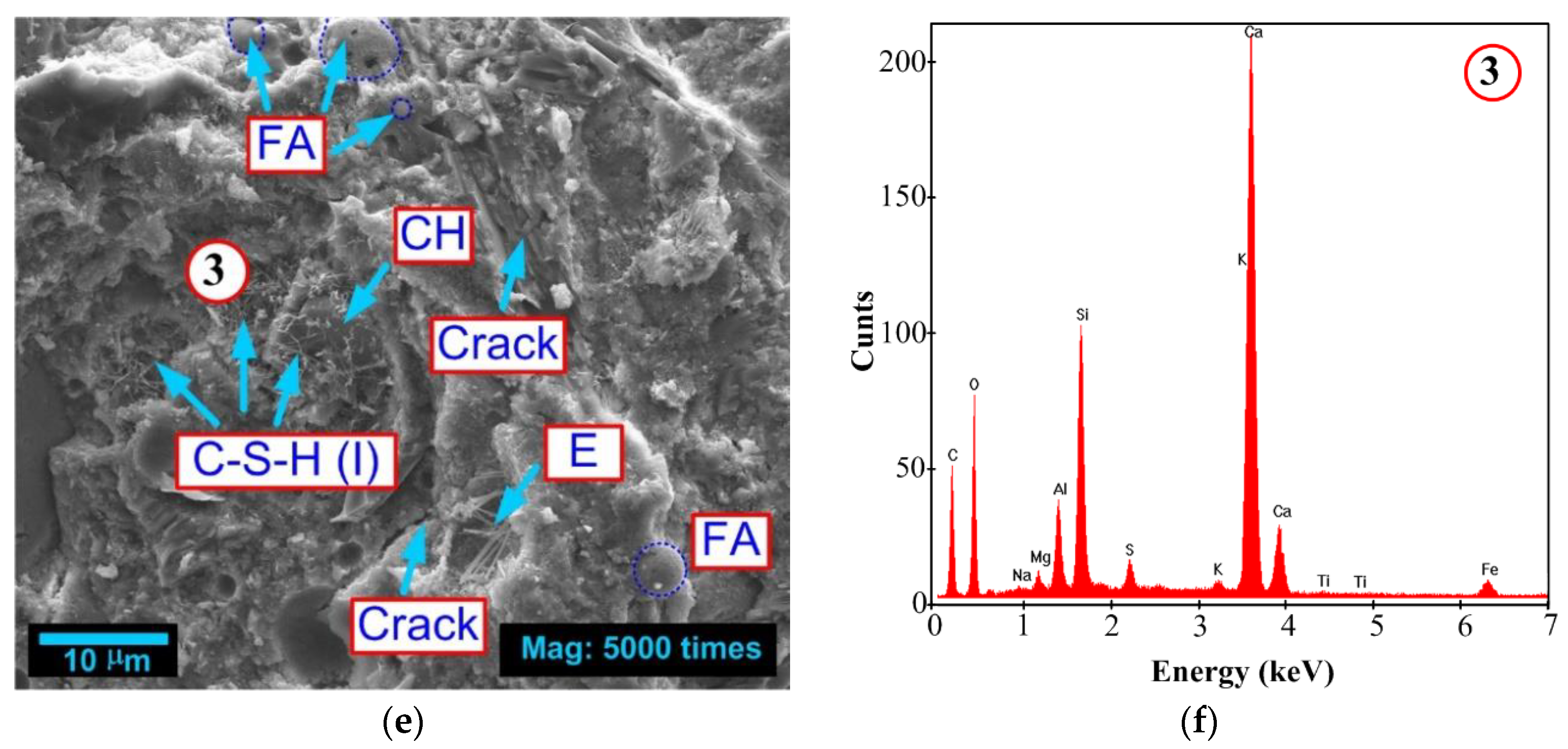
4.3. Evaluation of the Microstructure of Analyzed Concretes
- more compact material structure (Figure 14),
- higher compressive strength of concrete (Figure 8a), and
- higher macroscopic fracture toughness of the composite (Figure 8b).
5. Conclusions
- The LCFA as an active additive in the amount of 20% and 30% causes changes in the values of fracture toughness at shear and compressive strength of concrete composites.
- The analyzed parameters of KIIc and fcm depend on the curing time of individual concrete sample.
- At early age the maximum fracture toughness occurs in reference concrete. It is 2.26 MN/m3/2 at 3 days, and 3.18 MN/m3/2 at 7 days.
- Twenty percent LCFA additive ensures high fracture toughness in mature concrete. It is 4.39 MN/m3/2 at 28 days, 5.33 MN/m3/2 at 90 days, 5.70 MN/m3/2 at 180 days, and 6.14 MN/m3/2 at 365 days.
- Concrete with 30% LCFA additive are characterized by high fracture toughness only after 180 days of curing. It is 5.16 MN/m3/2 at 180 days, and 5.58 MN/m3/2 at 365 days.
- The microstructure of mature concrete specimens is characterized by: the content of the portlandite phase in the reference concrete with fractures at the phase boundaries and a high content of the C-S-H phase of types (I) and (II), as well as the visible transformation processes of the phase CH in the phase C-S-H in the concrete LCFA-20, weakly developed phases, and unreacted LCFA grains and microcracks in concrete with 30% LCFA additive (Figure 14).
- Twenty percent LCFA additive causes a strong pozzolanic reaction in the cement matrix, thanks to which the molar ratio in this material is reduced to the level of 1.2, while, in concrete with a higher content of the additive, it is 2.0, and in non-modified concrete at 7.0.
- The presented test results may be helpful in selecting the composition of concrete mixtures containing LCFA to be used in concrete and reinforced concrete structures subjected to shear loads.
Funding
Conflicts of Interest
References
- Malinowski, R.; Garfinkel, Y. Prehistory of Concrete. Concr. Inter. 1990, 13, 62–68. [Google Scholar]
- USGS. Mineral Commodity Summaries; U. S. Geological Survey: Reston, VA, USA, 2020. Available online: https://www.usgs.gov/centers/nmic/mineral-commodity-summaries (accessed on 18 February 2020).
- Golewski, G.L. Energy Savings Associated with the Use of Fly Ash and Nanoadditives in the Cement Composition. Energies 2020, 13, 2184. [Google Scholar] [CrossRef]
- Golewski, G.; Sadowski, T. The Fracture Toughness the KIIIc of Concretes with Fly Ash (FA) Additive. Constr. Build. Mater. 2017, 143, 444–454. [Google Scholar] [CrossRef]
- Ikponmwosa, E.E.; Ehikhuenmen, S.O.; Irene, K.K. Comparative Study and Empirical Modelling of Pulverized Coconut Shell, Periwinkle Shell and Palm Kernel Shell as a Pozzolans in Concrete. Acta Polytech. 2019, 59, 560–572. [Google Scholar] [CrossRef]
- Zhang, P.; Li, Q.; Chen, Y.; Shi, Y.; Ling, Y.-F. Durability of Steel Fiber-Reinforced Concrete Containing SiO2 Nano-Particles. Materials 2019, 12, 2184. [Google Scholar] [CrossRef]
- Góra, J.; Franus, M.; Barnat-Hunek, D.; Franus, W. Utilization of Recycled Liquid Crystal Display (LCD) Panel Waste in Concrete. Materials 2019, 12, 2941. [Google Scholar] [CrossRef]
- Szeląg, M. Evaluation of Cracking Patterns in Cement Composites—From Basics to Advances: A Review. Materials 2020, 13, 2490. [Google Scholar] [CrossRef]
- Raheel, M.; Rahman, F.; Ali, Q. A Stoichiometric Approach to Find Optimum Amount of Fly Ash Needed in Cement Concrete. SN Appl. Sci. 2020, 2, 1–9. [Google Scholar] [CrossRef]
- Biçer, A. Effect of Production Temperature on Thermal and Mechanical Properties of Polystyrene–Fly Ash Composites. Adv. Compos. Lett. 2020, 29, 1–8. [Google Scholar] [CrossRef]
- Xu, S.; Malik, M.A.; Qi, Z.; Huang, B.-T.; Li, Q.; Sarkar, M. Influence of the PVA Fibers and SiO2 NPs on the Structural Properties of Fly Ash Based Sustainable Geopolymer. Constr. Build. Mater. 2018, 164, 238–245. [Google Scholar] [CrossRef]
- Suchorab, Z.; Franus, M.; Barnat-Hunek, D. Properties of Fibrous Concrete Made with Plastic Optical Fibers from E-Waste. Materials 2020, 13, 2414. [Google Scholar] [CrossRef]
- Kovacik, J.; Marsavina, L.; Linul, E. Poisson’s Ratio of Closed-Cell Aluminum Foams. Materials 2018, 11, 1904. [Google Scholar] [CrossRef] [PubMed]
- Xie, T.; Yang, G.; Zhao, X.; Xu, J.; Fang, C. A Unified Model for Predicting the Compressive Strength of Recycled Aggregate Concrete Containing Supplementary Cementitious Materials. J. Clean. Prod. 2020, 251, 119752. [Google Scholar] [CrossRef]
- Szeląg, M. Application of an Automated Digital Image-Processing Method for Quantitative Assessment of Cracking Patterns in a Lime Cement Matrix. Sensors 2020, 20, 3859. [Google Scholar] [CrossRef] [PubMed]
- Seghir, N.T.; Mellas, M.; Sadowski, L.; Żak, A. Effects of Marble Powder on the Properties of the Air-Cured Blended Cement Paste. J. Clean. Prod. 2018, 183, 858–868. [Google Scholar] [CrossRef]
- Petrounias, P.; Giannakopoulou, P.P.; Rogkala, A.; Lampropoulou, P.; Tsikouras, B.; Rigopoulos, I.; Hatzipanagiotou, K. Petrographic and Mechanical Characteristics of Concrete Produced by Different Type of Recycled Materials. Geoscience 2019, 9, 264. [Google Scholar] [CrossRef]
- Zhang, P.; Han, S.; Golewski, G.L.; Wang, X. Nanoparticle-Reinforced Building Materials with Applications in Civil Engineering. Adv. Mech. Eng. 2020, 12, 1–4. [Google Scholar] [CrossRef]
- Golewski, G.L. Determination of Fracture Toughness in Concretes Containing Siliceous Fly Ash during Mode III Loading. Struct. Eng. Mech. 2017, 62, 1–9. [Google Scholar] [CrossRef]
- Zeyad, A.M.; Tayeh, B.A.; Yusuf, M.O. Strength and Transport Characteristics of Volcanic Pumice Powder Based High Strength Concrete. Constr. Build. Mater. 2019, 216, 314–324. [Google Scholar] [CrossRef]
- Giergiczny, Z. Fly Ash and Slag. Cem. Concr. Res. 2019, 124, 105826. [Google Scholar] [CrossRef]
- Szcześniak, A.; Zychowicz, J.; Stolarski, A. Influence of Fly Ash Additive on the Properties of Concrete with Slag Cement. Materials 2020, 13, 3265. [Google Scholar] [CrossRef] [PubMed]
- Zhang, P.; Gao, Z.; Wang, J.; Guo, J.; Hu, S.; Ling, Y. Properties of Fresh and Hardened Fly Ash/Slag Based Geopolymer Concrete: A Review. J. Clean. Prod. 2020, 270, 122389. [Google Scholar] [CrossRef]
- Golewski, G.L. Studies of Natural Radioactivity of Concrete with Siliceous Fly Ash Addition. Cem. Wapno Beton 2015, 2, 106–114. [Google Scholar]
- Zhang, R.; Guo, F.; Xia, Y.; Tan, J.; Xing, Y.; Gui, X. Recovering Unburned Carbon From Gasification Fly Ash Using Saline Water. Waste Manag. 2019, 98, 29–36. [Google Scholar] [CrossRef] [PubMed]
- Szostak, B.; Golewski, G.L. Effect of Nano Admixture of CSH on Selected Strength Parameters of Concrete Including Fly Ash. IOP Conf. Ser. Mater. Sci. Eng. 2018, 416, 012105. [Google Scholar] [CrossRef]
- Szymanowski, J.; Sadowski, L. The Development of Nanoalumina-Based Cement Mortars for Overlay Applications in Concrete Floors. Materials 2019, 12, 3465. [Google Scholar] [CrossRef]
- Carević, I.; Serdar, M.; Štirmer, N.; Ukrainczyk, N. Preliminary Screening of Wood Biomass Ashes for Partial Resources Replacements in Cementitious Materials. J. Clean. Prod. 2019, 229, 1045–1064. [Google Scholar] [CrossRef]
- Shaban, W.M.; Yang, J.; Kim, K.-H.; Liu, Q.-F.; Wang, H.; Wang, L.; Xie, J.; Li, L. Properties of Recycled Concrete Aggregates Strengthened by Different Types of Pozzolan Slurry. Constr. Build. Mater. 2019, 216, 632–647. [Google Scholar] [CrossRef]
- Jonbi, J.; Fulazzaky, M.A. Modeling the Water Absorption and Compressive Strength of Geopolymer Paving Block: An Empirical Approach. Measurement 2020, 158, 107695. [Google Scholar] [CrossRef]
- Chindaprasirt, P.; Rukzon, S. Strength, Porosity and Corrosion Resistance of Ternary Blend Portland Cement, Rice Husk Ash and Fly Ash Mortar. Constr. Build. Mater. 2008, 22, 1601–1606. [Google Scholar] [CrossRef]
- Li, Y.; Fu, T.; Wang, R.; Li, Y. Effect of Superabsorbent Polymer on Interfacial Transition Zone and Mechanical Properties of Ultra-High Performance Concrete. Constr. Build. Mater. 2020, 231, 117142. [Google Scholar] [CrossRef]
- Golewski, G.L. A Novel Specific Requirements for Materials Used in Reinforced Concrete Composites Subjected to Dynamic Loads. Compos. Struct. 2019, 223, 110939. [Google Scholar] [CrossRef]
- Golewski, G.L. A New Principles for Implementation and Operation of Foundations for Machines: A Review of Recent Advances. Struct. Eng. Mech. 2019, 71, 317–327. [Google Scholar]
- Zargar, M.; Gholami, H.; Soltani, M.; Ghane, M. Mechanical View of Corrosion Phenomenon in Concrete Quadrangular of Power Transmission Lines. Int. J. Mod. Stud. Mech. Eng. 2019, 5, 30–43. [Google Scholar]
- Nadeem, A.; Memon, S.A.; Lo, T.Y. The Performance of Fly Ash and Metakaolin Concrete at Elevated Temperatures. Constr. Build. Mater. 2014, 62, 67–76. [Google Scholar] [CrossRef]
- Stolarski, A.; Cichorski, W.; Szcześniak, A. Non-Classical Model of Dynamic Behaviour of Concrete. Appl. Sci. 2019, 9, 2590. [Google Scholar] [CrossRef]
- Szcześniak, A.; Stolarski, A. Dynamic Relaxation Method for Load Capacity Analysis of Reinforced Concrete Elements. Appl. Sci. 2018, 8, 396. [Google Scholar] [CrossRef]
- Wang, Y.-S.; Alrefaei, Y.; Dai, J.-G. Influence of Coal Fly Ash on the Early Performance Enhancement and Formation Mechanisms of Silico-Aluminophosphate Geopolymer. Cem. Concr. Res. 2020, 127, 105932. [Google Scholar] [CrossRef]
- Zhang, W.; Zhang, Y.; Gao, L. Effect of Low-Calcium Fly Ash on Sulfate Resistance of Cement Paste Under Different Exposure Conditions. Adv. Concr. Constr. 2019, 7, 175–181. [Google Scholar]
- Randazzo, L.; Ricca, M.; Ruffolo, S.; Aquino, M.; Petriaggi, B.D.; Enei, F.; La Russa, M.F. An Integrated Analytical Approach to Define the Compositional and Textural Features of Mortars Used in the Underwater Archeological Site of Castrum Novum (Santa Marinella, Rome, Italy). Minerals 2019, 9, 268. [Google Scholar] [CrossRef]
- Golewski, G.L. Generalized Fracture Toughness and Compressive Strength of Sustainable Concrete Including Low Calcium Fly Ash. Materials 2017, 10, 1393. [Google Scholar] [CrossRef] [PubMed]
- Golewski, G.L. An Analysis of Fracture Toughness in Concrete with Fly Ash Addition, Considering all Models of Cracking. IOP Conf. Ser. Mater. Sci. Eng. 2018, 416, 012029. [Google Scholar] [CrossRef]
- Maslehuddin, M.; Saricimen, H.; Al-Mana, A.I. Effect of Fly Ash Addition on the Corrosion Resisting Characteristics of Concrete. ACI Mater. J. 1987, 84, 42–50. [Google Scholar]
- Zhang, M.H. Microstructure, Crack Propagation, and Mechanical Properties of Cement Pastes Containing High Volumes of Fly Ashes. Cem. Concr. Res. 1995, 25, 1165–1178. [Google Scholar] [CrossRef]
- Papadakis, V.G. Effect of Fly Ash on Portland Cement Systems. Part I. Low-Calcium Fly Ash. Cem. Concr. Res. 1999, 29, 1727–1736. [Google Scholar] [CrossRef]
- Siddique, R. Effect of Fine Aggregate Replacement with Class F Fly Ash on the Mechanical Properties of Concrete. Cem. Concr. Res. 2003, 33, 539–547. [Google Scholar] [CrossRef]
- Golewski, G.L. Effect of Fly Ash Addition on the Fracture Toughness of Plain Concrete at Third Model of Fracture. J. Civ. Eng. Manag. 2017, 23, 613–620. [Google Scholar] [CrossRef]
- Dragaš, J.S.; Ignjatović, I.; Tošić, N.; Marinković, S. Mechanical and Time-Dependent Properties of High-Volume Fly Ash Concrete for Structural Use. Mag. Concr. Res. 2016, 68, 1–14. [Google Scholar] [CrossRef]
- Gastaldini, A.L.G.; Isaia, G.; Saciloto, A.; Missau, F.; Hoppe, T. Influence of Curing Time on the Chloride Penetration Resistance of Concrete Containing Rice Husk Ash: A Technical and Economical Feasibility Study. Cem. Concr. Compos. 2010, 32, 783–793. [Google Scholar] [CrossRef]
- Nath, P.; Sarker, P. Effect of Fly Ash on the Durability Properties of High Strength Concrete. Procedia Eng. 2011, 14, 1149–1156. [Google Scholar] [CrossRef]
- Berry, E.E.; Hemmings, R.T.; Zhang, M.-H.; Cornelius, B.J. Hydration in High-Volume Fly Ash Concrete Binders. ACI Mater. J. 1994, 91, 382–389. [Google Scholar]
- Lee, H.J.; Lee, J.H.; Kim, D.-G. Study on the Change in Microstructure of Fly Ash Concrete Depending on Ages and Degree of Hydration Using XRD and SEM. Adv. Mater. Res. 2012, 486, 350–355. [Google Scholar] [CrossRef]
- Taylor, P.; Tait, R. Effects of Fly Ash on Fatigue and Fracture Properties of Hardened Cement Mortar. Cem. Concr. Compos. 1999, 21, 223–232. [Google Scholar] [CrossRef]
- Golewski, G.L.; Sadowski, T. Macroscopic Evaluation of Fracture Processes in Fly Ash Concrete. Solid State Phenom. 2016, 254, 188–193. [Google Scholar] [CrossRef]
- Golewski, G. Measurement of Fracture Mechanics Parameters of Concrete Containing Fly Ash Thanks to Use of Digital Image Correlation (DIC) Method. Measurement 2019, 135, 96–105. [Google Scholar] [CrossRef]
- Golewski, G. Estimation of the Optimum Content of Fly Ash in Concrete Composite Based on the Analysis of Fracture Toughness Tests Using Various Measuring Systems. Constr. Build. Mater. 2019, 213, 142–155. [Google Scholar] [CrossRef]
- Brandt, A.M.; Prokopski, G. Critical Values of Stress Intensity Factor in Mode II Fracture of Cementitious Composites. J. Mater. Sci. 1990, 25, 3605–3610. [Google Scholar] [CrossRef]
- Berto, F.; Ayatollahi, M.; Marsavina, L. Mixed Mode Fracture. Theoret. Appl. Fract. Mech. 2017, 91, 1. [Google Scholar] [CrossRef]
- Khiem, N.; Tran, H.T.; Nam, D. Modal Analysis of Cracked Continuous Timoshenko Beam Made of Functionally Graded Material. Mech. Based Des. Struct. Mach. 2019, 48, 459–479. [Google Scholar] [CrossRef]
- Farid, H.M.; Fakoor, M. Mixed Mode I/II Fracture Criterion to Anticipate Behavior of the Orthotropic Materials. Steel Compos. Struct. 2020, 34, 671–679. [Google Scholar]
- Khansari, N.M.; Fakoor, M.; Berto, F. Probabilistic Micromechanical Damage Model for Mixed Mode I/II Fracture Investigation of Composite Materials. Theor. Appl. Fract. Mech. 2019, 99, 177–193. [Google Scholar] [CrossRef]
- Żebrowski, W.; Wolka, P.; Kurpinska, M. The Influence of the Aircraft Operating Fluids on the Mechanical Parameters of the Airport Surface Concrete. Materials 2020, 13, 3081. [Google Scholar] [CrossRef] [PubMed]
- Szeląg, M. Development of Cracking Patterns in Modified Cement Matrix with Microsilica. Materials 2018, 11, 1928. [Google Scholar] [CrossRef] [PubMed]
- Golewski, G.L.; Sadowski, T. A Study of Mode III Fracture Toughness in Young and Mature Concrete with Fly Ash Additive. Solid State Phenom. 2016, 254, 120–125. [Google Scholar] [CrossRef]
- Golewski, G.L. Effect of Curing Time on the Fracture Toughness of Fly Ash Concrete Composites. Compos. Struct. 2018, 185, 105–112. [Google Scholar] [CrossRef]
- Golewski, G.L.; Sadowski, T. Experimental Investigation and Numerical Modeling Fracture Processes in Fly Ash Concrete at Early Age. Solid State Phenom. 2012, 188, 158–163. [Google Scholar] [CrossRef]
- Zhang, P.; Gao, J.-X.; Dai, X.-B.; Zhang, T.-H.; Wang, J. Fracture Behavior of Fly Ash Concrete Containing Silica Fume. Struct. Eng. Mech. 2016, 59, 261–275. [Google Scholar] [CrossRef]
- Wang, J.; Wu, Q.; Guan, J.; Zhang, P.; Fang, H.; Hu, S. Numerical Simulation on Size Effect of Fracture Toughness of Concrete Based on Mesomechanics. Materials 2020, 13, 1370. [Google Scholar] [CrossRef]
- Sadowski, T.; Golewski, G. A Failure Analysis of Concrete Composites Incorporating Fly Ash during Torsional Loading. Compos. Struct. 2018, 183, 527–535. [Google Scholar] [CrossRef]
- Aliha, M. On Predicting Mode II Fracture Toughness (KIIc) of Hot Mix Asphalt Mixtures Using the Strain Energy Density Criterion. Theor. Appl. Fract. Mech. 2019, 99, 36–43. [Google Scholar] [CrossRef]
- D’Antino, T.; Santandrea, M.; Carloni, C. Advances in Knowledge of the Fracture Properties of Cohesive Materials: Fired-Clay and Tuff Bricks. J. Eng. Mech. 2020, 146, 04020079. [Google Scholar] [CrossRef]
- Sadrmomtazi, A.; Lotfi-Omran, O.; Nikbin, I.M. Influence of Cement Content and Maximum Aggregate Size on the Fracture Parameters of Magnetite Concrete Using WFM, SEM and BEM. Theor. Appl. Fract. Mech. 2020, 107, 102482. [Google Scholar] [CrossRef]
- Dehestani, A.; Hosseini, M.; Beydokhti, A.T. Effect of Wetting–Drying Cycles on Mode I and Mode II Fracture Toughness of Cement Mortar and Concrete. Theor. Appl. Fract. Mech. 2020, 106, 102448. [Google Scholar] [CrossRef]
- Wu, Z.-M.; Yu, R.C.; Sun, C.-Y.; Wang, Y.-J.; Zhang, X.; Fei, X.-D. A New Test Method for the Complete Load-Displacement Curve of Concrete Under Mixed Mode I-II Fracture. Theor. Appl. Fract. Mech. 2020, 108, 102629. [Google Scholar] [CrossRef]
- Ayatollahi, M.R.; Saboori, B. T-Stress Effects in Mixed Mode I/II/III Brittle Frature. Eng. Fract. Mech. 2015, 144, 32–45. [Google Scholar] [CrossRef]
- Linul, E.; Marsavina, L.; Stoia, D.I. Mode I and II Fracture Toughness Investigation of Laser-Sintered Polyamide. Theor. Appl. Fract. Mech. 2020, 106, 102497. [Google Scholar] [CrossRef]
- Fakoor, M.; Farid, H.M. Mixed-Mode I/II Fracture Criterion for Crack Initiation Assessment of Composite Materials. Acta Mech. 2018, 230, 281–301. [Google Scholar] [CrossRef]
- Golewski, G.; Sadowski, T. Fracture Toughness at Shear (Mode II) of Concretes Made of Natural and Broken Aggregates. Brittle Matrix Compos. 2006, 8, 537–546. [Google Scholar] [CrossRef]
- Aliha, M.; Razmi, A.; Mousavi, A. Fracture Study of Concrete Composites with Synthetic Fibers Additive Under Modes I and III Using ENDB Specimen. Constr. Build. Mater. 2018, 190, 612–622. [Google Scholar] [CrossRef]
- Aliha, M.R.M.; Sarbijan, M.; Bahmani, A. Fracture Toughness Determination of Modified HMA Mixtures with Two Novel Disc Shape Configurations. Constr. Build. Mater. 2017, 155, 789–799. [Google Scholar] [CrossRef]
- Wu, B.; Tang, K. Modelling on Crack Propagation Behaviours at Concrete Matrix-Aggregate Interface. Fatigue Fract. Eng. Mater. Struct. 2019, 42, 1803–1814. [Google Scholar] [CrossRef]
- Wang, R.; He, X.; Li, Y. Evaluation of Microcracks in the Interfacial Transition Zone of Recycled Rubber Concrete. Struct. Concr. 2019, 20, 1684–1694. [Google Scholar] [CrossRef]
- Golewski, G.L. The Influence of Microcrack Width on the Mechanical Parameters in Concrete with the Addition of Fly Ash: Consideration of Technological and Economical Benefits. Constr. Build. Mater. 2019, 197, 849–861. [Google Scholar] [CrossRef]
- Wu, C.-H.; Huang, C.-H.; Kan, Y.-C.; Yen, T. Effects of Fineness and Dosage of Fly Ash on the Fracture Properties and Strength of Concrete. Appl. Sci. 2019, 9, 2266. [Google Scholar] [CrossRef]
- Reinhardt, H.W.; Ošbolt, J.; Shilang, X.; Dinku, A. Shear of Structural Concrete Members and Pure Mode II Testing. Adv. Cem. Based Mater. 1997, 5, 75–85. [Google Scholar] [CrossRef]
- Reinhardt, H.W. A New Compact Shear Specimen. Int. J. Fract. 1981, 17, R105–R107. [Google Scholar]
- Reinhardt, H.-W.; Xu, S. Experimental Determination of Kiic of Normal Strength Concrete. Mater. Struct. 1998, 31, 296–302. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Pfeiffer, P.A. Shear Fracture Tests of Concrete. Mater. Struct. 1986, 19, 111–121. [Google Scholar] [CrossRef]
- Murakami, Y.; Keer, L.M. Stress Intensity Factors Handbook; Pergamon Press: Oxford, UK, 1987. [Google Scholar]
- Prokopski, G. Influence of Water-Cement Ratio on Micro-Cracking of Ordinary Concrete. J. Mater. Sci. 1991, 26, 6352–6356. [Google Scholar] [CrossRef]
- Gil, D.M.; Golewski, G. Potential of Siliceous Fly Ash and Silica Fume as a Substitute for Binder in Cementitious Concretes. E3S Web Conf. 2018, 49, 00030. [Google Scholar] [CrossRef]
- Gil, D.M.; Golewski, G.L. Effect of Silica Fume and Siliceous Fly Ash Addition on the Fracture Toughness of Plain Concrete in Mode I. IOP Conf. Ser. Mater. Sci. Eng. 2018, 416, 012065. [Google Scholar] [CrossRef]
- Nogueira, C.L. A New Method to Test Concrete Tensile and Shear Strength with Cylindrical Specimens. ACI Mater. J. 2018, 115, 909–923. [Google Scholar] [CrossRef]
- Zhou, C.; Zhu, Z.; Zhu, A.; Zhou, L.; Fan, Y.; Lang, L. Deterioration of Mode II Fracture Toughness, Compressive Strength and Elastic Modulus of Concrete under the Environment of Acid Rain and Cyclic Wetting-Drying. Constr. Build. Mater. 2019, 228, 116809. [Google Scholar] [CrossRef]
- Beaudoin, J.; Gu, P.; Myers, R. The Fracture of C-S-H and C-S-H/CH Mixtures 11Communicated by M. Daimon. Cem. Concr. Res. 1998, 28, 341–347. [Google Scholar] [CrossRef]
- Beaudoin, J. Comparison of Mechanical Properties of Compacted Calcium Hydroxide and Portland Cement Paste Systems. Cem. Concr. Res. 1983, 13, 319–324. [Google Scholar] [CrossRef]
- Mindess, S. The Strength and Fracture of Concrete: The Role of Calcium Hydroxide. In Materials Science of Concrete: Calcium Hyroxide in Concrete; Special Volume; Skalny, J., Gebauer, J., Odler, I., Eds.; The American Ceramic Society: Westerville, OH, USA, 2001; pp. 143–154. [Google Scholar]
- Ziegeldorf, S. Fracture Mechanics Parameters of Hardened Cement Paste, Aggregates and Interfaces. In Fracture Mechanics of Concrete; Wittmann, F.H., Ed.; Elsevier Science Publishers: Amsterdam, The Netherlands, 1983; pp. 371–409. [Google Scholar]
- Jiang, H.; Chen, M.; Sha, Z.; Xiao, J.; Feng, J. Numeric Analysis on Shear Behavior of High-Strength Concrete Single-Keyed Dry Joints with Fixing Imperfections in Precast Concrete Segmental Bridges. Materials 2020, 13, 2914. [Google Scholar] [CrossRef] [PubMed]
- Ikramullah; Rizal, S.; Nakai, Y.; Shiozawa, D.; Shawkataly, A.K.; Huzni, S.; Thalib, S. Evaluation of Interfacial Fracture Toughness and Interfacial Shear Strength of Typha Spp. Fiber/Polymer Composite by Double Shear Test Method. Materials 2019, 12, 2225. [Google Scholar] [CrossRef]
- Sucharda, O. Identification of Fracture Mechanic Properties of Concrete and Analysis of Shear Capacity of Reinforced Concrete Beams without Transverse Reinforcement. Materials 2020, 13, 2788. [Google Scholar] [CrossRef] [PubMed]
- Ungermann, J.; Adam, V.; Classen, M. Fictitious Rough Crack Model (FRCM): A Smeared Crack Modelling Approach to Account for Aggregate Interlock and Mixed Mode Fracture of Plain Concrete. Materials 2020, 13, 2774. [Google Scholar] [CrossRef]
- Rajchel, M.; Kulpa, M.; Siwowski, T. Experimental Study on a Novel Shear Connection System for FRP-Concrete Hybrid Bridge Girder. Materials 2020, 13, 2045. [Google Scholar] [CrossRef]
- Zhang, J.-H.; Li, S.-S.; Xie, W.; Guo, Y.-D. Experimental Study on Shear Capacity of High Strength Reinforcement Concrete Deep Beams with Small Shear Span–Depth Ratio. Materials 2020, 13, 1218. [Google Scholar] [CrossRef] [PubMed]
- Kurpinska, M.; Grzyl, B.; Kristowski, A. Cost Analysis of Prefabricated Elements of the Ordinary and Lightweight Concrete Walls in Residential Construction. Materials 2019, 12, 3629. [Google Scholar] [CrossRef] [PubMed]
- Lam, L.; Wong, Y.; Poon, C. Effect of Fly Ash and Silica Fume on Compressive and Fracture Behaviors of Concrete. Cem. Concr. Res. 1998, 28, 271–283. [Google Scholar] [CrossRef]
- Bharatkumar, B.H.; Raghuprasad, B.K.; Ramachandramurthy, D.S.; Narayanan, R.; Gopalakrishnan, S. Effect of Fly Ash and Slag on the Fracture Characteristics of High Performance Concrete. Mater. Struct. 2005, 38, 63–72. [Google Scholar] [CrossRef]
- Vejmelkova, E.; Pavlikova, M.; Keepert, M.; Kersner, Z.; Rovnanikova, P.; Ondracek, M.; Sedlmajer, M.; Cerny, R. Fly Ash Influence on the Properties of High Performance Concrete. Cem. Wapno Beton 2009, 4, 189–204. [Google Scholar]
- Tang, W.; Lo, T.Y.; Chan, W.K. Fracture Properties of Normal and Lightweight High-Strength Concrete. Mag. Concr. Res. 2008, 60, 237–244. [Google Scholar] [CrossRef]
- Rao, G.A.; Rao, A.S. Toughness Indices of Steel Fiber Reinforced Concrete Under Mode II Loading. Mater. Struct. 2009, 42, 1173–1184. [Google Scholar] [CrossRef]
- Watkins, J. Fracture Toughness Test for Soil-Cement Samples in Mode II. Int. J. Fract. 1983, 23, R135–R138. [Google Scholar] [CrossRef]
- Golewski, G.; Golewski, P.; Sadowski, T. Numerical Modelling Crack Propagation under Mode II Fracture in Plain Concretes Containing Siliceous Fly-Ash Additive Using XFEM Method. Comput. Mater. Sci. 2012, 62, 75–78. [Google Scholar] [CrossRef]
- BSI. EN 12390-3: 2011+AC: 2012. In Testing Hardened Concrete–Part 3: Compressive Strength of Test Specimens; British Standards Institution (BSI): London, UK, 2009. [Google Scholar]
- Bochenek, A.; Prokopski, G. The Investigation of Aggregate Grain Size Effect on Fracture Toughness of Ordinary Concrete Structures. Int. J. Fract. 1989, 41, 197–205. [Google Scholar] [CrossRef]
- Prokopski, G. Effect of Coarse Aggregate Quantity on Fracture Toughness of Concretes. J. Mater. Sci. 1993, 28, 5717–5721. [Google Scholar] [CrossRef]
- Tkaczewska, E.; Małolepszy, J. Hydration of Coal–Biomass Fly Ash Cement. Constr. Build. Mater. 2009, 23, 2694–2700. [Google Scholar] [CrossRef]
- Siddique, R. Properties of Self-Compacting Concrete Containing Class F Fly Ash. Mater. Des. 2011, 32, 1501–1507. [Google Scholar] [CrossRef]






| Type of Tested Concrete | Addition of LCFA (%) | Reference |
|---|---|---|
| Plain | 0, 25, 45 | [107] |
| Plain | 0, 10, 20, 30 | [57] |
| Plain | 0, 20, 30 | [55,56] |
| High Performance | 0, 25 | [108] |
| High Performance | 0, 10, 40 | [109] |
| Lightweight High Strength | As coarse aggregate | [110] |
| Material | Class | Specific Surface (m2/g) | Specific Gravity (g/cm3) | Acquisition Place |
|---|---|---|---|---|
OPC | 32.5 R * | 0.33 | 3.11 | Chełm Cement Plant, Chełm, Poland |
LCFA | F ** | 0.36 | 2.14 | Puławy Power Plant, Puławy, Poland |
| Material | Type and Size | Specific Density (g/cm3) | Compressive Strength (MPa) | Deposit Occurrence |
|---|---|---|---|---|
Fine aggregate | Pit sand 0–2 mm | 2.60 | 33 | Markuszów deposit, Markuszów, Poland |
Coarse aggregate | Gravel 2–8 mm | 2.65 | 34 | Las Suwalski deposit, Las Suwalski, Poland |
| Concrete Series | OPC | LCFA | %LCFA | Sand | Gravel | Water | Water/Binder | Plasticizer |
|---|---|---|---|---|---|---|---|---|
| LCFA-00 | 352 | 0 | 0 | |||||
| LCFA-20 | 282 | 70 | 20 | 676 | 1205 | 141 | 0.4 | 2 |
| LCFA-30 | 246 | 106 | 30 |
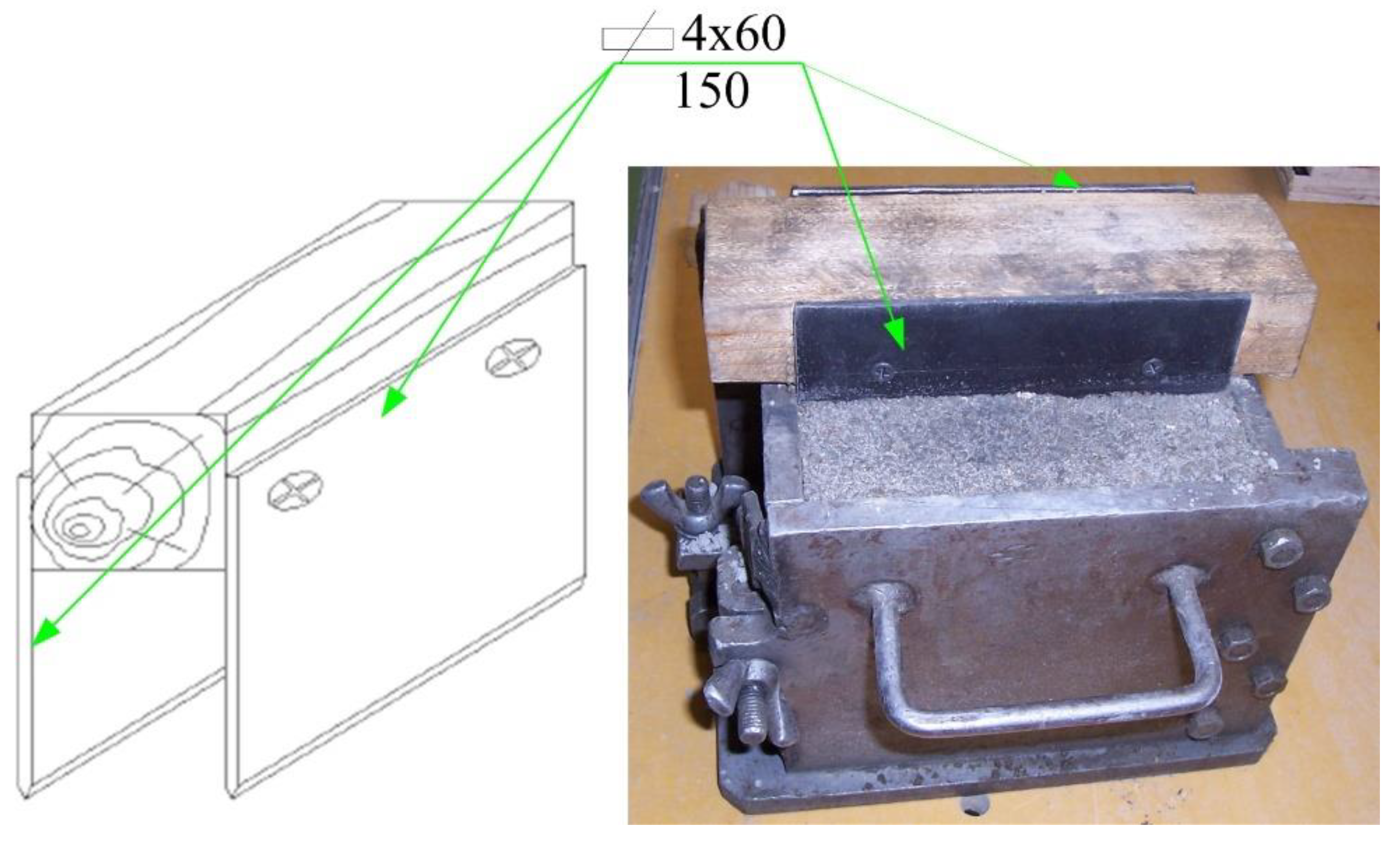
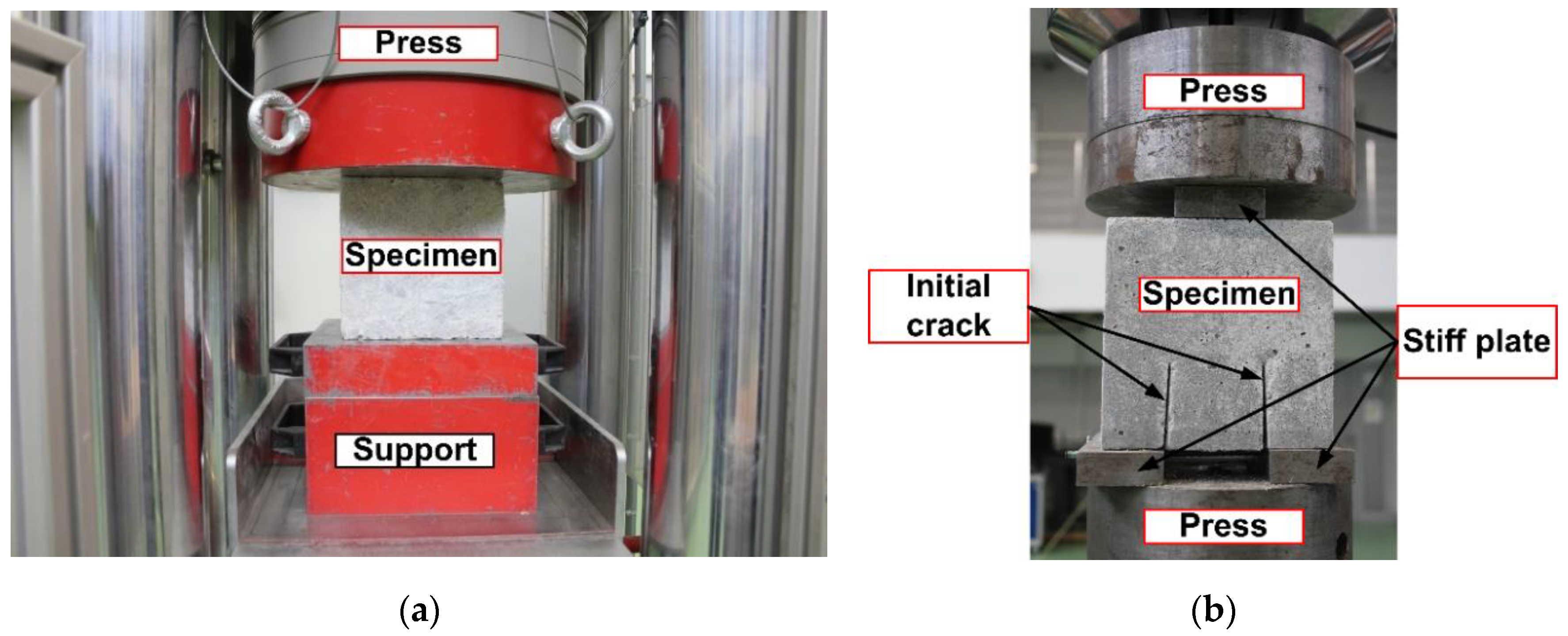
| Type of Test | Geometry of Specimens (mm) | Load Diagram | Curing Time of Specimens (days) | Number of Specimens |
|---|---|---|---|---|
| Compression | 150 × 150 × 150 |  | 3, 7, 28, 90, 180, 365 | 6 in all periods |
| Fracture toughness | 150 × 150 × 150 | 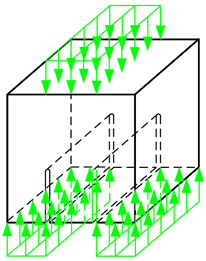 | 3, 7, 28, 90, 180, 365 | 6 in all periods |
| Concrete Series | Age (Days) | fcm (MPa) | ν(fcm) (%) | KIIc (MN/m3/2) | ν(KIIc) (%) |
|---|---|---|---|---|---|
| LCFA-00 | 3 | 24.23 | 10.73 | 2.26 | 16.81 |
| 7 | 33.18 | 7.74 | 3.18 | 12.89 | |
| 28 | 47.51 | 4.58 | 4.24 | 9.43 | |
| 90 | 55.13 | 4.55 | 4.93 | 7.10 | |
| 180 | 57.22 | 4.33 | 5.12 | 5.86 | |
| 365 | 59.25 | 4.15 | 5.31 | 4.52 | |
| LCFA-20 | 3 | 16.95 | 17.99 | 1.48 | 22.97 |
| 7 | 30.12 | 10.06 | 2.93 | 13.65 | |
| 28 | 48.96 | 6.17 | 4.39 | 11.62 | |
| 90 | 59.35 | 4.72 | 5.33 | 7.69 | |
| 180 | 62.81 | 4.01 | 5.70 | 5.61 | |
| 365 | 67.29 | 3.49 | 6.14 | 4.72 | |
| LCFA-30 | 3 | 14.23 | 25.23 | 1.05 | 23.81 |
| 7 | 30.06 | 11.88 | 2.40 | 12.92 | |
| 28 | 45.10 | 7.87 | 3.65 | 11.51 | |
| 90 | 55.11 | 5.63 | 4.68 | 8.12 | |
| 180 | 58.83 | 4.86 | 5.16 | 6.59 | |
| 365 | 63.27 | 3.95 | 5.58 | 4.84 |
| Type of Tested Concrete | KIIc (MN/m3/2) | Reference |
|---|---|---|
| LCFA-00 | 4.24 | Own tests |
| LCFA-20 | 4.39 | Own tests |
| LCFA-30 | 3.65 | Own tests |
| Basalt concrete | 4.45 | [58,115,116] |
| Limestone concrete | 4.64 | [58,116] |
| Granite concrete | 5.14 | [58,115,116] |
| Burned shale concrete | 3.54 | [58,115,116] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Golewski, G.L. Changes in the Fracture Toughness under Mode II Loading of Low Calcium Fly Ash (LCFA) Concrete Depending on Ages. Materials 2020, 13, 5241. https://doi.org/10.3390/ma13225241
Golewski GL. Changes in the Fracture Toughness under Mode II Loading of Low Calcium Fly Ash (LCFA) Concrete Depending on Ages. Materials. 2020; 13(22):5241. https://doi.org/10.3390/ma13225241
Chicago/Turabian StyleGolewski, Grzegorz Ludwik. 2020. "Changes in the Fracture Toughness under Mode II Loading of Low Calcium Fly Ash (LCFA) Concrete Depending on Ages" Materials 13, no. 22: 5241. https://doi.org/10.3390/ma13225241
APA StyleGolewski, G. L. (2020). Changes in the Fracture Toughness under Mode II Loading of Low Calcium Fly Ash (LCFA) Concrete Depending on Ages. Materials, 13(22), 5241. https://doi.org/10.3390/ma13225241





