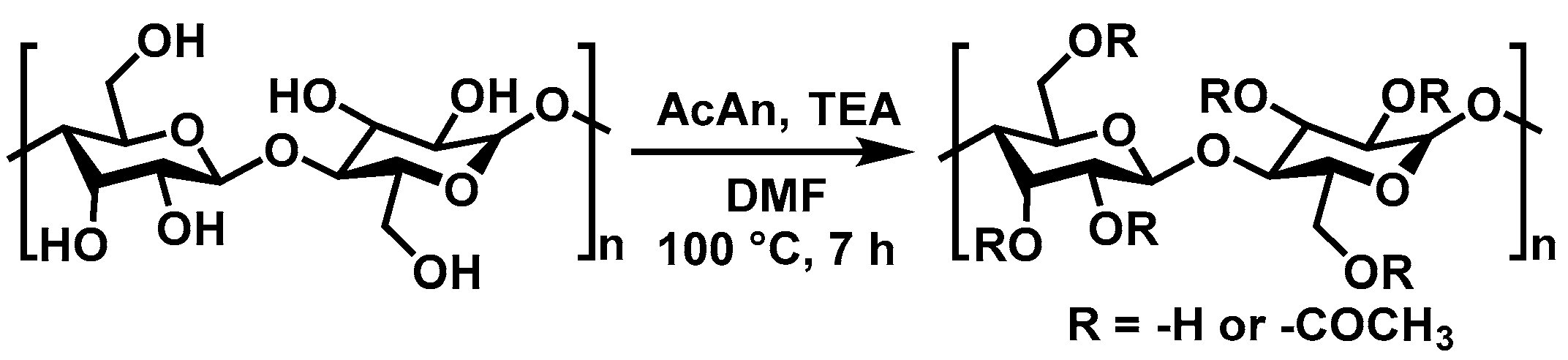
3.1. Composite Processing
In processing TWC composites, sheets of longitudinally cut basswood veneers were delignified resulting in a mass loss and a color change to white as the lignin phase is decomposed, but a preservation of the interconnected cellular architecture of the wood as shown in other studies [
2,
3,
11]. Subsequent filling of the cellular architecture of the delignified wood template with a PMMA-based resin serves to both provide mechanical reinforcement of the wood template as well as provide a refractive index matched medium by which photons can transmit through the filled wood template.
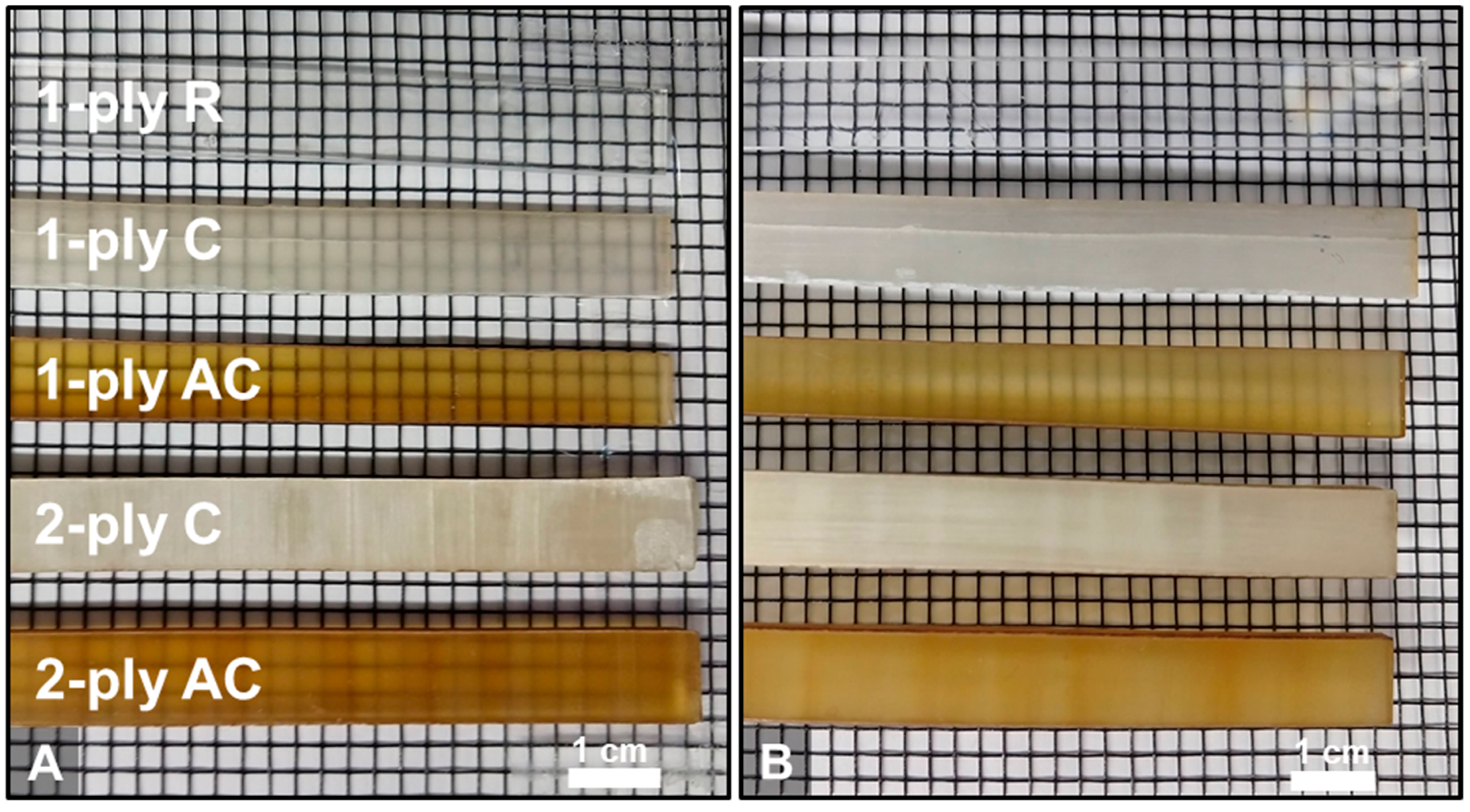
During delignification, a weight decrease of ~23% and complete loss of color was observed, indicating successful oxidation of the lignin. After the acetylation reaction, a weight percentage gain of ~6% was measured. The acetylation of the delignified wood template produced a brown color in solution and within the template that could not be removed, even with rigorous solvent washing. A byproduct, perhaps a salt complex of the triethylamine catalyst, may be responsible for the brown color throughout the substrate, seen in
Figure 1, which has previously been reported in the literature [
15]. According to Li et al., the color that manifests during the acetylation of the delignified wood template could be removed with an oxidation reaction [
5]. The additional oxidation was not attempted on this system. Instead, the acetylated delignified wood template was processed into a TWC with the color retained. A variance in transmittance when placed on a grid and raised 1 cm above the grid can be seen for the fabricated composites (
Figure 1). Despite the brown color, the underlying grid lines are qualitatively sharper for 1-ply AC and 2-ply AC when compared with 1-ply C and 2-ply C, respectively. Additionally, when raised 1 cm from the grid, the lines of the grid can only still be distinguished through the 1-ply AC and the 1-ply resin samples indicating a qualitative improvement of haze for the 1-ply AC sample relative to the 1-ply C sample.
3.2. Fourier Transform Infrared Spectroscopy
The C and AC substrates were analyzed via FTIR (
Figure 2). The broad peak ~3300 cm
−1 corresponds to –OH stretching. In the spectrum for delignified wood templates (C), the broad –OH peak is larger than the corresponding peak for the AC after the acetylation reaction, indicating that substitution at the hydroxyl groups occurred, as expected. The three sharper peaks at 1738 cm
−1, 1370 cm
−1, and 1225 cm
−1 are more pronounced in the AC spectrum than in the C spectrum (
Figure 2). Acetyl groups (–COCH
3) contain strong signaling of motifs that are not typically present on the cellulosic chains [
19]. The peak at 1370 cm
−1 is the C–H bond vibration of non-hydroxyl substituted methyl groups, which serve to decrease the hydrophilicity of the cellulosic substrate [
15]. The peak at 1225 cm
−1 is the stretching mode of the C–O bond of the ester linkage formed on the cellulosic chain with the esterification of the alcohol [
15]. The peak at 1738 cm
−1 corresponds to the stretching mode of the C=O carbonyl group. In the C sample trace, a small peak centered on ~1738 cm
−1 corresponds to low signaling of a carbonyl group, which can be attributed to decomposed lignin residue or oxidized chain end groups of hemicelluloses [
20].
3.3. Ultraviolet-Visible Spectroscopy
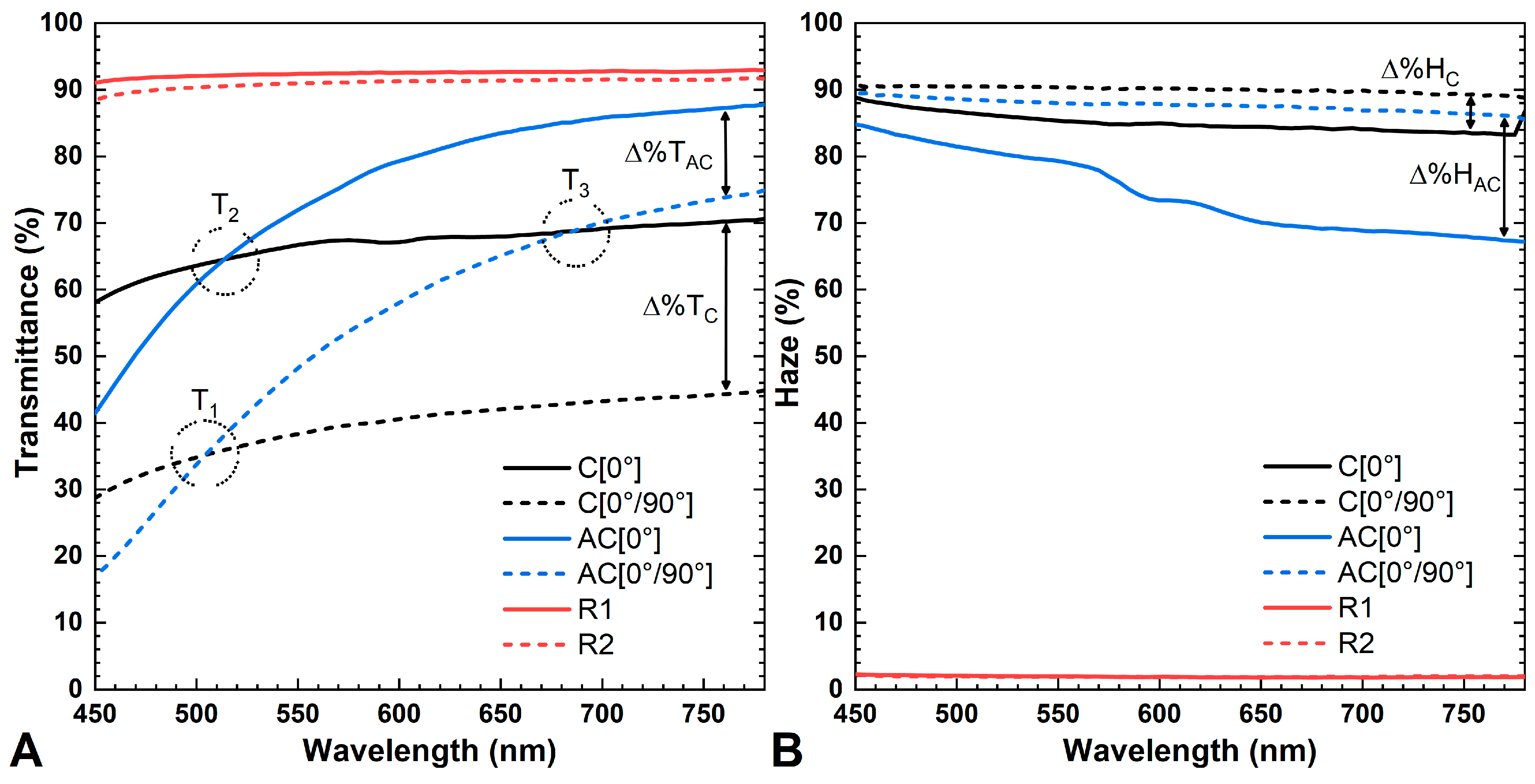
The percentage transmittance and percentage haze as a function of wavelength for each composite sample formulation is presented in
Figure 3a and
Figure 3b, respectively, and averages with ANOVA results are presented in
Table 2. Measuring transmittance and haze in accordance with ASTM D1003 was independent of orientation for 1-ply TWCs, so orientation in the [0°] was arbitrarily chosen for reporting the overall transmittance and haze for the [0°] and [90°] single-ply TWCs. A drop off in transmittances at wavelengths <450 nm was observed, which is likely due to the resin photoinitiator absorption by 405 nm light tailing into longer wavelengths near the violet-blue border wavelength at 450 nm [
21]. This drop-off is consistent across all samples. Therefore, for the purposes of analysis, only transmittance and haze measurements at wavelengths >450 nm are presented in
Figure 3.
As anticipated, R1 and R2 both exhibited high transmittances with minima of 91.1% and 88.5% and maxima of 93.0% and 91.8%, respectively. The average transmittance gap between R1 and R2 was 1.4 ± 0.3%. The transmittance for all TWCs had maxima in the upper red region (780 nm) of the visible spectrum and minima at 450 nm. C[0°] and C[0°/90°] had transmittance maxima of 70.6% and 44.9% and minima of 58.1% and 28.7%, respectively. The average gap in transmittance between C[0°] and C[0°/90°], Δ%T
C, was 27.1 ± 1.3%. AC[0°] and AC[0°/90°] had transmittance maxima of 87.8% and 74.9% and minima of 41.5% and 17.0%, respectively. The average gap in transmittance between AC[0°] and AC[0°/90°], Δ%T
AC, was 20.3 ± 4.8%. The AC samples had higher maxima than the C samples and lower minima in the case of each ply, but when comparing the gaps between 1-ply and 2-ply specimens, the AC samples had a smaller gap than the C samples (p-value < 0.05), suggesting that there was a lesser dependence on thickness when the delignified wood templates were acetylated. As expected, the brown color of the AC samples (
Figure 1) encourages absorption in the green, blue, and violet region, causing the transmittance to decrease with wavelength at a faster rate than the C samples [
22]. Changes in transmittance with wavelength for C and AC samples are denoted by the intersection points on the spectra at T
1, T
2, and T
3. The intersection T
1 occurs at 505 nm for the 2-ply systems and T
2 at 515 nm for the 1-ply TWCs, above which the 1-ply and 2-ply AC samples exhibit higher transmittances and below which the C samples have the higher transmittances (
Figure 3a). The intersection, T
3, at 687 nm is notable, as it shows the point where AC[0°/90°] and C[0°] have equivalent transmittances, despite AC[0°/90°] having twice the thickness of C[0°].
The haze of a specimen is a measurement of light scattering. For high-clarity materials, a low haze is desirable. Haze in composites can occur from incompatibilities at the interface or discontinuities in the bulk phases that allow for air or void volume that can increase light scattering and reduce transmission. For single R1 and R2, the haze values were low, with the respective maxima of 2.3% and 2.2%, and minima of 1.8% and 1.9%. The gap in haze for R1 and R2 was effectively zero containing positive and negative difference values at various wavelengths resulting in an average difference of 0.02 ± 0.09%. The haze measurements for all TWCs from 780 nm to 450 nm followed the reverse trend to transmittance, with the highest haze values at 450 nm and the lowest haze at 780 nm, as can be seen in
Figure 3b. C[0°] and C[0°/90°] had haze maxima of 88.9% and 90.8% and minima of 83.3% and 88.8%, respectively. The average gap in haze between C[0°] and C[0°/90°], Δ%H
C, was 4.9 ± 1.1%. AC[0°] and AC[0°/90°] had haze maxima of 84.8% and 89.4% and minima of 67.2% and 85.6%, respectively. The average gap in haze between AC[0°] and AC[0°/90°], Δ%H
AC, was 13.3 ± 5.0%.
Statistical analysis confirmed that each haze spectrum is distinct from one another (p-value < 0.05) apart from R1 and R2 (p-value = 0.40). Each of the AC samples exhibited lower haze than the respective C samples (p-value < 0.05), suggesting improvement at the interface and, consequently, a reduction in photon scattering. The behavior of AC[0°] is notable, as the value for haze dropped the most across the visible spectrum (p-value < 0.05) to 67.2% at 780 nm. According to Li et al., acetylated TWCs of similar thickness demonstrated haze values down into 45–50%, though this system concerned a different wood species (balsa) and a different resin (pure PMMA, instead of a PMMA-based photocurable resin) [
5].
3.4. Mechanical Properties
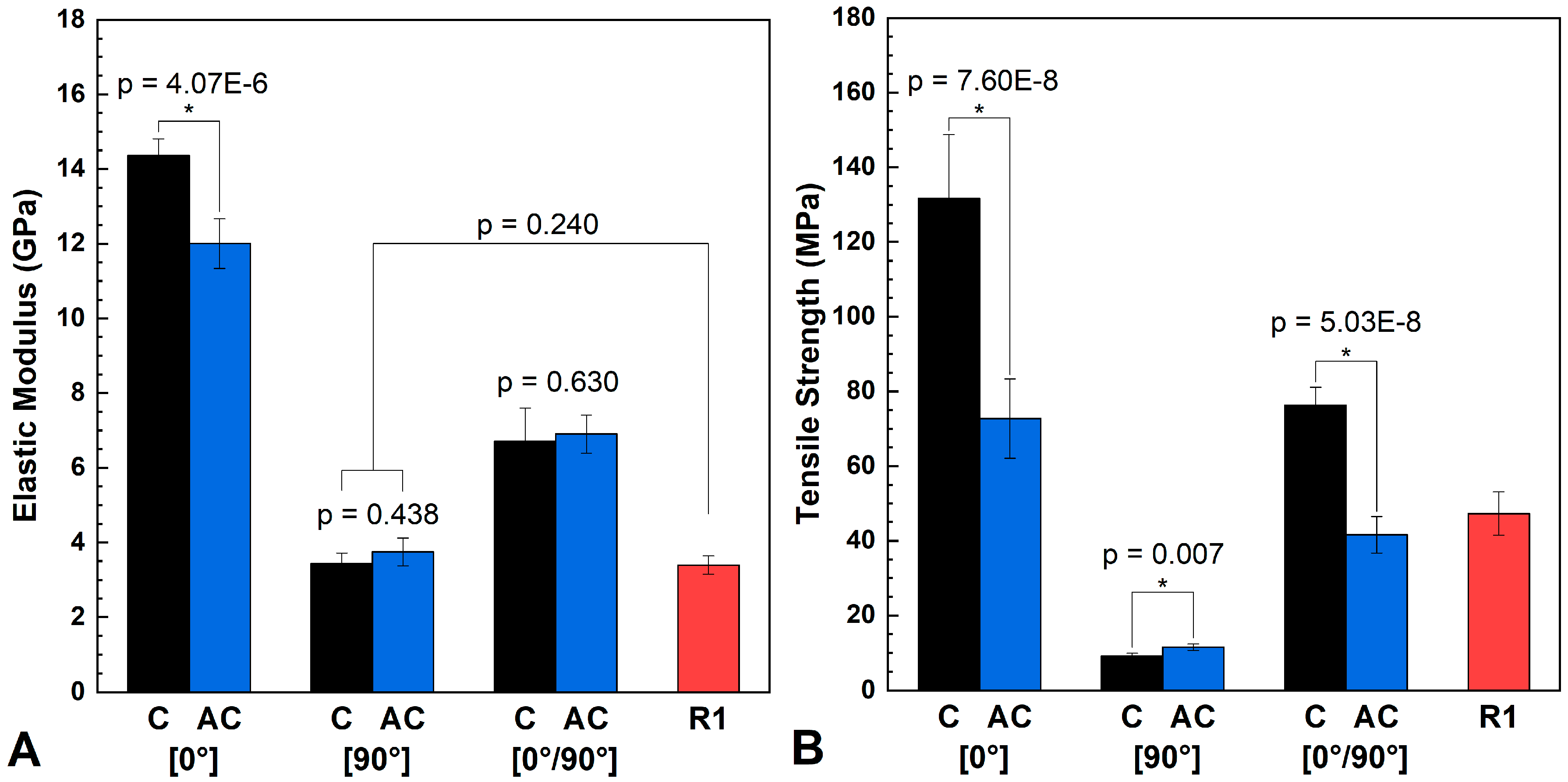
The mechanical response of the TWCs is shown in
Figure 4 and select numerical data can be found in
Table 3 and
Table 4. In
Figure 4, it is evident that the tensile modulus in the [0°] orientation was reduced for the AC samples. In the [90°] and [0°/90°] orientations, however, the same decrease for AC samples is not observed. It is notable that the modulus of the resin (R1) is similar to that of the [90°] C and AC samples. Anisotropic fibrous materials, such as wood, have high stiffness in the fiber (i.e., longitudinal) direction, often requiring compositing or lamination in order to improve the bi-directional properties of the material. Removal of lignin from wood is known to reduce the mechanical integrity of the resulting template. However, filling in the cellular architecture with a stiff resin can restore tensile stiffness [
7]. Two-way ANOVA for elastic modulus of all TWC formulations indicated that there were main effects of specimen type (C and AC samples) and orientation (0°, 90°, and 0°/90°), with an interaction between the two (p-value < 0.05). Comparisons of the C and AC samples at specific orientations showed that, in the 0° orientation, the difference between samples was significant (p-value = 4.07 × 10
−6). In the 90° and the 0°/90° orientations, however, the difference in stiffness was not statistically significant (p-value = 0.438 and 0.630, respectively). An additional one-way ANOVA determined that the elastic modulus for C[90°], AC[90°], and resin were not statistically different from each other (p-value = 0.240). This similarity indicates that the contribution of stiffness response in the 90° orientation is likely due to the resin only.
Similar to the stiffness response, tensile strength exhibited a trend of orientation dependence with the [0°] samples giving higher strengths along the fiber direction. Between the C and AC samples, however, there was a more pronounced decrease for the [0°] and [0°/90°] for the AC samples. There was not an observed decrease for the AC samples in the [90°] orientation, though both of the [90°] samples were weaker than any other TWC and even the resin due to facile fiber separation in that applied load direction. With two-way ANOVA for the tensile strength, the dependent variables were log-transformed to satisfy ANOVA assumptions of normality and homoscedasticity. In this analysis, there were main effects of specimen type (C and AC samples) and orientation (0°, 90°, and 0°/90°), with an interaction between the two main effects (p-value < 0.05). Comparisons of the C and AC samples at specific orientations showed that there was statistical significance between all orientations and tensile strengths, with C[0°] and C[0°/90°] being larger than the AC samples at the same orientations (p-value = 7.60E-6 and 5.03E-8, respectively). AC[90°] had a strength advantage over C[90°] (p-value = 0.007), suggesting an improvement of interfacial bonding between AC and the resin.
Two-way ANOVA for Poisson’s ratio of all TWC formulations indicated that there were neither statistically significant main effects of specimen type nor interactions between specimen type and orientation (p-value > 0.05). The only main effect on Poisson’s ratio was sample orientation, as expected, with samples tested in the 90° being different from the 0°, and 0°/90° samples being statistically similar (p-value < 0.05).
When specifically comparing chemical treatments at varying orientations, acetylation influenced the stiffness and strength of the resulting TWCs. Along the fiber direction (0°), acetylation had the largest effect on the mechanical properties, with the stiffness and strength of AC[0°] samples being significantly reduced relative to C[0°]. This reduction is likely due to the disruption of the hydrogen bonding of cellulose and hemicellulose chains through substitution with hydrophobic acetyl moieties. In the 90° orientations, no statistical difference in stiffness exists between C[90°], AC[90°], or resin samples, but the tensile strength of AC[90°] was greater than C[90°], but both were greatly reduced compared to the resin. When applying a load orthogonal to the alignment of cellulosic fibers, it was expected that a weaker mechanical response would be observed. The stiffness of the 90° specimens were statistically similar to the resin, suggesting that the resin matrix around the fibers was largely responsible for the stiffness under the applied load. Additionally, the homogenous resin exhibited a higher strength relative to either 90° TWC, which is likely a result of critical flaw sizes within the TWCs, resulting in diminished strength responses. Interestingly, AC[90°] was stronger than C[90°], meaning that the acetylation of the delignified wood template likely improved the interfacial interactions between the template and resin matrix.
In the cross-ply system, the stiffness was similar between C and AC samples, but C[0°/90°] had a higher strength than AC[0°/90°]. Following the individual ply-level system, the 0° direction was the strength-dominating orientation, meaning that the C samples were expected to be stronger relative to AC samples. The stiffness response of C[0°/90°] and AC[0°/90°] were not statistically different (p-value = 0.630), which was identical to the trend of the stiffness response in the 90° TWC samples. This result indicates that, despite the reduced stiffness of AC[0°] relative to C[0°], the addition of a 90° ply homogenizes the elastic response in these 2-ply TWCs.
3.5. Mechanical Modeling
Using the 1-ply mechanical response data from
Table 3, the prediction for the 2-ply elastic response was calculated using classical lamination plate theory and the tensile response was calculated using the rule of mixtures. The predicted and experimental responses are shown in
Table 4. The prediction of the tensile strength by Monte Carlo simulation (
n = 100,000) was in relatively good agreement for all samples with difference in means of 5.9 MPa and 0.5 MPa for C[0°/90°] and AC[0°/90°], respectively. The difference in means for the stiffness response was found to be 0.29 GPa and 0.64 GPa for C[0°/90°] and AC[0°/90°], respectively.
Using an unequal variances t-test the prediction of elastic modulus for the C[0°/90°] and AC[0°/90°] TWCs were found to be similar to the experimentally obtained values (p-value = 0.50 and p-value = 0.07, respectively). The predicted and experimental tensile strength of the 2-ply TWCs were also determined to be statistically similar for the C[0°/90°] and AC[0°/90°] TWCs (p-value = 0.053 and p-value = 0.838, respectively). The variance of the predicted tensile strength increased relative to the experimental values when subjected to a Monte Carlo simulation, while the variance in predicted elastic modulus did not. This increase in variance for strength is likely a result of the disparity in magnitude of the standard deviations of the input tensile strengths (
Table 3). For
the standard deviations were >10 MPa, while for
, they were <1 MPa, which could contribute to a larger variance in a Monte Carlo simulation. The model using classical lamination plate theory was able to successfully predict the effective elastic modulus for both C[0°/90°] and AC[0°/90°]. The larger experimental deviation from the predicted stiffness output for AC[0°/90°] was attributed to the statistically lower (AC[0°]) or indistinguishable (AC[90°]) elastic moduli compared to C samples in the 1-ply orientation. This model does not account for the adhesion interactions of the cross-plied sheets infiltrated with resin. If the interaction between the AC substrate and the resin were improved, the interface between the cross-plied sheets could be stronger than expected.
3.6. Mechanical–Optical Response
When considering infrastructure materials that serve optical purposes (e.g., windows), it is desirable to understand the correlation, if any, between optical and mechanical responses. As a reference, soda-lime glass, commonly used for windows, has an average transmittance of ~90% across the visible light spectrum, an elastic modulus between 68–72 GPa, and a tensile strength between 31–35 MPa [
23,
24]. Although the TWCs presented here have singular mechanical response values, there is a strong dependence of wavelength on the optical response. Instead of reporting transmittance and haze values at single wavelengths,
Figure 5 shows the total span of transmittance values for each specimen with the spectral color shown. Similar to the discussion of the UV-Vis traces, wavelengths <450 nm are omitted (no violet) and all 1-ply samples simplified to the [0°] notation as the optical measurements were not orientation dependent. For every specimen, the maximum transmittance values were at the highest recorded wavelength (780 nm—red) and declined with wavelength. The color segments shown are effectively the point-to-point decreasing slope for each region of color in the visible spectrum projected on a line. The solid central line corresponds to the mean elastic moduli or tensile strength for that specimen and the surrounding translucent bar is the first standard deviation. A rationale behind presenting the projection of optical response is due to the multiple intersections, T
1, T
2, and T
3, in
Figure 3a, where superior optical performance of AC vs. C samples is wavelength dependent.
Figure 5 graphically illustrates the ideal design and performance targets for TWCs, namely high stiffness and strength coupled with high, narrowly distributed transmittance values. As expected, the resin satisfies the high, narrowly distributed transmittance, but falls short of good mechanical properties when compared to the TWCs. Both C[0°] and C[0°/90°] have narrower transmittance distributions and higher strengths than either AC[0°] or AC[0°/90°], but do not achieve higher maximum transmittances. It is evident that, while further development of TWCs is required to push the bounds and achieve a mechanically robust and high transmittance composite material, these plots serve as useful assessment tools for evaluating high-performance TWCs that seek to maximize both optical and mechanical performance.