1. Introduction
One of the remarkable additive manufacturing (AM) processes that allows direct production by means of material layer addition is direct metal laser sintering, which was developed to overcome the disadvantages of traditional processes. DMLS process allows the direct production of components at net shape quality. The major advantage of AM process is fewer post-production processes, no geometrical restrictions, and a smallest possible feature size of about 100 µm. DMLS uses laser based processing techniques with different variety of 3D printed materials such as polymers, metals, ceramics and composites. The main objective of this research work was to fill the observed research gap on avoiding undesirable thermal strains, thermal fatigue and thermal damage on disc surface due to sudden variations in thermal boundary conditions. This research work designed a disc brake with radial grooves on disc surface. These modifications are achieved by using additive manufacturing based 3D printed DMLS processes in fabrication. Murr et al. [
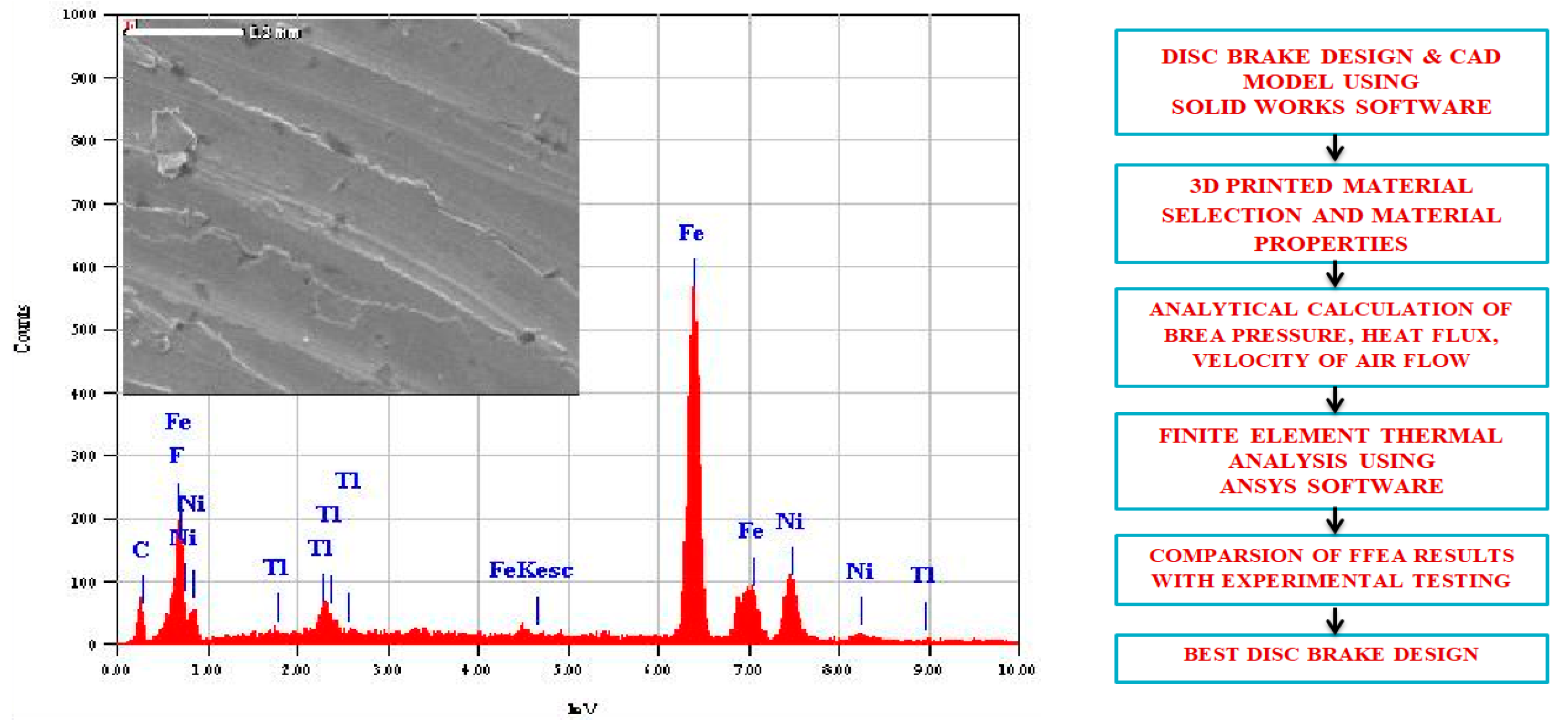
1] used a DMLS machine equipped with powder feed, bed, and laser energy sources for sintering and melting. A CAD model of the energy provided to metal powder during DMLS is based on full melting of powdered state of material by a high power laser beam (100–500 W). The starting 3D printed material in the form of powder is deposited on a substrate plate; the deposited layer is some tens of microns (25–50 µm). Different types of metals can be processed through DMLS; most common metals used for this process are aluminum alloys, nickel alloys, tool steels and stainless steels. In this present work, maraging steel was considered to study thermal behavior of 3D printed metal under sudden increase and decrease of temperature conditions. Maraging steel evolves from a group of martensitic steels with less carbon content. These steels are designated from the combination of “martensitic” and “aging”, since these steels go through different aging heat treatments to improve strength and hardness. Maraging steel is most suitable for DMLS, since they have good weldability property at micro level due to micron-sized melt-pool in the DMLS process with high cooling rates. The property of these steels is proven to be well-matched with typical heavy duty applications in aerospace, automobile and tooling industries. The most commercially available grades for maraging are 200, 250, 300 and 350, which specify yield stress in kilo-pound per square inch with nominal yield stress for maraging being from 1500 to 2500 MPa. Vickers Hardness value in 10 kgf is HV123 (10 Kg), density is 8.2082 g/cc, temperatures range is from 450 to 650 °C for ageing treatment and proper selection of working temperatures must be made for desirable strength for reasonable time interval. Maraging steels are applied for applications with less cobalt and nickel content and have been produced to decrease costs. The strength of Martensite is controlled by carbon content, while Ni and Al have less effect than other alloying elements. Electron dispersive X-ray spectroscopy (EDX) reveals 3D printed Maraging Steel (MS1) characterization, as shown in
Figure 1. Each element has unique emission spectrum. Measuring the spectrum peak intensities after an appropriate calibration, a quantitative estimation of chemical composition can be attained for material composition of maraging steel given in
Table 1. The requirement for metallic 3D printing is expected to grow faster than plastic. In the present context, a research gap has been observed to avoid these undesirable thermal strains, thermal fatigue, and thermal damage on disc surface due to sudden variations in thermal boundary conditions. Thus, this research work designed a disc brake with modifications on disc surface with radial grooves and these modifications were achieved using DMLS processes for easy fabrication. Today, automotive manufacturers use the 3D printing technology for prototyping and functional parts manufacturing. In
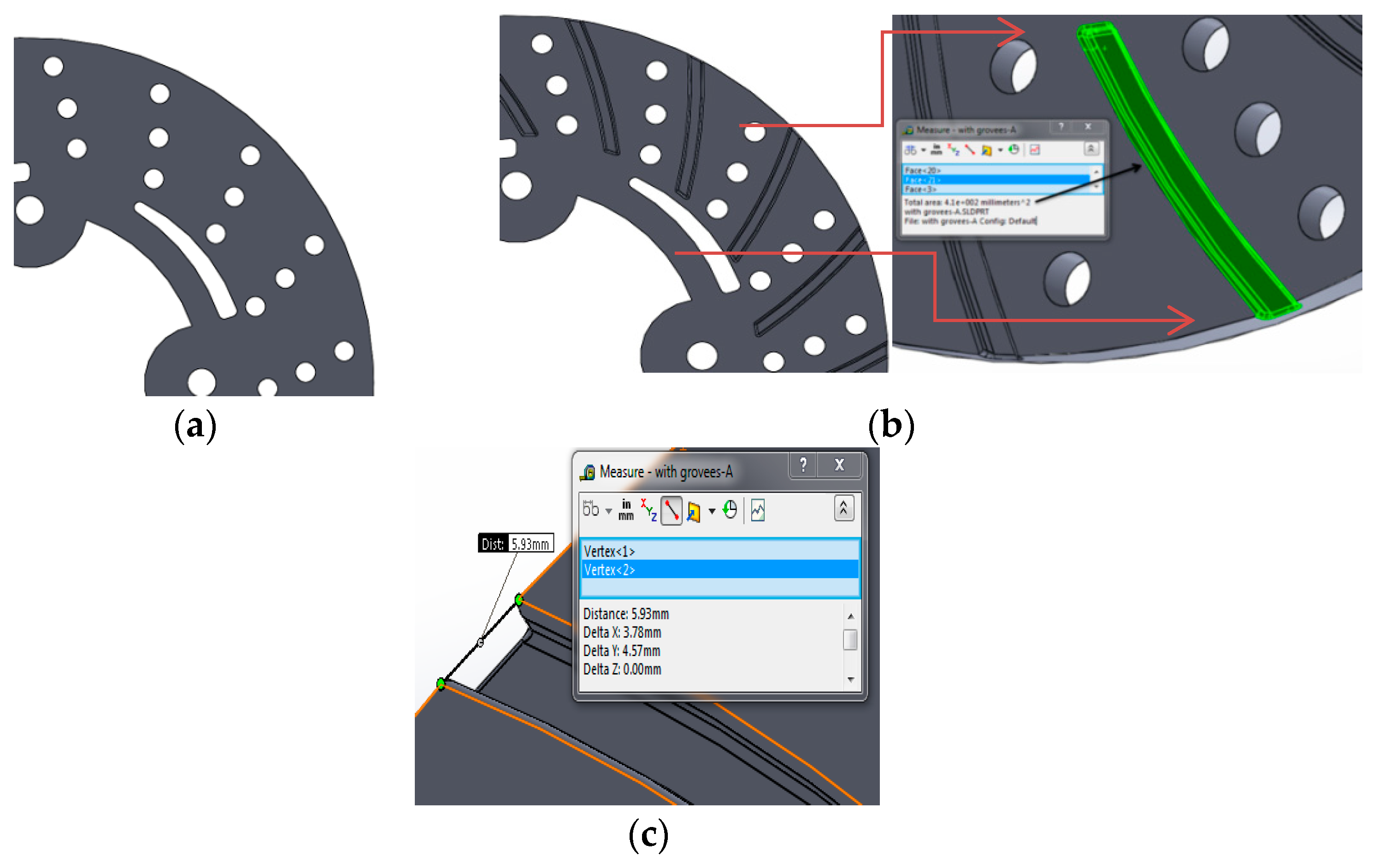
Figure 2, 3D-CAD geometries are shown without and with radial grooves having optimal dimensions of grooves. 3D printing can also improve quality through lighter parts, better ergonomics and more design freedom. Automotive companies also found overall product cycle times decreases by experimenting with 3D printing for assembly fixture, customized fixtures and tooling, making parts cheaper, lighter and faster is often a key goal of automotive industry, indicating opportunities for 3D printing manufacturing.
2. Development of Disc Brake by DMLS
DMLS based additive manufacturing process refers to digital 3D CAD data being used to build up a solid model in layers by depositing molten metal, and helps in developing complex products that can be light and stable [
2]. The solid model during laser sintering is prepared by positioning and slicing through 3D printing software, namely Magics RP. The STL format of CAD data is converted into layer data by means of buildup processor available in 3DP machine. DMLS machine has building volume of 250 mm × 250 mm × 325 mm, equipped with fiber laser of 400 W having scanning speed of 7 m/s [
3]. During DMLS, metal powder upon exposure to laser power beam melt droplets are created and, due to moving beam, melt pools are created and can be regarded as small castings. 3D printed maraging steel properties are given in
Table 2. After getting final 3D printed disc brake of maraging steel, it was heat treated for enhancing hardness and mechanical properties, dimensional stability, reduction in residual stresses, corrosion cracking, and fatigue. Ageing temperature is 490 °C for 5–8 h with air cooling. The 3D printing machine EOS M-290 is a flexible, fast and cost-effective production system for metallic parts. In this work, 3D printed maraging steel (MS1) was considered due to its remarkable properties, such as excellent strength, high toughness, excellent surface finish, and good thermal conductivity. Herzog et al. [
4] showed that maraging steel provides applications in critical parts in automotive, aerospace, structural component, tooling, machine tools, fasteners and production sectors.
The of brake disc developed by EOS M-290 system and disc brake developed by DMLS process are depicted in
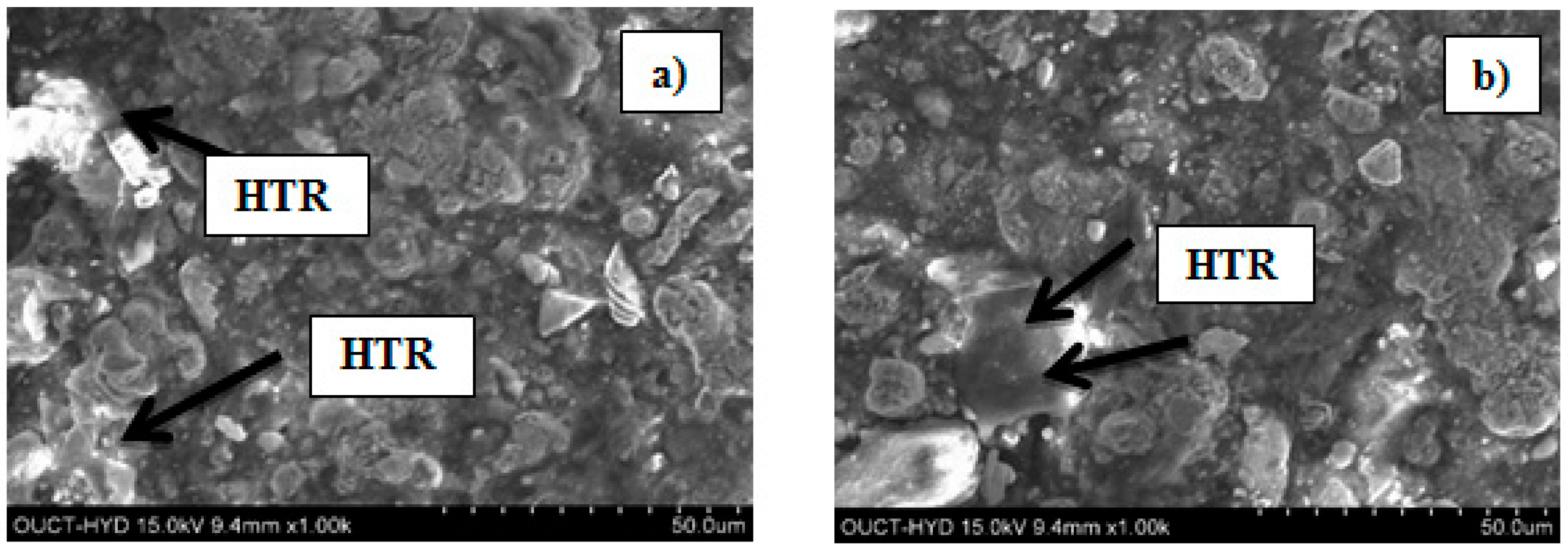
Figure 3. Micrographs of disc brake surface, powder morphology analysis of top surfaces of disc brake under heat flux and temperature surface conditions were carried out by Zeiss EVO 50 (ZEISS, Jena, Vienna, Germany) scanning electron microscope (SEM). SEM micrograph of brake disc surface, shown in
Figure 4 at radii 50 mm and 100 mm, represents the high temperature region of maraging steel by means of high energy collimated electron beam Leitz Aristomet (Leitz, Hicksville, NY, USA) microscope with high resolution and high depth of focus. Differential scanning calorimetry analyses, shown in
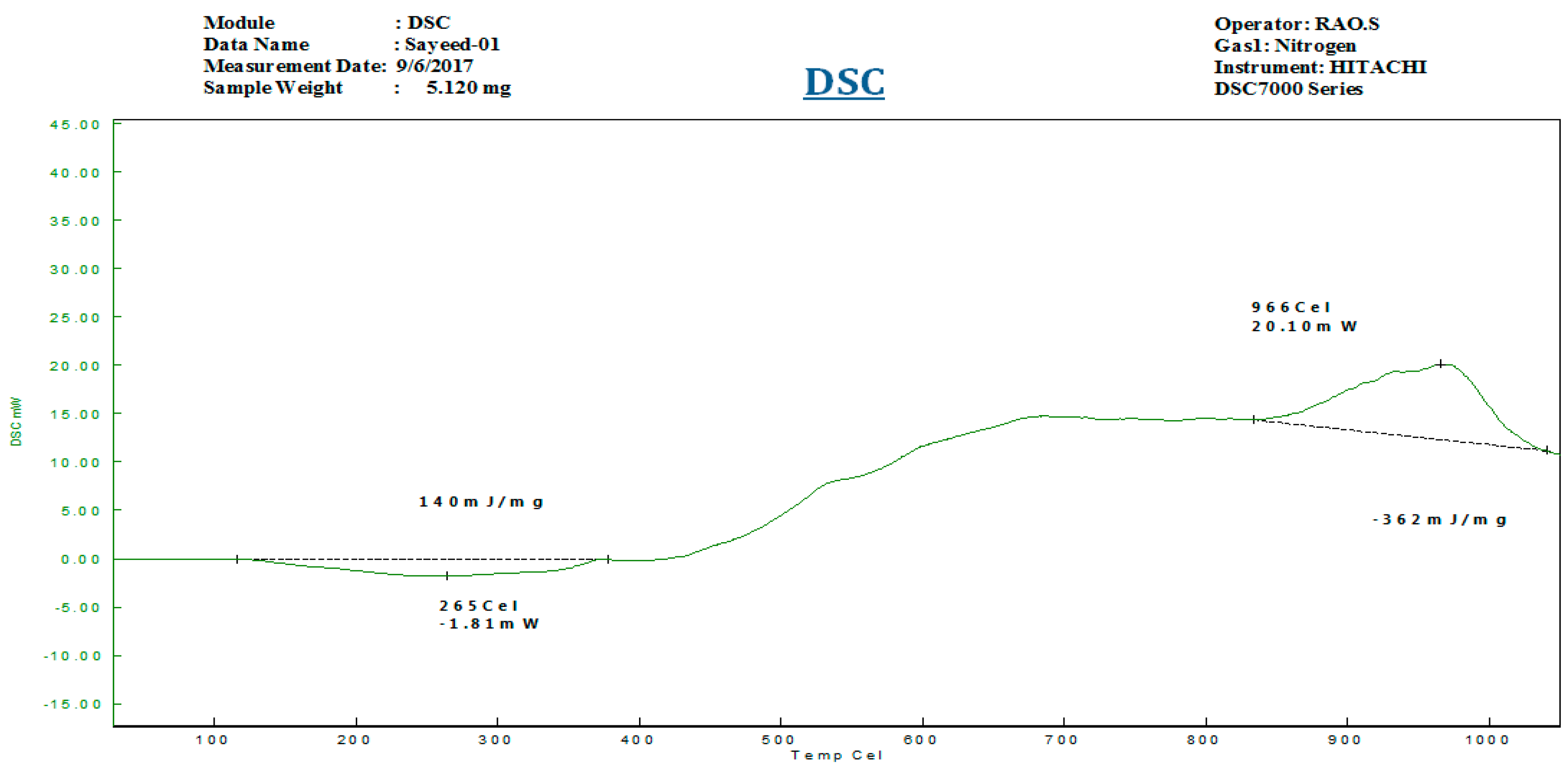
Figure 5, were performed using HITACHI (Hitachi Systems, Chiyoda, Tokyo, Japan), Differential Scanning Calorimeter DSC7000 Series, TGDSC-DTA equipment at KELVIN LABS (Kelvin Lab Inc, Hyderabad, Telengana, India), in nitrogen gas atmosphere at three different heating rates (30, 40, and 50 °C/min) between 30 and 1000 °C, with scanning rate 0.01 °C to 150 °C/min, TG measurement range as ±400 mg. Maraging specimens were prepared by cutting small samples having a weight of about 5.120 mg. DSC tests were conducted to assess phase changes and reactions sequence. The DSC testing instrument consists of empty crucible and another one containing the maraging specimen. They are simultaneously heated and kept at the certain temperature. A DSC curve reveals heat flow, i.e. amount of energy exchanged by specimen versus subjected temperature. DSC makes it possible to study phase transformation sequence under precise non-isothermal temperatures. It was observed in DSC curve that MS1 specimen is stable. No phase change was observed during the testing temperature between 300 and 1000 °C. Typical DSC Specifications are given in
Table 3.
3. Previous Studies on Thermal Analysis of Disc Brake
Belhocine et al. [
5] presented a simulated thermal behavior in brake disc and determined the initial flux entering the disc to evaluate convective coefficient and visualize disc temperature (3D). We conclude that temperature is influenced by construction and materials and mode. Choi et al. [
6] reported that sudden rise and fall of temperature change in metal parts of sliding systems induces uneven thermal stresses due to thermal expansion. This phenomenon is particularly evident in disc brakes under high thermal loads. This paper deals with the finite element modeling of frictional heating process in disc brakes to study the temperature and stress distributions during operation. Andinet et al. [
7] presented factors influencing braking performance of train during braking time and found major factors are temperatures and friction coefficient between pad and brake disc. Thermal transient analysis of disc braking system was performed to evaluate nodal temperature under different thermal and operating conditions. Balaji et al. [
8] reported thermal degradation is significant to determine thermal stability of product considering the brake application. The present paper deals with the role of various fibers such as aramid, acrylic and cellulose fibers. Thermogravimetric analysis has shown that composite NA03 had minimum weight loss and more thermal stable. Grzes et al. [
9] and Marko et al. [
10] reported the influence of the pad cover angle on temperature fields on disc brake. A three-dimensional finite element (FE) model of pad–disc system was developed and calculations were carried out for single braking process at constant deceleration with contact pressure corresponds with cover angle of the pad and the evaluated distributions of temperature for both contact surface of pad and disc surface. They developed and evaluated three-dimensional (3D) thermal–structure coupling model and implemented transient thermal analysis of thermo-elastic contact of disc brakes with variation in frictionally generated heat. They found the source of thermal fatigue was the thermo-elastic problem using finite element method. The results demonstrate that the maximum surface equivalent stress may exceed the material yield strength during an emergency braking, which may cause a plastic damage accumulation in a brake disc, while residual tensile hoop stress is incurred on cooling. FE structural and thermal analysis has been carried out with the dimensions and specifications of Corolla car model. The demand of this automobile car is increasing worldwide due to its low cost, high fuel economy and different towing capacity as per the requirement of roads. The maximum speed of this car is 200 kph. This high speed is the reason this car was considered for structural–thermal analysis of disc brake, and maximum speed was used for thermal analysis. Dimensions of disc and pads given in
Table 4 were used to develop the 3D model in solid works with and without radial grooves. The average stopping distance with fully loaded disc brake at 25 °C traveling speed varies from 100 to 200 m under the experimental test conditions, requiring an average of 81 m stopping distance with the deceleration rate 8 m/s
2 in 4.5 s. For the analysis, speed of the car reduced from 33.34 to 0 m/s, within 4.5 s. Single stop cycle of braking was used for thermal and structural analysis since material regains its original elastic condition after brake force is removed. Lopez et al. [
11] made several assumptions to simplify thermal analysis complexity and output surface temperature was measured experimentally and compared with FE analysis [
12,
13]. Heat dissipated through brake disc surface during application of brake and heat flux applied to surface was considered with and without radial grooves. Huajiang et al. [
14] considered heat transfer convection only after brake application was completed and car accelerated to regain its original speed. These areas include an effective surface area for applying braking pressure with and without radial grooves on disc surface. The remaining surface area of the disc was considered insulated for the purpose of comparison of surface temperatures with and without grooves under the brake pressure of 1 MPa.
4. Temperature Distributions in Disc Brake
To investigate temperature behavior of brake discs, it is necessary to obtain temperature distributions as a function of braking time, speed, and braking pressure. This research attempted to incorporate radial groove features on disc surface by using DMLS and to predict the temperature response due to these design modifications. Rotary motion of the brake disc causes the sliding surface contact between pad and disc to generates heat. Hudson et al. [
15] showed that the surface temperature due to friction generated heat should be considered. The friction force is determined from pressure distribution at contact surfaces of disc and pad. According to Mahmoud et al. [
16], the kinetic energy of a car, once the brakes are applied to the pads, which press against brake rotor, is converted to thermal energy [
17]. In the case of disc brakes, it is kinetic energy converted to thermal energy.
is total mass of vehicle and
is initial speed of vehicle and the heat dissipated by each disc is
, i.e., the rate of heat generated due to friction is equal to friction power, and this frictional heat is absorbed by brake disc and pads. If it is assumed that whole friction power is transferred to heat energy, then heat partition
coefficient needs to be considered. Thermal energy generated at friction surface of brake can be transferred to both rotor and pads. This partitioning of energy is dependent on thermal resistances of brake discs and pad that are further dependent on density, material thermal conductivities and heat capacities. The brake pad thermal resistance must be more than rotor thermal resistance to avoid brake fluid from high temperatures. The partitioning coefficient (γ) for thermal input to brake disc and pad was determined from thermal effusivity ξ given by
The friction contact area of pad and disc are determined from equations given as
The total heat generated on frictional contact interface
equals the heat flux into the disc
and pad
, and braking energy, which is termed as heat partition coefficient
, is determined from the following equation:
where
and
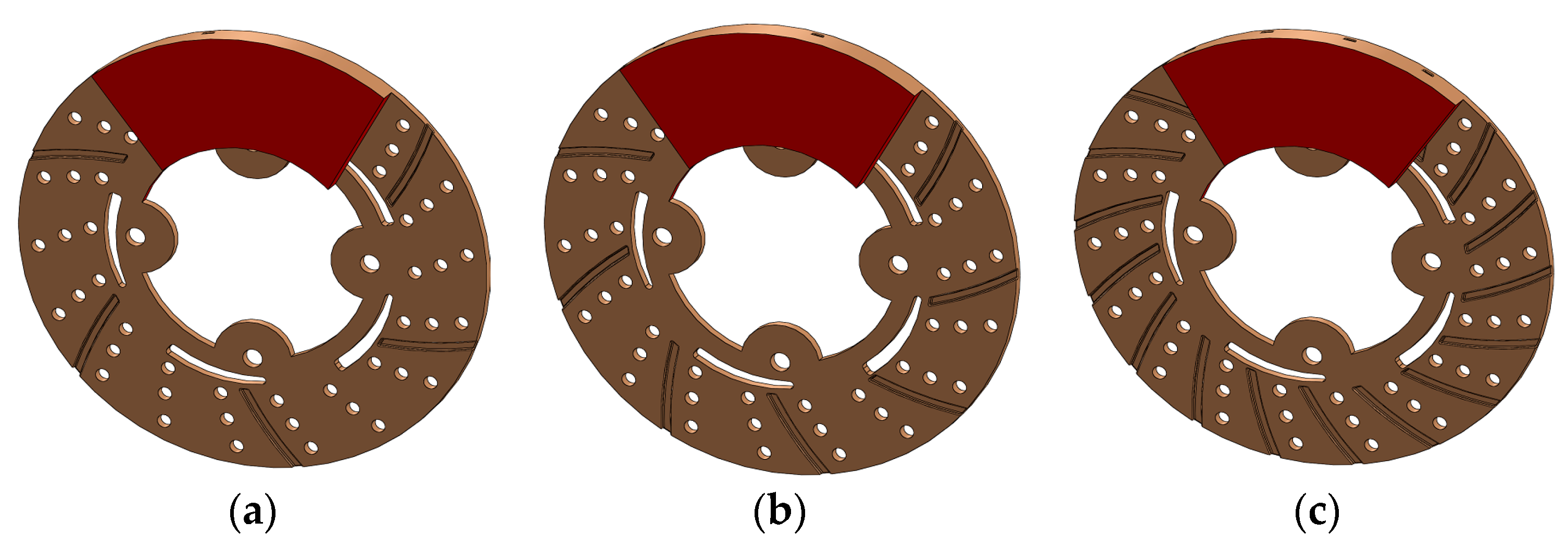
are thermal effusivities of disc and pad; this partitioning of thermal energy is dependent on thermal resistances of pad and brake disc rotor, which are related to their thermal conductivities, materials densities, and heat capacities. Solid works CAD models of disc and pad with 6, 9 and 18 radial grooves on disc surface are shown in
Figure 6. The heat flux generated by pressing pad against rubbing surface of rotor is only source of heat input to the disc; magnitude of this heat flux was calculated from basic energy principle and input of energy is in terms of rotor disc speed, radius of rotor, coefficient of friction and pressure distribution [
18]. The frictional heat generation due to friction of contact surfaces of two surfaces of brake system, coefficient of friction, speed of vehicle, geometry of the disc rotor and pad, and pressure distribution at the sliding surfaces. In uniform pressure distribution, heat flux
on contact area under the pressure distribution is taken into account in thermal and structural analysis [
19].
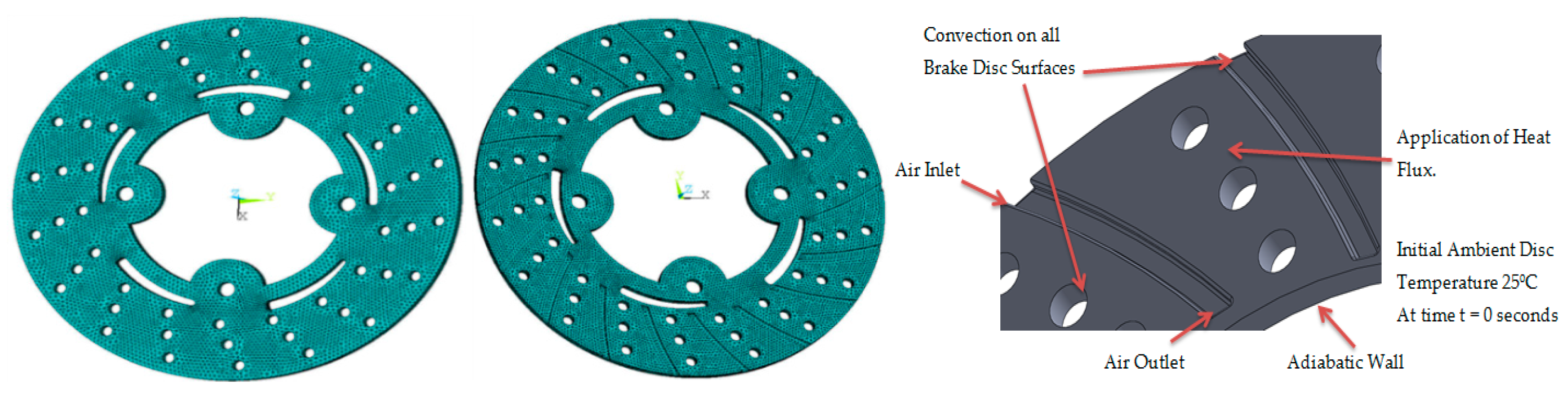
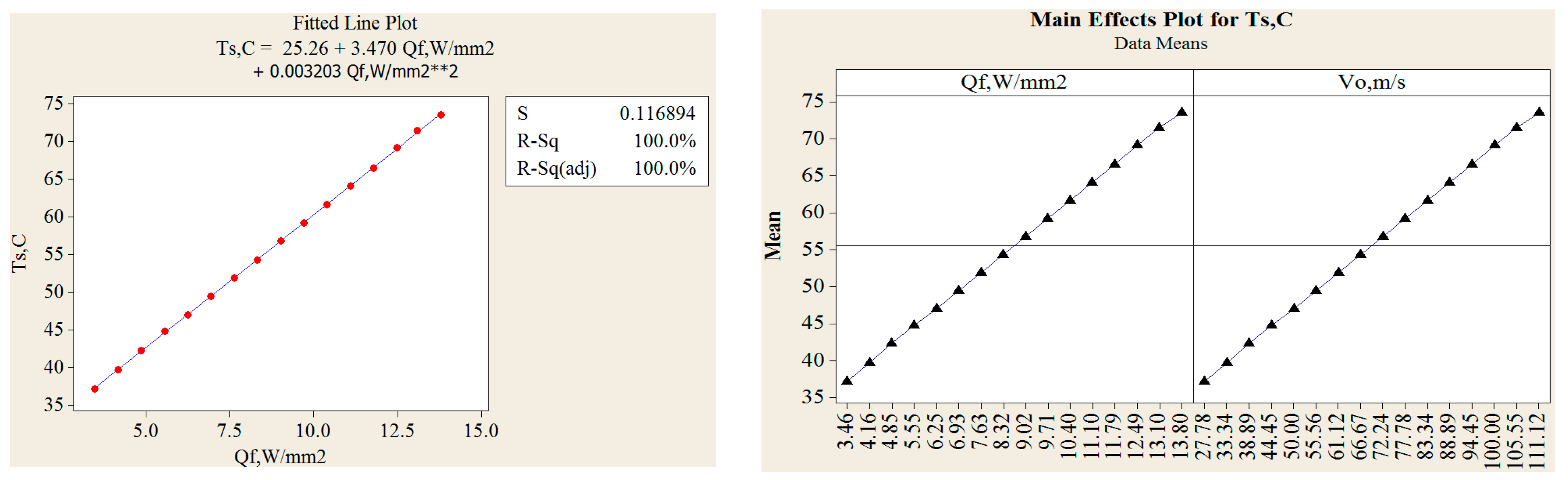
In the present thermal analysis, ambient temperature was assumed at 25 °C and brake disc surface temperature was 37.2 °C at 100 kph. It was assumed that heat dissipation from brake disc surface to atmosphere through convection process is governed by
, where Q
f is in Watts; hc is the convection heat transfer coefficient; Input parameters and dimensions of brake discs are tabulated in
Table 5, Acd and Acp are the contact surface area of the disc and pads, respectively, in m
2; Ts is surface temperature of brake disc; and Ta is ambient air temperature in °C. Heat transfer coefficient is applied to brake discs as heat flux boundary condition. Thus, increasing the rate of heat transfer from surface brake discs reduces disc surface temperature on total surface area of the brake discs [
20].
Density of air
(kg/mm
3) is given by
, where
is the mass flow rate of air (m
3/s) and
is the average air velocity (m/s). Convective heat transfer coefficient at different air velocities are obtained from the formula [
21].
Radial grooves areas at inlet and outlet are
,
, respectively. The Convective heat transfer
into disc surface can be calculated using Equation (6) [
22].
where Ø is rate coverage sector of braking forces between the front and rear axle,
is braking effectiveness, A
cd is disc surface swept by brake pad (m
2), ε
p is factor load distributed on brake disc surface, m is mass of vehicle (kg), g = 9.81 is acceleration of gravity (m/s
2), V
0 is initial speed of vehicle (m/s), and ad is the deceleration of the vehicle (m/s
2).The disc brake groove passage and sector chosen for numerical analysis are shown in
Figure 1. The dimensions of grooves on disc surface are 3.5 mm by 2.5 mm each, with outer and inner diameters as 240 mm and 120 mm, respectively. Heat transfer coefficient
associated with laminar flow for radial and non-radial grooves on brake discs was calculated for Re < 2.4 × 10
5, where do is outer diameter of discs mm, Re is Reynolds number, and Ka is thermal conductivity of air, W/m °C. Experimental validation was done on modified brake disc with and without radial grooves brake using non-contact thermometer Fluke-561, Infrared thermometer, which can measure contact and ambient temperatures. IR thermometer is used to measure hot moving energized, hard-to-reach objects instantly. Experimental results are given in
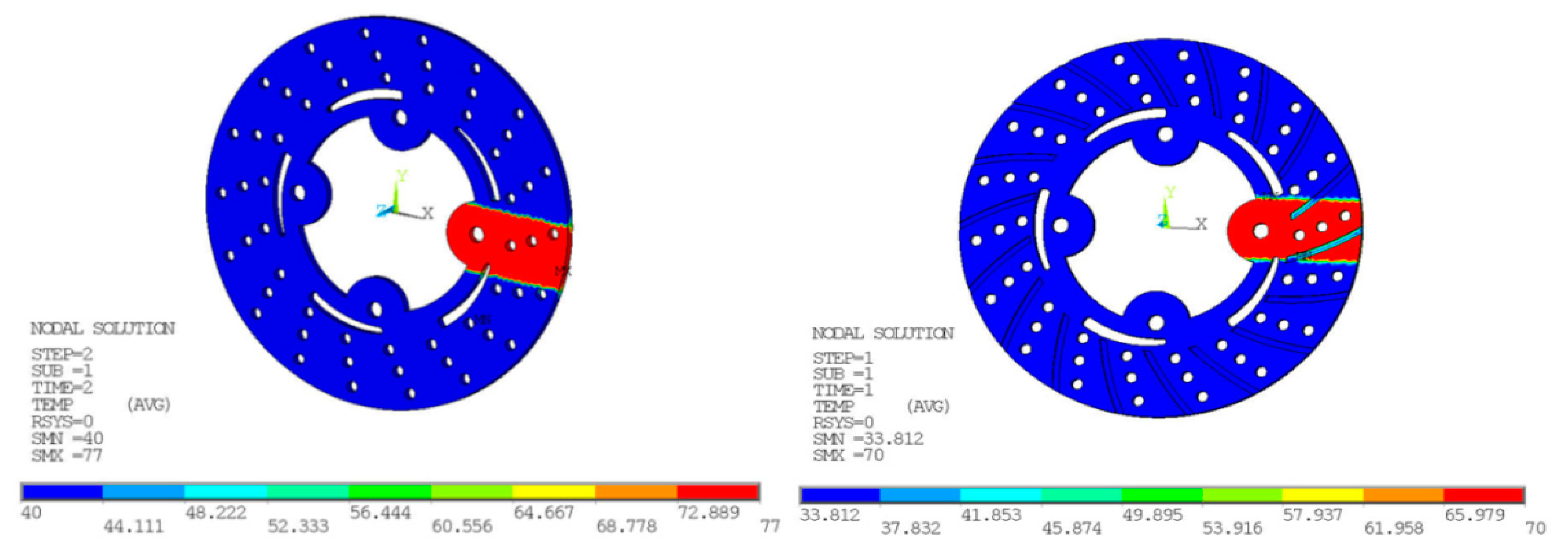
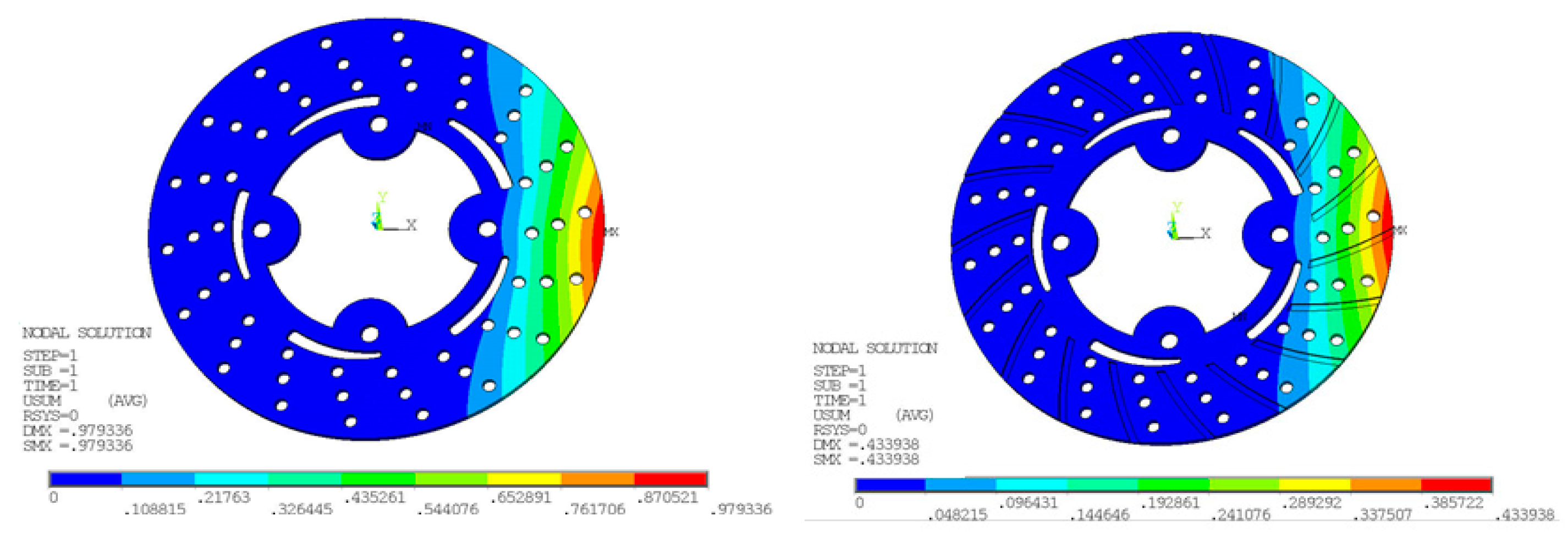
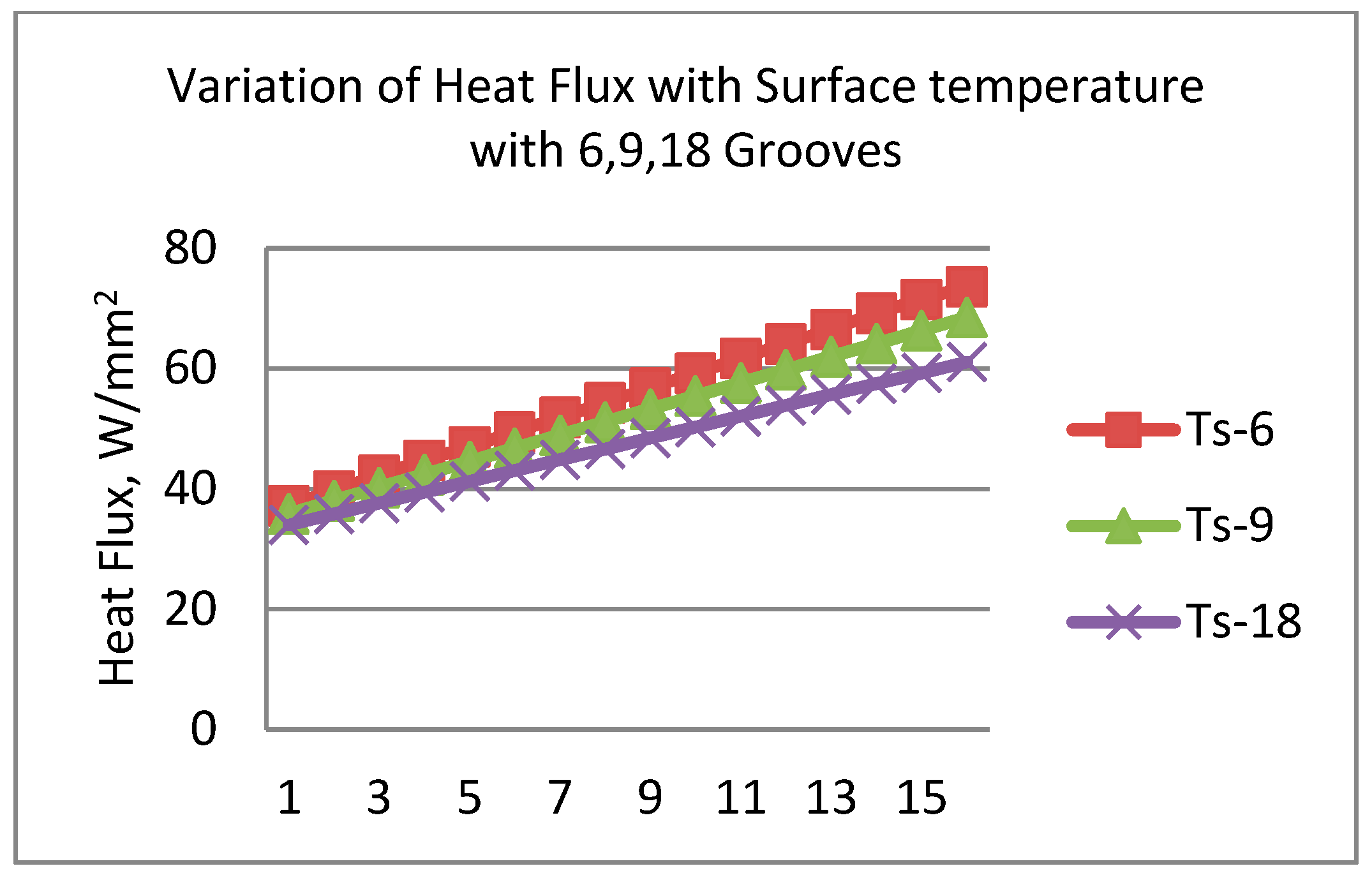
Table 6 for generation of heat on disc surfaces. Disc surface temperatures increase with increasing braking time for different disc design configurations.