Elucidating Grinding Mechanism by Theoretical and Experimental Investigations
Abstract
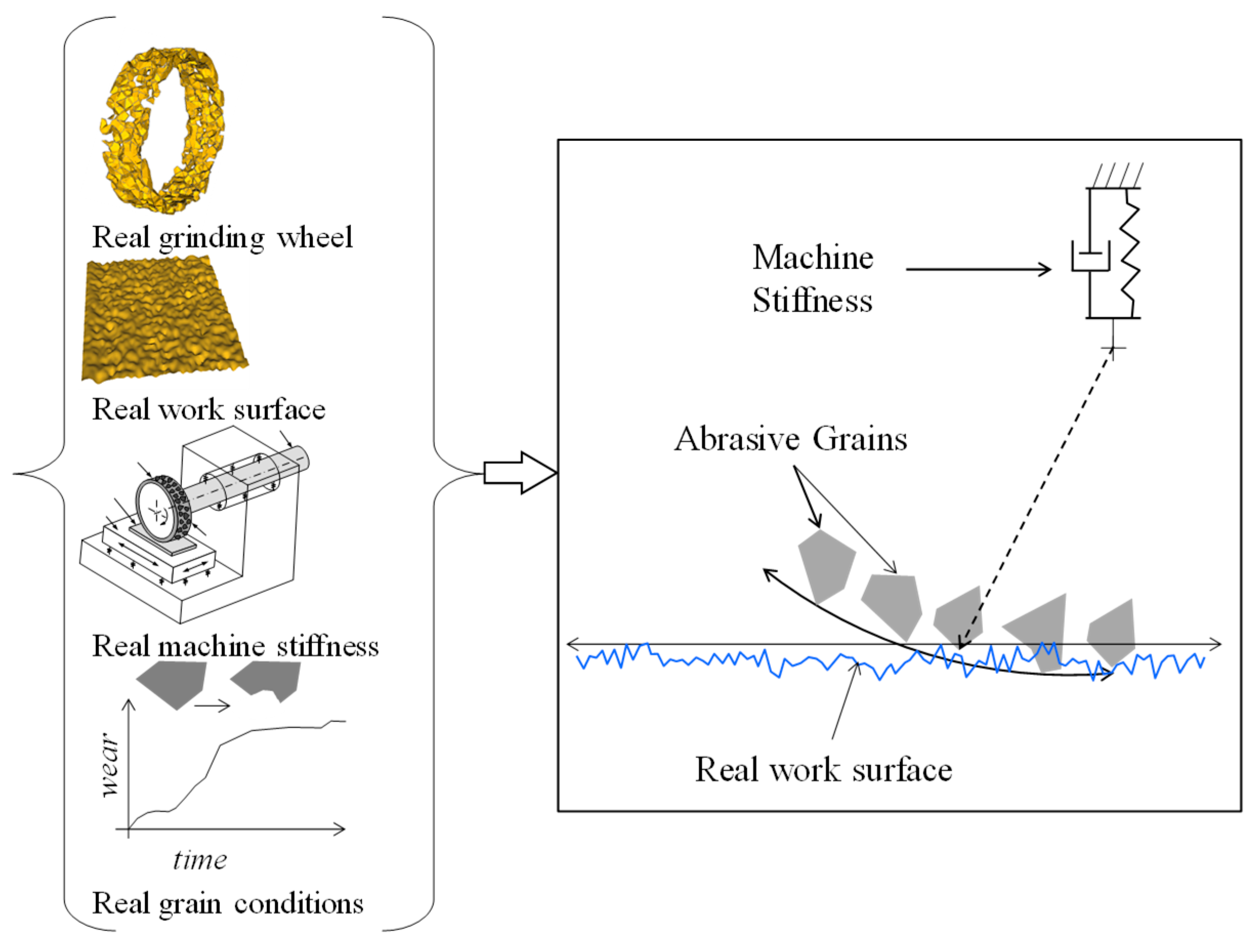
:1. Introduction
2. Literature Review on Grinding Mechanism
3. Preliminary Experimentation
4. Theoretical Model of Grinding Mechanism
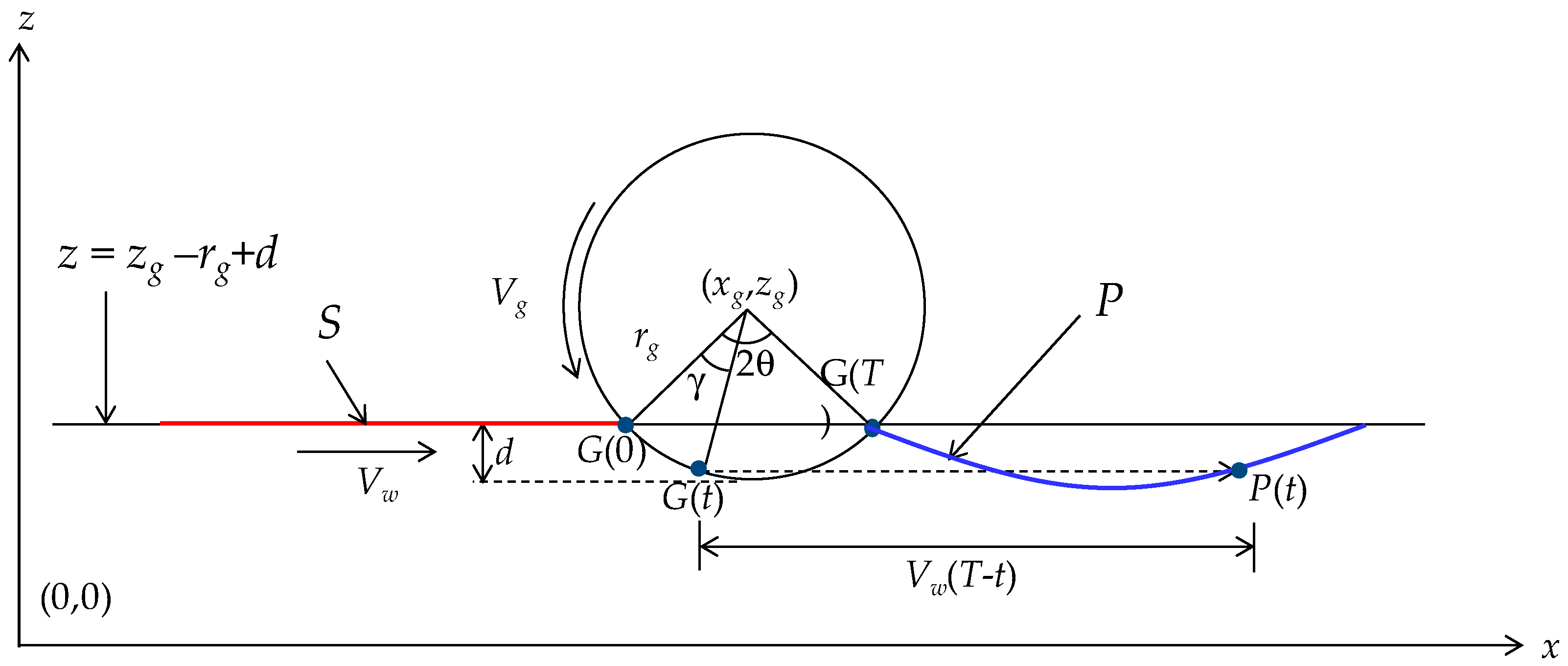
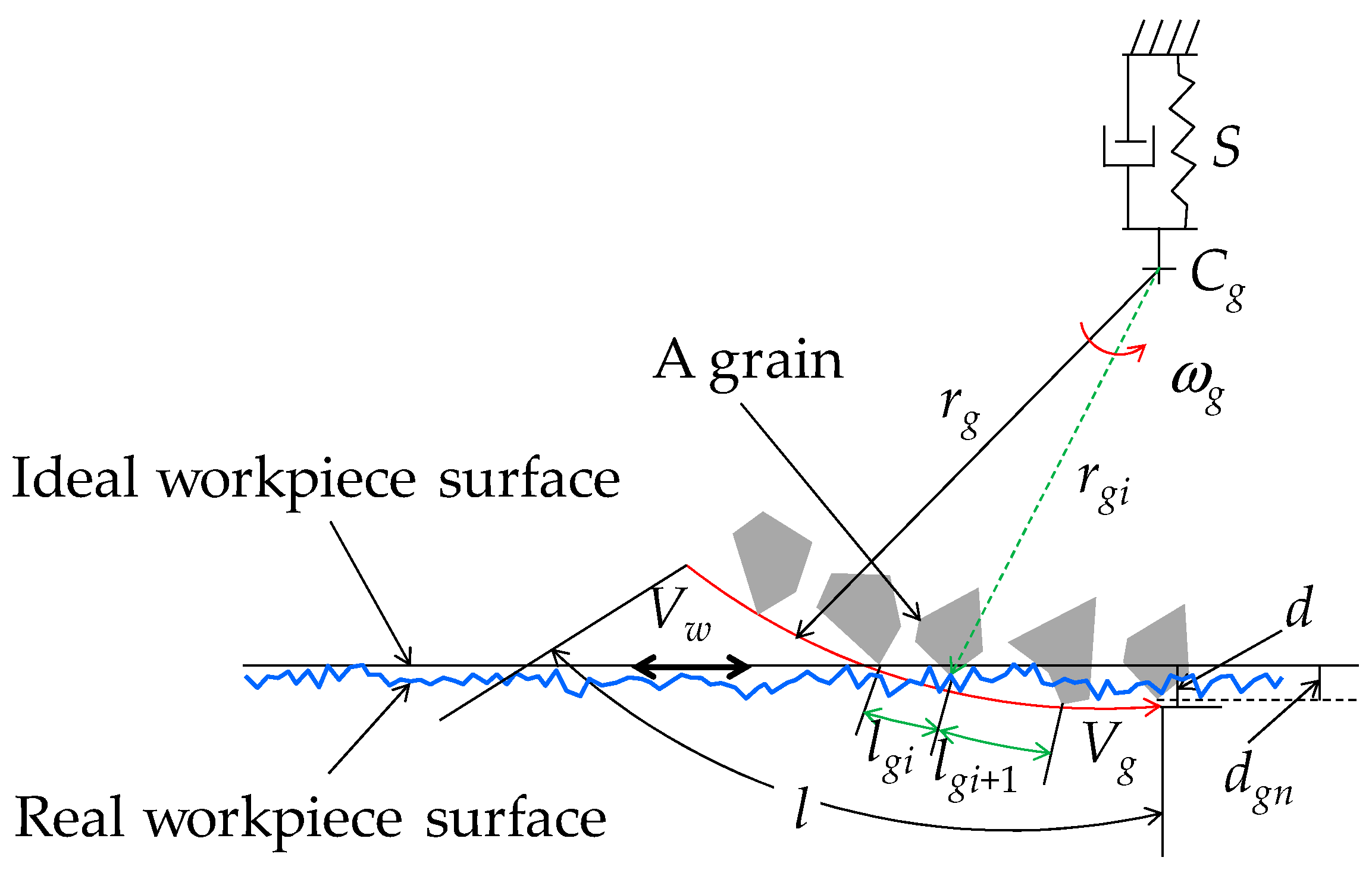
4.1. Effect of a Single Grain
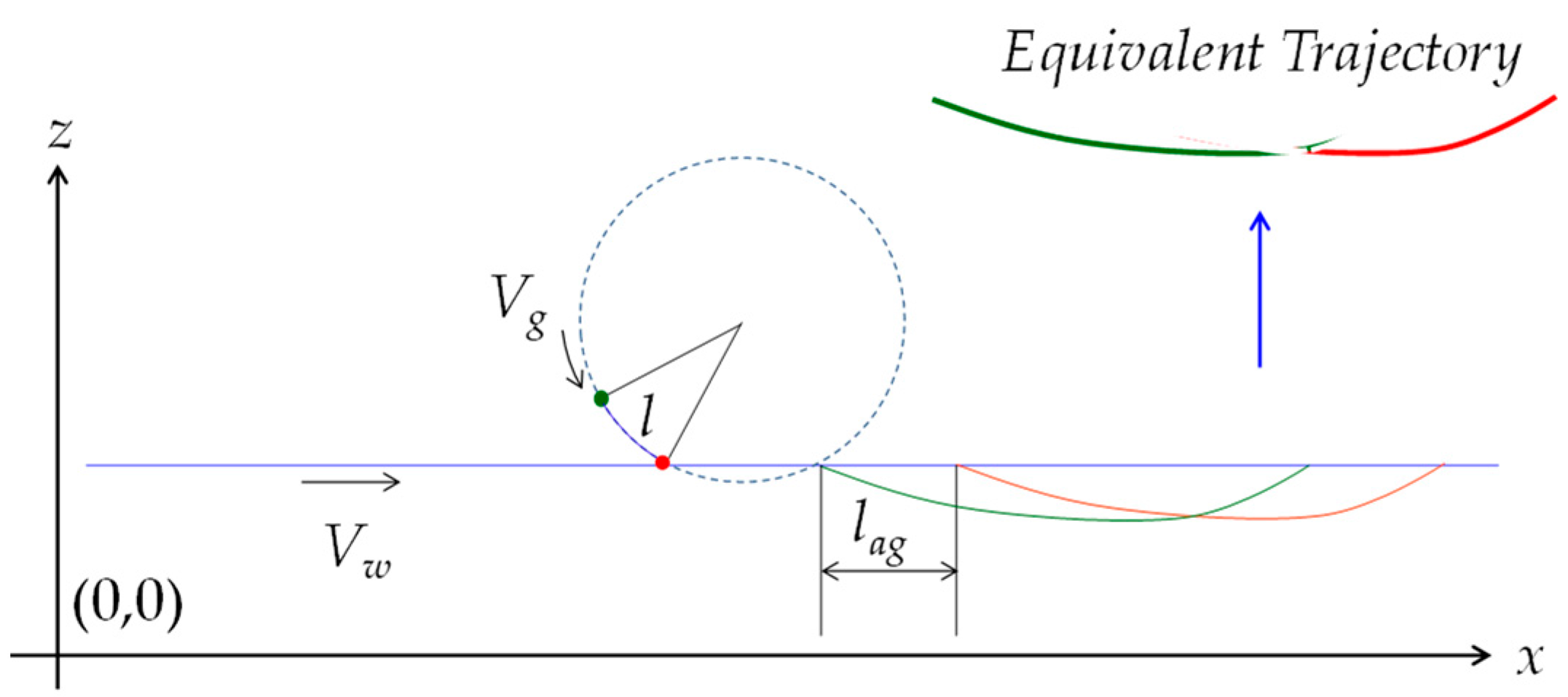
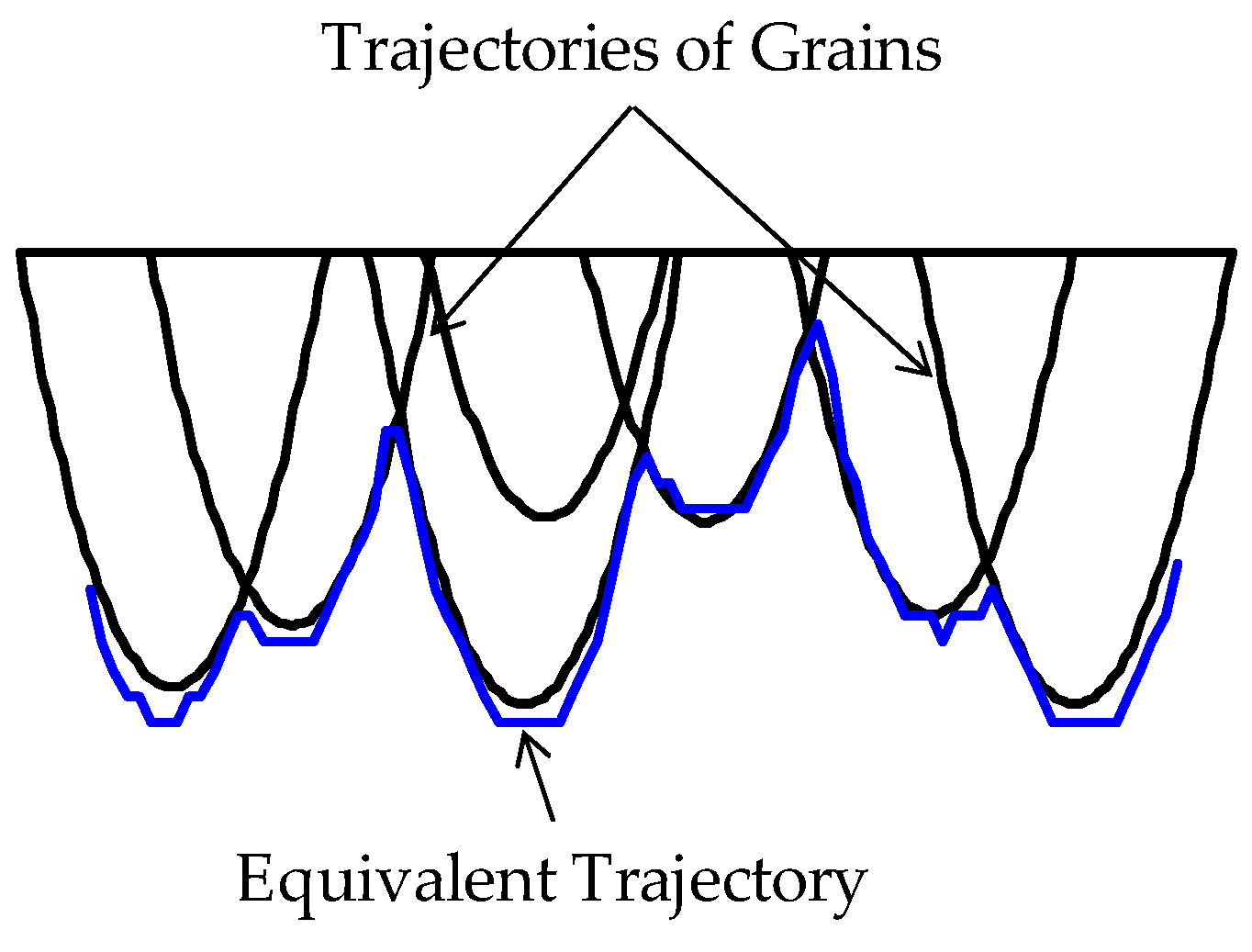
4.2. Effect of Two Consecutive Grains
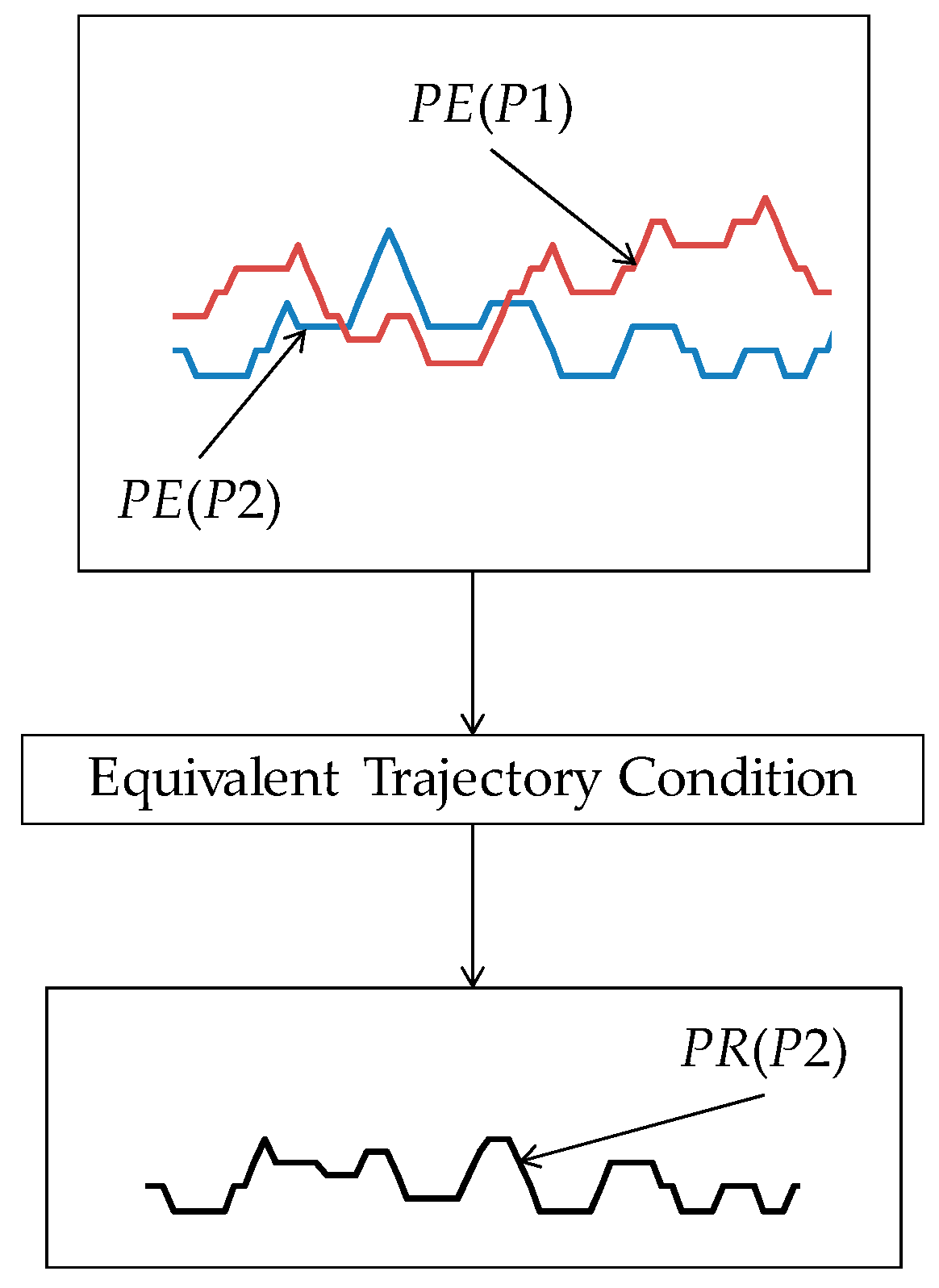
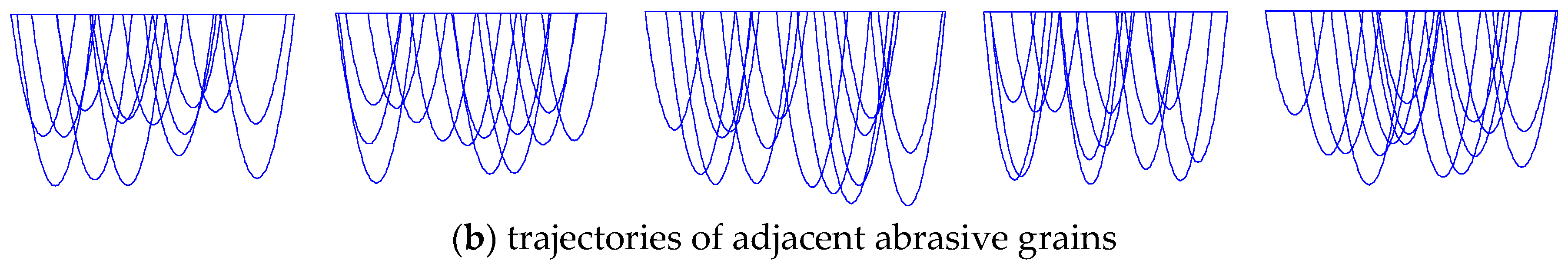
4.3. Effect of Several Grains
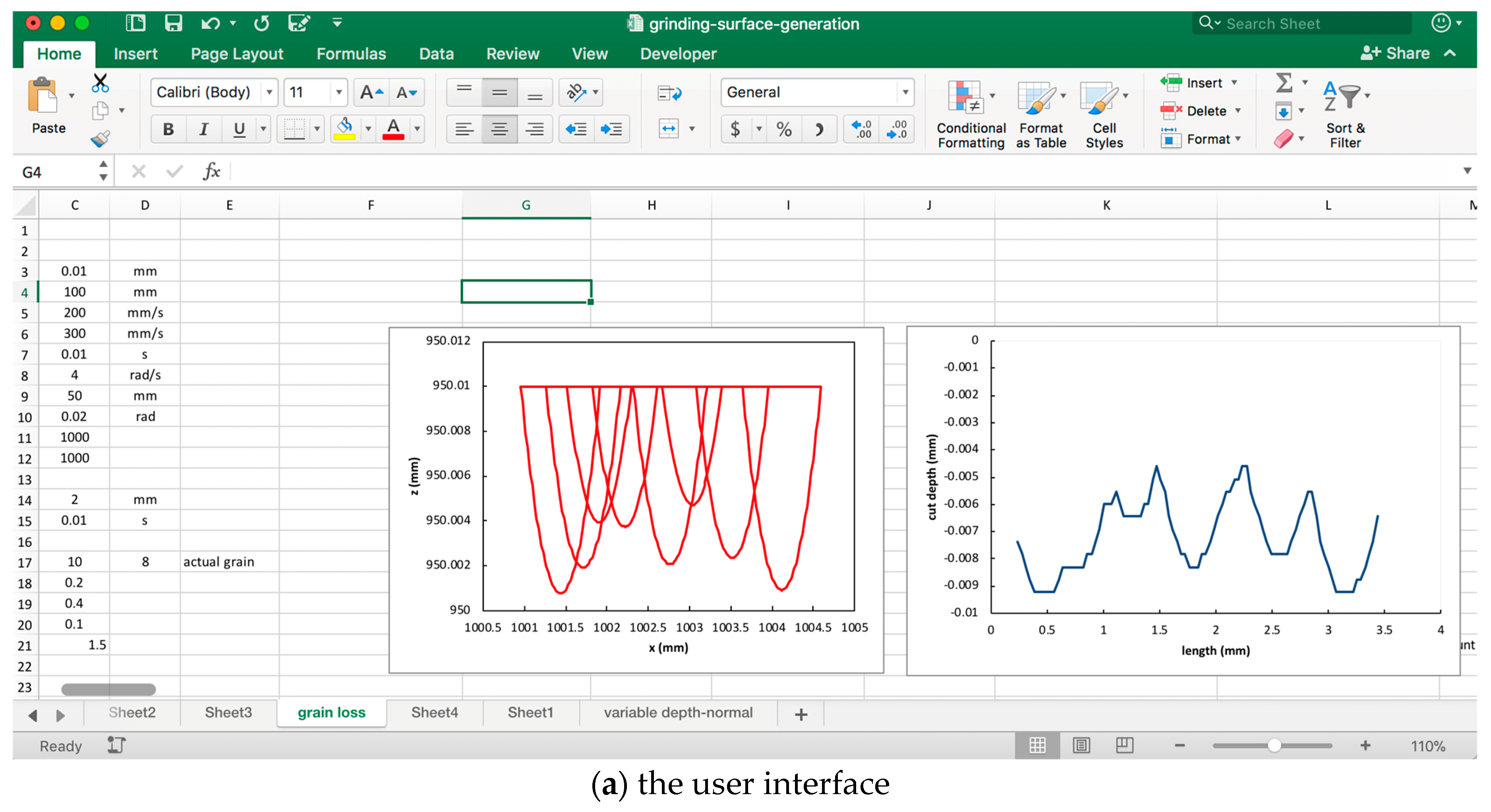
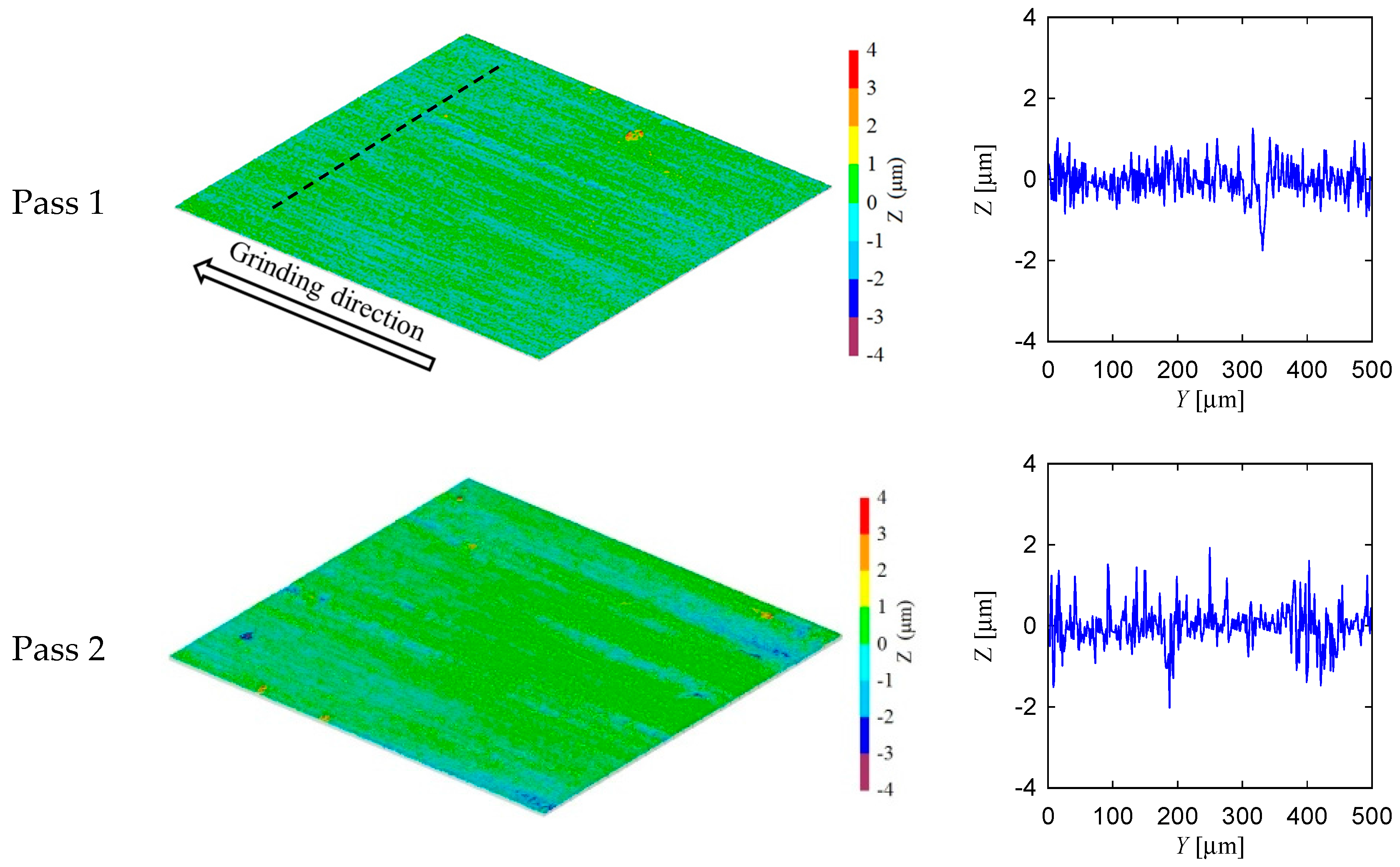
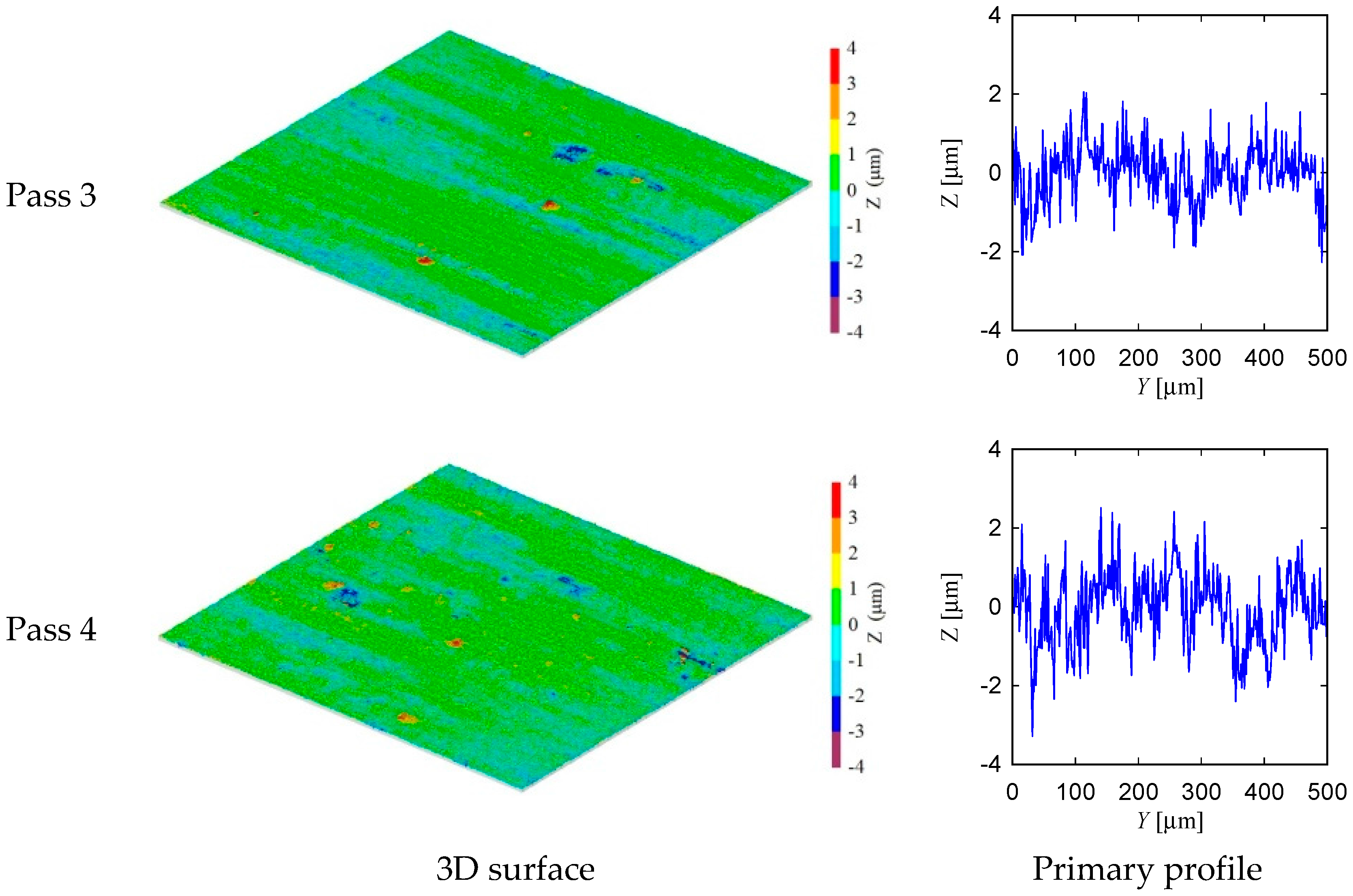
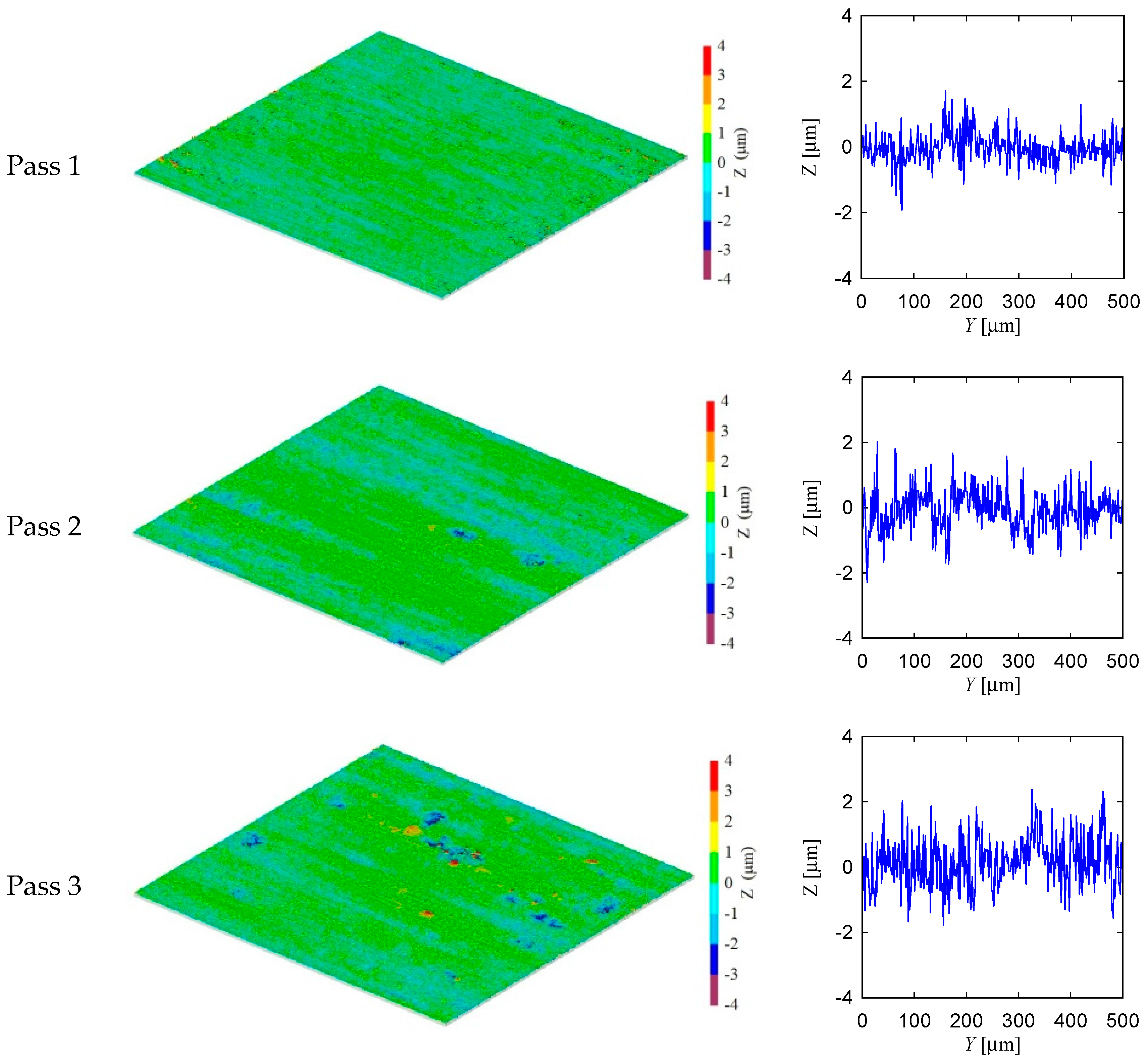
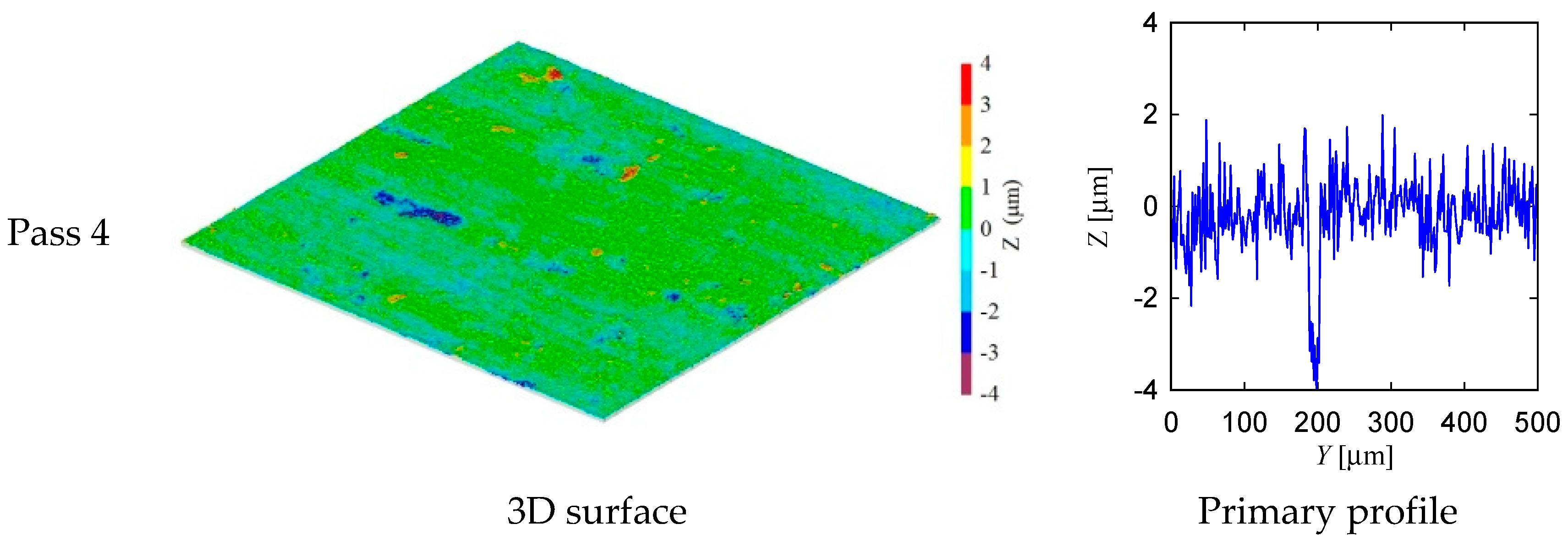
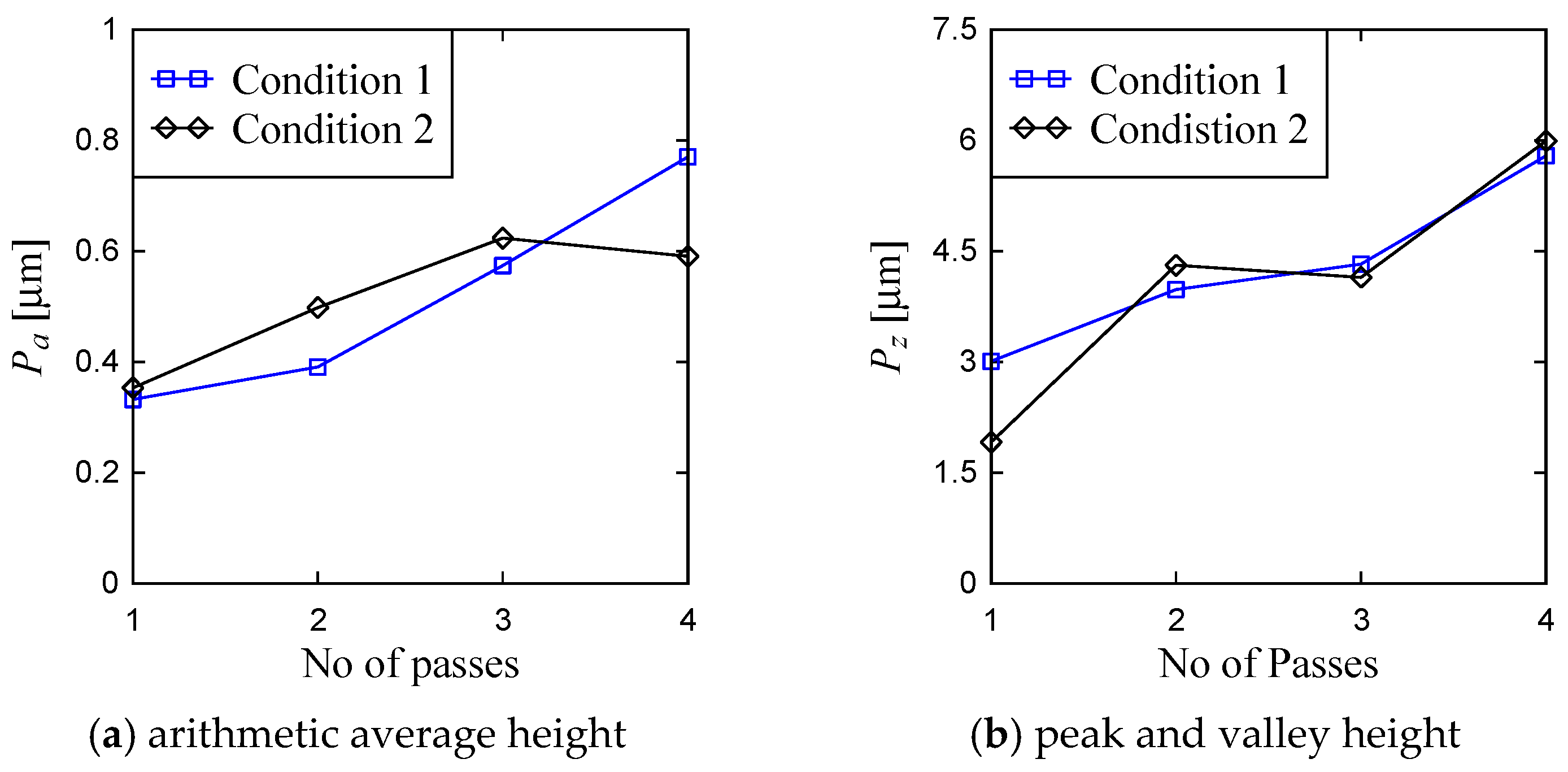
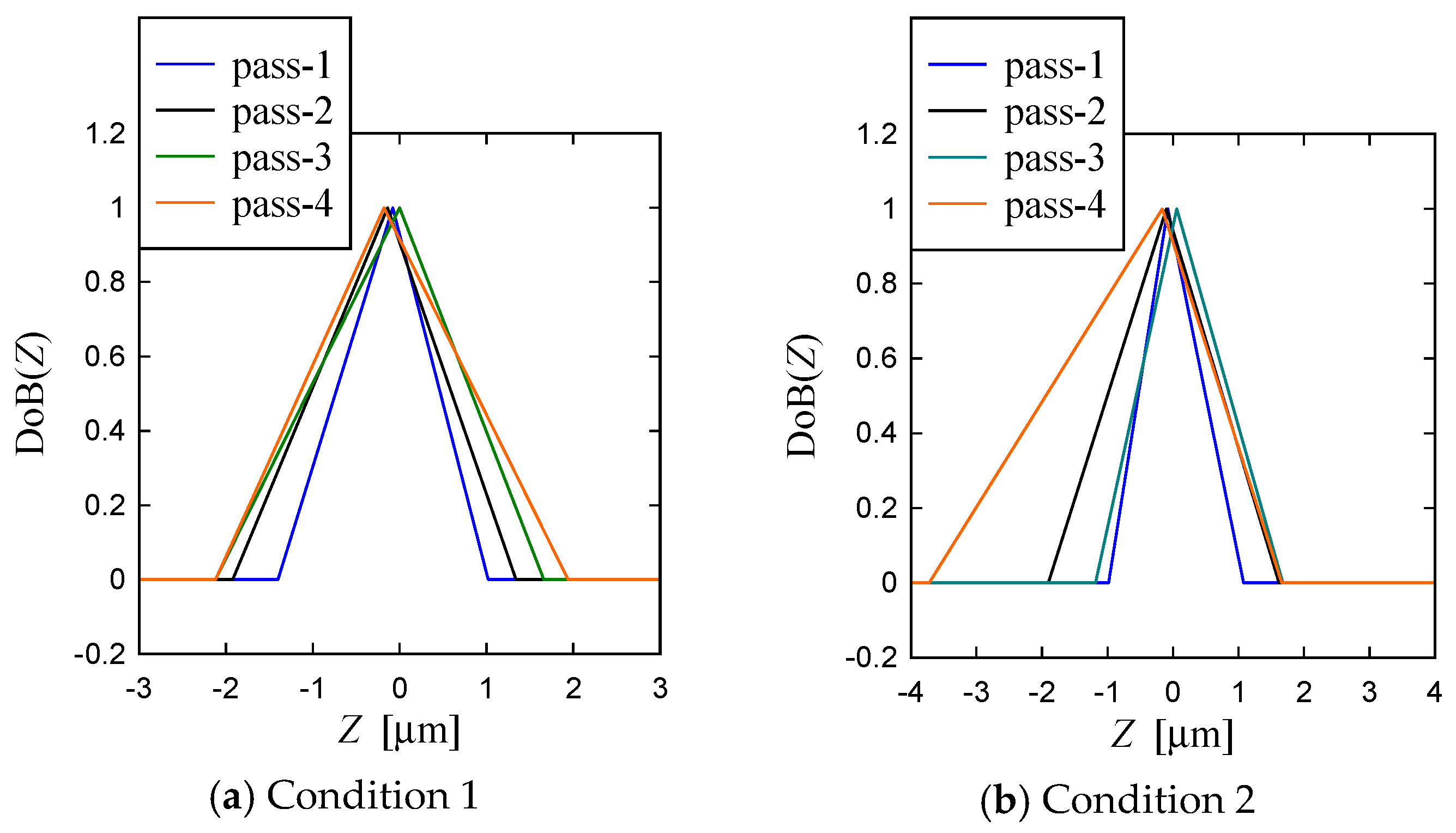
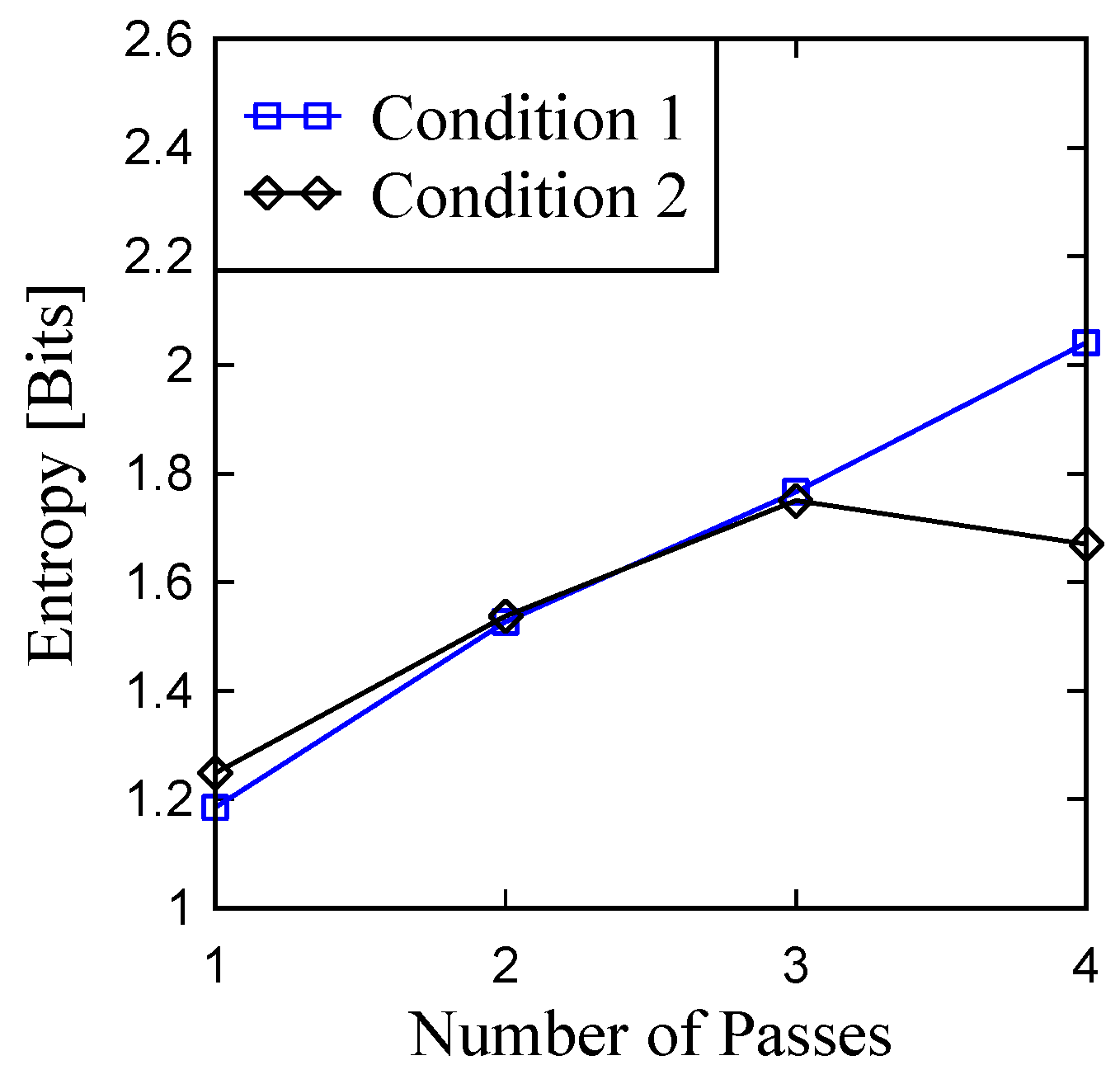
5. Experimental Investigation
6. Concluding Remarks
Authors Contributions
Conflicts of Interest
References
- Zhong, Z.W.; Venkatesh, V.C. Recent developments in grinding of advanced materials. Int. J. Adv. Manuf. Technol. 2009, 41, 468–480. [Google Scholar] [CrossRef]
- Tamaki, J.; Kubo, A.; Sharif Ullah, A.M.M. Wear Characteristics of Nano-polycrystalline Diamond Tool in Cutting of Tungsten Carbide. Int. J. Mechatron. Manuf. Syst. 2014, 7, 227–245. [Google Scholar]
- Gui, Y.; Zhao, J.; Chen, J.; Jiang, Y. Preparation and Characterization of Ni Spines Grown on the Surface of Cubic Boron Nitride Grains by Electroplating Method. Materials 2016, 9, 153. [Google Scholar] [CrossRef] [PubMed]
- Matsui, S.; Syoji, K. A Statistical Approach to Grinding Mechanism: Undeformed Chip Length in Grinding. J. Jpn. Soc. Precis. Eng. 1970, 36, 115–120. (In Japanese) [Google Scholar] [CrossRef]
- Matsui, S.; Syoji, K. Statistical Approach to Grinding Mechanism (2nd Report): Grain Depth of Cut in Grinding. J. Jpn. Soc. Precis. Eng. 1970, 36, 196–201. (In Japanese) [Google Scholar] [CrossRef]
- Matsui, S.; Syoji, K. Statistical Approach to Grinding Mechanism (3rd Report): On a Few Experiments. J. Jpn. Soc. Precis. Eng. 1971, 37, 708–714. (In Japanese) [Google Scholar] [CrossRef]
- Matsui, S. Statistical Approach to Grinding Mechanism: Theoretical Analysis in the Case where the Value of u/V is not negligible as compared with the Value 1. J. Jpn. Soc. Precis. Eng. 1980, 46, 298–304. [Google Scholar] [CrossRef]
- Matsui, S.; Tamaki, J. Influence of Elastic and Plastic Behaviors at the Grain Cutting Edge-Workpiece Contact Zone on Grinding Mechanism. J. Jpn. Soc. Precis. Eng. 1980, 46, 177–183. (In Japanese) [Google Scholar] [CrossRef]
- Shimada, K.; Liew, P.J.; Zhou, T.; Yan, J.; Kuriyagawa, T. Statistical Approach Optimizing Slant Feed Grinding. J. Adv. Mech. Des. Syst. Manuf. 2012, 6, 898–907. [Google Scholar] [CrossRef]
- Hecker, R.L.; Liang, S.Y. Predictive modeling of surface roughness in grinding. Int. J. Mach. Tools Manuf. 2003, 43, 755–761. [Google Scholar] [CrossRef]
- Agarwal, S.; Rao, P.V. A probabilistic approach to predict surface roughness in ceramic grinding. Int. J. Mach. Tools Manuf. 2005, 45, 609–616. [Google Scholar] [CrossRef]
- Nguyen, T.A.; Butler, D.L. Simulation of surface grinding process, Part 2: Interaction of the abrasive grain with the workpiece. Int. J. Mach. Tools Manuf. 2005, 45, 1329–1336. [Google Scholar] [CrossRef]
- Chakrabarti, S.; Paul, S. Numerical modelling of surface topography in superabrasive grinding. Int. J. Adv. Manuf. Technol. 2008, 39, 29–38. [Google Scholar] [CrossRef]
- Nguyen, A.T.; Butler, D.L. Correlation of grinding wheel topography and grinding performance: A study from a viewpoint of three-dimensional surface characterization. J. Mater. Process. Technol. 2008, 208, 14–23. [Google Scholar] [CrossRef]
- Oliveira, J.F.G.; Franҫa, T.V.; Wang, J.P. Experimental analysis of wheel/workpiece dynamic interactions in grinding. CIRP Ann. Manuf. Technol. 2008, 57, 329–332. [Google Scholar] [CrossRef]
- Li, X.; Rong, Y. Kinematics Simulation of Grinding Process Based on Virtual Wheel Model and Micro-Cutting Analysis. In Proceedings of the ASME 2009 International Manufacturing Science and Engineering Conference, West Lafayette, IN, USA, 4–7 October 2009; Volume 1, pp. 341–346. [Google Scholar]
- Heinzel, C.; Rickens, K. Engineered wheels for grinding of optical glass. CIRP Ann. Manuf. Technol. 2009, 58, 315–318. [Google Scholar] [CrossRef]
- Durgumahanti, U.S.P.; Singh, V.; Rao, P.V. A New Model for Grinding Force Prediction and Analysis. Int. J. Mach. Tools Manuf. 2010, 50, 231–240. [Google Scholar] [CrossRef]
- Stępień, P. Deterministic and stochastic components of regular surface texture generated by a special grinding process. Wear 2011, 271, 514–518. [Google Scholar] [CrossRef]
- Aurich, J.C.; Kirsch, B. Kinematic simulation of high-performance grinding for analysis of chip parameters of single grains. CIRP J. Manuf. Sci. Technol. 2012, 5, 164–174. [Google Scholar] [CrossRef]
- Jiang, J.; Ge, P.; Hong, J. Study on micro-interacting mechanism modeling in grinding process and ground surface roughness prediction. Int. J. Adv. Manuf. Technol. 2013, 67, 1035–1052. [Google Scholar] [CrossRef]
- Darafon, A.; Warkentin, A.; Bauer, R. 3D metal removal simulation to determine uncut chip thickness, contact length, and surface finish in grinding. Int. J. Adv. Manuf. Technol. 2013, 66, 1715–1724. [Google Scholar] [CrossRef]
- Sousa, F.J.P.; Hosse, D.S.; Reichenbach, I.; Aurich, J.C.; Seewig, J. Influence of kinematics and abrasive configuration on the grinding process of glass. J. Mater. Process. Technol. 2013, 213, 728–739. [Google Scholar] [CrossRef]
- Cao, Y.; Guan, J.; Li, B.; Chen, X.; Yang, J.; Gan, C. Modeling and simulation of grinding surface topography considering wheel vibration. Int. J. Adv. Manuf. Technol. 2013, 66, 937–945. [Google Scholar] [CrossRef]
- Chen, J.; Fang, Q.; Li, P. Effect of grinding wheel spindle vibration on surface roughness and subsurface damage in brittle material grinding. Int. J. Mach. Tools Manuf. 2015, 91, 12–23. [Google Scholar] [CrossRef]
- Osa, J.L.; Sánchez, J.A.; Ortega, N.; Iordanoff, I.; Charles, J.L. Discrete-element modelling of the grinding contact length combining the wheel-body structure and the surface-topography models. Int. J. Mach. Tools Manuf. 2016, 110, 43–54. [Google Scholar] [CrossRef]
- Wang, X.; Yu, T.; Dai, Y.; Shi, Y.; Wang, W. Kinematics modeling and simulating of grinding surface topography considering machining parameters and vibration characteristics. Int. J. Adv. Manuf. Technol. 2016, 87, 2459–2470. [Google Scholar] [CrossRef]
- McDonald, A.; Mohamed, A.-M.O.; Warkentin, A.; Bauer, R.J. Kinematic simulation of the uncut chip thickness and surface finish using a reduced set of 3D grinding wheel measurements. Precis. Eng. 2017, 49, 169–178. [Google Scholar] [CrossRef]
- Ding, W.; Dai, C.; Yu, T.; Xu, J.; Fu, Y. Grinding performance of textured monolayer CBN wheels: Undeformed chip thickness nonuniformity modeling and ground surface topography prediction. Int. J. Mach. Tools Manuf. 2017, 122, 66–80. [Google Scholar] [CrossRef]
- Ullah, A.M.M.S.; Chowdhury, M.A.K.; Kubo, A. A surface generation mechanism of grinding. Appl. Mech. Mater. 2017, 860, 13–18. [Google Scholar] [CrossRef]
- Jiang, J.L.; Ge, P.Q.; Bi, W.B.; Zhang, L.; Wang, D.X.; Zhang, Y. 2D/3D ground surface topography modeling considering dressing and wear effects in grinding process. Int. J. Mach. Tools Manuf. 2013, 74, 29–40. [Google Scholar] [CrossRef]
- Chowdhury, M.A.K.; Kubo, A.; Tamaki, J.; Ullah, A.M.M.S. Computer-aided Simulation of Rotary Diamond Dressing Based on Kinematic Analysis. J. Adv. Mech. Des. Syst. Manuf. 2013, 7, 506–520. [Google Scholar] [CrossRef]
- Kubo, A.; Tamaki, J.; Ullah, A.M.M.S. Kinematic analysis of dressing process using prismatic diamond rotary dresser. J. Jpn. Soc. Abras. Technol. 2015, 59, 197–204. (In Japanese) [Google Scholar]
- Ullah, A.M.M.S.; Shamsuzzaman, M. Fuzzy Monte Carlo Simulation using Point-Cloud Based Probability-Possibility Transformation. Simulation 2013, 89, 860–875. [Google Scholar] [CrossRef]
- Ullah, A.M.M.S.; Fuji, A.; Kubo, A.; Tamaki, J.; Kimura, M. On the surface metrology of bimetallic components. Mach. Sci. Technol. 2015, 19, 339–359. [Google Scholar] [CrossRef]
- Ullah, A.M.M.S. Surface Roughness Modeling Using Q-Sequence. Math. Comput. Appl. 2017, 22, 33. [Google Scholar] [CrossRef]
- Chowdhury, M.A.K.; Ullah, A.M.M.S.; Anwar, S. Drilling High Precision Holes in Ti6Al4V Using Rotary Ultrasonic Machining and Uncertainties Underlying Cutting Force, Tool Wear, and Production Inaccuracies. Materials 2017, 10, 1069. [Google Scholar] [CrossRef] [PubMed]
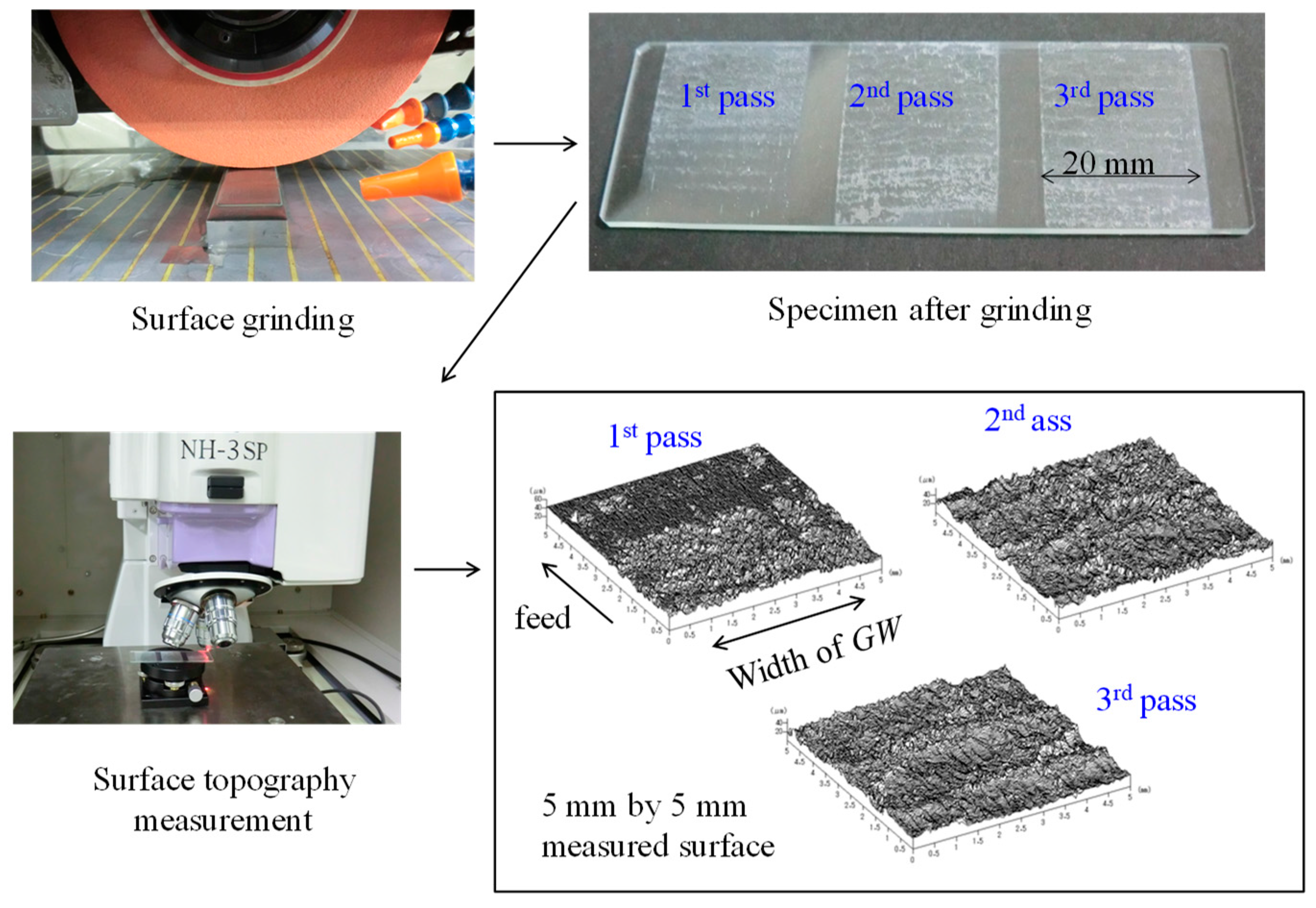

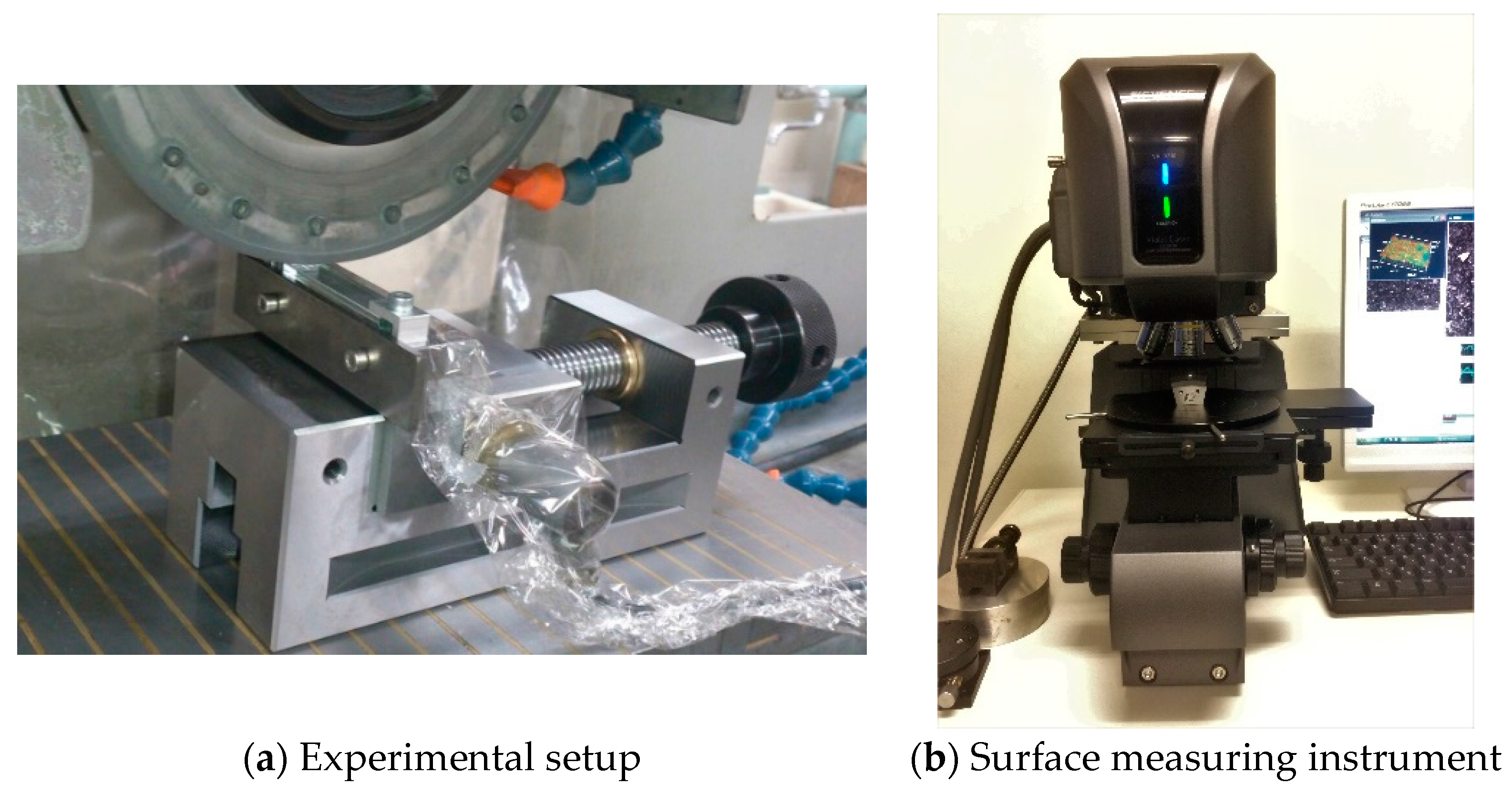
| Grinding Machine | Surface Grinding Machine, PSG-52DX made by Okamoto Machine Tool Works Ltd. (Gunma, Japan) |
| Grinding wheel | CBN170M100B Diameter D = 180 mm, Width B = 10 mm |
| Workpiece | Ordinary Glass |
| Grinding conditions | Rotational speed of grinding wheel, N = 300 rpm Workpiece speed (feed rate) Vw = 40, 100 mm/s Depth of cut, d = 6 μm Down cut |
| Coolant | Chemical Solution WS90 made by Yushiro Chemical Industry Co. Ltd. (Tokyo, Japan) |
| Measuring Instrument | Laser Scanning Microscope VK-9700 made by Keyence Corporation (Osaka, Japan) |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ullah, A.S.; Caggiano, A.; Kubo, A.; Chowdhury, M.A.K. Elucidating Grinding Mechanism by Theoretical and Experimental Investigations. Materials 2018, 11, 274. https://doi.org/10.3390/ma11020274
Ullah AS, Caggiano A, Kubo A, Chowdhury MAK. Elucidating Grinding Mechanism by Theoretical and Experimental Investigations. Materials. 2018; 11(2):274. https://doi.org/10.3390/ma11020274
Chicago/Turabian StyleUllah, AMM Sharif, Alessandra Caggiano, Akihiko Kubo, and M. A. K. Chowdhury. 2018. "Elucidating Grinding Mechanism by Theoretical and Experimental Investigations" Materials 11, no. 2: 274. https://doi.org/10.3390/ma11020274
APA StyleUllah, A. S., Caggiano, A., Kubo, A., & Chowdhury, M. A. K. (2018). Elucidating Grinding Mechanism by Theoretical and Experimental Investigations. Materials, 11(2), 274. https://doi.org/10.3390/ma11020274







