Free Vibration Analysis of Moderately Thick Orthotropic Functionally Graded Plates with General Boundary Restraints
Abstract
:1. Introduction
2. Theoretical Formulation
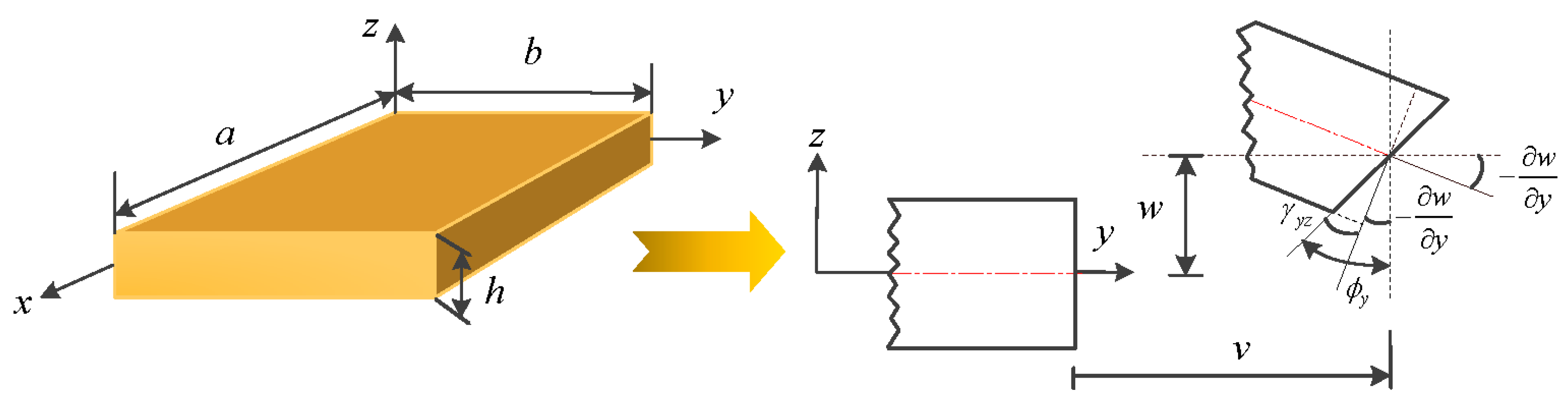
2.1. Model Description
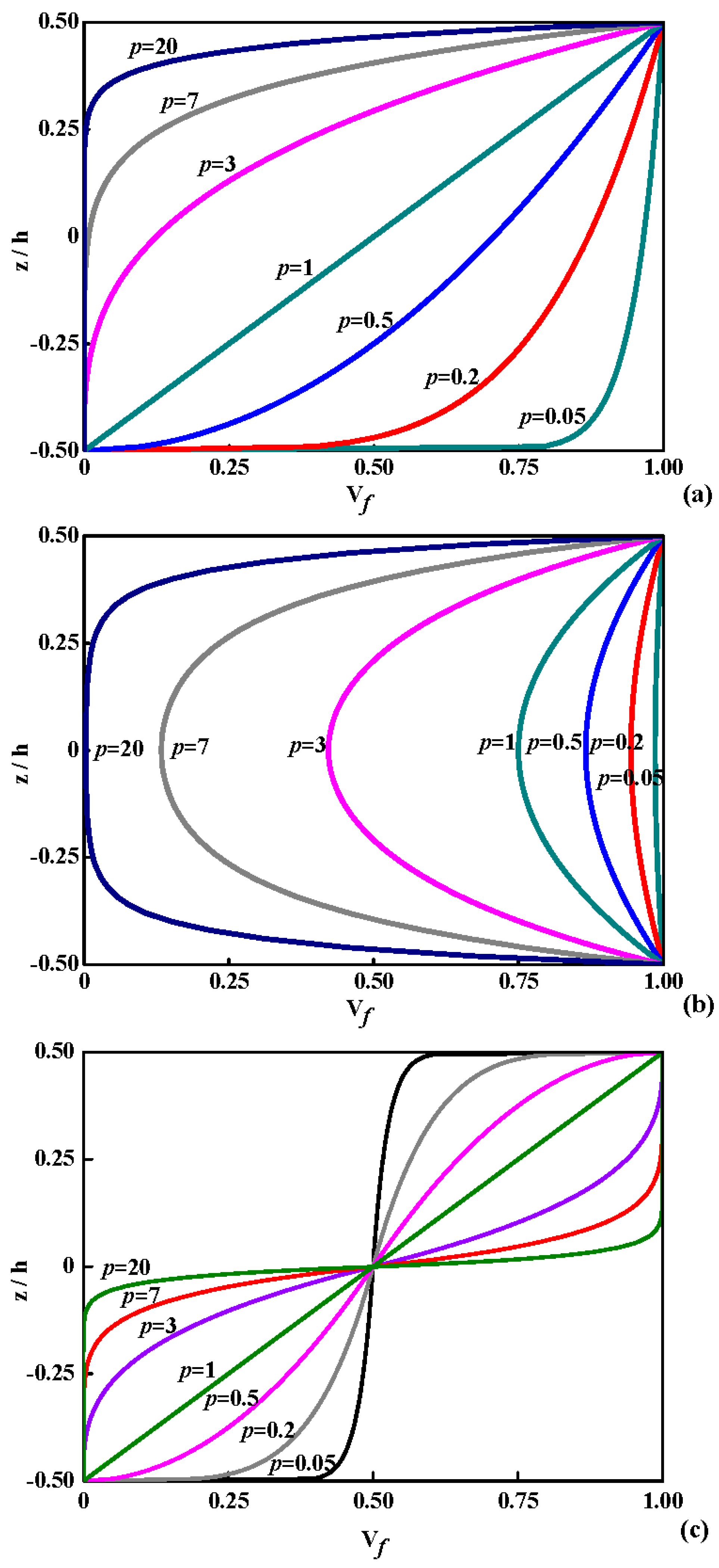
2.2. Material Properties
2.3. Stress–Strain Relations and Stress Resultants
2.4. Energy Functions
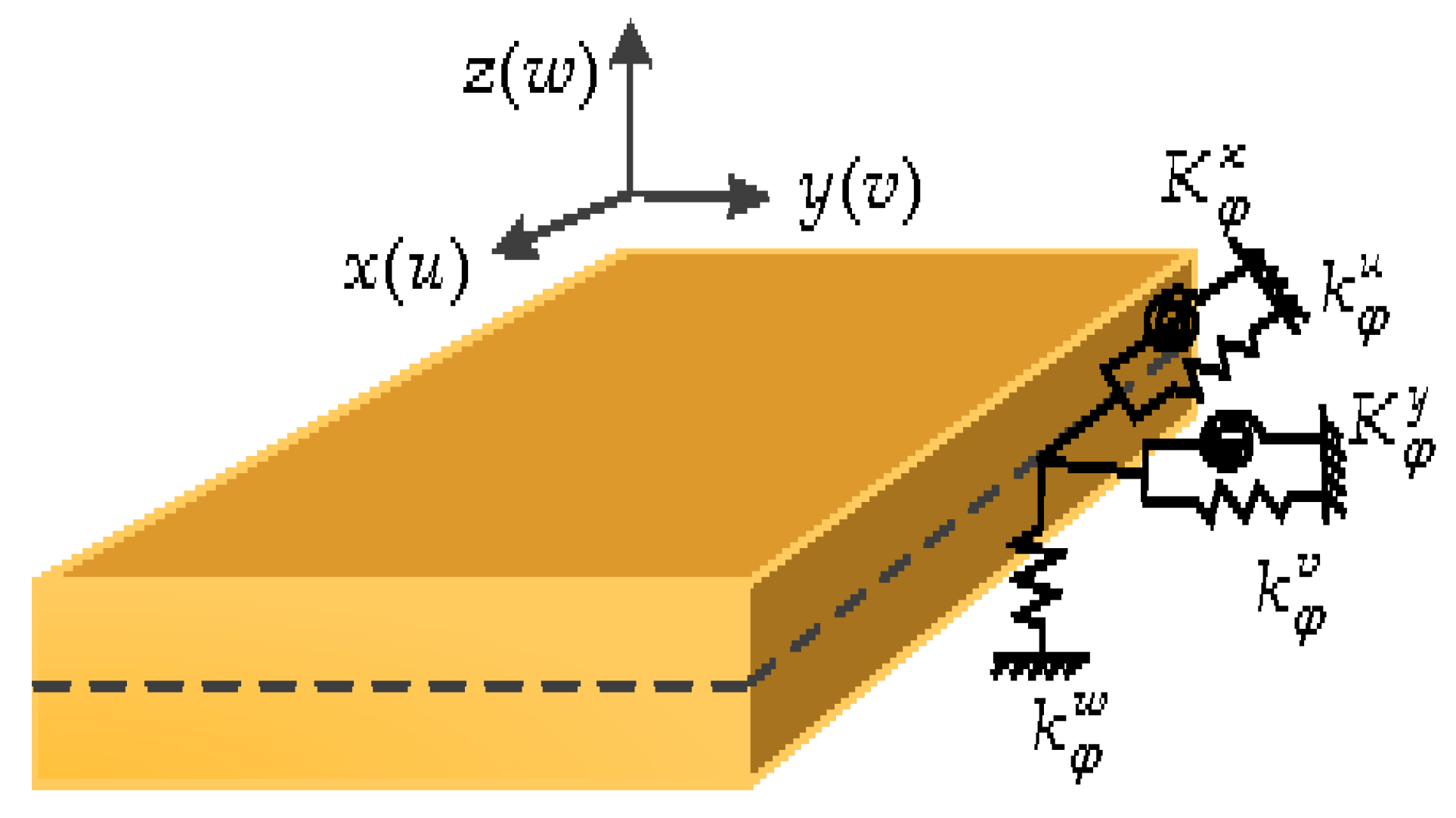
2.5. Governing Equations and Boundary Restraints
2.6. Admissible Displacement Functions
3. Numerical Results and Discussion
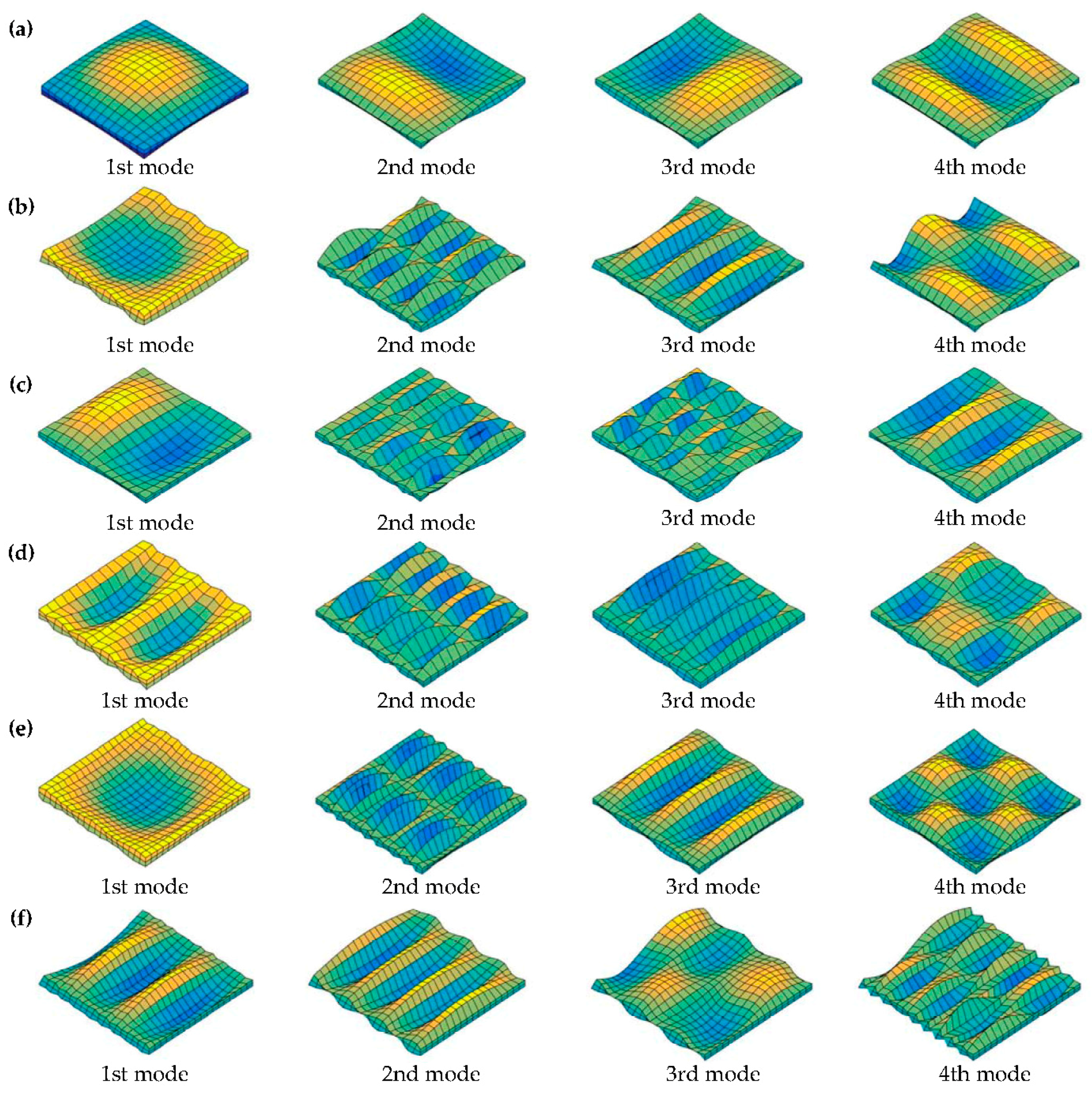
3.1. OFG Plates with General Boundary Restraints
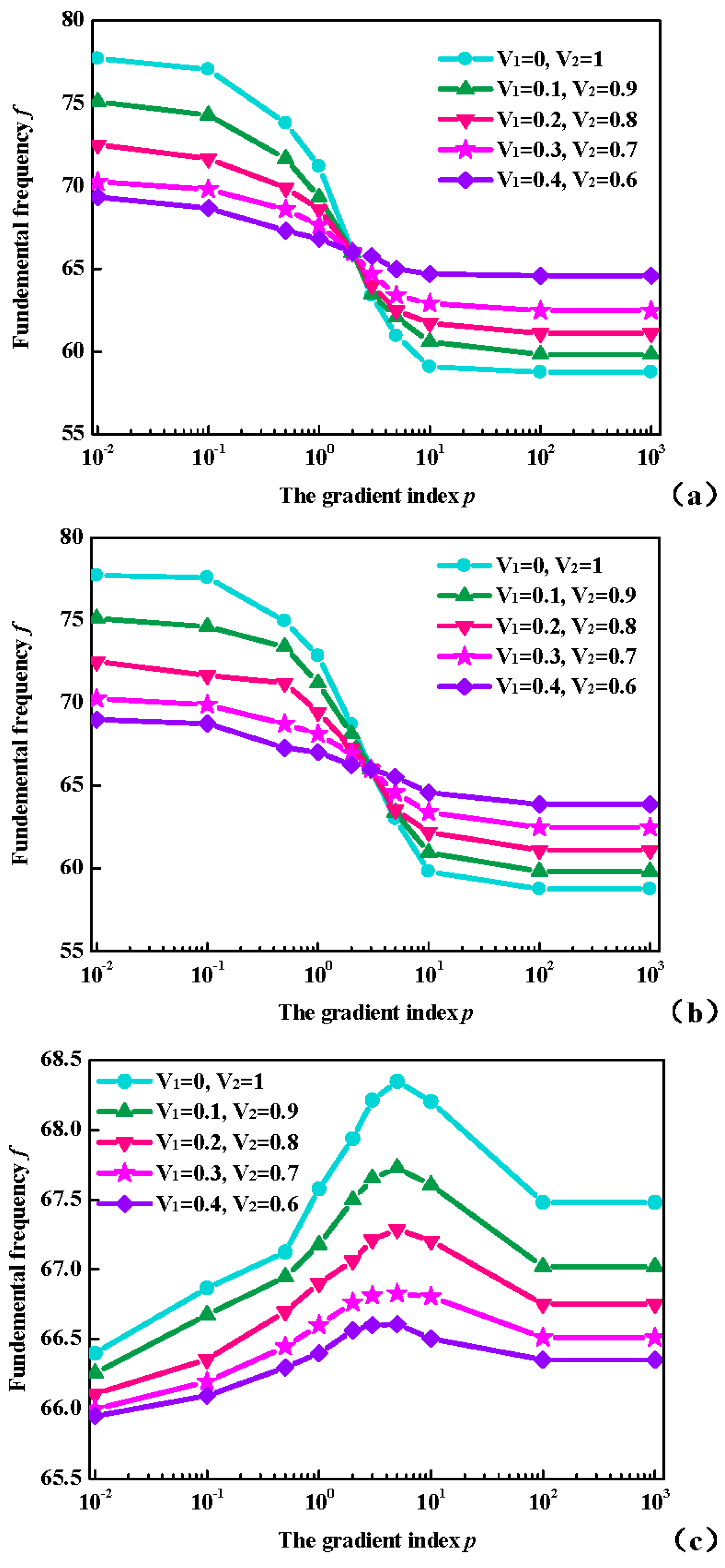
3.2. Volume Fraction Analysis
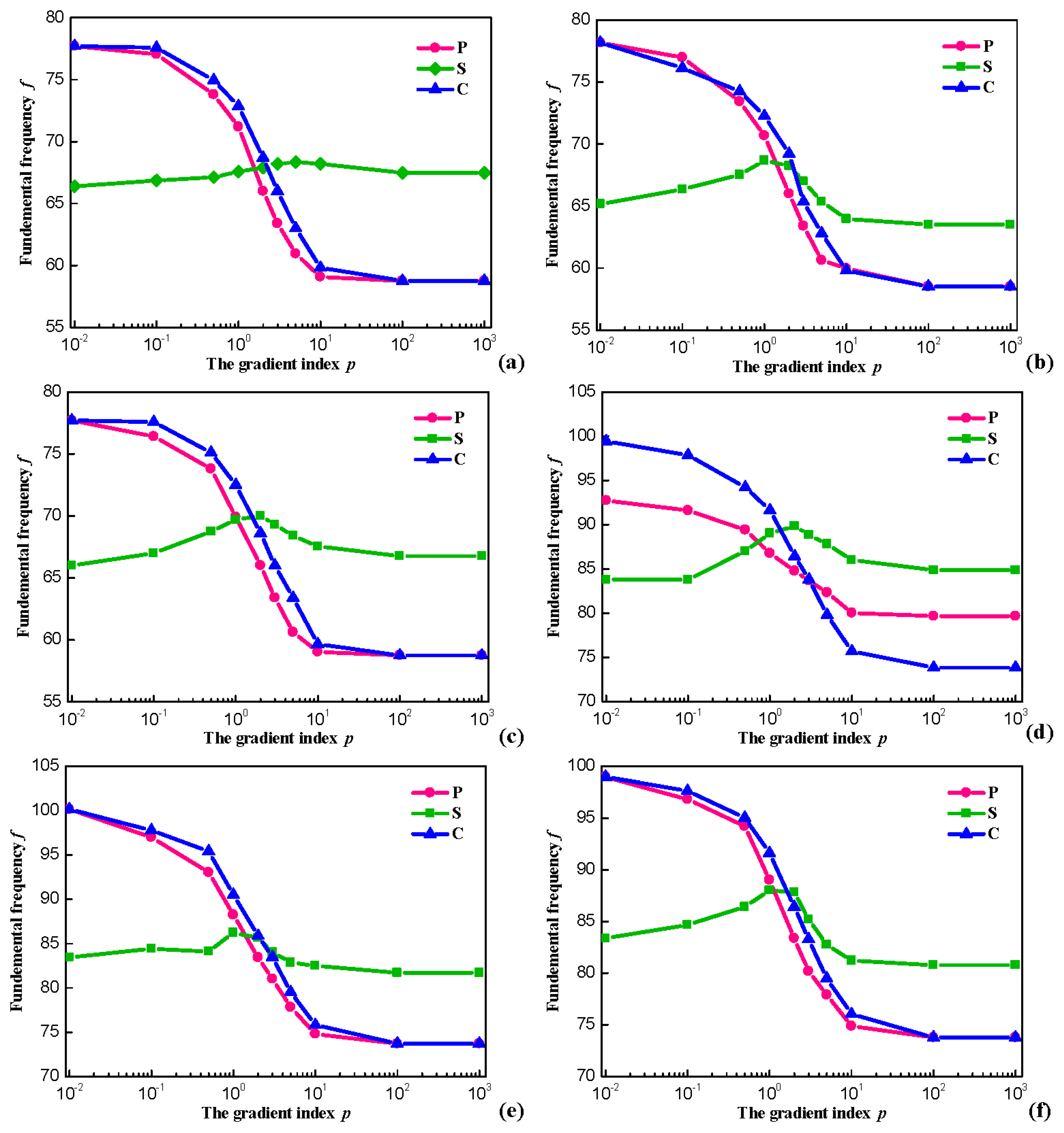
3.3. Fundamental Frequencies Analysis
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
References
- Xiao, H.T.; Yue, Z.Q. Stresses and Displacements in Functionally Graded Materials of Semi-Infinite Extent Induced by Rectangular Loadings. Materials 2012, 5, 210–226. [Google Scholar] [CrossRef] [PubMed]
- Lima, D.D.; Mantri, S.A.; Mikler, C.V.; Contieri, R.; Yannetta, C.J.; Campo, K.N.; Lopes, E.S.; Styles, M.J.; Borkar, T.; Caram, R.; et al. Laser additive processing of a functionally graded internal fracture fixation plate. Mater. Des. 2017, 130, 8–15. [Google Scholar] [CrossRef]
- Shi, Z.Y.; Yao, X.L.; Pang, F.Z.; Wang, Q.S. An exact solution for the free-vibration analysis of functionally graded carbon-nanotube-reinforced composite beams with arbitrary boundary conditions. Sci. Rep. 2017, 7, 18. [Google Scholar] [CrossRef] [PubMed]
- Tornabene, F.; Fantuzzi, N.; Bacciocchi, M. Linear Static Behavior of Damaged Laminated Composite Plates and Shells. Materials 2017, 10, 811. [Google Scholar] [CrossRef] [PubMed]
- Sekkal, M.; Fahsi, B.; Tounsi, A.; Mahmoud, S.R. A novel and simple higher order shear deformation theory for stability and vibration of functionally graded sandwich plate. Steel Compos. Struct. 2017, 25, 389–401. [Google Scholar]
- Shabana, Y.M.; Noda, N. Thermo-elasto-plastic stresses of functionally graded material plate with a substrate and a coating. J. Therm. Stress. 2002, 25, 1133–1146. [Google Scholar] [CrossRef]
- Chen, Y.M.; Chen, G.; Xie, X.P. Weak Galerkin finite element method for Biot's consolidation problem. J. Comput. Appl. Math. 2018, 330, 398–416. [Google Scholar] [CrossRef]
- Sidhoum, I.A.; Boutchicha, D.; Benyoucef, S.; Tounsi, A. An original HSDT for free vibration analysis of functionally graded plates. Steel Compos. Struct. 2017, 25, 735–745. [Google Scholar]
- Dinh, D.N.; Nguyen, P.D. The Dynamic Response and Vibration of Functionally Graded Carbon Nanotube-Reinforced Composite (FG-CNTRC) Truncated Conical Shells Resting on Elastic Foundations. Materials 2017, 10, 1194. [Google Scholar] [CrossRef] [PubMed]
- Fallah, A.; Aghdam, M.M.; Kargarnovin, M.H. Free vibration analysis of moderately thick functionally graded plates on elastic foundation using the extended Kantorovich method. Arch. Appl. Mech. 2013, 83, 177–191. [Google Scholar] [CrossRef]
- Zhao, X.; Lee, Y.Y.; Liew, K.M. Free vibration analysis of functionally graded plates using the element-free kp-Ritz method. J. Sound Vib. 2009, 319, 918–939. [Google Scholar] [CrossRef]
- Dong, C.Y. Three-dimensional free vibration analysis of functionally graded annular plates using the Chebyshev-Ritz method. Mater. Des. 2008, 29, 1518–1525. [Google Scholar] [CrossRef]
- Ye, T.G.; Jin, G.Y.; Su, Z.; Jia, X.Z. A unified Chebyshev-Ritz formulation for vibration analysis of composite laminated deep open shells with arbitrary boundary conditions. Arch. Appl. Mech. 2014, 84, 441–471. [Google Scholar] [CrossRef]
- Vel, S.S.; Batra, R.C. Three-dimensional exact solution for the vibration of functionally graded rectangular plates. J. Sound Vib. 2004, 272, 703–730. [Google Scholar] [CrossRef]
- Ferreira, A.J.M.; Batra, R.C.; Roque, C.M.C.; Qian, L.F.; Jorge, R.M.N. Natural frequencies of functionally graded plates by a meshless method. Compos. Struct. 2006, 75, 593–600. [Google Scholar] [CrossRef]
- Ferreira, A.J.M.; Batra, R.C.; Roque, C.M.C.; Qian, L.F.; Martins, P. Static analysis of functionally graded plates using third-order shear deformation theory and a meshless method. Compos. Struct. 2005, 69, 449–457. [Google Scholar] [CrossRef]
- Vaghefi, R.; Baradaran, G.H.; Koohkan, H. Three-dimensional static analysis of thick functionally graded plates by using meshless local Petrov-Galerkin (MLPG) method. Eng. Anal. Bound. Elem. 2010, 34, 564–573. [Google Scholar] [CrossRef]
- Naskar, T.; Kumar, J. Predominant modes for Rayleigh wave propagation using the dynamic stiffness matrix approach. J. Geophys. Eng. 2017, 14, 1032–1041. [Google Scholar] [CrossRef]
- Rouzegar, J.; Abbasi, A. A refined finite element method for bending of smart functionally graded plates. Thin Walled Struct. 2017, 120, 386–396. [Google Scholar] [CrossRef]
- Nikbakht, S.; Salami, S.J.; Shakeri, M. Three dimensional analysis of functionally graded plates up to yielding, using full layer-wise finite element method. Compos. Struct. 2017, 182, 99–115. [Google Scholar] [CrossRef]
- Chi, S.H.; Chung, Y.L. Mechanical behavior of functionally graded material plates under transverse load—Part I: Analysis. Int. J. Solids Struct. 2006, 43, 3657–3674. [Google Scholar] [CrossRef]
- Chi, S.H.; Chung, Y.L. Mechanical behavior of functionally graded material plates under transverse load—Part II: Numerical results. Int. J. Solids Struct. 2006, 43, 3675–3691. [Google Scholar] [CrossRef]
- Qian, L.F.; Batra, R.C.; Chen, L.M. Static and dynamic deformations of thick functionally graded elastic plates by using higher-order shear and normal deformable plate theory and meshless local Petrov-Galerkin method. Compos. Part B Eng. 2004, 35, 685–697. [Google Scholar] [CrossRef]
- Liu, D.Y.; Wang, C.Y.; Chen, W.Q. Free vibration of FGM plates with in-plane material inhomogeneity. Compos. Struct. 2010, 92, 1047–1051. [Google Scholar] [CrossRef]
- Ramirez, F.; Heyliger, P.R.; Pan, E. Static analysis of functionally graded elastic anisotropic plates using a discrete layer approach. Compos. Part B Eng. 2006, 37, 10–20. [Google Scholar] [CrossRef]
- Zhang, W.; Yang, J.; Hao, Y.X. Chaotic vibrations of an orthotropic FGM rectangular plate based on third-order shear deformation theory. Nonlinear Dyn. 2010, 59, 619–660. [Google Scholar] [CrossRef]
- Huang, M.; Ma, X.Q.; Sakiyama, T.; Matuda, H.; Morita, C. Free vibration analysis of orthotropic rectangular plates with variable thickness and general boundary conditions. J. Sound Vib. 2005, 288, 931–955. [Google Scholar] [CrossRef]
- Li, W.L. Comparison of Fourier sine and cosine series expansions for beams with arbitrary boundary conditions. J. Sound Vib. 2002, 255, 185–194. [Google Scholar] [CrossRef]
- Li, W.L.; Zhang, X.F.; Du, J.T.; Liu, Z.G. An exact series solution for the transverse vibration of rectangular plates with general elastic boundary supports. J. Sound Vib. 2009, 321, 254–269. [Google Scholar] [CrossRef]
- Wang, Q.S.; Shi, D.Y.; Liang, Q.; Shi, X.J. A unified solution for vibration analysis of functionally graded circular, annular and sector plates with general boundary conditions. Compos. Part B Eng. 2016, 88, 264–294. [Google Scholar] [CrossRef]
- Ye, T.G.; Jin, G.Y.; Shi, S.X.; Ma, X.L. Three-dimensional free vibration analysis of thick cylindrical shells with general end conditions and resting on elastic foundations. Int. J. Mech. Sci. 2014, 84, 120–137. [Google Scholar] [CrossRef]
- Shi, D.Y.; Wang, Q.S.; Shi, X.J.; Pang, F.Z. A series solution for the in-plane vibration analysis of orthotropic rectangular plates with non-uniform elastic boundary constraints and internal line supports. Arch. Appl. Mech. 2015, 85, 51–73. [Google Scholar] [CrossRef]
- Xing, Y.F.; Liu, B. Exact solutions for the free in-plane vibrations of rectangular plates. Int. J. Mech. Sci. 2009, 51, 246–255. [Google Scholar] [CrossRef]
- Su, Z.; Jin, G.Y.; Ye, T.G. Vibration analysis and transient response of a functionally graded piezoelectric curved beam with general boundary conditions. Smart Mater. Struct. 2016, 25, 065003. [Google Scholar] [CrossRef]
- Jin, G.Y.; Ye, T.G.; Shi, S.X. Three-Dimensional Vibration Analysis of Isotropic and Orthotropic Open Shells and Plates with Arbitrary Boundary Conditions. Shock Vib. 2015, 2015, 896204. [Google Scholar] [CrossRef]
- Chen, Y.H.; Jin, G.Y.; Liu, Z.G. Free vibration analysis of circular cylindrical shell with non-uniform elastic boundary constraints. Int. J. Mech. Sci. 2013, 74, 120–132. [Google Scholar] [CrossRef]
- Dai, L.; Yang, T.J.; Du, J.T.; Li, W.L.; Brennan, M.J. An exact series solution for the vibration analysis of cylindrical shells with arbitrary boundary conditions. Appl. Acoust. 2013, 74, 440–449. [Google Scholar] [CrossRef]
- Zenkour, A.M. Generalized shear deformation theory for bending analysis of functionally graded plates. Appl. Math. Modell. 2006, 30, 67–84. [Google Scholar] [CrossRef]
- Hachemi, H.; Kaci, A.; Houari, M.S.A.; Bourada, M.; Tounsi, A.; Mahmoud, S.R. A new simple three-unknown shear deformation theory for bending analysis of FG plates resting on elastic foundations. Steel Compos. Struct. 2017, 25, 717–726. [Google Scholar]
- Erber, T. Hooke’s law and fatigue limits in micromechanics. Eur. J. Phys. 2001, 22, 491–499. [Google Scholar] [CrossRef]
- Gallistl, D.; Huber, P.; Peterseim, D. On the stability of the Rayleigh-Ritz method for eigenvalues. Numer. Math. 2017, 137, 339–351. [Google Scholar] [CrossRef]
| Metal (Al) | Ceramic (ZrO2) | |||||
|---|---|---|---|---|---|---|
| Properties | (GPa) | (kg/m3) | (GPa) | (kg/m3) | ||
| 70 | 0.3 | 2702 | 200 | 0.3 | 5700 | |
| h/a | Method | Mode Number | ||||
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | ||
| CCCC boundary restraint | ||||||
| 0.1 | Present | 12.767 | 13.242 | 14.454 | 16.649 | 19.937 |
| Ref. [35] | 12.767 | 13.243 | 14.451 | 16.647 | 19.938 | |
| 0.2 | Present | 7.5324 | 8.0879 | 9.3818 | 10.206 | 11.430 |
| Ref. [35] | 7.5325 | 8.0882 | 9.3822 | 10.210 | 11.435 | |
| 0.3 | Present | 5.2981 | 5.8807 | 6.8086 | 7.0848 | 8.7976 |
| Ref. [35] | 5.2982 | 5.8807 | 6.8086 | 7.0848 | 8.7975 | |
| SSSS boundary restraint | ||||||
| 0.1 | Present | 8.2283 | 8.3304 | 8.8058 | 10.181 | 12.615 |
| Ref. [35] | 8.2286 | 8.3304 | 8.8058 | 10.182 | 12.616 | |
| 0.2 | Present | 4.1653 | 6.0783 | 6.5920 | 7.8472 | 8.3304 |
| Ref. [35] | 4.1652 | 6.0783 | 6.5922 | 7.8472 | 8.3304 | |
| 0.3 | Present | 2.7768 | 4.6194 | 5.1094 | 5.5535 | 5.5535 |
| Ref. [35] | 2.7768 | 4.6197 | 5.1096 | 5.5536 | 5.5536 | |
| FFFF boundary restraint | ||||||
| 0.1 | Present | 1.2016 | 1.6451 | 3.2673 | 3.5279 | 5.8824 |
| Ref. [35] | 1.2016 | 1.6450 | 3.2673 | 3.5278 | 5.8822 | |
| 0.2 | Present | 1.1741 | 1.5491 | 3.0822 | 3.2398 | 3.9205 |
| Ref. [35] | 1.1742 | 1.5490 | 3.0822 | 3.2398 | 3.9204 | |
| 0.3 | Present | 1.1337 | 1.4422 | 2.6131 | 2.8420 | 2.9385 |
| Ref. [35] | 1.1337 | 1.4422 | 2.6132 | 2.8421 | 2.9387 | |
| Mode | p = 0 | p = 5 | p = | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 9 × 9 | 11 × 11 | 9 × 9 | 11 × 11 | 9 × 9 | 11 × 11 | |||||||
| Present | TSDT [15] | Present | TSDT [15] | Present | TSDT [15] | Present | TSDT [15] | Present | TSDT [15] | Present | TSDT [15] | |
| CCCC boundary restraint | ||||||||||||
| 1 | 0.3598 | 0.3596 | 0.3597 | 0.3598 | 0.3148 | 0.3151 | 0.3154 | 0.3154 | 0.3086 | 0.3090 | 0.3089 | 0.3092 |
| 2 | 0.6262 | 0.6263 | 0.6282 | 0.6282 | 0.5440 | 0.5441 | 0.5456 | 0.5458 | 0.5379 | 0.5382 | 0.5396 | 0.5398 |
| 3 | 0.6262 | 0.6263 | 0.6282 | 0.6282 | 0.5440 | 0.5441 | 0.5456 | 0.5458 | 0.5379 | 0.5382 | 0.5396 | 0.5398 |
| 4 | 0.8462 | 0.8462 | 0.8486 | 0.8486 | 0.7336 | 0.7338 | 0.7357 | 0.7358 | 0.7270 | 0.7272 | 0.7290 | 0.7291 |
| 5 | 0.8697 | 0.8696 | 0.8687 | 0.8687 | 0.7595 | 0.7597 | 0.7590 | 0.7590 | 0.7471 | 0.7472 | 0.7462 | 0.7464 |
| 6 | 0.8697 | 0.8696 | 0.8687 | 0.8687 | 0.7595 | 0.7597 | 0.7590 | 0.7590 | 0.7471 | 0.7472 | 0.7462 | 0.7464 |
| SSSS boundary restraint | ||||||||||||
| 1 | 0.2463 | 0.2467 | 0.2465 | 0.2462 | 0.2244 | 0.2240 | 0.2236 | 0.2236 | 0.2120 | 0.2120 | 0.2117 | 0.2116 |
| 2 | 0.4472 | 0.4474 | 0.4485 | 0.4483 | 0.3916 | 0.3914 | 0.3920 | 0.3921 | 0.3846 | 0.3845 | 0.3854 | 0.3852 |
| 3 | 0.4472 | 0.4476 | 0.4485 | 0.4484 | 0.3916 | 0.3915 | 0.3920 | 0.3922 | 0.3846 | 0.3846 | 0.3854 | 0.3853 |
| 4 | 0.5405 | 0.5407 | 0.5398 | 0.5397 | 0.4866 | 0.4869 | 0.4862 | 0.4861 | 0.4647 | 0.4646 | 0.4641 | 0.4638 |
| 5 | 0.5405 | 0.5408 | 0.5398 | 0.5398 | 0.4866 | 0.4869 | 0.4862 | 0.4861 | 0.4647 | 0.4647 | 0.4641 | 0.4638 |
| 6 | 0.6505 | 0.6509 | 0.6465 | 0.6470 | 0.5691 | 0.5693 | 0.5661 | 0.5659 | 0.5595 | 0.5593 | 0.5562 | 0.5560 |
| CFCF boundary restraint | ||||||||||||
| 1 | 0.2379 | 0.2383 | 0.2358 | 0.2362 | 0.2087 | 0.2085 | 0.2101 | 0.2103 | 0.2054 | 0.2055 | 0.2041 | 0.2044 |
| 2 | 0.2608 | 0.2611 | 0.2611 | 0.2614 | 0.2304 | 0.2300 | 0.2305 | 0.2306 | 0.2230 | 0.2233 | 0.2248 | 0.2250 |
| 3 | 0.4228 | 0.4231 | 0.4225 | 0.4227 | 0.3672 | 0.3699 | 0.3694 | 0.3696 | 0.3631 | 0.3636 | 0.3629 | 0.3632 |
| 4 | 0.4250 | 0.4246 | 0.4225 | 0.4248 | 0.3773 | 0.3772 | 0.3765 | 0.3764 | 0.3656 | 0.3649 | 0.3655 | 0.3657 |
| 5 | 0.5271 | 0.5278 | 0.5307 | 0.5310 | 0.4594 | 0.4592 | 0.4620 | 0.4617 | 0.4543 | 0.4536 | 0.4560 | 0.4563 |
| 6 | 0.5604 | 0.5603 | 0.5668 | 0.5669 | 0.4902 | 0.4900 | 0.4953 | 0.4952 | 0.4821 | 0.4813 | 0.4869 | 0.4871 |
| SCSC boundary restraint | ||||||||||||
| 1 | 0.3068 | 0.3066 | 0.3069 | 0.3066 | 0.2709 | 0.2708 | 0.2710 | 0.2709 | 0.2631 | 0.2635 | 0.2632 | 0.2635 |
| 2 | 0.4507 | 0.4504 | 0.4510 | 0.4509 | 0.3942 | 0.3940 | 0.3946 | 0.3944 | 0.3868 | 0.3871 | 0.3871 | 0.3875 |
| 3 | 0.5584 | 0.5579 | 0.5578 | 0.5578 | 0.4932 | 0.4930 | 0.4934 | 0.4930 | 0.4790 | 0.4794 | 0.4790 | 0.4793 |
| 4 | 0.6068 | 0.6064 | 0.6080 | 0.6082 | 0.5286 | 0.5285 | 0.5305 | 0.5302 | 0.5206 | 0.5211 | 0.5224 | 0.5226 |
| 5 | 0.8009 | 0.8004 | 0.7995 | 0.7997 | 0.6970 | 0.6967 | 0.6994 | 0.6991 | 0.6872 | 0.6878 | 0.6867 | 0.6871 |
| 6 | 0.8123 | 0.8118 | 0.8120 | 0.8126 | 0.7096 | 0.7094 | 0.7106 | 0.7101 | 0.6968 | 0.6975 | 0.6978 | 0.6982 |
| p | h/a | Mode | Boundary Restraints | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| SSSS | SSSC | SSSF | CFCC | CFCS | CFCF | CFSS | CFSF | |||
| 0.5 | 0.2 | 1 | 149.27 | 149.27 | 148.52 | 183.54 | 190.92 | 189.42 | 182.01 | 189.43 |
| 2 | 197.60 | 197.60 | 197.79 | 263.85 | 251.08 | 249.90 | 261.16 | 249.27 | ||
| 3 | 268.70 | 268.70 | 267.95 | 297.59 | 340.27 | 341.19 | 296.66 | 340.73 | ||
| 4 | 283.64 | 283.64 | 283.54 | 360.97 | 358.36 | 358.11 | 363.46 | 358.11 | ||
| 0.3 | 1 | 73.802 | 73.08 | 73.436 | 89.413 | 93.036 | 94.231 | 89.782 | 94.232 | |
| 2 | 103.90 | 103.90 | 103.31 | 140.17 | 131.40 | 131.22 | 138.48 | 131.22 | ||
| 3 | 148.69 | 150.15 | 149.87 | 157.01 | 188.64 | 189.83 | 158.70 | 189.83 | ||
| 4 | 158.85 | 158.85 | 158.81 | 200.97 | 201.05 | 194.98 | 201.41 | 201.98 | ||
| 0.5 | 1 | 22.872 | 22.871 | 23.486 | 25.853 | 28.699 | 29.260 | 26.981 | 38.988 | |
| 2 | 36.633 | 36.056 | 35.439 | 50.398 | 46.378 | 45.522 | 51.837 | 45.508 | ||
| 3 | 57.082 | 57.082 | 56.925 | 57.403 | 71.642 | 71.353 | 57.263 | 71.345 | ||
| 4 | 60.526 | 60.526 | 60.548 | 75.468 | 76.557 | 76.637 | 75.184 | 77.240 | ||
| 2 | 0.2 | 1 | 139.82 | 138.54 | 137.19 | 175.35 | 173.10 | 173.92 | 177.47 | 173.92 |
| 2 | 176.40 | 176.40 | 175.66 | 218.98 | 224.11 | 222.62 | 219.83 | 222.62 | ||
| 3 | 219.76 | 220.94 | 219.01 | 289.21 | 277.88 | 275.82 | 289.21 | 279.37 | ||
| 4 | 235.79 | 235.79 | 235.47 | 289.58 | 299.38 | 300.53 | 289.34 | 288.57 | ||
| 0.3 | 1 | 65.998 | 65.998 | 65.164 | 82.771 | 83.441 | 83.373 | 84.466 | 83.809 | |
| 2 | 89.699 | 89.699 | 89.101 | 112.91 | 113.12 | 113.57 | 112.24 | 113.57 | ||
| 3 | 117.20 | 117.20 | 117.80 | 156.24 | 148.17 | 146.97 | 152.31 | 146.97 | ||
| 4 | 126.79 | 126.79 | 128.58 | 157.38 | 160.39 | 160.18 | 156.24 | 160.19 | ||
| 0.5 | 1 | 19.385 | 19.385 | 19.526 | 25.401 | 24.863 | 24.626 | 25.130 | 24.603 | |
| 2 | 29.511 | 29.510 | 29.624 | 37.273 | 36.784 | 36.587 | 37.560 | 36.582 | ||
| 3 | 41.343 | 41.343 | 41.060 | 54.783 | 52.479 | 52.129 | 54.783 | 52.125 | ||
| 4 | 47.861 | 45.759 | 45.111 | 55.233 | 65.442 | 57.663 | 57.311 | 61.643 | ||
| 10 | 0.2 | 1 | 126.24 | 126.24 | 124.07 | 170.09 | 159.65 | 159.72 | 172.19 | 159.72 |
| 2 | 155.05 | 155.70 | 155.24 | 195.56 | 197.08 | 197.04 | 195.59 | 197.09 | ||
| 3 | 181.92 | 181.92 | 181.99 | 234.65 | 230.57 | 230.56 | 235.40 | 247.57 | ||
| 4 | 213.04 | 195.00 | 196.87 | 287.83 | 248.14 | 247.57 | 285.93 | 268.55 | ||
| 0.3 | 1 | 59.038 | 59.039 | 59.980 | 81.646 | 72.792 | 74.173 | 80.591 | 74.541 | |
| 2 | 76.164 | 76.052 | 77.799 | 99.184 | 96.254 | 96.254 | 97.498 | 95.994 | ||
| 3 | 91.501 | 91.798 | 91.059 | 122.82 | 116.05 | 115.18 | 121.14 | 114.86 | ||
| 4 | 100.93 | 100.93 | 92.095 | 153.18 | 126.44 | 127.23 | 152.02 | 127.10 | ||
| 0.5 | 1 | 16.608 | 16.608 | 16.210 | 21.258 | 20.341 | 20.629 | 24.613 | 20.501 | |
| 2 | 24.392 | 23.587 | 23.378 | 30.328 | 29.454 | 29.473 | 31.454 | 29.251 | ||
| 3 | 28.855 | 28.855 | 28.908 | 42.315 | 36.206 | 36.074 | 41.992 | 36.187 | ||
| 4 | 33.175 | 33.175 | 33.255 | 54.897 | 41.721 | 42.218 | 54.944 | 42.077 | ||
| p | h/a | Mode | Boundary Restraints | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| SSSS | SSSC | SSSF | CFCC | CFCS | CFCF | CFSS | CFSF | |||
| 0.5 | 0.2 | 1 | 143.27 | 143.27 | 142.55 | 172.14 | 180.56 | 180.56 | 172.96 | 180.44 |
| 2 | 169.61 | 169.61 | 169.43 | 217.36 | 212.08 | 213.37 | 215.25 | 213.37 | ||
| 3 | 215.70 | 215.70 | 215.71 | 288.85 | 268.79 | 273.19 | 282.80 | 275.78 | ||
| 4 | 261.46 | 263.40 | 261.78 | 293.42 | 343.84 | 318.69 | 293.74 | 323.83 | ||
| 0.3 | 1 | 39.957 | 39.799 | 39.959 | 37.882 | 49.638 | 50.648 | 46.905 | 50.649 | |
| 2 | 50.756 | 50.756 | 50.259 | 66.126 | 65.693 | 64.701 | 64.718 | 64.685 | ||
| 3 | 71.395 | 71.395 | 71.466 | 94.250 | 88.635 | 87.408 | 95.658 | 89.695 | ||
| 4 | 93.545 | 93.545 | 94.043 | 99.961 | 118.79 | 118.38 | 99.716 | 118.38 | ||
| 0.5 | 1 | 13.742 | 13.742 | 13.918 | 10.946 | 10.722 | 12.523 | 10.099 | 12.520 | |
| 2 | 21.131 | 21.130 | 21.214 | 17.774 | 17.161 | 17.210 | 18.616 | 17.424 | ||
| 3 | 26.870 | 29.640 | 30.154 | 28.204 | 26.478 | 26.538 | 28.033 | 26.390 | ||
| 4 | 32.248 | 32.248 | 31.950 | 31.325 | 37.018 | 36.416 | 30.482 | 37.678 | ||
| 2 | 0.2 | 1 | 131.95 | 131.95 | 131.92 | 171.11 | 167.09 | 170.62 | 168.99 | 167.34 |
| 2 | 153.97 | 153.97 | 153.22 | 195.56 | 194.12 | 193.80 | 196.22 | 193.67 | ||
| 3 | 189.64 | 189.64 | 189.12 | 234.63 | 239.11 | 239.76 | 234.59 | 239.76 | ||
| 4 | 214.35 | 214.35 | 214.52 | 281.09 | 271.88 | 272.33 | 283.23 | 273.38 | ||
| 0.3 | 1 | 34.865 | 34.865 | 31.766 | 46.420 | 44.966 | 44.964 | 45.004 | 41.363 | |
| 2 | 44.032 | 44.009 | 35.233 | 52.573 | 55.703 | 56.658 | 56.257 | 56.661 | ||
| 3 | 59.393 | 59.393 | 59.309 | 73.363 | 73.826 | 70.840 | 73.412 | 76.400 | ||
| 4 | 69.760 | 69.759 | 70.258 | 94.390 | 88.247 | 87.151 | 93.799 | 87.154 | ||
| 0.5 | 1 | 7.9456 | 7.9443 | 8.0661 | 16.104 | 10.235 | 10.082 | 11.810 | 10.080 | |
| 2 | 11.244 | 11.245 | 11.670 | 19.577 | 14.729 | 14.280 | 13.584 | 14.274 | ||
| 3 | 16.165 | 16.522 | 15.924 | 27.897 | 21.322 | 20.896 | 20.420 | 20.891 | ||
| 4 | 20.059 | 19.795 | 20.059 | 28.088 | 26.279 | 25.679 | 28.050 | 25.608 | ||
| 10 | 0.2 | 1 | 121.99 | 121.78 | 121.99 | 164.35 | 153.67 | 153.22 | 164.34 | 153.22 |
| 2 | 139.33 | 139.32 | 138.89 | 179.97 | 177.43 | 175.93 | 180.63 | 175.97 | ||
| 3 | 163.88 | 163.88 | 164.16 | 203.80 | 207.55 | 208.46 | 204.18 | 208.41 | ||
| 4 | 176.79 | 177.01 | 177.53 | 234.65 | 222.20 | 223.76 | 235.40 | 224.36 | ||
| 0.3 | 1 | 26.254 | 30.634 | 30.422 | 43.323 | 38.697 | 38.705 | 43.546 | 38.856 | |
| 2 | 30.635 | 38.260 | 37.712 | 51.818 | 47.346 | 47.606 | 50.407 | 47.328 | ||
| 3 | 48.066 | 48.067 | 48.033 | 63.056 | 61.108 | 58.731 | 61.700 | 60.328 | ||
| 4 | 51.483 | 51.482 | 51.025 | 74.388 | 64.981 | 65.664 | 74.191 | 62.648 | ||
| 0.5 | 1 | 6.5105 | 6.5123 | 6.6952 | 12.656 | 8.0167 | 8.3461 | 10.053 | 7.8646 | |
| 2 | 8.9101 | 8.9129 | 9.1915 | 12.891 | 10.870 | 11.210 | 12.916 | 11.243 | ||
| 3 | 12.426 | 12.430 | 12.199 | 16.145 | 15.570 | 15.098 | 16.666 | 15.386 | ||
| 4 | 12.468 | 12.468 | 13.262 | 21.249 | 16.497 | 16.222 | 20.417 | 15.884 | ||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fu, Y.; Yao, J.; Wan, Z.; Zhao, G. Free Vibration Analysis of Moderately Thick Orthotropic Functionally Graded Plates with General Boundary Restraints. Materials 2018, 11, 273. https://doi.org/10.3390/ma11020273
Fu Y, Yao J, Wan Z, Zhao G. Free Vibration Analysis of Moderately Thick Orthotropic Functionally Graded Plates with General Boundary Restraints. Materials. 2018; 11(2):273. https://doi.org/10.3390/ma11020273
Chicago/Turabian StyleFu, Yu, Jianjun Yao, Zhenshuai Wan, and Gang Zhao. 2018. "Free Vibration Analysis of Moderately Thick Orthotropic Functionally Graded Plates with General Boundary Restraints" Materials 11, no. 2: 273. https://doi.org/10.3390/ma11020273
APA StyleFu, Y., Yao, J., Wan, Z., & Zhao, G. (2018). Free Vibration Analysis of Moderately Thick Orthotropic Functionally Graded Plates with General Boundary Restraints. Materials, 11(2), 273. https://doi.org/10.3390/ma11020273





