Configuration Optimization Design of Ti6Al4V Lattice Structure Formed by SLM
Abstract
1. Introduction
2. Model Establishment
2.1. Mathematical Model
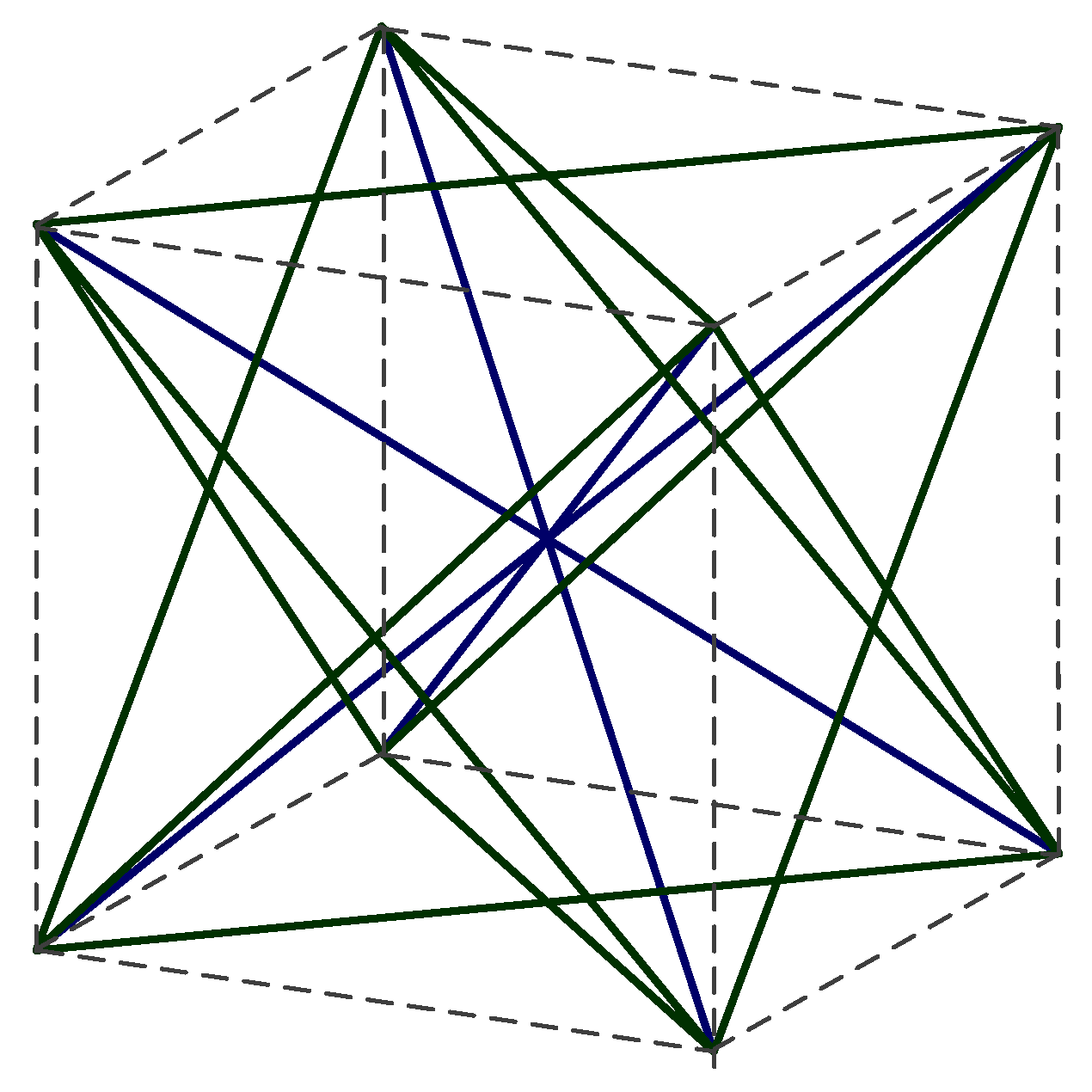
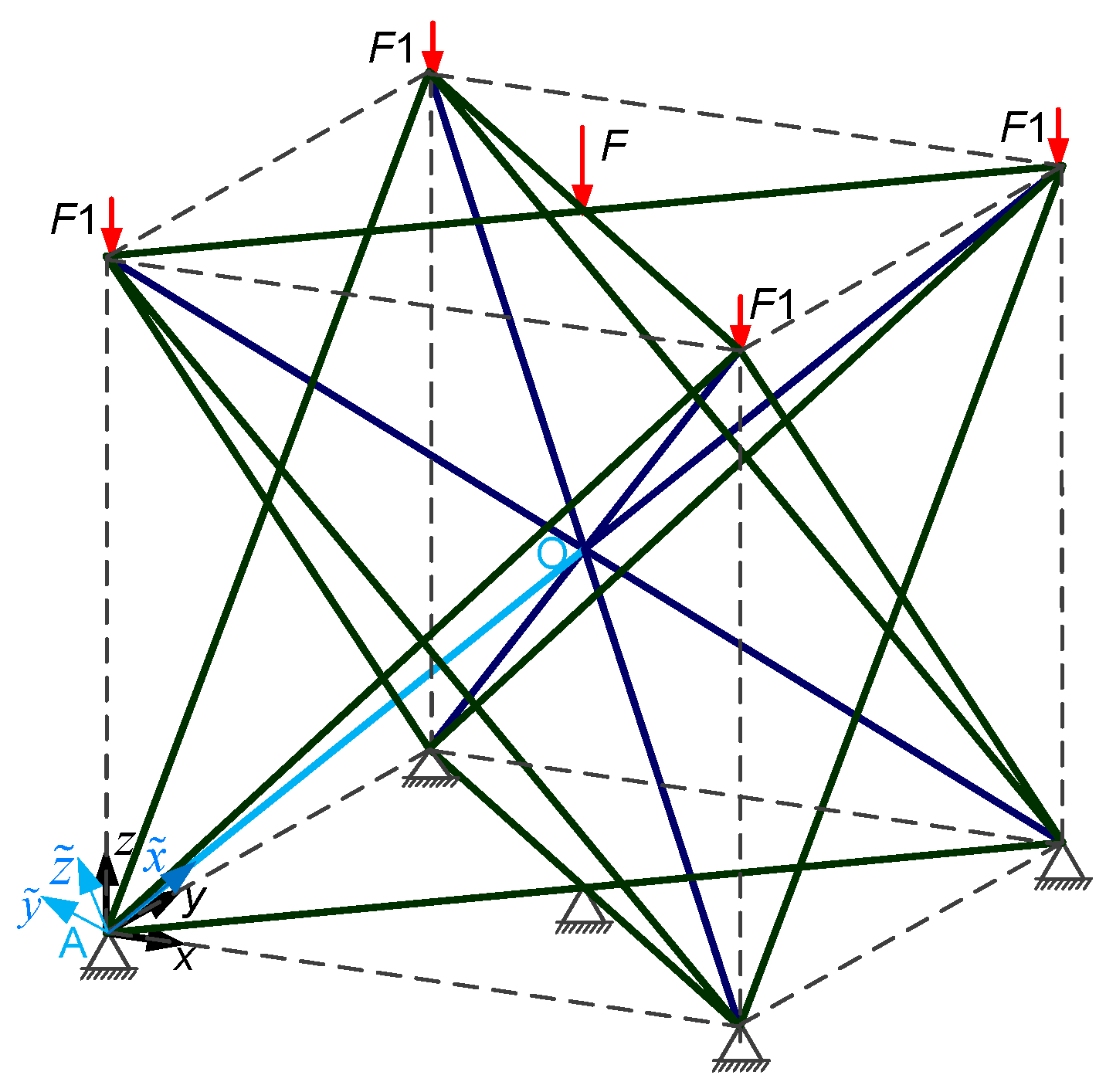
2.1.1. Establishment of the Ground Structure
2.1.2. Solution for the Axial Force FN
- All of the struts in the unit cells are homogeneous with circular cross-sections.
- The material is isotropic, and the compressive and tensile stress–strain relationships are equivalent for all of the strut elements.
- The strut element experiences only axial tension, compression, and bending; the effects of torsion are negligible.
3. Model Optimization
3.1. Design Variables
3.2. Objective Function
3.3. Constraints
3.3.1. Force Constraints
3.3.2. Cross-Sectional Area Constraints
3.4. Solution Process Based on the Firefly Algorithm
3.4.1. Solution of the Optimization Model
3.4.2. Establishment of the Optimization Mathematical Model
3.5. Optimization Results
4. FEA
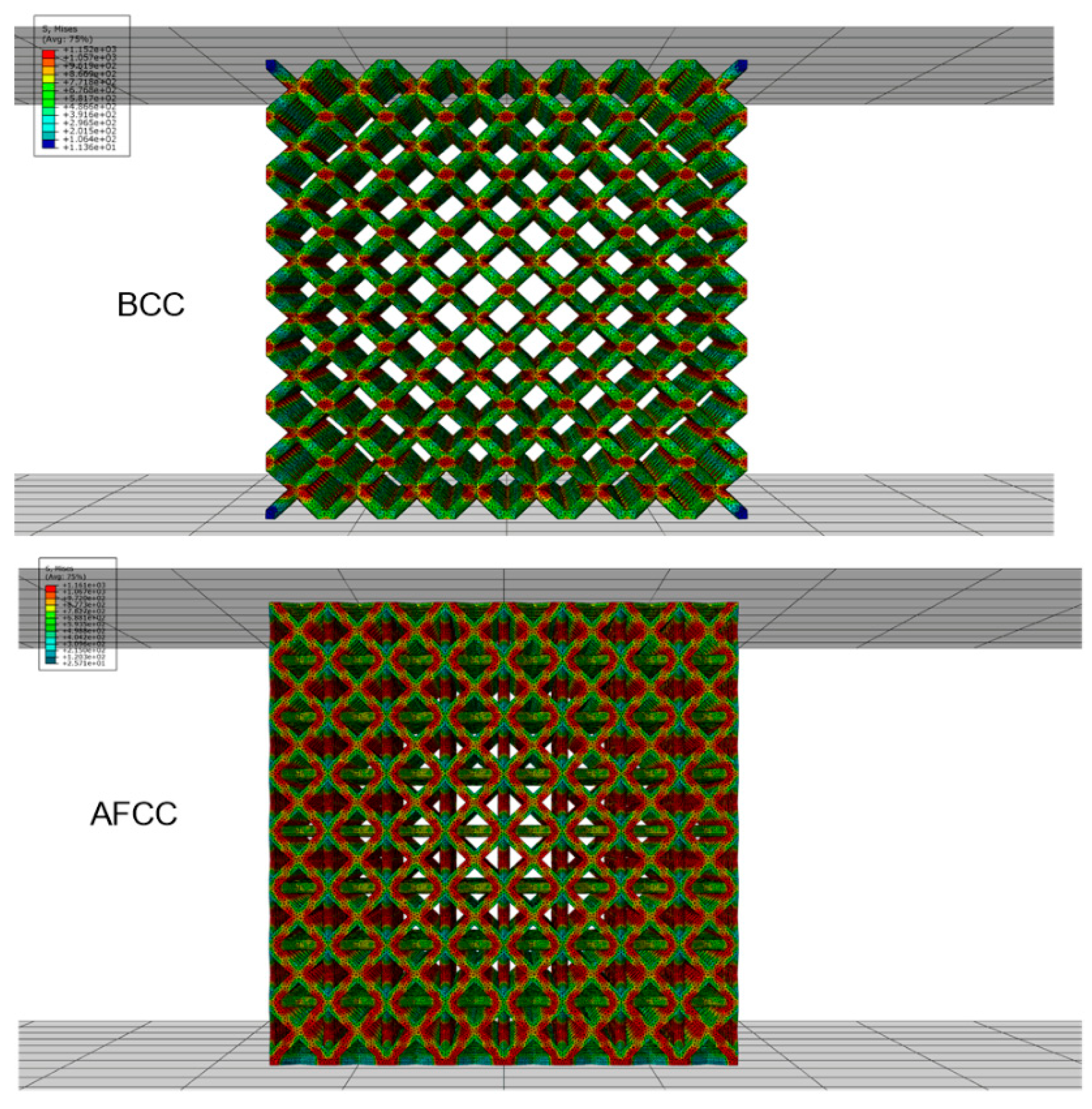
4.1. FEA Model
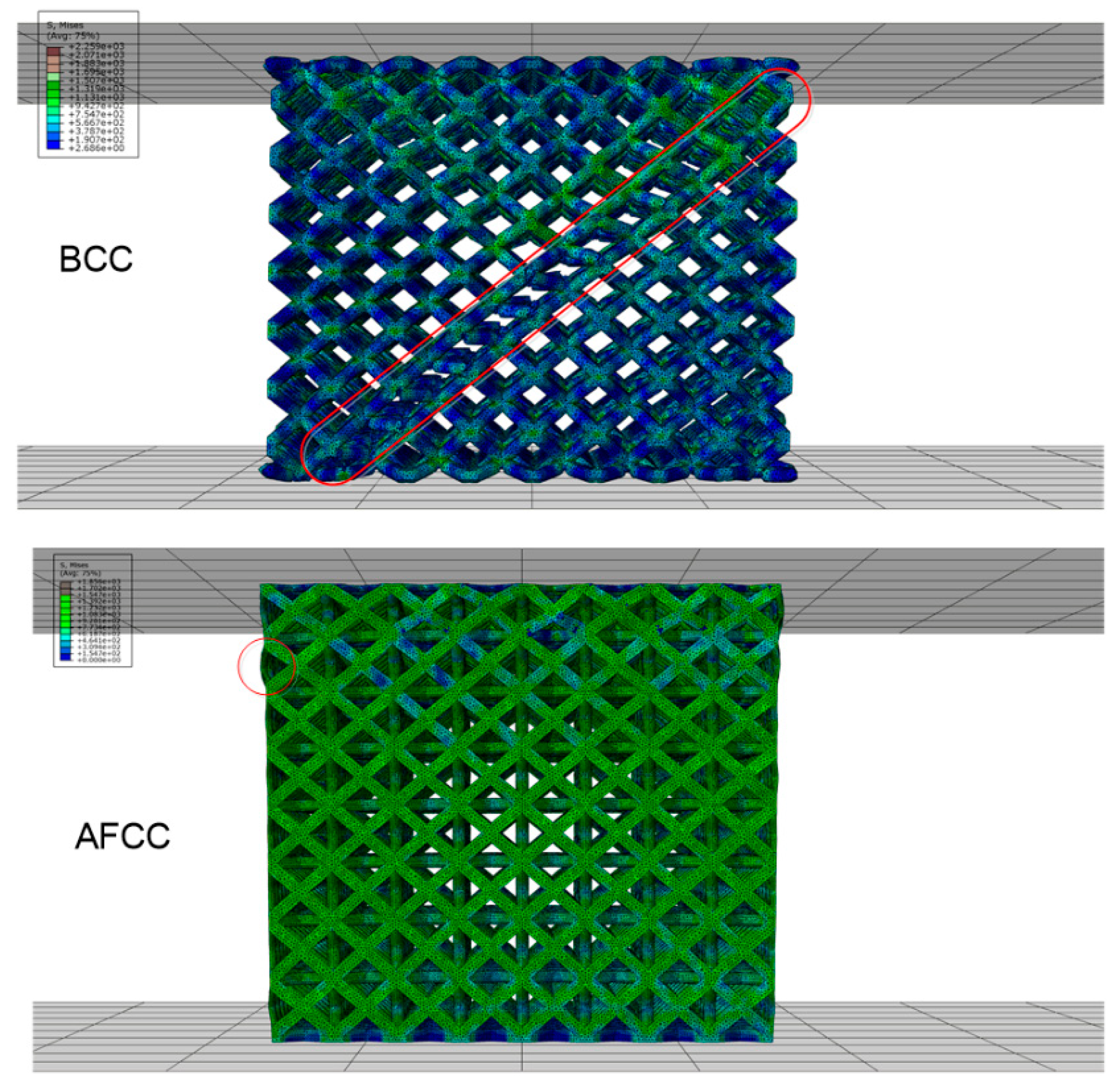
4.2. FEA Results
5. Experimental Study
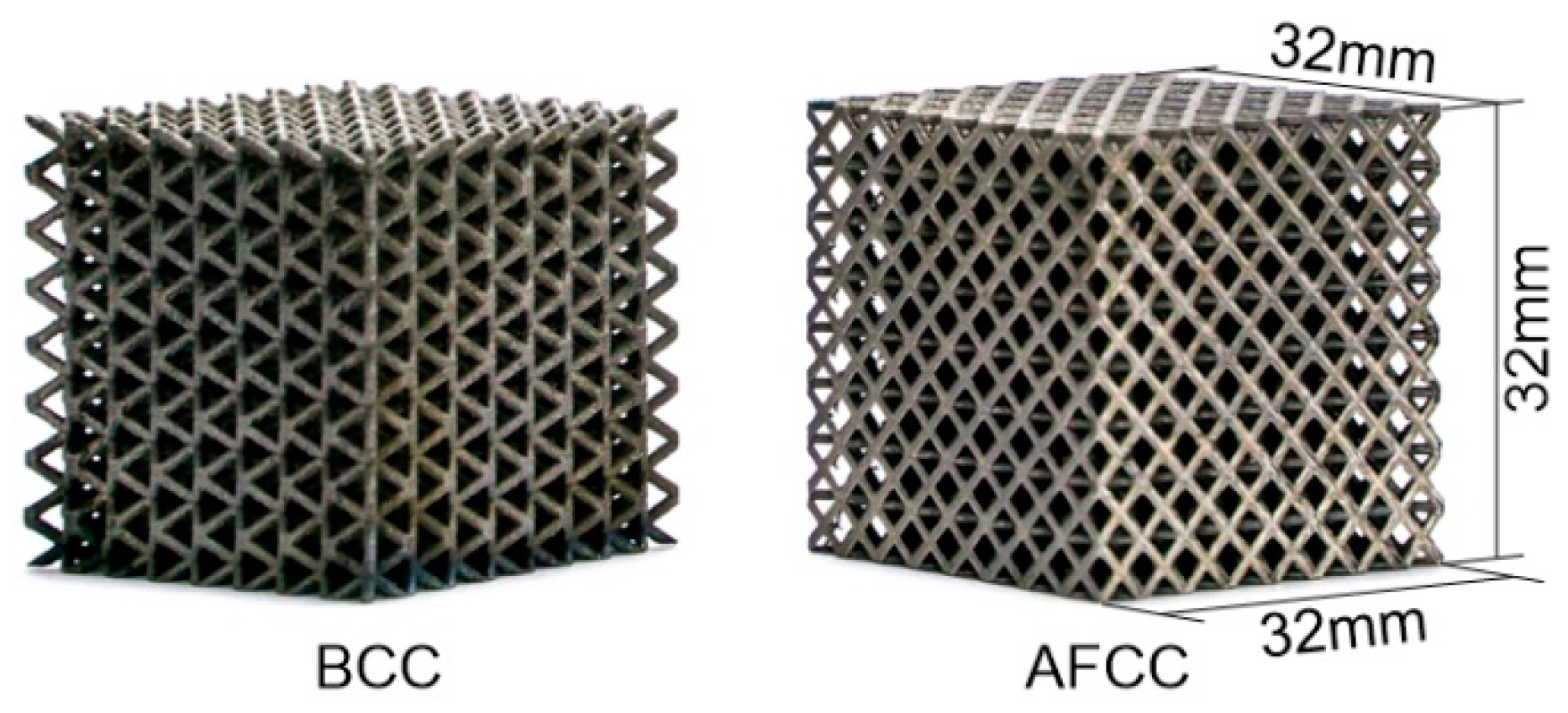
5.1. Manufacturing of Sample and Experiment Conditions
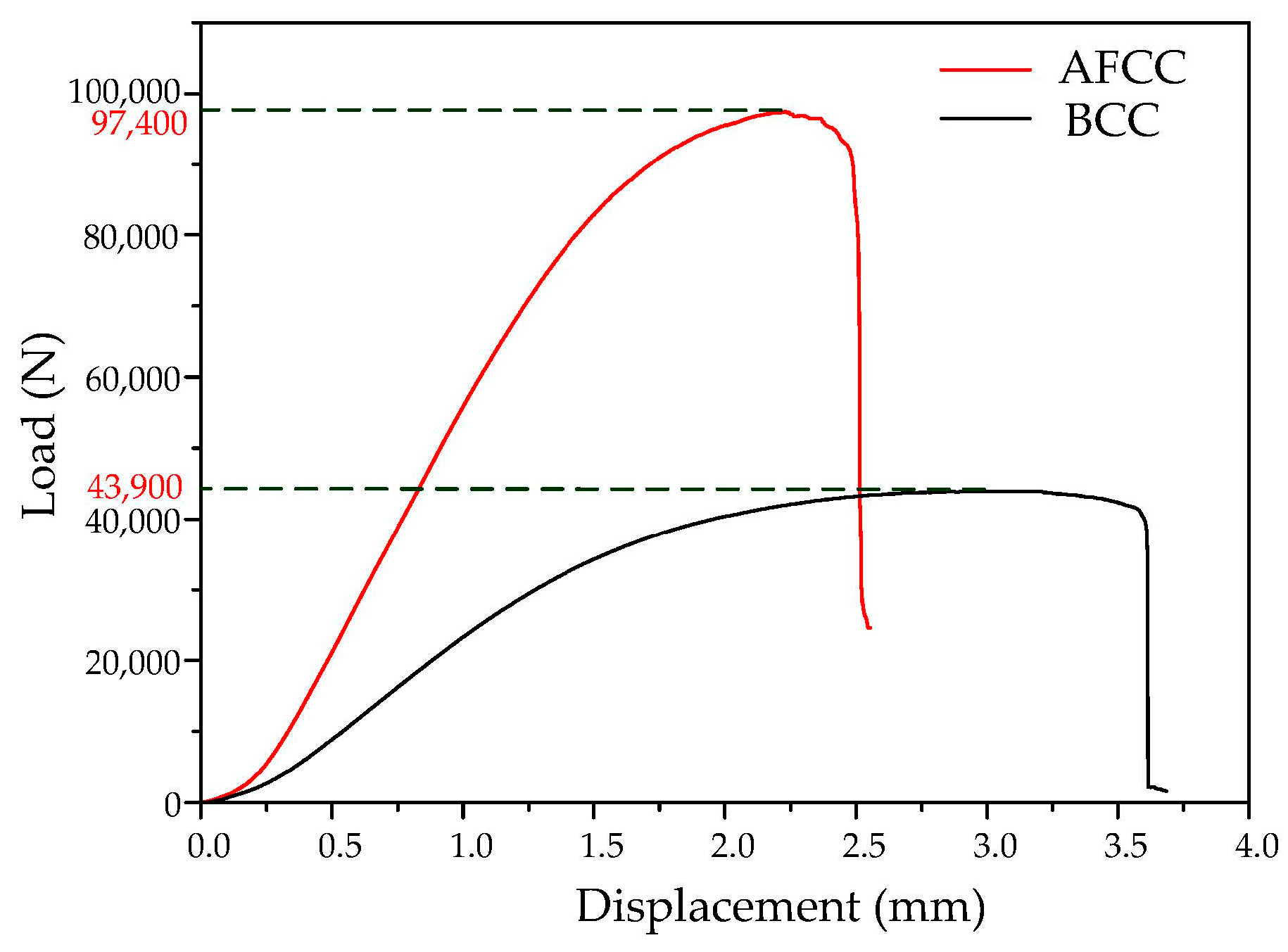
5.2. Experimental Results
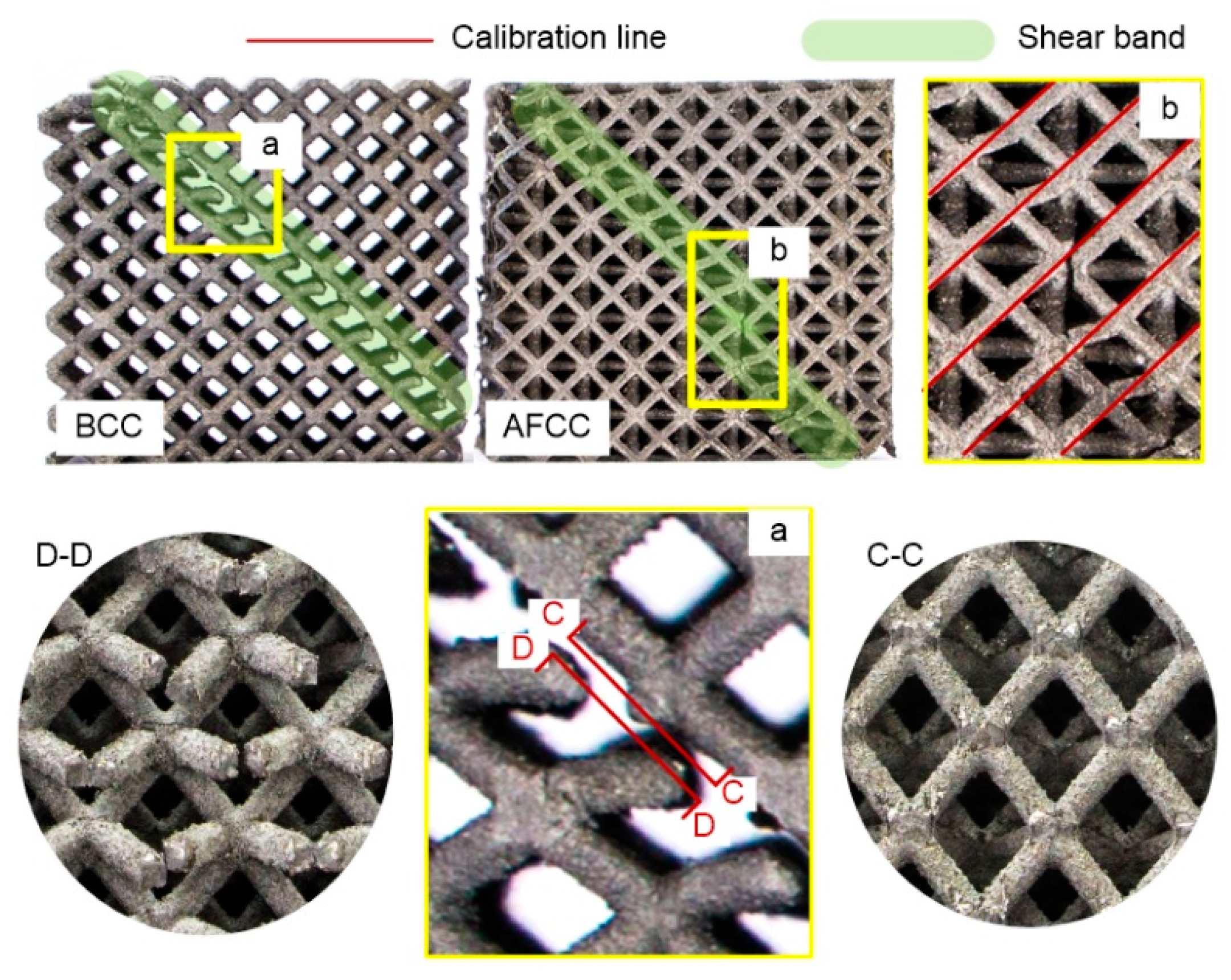
5.3. Destructive Failure Model
- (1)
- The process when the stress reaches its peak.
- (2)
- The stress decreases with a shear slip band along the 45° direction.
- (3)
- The stress increases to a relatively small peak, and the fracture occurs along the 45° direction.
- (4)
- The occurrence of global structural damage.
5.3.1. Mechanical Performance
5.3.2. Energy Absorption Performance
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Evans, A.G.; Hutchinson, J.W.; Fleck, N.A.; Ashby, M.F.; Wadley, H.N.G. The topological design of multifunctional cellular metals. Prog. Mater. Sci. 2001, 46, 309–327. [Google Scholar] [CrossRef]
- Valdevit, L.; Jacobsen, A.J.; Greer, J.R.; Carter, W.B. Protocols for the Optimal Design of Multi-Functional Cellular Structures: From Hypersonics to Micro-Architected Materials. J. Am. Ceram. Soc. 2011, 94 (Suppl. S1), 15–34. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, L.; Daynes, S.; Zhang, H.Y.; Feih, S.; Wang, M.Y. Design of graded lattice structure with optimized mesostructures for additive manufacturing. Mater. Des. 2018, 142, 114–123. [Google Scholar] [CrossRef]
- Xiong, J.; Mines, R.; Ghosh, R.; Vaziri, A.; Ma, L.; Ohrndorf, A.; Christ, H.J.; Wu, L. Advanced Micro-Lattice Materials. Adv. Eng. Mater. 2015, 17, 1253–1264. [Google Scholar] [CrossRef]
- Ushijima, K.; Cantwell, W.J.; Mines, R.A.W.; Tsopanos, S.; Smith, M. An investigation into the compressive properties of stainless steel micro-lattice structures. J. Sandw. Struct. Mater. 2010, 12, 3907–3912. [Google Scholar] [CrossRef]
- Zheng, X.; Fu, Z.; Du, K.; Wang, C.; Yi, Y. Minimal surface designs for porous materials: from microstructures to mechanical properties. J. Mater. Sci. 2018, 2, 1–15. [Google Scholar] [CrossRef]
- Deshpande, V.S.; Fleck, N.A.; Ashby, M.F. Effective properties of the octet-truss lattice material. J. Mech. Phys. Solids 2001, 49, 1747–1769. [Google Scholar] [CrossRef]
- Neff, C.; Hopkinson, N.; Crane, N.B. Selective Laser Sintering of Diamond Lattice Structures: Experimental Results and FEA Model Comparison. In Proceedings of the Solid Freeform Fabrication Symposium, Austin, TX, USA, 3–5 August 2015; pp. 1104–1117. [Google Scholar]
- Zheng, Q.; Ju, S.; Jiang, D. Anisotropic mechanical properties of diamond lattice composites structures. Compos. Struct. 2014, 109, 23–30. [Google Scholar] [CrossRef]
- Bai, L.; Xiong, F.; Chen, X.H.; Yi, C.Y.; Zhang, J.F.; Chen, R. Multi objective Structural Optimization Design of Ti6Al4V Lattice Structure Formed by SLM. J. Mech. Eng. 2018, 54, 156–165. (In Chinese) [Google Scholar] [CrossRef]
- Maskery, I.; Aremu, A.; Simonelli, M.; Tuck, C.; Wildman, R. Mechanical properties of Ti-6Al-4V selectively laser melted parts with Body-Centred-Cubic lattices of varying cell size. Exp. Mech. 2015, 55, 1261–1272. [Google Scholar] [CrossRef]
- Wallach, J.C.; Gibson, L.J. Mechanical behavior of a three-dimensional truss material. Int. J. Solids Struct. 2001, 38, 7181–7196. [Google Scholar] [CrossRef]
- Kooistra, G.W.; Deshpande, V.S.; Wadley, H.N.G. Compressive behavior of age hardenable tetrahedral lattice truss structures made from aluminium. Acta. Mater. 2004, 52, 4229–4237. [Google Scholar] [CrossRef]
- Zheng, H.Y.; Wu, L.Z.; Ma, L.; Wang, X.Z. Impact resistance of Kagome lattice structure sandwich panels. Eng. Mech. 2007, 24, 86–92. [Google Scholar]
- Mazur, M.; Leary, M.; Sun, S.; Martin, V.; Darpan, S.; Milan, B. Deformation and failure behaviour of Ti-6Al-4V lattice structures manufactured by selective laser melting (SLM). Int. J. Adv. Manuf. Tech. 2016, 84, 1391–1411. [Google Scholar] [CrossRef]
- Stolpe, M. Truss optimization with discrete design variables: A critical review. Struct. Multidiscip. Optim. 2016, 53, 1–26. [Google Scholar] [CrossRef]
- Achtziger, W.; Stolpe, M. Global optimization of truss topology with discrete bar areas—Part I: Theory of relaxed problems. Comput. Optim. Appl. 2008, 40, 247–280. [Google Scholar] [CrossRef]
- Hagishita, T.; Ohsaki, M. Topology optimization of trusses by growing ground structure method. Struct. Multidiscip. Optim. 2009, 37, 377–393. [Google Scholar] [CrossRef]
- Roberts, A.P.; Garboczi, E.J. Elastic properties of model random three-dimensional open-cell solids. J. Mech. Phys. Solids 2002, 50, 33–55. [Google Scholar] [CrossRef]
- Bauchau, O.A.; Craig, J.I. Structural Analysis: With Applications to Aerospace Structures; Springer: Berlin, Germany, 2009; pp. 173–176. [Google Scholar]
- Wu, Y.; Li, Q.; Hu, Q.; Borgart, A. Size and Topology Optimization for Trusses with Discrete Design Variables by Improved Firefly Algorithm. Math. Probl. Eng. 2017, 2017, 1457297. [Google Scholar]
- Chougrani, L.; Pernot, J.P.; Véron, P.; Abed, S. Lattice structure lightweight triangulation for additive manufacturing. Comput.-Aided Des. 2017, 90, 95–104. [Google Scholar] [CrossRef]
- Zhang, Y.; Outeiro, J.C.; Mabrouki, T. On the Selection of Johnson-cook Constitutive Model Parameters for Ti-6Al-4V Using Three Types of Numerical Models of Orthogonal Cutting. Procedia Cirp. 2015, 31, 112–117. [Google Scholar] [CrossRef]
- Zhang, S.; Gui, R.; Wei, Q.; Shi, Y. Cracking Behavior and Formation Mechanism of TC4 Alloy Formed by Selective Laser Melting. J. Mech. Eng. 2013, 49, 21–27. [Google Scholar] [CrossRef]
- Qiu, C.; Yue, S.; Adkins, N.; Ward, M.; Hassanin, H.; Lee, P.; Withers, P.J.; Attallah, M.M. Influence of processing on strut structure and compressive properties of cellular lattice structures fabricated by selective laser melting. Mater. Sci. Eng. A 2015, 628, 188–197. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Fang, D.N.; Zhang, Y.H.; Cui, X.D. Mechanical Properties and Multifunctional Design of Lightweight Lattice Materials; Science Press: Beijing, China, 2009. [Google Scholar]

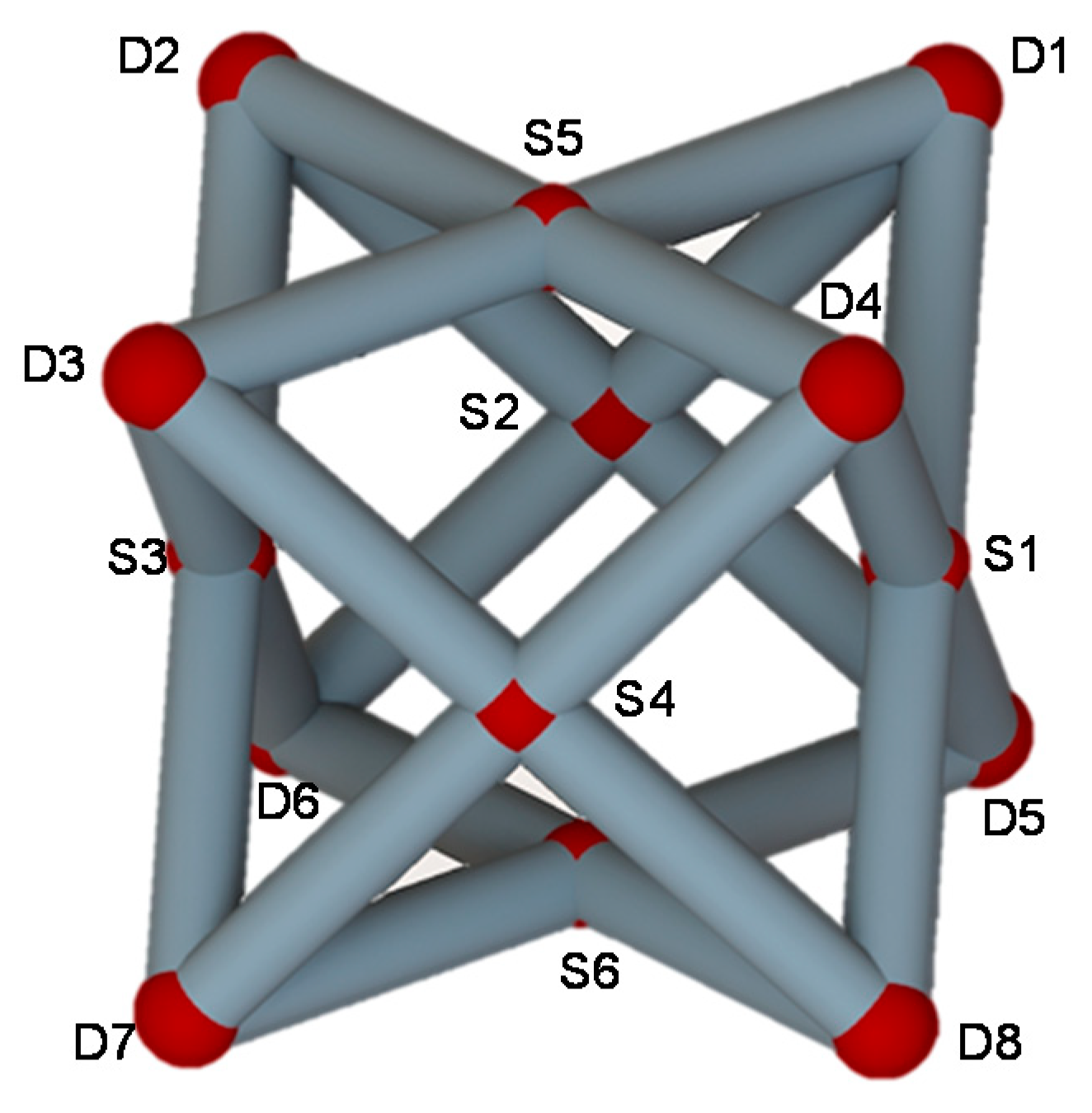





| Element | A (mm2) | Element | A (mm2) | Element | A (mm2) | Element | A (mm2) |
|---|---|---|---|---|---|---|---|
| D3S5 | 0.5 | D8S1 | 0.5 | D7S3 | 0.5 | D3B | 0 |
| D4S5 | 0.4 | D5S1 | 0.6 | D6S3 | 0.5 | D4B | 0 |
| D1S5 | 0.4 | D1S1 | 0.5 | D2S3 | 0.5 | D1B | 0 |
| D2S5 | 0.4 | D4S1 | 0.4 | D3S3 | 0.5 | D2B | 0 |
| D7S4 | 0.5 | D7S6 | 0 | D5S2 | 0.4 | D7B | 0 |
| D8S4 | 0.6 | D8S6 | 0 | D1S2 | 0.5 | D8B | 0 |
| D4S4 | 0.5 | D5S6 | 0 | D2S2 | 0.4 | D5B | 0 |
| D3S4 | 0.5 | D6S6 | 0 | D6S2 | 0.5 | D6B | 0 |
| Element | R (mm) | Element | R (mm) | Element | R (mm) | Element | R (mm) |
|---|---|---|---|---|---|---|---|
| D3S5 | 0.4 | D8S1 | 0.4 | D7S3 | 0.4 | D3B | 0 |
| D4S5 | 0.4 | D5S1 | 0.4 | D6S3 | 0.4 | D4B | 0 |
| D1S5 | 0.4 | D1S1 | 0.4 | D2S3 | 0.4 | D1B | 0 |
| D2S5 | 0.4 | D4S1 | 0.4 | D3S3 | 0.4 | D2B | 0 |
| D7S4 | 0.4 | D7S6 | 0 | D5S2 | 0.4 | D7B | 0 |
| D8S4 | 0.4 | D8S6 | 0 | D1S2 | 0.4 | D8B | 0 |
| D4S4 | 0.4 | D5S6 | 0 | D2S2 | 0.4 | D5B | 0 |
| D3S4 | 0.4 | D6S6 | 0 | D6S2 | 0.4 | D6B | 0 |
| Model | Model Size Length × Width × Height (mm3) | Unit Cell Number Length × Width × Height | Relative Density | Cell Strut Radius R (mm) |
|---|---|---|---|---|
| BCC | 32 × 32 × 32 | 8 × 8 × 8 | 0.26 | 0.44 |
| AFCC | 32 × 32 × 32 | 8 × 8 × 8 | 0.26 | 0.4 |
| Actual Power (W) | Scanning Interval (mm) | Layer Thickness (μm) | Density (%) |
|---|---|---|---|
| 275 | 0.12 | 30 | 99.5 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bai, L.; Zhang, J.; Chen, X.; Yi, C.; Chen, R.; Zhang, Z. Configuration Optimization Design of Ti6Al4V Lattice Structure Formed by SLM. Materials 2018, 11, 1856. https://doi.org/10.3390/ma11101856
Bai L, Zhang J, Chen X, Yi C, Chen R, Zhang Z. Configuration Optimization Design of Ti6Al4V Lattice Structure Formed by SLM. Materials. 2018; 11(10):1856. https://doi.org/10.3390/ma11101856
Chicago/Turabian StyleBai, Long, Junfang Zhang, Xiaohong Chen, Changyan Yi, Rui Chen, and Zixiang Zhang. 2018. "Configuration Optimization Design of Ti6Al4V Lattice Structure Formed by SLM" Materials 11, no. 10: 1856. https://doi.org/10.3390/ma11101856
APA StyleBai, L., Zhang, J., Chen, X., Yi, C., Chen, R., & Zhang, Z. (2018). Configuration Optimization Design of Ti6Al4V Lattice Structure Formed by SLM. Materials, 11(10), 1856. https://doi.org/10.3390/ma11101856








