Development of a Model for Dynamic Recrystallization Consistent with the Second Derivative Criterion
Abstract
:1. Introduction
2. Criteria for Consistency with the SDC
2.1. Mathematical Implications of the SDC
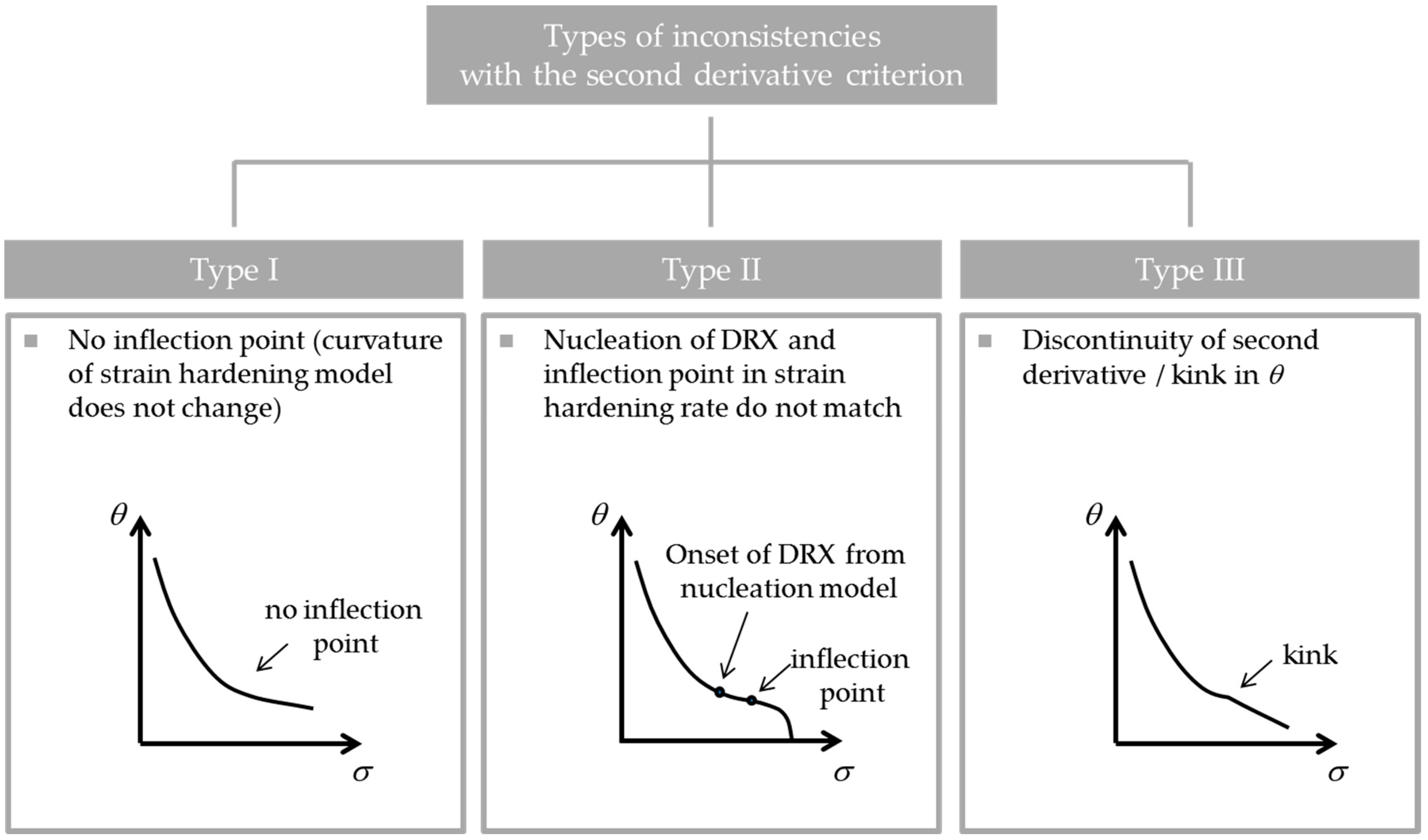
2.2. Inconsistencies with the SDC
- (i)
- The hardening model does not produce an inflection point in the strain-hardening rate.
- (ii)
- The point at which the criterion identifies the location of the point of inflection does not match the actual point of inflection in the experimentally measured strain-hardening rate.
- (iii)
- The derivatives of the strain-hardening rate are discontinuous.
2.2.1. Type I Inconsistency
2.2.2. Type II Inconsistency
2.2.3. Type III Inconsistency
2.3. Inconsistencies in Strain-Hardening Models for Hot Working
- (i)
- Model for strain-hardening and dynamic recovery
- (ii)
- Nucleation criterion for DRX
- (iii)
- Function describing the dynamically recrystallized volume fraction as a function of strain or time
- (iv)
- Rule of mixture to determine the macroscopic flow stress when recrystallized and non-recrystallized grains coexist
3. Material and Experimental Methodology
4. Experimental Results
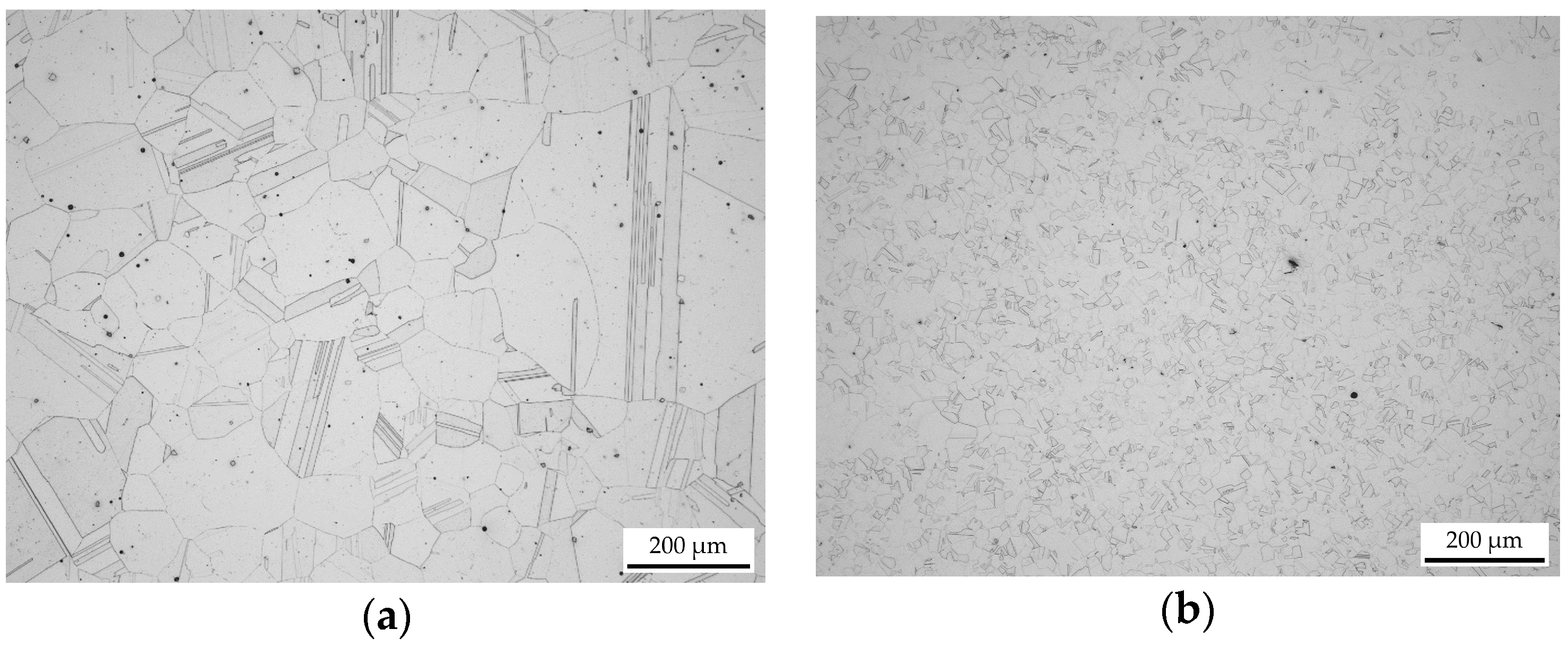
4.1. Microstructure Characterization
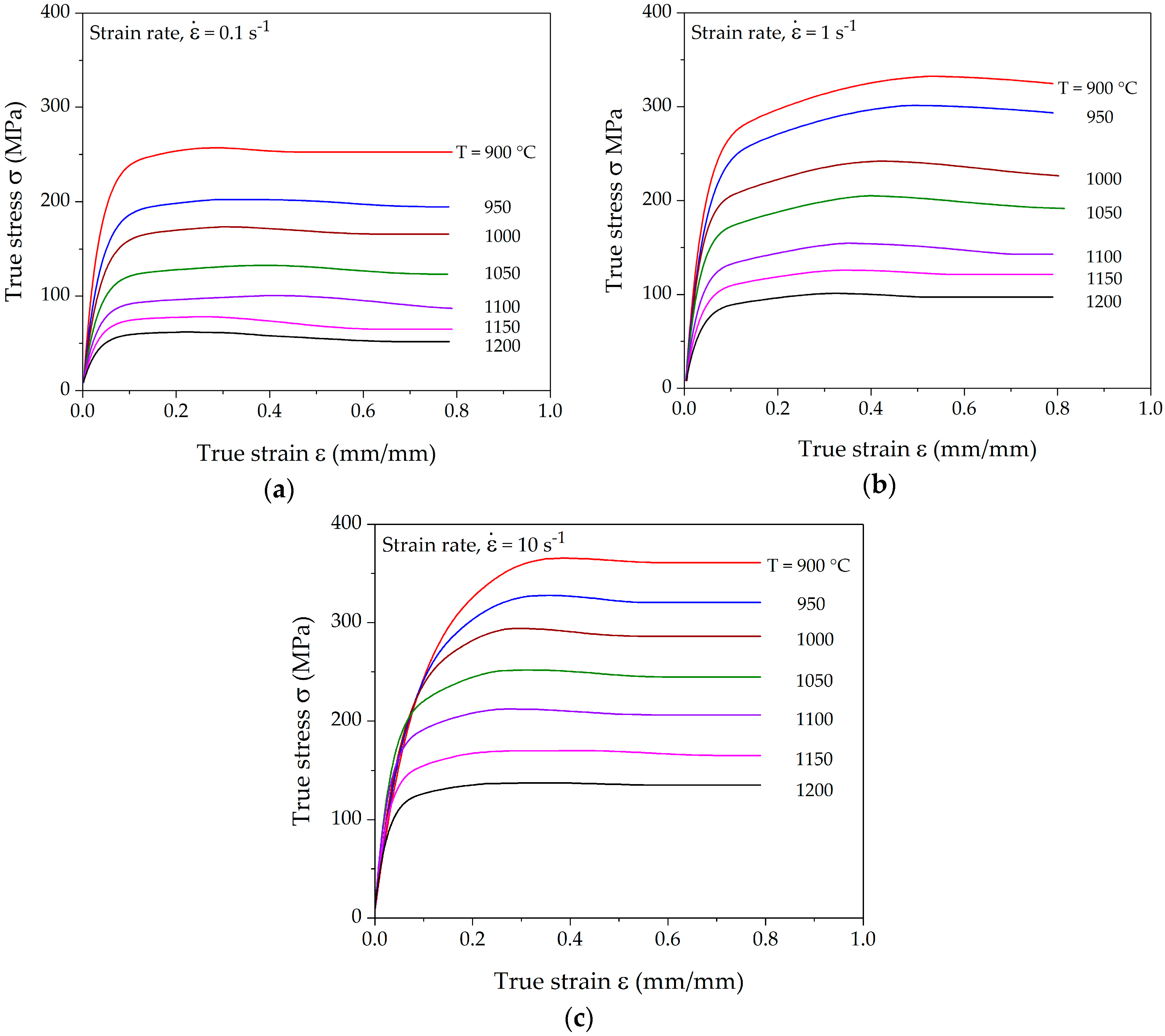
4.2. Hot Deformation Behavior
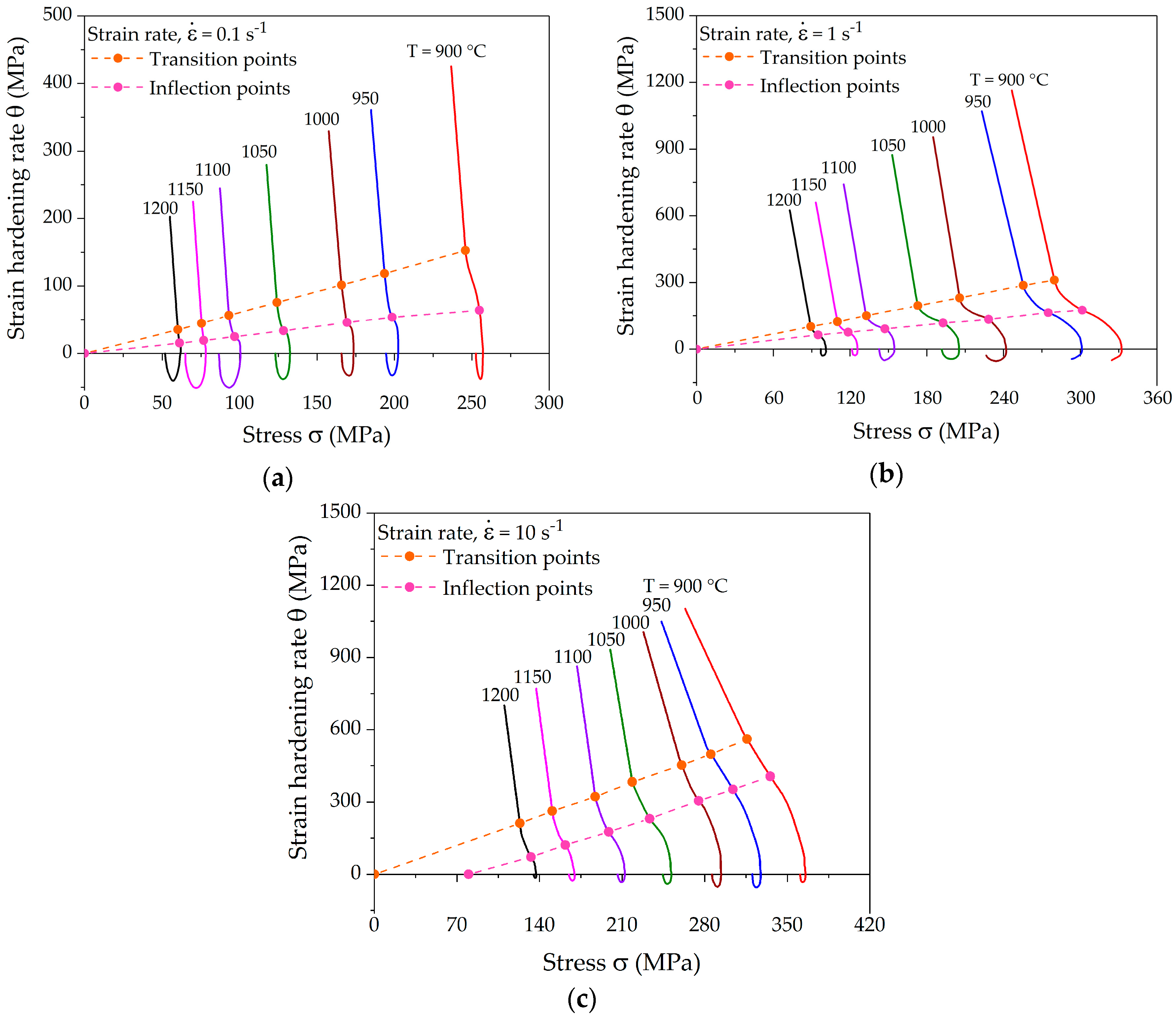
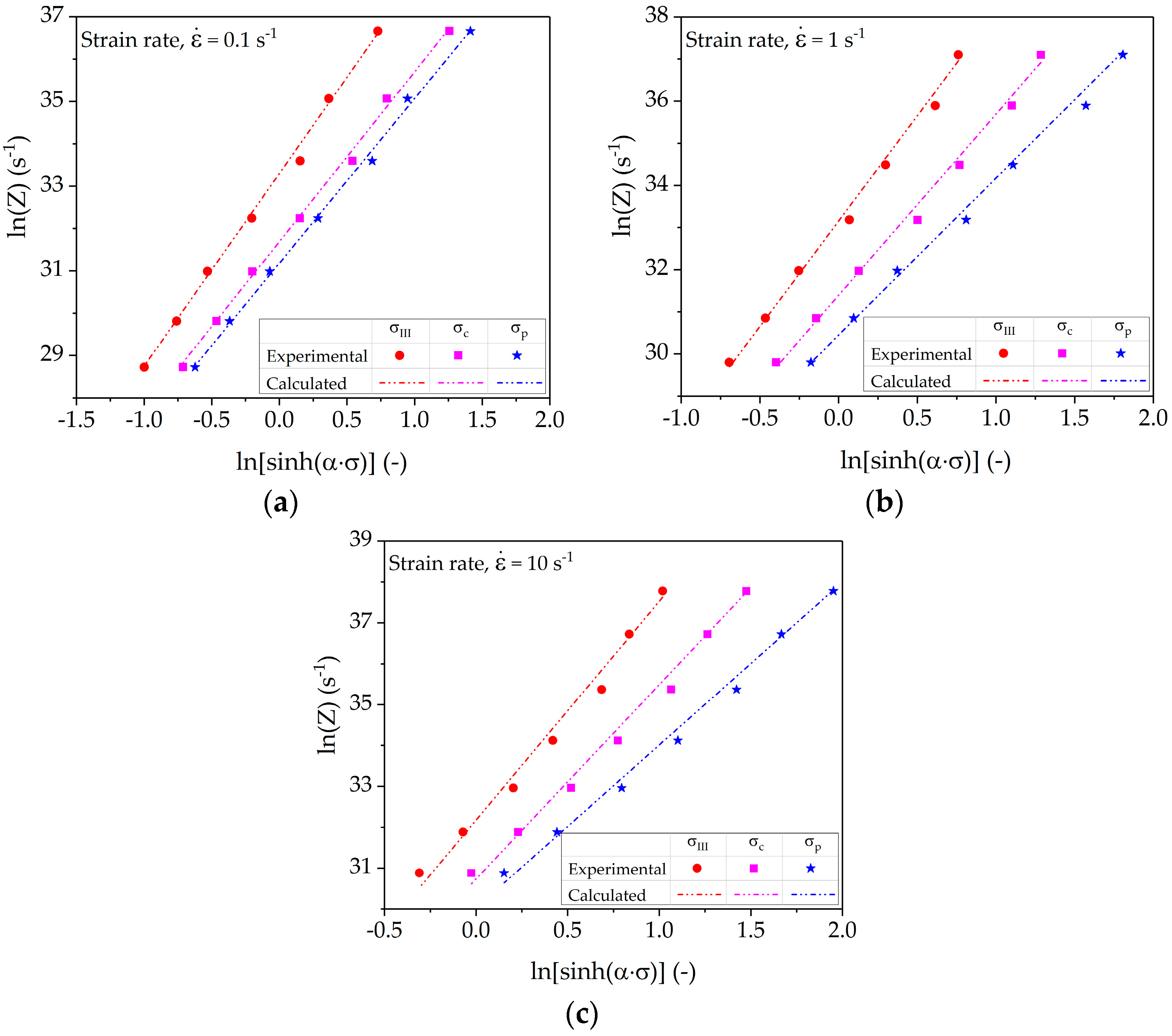
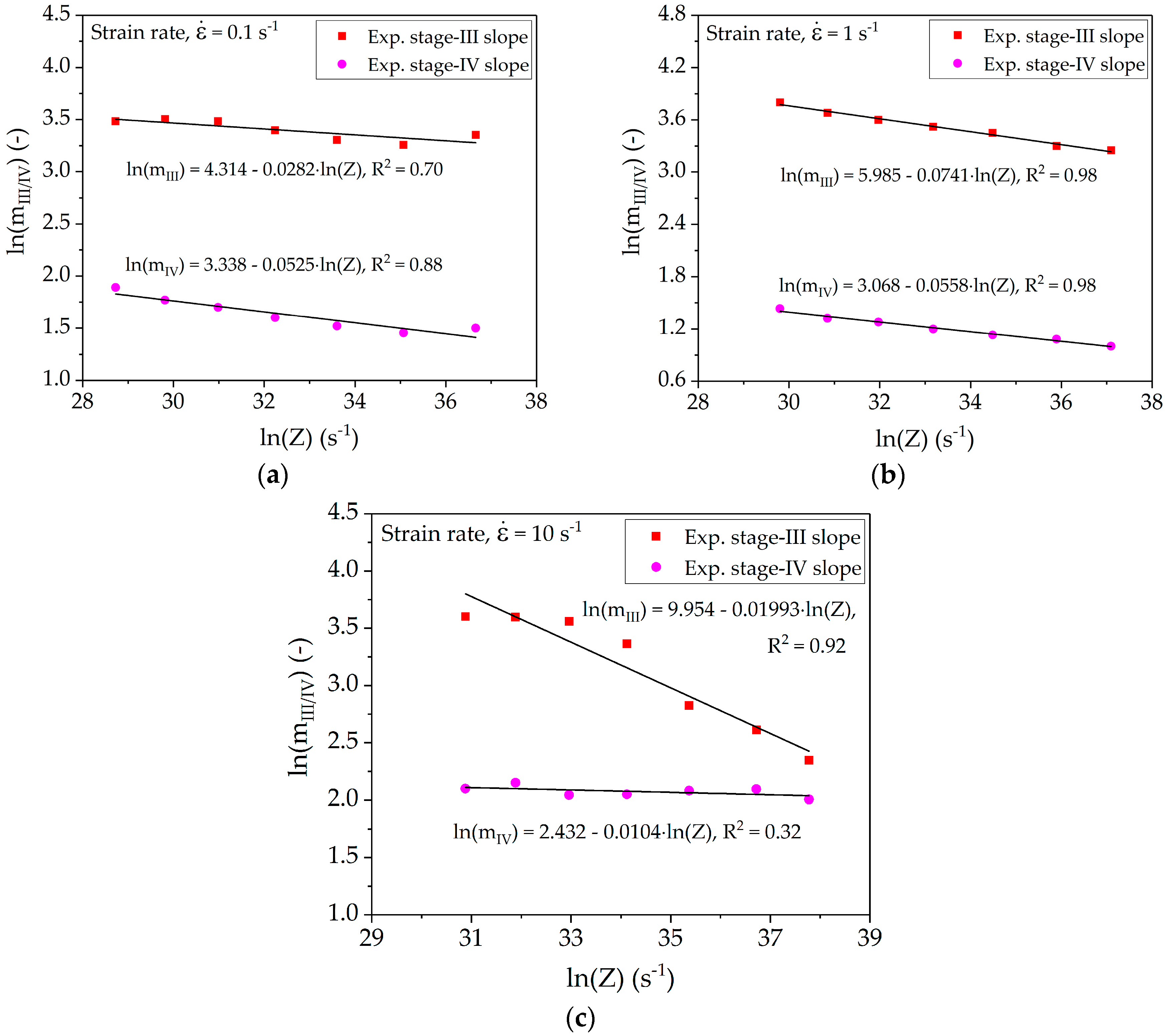
4.3. Dependence of Characteristic Points on the Zener-Hollomon-Parameter
5. Thermodynamically Consistent Strain-Hardening Model for Hot Working
- (i)
- The hardening model needs to take all three hardening stages into account.
- (ii)
- The criterion for the initiation of DRX has to match the inflection in the strain-hardening rate.
- (iii)
- The first three derivatives of the flow stress function with respect to the strain have to be continuous. The function describing the DRX kinetics and its first three derivatives have to vanish at the critical point.
- (iv)
- In the case of an Avrami-type approach, the Avrami exponent has to be greater than 3.
5.1. Strain-Hardening Model
5.2. Nucleation Criterion
5.3. Flow Stress Model
5.4. Dynamic Recrystallization Model
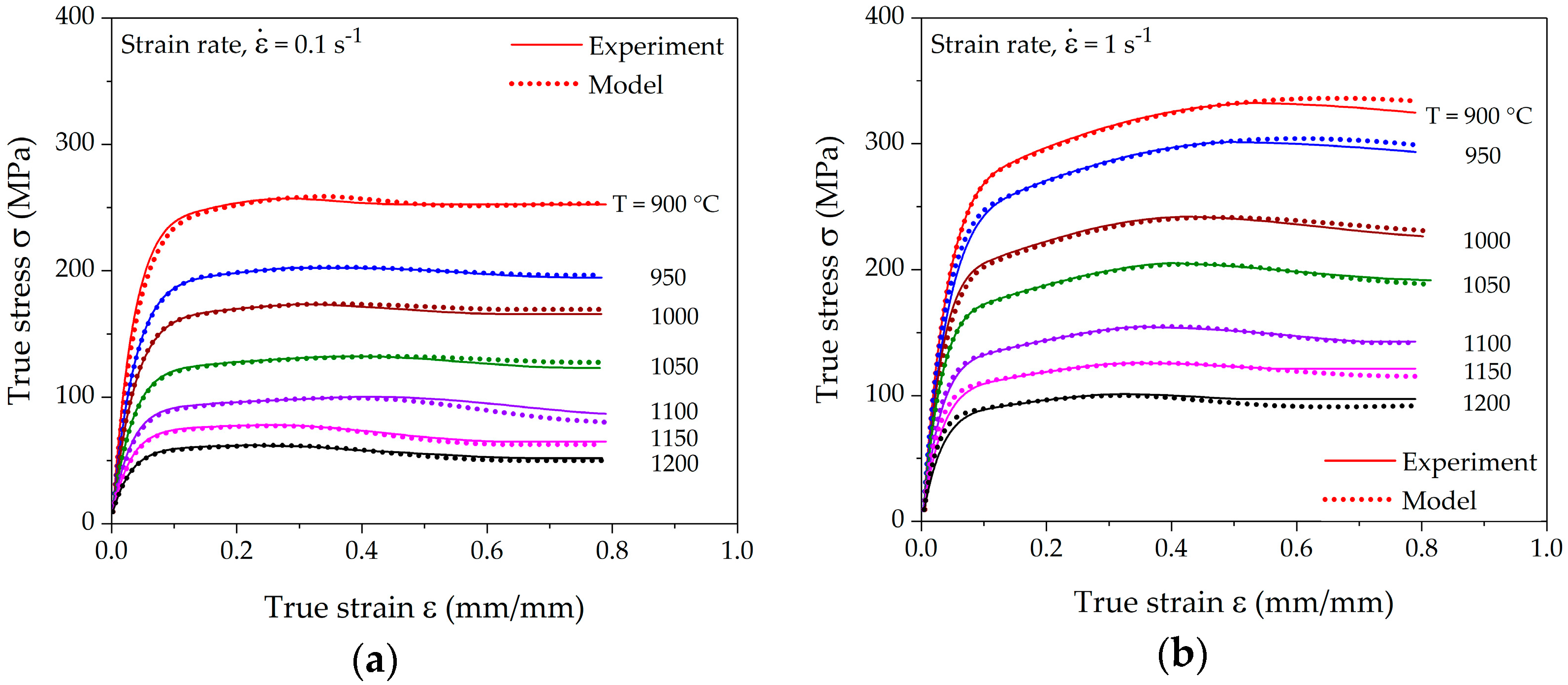
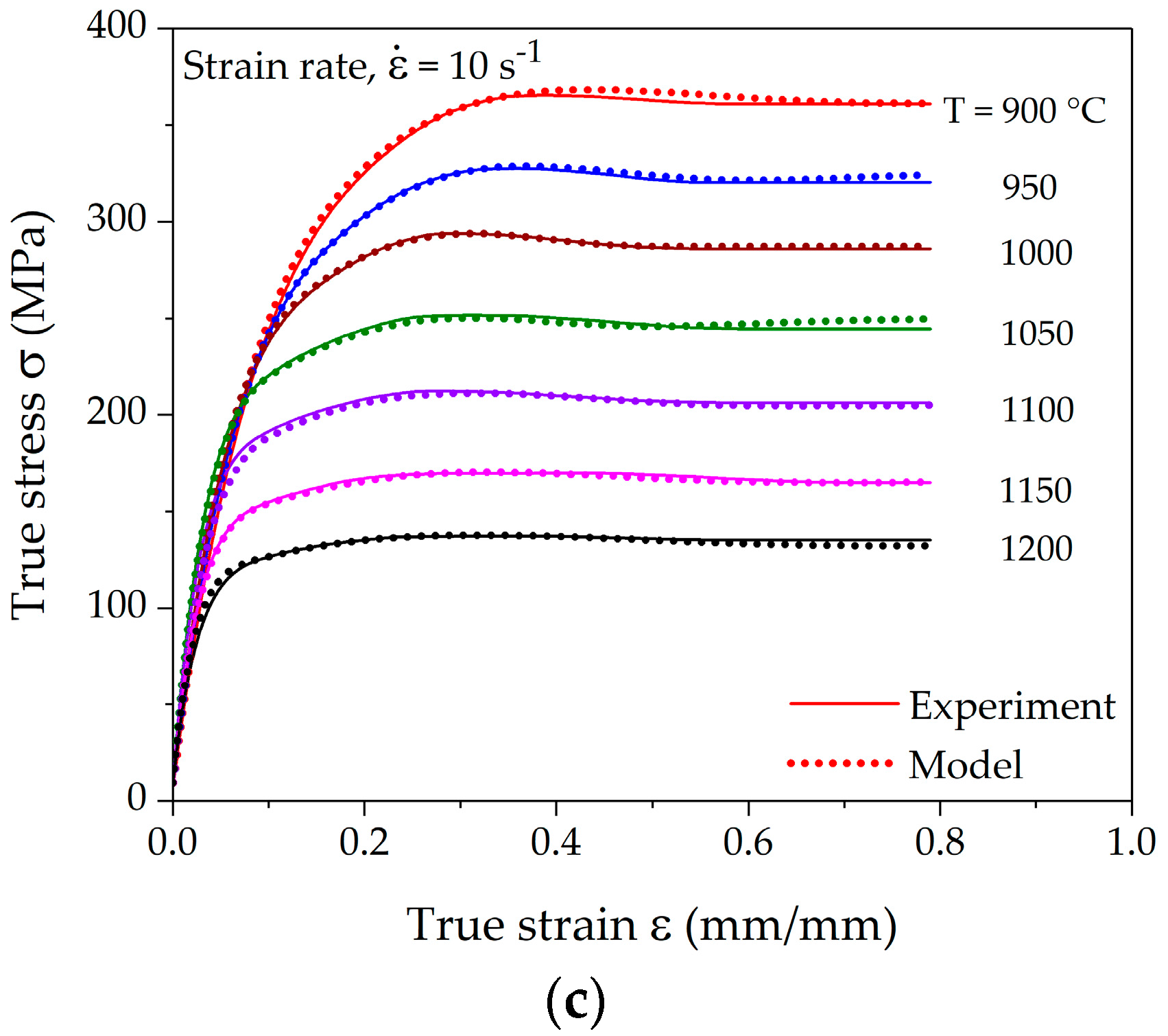
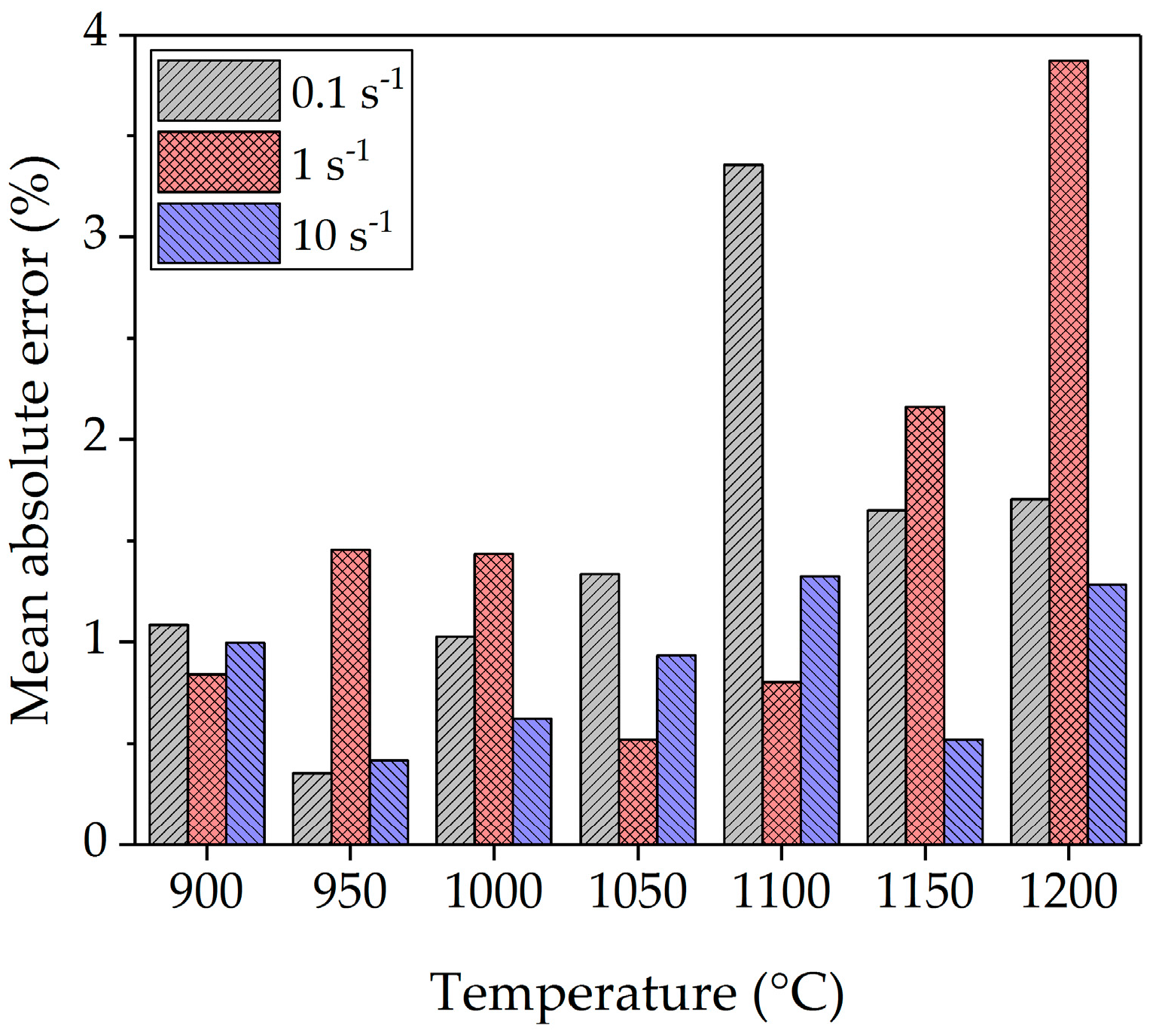
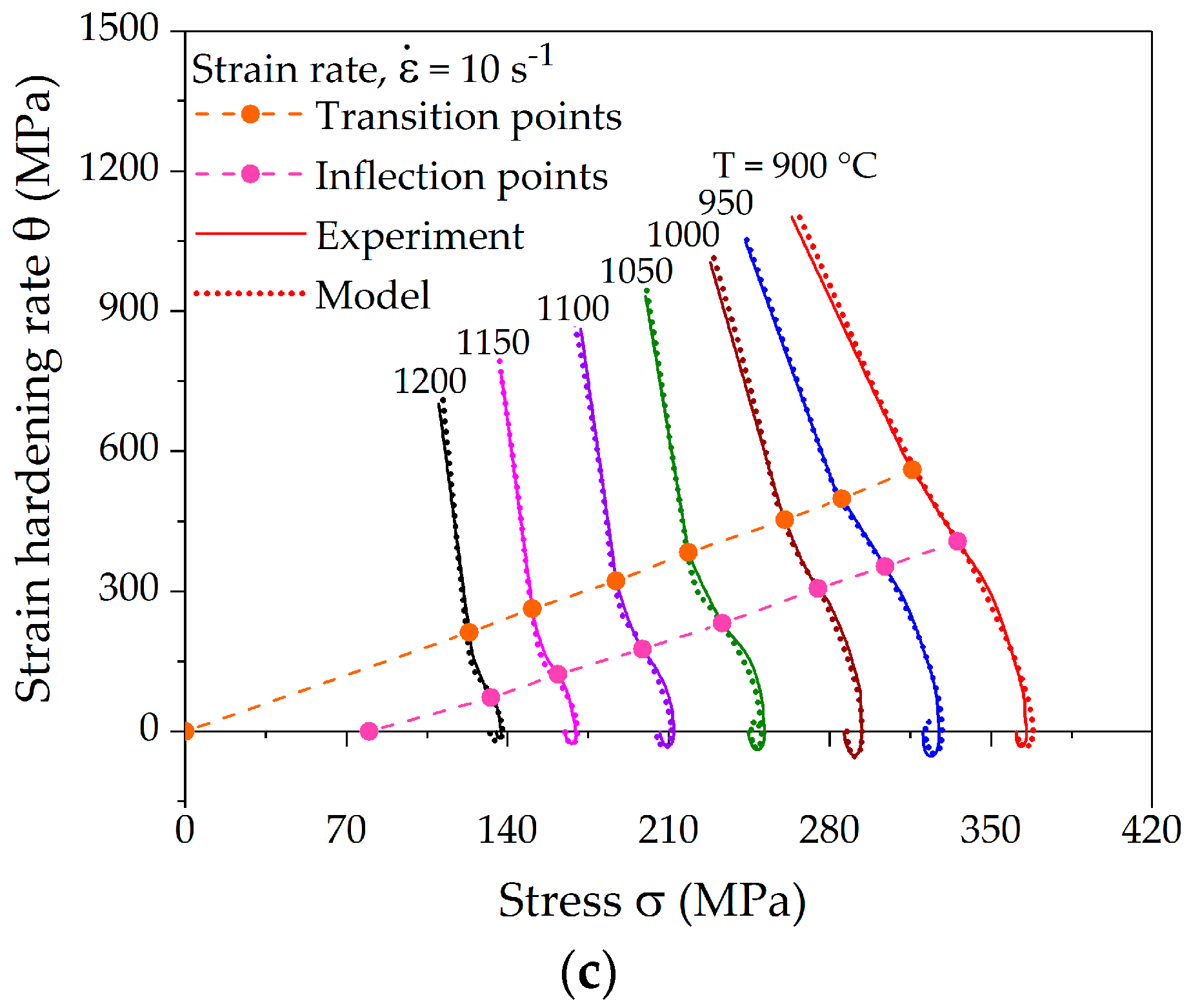
6. Model Validation and Discussion
7. Conclusions
- 1.
- We identified three inconsistencies of flow stress models for dynamically recrystallizing microstructures with the second derivative criterion (SDC):
- (i)
- The hardening model does not produce an inflection point in the strain-hardening rate.
- (ii)
- The predicted point of inflection does not match the inflection in the strain-hardening rate.
- (iii)
- The derivatives of the strain-hardening rate are discontinuous.
- 2.
- A new, single-internal-variable model consistent with the SDC was proposed. The model is based on the course of the strain-hardening rate as a function of stress, which is modeled using three distinct model functions. The transition point between stages III and IV and the critical stress for DRX are modeled as linear functions in the Kocks-Mecking space.
- 3.
- 4.
- The comparison of the modeling results with the experimental data shows a reasonable accuracy in the Kocks-Mecking plots and the flow stress.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Haupt, P. On the Thermomechanical Modelling of Inelastic Material Behaviour. In IUTAM Symposium on Micro- and Macrostructural Aspects of Thermoplasticity; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1999; pp. 3–14. [Google Scholar]
- Lemaitre, J.; Chaboche, J.L. Mechanics of Solid Materials; Cambridge University Press: Cambridge, UK, 1994. [Google Scholar]
- Sandström, R.; Lagneborg, R. A model for hot working occurring by recrystallization. Acta Metall. 1975, 23, 387–398. [Google Scholar] [CrossRef]
- Roberts, W.; Ahlblom, B. A nucleation criterion for dynamic recrystallization during hot working. Acta Metall. 1978, 26, 801–813. [Google Scholar] [CrossRef]
- Sellars, C.M. The Physical Metallurgy of Hot Working. In Proceedings of the Conference on Hot Working and Forming Processes, Sheffield, England; The Society: London, UK, 1979; pp. 3–15. [Google Scholar]
- Laasraoui, A.; Jonas, J.J. Prediction of steel flow stresses at high temperatures and strain rates. MTA 1991, 22, 1545–1558. [Google Scholar] [CrossRef]
- Sommitsch, C.; Mitter, W. On modelling of dynamic recrystallisation of fcc materials with low stacking fault energy. Acta Mater. 2006, 54, 357–375. [Google Scholar] [CrossRef]
- Brown, A.A.; Bammann, D.J. Validation of a model for static and dynamic recrystallization in metals. Int. J. Plast. 2012, 32–33, 17–35. [Google Scholar] [CrossRef]
- Poliak, E.I.; Jonas, J.J. A one-parameter approach to determining the critical conditions for the initiation of dynamic recrystallization. Acta Mater. 1996, 44, 127–136. [Google Scholar] [CrossRef]
- Mejía, I.; Bedolla-Jacuinde, A.; Maldonado, C.; Cabrera, J.M. Determination of the critical conditions for the initiation of dynamic recrystallization in boron microalloyed steels. Mater. Sci. Eng. A 2011, 528, 4133–4140. [Google Scholar] [CrossRef]
- Ebrahimi, R.; Solhjoo, S. Characteristic points of stress-strain curve at high temperature. J. Southeast Univ. 2007, 8, 311–316. [Google Scholar]
- Gottstein, G.; Frommert, M.; Goerdeler, M.; Schäfer, N. Prediction of the critical conditions for dynamic recrystallization in the austenitic steel 800H. Mater. Sci. Eng. A 2004, 387–389, 604–608. [Google Scholar] [CrossRef]
- Poliak, E.I.; Jonas, J.J. Initiation of dynamic recrystallization in constant strain rate hot deformation. ISIJ Int. 2003, 43, 684–691. [Google Scholar] [CrossRef]
- Yazdipour, N.; Davies, C.; Hodgson, P.D. Microstructural modeling of dynamic recrystallization using irregular cellular automata. Comput. Mater. Sci. 2008, 44, 566–576. [Google Scholar] [CrossRef]
- Zahiri, S.H.; Davies, C.H.; Hodgson, P.D. A mechanical approach to quantify dynamic recrystallization in polycrystalline metals. Scr. Mater. 2005, 52, 299–304. [Google Scholar] [CrossRef]
- Poliak, E.I.; Jonas, J.J. Critical strain for dynamic recrystallization in variable strain rate hot deformation. ISIJ Int. 2003, 43, 692–700. [Google Scholar] [CrossRef]
- Kim, S.-I.; Lee, Y.; Lee, D.-L.; Yoo, Y.-C. Modeling of AGS and recrystallized fraction of microalloyed medium carbon steel during hot deformation. Mater. Sci. Eng. A 2003, 355, 384–393. [Google Scholar] [CrossRef]
- Bambach, M. Conditions for consistent implementation of flow stress models incorporating dynamic recrystallization into finite element simulation codes. MSF 2013, 762, 325–330. [Google Scholar] [CrossRef]
- Bambach, M. Implications from the Poliak–Jonas criterion for the construction of flow stress models incorporating dynamic recrystallization. Acta Mater. 2013, 61, 6222–6233. [Google Scholar] [CrossRef]
- Kolmogorov, A. On the statistical theory of the crystallization of metals. Bull. Acad. Sci. USSR Math. Ser. 1937, 3, 355–359. [Google Scholar]
- Johnson, W.A.; Mehl, R. Reaction Kinetics in Processes of Nucleation and Growth. Trans. Metall. Soc. AIME 1939, 135, 396–415. [Google Scholar]
- Avrami, M. Kinetics of Phase Change. I General Theory. J. Chem. Phys. 1939, 7, 1103–1112. [Google Scholar] [CrossRef]
- Cahn, J.W. The kinetics of grain boundary nucleated reactions. Acta Metall. 1956, 4, 449–459. [Google Scholar] [CrossRef]
- Speich, G.R.; Fisher, R.M. Recrystallization in rapidly heated 3 1/4% silicon iron. In Recrystallization, Grain Growth and Textures; Margolin, H., Ed.; American Society for Metals (ASM): Cleveland, OH, USA, 1966; pp. 563–598. [Google Scholar]
- Bambach, M. Process and Materials Modeling in Metal Forming; Shaker Verlag Aachen: Herzogenrath, Germany, 2016. [Google Scholar]
- Mecking, H.; Kocks, U.F. A Mechanism for Static and Dynamic Recovery. In Strength of Metals and Alloys; Elsevier: Amsterdam, The Netherlands, 1979; pp. 345–350. [Google Scholar]
- Estrin, Y.; Tóth, L.S.; Molinari, A.; Bréchet, Y. A dislocation-based model for all hardening stages in large strain deformation. Acta Mater. 1998, 46, 5509–5522. [Google Scholar] [CrossRef]
- Mecking, H.; Kocks, U.F. Kinetics of flow and strain-hardening. Acta Metall. 1981, 29, 1865–1875. [Google Scholar] [CrossRef]
- Yanagimoto, J.; Karhausen, K.; Brand, A.J.; Kopp, R. Incremental Formulation for the Prediction of Flow Stress and Microstructural Change in Hot Forming. J. Manuf. Sci. Eng. 1998, 120, 316. [Google Scholar] [CrossRef]
- Karhausen, K.; Kopp, R. Model for integrated process and microstructure simulation in hot forming. Steel Res. 1992, 63, 247–256. [Google Scholar] [CrossRef]
- Takaki, T.; Hisakuni, Y.; Hirouchi, T.; Yamanaka, A.; Tomita, Y. Multi-phase-field simulations for dynamic recrystallization. Comput. Mater. Sci. 2009, 45, 881–888. [Google Scholar] [CrossRef]
- Ding, R.; Guo, Z.X. Microstructural modelling of dynamic recrystallisation using an extended cellular automaton approach. Comput. Mater. Sci. 2002, 23, 209–218. [Google Scholar] [CrossRef]
- Lin, J.; Liu, Y. A set of unified constitutive equations for modelling microstructure evolution in hot deformation. J. Mater. Process. Technol. 2003, 143–144, 281–285. [Google Scholar] [CrossRef]
- Jonas, J.J.; Quelennec, X.; Jiang, L.; Martin, É. The Avrami kinetics of dynamic recrystallization. Acta Mater. 2009, 57, 2748–2756. [Google Scholar] [CrossRef]
- Beynon, J.H.; Sellars, C.M. Modelling Microstructure and Its Effects during Multipass Hot Rolling. ISIJ Int. 1992, 32, 359–367. [Google Scholar] [CrossRef]
- Cabrera, J.M.; Ponce, J.; Prado, J.M. Modeling thermomechanical processing of austenite. J. Mater. Process. Technol. 2003, 143, 403–409. [Google Scholar] [CrossRef]
- Grätz, K.; Miessen, C.; Gottstein, G. Analysis of steady-state dynamic recrystallization. Acta Mater. 2014, 67, 58–66. [Google Scholar] [CrossRef]
- Gottstein, G. A Different View on Dynamic Recrystallization. Mater. Sci. Forum 2012, 715, 235–242. [Google Scholar] [CrossRef]
- Cao, Y.; Di, H.; Misra, R. The impact of aging pre-treatment on the hot deformation behavior of alloy 800H at 750 °C. J. Nucl. Mater. 2014, 452, 77–86. [Google Scholar] [CrossRef]
- Bergström, Y. A dislocation model for the strain-ageing behaviour of steel. Mater. Sci. Eng. 1972, 9, 101–110. [Google Scholar] [CrossRef]
- Lohmar, J.; Bambach, M. Influence of Different Interpolation Techniques on the Determination of the Critical Conditions for the Onset of Dynamic Recrystallisation. MSF 2013, 762, 331–336. [Google Scholar] [CrossRef]
- Bambach, M.; Heppner, S.; Steinmetz, D.; Roters, F. Assessing and ensuring parameter identifiability for a physically-based strain hardening model for twinning-induced plasticity. Mech. Mater. 2015, 84, 127–139. [Google Scholar] [CrossRef]
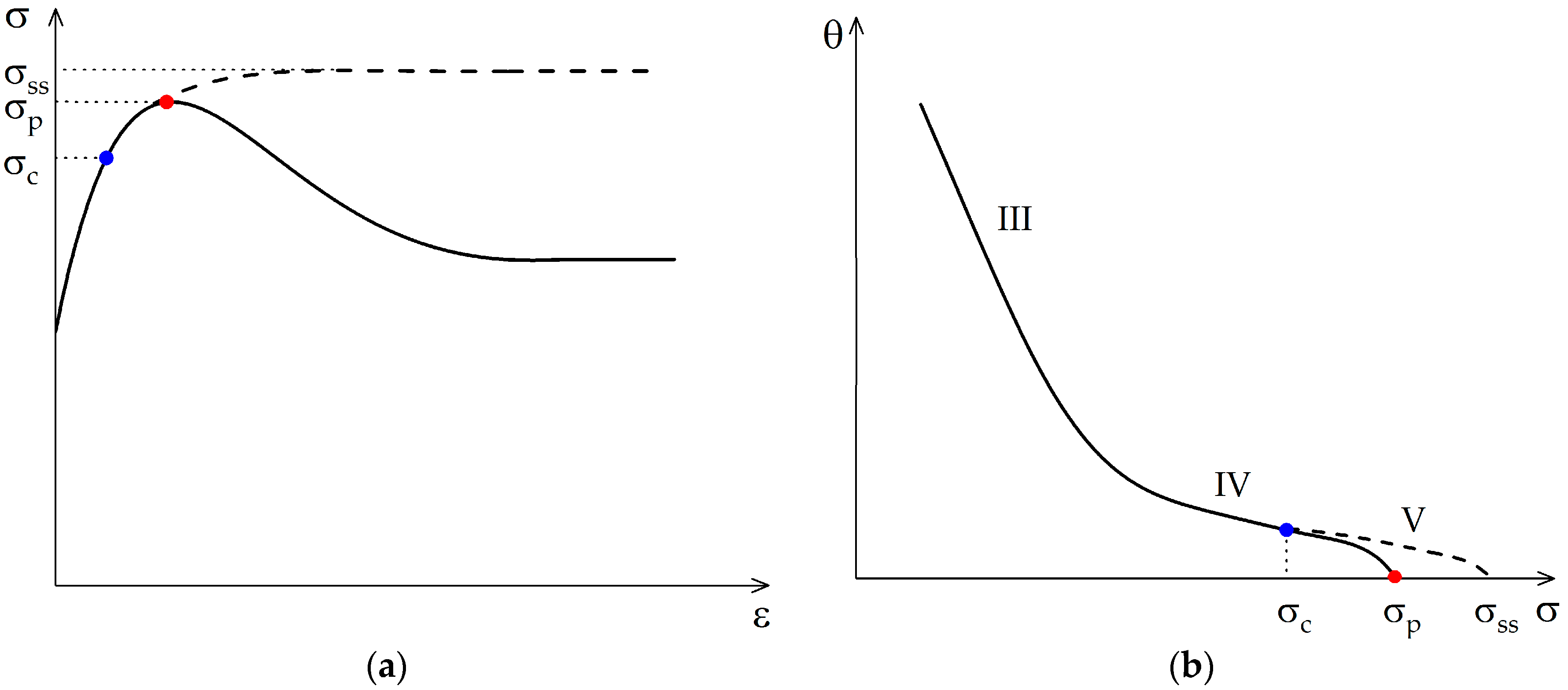

| Element | Ni | Cr | Mn | Si | Ti | Al | C, Co, Cu, S | Fe |
|---|---|---|---|---|---|---|---|---|
| wt. % | 30.25 | 20.51 | 0.69 | 0.50 | 0.36 | 0.26 | <0.09 | Bal. |
| Characteristic Points | Strain Rate, s−1 | A, s−1 | α, MPa−1 | n |
|---|---|---|---|---|
| σIII | 0.1 | 2.9∙1014 | 0.0060 | 4.55 |
| 1.0 | 2.50∙1014 | 0.0053 | 5.00 | |
| 10 | 9.4∙1013 | 0.0055 | 5.35 | |
| σc | 0.1 | 5.8∙1013 | 0.0077 | 4.01 |
| 1.0 | 4.3∙1013 | 0.0065 | 4.32 | |
| 10 | 2.2∙1013 | 0.0064 | 4.75 | |
| σp | 0.1 | 3.4∙1013 | 0.0082 | 3.89 |
| 1.0 | 2.1∙1013 | 0.0072 | 3.72 | |
| 10 | 1.1∙1013 | 0.0072 | 3.98 |
| Parameter | Strain Rate, s−1 | ||
|---|---|---|---|
| 0.1 | 1.0 | 10 | |
| bIII | 4042.44 | 5867.55 | 5408.66 |
| bIV | 732.89 | 737.75 | 2048.08 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Imran, M.; Kühbach, M.; Roters, F.; Bambach, M. Development of a Model for Dynamic Recrystallization Consistent with the Second Derivative Criterion. Materials 2017, 10, 1259. https://doi.org/10.3390/ma10111259
Imran M, Kühbach M, Roters F, Bambach M. Development of a Model for Dynamic Recrystallization Consistent with the Second Derivative Criterion. Materials. 2017; 10(11):1259. https://doi.org/10.3390/ma10111259
Chicago/Turabian StyleImran, Muhammad, Markus Kühbach, Franz Roters, and Markus Bambach. 2017. "Development of a Model for Dynamic Recrystallization Consistent with the Second Derivative Criterion" Materials 10, no. 11: 1259. https://doi.org/10.3390/ma10111259
APA StyleImran, M., Kühbach, M., Roters, F., & Bambach, M. (2017). Development of a Model for Dynamic Recrystallization Consistent with the Second Derivative Criterion. Materials, 10(11), 1259. https://doi.org/10.3390/ma10111259






