A Mesoscopic Analytical Model to Predict the Onset of Wrinkling in Plain Woven Preforms under Bias Extension Shear Deformation
Abstract
:1. Introduction
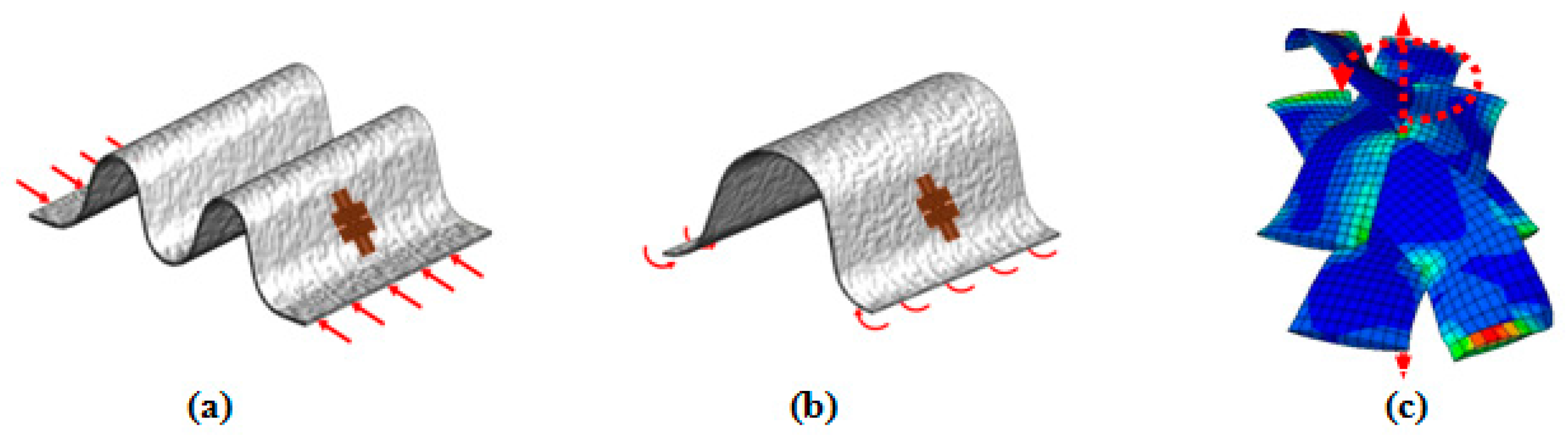
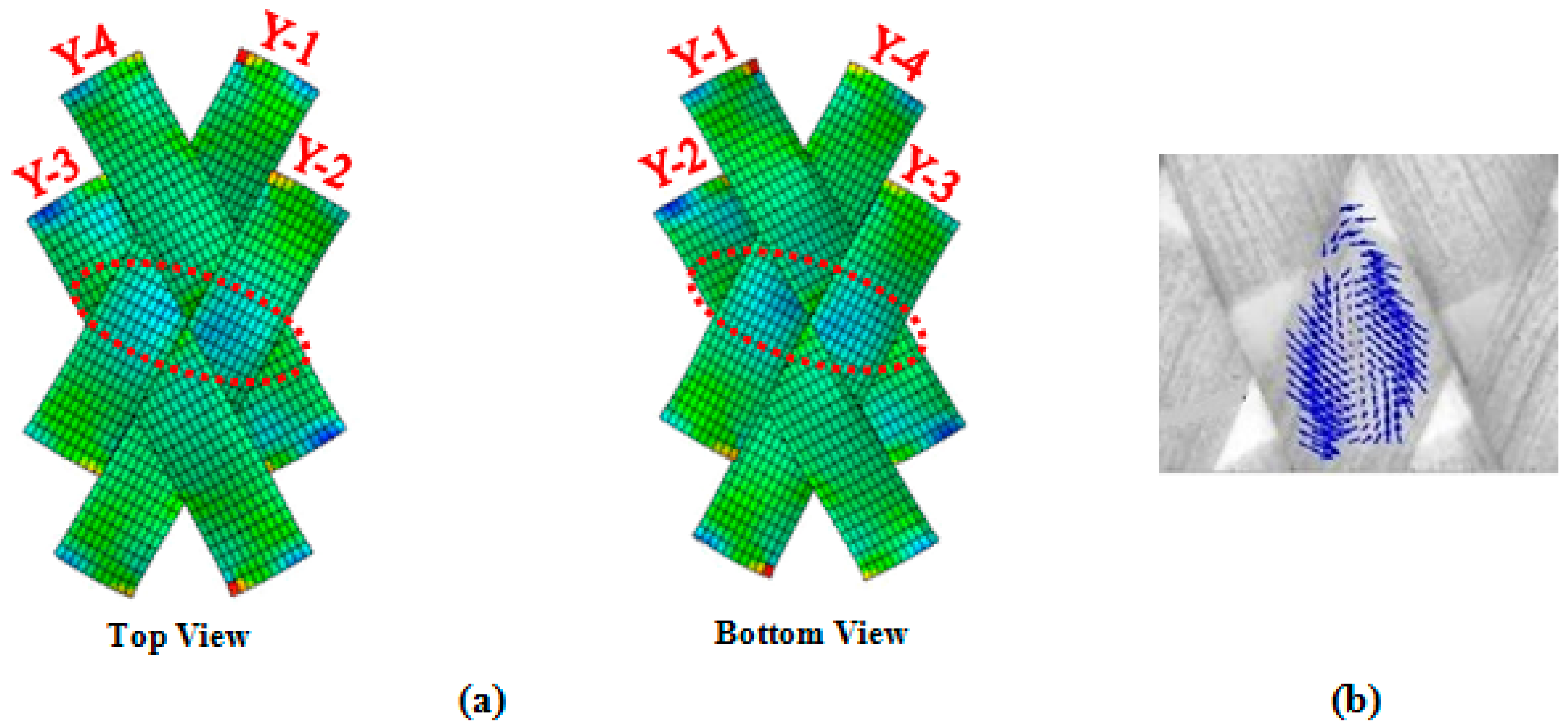
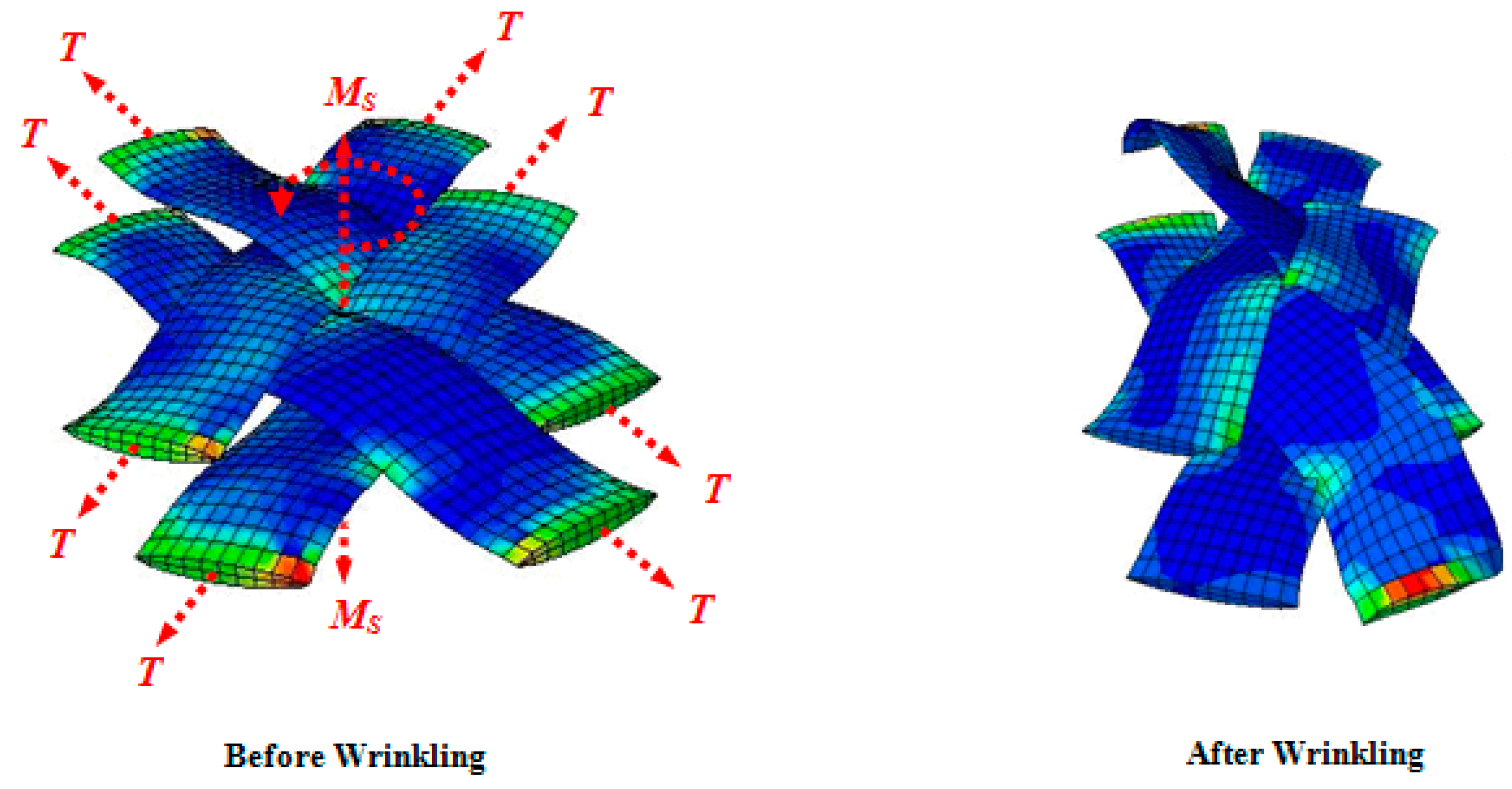
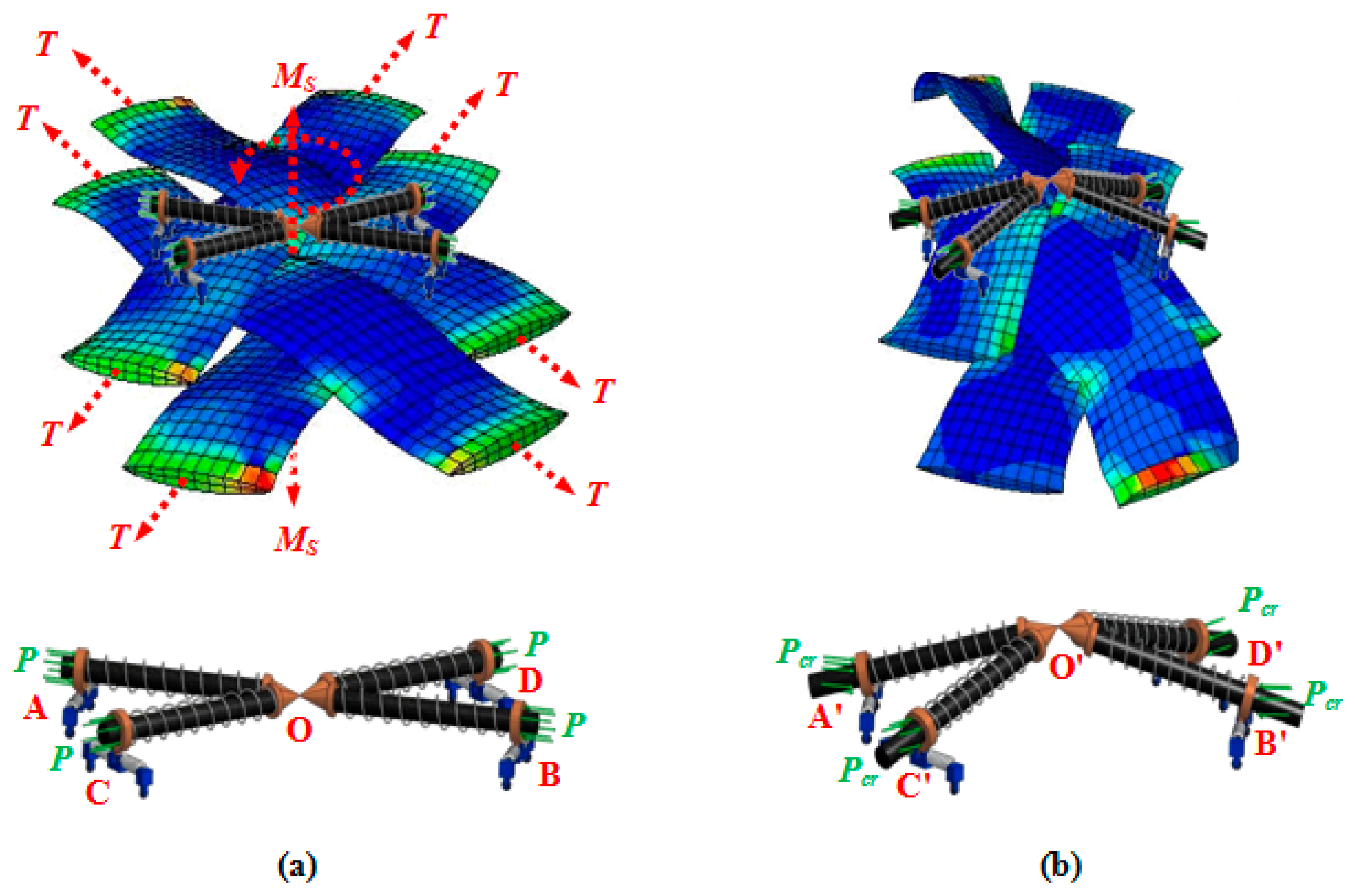
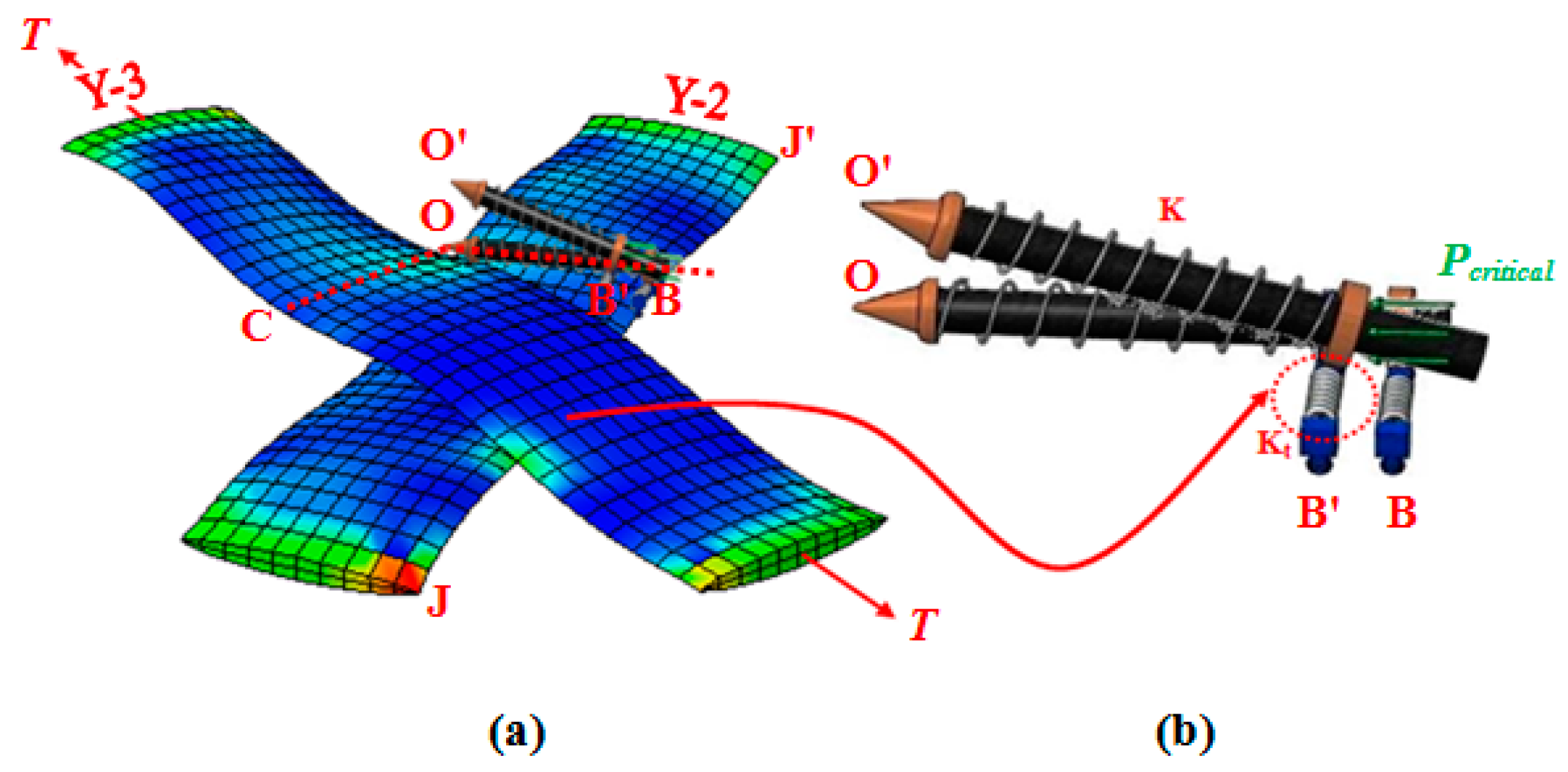
2. Mechanism of Wrinkle Formation in the BET
3. Prediction of Fabric Wrinkling Based on an Instability Analysis
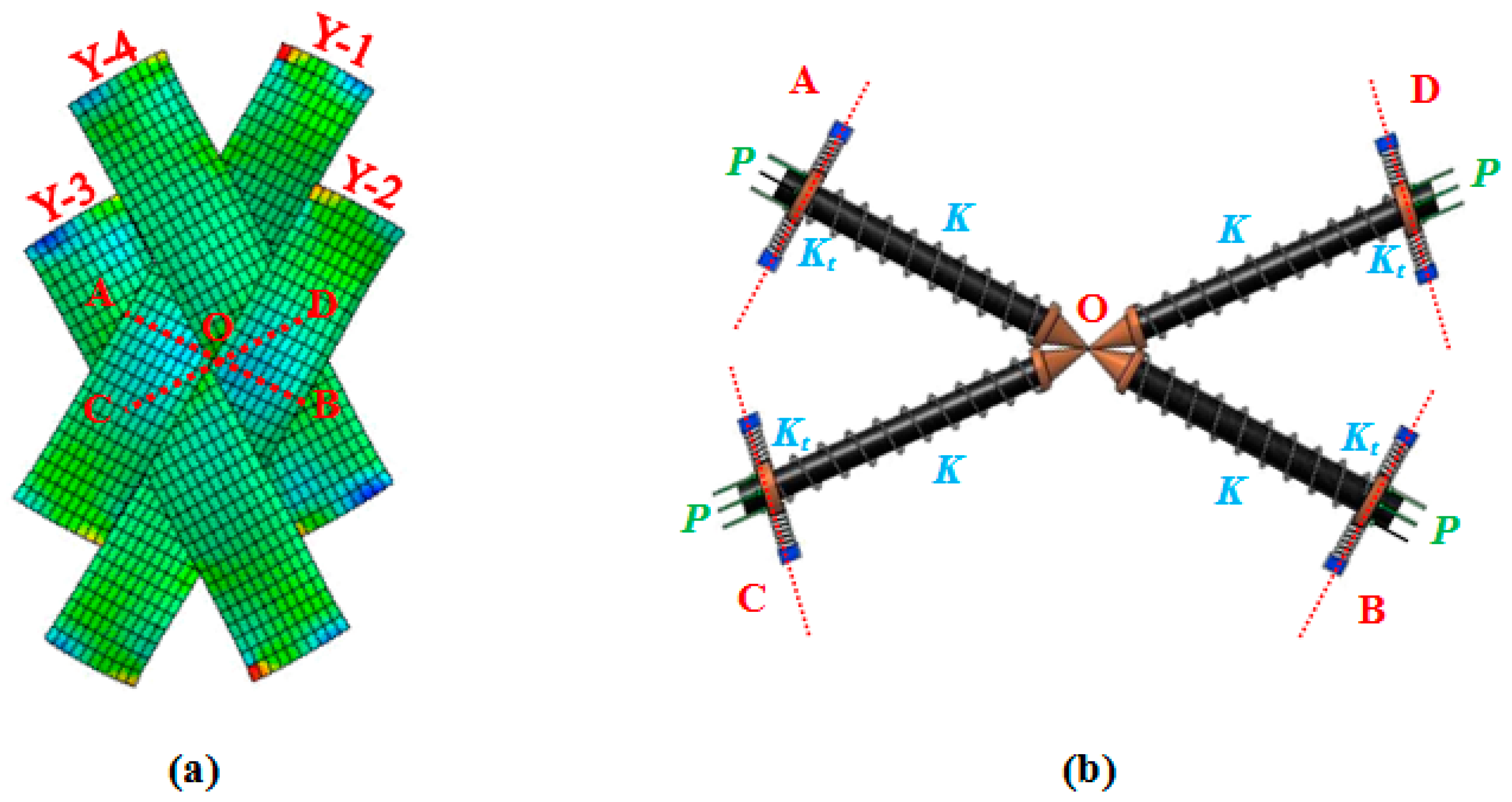
3.1. Analogy-Based/Equivalent Structure
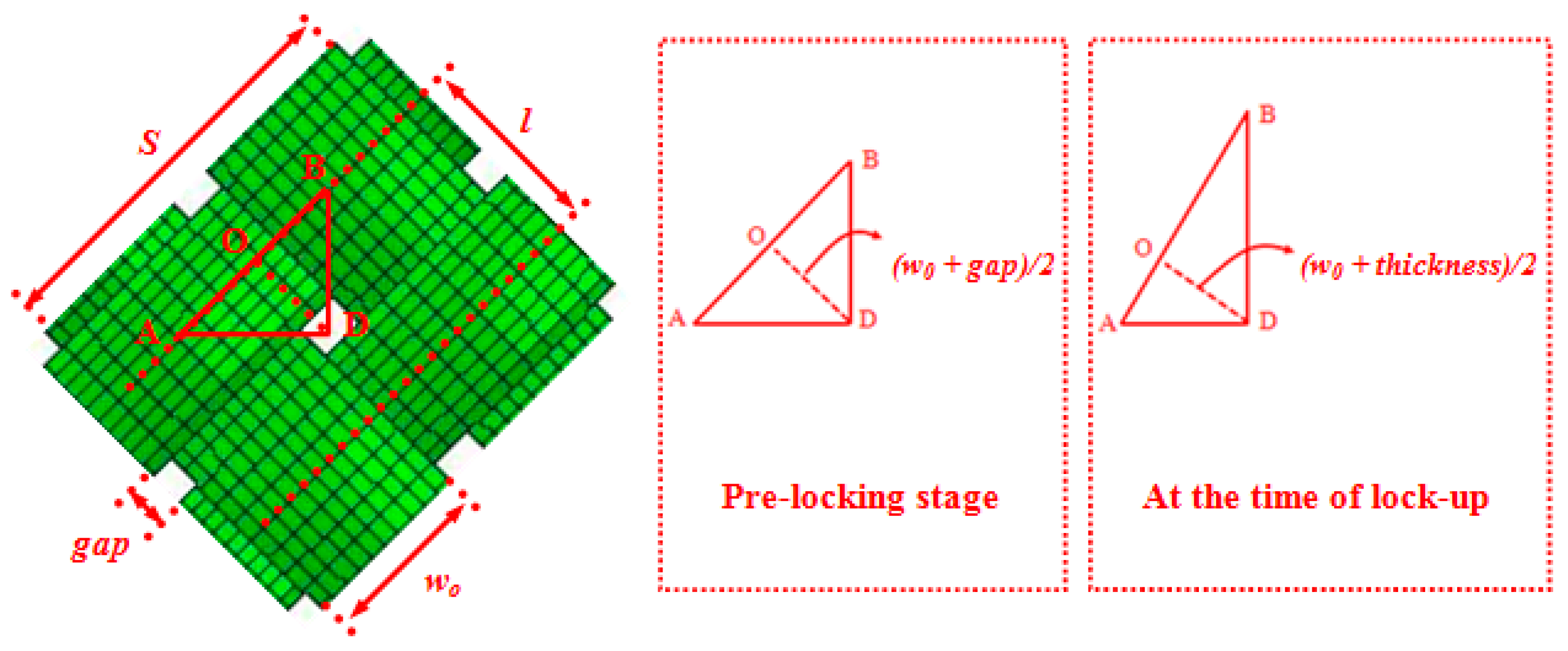
3.1.1. Characterization of Stiffness Elements of the PWCPE Structure
3.2. Determination of Critical Compressive Force (Pcr) and Shear Angle at the Onset of Wrinkling

4. Experimental Evaluation
4.1. Geometric Characterization
4.2. Meso-Mechanical Characterization of the Fabric
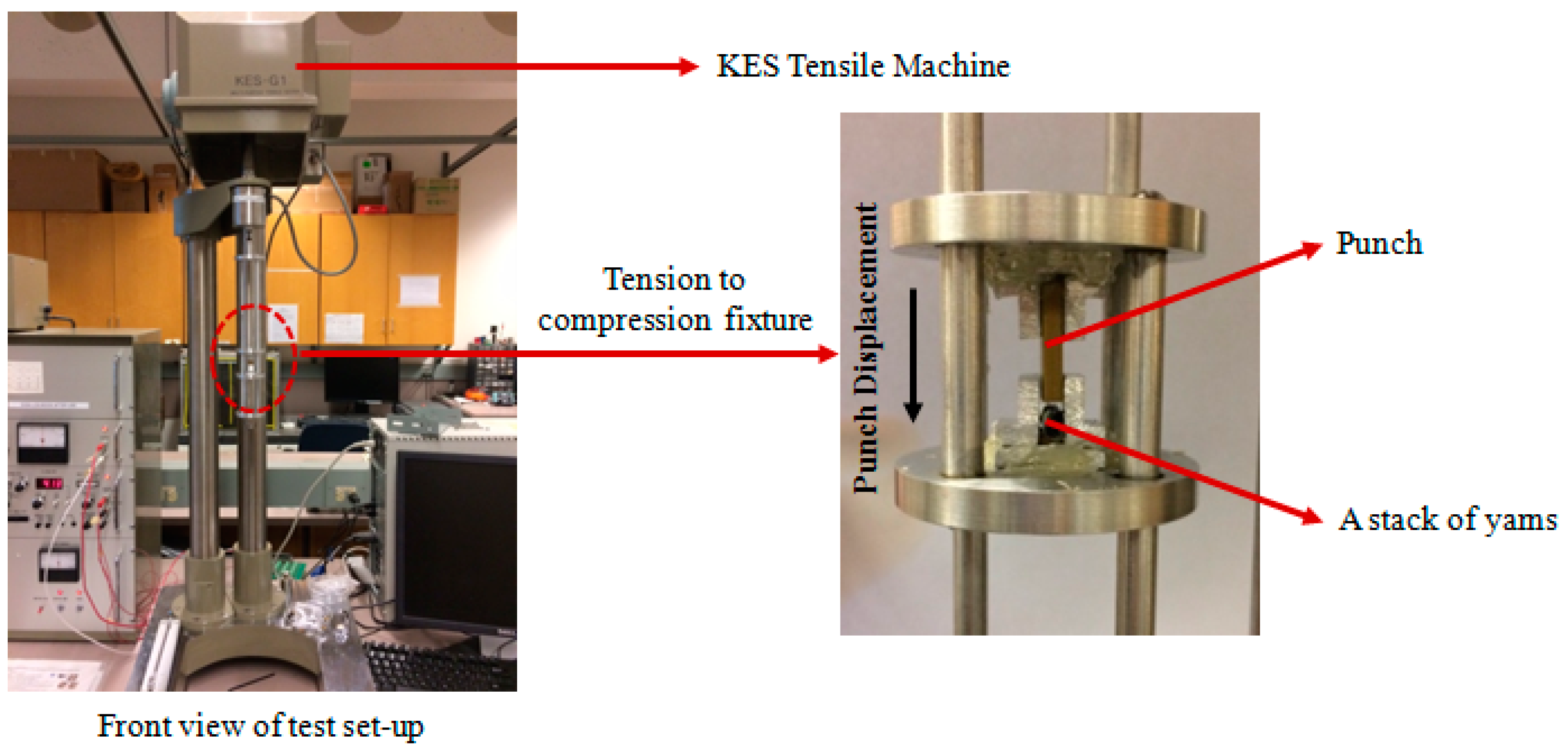
4.2.1. Effective Flexural Rigidity of Yarn in Longitudinal Direction (Qb)
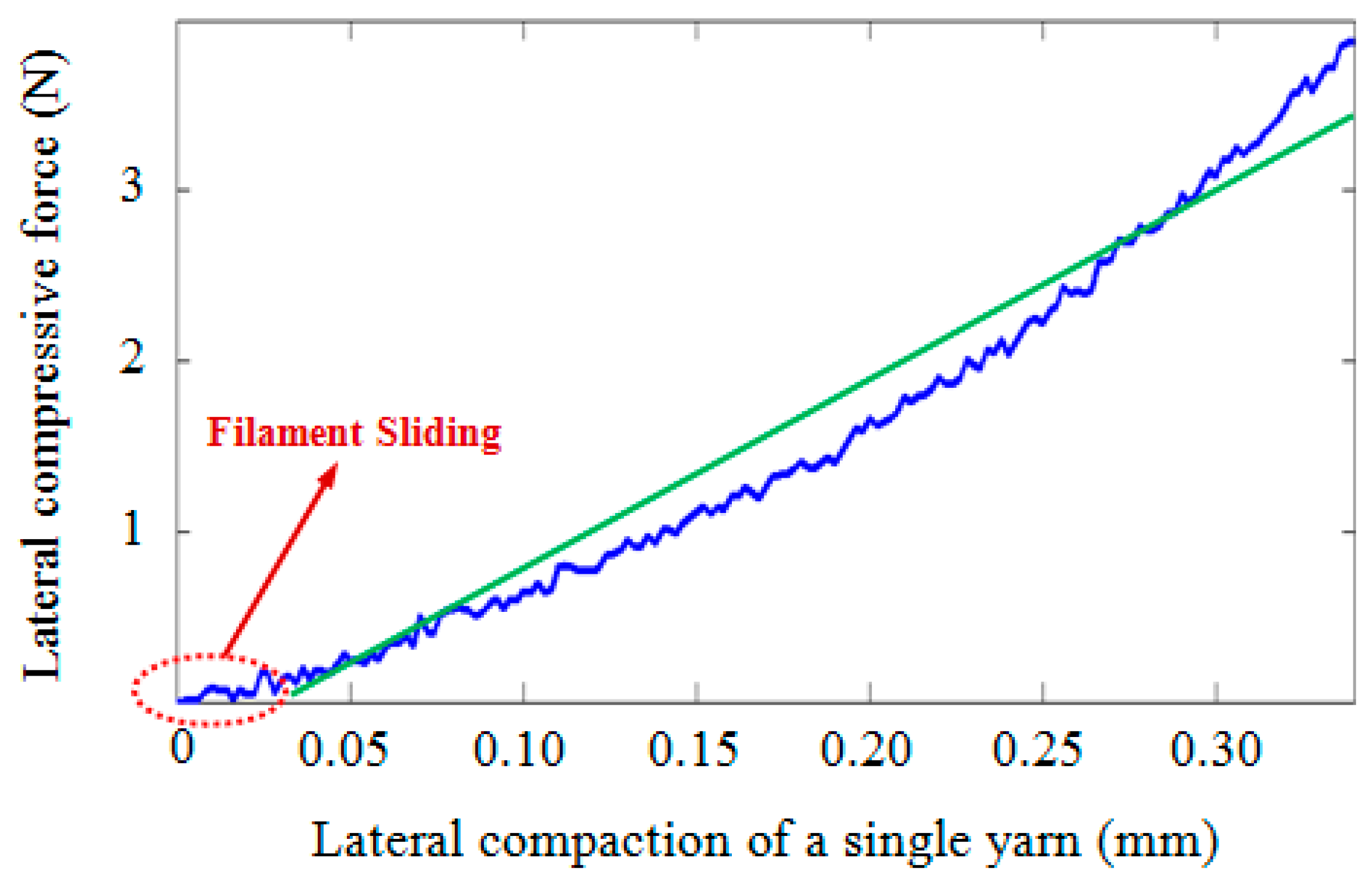
4.2.2. Effective Lateral Stiffness of Yarn (K)
Direct Method
Inverse Method
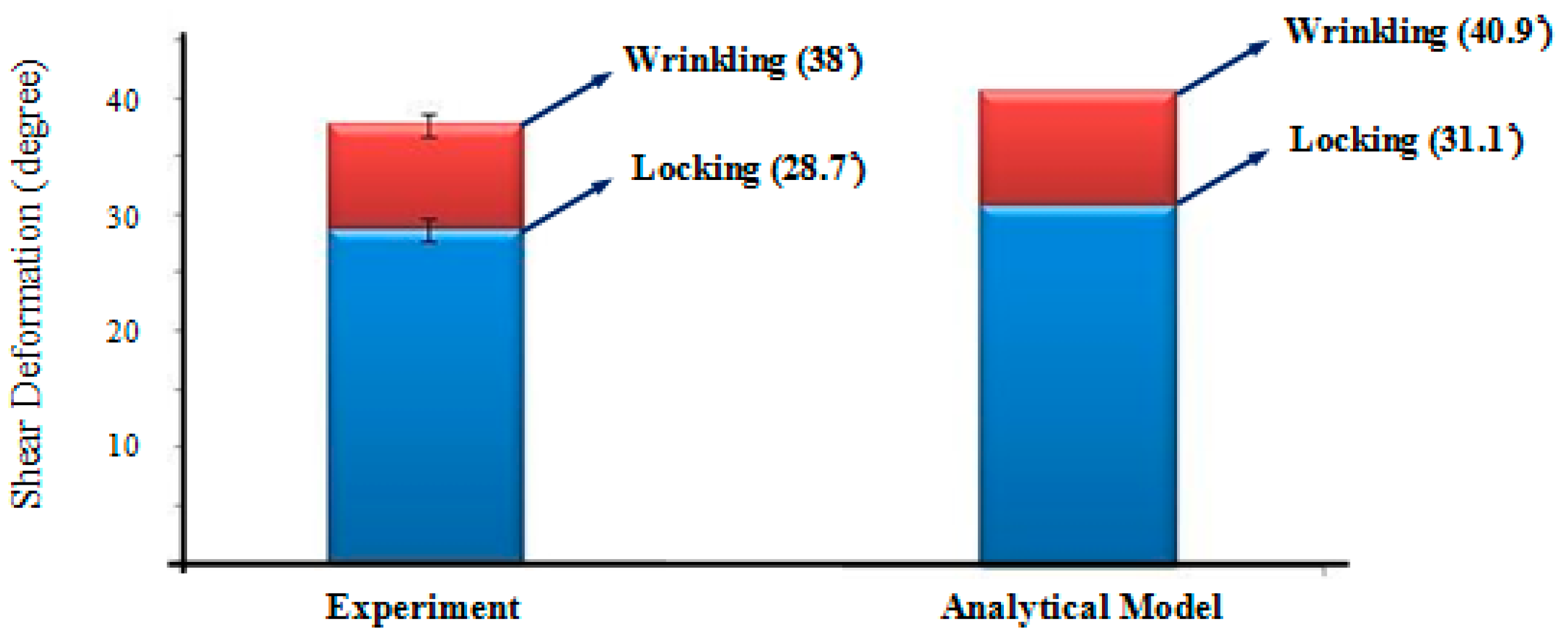
4.3. Bias Extension Test for Validating the Analytical Wrinkle Model
5. Concluding Remarks
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| Locking angle | Pcr | Critical lateral compressive force between yarns | |
| Initial yarn width | Qb | Effective flexural rigidity | |
| Yarn thickness | Deflection of the yarn as a beam at its mid-span | ||
| Initial distance between the central axis of the adjacent parallel yarns | F | An arbitrary vertical force | |
| Kt | Stiffness of rotation springs | T | Tension along the yarn |
| K | Lateral stiffness of yarns | S | Length of yarn in the representative PWCP element |
| P | Quasi-static compressive force between yarns | w | Instantaneous width of yarn |
| Δ | Compaction of the yarn due to lateral compressive force | Lumped parameter notation | |
| θ | Angle of rods with respect to the plane of fabric | Total potential energy of the system | |
| Hessian matrix | Critical yarn compaction | ||
| Wrinkling angle | x | Distance from the constraint to any arbitrary point on the yarn | |
| y(x) | Deflection of the yarn corresponding to x | q | Weight per length of the yarn |
| L’ | Length of the yarn in banding test | Fshear | Shear force |
| dWshear | Increment of the work done on the fabric element | Shear stress | |
| dV | Volume of fabric element |
References
- Chou, T.W.; Ko, F.K. Textile Structural Composites. In Composite Material Series; Elsevier Science Publisher: Amsterdam, The Netherlands, 1988; p. 388. [Google Scholar]
- Zhu, B. Sheet Forming of Woven Textile Composite Preforms: Formability and Wrinkling. Ph.D. Thesis, Hong Kong University of Science and Technology, Hong Kong, China, 2007. [Google Scholar]
- Boisse, P.; Hamila, N.; Vidal-Sallé, E.; Dumont, F. Simulation of wrinkling during textile composite reinforcement forming. Influence of tensile, in-plane shear and bending stiffnesses. Compos. Sci. Technol. 2011, 71, 683–692. [Google Scholar] [CrossRef]
- Boisse, P.; Zouari, B.; Daniel, J.L. Importance of in-plane shear rigidity in finite element analyses of woven fabric composite preforming. Compos. Part A Appl. Sci. Manuf. 2006, 37, 2201–2212. [Google Scholar] [CrossRef]
- Badel, P.; Vidal-Sallé, E.; Boisse, P. Computational determination of in-plane shear mechanical behaviour of textile composite reinforcements. Comput. Mater. Sci. 2007, 40, 439–448. [Google Scholar] [CrossRef]
- Kashani, M.H.; Hosseini, A.; Sassani, F.; Ko, F.K.; Milani, A.S. The Role of Intra-Yarn Shear in Integrated Multi-Scale Deformation Analyses of Woven Fabrics: A Critical Review. Crit. Rev. Solid State Mater. Sci. 2017, 1–20. [Google Scholar] [CrossRef]
- Boisse, P.; Hamila, N.; Guzman-Maldonado, E.; Madeo, A.; Hivet, G.; Dell’Isola, F. The bias-extension test for the analysis of in-plane shear properties of textile composite reinforcements and prepregs: A review. Int. J. Mater. Form. 2017, 10, 473–492. [Google Scholar] [CrossRef]
- Prodromou, A.G.; Chen, J. On the relationship between shear angle and wrinkling of textile composite preforms. Compos. Part A Appl. Sci. Manuf. 1997, 28A, 491–503. [Google Scholar] [CrossRef]
- Lightfoot, J.S.; Wisnom, M.R.; Potter, K. Defects in woven preforms: Formation mechanisms and the effects of laminate design and layup protocol. Compos. Part A Appl. Sci. Manuf. 2013, 51, 99–107. [Google Scholar] [CrossRef]
- Launay, J.; Hivet, G.; Duong, A.V.; Boisse, P. Experimental analysis of the influence of tensions on in plane shear behaviour of woven composite reinforcements. Compos. Sci. Technol. 2008, 68, 506–515. [Google Scholar] [CrossRef]
- Zhu, B.; Yu, T.X.; Teng, J.; Tao, X.M. Theoretical modeling of large shear deformation and wrinkling of plain woven composite. J. Compos. Mater. 2008, 43, 125–138. [Google Scholar] [CrossRef]
- Zhu, B.; Yu, T.X.; Zhang, H.; Tao, X.M. Experimental investigation of formability of woven textile composite preform in stamping operation. Int. J. Mater. Form. 2008, 1, 969–972. [Google Scholar] [CrossRef]
- Liu, L.; Chen, J.; Li, X.; Sherwood, J. Two-dimensional macro-mechanics shear models of woven fabrics. Compos. Part A Appl. Sci. Manuf. 2005, 36, 105–114. [Google Scholar] [CrossRef]
- Kaynia, N. Instability-Induced Transformation of Interfacial Layers in Composites and Its Multifunctional Applications. Ph.D. Disseration, Massachusetts Institute of Technology, Cabbridge, MA, USA, February 2016. [Google Scholar]
- Gambhir, M.L. Stability Analysis and Design of Structures; Springer: Berlin, Germany, 2004. [Google Scholar] [CrossRef]
- Zhu, B.; Yu, T.X.; Tao, X.M. An experimental study of in-plane large shear deformation of woven fabric composite. Compos. Sci. Technol. 2007, 67, 252–261. [Google Scholar] [CrossRef]
- Cornelissen, B.; Akkerman, R. Analysis of yarn bending behaviour. In Proceedings of the 17th International Conference on Composite Materials, Edinburgh, UK, 27–31 July 2009. [Google Scholar]
- Zamani, A.R.; Oyadiji, S.O. Analytical modelling of Kirschner wires in Ilizarov circular external fixator as pretensioned slender beams. J. R. Soc. Interface 2009, 6, 243–256. [Google Scholar] [CrossRef] [PubMed]
- Jones, R.M. Buckling of Bars, Plates and Shells; Bull Ridge Publishing: Ann Arbor, MI, USA, 2006; p. 824. [Google Scholar]
- Perelmuter, A.V.; Slivker, V. Handbook of Mechanical Stability in Engineering; World Scientific Publishing: Toh Tuck Link, Singapore, 2013. [Google Scholar]
- Syerko, E.; Comas-Cardona, S.; Binetruy, C. Models of mechanical properties/behavior of dry fibrous materials at various scales in bending and tension: A review. Compos. Part A Appl. Sci. Manuf. 2012, 43, 1365–1388. [Google Scholar] [CrossRef]
- Haghi Kashani, M.; Hosseini, A.; Sassani, F.; Ko, F.K.; Milani, A. Understanding Different Types of Coupling in Mechanical Behavior of Woven Fabric Reinforcement: A Critical Review and Analysis. Compos. Struct. 2017, 179, 558–567. [Google Scholar] [CrossRef]






| Yarn Material | Geometric Characteristics | Mechanical Characteristics | ||||
|---|---|---|---|---|---|---|
| w0 (mm) | S (mm) | l (mm) | t (mm) | Flexural Rigidity— (N·mm2) | Lateral Stiffness—K (N/mm) | |
| Carbon fiber | 1.4 | 4.1 | 2.05 | 0.355 | 2.75 | 11.06 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hosseini, A.; Kashani, M.H.; Sassani, F.; Milani, A.S.; Ko, F. A Mesoscopic Analytical Model to Predict the Onset of Wrinkling in Plain Woven Preforms under Bias Extension Shear Deformation. Materials 2017, 10, 1184. https://doi.org/10.3390/ma10101184
Hosseini A, Kashani MH, Sassani F, Milani AS, Ko F. A Mesoscopic Analytical Model to Predict the Onset of Wrinkling in Plain Woven Preforms under Bias Extension Shear Deformation. Materials. 2017; 10(10):1184. https://doi.org/10.3390/ma10101184
Chicago/Turabian StyleHosseini, Abbas, Masoud Haghi Kashani, Farrokh Sassani, Abbas S. Milani, and Frank Ko. 2017. "A Mesoscopic Analytical Model to Predict the Onset of Wrinkling in Plain Woven Preforms under Bias Extension Shear Deformation" Materials 10, no. 10: 1184. https://doi.org/10.3390/ma10101184
APA StyleHosseini, A., Kashani, M. H., Sassani, F., Milani, A. S., & Ko, F. (2017). A Mesoscopic Analytical Model to Predict the Onset of Wrinkling in Plain Woven Preforms under Bias Extension Shear Deformation. Materials, 10(10), 1184. https://doi.org/10.3390/ma10101184






