Thermal Stability of Hexamethyldisiloxane (MM) for High-Temperature Organic Rankine Cycle (ORC) †
Abstract
:1. Introduction
2. Methodology
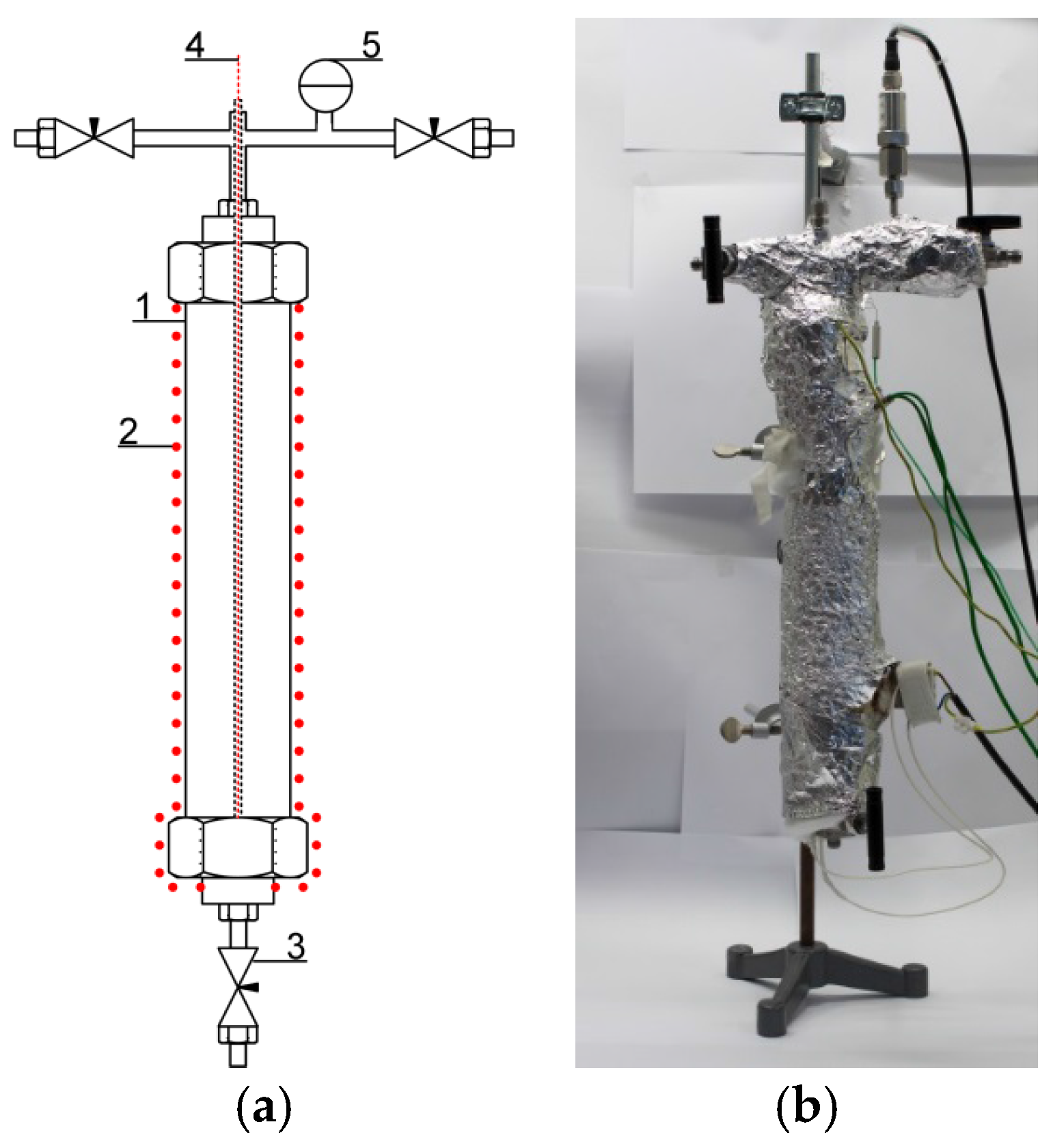
2.1. Test Rig and Experimental Procedure
2.2. Evaluation Method
3. Discussion
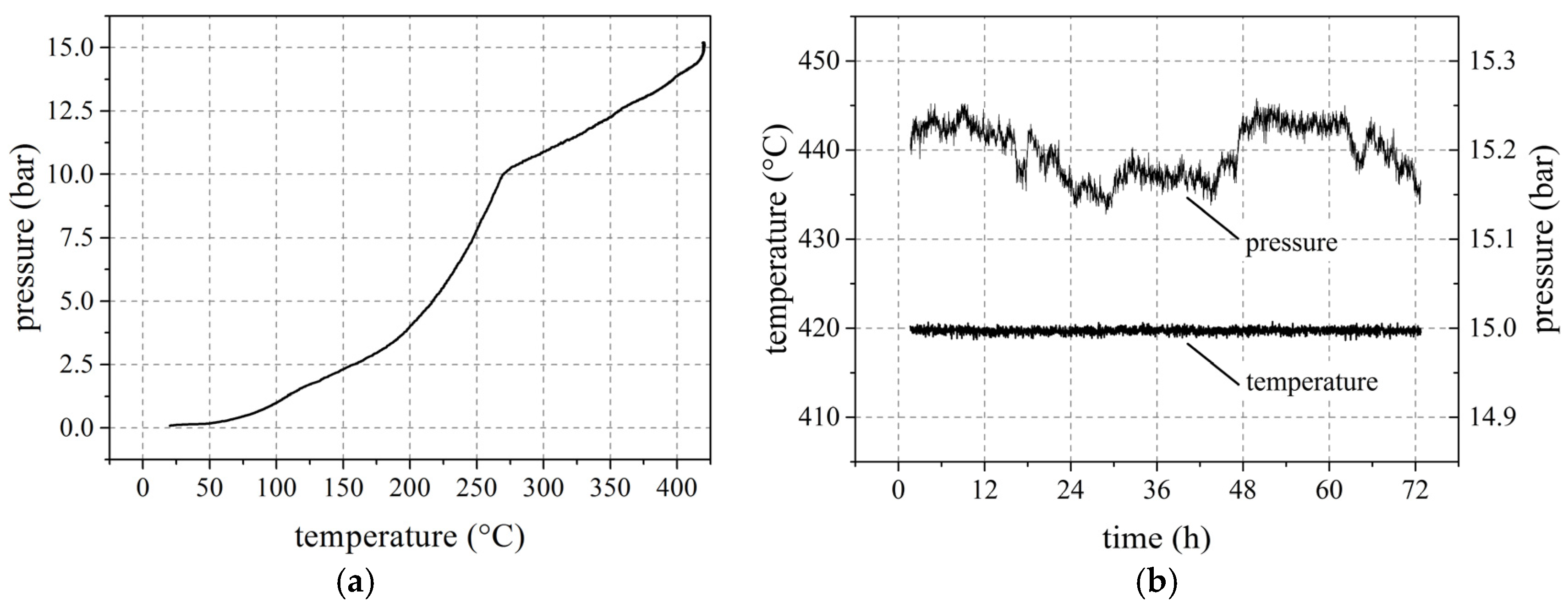
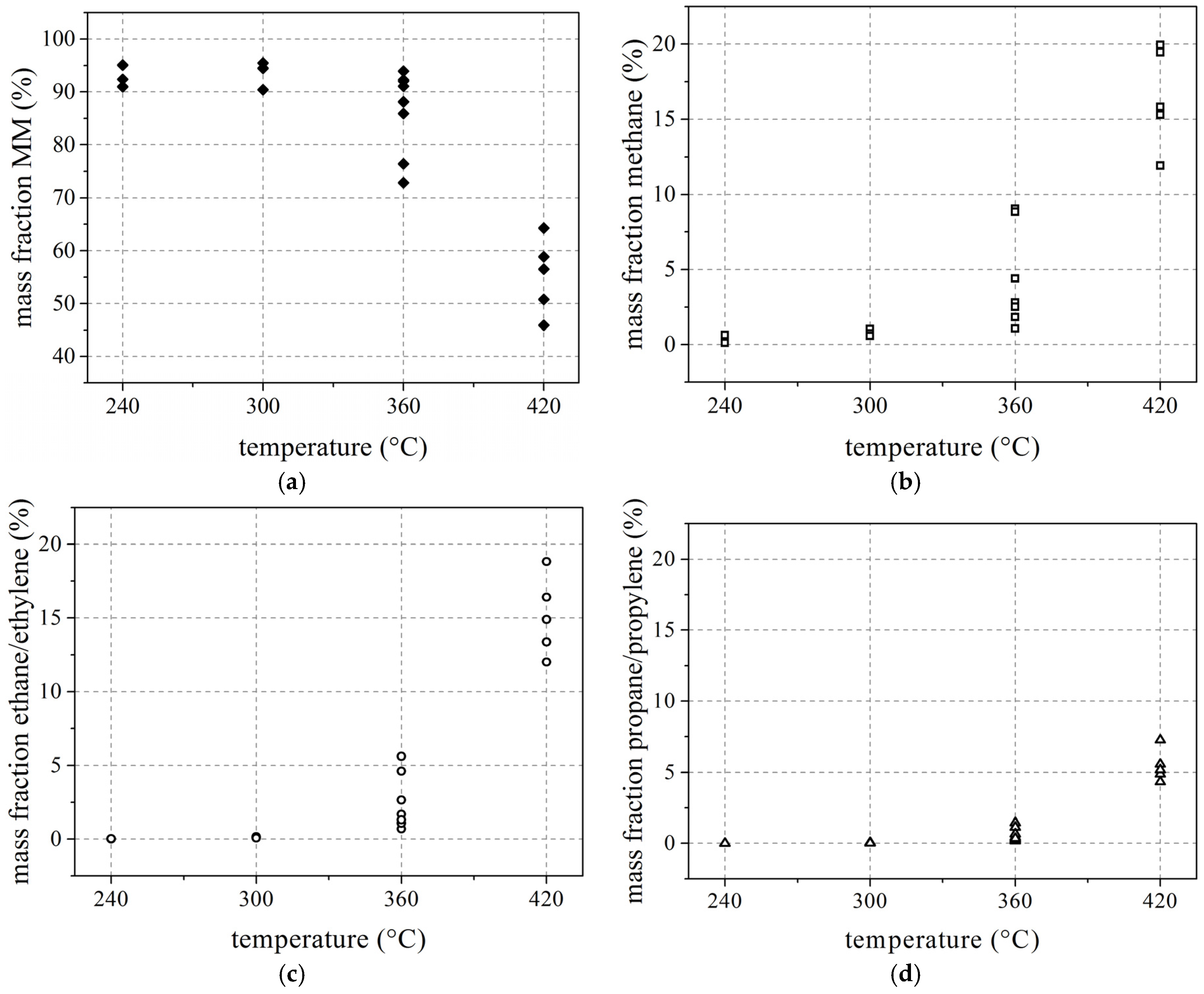
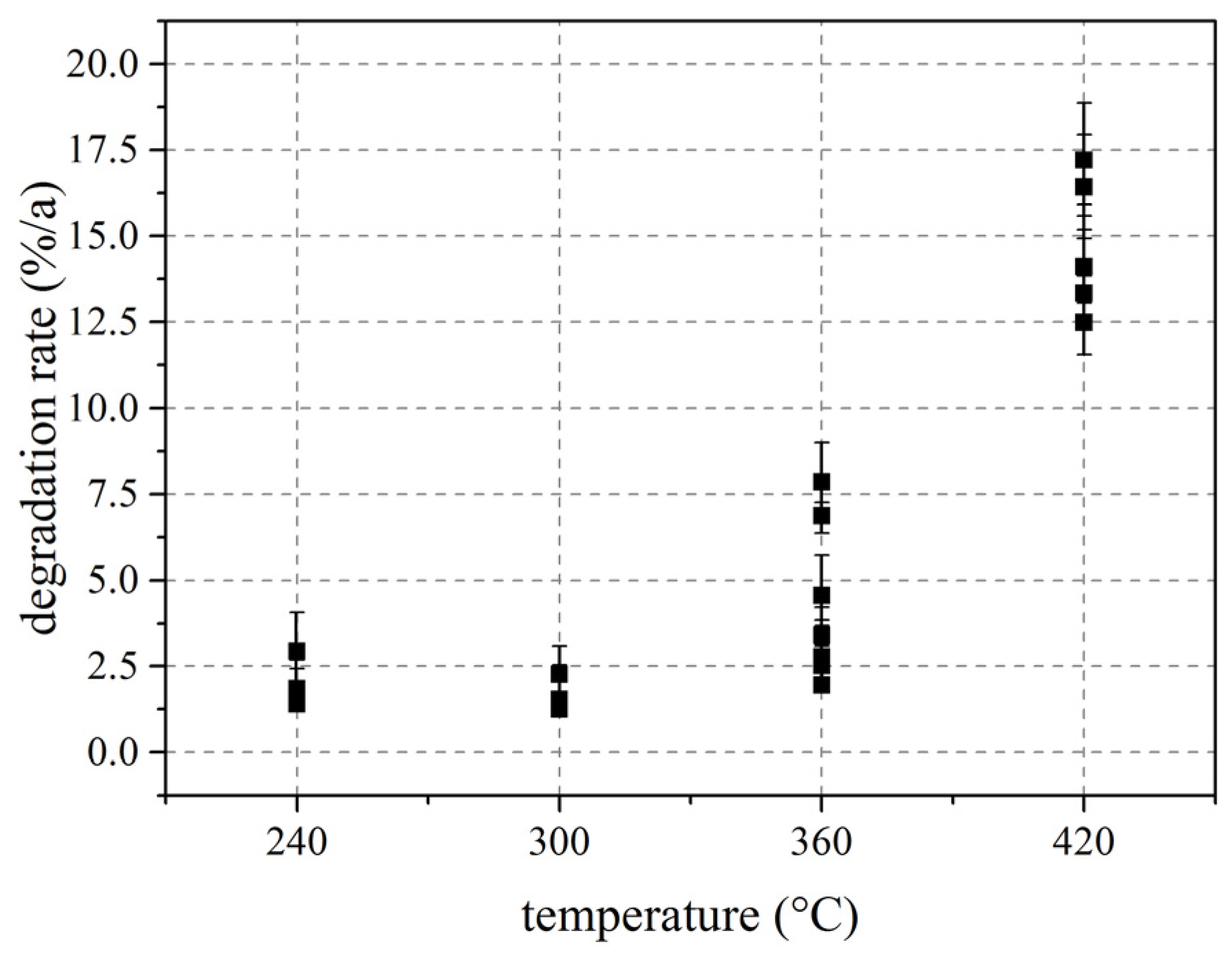
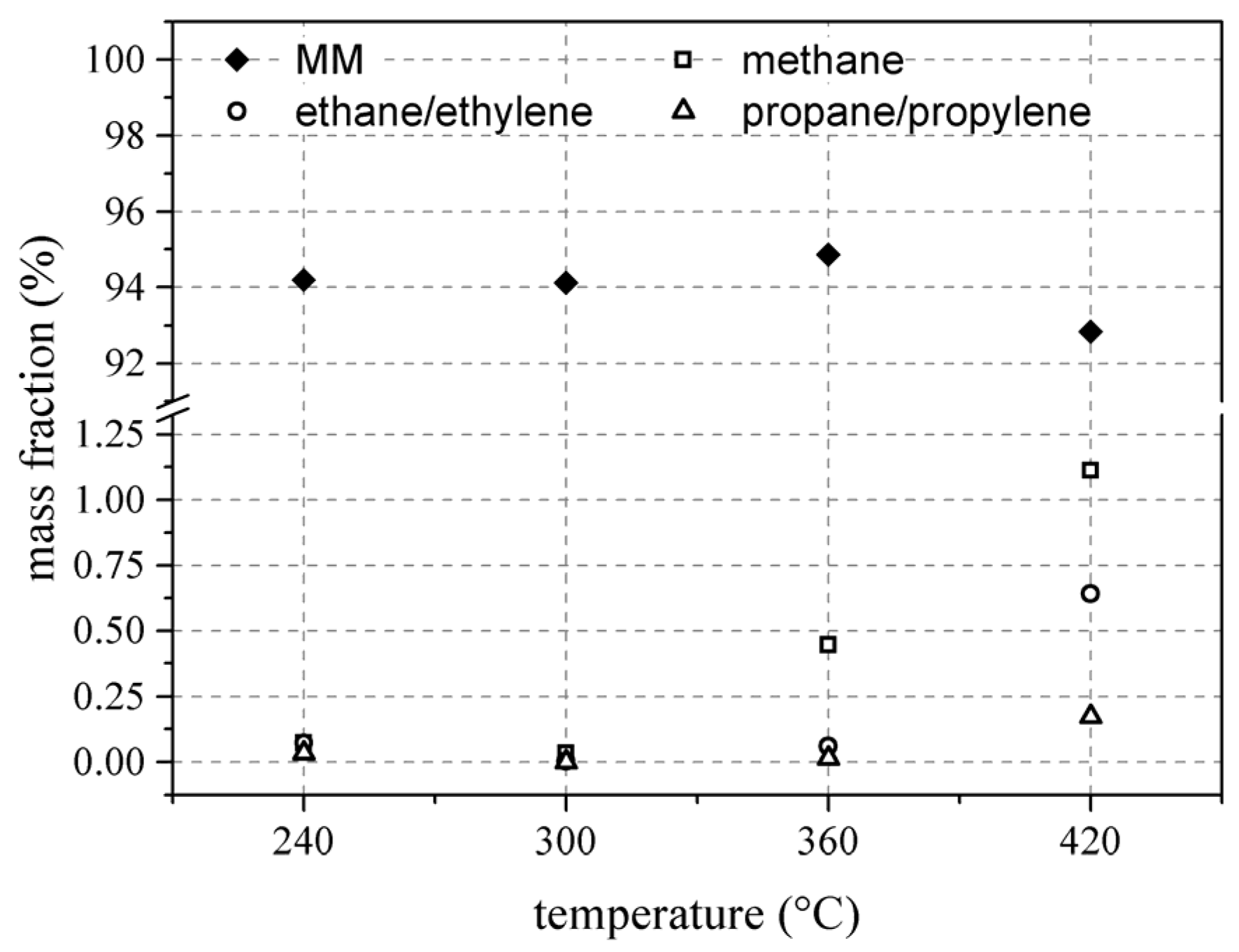
3.1. Influence of Temperature on Thermal Degradation
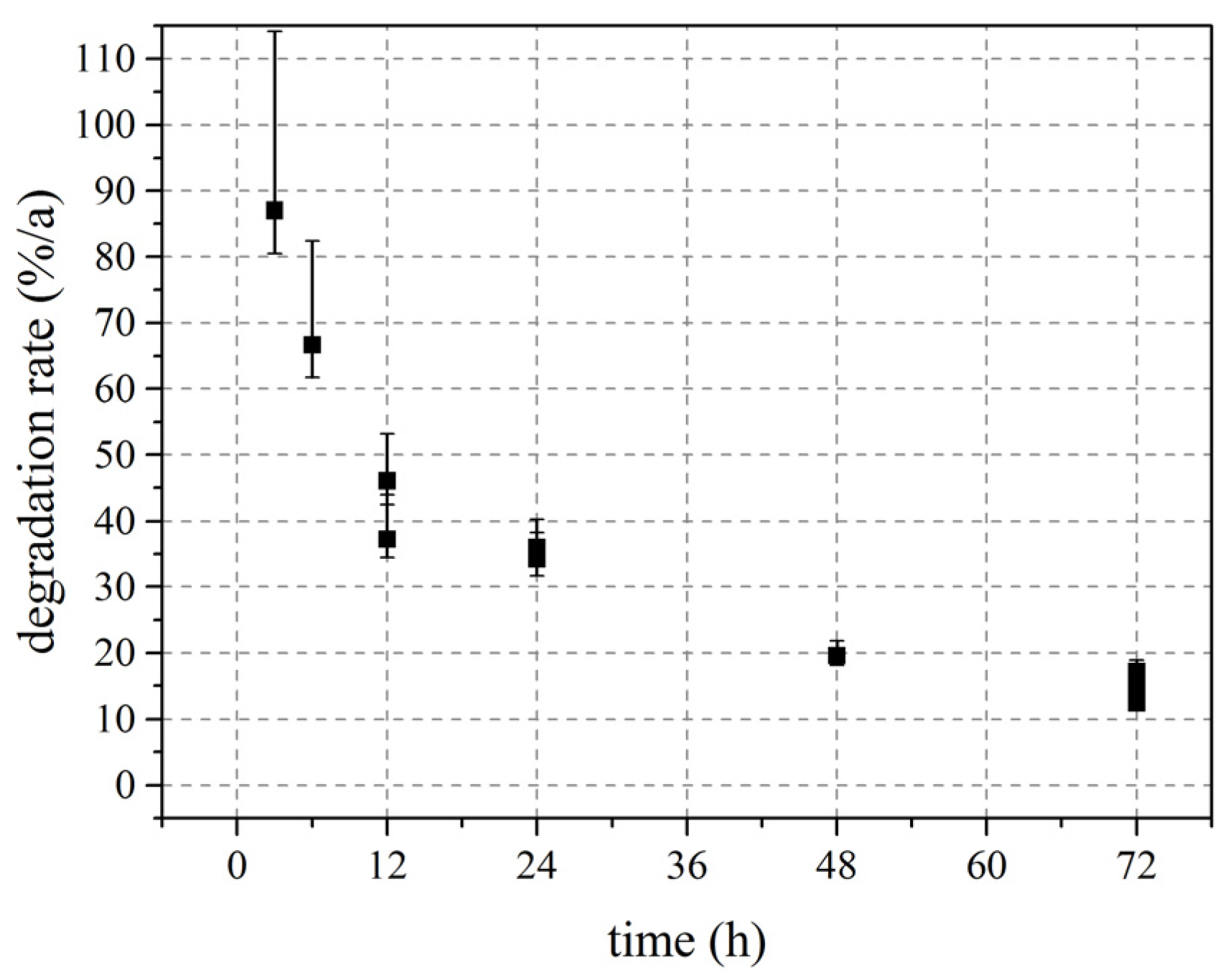
3.2. Influence of Time on Thermal Degradation
4. Materials and Methods
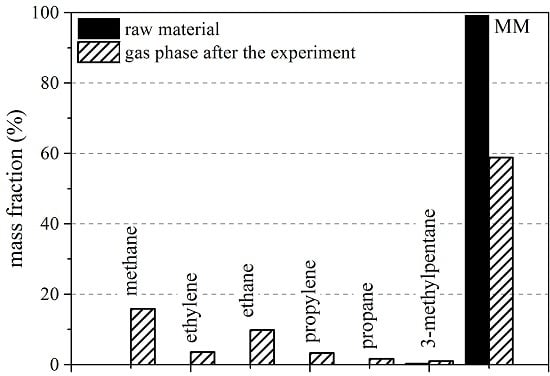
4.1. Qualitative Evaluation of Degradation Products
4.2. Quantitative Evaluation of Degradation
- volume of the reactor and mass of MM in the reactor, ±2.0%;
- mass fraction of MM in liquid and vapor phase, ±2.0%;
- density of MM in liquid and vapor phase, ±1.0%.
4.3. Impact on the Set-Up of Organic Rankine Cycle Power Units
5. Conclusions
- The main degradation products of MM are low molecular hydrocarbons like methane, ethane/ethylene and propane/propylene.
- The degradation rate is strongly influenced not only by the temperature but also by the retention time.
- MM is stable up to a temperature of 300 °C with annual degradation rates of less than 3.5%.
- Methane can be used as indicator for ongoing thermal degradation of MM.
- The design of direct contact evaporators based on MM requires special care concerning film temperature and avoiding hot spots in the evaporator.
- Free water molecules and contaminants may influence the degradation rates significantly and, therefore, a special start-up procedure including a boil-out of those substances is necessary.
- Thermal stability can be included as parameter in ORC working fluid database and the maximum temperature can be used as boundary condition for ORC design and simulation.
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| C | Carbon |
| MDM | Octamethyltrisiloxane |
| MD2M | Decamethyltetrasiloxane |
| MM | Hexamethyldisiloxane |
| ORC | Organic Rankine cycle |
| Si | Silicon |
| t | Time |
| T | Temperature |
References
- Obernberger, I.; Thornhofer, P.; Reisenhofer, E. Description and evaluation of the new 1000 kWel Organic Rankine Cycle process integrated in the biomass CHP plant in Lienz, Austria. Euroheat Power 2002, 10, 18–25. [Google Scholar]
- Obernberger, I. Decentralized biomass combustion: State of the art and future development. Biomass Bioenergy 1998, 14, 33–56. [Google Scholar] [CrossRef]
- Preißinger, M.; Heberle, F.; Brüggemann, D. Thermodynamic analysis of double-stage biomass fired Organic Rankine Cycle for micro-cogeneration. Int. J. Energy Res. 2012, 36, 944–952. [Google Scholar] [CrossRef]
- Mang, T.; Dresel, W. Lubricants and Lubrication, 2nd ed.; Wiley-VCH: Weinheim, Germany, 2007. [Google Scholar]
- Yang, K.; Zhang, H.; Song, S.; Yang, F.; Liu, H.; Zhao, G.; Zhang, J.; Yao, B. Effects of Degree of Superheat on the Running Performance of an Organic Rankine Cycle (ORC) Waste Heat Recovery System for Diesel Engines under Various Operating Conditions. Energies 2014, 7, 2123–2145. [Google Scholar] [CrossRef]
- Yang, K.; Zhang, H.; Song, S.; Zhang, J.; Wu, Y.; Zhang, Y.; Wang, H.; Chang, Y.; Bei, C. Performance Analysis of the Vehicle Diesel Engine-ORC Combined System Based on a Screw Expander. Energies 2014, 7, 3400–3419. [Google Scholar] [CrossRef]
- Zhang, C.; Shu, G.; Tian, H.; Wei, H.; Liang, X. Comparative study of alternative ORC-based combined power systems to exploit high temperature waste heat. Energy Convers. Manage. 2015, 89, 541–554. [Google Scholar] [CrossRef]
- Camporeale, S.M.; Pantaleo, A.M.; Ciliberti, P.D.; Fortunato, B. Cycle configuration analysis and techno-economic sensitivity of biomass externally fired gas turbine with bottoming ORC. Energy Convers. Manag. 2015, 105, 1239–1250. [Google Scholar] [CrossRef]
- Chowdhury, J.; Nguyen, B.; Thornhill, D. Modelling of Evaporator in Waste Heat Recovery System using Finite Volume Method and Fuzzy Technique. Energies 2015, 8, 14078–14097. [Google Scholar] [CrossRef]
- Guillen, D.; Klockow, H.; Lehar, M.; Freund, S.; Jackson, J. Development of a Direct Evaporator for the Organic Rankine Cycle. In Energy Technology 2011; Neelameggham, N.R., Belt, C.K., Jolly, M., Reddy, R.G., Yurko, J.A., Eds.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2011; pp. 25–35. [Google Scholar]
- Bei, C.; Zhang, H.; Yang, F.; Song, S.; Wang, E.; Liu, H.; Chang, Y.; Wang, H.; Yang, K. Performance Analysis of an Evaporator for a Diesel Engine—Organic Rankine Cycle (ORC) Combined System and Influence of Pressure Drop on the Diesel Engine Operating Characteristics. Energies 2015, 8, 5488–5515. [Google Scholar] [CrossRef]
- Weith, T.; Heberle, F.; Preißinger, M.; Brüggemann, D. Performance of Siloxane Mixtures in a High-Temperature Organic Rankine Cycle Considering the Heat Transfer Characteristics during Evaporation. Energies 2014, 7, 5548–5565. [Google Scholar] [CrossRef]
- Colonna, P.; Casati, E.; Trapp, C.; Mathijssen, T.; Larjola, J.; Turunen-Saaresti, T.; Uusitalo, A. Organic Rankine Cycle Power Systems: From the Concept to Current Technology, Applications, and an Outlook to the Future. J. Eng. Gas Turbines Power 2015, 137. [Google Scholar] [CrossRef]
- Di Nanno, L.R.; Di Bella, F.A.; Koplow, M.D. An RC-1 Organic Rankine Bottoming Cycle for an Adiabatic Diesel Engine; Technical Report, DOE/NASA/0302-1; Nasa Lewis Research Center: Cleveland, OH, USA, 1983. [Google Scholar]
- Erhart, T.; Gölz, J.; Eickler, U.; Van Den Broek, M. Fluid stability in large scale ORCs using siloxanes-long-term experiences and fluid recycling. In Proceedings of the 3rd International Seminar on ORC Power Systems, ASME-ORC 2015, Brussels, Belgium, 12–14 October 2015.
- Colonna, P.; Nannan, N.; Guardone, A.; Lemmon, E. Multiparameter equations of state for selected siloxanes. Fluid Phase Equilib. 2006, 244, 193–211. [Google Scholar] [CrossRef]
- Angelino, G. Cyclic Methylsiloxanes as Working Fluids for Space Power Cycles. J. Sol. Energy Eng. 1993, 115, 130–137. [Google Scholar] [CrossRef]
- Dvornic, P.R. Thermal properties of polysiloxanes. In Silicon Containing Polymers: The Science and Technology of Their Synthesis and Applications; Jones, R.G., Ed.; Kluwer Acad. Publ.: Dordrecht, The Netherlands, 2000; pp. 185–212. [Google Scholar]
- Dvornic, P.R. High temperature stability of cyclosiloxanes. In Silicon Compounds: Silanes and Silicones: A Survey of Properties and Chemistry, 2nd ed.; Gelest Inc.: Morrisville, PA, USA, 2008; pp. 441–454. [Google Scholar]
- Ginosar, D.M.; Petkovic, L.M.; Guillen, D.P. Thermal Stability of Cyclopentane as an Organic Rankine Cycle Working Fluid. Energy Fuels 2011, 25, 4138–4144. [Google Scholar] [CrossRef]
- Sealed Glass Tube Method to Test the Chemical Stability of Materials for Use within Refrigerant Systems; American Society of Heating, Refrigerating and Air-Conditioning Engineers, Inc.: Atlanta, GA, USA, 2007.
- Buravtsev, N.; Grigorev, A.; Kolbanovskii, Y.; Ovsyannikov, A. Thermal and thermooxidative stability of ozone-safe freons. Russ. J. Org. Chem. 1994, 30, 1880–1890. [Google Scholar]
- Grassie, N.; Macfarlane, I. The thermal degradation of polysiloxanes—I. Poly(dimethylsiloxane). Eur. Polym. J. 1978, 14, 875–884. [Google Scholar] [CrossRef]
- Grassie, N.; Macfarlane, I.; Francey, K. The thermal degradation of polysiloxanes—II. Poly(methylphenylsiloxane). Eur. Polym. J. 1979, 15, 415–422. [Google Scholar] [CrossRef]
- Deshpande, G.; Rezac, M.E. Kinetic aspects of the thermal degradation of poly(dimethyl siloxane) and poly(dimethyl diphenyl siloxane). Polym. Degrad. Stab. 2002, 76, 17–24. [Google Scholar] [CrossRef]
- Zhou, W.; Yang, H.; Guo, X.; Lu, J. Thermal degradation behaviors of some branched and linear polysiloxanes. Polym. Degrad. Stab. 2006, 91, 1471–1475. [Google Scholar] [CrossRef]
- Kuramochi, H.; Imai, F.; Kondoh, H.; Kunimori, K.; Uchijima, T.; Nozoye, H. Decomposition of cyclopentane on Ni(755): Peculiar decomposition behavior of cyclopentane. Surf. Sci. 1993, 287–288, 217–221. [Google Scholar]
- Angelino, G.; Invernizzi, C.M.; Macchi, E. Organic working fluid optimization for space power cycles. In Modern Research Topics in Aerospace Propulsion: In honor of Corrado Casci; Angelino, G., Ed.; Springer: New York, NY, USA, 1991; pp. 297–326. [Google Scholar]
- Calderazzi, L.; Di Paliano, P.C. Thermal stability of R-134a, R-141b, R-13I1, R-7146, R-125 associated with stainless steel as a containing material. Int. J. Refrig. 1997, 20, 381–389. [Google Scholar] [CrossRef]
- Pasetti, M.; Invernizzi, C.M.; Iora, P. Thermal stability of working fluids for organic Rankine cycles: An improved survey method and experimental results for cyclopentane, isopentane and n-butane. Appl. Therm. Eng. 2014, 73, 764–774. [Google Scholar] [CrossRef]
- Lasala, S.; Invernizzi, C.; Iora, P.; Chiesa, P.; Macchi, E. Thermal Stability Analysis of Perfluorohexane. Energy Procedia 2015, 75, 1575–1582. [Google Scholar] [CrossRef]
- Stephan, P.; Mayinger, F.; Schaber, K.; Stephan, K. Thermodynamik: Grundlagen und Technische Anwendungen; Band 1: Einstoffsysteme, 18th ed.; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Manders, W.F.; Bellama, J.M. Multiphoton infrared laser-induced degradation of polydimethylsiloxane and hexamethyldisiloxane. J. Polym. Sci. Polym. Chem. Ed. 1985, 23, 351–357. [Google Scholar] [CrossRef]
- Heberle, F.; Brüggemann, D.; Obermeier, A. Mögliche Emissionen bei der Strom- und Wärmeerzeugung aus Geothermie durch den Einsatz von F-Gasen im Energiewandlungsprozess mittels ORC; Climate Change 16/2012; Umweltbundesamt: Dessau-Roßlau, Germany, 2012. (In German) [Google Scholar]
- Benato, A.; Kærn, M.R.; Pierobon, L.; Stoppato, A.; Haglind, F. Analysis of hot spots in boilers of organic Rankine cycle units during transient operation. Appl. Energy 2015, 151, 119–131. [Google Scholar] [CrossRef]


© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Preißinger, M.; Brüggemann, D. Thermal Stability of Hexamethyldisiloxane (MM) for High-Temperature Organic Rankine Cycle (ORC). Energies 2016, 9, 183. https://doi.org/10.3390/en9030183
Preißinger M, Brüggemann D. Thermal Stability of Hexamethyldisiloxane (MM) for High-Temperature Organic Rankine Cycle (ORC). Energies. 2016; 9(3):183. https://doi.org/10.3390/en9030183
Chicago/Turabian StylePreißinger, Markus, and Dieter Brüggemann. 2016. "Thermal Stability of Hexamethyldisiloxane (MM) for High-Temperature Organic Rankine Cycle (ORC)" Energies 9, no. 3: 183. https://doi.org/10.3390/en9030183
APA StylePreißinger, M., & Brüggemann, D. (2016). Thermal Stability of Hexamethyldisiloxane (MM) for High-Temperature Organic Rankine Cycle (ORC). Energies, 9(3), 183. https://doi.org/10.3390/en9030183