Design and Implementation of Three-Winding Coupled Inductor Applied in High Step-Up DC/DC Converter Combined with Voltage Multipliers
Abstract
1. Introduction
- With analysis of the operating modes of the converter, accurate models and simplified models for currents in the three windings are established. (Section 2)
- A design methodology for the coupled inductor is established. The boost inductance of the converter is determined based on the ripple in the input current of the converter. Furthermore, a product areas (AP) method of selecting the magnetic core is obtained. The impact of the winding arrangement on the coupled inductor and a method of estimating losses in the three windings are achieved also. (Section 3)
- A prototype of a 200 W DC/DC converter with an input of 20 V and output of 200 V is prepared and tested. The correctness of the current models and design method are verified. (Section 4)
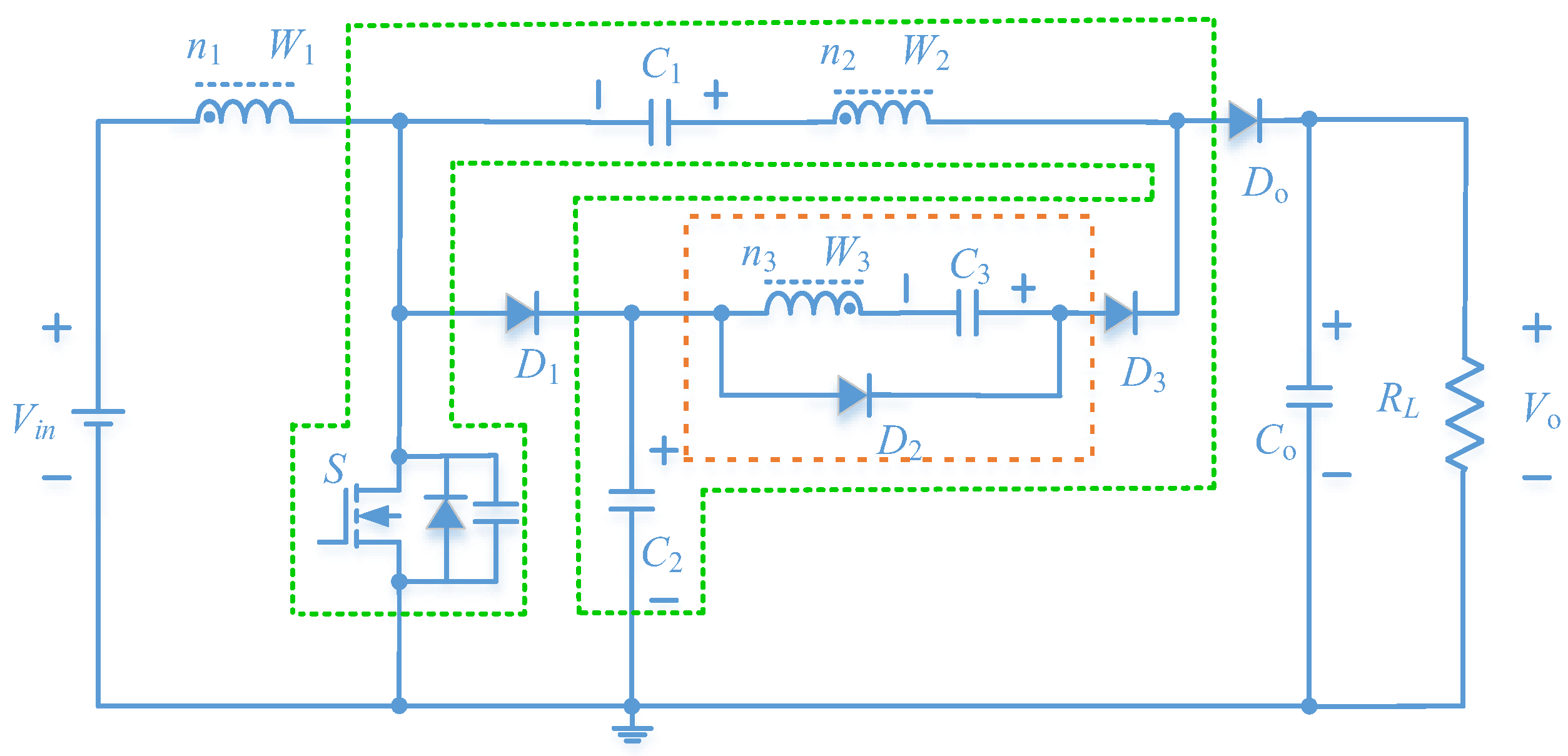
2. Current Models of the Three-Winding Coupled Inductor
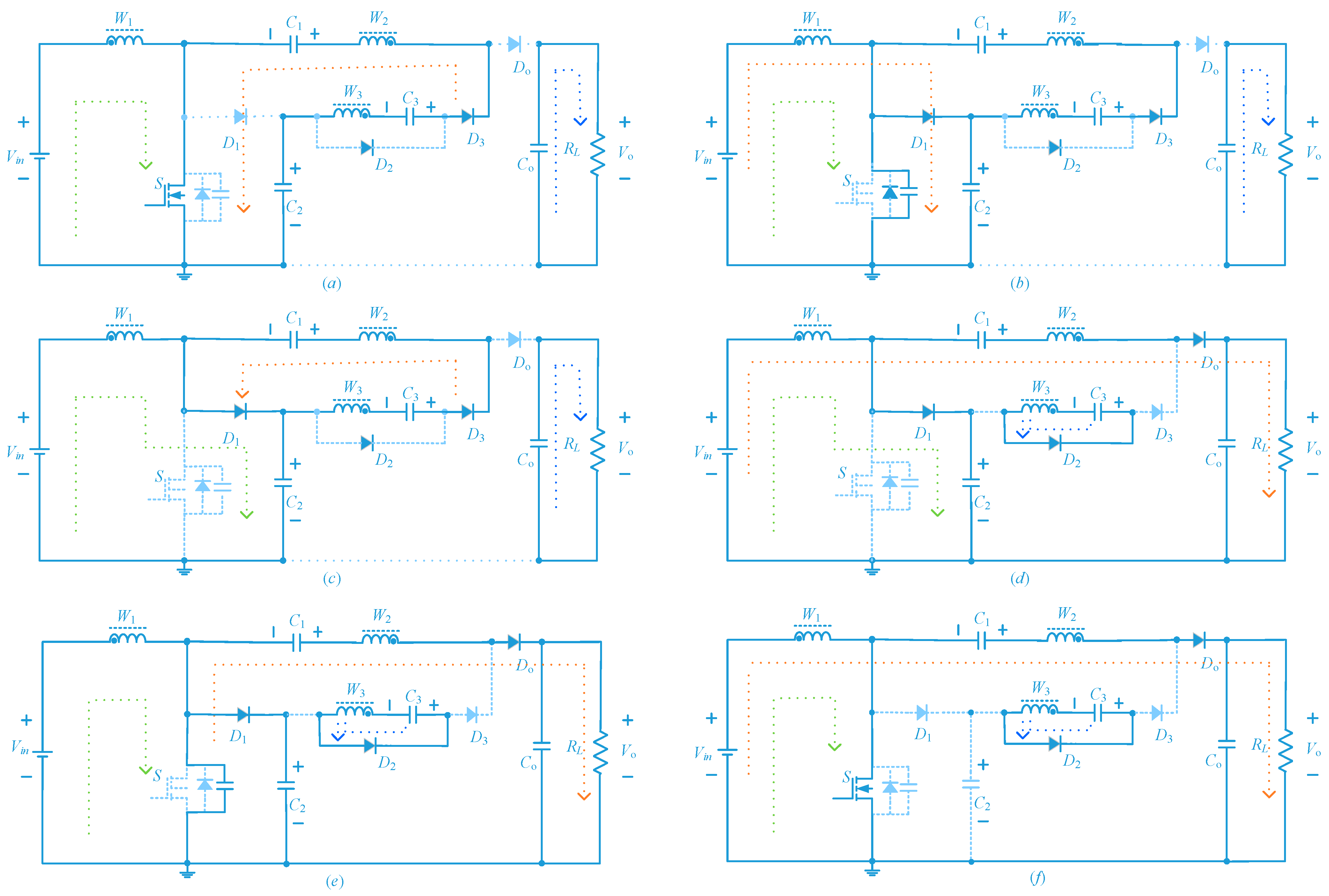
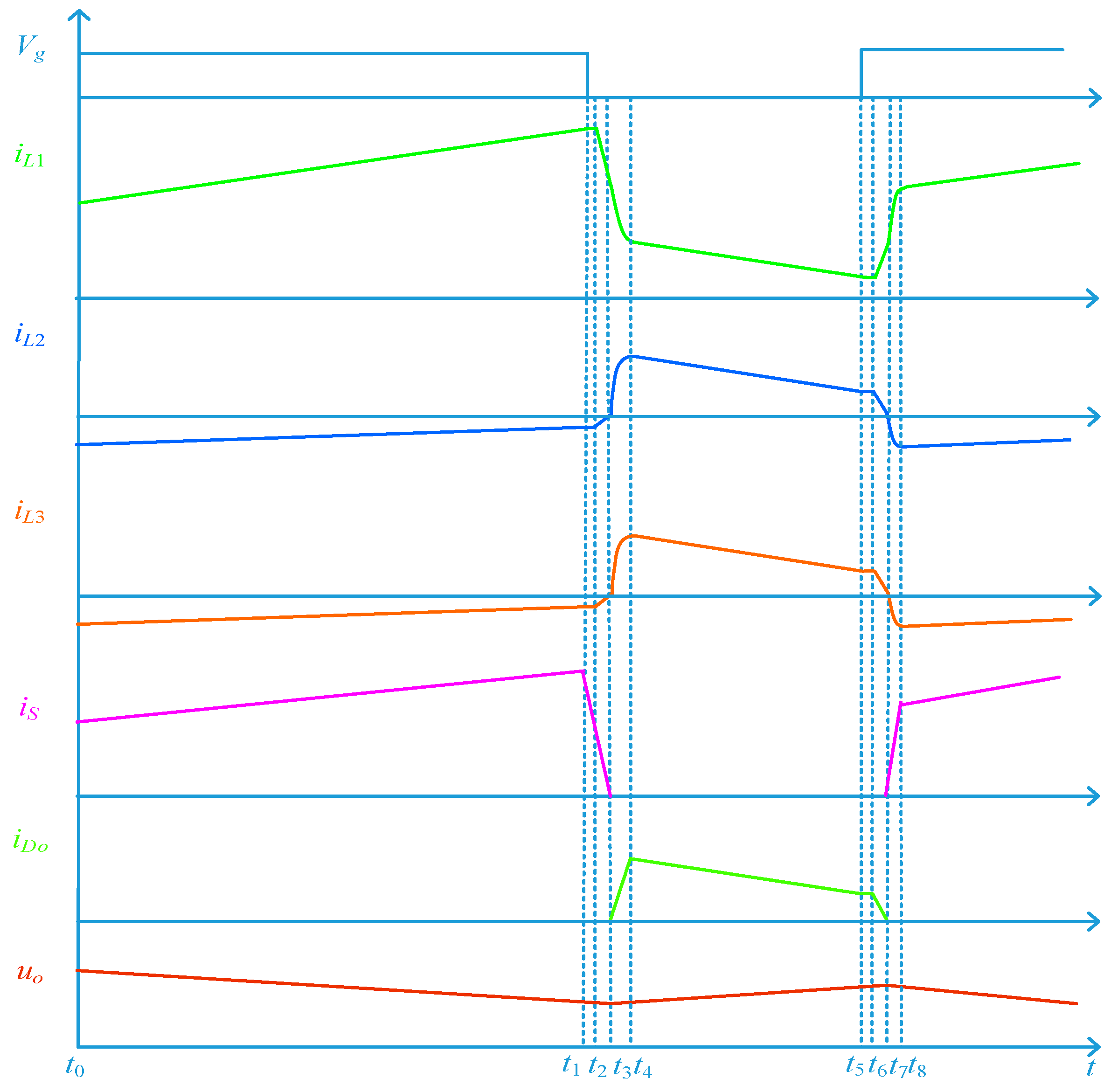
2.1. Operating Modes of the High Step-Up DC/DC Boost Converter
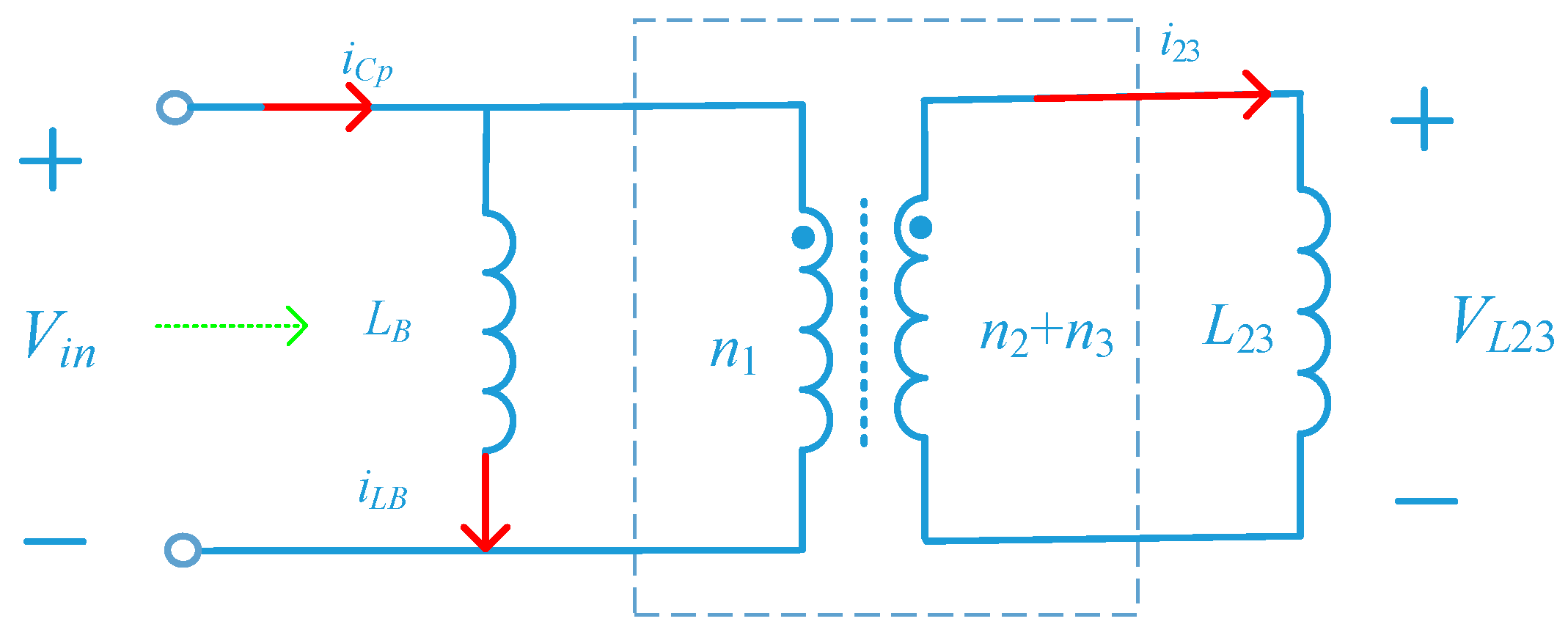
2.2. Simplified Models for Currents in the Three Windings of the Coupled Inductor
3. Design on the Three-Winding Coupled Inductor
3.1. Determining the Required Inductance of Winding W1 in the Coupled Inductor
3.2. Product Areas (AP) Method for Magnetic Core Selecting
3.3. Winding Arrangement for the Coupled Inductor
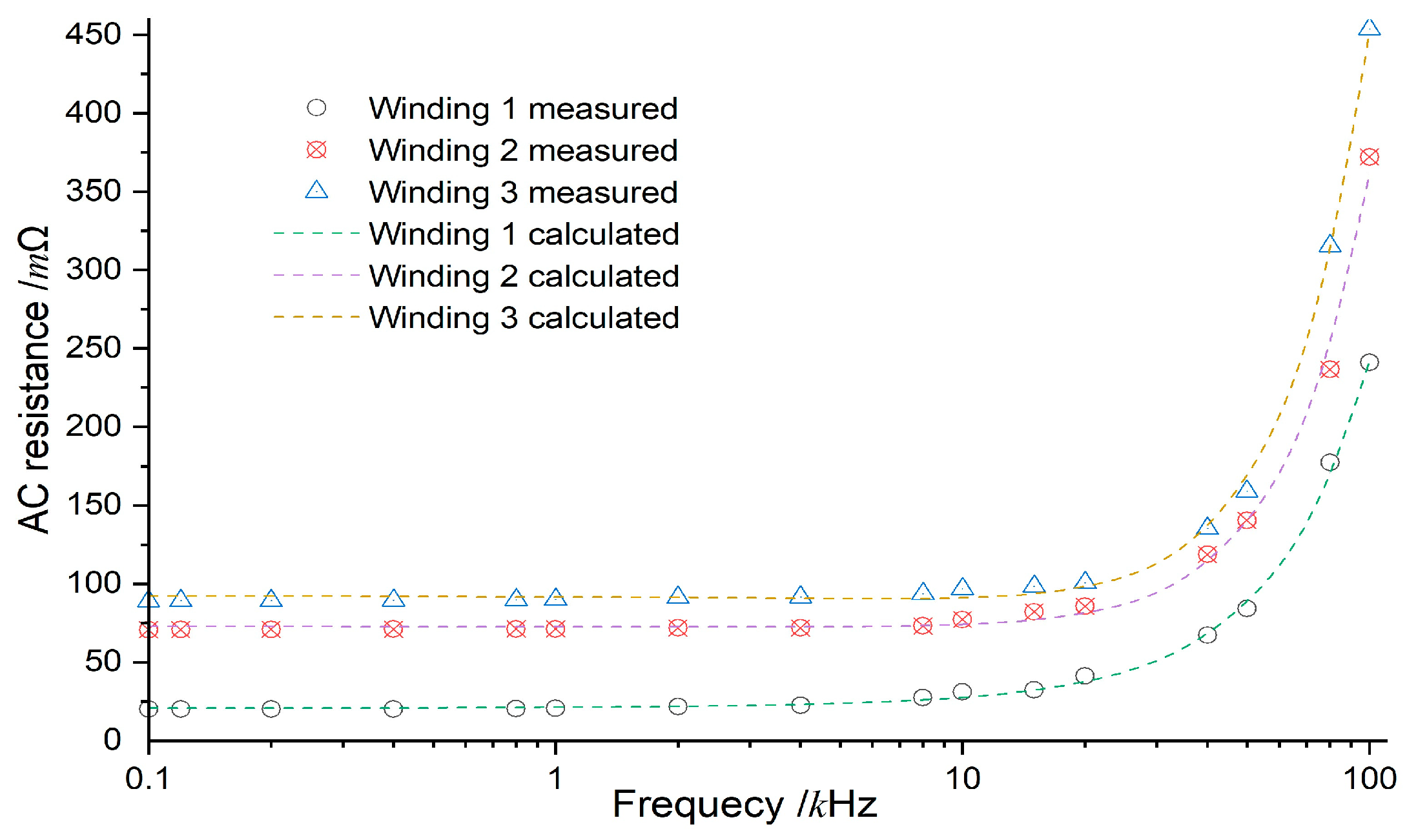
3.4. Loss Evaluation for the Three-Winding Coupled Inductor
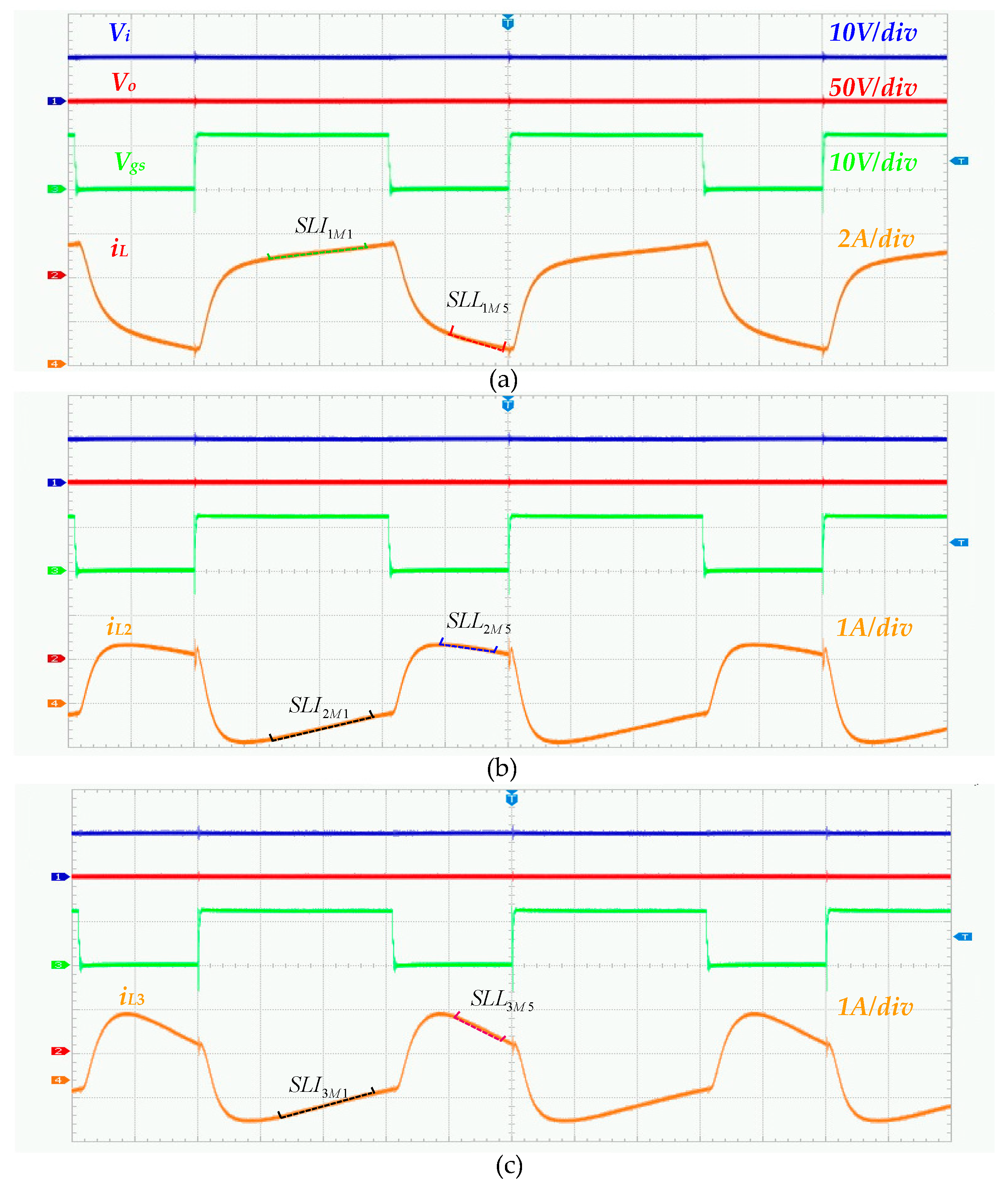
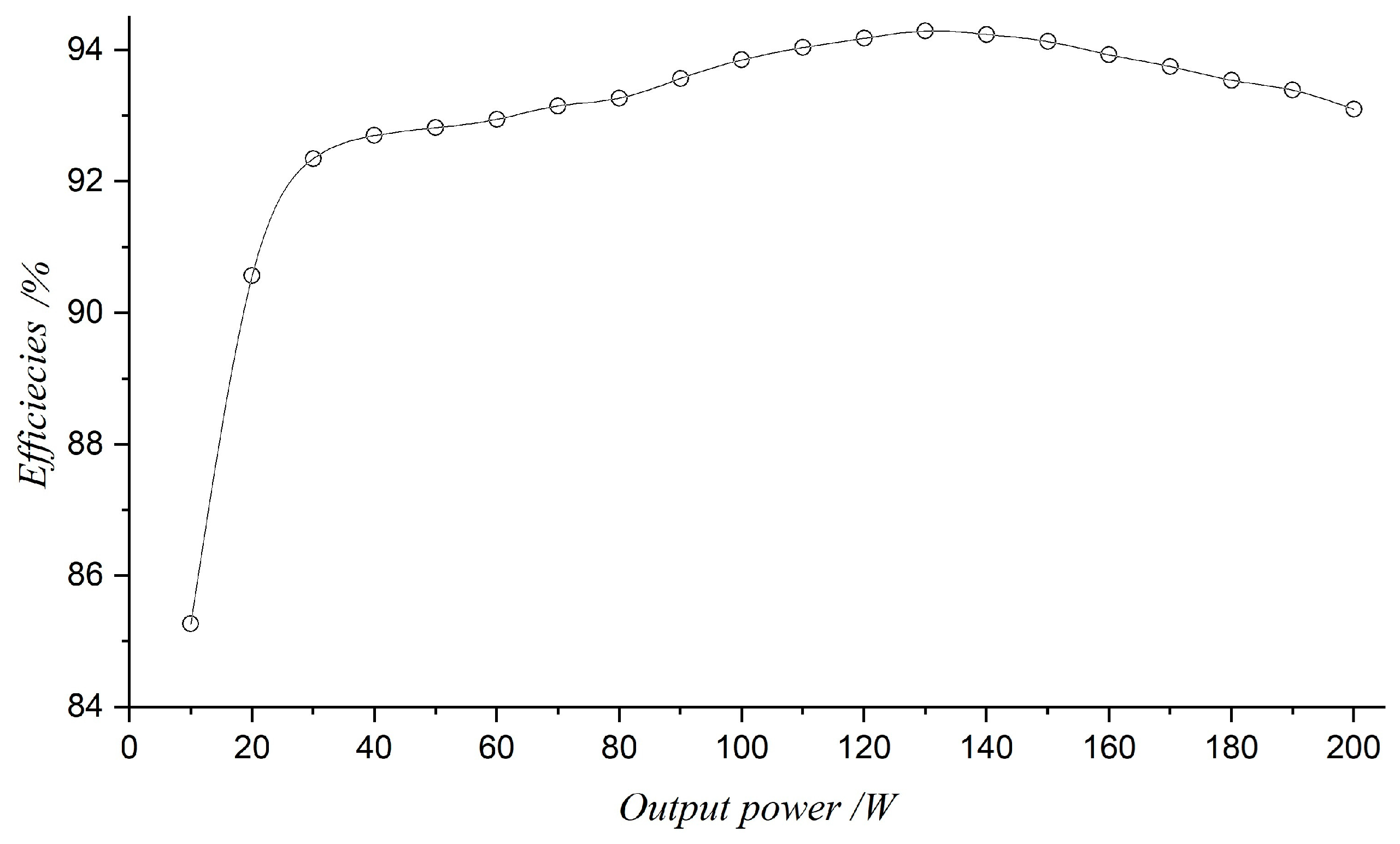
4. Experimental Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Voltage Gain of the High Step-Up DC/DC Converter
References
- Tseng, K.-C.; Hsu, H.-L.; Su, Y.-H.; Cheng, C.-A. A Novel Isolated High-Step-Up Interleaved Converter for Renewable Energy Systems. IEEE J. Emerg. Sel. Top. Ind. Electron. 2023, 4, 1235–1243. [Google Scholar] [CrossRef]
- Akhlaghi, Z.; Karimi, A.; Adib, E. High step up DC–DC converter with low conduction losses and reduced switching losses. IET Renew. Power Gener. 2024, 18, 654–662. [Google Scholar] [CrossRef]
- Karimi Hajiabadi, M.; Lahooti Eshkevari, A.; Mosallanejad, A. A bidirectional two-input step-up DC–DC converter with high gain, continuous input current, and common-ground for EVs and renewable energy systems. IET Power Electron. 2023, 16, 458–471. [Google Scholar] [CrossRef]
- Izadi, M.; Mosallanejad, A.; Lahooti Eshkevari, A. A non-isolated quadratic boost converter with improved gain, high efficiency, and continuous input current. IET Power Electron. 2023, 16, 193–208. [Google Scholar] [CrossRef]
- Karimi Hajiabadi, M.; Mosallanejad, A.; Salemnia, A. Ultra-high gain quadratic boost DC–DC converter based on a three-winding coupled inductor with reduced voltage stress for fuel cell-based systems. IET Power Electron. 2023, 16, 2666–2681. [Google Scholar] [CrossRef]
- Liu, H.; Li, F. A Novel High Step-up Converter with a Quasi-active Switched-Inductor Structure for Renewable Energy Systems. IEEE Trans. Power Electron. 2016, 31, 5030–5039. [Google Scholar] [CrossRef]
- Tang, Y.; Fu, D.; Wang, T.; Xu, Z. Hybrid switched-inductor converters for high step-up conversion. IEEE Trans. Ind. Electron. 2015, 62, 1480–1490. [Google Scholar] [CrossRef]
- He, L.Z.; Zheng, Z.P. High step-up DC-DC converter with switched-capacitor and its zero-voltage switching realization. IET Power Electron. 2017, 10, 630–636. [Google Scholar] [CrossRef]
- Salvador, M.A.; De Andrade, J.M.; Lazzarin, T.B.; Coelho, R.F. Nonisolated high-step-up DC-DC converter derived from switched-inductors and switched-capacitors. IEEE Trans. Ind. Electron. 2020, 67, 8506–8516. [Google Scholar] [CrossRef]
- Zhu, X.; Jiang, L.; Zhang, B.; Jin, K. The Resonant Modular Multilevel DC-DC Converter Adopting Switched-Inductor Cells for High Step-Up Ratio. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 6634–6647. [Google Scholar] [CrossRef]
- Gu, Y.; Chen, Y.; Zhang, B.; Qiu, D.; Xie, F. High Step-Up DC-DC Converter with Active Switched LC-Network for Photovoltaic Systems. IEEE Trans. Energy Convers. 2019, 34, 321–329. [Google Scholar] [CrossRef]
- Narayana, C.L.; Suryawanshi, H.M.; Nachankar, P.; Vijaya Vardhan Reddy, P.; Govind, D. A Quintupler Boost High Conversion Gain Soft-Switched Converter for DC Microgrid. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 1287–1291. [Google Scholar] [CrossRef]
- Rezaie, M.; Abbasi, V. Ultrahigh Step-Up DC-DC Converter Composed of Two Stages Boost Converter, Coupled Inductor, and Multiplier Cell. IEEE Trans. Ind. Electron. 2022, 69, 5867–5878. [Google Scholar] [CrossRef]
- Lu, F.; He, L.; Cheng, B. High Step-Up IPOS DC/DC Converter Based Efficiency Optimization Control Strategy. IEEE Trans. Ind. Electron. 2023, 70, 3674–3684. [Google Scholar] [CrossRef]
- Kumar, M.; Yadav, V.K.; Mathuria, K.; Verma, A.K. A Soft Switched High Gain Boost Converter With Coupled Inductor for Photovoltaic Applications. IEEE J. Emerg. Sel. Top. Ind. Electron. 2023, 4, 827–835. [Google Scholar] [CrossRef]
- Semiromizadeh, J.; Adib, E.; Izadi, H. A ZVS High Step-Up DC-DC Converter for Renewable Energy Systems With Simple Gate Drive Requirements. IEEE Trans. Ind. Electron. 2022, 69, 11253–11261. [Google Scholar] [CrossRef]
- Schmitz, L.; Martins, D.C.; Coelho, R.F. Three-Terminal Gain Cells Based on Coupled Inductor and Voltage Multiplers for High Step-Up Conversion. IEEE Trans. Circuits Syst. II Express Briefs 2023, 70, 236–240. [Google Scholar] [CrossRef]
- Ajami, A.; Ardi, H.; Farakhor, A. A Novel High Step-up DC/DC Converter Based on Integrating Coupled Inductor and Switched-Capacitor Techniques for Renewable Energy Applications. IEEE Trans. Power Electron. 2015, 30, 4255–4263. [Google Scholar] [CrossRef]
- Ding, J.; Zhao, S.; Gao, S.; Yin, H. A Single-Switch High Step-Up DC-DC Converter Based on Three-Winding Coupled Inductor and Pump Capacitor Unit. IEEE Trans. Power Electron. 2022, 37, 3053–3061. [Google Scholar] [CrossRef]
- Rao, B.T.; De, D. A Coupled Inductor-Based High-Gain ZVS DC-DC Converter With Reduced Voltage Stresses. IEEE Trans. Power Electron. 2023, 38, 15956–15967. [Google Scholar] [CrossRef]
- Asghari, A.; Yegane, Z.J. A High Step-Up DC-DC Converter with High Voltage Gain and Zero-Voltage Transition. IEEE Trans. Ind. Electron. 2024, 71, 6946–6954. [Google Scholar] [CrossRef]
- Yang, M.; Weng, Y.; Li, H.; Lin, J.; Yan, X.; Jin, T. A Novel Three-Winding Coupled Inductor-Based High-Gain DC-DC Converter With Low Switch Stress and Continuous Input Current. IEEE Trans. Power Electron. 2023, 38, 15781–15791. [Google Scholar] [CrossRef]
- Sarvghadi, P.; Varjani, A.Y.; Shahparasti, M. A High Step-Up Transformerless DC-DC Converter With New Voltage Multiplier Cell Topology and Coupled Inductor. IEEE Trans. Ind. Electron. 2022, 69, 10162–10171. [Google Scholar] [CrossRef]
- Nouri, T.; Nouri, N.; Vosoughi, N. A Novel High Step-Up High Efficiency Interleaved DC-DC Converter with Coupled Inductor and Built-In Transformer for Renewable Energy Systems. IEEE Trans. Ind. Electron. 2020, 67, 6505–6516. [Google Scholar] [CrossRef]
- Habibi, S.; Rahimi, R.; Ferdowsi, M.; Shamsi, P. Coupled Inductor-Based Single-Switch Quadratic High Step-Up DC-DC Converters With Reduced Voltage Stress on Switch. IEEE J. Emerg. Sel. Top. Ind. Electron. 2023, 4, 434–446. [Google Scholar] [CrossRef]
- Mohseni, P.; Rahimpour, S.; Dezhbord, M.; Islam, M.R.; Muttaqi, K.M. An Optimal Structure for High Step-Up Nonisolated DC-DC Converters with Soft-Switching Capability and Zero Input Current Ripple. IEEE Trans. Ind. Electron. 2022, 69, 4676–4686. [Google Scholar] [CrossRef]
- Hasanpour, S.; Siwakoti, Y.P.; Blaabjerg, F. A New High Efficiency High Step-Up DC/DC Converter for Renewable Energy Applications. IEEE Trans. Ind. Electron. 2023, 70, 1489–1500. [Google Scholar] [CrossRef]
- Li, H.; Li, C.; Sun, X.; Cheng, L.; Li, W. An Interleaved High Step-Up DC/DC Converter-Based Three-Winding Coupled Inductors With Symmetrical Structure. IEEE Trans. Power Electron. 2023, 38, 6642–6652. [Google Scholar] [CrossRef]
- Guo, X.; Wu, S.; Zhang, Y.; Dou, C.; Chi, Y. Optimal Design of High Frequency Transformer for High Power Density Flyback Converter. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 4399–4403. [Google Scholar] [CrossRef]
- Liu, Y.-C.; Chen, C.; Chen, K.-D.; Syu, Y.-L.; Lu, D.-J.; Kim, K.A.; Chiu, H.-J. Design and Implementation of a Planar Transformer with Fractional Turns for High Power Density LLC Resonant Converters. IEEE Trans. Power Electron. 2021, 36, 5191–5203. [Google Scholar] [CrossRef]
- Guo, Z.; Yu, R.; Xu, W.; Feng, X.; Huang, A.Q. Design and Optimization of a 200-kW Medium-Frequency Transformer for Medium-Voltage SiC PV Inverters. IEEE Trans. Power Electron. 2021, 36, 10548–10560. [Google Scholar] [CrossRef]
- Yao, P.; Jiang, X.; Xue, P.; Li, S.; Lu, S.; Wang, F. Design Optimization of Medium-Frequency Transformer for DAB Converters with DC Bias Capacity. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 5043–5054. [Google Scholar] [CrossRef]
- Zhou, X.; Tian, Y.; Quan, Y.; Zhang, X.; Liu, T.; Feng, J.; Wang, D.; Cheng, X.; Zheng, L.; Yu, Y. A Design Method of Partially Interleaved Winding Structure With Low Leakage Inductance for Planar Transformer Application. IEEE Trans. Power Electron. 2023, 38, 6366–6379. [Google Scholar] [CrossRef]
- Liang, W.; Hu, X.; Chen, H.; Wu, G.; Zhang, M.; Tan, G. High-voltage-gain DC-DC converter with three-winding coupled inductor. Chin. J. Electr. Eng. 2019, 5, 10–23. [Google Scholar] [CrossRef]
- Erickson, R.W.; Maksimović, D. Fundamentals of Power Electronics, 3rd ed.; Springer Nature: Cham, Switzerland, 2020; pp. 23–24. [Google Scholar]
- Dowell, P.L. Effects of Eddy Currents in Transformer Windings. Proc. Inst. Electr. Eng. 1966, 113, 1387. [Google Scholar] [CrossRef]
- Geng, S.Q.; Chu, M.H.; Wang, W.S.; Wan, P.Y.; Peng, X.H.; Lu, H.; Li, P.K. Modelling and optimization of winding resistance for litz wire inductors. IET Power Electron. 2021, 14, 1834–1843. [Google Scholar] [CrossRef]
- Steinmetz, C.P. On the Law of Hysteresis. Proceeding IEEE 1984, 72, 197–221. [Google Scholar] [CrossRef]
- Hasanpour, S.; Forouzesh, M.; Siwakoti, Y.; Blaabjerg, F. A Novel Full Soft-Switching High-Gain DC/DC Converter Based on Three-Winding Coupled-Inductor. IEEE Trans. Power Electron. 2021, 36, 12656–12669. [Google Scholar] [CrossRef]
- Farsijani, M.; Abbasian, S.; Hafezi, H.; Abrishamifar, A. A High Step-Up Cost Effective DC-to-DC Topology Based on Three-Winding Coupled-Inductor. IEEE J. Emerg. Sel. Top. Ind. Electron. 2023, 4, 50–59. [Google Scholar] [CrossRef]

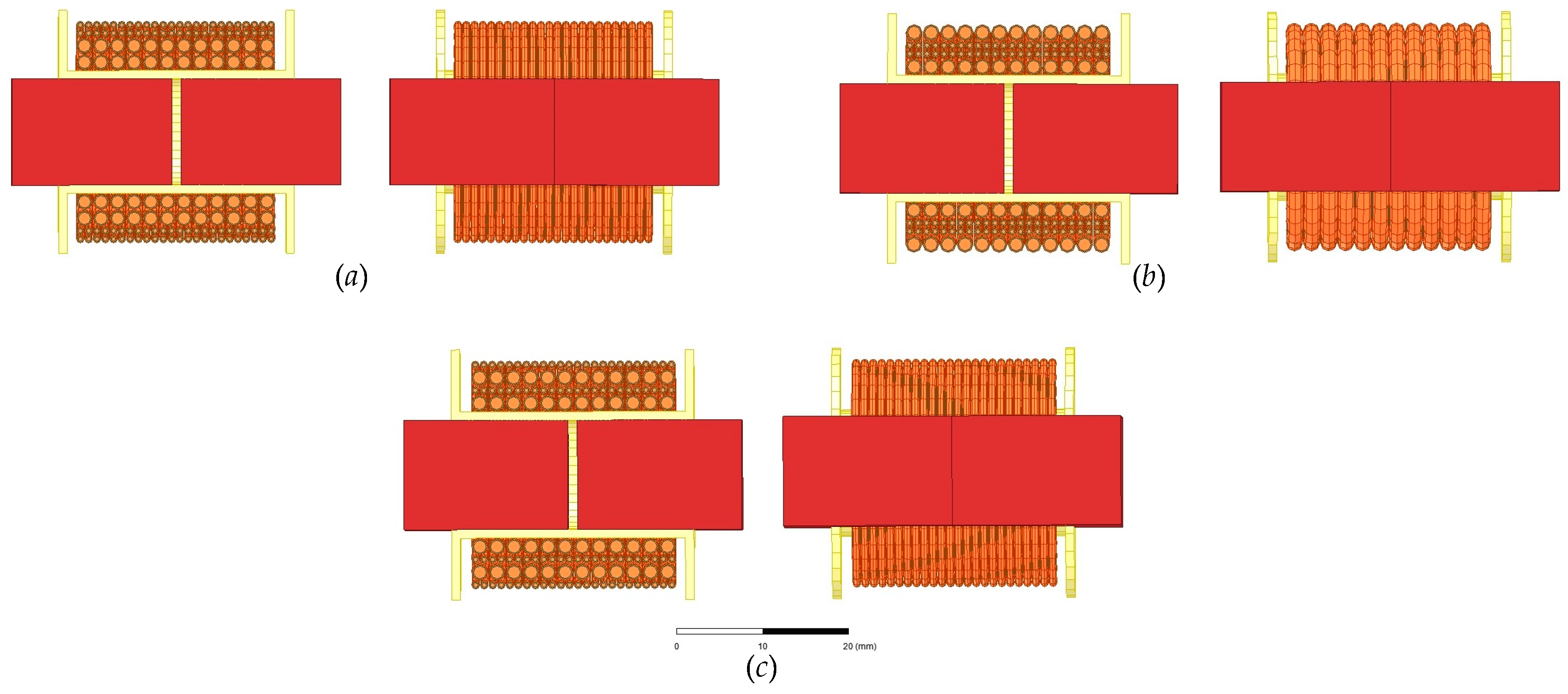


| Specifications | Values |
|---|---|
| Input voltage Vin | 20 V |
| Ripple in current of winding W1 iL1rip | 10 A |
| Output voltage Vo | 200 V |
| Switching frequency fs | 40 kHz |
| Output maximum power Po | 200 W |
| Windings | RMS Value of Current | Litz Wires |
|---|---|---|
| W1 | 10.81 A | 200 strands/AWG40 |
| W2 and W3 | 2.05 A | 50 strands/AWG40 |
| Winding Structure | Inductance of Winding W1 | Leakage Inductance |
|---|---|---|
| W1-W2-W3 | 105.94 µH | 5.27 µH |
| 1/2W1-W2-W3-1/2W1 | 107.33 µH | 3.03 µH |
| 1/2W1-W2-1/2W1-W3 | 106.65 µH | 2.91 µH |
| Converter | Voltage Gain | Rated Power | Switching Frequency | Magnetic Core | Turn Ratio n1:n2:n3 | Inductance of Winding W1 | Urcore |
|---|---|---|---|---|---|---|---|
| [39] | 160 W | 50 kHz | EE42/21/20 | 29:11:24 | 220 µH | 7.05 W/cm3 | |
| [27] | 200 W | 60 kHz | EE42/21/20 | 1:0.61:0.63 | 200 µH | 8.81 W/cm3 | |
| [40] | 200 W | 50 kHz | ETD44/22/15 | 20:40:40 | 130 µH | 11.23 W/cm3 | |
| [20] | 250 W | 70 kHz | EE42/21/15 | 1:1:1 | 16 µH | 14.45 W/cm3 | |
| [19] | 500 W | 50 kHz | EE55/28/21 | 18:7:7 | 80 µH | 9.61 W/cm3 | |
| This paper | 200 W | 40 kHz | ETD39/20/13 | 24:24:24 | 105 µH | 17.39 W/cm3 |
| Components | Parameters |
|---|---|
| Magnetic Core | ETD39/PC95 |
| n1:n2:n3 | 24:24:24 |
| Inductance of winding 1 | 105.04 μF |
| MOSFET S | IRFB38N20DPBF |
| D1, D2, D3 and Do | MUR1640CTG |
| Cin | 94.0 μF |
| Co | 200.0 μF |
| C1 | 100.0 μF |
| C2 and C3 | 47.0 μF |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, J.; Wang, J.; Qin, Y.; Ding, S.; Ji, B. Design and Implementation of Three-Winding Coupled Inductor Applied in High Step-Up DC/DC Converter Combined with Voltage Multipliers. Energies 2025, 18, 1938. https://doi.org/10.3390/en18081938
Song J, Wang J, Qin Y, Ding S, Ji B. Design and Implementation of Three-Winding Coupled Inductor Applied in High Step-Up DC/DC Converter Combined with Voltage Multipliers. Energies. 2025; 18(8):1938. https://doi.org/10.3390/en18081938
Chicago/Turabian StyleSong, Jiuxu, Jiahao Wang, Yuanzhong Qin, Shuai Ding, and Bing Ji. 2025. "Design and Implementation of Three-Winding Coupled Inductor Applied in High Step-Up DC/DC Converter Combined with Voltage Multipliers" Energies 18, no. 8: 1938. https://doi.org/10.3390/en18081938
APA StyleSong, J., Wang, J., Qin, Y., Ding, S., & Ji, B. (2025). Design and Implementation of Three-Winding Coupled Inductor Applied in High Step-Up DC/DC Converter Combined with Voltage Multipliers. Energies, 18(8), 1938. https://doi.org/10.3390/en18081938





