Evaluation of Thermal Stress and Performance for Solid Oxide Electrolysis Cells Employing Graded Fuel Electrodes
Abstract
1. Introduction
2. Model Development
- (1)
- The operating condition is steady state.
- (2)
- The incoming and outgoing gases are considered to be incompressible and ideal, and the flow pattern is laminar.
- (3)
- All cell layers are considered to be linearly elastic materials and isotropic.
- (4)
- Each interface between the cell layers is continuous, i.e., there are no fractures present.
- (5)
- The thermophysical properties (e.g., modulus of elasticity, CTE, and Poisson’s ratio) of all SOEC components are varied solely with temperature.
- (6)
- The fuel electrode graded factors change linearly only with singular variables in the cell thickness direction, which means that, when one graded factor is altered, the other factors remain unchanged.
2.1. Geometric Model
2.2. Governing Equations
2.2.1. Electrochemical Reactions
2.2.2. Gas Flow and Momentum Equations
2.2.3. Mass Conservation Equation
2.2.4. Heat Conservation Equation
2.2.5. Thermal Stress–Strain Equation
2.3. Boundary Conditions and Model Validation
3. Results and Discussion
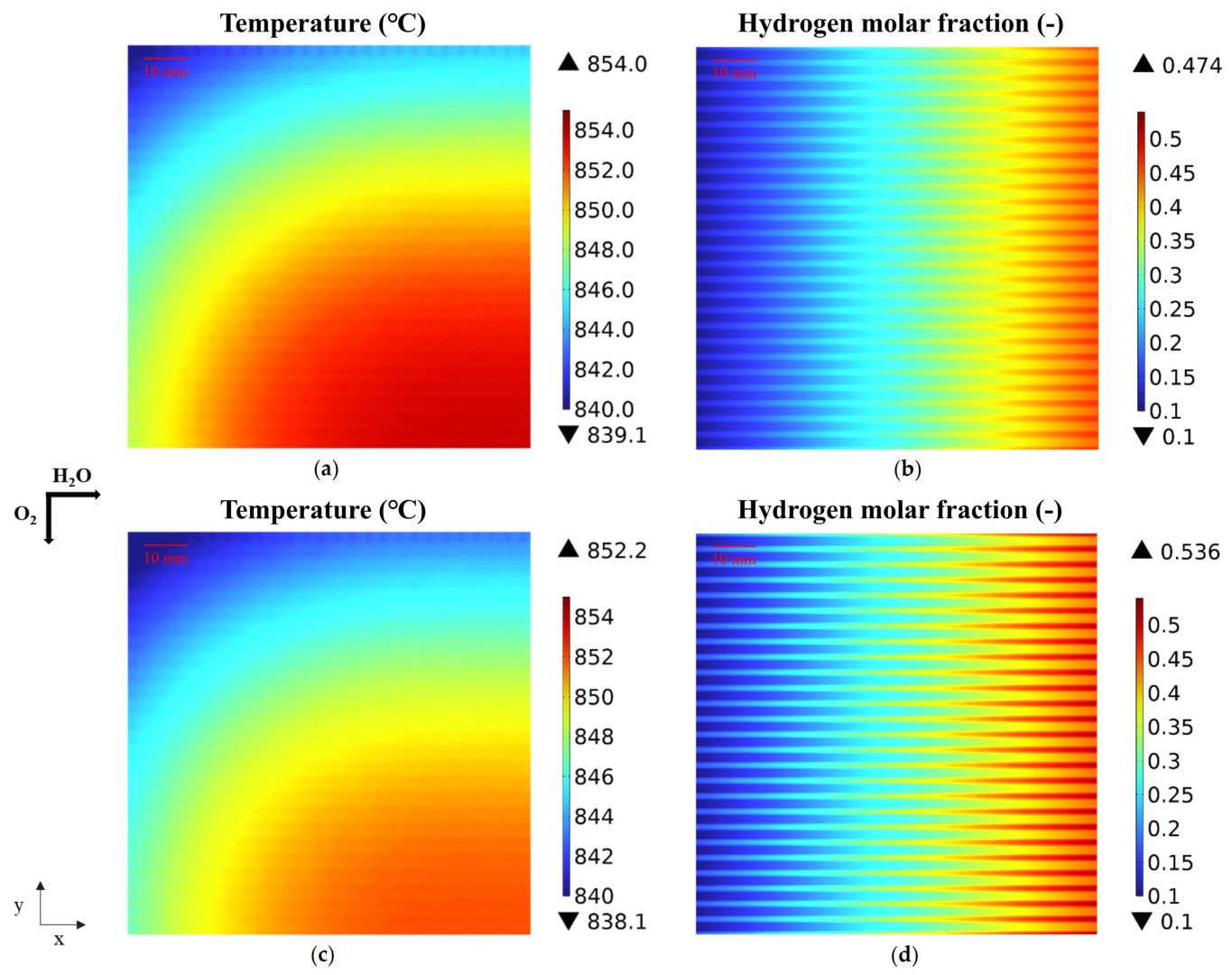
3.1. Predicted Performance with the Base-Case Porous Fuel Electrode (Case 0)
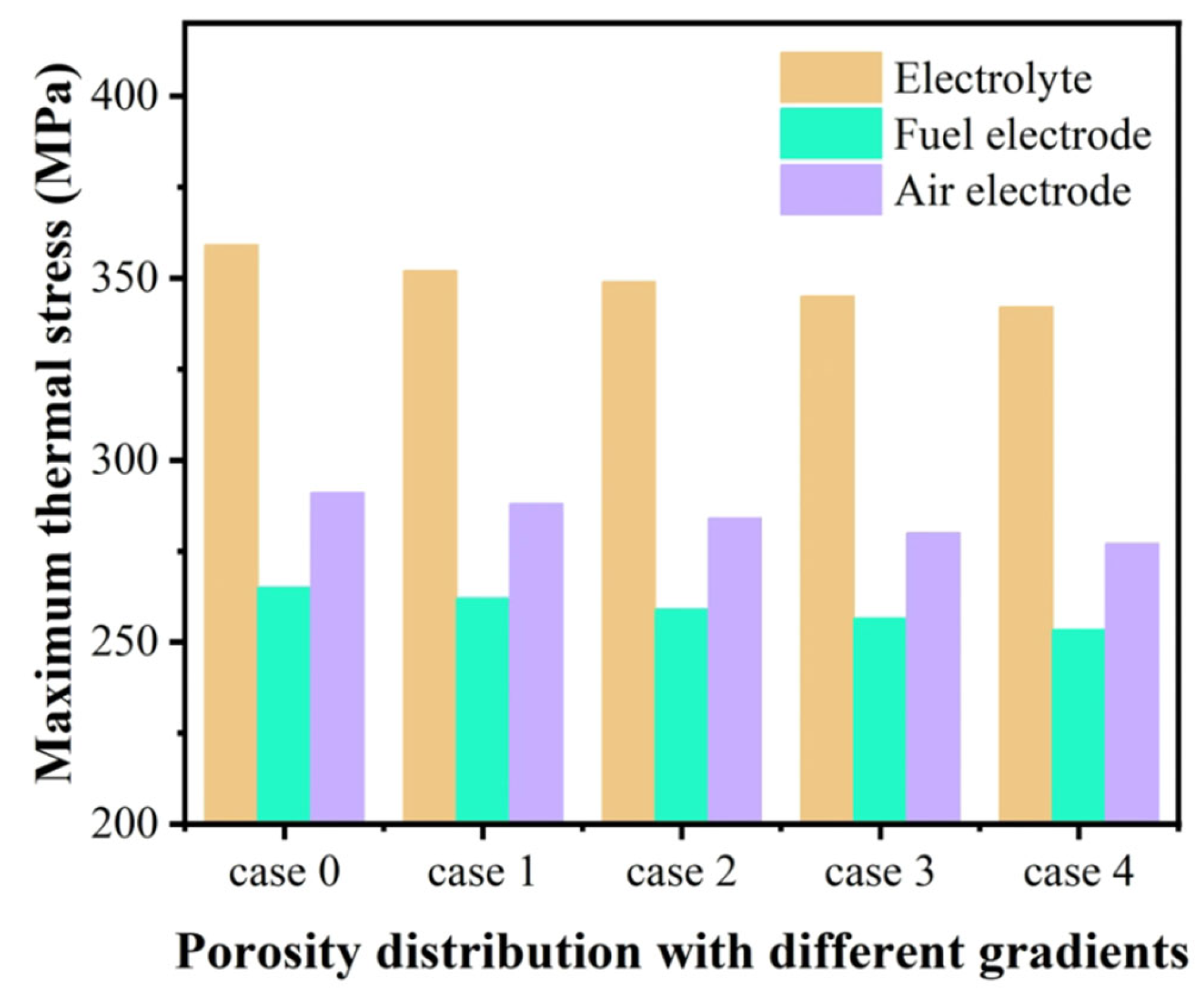
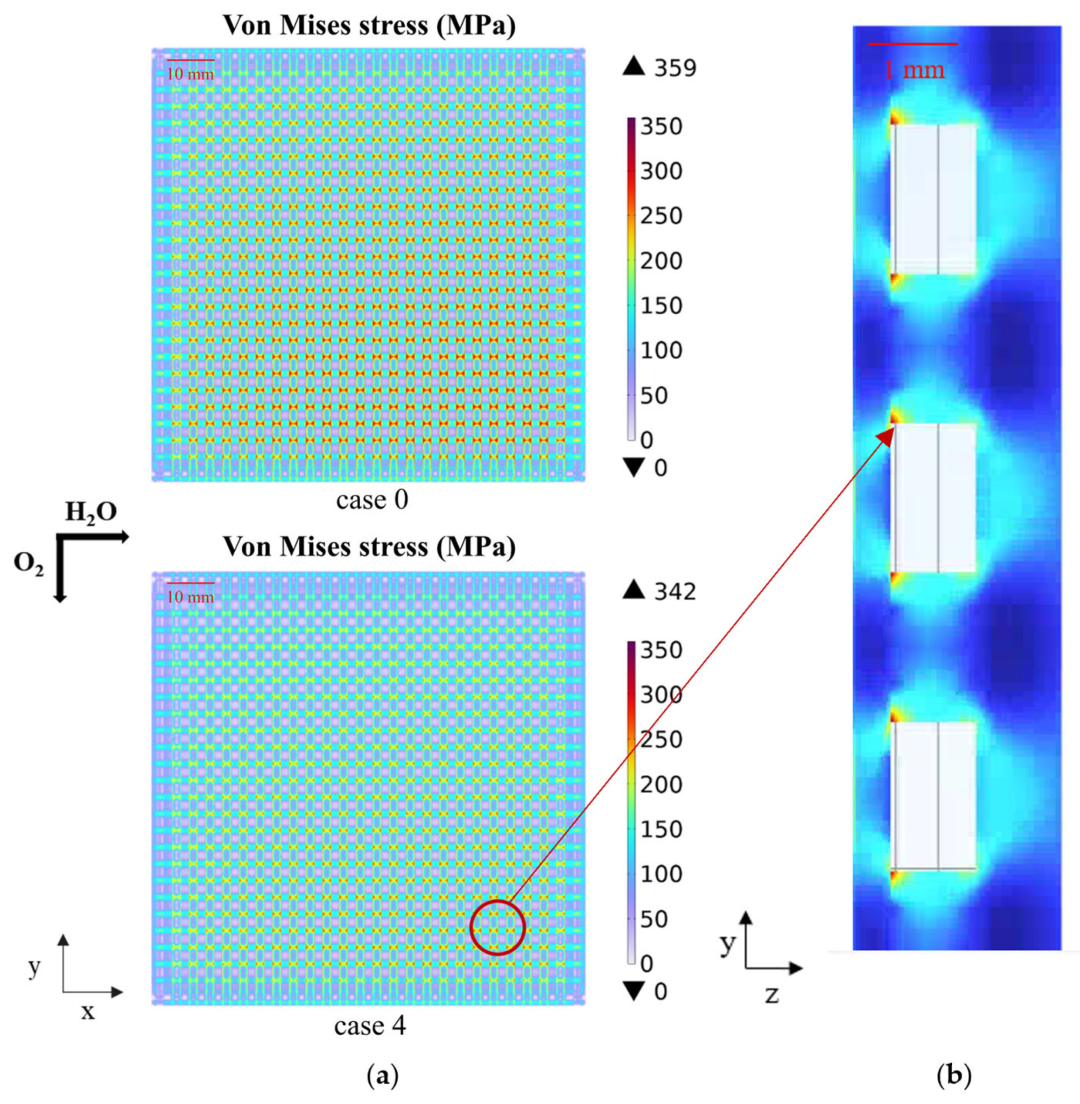
3.2. Effect of Graded Porosity
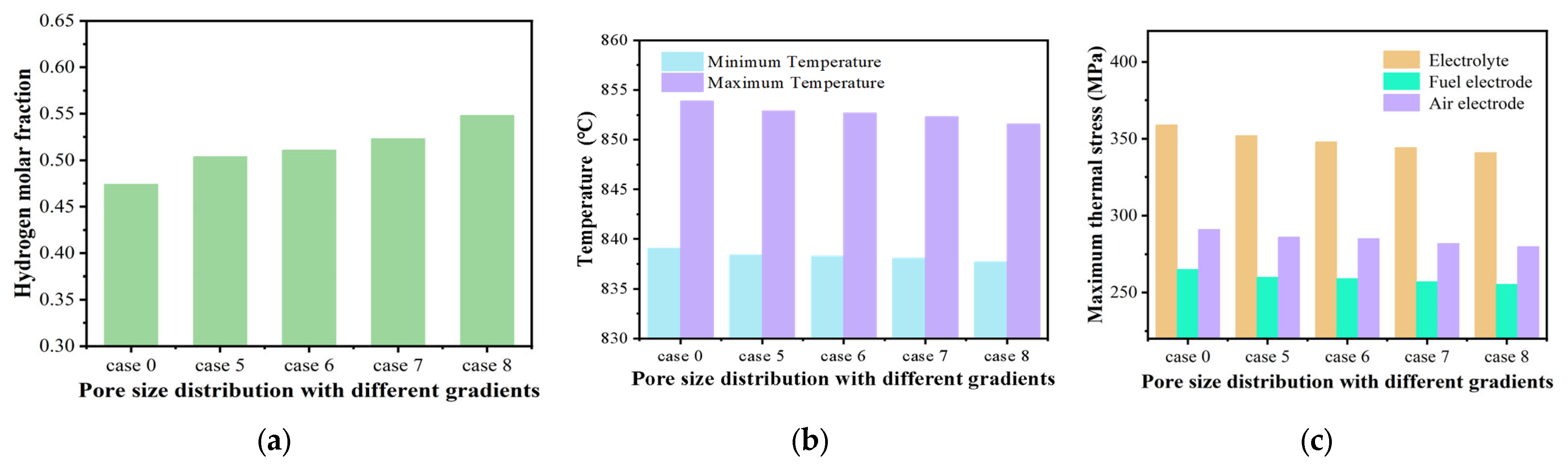
3.3. Effect of Graded Pore Size
3.4. Effect of Graded Ni Composition
3.5. Limitations and Prospects
4. Conclusions
- (1)
- The graded porosity can effectively improve heat distribution and local overheating, enhance reaction rates, and alleviate thermal stress concentration. The hydrogen molar fraction of an SOEC using a graded porosity of 0.3–0.5 is enhanced by 13%, and the maximum thermal stress is decreased by 4.7%.
- (2)
- The graded pore size may significantly improve gas diffusion and electrochemical reaction kinetics, thereby enhancing the overall performance of the SOEC. The hydrogen molar fraction of an SOEC with a graded pore size of 1–10 μm is enhanced by 15.6%, while the maximum thermal stress is decreased by about 5.0%.
- (3)
- The graded Ni composition in the fuel electrode is able to increase the catalytic activity and electronic/ion conductivity in the TPB region, thereby improving the electrochemical reaction rate and hydrogen production. The graded Ni component fuel electrode improves hydrogen production performance, but the maximum thermal stress increases as well.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Dawood, F.; Anda, M.; Shafiullah, G.M. Hydrogen production for energy: An overview. Int. J. Hydrogen Energy 2020, 45, 3847–3869. [Google Scholar] [CrossRef]
- Liu, W.; Wan, Y.; Xiong, Y.; Gao, P. Green hydrogen standard in China: Standard and evaluation of low-carbon hydrogen, clean hydrogen, and renewable hydrogen. Int. J. Hydrogen Energy 2022, 47, 24584–24591. [Google Scholar] [CrossRef]
- Xu, Y.; Cai, S.; Chi, B.; Tu, Z. Technological limitations and recent developments in a solid oxide electrolyzer cell: A review. Int. J. Hydrogen Energy 2024, 50, 548–591. [Google Scholar] [CrossRef]
- Cai, W.; Xiao, L.; Deng, T.; Hang, Q.; Pan, B.; Yuan, J.; Xie, C. Analysis of residual stress for thin-layered electrolyte co-sintered with porous electrodes applied in solid oxide cells. Thin-Walled Struct. 2025, 211, 113140. [Google Scholar] [CrossRef]
- Lim, K.; Kim, Y.; Salihi, H.; Ju, H. Integrated modeling of electrochemical, thermal, and structural behavior in solid oxide electrolysis cells. Int. J. Heat Mass Transf. 2024, 224, 125235. [Google Scholar] [CrossRef]
- Fang, X.; Lin, Z. Numerical study on the mechanical stress and mechanical failure of planar solid oxide fuel cell. Appl. Energy 2018, 229, 63–68. [Google Scholar] [CrossRef]
- Cai, W.; Zhou, R.; Yuan, J.; Tu, Y.; Zhang, Z. The electrochemical performance and thermal behavior analysis of SOFC containing thin gadolinium-doped ceria diffusion barrier layer. Int. Commun. Heat Mass Transf. 2024, 155, 107577. [Google Scholar] [CrossRef]
- Ilyushin, Y.V.; Boronko, E.A. Analysis of Energy Sustainability and Problems of Technological Process of Primary Aluminum Production. Energies 2025, 18, 2194. [Google Scholar] [CrossRef]
- Spreafico, C. Additive manufacturing of solid oxide fuel cells. A comprehensive review of patent literature. J. Power Sources 2025, 625, 235702. [Google Scholar] [CrossRef]
- Miyake, M.; Iwami, M.; Takeuchi, M.; Nishimoto, S.; Kameshima, Y. Electrochemical performance of Ni0.8Cu0.2/Ce0.8Gd0.2O1.9 cermet anodes with functionally graded structures for intermediate-temperature solid oxide fuel cell fueled with syngas. J. Power Sources 2018, 390, 181–185. [Google Scholar] [CrossRef]
- Wang, C. A Computational Analysis of Functionally Graded Anode in Solid Oxide Fuel Cell by Involving the Correlations of Microstructural Parameters. Energies 2016, 9, 408. [Google Scholar] [CrossRef]
- Kim, J.W.; Bae, K.; Kim, H.J.; Son, J.; Kim, N.; Stenfelt, S.; Prinz, F.B.; Shim, J.H. Three-dimensional thermal stress analysis of the re-oxidized Ni-YSZ anode functional layer in solid oxide fuel cells. J. Alloys Compd. 2018, 752, 148–154. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, N.; Qiao, J.; Sun, K.; Xu, P. Improved SOFC performance with continuously graded anode functional layer. Electrochem. Commun. 2009, 11, 1120–1123. [Google Scholar] [CrossRef]
- McCoppin, J.; Barney, I.; Mukhopadhyay, S.; Miller, R.; Reitz, T.; Young, D. Compositional control of continuously graded anode functional layer. J. Power Sources 2012, 215, 160–163. [Google Scholar] [CrossRef]
- He, S.; Dai, H.; Cai, G.; Chen, H.; Guo, L. Optimization of La0.75Sr0.25Cr0.5Mn0.5O3-δ-Ce0.8Sm0.2O1.9 compositionally graded anode functional layer. Electrochim. Acta 2015, 152, 155–160. [Google Scholar] [CrossRef]
- Marcano, D.; Mauer, G.; Vaßen, R.; Weber, A. Manufacturing of high performance solid oxide fuel cells (SOFCs) with atmospheric plasma spraying (APS) and plasma spray-physical vapor deposition (PS-PVD). Serf. Coat. Technol. 2017, 318, 170–177. [Google Scholar] [CrossRef]
- Sai Charan, M.; Naik, A.K.; Kota, N.; Laha, T.; Roy, S. Review on developments of bulk functionally graded composite materials. Int. Mater. Rev. 2022, 67, 797–863. [Google Scholar] [CrossRef]
- Cai, W.; Zhou, R.; Wang, C.; Xie, C.; Xiao, L.; Zhang, Z.; Yang, C.; Yuan, J. On characteristics and research development of coupled fuel cell stack performance and stress. Appl. Energy 2025, 388, 125719. [Google Scholar] [CrossRef]
- Abdullah, T.; Liu, L. Simulation-based microstructural optimization of solid oxide fuel cell for low temperature operation. Int. J. Hydrogen Energy 2016, 41, 13632–13643. [Google Scholar] [CrossRef]
- Li, J.; Lin, Z. Effects of electrode composition on the electrochemical performance and mechanical property of micro-tubular solid oxide fuel cell. Int. J. Hydrogen Energy 2012, 37, 12925–12940. [Google Scholar] [CrossRef]
- Bain, A.T.; Roberts, R.; Deiner, J.; Fellner, J. Computational Optimization of Functionally Graded Electrodes for Solid Oxide Fuel Cells. ECS Meet. Abstr. 2022, MA2022-02, 1794. [Google Scholar] [CrossRef]
- Xu, M.; Li, T.S.; Yang, M.; Andersson, M.; Fransson, I.; Larsson, T.; Sundén, B. Modeling of an anode supported solid oxide fuel cell focusing on thermal stresses. Int. J. Hydrogen Energy 2016, 41, 14927–14940. [Google Scholar] [CrossRef]
- Kamkeng, A.D.N.; Wang, M. Long-term performance prediction of solid oxide electrolysis cell (SOEC) for CO2/H2O co-electrolysis considering structural degradation through modelling and simulation. Chem. Eng. J. 2022, 429, 132158. [Google Scholar] [CrossRef]
- Liu, C.; Dang, Z.; Xi, G. Numerical study on thermal stress of solid oxide electrolyzer cell with various flow configurations. Appl. Energy 2024, 353, 122041. [Google Scholar] [CrossRef]
- Ni, M.; Leung, M.K.H.; Leung, D.Y.C. Micro-scale modelling of solid oxide fuel cells with micro-structurally graded electrodes. J. Power Sources 2007, 168, 369–378. [Google Scholar] [CrossRef]
- Yan, Z.; He, A.; Hara, S.; Shikazono, N. Design and optimization of functionally graded electrodes for solid oxide fuel cells (SOFCs) by mesoscale modeling. Int. J. Hydrogen Energy 2022, 47, 16610–16625. [Google Scholar] [CrossRef]
- Mukhopadhyay, M.; Maji, P.S.; Saravanan, R.; Mukhopadhyay, J. Designing tortuous gas diffusion path for hydrogen oxidation reaction and stability of solid oxide fuel cell: An engineered microstructural aspect in anode functional layer. Int. J. Hydrogen Energy 2024, 87, 1140–1154. [Google Scholar] [CrossRef]
- Sun, M.; Li, A.; Zhang, X.; Fei, Y.; Zhu, L.; Huang, Z. Influence of operating conditions on the fuel electrode degradation of solid oxide electrolysis cell investigated by phase field model with wettability analysis. J. Power Sources 2023, 587, 233700. [Google Scholar] [CrossRef]
- Damm, D.L.; Fedorov, A.G. Local thermal non-equilibrium effects in porous electrodes of the hydrogen-fueled SOFC. J. Power Sources 2006, 159, 1153–1157. [Google Scholar] [CrossRef]
- Gari, A.A.; Ahmed, K.I.; Ahmed, M.H. Performance and thermal stress of tubular functionally graded solid oxide fuel cells. Energy Rep. 2021, 7, 6413–6421. [Google Scholar] [CrossRef]
- Lee, S.; Park, I.; Lee, H.; Shin, D. Continuously gradient anode functional layer for BCZY based proton-conducting fuel cells. Int. J. Hydrogen Energy 2014, 39, 14342–14348. [Google Scholar] [CrossRef]
- Greene, E.S.; Chiu, W.K.S.; Medeiros, M.G. Mass transfer in graded microstructure solid oxide fuel cell electrodes. J. Power Sources 2006, 161, 225–231. [Google Scholar] [CrossRef]
- Luo, Y.; Jiang, W.; Zhang, Q.; Zhang, W.Y.; Hao, M. Effects of anode porosity on thermal stress and failure probability of planar solid oxide fuel cell with bonded compliant seal. Int. J. Hydrogen Energy 2016, 41, 7464–7474. [Google Scholar] [CrossRef]
- Saied, M.; Ahmed, K.; Ahmed, M.; Nemat-Alla, M.; El-Sebaie, M. Investigations of solid oxide fuel cells with functionally graded electrodes for high performance and safe thermal stress. Int. J. Hydrogen Energy 2017, 42, 15887–15902. [Google Scholar] [CrossRef]
- Zeng, S.; Xu, M.; Parbey, J.; Yu, G.; Andersson, M.; Li, Q.; Li, B.; Li, T. Thermal stress analysis of a planar anode-supported solid oxide fuel cell: Effects of anode porosity. Int. J. Hydrogen Energy 2017, 42, 20239–20248. [Google Scholar] [CrossRef]
- Gao, M.; Wang, K.; Song, D.; Wang, M.; Li, H.; Tu, S. Stress relief in solid oxide fuel cells by leveraging on the gradient porosity design in anode layers. Int. J. Heat Mass Transf. 2024, 232, 125900. [Google Scholar] [CrossRef]
- Osman, S.; Ahmed, K.; Nemattalla, M.; Ookawara, S.; Ahmed, M. Performance and thermal stresses in functionally graded anode-supported honeycomb solid-oxide fuel cells. Int. J. Hydrogen Energy 2021, 46, 33010–33027. [Google Scholar] [CrossRef]
- Ma, S.; Xue, D.; Li, Q.; Zheng, J.; Feng, C.; Li, G. Stress Analysis of Solid Oxide Fuel Cell Electrodes Using Functional Gradient Materials. J. Electrochem. Soc. 2023, 170, 034502. [Google Scholar] [CrossRef]
- Liu, Y.; Xiao, L.; Wang, H.; Ou, D.; Yuan, J. Numerical Study of H2 Production and Thermal Stress for Solid Oxide Electrolysis Cells with Various Ribs/Channels. Energies 2024, 17, 510. [Google Scholar] [CrossRef]
- Qu, Z.; Aravind, P.V.; Boksteen, S.Z.; Dekker, N.J.J.; Janssen, A.H.H.; Woudstra, N.; Verkooijen, A.H.M. Three-dimensional computational fluid dynamics modeling of anode-supported planar SOFC. Int. J. Hydrogen Energy 2011, 36, 10209–10220. [Google Scholar] [CrossRef]
- Lee, S.; Kim, H.; Yoon, K.J.; Son, J.; Lee, J.; Kim, B.; Choi, W.; Hong, J. The effect of fuel utilization on heat and mass transfer within solid oxide fuel cells examined by three-dimensional numerical simulations. Int. J. Heat Mass Transf. 2016, 97, 77–93. [Google Scholar] [CrossRef]
- Costamagna, P.; Honegger, K. Modeling of Solid Oxide Heat Exchanger Integrated Stacks and Simulation at High Fuel Utilization. J. Electrochem. Soc. 1998, 145, 3995. [Google Scholar] [CrossRef]
- Chen, B.; Xu, H.; Ni, M. Modelling of SOEC-FT reactor: Pressure effects on methanation process. Appl. Energy 2017, 185, 814–824. [Google Scholar] [CrossRef]
- Rehman, M.; Hafeez, M.B.; Krawczuk, M. A Comprehensive Review: Applications of the Kozeny–Carman Model in Engineering with Permeability Dynamics. Arch. Comput. Methods Eng. 2024, 31, 3843–3855. [Google Scholar] [CrossRef]
- Zhang, J.; Lei, L.; Liu, D.; Zhao, F.; Chen, F.; Wang, H. Numerical investigation of solid oxide electrolysis cells for hydrogen production applied with different continuity expressions. Energy Convers. Manag. 2017, 149, 646–659. [Google Scholar] [CrossRef]
- Szmyt, W.; Guerra-Nuñez, C.; Dransfeld, C.; Utke, I. Solving the inverse Knudsen problem: Gas diffusion in random fibrous media. J. Membr. Sci. 2021, 620, 118728. [Google Scholar] [CrossRef]
- Khirevich, S.; Höltzel, A.; Daneyko, A.; Seidel-Morgenstern, A.; Tallarek, U. Structure–transport correlation for the diffusive tortuosity of bulk, monodisperse, random sphere packings. J. Chromatogr. A 2011, 1218, 6489–6497. [Google Scholar] [CrossRef]
- Xu, Z.; Zhang, X.; Li, G.; Xiao, G.; Wang, J. Comparative performance investigation of different gas flow configurations for a planar solid oxide electrolyzer cell. Int. J. Hydrogen Energy 2017, 42, 10785–10801. [Google Scholar] [CrossRef]
- Zhang, B.; Harun, N.F.; Zhou, N.; Oryshchyn, D.; Colon-Rodriguez, J.J.; Shadle, L.; Bayham, S.; Tucker, D. A real-time distributed solid oxide electrolysis cell (SOEC) model for cyber-physical simulation. Appl. Energy 2025, 388, 125607. [Google Scholar] [CrossRef]
- Hsieh, C.; Tuan, W. Elastic and thermal expansion behavior of two-phase composites. Mater. Sci. Eng. A 2006, 425, 349–360. [Google Scholar] [CrossRef]
- Chi, H.; Xiao, L.; Deng, T.; Pan, B.; Yuan, J. Modeling and characterization of sintered YSZ/NiO porous electrode structural properties using coarse-graining molecular dynamics method. Ceram. Int. 2024, 50, 26205–26219. [Google Scholar] [CrossRef]
- Choi, S.; Cheung, C.; Graeve, O.A. Fabrication of continuous linear pores in an SOFC anode using unidirectional carbon fibers as sacrificial templates. J. Am. Ceram. Soc. 2021, 104, 3030–3041. [Google Scholar] [CrossRef]
- Hedayat, N.; Du, Y.; Ilkhani, H. Pyrolyzable pore-formers for the porous-electrode formation in solid oxide fuel cells: A review. Ceram. Int. 2018, 44, 4561–4576. [Google Scholar] [CrossRef]

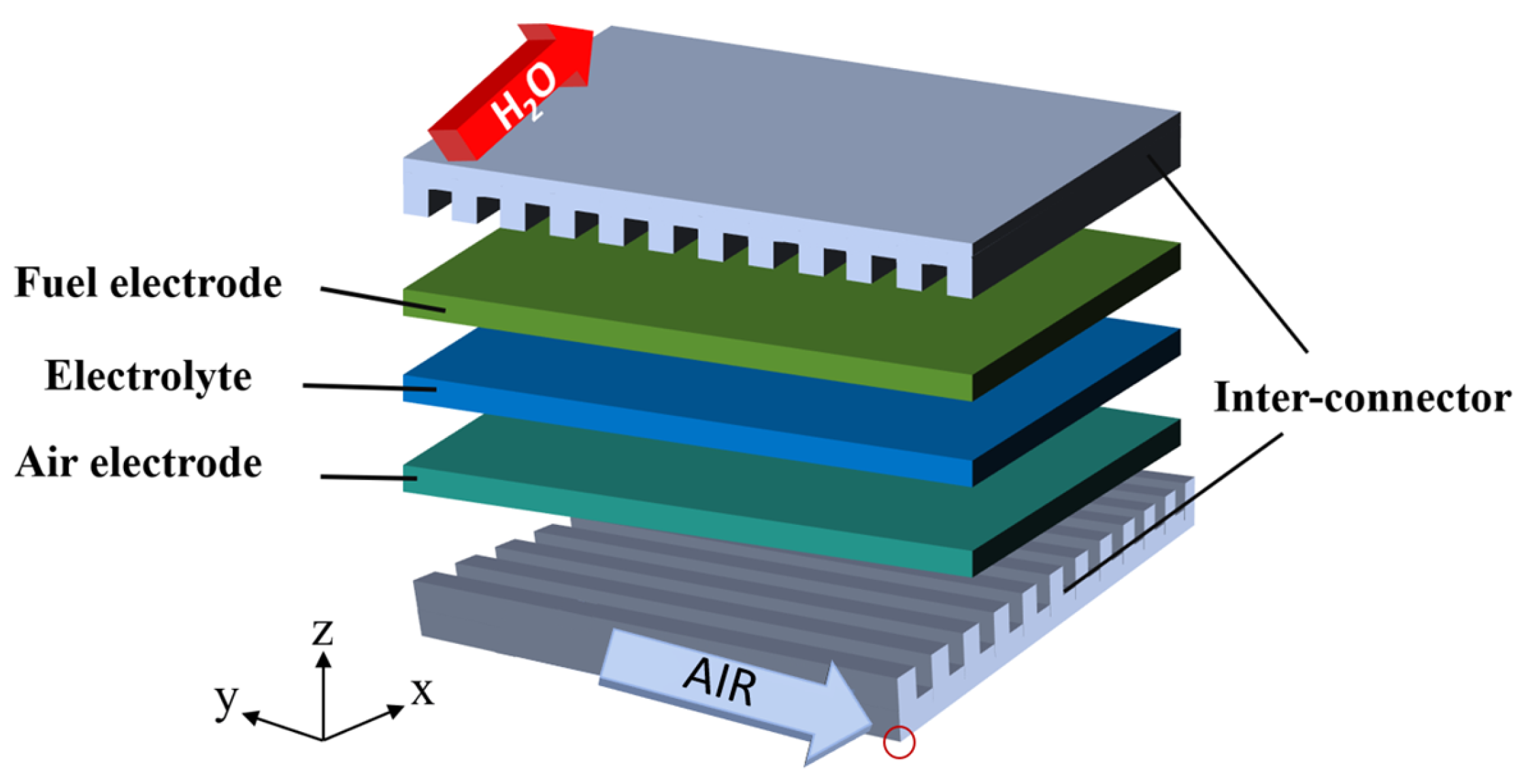
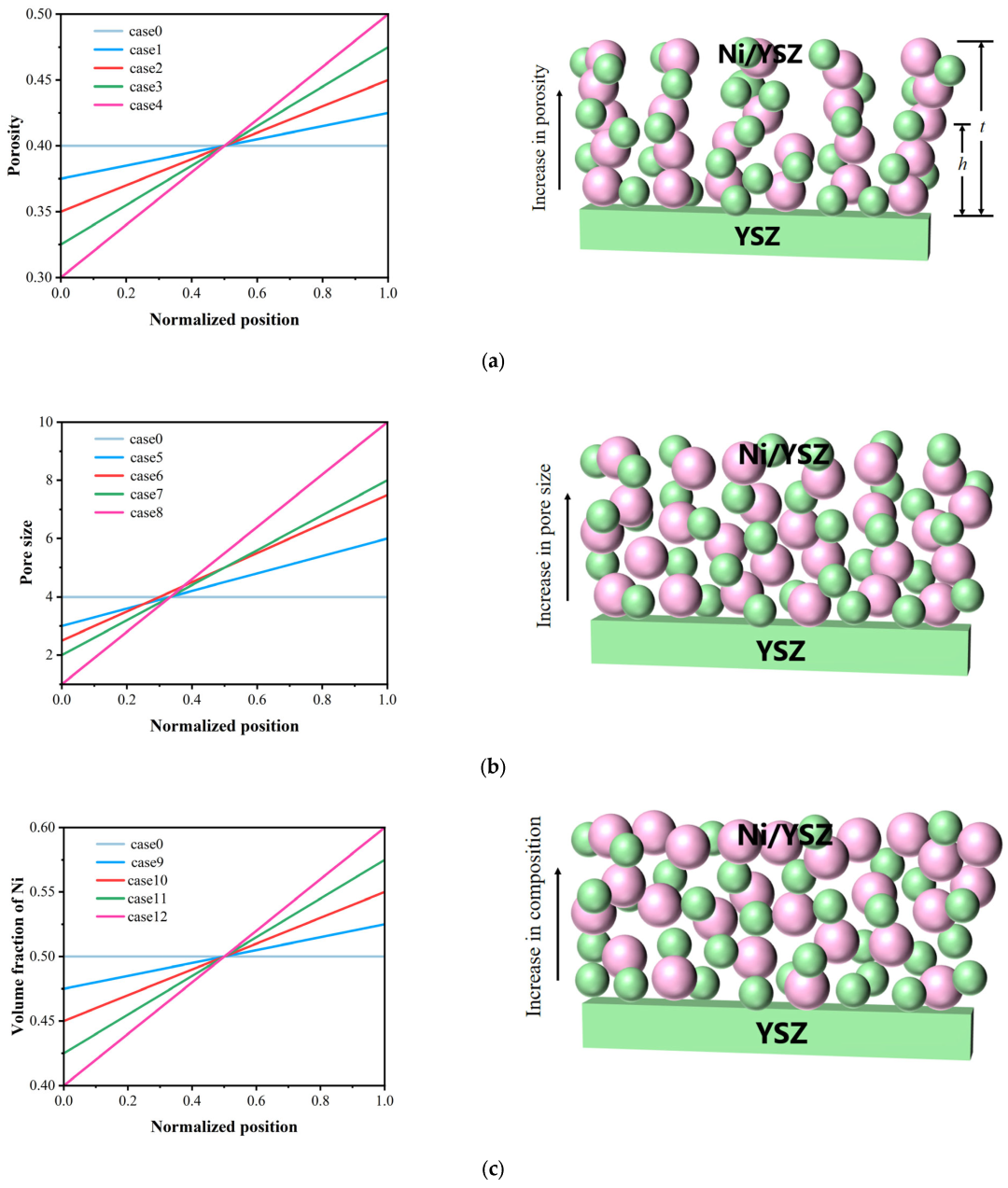

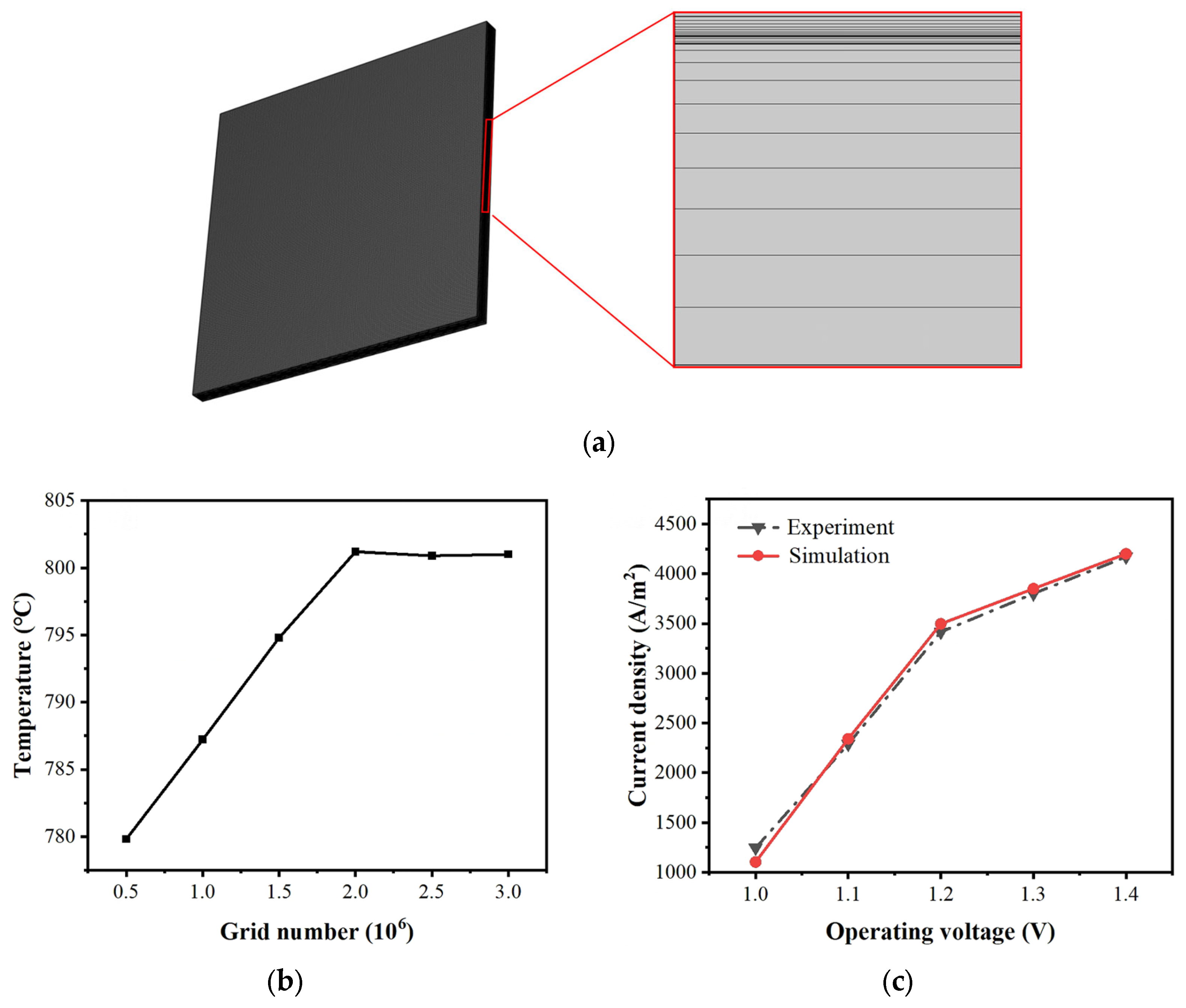
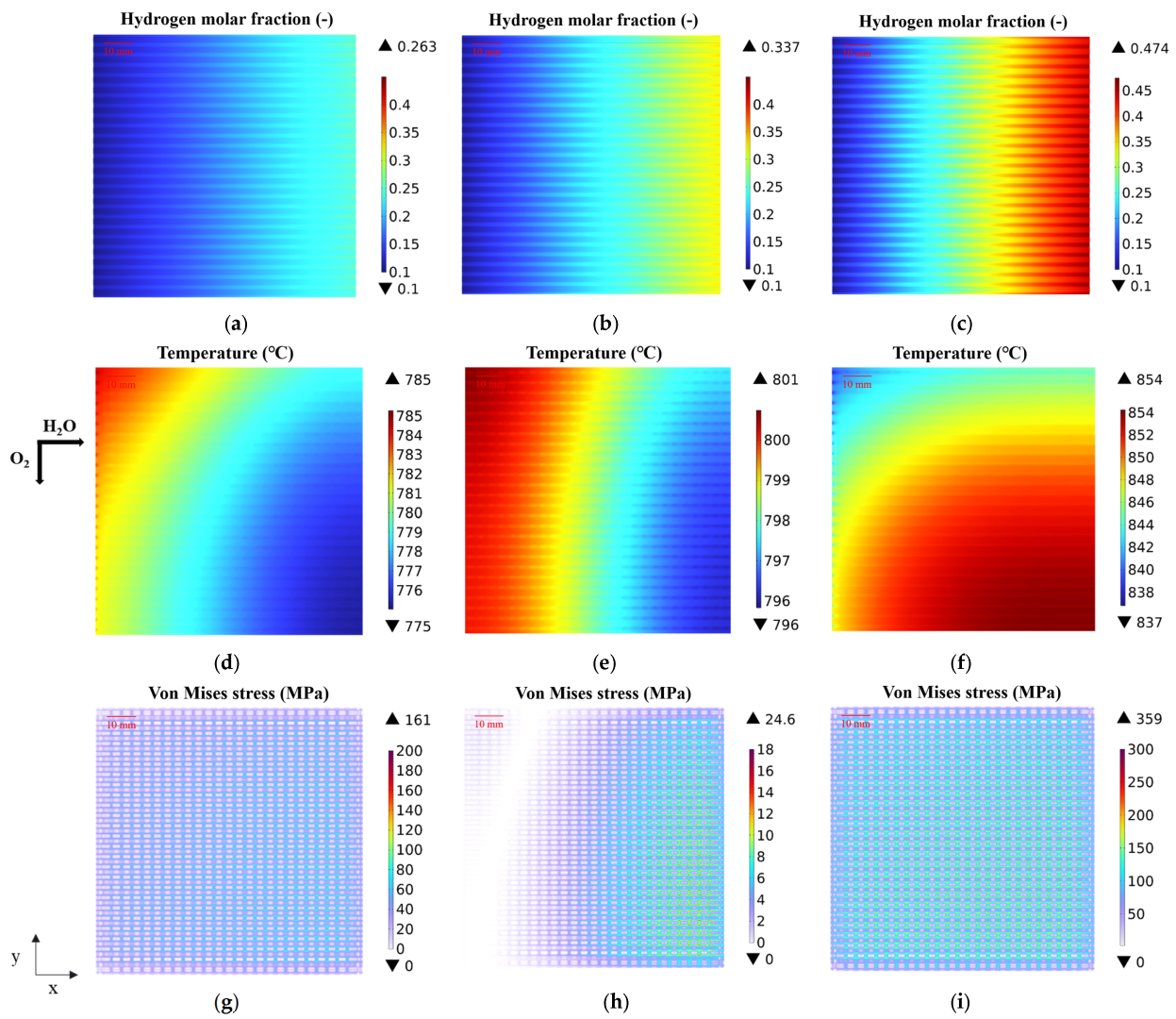



| Parameter | Value | Unit |
|---|---|---|
| Cell width | 91 | mm |
| Cell length | 91 | mm |
| Channel height | 1.0 | mm |
| Channel width | 1.75 | mm |
| Rib width | 1.75 | mm |
| Number of channels | 26 | - |
| Fuel electrode thickness | 410 | μm |
| Electrolyte thickness | 10 | μm |
| Air electrode thickness | 25 | μm |
| Parameter | Fuel Electrode | Electrolyte | Air Electrode | Interconnector |
|---|---|---|---|---|
| Material | Ni-YSZ | YSZ | LSM | Stainless steel |
| Density (kg/m3) | 7740 | 6000 | 5300 | 7700 |
| Specific heat capacity (J/(kg · K)) | 595 | 400 | 607 | 600 |
| Thermal conductivity (W/(m · K)) | 6.23 | 2.7 | 10 | 44.5 |
| Electronic/ionic conductivity (1/(Ω · m)) | 769,000 | |||
| CTE (1/K) | 12.2 | 10.3 | 11.7 | 15.5 |
| Young’s modulus (GPa) | 57 | 185 | 35 | 214 |
| Poisson’s ratio | 0.28 | 0.32 | 0.25 | 0.3 |
| γ/A·m−2 | Eact/J·mol−1 | |
|---|---|---|
| Fuel electrode | 1.34 × 1010 | 1 × 105 |
| Air electrode | 2.05 × 108 | 1.2 × 105 |
| Boundary | Momentum | Thermal | Species | Electric Potential |
|---|---|---|---|---|
| Top IC wall | - | symmetry | - | Φtop = Vcell |
| Bottom IC wall | - | symmetry | - | Φbottom = 0 |
| Air inlet | 0.33 L/min | 800 °C | 21% O2, 79% N2 | - |
| Air outlet | pressure | convection | convection | - |
| Fuel inlet | 0.217 L/min | 800 °C | 90% H2O, 10% H2 | - |
| Fuel outlet | pressure | convection | convection | - |
| Side walls | - | insulation | - | - |
| Case | Xn | X0 | m |
|---|---|---|---|
| Case 0 | no graded porosity (−) | 0.400 | 0 |
| Case 1 | graded porosity (−) | 0.375 | 0.425 |
| Case 2 | graded porosity (−) | 0.350 | 0.450 |
| Case 3 | graded porosity (−) | 0.325 | 0.475 |
| Case 4 | graded porosity (−) | 0.300 | 0.500 |
| Case | Xn | X0 | m |
|---|---|---|---|
| Case 0 | no graded pore size (μm) | 4.00 | 0 |
| Case 5 | graded pore size (μm) | 3.00 | 6.00 |
| Case 6 | graded pore size (μm) | 2.50 | 7.50 |
| Case 7 | graded pore size (μm) | 2.00 | 8.00 |
| Case 8 | graded pore size (μm) | 1.00 | 10.0 |
| Case | Xn | X0 | m |
|---|---|---|---|
| Case 0 | no graded Ni composition (−) | 0.500 | 0 |
| Case 9 | graded Ni composition (−) | 0.475 | 0.525 |
| Case 10 | graded Ni composition (−) | 0.450 | 0.550 |
| Case 11 | graded Ni composition (−) | 0.425 | 0.575 |
| Case 12 | graded Ni composition (−) | 0.400 | 0.600 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, F.; Xiao, L.; Zhou, R.; Liu, Q.; Yuan, J. Evaluation of Thermal Stress and Performance for Solid Oxide Electrolysis Cells Employing Graded Fuel Electrodes. Energies 2025, 18, 2790. https://doi.org/10.3390/en18112790
Liu F, Xiao L, Zhou R, Liu Q, Yuan J. Evaluation of Thermal Stress and Performance for Solid Oxide Electrolysis Cells Employing Graded Fuel Electrodes. Energies. 2025; 18(11):2790. https://doi.org/10.3390/en18112790
Chicago/Turabian StyleLiu, Fangzheng, Liusheng Xiao, Ruidong Zhou, Qi Liu, and Jinliang Yuan. 2025. "Evaluation of Thermal Stress and Performance for Solid Oxide Electrolysis Cells Employing Graded Fuel Electrodes" Energies 18, no. 11: 2790. https://doi.org/10.3390/en18112790
APA StyleLiu, F., Xiao, L., Zhou, R., Liu, Q., & Yuan, J. (2025). Evaluation of Thermal Stress and Performance for Solid Oxide Electrolysis Cells Employing Graded Fuel Electrodes. Energies, 18(11), 2790. https://doi.org/10.3390/en18112790






