Stand-Alone Operation of Multi-Phase Doubly-Fed Induction Generator Supplied by SiC-Based Current Source Converter

Abstract

This paper investigates the performance of a five-phase silicon carbide (SiC)-based current-source converter (CSC) integrated with a Doubly Fed Induction Generator (DFIG) for wind energy applications. The study explores both healthy and faulty operation, focusing on system behavior under transient conditions and various load scenarios in stand-alone mode. A novel five-phase space vector PWM strategy in dual coordinate planes is introduced, which enables stable control during normal and open-phase fault conditions. Experimental results demonstrate improved stator voltage and current quality, particularly in terms of reduced Total Harmonic Distortion (THD), compared to traditional voltage-source converter-based systems. Furthermore, the system maintains operational stability under a single-phase open fault, despite increased oscillations in stator quantities. The results highlight the potential of five-phase CSC-DFIG systems as a robust and efficient alternative for wind power plants, particularly in configurations involving long cable connections and requiring low generator losses. Future work will focus on enhancing fault-tolerant capabilities and expanding control strategies for improved performance under different operating conditions.

1. Introduction

The growing demand for electricity and the increasing environmental concerns are driving the search for renewable energy sources. As reported by the Global Wind Energy Council (GWEC) in 2024, the global wind power capacity crossed 1 TW, a figure that the GWEC also anticipates will double before 2030. This presents an opportunity to advance the technology used in Wind Energy Conversion Systems (WECSs), including power sources, converters, and control systems [1,2].

Doubly Fed Induction Generators (DFIGs) are now widely used in WECS instead of permanent magnet synchronous generators or induction generators due to their several features, such as the ability to actively control speed and separate control of active and reactive power output. This contributes to improving grid stability and integrating renewable energy sources. The described benefits of DFIG allow optimal performance in offshore wind energy conditions, known for their higher wind speeds and variability in wind patterns [3,4,5].

Wind power plants, both onshore and offshore, require highly fault-tolerant generators to reduce downtime in power generation. The multi-phase generators offer several advantages over traditional three-phase systems under such conditions. Those include improved fault tolerance, higher torque density per unit volume, reduced torque fluctuations, and the ability to operate even with the loss of one or two excitation phases [6].

The power electronic converters used with DFIGs can be divided into two categories: Voltage Source Converters (VSCs) and Current Source Converters (CSCs). CSCs present an intriguing alternative to VSCs with sine-wave filters, particularly since the efficiencies of both systems are comparable [7]. CSCs are recognized for their inherent advantages, including low output voltage and current total harmonic distortion (THD < 2.5%), low derivative of the output voltage, four-quadrant operation, and short-circuit protection achieved through the use of a large inductance in the DC-link circuit. The current source converter can deliver machine-friendly current and voltage, which positively impacts winding insulation and machine bearings, as well as reduces electromagnetic and acoustic noise [8,9,10]. Additionally, in the context of a five-phase circuit, the number of passive components is reduced compared to VSC solutions with sine wave filters. Furthermore, by utilizing modern silicon carbide (SiC) transistors and increasing the switching frequency, the size and weight of the inductor in the DC-link circuit of the CSC can be minimized, along with the capacitance of the filtering capacitors.

Despite their limited popularity, CSCs show promise as an alternative for medium-voltage drives with induction machines (IMs) [9,11], as well as for machines connected by long cables between the converter and motor terminals [7]. The ability to operate with long connection cables can be particularly advantageous in wind power plants, as the converter could be located at the base of the tower rather than in the nacelle. In three-phase systems, current-source inverters have been proposed for applications in energy production and grid integration, such as photovoltaics [12], wind energy generation [13], and high-voltage DC transmission [14]. However, five-phase current-source converters have not been extensively discussed in the literature, likely due to their generally low demand and the challenges associated with such systems, including the development of new PWM and control strategies related to dual coordinate systems.

This article focuses on the stand-alone operation of a five-phase power generation system. The system comprises a five-phase, SiC-based current converter and a Doubly Fed Induction Generator with a five-phase slip-ring rotor and a three-phase stator. The main objective of this work is to highlight the advantages of a five-phase DFIG fed by a CSC, compared to traditional three-phase generators fed by a VSC. The authors examine steady-state characteristics in terms of THD and harmonic spectra for stator voltages and currents. In addition, closed-loop performance is verified over +/−30% of synchronous speed and various load points, which are typical working conditions for a DFIG employed in a wind power plant. To assess the system’s effectiveness in WECS application, the authors measure the stator quantities during speed variations, resistive load changes, and application of nonlinear loads.

The main contributions of this work are as follows.

• Combination of the advantages of a five-phase DFIG with a five-phase current source converter, resulting in increased fault resistance and higher power quality.
• Presentation of a five-phase space vector PWM strategy in dual coordinates and possible faulty operation.
• The experimental investigation of a five-phase CSC and a DFIG system working in a stand-alone system, which included open-loop and closed-loop operation, different load types, and a wide range of mechanical speeds similar to a typical WECS application. To the authors’ knowledge, this has never been reported in the literature.
• Presentation of healthy and faulty (open-phase) operation of a five-phase CSC and a DFIG system that works in a standalone system.

2. Five Phase Doubly Fed Induction Generator

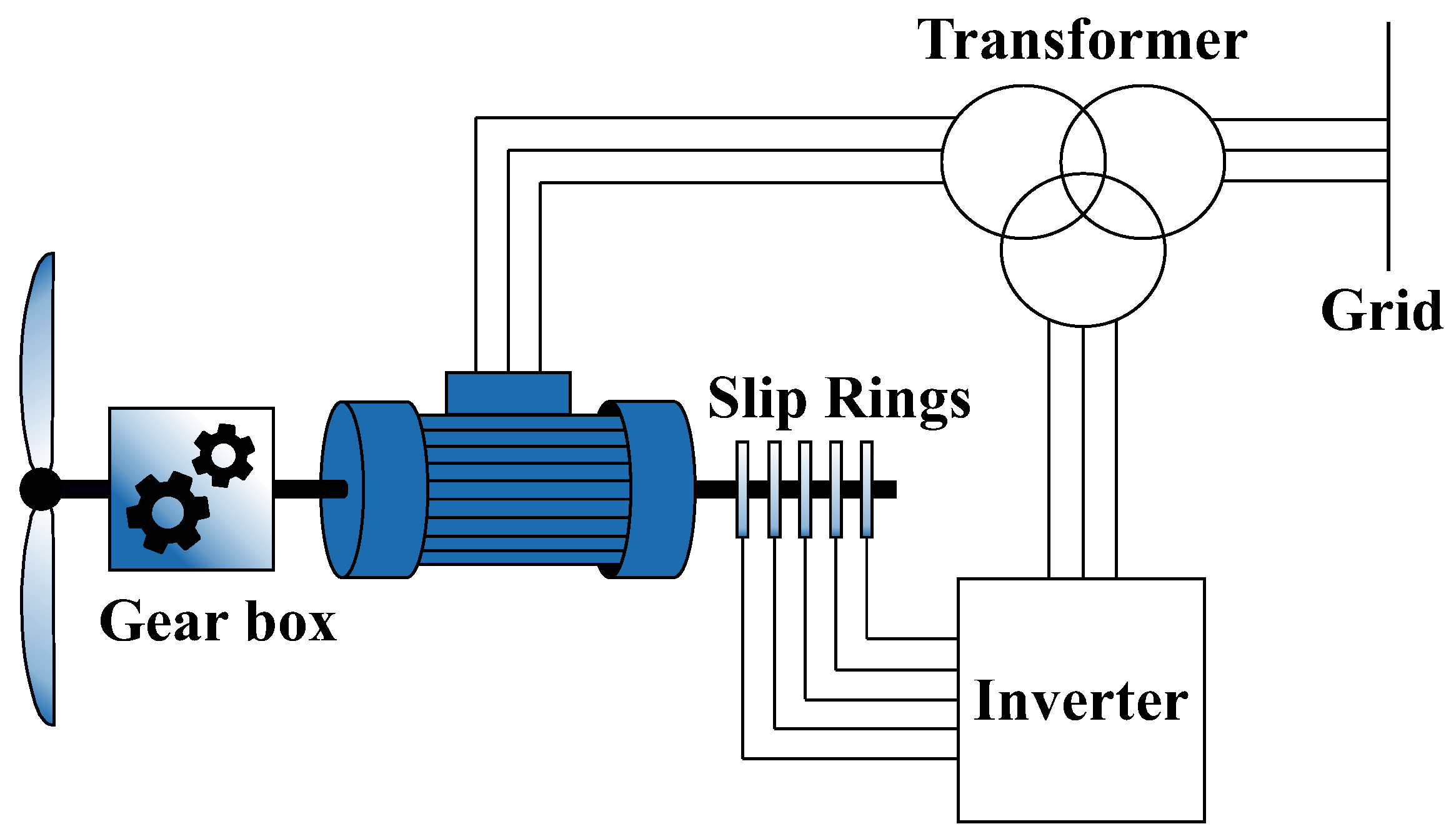
Figure 1 presents a general schematic of a wind power conversion system using five-phase components. Such a WECS consists of the following main parts: a multi-phase doubly fed induction generator, the rotor side converter (RSC), and the grid side converter (GSC). The DFIG operates on the principle of feeding alternating current into both the stator and rotor windings. This allows for variable speed operation and improved power control. The RSC is responsible for controlling the generator’s rotor currents. The GSC manages the DC link voltage and the grid power factor. In the context of this paper, the DFIG operates in a stand-alone configuration, rendering the GSC’s grid functions irrelevant.

One of the primary advantages of DFIG systems is their ability to operate at variable speeds. This allows maximum power to be extracted from wind turbines as wind conditions change. In addition, DFIGs offer improved energy efficiency and reduced mechanical stress on the components of the wind turbine. However, a significant drawback of traditional DFIG designs is the need for slip rings and brushes to connect the rotor windings, which can lead to increased maintenance requirements. To address this issue, brushless alternatives such as the Brushless Doubly Fed Induction Generator (BDFIG) have been developed, offering potential improvements in reliability and reduced maintenance costs [15]. Another solution to this problem is the use of multi-phase rotor windings [4].

In addition, traditional VSCs used in the DFIG can introduce harmonic distortion into the grid. This can degrade power quality. Using CSCs can help reduce the total harmonic distortion of the generator.

The DFIG used in this paper has a six-pole, three-phase stator winding and a five-phase slip-ring rotor winding. The main parameters of the generator are listed in

Table 1. Selected parameters of 5-phase DFIG.

Parameter	Symbol	Value
Power	$P_n$	4 kW
Stator voltage	$Un$	400 V
Synchronous speed	$nn$	1000 rpm
Pair poles	p	3
Stator phases	$ms$	3
Rotor phases	$mr$	5
Stator resistance	$Rs$	2.5 $\mathsf{\Omega }$
Rotor resistance	$Rr$	2.27 $\mathsf{\Omega }$
Stator magnetizing inductance	$Lms$	0.33 H
Stator leakage inductance	$Lls$	0.045 H
Rotor magnetizing inductance	$Lmr$	0.25 H
Rotor leakage inductance	$Llr$	0.03 H
Mutual inductance	$Lsr$	0.29 H

. The development of this generator is described in detail in [16].

The DFIG model in a natural reference frame consists of stator parameters in a symmetric three-phase system and rotor parameters in a symmetric five-phase system. The voltage equations are defined as follows:

$$U_s=R_s{I}_s+{\displaystyle \frac{d}{dt}}{\psi }_s,$$

(1)

$$U_r=R_r{I}_r+{\displaystyle \frac{d}{dt}}{\psi }_r.$$

(2)

where:

$$U_s={\left[\begin{array}{ccc}{U}_{as}& U_{bs}& U_{cs}\end{array}\right]}^T,$$

(3)

$$U_r={\left[\begin{array}{ccccc}{U}_{ar}& U_{br}& U_{cr}& U_{dr}& U_{er}\end{array}\right]}^T,$$

(4)

$$I_s={\left[\begin{array}{ccc}{i}_{as}& i_{bs}& i_{cs}\end{array}\right]}^T,$$

(5)

$$I_r={\left[\begin{array}{ccccc}{i}_{ar}& i_{br}& i_{cr}& i_{dr}& i_{er}\end{array}\right]}^T.$$

(6)

$R_s$, $R_r$—stator and rotor diagonal resistance matrices, ${\psi }_s$, ${\psi }_r$—stator and rotor flux linkage vectors. The flux linkage equation for the stator and rotor winding is defined as:

$$\left[\begin{array}{c}{\psi }_s\\ {\psi }_r\end{array}\right]=\left[\begin{array}{cc}{L}_{ss}& L_{sr\left(\phi \right)}\\ L_{rs\left(\phi \right)}& L_{rr}\end{array}\right]\left[\begin{array}{c}{I}_s\\ I_r\end{array}\right].$$

(7)

where $L_{ss}$—stator winding inductance matrix, $L_{rr}$—rotor winding inductance matrix, $L_{sr}=L_{rs}$—stator and rotor mutual winding inductance matrix, $\phi$—rotor angle.

The mechanical equation for DFIG is defined as:

$$T_{em}=T_l+J{\displaystyle \frac{d\omega }{dt}}+D\omega .$$

(8)

where $T_{em}$—electromagnetic torque, $T_l$—load torque, J—moment of inertia, D—the mechanical damping constant, $\omega ={\displaystyle \frac{d\phi }{dt}}$—rotor angular velocity.

The electromagnetic torque is determined below:

$$T_{em}={\displaystyle \frac{1}{2}}\left[\begin{array}{cc}{I}_s^T& I_r^T\end{array}\right]{\displaystyle \frac{\partial }{\partial \phi }}\left[\begin{array}{cc}{L}_{ss}& L_{sr\left(\phi \right)}\\ L_{rs\left(\phi \right)}& L_{rr}\end{array}\right]\left[\begin{array}{c}{I}_s\\ I_r\end{array}\right].$$

(9)

The above model was implemented in Simulink as a Simscape component (Figure 2). This block uses the physical modeling language of Simscape written as a text file and compiled at run-time. The key sections of this model are the following:

• A declaration specifying the types and names of nodes, variables, and parameters (e.g., as = foundation.electrical.electrical; vas = 0, `V’; Rs = 2.5, ‘Ohm’;).
• Branches that establish relationships between variables and terminals, encompassing both through and across variables (e.g., ias: xs.i -> as.i;).
• An equation section, where the differential equations are defined (e.g., vas == Rs*ias + phias.der;).

where xs, as—stator nodes, vas, ias, phias—stator voltage, current and flux variables, Rs—stator resistance and der is the derivative operator.

The dynamic behavior of the generator was simulated under the following conditions.

• The rotor winding was supplied by five controlled voltage sources with a phase difference of 2$\pi$/5.
• The rotor supply voltage frequency was set to 10 Hz and the mechanical speed was 801 rpm.
• The step change in the rotor supply voltage was tested for a generator that operates in a standalone mode.
• The stator winding was connected to a symmetric RC load (R = 140 $\mathsf{\Omega }$ and C = 50 $\mathsf{\mu }$F per phase).

The comparison of simulated and measured stator voltage and current is presented in Section 4.2.

Figure 2. Simulink model of multi-phase DFIG working in a stand-alone mode.

Figure 2. Simulink model of multi-phase DFIG working in a stand-alone mode.

3. Five Phase Current-Source Converter

3.1. Fundamentals of CSC

Figure 3 presents the general scheme of the phase-phase current-source converter. The source of the unidirectional current $i_d$ in the DC-link circuit is an inductor with significant inductance $L_d$. The regulation of the DC-link voltage $e_d$ can be achieved through the following methods:

• Controlled rectifier bridge—This configuration allows for four-quadrant operation [17].
• Uncontrolled rectifier bridge with a chopper circuit—In this case, energy cannot flow from the machine back to the three-phase grid.

Figure 3. General scheme of five-phase current-source converter.

Figure 3. General scheme of five-phase current-source converter.

The five-phase current-source converter contains ten unidirectional switches with the diodes in series that connect the DC-link circuit to the inverter’s output. The capacitors as an integral part of the CSC are connected to the inverter’s output terminals. In thyristor-based current-source inverters, these capacitors ensured proper commutation of the thyristor, whereas in modern inverters, they filter the output voltage. In a current-source inverter, the control of gate signals should ensure the continuity of the DC-link current, always through a sequence of switching with two conducting transistors (one upper and one lower at the same time). The differential equations for the DC-link circuit and the output voltage of the current-source inverter are presented below:

$${\displaystyle \frac{d{i}_d}{dt}}=-{\displaystyle \frac{R_d}{L_d}}{i}_d+{\displaystyle \frac{1}{L_d}}\left(e_d-u_d\right),$$

(10)

$${\displaystyle \frac{d{U}_c}{dt}}={\displaystyle \frac{1}{C_M}}\left(I_f-I_r\right).$$

(11)

where $i_d$—DC-link current, $R_d$, $L_d$—resistance and inductance of DC-link inductor, $e_d$—regulated input voltage for DC-link circuit, $u_d$—direct DC voltage, $U_c$—output inverter voltage vector, $C_M$—output filter capacity, $I_f$—converter current vector, $I_r$—rotor current vector. From the equality of active power on the DC and AC sides of the current-source converter, the direct DC voltage $u_d$ value is given as follows:

$$u_d={\displaystyle \frac{|I_r\left|\right|U_r|cos\left(\delta \right)}{i_d}}.$$

(12)

where $\delta$—the angle between current and voltage rotor vectors. Considering the dynamics of voltage $U_c$ variations across the passive filter, the rotor voltage at the generator rotor terminals is defined as follows:

$$U_r=R_c\left(I_f-I_r\right)+U_c.$$

(13)

The inductance value of the DC choke $L_d$ can be calculated using the following equation, based on the assumed current ripple $\Delta i_d$ in the DC circuit:

$$L_d={\displaystyle \frac{1}{\Delta i_d}}{\int }_{t_1}^{t_2}\left(e_d-u_d\right)dt.$$

(14)

where $t_1$, $t_2$ are times derived from the converter switching frequency.

The literature contains several studies discussing the control of three-phase induction machines supplied by current-source converters [18,19,20,21,22,23,24,25]. The control methods presented in these studies can be categorized based on the choice of control variables:

• Current control, where the control variables are the components of the inverter’s output current vector.
• Voltage control, where the control variables are the DC-link voltage and the motor slip.

In current control, the DC-link current can be maintained at a nominal level or can vary depending on the operating point of the load [18,19,20,21,22,23,24,25,26,27]. In the latter case, an additional controller for the inductor current is necessary. When the inductor current is kept at the nominal level, the modulation index is adjusted to change the desired amplitude of the inverter’s output current. Furthermore, maintaining the inductor current at the nominal level generates significant losses in the semiconductors and in the resistance of the inductor, especially when operating at a point other than the nominal.

Some recent articles discuss the technical details of the operational features and control of the DFIG-CSC three-phase system [28,29]. Most of these studies are related to field-oriented control and focus mainly on theoretical analysis. The paper [30] describes feedback control for a three-phase DFIG, which can be categorized as a type of voltage control for CSC. In this paper, a relatively simple current control scheme with only two PI controllers is proposed, assuming that the converter operates at the maximum modulation index, which can be expressed as:

$$M={\displaystyle \frac{|I_f|}{i_d}}.$$

(15)

3.2. SVPWM for Five-Phase CSC in Normal Operation

The output current generated in the CSC is determined by the current states of the switches and the DC-link current value. The control of the transistors in the CSC must ensure the continuity of the current in the DC-link circuit to prevent a large derivative value: $d{i}_d/dt$. In a CSC, only two transistors (one upper and one lower) are always conducting while generating either an active or passive vector. There are two general switching combinations:

• When two transistors in different phases are switched on, energy flows between the inverter and the load.
• When two transistors in the same phase are switched on, no energy flows to the load.

In a five-phase CSC, the output current can be formed using 25 vectors: 20 active and 5 passive. Among the active vectors, long and short vectors can be distinguished. The output current i(k) in any phase of the inverter (k = a...e) can be determined using the following relationship:

$$i_{\left(k\right)}=i_d(S_{\left(k\right)U}-S_{\left(k\right)L}),$$

(16)

where $S_{\left(k\right)U}$,$S_{{\left(k\right)}_L}$—the gate signals of the upper (U) and lower (L) transistors in phase k (values: 1—on, 0—off). In the higher-level control system, the reference output current of the inverter is defined as a space vector in the orthogonal stationary coordinate system: ${\alpha }^{\left(1\right)}-{\beta }^{\left(1\right)}-{\alpha }^{\left(2\right)}-{\beta }^{\left(2\right)}$. The ${\alpha }^{\left(x\right)}-{\beta }^{\left(x\right)}$ components of the active vectors can be determined using the phase values (for specific switching states) and the Clarke transformation matrix for the five-phase system [31,32]:

$$\begin{array}{cc}\hfill \left[\begin{array}{c}{i}_0^{\left(0\right)}\\ i_{\alpha }^{\left(1\right)}\\ i_{\beta }^{\left(1\right)}\\ i_{\alpha }^{\left(2\right)}\\ i_{\beta }^{\left(2\right)}\end{array}\right]& =\sqrt{{\displaystyle \frac{2}{5}}}·\mathbf{T}·\left[\begin{array}{c}{i}_{fa}\\ i_{fb}\\ i_{fc}\\ i_{fd}\\ i_{fe}\end{array}\right],\gamma ={\displaystyle \frac{2}{5}}\pi ,\hfill \end{array}$$

(17)

$$\begin{array}{c}\hfill \mathbf{T}=\left[\begin{array}{ccccc}\sqrt{{\displaystyle \frac{1}{2}}}& \sqrt{{\displaystyle \frac{1}{2}}}& \sqrt{{\displaystyle \frac{1}{2}}}& \sqrt{{\displaystyle \frac{1}{2}}}& \sqrt{{\displaystyle \frac{1}{2}}}\\ 1& cos\left(\gamma \right)& cos\left(2\gamma \right)& cos\left(3\gamma \right)& cos\left(4\gamma \right)\\ 0& sin\left(\gamma \right)& sin\left(2\gamma \right)& sin\left(3\gamma \right)& sin\left(4\gamma \right)\\ 1& cos\left(2\gamma \right)& cos\left(4\gamma \right)& cos\left(6\gamma \right)& cos\left(8\gamma \right)\\ 0& sin\left(2\gamma \right)& sin\left(4\gamma \right)& sin\left(6\gamma \right)& sin\left(8\gamma \right)\end{array}\right].\end{array}$$

(18)

Despite the similarities between three-phase and five-phase converter designs, generating output currents in systems with more than three phases introduces challenges that must be addressed when developing a PWM algorithm. Each valid switching state simultaneously generates active vectors in two planes: ${\alpha }^{\left(1\right)}-{\beta }^{\left(1\right)}$ and ${\alpha }^{\left(2\right)}-{\beta }^{\left(2\right)}$. Consequently, the output current of a five-phase CSC is defined in two orthogonal coordinate systems. In a five-phase current-source converter, two output current vectors can be independently formed using four active vectors. Their activation times can be determined by transforming the equation:

$$I^{*}={\displaystyle \frac{i_d}{T_s}}\mathbf{I}·\mathbf{T}$$

(19)

where $\mathbf{T}$ is the matrix of activation times for the four active vectors, $\mathbf{I}$ is the matrix of the four selected active vectors, $I^{*}$ is the matrix of reference current vectors and $\mathit{Ts}$ is the switching period.

3.3. SVPWM for Five-Phase CSC Under Single Open-Phase Fault

The operation of five-phase systems under open-phase fault conditions is a well-studied topic for voltage-source converters, as documented in works such as [33,34,35]. However, for current-source converters, this area remains largely unexplored, with only one known study based on simulation results [11]. The contribution of the authors in this paper includes addressing this research gap. Five-phase systems offer a key advantage: the ability to operate even when one phase loses supply. Enabling operation under an open-phase fault condition in a current-source inverter requires modifications to the PWM strategy to avoid using transistors in the faulty phase. In a current-source inverter, the continuity of the DC-link inductor current must be maintained. The PWM modification for an open-phase fault involves reducing the number of active vectors from four to three, using a different transformation matrix, and redefining the representation of active vectors. For this study, a transformation matrix for the four-phase operation was used. The $T_{PF}$ (post-fault transformation) matrix is introduced to handle the open-phase fault condition in five-phase induction machines. This matrix is derived from the vector space decomposition (VSD) approach, which is commonly used for multiphase systems. Unlike the classical Clarke transformation used in three-phase systems, which focus on the symmetrical transformation of three-phase currents into two orthogonal components, the $T_{PF}$ matrix is specifically designed to preserve the symmetry of the prefault equations as much as possible in the five-phase case. This transformation matrix allows the use of the same equations ${\alpha }^{\left(1\right)}-{\beta }^{\left(1\right)}$ and ${\alpha }^{\left(2\right)}-{\beta }^{\left(2\right)}$ in both pre- and post-fault conditions, reducing the complexity of the control strategy during fault conditions. The derivation of this matrix can be found in [33,35]. During fault operation, the components in the secondary coordinate system are constrained as follows:

$$i_{\alpha }^{\left(2\right)}=-i_{\alpha }^{\left(1\right)},$$

(20)

$$i_{\beta }^{\left(2\right)}=0.$$

(21)

In the case of an open-phase fault, the activation times of the three vectors are calculated using the same Equation (19), but with the post-fault matrix $T_{PF}$ (23) and by forcing the components in the second coordinate system according to (20) and (21). The complete post-fault transformation, assuming $i_{fa}=0$, is presented in Equation (22).

The operational performance of five-phase current-source converters under open-phase fault conditions has not yet been extensively reported in the literature. Existing research on fault-tolerant operation in five-phase systems predominantly focuses on voltage-source converters rather than current-source converters. Therefore, the results presented on the open-phase operation of five-phase current-source converters are a novel contribution to the field of multi-phase drive systems.

$$\begin{array}{cc}\hfill \left[\begin{array}{c}{i}_{\alpha }^{\left(1\right)}\\ i_{\beta }^{\left(1\right)}\\ i_{\alpha }^{\left(2\right)}\\ i_{\beta }^{\left(2\right)}\end{array}\right]& =\sqrt{{\displaystyle \frac{2}{5}}}{\mathbf{T}}_{\mathbf{PF}}\left[\begin{array}{c}{i}_{fb}\\ i_{fc}\\ i_{fd}\\ i_{fe}\end{array}\right],\hfill \end{array}$$

(22)

$$\begin{array}{cc}\hfill {\mathbf{T}}_{\mathbf{PF}}& =\left[\begin{array}{cccc}cos\left(\gamma \right)-1& cos\left(2\gamma \right)-1& cos\left(3\gamma \right)-1& cos\left(4\gamma \right)-1\\ sin\left(\gamma \right)& sin\left(2\gamma \right)& sin\left(3\gamma \right)& sin\left(4\gamma \right)\\ sin\left(2\gamma \right)& cos\left(4\gamma \right)& sin\left(6\gamma \right)& sin\left(8\gamma \right)\\ 1& 1& 1& 1\end{array}\right].\hfill \end{array}$$

(23)

3.4. Proposed Control Structure

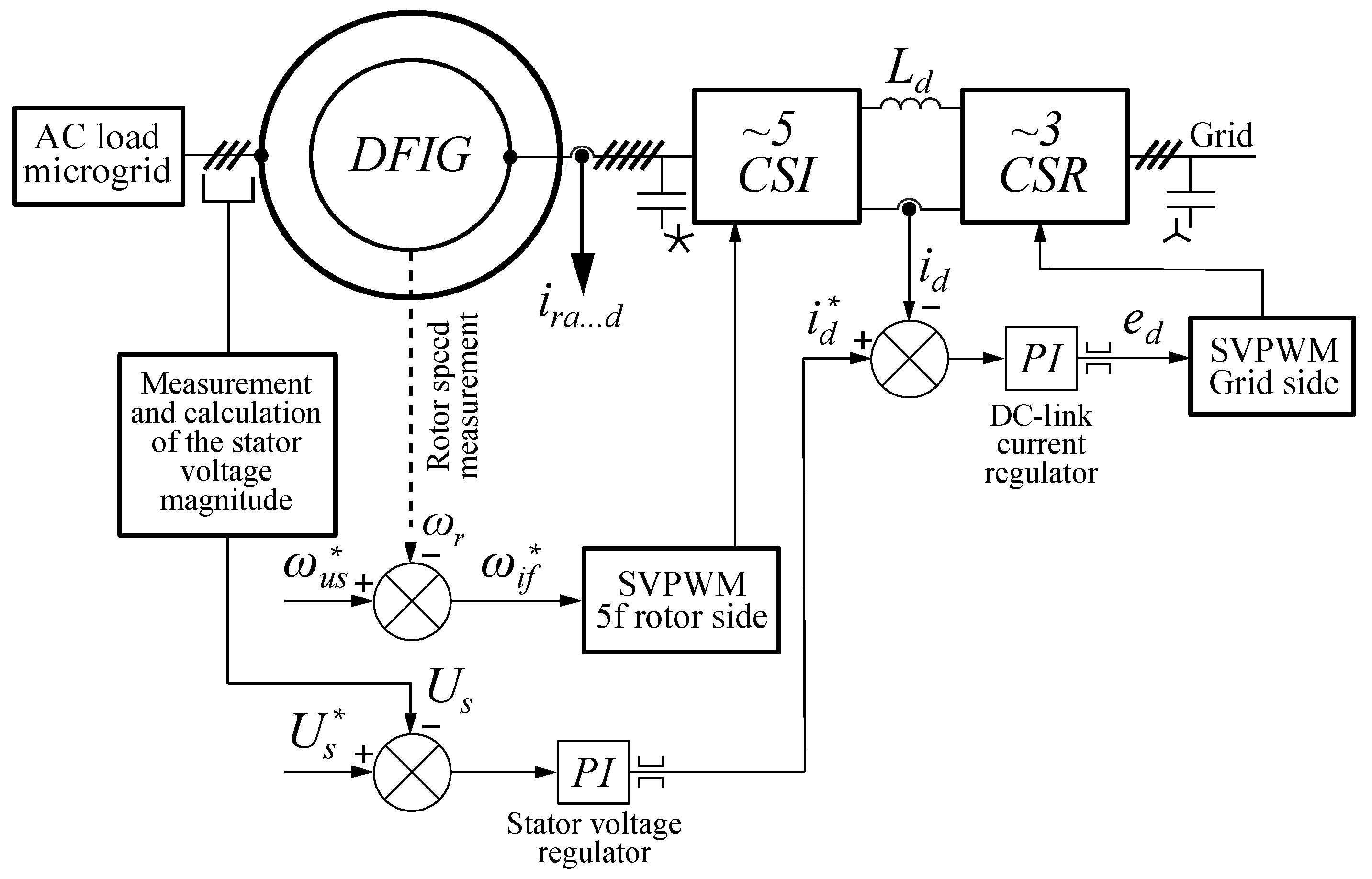
Figure 4 presents the control scheme of a five-phase DFIG supplied by a current-source converter for the case of stand-alone generator operation. The measured quantities are stator voltage, the rotor angular velocity of the DFIG generator and the DC-link current of the converter. The overall reference quantity for the control system is the amplitude of the stator voltage, which is fed to the input of the PI controller. The limited output of this controller is the reference current value in the DC-link circuit, which is regulated by a second PI controller. The output of the current regulator in the DC-link circuit is the reference value of $e_d$ voltage produced by the grid inverter. In the case of current excitation in the form of a CSC, the number of regulators is reduced compared to a VSC and the need for decoupling is reduced. The output current frequency of the converter ${\omega }_{if}^{*}$ is calculated from the slip and the reference frequency of the stator voltage ${\omega }_{us}^{*}$ is set to 50 Hz. In the SVPWM 5f block, based on the set frequency, the position angle of the output current vector is calculated, then the on and off times of the individual transistors. The five-phase systems are distinguished by two stationary $\alpha -\beta$ coordinate systems. Applying a suitable modulation method in such a case is challenging because each active vector simultaneously generates an output current in two coordinate systems (the first associated with the fundamental harmonic, the second with the third harmonic). The authors applied vector pulse width modulation with four active vectors, with the possibility of generating vectors independently in two $\alpha -\beta$ coordinate systems. The optimized modulation method presented in [36] for CSC was adopted. In the modulation algorithm, the overlap-time $t_d$ compensation was applied, the value of which is given in

Table 2. Selected parameters of 5-phase CSC.

Parameter	Symbol	Value
Switching frequency	$f_s$	20 kHz
DC-link rated current	$I_{dn}$	12 A
DC-link inductor inductance	$L_d$	20 mH
DC-link inductor saturation current	$I_{ds}$	20 A
Filter capacitance	$C_{M,G}$	10 $\mathsf{\mu }$F
Pulse-width modulation (5f side)	Dual Space Vector with 3rd harmonic compensation	[-]
Overlap-time (compensated)	$t_d$	0.3 $\mathsf{\mu }$s
Microprocessor unit	DSP + FPGA	[-]
Incremental encoder	FNC 30B	[-]
Transistors	$C2M0040120D$	[-]
Diodes	$C4D40120D$	[-]

.

4. Measurement Analysis

The performance of a five-phase power generation system, which consists of a current-source converter and a doubly fed induction generator (DFIG), was experimentally evaluated under open-loop and closed-loop operation. The open-loop analysis focused on total harmonic distortion (THD) and spectral measurements of stator voltage and current, with results compared to simulations. The closed-loop analysis included dynamic scenarios such as resistive and non-linear load switching, as well as speed variations crossing synchronous speed.

Both normal and fault conditions, with a single open-phase fault in phase A, were investigated. However, the study does not address the diagnosis or detection of open-phase faults in the current-source converter.

4.1. Test-Bench Description

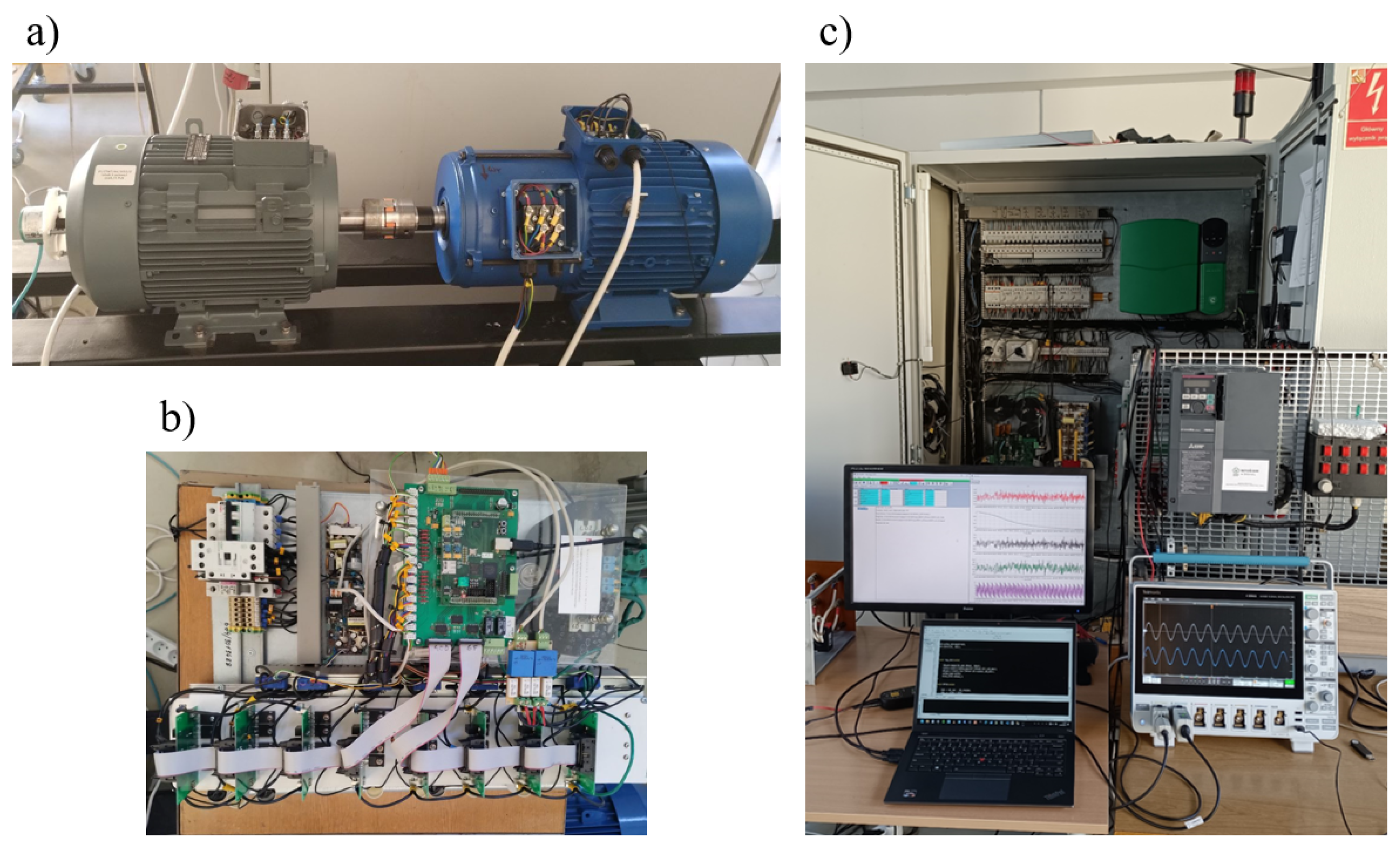
The prototype DFIG consists of a six-pole, three-phase stator winding and a five-phase slip-ring rotor winding. The generator case is repurposed from a 5.5 kW Sg132m-6 induction motor with modifications to accommodate five slip rings. The five-phase winding is arranged in 30 slots inside the inner rotor in a star configuration with a rated voltage of 400 V. The DFIG, driven by a 5.5 kW, 1500 rpm induction motor, is shown in Figure 5a.

The five-phase CSC consists of ten SiC transistors and diodes in series. The choke in the DC-link circuit has an inductance of 20 mH. The inverter operates at a frequency of 20 kHz. The filter capacitance is 10 uF on both the grid side and the rotor side. The microprocessor unit consists of the ADSP21363 signal processor and the CYCLONE II FPGA.

The former performs all floating-point operations, including SVPWMs, control algorithms, and scaling measurements. The second handles the ADCs (MAX1324) and provides signals to the transistor gate drivers. The prototype of the five-phase CSC is presented in Figure 5b.

The authors used a dedicated console written in C language, operating on a laptop PC. This configuration enabled the control of the inverter and the analysis of the electrical quantities supplied to the five-phase rotor winding. The motor speed was regulated by a three-phase, commercial-grade inverter located in the cabinet (Figure 5c).

A Tektronix MS046 oscilloscope was used to record three-phase voltages, currents, and power on the stator side. A Zimmer ZES power analyzer was used to measure THD and harmonic spectrum. To achieve different load points, the three-phase stator of the DFIG was connected to a variable load.

4.2. Open-Loop Performance

The generator operated across a range of speeds within +/−30% of synchronous speed and was evaluated under various load conditions, reflecting a typical WECS workload. The open-loop operation of the CSC-DFIG system was performed with an active frequency calculation for the converter, derived from the machine’s slip, as detailed in Section 3.4.

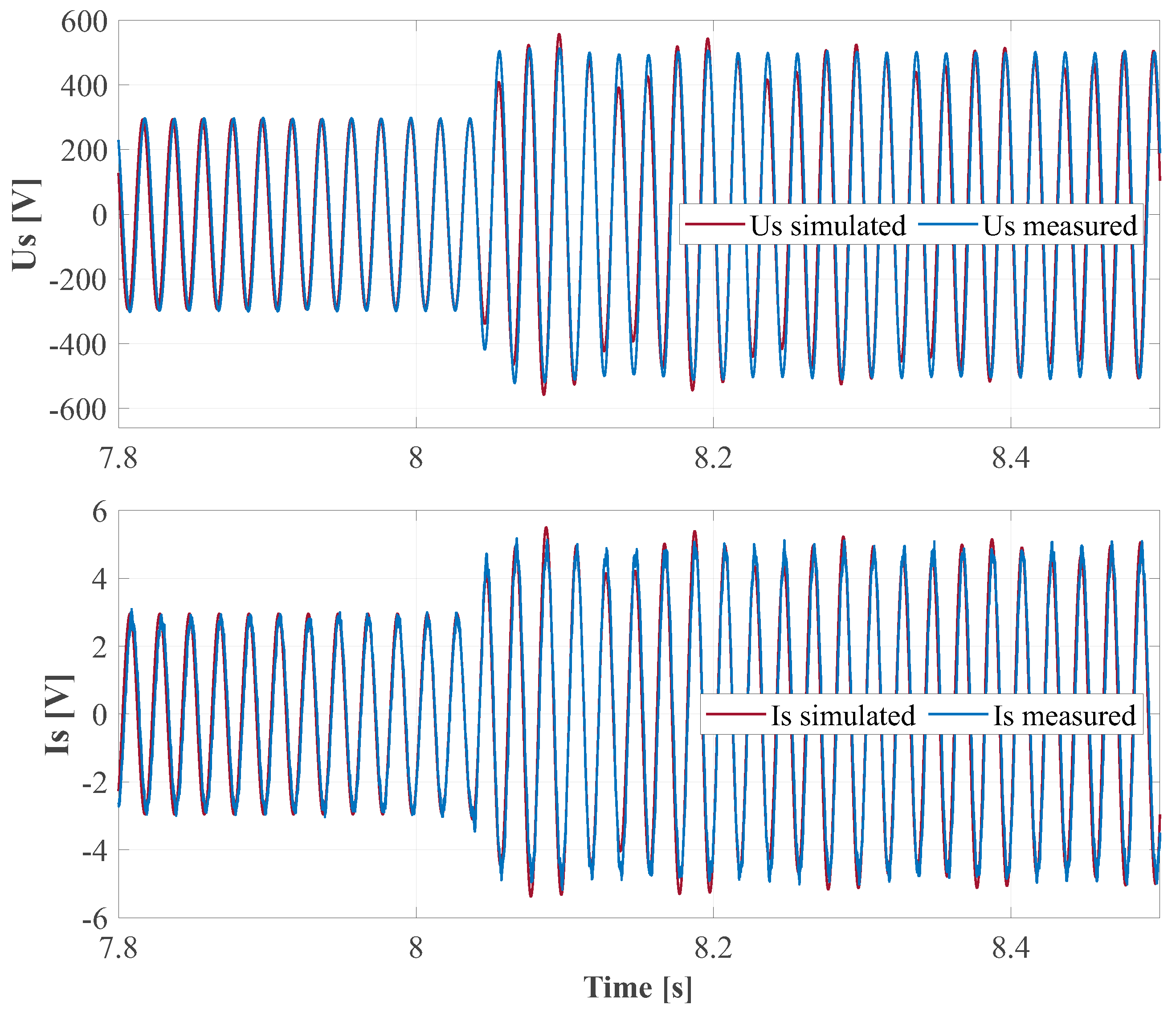
THD measurements of the stator voltage and current waveforms were collected in steady-state conditions. These results were compared to a setup in which the rotor was driven by a five-phase voltage-source converter, operating at the same frequency and constructed using SiC technology. Figure 6 illustrates a comparison between the simulated and measured stator voltage and current responses to a step change in the rotor supply. The detailed simulation conditions are listed at the end of Section 2. The results exhibit satisfactory agreement, with discrepancies during the transient period likely resulting from mismatches in the machine’s inductance parameters [37,38,39].

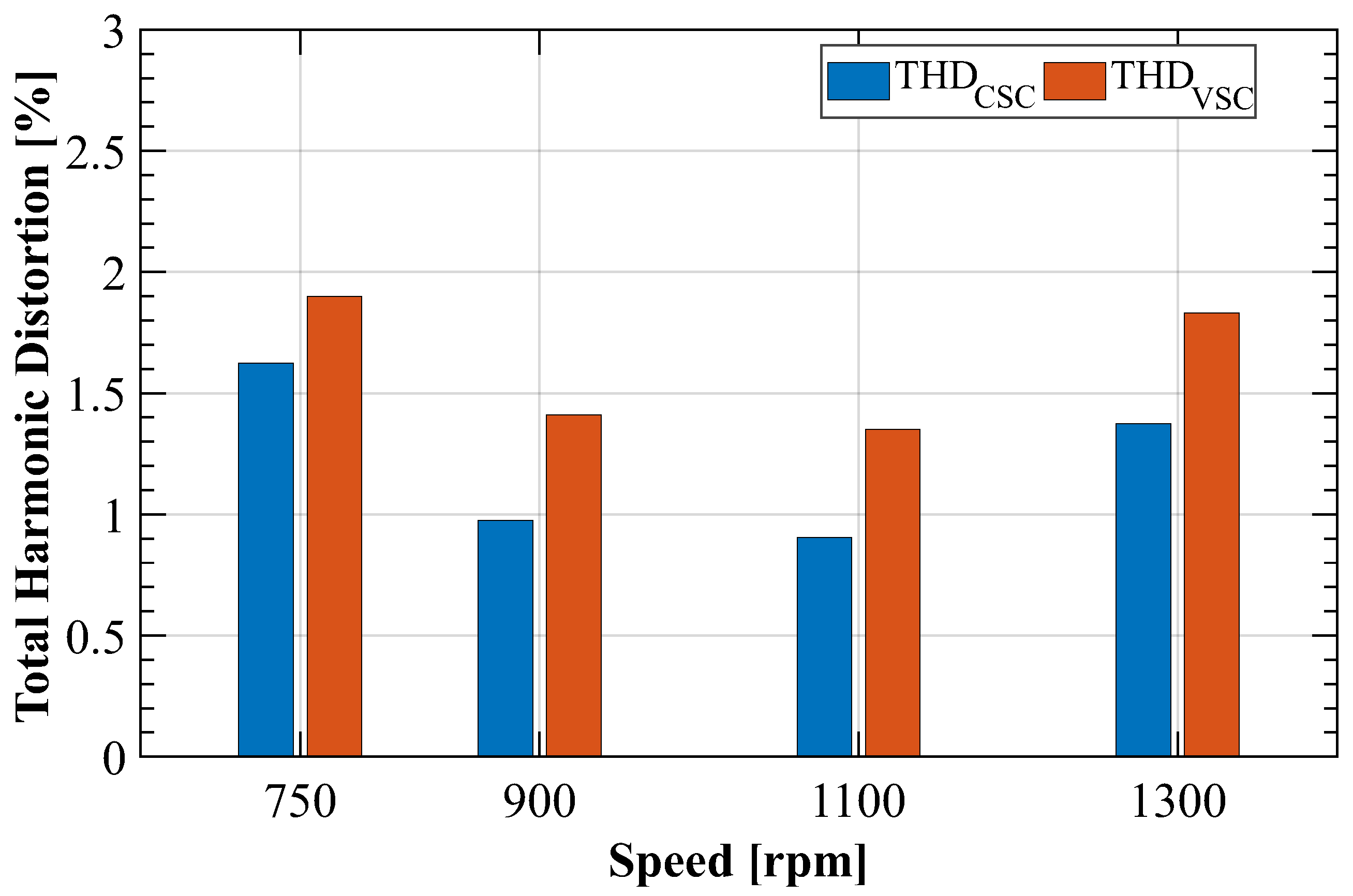
Figure 7 shows the THD values for the stator voltage under different rotor speeds and a load of 1.2 kW. In each case, the THD value is slightly lower in favor of the CSC and the average difference is approximately 0.4%. The THD values of the stator current under the same conditions are presented in Figure 8. The difference in favor of the current inverter is approximately 0.5% in the speed range up to synchronous; at 1300 rpm, the difference was 0.3%. Figure 9 presents the harmonic spectrum of stator voltage under different rotor speeds and a load of 1.2 kW.

4.3. Closed-Loop Performance

The closed-loop operation of the studied generator system in stand-alone mode was evaluated under four distinct scenarios:

• Rotor speed variation from 0.7 to 1.3 times the synchronous speed under a resistive load;
• A change in nonlinear load induced by the direct online starting of an induction motor;
• Normal (healthy) and faulty operation during a step change in the resistive load.

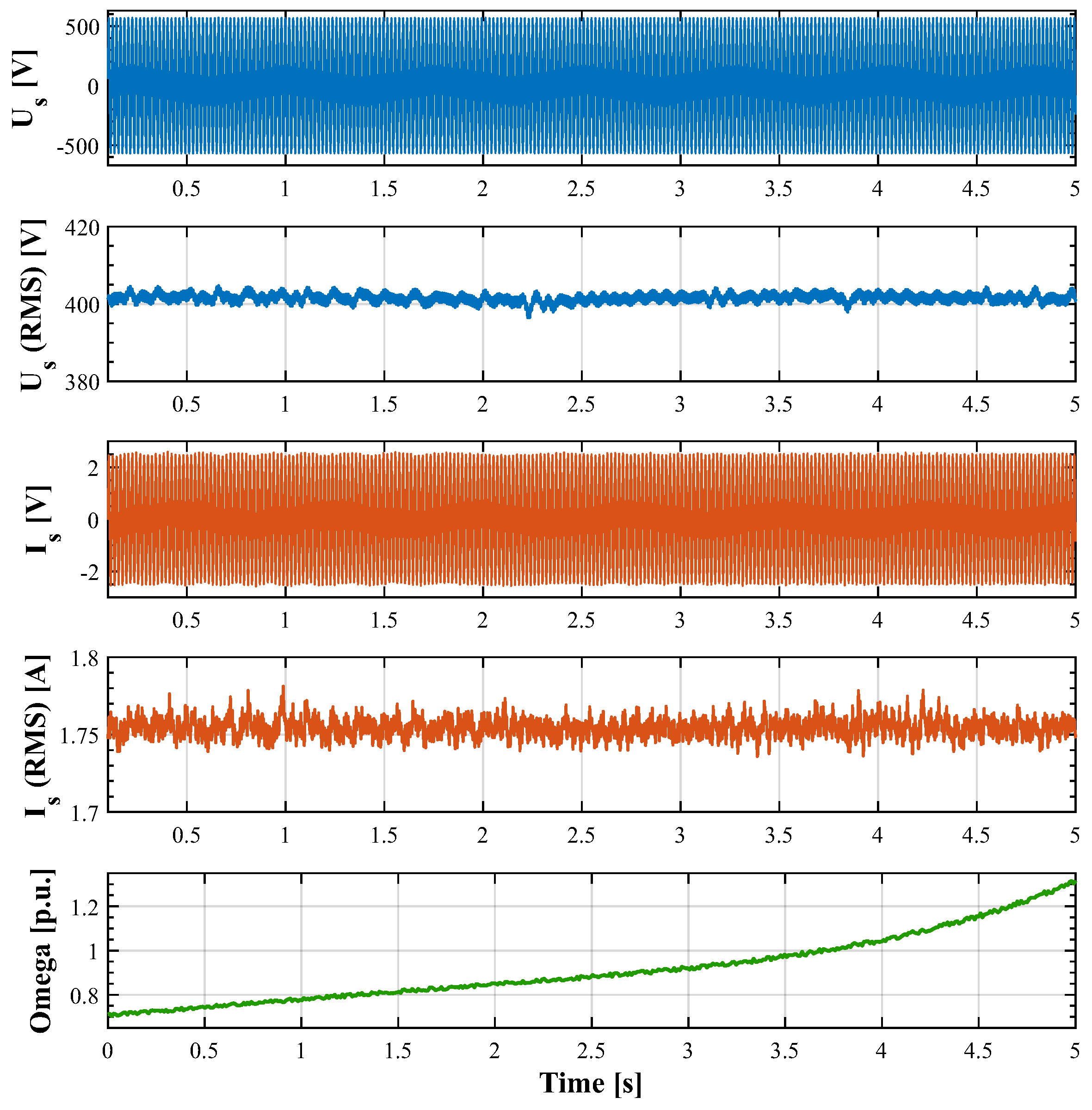
These scenarios simulate the typical operating conditions of a wind power plant, where DFIGs operate in sub-synchronous and super-synchronous modes due to fluctuating wind conditions. Figure 10 illustrates the instantaneous and RMS waveforms of the stator voltage and current, along with the generator rotor speed. The rotor speed is varied from 0.7 to 1.3 times the synchronous speed, with a 1.2 kW resistive load connected to the stator. The RMS stator voltage remains accurately regulated (<1.25% error) at the reference level (400 V), even as the rotor speed crosses synchronous speed.

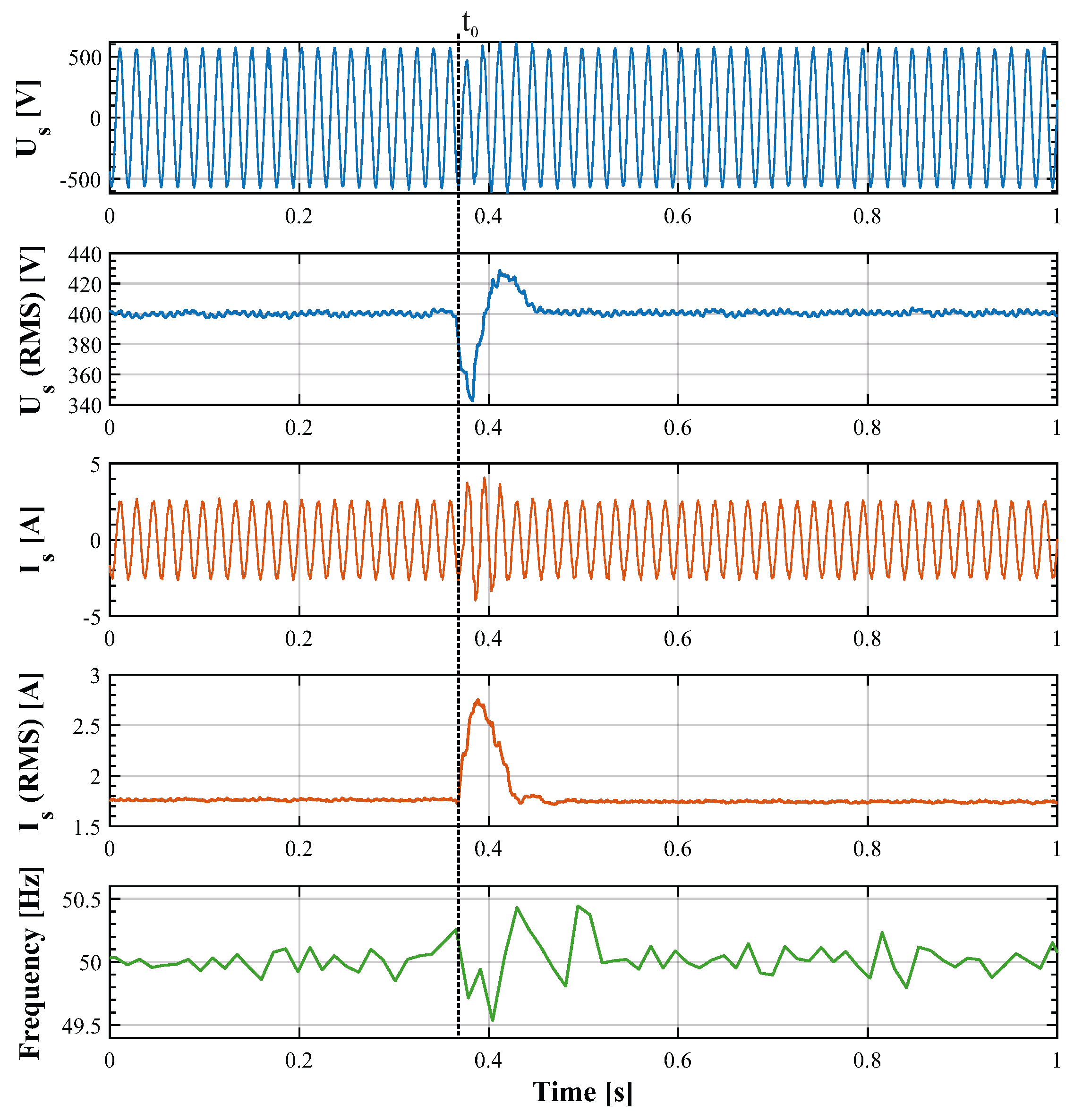
Subsequently, a nonlinear load was introduced to the microgrid through direct online startup of an induction motor. Figure 11 displays the corresponding RMS waveforms of the stator voltage and current, along with the frequency response during this transient event. The label $t_0$ indicates the moment of the startup. The regulation error of the stator voltage did not exceed 15%, and the maximum frequency error was approximately 1%. These values can be adjusted by tuning the parameters of the PI controller.

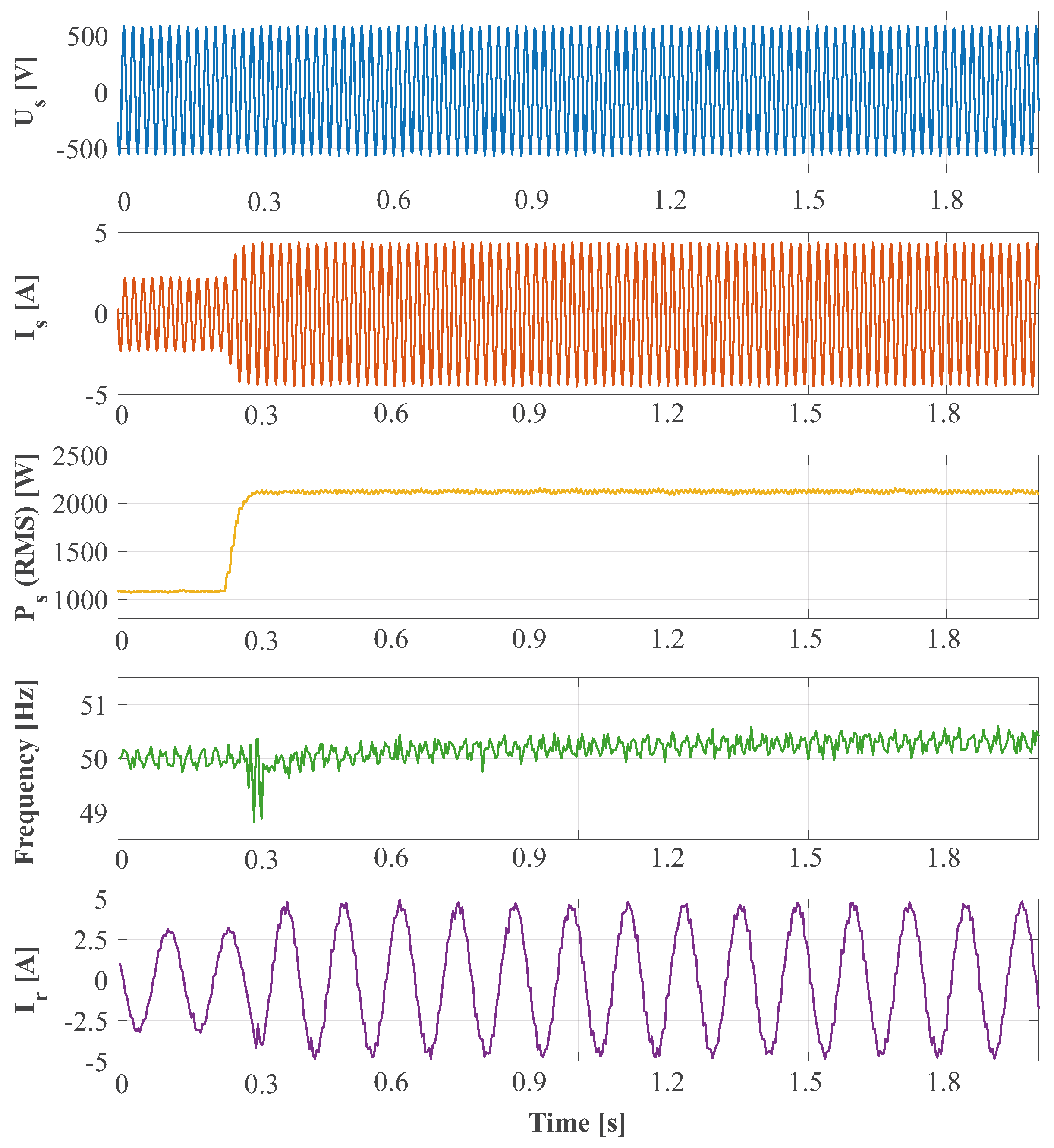
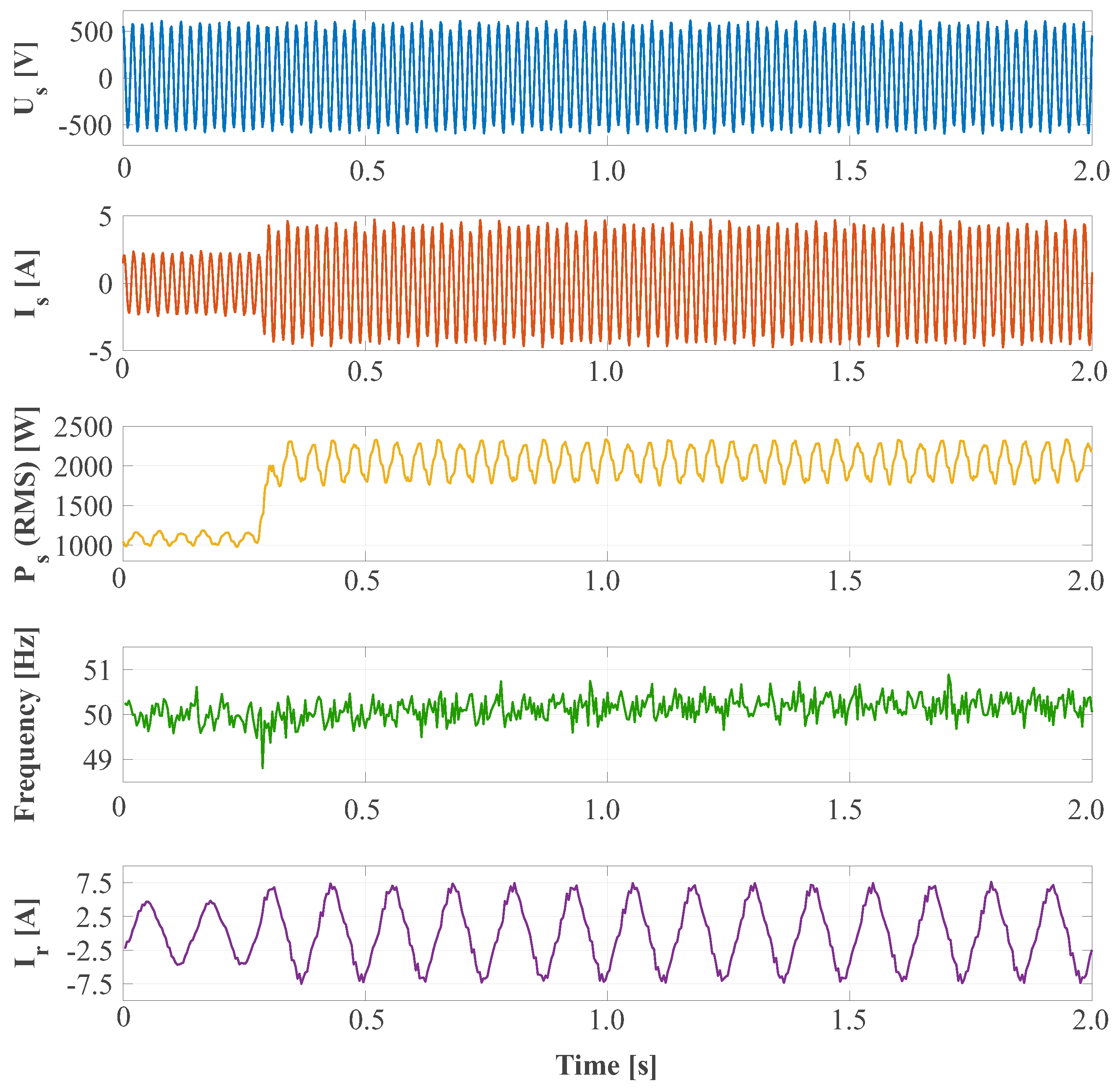
The final test involved a load change from 1.1 kW to 2.1 kW. This step change in the resistive load connected to the three-phase stator of the generator was conducted under two conditions:

• Normal (healthy) condition: All five phases of the DFIG rotor were supplied by the current-source converter (CSC) (Figure 12).
• Faulty condition: A single open-phase fault was introduced in phase A (Figure 13).

In healthy conditions, the average RMS stator power after the load step was 2120 W, with a standard deviation of 12.89 W. The frequency error remained within 1.2%, achieved with a rotor current amplitude of 5 A. Under the faulty condition, the multi-phase generator maintained operational capability despite the open-phase fault, though increased oscillations were observed in the stator quantities. The average RMS stator power after the load step decreased to 2054 W, with a higher standard deviation of 176 W. The frequency error increased to 1.8%, accompanied by a rise in the rotor current amplitude to 7.6 A. The increased rotor current to 7.6 A under fault conditions is a direct consequence of the open-phase fault, which reduces the number of active phases supplying the machine. In this situation, the closed-loop control system responds by increasing the inverter’s output current to maintain the required stator voltage magnitude, compensating for the lost phase [40].

5. Conclusions

This study demonstrates the potential of five-phase silicon carbide (SiC)-based current-source converters (CSCs) to enhance the performance of Doubly Fed Induction Generators (DFIGs) for wind energy systems. The experimental results validate the reliable operation of the system during transient states, with improved stator voltage and current quality, particularly in reducing THD, compared to conventional voltage-source converters.

The key contributions of this paper are as follows. The integration of a five-phase Doubly Fed Induction Generator (DFIG) with a five-phase Current Source Converter (CSC) is presented, leveraging the strengths of both technologies to enhance fault resilience and improve the quality of power output. This integrated DFIG/CSC system offers several advantages, including improved reliability and reduced maintenance requirements for five-phase generators, increased control resolution compared to conventional three-phase converters, and the generation of machine-friendly current and voltage waveforms at the output of the CSC. These features are particularly beneficial in offshore wind energy conversion systems.

In addition, the paper proposes a novel five-phase space vector PWM strategy that operates in dual coordinate planes. This strategy enables stable system performance under both healthy and faulty conditions, during step changes in the resistive load connected to the stator.

The work also includes an experimental evaluation of the five-phase CSC-DFIG system operating in a stand-alone configuration. The analysis encompasses both open-loop and closed-loop control scenarios, with various load conditions represented by step changes in resistive and nonlinear loads connected to the stator. The system is tested across a range of mechanical speeds from 0.7 to 1.3 times the synchronous speed, effectively replicating the dynamic behavior of real-world wind energy conversion systems. To the authors’ knowledge, such an experimental investigation has not been previously reported. Finally, the robustness of the system under fault conditions is examined, particularly during a single-phase open fault. The results show that despite increased oscillations in stator parameters, the generator maintains operational stability, underscoring its practical viability in challenging environments.

Future work will expand on this foundation, focusing on advanced fault-tolerant control strategies for the CSC to further enhance performance and reliability. The independent current generation in two $\alpha -\beta$ planes in a five-phase converter opens new possibilities for optimizing performance during fault conditions and for multi-generator systems fed by a single converter. The use of a current-source converter appears to be an interesting alternative, especially for long cable connections, as it eliminates the need for additional filters. In addition, the output current and voltage waveforms are “generator-friendly”, which contributes to decreased losses in the machine. As the field of multi-phase generation continues to evolve, increasing the number of rotor phases in DFIGs proves to be a path toward higher reliability and innovative machine design [16]. This research marks a step in that direction, showcasing the practical benefits and potential of five-phase CSC-driven systems in modern wind power applications.