Energy and Exergy Analysis of a Photovoltaic-Thermal Geothermal Heat Pump Coupled with Radiant Ceiling and Fresh Air System
Abstract
1. Introduction
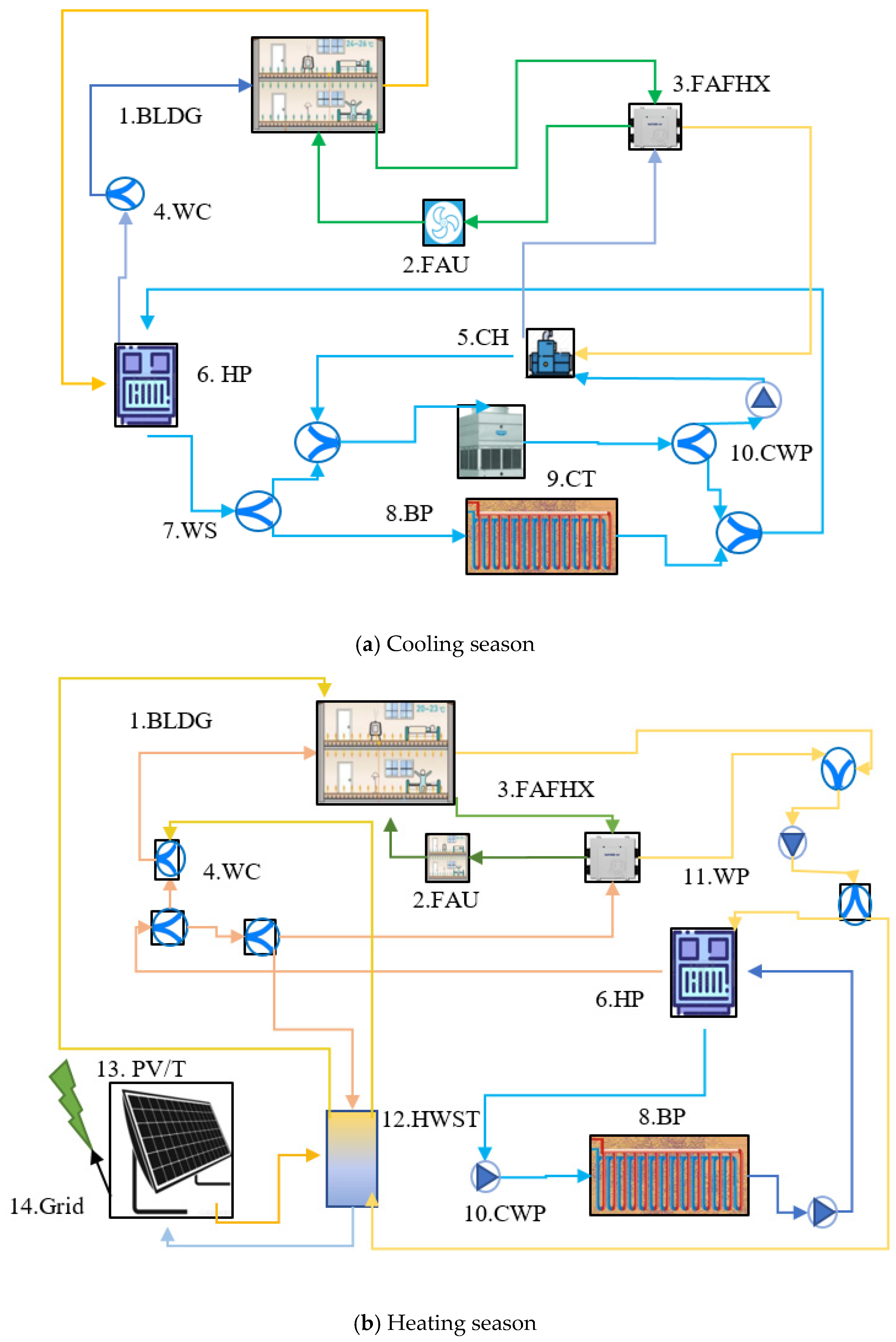
2. Description of the System
3. Mathematical Models
3.1. Exergy Model of the PV/T System
3.2. Exergy Model of Each Component During the Cooling Season
3.3. Exergy Model of Each Component During the Heating Season
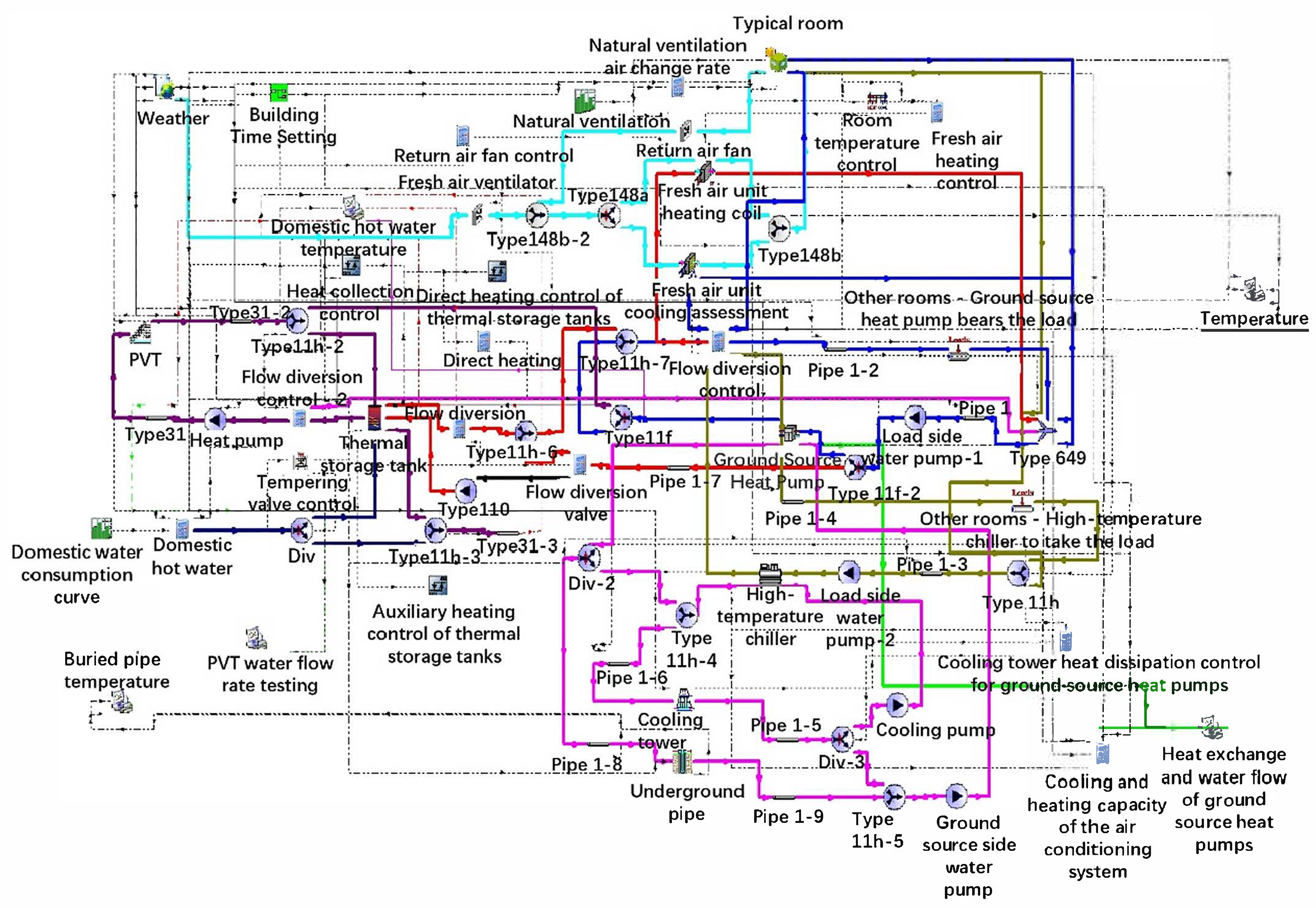
3.4. Mathematical Model Under TRNSYS Environment
4. Model Validation
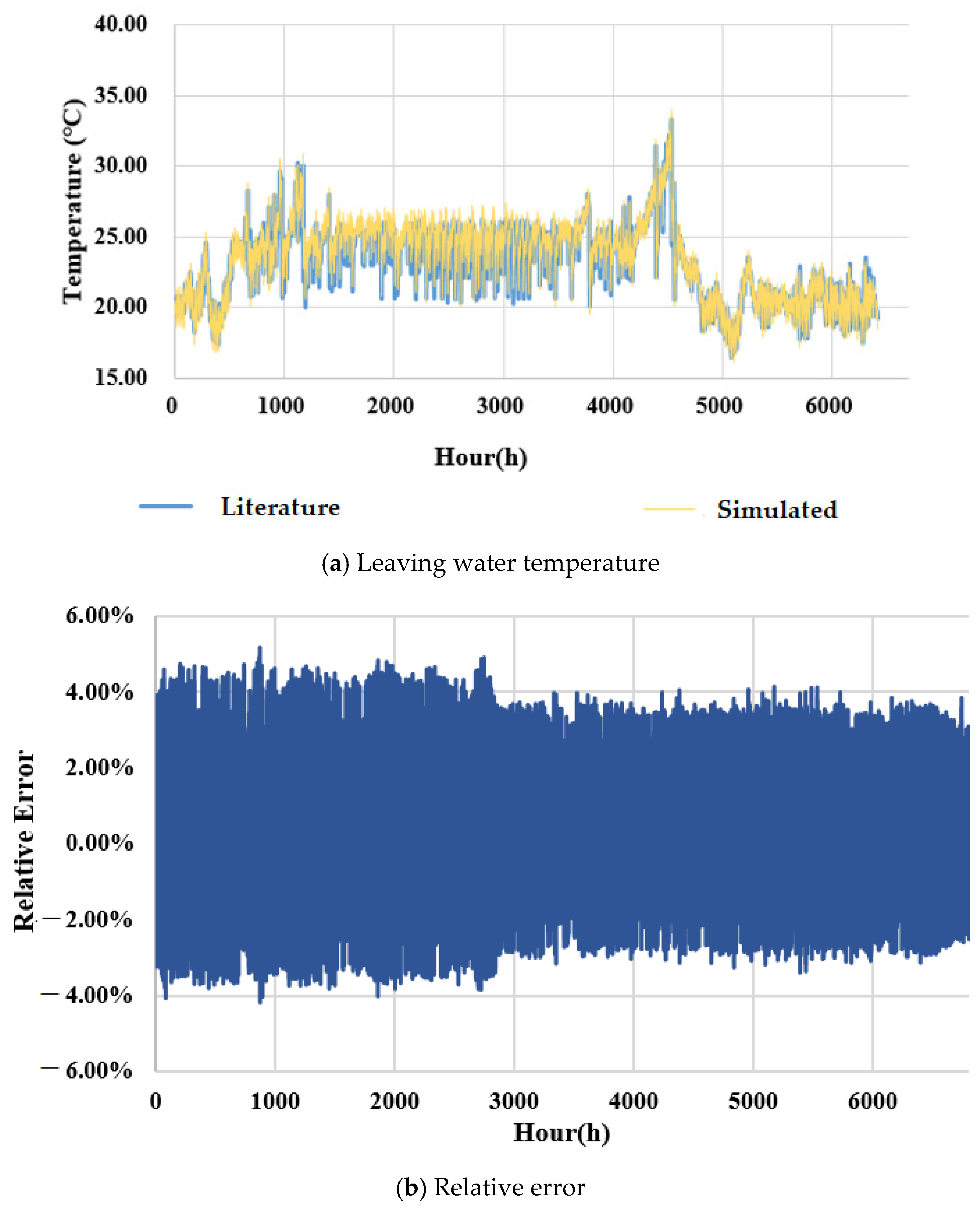
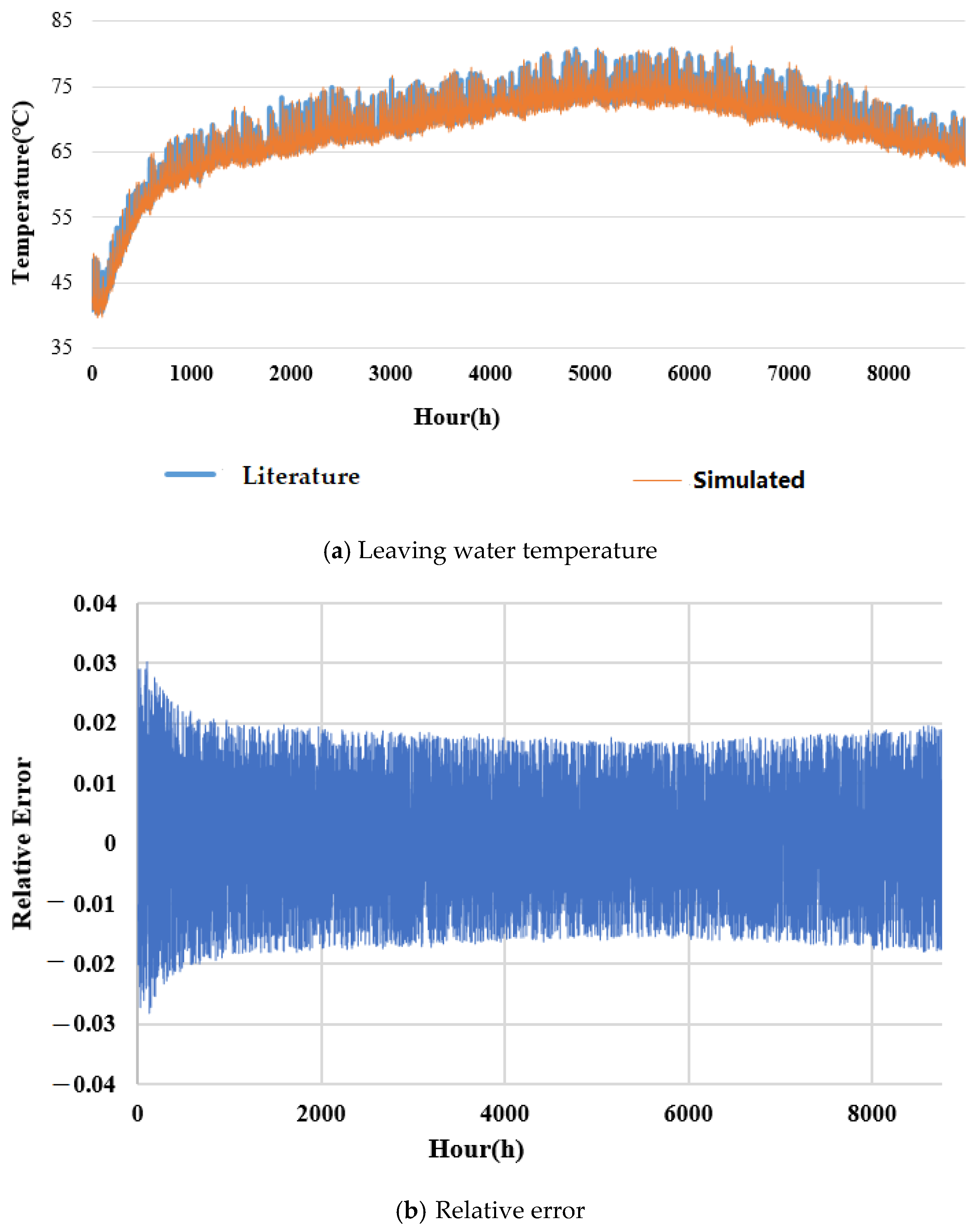
4.1. Validation on the Ground Source Heat Pump and Radiant System
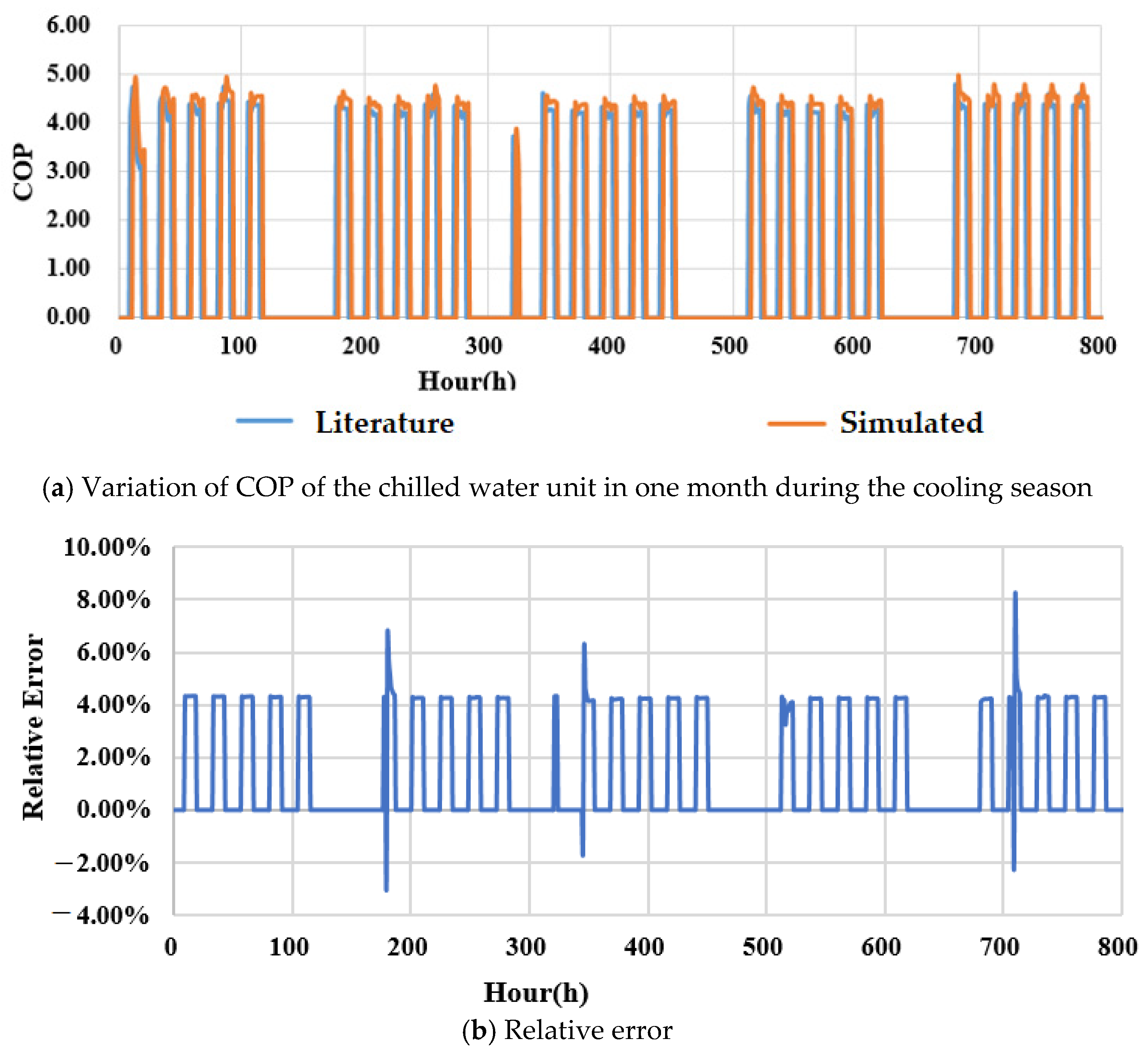
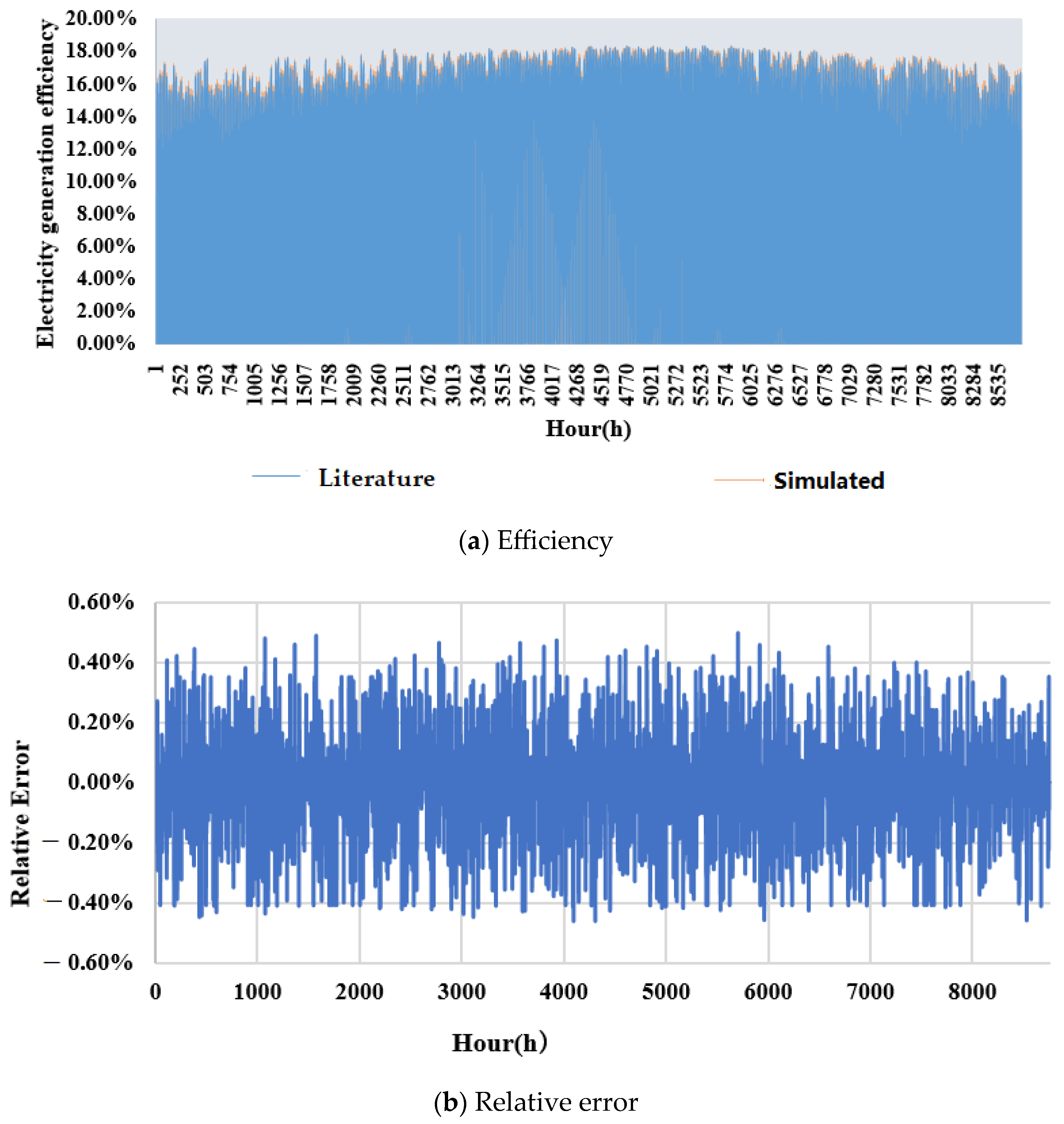
4.2. Validation on the PV/T System
5. Results and Discussion
5.1. Exergy Performance of the System and Each Component
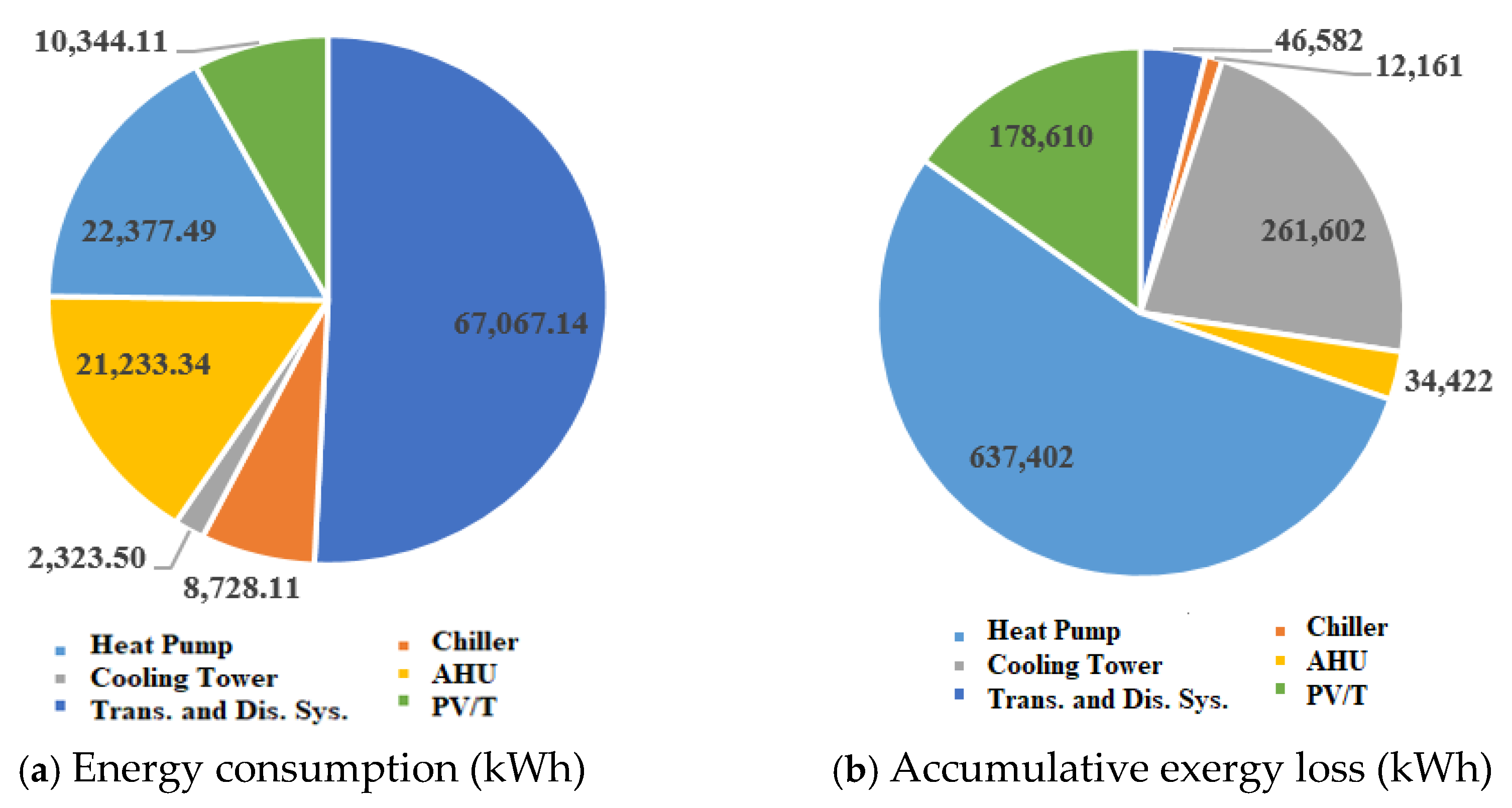
5.2. Energy Consumption of the System and Each Component
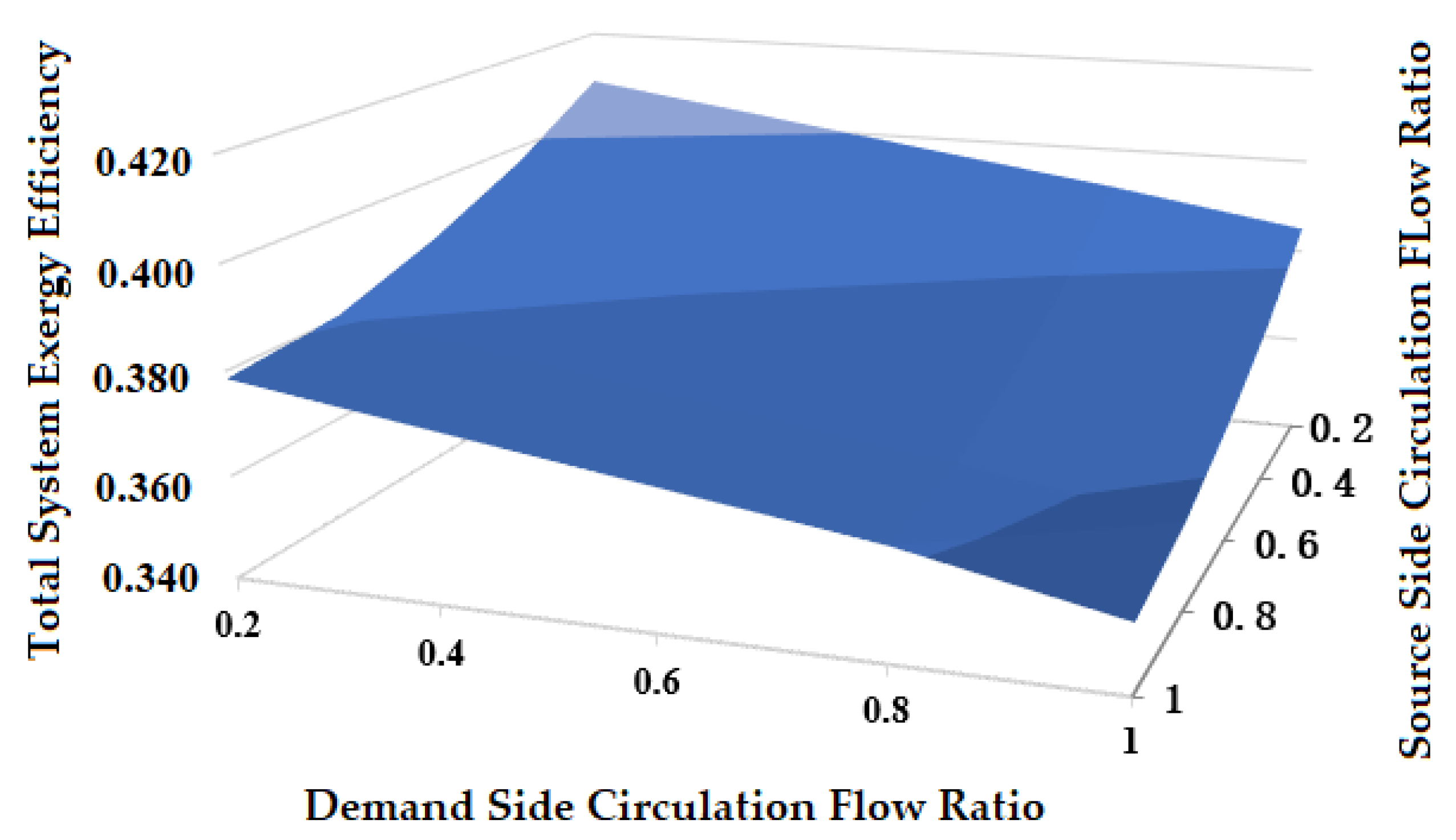
5.3. Optimization on the Exergy Performance of the System
5.3.1. Optimization on the Temperature Difference Between the Supply and Chilled Water Temperature
5.3.2. Optimization on the Bearing Load Ratio of Ground Source Heat Pump vs. PV/T System in Heating Season
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Area of the radiant ceiling, m2 | |
| Inlet enthalpy of the compressor, kW | |
| Outlet enthalpy of the compressor, kW | |
| Inlet enthalpy of the condenser, kW | |
| Outlet enthalpy of the condenser, kW | |
| Inlet enthalpy of the radiant ceiling system, kW | |
| Outlet enthalpy of the radiant ceiling system, kW | |
| Outlet enthalpy of the buried pipe system, kW | |
| Inlet enthalpy of the buried pipe system, kW | |
| Exergy loss of the radiant ceiling system, kW | |
| Exergy loss of the compressor, kW | |
| Exergy loss of the buried pipe, kW | |
| Exergy loss of the condenser, kW | |
| Exergy loss of the evaporator, kW | |
| Exergy loss of the overall system, kW | |
| Radiation heat transfer factor, taken as 0.87 | |
| PV/T panel heat transfer medium flow rate, kg/s | |
| Ground source heat pump load side heat transfer medium flow rate, kg/s | |
| Ground source heat pump source side water flow rate, kg/s | |
| Mass flow rate of the refrigerant, kg/s | |
| Heating water flow rate of the fresh air unit, kg/s | |
| Cooling water flow rate of the fresh air unit, kg/s | |
| Water flow rate at the radiant ceiling terminal, kg/s | |
| Water flow rate of the heat exchanger at the source side, kg/s | |
| Mass flow rate from the hot water storage tank, kg/s | |
| Heat gain/loss from/to the ground, kW | |
| Heat exchange rate between the cooling water and the outside air, kJ | |
| Convective heat transfer between the ceiling and the indoor air, kW | |
| Heating load, kW | |
| Cooling load, kW | |
| Radiant heat transfer between the ceiling and other surfaces, kW | |
| Load side entering water temperature of the heating pump system, ºC | |
| Load side leaving water temperature of the heating pump system, ºC | |
| Source side entering water temperature of the heating pump system, ºC | |
| Source side leaving water temperature of the heating pump system, ºC | |
| Entering cooling water temperature, K | |
| Leaving cooling water temperature, K | |
| Average cooling water temperature inside the cooling tower, K | |
| Load shared by unit i, kW | |
| Indoor air temperature, K | |
| Ambient air temperature, K | |
| Radiant ceiling surface temperature, K | |
| Leaving water temperature of the radiant system, K | |
| Surface temperature, excluding the radiant ceiling surface, K | |
| Soil temperature, K | |
| Entering water temperature of the radiant system, K | |
| Entering water temperature to the fresh air unit, K | |
| Leaving water temperature from the fresh air unit, K | |
| Input power to the fresh air unit, kW | |
| Input power to PV/T collector, kW | |
| Input power to the cooling tower, kW | |
| Input power to the heat pump unit, kW | |
| Real time power input to heat pump unit i, kW | |
| Input power to the chilled water pump, kW | |
| Input power to the cooling water pump, kW | |
| Stefan–Boltzmann constant, equal to 5.67 × 10−8 W/(m2∙K4) |
References
- Lin, Y.; Bu, Z.; Yang, W.; Zhang, H.; Francis, V.; Li, C. A Review on the Research and Development of Solar-Assisted Heat Pump for Buildings in China. Buildings 2022, 12, 1435. [Google Scholar] [CrossRef]
- Lamnatou, C.; Chemisana, D.; Cristofari, C. Smart grids and smart technologies in relation to photovoltaics, storage systems, buildings and the environment. Renew. Energy 2022, 185, 1376–1391. [Google Scholar] [CrossRef]
- Qi, Z.; Gao, Q.; Liu, Y.; Yan, Y.Y.; Spitler, J.D. Status and development of hybrid energy systems from hybrid ground source heat pump in China and other countries. Renew. Sustain. Energy Rev. 2014, 29, 37–51. [Google Scholar] [CrossRef]
- Penrod, E.B.; Prasanna, K.V. Design of a flat-plate collector for a solar earth heat pump. Sol. Energy 1962, 6, 9–22. [Google Scholar] [CrossRef]
- Liu, L.; Zhu, N.; Zhao, J. Thermal equilibrium research of solar seasonal storage system coupling with ground-source heat pump. Energy 2016, 99, 83–90. [Google Scholar] [CrossRef]
- Girard, A.; Gago, E.J.; Muneer, T.; Caceres, G. Higher ground source heat pump COP in a residential building through the use of solar thermal collectors. Renew. Energy 2015, 80, 26–39. [Google Scholar] [CrossRef]
- Zhu, N.; Wang, J.; Liu, L. Performance evaluation before and after solar seasonal storage coupled with ground source heat pump. Energy Convers. Manag. 2015, 103, 924–933. [Google Scholar] [CrossRef]
- Kaygusuz, K. Experimental and theoretical investigation of a solar heating system with heat pump. Renew. Energy 2000, 21, 79–102. [Google Scholar] [CrossRef]
- Bakirci, K.; Ozyurt, O.; Comakli, K.; Comakli, O. Energy analysis of a solar-ground source heat pump system with vertical closed-loop for heating applications. Energy 2011, 36, 3224–3232. [Google Scholar] [CrossRef]
- Al-Juwayhel, F.I. Simulation of Dynamic Performance of Air-Source, Earth-Source and Solar Assisted Earth-Source Heat Pump System. Ph.D. Thesis, Oklahoma State University, Stillwater, OK, USA, 1981. [Google Scholar]
- Coskun, S. Performance analysis of a solar-assisted ground source heat pump system in climatic conditions of Turkey. Therm. Sci. 2020, 24, 977–989. [Google Scholar] [CrossRef]
- Biglarian, H.; Saidi, M.H.; Abbaspour, M. Economic and environmental assessment of a solar-assisted ground source heat pump system in a heating-dominated climate. Int. J. Environ. Sci. Technol. 2019, 16, 3091–3098. [Google Scholar] [CrossRef]
- Berardi, U.; Heidarinejad, G.; Rayegan, S.; Pasdarshahri, H. Enhancing the cooling potential of a solar-assisted desiccant cooling system by ground source free cooling. Build. Simul. 2020, 13, 1125–1144. [Google Scholar] [CrossRef]
- Zhang, C.; Nielsen, E.; Fan, J.; Furbo, S.; Li, Q. Experimental investigation on a combined solar and ground source heat pump system for a single-family house: Energy flow analysis and performance assessment. Energy Build. 2021, 241, 110–958. [Google Scholar] [CrossRef]
- Maranghi, F.; Gosselin, L.; Raymond, J.; Bourbonnais, M. Modeling of solar-assisted ground-coupled heat pumps with or without batteries in remote high north communities. Renew. Energy 2023, 207, 484–498. [Google Scholar] [CrossRef]
- Ma, L. Energy Efficiency Experiment and Simulation Analysis of Ground Source Heat Pump and Capillary Radiation Terminal Composite System. Master’s Thesis, Huazhong University of Science and Technology, Wuhan, China, 2017. [Google Scholar]
- Banjac, M. Achieving sustainable work of the heat pump with the support of an underground water tank and solar collectors. Energy Build. 2015, 98, 19–26. [Google Scholar] [CrossRef]
- Cui, M.; Liu, J.; Kim, M.K.; Wu, X. Application potential analysis of different control strategies for radiant floor cooling systems in office buildings in different climate zones of China. Energy Build. 2023, 282, 112772. [Google Scholar] [CrossRef]
- Ramdani, H.; Ould-Lahoucine, C. Study on the overall energy and exergy performances of a novel water-based hybrid photovoltaic-thermal solar collector. Energy Convers. Manag. 2020, 222, 113–138. [Google Scholar] [CrossRef]
- Arslan, E.; Aktaş, M.; Can, Ö.F. Experimental and numerical investigation of a novel photovoltaic thermal (PV/T) collector with the energy and exergy analysis. J. Clean. Prod. 2020, 276, 123–155. [Google Scholar] [CrossRef]
- Suleman, F.; Dincer, I.; Agelin-Chaab, M. Energy and exergy analyses of an integrated solar heat pump system. Appl. Therm. Eng. 2014, 73, 559–566. [Google Scholar] [CrossRef]
- Ozcan, H.G.; Varga, S.; Gunerhan, H.; Hepbasli, A. Numerical and experimental work to assess dynamic advanced exergy performance of an on-grid solar photovoltaic-air source heat pump-battery system. Energy Convers. Manag. 2021, 227, 113605. [Google Scholar] [CrossRef]
- Li, S.; Yang, H.; Yuan, J.; Zeng, X.; Yang, Z.; Lu, J. Thermodynamic and economic analyses of modified ejector enhanced solar-air composite dual-source heat pump system in residential buildings. Energy Build. 2024, 308, 113978. [Google Scholar] [CrossRef]
- Gao, J.; Zhao, Y.; Wu, F.; Adnouni, M.; Sun, Y.; Li, S.; Yu, Z.; Zhang, X. Experimental investigation on efficient heating method of solar composite heat pump based on evaporative thermal accumulator. Energy 2025, 317, 134627. [Google Scholar] [CrossRef]
- Petrucci, G.A.; Chenche, L.E.P.; Gomez, A.O.C.; Loayza, D.M.J.; Flores, J.M.B.; Pabon, J.J.G. Energy analysis of a direct expansion heat pump assisted by a thermal photovoltaic panel for hot water production in several regions of Brazil. Energy Convers. Manag. 2024, 319, 118969. [Google Scholar] [CrossRef]
- Yousaf, S.; Bradshaw, C.R.; Kamalapurkar, R.; San, O. A gray-box model for unitary air conditioners developed with symbolic regression. Int. J. Refrig. 2024, 168, 696–707. [Google Scholar] [CrossRef]
- Ma, X.; Du, Y.; Li, B.; Zhang, C.; Wu, Y. Enhancing the performance of autocascade steam generating heat pumps through advanced exergy methods. Energy Convers. Manag. 2025, 332, 119705. [Google Scholar] [CrossRef]
- Gao, Y.; Yang, W.; Zhang, Y. Thermodynamic analysis of a new tandem dual-temperature air source heat pump with ejector. Int. J. Refrig. 2024, 168, 307–317. [Google Scholar] [CrossRef]
- EnergyPlus. Weather Data. Available online: https://energyplus.net/weather (accessed on 14 May 2025).
- JGJ/T 76-2019; Ministry of Housing and Urban-Rural Development of the People’s Republic of China (MOHURD). Standards for Design of Office Building. China Architecture & Building Press: Beijing, China, 2019.
- GB50495—2019; Ministry of Housing and Urban-Rural Development. Technical Specification for Solar Heating Engineering. China Architecture & Building Press: Beijing, China, 2019.
- Deng, Y.; Ding, L.; Luo, Y. Exergy analysis on a new style cold outdoor air processing system of water loop heat pump. World SCI-TECH RD 2009, 31, 655–657. Available online: https://kns.cnki.net/kcms2/article/abstract?v=XMmmt_ij6VEIqgrxWpuCNxEqx1VPl4zUbTBNxveMQa7I6lcrcbPyRy4uz8vWHhcNzkwGoQ80fU--U6ucB61d85o7UviTMicl6TUWpx8iD5IgqRrb7HFz7QHexmouSTSzGEmUFBUtbfEGSFzMVYCEPEI4gZUxUXEoPfkgUIypGpSXXGfHy9h8pg==&uniplatform=NZKPT&language=CHS (accessed on 19 May 2025).
- Wang, L. Exergy Analysis and Experimental Study of Solar Water Source Heat Pump Hybrid System. Master’s Thesis, Hunan University, Changsha, China, 2012. [Google Scholar]
- Gong, G.; Wang, L.; Su, H. Analysis and Experimental Study on Solar Water Source Heat Pump Composite System. Acta Energiae Solaris Sin. 2013, 6, 1051–1056. [Google Scholar]
- Yuan, C. Dual-Condition Exergy Analysis of Ground Source Heat Pump System. Master’s Thesis, Xi’an University of Architecture and Technology, Xi’an, China, 2013. [Google Scholar]
- Chen, D. Research on Operating Characteristics of Cooling Tower Assisted Composite Ground Source Heat Pump System. Master’s Thesis, Yangzhou University, Yangzhou, China, 2016. [Google Scholar]
- Liu, S. Research on Operation Control Optimization of Ground Source Heat Pump-Radiant Ceiling Air Conditioning System. Master’s Thesis, Xi’an University of Architecture and Technology, Xi’an, China, 2019. [Google Scholar]
- American Society of Heating, Refrigerating and Air-Conditioning Engineers, Inc. ASHRAE Handbook. HVAC System and Equipment; ASHRAE: Atlanda, GA, USA, 2024. [Google Scholar]
- Hu, W. Energy-Saving Analysis and Management of Ground Source Heat Pump Air Conditioning System. Master’s Thesis, Hunan University, Changsha, China, 2019. [Google Scholar]
- Lin, Y.; Feng, H.; Yang, W.; Hao, X.; Tian, L.; Yuan, X. Thermal performance optimization of a semi-nested building coupled with an earth-to-air heat exchanger using iterative Taguchi method. Renew. Energy 2022, 195, 1275–1290. [Google Scholar] [CrossRef]
- Jordan, D.C.; Kurtz, S.R.; VanSant, K.; Newmiller, J. Compendium of photovoltaic degradation rates. Prog. Photovolt. Res. Appl. 2016, 24, 978–989. [Google Scholar] [CrossRef]
- Khanmohammdi, S.; Musharavati, F.; Sheykhmohammadi, M. Energy and exergy examinations of PV/T based hybrid system for power, heating and potable water production: Transient modeling. Renew. Energy 2022, 195, 540–553. [Google Scholar] [CrossRef]






| Item | Module | Quantity | Parameter | Value |
|---|---|---|---|---|
| 1 | PV/T collector | 1 | Average heating capacity (kW) | 60.93 |
| Average thermal efficiency (%) | 40 | |||
| Heat loss from pipelines and heat storage devices (%) | 15 | |||
| Area compensation coefficient | 0.98 | |||
| Area (m2) | 276.29 | |||
| 2 | Monocrystalline silicon plate | 1 | Power generation efficiency % | 0.21 |
| Component efficiency % | 16.6 | |||
| 3 | Thermal storage/heat collection tank | 1 | Volume (m3) | 27.63 |
| heat loss coefficient of the hot Water tank | 0.4 | |||
| 4 | Heat collector pump | 2 | Flow rate (m3/h) | 16.58 |
| Input power (kW) | 1.29 | |||
| 5 | Source side circulation pump | 1 | Flow rate (m3/h) | 68.75 |
| Input power (kW) | 6.69 | |||
| 6 | Cooling water pump of the Chiller | 1 | Flow rate (m3/h) | 23.21 |
| Input power (kW) | 2.26 | |||
| 7 | Chilled water pump | 1 | Flow rate (m3/h) | 20.62 |
| Input power (kW) | 2.57 | |||
| 8 | Chilled water pump of the Chiller | 2 | Flow rate (m3/h) | 58.42 |
| Input power (kW) | 7.28 | |||
| 9 | Heat pump | 1 | Heating capacity (kW) | 374 |
| Cooling capacity (kW) | 540 | |||
| Heating COP | 4.85 | |||
| 10 | Chiller | 1 | Cooling COP | 5.66 |
| Cooling capacity (kW) | 120 | |||
| 11 | Cooling tower | 1 | Cooling water flow rate (m3/h) | 55 |
| Fan flow rate (m3/h) | 33000 | |||
| Input power (kW) | 1.5 | |||
| 12 | Fresh air unit | 12 | Flow rate (m3/h) | 660 |
| Input power (kW) | 0.18 |
| Radiant System Parameter | Value | Buried Pipe Parameter | Value |
|---|---|---|---|
| Tube spacing (mm) | 10 | Thermal conductivity of backfill material (W/(m·K)) | 2.1 |
| Tube inner diameter (mm) | 26 | Drilling diameter (mm) | 110 |
| Tube outer diameter (mm) | 32 | Drilling spacing (m) | 0.06 |
| Air layer thickness (mm) | 5 | Hole depth (m) | 60 |
| Return water temperature in summer (°C) | 19 | Borehole thermal resistance (°C/W) | 0.099 |
| Return water temperature in winter (°C) | 30 | Thermal conductivity of the U-shaped tube (W/(m·k)) | 30 |
| Location | Wuhan | Pipe type | Single U pipe |
| Mode | Source | Average Source Side Supply Water Temperature (°C) | Average Source Side Return Water Temperature (°C) | Average Supply Water Temperature of Radiant System (°C) | Average Return Water Temperature of Radiant System (°C) | Maximum Cooling Load (kW) | Maximum Heating Load (kW) |
|---|---|---|---|---|---|---|---|
| Cooling | From [16] | 30.1 | 26.8 | 14.8 | 21.5 | 15.68 | - |
| Simulated | 29.8 | 26.7 | 14.2 | 22.2 | 16.56 | - | |
| Heating | From [16] | 12.5 | 14.6 | 33.7 | 30.1 | - | 16.89 |
| Simulated | 12.8 | 15.1 | 34.1 | 29.7 | - | 17.21 |
| Parameter | Value |
|---|---|
| Collector incident angle (°) | 28 |
| Collector tilted angle (°) | 40 |
| Dimension (m × m) | 1.2 × 0.65 |
| Dimension of the PV cell (mm × mm) | 125 × 0.65 |
| Total heat collection area (m2) | 985 |
| Back flow channel/pipe diameter | 10/0.01 m |
| Collector inlet mass flow rate | 36 kg/h |
| Season | Component | Average Hourly Exergy Loss (kWh) | Accumulative Exergy Loss (kWh) | Exergy Loss Rate (−) | Average Exergy Efficiency (%) |
|---|---|---|---|---|---|
| Cooling season | PV/T system | 25.95 | 103,822.42 | 0.035 | 65.8 |
| Hot water storage tank | 4.8 | 19,193.31 | 0.006 | 32.2 | |
| Compressor | 18.61 | 74,561.97 | 0.025 | 75.1 | |
| Evaporator | 12.93 | 51,717.14 | 0.017 | 78.2 | |
| Condenser | 2.76 | 4753.06 | 0.004 | 36.3 | |
| Transmission and distribution system | 252.36 | 415,156.6 | 0.338 | 42.3 | |
| Radiant system | 319.32 | 501,973.51 | 0.428 | 28.5 | |
| Fresh air unit | 16.19 | 31,543 | 0.022 | 43.3 | |
| Chiller | 35.3 | 60,806.89 | 0.047 | 48.9 | |
| Buried pipe | 3.23 | 2810.81 | 0.004 | 49.2 | |
| Cooling tower | 55.24 | 221,052.75 | 0.074 | 36.3 | |
| Total | 746.69 | 1,487,391.46 | 1 | 34.5 | |
| Heating season | PV/T system | 67.99 | 74,788.28 | 0.091 | 67.2 |
| Hot water storage tank | 17.83 | 71,338.54 | 0.087 | 53.6 | |
| Compressor | 16.82 | 44,593.15 | 0.054 | 83.2 | |
| Evaporator | 18.68 | 49,548.05 | 0.060 | 32.3 | |
| Condenser | 15 | 63,520.79 | 0.077 | 81.2 | |
| Transmission and distribution system | 221.36 | 197,126.5 | 0.240 | 36.5 | |
| Radiant system | 299.07 | 284,415.17 | 0.347 | 32.3 | |
| Fresh air unit | 3.27 | 2878.80 | 0.04 | 46.6 | |
| Chiller | - | - | - | - | |
| Buried pipe | 3.16 | 4933.03 | 0.006 | 47.6 | |
| Cooling tower | 4.81 | 7621.10 | 0.034 | 9.2 | |
| Total | 667.99 | 800,763.41 | 1.000 | 36.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, Y.; Bu, Z.; Yang, W.; Chan, M.; Tian, L.; Dai, M. Energy and Exergy Analysis of a Photovoltaic-Thermal Geothermal Heat Pump Coupled with Radiant Ceiling and Fresh Air System. Energies 2025, 18, 2715. https://doi.org/10.3390/en18112715
Lin Y, Bu Z, Yang W, Chan M, Tian L, Dai M. Energy and Exergy Analysis of a Photovoltaic-Thermal Geothermal Heat Pump Coupled with Radiant Ceiling and Fresh Air System. Energies. 2025; 18(11):2715. https://doi.org/10.3390/en18112715
Chicago/Turabian StyleLin, Yaolin, Zhenyan Bu, Wei Yang, Melissa Chan, Lin Tian, and Mingqi Dai. 2025. "Energy and Exergy Analysis of a Photovoltaic-Thermal Geothermal Heat Pump Coupled with Radiant Ceiling and Fresh Air System" Energies 18, no. 11: 2715. https://doi.org/10.3390/en18112715
APA StyleLin, Y., Bu, Z., Yang, W., Chan, M., Tian, L., & Dai, M. (2025). Energy and Exergy Analysis of a Photovoltaic-Thermal Geothermal Heat Pump Coupled with Radiant Ceiling and Fresh Air System. Energies, 18(11), 2715. https://doi.org/10.3390/en18112715








