Elimination Methods for High-Frequency Harmonics on the DC Side of Modular Multilevel Converters from the Perspective of Valve Control
Abstract
1. Introduction
2. Mechanism Analysis of DC Voltage High-Frequency Harmonics Caused by Discrete Carrier Quantization Error
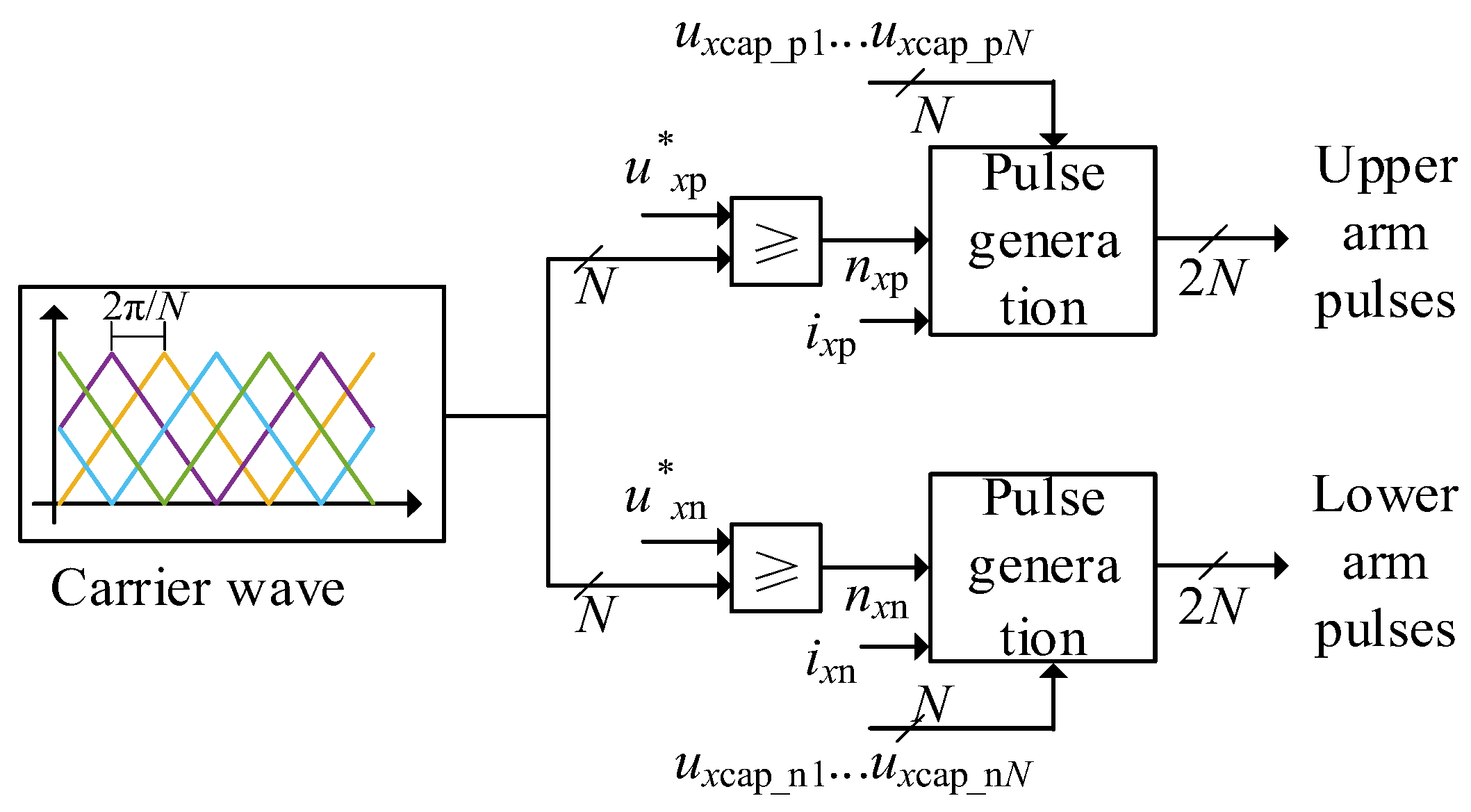
2.1. Principle of CPS Modulation and Ideal Waveforms
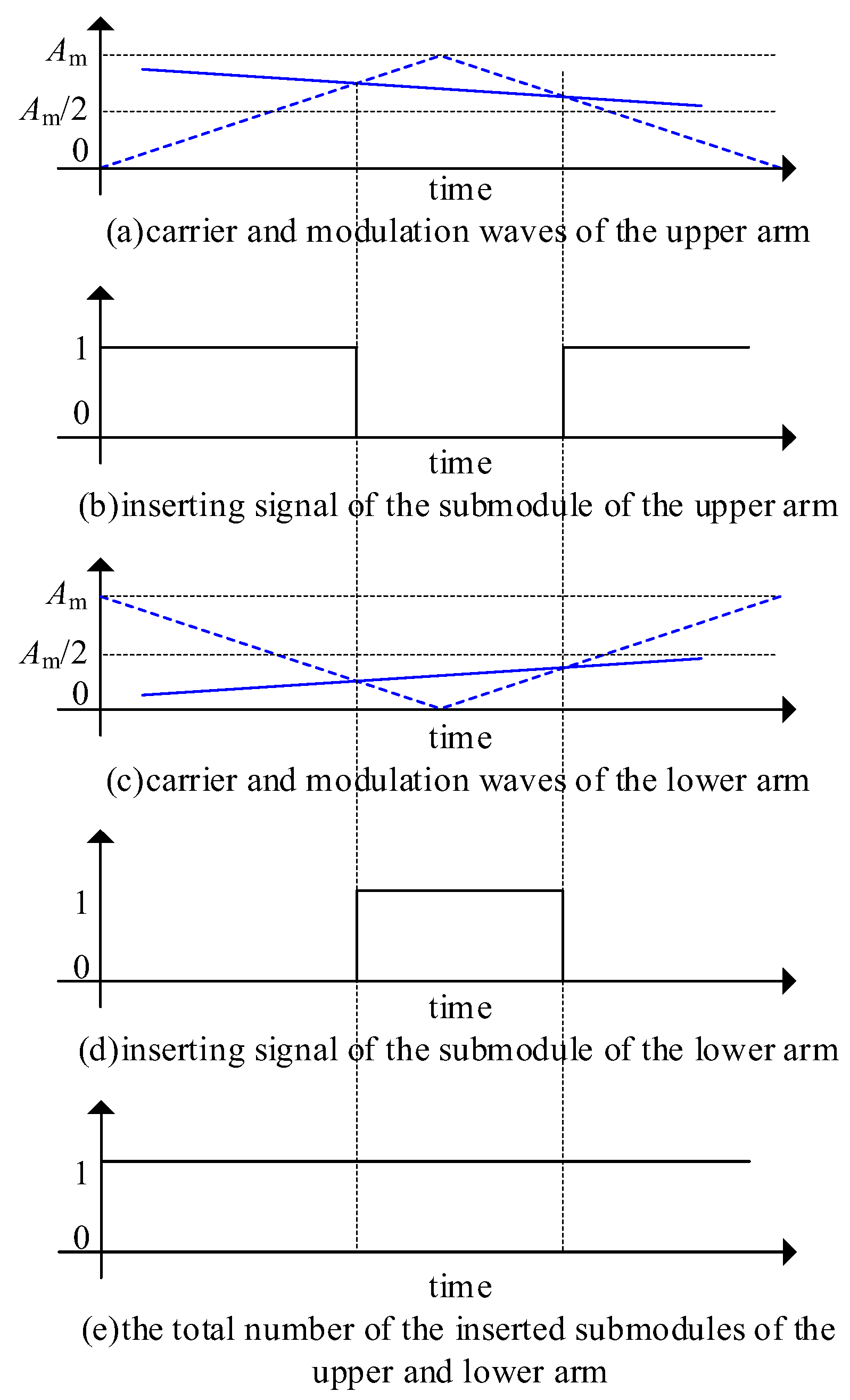
2.2. High-Frequency Harmonics Caused by Discretization of Triangular Carriers
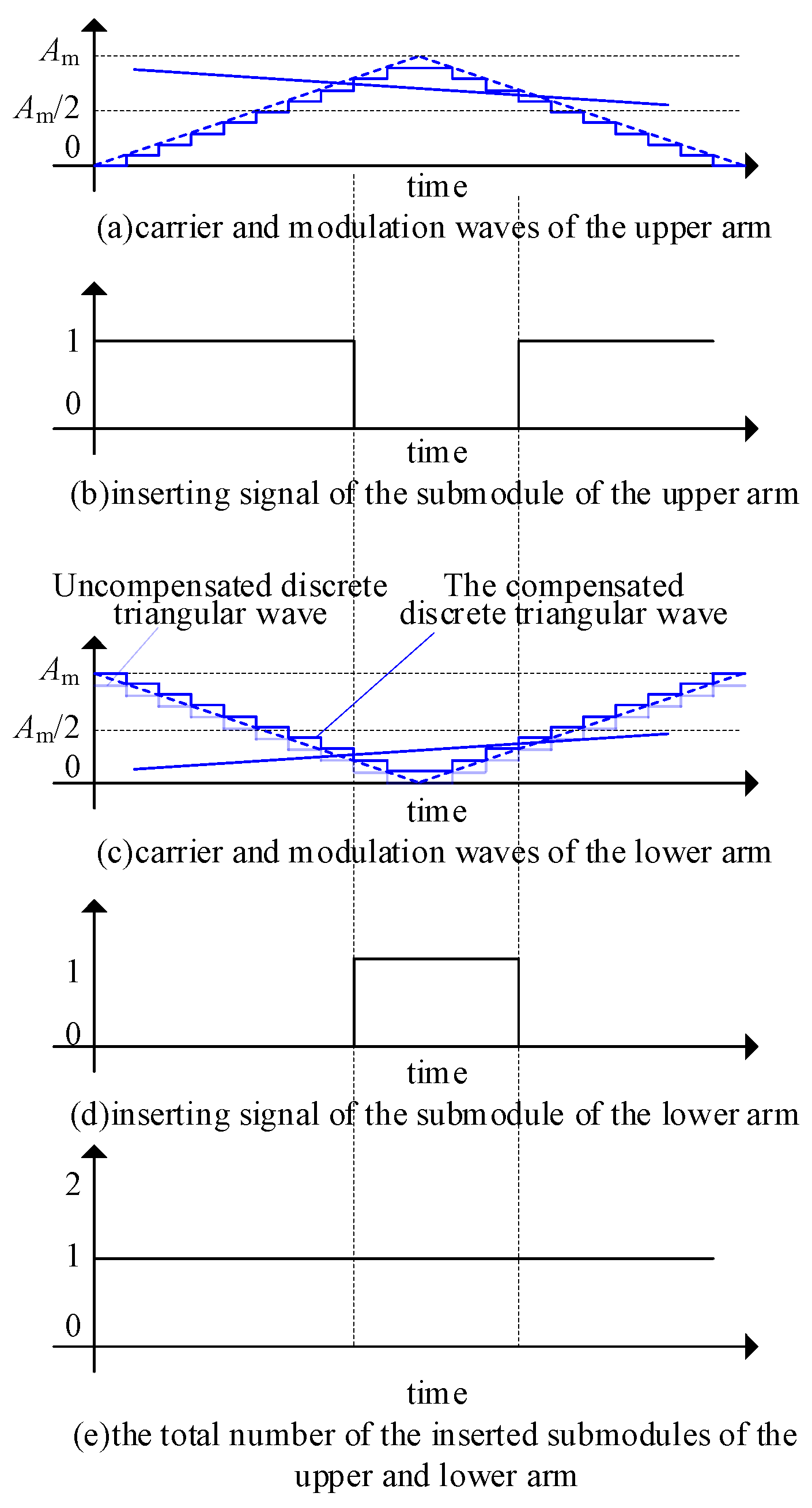
3. Discrete Carrier Compensation Method Based on Amplitude Symmetry
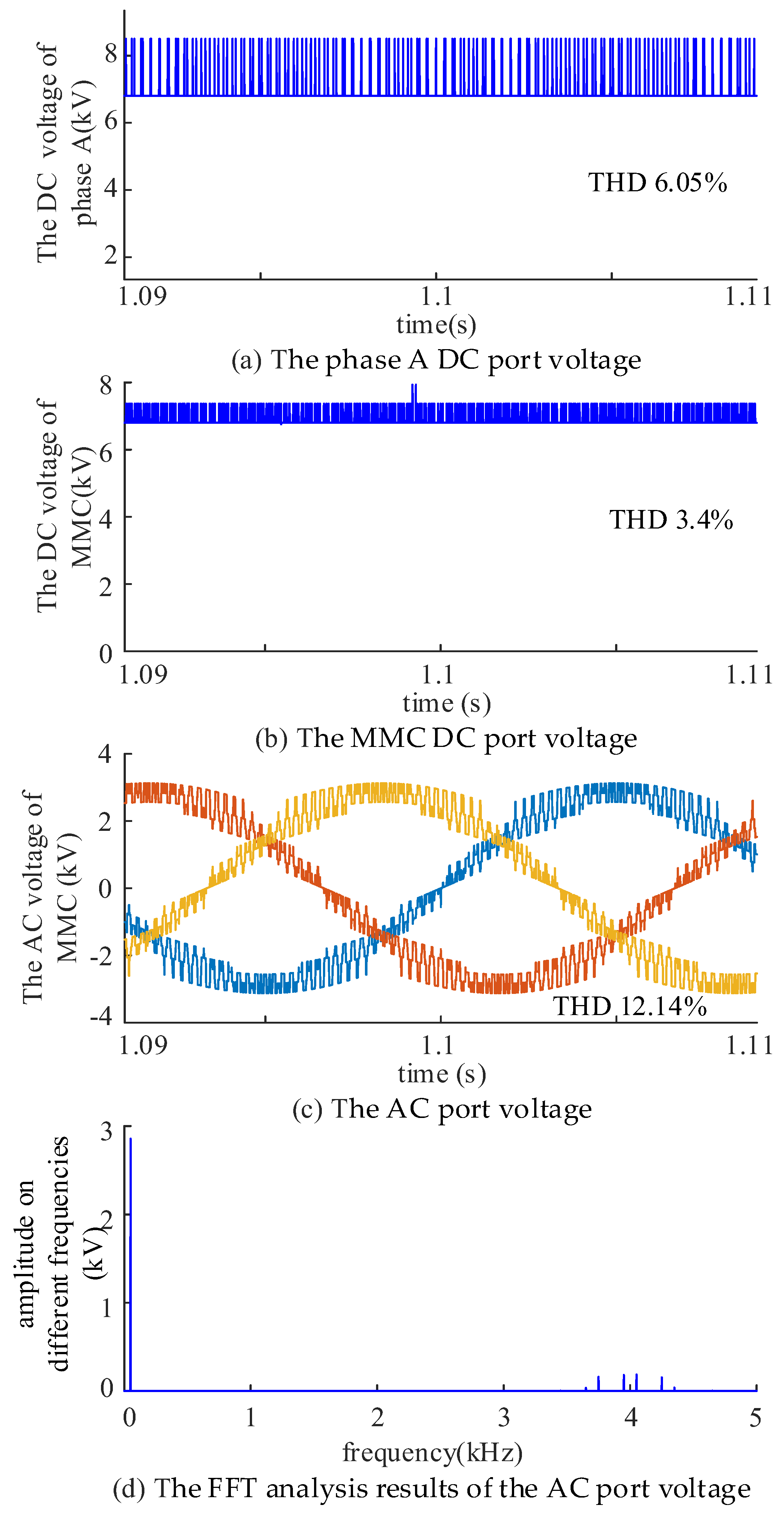
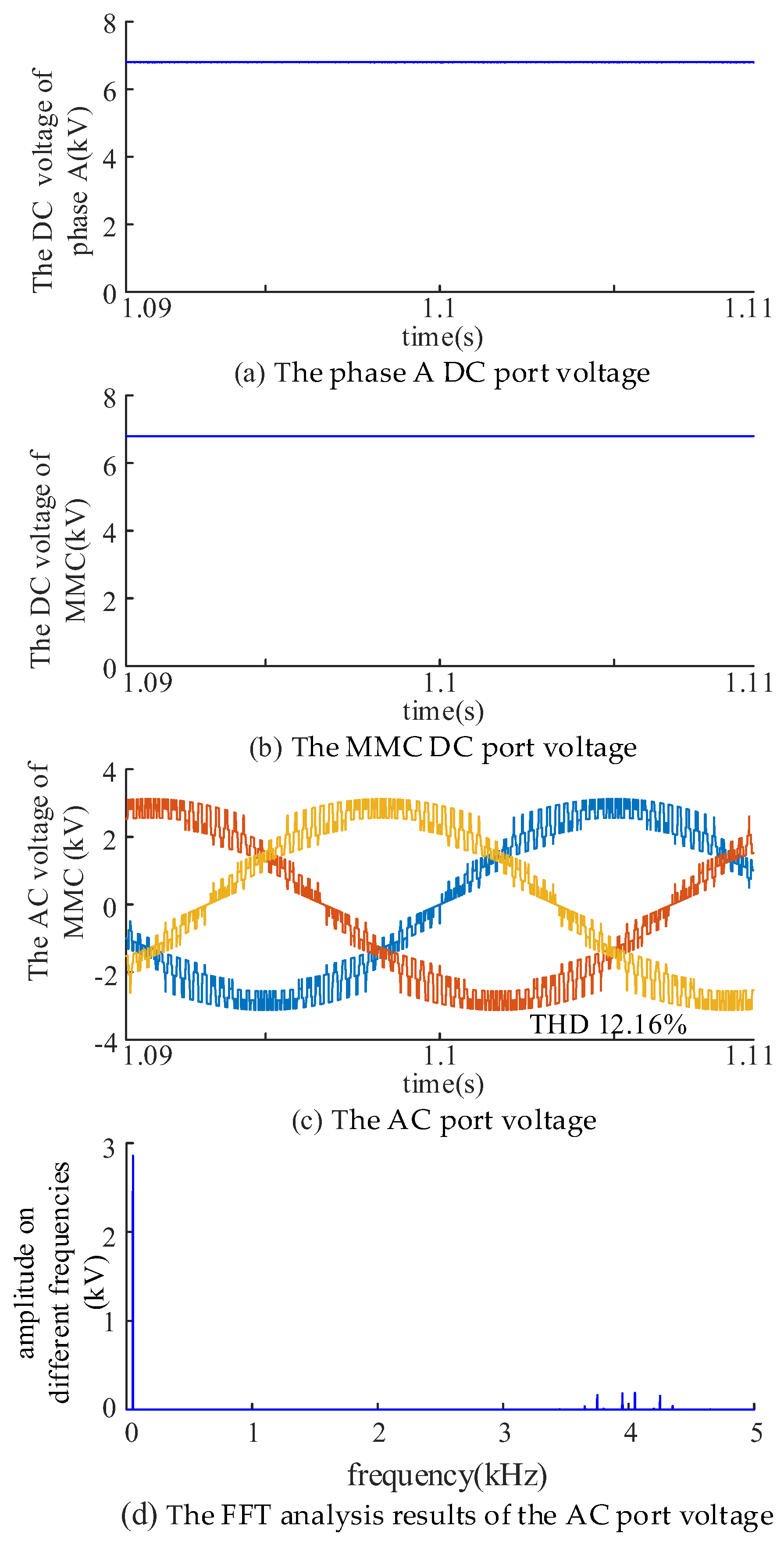
4. Case Study
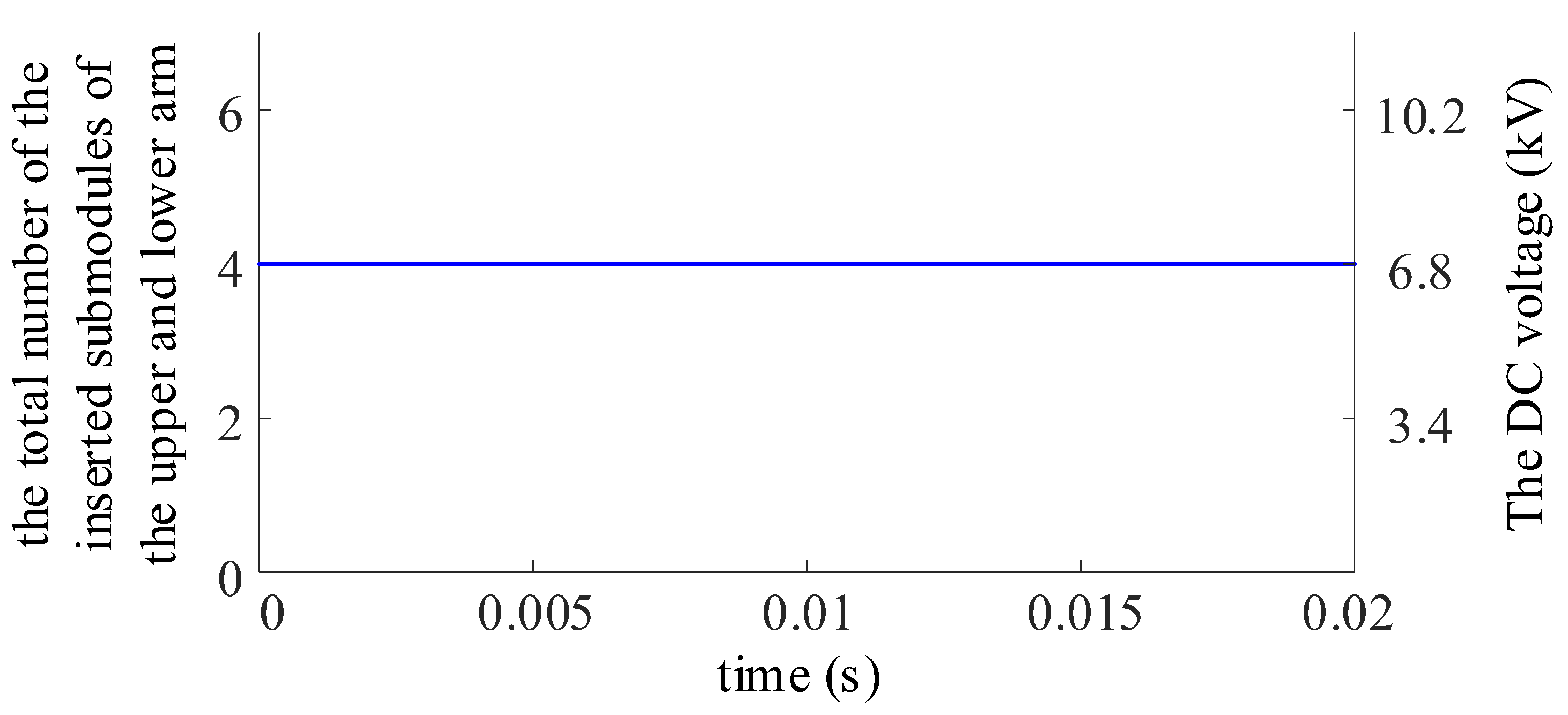
5. Simulation Verification
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Shu, Y.; Zhao, Y.; Zhao, L.; Qiu, B.; Liu, M.; Yang, Y. Study on low carbon energy transition path toward carbon peak and carbon neutrality. Proc. CSEE 2023, 43, 1663–1672. [Google Scholar]
- Kouro, S.; Malinowski, M.; Gopakumar, K.; Pou, J.; Franquelo, L.G.; Wu, B.; Rodriguez, J.; Pérez, M.A.; Leon, J.I. Recent Advances and Industrial Applications of Multilevel Converters. IEEE Trans. Ind. Electron. 2010, 57, 2553–2580. [Google Scholar] [CrossRef]
- Debnath, S.; Qin, J.; Bahrani, B.; Saeedifard, M.; Barbosa, P. Operation, Control, and Applications of the Modular Multilevel Converter: A Review. IEEE Trans. Power Electron. 2015, 30, 37–53. [Google Scholar] [CrossRef]
- Pang, H.; Wei, X. Research on Key Technology and Equipment for Zhangbei 500kV DC Grid. In Proceedings of the 2018 International Power Electronics Conference (IPEC-Niigata 2018-ECCE Asia), Niigata, Japan, 20–24 May 2018; pp. 2343–2351. [Google Scholar]
- Li, J.; Zhu, B.; Guo, Y.; Liang, Z.; Wu, H.; Liu, H. The Simulation of Kunliulong Flexible DC Project with Single Station Operation and out of Operation. In Proceedings of the 2021 IEEE/IAS Industrial and Commercial Power System Asia (I&CPS Asia), Chengdu, China, 18–21 July 2021; pp. 1028–1034. [Google Scholar]
- Huifan, X.; Yang, S.; Guanghu, X.; Tao, L.; Weihuang, H.; Xuehua, L.; Yangzheng, W. Abnormal Analysis and Optimization Measures of Valve-controlled Trigger of Kunliulong Hybrid Multi-terminal UHVDC Project. In Proceedings of the 2023 8th Asia Conference on Power and Electrical Engineering (ACPEE), Tianjin, China, 14–16 April 2023; pp. 1551–1556. [Google Scholar]
- Saad, H.; Fillion, Y.; Deschanvres, S.; Vernay, Y.; Dennetière, S. On Resonances and Harmonics in HVDC-MMC Station Connected to AC Grid. IEEE Trans. Power Deliv. 2017, 32, 1565–1573. [Google Scholar] [CrossRef]
- Li, Y.; Pang, H.; Kong, M.; Lu, J.; Ji, K.; Tang, G. Compensation control and parameters design for high-frequency resonance suppression of MMC-HVDC system. CSEE J. Power Energy Syst. 2021, 7, 1161–1175. [Google Scholar]
- Wu, H.; Wang, X.; Kocewiak, Ł.; Harnefors, L. AC Impedance Modeling of Modular Multilevel Converters and Two-Level Voltage-Source Converters: Similarities and Differences. In Proceedings of the 2018 IEEE 19th Workshop on Control and Modeling for Power Electronics (COMPEL), Padua, Italy, 25–28 June 2018; pp. 1–8. [Google Scholar]
- Yue, B.; Lyu, J.; Yin, J.; Yuan, B.; Li, X.; Yu, H.; Yin, C.; Wang, B. Review of suppression strategies for high-frequency oscillations in MMC-HVDC systems. In Proceedings of the 10th Renewable Power Generation Conference (RPG 2021), Online Conference, 14–15 October 2021; pp. 1015–1019. [Google Scholar]
- Wen, B.; Boroyevich, D.; Burgos, R.; Mattavelli, P.; Shen, Z. Small-Signal Stability Analysis of Three-Phase AC Systems in the Presence of Constant Power Loads Based on Measured d-q Frame Impedances. IEEE Trans. Power Electron. 2015, 30, 5952–5963. [Google Scholar] [CrossRef]
- Canay, I.M. A Novel Approach to the Torsional Interaction and Electrical Damping of the Synchronous Machine. Part I: Theory. IEEE Power Eng. Rev. 1982, 10, 24. [Google Scholar] [CrossRef]
- Yuan, H.; Yuan, X.; Hu, J. Modeling of Grid-Connected VSCs for Power System Small-Signal Stability Analysis in DC-Link Voltage Control Timescale. IEEE Trans. Power Syst. 2017, 32, 3981–3991. [Google Scholar] [CrossRef]
- Li, Y.; An, T.; Zhang, D.; Pei, X.; Ji, K.; Tang, G. Analysis and Suppression Control of High Frequency Resonance for MMC-HVDC System. IEEE Trans. Power Deliv. 2021, 36, 3867–3881. [Google Scholar] [CrossRef]
- Hao, Q.; Li, Z.; Gao, F.; Zhang, J. Reduced-Order Small-Signal Models of Modular Multilevel Converter and MMC-Based HVdc Grid. IEEE Trans. Ind. Electron. 2019, 66, 2257–2268. [Google Scholar] [CrossRef]





| Parameters and Units | Value |
|---|---|
| Rated DC voltage/kV | 6.8 |
| Rated AC voltage/kV | 3.5 |
| Rated submodule voltage/kV | 1.7 |
| Number of arm submodules | 4 |
| Carrier frequency | 1000 |
| Number of triangular wave steps | 128 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huai, Q.; Ji, Y.; Yang, M.; Jie, Z.; Yuan, X.; Xu, X. Elimination Methods for High-Frequency Harmonics on the DC Side of Modular Multilevel Converters from the Perspective of Valve Control. Energies 2025, 18, 2655. https://doi.org/10.3390/en18102655
Huai Q, Ji Y, Yang M, Jie Z, Yuan X, Xu X. Elimination Methods for High-Frequency Harmonics on the DC Side of Modular Multilevel Converters from the Perspective of Valve Control. Energies. 2025; 18(10):2655. https://doi.org/10.3390/en18102655
Chicago/Turabian StyleHuai, Qing, Yirun Ji, Minxiang Yang, Ziyao Jie, Xi Yuan, and Xiang Xu. 2025. "Elimination Methods for High-Frequency Harmonics on the DC Side of Modular Multilevel Converters from the Perspective of Valve Control" Energies 18, no. 10: 2655. https://doi.org/10.3390/en18102655
APA StyleHuai, Q., Ji, Y., Yang, M., Jie, Z., Yuan, X., & Xu, X. (2025). Elimination Methods for High-Frequency Harmonics on the DC Side of Modular Multilevel Converters from the Perspective of Valve Control. Energies, 18(10), 2655. https://doi.org/10.3390/en18102655





