X-Rotor, an Innovative Offshore Wind Turbine to Reduce Cost of Energy †
Abstract
1. Introduction
- (1)
- The aerodynamic efficiency is intrinsically lower because the angle of a blade to the incident wind flow must vary over each rotational cycle and cannot be maintained at an optimum value. This typically means that the swept area of a VAWT must be 15% to 20% larger than that of a HAWT in order to produce the same power.
- (2)
- The optimum rotor speed is roughly half that of comparable HAWTs. Due to the intrinsic differences in aerodynamics, VAWTs typically have reduced rotor speed. Combined with the fact that the drive-train has approximately double the rated torque for any given power rating, VAWT drive-trains tend to be much heavier and more expensive.
- (a)
- The tip speeds of the secondary rotors must be kept comfortably below the speed of sound; that is, the product of the tip speed ratios of the primary and secondary rotors is subject to an upper limit.
- (b)
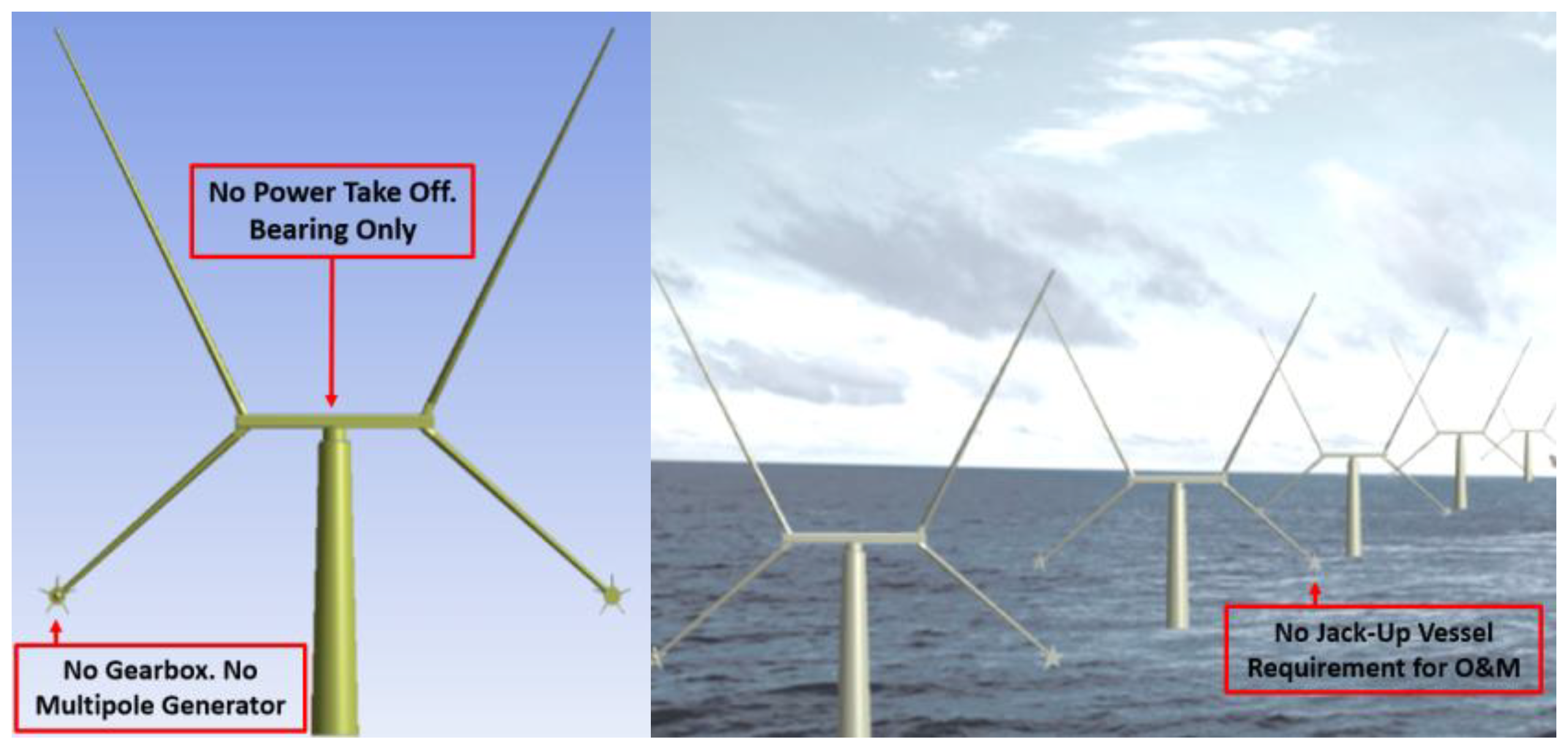
- The rotational speed of the secondary rotors must be sufficiently high that neither a gearbox nor a multi-pole generator is required; that is, a conventional generator suffices without a gearbox.
- (c)
- The induced wind speed acting on the secondary rotors should be sufficiently high that a low secondary rotor radius suffices to meet (2) and structural requirements.
- (d)
- The overall efficiency of power conversion of the combined primary and secondary rotors must be high.
- (e)
- Mounting the secondary rotors on primary rotor blades that are pitched to regulate the turbine should be avoided.
- (1)
- Large reductions in the cost of drive-trains through an easily scalable approach to power take-off (PTO) that does not require a gearbox or multi-pole generator while achieving comparable efficiency levels in power conversion innovations.
- (2)
- Large reductions in Operation and Maintenance (O&M) costs through having no heavy components situated at great height above sea level and through general simplification of the machinery.
2. The X-Rotor Concept
2.1. Overview
2.2. Secondary Rotors
2.3. Primary Rotor
2.4. Control
- (1)
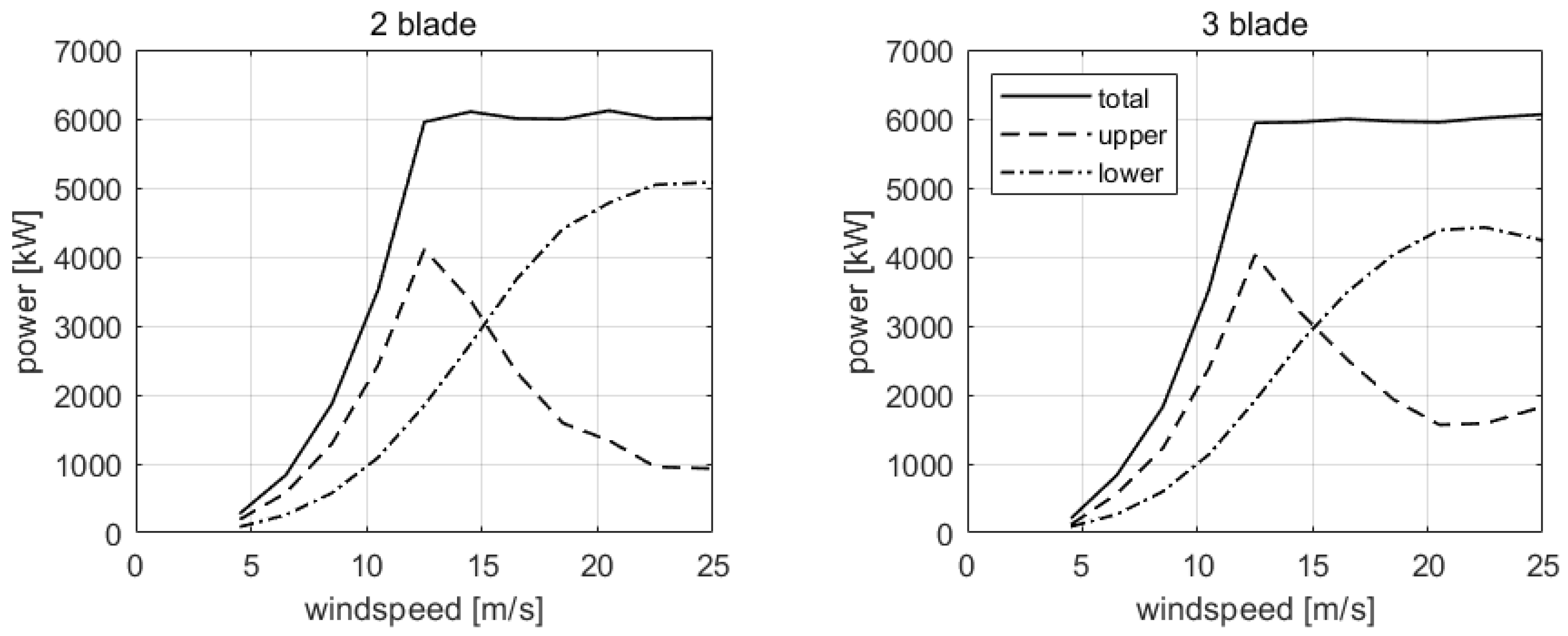
- In above-rated conditions, switch control of the secondary rotors from maintaining a constant tip speed ratio to maintaining a constant rotational speed. Although the total power generated would no longer vary with wind speed, there would be little reduction in the primary rotor torque.
- (2)
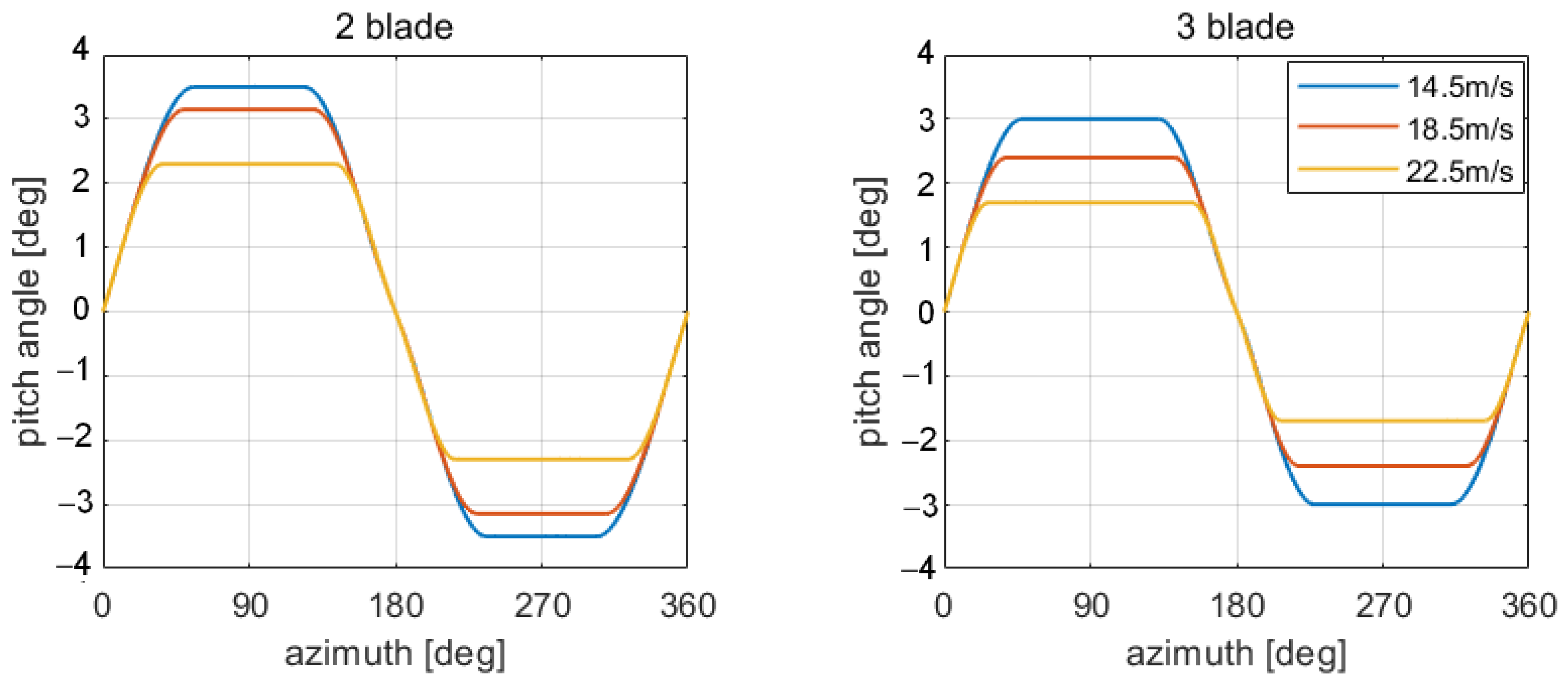
- In below-rated conditions, vary the pitch cyclically to increase the efficiency of the primary rotor by possibly 5% [9]. It would be straightforward to estimate the azimuthal angle of the primary rotor from the cyclically varying power generated by each secondary rotor.
- (3)
- In above-rated conditions, vary the pitch cyclically to reduce loadings on the blade while achieving only a slightly lower efficiency [10].
2.5. Power Converter System
2.6. O&M
2.7. Integrated X-Rotor Design
3. Baseline 5 MW Wind Turbine Designs for the X-Rotor
3.1. Turbine Operational Regime and Aerodynamic Loads
3.2. Structural Analysis of Primary Rotor Blades for Two-Blade Design
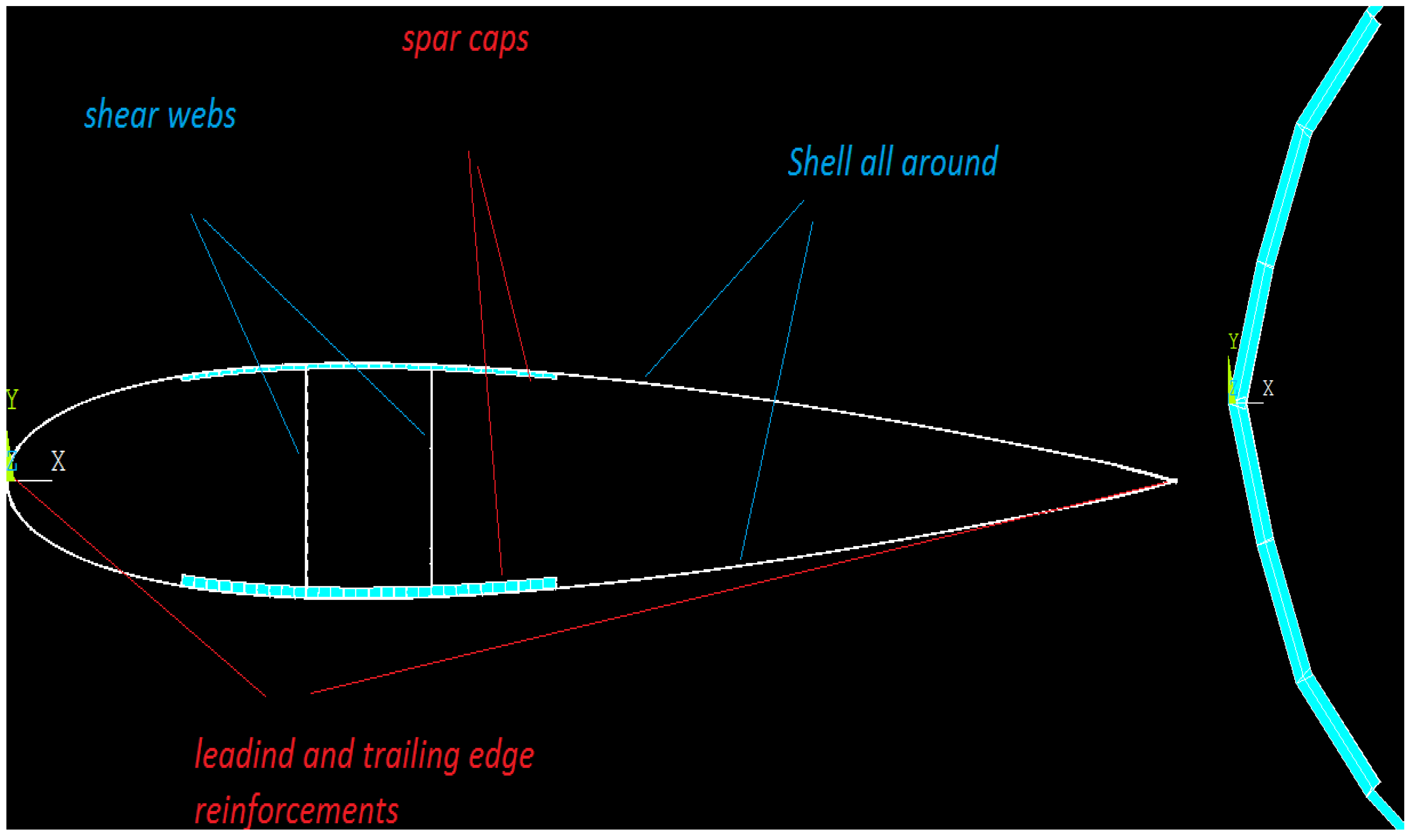
3.2.1. Primary Rotor Blade Design
3.2.2. Ultimate Limit State Analysis
3.2.3. Buckling Verification
3.2.4. Modal Analysis and Dynamic Response Simulation
3.3. Rotor Loads on Tower and Substructure
3.4. Secondary Rotor Design
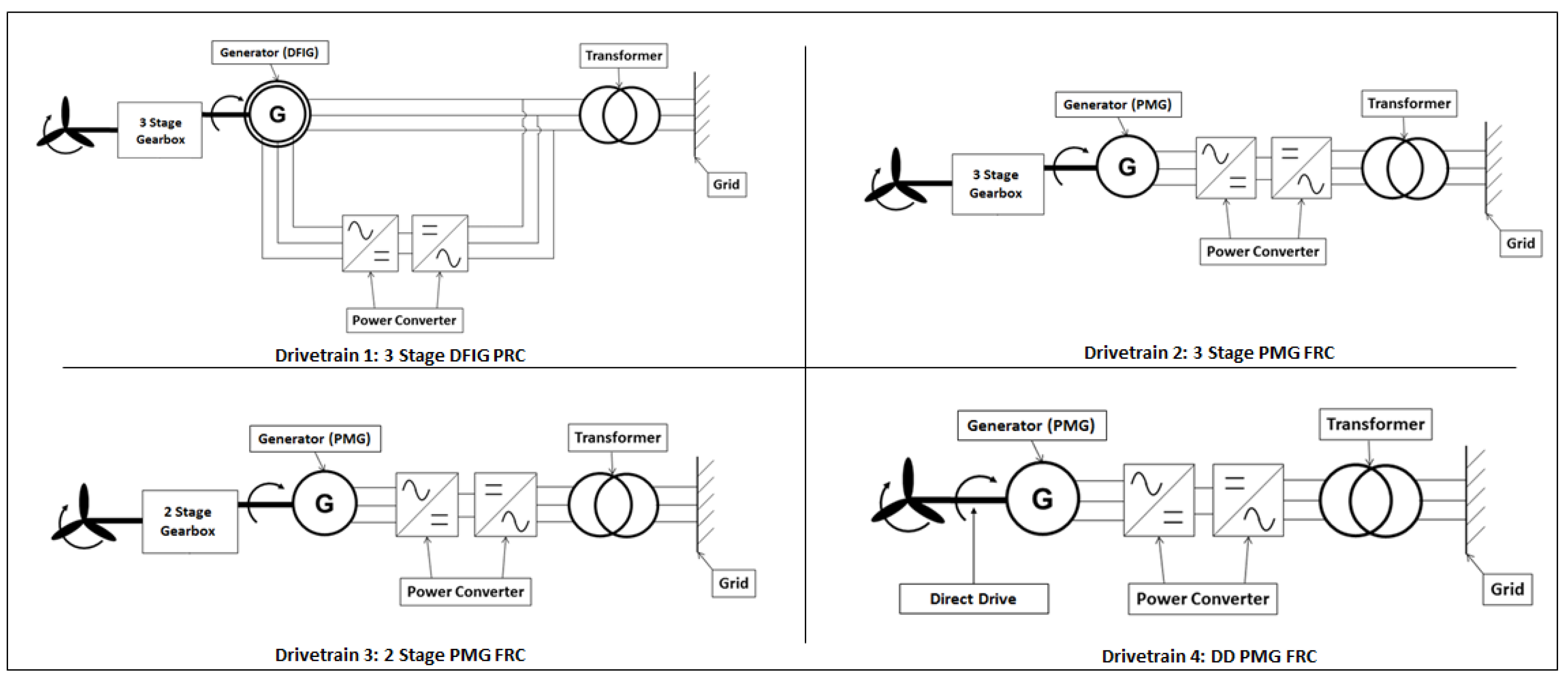
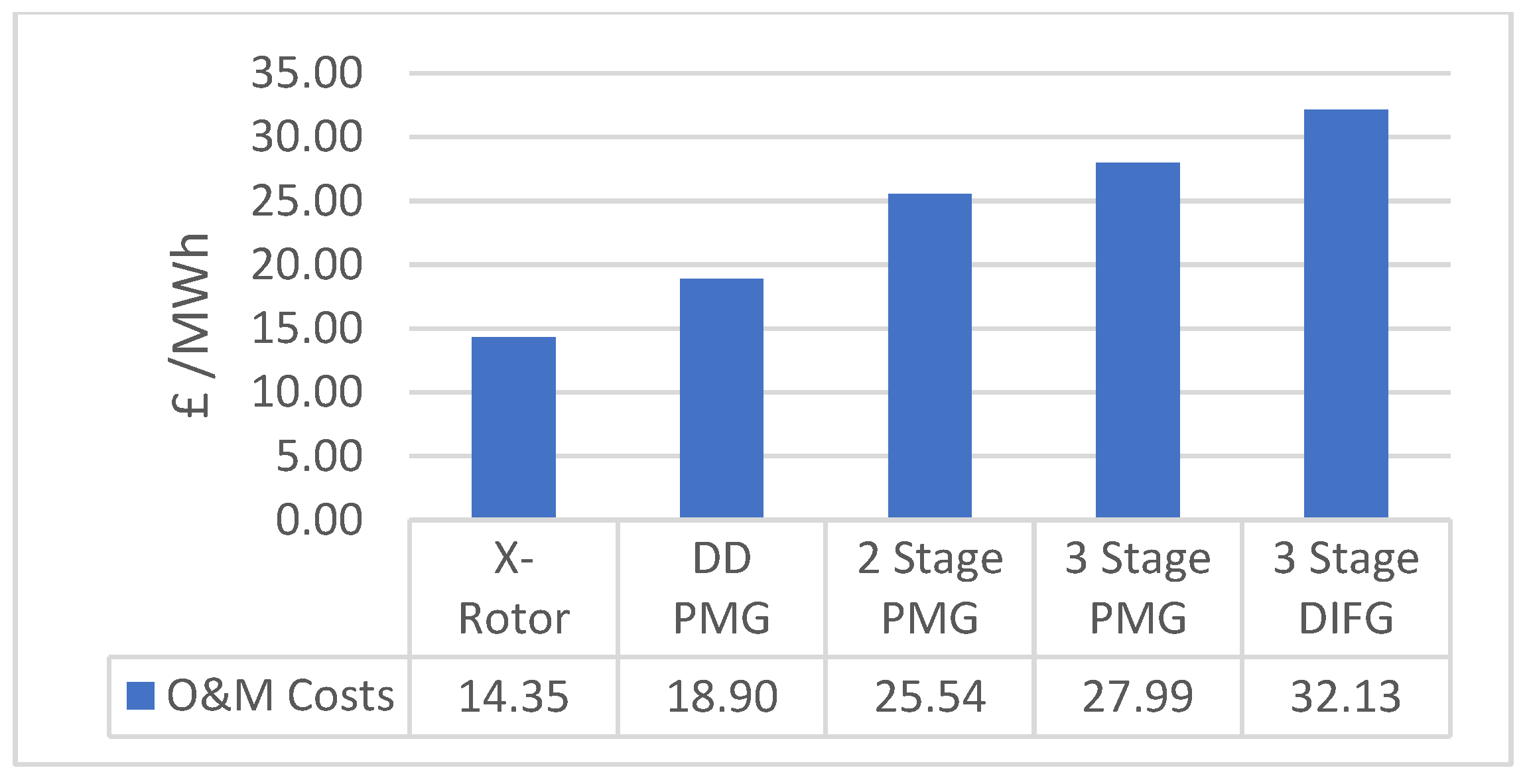
4. O&M Cost Analysis
5. CoE Discussion
6. X-Rotor’s Alternative Configurations (Beyond 5 MW)
7. Conclusions
- –
- CAPEX savings from 5% for a 3-stage DFIG-based power-train to 35% for a DD PMG-based power-train.
- –
- OPEX savings from 55% for a 3-stage DFIG-based power-train to 35% for a DD PMG-based power-train.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. FE Blade Design
| Section | NACA Profile | Spars Thickness Upper Blades [mm] | Spars Thickness Lower Blades [mm] | Shear Webs Thickness Upper Blades [mm] | Shear Webs Thickness Lower Blades [mm] | Shell Thickness All Blades [mm] | Reinforcements Thickness All Blades [mm] |
|---|---|---|---|---|---|---|---|
| 1 | 0025 | 55 | 20 | 20 | 10 | 5 | 5 |
| 2 | 0024 | 53 | 19.41 | 19 | 10 | 5 | 5 |
| 3 | 0023 | 51 | 18.82 | 19 | 10 | 5 | 5 |
| 4 | 0022 | 48 | 18.23 | 18 | 10 | 5 | 5 |
| 5 | 0021 | 46 | 17.64 | 17 | 10 | 5 | 5 |
| 6 | 0020 | 45 | 17.05 | 17 | 10 | 5 | 5 |
| 7 | 0019 | 43 | 16.47 | 16 | 10 | 5 | 5 |
| 8 | 0018 | 40 | 15.88 | 15 | 10 | 5 | 5 |
| 9 | 0017 | 37 | 15.29 | 14 | 10 | 5 | 5 |
| 10 | 0016 | 35 | 14.71 | 13 | 10 | 5 | 5 |
| 11 | 0015 | 30 | 14.11 | 10 | 10 | 5 | 5 |
| 12 | 0014 | 25 | 13.52 | 10 | 10 | 5 | 5 |
| 13 | 0013 | 20 | 12.94 | 10 | 10 | 5 | 5 |
| 14 | 0012 | 15 | 12.35 | 10 | 10 | 5 | 5 |
| 15 | 0011 | 10 | 11.76 | 10 | 10 | 5 | 5 |
| 16 | 0010 | 10 | 11.17 | 10 | 10 | 5 | 5 |
| 17 | 0009 | 10 | 10.58 | 10 | 10 | 5 | 5 |
| 18 | 0008 | 10 | 10 | 10 | 10 | 5 | 5 |
| Section | NACA Profile | Spars Thickness Upper Blades [mm] | Spars Thickness Lower Blades [mm] | Shear Webs Thickness Upper Blades [mm] | Shear Webs Thickness Lower Blades [mm] | Shell Thickness All Blades [mm] | Reinforcements Thickness All Blades [mm] |
|---|---|---|---|---|---|---|---|
| 1 | 0025 | 100 | 30 | 10 | 10 | 5 | 5 |
| 2 | 0024 | 95 | 28 | 10 | 10 | 5 | 5 |
| 3 | 0023 | 90 | 26 | 10 | 10 | 5 | 5 |
| 4 | 0022 | 85 | 24 | 10 | 10 | 5 | 5 |
| 5 | 0021 | 80 | 22 | 10 | 10 | 5 | 5 |
| 6 | 0020 | 75 | 21 | 10 | 10 | 5 | 5 |
| 7 | 0019 | 70 | 19 | 10 | 10 | 5 | 5 |
| 8 | 0018 | 63 | 17 | 10 | 10 | 5 | 5 |
| 9 | 0017 | 56 | 15 | 10 | 10 | 5 | 5 |
| 10 | 0016 | 48 | 13 | 10 | 10 | 5 | 5 |
| 11 | 0015 | 39 | 11 | 10 | 10 | 5 | 5 |
| 12 | 0014 | 29 | 10 | 10 | 10 | 5 | 5 |
| 13 | 0013 | 19 | 10 | 10 | 10 | 5 | 5 |
| 14 | 0012 | 14 | 10 | 10 | 10 | 5 | 5 |
| 15 | 0011 | 10 | 10 | 10 | 10 | 5 | 5 |
| 16 | 0010 | 10 | 10 | 10 | 10 | 5 | 5 |
| 17 | 0009 | 10 | 10 | 10 | 10 | 5 | 5 |
| 18 | 0008 | 10 | 10 | 10 | 10 | 5 | 5 |
References
- Tjiu, W.; Marnoto, T.; Mat, S.; Ruslan, M.; Sopian, K. Darrieus vertical axis wind turbine for power generation II: Challenges in HAWT Looking Climate Change Policy. Renew. Energy 2015, 75, 560–571. [Google Scholar] [CrossRef]
- Willis, S. (James Ingram & Associates, Anglesey, UK); Ingram, J. (James Ingram & Associates, Anglesey, UK); Michon, M. (University of Sheffield, Sheffield, UK); Atallah, K. (University of Sheffield, Sheffield, UK); Kolios, A. (University of Cranfield, Cranfield, UK); Brennan, F. (University of Cranfield, Cranfield, UK); Feuchtwang, J. (University of Strathclyde, Glasgow, UK); Leithead, W. (University of Strathclyde, Glasgow, UK); Parker, M. (University of Strathclyde, Glasgow, UK); Anaya-Lara, O. (University of Strathclyde, Glasgow, UK); et al. Personal communication: ETI WI1001 NOVA Phase 1 Feasibility Study Final Report to Energy Technology Institute, UK, 2010.
- Hitter, U. Wind-Driven Power Plant. US4197056, 8 April 1980. [Google Scholar]
- St.-Germain, J. Wind Machine with Electric Generators and Secondary Rotors Located on Rotating Vertical Blades. US5151610, 29 September 1992. [Google Scholar]
- Madsen, H.A.; Rasmussen, F. Wind Turbine Having Secondary Rotors. WO02086312, 31 October 2002. [Google Scholar]
- Jørgensen, B.H.; Madsen, P.H.; Giebel, G.; Martí, I.; Thomsen, K. DTU International Energy Report 2021: Perspectives on Wind Energy; DTU Wind Energy: Roskilde, Denmark, 2021. [Google Scholar]
- Baker, J.B. Wind Driven Generators: Combined Horizontal and Vertical Wind Turbines. GB2477750, 17 August 2011. [Google Scholar]
- Leithead, W.; Camciuc, A.; Amiri, A.K.; Carroll, J. The X-Rotor Offshore Wind Turbine Concept. In Proceedings of the 16th Deep Sea Offshore Wind R&D Conference, Trondheim, Norway, 16–18 January 2019; Volume 1356, pp. 012031–012041. [Google Scholar] [CrossRef]
- Paraschivoiu, I.; Trifu, O.; Saeed, F. H-Darrieus Wind Turbine with Blade Pitch Control. Int. J. Rotating Mach. 2009, 2009, 505343. [Google Scholar] [CrossRef]
- Soraghan, C. Aerodynamic Modelling and Control of Vertical Axis Wind Turbines. Ph.D. Thesis, University of Strathclyde, Glasgow, UK, 2014. [Google Scholar]
- Merkhouf, A.; Doyon, P.; Upadhyay, S. Variable Frequency Transformer—Concept and Electromagnetic Design Evaluation. IEEE Tran Energy Convers. 2008, 23, 989–996. [Google Scholar] [CrossRef]
- Jamieson, P. Top-level rotor optimisations based on actuator disc theory. Wind. Energy Sci. 2020, 5, 807–818. [Google Scholar] [CrossRef]
- IEC 61400-1; Wind Turbine—Part 1: Design Requirements. International Electrotechnical Commission: Geneva, Switzerland, 2005.
- Guidelines for Design of Wind, Turbines, 2nd ed.; DNV/Riso: Oslo, Norway, 2002.
- ANSYS 17.1; ANSYS Inc.: Canonsburg, PA, USA, 2016.
- COM(2015) 80 Final—A Framework Strategy for a Resilient Energy Union with a Forward; European Environment Agency: Copenhagen, Denmark, 2015.
- Chidambaram, S.; Kamaraj, A.; Kumar, R.S.; Karthik, V. Shear Fracture and Industrial Overload Failure of Mechanical Fuse Shear Pin. IOP Conf. Ser. Mater. Sci. Eng. 2018, 377, 012004. [Google Scholar] [CrossRef]
- Chen, I.W.; Wong, B.L.; Lin, Y.H.; Chau, S.W.; Huang, H.H. Design and analysis of jacket substructures for offshore wind turbines. Energy 2016, 9, 264. [Google Scholar] [CrossRef]
- Arshad, M.; O’Kelly, B.C. Offshore wind-turbine structures: A review. Energy 2013, 166, 139–152. [Google Scholar] [CrossRef]
- De Vries, W. Offshore Foundations and Support Structures; WP4 Final Report, FP6 Project Upwind; Upwind: San Francisco, CA, USA, 2011. [Google Scholar]
- Oest, J.; Sandal, K.; Schafhirt, S.; Stieng, L.E.S.; Muskulus, M. On gradient-based optimization of jacket structures for offshore wind turbines. Wind. Energy 2018, 21, 953–967. [Google Scholar] [CrossRef]
- Standard E, Fatigue, Eurocode 3: Design of Steel Structures—Part 1-9: Fatigue; National Standards Authority of Ireland: Ireland, UK, 2005.
- Natarajan, A.; Stolpe, M.; Wandji, W.N. Structural optimization based design of jacket type sub-structures for 10 MW offshore wind turbines. Ocean. Eng. 2019, 172, 629–640. [Google Scholar] [CrossRef]
- Carroll, J.; McDonald, A.; Dinwoodie, I.; McMillan, D.; Revie, M.; Lazakis, I. Availability, operation and maintenance costs of offshore wind turbines with different drive train configurations. Wind. Energy 2016, 20, 361–378. [Google Scholar] [CrossRef]
- Dalgic, Y.; Lazakis, I.; Dinwoodie, I.; McMillan, D.; Revie, M. Advanced logistics planning for offshore wind farm operation and maintenance activities. Ocean. Eng. 2015, 101, 211–226. [Google Scholar] [CrossRef]
- BMU and PTJ, FINO 1 Meteorological Dataset 2004–2012. Available online: http://fino.bsh.de (accessed on 1 December 2014).
- Carroll, J.; McDonald, A.; McMillan, D. Failure rate, repair time and unscheduled O&M cost analysis of offshore wind turbines. Wind. Energy 2016, 19, 1107–1119. [Google Scholar]
- Crabtree, C.; Zappalá, D.; Hogg, S. Wind energy: UK experiences and offshore operational challenges. Proc. Inst. Mech. Eng. 2015, 229, 727–746. [Google Scholar] [CrossRef]
- Carroll, J.; McDonald, A.; McMillan, D.; Stehly, T.; Mone, C.; Maples, B. Cost of Energy for Offshore Wind Turbines with Different Drive Train Types, Conference Paper; EWEA: Brussels, Belgium, 2015. [Google Scholar]



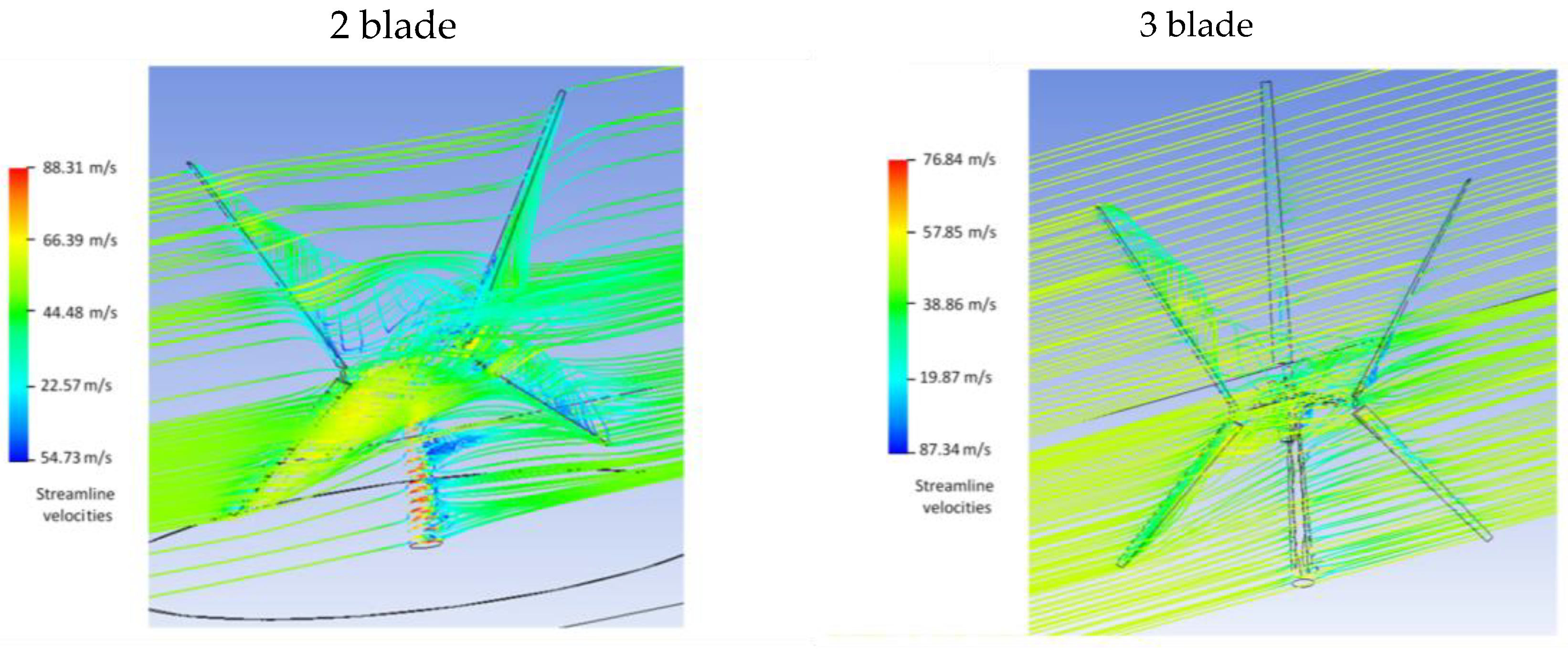
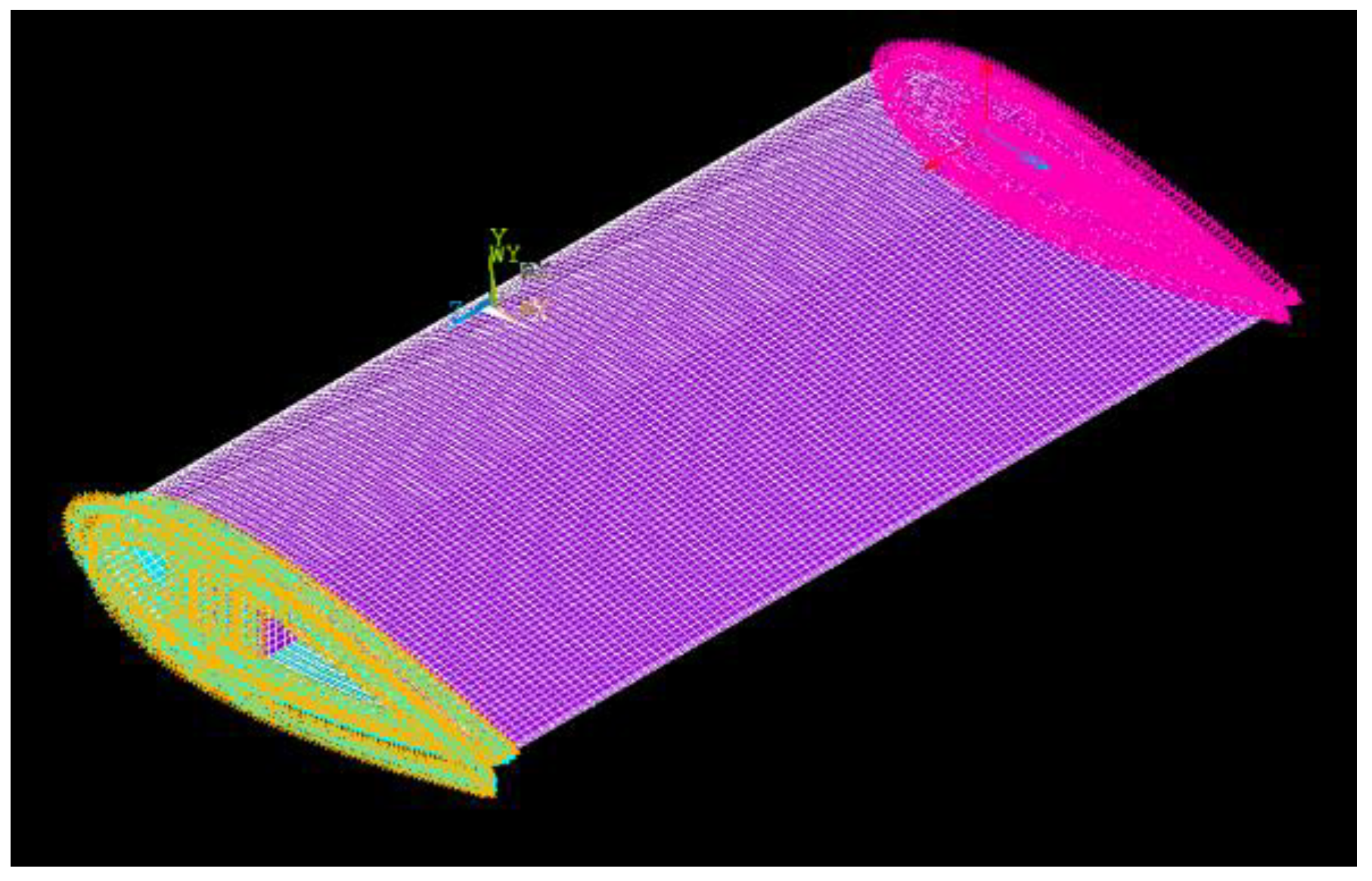

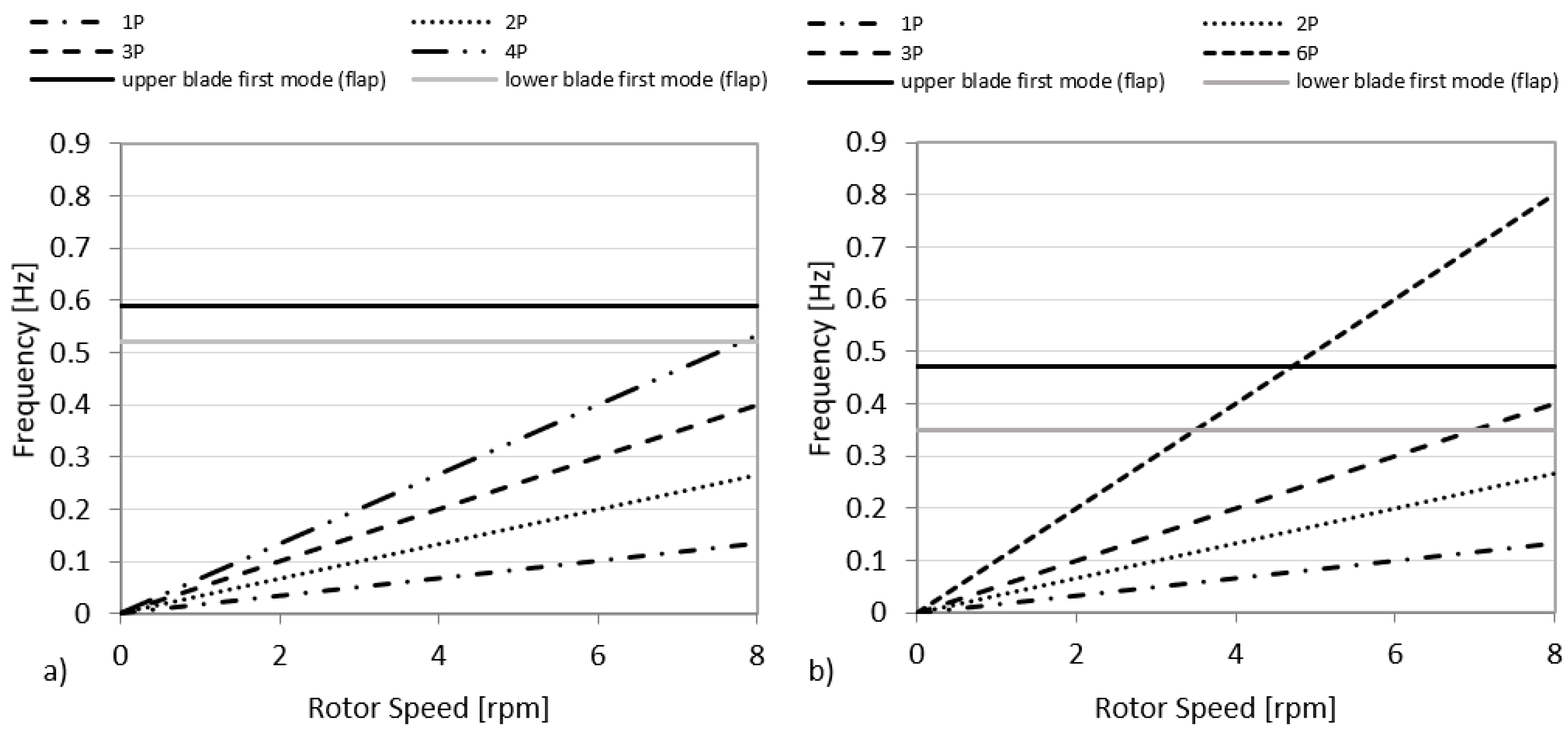
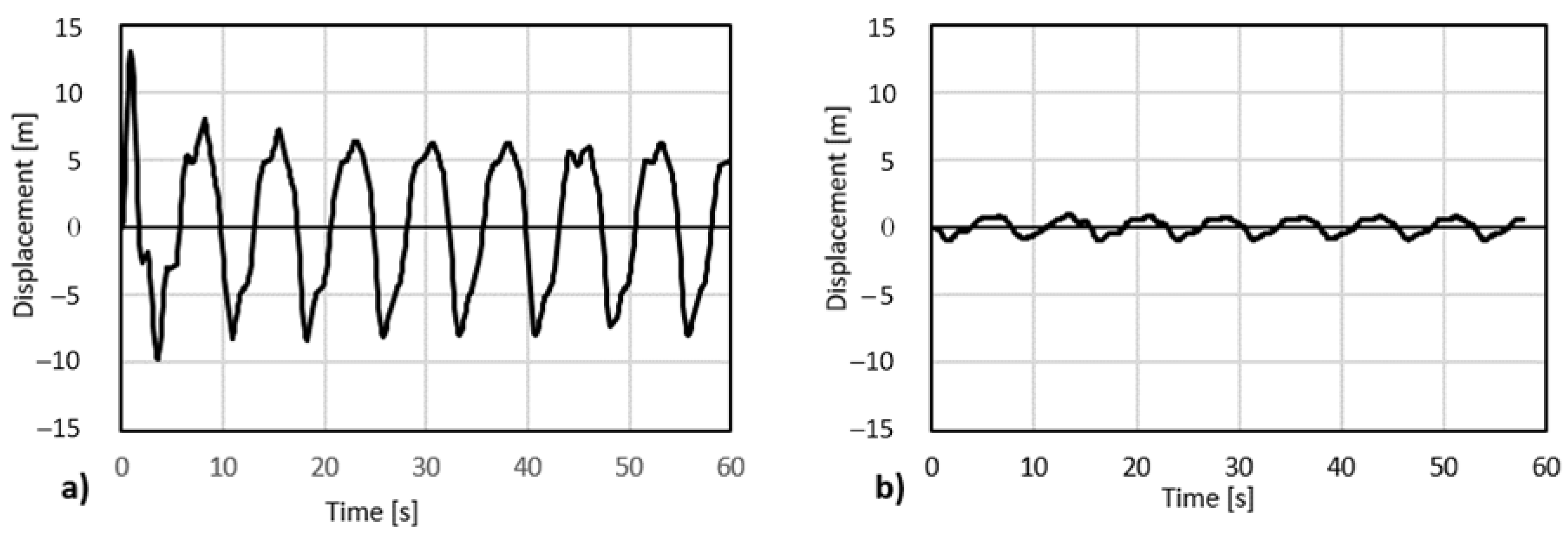
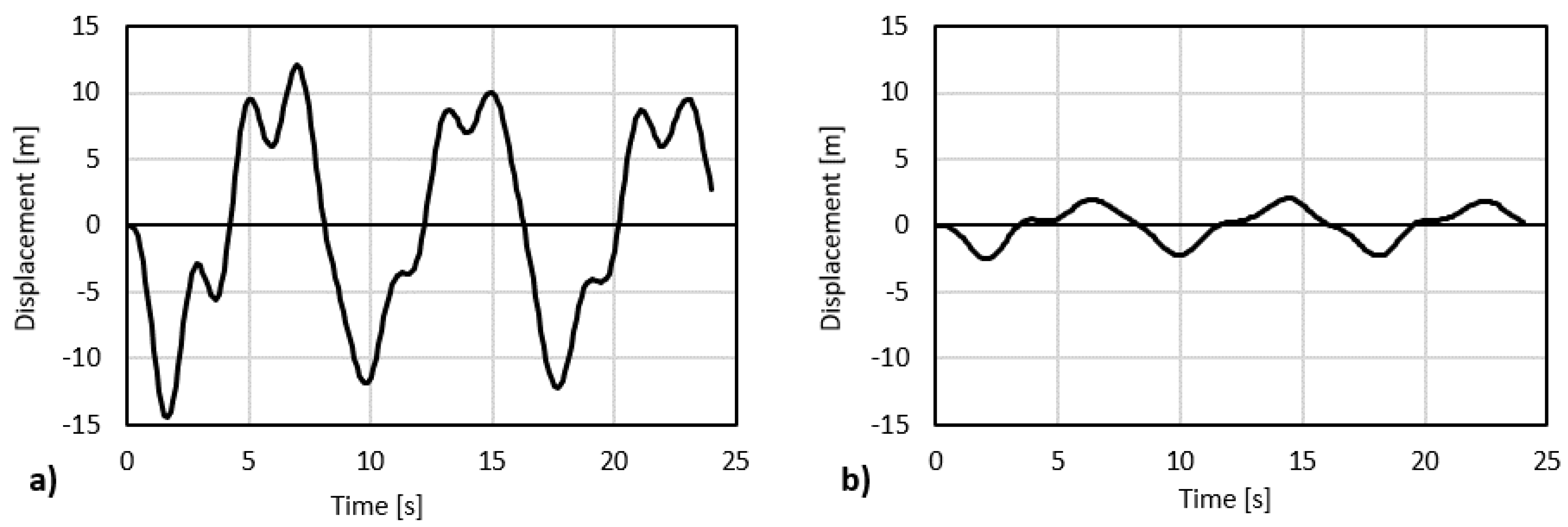
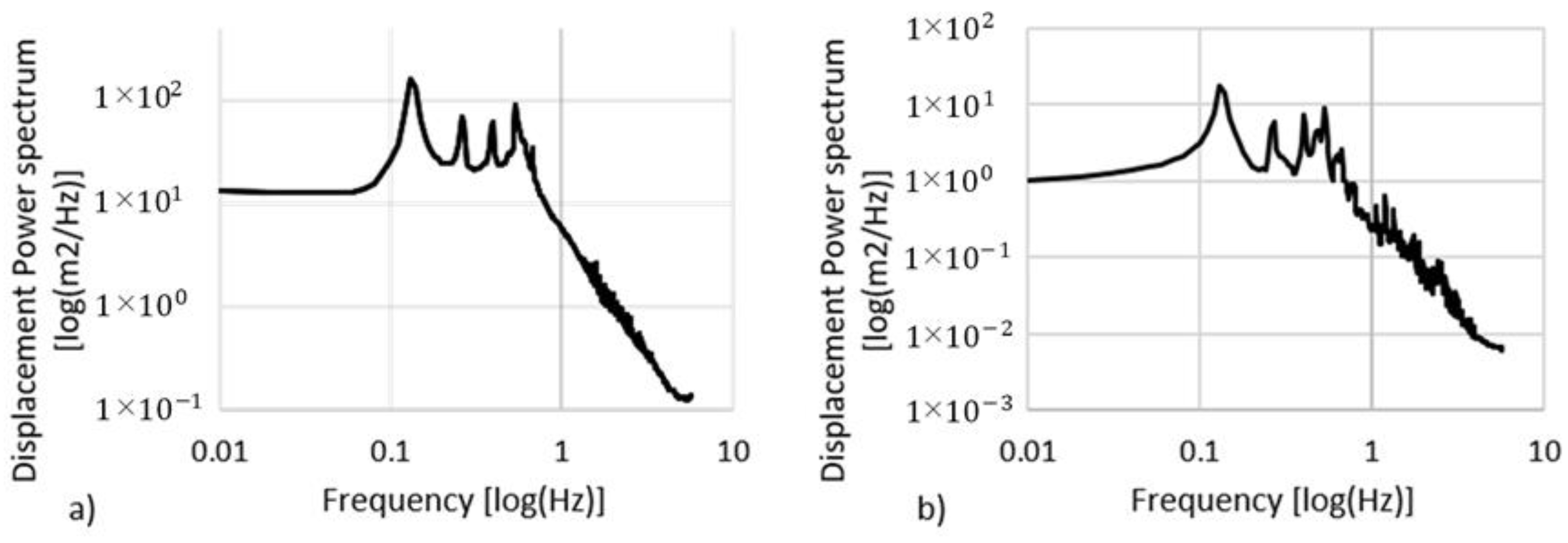
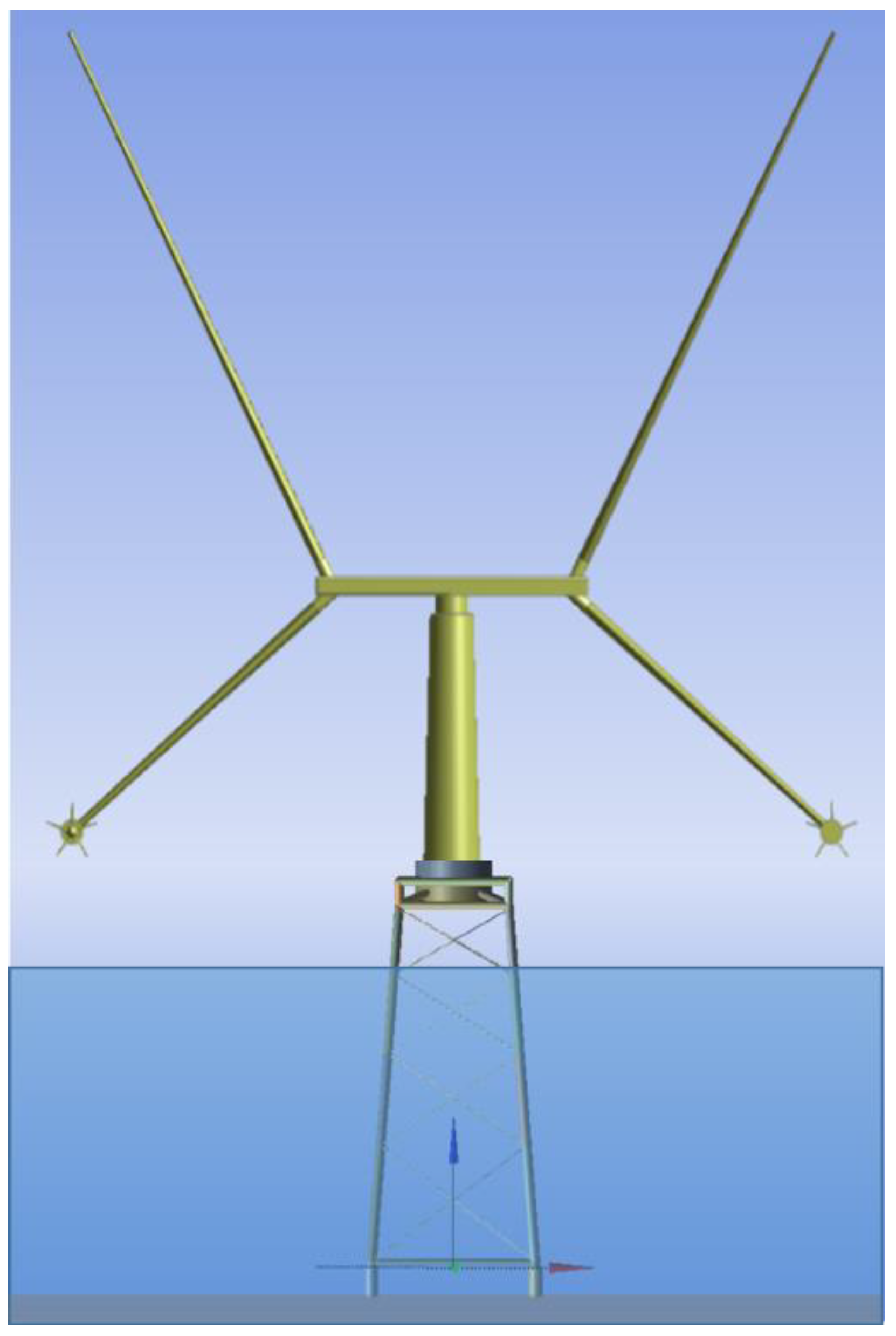


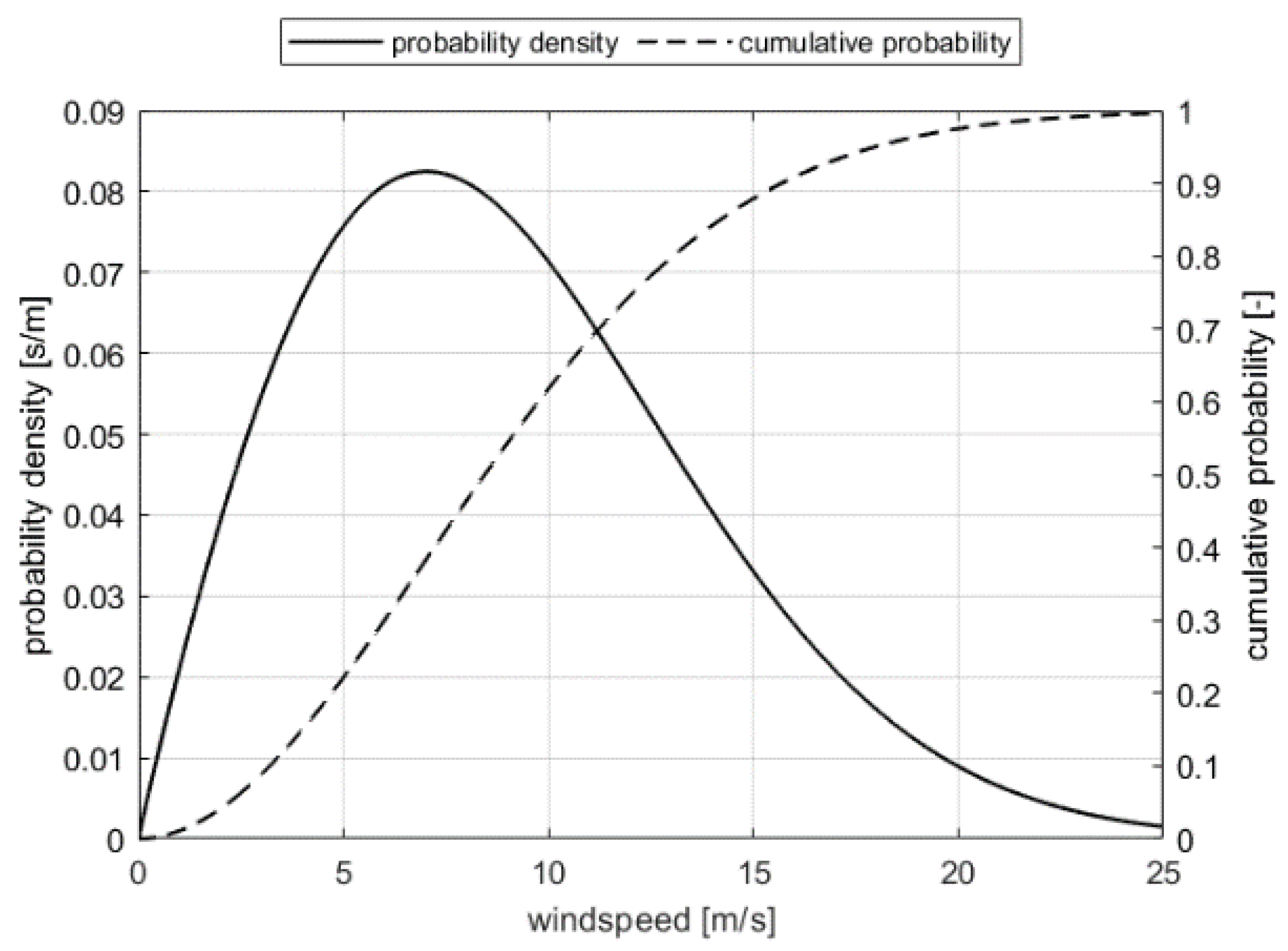


| Rotor | Cone Angle [deg] | Chord (Root) [m] | Chord (Tip) [m] | Thickness (Root) [%] | Thickness (Tip) [%] | |
|---|---|---|---|---|---|---|
| Two Bladed | Upper | 30 | 10 | 5 | 25 | 8 |
| Lower | 50 | 14 | 7 | 25 | 8 | |
| Three Bladed | Upper | 30 | 6.67 | 3.33 | 25 | 8 |
| Lower | 50 | 9.33 | 4.67 | 25 | 8 |
| Load Case | Wind Speed [m/s] | Rpm | Pitch Amplitude [deg] | ||
|---|---|---|---|---|---|
| 2-Blade | 3-Blade | 2-Blade | 3-Blade | ||
| 1 | 4.5 | 2.88 | 2.7 | 0 | 0 |
| 2 | 6.5 | 4.16 | 3.9 | 0 | 0 |
| 3 | 8.5 | 5.44 | 5.1 | 0 | 0 |
| 4 | 10.5 | 6.72 | 6.3 | 0 | 0 |
| 5 | 12.5 | 8 | 7.5 | 0 | 0 |
| 6 | 14.5 | 8 | 7.5 | 3.5 | 3 |
| 7 | 16.5 | 8 | 7.5 | 3.5 | 2.7 |
| 8 | 18.5 | 8 | 7.5 | 3.15 | 2.4 |
| 9 | 20.5 | 8 | 7.5 | 2.75 | 2 |
| 10 | 22.5 | 8 | 7.5 | 2.3 | 1.7 |
| 11 | 25 | 8 | 7.5 | 1.8 | 1.1 |
| Shell | Spar Cap | Reinforcement | Shear Webs | |
|---|---|---|---|---|
| Property | Triaxial | Uniaxial (CF) | Uniaxial (GF) | Biaxial |
| E11 [GPa] | 21.790 | 115.00 | 41.630 | 13.920 |
| E22 [GPa] | 14.670 | 7.560 | 14.930 | 13.920 |
| ν12 [-] | 0.478 | 0.30 | 0.241 | 0.533 |
| G12 [GPa] | 9.413 | 3.96 | 5.047 | 11.500 |
| Ρ [Kg/3] | 1845.000 | 1578 | 1915.000 | 1845.000 |
| σ11-Ten [MPa] | 480.400 | 1317.60 | 876.100 | 223.200 |
| σ11-Comp [MPa] | 393.000 | 620.13 | 625.800 | 209.200 |
| σ22-Ten [MPa] | 90.400 | 21.88 | 74.030 | 223.200 |
| σ22-Comp [MPa] | 152.700 | 76.25 | 189.400 | 209.200 |
| τ12 [MPa] | 114.000 | 45.53 | 56.580 | 140.300 |
| Blade | Two-Bladed | Three-Bladed | ||
|---|---|---|---|---|
| Flap [MNm] | Edge [MNm] | Flap [MNm] | Edge [MNm] | |
| Upper | 79.6 | 3.12 | 50 | 0.7 |
| Lower | 45.4 | 2.26 | 26.5 | 0.41 |
| Variant | 2-Blade Design [kg] | 3-Blade Design [kg] |
|---|---|---|
| Upper blade | 40,500 | 39,947 |
| Lower blade | 23,384 | 16,266 |
| Upper rotor | 81,000 | 104,841 |
| Lower rotor | 46,768 | 48,798 |
| Total rotor | 127,768 | 153,639 |
| Mode Number | Buckling Load Factor | |||
|---|---|---|---|---|
| Two-Bladed Design | Three-Blade Design | |||
| Upper Blade | Lower Blade | Upper Blade | Lower Blade | |
| 1 | 2.7 | 9.9 | 3.6 | 7.3 |
| 2 | 4.6 | 15.9 | 6.7 | 12.2 |
| 3 | 6.4 | 23.6 | 10.0 | 17.7 |
| 4 | 8.6 | 31.8 | 13.4 | 23.5 |
| 5 | 10.9 | 41.1 | 16.9 | 30.0 |
| Mode Number | Natural Frequencies [Hz] | |||
|---|---|---|---|---|
| Two-Bladed Design | Three-Bladed Design | |||
| Upperr Blade | Lower Blade | Upper Blade | Lower Blade | |
| 1 | 0.60 | 0.52 | 0.47 | 0.35 |
| 2 | 0.69 | 0.77 | 0.66 | 0.60 |
| 3 | 1.59 | 2.61 | 1.12 | 2.02 |
| 4 | 1.82 | 2.70 | 1.83 | 2.31 |
| X-Rotor Variant | Fx [N] | Fy [N] | Fz [N] | Mx [Nm] | My [Nm] | Mz [Nm] |
|---|---|---|---|---|---|---|
| 2-blade | −54,871 | −106,315 | −3.79 × 106 | −6.34 × 107 | −1.07 × 107 | 861,663 |
| 3-blade | −32,899 | 27,572 | −2.52 × 106 | −3.54 × 107 | −4 × 106 | 1.14 × 106 |
| Two-Blade | Three-Blade | |||
|---|---|---|---|---|
| Unit Force [1 × 106 N] | Unit Moment [1 × 107 NM] | Unit Force [1 × 106 N] | Unit Moment [1 × 107 NM] | |
| Jacket leg | 14.5 | 1.8 | 55 | 6 |
| Tower | 22 | 4 | 65 | 12 |
| Load Case | Cycle Count [Million] | Stress Range | Damage | |||
|---|---|---|---|---|---|---|
| 2-Blade | 3-Blade | 2-Blade | 3-Blade | 2-Blade | 3-Blade | |
| 1 | 8.60 | 12.13 | 4.34 | 2.40 | 0 | 0 |
| 2 | 14.22 | 20.05 | 9.05 | 4.26 | 0 | 0 |
| 3 | 18.04 | 25.42 | 15.49 | 7.32 | 0 | 0 |
| 4 | 19.10 | 26.90 | 23.64 | 11.18 | 0 | 0 |
| 5 | 17.57 | 24.70 | 33.48 | 16.56 | 0.23 | 0 |
| 6 | 12.35 | 17.36 | 36.29 | 28.58 | 0.24 | 0 |
| 7 | 7.69 | 11.19 | 37.03 | 34.19 | 0.17 | 0.16 |
| 8 | 4.73 | 6.65 | 38.79 | 38.56 | 0.13 | 0.18 |
| 9 | 2.60 | 3.65 | 41.44 | 41.31 | 0.10 | 0.14 |
| 10 | 1.32 | 1.86 | 44.63 | 43.82 | 0.07 | 0.09 |
| 11 | 0.51 | 0.72 | 49.46 | 46.61 | 0.05 | 0.05 |
| Total | 106.99 | 150.64 | - | - | 0.999 | 0.62 |
| Tower Base Outer Radius [m] | Jacket Upper Width [m] | Jacket Lower Width [m] | Jacket Mass [×103 kg] | First Natural Frequency [Hz] | |
|---|---|---|---|---|---|
| 2-blade | 3.79 | 8.00 | 12.00 | 940 | 0.77 |
| 3-blade | 2.60 | 20.00 | 30.00 | 655 | 0.45 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leithead, W.E.; Amiri, A.M.K.; Camciuc, A.; Morgan, L.; Carroll, J.; Feuchtwang, J. X-Rotor, an Innovative Offshore Wind Turbine to Reduce Cost of Energy. Energies 2025, 18, 2549. https://doi.org/10.3390/en18102549
Leithead WE, Amiri AMK, Camciuc A, Morgan L, Carroll J, Feuchtwang J. X-Rotor, an Innovative Offshore Wind Turbine to Reduce Cost of Energy. Energies. 2025; 18(10):2549. https://doi.org/10.3390/en18102549
Chicago/Turabian StyleLeithead, William E., Abbas Mehrad Kazemi Amiri, Arthur Camciuc, Laurence Morgan, James Carroll, and Julian Feuchtwang. 2025. "X-Rotor, an Innovative Offshore Wind Turbine to Reduce Cost of Energy" Energies 18, no. 10: 2549. https://doi.org/10.3390/en18102549
APA StyleLeithead, W. E., Amiri, A. M. K., Camciuc, A., Morgan, L., Carroll, J., & Feuchtwang, J. (2025). X-Rotor, an Innovative Offshore Wind Turbine to Reduce Cost of Energy. Energies, 18(10), 2549. https://doi.org/10.3390/en18102549








