Performance Analysis of Wave Rotor Combustor Integration into Baseline Engines: A Comparative Study of Pressure-Gain and Work Cycles
Abstract
1. Introduction
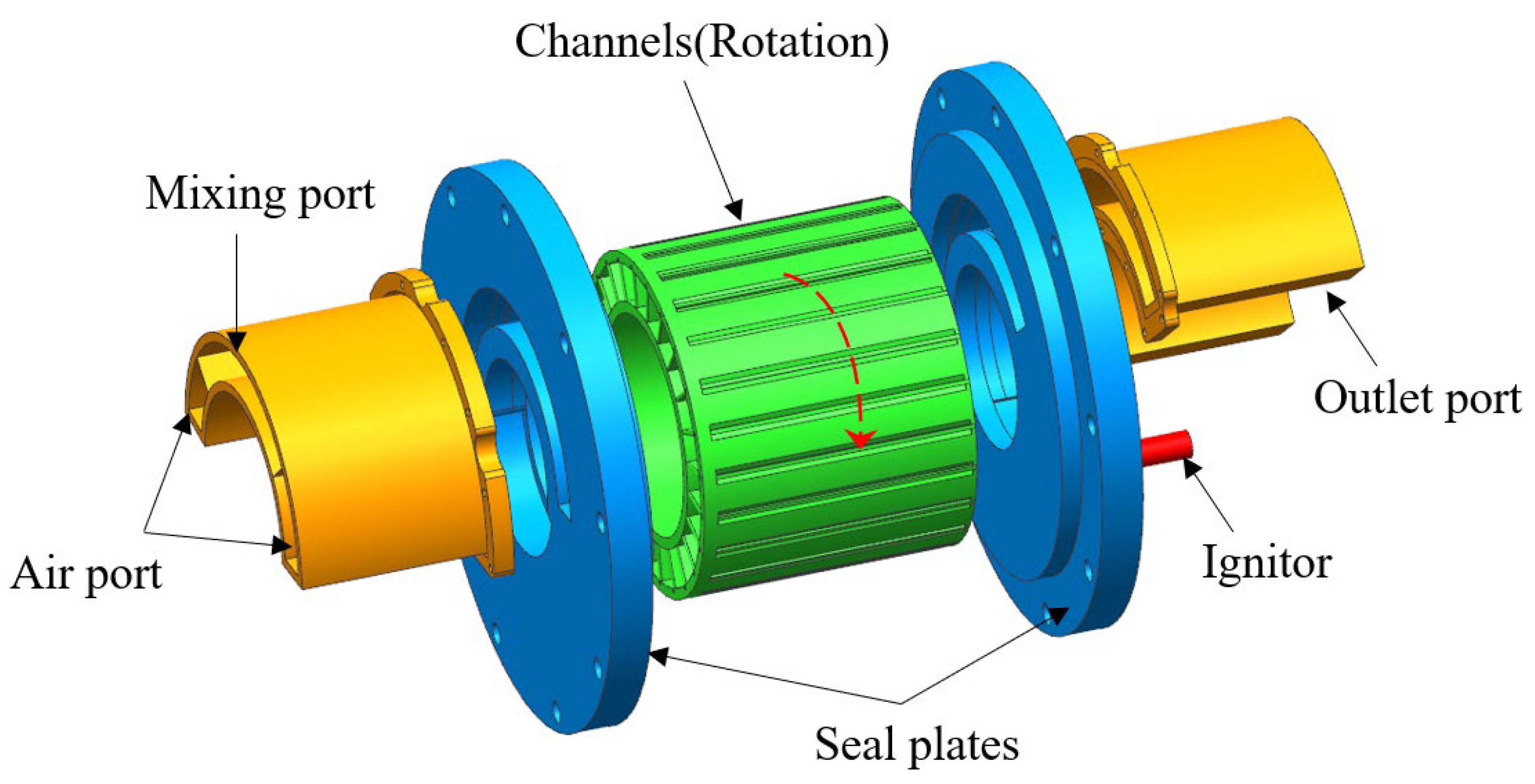
2. Wave Rotor Combustor
3. Establishment of Thermodynamic Cycle
3.1. Assumptions
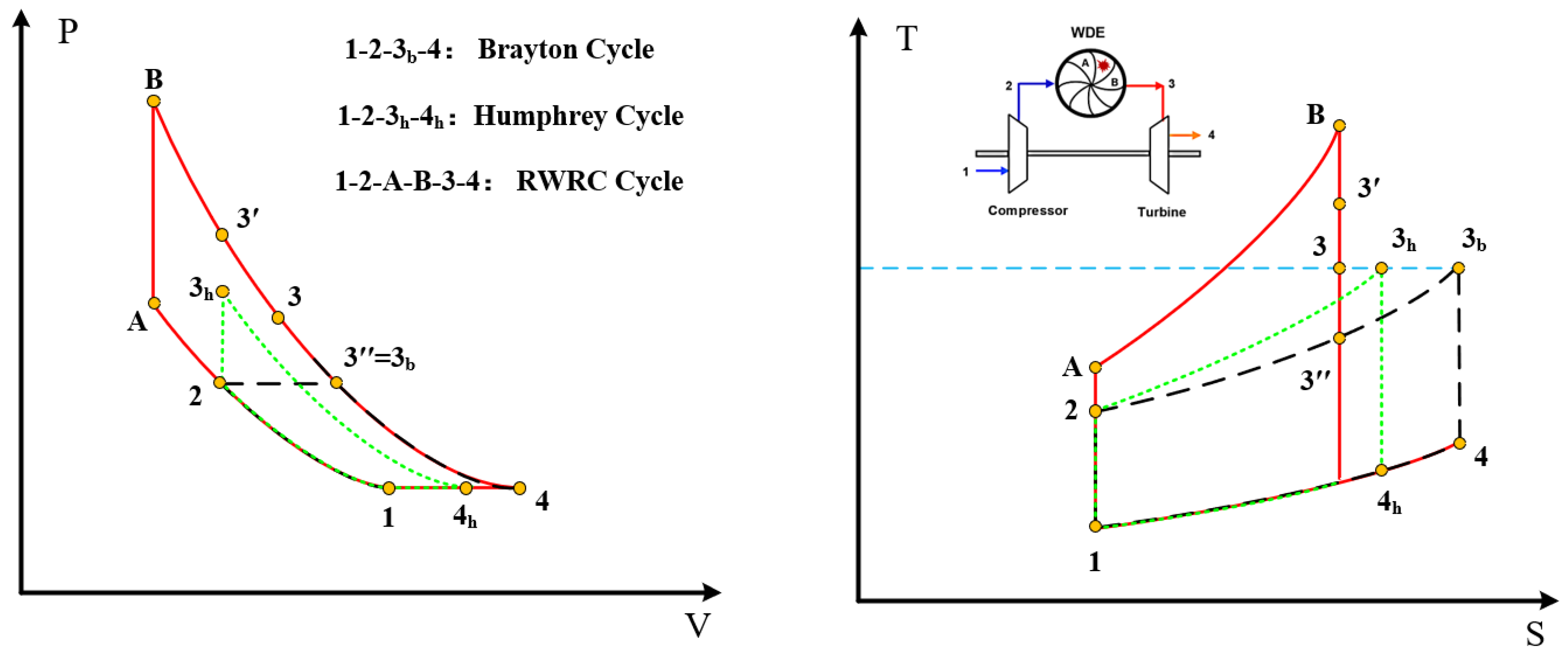
3.2. The Baseline Engine Cycle
3.3. The Wave Rotor Pressure-Gain Cycle (WRPGC)
3.4. The Wave Rotor Work Cycle (WRWC)
4. Results and Discussions
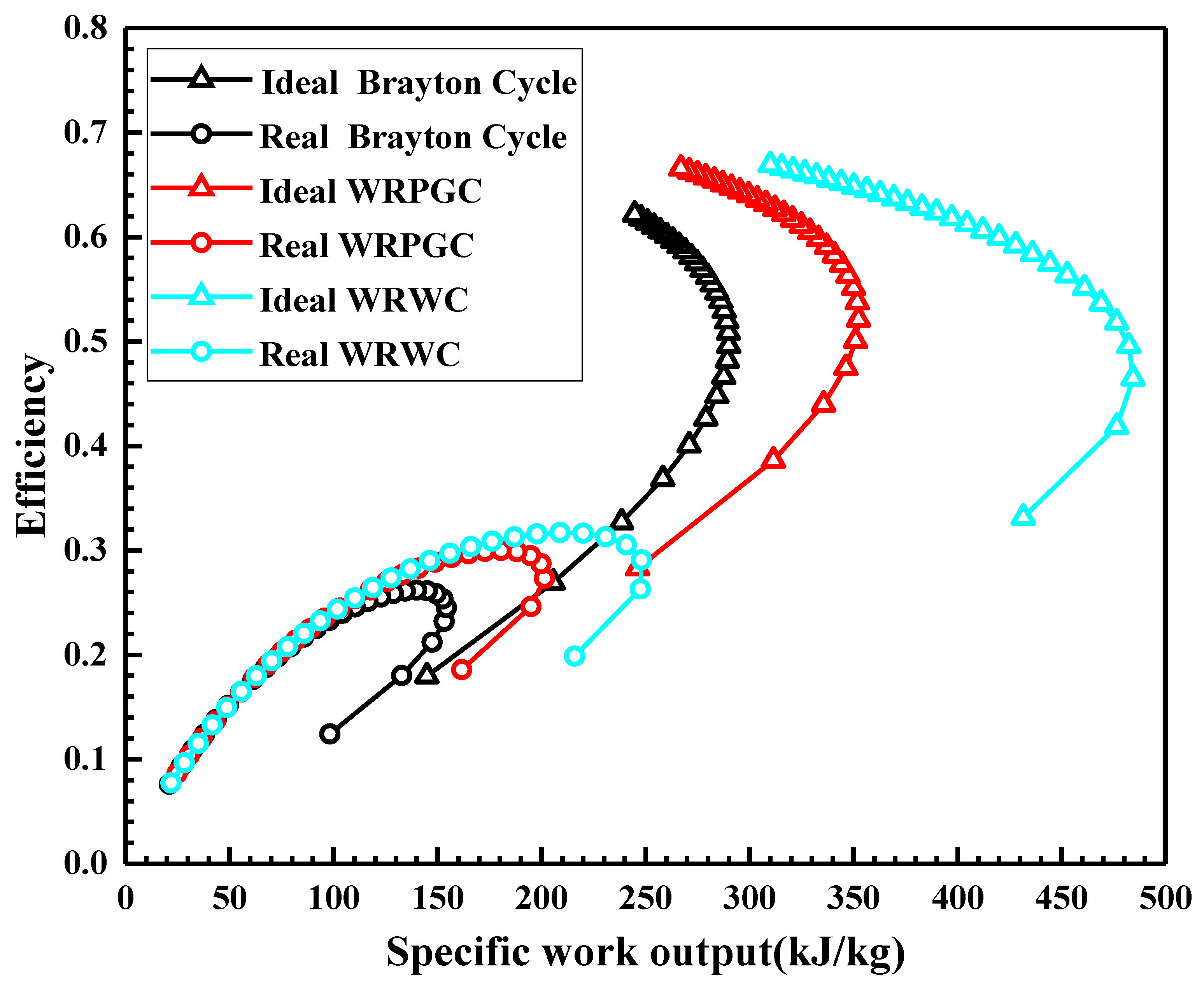
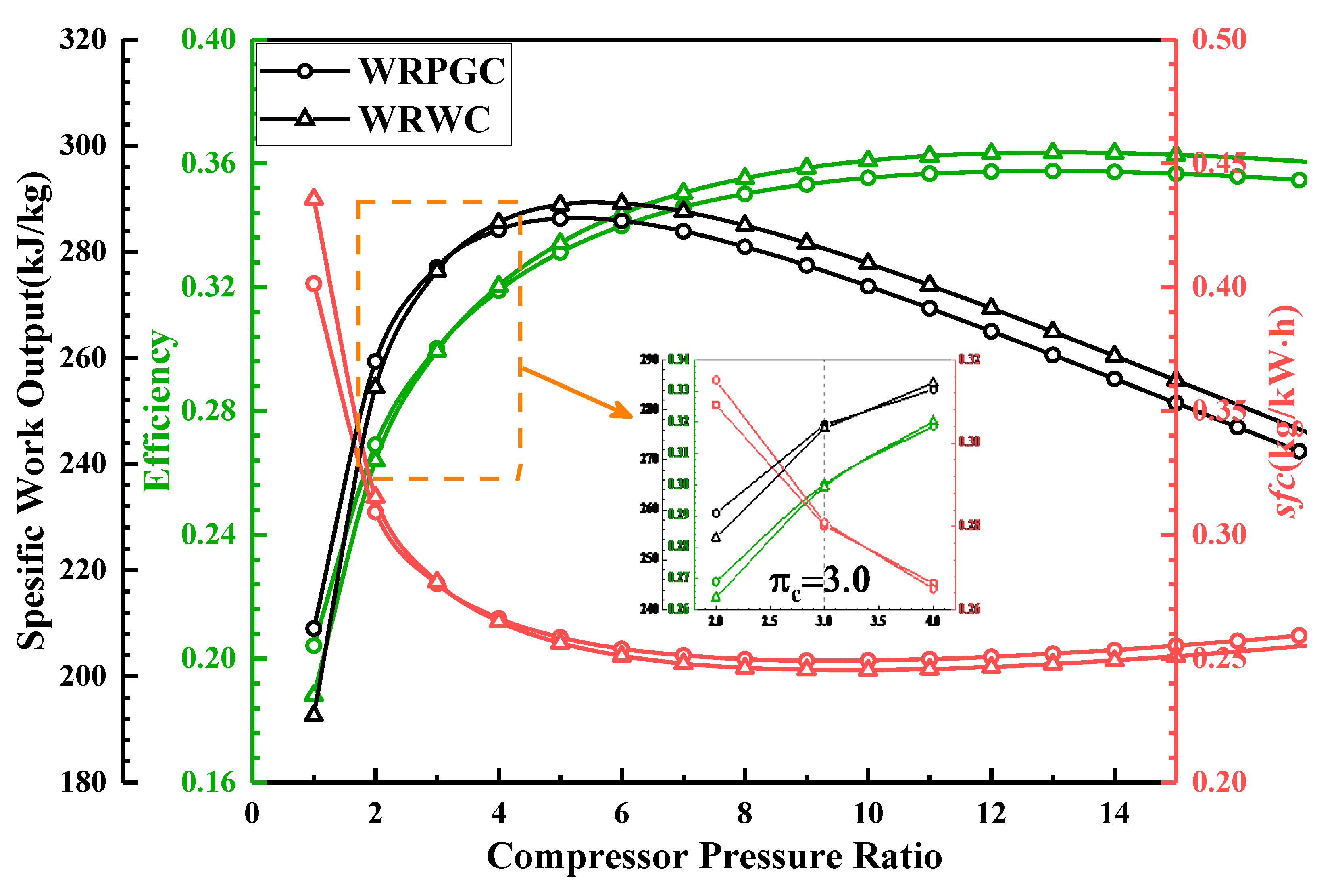
4.1. Comparative Study of Different Thermodynamic Cycles
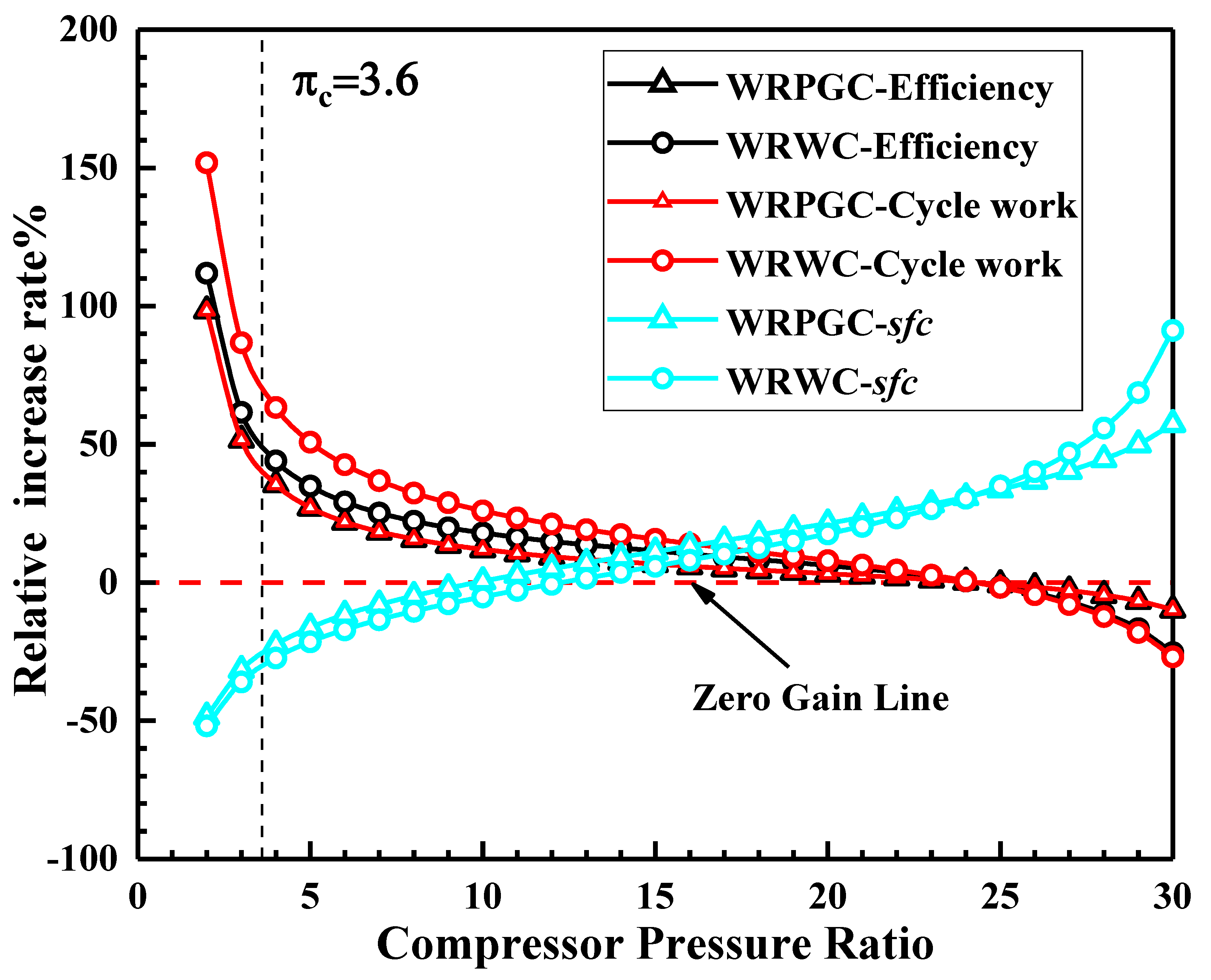
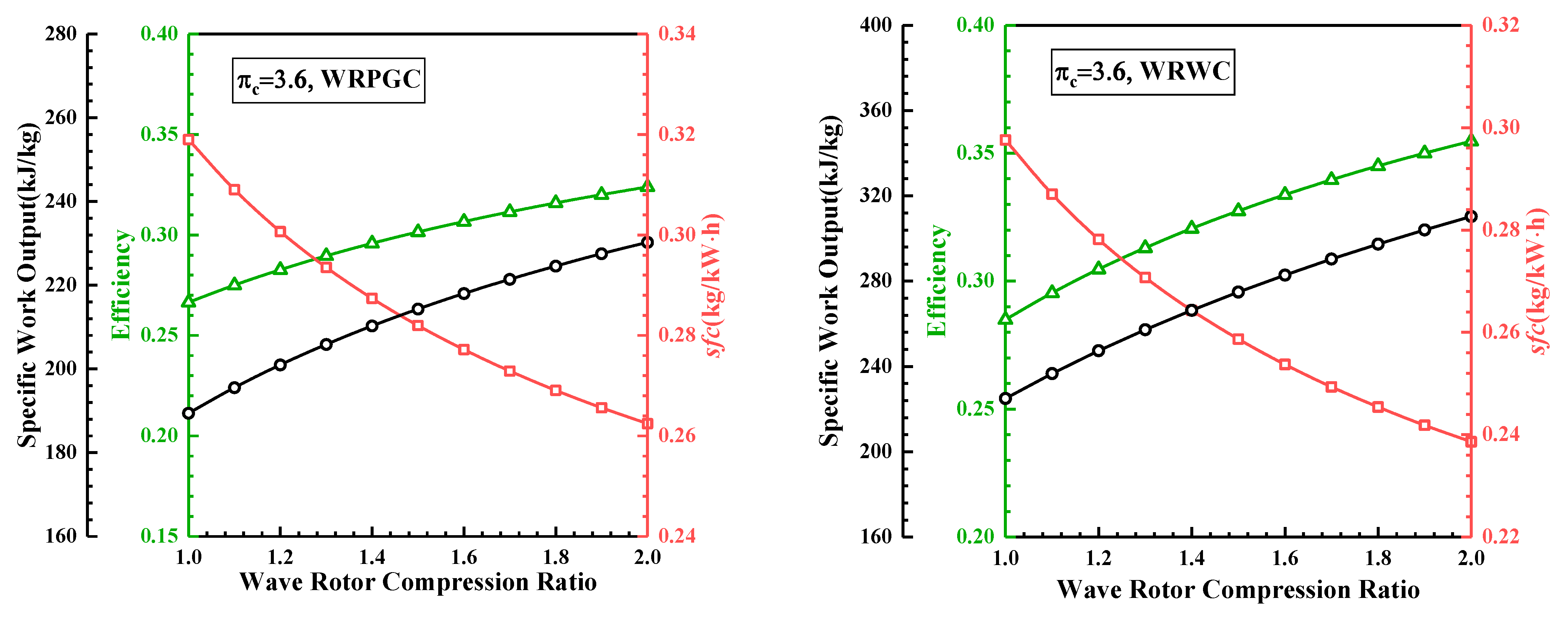
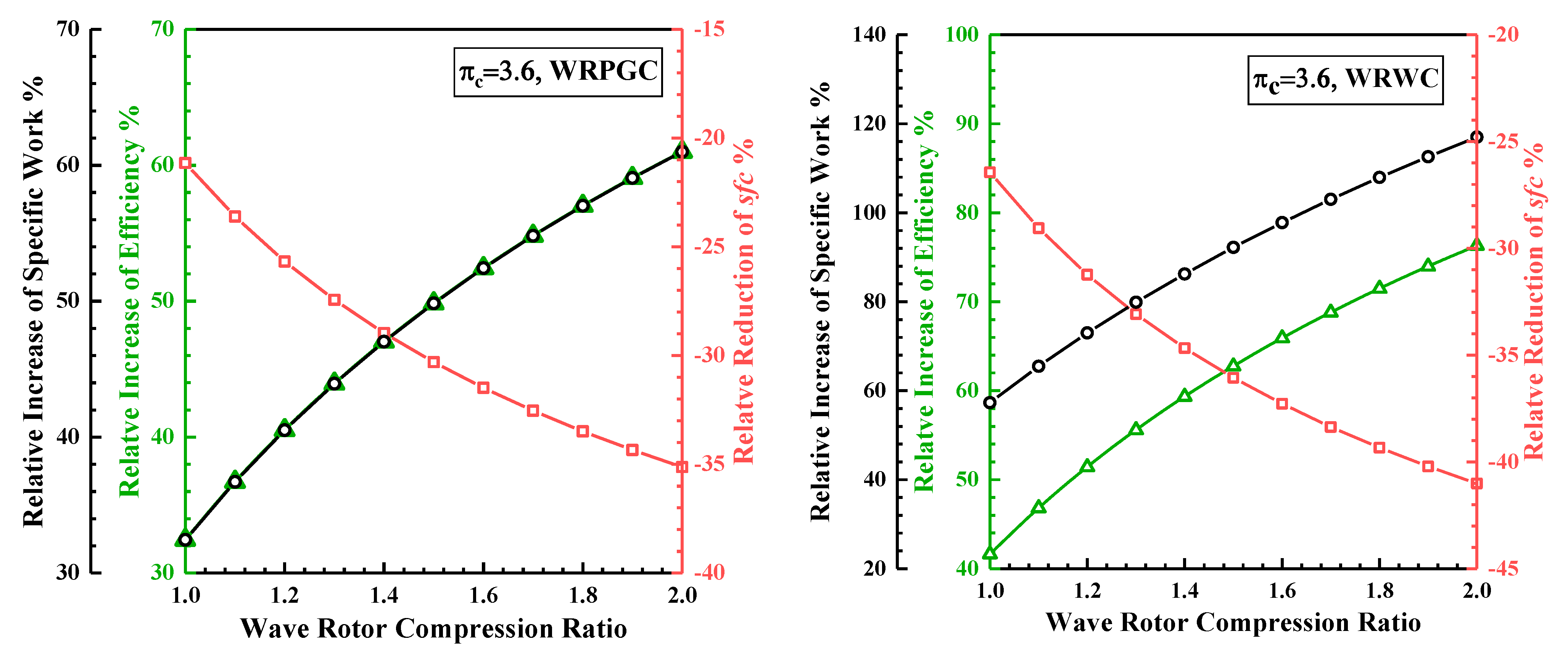
4.2. Performance Influence of Wave Rotor Precompression Ratio
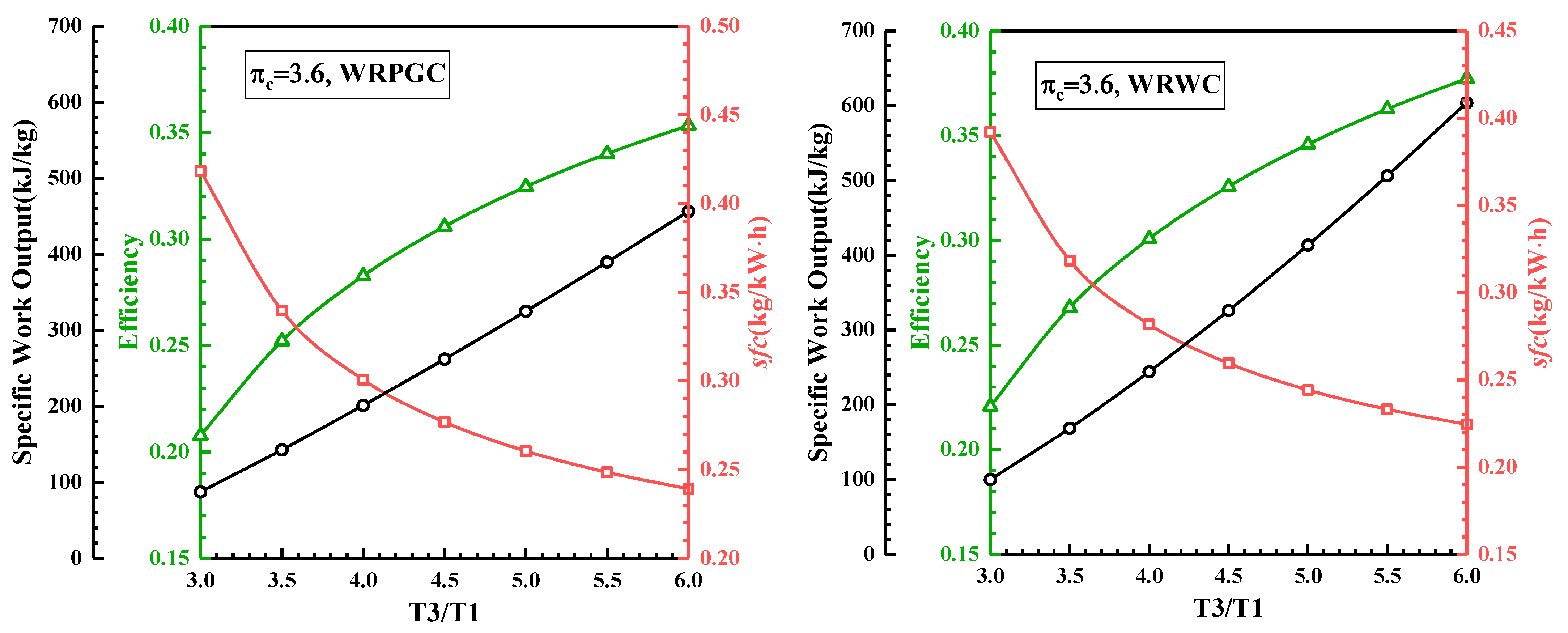
4.3. Performance Influence of Turbine Inlet Temperature
4.4. Performance Influence of Fixed Peak Cycle Temperature
5. Conclusions
- (1)
- Based on the theoretical analysis of the WRC thermodynamic cycle, this study proposes the concepts of the wave rotor as a “wave rotor pressure-gain cycle” and a “wave rotor work cycle”. Performance parameters under different thermodynamic conditions are calculated;
- (2)
- When the compressor pressure ratio exceeds 24.0, the thermal efficiency of the WRC cycle starts to fall below that of the Brayton cycle. For the baseline engine compressor pressure ratio of 3.6, the WRC cycle realizes performance improvements of 40.5% in the pressure-gain cycle and 49.5% in the work cycle, respectively;
- (3)
- When the wave rotor precompression ratio is increased from 1.2 to 1.8, or when the turbine inlet temperature ratio is increased from 4.0 to 6.0, the WRWC has a better performance improvement compared to the WRPGC. Particularly, the increase in work output and the decrease in the specific fuel consumption are more significant with the increase in the turbine inlet temperature;
- (4)
- As the wave rotor peak cycle temperature is fixed, there is a small range of pressure ratios wherein the WRPGC exhibits a better work output and thermodynamic performance compared to the WRWC. The WRPGC is more suitable for integration into low-pressure-ratio baseline engines.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kim, I.; Jin, H.; Ri, K.; Hyon, S.; Huang, C. Design methodology for combustor in advanced gas turbine engines: A review. Aircr. Eng. Aerosp. Technol. 2024, 96, 285–296. [Google Scholar] [CrossRef]
- Ergin, C.C.; Verstraete, T.; Saracoglu, B. The Design and Optimization of Additively Manufactured Radial Compressor of a Miniature Gas Turbine Engine. ASME J. Fluids Eng. 2024, 146, 7. [Google Scholar] [CrossRef]
- Haque, M.A.; Nemitallah, M.A.; Abdelhafez, A.; Mansir, I.B.; Habib, M.A. Review of fuel/oxidizer-flexible combustion in gas turbines. Energy Fuels 2020, 34, 10459–10485. [Google Scholar] [CrossRef]
- Abd El-Maksoud, R.M. Binary Brayton cycle with two isothermal processes. Energy Convers. Manage 2013, 73, 303–308. [Google Scholar]
- Jiang, Y.; Liese, E.; Zitney, S.E.; Bhattacharyya, D. Optimal design of microtube recuperators for an indirect supercritical carbon dioxide recompression closed Brayton cycle. Appl. Energy 2018, 216, 634–648. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, H.; Han, W.; Bai, W.; Yang, Y.; Yao, M.; Wang, Y. Improved design of supercritical CO, Brayton cycle for coal-fired powerplant. Energy 2018, 155, 1–14. [Google Scholar] [CrossRef]
- Lefbvre A, H. Gas Turbine Combustion; Hemisphere Pubulishing Corporation: New York, NY, USA, 1983; pp. 94–95. [Google Scholar]
- Taamallah, S.; Vogiatzaki, K.; Alzahrani, F.M.; Mokheimer, E.M.; Habib, M.A.; Ghoniem, A.F. Fuel flexibility, stability and emissions in premixed hydrogen-rich gas turbine combustion: Technology, fundamentals, and numerical simulations. Appl. Energy 2015, 154, 1020–1047. [Google Scholar] [CrossRef]
- Koff, B. Gas Turbine Technology Evolution: A Designer’s Perspective. J. Propuls. Power 2004, 20, 577–595. [Google Scholar] [CrossRef]
- Akbari, P.; Nalim, R.; Mueller, N. A review of wave rotor technology and its applications. In Proceedings of the 2004 ASME International Mechanical Engineering Congress, Anaheim, CA, USA, 13–19 November 2004; pp. 1–23. [Google Scholar]
- Kailasanath, K. Review of propulsion applications of detonation waves. AIAA J. 2000, 38, 1698–1708. [Google Scholar] [CrossRef]
- Stathopoulos, P. Comprehensive thermodynamic analysis of the humphrey cycle for gas turbines with pressure gain combustion. Energies 2018, 11, 3521. [Google Scholar] [CrossRef]
- Heiser, W.H.; Pratt, D.T. Thermodynamic Cycle Analysis of Pulse Detonation Engines. J. Propuls. Power 2002, 18, 68–76. [Google Scholar] [CrossRef]
- Chen, L.; Lin, J.; Sun, F.; Wu, C. Efficiency of an Atkinson Engine at Maximum Power Density. Energy Convers. Manag. 1998, 39, 337–341. [Google Scholar] [CrossRef]
- Nalim, M.R.; Paxson, D.E. A Numerical Investigation of Premixed Combustion in Wave Rotors. J. Eng. Gas Turbines Power 1997, 119, 668–675. [Google Scholar] [CrossRef]
- Nalim, M.R. Assessment of Combustion Modes for Internal Combustion Wave Rotors. J. Eng. Gas. Turbines Power 1999, 121, 265–271. [Google Scholar] [CrossRef]
- Goldstein, A.W.; Klapproth, J.F.; Hartmann, M.J. Ideal Performance of Valved-Combustors and Applicability to Several Engine Types. Trans. ASME 1958, 80, 1027–1036. [Google Scholar] [CrossRef]
- Akbari, P.; Nalim, M.R. Review of Recent Developments in Wave Rotor Combustion Technology. J. Propuls. Power 2009, 25, 833–844. [Google Scholar] [CrossRef]
- Smith, C.; Snyder, P.; Emmerson, C.; Nalim, M. Impacts of the Constant Volume Combustor on a Supersonic Turbofan Engine. In Proceedings of the 38th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Indianapolis, IN, USA, 7–10 July 2002. [Google Scholar] [CrossRef]
- Li, H.; Akbari, P.; Nalim, R. Air-Standard Thermodynamic Analysis of Gas Turbine Engines Using Wave Rotor Combustion. In Proceedings of the 43rd AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Cincinnati, OH, USA, 9 July 2007. [Google Scholar]
- Nalim, R.; Li, H.; Akbari, P. Air-Standard Aerothermodynamic Analysis of Gas Turbine Engines with Wave Rotor Combustion. J. Eng. Gas Turbines Power-Trans. ASME 2009, 131, 445–456. [Google Scholar] [CrossRef]
- Antonios, F. Gas turbine performance enhancement for naval ship propulsion using wave rotors. J. Mar. Eng. Technol. 2022, 21, 297–309. [Google Scholar] [CrossRef]
- Akbari, P.; Agoos, I. Two-Stage Wave Disk Engine Concept and Performance Prediction; SAE Technical Paper; SAE: Warrendale, PA, USA, 2017. [Google Scholar]
- Akbari, P.; Polanka, M.D. Performance of an Ultra-Compact Disk-Shaped Reheat Gas Turbine for Power Generation. In Proceedings of the 2018 Joint Propulsion Conference, Cincinnati, OH, USA, 9–11 July 2018. [Google Scholar]
- Sun, G.; Akbari, P.; Gower, B.; Mueller, N. Thermodynamics of the Wave Disk Engine. In Proceedings of the 48th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Atlanta, GA, USA, 30 July–1 August 2012. [Google Scholar]
- Li, J.Z.; Gong, E.L.; Wen, Q.; Wang, J.H. Effect of internal combustion wave rotor technology on performance of gas turbine engine. J. Aerosp. Power 2012, 27, 1928–1934. [Google Scholar]
- Briones, A.M.; Sekar, B.; Erdmann, T. Effects of Centrifugal Force on Flame Propagation Characteristics of Wave Rotor Combustor. J. Propuls. Technol. 2022, 43, 269–275. [Google Scholar]
- Gong, E.; Li, J.; Liu, B.; Han, Q. Analysis of Wave Rotor Combustor Leakage and Seal Problems. J. Propuls. Technol. 2016, 37, 1952–1957. [Google Scholar]
- Li, J.; Gong, E.; Yuan, L.; Li, W.; Zhang, K. Experimental investigation on pressure rise characteristics in an ethylene fueled wave rotor combustor. Energy Fuels 2017, 31, 10165–10177. [Google Scholar] [CrossRef]
- Paxson, D.; Kaemming, T. Foundational Performance Analyses of Pressure Gain Combustion Thermodynamic Benefits for Gas Turbines. In Proceedings of the 50th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, Nashville, TN, USA, 9–12 January 2012. [Google Scholar]
- Paxson, D.E.; Kaemming, T. Influence of Unsteadiness on the Analysis of Pressure Gain Combustion Devices. J. Propuls. Power 2014, 30, 377–383. [Google Scholar] [CrossRef]
- Wilson, J.; Paxson, D.E. Jet Engine Performance Enhancement Through Use of a Wave-Rotor Topping Cycle; Nasa Tm 4486; NASA: Washington, DC, USA, 1993.
- Jagannath, R.; Bane, S.P.; Feyz, M.E.; Nalim, M.R. Assessment of Incidence Loss and Shaft Work Production for Wave Rotor Combustor with Non-Axial Channels. In Proceedings of the 55th AIAA Aerospace Sciences Meeting, American Institute of Aeronautics and Astronautics, Grapevine, TX, USA, 9–13 January 2017. [Google Scholar]
- Hawthorne, W.R. R. Tom Sawyer Award Lecture: Reflections on United Kingdom Aircraft Gas Turbine History. J. Eng. Gas Turbines Power 1994, 116, 495–510. [Google Scholar] [CrossRef]
- Shen, W.; Tong, J. Engineering Thermodynamics; Higher Education Press: Beijing, China, 2007. [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, R.; Gong, E.; Li, J.; Yao, Q.; Nie, Z. Performance Analysis of Wave Rotor Combustor Integration into Baseline Engines: A Comparative Study of Pressure-Gain and Work Cycles. Energies 2024, 17, 2074. https://doi.org/10.3390/en17092074
Zheng R, Gong E, Li J, Yao Q, Nie Z. Performance Analysis of Wave Rotor Combustor Integration into Baseline Engines: A Comparative Study of Pressure-Gain and Work Cycles. Energies. 2024; 17(9):2074. https://doi.org/10.3390/en17092074
Chicago/Turabian StyleZheng, Renchuan, Erlei Gong, Jianzhong Li, Qian Yao, and Zhaolong Nie. 2024. "Performance Analysis of Wave Rotor Combustor Integration into Baseline Engines: A Comparative Study of Pressure-Gain and Work Cycles" Energies 17, no. 9: 2074. https://doi.org/10.3390/en17092074
APA StyleZheng, R., Gong, E., Li, J., Yao, Q., & Nie, Z. (2024). Performance Analysis of Wave Rotor Combustor Integration into Baseline Engines: A Comparative Study of Pressure-Gain and Work Cycles. Energies, 17(9), 2074. https://doi.org/10.3390/en17092074






