Study on the Influence of Circulating Water Bypass on the Thermal and Anti-Freezing Characteristics of High-Level Wet Cooling Tower
Abstract
1. Introduction
2. Models and Methods
2.1. Model Geometry
2.2. Numerical Model
2.2.1. Heat and Mass Transfer Model
2.2.2. Boundary Conditions and Solution Methods
3. Results and Discussion
3.1. Impact of Water Bypass Percentage on the Cooling Performance
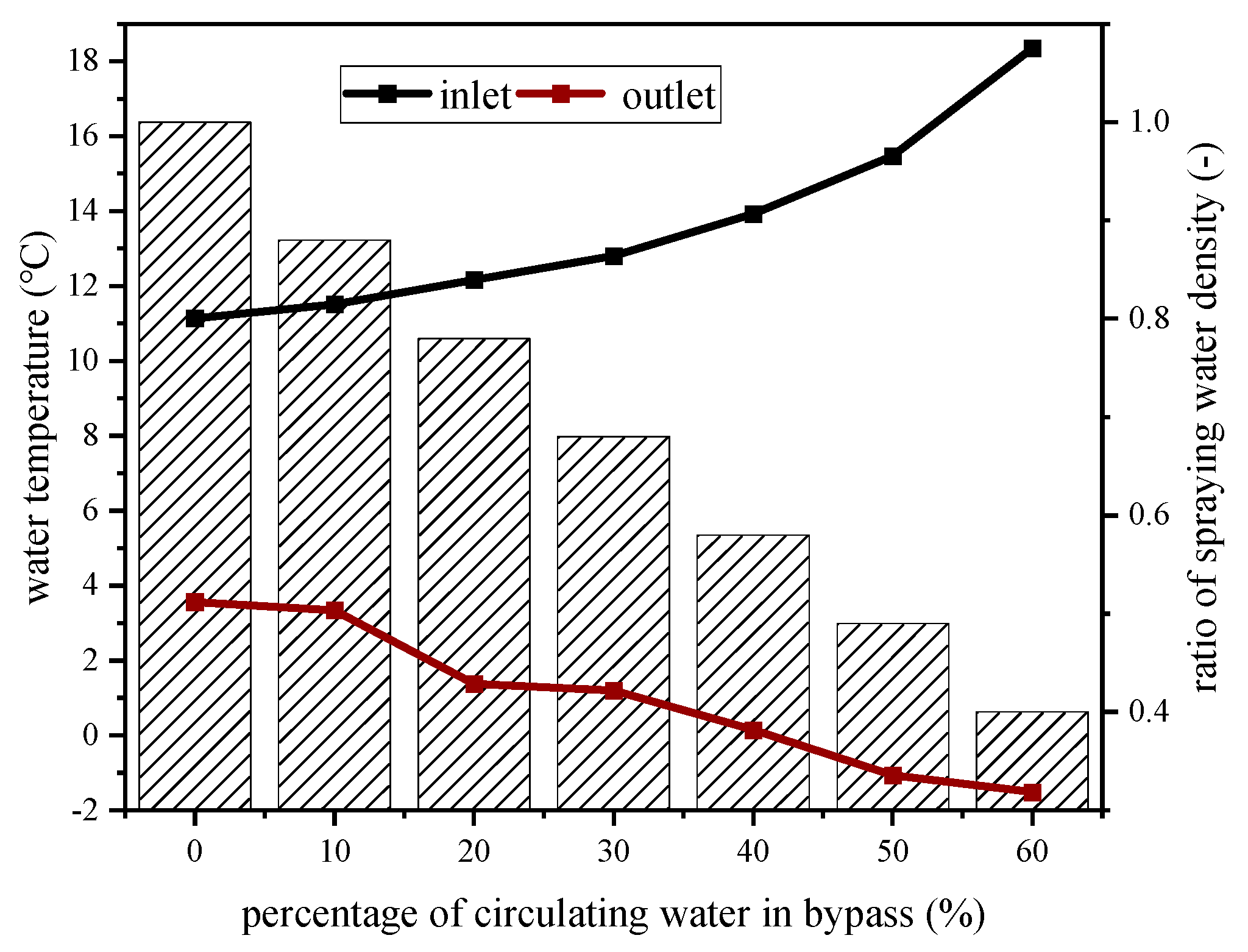
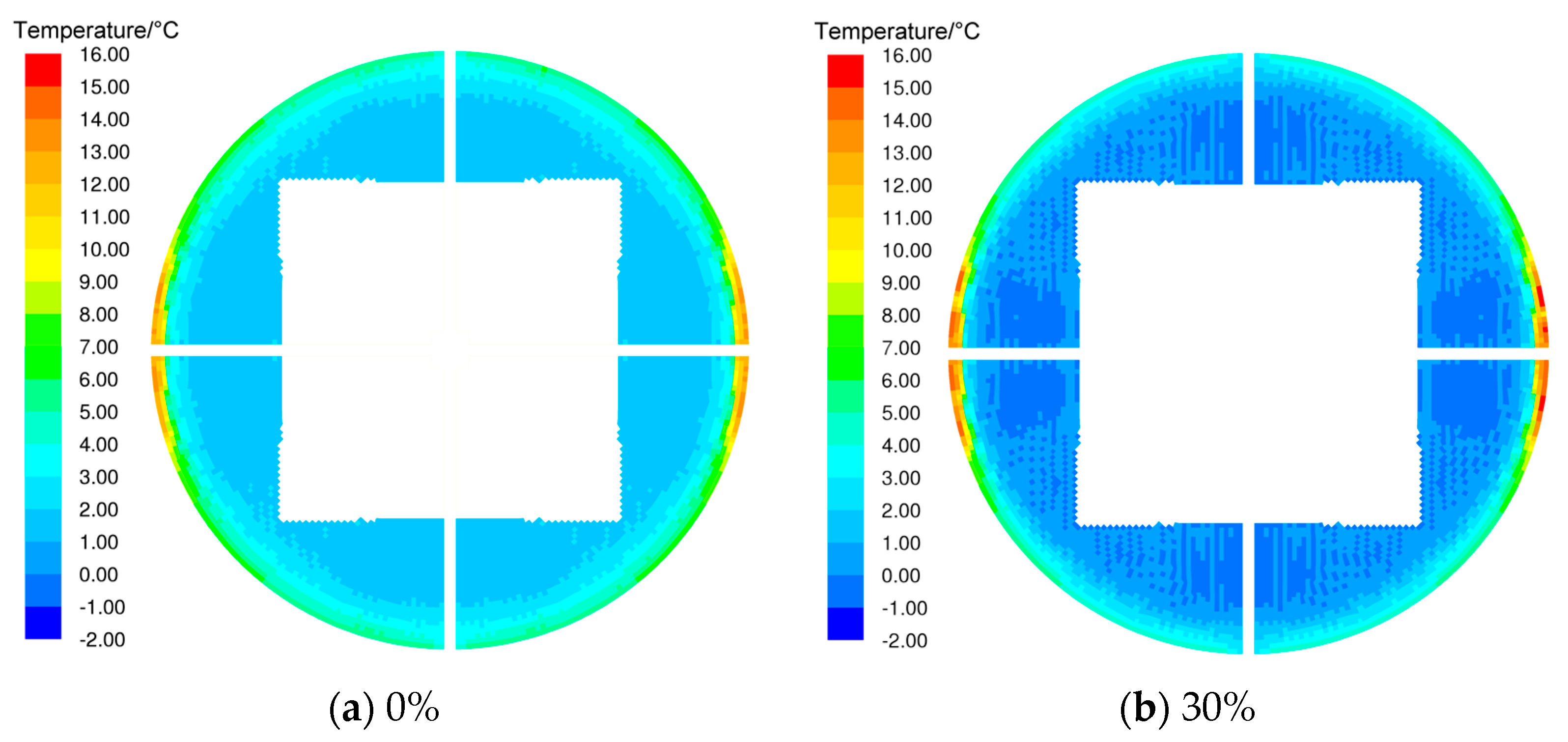
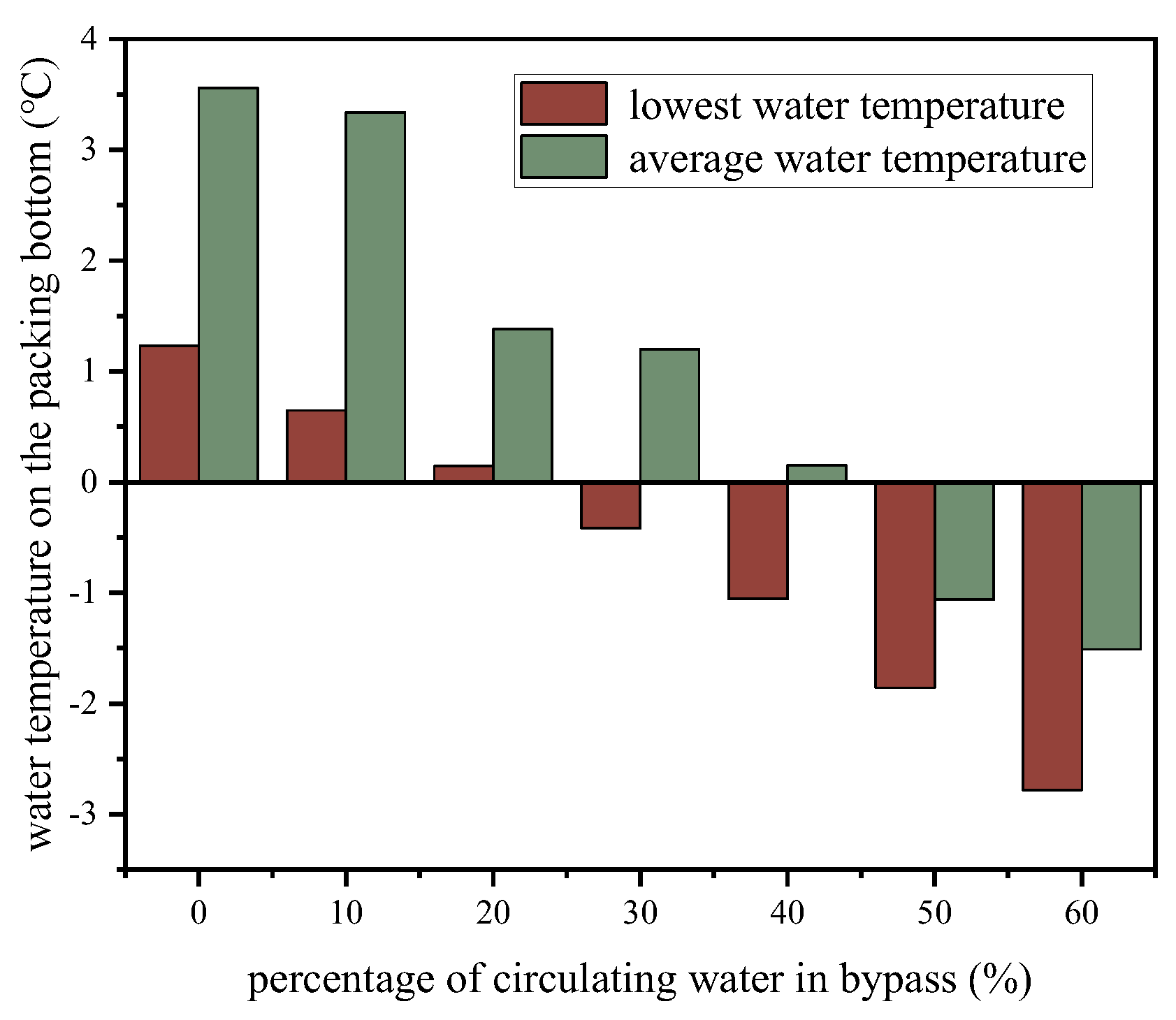
3.2. Impact of Water Bypass Percentage on the Anti-Freezing Characteristics
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lu, H.; Huang, S.; Li, H.; Cheng, Z.; Chang, X.; Dong, L.; Kong, D.; Jing, X. Numerical Simulation of Combustion Characteristics in a 660 MW Tangentially Fired Pulverized Coal Boiler Subjected to Peak-Load Regulation. Case Stud. Therm. Eng. 2023, 49, 103168. [Google Scholar] [CrossRef]
- Ezhov, V.; Zakharov, A.; Ezhova, T.; Kravchenko, S.; Amelin, V. Features of Calculation Technique of the Cooling Tower in the Winter Period Taking into Account the Desigh of the Head Wall. IOP Conf. Ser. Mater. Sci. Eng. 2020, 1001, 012066. [Google Scholar] [CrossRef]
- Ma, L.; Ren, J. The Influence and Analysis of Natural Crosswind on Cooling Characteristics of the High Level Water Collecting Natural Draft Wet Cooling Tower. In Proceedings of the 2017 3rd International Conference on Environmental Science and Material Application (ESMA2017), Singapore, 28–30 July 2017; IoP Publishing: Bristol, UK, 2018; Volume 108, p. 052116. [Google Scholar]
- Goshayshi, H.R.; Missenden, J.F. The Investigation of Cooling Tower Packing in Various Arrangements. Appl. Therm. Eng. 2000, 20, 69–80. [Google Scholar] [CrossRef]
- Shahali, P.; Rahmati, M.; Alavi, S.R.; Sedaghat, A. Experimental Study on Improving Operating Conditions of Wet Cooling Towers Using Various Rib Numbers of Packing. Int. J. Refrig. 2016, 65, 80–91. [Google Scholar] [CrossRef]
- Yang, J.; Gao, M.; Wang, M.; Wang, W.; He, S.; Jiang, G.; Sun, G. Synergistic Optimization of Partition Water Distribution, Non-Equidistant Fillings and Dry-Wet Hybrid Rain Zone for Wet Cooling Towers. Appl. Therm. Eng. 2023, 231, 120940. [Google Scholar] [CrossRef]
- Gilani, N.; Parpanji, F. Parametric Study on the Outlet Water Temperature in a Shower Cooling Tower and Its Application in Different Iranian Provincial Capitals. Int. J. Therm. Sci. 2018, 124, 174–186. [Google Scholar] [CrossRef]
- Al-Waked, R.; Behnia, M. Enhancing Performance of Wet Cooling Towers. Energy Convers. Manag. 2007, 48, 2638–2648. [Google Scholar] [CrossRef]
- Chen, Y.L.; Shi, Y.F.; Hao, J.G.; Chang, H.; Sun, F.Z. Crosswinds Effect on the Thermal Performance of Wet Cooling Towers Under Variable Operating Conditions. In Proceedings of the 2017 International Conference on Power and Energy Engineering, Ottawa, ON, Canada, 13–15 September 2017; IOP Conference Series: Earth and Environmental Science. IOP Publishing: Bristol, UK, 2018; Volume 114, p. 012007. [Google Scholar]
- Chen, G.; Zhao, Y.; Li, W.; Ge, W. The Efficiency of High-Level Water Collecting Cooling Tower with the Installation of Cross Wall Affect by the Evolution of Aerodynamic Field. Appl. Therm. Eng. 2019, 161, 114181. [Google Scholar] [CrossRef]
- Zhao, S.-A.; Wang, M.-R.; Li, L.-J.; Du, C.-Q. Effect of Cross Wind on Performances of Natural Draft Counterflow Cooling Tower Outlet. Heat Transf. Eng. 2017, 38, 1108–1116. [Google Scholar] [CrossRef]
- Mondal, P.K.; Mukherjee, S.; Kundu, B.; Wongwises, S. Investigation of the Crosswind-Influenced Thermal Performance of a Natural Draft Counterflow Cooling Tower. Int. J. Heat Mass Transf. 2015, 85, 1049–1057. [Google Scholar] [CrossRef]
- Alavi, S.R.; Rahmati, M. Experimental Investigation on Thermal Performance of Natural Draft Wet Cooling Towers Employing an Innovative Wind-Creator Setup. Energy Conv. Manag. 2016, 122, 504–514. [Google Scholar] [CrossRef]
- Rahmati, M.; Alavi, S.R.; Sedaghat, A. Thermal Performance of Natural Draft Wet Cooling Towers under Cross-Wind Conditions Based on Experimental Data and Regression Analysis. In Proceedings of the 2016 6th Conference on Thermal Power Plants (CTPP), Tehran, Iran, 19–20 January 2016; IEEE: New York, NY, USA, 2016; pp. 1–5. [Google Scholar]
- Al-Waked, R. Crosswinds Effect on the Performance of Natural Draft Wet Cooling Towers. Int. J. Therm. Sci. 2010, 49, 218–224. [Google Scholar] [CrossRef]
- Sharifullin, V.N.; Badriev, A.I. Aerodynamic Characteristics of the Cooling Tower under the Nonuniform Distribution of the Water and Air Flows. Therm. Eng. 2019, 66, 569–574. [Google Scholar] [CrossRef]
- Williamson, N.; Armfield, S.; Behnia, M. Numerical Simulation of Flow in a Natural Draft Wet Cooling Tower—The Effect of Radial Thermofluid Fields. Appl. Therm. Eng. 2008, 28, 178–189. [Google Scholar] [CrossRef]
- Li, H.-W.; Duan, W.-B.; Wang, S.-B.; Zhang, X.-L.; Sun, B.; Hong, W.-P. Numerical Simulation Study on Different Spray Rates of Three-Area Water Distribution in Wet Cooling Tower of Fossil-Fuel Power Station. Appl. Therm. Eng. 2018, 130, 1558–1567. [Google Scholar] [CrossRef]
- Zhang, D.; Zhang, Z.; Han, Q.; Wu, F.; He, S.; Gao, M. Numerical Simulation on Synergetic Optimization of Non-Equidistant Fillings and Non-Uniform Water Distribution for Wet Cooling Towers. Int. J. Heat Mass Transf. 2021, 179, 121676. [Google Scholar] [CrossRef]
- Li, J.-Y.; Wang, H.; Chen, Z.-C.; Sheng, W. The full model numerical simulation of super large-scale cooling tower for partition water distribution system. J. Eng. Thermophys. 2016, 37, 1888–1894. (In Chinese) [Google Scholar]
- Dong, H.; Wan, D.; Liu, M.; Chen, T.; Gao, S.; Zhao, Y. Evaluation of the Hot Air Recirculation Effect and Relevant Empirical Formulae Applicability for Mechanical Draft Wet Cooling Towers. Energies 2020, 13, 3347. [Google Scholar] [CrossRef]
- Zhao, Y.; Sun, F.; Li, Y.; Long, G.; Yang, Z. Numerical Study on the Cooling Performance of Natural Draft Dry Cooling Tower with Vertical Delta Radiators under Constant Heat Load. Appl. Energy 2015, 149, 225–237. [Google Scholar] [CrossRef]
- Wang, Z.; Yue, Z.; Wang, W.; Ma, C.; Li, X.; Guo, C.; Zhao, Y. Anti-Freezing Study of High-Level Water-Collecting Natural Draft Wet-Cooling Tower Based on Its Water Temperature Distribution Characteristics. Energies 2024, 17, 651. [Google Scholar] [CrossRef]
- Zheng, S.; Jin, T.; Fan, J. Numerical Study of Natural Draft Wet Cooling Tower. In Proceedings of the Mems, Nano and Smart Systems, PTS 1–6; Yuan, L., Ed.; Trans Tech Publications Ltd.: Stafa, Switzerland, 2012; Volume 403–408, pp. 3191–3194. [Google Scholar]
- Al-Waked, R.; Behnia, M. CFD Simulation of Wet Cooling Towers. Appl. Therm. Eng. 2006, 26, 382–395. [Google Scholar] [CrossRef]
- Chen, G.; Zhao, Y.; Ge, W.; Li, W. Critical Guidelines to Cope with the Adverse Impacts of the Inner Peripheral Vortex in the High-Level Water Collecting Natural Draft Wet Cooling Tower. Appl. Therm. Eng. 2020, 168, 114819. [Google Scholar] [CrossRef]
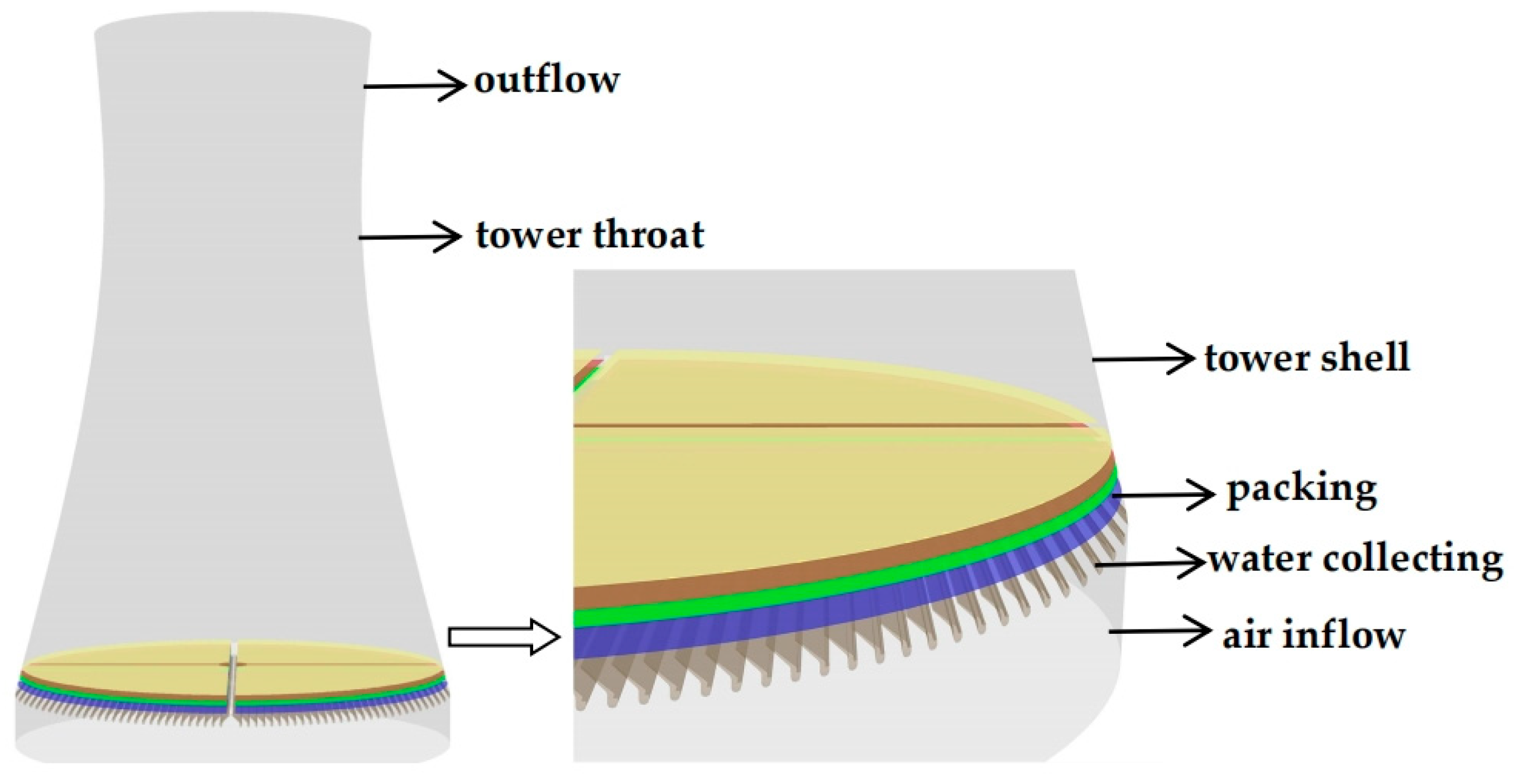
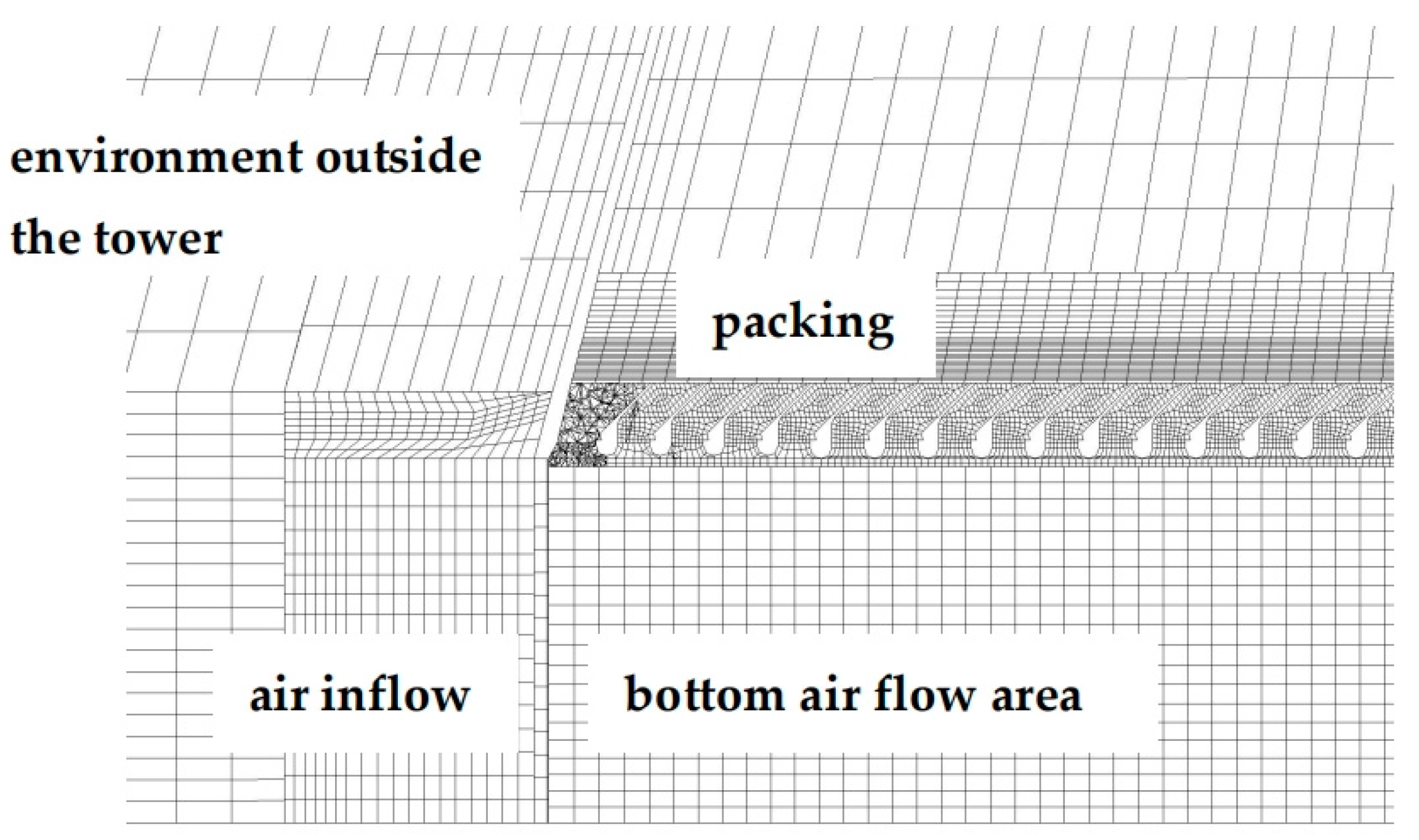
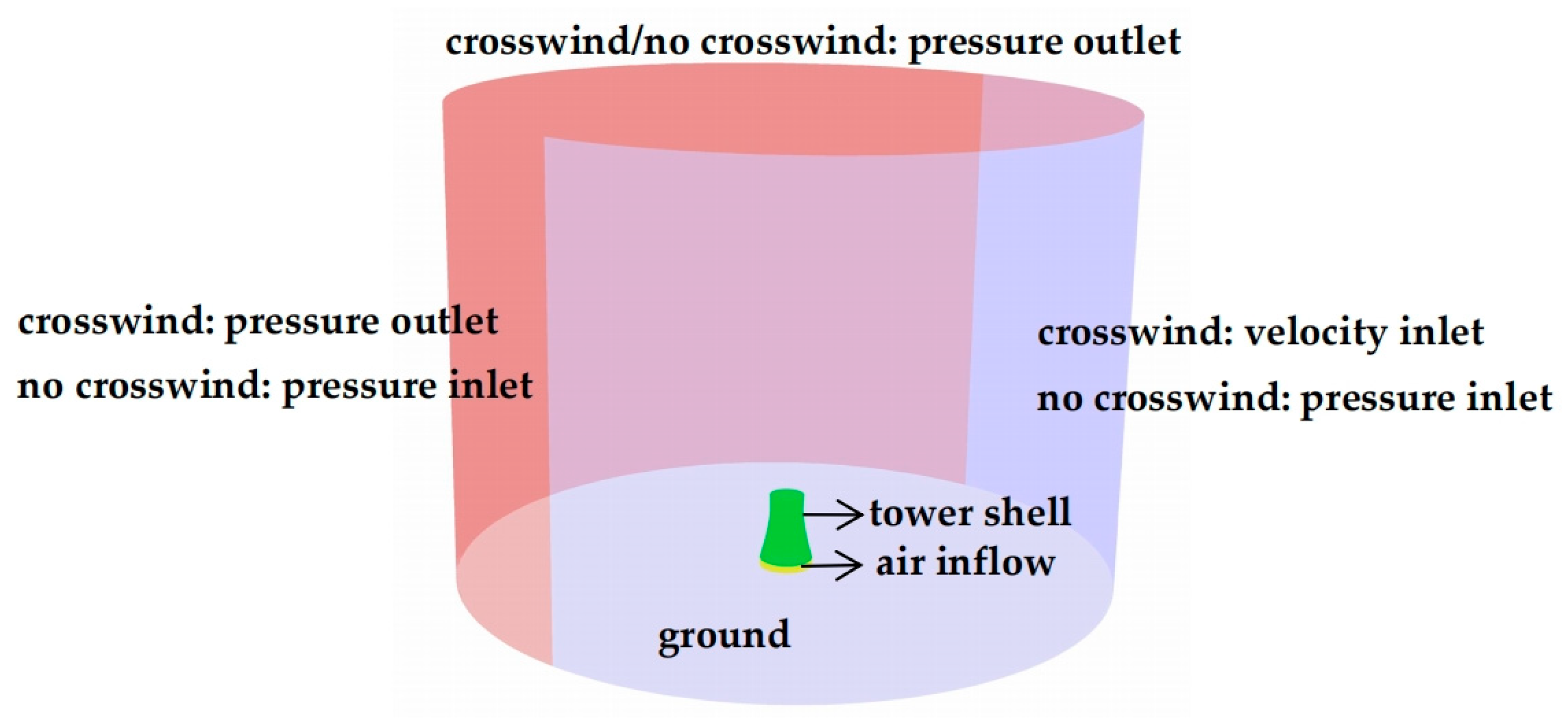


| Projects | Unit | Realm |
|---|---|---|
| Area available for water spraying | m2 | 9000 |
| Cooling tower top elevation | m | 158.22 |
| Throat elevation | m | 126.58 |
| Packing top elevation | m | 18.05 |
| Packing bottom elevation | m | 16.55 |
| Height of air inlet | m | 13.55 |
| Diameter of zero-meter layer | m | 121.66 |
| Throat diameter | m | 71.68 |
| Diameter of air outlet at the top of the tower | m | 73.57 |
| Radius of top layer of packing | m | 55.07 |
| Radius of bottom layer of packing | m | 55.45 |
| Grid Number | 2.8 Million | 3.5 Million | 4.3 Million |
|---|---|---|---|
| Outlet water temperature, °C | 15.38 | 15.35 | 15.35 |
| Absolute error, °C | 0.15 | 0.12 | 0.12 |
| Circulating water temperature drop, °C | 8.67 | 8.67 | 8.67 |
| Operation Conditions | C1 | C2 |
|---|---|---|
| Ambient temperature, °C | 1.2 | 25.4 |
| Relative humidity, % | 68 | 64 |
| Crosswind velocity, m/s | 2.9 | 3.4 |
| Atmosphere pressure, kPa | 102.22 | 100.04 |
| Circulating water flow rate, m3/h | 20,700 | 82,776 |
| Water distribution modes | Outer ring | Full |
| Test/design inlet water temperature, °C | 20.08 | 35.13 |
| Test/design outlet water temperature, °C | 15.12 | 27.12 |
| Circulating water temperature drop, °C | 4.96 | 8.01 |
| Outlet water temperature calculated by numerical model, °C | 14.98 | 27.01 |
| Absolute error, °C | 0.14 | 0.11 |
| Percentage of circulating water in bypass (%) | 0 | 10 | 20 | 30 | 40 | 50 | 60 |
| Air mass flow (kg/s) | 15,324 | 15,221 | 15,198 | 15,084 | 14,957 | 14,845 | 14,752 |
| Percentage of circulating water in bypass (%) | 0 | 10 | 20 | 30 | 40 | 50 | 60 |
| Temperature drop (°C) | 7.58 | 8.17 | 10.78 | 11.60 | 13.78 | 16.53 | 19.86 |
| Mixed temperature (°C) | 3.56 | 4.16 | 3.54 | 4.68 | 5.66 | 7.21 | 10.41 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Yue, Z.; Wang, W.; Ma, C.; Li, X.; Guo, C.; Zhao, Y. Study on the Influence of Circulating Water Bypass on the Thermal and Anti-Freezing Characteristics of High-Level Wet Cooling Tower. Energies 2024, 17, 2073. https://doi.org/10.3390/en17092073
Wang Z, Yue Z, Wang W, Ma C, Li X, Guo C, Zhao Y. Study on the Influence of Circulating Water Bypass on the Thermal and Anti-Freezing Characteristics of High-Level Wet Cooling Tower. Energies. 2024; 17(9):2073. https://doi.org/10.3390/en17092073
Chicago/Turabian StyleWang, Zhonghua, Zenggang Yue, Wei Wang, Chenghui Ma, Xiaoguang Li, Changmin Guo, and Yuanbin Zhao. 2024. "Study on the Influence of Circulating Water Bypass on the Thermal and Anti-Freezing Characteristics of High-Level Wet Cooling Tower" Energies 17, no. 9: 2073. https://doi.org/10.3390/en17092073
APA StyleWang, Z., Yue, Z., Wang, W., Ma, C., Li, X., Guo, C., & Zhao, Y. (2024). Study on the Influence of Circulating Water Bypass on the Thermal and Anti-Freezing Characteristics of High-Level Wet Cooling Tower. Energies, 17(9), 2073. https://doi.org/10.3390/en17092073







