Active Energy Meters Tested in Realistic Non-Sinusoidal Conditions Recorded on the Field and Reproduced in Laboratory †
Abstract
1. Introduction
- Section 2 describes the instrumentation and the methods employed for recording test waveforms and the for the testing of energy meters with either standardised or recorded waveforms in a metrologically traceable framework;
- Section 3 shows the recordings performed at an installation site and the outcome of tests performed on both three-phase and single-phase energy meter models;
- Section 4 discusses the results and the discrepancies between the errors measured under the standardised and the field-recorded conditions.
2. Materials and Methods
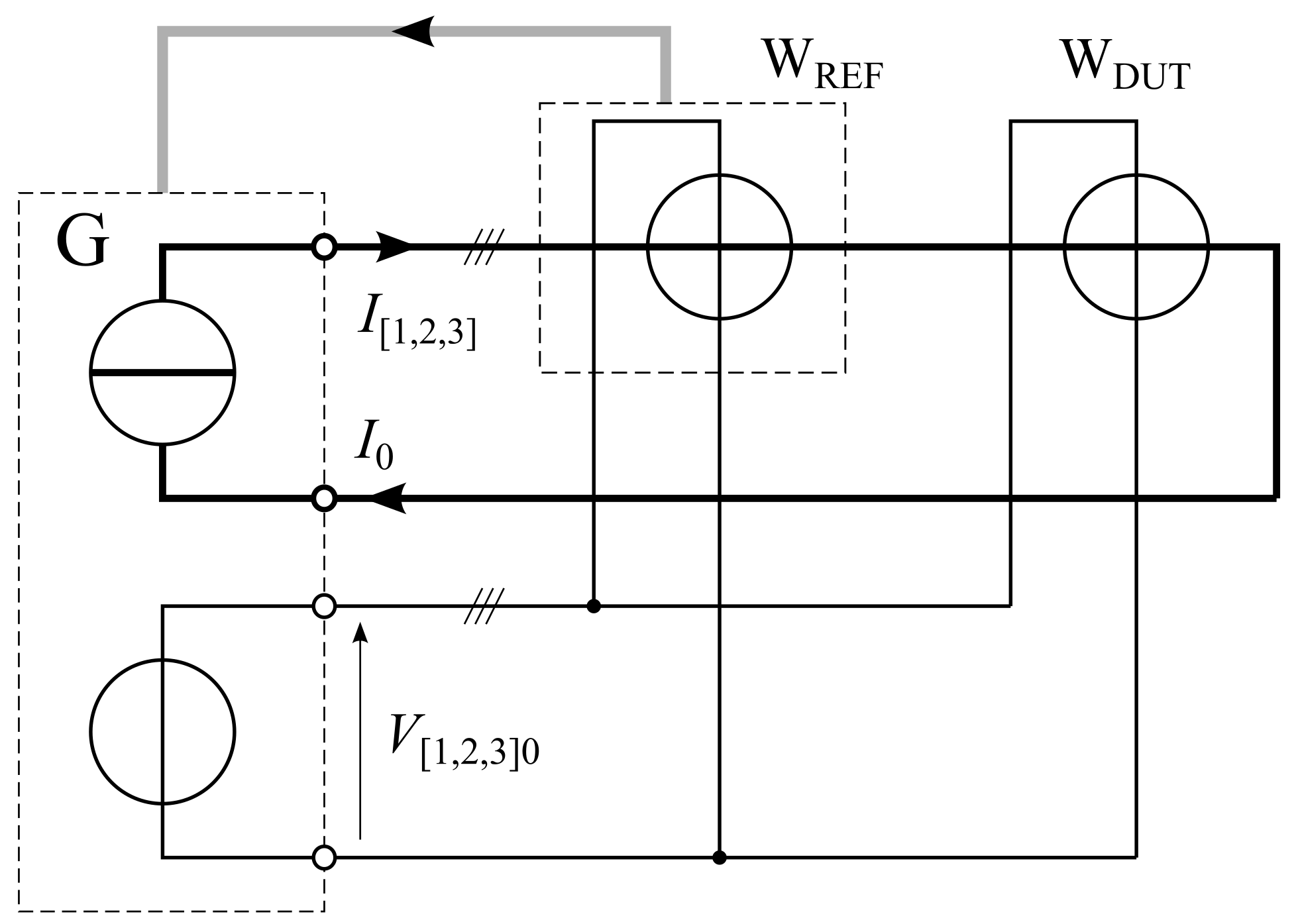
2.1. The Calibration Test Bed
2.2. Meters under Test
2.3. Standardised Sinusoidal and Distorted Waveforms
2.4. On-Field Recorded Waveforms
2.4.1. Recording
2.4.2. Reproduction
3. Results
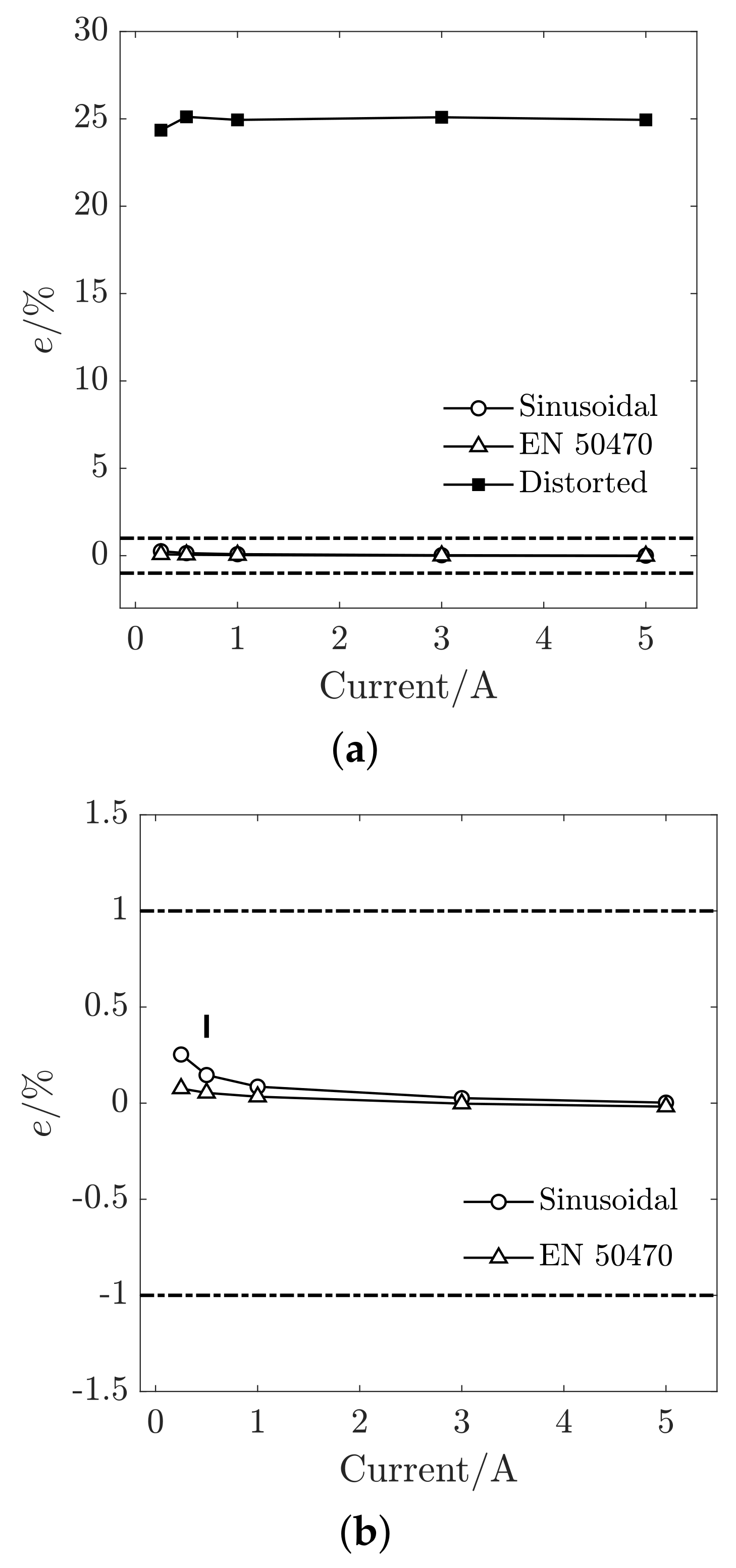
3.1. Tests with Standardised Waveforms
3.2. Tests with On-Field Recorded Waveforms
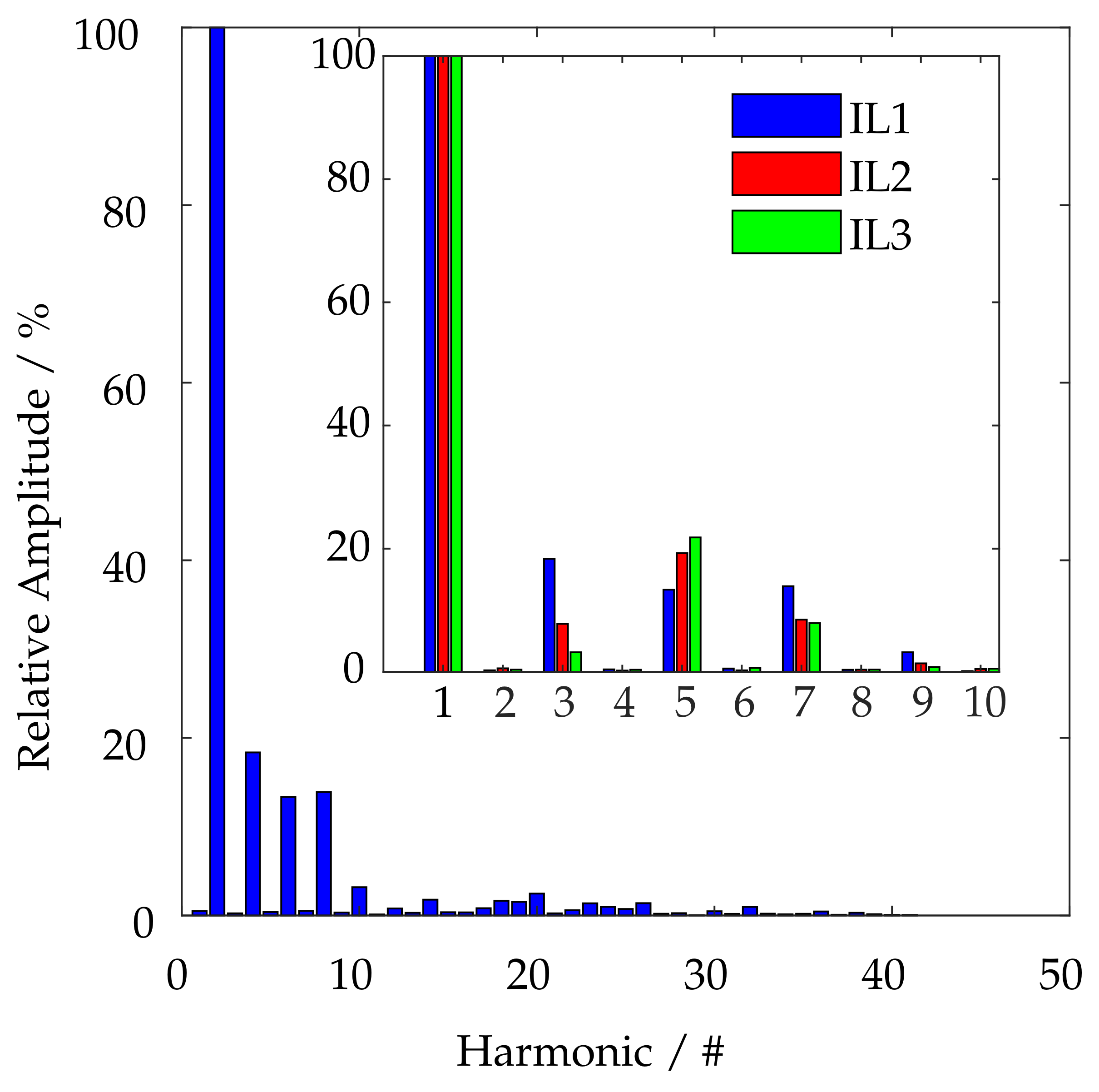
3.2.1. Recorded Waveforms in the Field
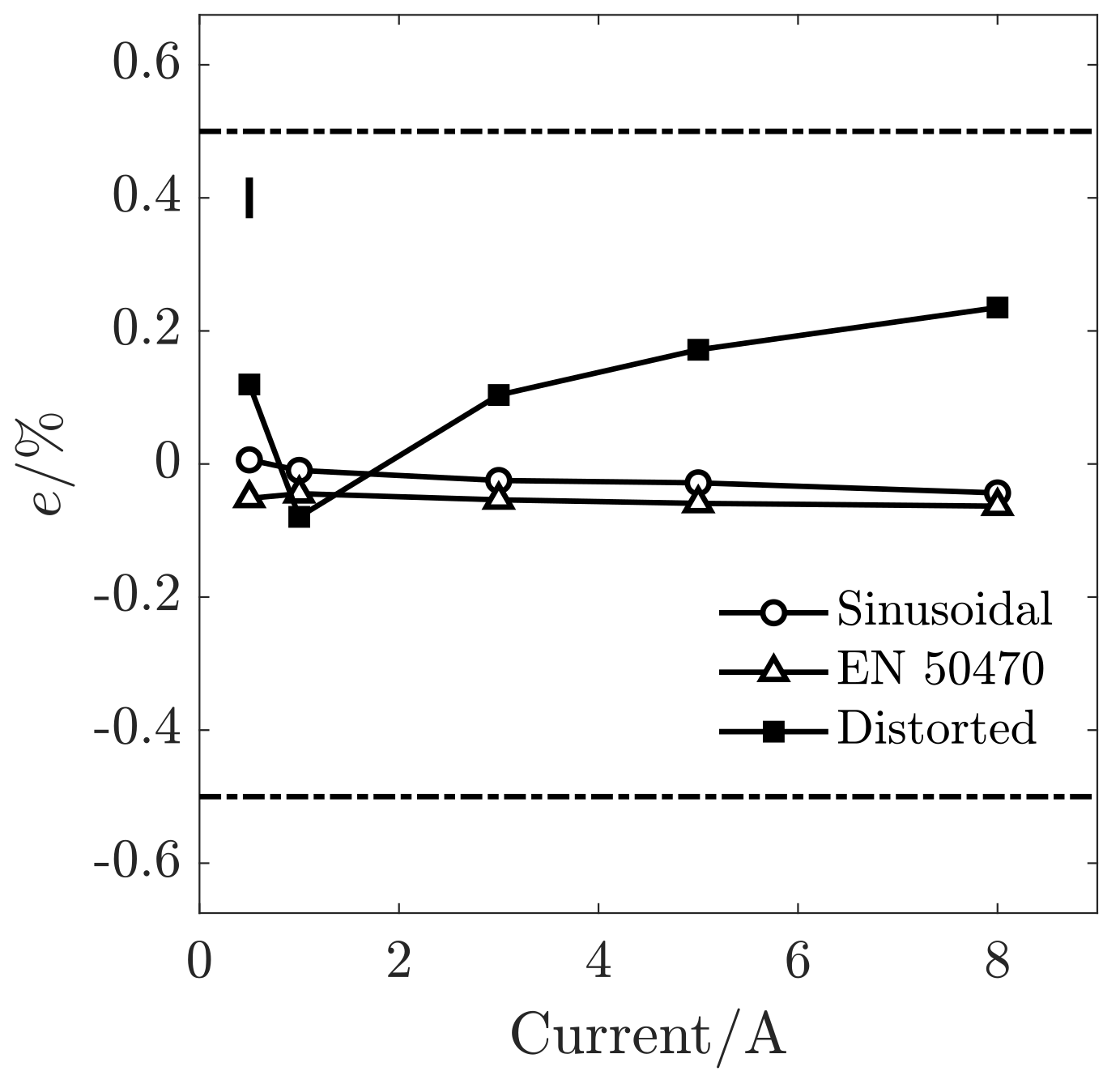
3.2.2. Measurements in the Laboratory
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Bollen, M.H.J. What is power quality? Electr. Power Syst. Res. 2003, 66, 5–14. [Google Scholar] [CrossRef]
- Lumbreras, D.; Gálvez, E.; Collado, A.; Zaragoza, J. Trends in Power Quality, Harmonic Mitigation and Standards for Light and Heavy Industries: A Review. Energies 2020, 13, 5792. [Google Scholar] [CrossRef]
- Salem, W.A.A.; Ibrahim, W.G.; Abdelsadek, A.M.; Nafeh, A.A. Grid connected photovoltaic system impression on power quality of low voltage distribution system. Cogent Eng. 2022, 9, 2044576. [Google Scholar] [CrossRef]
- Silva, J.I.; Sousa, V.; Sarmiento, P.; Gómez, J.R.; Viego, P.R.; Quispe, E.C. Effects of power electronics devices on the energy quality of an administrative building. Int. J. Power Electron. Drive Syst. 2019, 10, 1951. [Google Scholar] [CrossRef]
- Khalid, M.R.; Alam, M.S.; Sarwar, A.; Asghar, M.S.J. A Comprehensive review on electric vehicles charging infrastructures and their impacts on power-quality of the utility grid. eTransportation 2019, 1, 100006. [Google Scholar] [CrossRef]
- Cetina, Q.; Roscoe, R.A.J.; Wright, P.S. Challenges for smart electricity meters due to dynamic power quality conditions of the grid: A review. In Proceedings of the 2017 IEEE International Workshop on Applied Measurements for Power Systems (AMPS), Liverpool, UK, 20–22 September 2017; pp. 1–6. [Google Scholar] [CrossRef]
- Ten Have, B.; Hartman, T.; Moonen, N.; Leferink, F. Misreadings of static energy meters due to conducted EMI caused by fast changing current. In Proceedings of the 2019 Joint International Symposium on Electromagnetic Compatibility, Sapporo and Asia-Pacific International Symposium on Electromagnetic Compatibility (EMC Sapporo/APEMC), Sapporo, Japan, 3–7 June 2019; pp. 445–448. [Google Scholar] [CrossRef]
- Ten Have, B.; Hartman, T.; Moonen, N.; Keyer, C.; Leferink, F. Faulty readings of static energy meters caused by conducted electromagnetic interference from a water pump. Renew. Energy Power Qual. J. 2019, 17, 15–19. [Google Scholar] [CrossRef]
- 2014/32/EU; Directive 2014/32/EU of the European Parliament and of the Council of 26 February 2014 on the Harmonization of the Laws of the Member States Relating to the Making Available on the Market of Measuring Instruments. European Parliament and the Council of the European Union: Brussels, Belgium, 2014.
- EN 50470-1:2006+A1:2018; Electricity Metering Equipment (A.C.)—Part 1: General Requirements, Tests and Test Conditions—Metering Equipment (Class Indexes A, B and C). CENELEC: Brussels, Belgium, 2018.
- EN 50470-3:2022-06; Electricity Metering Equipment (A.C.)—Part 3: Particular Requirements—Static Meters for Active Energy (Class Indexes A, B and C). CENELEC: Brussels, Belgium, 2022.
- EN 62052-11:2021-04; Electricity Metering Equipment—General Requirements, Tests and Test Conditions—Part 11: Metering Equipment. IEC: Geneve, Switzerland, 2021.
- EN 62053-21:2021-04; Electricity Metering Equipment—Particular Requirements—Part 21: Static Meters for AC Active Energy (Classes 0, 5, 1 and 2). IEC: Geneve, Switzerland, 2022.
- CEI 13-71; Sistemi di Misura dell’Energia Elettrica (c.a.)—Guida Alla Composizione, Installazione e Verifica. CEI: Milano, Italy, 2022.
- Quijano Cetina, R.; Seferi, Y.; Blair, S.M.; Wright, P.S. Energy metering integrated circuit behavior beyond standards requirements. Energies 2021, 14, 390. [Google Scholar] [CrossRef]
- Bartolomei, L.; Cavaliere, D.; Mingotti, A.; Peretto, L.; Tinarelli, R. Testing of Electrical Energy Meters Subject to Realistic Distorted Voltages and Currents. Energies 2020, 13, 2023. [Google Scholar] [CrossRef]
- Olencki, A.; Mróz, P. Testing Of Energy Meters Under Three-Phase Determined And Random Nonsinusoidal Conditions. Metrol. Meas. Syst. 2014, 21, 217–232. [Google Scholar] [CrossRef]
- Ferrero, A.; Muscas, C. On the selection of the “best” test waveform for calibrating electrical instruments under nonsinusoidal conditions. IEEE Trans. Instrum. Meas. 2000, 49, 382–387. [Google Scholar] [CrossRef]
- Ferrero, A.; Faifer, M.; Salicone, S. On Testing the Electronic Revenue Energy Meters. IEEE Trans. Instrum. Meas. 2009, 58, 3042–3049. [Google Scholar] [CrossRef]
- Gallo, D.; Landi, C.; Pasquino, N.; Polese, N. A New Methodological Approach to Quality Assurance of Energy Meters Under Nonsinusoidal Conditions. IEEE Trans. Instrum. Meas. 2007, 56, 1694–1702. [Google Scholar] [CrossRef]
- Durante, L.; Ghosh, P.K. Active power measurement in nonsinusoidal environments. IEEE Trans. Power Syst. 2000, 15, 1142–1147. [Google Scholar] [CrossRef]
- van den Brom, H.E.; van Leeuwen, R.; Marais, Z.; ten Have, B.; Hartman, T.; Azpúrua, M.A.; Pous, M.; Kok, G.J.; van Veghel, M.G.; Kolevatov, I.; et al. EMC testing of electricity meters using real-world and artificial current waveforms. IEEE Trans. Electromagn. Compat. 2021, 63, 1865–1874. [Google Scholar] [CrossRef]
- Callegaro, L.; Aprile, G.; Cultrera, A.; Galliana, F.; Germito, G.; Serazio, D.; Trinchera, B. A calibration-verification testbed for electrical energy meters under low power quality conditions. Meas. Sens. 2021, 18, 100188. [Google Scholar] [CrossRef]
- Cultrera, A.; Germito, G.; Serazio, D.; Galliana, F.; Trinchera, B.; Aprile, G.; Chirulli, M.; Callegaro, L. Laboratory reproduction of on-field low power quality conditions for the calibration/verification of electrical energy meters. In Proceedings of the 25th IMEKO TC-4 International Symposium on Measurement of Electrical Quantities, Brescia, Italy, 12–14 September 2022; pp. 29–32. [Google Scholar]
- Djokić, B.; Parks, H. Calibration of Electrical Instruments Under Nonsinusoidal Conditions at NRC Canada. IEEE Trans. Instrum. Meas. 2021, 70, 1–6. [Google Scholar] [CrossRef]
- Yang, S.; Yan, A.Y.K.; Ng, C.M.N. High accuracy and traceable power quality instrument calibration using high-speed digitizing technique. In Proceedings of the Conference on Precision Electromagnetic Measurements (CPEM), Ottawa, ON, Canada, 10–15 July 2016; pp. 1–2. [Google Scholar] [CrossRef]
- Macedo, J.R.; Xavier, G.L.; Gondin, I.N.; Oliveira, L.T.; de Oliveira, R.F. An update on the performance of active energy meters under non-sinusoidal conditions. Electr. Eng. 2020, 102, 1785–1794. [Google Scholar] [CrossRef]


| Label | EN Class | / | / | N. of Phases |
|---|---|---|---|---|
| DUT-1 | C | 1 | 10 | 3 |
| DUT-2 | B | 5 | 6 | 3 |
| DUT-3 | B | 5 | 40 | 1 |
| DUT-4 | B | 5 | 40 | 1 |
| DUT-5 | B | 5 | 65 | 1 |
| DUT-6 | B | 5 | 45 | 1 |
| Phase | UL/V | THDV/% | IL/A | THDI/% | θ/∘ | P/ | Q/kvar | S/kVA |
|---|---|---|---|---|---|---|---|---|
| 1 | 229.97 | 1.23 | 10.57 | 26.89 | 95.09 | −0.205 | −2.293 | 2.431 |
| 2 | 231.52 | 1.42 | 10.26 | 22.38 | 68.55 | 0.842 | −2.155 | 2.376 |
| 3 | 231.19 | 1.21 | 10.41 | 22.74 | 64.04 | 1.22 | −2.107 | 2.407 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cultrera, A.; Germito, G.; Serazio, D.; Galliana, F.; Trinchera, B.; Aprile, G.; Chirulli, M.; Callegaro, L. Active Energy Meters Tested in Realistic Non-Sinusoidal Conditions Recorded on the Field and Reproduced in Laboratory. Energies 2024, 17, 1403. https://doi.org/10.3390/en17061403
Cultrera A, Germito G, Serazio D, Galliana F, Trinchera B, Aprile G, Chirulli M, Callegaro L. Active Energy Meters Tested in Realistic Non-Sinusoidal Conditions Recorded on the Field and Reproduced in Laboratory. Energies. 2024; 17(6):1403. https://doi.org/10.3390/en17061403
Chicago/Turabian StyleCultrera, Alessandro, Gabriele Germito, Danilo Serazio, Flavio Galliana, Bruno Trinchera, Giulia Aprile, Martino Chirulli, and Luca Callegaro. 2024. "Active Energy Meters Tested in Realistic Non-Sinusoidal Conditions Recorded on the Field and Reproduced in Laboratory" Energies 17, no. 6: 1403. https://doi.org/10.3390/en17061403
APA StyleCultrera, A., Germito, G., Serazio, D., Galliana, F., Trinchera, B., Aprile, G., Chirulli, M., & Callegaro, L. (2024). Active Energy Meters Tested in Realistic Non-Sinusoidal Conditions Recorded on the Field and Reproduced in Laboratory. Energies, 17(6), 1403. https://doi.org/10.3390/en17061403






