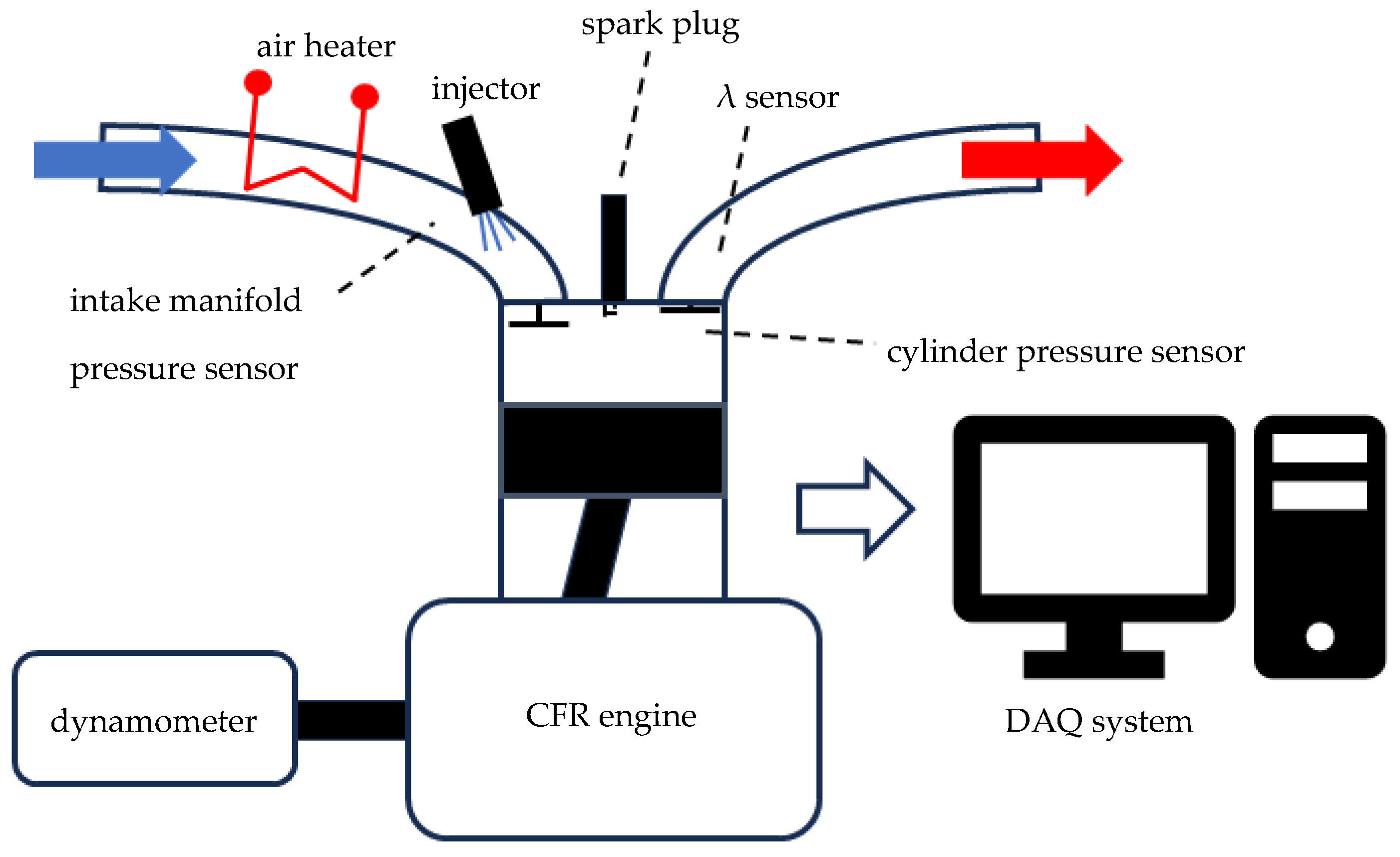
1. Introduction
With more than 1 billion vehicles in the world today [
1] and counting, sustainable fuels could play an important role in the reduction in the carbon footprint of the world’s existing vehicle fleet. In SI engines, one of the most important aspects of fuel quality is its resistance to knock, indicated by the octane number. Knocking combustion occurs when the mixture of air and fuel in front of the advancing flame front undergoes spontaneous ignition. This results in rapid pressure oscillations, resulting in loss of engine power and efficiency, and even posing a risk of engine damage or failure. Knocking combustion is avoided by the engine control unit (ECU), which adapts the spark ignition timing when the onset of knock is detected. Application of knock limited spark advance (KLSA) strategies comes at the cost of engine efficiency, since the spark ignition timing is adjusted to move away from the optimum spark timing for maximum brake torque (MBT). Fuels with higher knock-resistive properties are therefore beneficial for SI engine efficiency.
A fuel’s knock-resistant properties are defined by various parameters, such as chemical structure, latent heat of vaporization (HOV), and laminar flame speed. The operating conditions, often related to the engine load, pressure and temperature in the combustion chamber, as well as engine speed, also affect the tendency for a fuel to knock.
Investigation of the knock phenomenon dates back to the early 1900s. Around 1930, the Cooperative Fuel Research Committee introduced the Cooperative Fuel Research (CFR) engine and the octane scale. This scale assesses the autoignition characteristics of a sample fuel by comparing it to a reference blend with a specified octane number. The reference blends consist of binary combinations of 2,2,4-trimethylpentane (also known as iso-octane) and n-heptane, referred to as primary reference fuels (PRFs). According to the definition, the octane number (ON) of a PRF blend is equivalent to the volumetric ratio of iso-octane in a mixture of iso-octane and n-heptane, as expressed in Equation (1). For instance, a blend comprising 95% iso-octane and 5% n-heptane would have an ON of 95.
Two traditional test methods were developed to assign a fuel’s octane rating. The initial ON development involved the research octane number (RON) test method first, with the motor octane number (MON) test being introduced later. The MON method was developed in response to on-road testing that revealed the inadequacy of RON alone, since some fuels that met RON specifications did not seem to meet performance expectations on the road. The MON test provides additional information on a fuel’s resistance to knocking under different driving conditions by simulating higher-speed and heavier-load engine operation. The main differences between RON and MON conditions are listed in
Table 1. Due to the constant inlet mixture temperature, the MON test cancels the cooling effect of components with a high latent heat of vaporization, making it possible to isolate the antiknock impact of the fuel chemistry.
Generally, gasoline tends to have a higher RON in comparison to its corresponding MON. The disparity between these numbers is defined as “fuel sensitivity” (S), represented by Equation (2).
This phenomenon is rooted in the negative temperature coefficient (NTC) reactivity of paraffinic fuels [
2], including primary reference fuels, influencing their ignition delay as temperatures increase. Contemporary gasolines are composed of a blend of normal-, iso-, and cyclo-paraffins, oxygenates, olefins, and aromatics; the latter two components usually lacking a distinct NTC region, or not having it at all [
3]. As a result, during the MON test, paraffinic fuels exhibit higher resistance to autoignition, leading to higher MON values compared to their oxygenated counterparts with the same RON [
3,
4].
In the RON and MON measurement setup as described in ATSM-D2699 and D-2700, respectively [
5,
6], knock intensity is assessed by analysing the output signal from the so-called detonation pickup-model D1 sensor. This system comprises a magnetorestrictive rod mounted in a coil, generating a voltage in response to changes in pressure within the combustion chamber. The sensor’s signal undergoes processing through a detonation meter, which, in turn, produces a signal for the knock meter—a display indicating the intensity of knock. It is a known fact that this system acts as a low-pass signal filter with a 2.85 kHz limit [
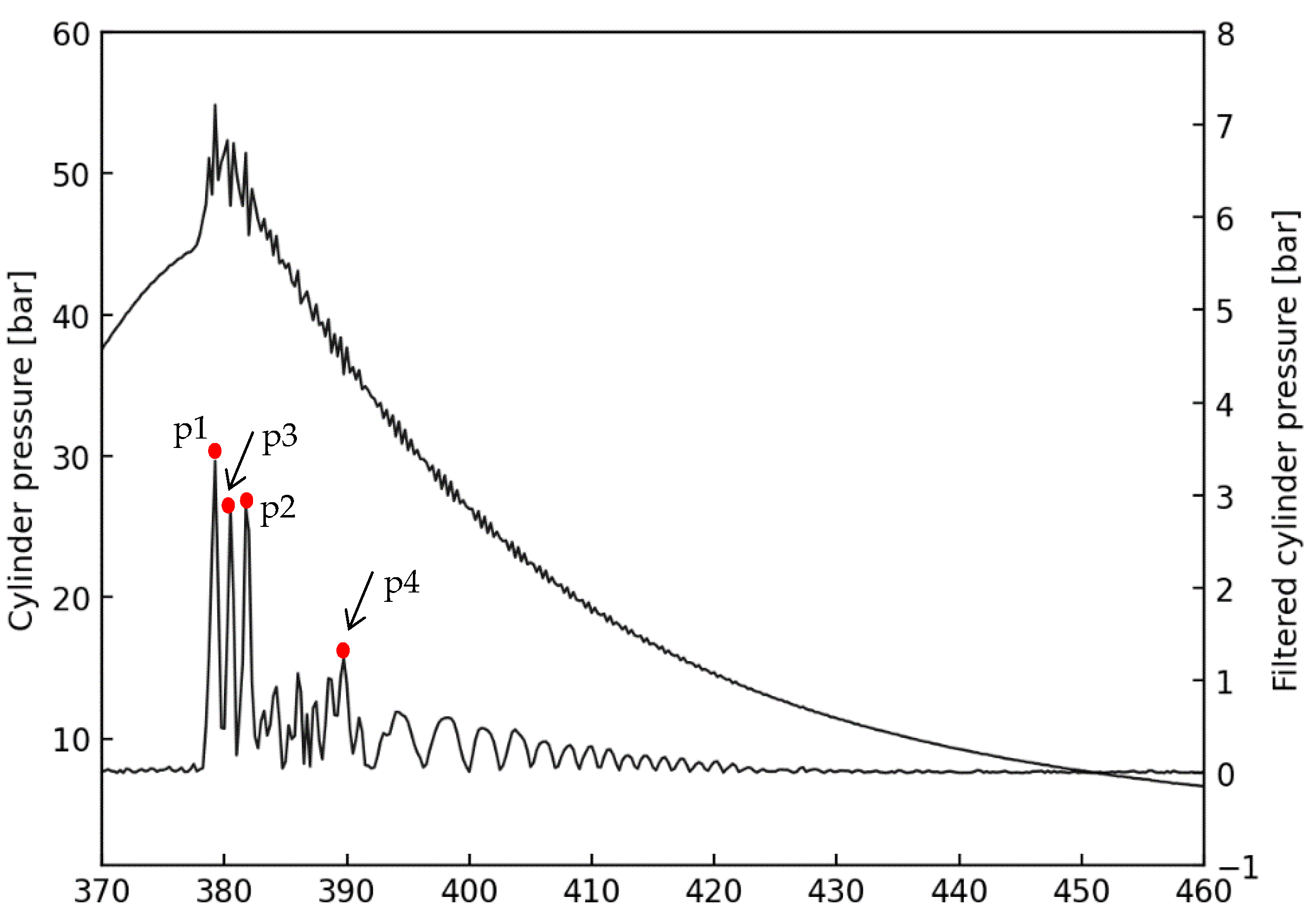
7]. It measures
, the sudden pressure increase at the so-called “knock point” where the cylinder pressure trace bends upwards, moving away from the normal cylinder pressure trace, when knocking combustion occurs as illustrated in
Figure 1.
Arrigoni was one of the first scientists to investigate the knock phenomenon in depth in the 1970s [
8], and they noticed high-frequency pressure oscillations around top dead centre (TDC) when knocking combustion occurred. These oscillations usually occur within a frequency range of 3.5 to 15 kHz and are represented by the pressure spikes in
Figure 1. In a contemporary production SI engine, those high-frequency oscillations cause engine vibrations that are picked up by a so-called knock sensor. The majority of knock sensor technology is based on based on piezo-ceramic material, combined with seismic mass, generating a signal to the engine’s ECU when the sensor is excited by the high-frequency pressure oscillations—not necessarily the
—during knocking combustion. Next to the fact that the D1 pickup system’s frequency spectrum is limited to 2.85 kHz, knock sensors are generally mounted on the engine block surface in contrast to the D1′s mounting position, flush with the combustion chamber wall. Arrigoni’s work indicated that there is a good correlation between the knock meter signal and the high-frequency pressure oscillations, and later work from Yates et al. [
9] revealed an inconsistent correlation between the pressure oscillations and the corresponding octane number for the Research and Motor method reference conditions.
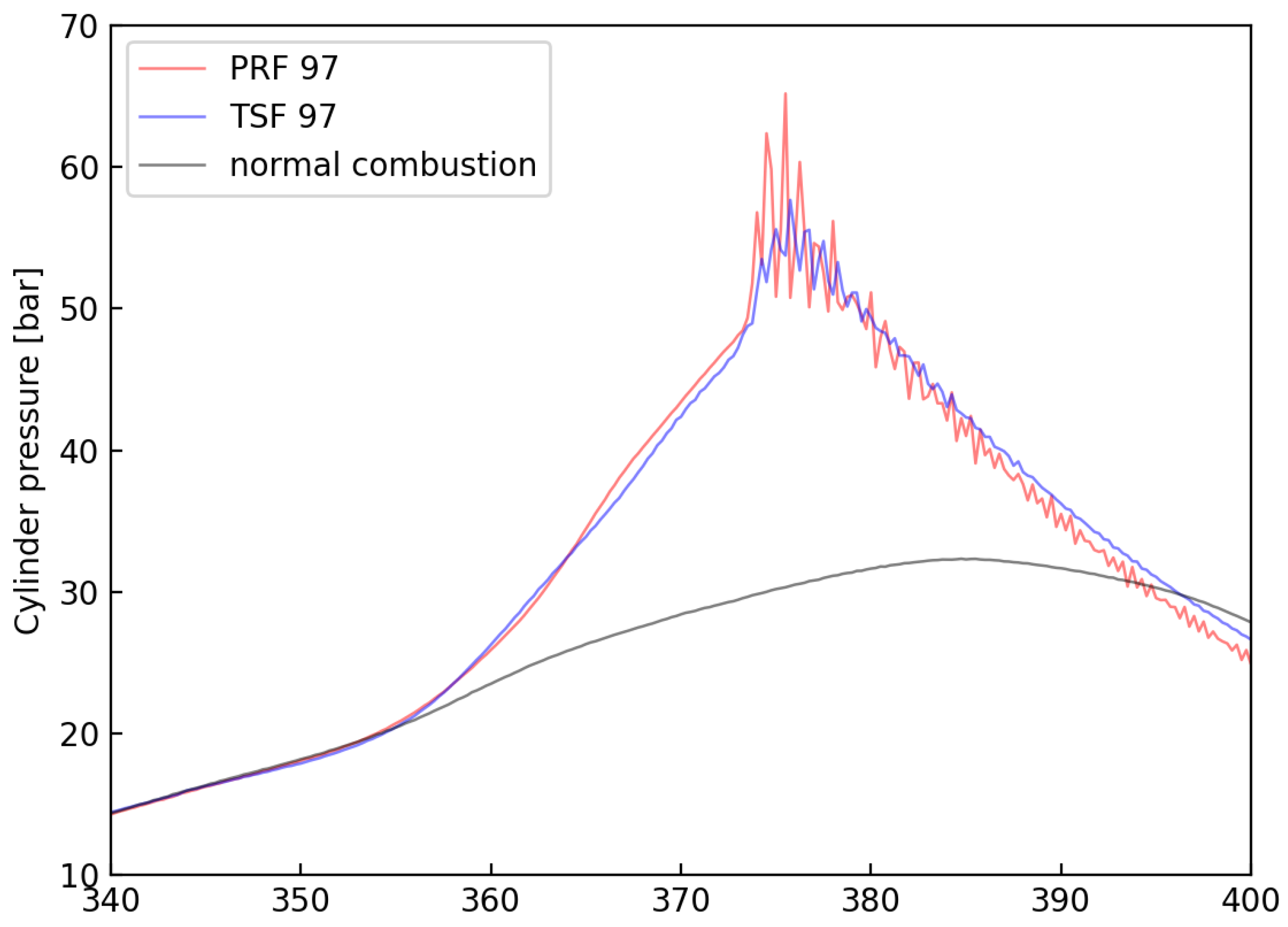
The shortcomings of the ASTM RON/MON methodology further become evident in
Figure 2, which illustrates a knocking combustion cycle of a PRF and a toluene standardization fuel (TSF), both sharing the same RON of 97. Both fuels show the same
(identical RON) after the knock point, but feature a different oscillation intensity. In this case, the engine’s knock sensor would “measure” another kind of “RON” than what would be measured by the D1 pickup.
The inconsistency between the knock intensity signal from the knock sensor and the conditions used for rating fuels for octane values suggests a potential difference between octane rating and the knock limit typically detected by a modern knock sensor [
10]. Additionally, the conditions under which the RON and MON tests are conducted diverge considerably from those found in a modern-day engine. This further supports the argument that RON and MON are not reliable indicators of knock resistance for contemporary engines.
In the context of a pioneering second-generation SI biofuel initiative [
11,
12], a novel approach to octane rating was devised. This method hinges on the fuel’s oscillation characteristics, aiming to address the limitations of the ASTM octane rating system. This work introduces a novel approach that addresses the traditionally weak correlation between oscillation intensity and ASTM RON, a step not previously taken. The subsequent sections delve into the background of knock intensity, present the methodology description of past work, and discuss the results obtained with an improved method using different reference fuels.
3. Results
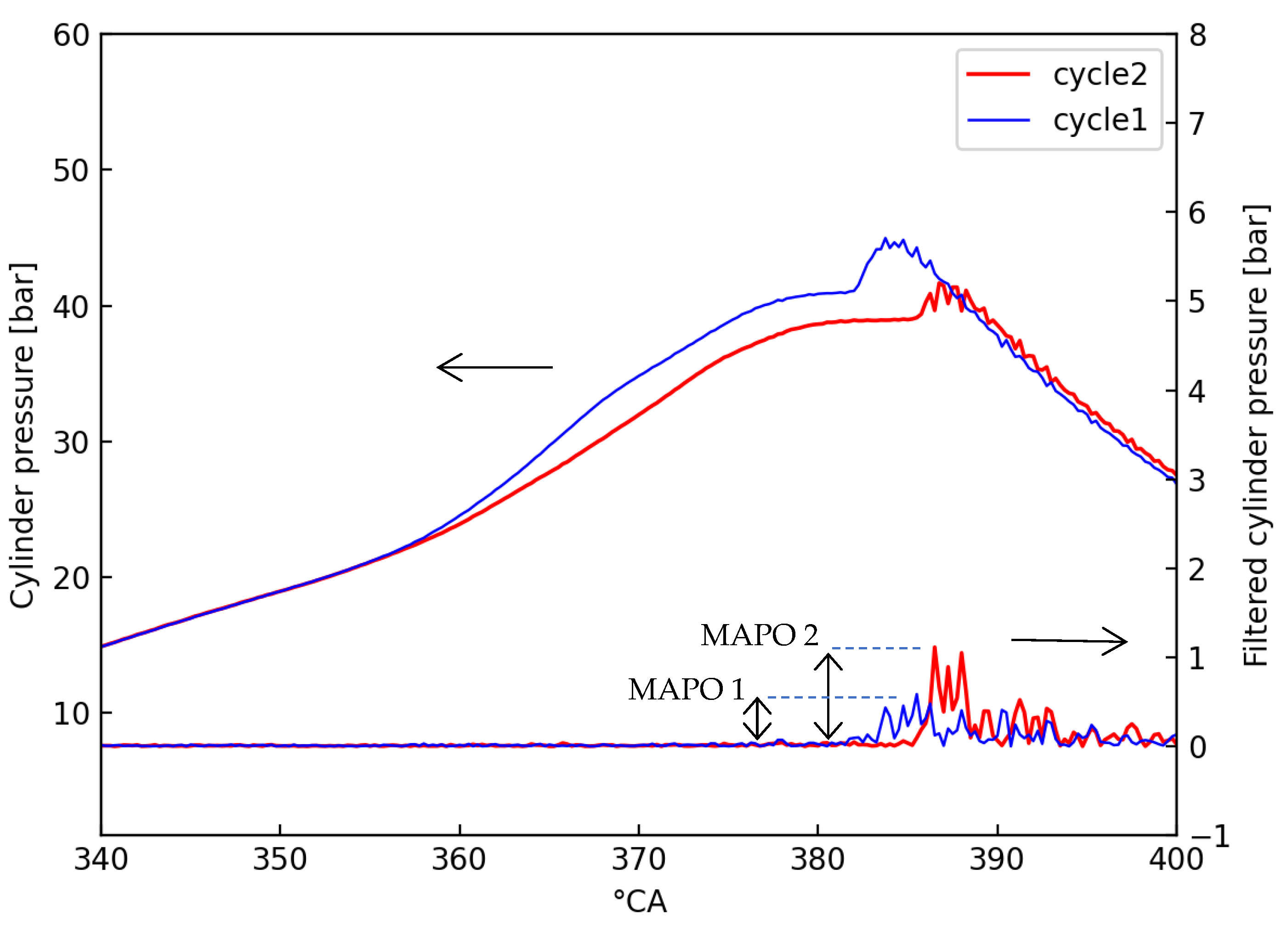
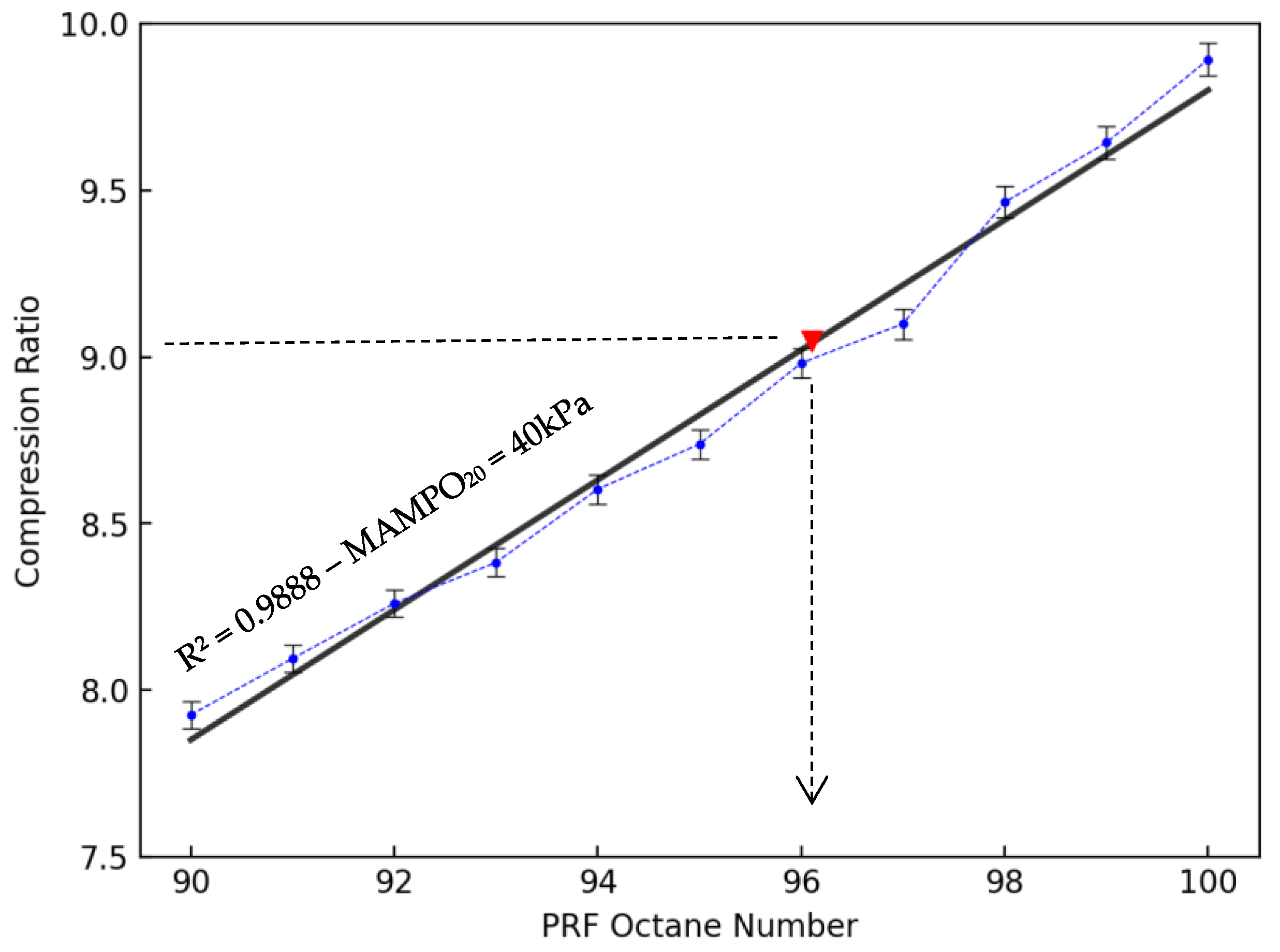
In accordance with the methodology that was used with PRFs, all TSFs were subjected to knocking combustion with a MAMPO
20 intensity slightly above and below 40 kPa.
Table 5 lists the interpolated compression ratios for which all TSFs would be knocking with a MAMPO
20 intensity of 40 kPa. Similar to the PRF scenario, a linear relationship was observed between the TSF’s octane number and the compression ratio needed to obtain a 40 kPa MAMPO
20 knocking intensity. The R² value of the regression line was found to be 0.9909, better than in the PRF case. The thus obtained TSF-based RON estimation can be expressed by Equation (6).
For clarity and to distinguish between the PRF-based method, the TSF-based MAMPO20 will from now on be indicated as MAMPO20T, where T stands for “TSF-based”.
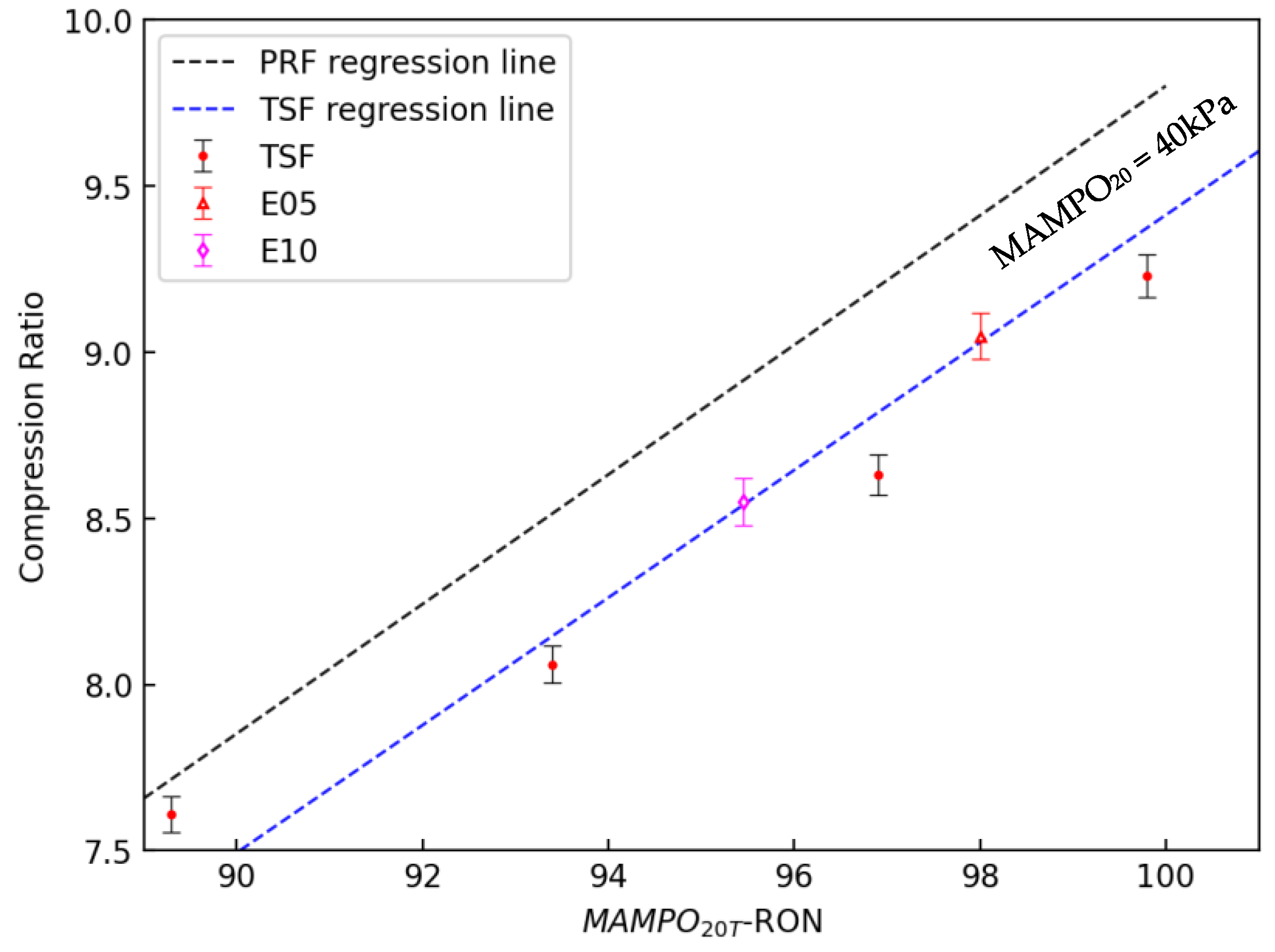
The regression line of the TSFs is juxtaposed with that of the PRFs in
Figure 7, with the earlier compression ratio measurements for E10 and E05 highlighted on the graph. The significant deviation of the TSF measurements from the TSF regression line can be attributed to the inclusion of three measurements above RON 100 in the regression, which, although utilized for the analysis, are not visible on the chart.
One can note that the offset between ASTM RON and MAMPO
20T RON is noticeably smaller for both gasoline types compared to the case when PRFs are used as a reference.
Table 6 gives a comparative overview.
The small differences between ASTM RON and MAMPO
20T RON seem to confirm the fact that TSFs correctly represent the oscillation behaviour of gasoline and that RON estimations can be made, based on oscillation behaviour during knock, within experimental uncertainty limits of ±0.7 RON, as mentioned in the work by Singh et al. [
22].
The method becomes particularly interesting when assessing RON values for fuels exceeding RON 100. Compared to the higher experimental uncertainty with the ASTM method using TSFs that can go up to ±1.7 RON, the uncertainty of the MAMPO20T method remains unchanged based on the performed uncertainty analysis.
Three distinct RON 100+ fuels underwent testing using the MAMPO
20T method, and the findings are juxtaposed with their corresponding ASTM RON values in
Table 7. The ASTM RON values were obtained from the SI Fuel database by Vom Lehn et al. [
23].
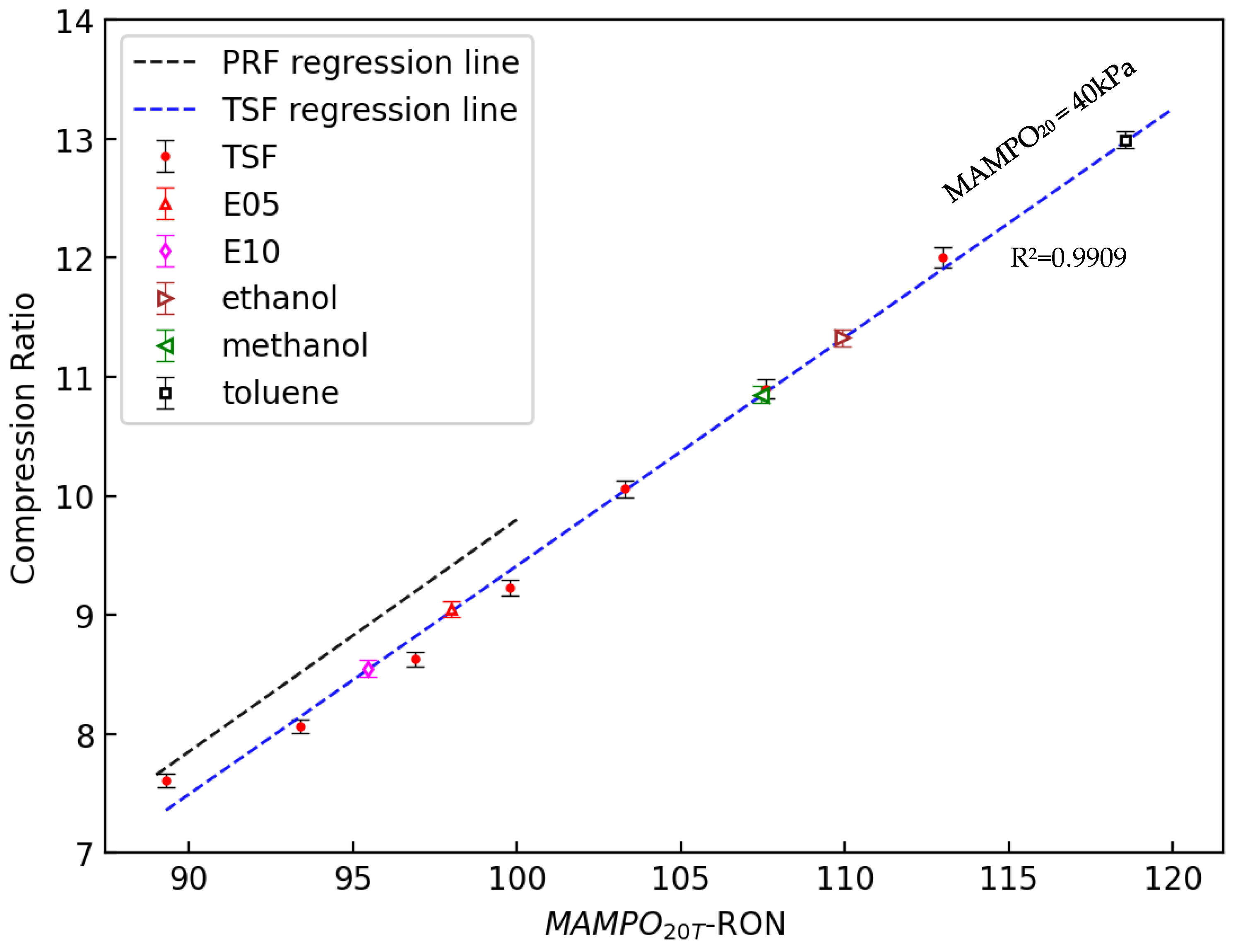
The chart in
Figure 8 gives a complete overview of the RON classification of all fuels used in the test and shows the same distinct linear relationship between compression ratio and octane number for PRFs and TSFs alike. Interestingly, ethanol is associated with higher MAMPO
20T RON values when compared to its respective ASTM RON, while the inverse is true for methanol and toluene.
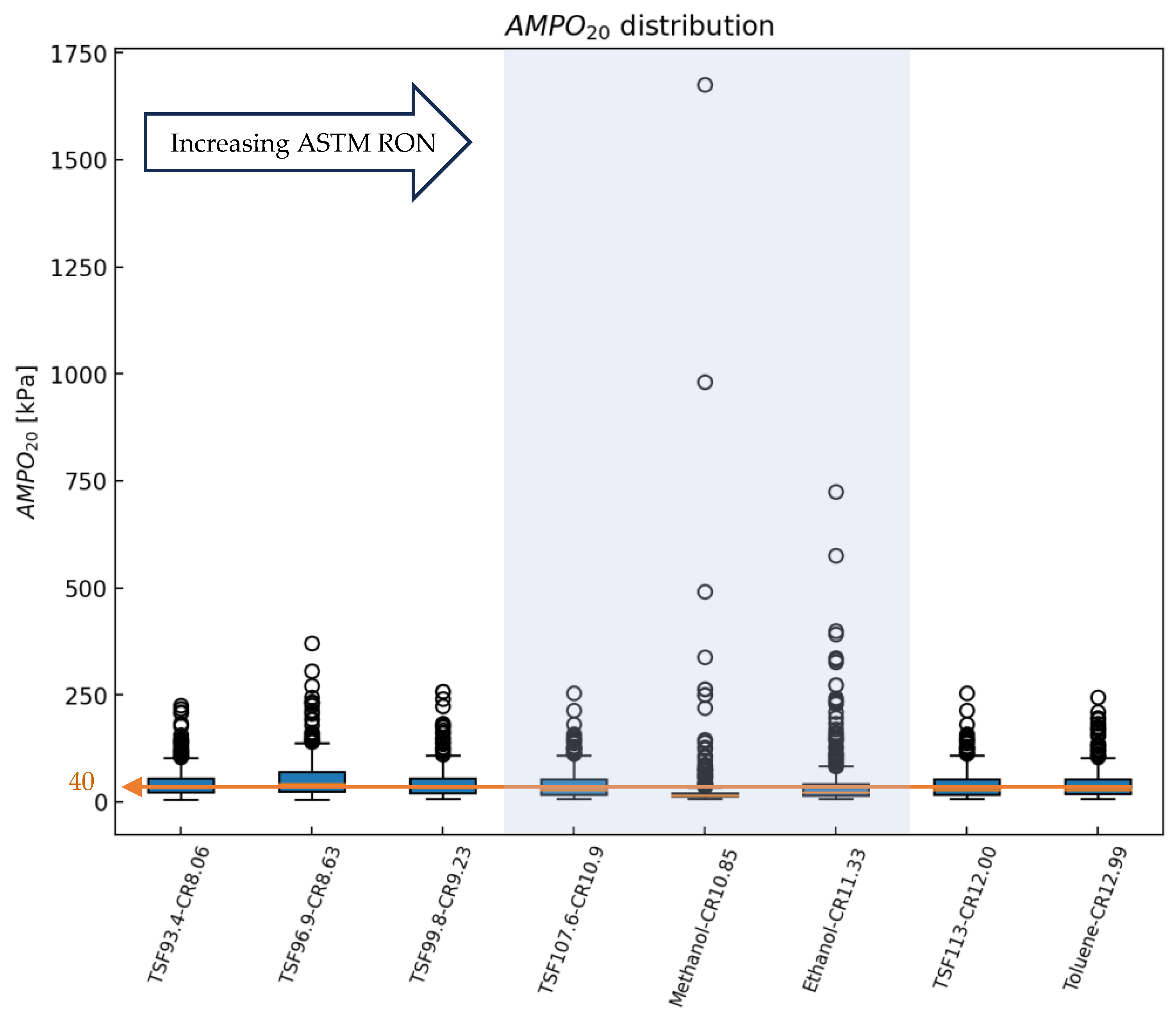
In order to explain this difference, the AMPO
20 distributions of different TSFs were compared to ethanol, methanol, and toluene in the box-whisker diagram of
Figure 9. Each circular marker shows the average of the 20 highest oscillation pressure peaks per combustion cycle. A total of 500 successive combustion cycles were plotted for every fuel. The mean AMPO
20 value for every fuel (MAMPO
20) was 40 kPa ± 5 kPa and is represented by the horizontal line in the graph, indicating that all fuels knock with comparable knock intensity. For every fuel, the needed compression ratio to obtain a MAMPO
20 of 40 kPa is listed on the x-axis.
Across all TSF fuels, it is evident that the oscillation behaviour remains consistent up to the TSF with RON 113. However, the divergence in oscillation behaviour between methanol, ethanol, and the TSFs is significant. In the shaded area on
Figure 9, the oscillation behaviours of TSF 107.6, methanol, and ethanol are compared. Although they feature a comparable ASTM RON, methanol demonstrates oscillation events that are one order of magnitude higher than the primary oscillations. Ethanol exhibits comparable but notably lower oscillation intensities. Consequently, ethanol demonstrates a higher MAMPO
20T RON compared to methanol, despite having an almost identical ASTM RON. The intense oscillation properties of methanol and ethanol could be subscribed to the respective high laminar flame speed velocities of both alcohols. Since ethanol features lower laminar flame speeds than methanol [
24], one can conclude that flame speed and oscillation intensity are likely connected. This seems to be confirmed by the oscillation behaviour of toluene, shown on the right side of the graph. In comparison, toluene and TSFs in general show lower laminar flame speeds than ethanol and methanol [
25,
26], and it is observed that toluene exhibits much milder pressure oscillations, similar to all TSFs used in the test. Consequently, high laminar flame speeds may well be a possible cause of the extreme outliers in the case of methanol and ethanol. Other factors influencing the oscillation behaviour may be found in deviations in end-gas autoignition characteristics as was found in the study by Han et al. [
26].
The utilization of TSFs as reference fuels ensures that the MAMPO20T scale does not disrupt the RON scale for current gasoline formulations. However, it does introduce discrepancies in the RON scale for experimental fuels exceeding RON 100. In such cases, fuels like ethanol and methanol, despite sharing the same ASTM RON, are classified differently. This disparity underscores the inadequacy of the ASTM RON test in capturing the behaviour of fuels during knock and confirms the possible added value of the MAMPO20T method as a “real life” octane classification method.
4. Discussion
The MAMPO20 method exhibits a strong correlation between the ASTM RON scale and oscillation behaviour during knock and shows a significant improvement to the historically weak correlations between AMPO and RON in the literature for PRFs and TSFs alike.
Due to the difference in oscillation behaviour of both reference fuel types, an offset exists between the regression lines of PRFs and TSFs. Since TSFs better represent the behaviour of real-world gasolines, they can be used as reference fuels to estimate RON numbers within acceptable error limits of ±0.7 ON. While the experimental uncertainty remains consistent with comparable measurement setups, conducting additional measurements, particularly with different fuels, is necessary to confirm repeatability.
In contrast to assertions in the literature [
27], utilizing a pressure oscillation system such as MAMPO
20T does not inherently disturb the RON scale, although discrepancies in RON may emerge with fuels exceeding RON 100.
The MAMPO
20T RON method, in contrast to the ASTM method, is able to distinguish the difference in oscillation behaviour between PRFs and TSFs that share the same ASTM RON. As a result, the approach links PRF reference fuels (illustrated by the dashed PRF regression line in
Figure 9) with a MAMPO
20T RON number higher than their corresponding ASTM RON. This is due to the requirement of higher compression ratios for PRFs compared to RON-equivalent TSFs to achieve the MAMPO
20 knock intensity of 40 kPa.
The divergence in classifying PRFs on the MAMPO20T RON scale can likely be attributed to differences in lower flame speeds of PRFs, which necessitate higher compression ratios to obtain equal knock intensities compared to oxygenated fuels. The absence of this distinction in the ASTM method underscores the credibility of the MAMPO20T method as a more accurate octane quantification system reflecting real-world conditions. Fuels with molecular structures similar to those of primary reference fuels would exhibit smaller deviations from ASTM RON when the PRF-based MAMPO RON method is used.
The MAMPO20T method seems viable for assessing fuels surpassing RON 100 without complications. Notably, this method discerns between ethanol and methanol, showcasing a MAMPO20T RON difference of 2.4, whereas the ASTM RON method indicates only a 0.1 RON difference.
This underscores how the MAMPO20T method can distinguish between two fuels with nearly identical ASTM RON values based on variations in oscillation behaviour. The inadequacy of the ASTM RON test to capture the behaviour of fuels during knock confirms the possible added value of the MAMPO20T method as a “real life” octane classification method for fuels below and above RON 100.
One limitation of the MAMPO20T method arises from its inability to measure knock intensity in real time through post-processing, rendering it impractical for real-time applications. Due to its reliance on an averaging algorithm, the method loses detailed cylinder pressure information upon application.
The systematic factors influencing high-frequency oscillation behaviour, while showing a connection to laminar flame speed, remain incompletely understood to date. Further investigation is necessary to gain deeper insights into this behaviour.
The existing approach utilizes a CFR engine where the compression ratio serves as the adjustable parameter for evaluation. Given that the MAMPO method can be implemented whenever cylinder chamber pressure can be monitored, future investigations could utilize a commercial engine with a fixed compression ratio. Other parameters such as ignition timing, boost pressure, or intake temperature could be manipulated instead, on the condition that the results are sufficiently reproducible.
Implementing a version of the MAMPO method in a production engine would enable a more direct assessment of a fuel’s octane behaviour within that specific engine. It would also allow a direct approach for an assessment of the engine’s octane requirement (OR) [
28] since the method enables a direct measurement of knock intensity, independent of the antiknock strategy of the engine’s ECU that relies on measurements of the knock sensor instead. It would also provide a solution to the contention that operating conditions deviate from real-world scenarios. In future research, the connection between MAMPO
20 knock intensity and knock sensor output will be explored and potential correlations will be assessed. This investigation could facilitate the quantification of knock intensity using a knock sensor, potentially replacing the need for pressure transducers entirely.