Design of Sinusoidal Leading Edge for Low-Speed Axial Fans Operating under Inflow Distortion
Abstract
1. Introduction
2. Flow Control Using Sinusoidal Leading Edges
2.1. Design Practices and Challenges for Sinusoidal Leading Edge Rotors
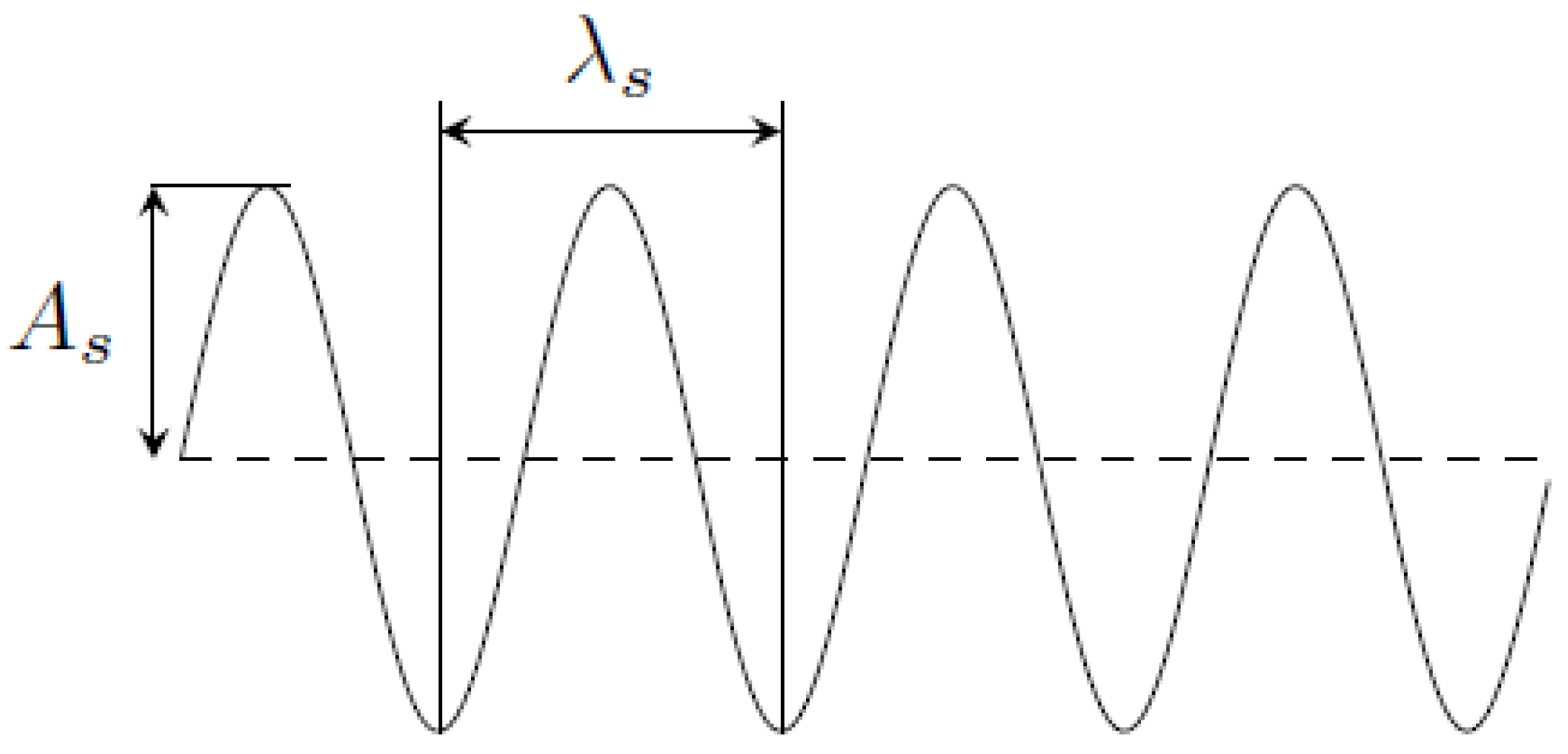
- The amplitude of the sinusoid is selected between 0.05c and 0.4c, where c is the chord length of the aerodynamic profile;
- Higher values of are associated with higher aerodynamic losses at low incidence;
- The effect of the wavelength is negligible in post-stall operations; however, low values of are responsible for increased losses;
- Wavelength is normally chosen between 0.1c and 0.4c;
- Low values of and large are preferred in noise control. Tubercles are, in fact, beneficial in diminishing tonal noise at peak frequencies, and modified airfoils show reduced broadband sound pressure levels. In isolated profiles, new peaks at higher frequencies may appear;
- Sinusoid leading edge aerodynamic performance is similar for Reynolds numbers between 0.1M and 1.5M;
- Airfoil thickness plays a role, suggesting that thicker profiles are less sensitive to performance degradation due to sinusoidal shape;
- Sinusoids act as stall delayers and post-stall stabilizers, with little to no effect in stable operations. It is, therefore, not recommended to apply this modification if stable operation is expected.
2.2. Sinousoidal Leading Edges in Fan Rotors
- Sinusoidal LEs induce additional vorticity that interferes with the secondary structures that are generated in the flow passage. They also generate radial motions that interfere with and dampen the tip leakage vortex;
- The sinusoids are locally applied to control the radial velocity distribution. As an example, only the outer 30% of the blade span is usually modified with the sinusoidal profile;
- Airfoil family, thickness, and camber distributions may substantially differ from the relevant works on isolated airfoils, which mostly focus on NACA 0021 and NACA 65-021.
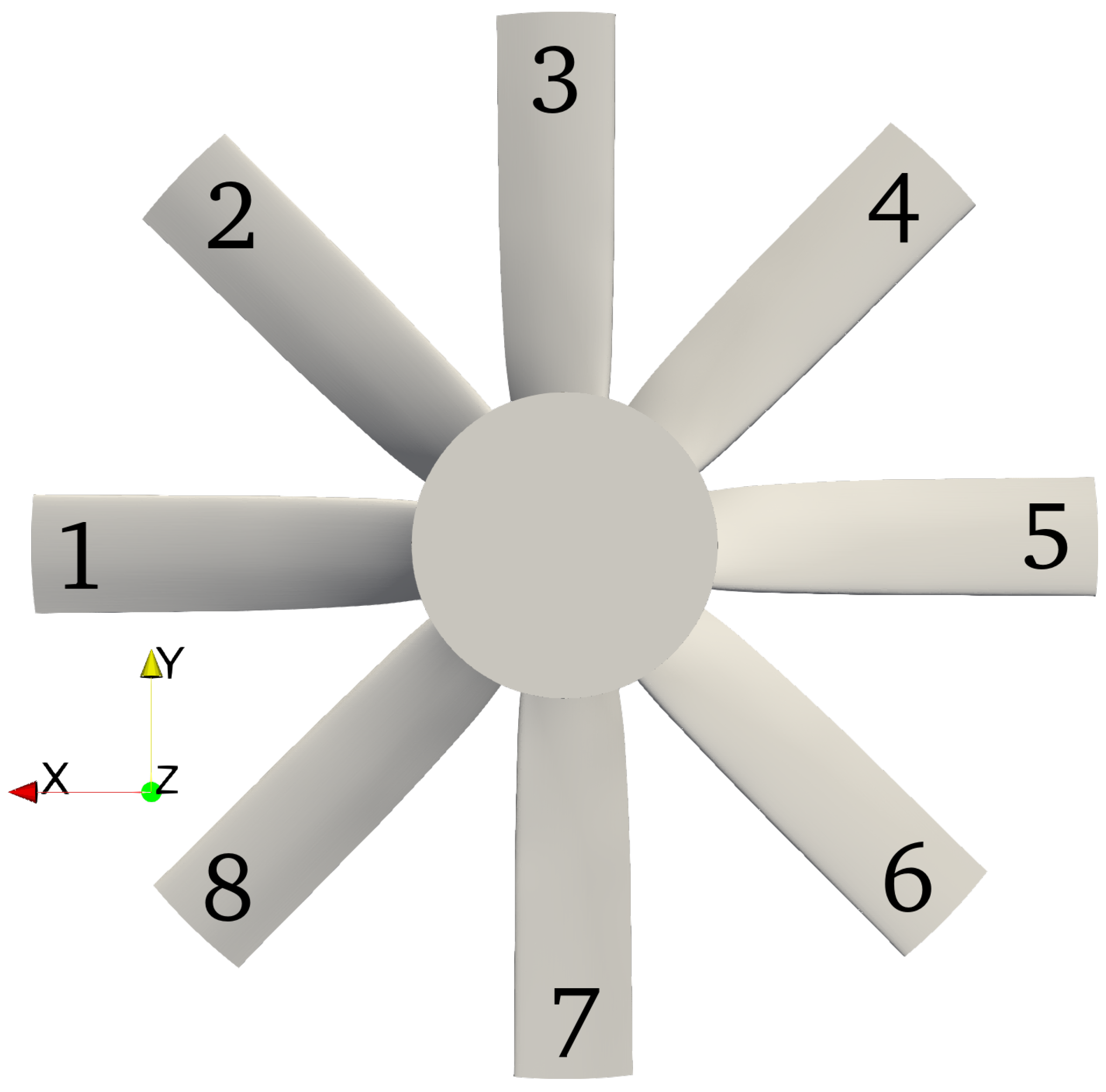
3. Design of Sinusoidal Leading Edge Rotor for a Low-Speed, High-Diameter Fan in an ACC Test Facility
4. Experimental and Numerical Setup
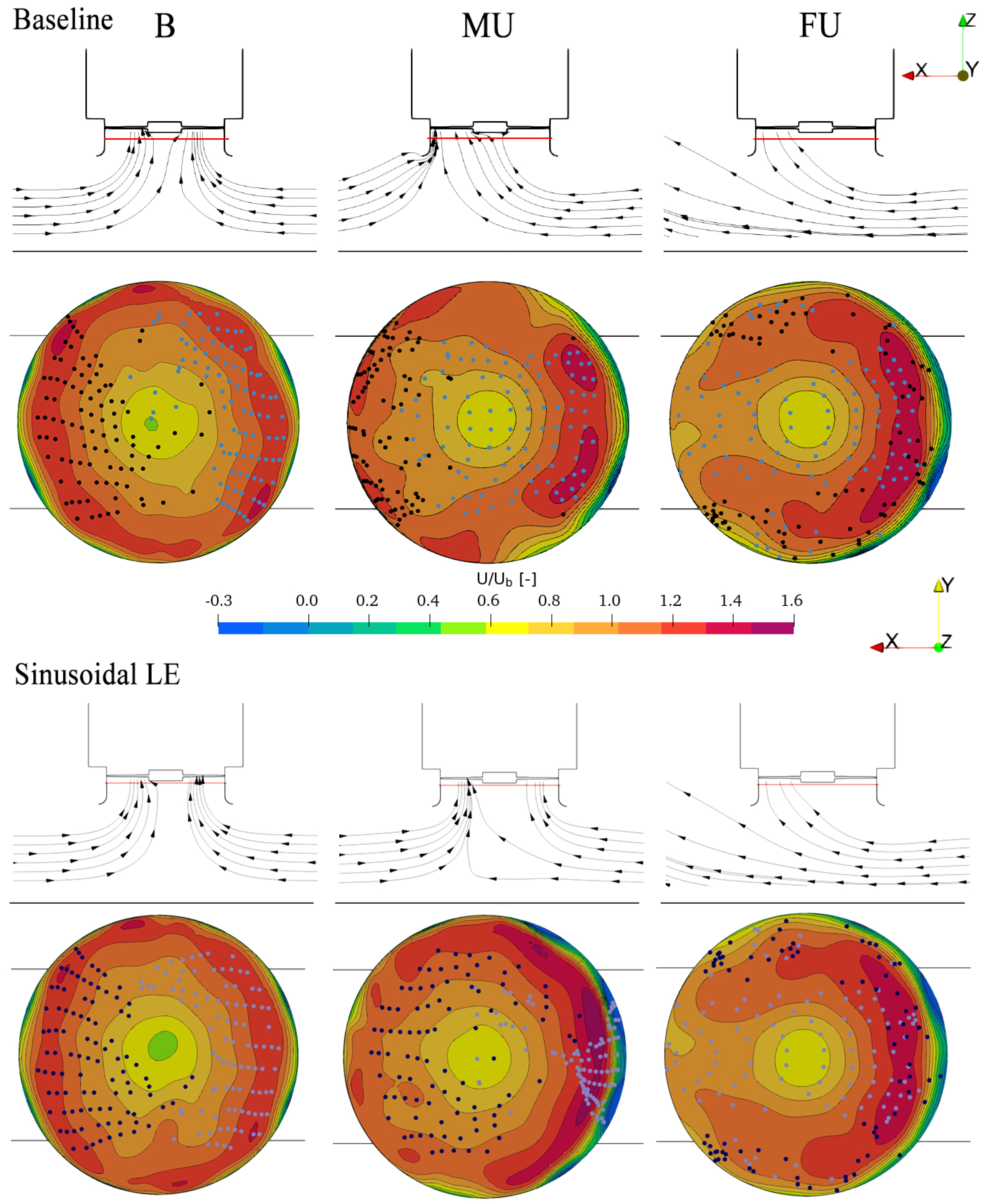
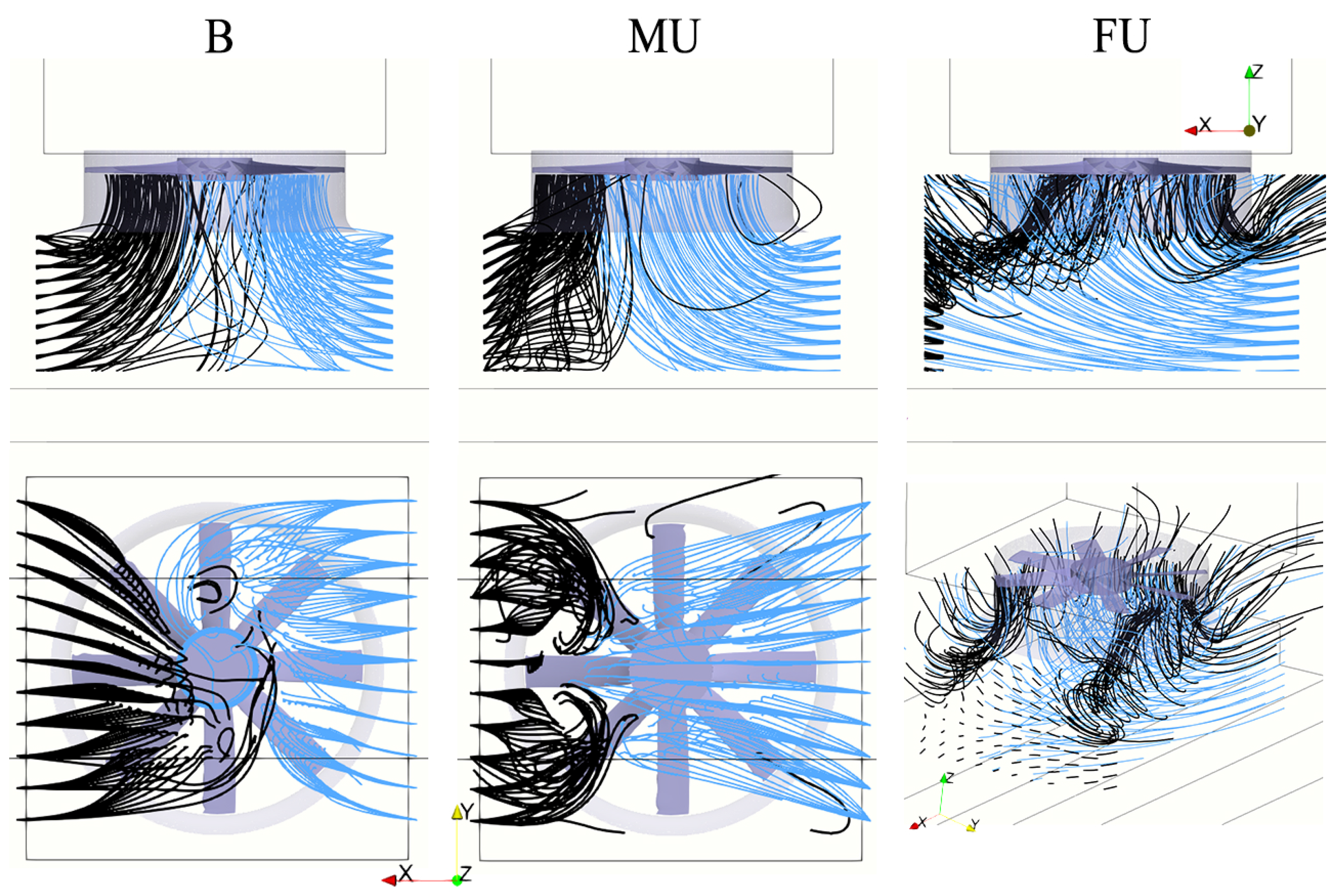
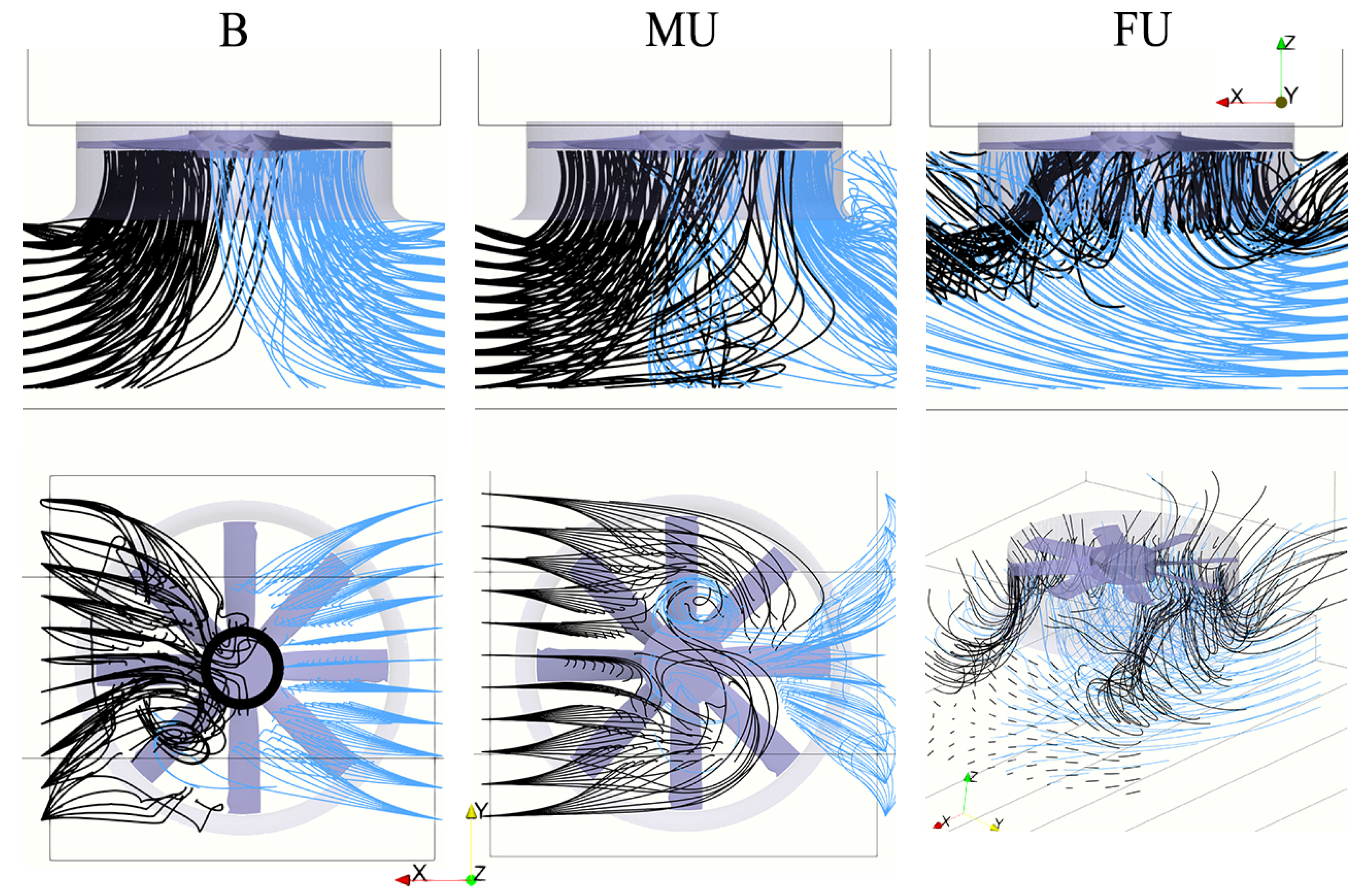
4.1. Scenario Definition
- i
- Balanced (B): The flow rate is equally divided between the two inlets;
- ii
- Mildly unbalanced (MU): the inflow has an imposed flow rate that is double that of the opposite;
- iii
- Fully unbalanced (FU): the full flow rate is imposed on one inlet only
4.2. Numerical Modeling
5. Validation
6. Results
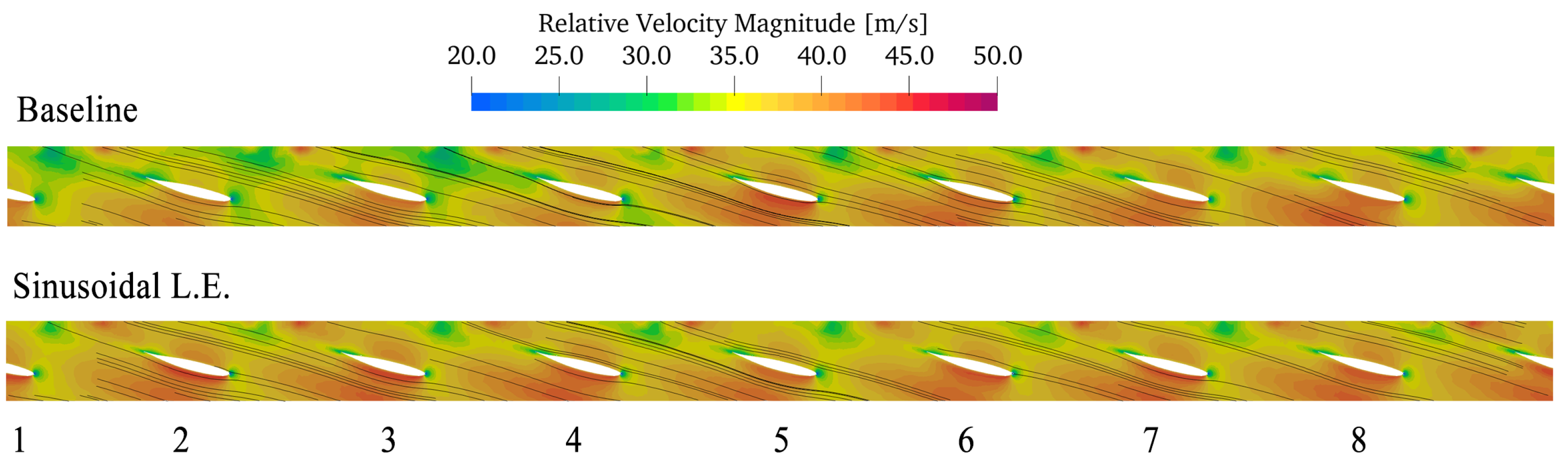
6.1. Fan Performance under Distorted Inflow Conditions
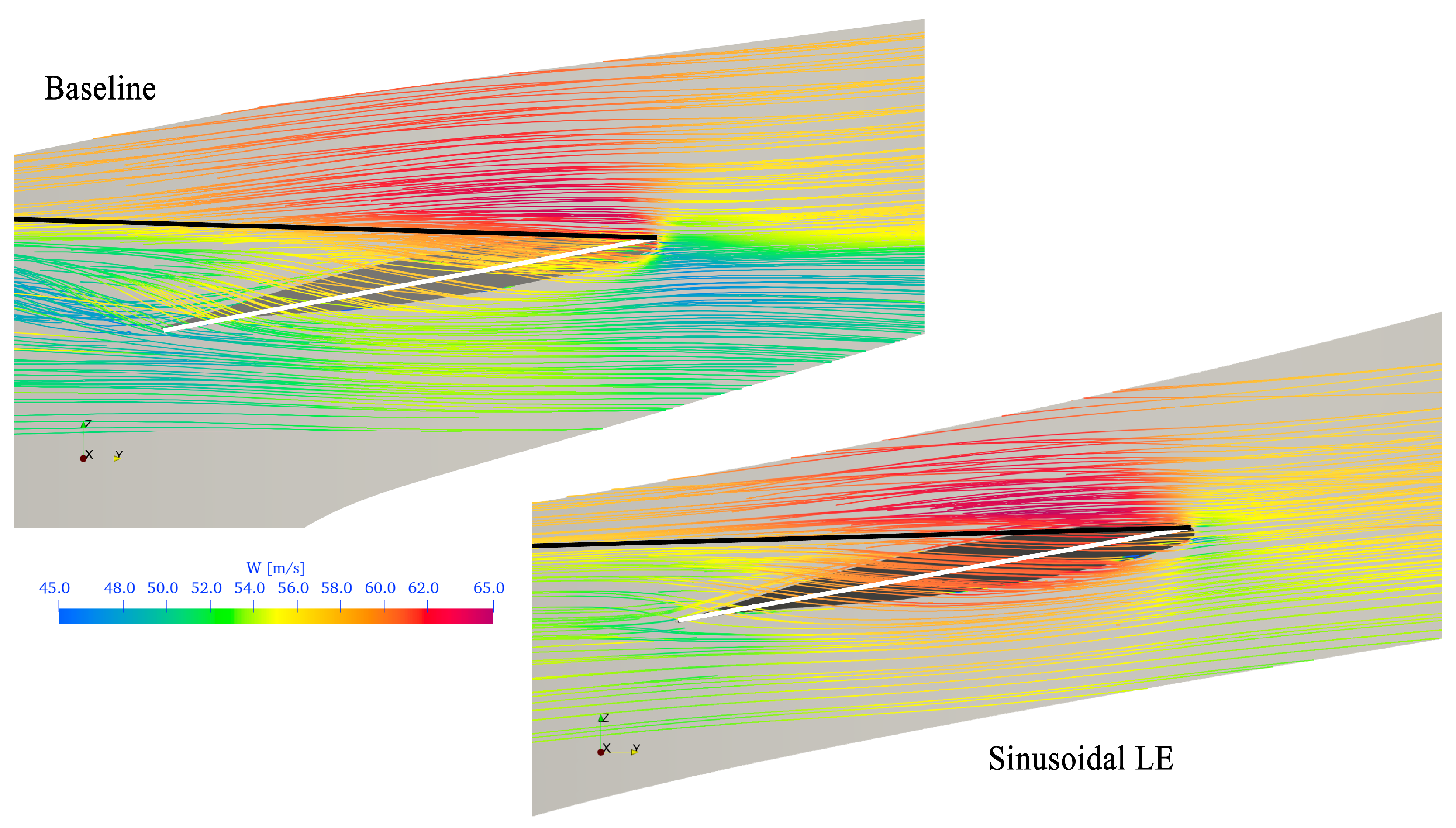
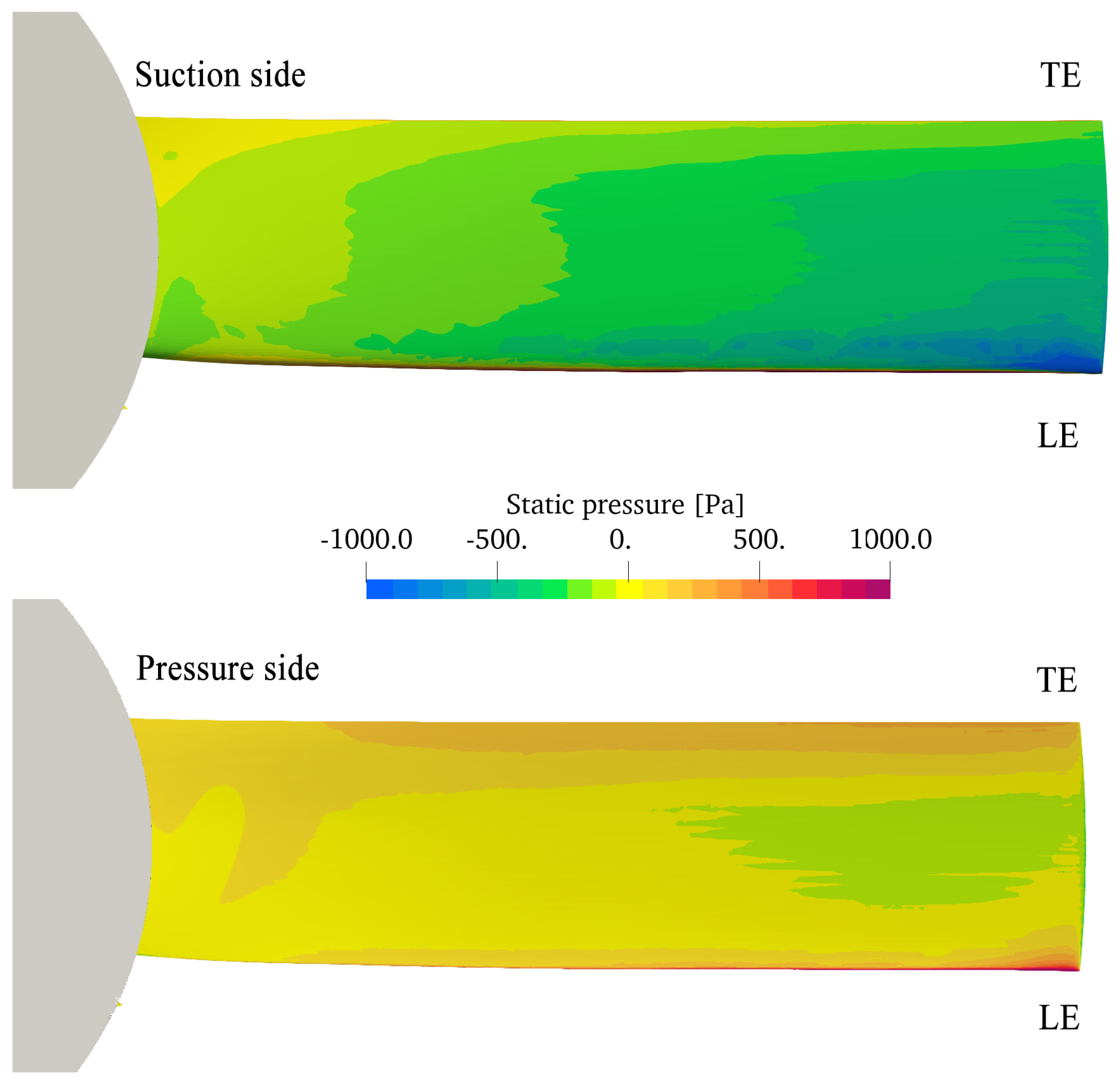
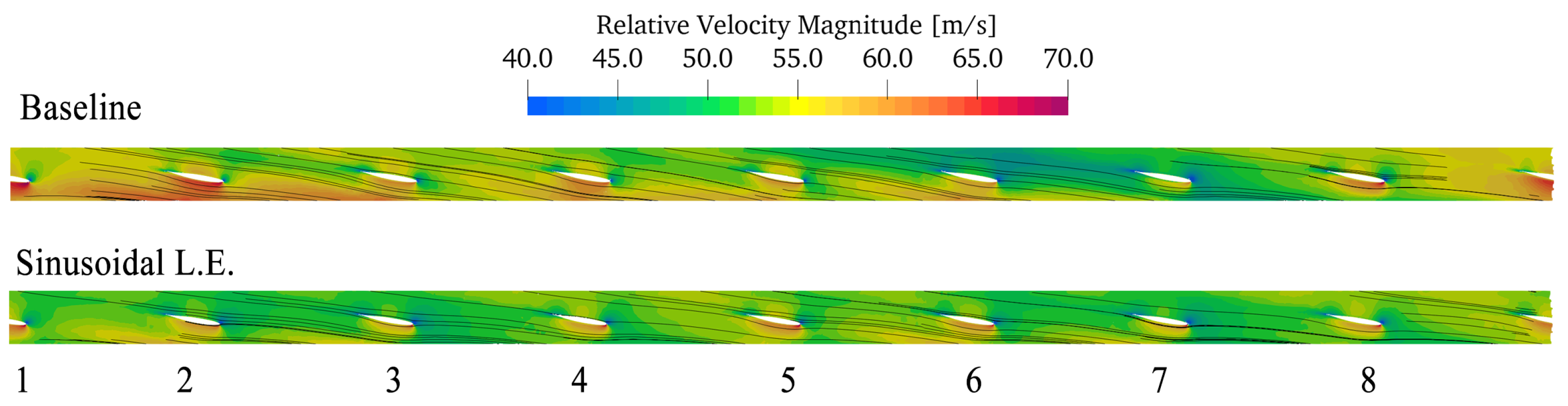
6.2. Flow Survey with Balanced and Distorted Inflow Conditions
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Astolfi, M.; Martelli, E.; Pierobon, L. 7—Thermodynamic and technoeconomic optimization of Organic Rankine Cycle systems. In Organic Rankine Cycle (ORC) Power Systems; Macchi, E., Astolfi, M., Eds.; Woodhead Publishing: Cambridge, UK, 2017; pp. 173–249. [Google Scholar]
- Butler, C.; Grimes, R. The effect of wind on the optimal design and performance of a modular air-cooled condenser for a concentrated solar power plant. Energy 2014, 68, 886–895. [Google Scholar] [CrossRef]
- Marincowitz, F.; Owen, M.; Muiyser, J. Multi-objective optimisation for wind resistant air-cooled condenser operation. Appl. Therm. Eng. 2023, 218, 119382. [Google Scholar] [CrossRef]
- Chen, L.; Yang, L.; Du, X.; Yang, Y. A novel layout of air-cooled condensers to improve thermo-flow performances. Appl. Energy 2016, 165, 244–259. [Google Scholar] [CrossRef]
- Venter, A.; Owen, M.; Muiyser, J. A numerical analysis of windscreen effects on air-cooled condenser fan performance. Appl. Therm. Eng. 2021, 186, 116416. [Google Scholar] [CrossRef]
- He, W.; Dai, Y.; Zhu, S.; Han, D.; Yue, C.; Pu, W. Influence from the blade installation angle of the windward axial fans on the performance of an air-cooled power plant. Energy 2013, 60, 416–425. [Google Scholar] [CrossRef]
- Bianchini, C.; Da Soghe, R.; Giannini, L.; Mazzei, L.; Gardner, A.; Wilde, J. Characterization of ACC Losses Due to Wind and Design of Optimal Wind-Screens. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Boston, MA, USA, 26–30 June 2023; Volume 86991, p. V006T10A008. [Google Scholar]
- Bekker, G.; Meyer, C.; Van der Spuy, S. Influence of pressure recovery on the performance of an induced draught air-cooled condenser under windless and windy conditions. Appl. Therm. Eng. 2022, 213, 118703. [Google Scholar] [CrossRef]
- Yang, L.; Du, X.; Zhang, H.; Yang, Y. Numerical investigation on the cluster effect of an array of axial flow fans for air-cooled condensers in a power plant. Chin. Sci. Bull. 2011, 56, 2272–2280. [Google Scholar] [CrossRef][Green Version]
- Louw, F.G.; Bruneau, P.R.; von Backström, T.W.; van der Spuy, S.J. The design of an axial flow fan for application in large air-cooled heat exchangers. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Copenhagen, Denmark, 11–15 June 2012; Volume 44694, pp. 771–785. [Google Scholar]
- Duvenhage, K.; Vermeulen, J.; Meyer, C.; Kröger, D. Flow distortions at the fan inlet of forced-draught air-cooled heat exchangers. Appl. Therm. Eng. 1996, 16, 741–752. [Google Scholar] [CrossRef]
- Thiart, G.; Von Backström, T. Numerical simulation of the flow field near an axial flow fan operating under distorted inflow conditions. J. Wind. Eng. Ind. Aerodyn. 1993, 45, 189–214. [Google Scholar] [CrossRef]
- Fourie, N.; Van Der Spuy, S.; Von Backström, T. Simulating the effect of wind on the performance of axial flow fans in air-cooled steam condenser systems. J. Therm. Sci. Eng. Appl. 2015, 7, 021011. [Google Scholar] [CrossRef]
- Van der Spuy, S.J.; von Backström, T.W.; Kröger, D.G. Performance of low noise fans in power plant air cooled steam condensers. Noise Control. Eng. J. 2009, 57, 341–347. [Google Scholar] [CrossRef][Green Version]
- Karpenko, M.; Stosiak, M.; Deptuła, A.; Urbanowicz, K.; Nugaras, J.; Królczyk, G.; Żak, K. Performance evaluation of extruded polystyrene foam for aerospace engineering applications using frequency analyses. Int. J. Adv. Manuf. Technol. 2023, 126, 5515–5526. [Google Scholar] [CrossRef]
- Bianchi, S.; Corsini, A.; Sheard, A. A critical review of passive noise control techniques in industrial fans. J. Eng. Gas Turbines Power 2014, 136, 044001. [Google Scholar] [CrossRef]
- Fish, F.E. Influence of hydrodynamic-design and propulsive mode on mammalian swimming energetics. Aust. J. Zool. 1994, 42, 79–101. [Google Scholar] [CrossRef]
- Fish, F.E.; Howle, L.E.; Murray, M.M. Hydrodynamic flow control in marine mammals. Integr. Comp. Biol. 2008, 48, 788–800. [Google Scholar] [CrossRef]
- Xin, H.; Rui, G.; Jin, J.F.; Liu, Y.R.; Yi, M.; Qian, C.; Zheng, Y. Numerical simulation and aerodynamic performance comparison between seagull aerofoil and NACA 4412 aerofoil under low-reynolds. Adv. Nat. Sci. 2010, 3, 244–250. [Google Scholar]
- Fish, F.E.; Battle, J.M. Hydrodynamic design of the humpback whale flipper. J. Morphol. 1995, 225, 51–60. [Google Scholar] [CrossRef]
- Watts, P.; Fish, F.E. The influence of passive, leading edge tubercles on wing performance. In Proceedings of the 12th International Symposium: Unmanned Untethered Submersible Technology, Annual International Symposium; Autonomous Undersea Systems Institute: Durham, NH, USA, 2001. [Google Scholar]
- Hansen, K.L.; Kelso, R.M.; Dally, B.B. Performance variations of leading-edge tubercles for distinct airfoil profiles. AIAA J. 2011, 49, 185–194. [Google Scholar] [CrossRef]
- Hansen, K.L. Effect of Leading Edge Tubercles on Airfoil Performance. Ph.D. Thesis, University of Adelaide, Adelaide, Australia, 2012. [Google Scholar]
- Xingwei, Z.; Chaoying, Z.; Tao, Z.; Wenying, J. Numerical study on effect of leading-edge tubercles. Aircr. Eng. Aerosp. Technol. 2013, 85, 247–257. [Google Scholar] [CrossRef]
- Miklosovic, D.; Murray, M.; Howle, L.; Fish, F. Leading-edge tubercles delay stall on humpback whale (Megaptera novaeangliae) flippers. Phys. Fluids 2004, 16, L39–L42. [Google Scholar] [CrossRef]
- Hansen, K.L.; Rostamzadeh, N.; Kelso, R.M.; Dally, B.B. Evolution of the streamwise vortices generated between leading edge tubercles. J. Fluid Mech. 2016, 788, 730–766. [Google Scholar] [CrossRef]
- Kim, H.; Kim, J.; Choi, H. Flow structure modifications by leading-edge tubercles on a 3D wing. Bioinspir. Biomim. 2018, 13, 066011. [Google Scholar] [CrossRef] [PubMed]
- Cai, C.; Zhou, T.; Liu, S.; Zuo, Z.; Zhang, Y.; Li, Q. Modeling of the compartmentalization effect induced by leading-edge tubercles. Phys. Fluids 2022, 34, 087104. [Google Scholar] [CrossRef]
- Seyhan, M.; Sarioglu, M.; Akansu, Y.E. Influence of leading-edge tubercle with amplitude modulation on NACA 0015 airfoil. AIAA J. 2021, 59, 3965–3978. [Google Scholar] [CrossRef]
- Hansen, K.; Kelso, R.; Doolan, C. Reduction of flow induced tonal noise through leading edge tubercle modifications. In Proceedings of the 16th AIAA/CEAS Aeroacoustics Conference, Stockholm, Sweden, 7–9 June 2010; p. 3700. [Google Scholar]
- Fan, M.; Dong, X.; Li, Z.; Sun, Z.; Feng, L. Numerical and experimental study on flow separation control of airfoils with various leading-edge tubercles. Ocean Eng. 2022, 252, 111046. [Google Scholar] [CrossRef]
- Corsini, A.; Delibra, G.; Sheard, A.G. On the role of leading-edge bumps in the control of stall onset in axial fan blades. J. Fluids Eng. 2013, 135, 081104. [Google Scholar] [CrossRef]
- Chaitanya, P.; Joseph, P.; Narayanan, S.; Vanderwel, C.; Turner, J.; Kim, J.W.; Ganapathisubramani, B. Performance and mechanism of sinusoidal leading edge serrations for the reduction of turbulence–aerofoil interaction noise. J. Fluid Mech. 2017, 818, 435–464. [Google Scholar] [CrossRef]
- Post, M.L.; Decker, R.; Sapell, A.R.; Hart, J.S. Effect of bio-inspired sinusoidal leading-edges on wings. Aerosp. Sci. Technol. 2018, 81, 128–140. [Google Scholar] [CrossRef]
- Miklosovic, D.S.; Murray, M.M.; Howle, L.E. Experimental evaluation of sinusoidal leading edges. J. Aircr. 2007, 44, 1404–1408. [Google Scholar] [CrossRef]
- Chong, T.P.; Vathylakis, A.; McEwen, A.; Kemsley, F.; Muhammad, C.; Siddiqi, S. Aeroacoustic and aerodynamic performances of an aerofoil subjected to sinusoidal leading edges. In Proceedings of the 21st AIAA/CEAS Aeroacoustics Conference, Dallas, TX, USA, 22–26 June 2015; p. 2200. [Google Scholar]
- Guerreiro, J.; Sousa, J. Low-Reynolds-number effects in passive stall control using sinusoidal leading edges. AIAA J. 2012, 50, 461–469. [Google Scholar] [CrossRef]
- Corsini, A.; Delibra, G.; Sheard, A.G. Leading edge bumps in ventilation fans. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, San Antonio, TX, USA, 3–7 June 2013; Volume 55188, p. V004T10A007. [Google Scholar]
- MinWaterCSP Website. Available online: https://minwatercsp.eu/ (accessed on 30 May 2023).
- Angelini, G.; Bonanni, T.; Corsini, A.; Delibra, G.; Tieghi, L.; Volponi, D. Optimization of an axial fan for air cooled condensers. Energy Procedia 2017, 126, 754–761. [Google Scholar] [CrossRef]
- Tieghi, L.; Delibra, G.; Corsini, A.; Van Der Spuy, J. Numerical investigation of CSP air cooled condenser fan. E3S Web Conf. 2020, 197, 11010. [Google Scholar] [CrossRef]
- Angelini, G.; Bonanni, T.; Corsini, A.; Delibra, G.; Tieghi, L.; Volponi, D. Effects of Fan Inflow Distortions on Heat Exchange in Air-Cooled Condensers: Unsteady Computations With Synthetic Blade Model. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Oslo, Norway, 11–15 June 2018; Volume 50985, p. V001T09A010. [Google Scholar]
- Tieghi, L.; Delibra, G.; van der Spuy, J.; Corsini, A. Leading Edge Bumps for Flow Control in Air-Cooled Condensers. Int. J. Turbomach. Propuls. Power 2023, 8, 9. [Google Scholar] [CrossRef]
- Barnabei, V.F.; Castorrini, A.; Corsini, A.; Van der Spuy, J.; Rispoli, F. Unsteady flow simulation of an axial fan for dry cooling in a CSP plant using the variational multiscale method. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Virtual, Online, 21–25 September 2020; Volume 84058, p. V001T10A014. [Google Scholar]
- Volponi, D.; Bonanni, T.; Tieghi, L.; Delibra, G.; Corsini, A.; Wilkinson, M.; Van Der Spuy, S.; Von Backström, T.W. CFD simulation results for the MinWaterCSP cooling fan. In Proceedings of the FAN 2018, Darmstadt, Germany, 18–20 April 2018; Available online: https://www.researchgate.net/profile/Sybrand-Van-Der-Spuy/publication/325036834_CFD_SIMULATION_RESULTS_FOR_THE_MINWATERCSP_COOLING_FAN/links/5c1c8feb458515a4c7edd381/CFD-SIMULATION-RESULTS-FOR-THE-MINWATERCSP-COOLING-FAN.pdf (accessed on 15 February 2024).
- Idelchik, I.E. Handbook of Hydraulic Resistance; Begell House: Washington, DC, USA, 1996. [Google Scholar]
- Tieghi, L.; Delibra, G.; van der Spuy, J.; Corsini, A. On the usage of tip appendages for flow control in air-cooled condensers. In Proceedings of the 18th Conference on Modelling Fluid Flow CMFF’22, Budapest, Hungary, 30 August–2 September 2022. [Google Scholar]
- Van der Spuy, S.; Els, D.; Tieghi, L.; Delibra, G.; Corsini, A.; Louw, F.; Zapke, A.; Meyer, C. Preliminary Evaluation of the 24 Ft. Diameter Fan Performance In the MinWaterCSP Large Cooling Systems Test Facility. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Virtual, Online, 7–11 June 2021; Volume 84898, p. V001T10A005. [Google Scholar]



| Ref. | Application | Method. | Param. | Major Findings |
|---|---|---|---|---|
| [21] | Wing | Panel code | - |
|
| [22,23] | NACA 65021 NACA 0021 | Exp. | = 0.03c–0.11c = 0.11c–0.43c Re = 120.000 |
|
| [24] | Wing | CFD | = 0.25c–0.5c = 0.05–0.1c Re = 100.000 |
|
| [25] | Model of whale flippers | Exp. | Real fin Re = 135.000–550.000 |
|
| [26] | NACA 0021 | Exp. CFD | Real fin Re = 180.000 |
|
| [27] | 3D wing (flippers) | Exp. | = 0.11c = 0.42c Re = 2.230 |
|
| [28] | Wavy & Fenced airfoils | CFD | = 0.06c = 0.4c Re = 185.000 |
|
| [29] | NACA 0015 | Exp. | = 0.0125c–0.05c = 0.0625c–0.5c Re = 63.000 |
|
| [30] | NACA 0021 | Exp. (noise) | = 0.03c–0.11c = 0.11c–0.43c Re = 120.000 |
|
| [31] | NACA 4415 turbine blades | CFD | = 0.1c = 0.2c Re = 50.000 |
|
| [32] | NACA 4415 | CFD | = 0.025c = 0.25c Re = 183.000 |
|
| Tip radius | 3680 mm |
| Tip clearance | 36.8 mm |
| Hub-to-tip ratio | 0.29 |
| Rotational speed | 151 rpm |
| Tip Reynolds number | |
| Number of blades | 8 |
| Rated volumetric flow rate | 333 m3/s |
| Rated static pressure rise | 117 Pa |
| Rated power | 57 kW |
| Q | Balanced | Mild Unbalance | Full Unbalance | |||
|---|---|---|---|---|---|---|
| [m3/s] | Base | Sin. LE | Base | Sin. LE | Base | Sin. LE |
| 135 | 224.9 | 212.6 | 222.8 | 217.1 | 224.7 | 221.3 |
| 192 | 195.4 | 192.3 | 194.1 | 192.1 | 183.7 | 179.9 |
| 281 | 119.6 | 123.0 | 128.1 | 115.4 | 123.1 | 110.3 |
| 308 | 94.6 | 94.6 | 106.3 | 95.8 | 103.1 | 81.2 |
| 333 | 68.7 | 68.5 | 66.9 | 68.9 | 59.4 | 43.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tieghi, L.; Delibra, G.; Van der Spuy, J.; Corsini, A. Design of Sinusoidal Leading Edge for Low-Speed Axial Fans Operating under Inflow Distortion. Energies 2024, 17, 1150. https://doi.org/10.3390/en17051150
Tieghi L, Delibra G, Van der Spuy J, Corsini A. Design of Sinusoidal Leading Edge for Low-Speed Axial Fans Operating under Inflow Distortion. Energies. 2024; 17(5):1150. https://doi.org/10.3390/en17051150
Chicago/Turabian StyleTieghi, Lorenzo, Giovanni Delibra, Johan Van der Spuy, and Alessandro Corsini. 2024. "Design of Sinusoidal Leading Edge for Low-Speed Axial Fans Operating under Inflow Distortion" Energies 17, no. 5: 1150. https://doi.org/10.3390/en17051150
APA StyleTieghi, L., Delibra, G., Van der Spuy, J., & Corsini, A. (2024). Design of Sinusoidal Leading Edge for Low-Speed Axial Fans Operating under Inflow Distortion. Energies, 17(5), 1150. https://doi.org/10.3390/en17051150







