Estimation of the Region of Attraction of Polynomial Swing Equation Using Sum of Squares Theory
Abstract
1. Introduction
2. Theoretical Basis of SOS
2.1. Basic Concepts
2.2. Key Result
3. The Polynomial Model of Power Systems
3.1. Polynomial Model of Single-Machine Systems
3.2. Polynomial Model of Multi-Machine Systems
3.3. Single-Machine Projection Polynomial Equation for Multi-Machine Systems
4. Estimation of the Region of Attraction Method in Power Systems
4.1. Fundamental Theory
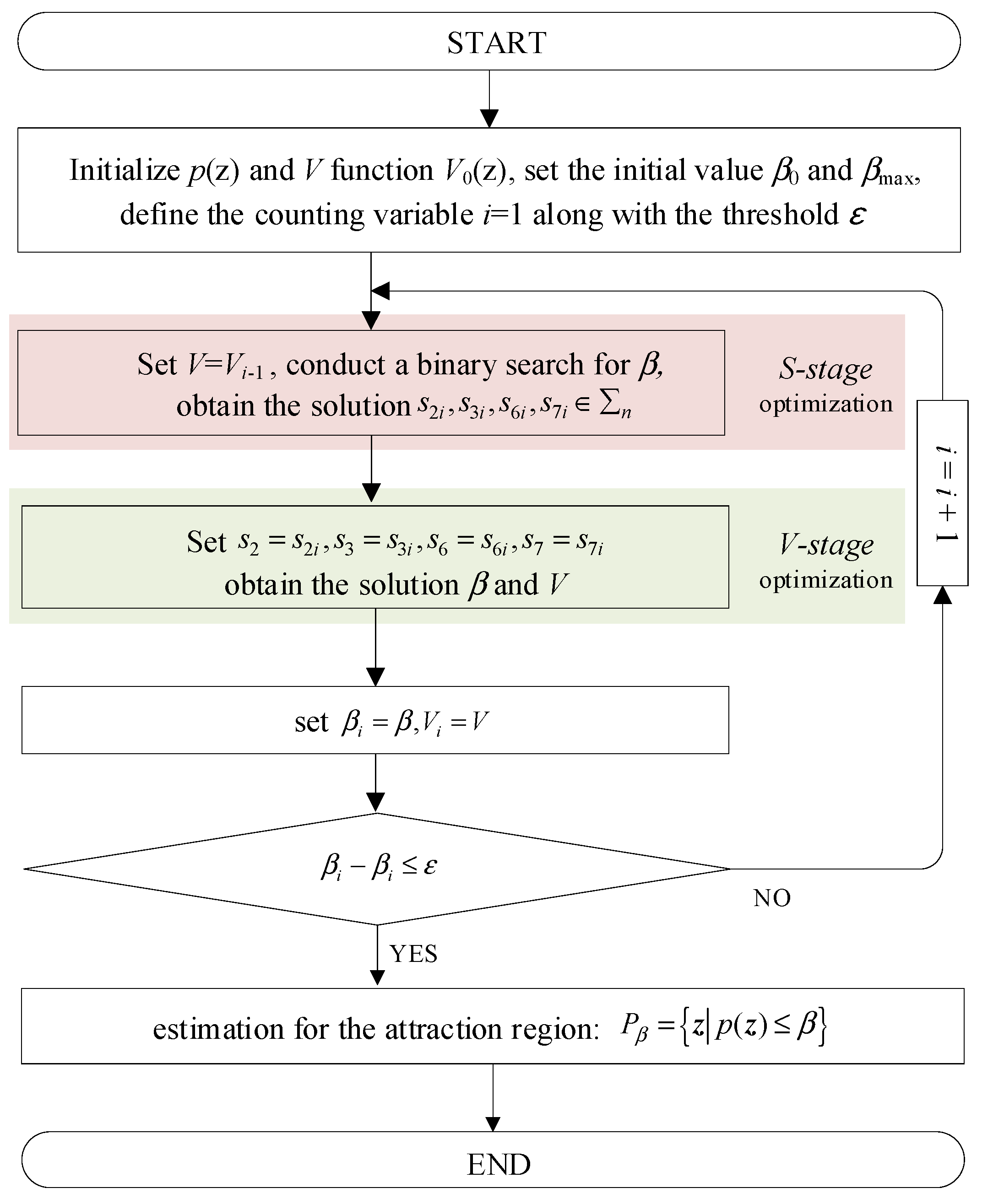
4.2. Methods and Procedures for Estimating the Region of Attraction of Power Systems
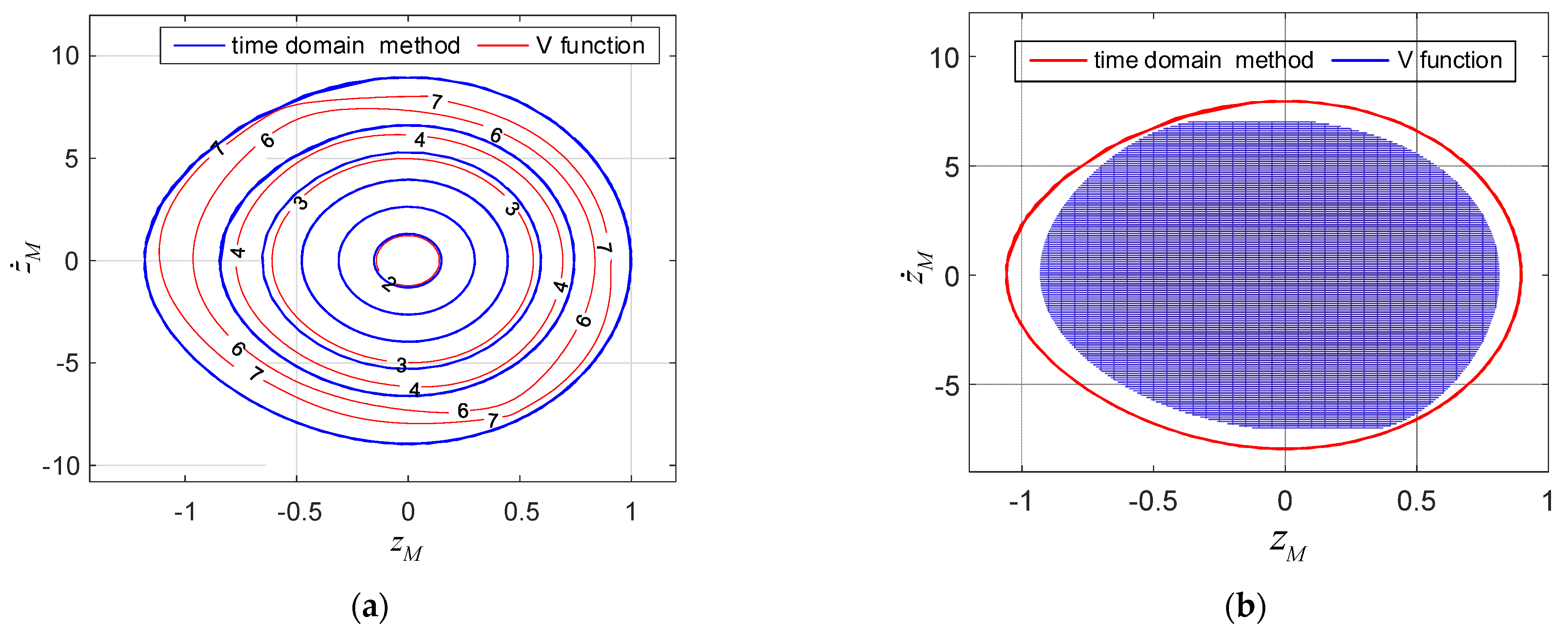
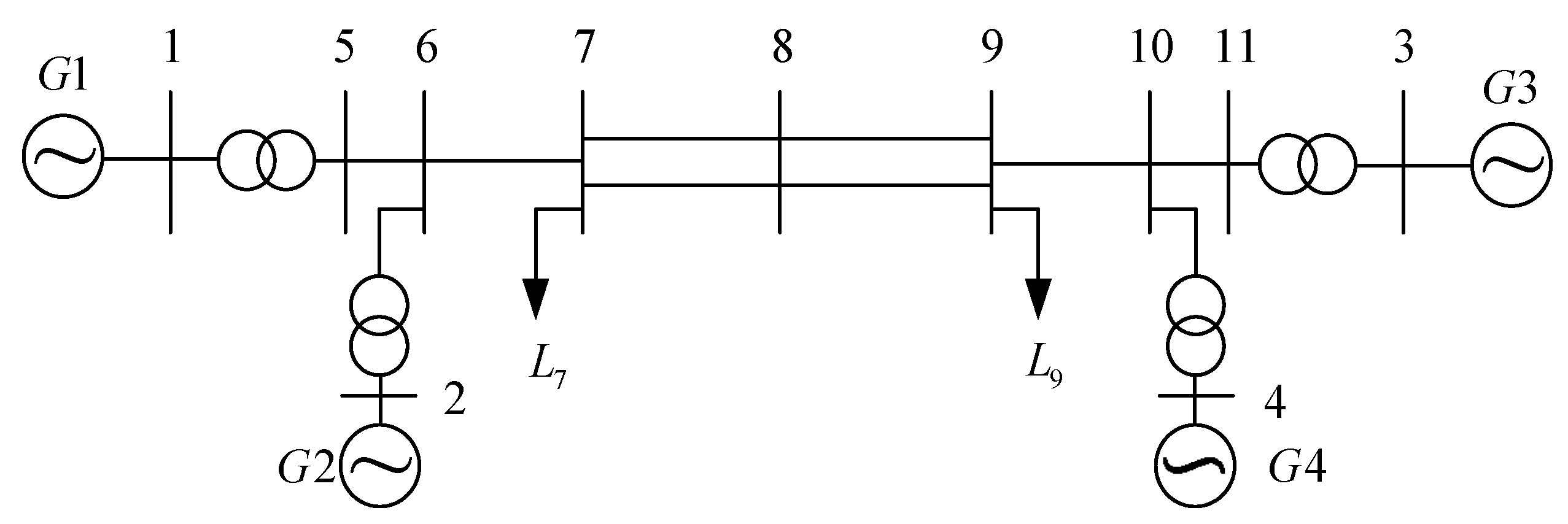
5. Case Study
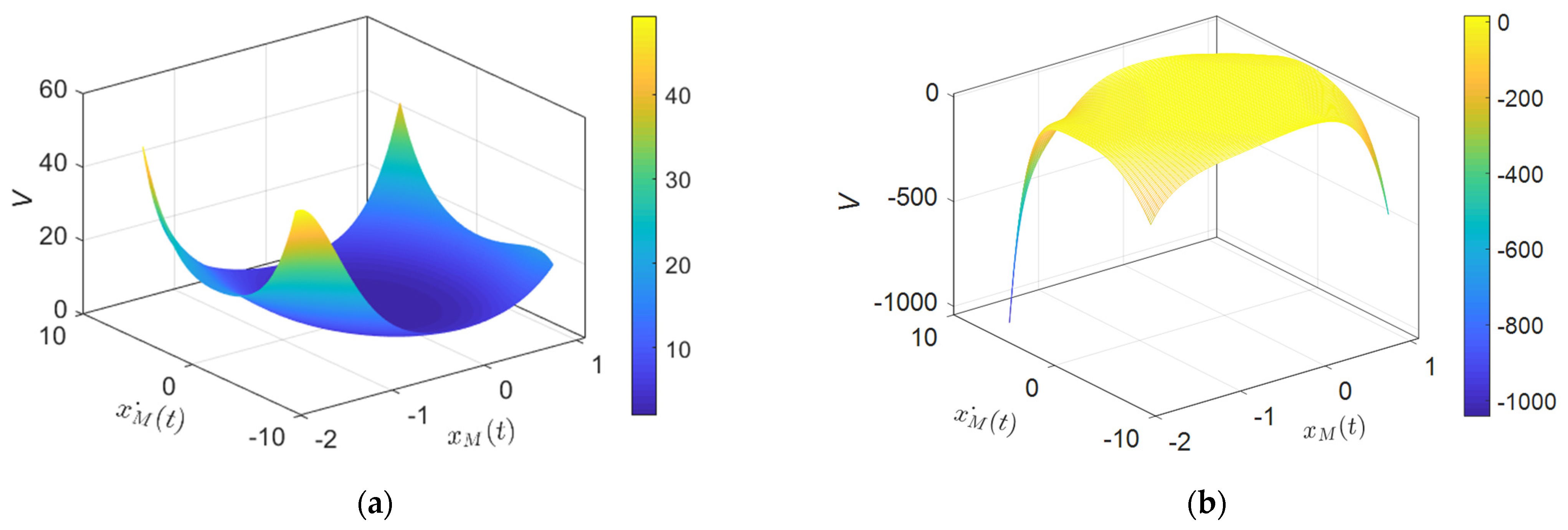
5.1. Single-Machine Infinite Bus System
5.2. IEEE 3-Machine Test Power System
5.3. IEEE 4-Machine Test Power System
6. Conclusions
- (1)
- The swing equations of the power system exhibit inherent polynomial structural characteristics. Based on the Taylor expansion formula, the power system model in both single-machine and multi-machine scenarios can be unified in a polynomial framework.
- (2)
- Based on the amplitude characteristics of angles in Z space, a single-machine projection equation governing the variation of maximum angle for multi-machines power system is capable of determining the stability of the polynomial system.
- (3)
- Case studies of single-machine and multi-machine systems compared to the time-domain method demonstrate that the proposed method can compute the Lyapunov function (V function) and effectively estimate the domain of attraction of the power system.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Khalil, H.K. Nonlinear Systems; Prentice Hall: Upper Saddle River, NJ, USA, 2002. [Google Scholar]
- Winfree, A.T. Biological rhythms and the behavior of populations of coupled oscillators. J. Theor. Biol. 1967, 16, 15–42. [Google Scholar] [CrossRef] [PubMed]
- Fouad, A.-A.; Vittal, V. Power System Transient Stability Analysis Using the Transient Energy Function Method; Pearson Education: Upper Saddle River, NJ, USA, 1991. [Google Scholar]
- Gassara, H.; Iben Ammar, I.; Ben Makhlouf, A.; Mchiri, L.; Rhaima, M. Design of Polynomial Observer-Based Control of Fractional-Order Power Systems. Mathematics 2023, 11, 4450. [Google Scholar] [CrossRef]
- Shakerighadi, B.; Ebrahimzadeh, E.; Blaabjerg, F.; Leth Bak, C. Large-signal stability modeling for the grid-connected VSC based on the Lyapunov method. Energies 2018, 11, 2533. [Google Scholar] [CrossRef]
- Parrilo, P.A. Structured Semidefinite Programs and Semialgebraic Geometry Methods in Robustness and Optimization; California Institute of Technology: Pasadena, CA, USA, 2000. [Google Scholar]
- Jarvis-Wloszek, Z.W. Lyapunov Based Analysis and Controller Synthesis for Polynomial Systems Using Sum-of-Squares Optimization; University of California, Berkeley: Berkeley, CA, USA, 2003. [Google Scholar]
- Tan, W. Nonlinear Control Analysis and Synthesis Using Sum-of-Squares Programming; ProQuest: Ann Arbor, MI, USA, 2006. [Google Scholar]
- Blekherman, G.; Parrilo, P.A.; Thomas, R.R. Semidefinite Optimization and Convex Algebraic Geometry; SIAM: Philadelphia, PA, USA, 2012. [Google Scholar]
- Tan, W.; Packard, A. Searching for control Lyapunov functions using sums of squares programming. In Proceedings of the 42nd Annual Allerton Conference on Communications, Control and Computing, Monticello, IL, USA, 29 September–1 October 2004; 2004; pp. 210–219. [Google Scholar]
- Papachristodoulou, A.; Prajna, S. A tutorial on sum of squares techniques for systems analysis. In Proceedings of the 2005, American Control Conference, Portland, OR, USA, 8–10 June 2005; pp. 2686–2700. [Google Scholar]
- Topcu, U.; Packard, A.; Seiler, P. Local stability analysis using simulations and sum-of-squares programming. Automatica 2008, 44, 2669–2675. [Google Scholar] [CrossRef]
- Khodadadi, L.; Samadi, B.; Khaloozadeh, H. Estimation of region of attraction for polynomial nonlinear systems: A numerical method. ISA Trans. 2014, 53, 25–32. [Google Scholar] [CrossRef] [PubMed]
- Ahmadi, A.A.; Majumdar, A. DSOS and SDSOS optimization: More tractable alternatives to sum of squares and semidefinite optimization. SIAM J. Appl. Algebra Geom. 2019, 3, 193–230. [Google Scholar] [CrossRef]
- Tacchi, M.; Cardozo, C.; Henrion, D.; Lasserre, J.B. Approximating regions of attraction of a sparse polynomial differential system. IFAC-PapersOnLine 2020, 53, 3266–3271. [Google Scholar] [CrossRef]
- Ameen, F.; Siddiq, A.; Trohák, A.; Benotsmane, R. A Scalable Hierarchical Dynamic PV Array Reconfiguration under Partial Shading. Energies 2024, 17, 181. [Google Scholar] [CrossRef]
- Anghel, M.; Milano, F.; Papachristodoulou, A. Algorithmic construction of Lyapunov functions for power system stability analysis. IEEE Trans. Circuits Syst. I Regul. Pap. 2013, 60, 2533–2546. [Google Scholar] [CrossRef]
- Mazumder, S.K.; de la Fuente, E.P. Dynamic stability analysis of power network. In Proceedings of the 2015 IEEE Energy Conversion Congress and Exposition (ECCE), Montreal, QC, Canada, 20–24 September 2015; pp. 5808–5815. [Google Scholar]
- Kundu, S.; Anghel, M. Stability and control of power systems using vector Lyapunov functions and sum-of-squares methods. In Proceedings of the 2015 European Control Conference (ECC), Linz, Austria, 15–17 July 2015; pp. 253–259. [Google Scholar]
- Han, D.; El-Guindy, A.; Althoff, M. Power systems transient stability analysis via optimal rational Lyapunov functions. In Proceedings of the 2016 IEEE Power and Energy Society General Meeting (PESGM), Boston, MA, USA, 17–21 July 2016; pp. 1–5. [Google Scholar]
- Mishra, C.; Thorp, J.S.; Centeno, V.A.; Pal, A. Stability region estimation under low voltage ride through constraints using sum of squares. In Proceedings of the 2017 North American Power Symposium (NAPS), Morgantown, WV, USA, 17–19 September 2017; pp. 1–6. [Google Scholar]
- Mishra, C.; Pal, A.; Thorp, J.S.; Centeno, V.A. Transient stability assessment of prone-to-trip renewable generation rich power systems using lyapunov’s direct method. IEEE Trans. Sustain. Energy 2019, 10, 1523–1533. [Google Scholar] [CrossRef]
- Izumi, S.; Somekawa, H.; Xin, X.; Yamasaki, T. Analysis of robust transient stability of power systems using sum of squares programming. Int. J. Electr. Power Energy Syst. 2020, 115, 105401. [Google Scholar] [CrossRef]
- Zhang, Z.; Schuerhuber, R.; Fickert, L.; Friedl, K.; Chen, G.; Zhang, Y. Domain of attraction’s estimation for grid connected converters with phase-locked loop. IEEE Trans. Power Syst. 2021, 37, 1351–1362. [Google Scholar] [CrossRef]
- Zhang, Q.; Gan, D. A Gronwall Inequality Based Approach to Transient Stability Assessment for Power Grids. arXiv 2023, arXiv:2311.02231. [Google Scholar]
- Chiang, H.-D.; Wu, F.F.; Varaiya, P.P. A BCU method for direct analysis of power system transient stability. IEEE Trans. Power Syst. 1994, 9, 1194–1208. [Google Scholar] [CrossRef]
- Topcu, U.; Packard, A.; Seiler, P.; Balas, G. Help on sos [ask the experts]. IEEE Control Syst. Mag. 2010, 30, 18–23. [Google Scholar]
- Anderson, P.M.; Fouad, A.A. Power System Control and Stability; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Kundur, P. Power system stability. Power Syst. Stab. Control. 2007, 10, 7–11. [Google Scholar]





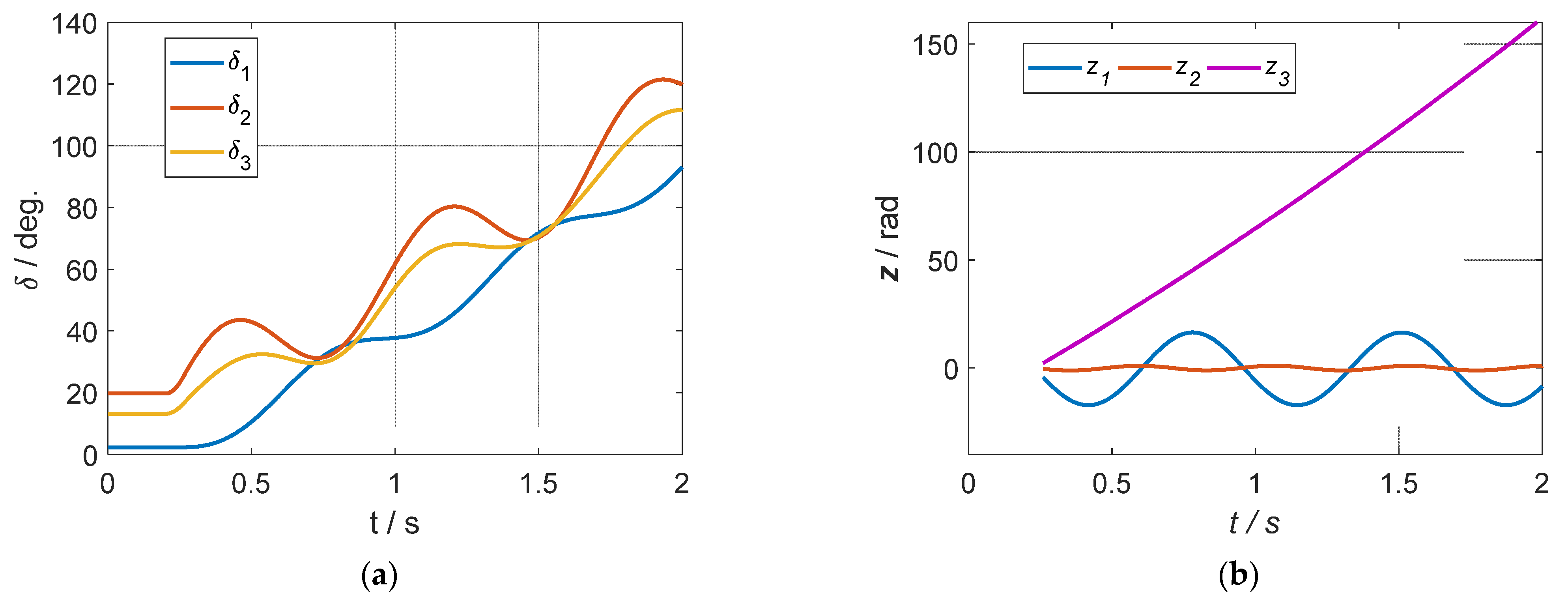
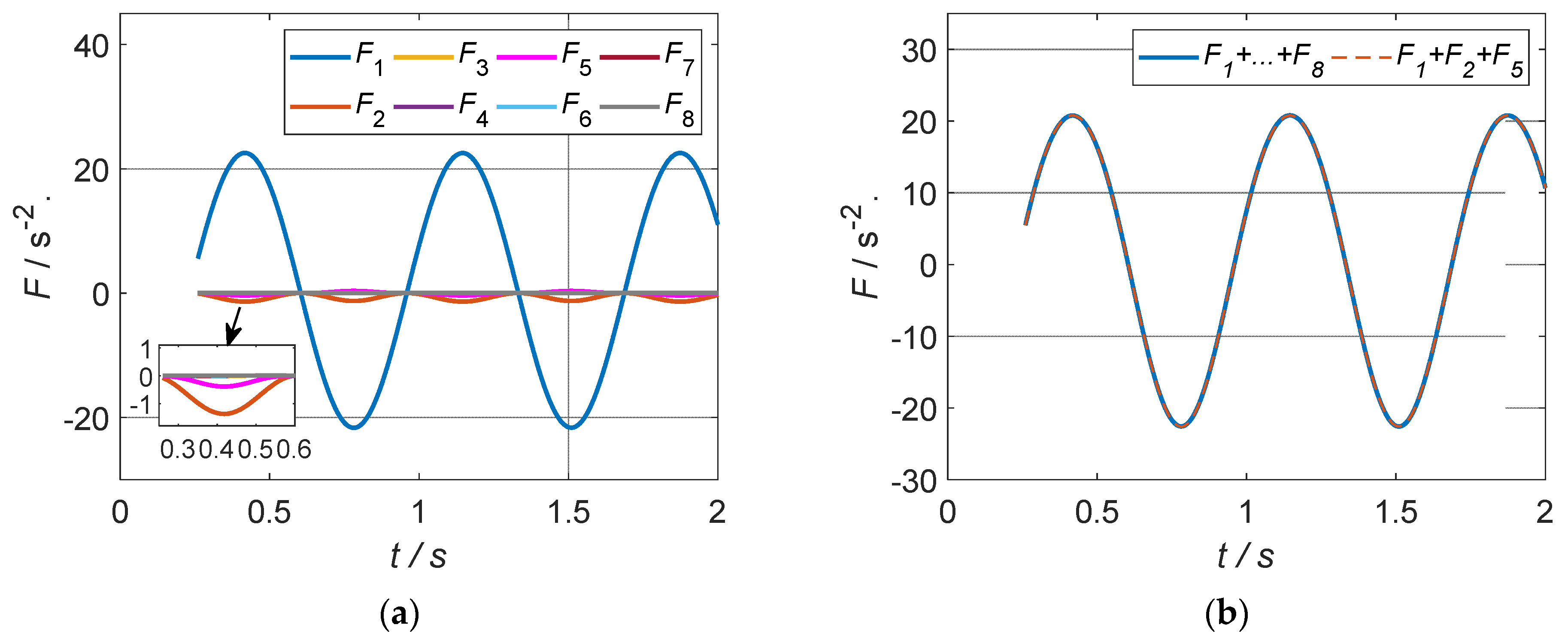

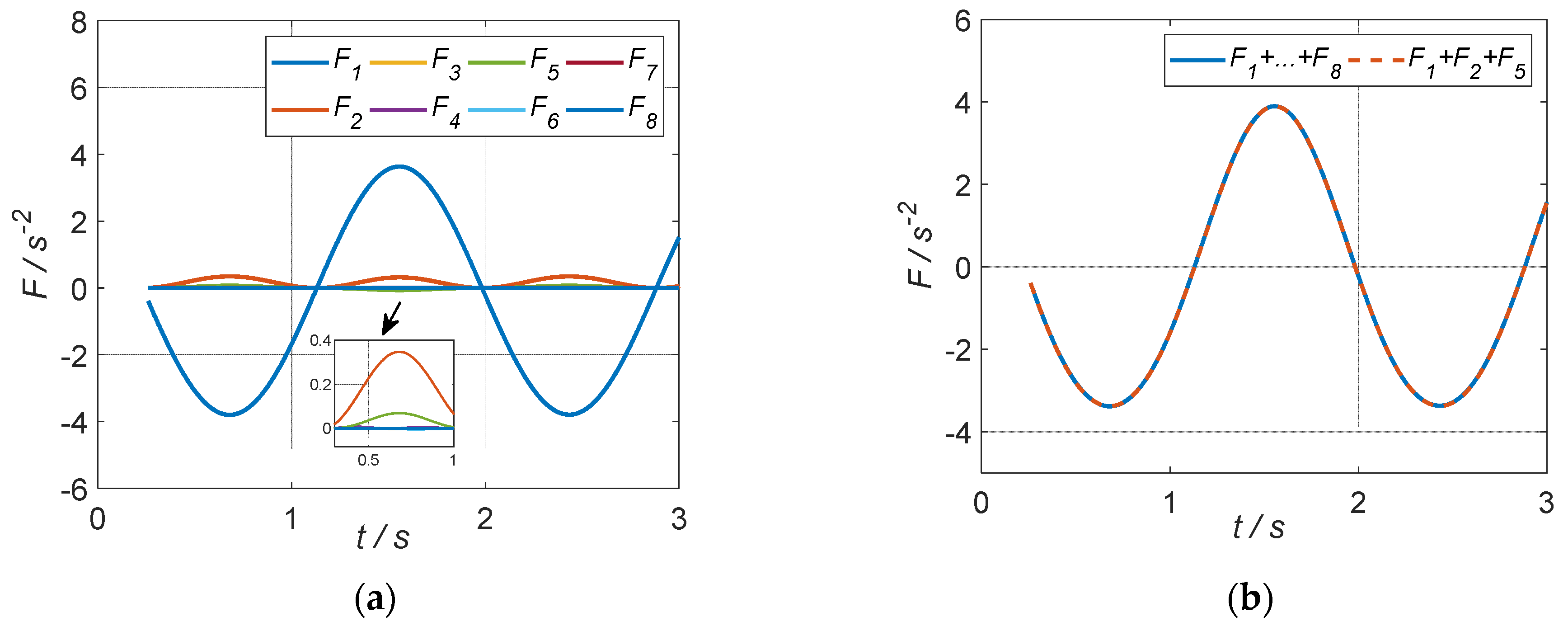


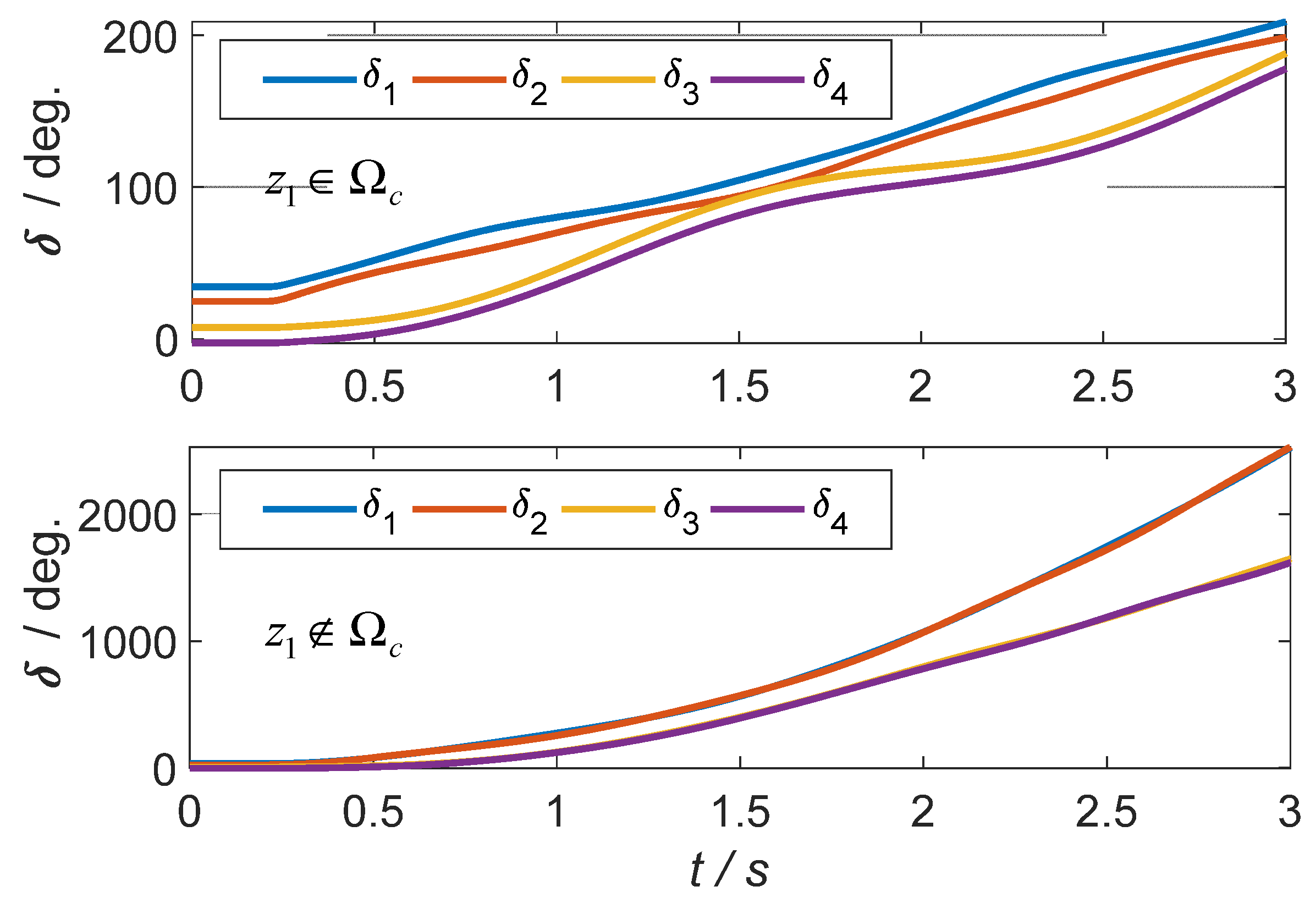
| H | D | PM | Pmax | ωR |
|---|---|---|---|---|
| 3.5 | 0 | 0.9 | 1.35 | 375.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Wu, H.; Zhan, X.; Gan, D. Estimation of the Region of Attraction of Polynomial Swing Equation Using Sum of Squares Theory. Energies 2024, 17, 1050. https://doi.org/10.3390/en17051050
Li J, Wu H, Zhan X, Gan D. Estimation of the Region of Attraction of Polynomial Swing Equation Using Sum of Squares Theory. Energies. 2024; 17(5):1050. https://doi.org/10.3390/en17051050
Chicago/Turabian StyleLi, Jing, Hao Wu, Xianwen Zhan, and Deqiang Gan. 2024. "Estimation of the Region of Attraction of Polynomial Swing Equation Using Sum of Squares Theory" Energies 17, no. 5: 1050. https://doi.org/10.3390/en17051050
APA StyleLi, J., Wu, H., Zhan, X., & Gan, D. (2024). Estimation of the Region of Attraction of Polynomial Swing Equation Using Sum of Squares Theory. Energies, 17(5), 1050. https://doi.org/10.3390/en17051050








